Finite Element Analysis of Variable Viscosity Impact on MHD Flow and Heat Transfer of Nanofluid Using the Cattaneo–Christov Model
Abstract
1. Introduction
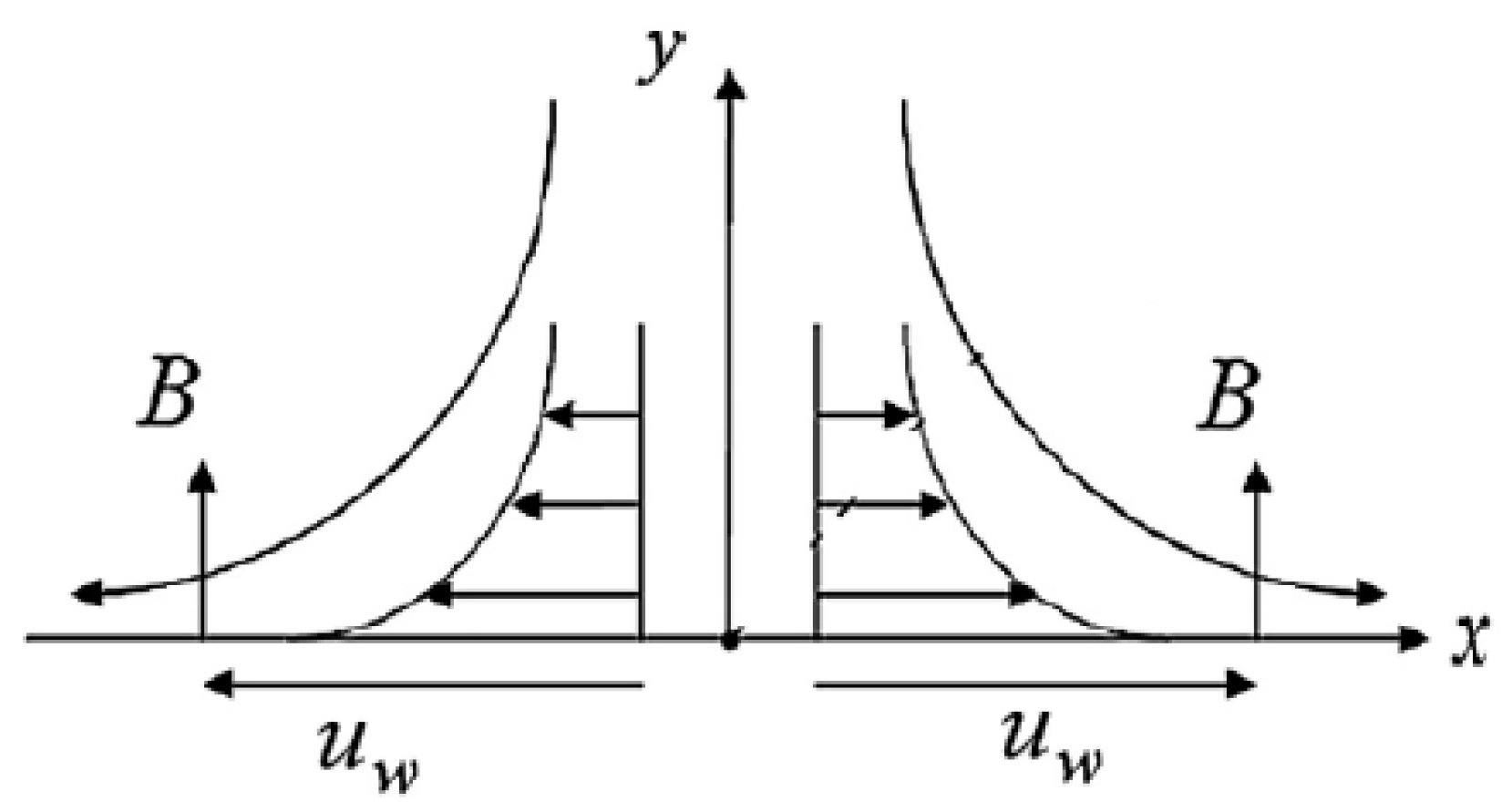
2. Problem Description
3. Interpretation of Method
3.1. Variational Formulations
3.2. Finite Element Formulation
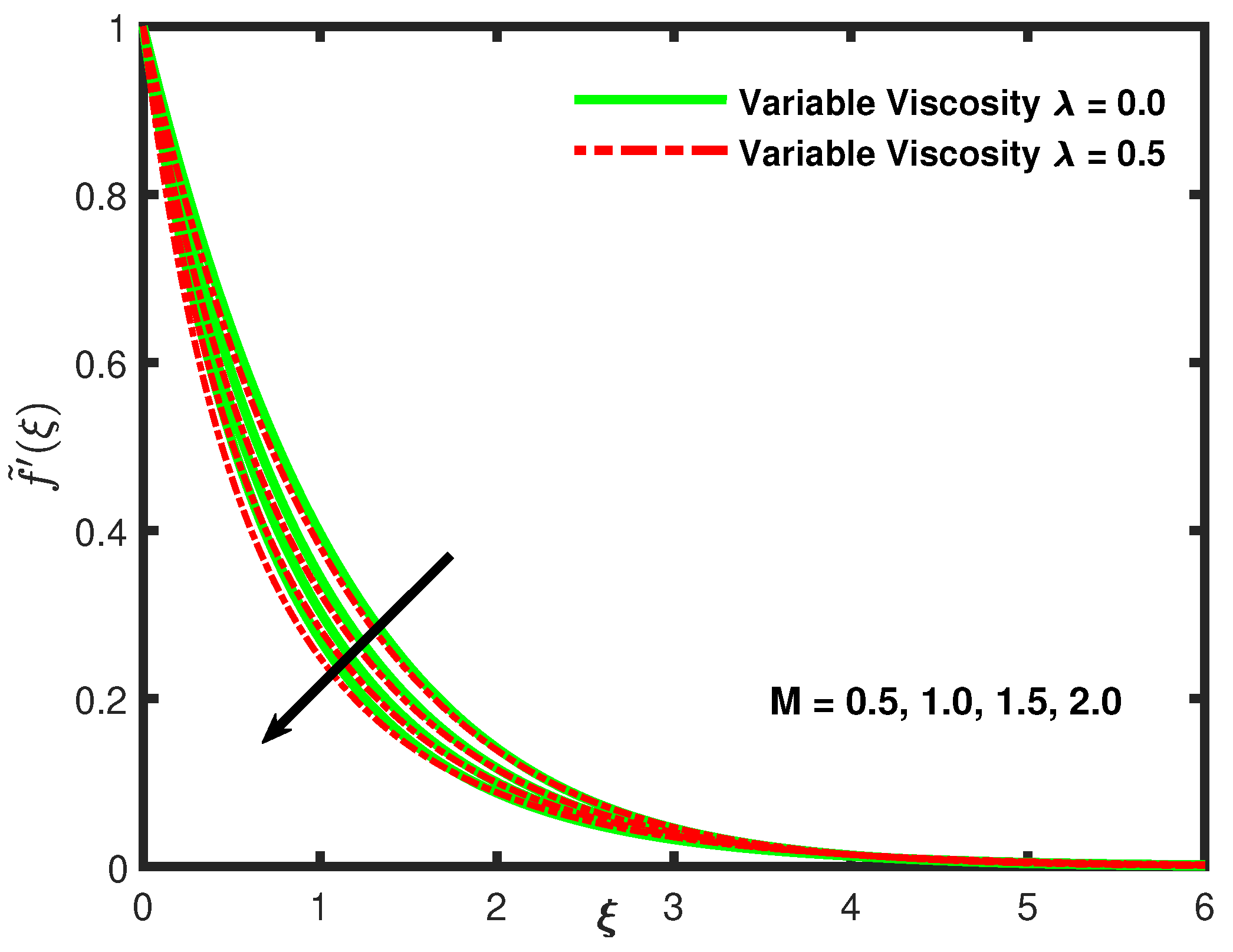
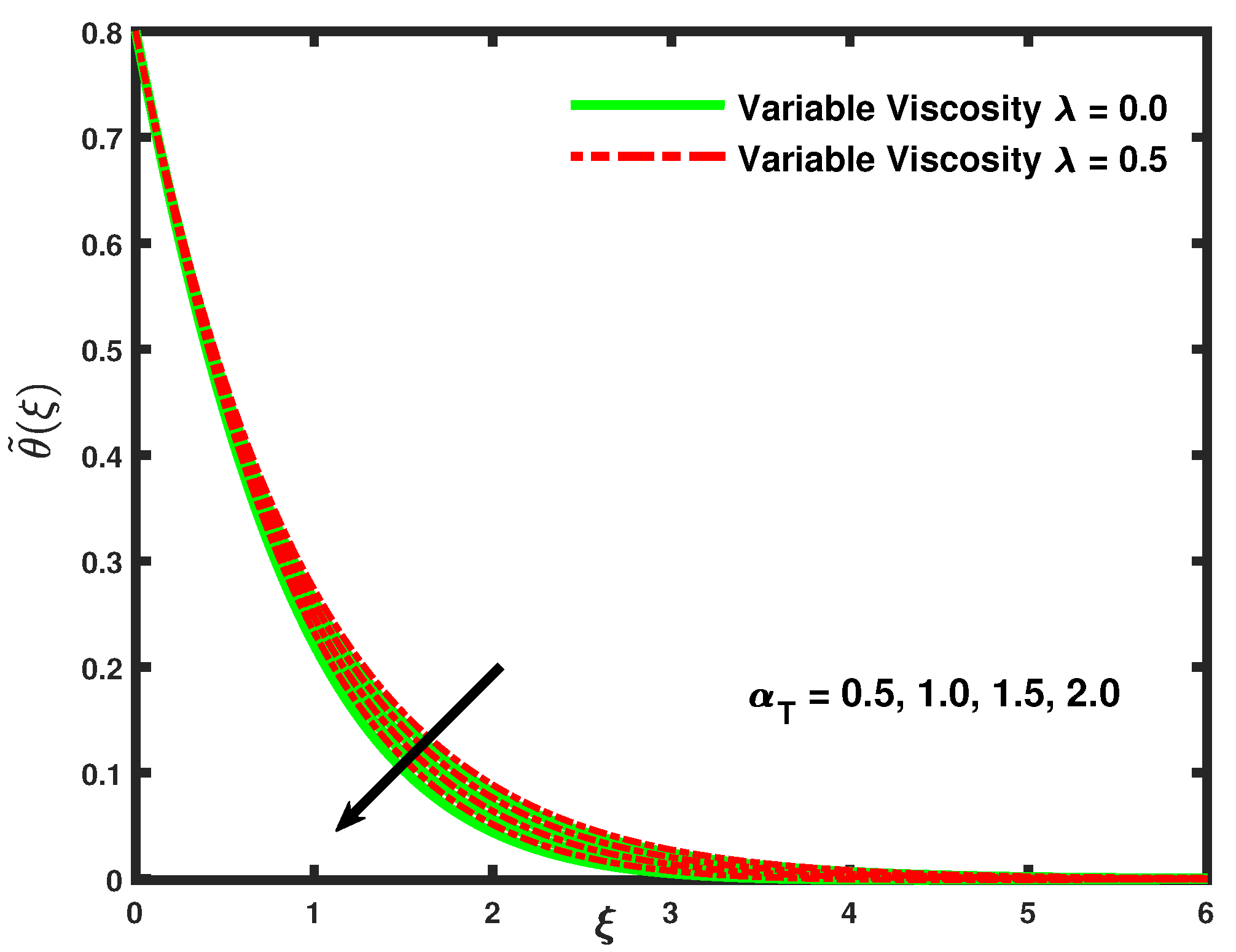
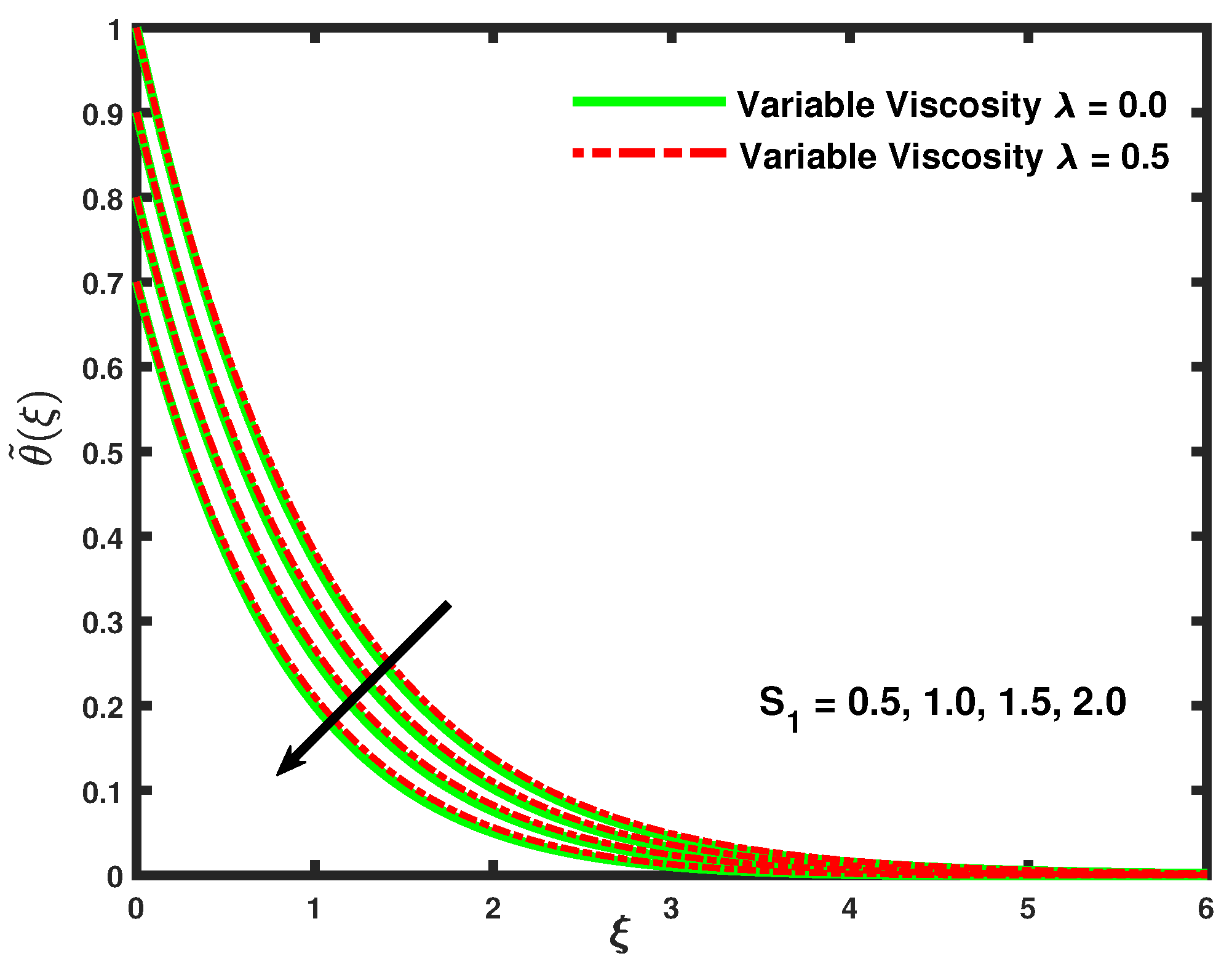
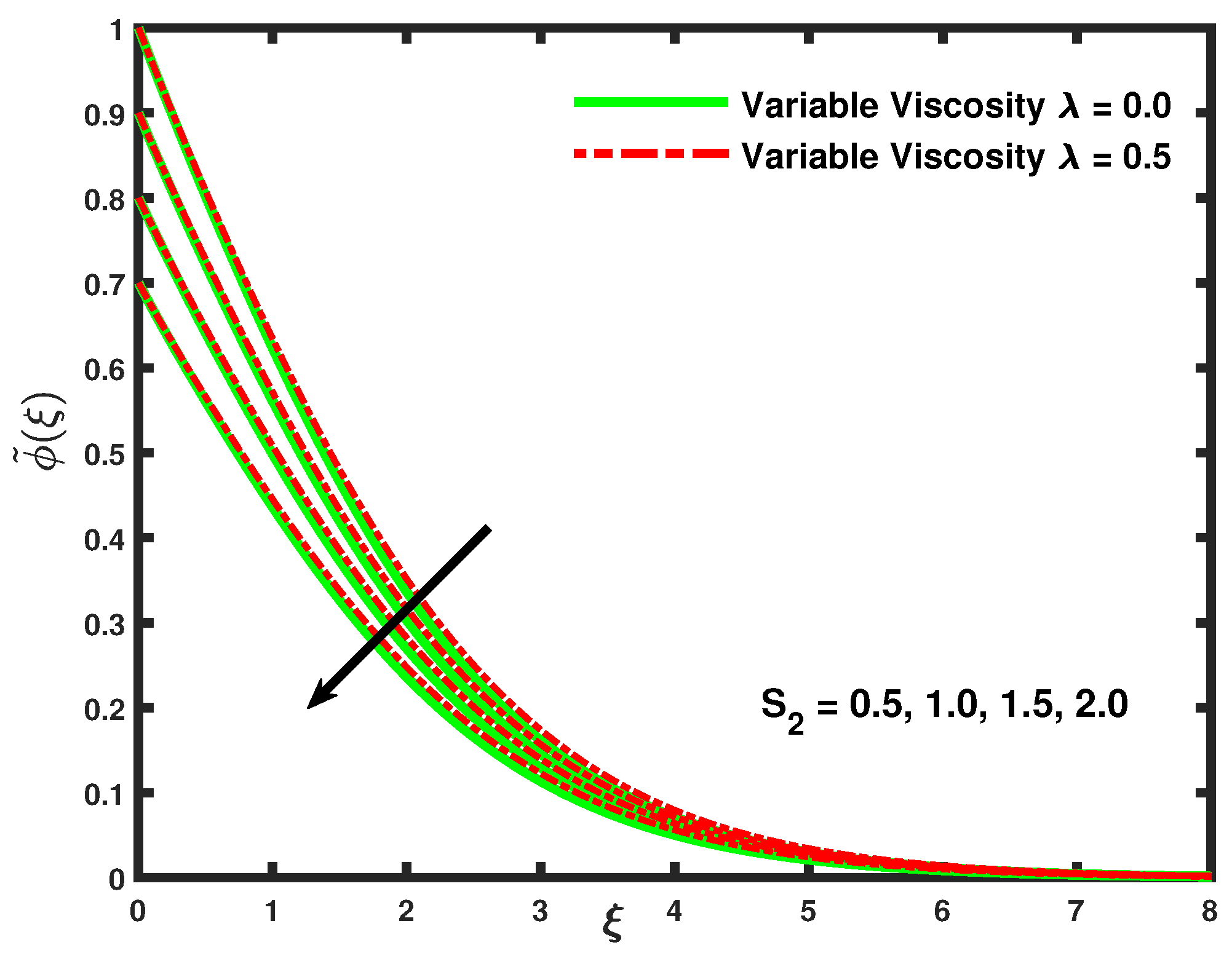
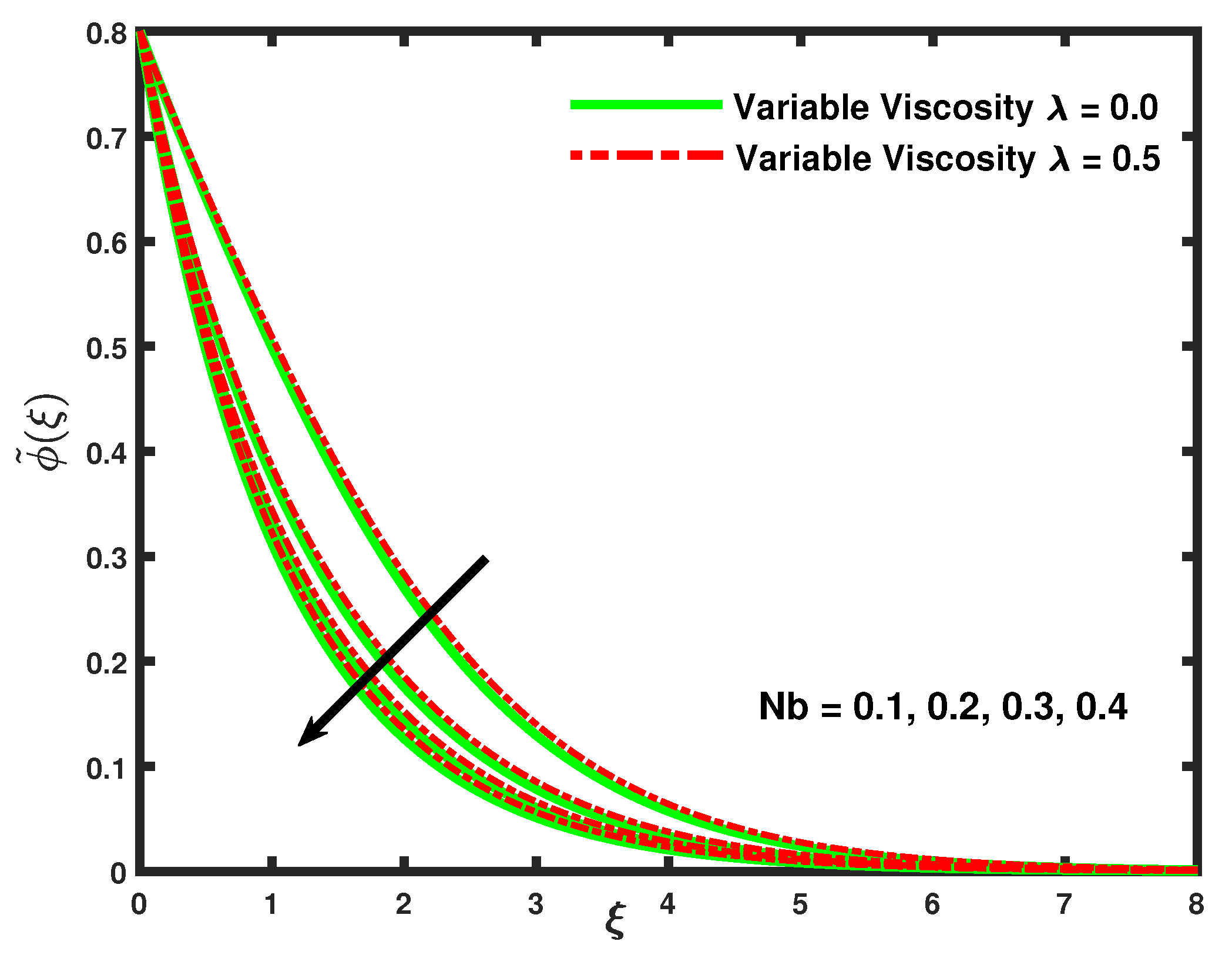
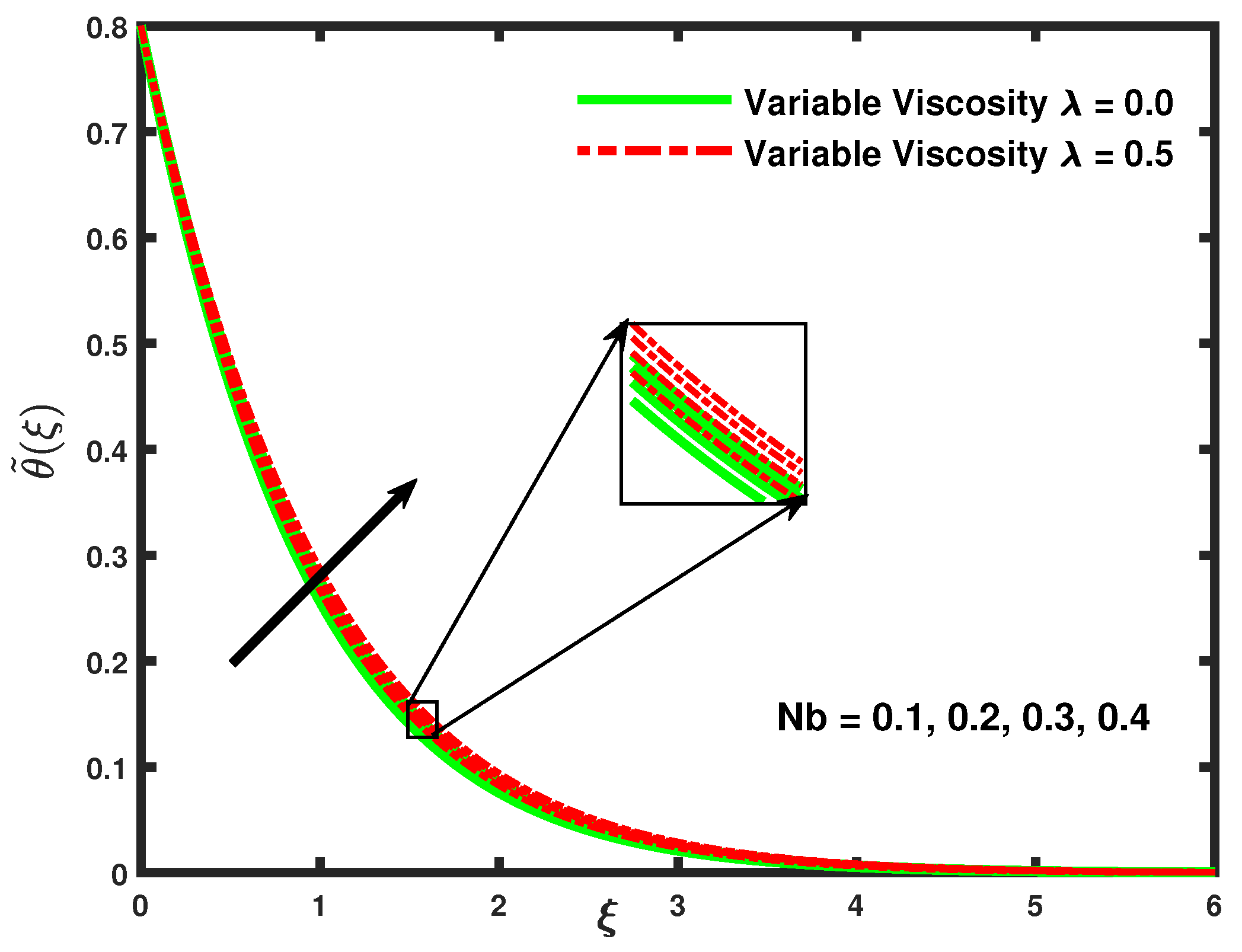
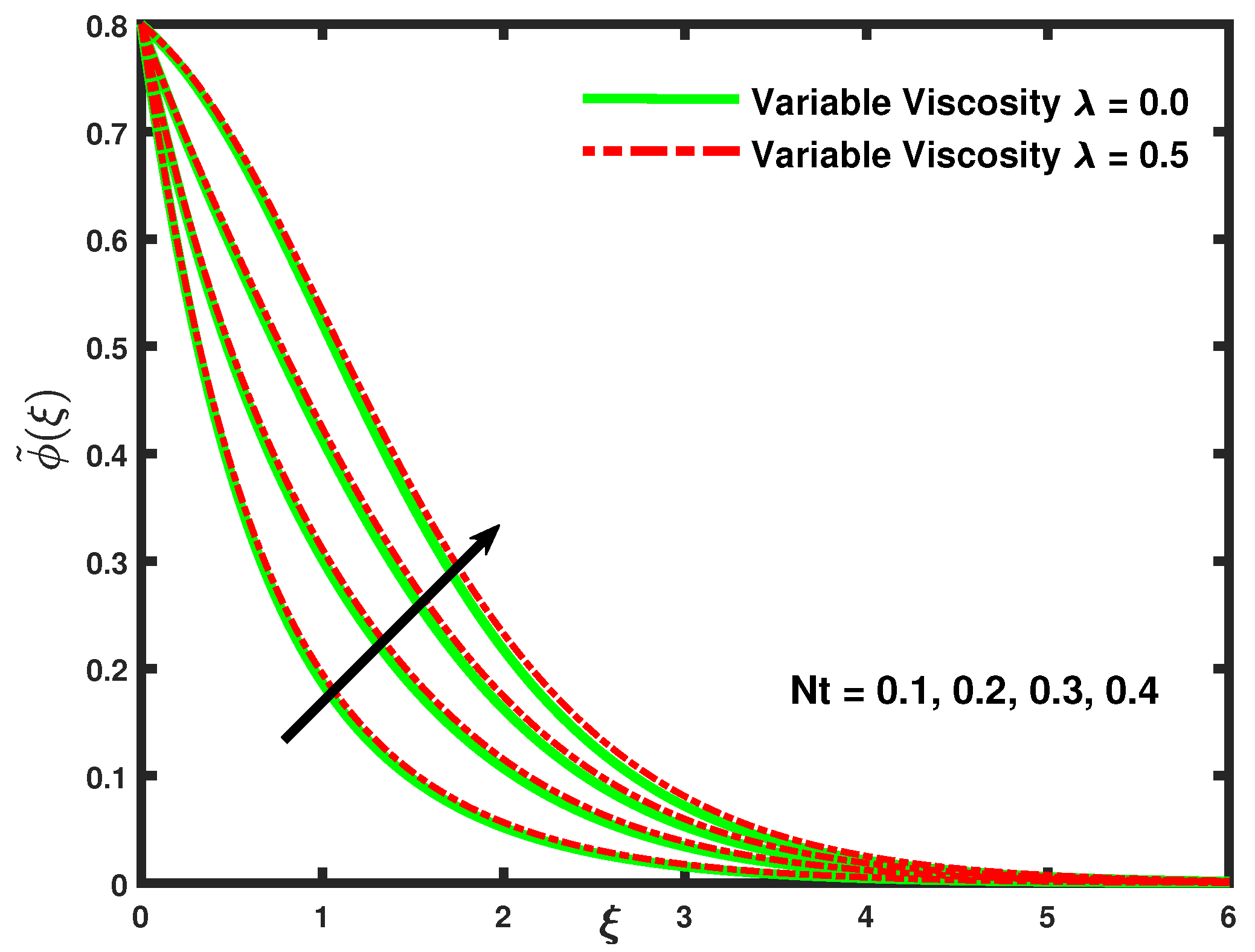
4. Results and Discussion
5. Concluding Remarks
- The increasing values of M and the viscosity parameter decrease the nanofluid velocity magnitude; however, there is a contrary impact on the concentration and temperature distributions.
- The increase in the concentration and thermal boundary layer thickness of the nanofluid sheet due to the increment in the viscosity parameter also increases the temperature and concentration of nanoparticles.
- Fluid temperature declines with increasing values of the thermal relaxation parameter. This shows that the Cattaneo–Christov heat flux model provides a better assessment of temperature distribution.
- The magnitude of the Nusselt number and the Sherwood number declines, and the thermal boundary layer thickness is reduced with increasing values of magnetic M and viscosity parameters.
- The increasing values of the Brownian motion parameter cause a decline in the nanoparticle concentration profile whereas for thermophoresis parameter , the results are contrary.
- The thermal relaxation parameter effectively increases the rate of heat transfer of the flow.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Viscosity of fluid | |
| Thermal Stratification | |
| Solutal Stratification | |
| Dimensionless parameters | |
| Buoyancy parameters | |
| Brownian diffusion coefficient | |
| Brownian motion parameter | |
| Curie temperature | |
| Lewis number | |
| Thermal conductivity of fluid | |
| Nanoparticle’s density | |
| Specific heat | |
| Prandtl number | |
| Thermal Grashof number | |
| Thermophoresis parameter | |
| Thermal relaxation parameter | |
| Thermal diffusibility | |
| Velocity components | |
| Velocity of sheet | |
| Temperature at surface | |
| Local Reynold number | |
| T | Non-dimensional temperature |
| M | Magnetic penetrability |
| Conductivity of fluid | |
| Thermal relaxation parameter | |
| Variable viscosity parameter | |
| Fluid density | |
| Temperature away from the surface |
References
- Sakiadis, B. Boundary-layer behavior on continuous solid surfaces: II. The boundary layer on a continuous flat surface. AiChE J. 1961, 7, 221–225. [Google Scholar] [CrossRef]
- Crane, L.J. Flow past a stretching plate. Z. Für Angew. Math. Und Phys. ZAMP 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Grubka, L.; Bobba, K. Heat transfer characteristics of a continuous, stretching surface with variable temperature. J. Heat Transf. 1985, 107, 248–250. [Google Scholar] [CrossRef]
- Banks, W.; Zaturska, M. Eigensolutions in boundary-layer flow adjacent to a stretching wall. IMA J. Appl. Math. 1986, 36, 263–273. [Google Scholar] [CrossRef]
- El-Hakiem, M. Effects of a transverse flows with variable viscosity in micropolar fluids. Heat Mass Transf. 1998, 34, 91–99. [Google Scholar] [CrossRef]
- Elbashbeshy, E.; Bazid, M. The effect of temperature-dependent viscosity on heat transfer over a continuous moving surface. J. Phys. D Appl. Phys. 2000, 33, 2716. [Google Scholar] [CrossRef]
- Christov, C. On frame indifferent formulation of the Maxwell-Cattaneo model of finite-speed heat conduction. Mech. Res. Commun. 2009, 36, 481–486. [Google Scholar] [CrossRef]
- Salahuddin, T.; Malik, M.Y.; Hussain, A.; Bilal, S.; Awais, M. MHD flow of Cattanneo–Christov heat flux model for Williamson fluid over a stretching sheet with variable thickness: Using numerical approach. J. Magn. Magn. Mater. 2016, 401, 991–997. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Alsaedi, A.; Waqas, M.; Yasmeen, T. Impact of Cattaneo–Christov heat flux model in flow of variable thermal conductivity fluid over a variable thicked surface. Int. J. Heat Mass Transf. 2016, 99, 702–710. [Google Scholar] [CrossRef]
- Malik, M.; Khan, M.; Salahuddin, T.; Khan, I. Variable viscosity and MHD flow in Casson fluid with Cattaneo–Christov heat flux model: Using Keller box method. Eng. Sci. Technol. Int. J. 2016, 19, 1985–1992. [Google Scholar] [CrossRef]
- Rahman, M.; Roşca, A.; Pop, I. Boundary layer flow of a nanofluid past a permeable exponentially shrinking/stretching surface with second order slip using Buongiorno’s model. Int. J. Heat Mass Transf. 2014, 77, 1133–1143. [Google Scholar] [CrossRef]
- Tibullo, V.; Zampoli, V. A uniqueness result for the Cattaneo–Christov heat conduction model applied to incompressible fluids. Mech. Res. Commun. 2011, 38, 77–79. [Google Scholar] [CrossRef]
- Li, J.; Zheng, L.; Liu, L. MHD viscoelastic flow and heat transfer over a vertical stretching sheet with Cattaneo-Christov heat flux effects. J. Mol. Liq. 2016, 221, 19–25. [Google Scholar] [CrossRef]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T.; Bin-Mohsin, B. Nonlinear radiation effects on MHD flow of nanofluid over a nonlinearly stretching/shrinking wedge. Neural Comput. Appl. 2017, 28, 2041–2050. [Google Scholar] [CrossRef]
- Ali, B.; Naqvi, R.A.; Nie, Y.; Khan, S.A.; Sadiq, M.T.; Rehman, A.U.; Abdal, S. Variable Viscosity Effects on Unsteady MHD an Axisymmetric Nanofluid Flow over a Stretching Surface with Thermo-Diffusion: FEM Approach. Symmetry 2020, 12, 234. [Google Scholar] [CrossRef]
- Vo, D.D.; Alsarraf, J.; Moradikazerouni, A.; Afrand, M.; Salehipour, H.; Qi, C. Numerical investigation of γ-AlOOH nano-fluid convection performance in a wavy channel considering various shapes of nanoadditives. Powder Technol. 2019, 345, 649–657. [Google Scholar] [CrossRef]
- Sarafraz, M.; Pourmehran, O.; Yang, B.; Arjomandi, M.; Ellahi, R. Pool boiling heat transfer characteristics of iron oxide nano-suspension under constant magnetic field. Int. J. Therm. Sci. 2020, 147, 106131. [Google Scholar] [CrossRef]
- Asadi, A.; Aberoumand, S.; Moradikazerouni, A.; Pourfattah, F.; Żyła, G.; Estellé, P.; Mahian, O.; Wongwises, S.; Nguyen, H.M.; Arabkoohsar, A. Recent advances in preparation methods and thermophysical properties of oil-based nanofluids: A state-of-the-art review. Powder Technol. 2019, 352, 209–226. [Google Scholar] [CrossRef]
- Pourmehran, O.; Sarafraz, M.; Rahimi-Gorji, M.; Ganji, D. Rheological behaviour of various metal-based nano-fluids between rotating discs: A new insight. J. Taiwan Inst. Chem. Eng. 2018, 88, 37–48. [Google Scholar] [CrossRef]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S.; Khan, S.A. Analysis of Magnetic Properties of Nano-Particles Due to a Magnetic Dipole in Micropolar Fluid Flow over a Stretching Sheet. Coatings 2020, 10, 170. [Google Scholar] [CrossRef]
- Ma, Y.; Shahsavar, A.; Moradi, I.; Rostami, S.; Moradikazerouni, A.; Yarmand, H.; Zulkifli, N.W.B.M. Using finite volume method for simulating the natural convective heat transfer of nano-fluid flow inside an inclined enclosure with conductive walls in the presence of a constant temperature heat source. Phys. A Stat. Mech. Appl. 2019, 123035. [Google Scholar] [CrossRef]
- Sarafraz, M.; Safaei, M.R. Diurnal thermal evaluation of an evacuated tube solar collector (ETSC) charged with graphene nanoplatelets-methanol nano-suspension. Renew. Energy 2019, 142, 364–372. [Google Scholar] [CrossRef]
- Tian, Z.; Rostami, S.; Taherialekouhi, R.; Karimipour, A.; Moradikazerouni, A.; Yarmand, H.; Zulkifli, N.W.B.M. Prediction of rheological behavior of a new hybrid nanofluid consists of copper oxide and multi wall carbon nanotubes suspended in a mixture of water and ethylene glycol using curve-fitting on experimental data. Phys. A Stat. Mech. Appl. 2020, 124101. [Google Scholar] [CrossRef]
- Alsarraf, J.; Moradikazerouni, A.; Shahsavar, A.; Afrand, M.; Salehipour, H.; Tran, M.D. Hydrothermal analysis of turbulent boehmite alumina nanofluid flow with different nanoparticle shapes in a minichannel heat exchanger using two-phase mixture model. Phys. A Stat. Mech. Appl. 2019, 520, 275–288. [Google Scholar] [CrossRef]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S. Finite Element Simulation of Multi-Slip Effects on Unsteady MHD Bioconvective Micropolar nanofluid Flow Over a Sheet with Solutal and Thermal Convective Boundary Conditions. Coatings 2019, 9, 842. [Google Scholar] [CrossRef]
- Ibrahim, W.; Shankar, B. MHD boundary layer flow and heat transfer of a nanofluid past a permeable stretching sheet with velocity, thermal and solutal slip boundary conditions. Comput. Fluids 2013, 75, 1–10. [Google Scholar] [CrossRef]
- Ranjbarzadeh, R.; Moradikazerouni, A.; Bakhtiari, R.; Asadi, A.; Afrand, M. An experimental study on stability and thermal conductivity of water/silica nanofluid: Eco-friendly production of nanoparticles. J. Clean. Prod. 2019, 206, 1089–1100. [Google Scholar] [CrossRef]
- Sarafraz, M.; Arya, H.; Saeedi, M.; Ahmadi, D. Flow boiling heat transfer to MgO-therminol 66 heat transfer fluid: Experimental assessment and correlation development. Appl. Therm. Eng. 2018, 138, 552–562. [Google Scholar] [CrossRef]
- Moradikazerouni, A.; Hajizadeh, A.; Safaei, M.R.; Afrand, M.; Yarmand, H.; Zulkifli, N.W.B.M. Assessment of thermal conductivity enhancement of nano-antifreeze containing single-walled carbon nanotubes: Optimal artificial neural network and curve-fitting. Phys. A Stat. Mech. Appl. 2019, 521, 138–145. [Google Scholar] [CrossRef]
- Das, K. Slip flow and convective heat transfer of nanofluids over a permeable stretching surface. Comput. Fluids 2012, 64, 34–42. [Google Scholar] [CrossRef]
- Sarafraz, M.; Pourmehran, O.; Yang, B.; Arjomandi, M. Assessment of the thermal performance of a thermosyphon heat pipe using zirconia-acetone nanofluids. Renew. Energy 2019, 136, 884–895. [Google Scholar] [CrossRef]
- Abdal, S.; Ali, B.; Younas, S.; Ali, L.; Mariam, A. Thermo-Diffusion and Multislip Effects on MHD Mixed Convection Unsteady Flow of Micropolar Nanofluid over a Shrinking/Stretching Sheet with Radiation in the Presence of Heat Source. Symmetry 2020, 12, 49. [Google Scholar] [CrossRef]
- Uddin, M.J.; Khan, W.; Ismail, A.M. Free convection boundary layer flow from a heated upward facing horizontal flat plate embedded in a porous medium filled by a nanofluid with convective boundary condition. Transp. Porous Media 2012, 92, 867–881. [Google Scholar] [CrossRef]
- Sarafraz, M.; Arya, A.; Nikkhah, V.; Hormozi, F. Thermal performance and viscosity of biologically produced silver/coconut oil nanofluids. Chem. Biochem. Eng. Q. 2016, 30, 489–500. [Google Scholar] [CrossRef]
- Besthapu, P.; Haq, R.U.; Bandari, S.; Al-Mdallal, Q.M. Mixed convection flow of thermally stratified MHD nanofluid over an exponentially stretching surface with viscous dissipation effect. J. Taiwan Inst. Chem. Eng. 2017, 71, 307–314. [Google Scholar] [CrossRef]
- Disu, A.; Dada, M. Reynold’s model viscosity on radiative MHD flow in a porous medium between two vertical wavy walls. J. Taibah Univ. Sci. 2017, 11, 548–565. [Google Scholar] [CrossRef][Green Version]
- Akbar, N.S.; Tripathi, D.; Khan, Z.H.; Bég, O.A. A numerical study of magnetohydrodynamic transport of nanofluids over a vertical stretching sheet with exponential temperature-dependent viscosity and buoyancy effects. Chem. Phys. Lett. 2016, 661, 20–30. [Google Scholar] [CrossRef]
- Akbar, N.S. Ferromagnetic CNT suspended H2O+ Cu nanofluid analysis through composite stenosed arteries with permeable wall. Phys. E Low-Dimens. Syst. Nanostruct. 2015, 72, 70–76. [Google Scholar] [CrossRef]
- Reddy, J.N. An Introduction to the Finite Element Method; TMH: New York, NY, USA, 1993. [Google Scholar]
- Gupta, D.; Kumar, L.; Beg, O.A.; Singh, B. Finite Element Analysis of Transient Heat and Mass Transfer in Mircostructural Boundary layer Flow from a Porous Stretching Sheet. Comput. Therm. Sci. Int. J. 2014, 6, 155–169. [Google Scholar] [CrossRef]
- Swapna, G.; Kumar, L.; Rana, P.; Singh, B. Finite element modeling of a double-diffusive mixed convection flow of a chemically-reacting magneto-micropolar fluid with convective boundary condition. J. Taiwan Inst. Chem. Eng. 2015, 47, 18–27. [Google Scholar] [CrossRef]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S. Finite Element Analysis of Thermo-Diffusion and Multi-Slip Effects on MHD Unsteady Flow of Casson Nano-Fluid over a Shrinking/Stretching Sheet with Radiation and Heat Source. Appl. Sci. 2019, 9, 5217. [Google Scholar] [CrossRef]
- Jalil, M.; Asghar, S.; Yasmeen, S. An exact solution of MHD boundary layer flow of dusty fluid over a stretching surface. Math. Probl. Eng. 2017, 2017, 2307469. [Google Scholar] [CrossRef]
- Ali, B.; Nie, Y.; Khan, S.A.; Sadiq, M.T.; Tariq, M. Finite Element Simulation of Multiple Slip Effects on MHD Unsteady Maxwell Nanofluid Flow over a Permeable Stretching Sheet with Radiation and Thermo-Diffusion in the Presence of Chemical Reaction. Processes 2019, 7, 628. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Pop, I. Boundary layer flow and heat transfer over an unsteady stretching vertical surface. Meccanica 2009, 44, 369–375. [Google Scholar] [CrossRef]
- Pal, D. Combined effects of non-uniform heat source/sink and thermal radiation on heat transfer over an unsteady stretching permeable surface. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1890–1904. [Google Scholar] [CrossRef]
- Abbasi, F.; Shehzad, S.; Hayat, T.; Alhuthali, M. Mixed convection flow of jeffrey nanofluid with thermal radiation and double stratification. J. Hydrodyn. Ser. B 2016, 28, 840–849. [Google Scholar] [CrossRef]
- Khan, W.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Anwar, M.; Khan, I.; Sharidan, S.; Salleh, M. Conjugate effects of heat and mass transfer of nanofluids over a nonlinear stretching sheet. Int. J. Phys. Sci. 2012, 7, 4081–4092. [Google Scholar] [CrossRef]
- Anantha Kumar, K.; Ramadevi, B.; Sugunamma, V. Impact of Lorentz force on unsteady bio convective flow of Carreau fluid across a variable thickness sheet with non-Fourier heat flux model. In Defect and Diffusion Forum; Trans Tech Publ: Zurich, Switzerland, 2018; Volume 387, pp. 474–497. [Google Scholar]



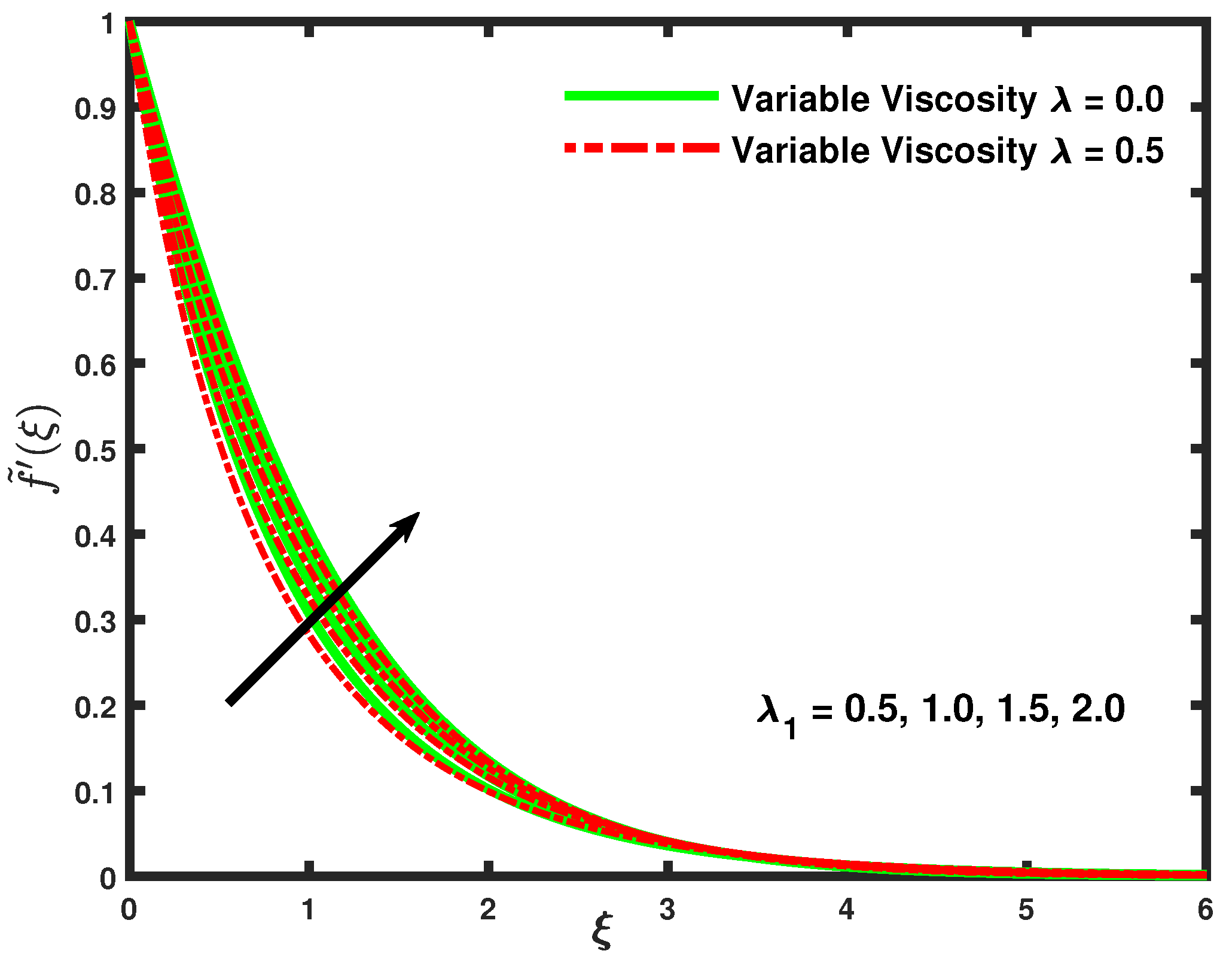
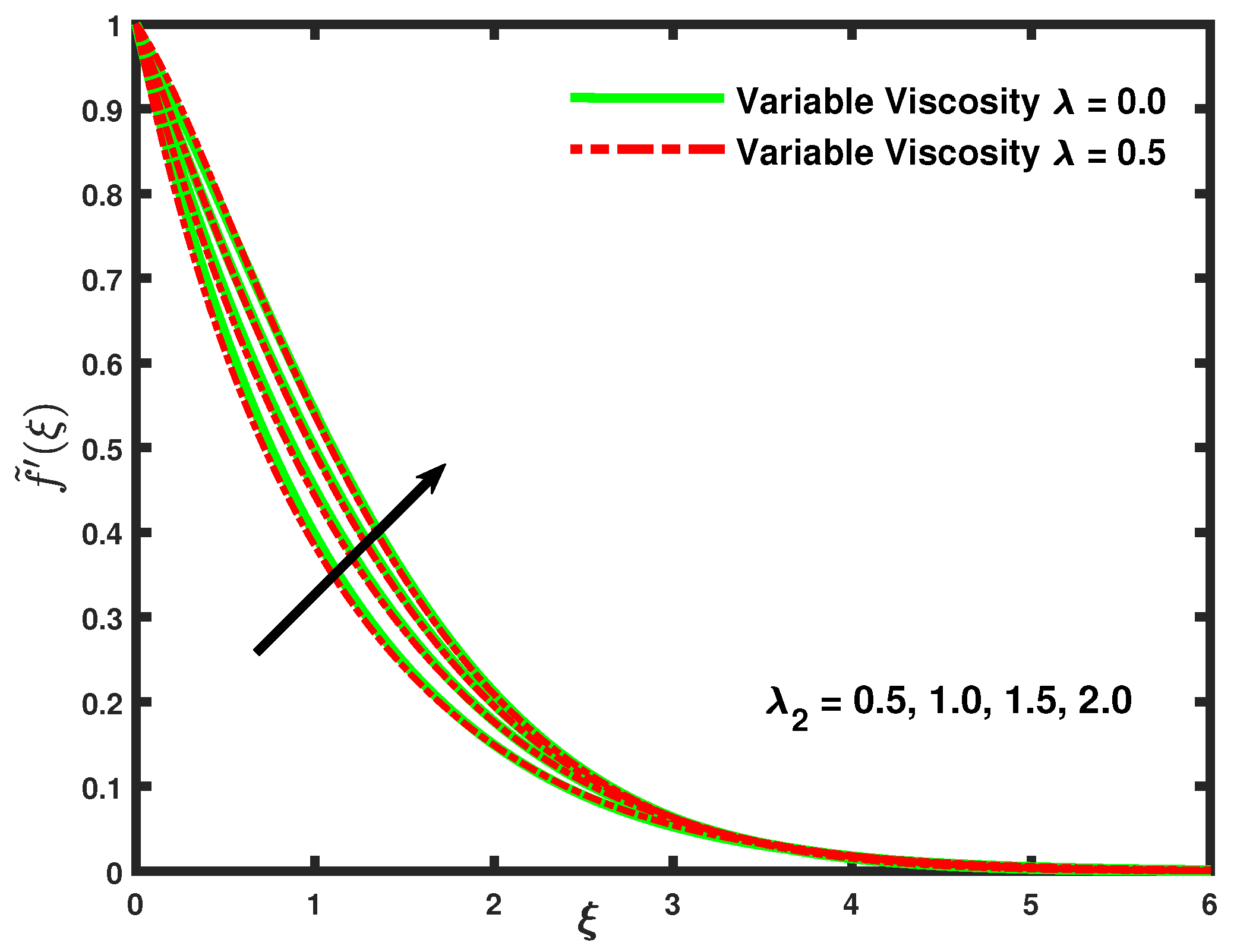


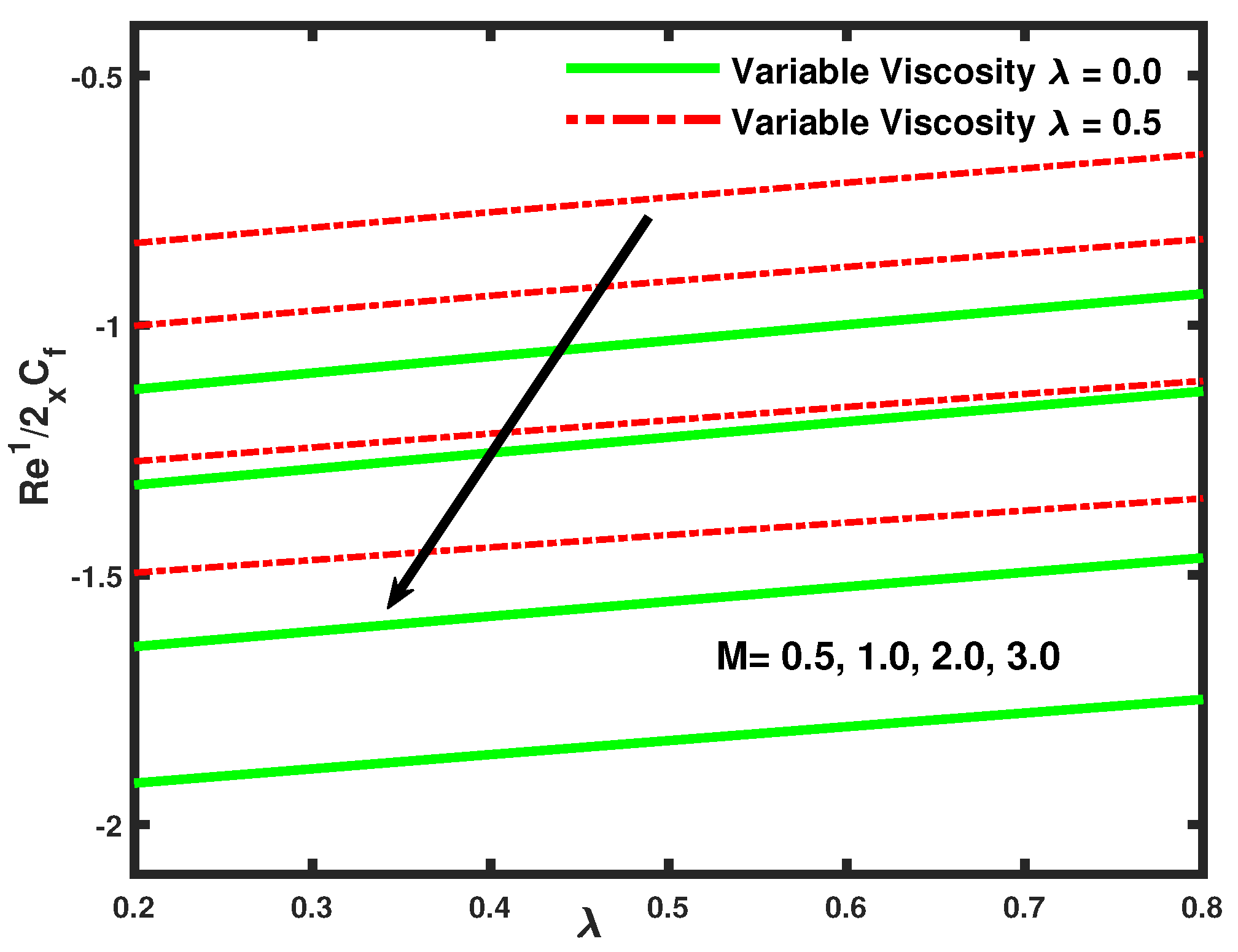
| Number of Elements | ||||
|---|---|---|---|---|
| 60 | 0.86976 | 0.04045 | 0.02302 | 0.13808 |
| 100 | 0.87027 | 0.04057 | 0.02315 | 0.13809 |
| 180 | 0.87049 | 0.04062 | 0.02321 | 0.13809 |
| 300 | 0.87056 | 0.04064 | 0.02323 | 0.13810 |
| 420 | 0.87058 | 0.04065 | 0.02324 | 0.13810 |
| 500 | 0.87059 | 0.04065 | 0.02324 | 0.13810 |
| 700 | 0.87060 | 0.04065 | 0.02324 | 0.13810 |
| M | Mudassar et al. [43] (a) | Liaqat et al. [42] | Bagh et al. [44] | FEM (Our results) (b) | Error |
|---|---|---|---|---|---|
| 0.0 | 1.000000 | 1.0000078 | 1.0000080 | 1.0000080 | 0.00002 |
| 0.2 | 1.095445 | 1.0954462 | 1.0954458 | 1.0954460 | 0.00001 |
| 0.5 | 1.224745 | 1.2247452 | 1.2247446 | 1.2247450 | 0.00001 |
| 1.0 | 1.414214 | 1.4142142 | 1.4142132 | 1.4142138 | 0.00002 |
| 1.2 | 1.483240 | 1.4832385 | 1.4832393 | 1.4832390 | 0.00003 |
| 1.5 | 1.581139 | 1.5811392 | 1.5811384 | 1.5811389 | 0.00001 |
| 2.0 | 1.732051 | 1.7320515 | 1.7320504 | 1.7320510 | 0.00002 |
| Ishak et al. [45] (a) | Liaqat et al. [42] | Bagh et al. [44] | Dulal Pal. [46] | FEM (Our results) (b) | Error | |
|---|---|---|---|---|---|---|
| 0.72 | 0.808631 | 0.808633 | 0.8086339299 | - | 0.8086339297 | 0.0003 |
| 1.00 | 1.000000 | 1.000008 | 1.0000080213 | 1.0000 | 1.0000080210 | 0.0008 |
| 3.00 | 1.923682 | 1.923677 | 1.9236777221 | 1.9236 | 1.9236777219 | 0.0003 |
| 10.0 | 3.720673 | 3.720668 | 3.7206681683 | 3.7207 | 3.7206681679 | 0.0002 |
| 100 | 12.294083 | 12.294051 | 12.294051659 | 12.2940 | 12.294051661 | 0.0003 |
| Abbasi et al. [47] | FEM (Our Results) | |||
|---|---|---|---|---|
| , | ||||
| 0.0 | 0.82852 | 0.37977 | 0.828512 | 0.379840 |
| 0.05 | - | - | 0.855730 | 0.357125 |
| 0.1 | - | - | 0.882813 | 0.334570 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, L.; Liu, X.; Ali, B. Finite Element Analysis of Variable Viscosity Impact on MHD Flow and Heat Transfer of Nanofluid Using the Cattaneo–Christov Model. Coatings 2020, 10, 395. https://doi.org/10.3390/coatings10040395
Ali L, Liu X, Ali B. Finite Element Analysis of Variable Viscosity Impact on MHD Flow and Heat Transfer of Nanofluid Using the Cattaneo–Christov Model. Coatings. 2020; 10(4):395. https://doi.org/10.3390/coatings10040395
Chicago/Turabian StyleAli, Liaqat, Xiaomin Liu, and Bagh Ali. 2020. "Finite Element Analysis of Variable Viscosity Impact on MHD Flow and Heat Transfer of Nanofluid Using the Cattaneo–Christov Model" Coatings 10, no. 4: 395. https://doi.org/10.3390/coatings10040395
APA StyleAli, L., Liu, X., & Ali, B. (2020). Finite Element Analysis of Variable Viscosity Impact on MHD Flow and Heat Transfer of Nanofluid Using the Cattaneo–Christov Model. Coatings, 10(4), 395. https://doi.org/10.3390/coatings10040395







