Exploring the Application of Multi-Resonant Bands Terahertz Metamaterials in the Field of Carbohydrate Films Sensing
Abstract
1. Introduction
2. Materials and Methods
2.1. Preparation of the Materials, Biological-Solution and Biological-Films Sample
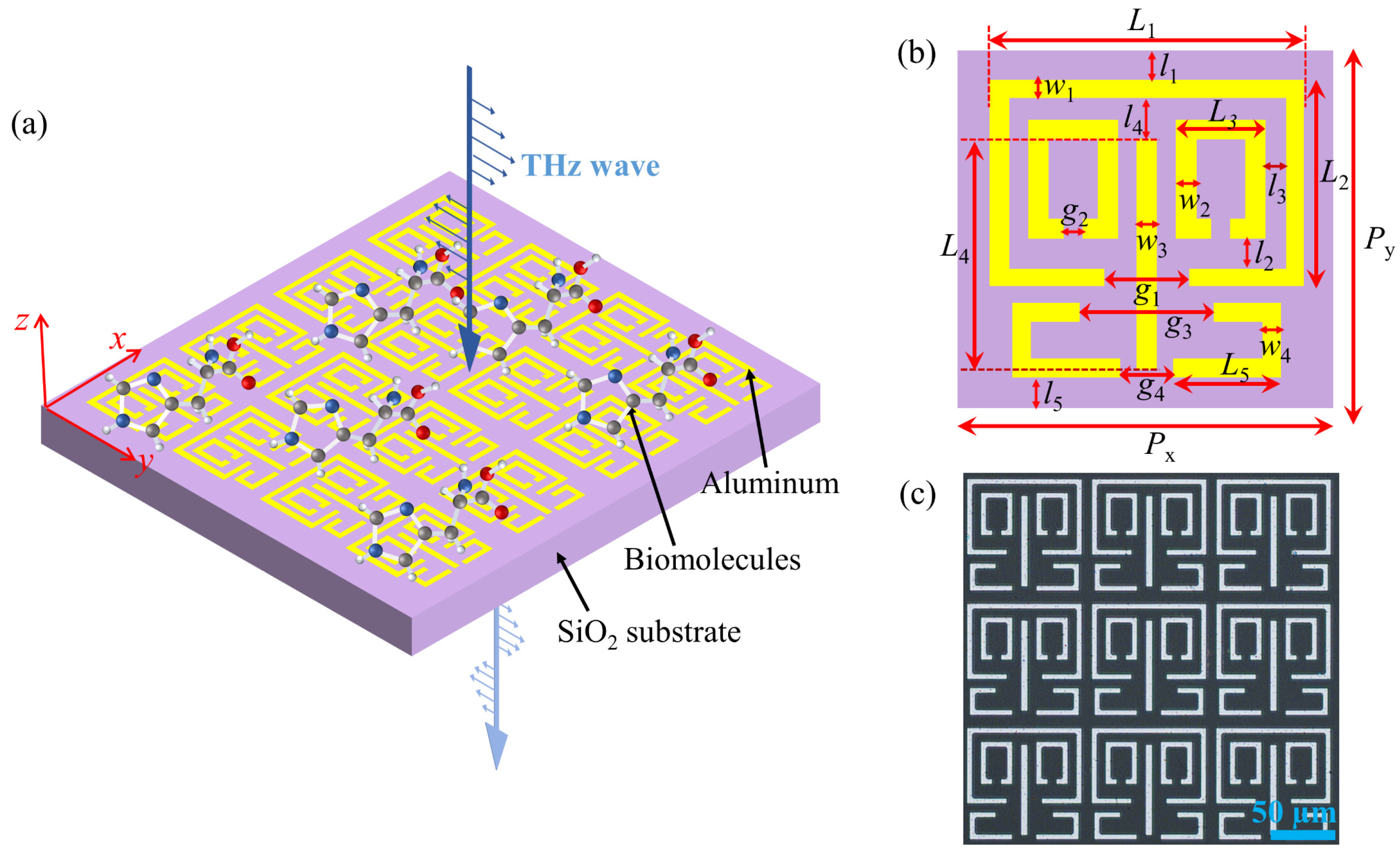
2.2. Metamaterials Structure and Design
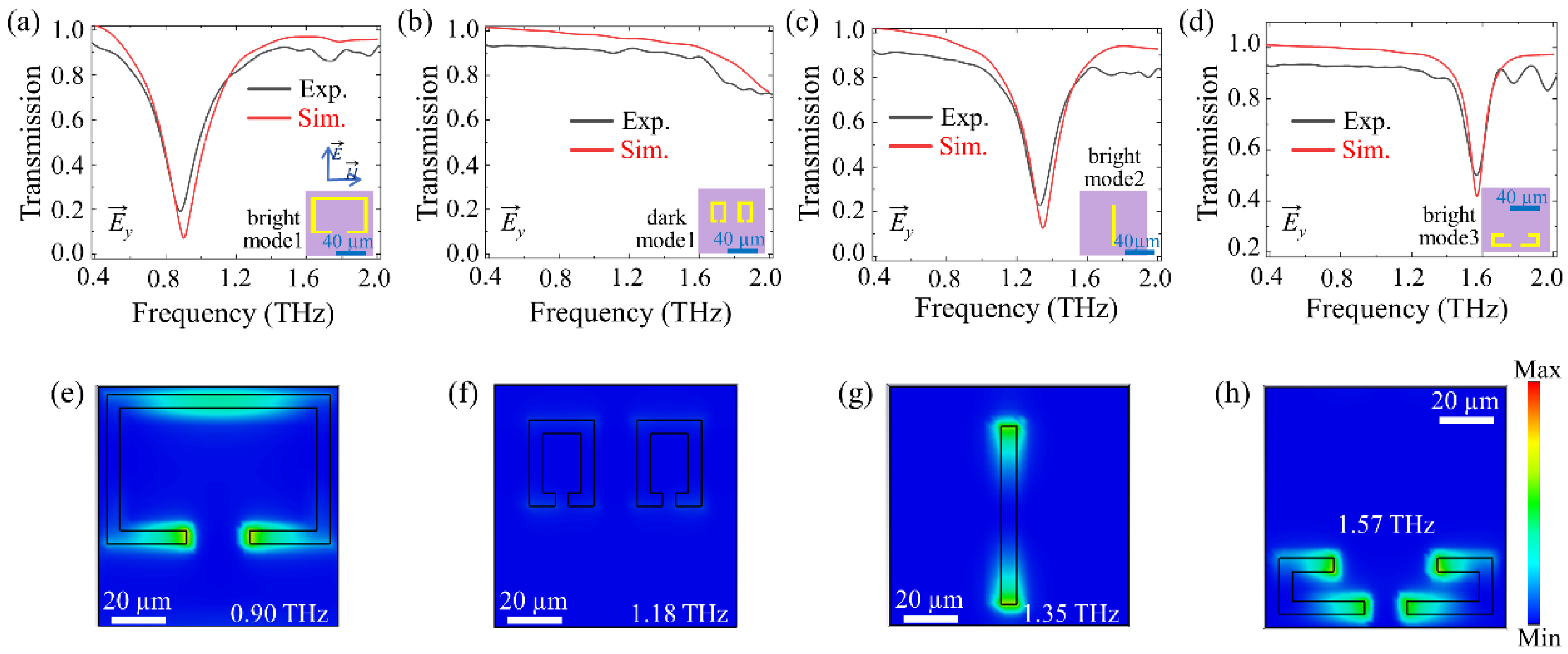
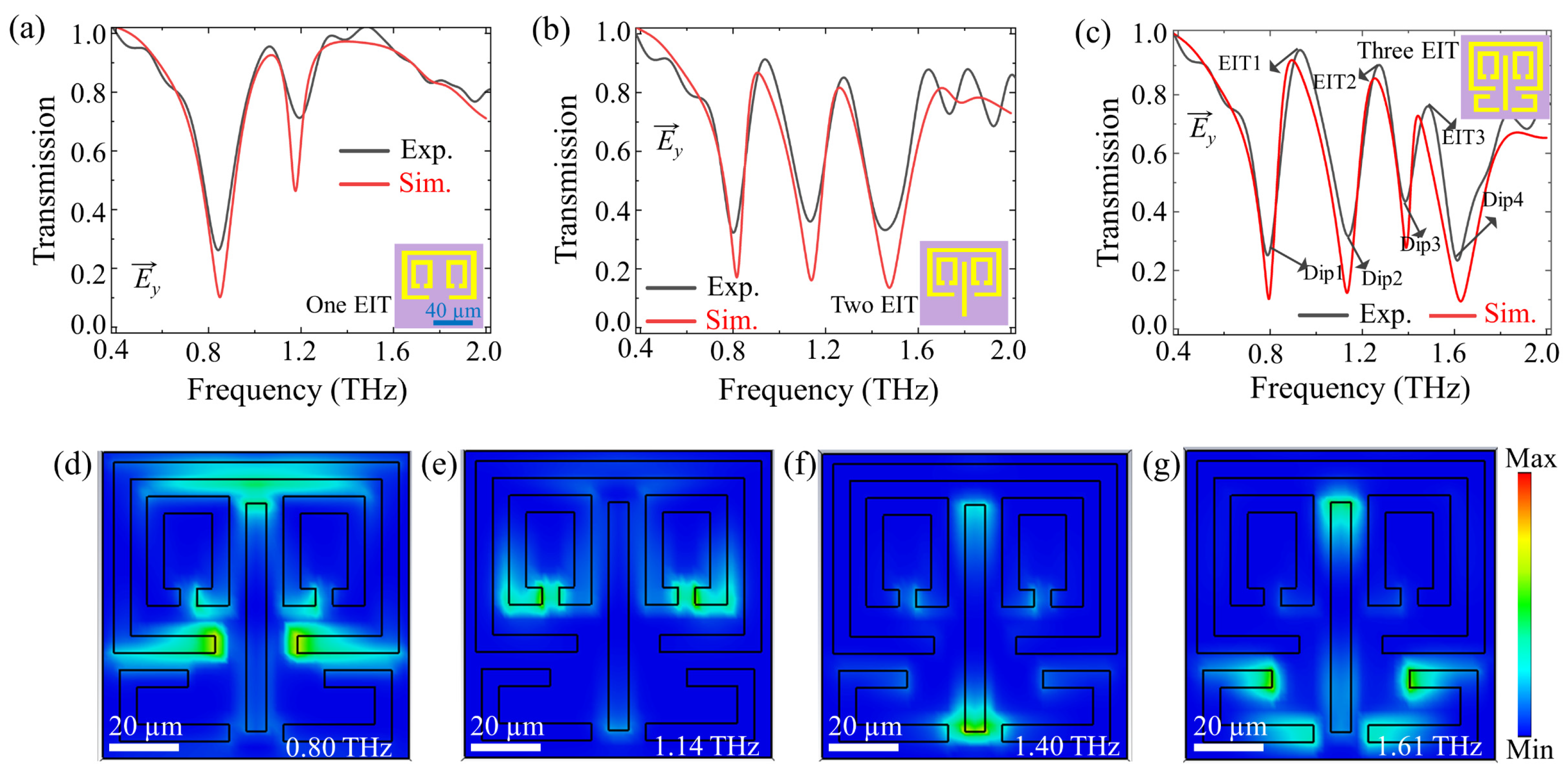
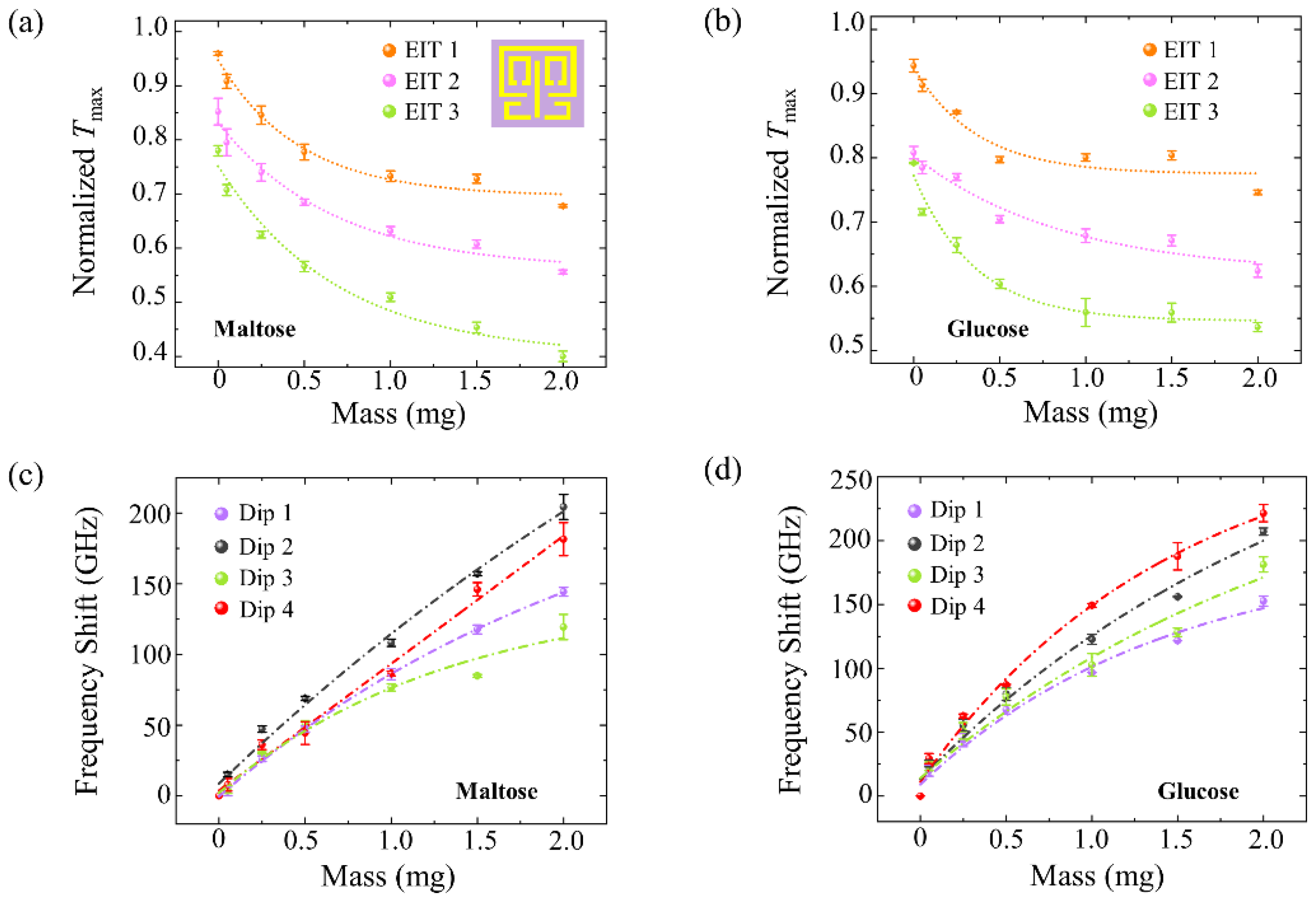
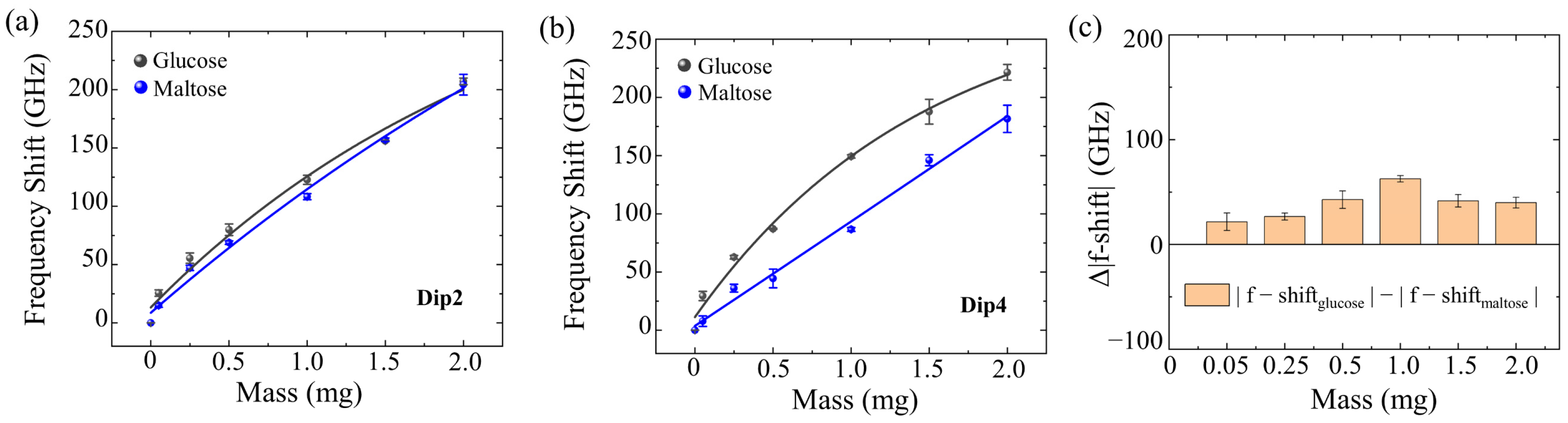
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wilke, I.; Sengupta, S. Terahertz spectroscopy: Principles and applications. Opt. Sci. Eng. 2007, 1, 41–72. [Google Scholar]
- Pawar, A.Y.; Sonawane, D.D.; Erande, K.B.; Derle, D.V. Terahertz technology and its applications. Drug Invent. Today 2013, 5, 157–163. [Google Scholar] [CrossRef]
- Cheon, H.; Yang, H.-J.; Lee, S.-H.; Kim, Y.A.; Son, J.-H. Terahertz molecular resonance of cancer DNA. Sci. Rep. 2016, 6, 37103. [Google Scholar] [CrossRef]
- Yang, X.; Zhao, X.; Yang, K.; Liu, Y.; Liu, Y.; Fu, W.; Luo, Y. Biomedical applications of terahertz spectroscopy and imaging. Trends Biotechnol. 2016, 34, 810–824. [Google Scholar] [CrossRef]
- Kim, Y.; Salim, A.; Lim, S. Millimeter-wave-based spoof localized surface plasmonic resonator for sensing glucose concentration. Biosensors 2021, 11, 358. [Google Scholar] [CrossRef]
- Jiang, L.; Zhang, K.; Yao, Y.; Li, S.; Li, J.; Tian, Z.; Zhang, W. Terahertz optoacoustic detection of aqueous salt solutions. iScience 2022, 25, 104668. [Google Scholar] [CrossRef]
- Bessou, M.; Chassagne, B.; Caumes, J.P.; Pradère, C.; Maire, P.; Tondusson, M.; Abraham, E. Three-dimensional terahertz computed tomography of human bones. Appl. Opt. 2012, 51, 6738–6744. [Google Scholar] [CrossRef] [PubMed]
- Walther, M.; Fischer, B.; Schall, M.; Helm, H.; Jepsen, P.U. Far-infrared vibrational spectra of all-trans, 9-cis and 13-cis retinal measured by thz time-domain spectroscopy. Chem. Phys. Lett. 2000, 332, 389–395. [Google Scholar] [CrossRef]
- Deng, X.; Shen, Y.; Liu, B.; Song, Z.; He, X.; Zhang, Q.; Ling, D.; Liu, D.; Wei, D. Terahertz metamaterial sensor for sensitive detection of citrate salt solutions. Biosensors 2022, 12, 408. [Google Scholar] [CrossRef]
- Lee, K.; Kang, H.; Lee, S.; Kim, H.S.; Kim, C.; Hun Kim, J.; Lee, T.; Son, J.H.; Park, Q.H.; Seo, M. Highly sensitive and selective sugar detection by terahertz nano-antennas. Sci. Rep. 2015, 5, 15459. [Google Scholar] [CrossRef]
- Seo, M.; Park, H.-R. Terahertz biochemical molecule-specific sensors. Adv. Opt. Mater. 2020, 8, 1900662. [Google Scholar] [CrossRef]
- Yang, K.; Yang, X.; Zhao, X.; Lamy de la Chapelle, M.; Fu, W. Thz spectroscopy for a rapid and label-free cell viability assay in a microfluidic chip based on an optical clearing agent. Anal. Chem. 2019, 91, 785–791. [Google Scholar] [CrossRef] [PubMed]
- Yang, K.; Li, J.; Lamy de la Chapelle, M.; Huang, G.; Wang, Y.; Zhang, J.; Xu, D.; Yao, J.; Yang, X.; Fu, W. A terahertz metamaterial biosensor for sensitive detection of micrornas based on gold-nanoparticles and strand displacement amplification. Biosens. Bioelectron. 2021, 175, 112874. [Google Scholar] [CrossRef] [PubMed]
- Yahiaoui, R.; Burrow, J.A.; Mekonen, S.M.; Sarangan, A.; Mathews, J.; Agha, I.; Searles, T.A. Electromagnetically induced transparency control in terahertz metasurfaces based on bright-bright mode coupling. Phys. Rev. B 2018, 97, 155403. [Google Scholar] [CrossRef]
- Manjappa, M.; Chiam, S.-Y.; Cong, L.; Bettiol, A.A.; Zhang, W.; Singh, R. Tailoring the slow light behavior in terahertz metasurfaces. Appl. Phys. Lett. 2015, 106, 181101. [Google Scholar] [CrossRef]
- Chen, H.T.; Padilla, W.J.; Zide, J.M.O.; Gossard, A.C.; Taylor, A.J.; Averitt, R.D. Active terahertz metamaterial devices. Nature 2006, 444, 597–600. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Wu, J.; Jin, B.; Jia, X.; Kang, L.; Xu, W.; Wang, H.; Chen, J.; Tonouchi, M.; Wu, P. Tunable electromagnetically induced transparency from a superconducting terahertz metamaterial. Appl. Phys. Lett. 2017, 110, 241105. [Google Scholar] [CrossRef]
- Simovski, C.R.; Belov, P.A.; Atrashchenko, A.V.; Kivshar, Y.S. Wire metamaterials: Physics and applications. Adv. Mater. 2012, 24, 4229–4248. [Google Scholar] [CrossRef]
- Choi, M.; Lee, S.H.; Kim, Y.; Kang, S.B.; Shin, J.; Kwak, M.H.; Lee, Y.H.; Park, N.; Min, B. A terahertz metamaterial with unnaturally high refractive index. Nature 2011, 470, 369–373. [Google Scholar] [CrossRef]
- Li, Q.; Liu, S.; Zhang, X.; Wang, S.; Chen, T. Electromagnetically induced transparency in terahertz metasurface composed of meanderline and u-shaped resonators. Opt. Express 2020, 28, 8792–8801. [Google Scholar] [CrossRef]
- Zhou, T.; Chen, S.; Zhang, X.; Zhang, X.; Hu, H.; Wang, Y. Electromagnetically induced transparency based on a carbon nanotube film terahertz metasurface. Opt. Express 2022, 30, 15436–15445. [Google Scholar] [CrossRef]
- Zheng, G.; Muhlenbernd, H.; Kenney, M.; Li, G.; Zentgraf, T.; Zhang, S. Metasurface holograms reaching 80% efficiency. Nat. Nanotechnol. 2015, 10, 308–312. [Google Scholar] [CrossRef] [PubMed]
- Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Planar photonics with metasurfaces. Science 2013, 339, 1232009. [Google Scholar] [CrossRef] [PubMed]
- Menzel, C.; Rockstuhl, C.; Lederer, F. An advanced jones calculus for the classification of periodic metamaterials. Phys. Rev. A 2010, 82, 3464–3467. [Google Scholar] [CrossRef]
- Yahiaoui, R.; Manjappa, M.; Srivastava, Y.K.; Singh, R. Active control and switching of broadband electromagnetically induced transparency in symmetric metadevices. Appl. Phys. Lett. 2017, 111, 021101. [Google Scholar] [CrossRef]
- Yue, Y.; He, F.; Chen, L.; Shu, F.; Jing, X.; Hong, Z. Analogue of electromagnetically induced transparency in a metal-dielectric bilayer terahertz metamaterial. Opt. Express 2021, 29, 21810–21819. [Google Scholar] [CrossRef]
- Krishnamoorthy, H.N.S.; Jacob, Z.; Narimanov, E.; Kretzschmar, I.; Menon, V.M. Topological transitions in metamaterials. Science 2012, 336, 205–209. [Google Scholar] [CrossRef]
- Sreekanth, K.V.; Biaglow, T.; Strangi, G. Directional spontaneous emission enhancement in hyperbolic metamaterials. J. Appl. Phys. 2013, 114, 134306. [Google Scholar] [CrossRef]
- Tao, H.; Chieffo, L.R.; Brenckle, M.A.; Siebert, S.M.; Liu, M.; Strikwerda, A.C.; Fan, K.; Kaplan, D.L.; Zhang, X.; Averitt, R.D.; et al. Metamaterials on paper as a sensing platform. Adv. Mater. 2011, 23, 3197–3201. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, X.; Huang, G.; Yang, X.; Zhang, Y.; Zhan, X.; Tian, H.; Xiong, Y.; Wang, Y.; Fu, W. Molecule-specific terahertz biosensors based on an aptamer hydrogel-functionalized metamaterial for sensitive assays in aqueous environments. ACS Sens. 2021, 6, 1884–1890. [Google Scholar] [CrossRef]
- Zhang, S.; Genov, D.A.; Wang, Y.; Liu, M.; Zhang, X. Plasmon-induced transparency in metamaterials. Phys. Rev. Lett. 2008, 101, 218–221. [Google Scholar] [CrossRef] [PubMed]
- Gu, J.; Singh, R.; Liu, X.; Zhang, X.; Ma, Y.; Zhang, S.; Maier, S.A.; Tian, Z.; Azad, A.K.; Chen, H.-T.; et al. Active control of electromagnetically induced transparency analogue in terahertz metamaterials. Nat. Commun. 2012, 3, 1151. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Jiang, T.; Sun, H.; Tong, M.; You, J.; Zheng, X.; Xu, Z.; Cheng, X. Ultrafast frequency shift of electromagnetically induced transparency in terahertz metaphotonic devices. Laser Photonics Rev. 2020, 14, 1900338. [Google Scholar] [CrossRef]
- Hu, Y.; Xiong, Y. High-q and tunable analog of electromagnetically induced transparency in terahertz all-dielectric metamaterial. Appl. Opt. 2022, 61, 1500–1506. [Google Scholar] [CrossRef] [PubMed]
- Shi, X.; Tong, Y.; Ding, Y. Polarization-independent and angle-insensitive tunable electromagnetically induced transparency in terahertz metamaterials. Appl. Opt. 2021, 60, 7784–7789. [Google Scholar] [CrossRef]
- Zhang, Y.; Qiu, F.; Liang, L.; Yao, H.; Yan, X.; Liu, W.; Huang, C.; Yao, J. Three-stimulus control ultrasensitive dirac point modulator using an electromagnetically induced transparency-like terahertz metasurface with graphene. Opt. Express 2022, 30, 24703–24715. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.; Rockstuhl, C.; Lederer, F.; Zhang, W. Coupling between a dark and a bright eigenmode in a terahertz metamaterial. Phys. Rev. B 2009, 79, 085111. [Google Scholar] [CrossRef]
- Liu, G.-B.; Zhang, H.; Li, H.-M. Electromagnetically induced transparency metamaterial with polarization independence and multi-transmission windows. Appl. Opt. 2020, 59, 9568–9573. [Google Scholar] [CrossRef]
- Lu, X.; Ge, H.; Jiang, Y.; Zhang, Y. A dual-band high-sensitivity thz metamaterial sensor based on split metal stacking ring. Biosensors 2022, 12, 471. [Google Scholar] [CrossRef]
- Chen, C.; Yan, F.; Liu, Z.; Gong, R.; Wang, R.; Li, L. Tunable terahertz slow light of a cavity-integrated guided-mode resonance grating. J. Opt. Soc. Am. B 2021, 38, 1710–1716. [Google Scholar] [CrossRef]
- Wang, B.; Guo, T.; Gai, K.; Yan, F.; Wang, R.; Li, L. Tunable terahertz group slowing effect with plasmon-induced transparency metamaterial. Appl. Opt. 2022, 61, 3218–3222. [Google Scholar] [CrossRef]
- Xiao, B.; Tong, S.; Fyffe, A.; Shi, Z. Tunable electromagnetically induced transparency based on graphene metamaterials. Opt. Express 2020, 28, 4048–4057. [Google Scholar] [CrossRef]
- Zeng, Y.; Ling, Z.; Liu, G.; Wang, L.; Lin, Q. Tunable plasmonically induced transparency with giant group delay in gain-assisted graphene metamaterials. Opt. Express 2022, 30, 14103–14111. [Google Scholar] [CrossRef]
- Beliaev, L.Y.; Takayama, O.; Melentiev, P.N.; Lavrinenko, A.V. Photoluminescence control by hyperbolic metamaterials and metasurfaces: A review. Opto-Electron. Adv. 2021, 4, 210031. [Google Scholar] [CrossRef]
- Buono, W.T.; Forbes, A. Nonlinear optics with structured light. Opto-Electron. Adv. 2022, 5, 210174. [Google Scholar] [CrossRef]
- Cao, T.; Lian, M.; Chen, X.; Mao, L.; Liu, K.; Jia, J.; Su, Y.; Ren, H.; Zhang, S.; Xu, Y.; et al. Multi-cycle reconfigurable thz extraordinary optical transmission using chalcogenide metamaterials. Opto-Electron. Sci. 2022, 1, 210010. [Google Scholar] [CrossRef]
- Fan, Y.; Shen, N.H.; Koschny, T.; Soukoulis, C.M. Tunable terahertz meta-surface with graphene cut-wires. ACS Photonics 2015, 2, 151–156. [Google Scholar] [CrossRef]
- Zhu, L.; Li, H.; Dong, L.; Zhou, W.; Rong, M.; Zhang, X.; Guo, J. Dual-band electromagnetically induced transparency (eit) terahertz metamaterial sensor. Opt. Mater. Express 2021, 11, 2109–2121. [Google Scholar] [CrossRef]
- Liu, S.; Xu, Z.; Yin, X.; Zhao, H. Analog of multiple electromagnetically induced transparency using double-layered metasurfaces. Sci. Rep. 2020, 10, 8469. [Google Scholar] [CrossRef]
- Sun, D.; Qi, L.; Liu, Z. Terahertz broadband filter and electromagnetically induced transparency structure with complementary metasurface. Results Phys. 2020, 16, 102887. [Google Scholar] [CrossRef]
- Chen, X.; Tian, Z.; Wang, J.; Yuan, Y.; Zhang, X.; Ouyang, C.; Gu, J.; Han, J.; Zhang, W. Hysteretic behavior in ion gel-graphene hybrid terahertz modulator. Carbon 2019, 155, 514–520. [Google Scholar] [CrossRef]
- Lee, K.S.; Lu, T.M.; Zhang, X.C. Tera tool terahertz time-domain spectroscopy. IEEE Circ. Dev. Mag. 2002, 18, 23–28. [Google Scholar]
- Xu, W.; Xie, L.; Zhu, J.; Xu, X.; Ye, Z.; Wang, C.; Ma, Y.; Ying, Y. Gold nanoparticle-based terahertz metamaterial sensors: Mechanisms and applications. ACS Photonics 2016, 3, 2308–2314. [Google Scholar] [CrossRef]
- Xu, W.; Xie, L.; Zhu, J.; Tang, L.; Singh, R.; Wang, C.; Ma, Y.; Chen, H.-T.; Ying, Y. Terahertz biosensing with a graphene-metamaterial heterostructure platform. Carbon 2019, 141, 247–252. [Google Scholar] [CrossRef]
- Ahmadivand, A.; Gerislioglu, B.; Ramezani, Z.; Kaushik, A.; Manickam, P.; Ghoreishi, S.A. Functionalized terahertz plasmonic metasensors: Femtomolar-level detection of SARS-CoV-2 spike proteins. Biosens. Bioelectron. 2021, 177, 112971. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, M.; Guo, G.; Xu, Y.; Yao, Z.; Zhang, S.; Yan, Y.; Tian, Z. Exploring the Application of Multi-Resonant Bands Terahertz Metamaterials in the Field of Carbohydrate Films Sensing. Biosensors 2023, 13, 606. https://doi.org/10.3390/bios13060606
Zhang M, Guo G, Xu Y, Yao Z, Zhang S, Yan Y, Tian Z. Exploring the Application of Multi-Resonant Bands Terahertz Metamaterials in the Field of Carbohydrate Films Sensing. Biosensors. 2023; 13(6):606. https://doi.org/10.3390/bios13060606
Chicago/Turabian StyleZhang, Min, Guanxuan Guo, Yihan Xu, Zhibo Yao, Shoujun Zhang, Yuyue Yan, and Zhen Tian. 2023. "Exploring the Application of Multi-Resonant Bands Terahertz Metamaterials in the Field of Carbohydrate Films Sensing" Biosensors 13, no. 6: 606. https://doi.org/10.3390/bios13060606
APA StyleZhang, M., Guo, G., Xu, Y., Yao, Z., Zhang, S., Yan, Y., & Tian, Z. (2023). Exploring the Application of Multi-Resonant Bands Terahertz Metamaterials in the Field of Carbohydrate Films Sensing. Biosensors, 13(6), 606. https://doi.org/10.3390/bios13060606




