Highly Sensitive Refractive Index Sensor Based on Polymer Bragg Grating: A Case Study on Extracellular Vesicles Detection
Abstract
1. Introduction
2. Sensor Configuration and Modeling
3. Results and Discussions
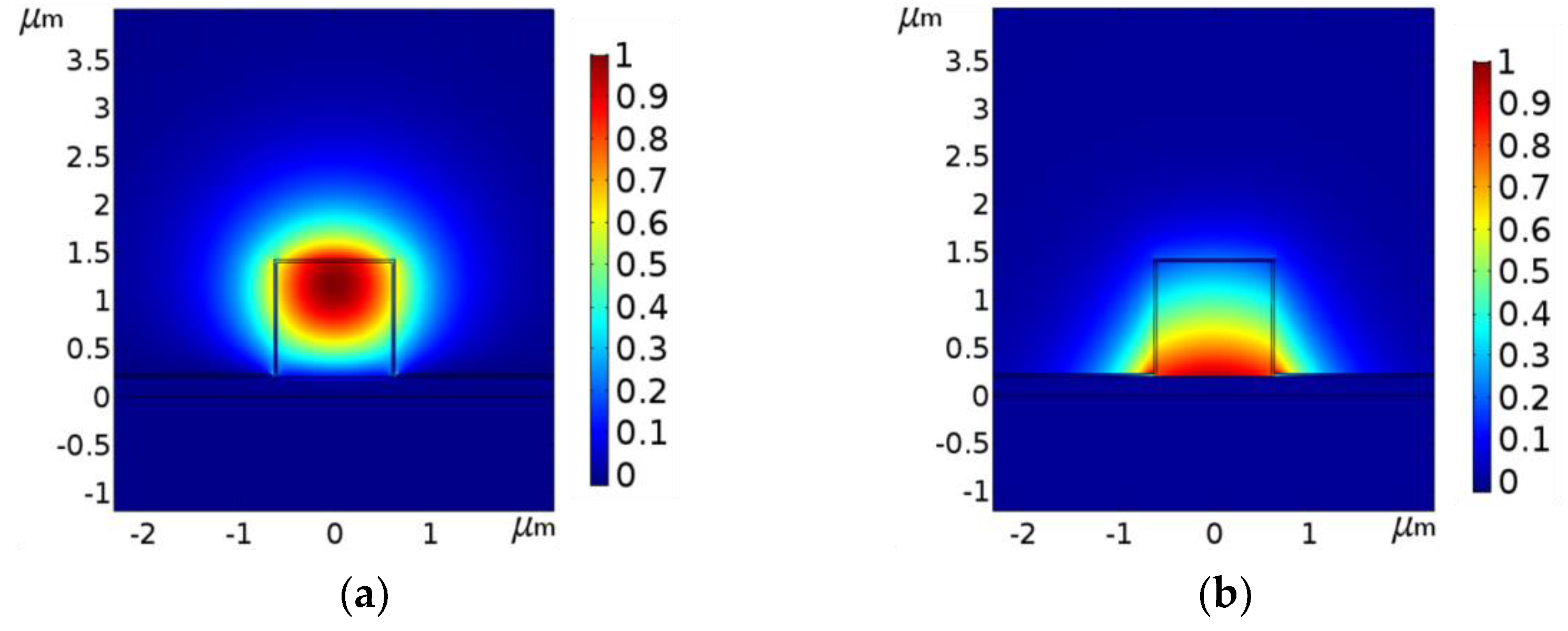
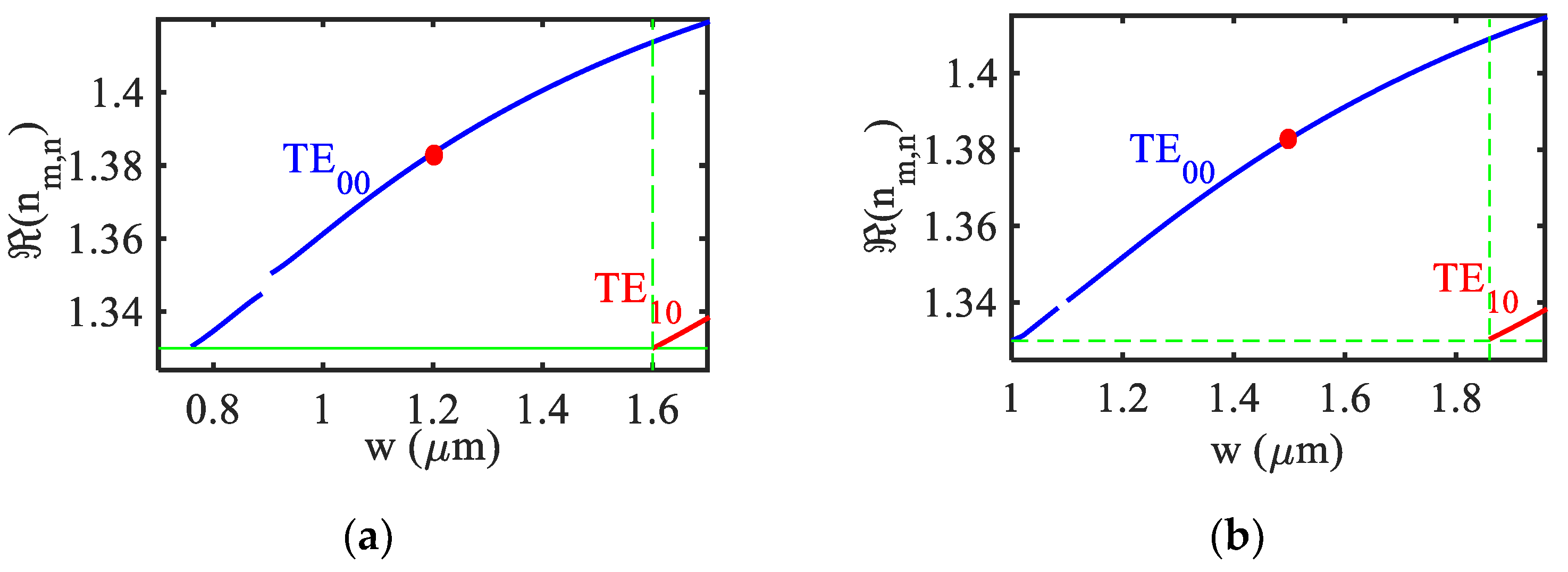
3.1. Design and Performance Estimation
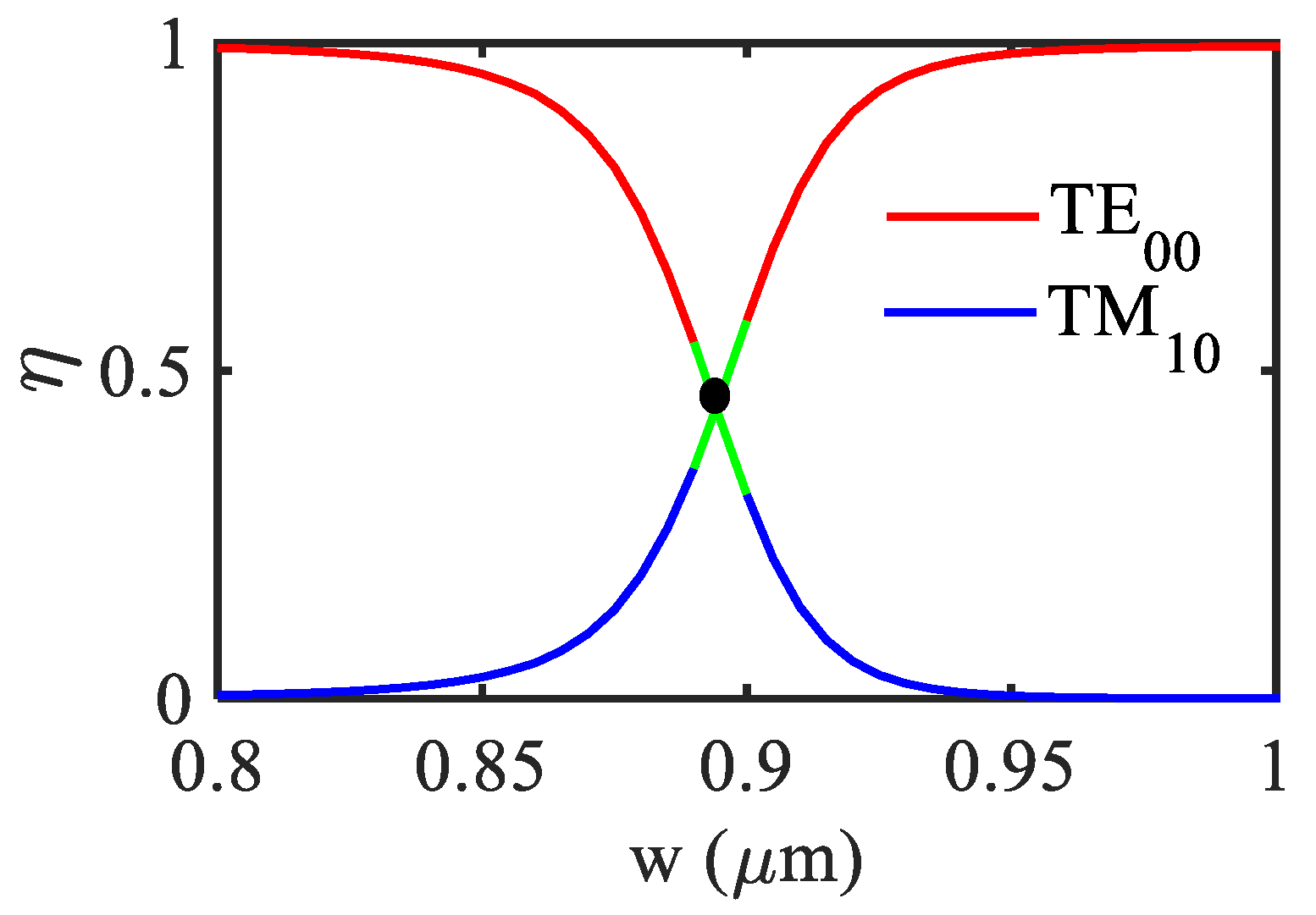
3.2. EV Detection: A Case of Study
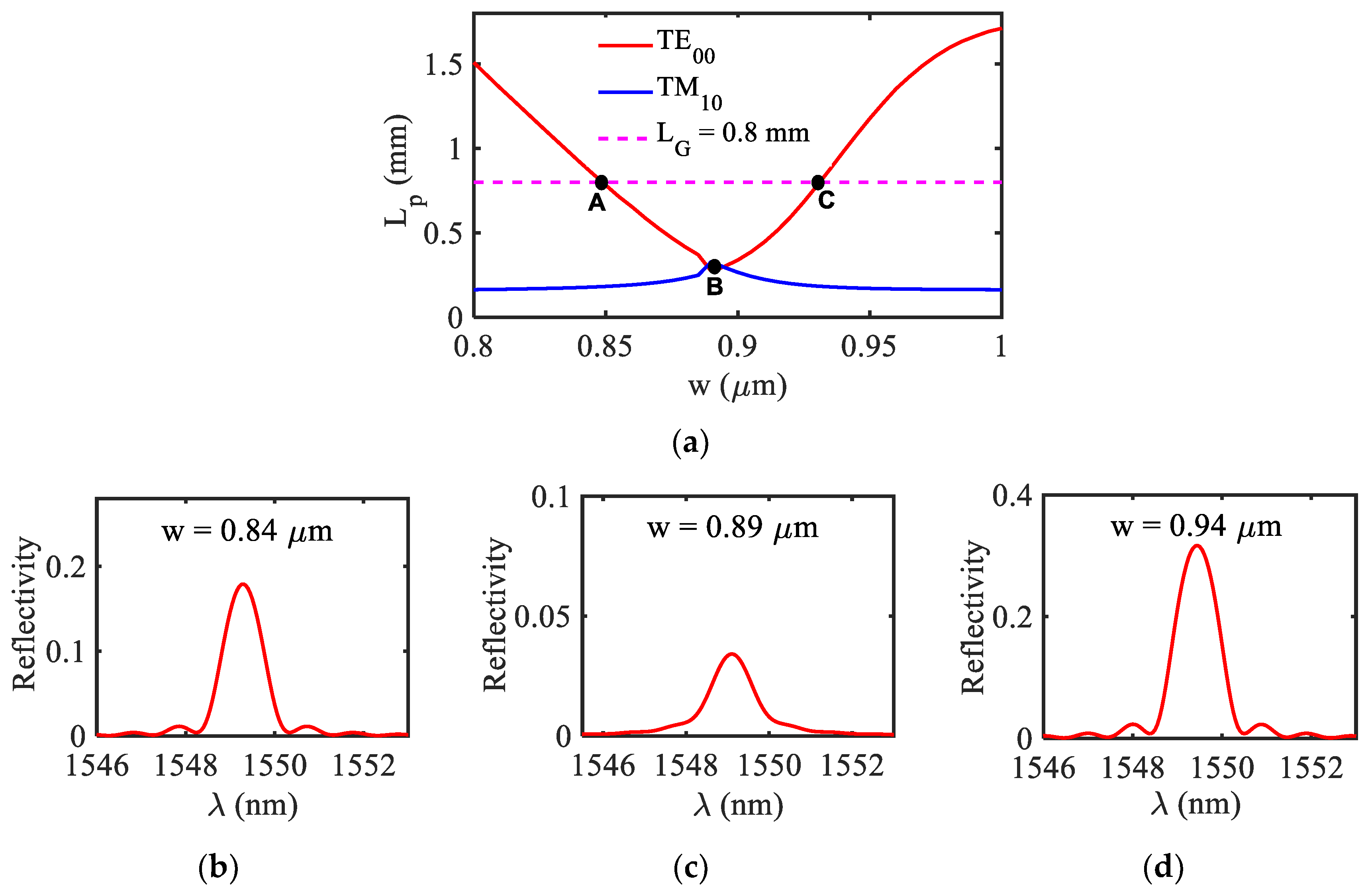
3.3. Fabrication Tolerances and Readout Concept
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Thomas-Peter, N.; Langford, N.K.; Datta, A.; Zhang, L.; Smith, B.J.; Spring, J.B.; Metcalf, B.J.; Coldenstrodt-Ronge, H.B.; Hu, M.; Nunn, J.; et al. Integrated photonic sensing. New J. Phys. 2011, 13, 055024. [Google Scholar] [CrossRef]
- Luan, E.; Shoman, H.; Ratner, D.M.; Cheung, K.C.; Chrostowski, L. Silicon Photonic Biosensors Using Label-Free Detection. Sensors 2018, 18, 3519. [Google Scholar] [CrossRef] [PubMed]
- Blanco, F.J.; Agirregabiria, M.; Berganzo, J.; Mayora, K.; Elizalde, J.; Calle, A.; Dominguez, C.; Lechuga, L.M. Microfluidic-optical integrated CMOS compatible devices for label-free biochemical sensing. J. Micromech. Microeng. 2006, 16, 1006. [Google Scholar] [CrossRef]
- Brunetti, G.; Conteduca, D.; Armenise, M.N.; Ciminelli, C. Novel Micro-Nano Optoelectronic Biosensor for Label-Free Real-Time Biofilm Monitoring. Biosensors 2021, 11, 361. [Google Scholar] [CrossRef]
- Sinibaldi, A. Cancer Biomarker Detection with Photonic Crystals-Based Biosensors: An Overview. J. Light. Technol. 2021, 39, 3871–3881. [Google Scholar] [CrossRef]
- Gavela, A.F.; García, D.G.; Ramirez, J.C.; Lechuga, L.M. Last Advances in Silicon-Based Optical Biosensors. Sensors 2016, 16, 285. [Google Scholar] [CrossRef]
- Ochoa, M.; Algorri, J.F.; Roldán-Varona, P.; Rodríguez-Cobo, L.; López-Higuera, J.M. Recent Advances in Biomedical Photonic Sensors: A Focus on Optical-Fibre-Based Sensing. Sensors 2021, 21, 6469. [Google Scholar] [CrossRef]
- Ahmed, I.; Marbán, E. Exosomes: Fundamental biology and roles in cardiovascular physiology. Annu. Rev. Physiol. 2016, 78, 67–83. [Google Scholar]
- Ignatiadis, M.; Sledge, G.W.; Jeffrey, S.S. Liquid biopsy enters the clinic—Implementation issues and future challenges. Nat. Rev. Clin. Oncol. 2021, 18, 297–312. [Google Scholar] [CrossRef]
- Wan, J.C.M.; Massie, C.; Garcia-Corbacho, J.; Mouliere, F.; Brenton, J.D.; Caldas, C.; Pacey, S.; Baird, R.; Rosenfeld, N. Liquid biopsies come of age: Towards implementation of circulating tumour DNA. Nat. Rev. Cancer 2017, 17, 223–238. [Google Scholar] [CrossRef]
- Lim, C.Z.J.; Zhang, L.; Zhang, Y.; Sundah, N.R.; Shao, H. New Sensors for Extracellular Vesicles: Insights on Constituent and Associated Biomarkers. ACS Sens. 2020, 5, 4–12. [Google Scholar] [CrossRef] [PubMed]
- Properzi, F.; Logozzi, M.; Fais, S. Exosomes: The future of biomarkers in medicine. Biomark. Med. 2013, 7, 769–778. [Google Scholar] [CrossRef] [PubMed]
- Fuhrmann, G.; Neuer, A.L.; Herrmann, I.K. Extracellular vesicles—A promising avenue for the detection and treatment of infectious diseases? Eur. J. Pharm. Biopharm. 2017, 118, 56–61. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Park, J.S.; Huang, C.-H.; Jo, A.; Cook, K.; Wang, R.; Lin, H.-Y.; Van Deun, J.; Li, H.; Min, J.; et al. An integrated magneto-electrochemical device for the rapid profiling of tumour extracellular vesicles from blood plasma. Nat. Biomed. Eng. 2021, 5, 678–689. [Google Scholar] [CrossRef]
- Im, H.; Shao, H.; Park, Y.I.; Peterson, V.M.; Castro, C.M.; Weissleder, R.; Lee, H. Label-free detection and molecular profiling of exosomes with a nano-plasmonic sensor. Nat. Biotechnol. 2014, 32, 490–495. [Google Scholar] [CrossRef]
- Conteduca, D.; Quinn, S.D.; Krauss, T.F. Dielectric metasurface for high-precision detection of large unilamellar vesicles. J. Opt. 2022, 23, 114002. [Google Scholar] [CrossRef]
- Fan, B.; Liu, F.; Li, Y.; Huang, Y.; Miura, Y.; Ohnishi, D. Refractive index sensor based on hybrid coupler with short-range surface plasmon polariton and dielectric waveguide. Appl. Phys. Lett. 2012, 100, 111108. [Google Scholar] [CrossRef]
- Sinibaldi, A.; Danz, N.; Descrovi, E.; Munzert, P.; Schulz, U.; Sonntag, F.; Dominici, L.; Michelotti, F. Direct comparison of the performance of Bloch surface wave and surface plasmon polariton sensors. Sens. Actuators B Chem. 2012, 174, 292–298. [Google Scholar] [CrossRef]
- Lei, Z.L.; Guo, B. 2D Material-Based Optical Biosensor: Status and Prospect. Adv. Sci. 2022, 9, 2102924. [Google Scholar] [CrossRef]
- Pal, A.; Jha, A. A theoretical analysis on sensitivity improvement of an SPR refractive index sensor with graphene and barium titanate nanosheets. Optik 2021, 231, 166378. [Google Scholar] [CrossRef]
- Saha, N.; Kumar, A. Toward 100 Micrometer per Refractive Index Unit Sensitive Sensor Using a Compact Long-Period Grating Inscribed Metal Clad Ridge Waveguide. J. Light. Technol. 2018, 36, 2024–2030. [Google Scholar] [CrossRef]
- Saha, N.; Kumar, A. Highly Sensitive Refractive Index Sensor Based on Mode Transition in a Dual Resonance Long Period Grating Inscribed Ridge Waveguide. J. Light. Technol. 2019, 37, 5576–5582. [Google Scholar] [CrossRef]
- Dwivedi, R.; Kumar, A. A compact and ultra high sensitive RI sensor using modal interference in an integrated optic waveguide with metal under-cladding. Sens. Actuators B Chem. 2017, 240, 1302–1307. [Google Scholar] [CrossRef]
- Butt, M.A.; Khonina, S.N.; Kazanskiy, N.L. Sensitivity Enhancement of Silicon Strip Waveguide Ring Resonator by Incorporating a Thin Metal Film. IEEE Sens. J. 2020, 20, 1355–1362. [Google Scholar] [CrossRef]
- Ciminelli, C.; Campanella, C.M.; Dell’Olio, F.; Campanella, C.E.; Armenise, M.N. Label-free optical resonant sensors for biochemical applications. Prog. Quantum Electron. 2013, 37, 51–107. [Google Scholar] [CrossRef]
- Dai, X.; Mihailov, S.J.; Callender, C.L.; Blanchetière, C.; Walker, R.B. Ridge-waveguide-based polarization insensitive Bragg grating refractometer. Meas. Sci. Technol. 2006, 17, 1752–1756. [Google Scholar] [CrossRef]
- Tripathi, S.M.; Kumar, A.; Marin, E.; Meunier, J.-P. Bragg grating based biochemical sensor using submicron Si/SiO2 waveguides for lab-on-a-chip applications: A novel design. Appl. Opt. 2009, 48, 4562–4567. [Google Scholar] [CrossRef]
- Heinsalu, S.; Isogai, Y.; Kawano, A.; Matsushima, Y.; Ishikawa, H.; Utaka, K. Proposal and analysis of ultra-high amplitude-sensitive refractive index sensor by thick silicon multi-slot sub-wavelength Bragg grating waveguide. Opt. Commun. 2022, 505, 127533. [Google Scholar] [CrossRef]
- Pérez-Armenta, C.; Ortega-Moñux, A.; Čtyroký, J.; Cheben, P.; Schmid, J.H.; Halir, R.; Molina-Fernández, I.; Wangüemert-Pérez, J.G. Narrowband Bragg filters based on subwavelength grating waveguides for silicon photonic sensing. Opt. Express 2020, 28, 37971–37985. [Google Scholar] [CrossRef]
- Saha, N.; Kumar, A.; Mukherjee, A. Enhancement of refractive index sensitivity of Bragg-gratings based optical waveguide sensors using a metal under-cladding. Opt. Commun. 2017, 396, 83–87. [Google Scholar] [CrossRef]
- Hessler, S.; Knopf, S.; Rommel, M.; Girschikofsky, M.; Schmauss, B.; Hellmann, R. Advancing the sensitivity of integrated epoxy-based Bragg grating refractometry by high-index nanolayers. Opt. Lett. 2020, 45, 5510–5513. [Google Scholar] [CrossRef] [PubMed]
- Kumar, A.; Gosciniak, J.; Volkov, V.S.; Papaioannou, S.; Kalavrouziotis, D.; Vyrsokinos, K.; Weeber, J.; Hassan, K.; Markey, L.; Dereux, A.; et al. Dielectric-loaded plasmonic waveguide components: Going practical. Laser Photonics Rev. 2013, 7, 938–951. [Google Scholar] [CrossRef]
- Rosenberger, M.; Girschikofsky, M.; Förthner, M.; Bellel, S.; Rommel, M.; Frey, L.; Schmauss, B.; Hellmann, R. TiO2 surface functionalization of COC based planar waveguide Bragg gratings for refractive index sensing. J. Opt. 2018, 20, 01LT02. [Google Scholar] [CrossRef]
- Rosenberger, M.; Koller, G.; Belle, S.; Schmauss, B.; Hellmann, R. Planar Bragg grating in bulk Polymethylmethacrylate. Opt. Express 2012, 20, 27288–27296. [Google Scholar] [CrossRef]
- Bundalo, I.-L.; Nielsen, K.; Markos, C.; Bang, O. Bragg grating writing in PMMA microstructured polymer optical fibers in less than 7 min. Opt. Express 2014, 22, 5270–5276. [Google Scholar] [CrossRef]
- Beadie, G.; Brindza, M.; Flynn, R.A.; Rosenberg, A.; Shirk, J.S. Refractive index measurements of poly(methyl methacrylate) (PMMA) from 0.4–1.6 μm. Appl. Opt. 2015, 54, F139–F143. [Google Scholar] [CrossRef]
- Evans, C.C. Nonlinear Optics in Titanium Dioxide: From Bulk to Integrated Optical Devices. Ph.D. Thesis, Harvard University, Cambridge, MA, USA, 2013. [Google Scholar]
- Jitender; Kumar, A.; Varshney, R.K.; Kumar, M. Calculation of propagation characteristics of surface plasmons in gold/silver nanowires. Appl. Opt. 2015, 54, 3715–3719. [Google Scholar] [CrossRef]
- Saha, N.; Kumar, A. Highly sensitive temperature sensor based on a long period grating inscribed metal clad ridge waveguide with PDMS surrounding. OSA Contin. 2019, 2, 946–956. [Google Scholar] [CrossRef]
- Dai, D.; Zhang, M. Mode hybridization and conversion in silicon-on-insulator nanowires with angled sidewalls. Opt. Express 2015, 23, 32452–32464. [Google Scholar] [CrossRef]
- Kaushalram, A.; Hegde, G.; Talabattula, S. Mode hybridization analysis in thin film lithium niobate strip multimode waveguides. Sci. Rep. 2020, 10, 16692. [Google Scholar] [CrossRef]
- Jia, S.; Zhang, R.; Li, Z.; Li, J. Clinical and biological significance of circulating tumor cells, circulating tumor DNA, and exosomes as biomarkers in colorectal cancer. Oncotarget 2017, 8, 55632–55645. [Google Scholar] [CrossRef] [PubMed]
- Chin, L.K.; Son, T.; Hong, J.-S.; Liu, A.-Q.; Skog, J.; Castro, C.M.; Weissleder, R.; Lee, H.; Im, H. Plasmonic Sensors for Extracellular Vesicle Analysis: From Scientific Development to Translational Research. ACS Nano 2020, 14, 14528–14548. [Google Scholar] [CrossRef] [PubMed]
- Panindre, P.; Mousavi, N.S.S.; Kumar, S. Design optimization of a single-mode microring resonator for label-free detection of biomarkers within a tunable spectral range of 2 nm. In Proceedings of theSPIE 10711, Biomedical Imaging and Sensing Conference, Yokohama, Japan, 25–27 April 2018; p. 1071126. [Google Scholar]
- Andersen, M.; Painter, L.R.; Nir, S. Dispersion Equation and Polarizability of Bovine Serum Albumin from Measurements of Refractive Indices. Biopolymers 1974, 13, 1261. [Google Scholar] [CrossRef]
- Arwin, H. Optical Properties of Thin Layers of Bovine Serum Albumin, γ-Globulin, and Hemoglobin. Appl. Spectrosc. 1986, 40, 313–318. [Google Scholar] [CrossRef]
- Ma, G.J.; Ferhan, A.R.; Jackman, J.A.; Cho, N.-J. Conformational flexibility of fatty acid-free bovine serum albumin proteins enables superior antifouling coatings. Commun. Mater. 2020, 1, 45. [Google Scholar] [CrossRef]
- Rauf, S.; Zhou, D.; Abell, C.; Klenerman, D.; Kang, D.-J. Building three-dimensional nanostructures with active enzymes by surface templated layer-by-layer assembly. Chem. Commun. 2006, 16, 1721–1723. [Google Scholar] [CrossRef]
- Claes, T.; Molera, J.G.; De Vos, K.; Schacht, E.; Baets, R.; Bienstman, P. Label-Free Biosensing With a Slot-Waveguide-Based Ring Resonator in Silicon on Insulator. IEEE Photonics J. 2009, 1, 197–204. [Google Scholar] [CrossRef]
- Gardiner, C.; Shaw, M.; Hole, P.; Smith, J.; Tannetta, D.; Redman, C.W.; Sargent, I.L. Measurement of refractive index by nanoparticle tracking analysis reveals heterogeneity in extracellular vesicles. J. Extracell. Vesicles 2014, 3, 25361. [Google Scholar] [CrossRef]
- Hoang, V.T.; Stępniewski, G.; Czarnecka, K.H.; Kasztelanic, R.; Long, V.C.; Xuan, K.D.; Shao, L.; Śmietana, M.; Buczyński, R. Optical Properties of Buffers and Cell Culture Media for Optofluidic and Sensing Applications. Appl. Sci. 2019, 9, 1145. [Google Scholar] [CrossRef]
- Barwicz, T.; Popovic, M.A.; Watts, M.R.; Rakich, P.T.; Ippen, E.P.; Smith, H.I. Fabrication of add-drop filters based on frequency-matched microring resonators. J. Light. Technol. 2006, 24, 2207–2218. [Google Scholar] [CrossRef]
- Greibe, T.; Anhøj, T.A.; Johansen, L.S.; Han, A. Quality control of JEOL JBX-9500FSZ e-beam lithography system in a multi-user laboratory. Microelectron. Eng. 2016, 155, 25–28. [Google Scholar] [CrossRef][Green Version]
- Tachibana, Y.; Ohsaki, H.; Hayashi, A.; Mitsui, A.; Hayashi, Y. TiO2− X sputter for high rate deposition of TiO2. Vacuum 2000, 59, 836–843. [Google Scholar] [CrossRef]
- Simionescu, O.-G.; Romanițan, C.; Tutunaru, O.; Ion, V.; Buiu, O.; Avram, A. RF Magnetron Sputtering Deposition of TiO2 Thin Films in a Small Continuous Oxygen Flow Rate. Coatings 2019, 9, 442. [Google Scholar] [CrossRef]
- Wang, Q.; Du, C.; Zhang, J.; Lv, R.; Zhao, Y. Sensitivity-enhanced temperature sensor based on PDMS-coated long period fiber grating. Opt. Commun. 2016, 377, 89–93. [Google Scholar] [CrossRef]

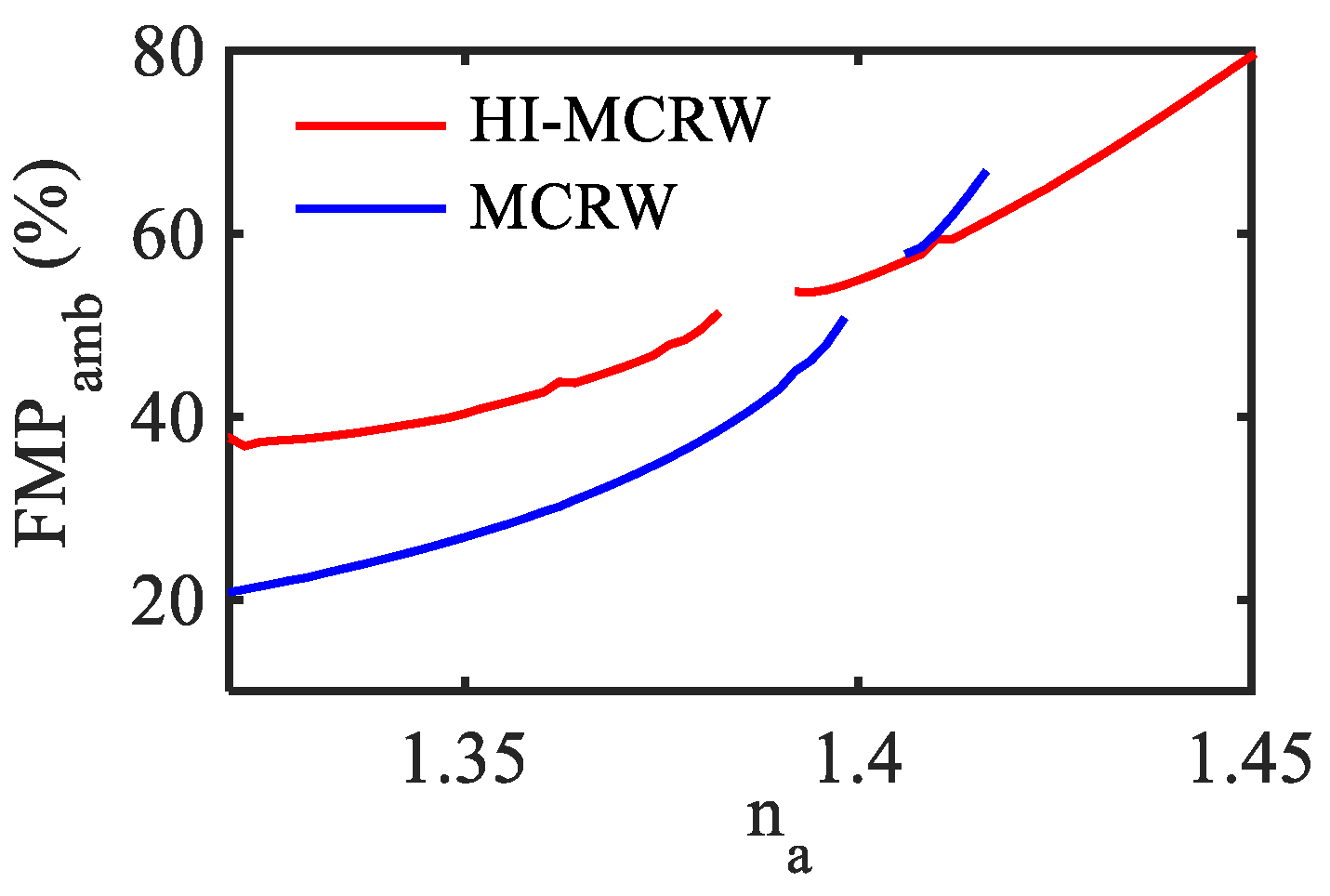

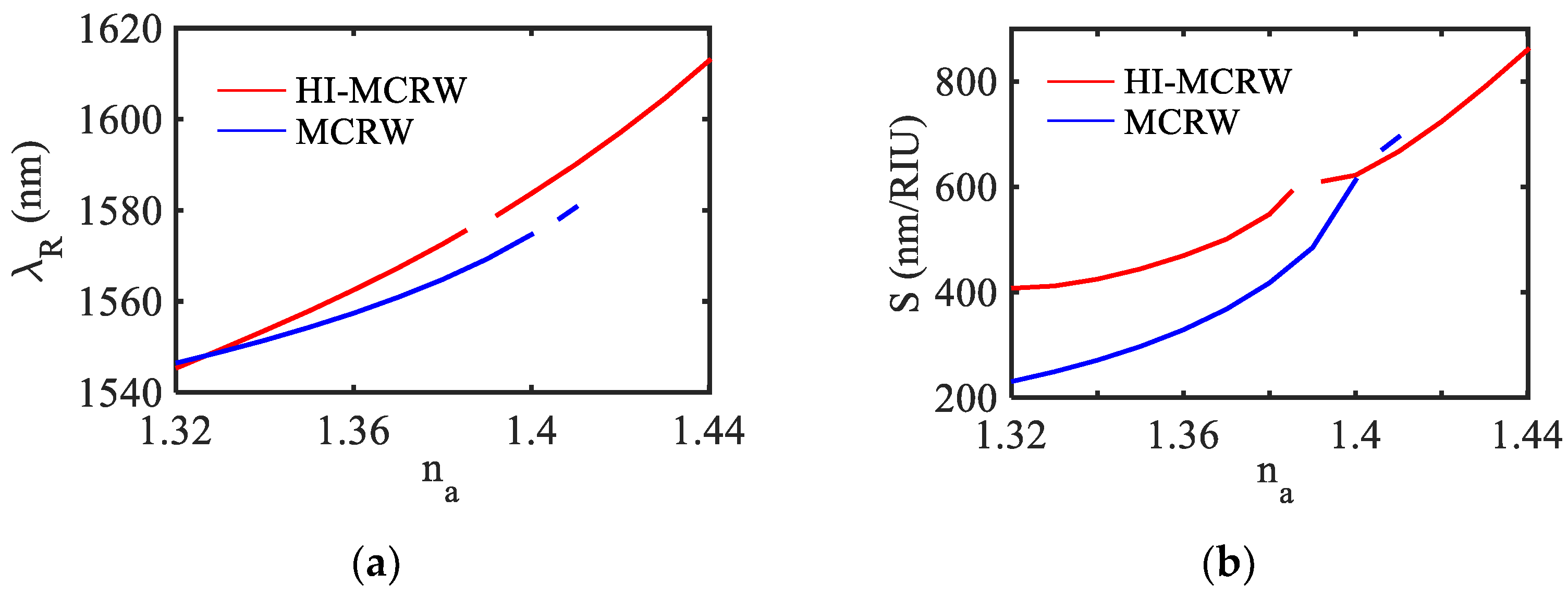
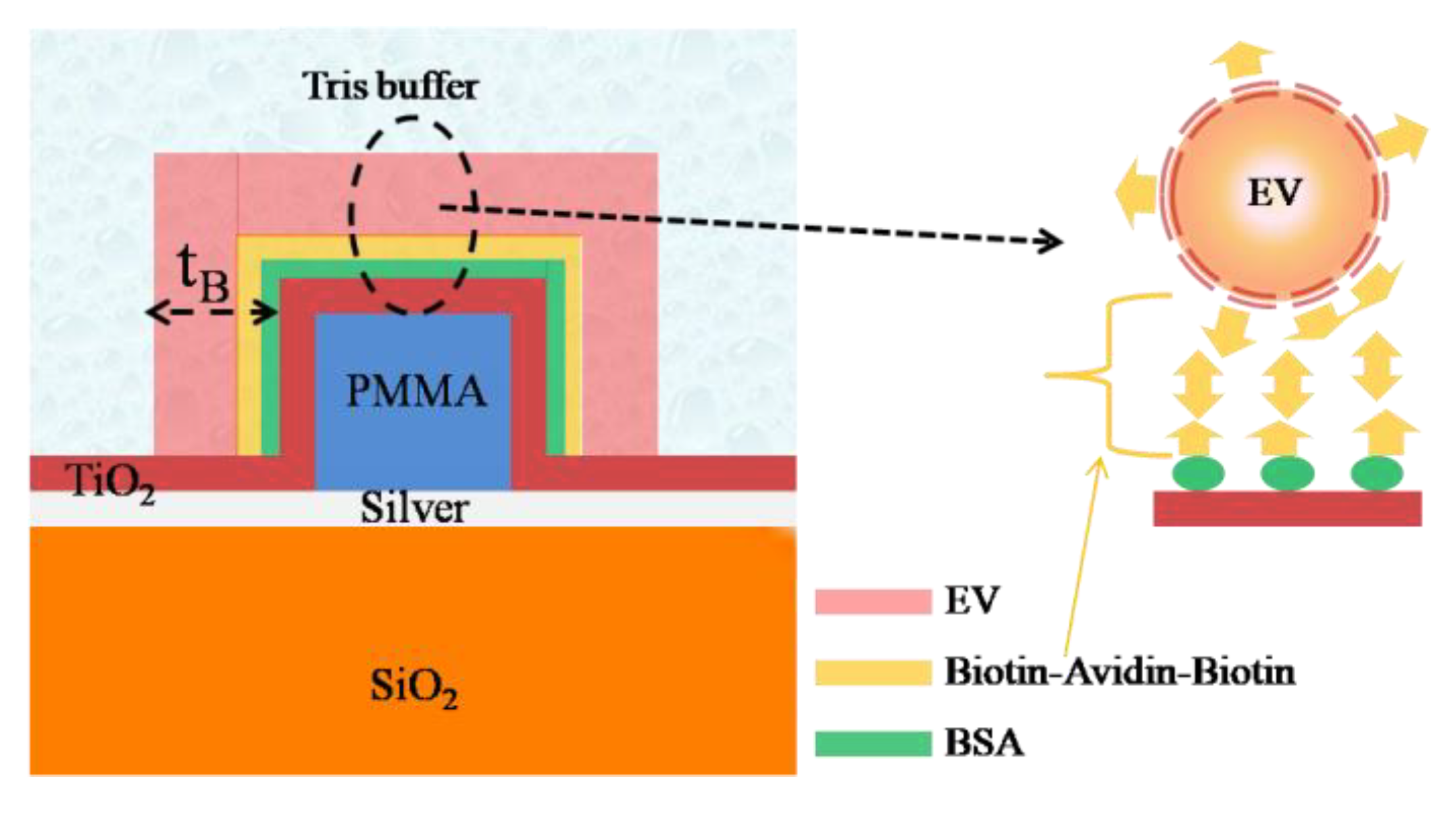

| Ref. | S (nm/RIU) | Dynamic Range (RIU) | Operating Principle |
|---|---|---|---|
| [26] | 7–25 | 1.3–1.402 | Open top ridge waveguide |
| [27] | 200–740 | 1.33–1.63 | SOI with photosensitive upper cladding |
| [29] | 507 | 1.3–1.34 | Sub-wavelength gratings |
| [30] | 237–578 | 1.3–1.4 | Metal clad ridge waveguide |
| [31] | 169–523 | 1.4–1.59 | High-index coated ridge waveguide |
| Proposed structure | 408–861 | 1.32–1.44 | High-index coated polymer waveguide with metal under cladding |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saha, N.; Brunetti, G.; Kumar, A.; Armenise, M.N.; Ciminelli, C. Highly Sensitive Refractive Index Sensor Based on Polymer Bragg Grating: A Case Study on Extracellular Vesicles Detection. Biosensors 2022, 12, 415. https://doi.org/10.3390/bios12060415
Saha N, Brunetti G, Kumar A, Armenise MN, Ciminelli C. Highly Sensitive Refractive Index Sensor Based on Polymer Bragg Grating: A Case Study on Extracellular Vesicles Detection. Biosensors. 2022; 12(6):415. https://doi.org/10.3390/bios12060415
Chicago/Turabian StyleSaha, Nabarun, Giuseppe Brunetti, Arun Kumar, Mario Nicola Armenise, and Caterina Ciminelli. 2022. "Highly Sensitive Refractive Index Sensor Based on Polymer Bragg Grating: A Case Study on Extracellular Vesicles Detection" Biosensors 12, no. 6: 415. https://doi.org/10.3390/bios12060415
APA StyleSaha, N., Brunetti, G., Kumar, A., Armenise, M. N., & Ciminelli, C. (2022). Highly Sensitive Refractive Index Sensor Based on Polymer Bragg Grating: A Case Study on Extracellular Vesicles Detection. Biosensors, 12(6), 415. https://doi.org/10.3390/bios12060415







