Bioimpedance Sensing of Implanted Stent Occlusions: Smart Stent
Abstract
:1. Introduction
2. Materials and Methods
2.1. Artery Model
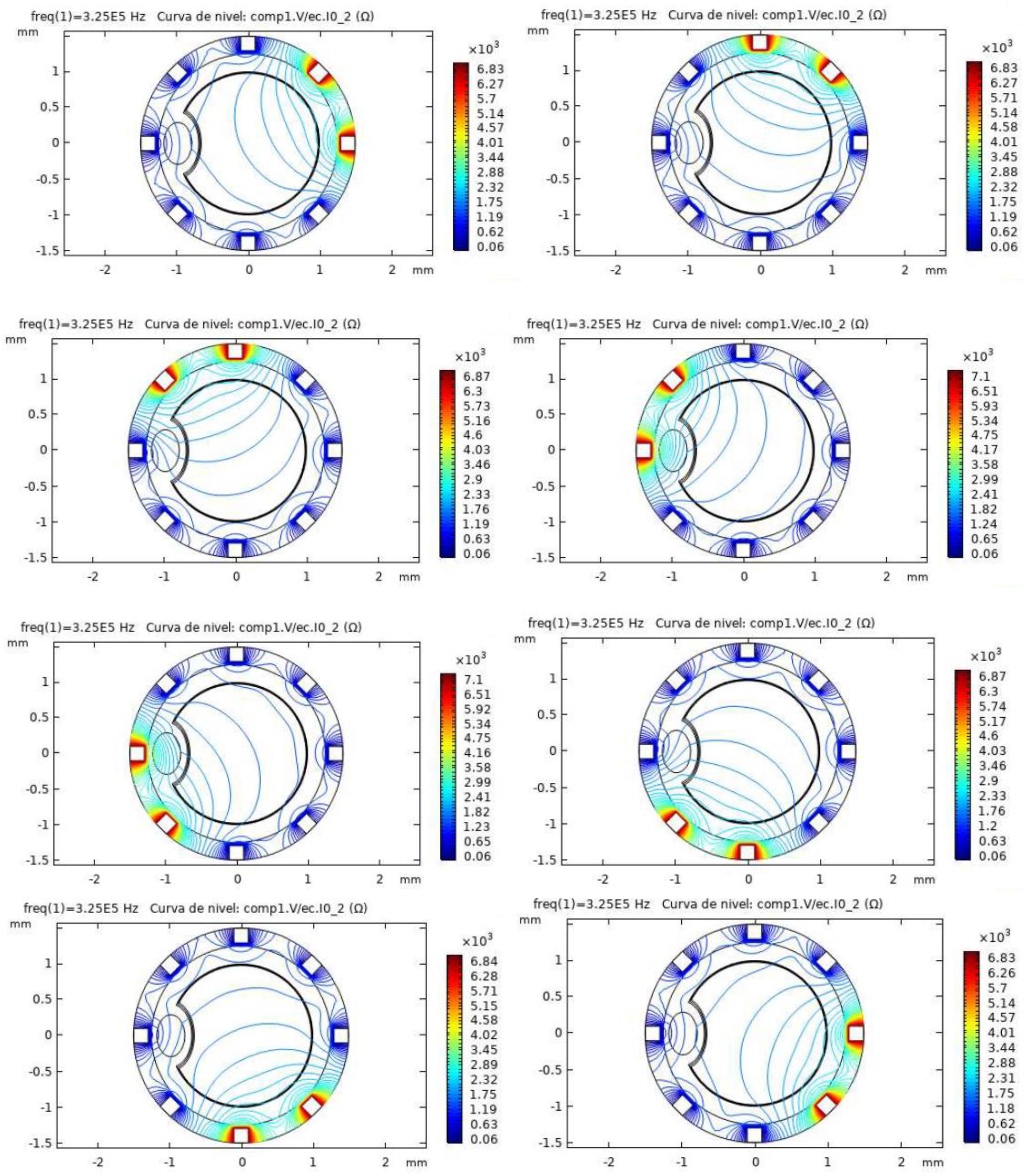
2.2. Electrical Impedance Tomography System Model and Simulations Performed
3. Results
3.1. Non-Pathological Model Simulations
3.2. Pathological Model Simulations
4. Discussion
4.1. Use of Bioimpedance for the Monitoring of Stent Occlusions
4.2. Practical Considerations and Future Work for the Implementation of the Smart Stent
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| CAD | Coronary Artery Disease |
| ISR | in-stent restenosis |
| IHD | Ischemic Heart Disease |
| MLD | Minimal Lumen Diameter |
| DES | Drug-Eluting Stent |
| EIT | Electrical Impedance Tomography |
References
- Liu, Y.-W.; Huang, M.-S.; Hsu, L.-W.; Chang, H.-Y.; Lee, C.-H.; Lee, C.-Y.; Chen, D.-P.; Li, Y.-H.; Chao, T.-H.; Su, P.-F.; et al. Genetic risk model for in-stent restenosis of second-and third-generation drug-eluting stents. iScience 2021, 24, 103082. [Google Scholar] [CrossRef] [PubMed]
- Hashemi, J.; Rai, S.; Ghafghazi, S.; Berson, R.E. Blood residence time to assess significance of coronary artery stenosis. Sci. Rep. 2020, 10, 11658. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.S.; Dean, L.S. In-Stent Restenosis. Cardiovasc. Ther. 2011, 29, 190–198. [Google Scholar] [CrossRef] [PubMed]
- Bonaa, K.H.; Mannsverk, J.; Wiseth, R.; Aaberge, L.; Myreng, Y.; Nygard, O.; Nilsen, D.W.; Klow, N.E.; Uchto, M.; Trovik, T.; et al. Drug-eluting or bare-metal stents for coronary artery disease. N. Engl. J. Med. 2016, 375, 1242–1252. [Google Scholar] [CrossRef]
- Holmes, D.R.; Firth, B.G.; Douglas, L. Wood Paradigm shifts in cardiovascular medicine. J. Am. Coll. Cardiol. 2004, 43, 507–512. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Zang, G.; Yin, T.; Ma, X.; Zhou, L.; Wu, L.; Daniel, R.; Wang, Y.; Qiu, J.; Wang, G. A novel mechanism of inhibiting in-stent restenosis with arsenic trioxide drug-eluting stent: Enhancing contractile phenotype of vascular smooth muscle cells via YAP pathway. Bioact. Mater. 2021, 6, 375–385. [Google Scholar] [CrossRef]
- Kastrati, A.; Schomig, A.; Elezi, S.; Schühlen, H.; Dirschinger, J.; Hadamitzky, M.; Wehinger, A.; Hausleiter, J.; Walter, H.; Neumann, F.J. Predictive factors of restenosis after coronary stent placement. J. Am. Coll. Cardiol. 1997, 30, 1428–1436. [Google Scholar] [CrossRef] [Green Version]
- Gaspar, T.; Halon, D.A.; Lewis, B.S.; Adawi, S.; Schliamser, J.E.; Rubinshtein, R.; Flugelman, M.Y.; Peled, N. Diagnosis of coronary in-stent restenosis with multidetector row spiral computed tomography. J. Am. Coll. Cardiol. 2005, 46, 1573–1579. [Google Scholar] [CrossRef] [Green Version]
- Ehara, M.; Kawai, M.; Surmely, J.F.; Matsubara, T.; Terashima, M.; Tsuchikane, E.; Kinoshita, Y.; Ito, T.; Takeda, Y.; Nasu, K.; et al. Diagnostic accuracy of coronary in-stent restenosis using 64-slice computed tomography: Comparison with invasive coronary angiography. J. Am. Coll. Cardiol. 2007, 49, 951–959. [Google Scholar] [CrossRef] [Green Version]
- Rivas Marchena, D.; Olmo Fernández, A.; Miguel, J.A.; Martinez, M.; Huertas Sánchez, G.; Yúfera García, A. Real-Time Electrical Bioimpedance Characterization of Neointimal Tissue for Stent Applications. Sensors 2017, 17, 1737. [Google Scholar] [CrossRef] [Green Version]
- Portillo Anaya, J.M.; Pérez, P.; Olmo Fernández, A.; Huertas Sánchez, G.; Yufera Garcia, A. Evaluation of Implanted Stent Occlusion Status based on Neointimal Tissue Bioimpedance Simulations. J. Sens. 2019, 2019, 7167186. [Google Scholar] [CrossRef]
- Ham, A.W.; Cormack, D.H. Tratado de Histología, 8th ed.; Edición Interamericana: Mexico D.F., Mexico, 1984. [Google Scholar]
- Mori, H.; Finn, A.V.; Atkinson, J.B.; Lutter, C.; Narula, J.; Virmani, R. Calcified Nodule: An Early and Late Cause of In-Stent Failure. JACC Cardiovasc. Interv. 2016, 9, 125–126. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Zhang, Y.T.; Mezic, I.; Meinhart, C.D.; Petzold, L. Numerical Simulation of an Electroosmotic Micromixer. In Proceedings of the ASME 2003 International Mechanical Engineering Congress and Exposition, Washington, DC, USA, 15–21 November 2003; Available online: https://www.academia.edu/13966744/NUMERICAL_SIMULATION_OF_AN_ELECTROOSMOTIC_MICROMIXER (accessed on 31 July 2021).
- Cummings, E.; Griffiths, S.; Nilson, R.; Paul, P. Conditions for Similitude between the Fluid Velocity and the Electric Field in Electroosmotic Flow. Anal. Chem. 2000, 72, 2526–2532. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cherepenin, V.; Karpov, A.; Korjenevsky, A.; Kornienko, V.; Mazaletskaya, A.; Mazourov, D.; Meister, D. A 3D electrical impedance tomography (EIT) system for breast cancer detection. Physiol. Meas. 2001, 22, 9–18. [Google Scholar] [CrossRef]
- Bachmann, M.C.; Morais, C.; Bugedo, G.; Bruhn, A.; Morales, A.; Borges, J.B.; Costa, E.; Retamal, J. Electrical impedance tomography in acute respiratory distress syndrome. Crit. Care 2018, 22, 263. [Google Scholar] [CrossRef] [Green Version]
- Isaacson, D.; Mueller, J.L.; Newell, J.C.; Siltanen, S. Imaging cardiac activity by the D-bar method for electrical impedance tomography. Physiol. Meas. 2006, 27, S43–S50. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Hanzaee, F.F.; Jiang, D.; Bayford, R.H.; Demosthenous, A. Electrical Impedance Tomography for Biomedical Applications: Circuits and Systems Review. IEEE Open J. Circuits Syst. 2021, 2, 380–397. [Google Scholar] [CrossRef]
- Khan, T.A.; Ling, S.H. Review on Electrical Impedance Tomography: Artificial Intelligence Methods and its Applications. Algorithms 2019, 12, 88. [Google Scholar] [CrossRef] [Green Version]
- Canales, D. Electrical Impedance Tomography (EIT) Image Reconstruction for the Human Forearm. Master’s Thesis, Instituto Tecnológico de Costa Rica, Escuela de Electrónica, Cartago, Costa Rica, 2016. [Google Scholar]
- Harikumar, R.; Prabu, R.; Raghavan, S. Electrical Impedance Tomography (EIT) and Its Medical Applications: A Review. Int. J. Soft Comput. Eng. 2013, 3, 193. [Google Scholar]
- Hua, P.; Webster, J.G.; Tompkins, W.J. Effect of the measurement method on noise handling and image quality of EIT imaging. In Proceedings of the 9th International IEEE EMBS Conference on Neural Engineering, San Francisco, CA, USA, 20–23 March 2019; pp. 1429–1430. [Google Scholar]
- Gisser, G.; Isaacson, D.; Newell, J.C. Current topics in impedance imaging. Clin. Physiol. Meas. 1987, 8, 38–46. [Google Scholar] [CrossRef]
- Fernández-Fuentes, X.; Mera, D.; Gómez, A.; Vidal-Franco, I. Towards a Fast and Accurate EIT Inverse Problem Solver: A Machine Learning Approach. Electronics 2018, 7, 422. [Google Scholar] [CrossRef] [Green Version]
- Pérez, A. Estudio de Diferentes Tecnologías de Fabricación de Electrodos Para Stents Inteligentes; Departamento de Tecnología Electrónica, Universidad de Sevilla: Sevilla, Spain, 2020. [Google Scholar]
- Kaikai, X. Silicon electro-optic micro-modulator fabricated in standard CMOS technology as components for all silicon monolithic integrated optoelectronic systems. J. Micromech. Microeng. 2021, 31, 054001. [Google Scholar]
- Juan-Colás, J.; Parkin, A.; Dunn, K.; Scullion, M.; Krauss, T.; Johnson, S. The electrophotonic silicon biosensor. Nat. Commun. 2016, 7, 12769. [Google Scholar] [CrossRef] [PubMed] [Green Version]
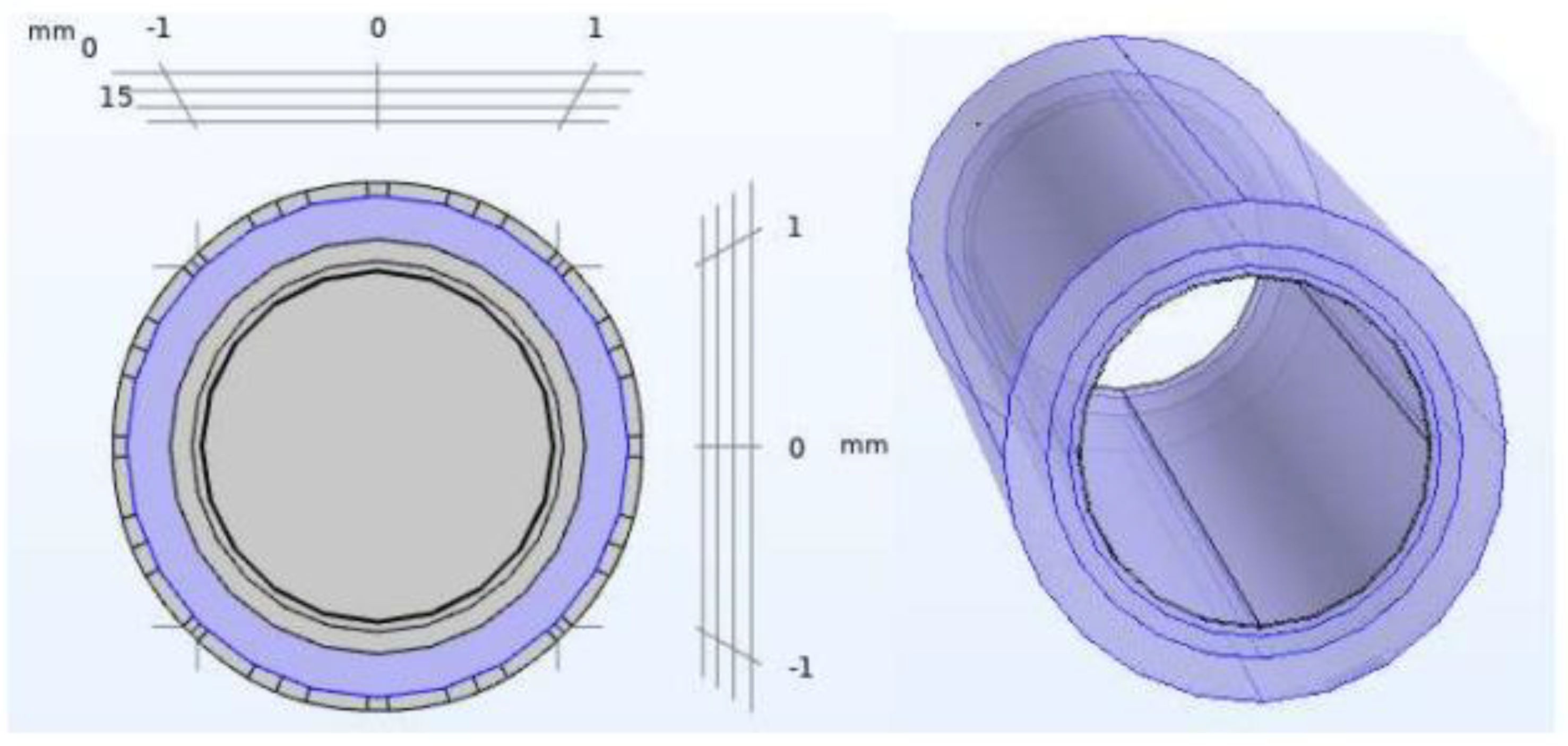

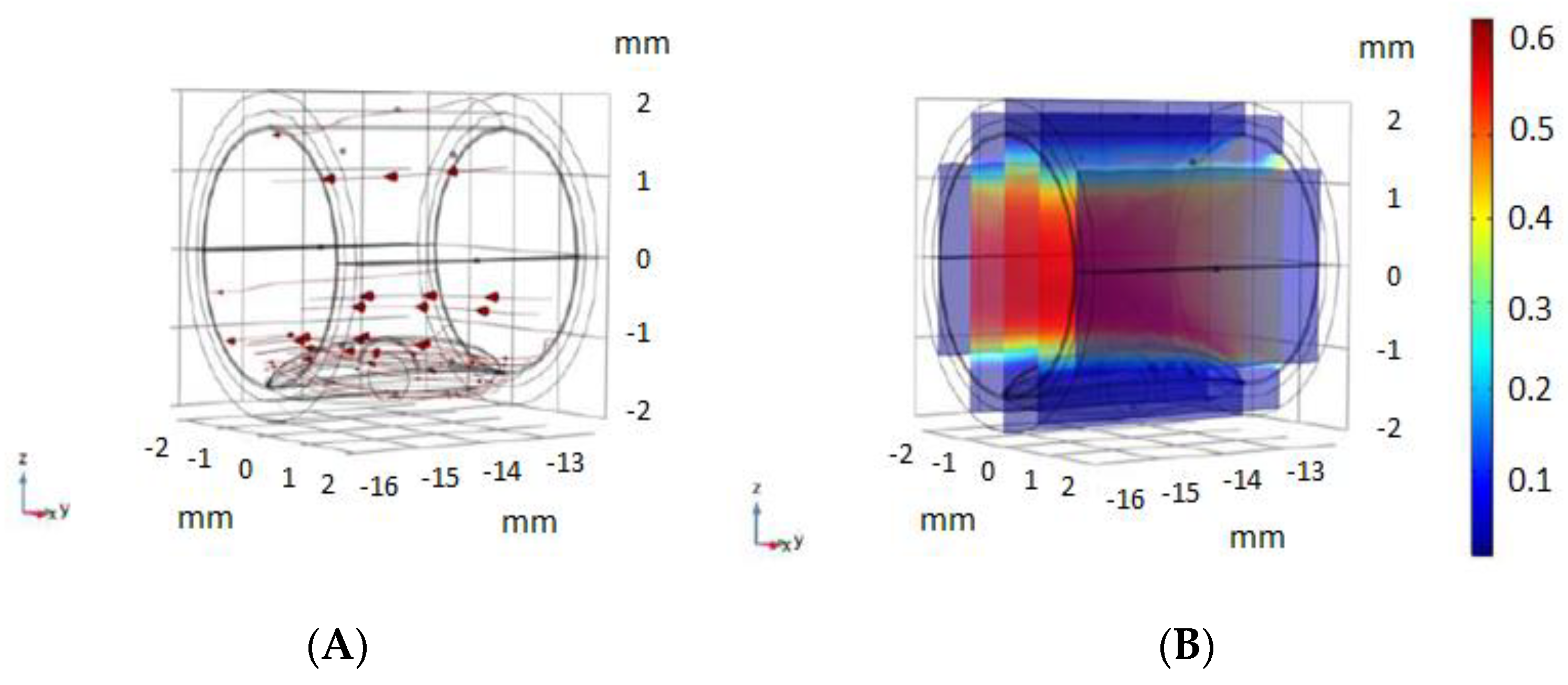
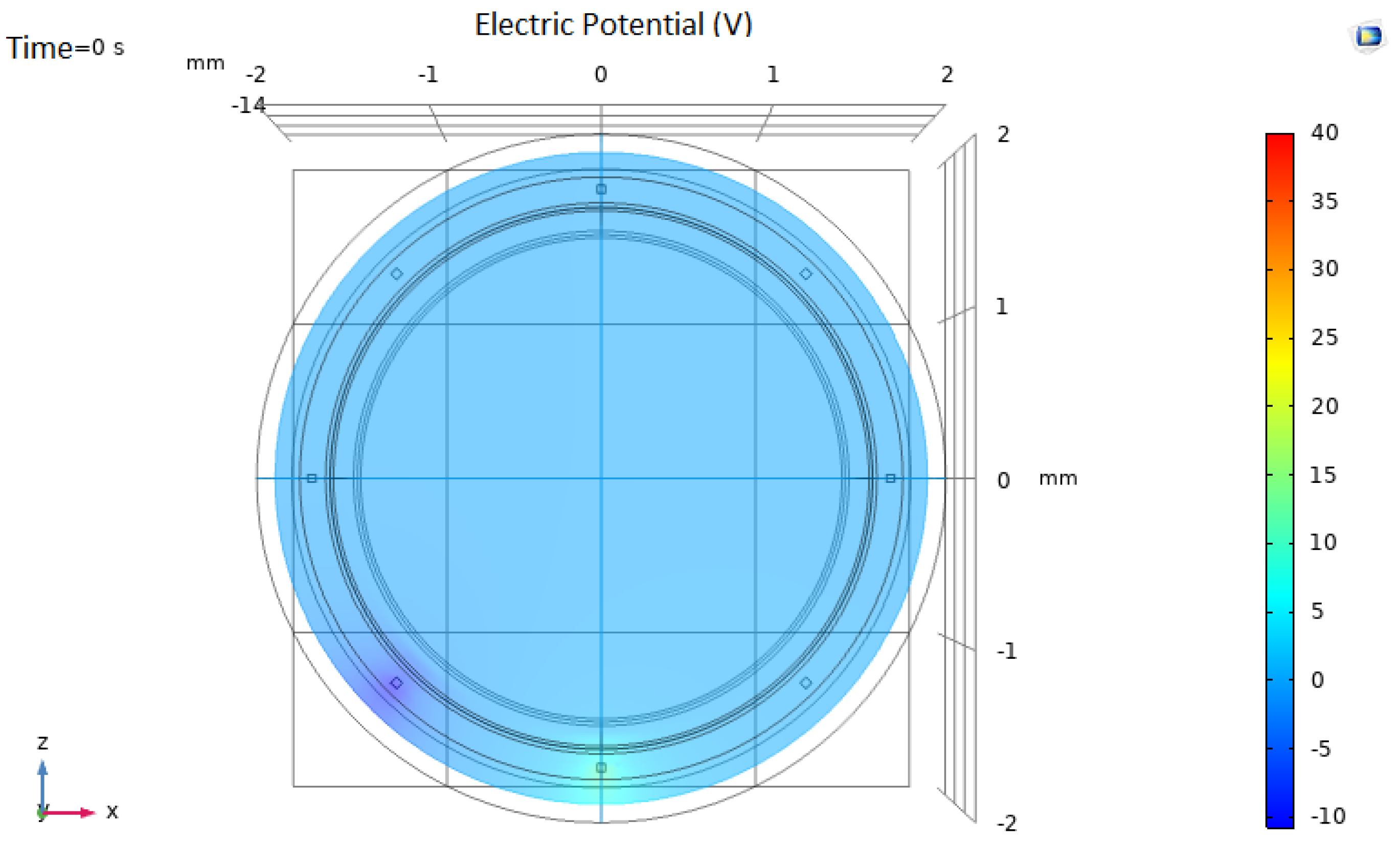


| Geometrical Properties | |
|---|---|
| Blood Cylinder, Inner Radius | 155.4 × 10−5 m |
| Endothelium layer, outer radius | 157.5 × 10−5 m |
| Fibre layer, outer radius | 160 × 10−5 m |
| Fat Layer, outer radius | 200 × 10−5 m |
| Cylinder length | 1600 × 10−5 m |
| Electrode side | 5 × 10−5 m |
| Electrical Conductivity (S/m) | ||||
|---|---|---|---|---|
| Frequency (Hz) | Fat | Muscle | Fibre | Endothelium |
| 100 | 0.035 | 0.2 | 0.25 | 0.05 |
| 101 | 0.038 | 0.202 | 0.25 | 0.05 |
| 102 | 0.041 | 0.267 | 0.25 | 0.05 |
| 103 | 0.042 | 0.321 | 0.25 | 0.05 |
| 104 | 0.043 | 0.341 | 0.25 | 0.05 |
| 105 | 0.043 | 0.362 | 0.3 | 0.050 |
| 106 | 0.044 | 0.503 | 0.350 | 0.146 |
| Electric relative Permittivity (εr) | ||||
|---|---|---|---|---|
| Frequency (Hz) | Fat | Muscle | Fibre | Endothelium |
| 100 | 9.91 × 106 | 2.62 × 107 | 2.0 × 104 | 3.61 × 103 |
| 101 | 5.03 × 106 | 2.57 × 107 | 2.0 × 104 | 3.61 × 103 |
| 102 | 1.52 × 105 | 9.33 × 106 | 1.0 × 104 | 3.61 × 103 |
| 103 | 1.93 × 104 | 4.35 × 105 | 1.0 × 103 | 3.61 × 103 |
| 104 | 9.12 × 102 | 2.59 × 104 | 1.0 × 102 | 3.61 × 103 |
| 105 | 1.01 × 102 | 8.09 × 103 | 2.0 × 101 | 3.58 × 103 |
| 106 | 50.8 | 1.84 × 103 | 10.0 | 1.85 × 103 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rodríguez, A.; Barroso, P.; Olmo, A.; Yúfera, A. Bioimpedance Sensing of Implanted Stent Occlusions: Smart Stent. Biosensors 2022, 12, 416. https://doi.org/10.3390/bios12060416
Rodríguez A, Barroso P, Olmo A, Yúfera A. Bioimpedance Sensing of Implanted Stent Occlusions: Smart Stent. Biosensors. 2022; 12(6):416. https://doi.org/10.3390/bios12060416
Chicago/Turabian StyleRodríguez, Antonio, Pablo Barroso, Alberto Olmo, and Alberto Yúfera. 2022. "Bioimpedance Sensing of Implanted Stent Occlusions: Smart Stent" Biosensors 12, no. 6: 416. https://doi.org/10.3390/bios12060416
APA StyleRodríguez, A., Barroso, P., Olmo, A., & Yúfera, A. (2022). Bioimpedance Sensing of Implanted Stent Occlusions: Smart Stent. Biosensors, 12(6), 416. https://doi.org/10.3390/bios12060416







