Kinetics of Isothermal Dumbbell Exponential Amplification: Effects of Mix Composition on LAMP and Its Derivatives
Abstract
:1. Introduction
2. Materials and Methods
2.1. Oligonucleotides: Dumbbells and Primers
2.2. Isothermal Dumbbell Exponential Amplification (IDEA)
3. Theoretical Model for IDEA Kinetics of LAMP Experiments
3.1. Evolution Rules for IDEA
3.2. Primer Limiting Kinetic Rates
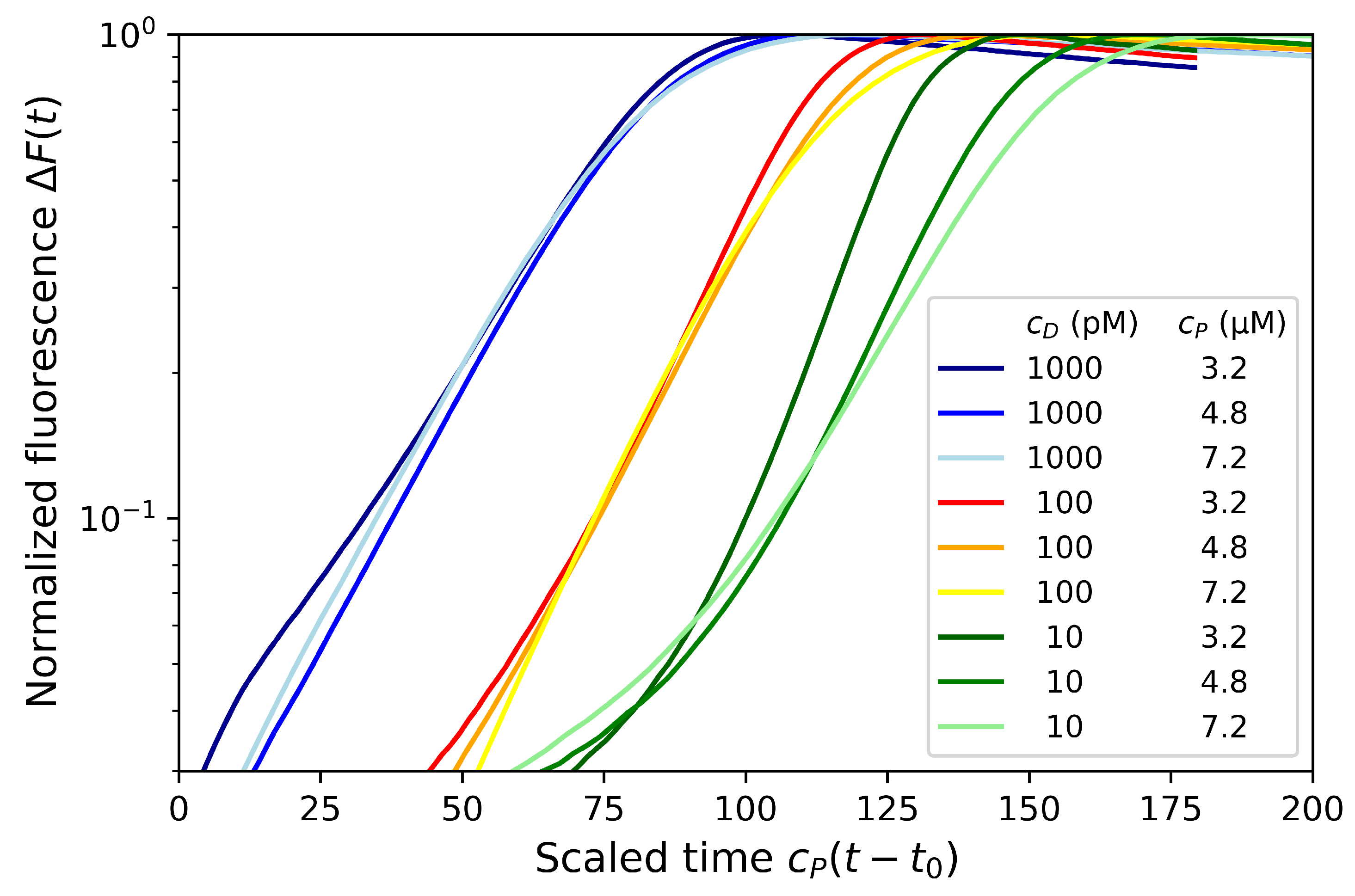
3.2.1. Constant Primer Concentration
3.2.2. Saturation Due to Finite Primer Concentration
3.3. Saturation Due to Finite dNTPs Concentration
3.4. Guidelines for Practitioners
- (i)
- Main assumptions and approximations of the model and their consequences:
- (ii)
- Key parameters for LAMP optimization:
- (iii)
- Experimental techniques to analyze LAMP:
- 1/
- The most standard technique is the use of intercalating dyes such as EvaGreen or SYBR Green. From the change of fluorescence due to the binding of the dye to the stem of hairpins, it is possible to measure the amount of stem length in the solution. By neglecting the loop parts (second term in the expression of in Equation (3)), we may consider that the measured fluorescence is proportional to the total length of hairpins . We have considered this technique to validate our model later on.
- 2/
- The detection of amplification by release of quenching (DARQ) is based on the use of a complementary strand to one of the primer and a couple of quencher-fluorophore attached on each strand [34,52]. Before the amplification, the fluorophore is quenched and no (or little) fluorescence is observed. During LAMP amplification, the complementary strand of the primer is released when the later is incorporated into a hairpin. Thus, in this case, the fluorescence is proportional to the concentration of hairpins formed during the amplification. Similar techniques have been developed [34].
- 3/
- Finally, by gel electrophoresis, the amount of hairpins of different stem lengths could be analyzed. This method is generally considered at the end of the amplification where different bands are observed corresponding to the different stem lengths present in the solution. In the dNTP-limited regime, the lack of dNTP could lead to a smearing of the band due to interrupted elongation of hairpin stems.
3.5. Fluorescence Detection with Intercalating Dyes
4. Experimental Results and Discussion
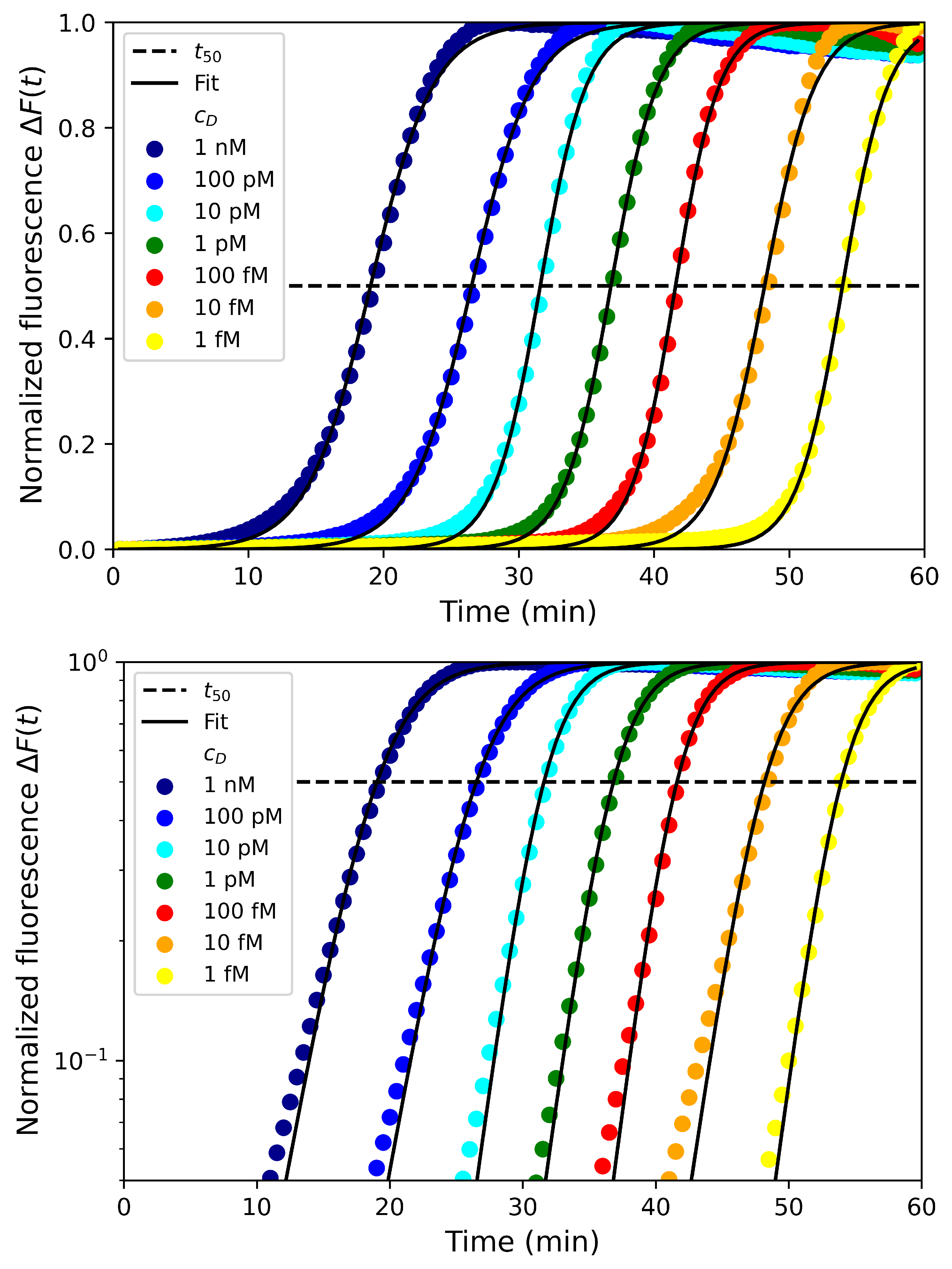
4.1. Effect of Fluorescent Dye Concentrations
4.2. Effect of Primer and dNTP Concentrations
4.3. Effect of Dumbbell Concentration and Length
5. Conclusions and Outlook
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mullis, K.; Faloona, F.; Scharf, S.; Saiki, R.; Horn, G.; Erlich, H. Specific Enzymatic Amplification of DNA In Vitro: The Polymerase Chain Reaction. Cold. Spring Harb. Symp. Quant. Biol. 1986, 51, 263–273. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Saiki, R.K.; Gelfand, D.H.; Stoffel, S.; Scharf, S.J.; Higuchi, R.; Horn, G.T.; Mullis, K.B.; Erlich, H.A. Primer-Directed Enzymatic Amplification of DNA with a Thermostable DNA Polymerase. Science 1988, 239, 487–491. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhao, Y.; Chen, F.; Li, Q.; Wang, L.; Fan, C. Isothermal Amplification of Nucleic Acids. Chem. Rev. 2015, 115, 12491–12545. [Google Scholar] [CrossRef] [PubMed]
- Notomi, T.; Okayama, H.; Masubuchi, H.; Yonekawa, T.; Watanabe, K.; Amino, N.; Hase, T. Loop-mediated isothermal amplification of DNA. Nucl. Acid. Res. 2000, 28, e63. [Google Scholar] [CrossRef] [Green Version]
- Augustine, R.; Hasan, A.; Das, S.; Ahmed, R.; Mori, Y.; Notomi, T.; Kevadiya, B.D.; Thakor, A.S. Loop-Mediated Isothermal Amplification (LAMP): A Rapid, Sensitive, Specific, and Cost-Effective Point-of-Care Test for Coronaviruses in the Context of COVID-19 Pandemic. Biology 2020, 9, 182. [Google Scholar] [CrossRef]
- Thompson, D.; Lei, Y. Mini review: Recent progress in RT-LAMP enabled COVID-19 detection. Sens. Actuators Rep. 2020, 2, 100017. [Google Scholar] [CrossRef]
- Rabe, B.A.; Cepko, C. SARS-CoV-2 detection using isothermal amplification and a rapid, inexpensive protocol for sample inactivation and purification. Proc. Natl. Acad. Sci. USA 2020, 117, 24450–24458. [Google Scholar] [CrossRef]
- Rodriguez-Manzano, J.; Malpartida-Cardenas, K.; Moser, N.; Pennisi, I.; Cavuto, M.; Miglietta, L.; Moniri, A.; Penn, R.; Satta, G.; Randell, P.; et al. Handheld Point-of-Care System for Rapid Detection of SARS-CoV-2 Extracted RNA in under 20 min. ACS Cent. Sci. 2021, 7, 307–317. [Google Scholar] [CrossRef]
- Tang, Z.; Nouri, R.; Dong, M.; Yang, J.; Greene, W.; Zhu, Y.; Yon, M.; Nair, M.S.; Kuchipudi, S.V.; Guan, W. Rapid detection of novel coronavirus SARS-CoV-2 by RT-LAMP coupled solid-state nanopores. Biosens. Bioelecton. 2022, 197, 113759. [Google Scholar] [CrossRef]
- Notomi, T.; Mori, Y.; Tomita, N.; Kanda, H. Loop-mediated isothermal amplification (LAMP): Principle, features, and future prospects. J. Microbiol. 2015, 53, 1–5. [Google Scholar] [CrossRef]
- Mori, Y.; Notomi, T. Loop-mediated isothermal amplifi cation (LAMP): A rapid, accurate, and cost-effective diagnostic method for infectious diseases. J. Infect. Chemother. 2009, 15, 62–69. [Google Scholar] [CrossRef] [PubMed]
- Francois, P.; Tangomo, M.; Hibbs, J.; Bonetti, E.-J.; Boehme, C.C.; Notomi, T.; Perkins, M.D.; Schrenzel, J. Robustness of a loop-mediated isothermal amplification reaction for diagnostic applications. FEMS Immunol. Med. Microbiol. 2011, 62, 41–48. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, H.; Xu, Y.; Fohlerova, Z.; Chang, H.; Iliescu, C.; Neuzil, P. LAMP-on-a-chip: Revising microfluidic platforms for loop-mediated DNA amplification. TrAC 2019, 113, 44–53. [Google Scholar] [CrossRef] [PubMed]
- Ma, Y.-D.; Li, K.-H.; Chen, Y.-H.; Lee, Y.-M.; Chou, S.-T.; Lai, Y.-Y.; Huang, P.-C.; Ma, H.-P.; Lee, G.-B. A sample-to-answer, portable platform for rapid detection of pathogens with a smartphone interface. Lab Chip 2019, 19, 3804–3814. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, H.Q.; Nguyen, V.D.; Nguyen, H.V.; Seo, T.S. Quantification of colorimetric isothermal amplification on the smartphone and its open-source app for point-of-care pathogen detection. Sci. Rep. 2020, 10, 15123. [Google Scholar] [CrossRef]
- Foudeh, A.M.; Didar, T.F.; Veres, T.; Tabrizian, M. Microfluidic designs and techniques using lab-on-a-chip devices for pathogen detection for point-of-care diagnostics. Lab Chip 2012, 12, 3249–3266. [Google Scholar] [CrossRef]
- Tomita, N.; Mori, Y.; Kanda, H.; Notomi, T. Loop-mediated isothermal amplification (LAMP) of gene sequences and simple visual detection of products. Nat. Protoc. 2008, 3, 877. [Google Scholar] [CrossRef]
- Li, Y.; Fan, P.; Zhou, S.; Zhang, L. Loop-mediated isothermal amplification (LAMP): A novel rapid detection platform for pathogens. Microb. Pathog. 2017, 107, 54–61. [Google Scholar] [CrossRef]
- Wong, Y.P.; Othman, S.; Lau, Y.L.; Radu, S.; Chee, H.Y. Loop-mediated isothermal amplification (LAMP): A versatile technique for detection of micro-organisms. J. Appl. Microbiol. 2017, 124, 626–643. [Google Scholar] [CrossRef] [Green Version]
- Leonardo, S.; Toldrà, A.; Campàs, M. Biosensors Based on Isothermal DNA Amplification for Bacterial Detection in Food Safety and Environmental Monitoring. Sensors 2021, 21, 602. [Google Scholar] [CrossRef]
- Cao, H.; Fang, X.; Li, H.; Li, H.; Kong, J. Ultrasensitive detection of mucin 1 biomarker by immuno-loop-mediated isothermal amplification. Talanta 2017, 164, 588–592. [Google Scholar] [CrossRef] [PubMed]
- Zhang, T.; Lin Xu, L.; Jiang, B.; Yuan, R.; Xiang, Y. Polymerization nicking-triggered LAMP cascades enable exceptional signal amplification for aptamer-based label-free detection of trace proteins in human serum. Anal. Chim. Acta 2020, 1098, 164–169. [Google Scholar] [CrossRef]
- Li, C.; Li, Z.; Jia, H.; Yan, J. One-step ultrasensitive detection of microRNAs with loop-mediated isothermal amplification (LAMP). Chem. Commun. 2011, 47, 2595–2597. [Google Scholar] [CrossRef] [PubMed]
- Du, W.; Lv, M.; Li, J.; Yu, R.; Jiang, J. A ligation-based loop-mediated isothermal amplification (ligation-LAMP) strategy for highly selective microRNA detection. Chem. Commun. 2016, 52, 12721. [Google Scholar] [CrossRef]
- Al-Maskri, A.A.A.; Ye, J.; Talap, J.; Hu, H.; Sun, L.; Yu, L.; Cai, S.; Zeng, S. Reverse transcription-based loop-mediated isothermal amplification strategy for real-time miRNA detection with phosphorothioated probes. Anal. Chim. Acta 2020, 1126, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Gao, K.; Zhang, P.; Wang, H.; Wang, H.; Su, F.; Li, Z. Ultrasensitive homogeneous detection of microRNAs in a single cell with specifically designed exponential amplification. Chem. Commun. 2021, 57, 5570. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, H.; Wang, H.; Wang, F.; Li, Z. CRISPR/Cas12a-Assisted Ligation-Initiated Loop-Mediated Isothermal Amplification (CAL-LAMP) for Highly Specific Detection of microRNAs. Anal. Chem. 2021, 93, 7942–7948. [Google Scholar] [CrossRef]
- Hua, X.; Fan, J.-J.; Yang, L.; Wang, J.; Wen, Y.; Su, L.; Zhang, X. Rapid detection of miRNA via development of consecutive adenines (polyA)-based electrochemical biosensors. Biosens. Bioelectron. 2022, 198, 113830. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, D.; Tian, W.; Qi, Y.; Ren, W.; Li, Z.; Liu, C. Facile Clamp-Assisted Ligation Strategy for Direct Discrimination and Background-Free Quantification of Site-Specific 5-Formylcytosine. Anal. Chem. 2020, 92, 3477–3482. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, C.; Zhang, S.; Cai, Y.; Hua, K.; Cui, Y. One-step determination of deletion mutation based on loop-mediated isothermal amplification. Anal. Biochem. 2021, 616, 114087. [Google Scholar] [CrossRef]
- Varona, M.; Anderson, J.L. Advances in Mutation Detection Using Loop-Mediated Isothermal Amplification. ACS Omega 2021, 6, 3463–3469. [Google Scholar] [CrossRef] [PubMed]
- Hambalek, J.A.; Kong, J.E.; Brown, C.; Munoz, H.E.; Horn, T.; Bogumil, M.; Quick, E.; Ozcan, A.; Di Carlo, D. Methylation-Sensitive Loop-Mediated Isothermal Amplification (LAMP): Nucleic Acid Methylation Detection through LAMP with Mobile Fluorescence Readout. ACS Sens. 2021, 6, 3242–3252. [Google Scholar] [CrossRef] [PubMed]
- Yin, Y.; Wu, Z.; Li, G.; Huang, J.; Guo, Q.; Meng, X. A DNA molecular diagnostic technology with LAMP-like sensitivity based on one pair of hairpin primers-mediated isothermal polymerization amplification. Anal. Chim. Acta 2020, 1134, 144–149. [Google Scholar] [CrossRef] [PubMed]
- Becherer, L.; Borst, N.; Bakheit, M.; Frischmann, S.; Zengerle, R.; von Stetten, F. Loop-mediated isothermal amplification (LAMP)–review and classification of methods for sequencespecific detection. Anal. Methods 2020, 12, 717. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Tang, Y.; Song, D.; Lu, B.; Guo, L.; Li, B. Establishment of Dual Hairpin Ligation-Induced Isothermal Amplification for Universal, Accurate, and Flexible Nucleic Acid Detection. Anal. Chem. 2021, 93, 3315–3323. [Google Scholar] [CrossRef]
- Luo, G.; Yi, T.; Wang, Q.; Guo, B.; Fang, L.; Zhang, G.; Guo, X. Stem-loop-primer assisted isothermal amplification enabling high-specific and ultrasensitive nucleic acid detection. Biosens. Bioelectron. 2021, 184, 113239. [Google Scholar] [CrossRef]
- Savonnet, M.; Buhot, A.; Cubizolles, M.; Roupioz, Y. Method for Detecting and Possibly Quantifying an Analyte with an Oligonucleotide with Double Stem-Loop Structure and Said. Oligonucleotide. Patent EP3878971A1, 15 September 2021. [Google Scholar]
- Aubret, M.; Savonnet, M.; Laurent, P.; Roupioz, Y.; Cubizolles, M.; Buhot, A. Development of an Innovative Quantification Assay Based on Aptamer Sandwich and Isothermal Dumbbell Exponential Amplification. Anal. Chem. 2022, 94, 3376–3385. [Google Scholar] [CrossRef]
- Martin, A.; Grant, K.B.; Stressmann, F.; Ghigo, J.-M.; Marchal, D.; Limoges, B. Ultimate Single-Copy DNA Detection Using Real-Time Electrochemical LAMP. ACS Sens. 2016, 1, 904–912. [Google Scholar] [CrossRef]
- Hardinge, P.; Kiddle, G.; Tisi, L.; Murray, J.A. Optimised LAMP allows single copy detection of 35Sp and NOSt in transgenic maize using Bioluminescent Assay in Real Time (BART). Sci. Rep. 2018, 8, 17590. [Google Scholar] [CrossRef]
- Tanner, N.A.; Evans, T.C., Jr. Loop-Mediated Isothermal Amplification for Detection of Nucleic Acids. Curr. Protoc. Mol. Biol. 2014, 105, 15.14.1–15.14.14. [Google Scholar] [CrossRef]
- Stolovitzky, G.; Cecchi, G. Efficiency of DNA replication in the polymerase chain reaction. Proc. Natl. Acad. Sci. USA 1996, 93, 12947–12952. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gevertz, J.L.; Dunn, S.M.; Roth, C.M. Mathematical Model of Real-Time PCR Kinetics. Biotechnol. Bioeng. 2005, 92, 346. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cobbs, G. Stepwise kinetic equilibrium models of quantitative polymerase chain reaction. BMC Bioinform. 2012, 13, 203. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Subramanian, S.; Gomez, R.D. An Empirical Approach for Quantifying Loop-Mediated Isothermal Amplification (LAMP) Using Escherichia coli as a Model System. PLoS ONE 2014, 9, e100596. [Google Scholar] [CrossRef] [Green Version]
- Gordon, M.I.; Klemer, D.P.; Fuller, S.L.; Chang, J.H.; Klemer, D.R.; Putnam, M.L. Mathematical modeling of a real-time isothermal amplification assay for Erwinia amylovora. Eng. Rep. 2019, 1, e12047. [Google Scholar] [CrossRef] [Green Version]
- Kaur, N.; Thota, N.; Toley, B.J. A stoichiometric and pseudo kinetic model of loop mediated isothermal amplification. Comput. Struct. Biotechnol. J. 2020, 18, 2336–2346. [Google Scholar] [CrossRef]
- Fiche, J.B.; Buhot, A.; Calemczuk, R.; Livache, T. Temperature Effects on DNA Chip Experiments from Surface Plasmon Resonance Imaging: Isotherms and Melting Curves. Biophys. J. 2006, 92, 935–946. [Google Scholar] [CrossRef] [Green Version]
- Patel, S.S.; Wong, I.; Johnson, K.A. Pre-Steady-State Kinetic Analysis of Processive DNA Replication Including Complete Characterization of an Exonuclease-Deficient Mutant. Biochemistry 1991, 30, 511–525. [Google Scholar] [CrossRef]
- Vashishtha, A.K.; Konigsberg, W.H. The effect of different divalent cations on the kinetics and fidelity of Bacillus stearothermophilus DNA polymerase. AIMS Biophys. 2018, 5, 125–143. [Google Scholar] [CrossRef]
- Wang, D.-G.; Brewster, J.D.; Paul, M.; Tomasula, P.M. Two Methods for Increased Specificity and Sensitivity in Loop-Mediated Isothermal Amplification. Molecules 2015, 20, 6048–6059. [Google Scholar] [CrossRef] [Green Version]
- Tanner, N.A.; Zhang, Y.; Evans, T.C., Jr. Simultaneous multiple target detection in real-time loop-mediated isothermal amplification. BioTechniques 2012, 53, 81–89. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Quyen, T.L.; Ngo, T.A.; Bang, D.D.; Madsen, M.; Wolff, A. Classification of Multiple DNA Dyes Based on Inhibition Effects on Real-Time Loop-Mediated Isothermal Amplification (LAMP): Prospect for Point of Care Setting. Front. Microbiol. 2019, 10, 2234. [Google Scholar] [CrossRef] [PubMed]
- Fedorov, A.A.; Murav’ev, A.V. A kinetic model for equilibrium binding of intercalation dye with DNA during its amplification. J. Phys. Conf. Ser. 2020, 1697, 012042. [Google Scholar] [CrossRef]
- Mao, F.; Leung, W.-Y.; Xin, X. Characterization of EvaGreen and the implication of its physicochemical properties for qPCR applications. BMC Biotechnol. 2007, 7, 76. [Google Scholar] [CrossRef] [Green Version]
- Shoute, L.C.T.; Loppnow, G.R. Characterization of the binding interactions between EvaGreen dye and dsDNA. Phys. Chem. Chem. Phys. 2018, 20, 4772–4780. [Google Scholar] [CrossRef]
- Nagamine, N.; Hase, T.; Notomi, T. Accelerated reaction by loop-mediated isothermal amplification using loop primers. Mol. Cell. Probes 2002, 16, 223–229. [Google Scholar] [CrossRef]
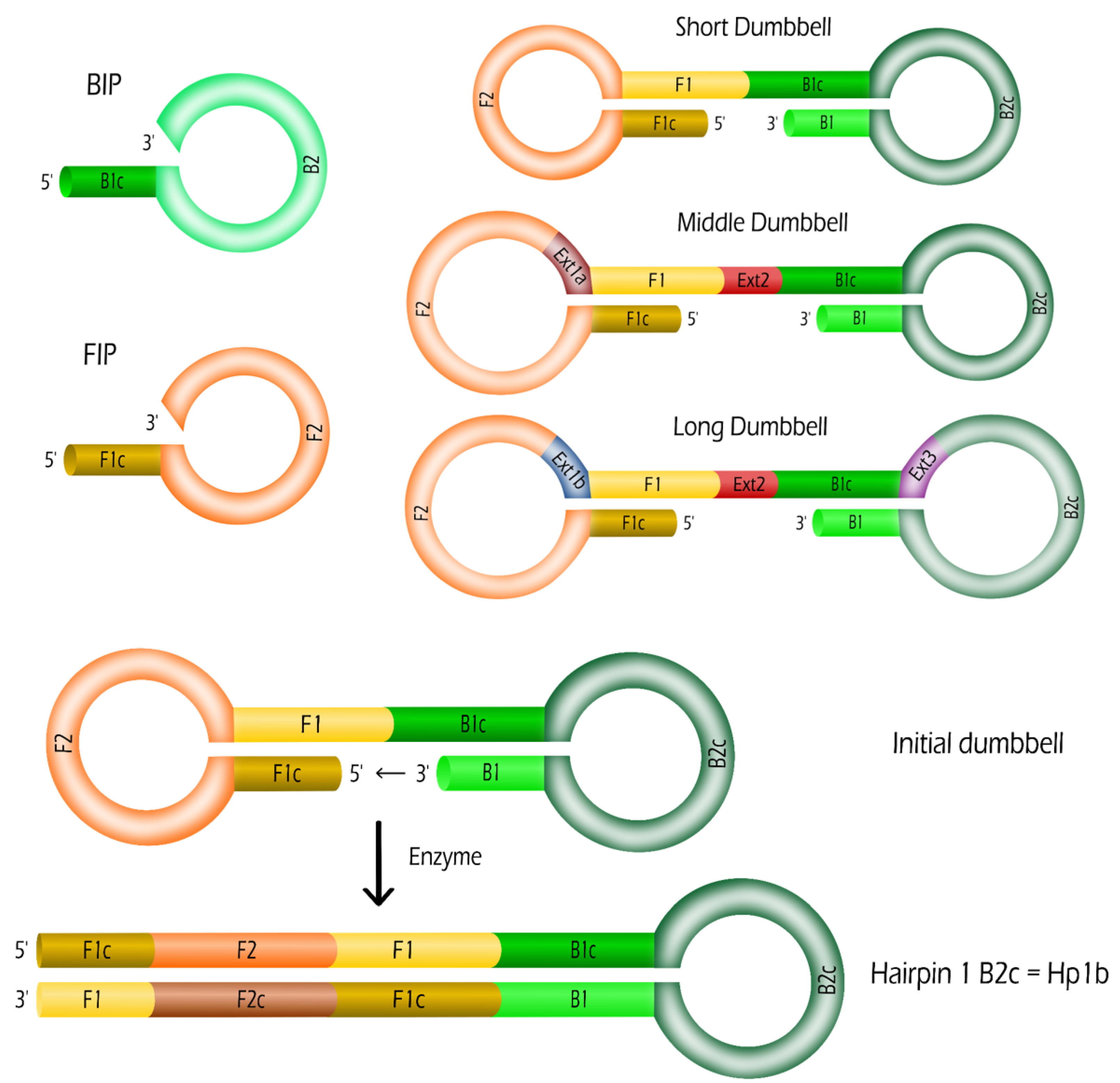


| Name | Length | Sequence (5-3) |
|---|---|---|
| B1 | 25 | GGG GGA AAG ATA TAA CTC AGA GAT G |
| B2 | 18 | GAA GGA GGG TCA GTG AGG |
| F1 | 21 | ATA AAC CGC GTC TTG GAT CCG |
| F2 | 24 | CGT GCA GTA CGC CAA CCT TTC TCA |
| FIP | 45 | F1c-F2 |
| BIP | 43 | B1c-B2 |
| Ext1a | 16 | TGC GCT GCC CCT CTT A |
| Ext1b | 23 | TGC GCT GCC CCT CTT ATA TCT TC |
| Ext2 | 3 | TGA |
| Ext3 | 30 | AGT TTA CAG CTC CTT AAG CCC CAT ATT GCC |
| SD | 134 | F1c-F2-F1-B1c-B2c-B1 |
| MD | 153 | F1c-F2-Ext1a-F1-Ext2-B1c-B2c-B1 |
| LD | 190 | F1c-F2-Ext1b-F1-Ext2-B1c-Ext3-B2c-B1 |
| Dumbbell | SD = 134 Bases | MD = 153 Bases | LD = 190 Bases | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Exp. | Theo. | Error | Exp. | Theo. | Error | Exp. | Theo. | Error | |
| 1 nM | |||||||||
| 100 pM | |||||||||
| 10 pM | |||||||||
| 1 pM | |||||||||
| 100 fM | |||||||||
| 10 fM | |||||||||
| 1 fM | |||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Savonnet, M.; Aubret, M.; Laurent, P.; Roupioz, Y.; Cubizolles, M.; Buhot, A. Kinetics of Isothermal Dumbbell Exponential Amplification: Effects of Mix Composition on LAMP and Its Derivatives. Biosensors 2022, 12, 346. https://doi.org/10.3390/bios12050346
Savonnet M, Aubret M, Laurent P, Roupioz Y, Cubizolles M, Buhot A. Kinetics of Isothermal Dumbbell Exponential Amplification: Effects of Mix Composition on LAMP and Its Derivatives. Biosensors. 2022; 12(5):346. https://doi.org/10.3390/bios12050346
Chicago/Turabian StyleSavonnet, Maud, Mathilde Aubret, Patricia Laurent, Yoann Roupioz, Myriam Cubizolles, and Arnaud Buhot. 2022. "Kinetics of Isothermal Dumbbell Exponential Amplification: Effects of Mix Composition on LAMP and Its Derivatives" Biosensors 12, no. 5: 346. https://doi.org/10.3390/bios12050346
APA StyleSavonnet, M., Aubret, M., Laurent, P., Roupioz, Y., Cubizolles, M., & Buhot, A. (2022). Kinetics of Isothermal Dumbbell Exponential Amplification: Effects of Mix Composition on LAMP and Its Derivatives. Biosensors, 12(5), 346. https://doi.org/10.3390/bios12050346







