Design Methodology of Passive In-Line Relays for Molecular Communication in Flow-Induced Microfluidic Channel
Abstract
1. Introduction
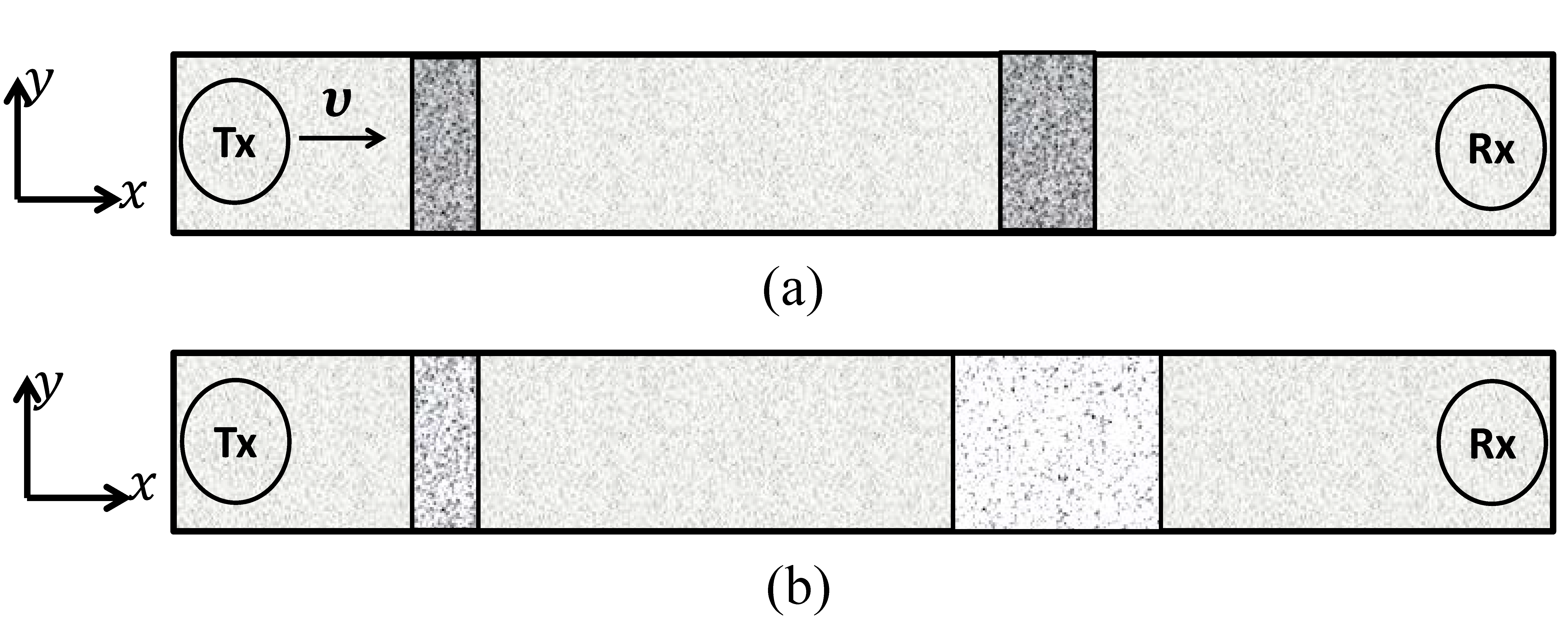
2. System Model
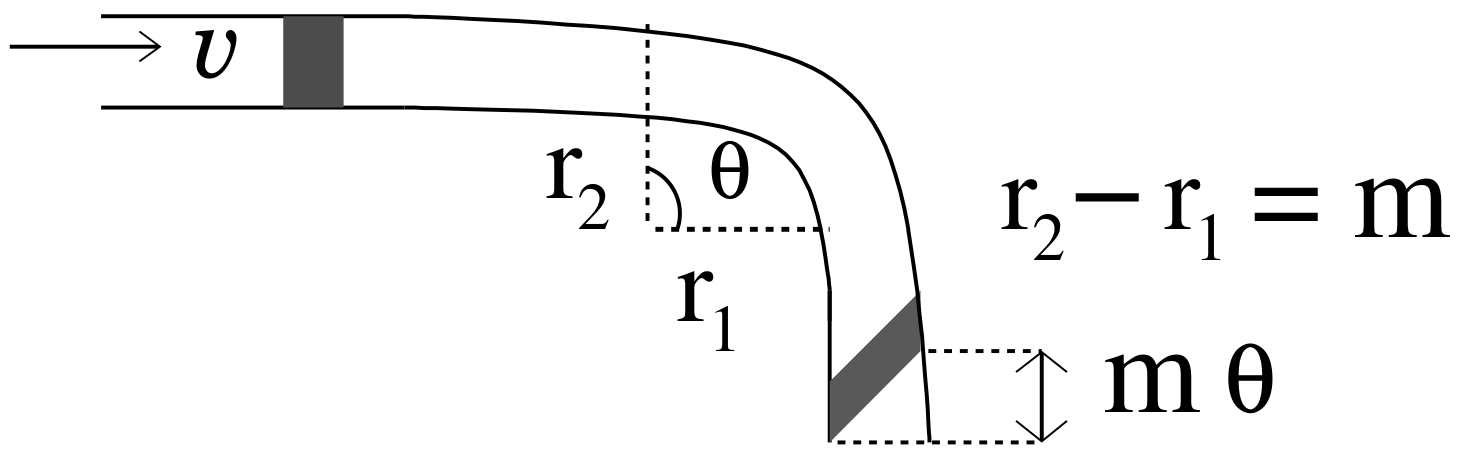
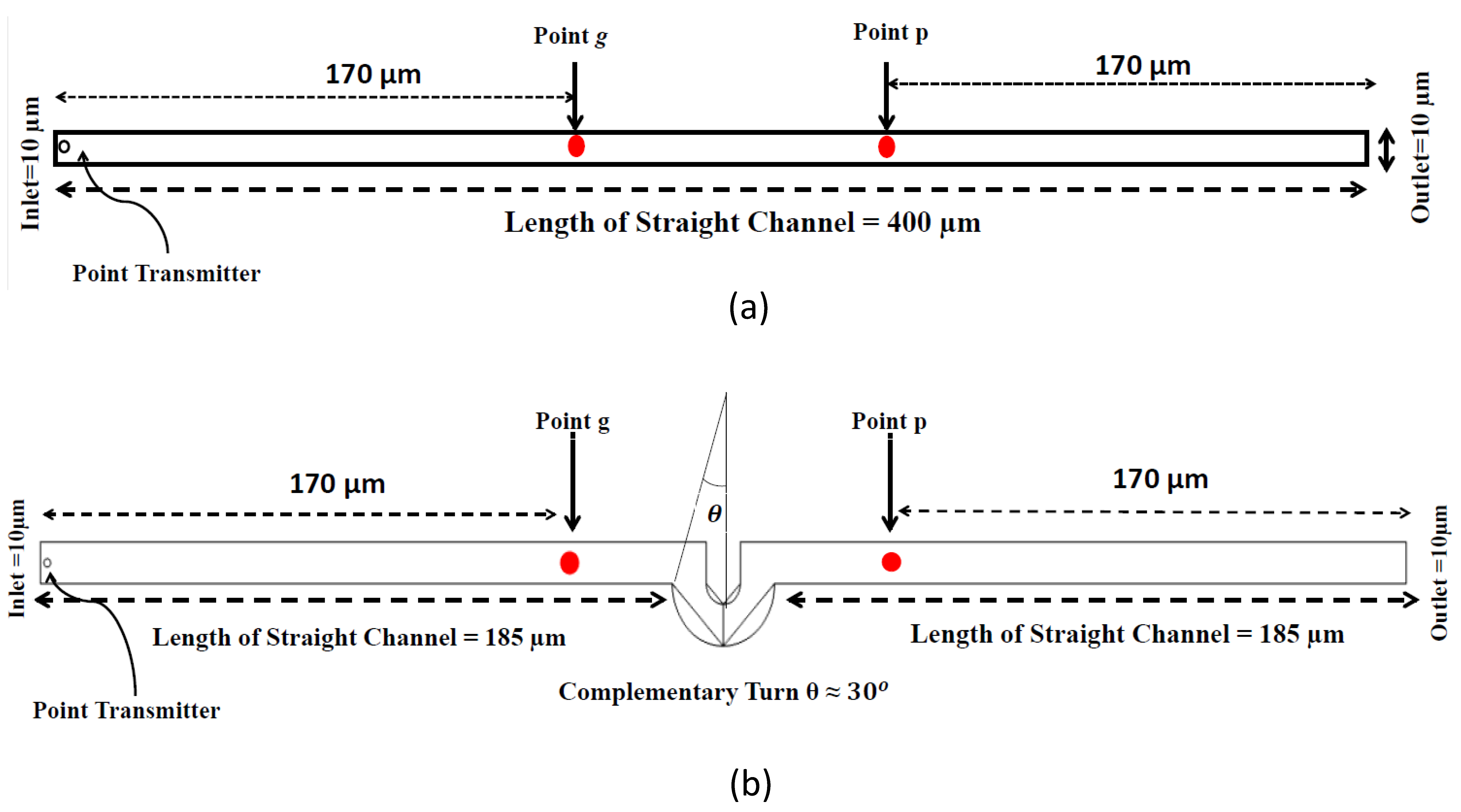
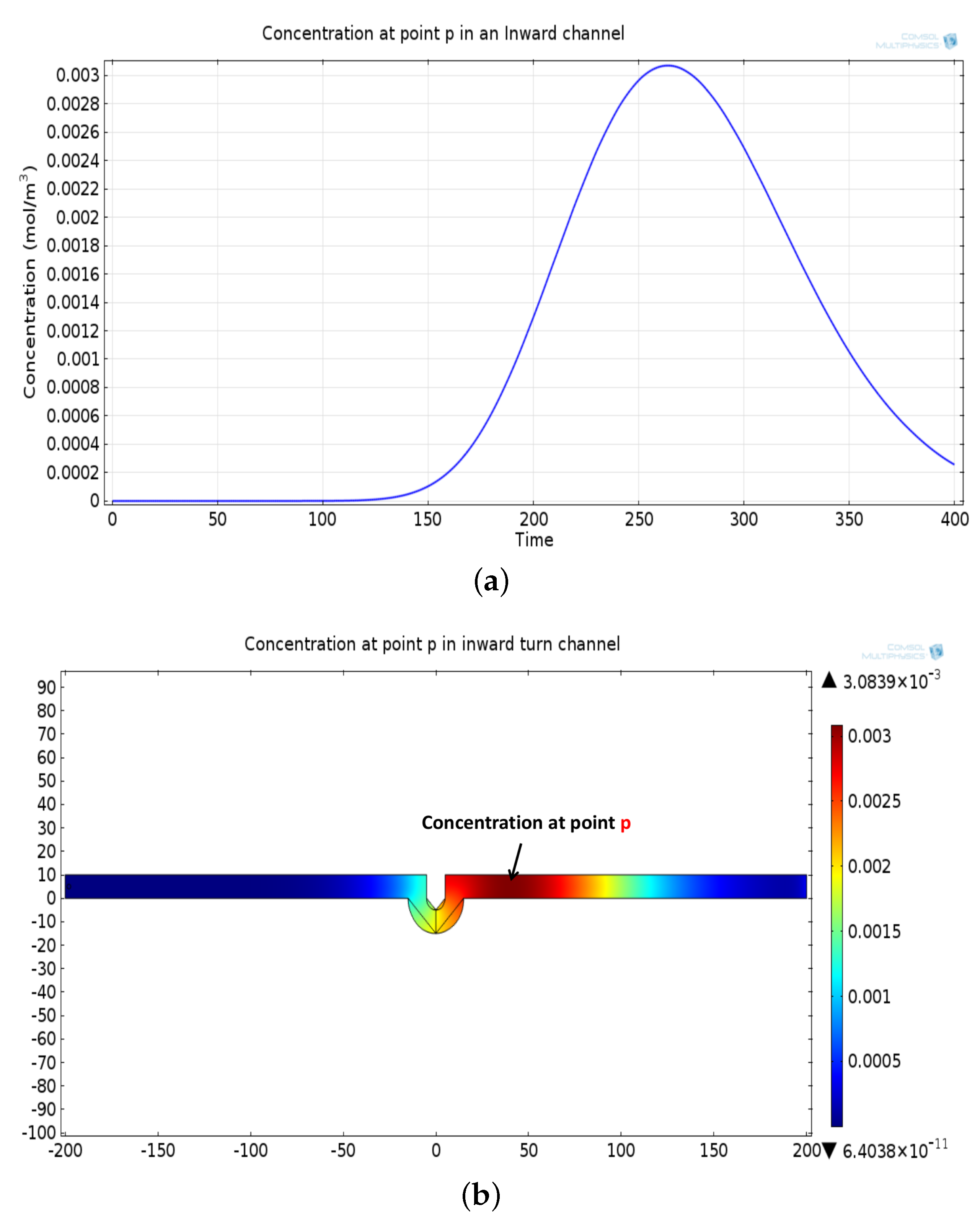
3. Design of Passive In-Line Relay
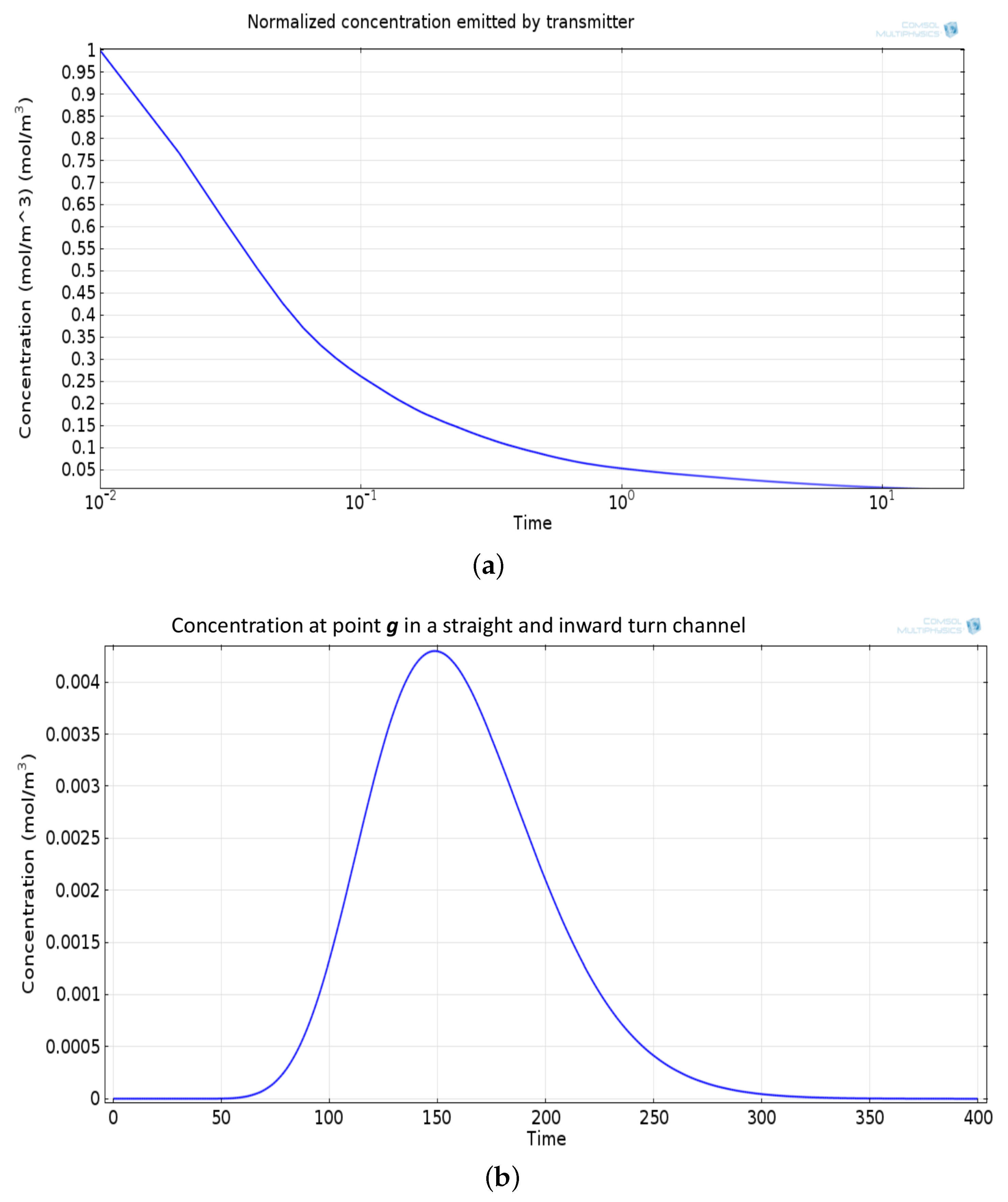
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Akyildiz, I.F.; Pierobon, M.; Balasubramaniam, S. Moving forward with molecular communication: From theory to human health applications. Proc. IEEE 2019, 107, 858–865. [Google Scholar] [CrossRef]
- Nakano, T.; Moore, M.J.; Wei, F.; Vasilakos, A.V.; Shuai, J. Molecular Communication and Networking: Opportunities and Challenges. IEEE Trans. NanoBiosci. 2012, 11, 135–148. [Google Scholar] [CrossRef] [PubMed]
- Nakano, T.; Okaie, Y.; Vasilakos, A.V. Transmission Rate Control for Molecular Communication among Biological Nanomachines. IEEE J. Sel. Areas Commun. 2013, 31, 835–846. [Google Scholar] [CrossRef]
- Nakano, T.; Suda, T.; Okaie, Y.; Moore, M.J.; Vasilakos, A.V. Molecular communication among biological nanomachines: A layered architecture and research issues. IEEE Trans. NanoBiosci. 2014, 13, 169–197. [Google Scholar] [CrossRef]
- Varshney, N.; Patel, A.; Haselmayr, W.; Jagannatham, A.K.; Varshney, P.K.; Nallanathan, A. Impact of Intermediate Nanomachines in Multiple Cooperative Nanomachine-Assisted Diffusion Advection Mobile Molecular Communication. IEEE Trans. Commun. 2019, 67, 4856–4871. [Google Scholar] [CrossRef]
- Alberts, B.; Johnson, A.; Lewis, J. Essential Cell Biology; Garland Science: New York, NY, USA, 2013. [Google Scholar]
- Einolghozati, A.; Sardari, M.; Beirami, A.; Fekri, F. Data gathering in networks of bacteria colonies: Collective sensing and relaying using molecular communication. In Proceedings of the IEEE INFOCOM Workshops, Orlando, FL, USA, 25–30 March 2012; pp. 256–261. [Google Scholar]
- Nakano, T.; Liu, J.-Q. Design and Analysis of Molecular Relay Channels: An Information Theoretic Approach. IEEE Trans. NanoBiosci. 2010, 9, 213–221. [Google Scholar] [CrossRef] [PubMed]
- Einolghozati, A.; Sardari, M.; Fekri, F. Relaying in diffusion-based molecular communication. In Proceedings of the IEEE International Symposium on Information Theory, Istanbul, Turkey, 7–12 July 2013; pp. 1844–1848. [Google Scholar]
- Wang, J.; Peng, M.; Liu, Y. Performance analysis of diffusion-based decode-and-forward relay with depleted molecule shift keying. Digit. Commun. Netw. 2020. [Google Scholar] [CrossRef]
- Wang, X.; Higgins, M.D.; Leeson, M.S. Relay analysis in molecular communications with time-dependent concentration. IEEE Commun. Lett. 2015, 19, 1977–1980. [Google Scholar] [CrossRef]
- Ahmadzadeh, A.; Noel, A.; Burkovski, A.; Schober, R. Amplify-and- forward relaying in two-hop diffusion-based molecular communication networks. In Proceedings of the IEEE GLOBECOM, San Diego, CA, USA, 6–10 December 2015; pp. 1–7. [Google Scholar]
- Tavakkoli, N.; Azmi, P.; Mokari, N. Optimal Positioning of Relay Node in Cooperative Molecular Communication Networks. IEEE Trans. Commun. 2017, 65, 5293–5304. [Google Scholar] [CrossRef]
- Tiwari, S.K.; Reddy, T.R.T.; Upadhyay, P.K.; Da Costa, D.B. Joint Optimization of Molecular Resource Allocation and Relay Positioning in Diffusive Nanonetworks. IEEE Access 2018, 6, 67681–67687. [Google Scholar] [CrossRef]
- Ahmadzadeh, A.; Noel, A.; Schober, R. Analysis and design of multi-hop diffusion-based molecular communication networks. IEEE Trans. Mol. Biol. Multi Scale Commun. 2015, 1, 144–157. [Google Scholar] [CrossRef]
- Manocha, P.; Chandwani, G.; Das, S. Dielectrophoretic Relay Assisted Molecular Communication for In-Sequence Molecule Delivery. IEEE Trans. NanoBiosci. 2016, 15, 1. [Google Scholar] [CrossRef] [PubMed]
- Manocha, P.; Chandwani, G.; Das, S. Characterization of Dielectrophoresis Based Relay-Assisted Molecular Communication Using Analogue Transmission Line. IEEE Access 2020, 8, 33352–33359. [Google Scholar] [CrossRef]
- Bukowska, D.M.; Derzsi, L.; Tamborski, S.; Szkulmowski, M.; Garstecki, P.; Wojtkowski, M. Assessment of the flow velocity of blood cells in a microfluidic device using joint spectral and time domain optical coherence tomography. Opt. Express 2013, 21, 24025–24038. [Google Scholar] [CrossRef] [PubMed]
- Yun, J.H.; Jung, H.W.; Chun, M.-S. The geometry effect on steady electrokinetic flows in curved rectangular microchannels. Phys. Fluids 2010, 22, 052004. [Google Scholar] [CrossRef]
- Bicen, A.O.; Akyildiz, I.F. System-theoretic analysis and least-squares design of microfluidic channels for flow-induced molecular communication. IEEE Trans. Signal Process. 2013, 61, 5000–5013. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Manocha, P.; Chandwani, G. Design Methodology of Passive In-Line Relays for Molecular Communication in Flow-Induced Microfluidic Channel. Biosensors 2021, 11, 65. https://doi.org/10.3390/bios11030065
Manocha P, Chandwani G. Design Methodology of Passive In-Line Relays for Molecular Communication in Flow-Induced Microfluidic Channel. Biosensors. 2021; 11(3):65. https://doi.org/10.3390/bios11030065
Chicago/Turabian StyleManocha, Puneet, and Gitanjali Chandwani. 2021. "Design Methodology of Passive In-Line Relays for Molecular Communication in Flow-Induced Microfluidic Channel" Biosensors 11, no. 3: 65. https://doi.org/10.3390/bios11030065
APA StyleManocha, P., & Chandwani, G. (2021). Design Methodology of Passive In-Line Relays for Molecular Communication in Flow-Induced Microfluidic Channel. Biosensors, 11(3), 65. https://doi.org/10.3390/bios11030065





