Influence of InAlN Nanospiral Structures on the Behavior of Reflected Light Polarization
Abstract
:1. Introduction
2. Experimental Details
3. Results and Discussion
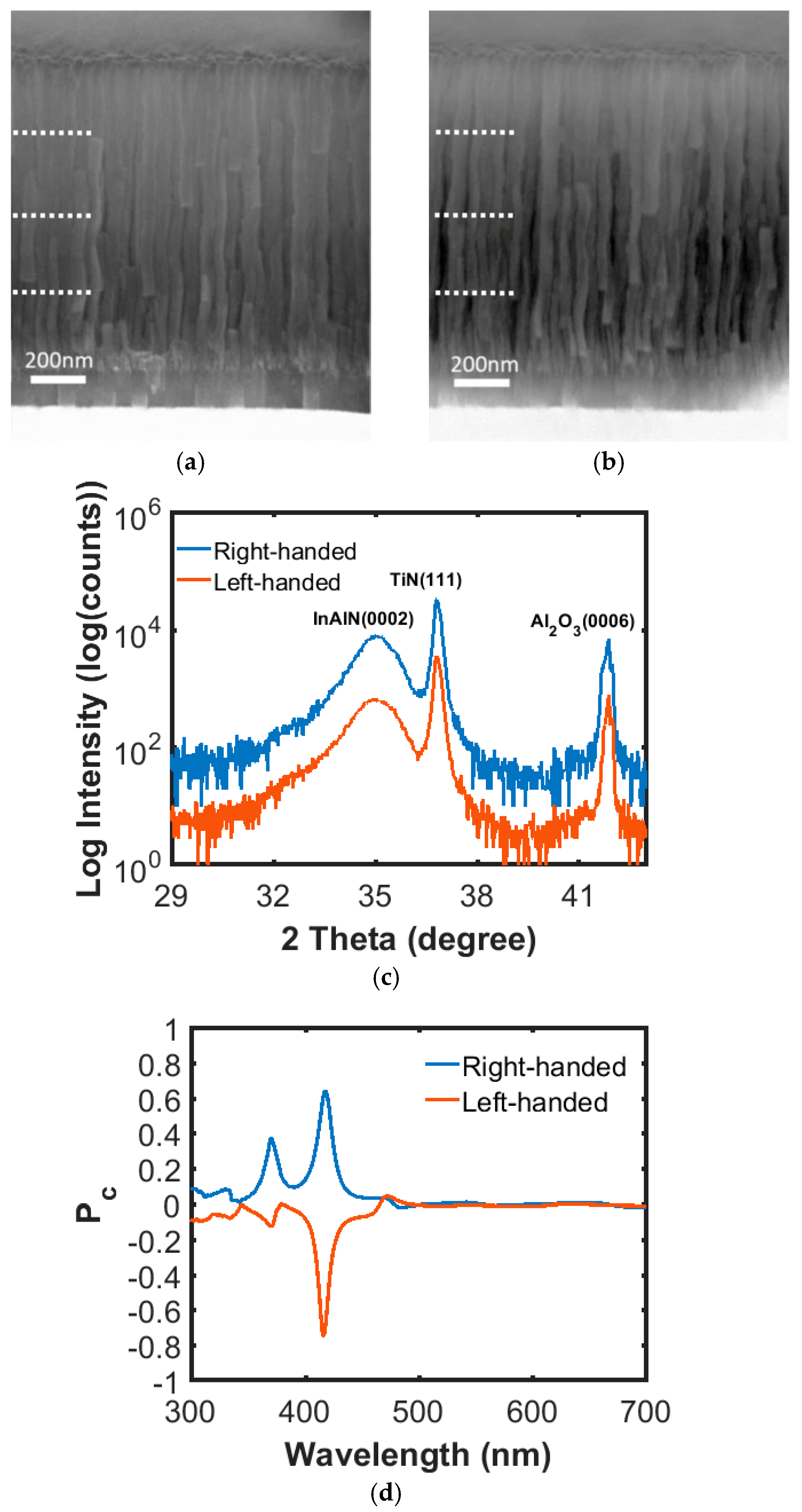
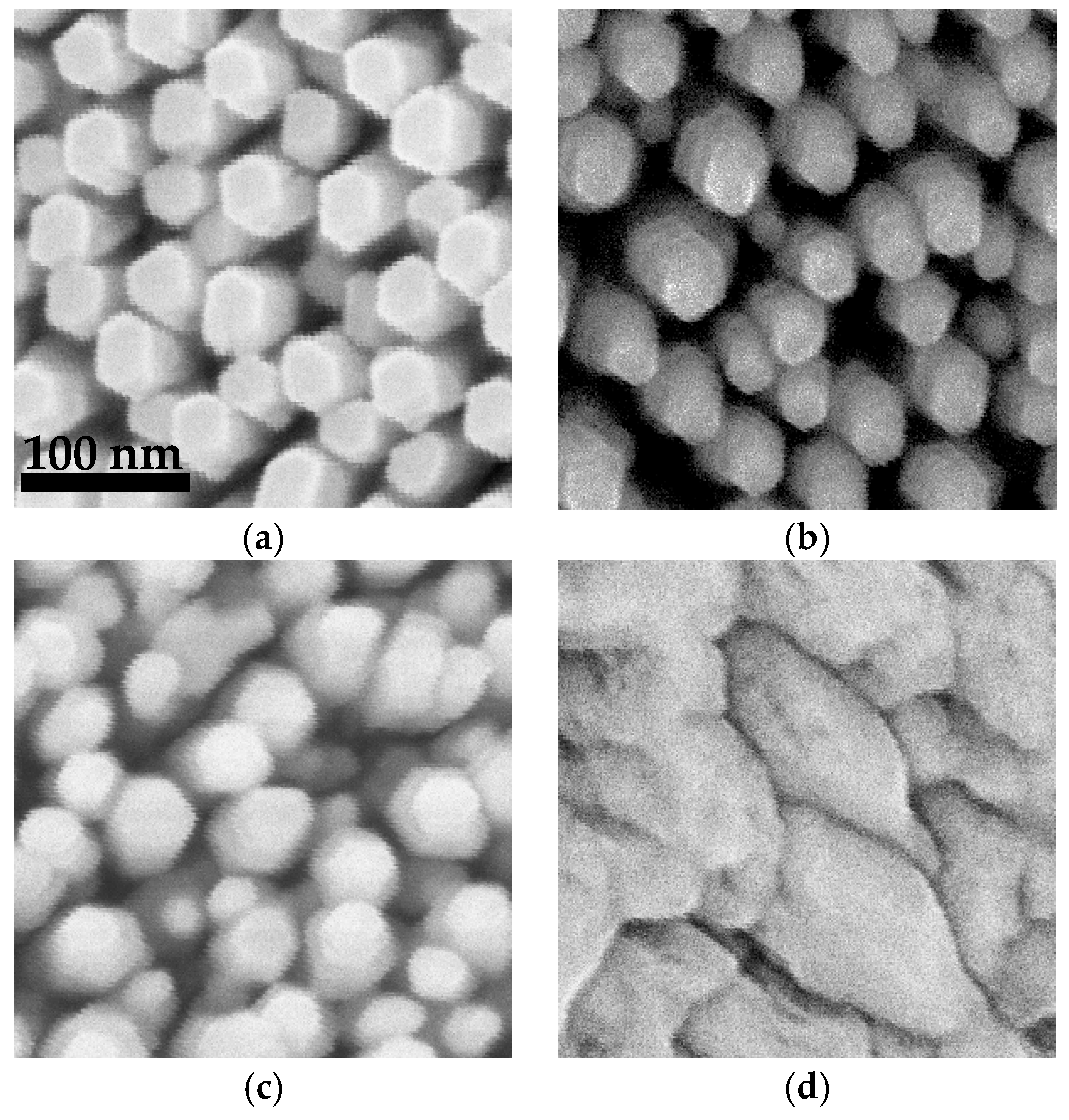
3.1. Nanospiral Chirality
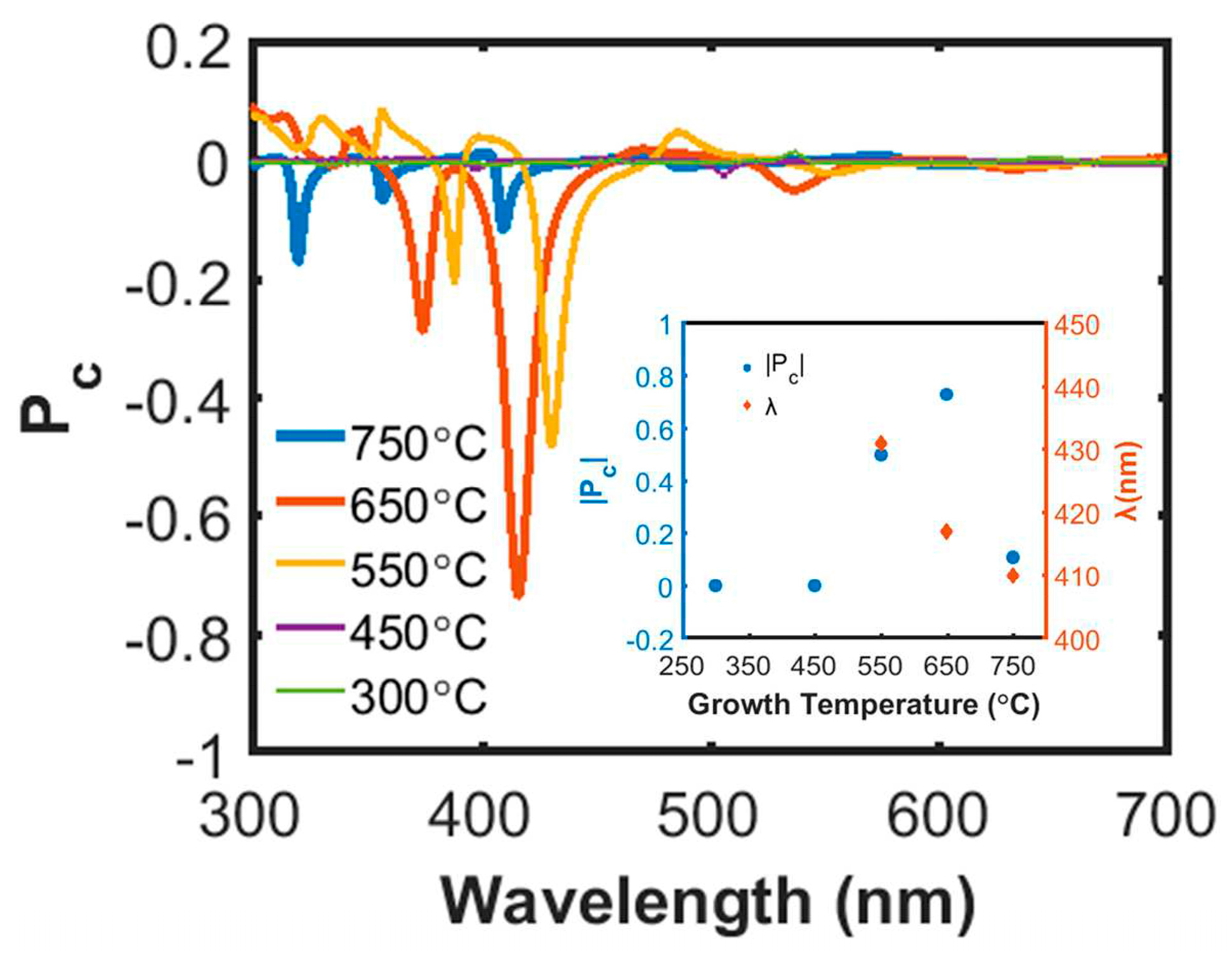
3.2. InAlN Growth Temperature
3.3. TiN Seed Layer
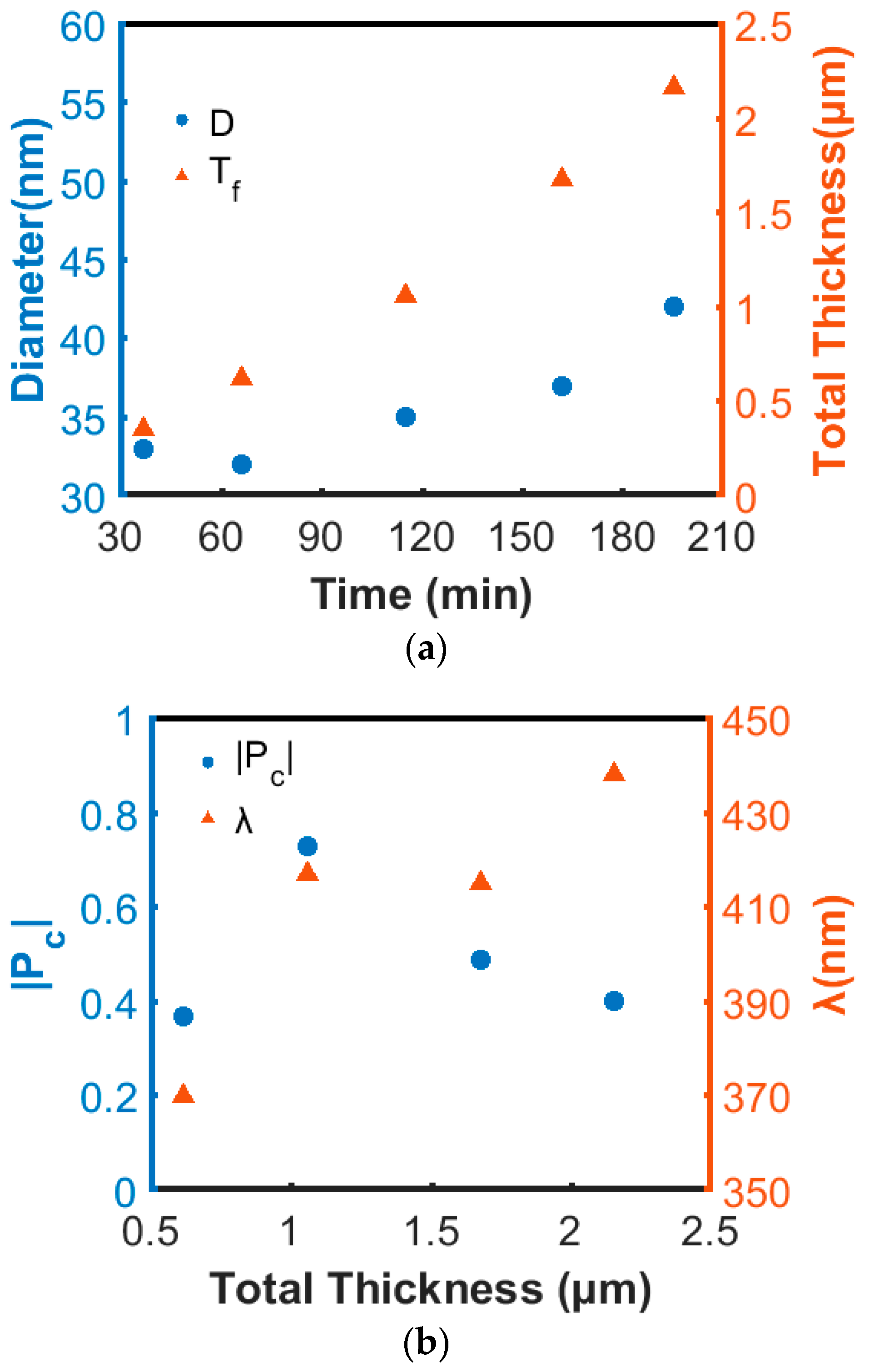
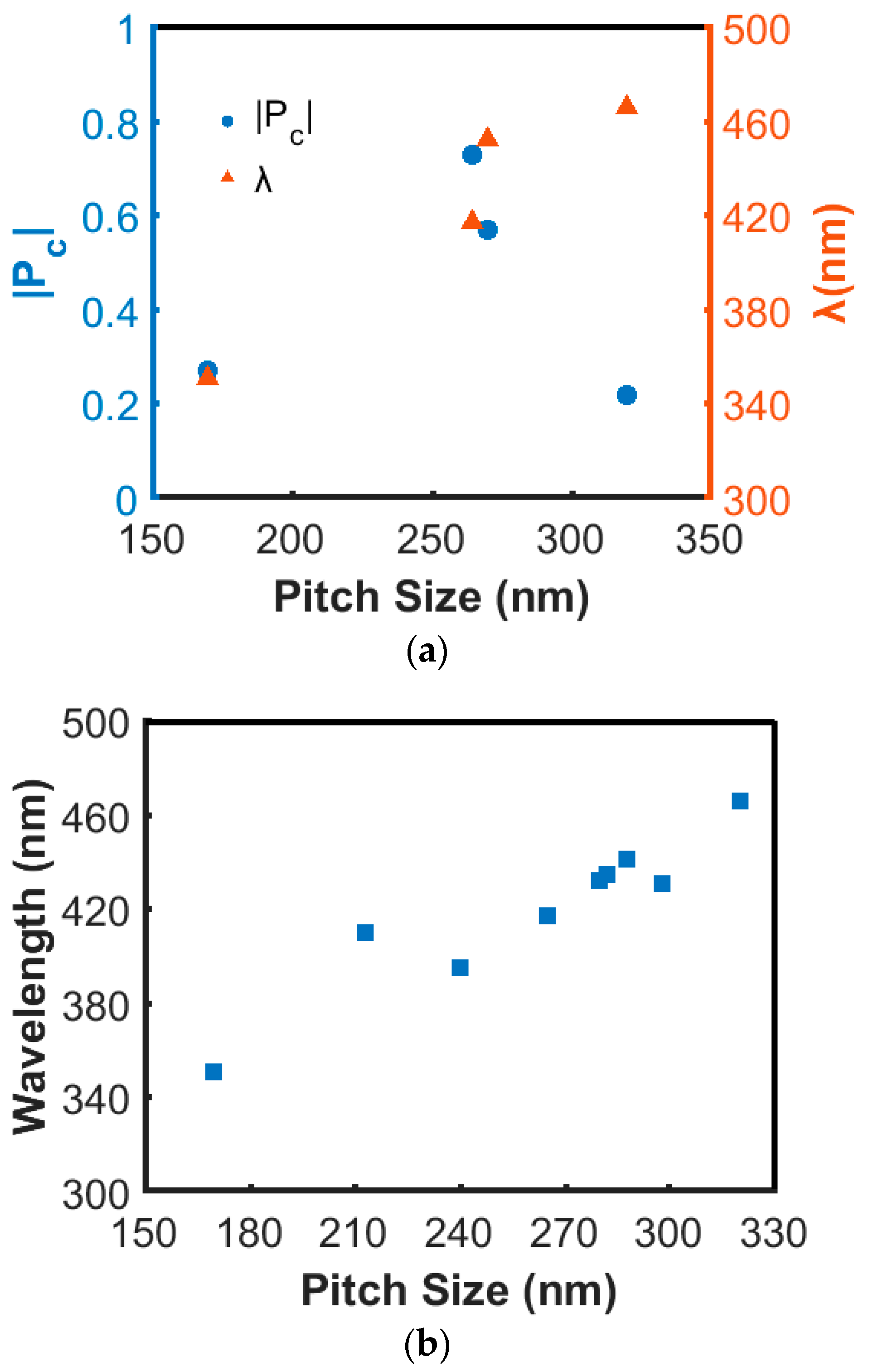
3.4. Total Thickness and Pitch of Nanospirals
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Arwin, H.; Magnusson, R.; Landin, J.; Järrendahl, K. Chirality-induced polarization effects in the cuticle of scarab beetles: 100 years after Michelson. Philos. Mag. 2012, 92, 1583–1599. [Google Scholar] [CrossRef]
- Sharma, V.; Crne, M.; Park, J.O.; Srinivasarao, M. Structural origin of circularly polarized iridescence in Jewwled Beetles. Science 2009, 325, 449–451. [Google Scholar] [CrossRef] [PubMed]
- Parker, A.R.; Townley, H.E. Biomimetics of photonic nanostructures. Nat. Photonics 2007, 2, 347–353. [Google Scholar] [CrossRef] [PubMed]
- Hodgkinson, I.J.; Lakhtakia, A.; Wu, Q.H. Experimental realization of sculptured-thin-film polarization discriminatory light-handedness inverters. Opt. Eng. 2000, 39, 2831–2834. [Google Scholar]
- Wu, Q.; Lakhtakia, A.; Hodgkinson, I.J. Circular polarization filters made of chiral sculptured thin films: Experimental and simulation results. Opt. Eng. 2000, 39, 1863–1868. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Radic, S. Phase-shifted fiber Bragg gratings and their application for wavelength demultiplexing. IEEE Photonics Technol. Lett. 1994, 6, 995–997. [Google Scholar] [CrossRef]
- Kim, Y.; Hong, K.; Yeom, J.; Hong, J.; Jung, J.-H.; Lee, Y.W.; Park, J.-H.; Le, B. A frontal projection-type three-dimensional display. Opt. Exp. 2012, 20, 20130–20138. [Google Scholar] [CrossRef] [PubMed]
- Krause, K.M.; Brett, M.J. Spatially graded nanostructured chiral films as tunable circular polarizers. Adv. Funct. Mater. 2008, 18, 3111–3118. [Google Scholar] [CrossRef]
- Hawkeye, M.M.; Brett, M.J. Glancing angle deposition: Fabrication, properties, and applications of micro- and nanostructured thin films. J. Vac. Sci. Technol. A 2007, 25, 1317–1335. [Google Scholar] [CrossRef]
- Deubel, M.; von Freymann, G.; Wegener, M.; Pereira, S.; Busch, K.; Soukoulis, C.M. Direct laser writing of three-dimensional photonic-crystal templates for telecommunications. Nat. Mater. 2004, 3, 444–447. [Google Scholar] [CrossRef] [PubMed]
- Gansel, J.K.; Thiel, M.; Rill, M.S.; Decker, M.B.; Saile, K.; Freymann, V.G.; Linden, S.; Wegener, M. Gold helix photonic metamaterial as broadband circular polarizer. Science 2009, 325, 1513–1515. [Google Scholar] [CrossRef] [PubMed]
- Pang, Y.K.; Lee, J.C.W.; Lee, H.F.; Tam, W.Y.; Chan, C.T.; Sheng, P. Chiral microstructures (spirals) fabrication by holographic lithography. Opt. Exp. 2005, 13, 7615–7620. [Google Scholar] [CrossRef]
- Hodgkinson, I.; Wu, Q.H. Inorganic Chiral Optical Materials. Adv. Mater. 2001, 13, 889–897. [Google Scholar] [CrossRef]
- Young, N.O.; Kowal, J. Optically active fluorite films. Nature 1959, 183, 104–105. [Google Scholar] [CrossRef]
- Hwang, J.; Song, M.H.; Park, B.; Nishimura, S.; Toyooka, T.; Wu, J.W.; Takanishi, Y.; Ishikawa, K.; Takezoe, H. Electro-tunable optical diode based on photonic bandgap liquid-crystal heterojunctions. Nat. Mater. 2005, 4, 383–387. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Potts, A.; Bagnall, D.M. Giant optical activity in dielectric planar metamaterials with two-dimensional chirality. J. Opt. A Pure Appl. Opt. 2006, 8, 878–890. [Google Scholar] [CrossRef]
- Robble, K.; Brett, M.J.; Lakhtakia, I. Chiral sculptured thin films. Nature 1996, 384, 616. [Google Scholar] [CrossRef]
- Valyukh, S.; Arwin, H.; Birch, J.; Järrendahl, K. Bragg reflection from periodic helicoidal media with laterally graded refractive index. Opt. Mater. 2017, 72, 334–340. [Google Scholar] [CrossRef]
- Radnóczi, G.Z.; Seppänen, T.; Pécz, B.; Hultman, L.; Birch, J. Growth of highly curved AlxIn1−xN nanocrystals. Phys. Stat. Sol. 2005, 202, R76–R78. [Google Scholar] [CrossRef]
- Hsiao, C.-L.; Magnusson, R.; Palisaitis, J.; Sandström, P.; Persson, P.O.Å.; Valyukh, S.; Hultman, L.; Järrendahl, K.; Birch, J. Curved-Lattice Epitaxial Growth of In1−xAlxN Nanospirals with Tailored Chirality. Nano Lett. 2015, 15, 294–300. [Google Scholar] [CrossRef] [PubMed]
- Magnusson, R.; Hsiao, C.L.; Birch, J.; Arwin, H.; Järrendahl, K. Chiral nanostructures producing near circular polarization. Opt. Mater. Exp. 2014, 4, 1389–1403. [Google Scholar] [CrossRef]
- Magnusson, R.; Birch, J.; Sandström, P.; Hsiao, C.L.; Arwin, H.; Järrendahl, K. Optical Mueller Matrix Modeling of Chiral AlxIn1−xN Nanospirals. Thin Solid Films 2014, 571, 447–452. [Google Scholar] [CrossRef]
- Hsiao, C.L.; Palisaitis, J.; Junaid, M.; Persson, P.O.Å.; Jensen, J.; Zhao, Q.-X.; Chen, L.C.; Chen, K.H.; Birch, J. Room-temperature heteroepitaxy of single-phase Al1−xInxN films with full composition range on isostructural wurtzite substrates. Thin Solid Films 2012, 524, 113–120. [Google Scholar] [CrossRef]
- Hsiao, C.L.; Palisaitis, J.; Junaid, M.; Chen, R.S.; Persson, P.O.Å.; Sandström, P.; Holtz, P.O.; Hultman, L.; Birch, J. Spontaneous formation of AlInN core-shell nanorod arrays by ultrahigh-vacuum magnetron sputter epitaxy. Appl. Phys. Exp. 2011, 4, 115002. [Google Scholar] [CrossRef]
- Palisaitis, J.; Hsiao, C.-L.; Hultman, L.; Birch, J.; Persson, P.O.Å. Core-shell formation in self-induced InAlN nanorods. Nanotechnology 2017, 7, 015303. [Google Scholar] [CrossRef] [PubMed]
- Hsiao, C.L.; Palisaitis, J.; Persson, P.O.Å.; Junaid, M.; Sandström, P.; Hultman, L.; Birch, J. Nucleation and core-shell formation mechanism of self-induced InxAl1−xN core-shell nanorods grown on sapphire substrates by magnetron sputter epitaxy. Vacuum 2016, 131, 39–43. [Google Scholar] [CrossRef]
- Serban, E.A.; Persson, P.O.Å.; Poenaru, I.; Junaid, M.; Hultman, L.; Birch, J.; Hsiao, C.L. Structural and compositional evolutions of InxAl1−xN core-shell nanorods grown on Si(111) substrates by reactive magnetron sputter epitaxy. Nanotechnology 2015, 26, 215602. [Google Scholar] [CrossRef] [PubMed]
- Palisaitis, J.; Hsiao, C.-L.; Hultman, L.; Birch, J.; Persson, P.O.Å. Direct observation of spinodal decomposition phenomena in InAlN alloy during in-situ STEM heating. Sci. Rep. 2017, 7, 44390. [Google Scholar] [CrossRef] [PubMed]
- Jeyachandran, Y.L.; Narayandass, S.K.; Mangalaraj, D.; Areva, S.; Mielczarski, J.A. Properties of titanium nitride films prepared by direct current magnetron sputtering. Mater. Sci. Eng. A 2007, 445–446, 223–236. [Google Scholar] [CrossRef]
- Martin, P.M. Handbook of Deposition Technologies for Films and Coatings: Science, Application and Technology, 3rd ed.; William Andrew: Norwich, UK, 2010; Chapter 5; pp. 253–296. [Google Scholar]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuo, Y.-H.; Magnusson, R.; Serban, E.A.; Sandström, P.; Hultman, L.; Järrendahl, K.; Birch, J.; Hsiao, C.-L. Influence of InAlN Nanospiral Structures on the Behavior of Reflected Light Polarization. Nanomaterials 2018, 8, 157. https://doi.org/10.3390/nano8030157
Kuo Y-H, Magnusson R, Serban EA, Sandström P, Hultman L, Järrendahl K, Birch J, Hsiao C-L. Influence of InAlN Nanospiral Structures on the Behavior of Reflected Light Polarization. Nanomaterials. 2018; 8(3):157. https://doi.org/10.3390/nano8030157
Chicago/Turabian StyleKuo, Yu-Hung, Roger Magnusson, Elena Alexandra Serban, Per Sandström, Lars Hultman, Kenneth Järrendahl, Jens Birch, and Ching-Lien Hsiao. 2018. "Influence of InAlN Nanospiral Structures on the Behavior of Reflected Light Polarization" Nanomaterials 8, no. 3: 157. https://doi.org/10.3390/nano8030157
APA StyleKuo, Y.-H., Magnusson, R., Serban, E. A., Sandström, P., Hultman, L., Järrendahl, K., Birch, J., & Hsiao, C.-L. (2018). Influence of InAlN Nanospiral Structures on the Behavior of Reflected Light Polarization. Nanomaterials, 8(3), 157. https://doi.org/10.3390/nano8030157






