Effects of Residual Stress Distribution on Interfacial Adhesion of Magnetron Sputtered AlN and AlN/Al Nanostructured Coatings on a (100) Silicon Substrate
Abstract
1. Introduction
2. Experimental Details
3. Results and Discussion
3.1. Residual Stress Measurement
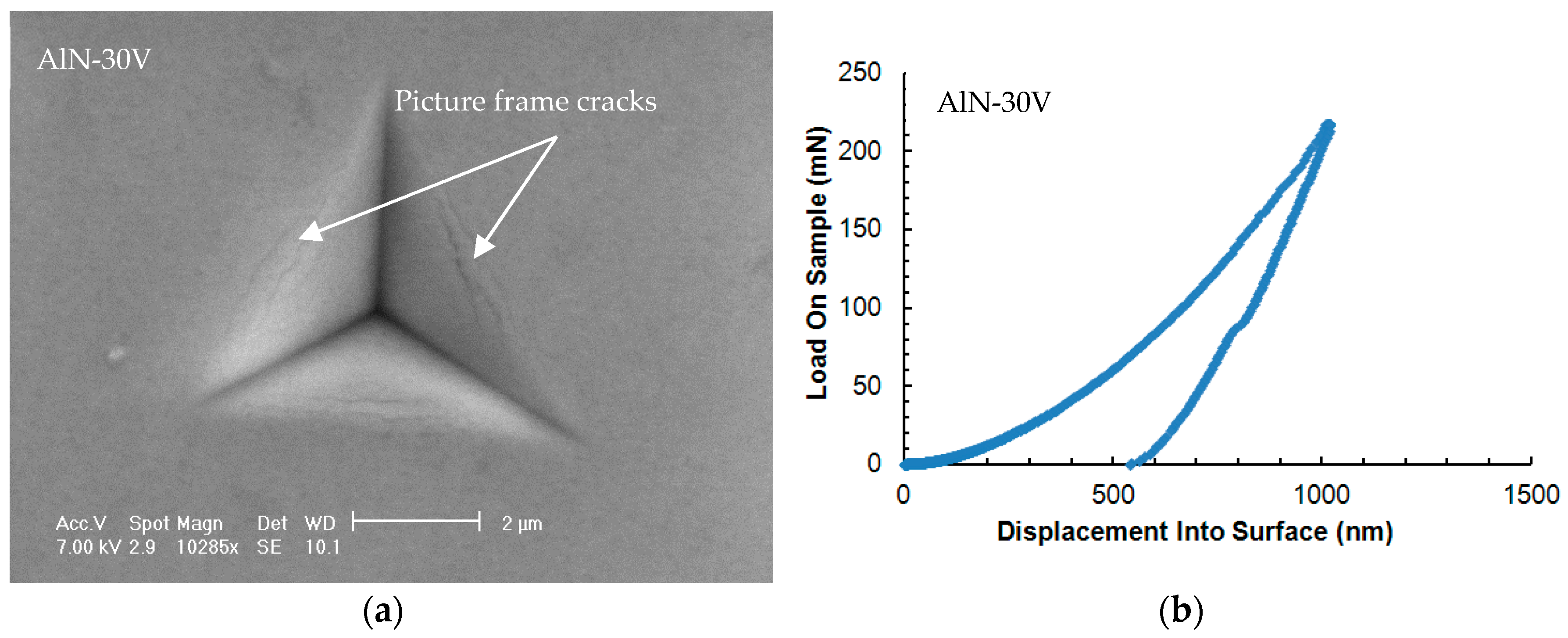
3.2. Nanoindentation Characterizations
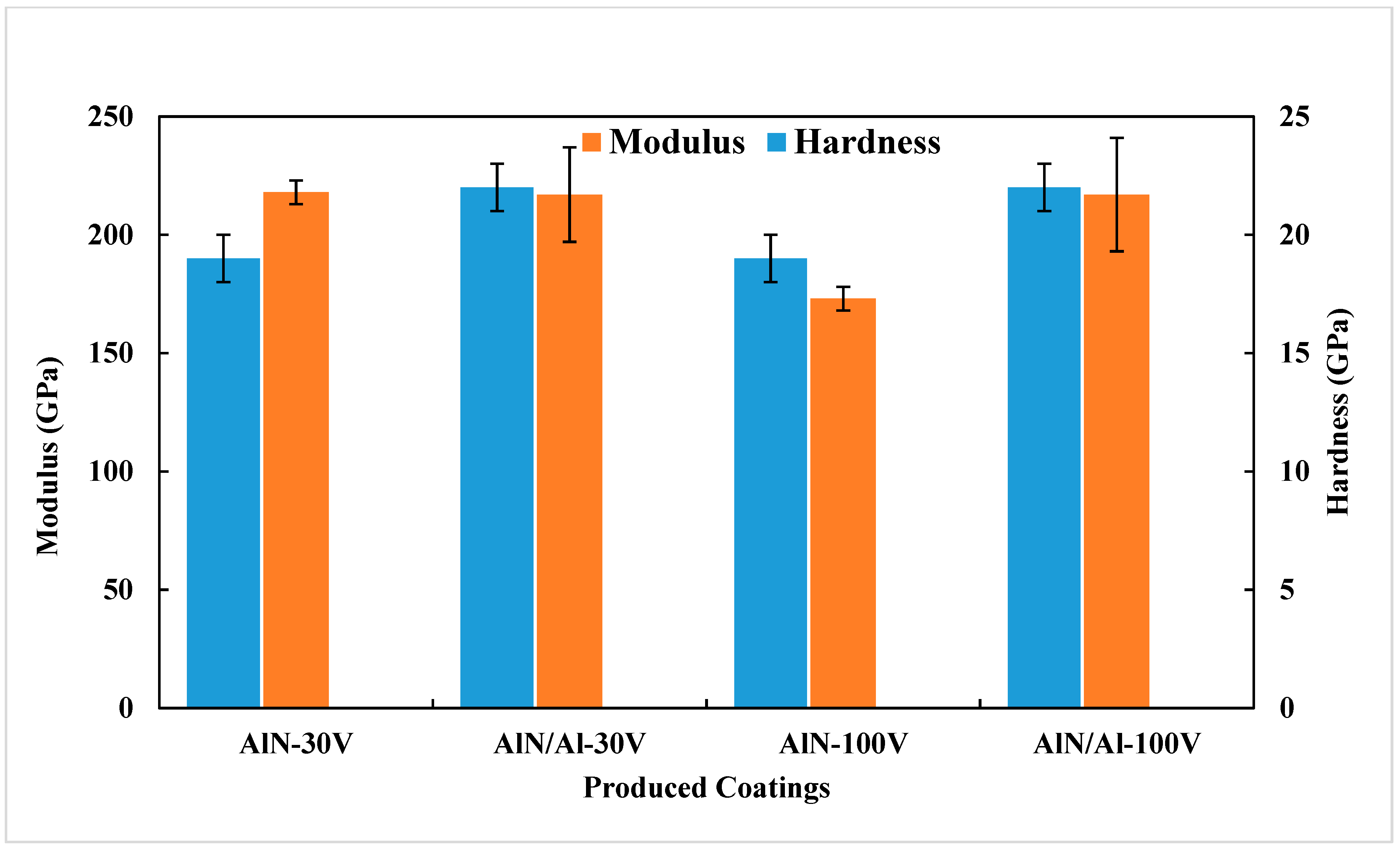
3.2.1. Hardness and Elastic Modulus
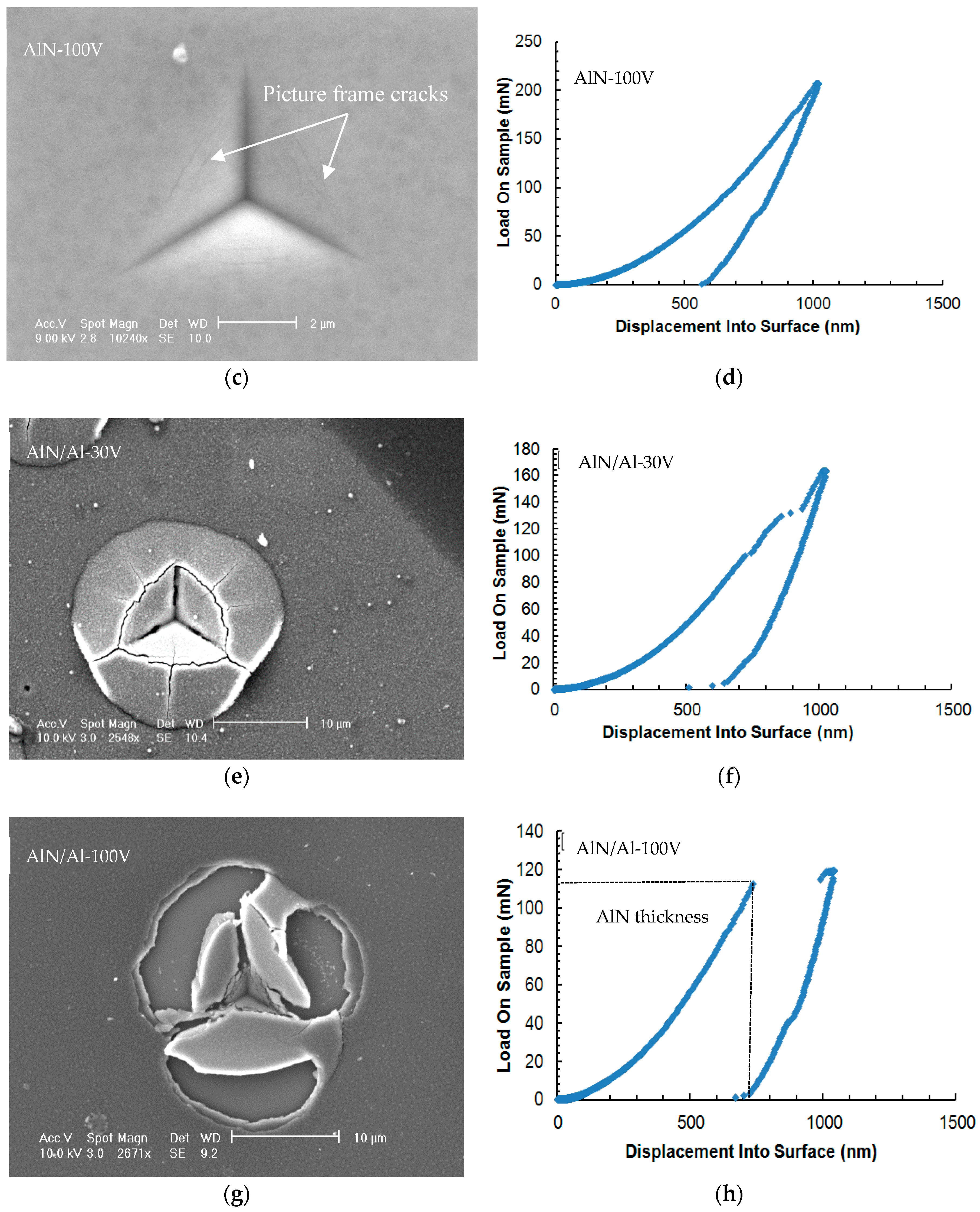
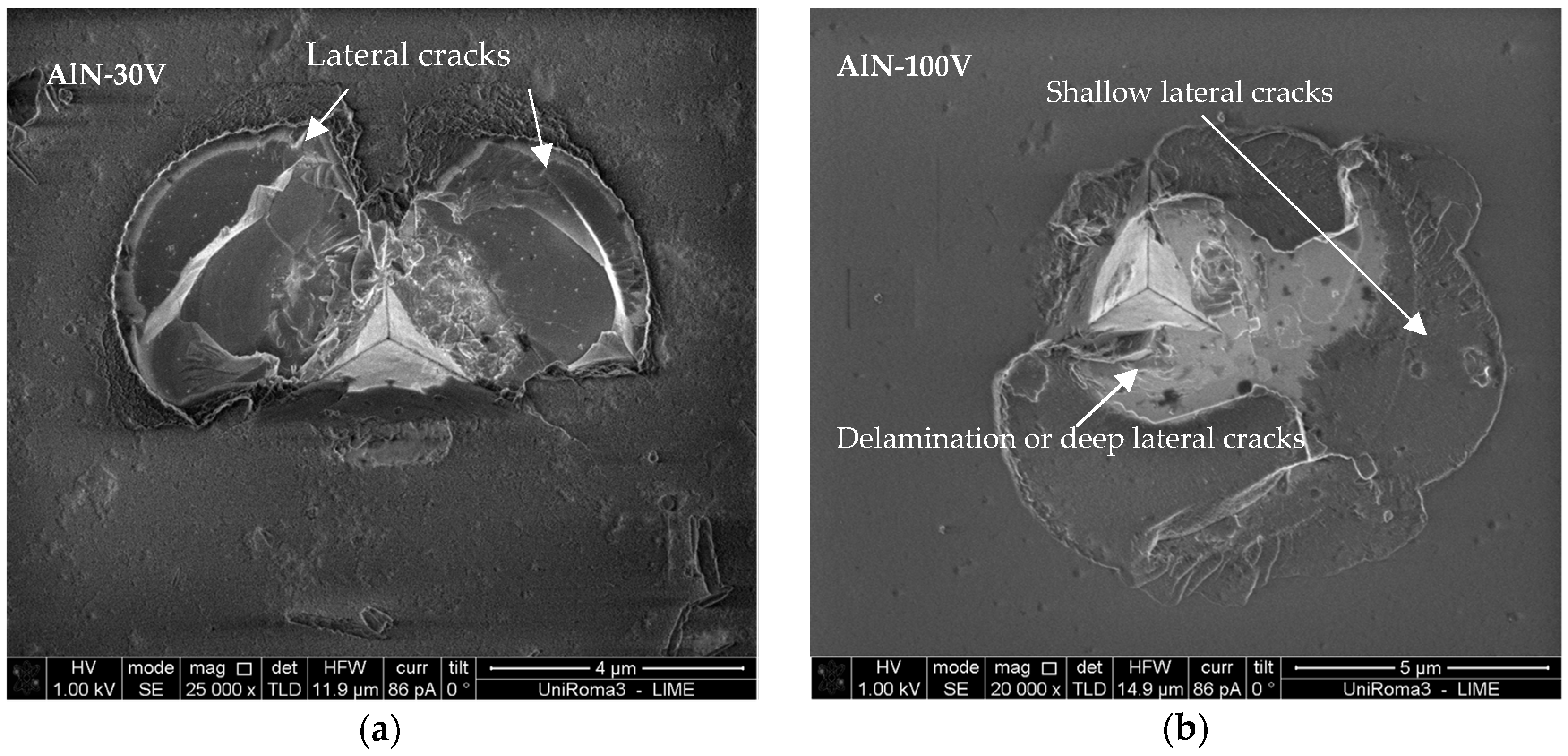
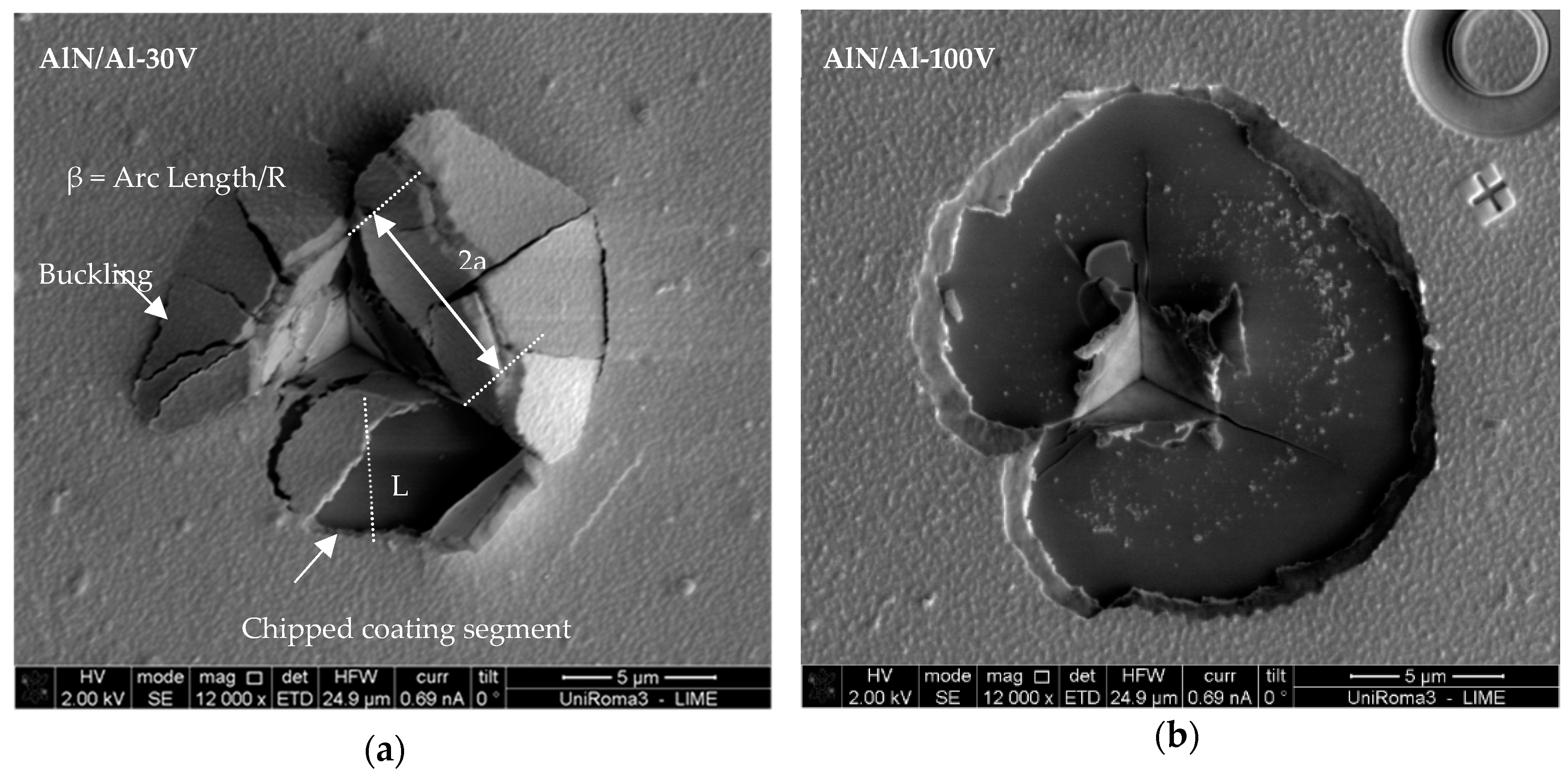
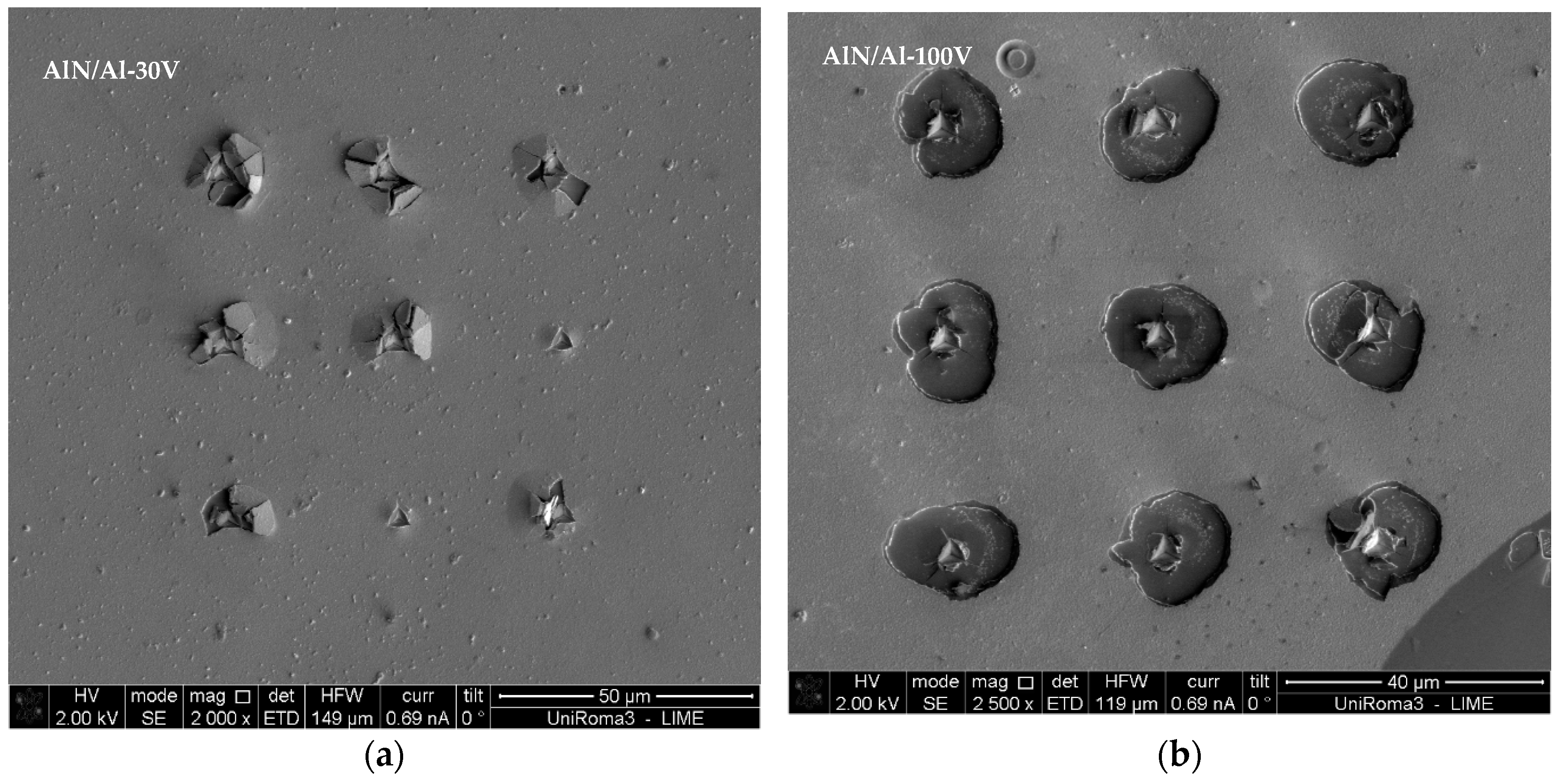
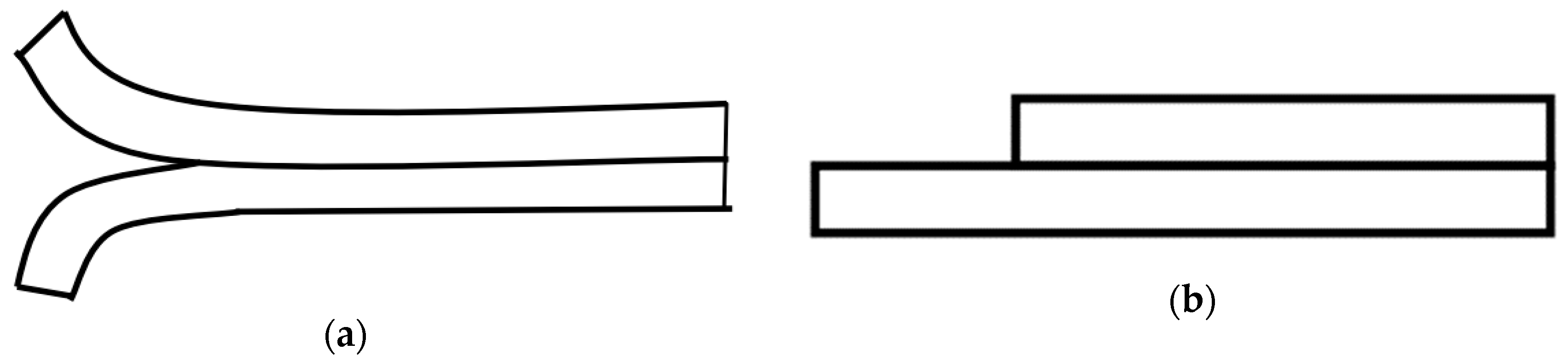
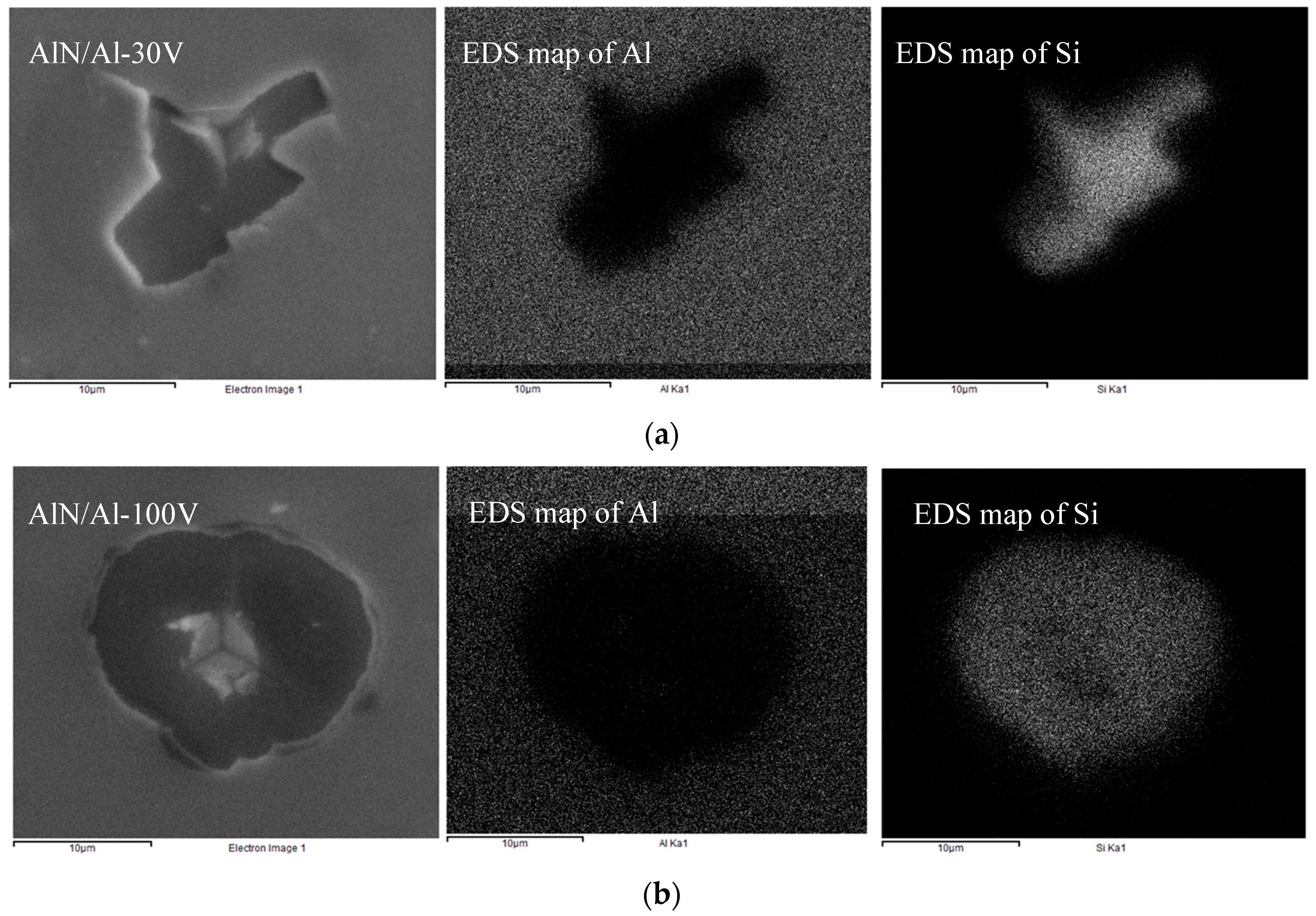
3.2.2. Quantitative Evaluation of Coatings’ Interfacial Adhesion
4. Conclusions
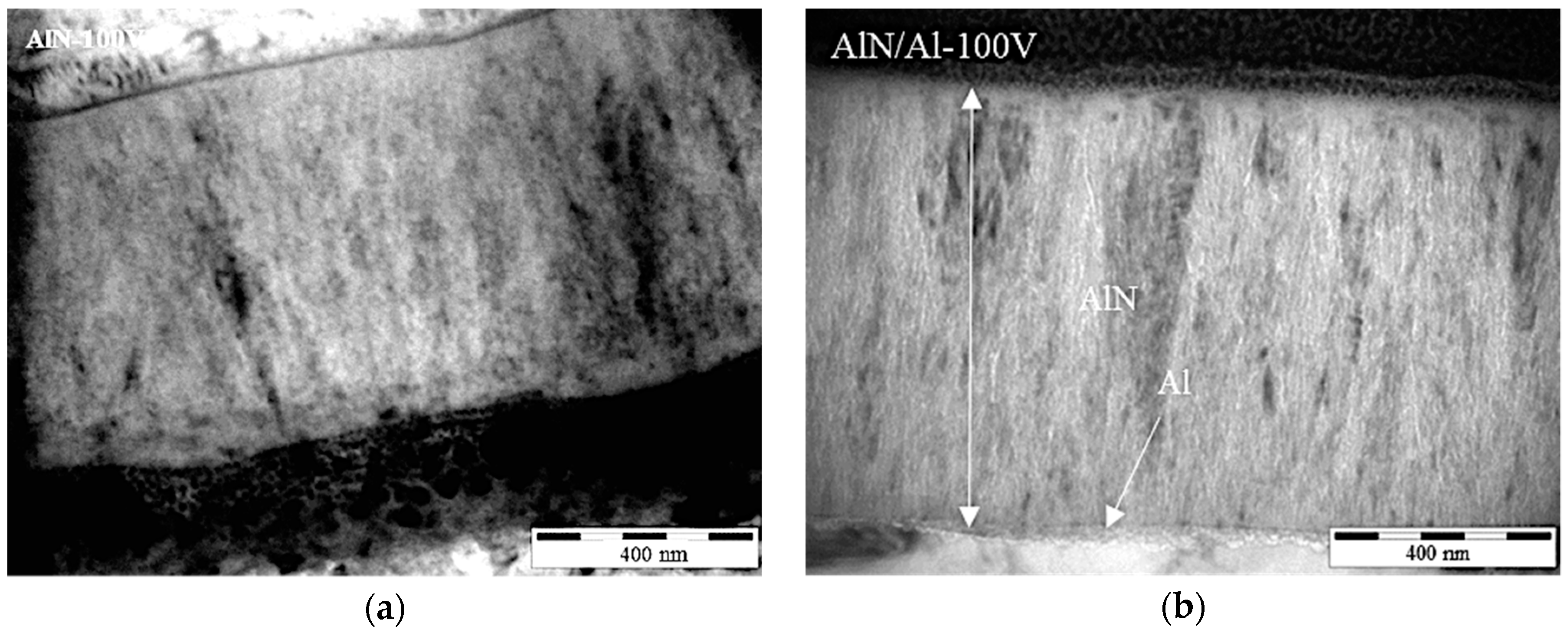
- An increase in the average compressive residual stress in AlN coatings on the silicon substrate was measured with an increase in bias voltage and coatings deposited with the Al bond layer. Higher bias voltage accelerates the gas ions toward the growing coating and the additional energy caused the enhancement of residual stresses. It is also possible that the different bias conditions affect the interface between Si and AlN, in addition to increasing the compressive residual stresses. The residual stress was further increased by adding an Al bond layer, as a result of its high thermal expansion coefficient in comparison with AlN and the silicon substrate. As a consequence, tensile thermal stresses during cooling were established in the Al, which caused additional compressive residual stresses in the AlN/Al coatings.
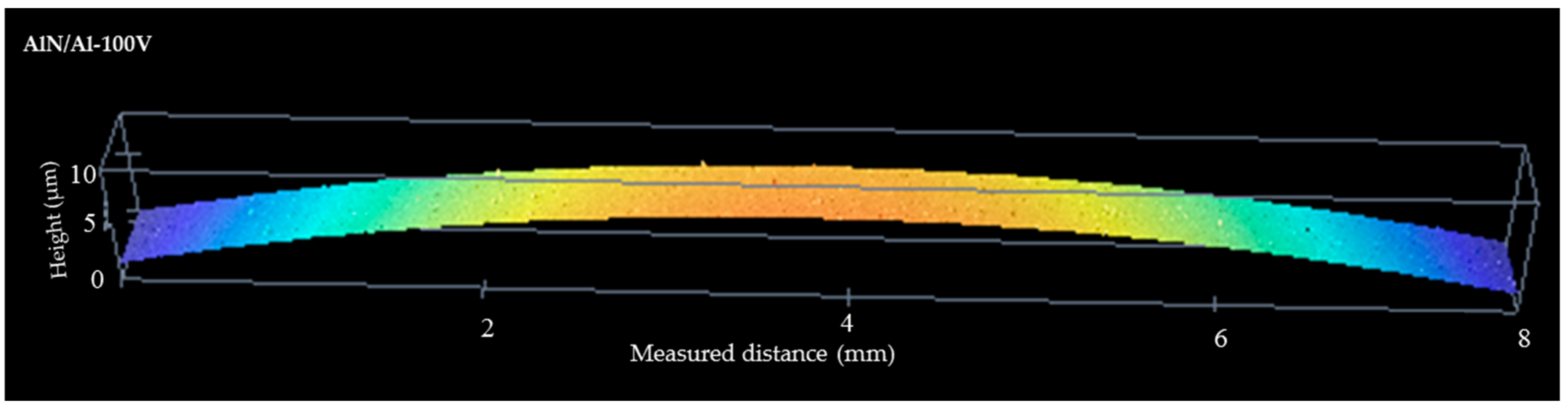
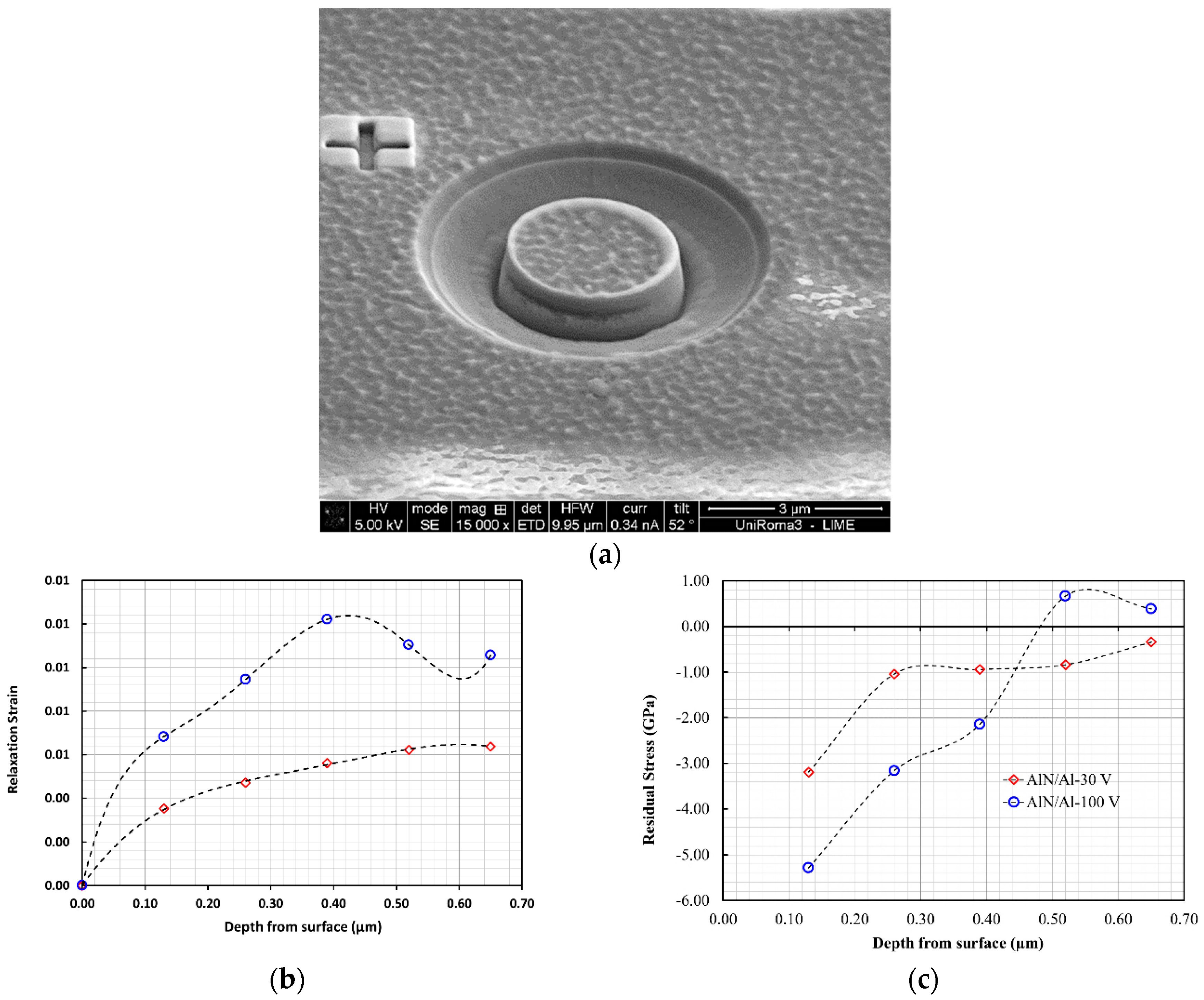
- FIB-DIC residual stress depth profiles showed that for the AlN/Al coatings, a strong residual stress gradient was present in the films, being the maximum compressive residual stress on the top surface, then decreasing towards the interface; a mild tensile stress was observed even for the AlN/Al-100V sample, which was the one showing more extended delamination.
- The coatings deposited without the Al bond layer showed significantly better adhesion in comparison to those with the bond layer, even though both groups of coatings had differences in compressive residual stresses, equivalent to thermal stresses in the bond layer. Adhesive failures could not be produced in the coatings without a bond layer (AlN-30V and AlN-100V), even with a cube-corner indenter, which means that the interface adhesion was higher in comparison with the coatings deposited with the Al bond layer. The fracture toughness corresponding to lateral cracks was measured to be 2.3 and 1.8 MPa m0.5 for the coatings AlN-30V and AlN-100V, having residual stresses of 1.2 and 3.5 GPa, respectively. The observed behavior could be related to more homogenous compressive residual stress distribution in the AlN top layer.
- The adhesion energy for interfacial separation was found to be 5 and 4 J/m2 for the coatings AlN/Al-30V and AlN/Al-100V, having an average residual stress of 1.5 and 3.9 GPa, respectively, with a corresponding interfacial fracture toughness of 0.71 and 0.65 MPa·m0.5, which was significantly lower than the estimated fracture toughness of the AlN material, thus explaining why delamination occurs for AlN/Al systems. The tensile residual stress on the bond layer, coupled with its plastic deformation during indentation, leads to interface delamination.
- These results suggested that in order to be beneficial for adhesion, a metallic bond layer should possess good hardness, a small difference in the thermal expansion coefficient, and a comparable elastic modulus with both the substrate and top coating. Refractory metals are a good prospect for the design of improved multilayer coating systems with optimal performance.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bian, Y.; Liu, M.; Ke, G.; Chen, Y.; DiBattista, J.; Chan, E.; Yang, Y. Aluminum nitride thin film growth and applications for heat dissipation. Surf. Coat. Technol. 2015, 267, 65–69. [Google Scholar] [CrossRef]
- Abadias, G.; Chason, E.; Keckes, J.; Sebastiani, M.; Thompson, G.B.; Barthel, E.; Doll, G.L.; Murray, C.E.; Stoessel, C.H.; Martinu, L. Stress in thin films and coatings: Current status, challenges, and prospects. J. Vac. Sci. Technol. A Vac. Surf. Films 2018, 36, 020801. [Google Scholar] [CrossRef]
- Ali, R.; Sebastiani, M.; Bemporad, E. Influence of Ti–TiN multilayer PVD-coatings design on residual stresses and adhesion. Mater. Des. 2015, 75, 47–56. [Google Scholar] [CrossRef]
- Renzelli, M.; Mughal, M.Z.; Sebastiani, M.; Bemporad, E. Design, fabrication and characterization of multilayer Cr-CrN thin coatings with tailored residual stress profiles. Mater. Des. 2016, 112, 162–171. [Google Scholar] [CrossRef]
- Kleinbichler, A.; Pfeifenberger, M.J.; Zechner, J.; Moody, N.R.; Bahr, D.F.; Cordill, M.J. New Insights into Nanoindentation-Based Adhesion Testing. JOM 2017, 69, 2237–2245. [Google Scholar] [CrossRef]
- Kriese, M.D.; Gerberich, W.W.; Moody, N.R. Quantitative adhesion measures of multilayer films: Part II. Indentation of W/Cu, W/W, Cr/W. J. Mater. Res. 1999, 14, 3019–3026. [Google Scholar] [CrossRef]
- Khanna, V. Adhesion–delamination phenomena at the surfaces and interfaces in microelectronics and MEMS structures and packaged devices. J. Phys. D Appl. Phys. 2010, 44, 034004. [Google Scholar] [CrossRef]
- Liu, D.; Pallon, L.K.H.; Pourrahimi, A.M.; Zhang, P.; Diaz, A.; Holler, M.; Schneider, K.; Olsson, R.T.; Hedenqvist, M.S.; Yu, S.; et al. Cavitation in strained polyethylene/aluminium oxide nanocomposites. Eur. Polym. J. 2017, 87, 255–265. [Google Scholar] [CrossRef]
- Stoney, G.G. The tension of metallic films deposited by electrolysis. Proc. R. Soc. Lond. Ser. A Contain. Pap. Math. Phys. Character 1909, 82, 172–175. [Google Scholar] [CrossRef]
- Janssen, G.C.A.M.; Abdalla, M.M.; van Keulen, F.; Pujada, B.R.; van Venrooy, B. Celebrating the 100th anniversary of the Stoney equation for film stress: Developments from polycrystalline steel strips to single crystal silicon wafers. Thin Solid Films 2009, 517, 1858–1867. [Google Scholar] [CrossRef]
- Advanced technical ceramics. Methods of test for ceramic coatings. Determination of internal stress by the Stoney formula. In BS DD CEN/TS 1071; BSI: London, UK, 2005. [Google Scholar]
- Daniel, R.; Martinschitz, K.; Keckes, J.; Mitterer, C. The origin of stresses in magnetron-sputtered thin films with zone T structures. Acta Mater. 2010, 58, 2621–2633. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; Sebastiani, M.; Bemporad, E. Focused ion beam ring drilling for residual stress evaluation. Mater. Lett. 2009, 63, 1961–1963. [Google Scholar] [CrossRef]
- Bemporad, E.; Brisotto, M.; Depero, L.; Gelfi, M.; Korsunsky, A.; Lunt, A.; Sebastiani, M. A critical comparison between XRD and FIB residual stressmeasurement techniques in thin films. Thin Solid Films 2014, 572, 224–231. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; Salvati, E.; Lunt, A.G.J.; Sui, T.; Mughal, M.Z.; Daniel, R.; Keckes, J.; Bemporad, E.; Sebastiani, M. Nanoscale residual stress depth profiling by Focused Ion Beam milling and eigenstrain analysis. Mater. Des. 2018, 145, 55–64. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- Toonder, J.D.; Malzbender, J.; With, G.D.; Balkenende, R. Fracture Toughness and Adhesion Energy of Sol-gel Coatings on Glass. J. Mater. Res. 2002, 17, 224–233. [Google Scholar] [CrossRef]
- Thouless, M.D. An analysis of spalling in the microscratch test. Eng. Fract. Mech. 1998, 61, 75–81. [Google Scholar] [CrossRef]
- Castanho, J.; Vieira, M. Effect of ductile layers in mechanical behaviour of TiAlN thin coatings. J. Mater. Process. Technol. 2003, 143, 352–357. [Google Scholar] [CrossRef]
- Chen, J.; Bull, S. Indentation Fracture and Toughness Assessment for Thin Optical Coatings on Glass. J. Phys. D Appl. Phys. 2007, 40, 5401. [Google Scholar] [CrossRef]
- Pharr, G.M.; Oliver, W.C.; Harding, D.S. New evidence for a pressure-induced phase transformation during the indentation of silicon. J. Mater. Res. 1991, 6, 1129–1130. [Google Scholar] [CrossRef]
- Haberl, B.; Bradby, J.; Swain, M.; Williams, J.; Munroe, P. Phase transformations induced in relaxed amorphous silicon by indentation at room temperature. Appl. Phys. Lett. 2004, 85, 5559–5561. [Google Scholar] [CrossRef]
- Ghidelli, M.; Sebastiani, M.; Johanns, K.E.; Pharr, G.M. Effects of indenter angle on micro-scale fracture toughness measurement by pillar splitting. J. Am. Ceram. Soc. 2017, 100, 5731–5738. [Google Scholar] [CrossRef]
- Morris, D.J.; Cook, R.F. Indentation fracture of low-dielectric constant films: Part I. Experiments and observations. J. Mater. Res. 2008, 23, 2429–2442. [Google Scholar] [CrossRef]
- Anstis, G.; Chantikul, P.; Lawn, B.R.; Marshall, D. A critical evaluation of indentation techniques for measuring fracture toughness: I, direct crack measurements. J. Am. Ceram. Soc. 1981, 64, 533–538. [Google Scholar] [CrossRef]
- Koumoulos, E.P.; Tofail, S.A.M.; Silien, C.; De Felicis, D.; Moscatelli, R.; Dragatogiannis, D.A.; Bemporad, E.; Sebastiani, M.; Charitidis, C.A. Metrology and nano-mechanical tests for nano-manufacturing and nano-bio interface: Challenges & future perspectives. Mater. Des. 2018, 137, 446–462. [Google Scholar]
- Bull, S.J. Nanoindentation of coatings. J. Phys. D Appl. Phys. 2005, 38, R393. [Google Scholar] [CrossRef]
- Iriarte, G.F.; Engelmark, F.; Ottosson, M.; Katardjiev, I. Influence of deposition parameters on the stress of magnetron sputter-deposited AlN thin films on Si (100) substrates. J. Mater. Res. 2003, 18, 423–432. [Google Scholar] [CrossRef]
- Jones, A.M.; McCabe, A.R.; Bull, S.J.; Dearnaley, G. The effects of deposition temperature and interlayer thickness on the adhesion of ion-assisted titanium nitride films produced with yttrium metal interlayers. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 1993, 80–81, 1397–1401. [Google Scholar] [CrossRef]
- Gerth, J.; Wiklund, U. The influence of metallic interlayers on the adhesion of PVD TiN coatings on high-speed steel. Wear 2008, 264, 885–892. [Google Scholar] [CrossRef]
- Suo, Z.; Hutchinson, J.W. Interface crack between two elastic layers. Int. J. Fract. 1990, 43, 1–18. [Google Scholar] [CrossRef]
- Ionescu, G.; Nae, I.; Ripeanu, R.; Dinita, A.; Stan, G. Studies on Tribological Behavior of Aluminum Nitride-Coated Steel. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2017; p. 012052. [Google Scholar]
- Steinwandel, J.; Hoeschele, J.; Staneff, T.; Heuberger, A. Method and Apparatus for Aluminum Nitride Coating of a Contact Surface, Especially a Cylinder Contact Surface of a Crankcase Made of an Aluminum Basic Alloy. U.S. Patents 6,180,189, 30 January 2001. [Google Scholar]
- Pina Bhatt, D.H. Performance Evaluation of TiAlN and CrN Coated Silicon Wafer by Magnetron Sputtering Method. Int. J. Nanotechnol. Appl. 2017, 11, 167–177. [Google Scholar]
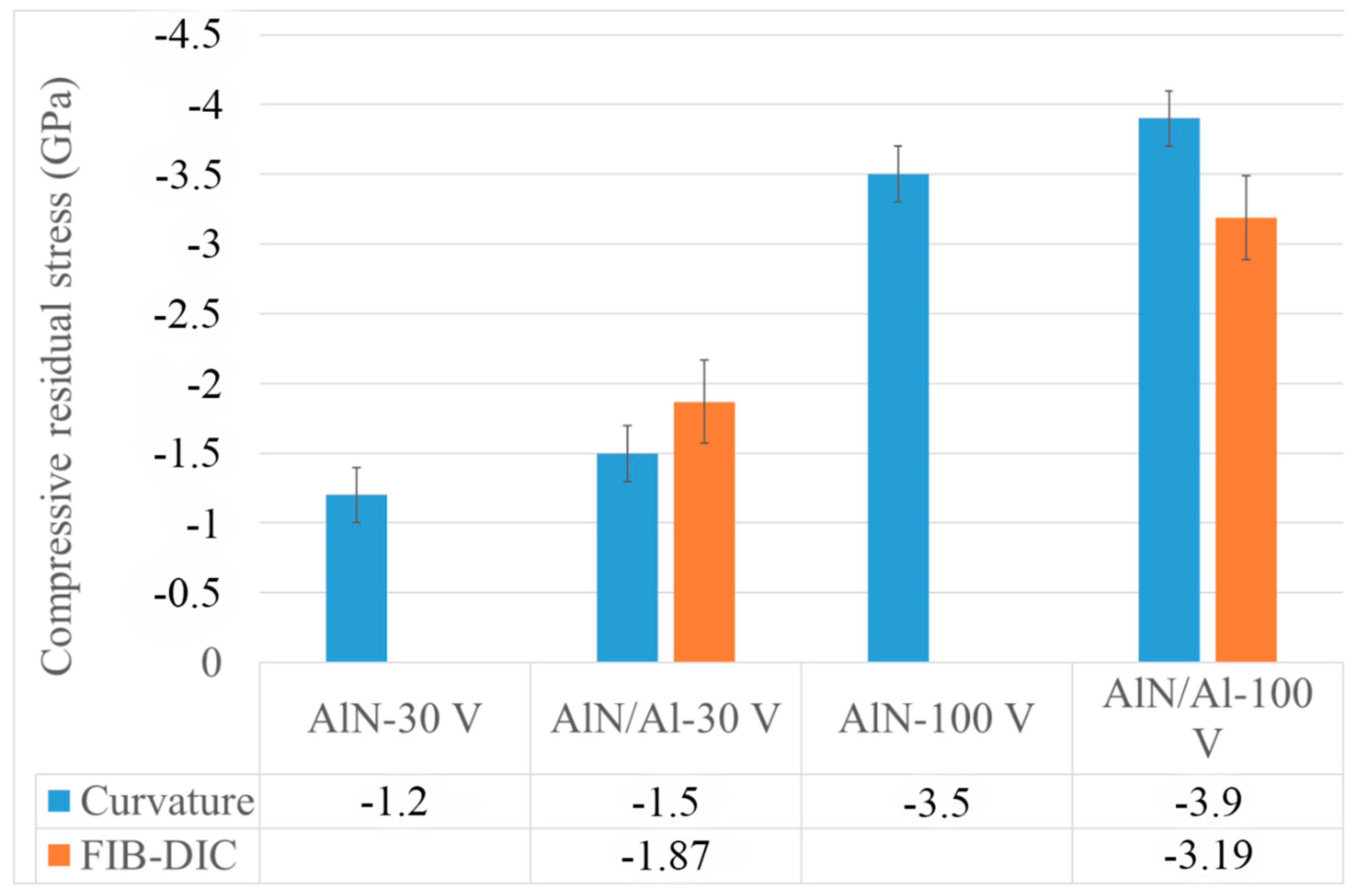
| Description of Coatings | Bias Voltage (V) | Bond Layer (Yes/No) | Thickness (μm) | Average Residual Stress (GPa) |
|---|---|---|---|---|
| AlN-30V | −30 | No | 0.75 ± 0.05 | −1.2 ± 0.2 |
| AlN/Al-30V | −30 | Yes | 0.76 ± 0.02 | −1.5 ± 0.2 |
| AlN-100V | −100 | No | 0.72 ± 0.03 | −3.5 ± 0.2 |
| AlN/Al-100V | −100 | Yes | 0.71 ± 0.04 | −3.9 ± 0.2 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, R.; Renzelli, M.; Khan, M.I.; Sebastiani, M.; Bemporad, E. Effects of Residual Stress Distribution on Interfacial Adhesion of Magnetron Sputtered AlN and AlN/Al Nanostructured Coatings on a (100) Silicon Substrate. Nanomaterials 2018, 8, 896. https://doi.org/10.3390/nano8110896
Ali R, Renzelli M, Khan MI, Sebastiani M, Bemporad E. Effects of Residual Stress Distribution on Interfacial Adhesion of Magnetron Sputtered AlN and AlN/Al Nanostructured Coatings on a (100) Silicon Substrate. Nanomaterials. 2018; 8(11):896. https://doi.org/10.3390/nano8110896
Chicago/Turabian StyleAli, Rashid, Marco Renzelli, M. Imran Khan, Marco Sebastiani, and Edoardo Bemporad. 2018. "Effects of Residual Stress Distribution on Interfacial Adhesion of Magnetron Sputtered AlN and AlN/Al Nanostructured Coatings on a (100) Silicon Substrate" Nanomaterials 8, no. 11: 896. https://doi.org/10.3390/nano8110896
APA StyleAli, R., Renzelli, M., Khan, M. I., Sebastiani, M., & Bemporad, E. (2018). Effects of Residual Stress Distribution on Interfacial Adhesion of Magnetron Sputtered AlN and AlN/Al Nanostructured Coatings on a (100) Silicon Substrate. Nanomaterials, 8(11), 896. https://doi.org/10.3390/nano8110896






