Development of Diopside-Modified Marl-Based Dielectric Composite for Microelectronics Applications
Abstract
1. Introduction
2. Materials and Methods
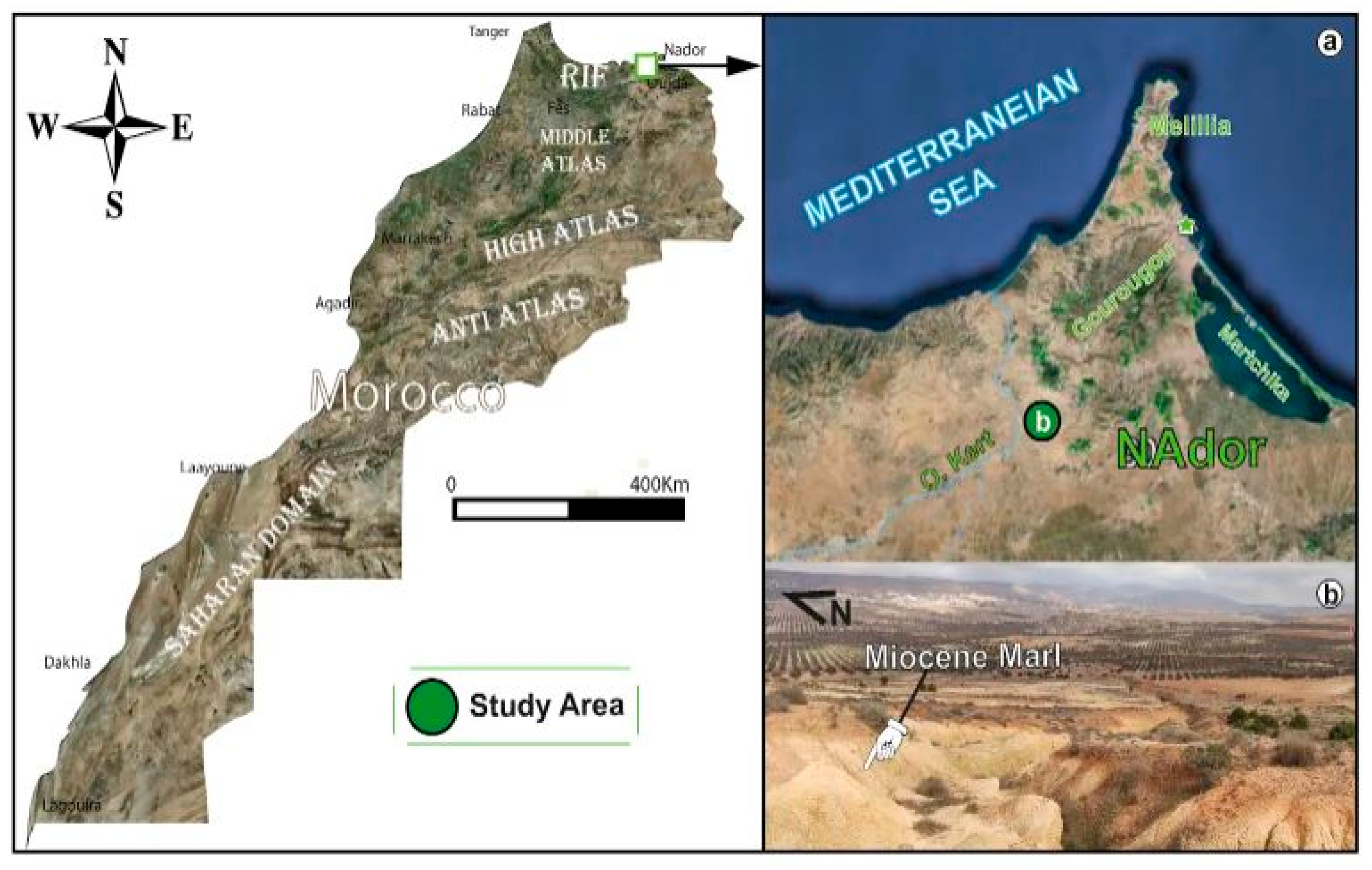
2.1. Types of Materials
2.2. Experimental Procedures
Procedures
2.3. Characterization Methods
3. Results and Discussions
3.1. Mineralogy and Granulometric Properties of Raw Marl
3.1.1. X-Ray Fluorescence
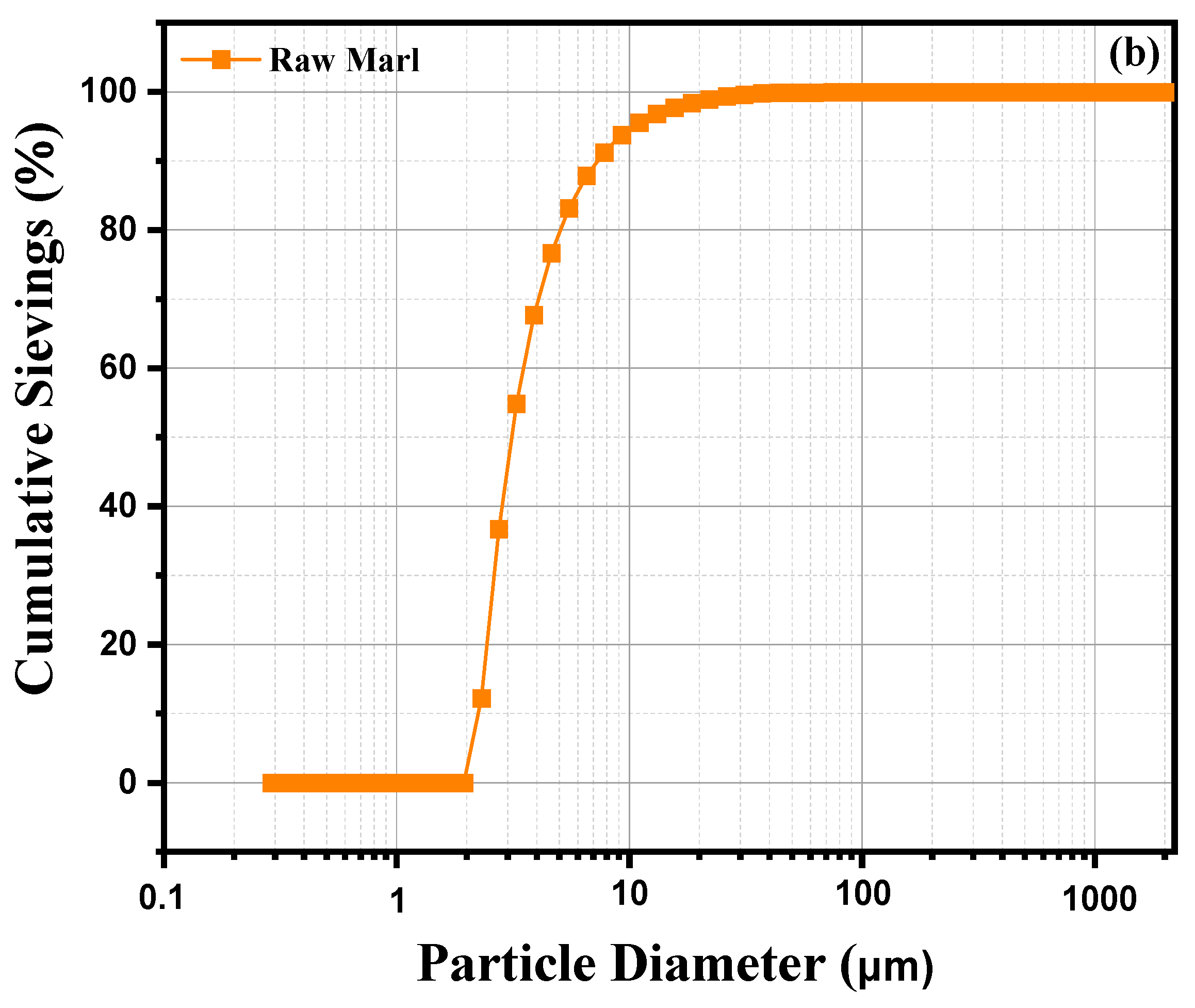
3.1.2. Laser Granulometry
3.2. Mineralogy and Microstructure Changes
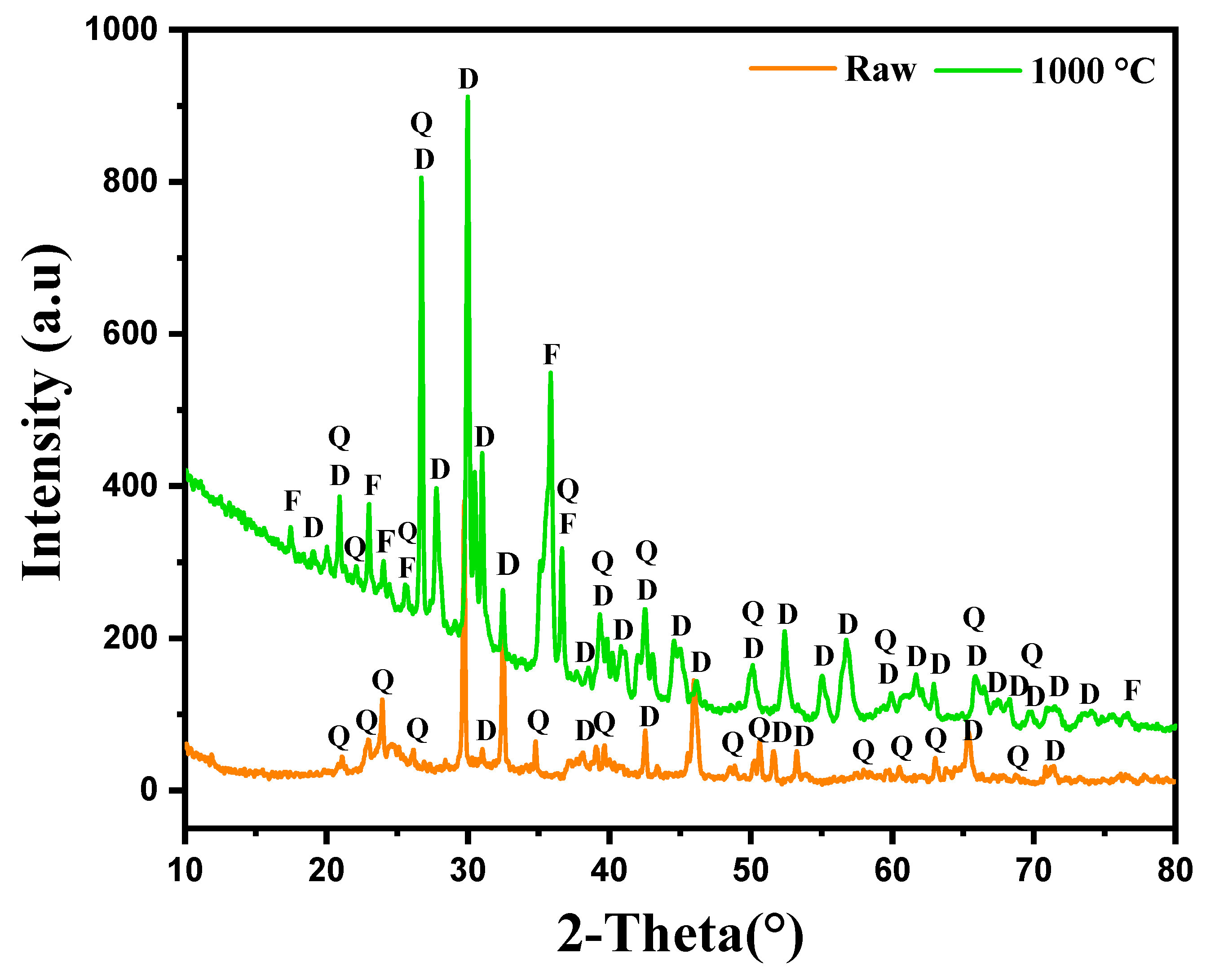
3.2.1. X-Ray Diffraction Analysis
3.2.2. IR Spectroscopy
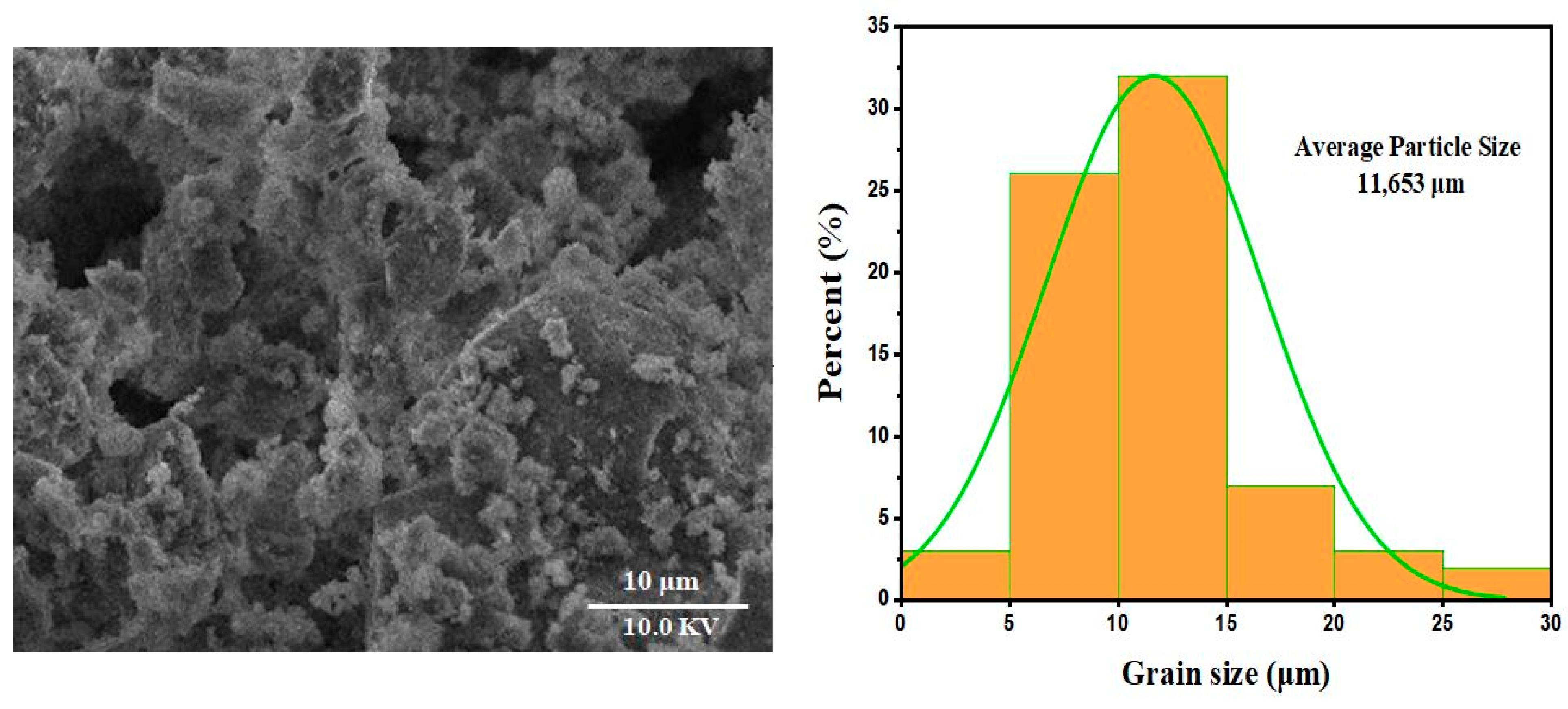
3.2.3. Scanning Electron Microscopy (SEM)
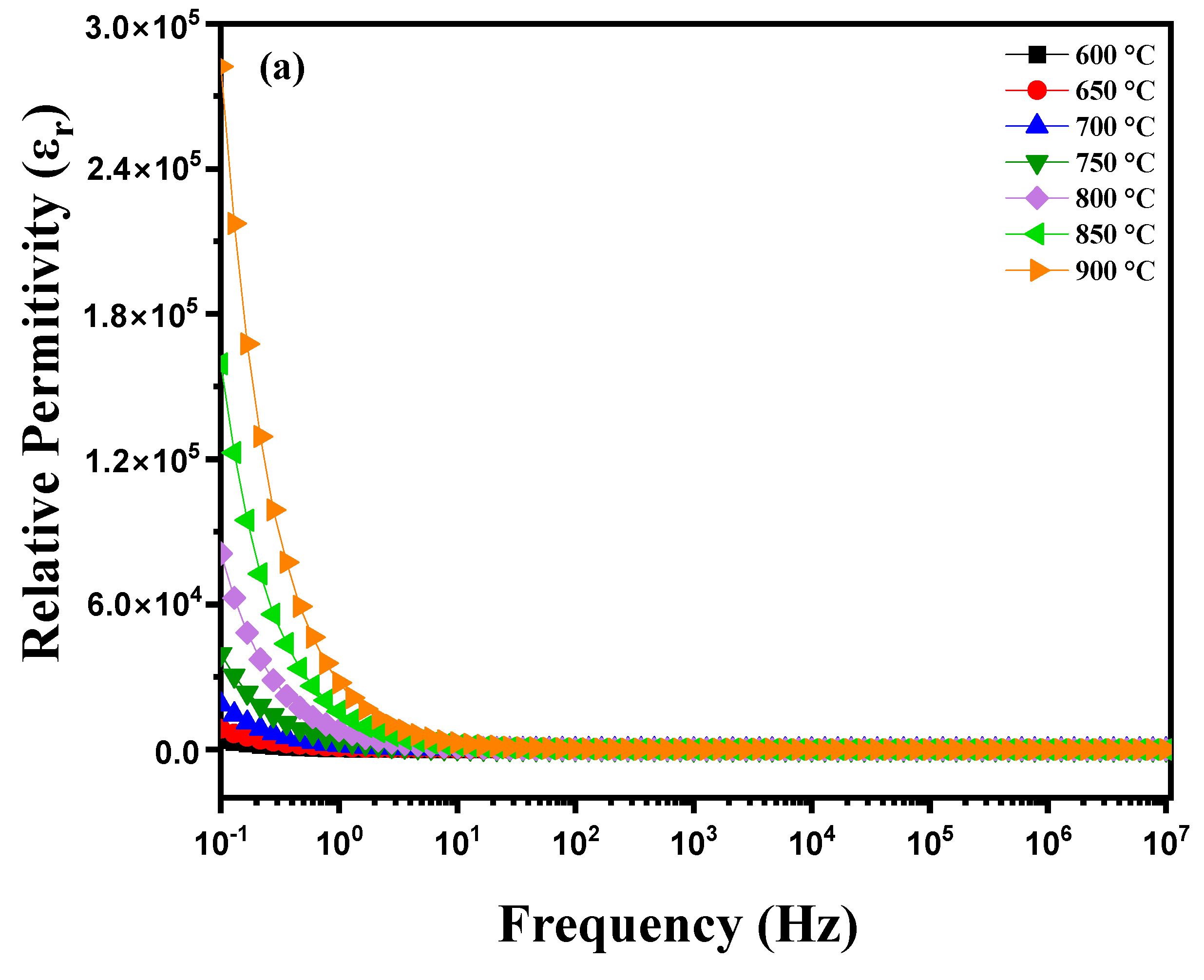
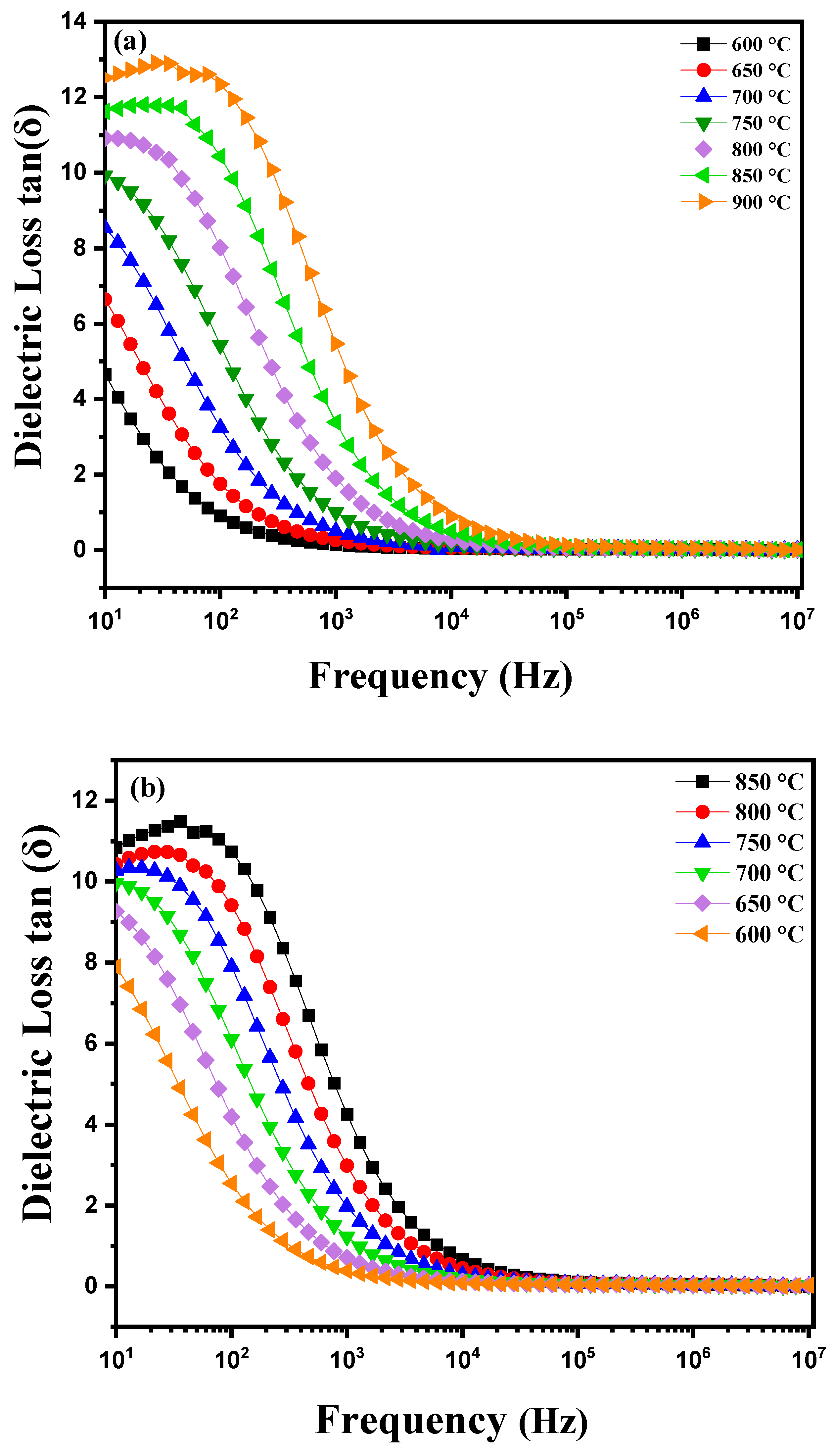
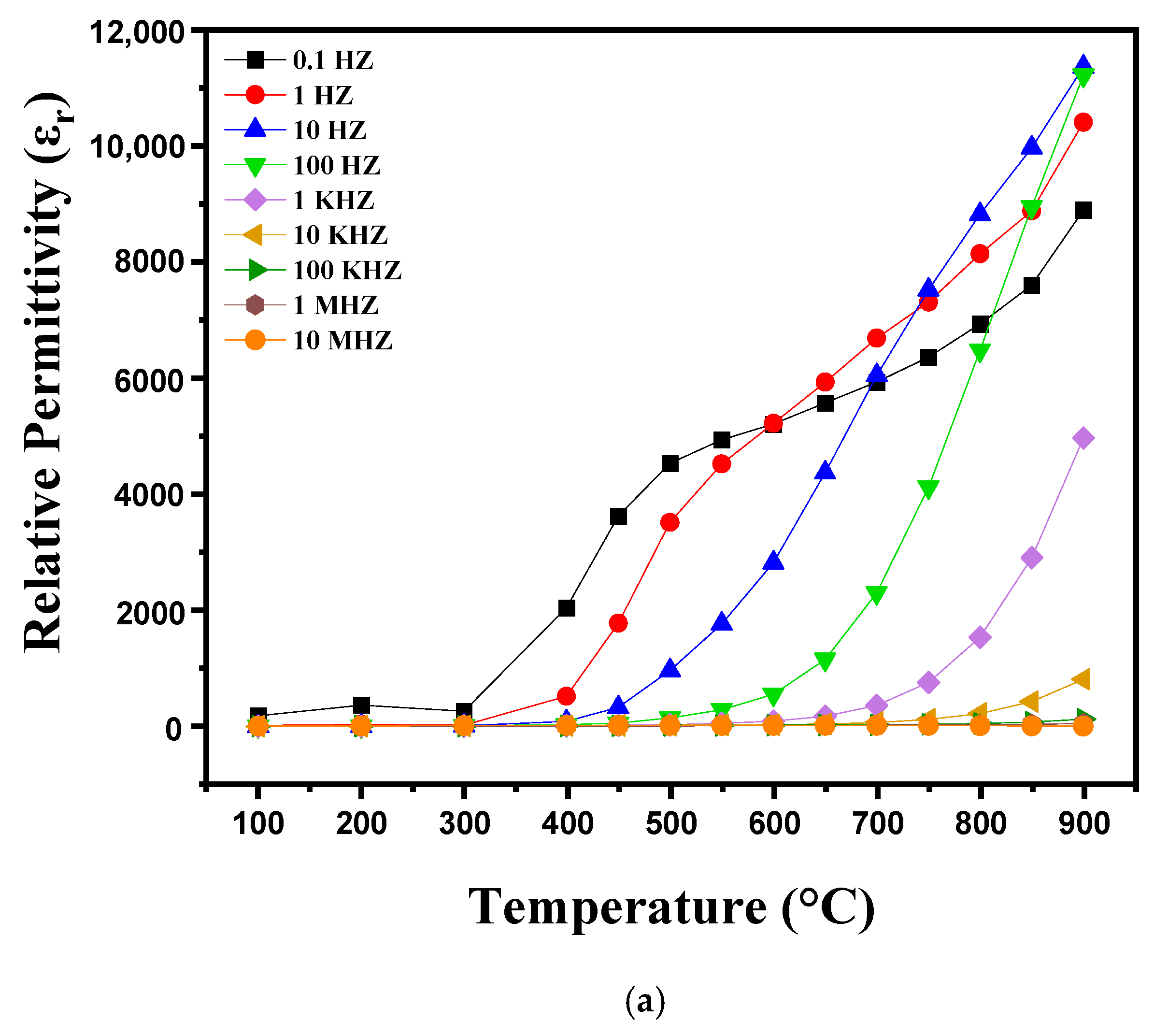
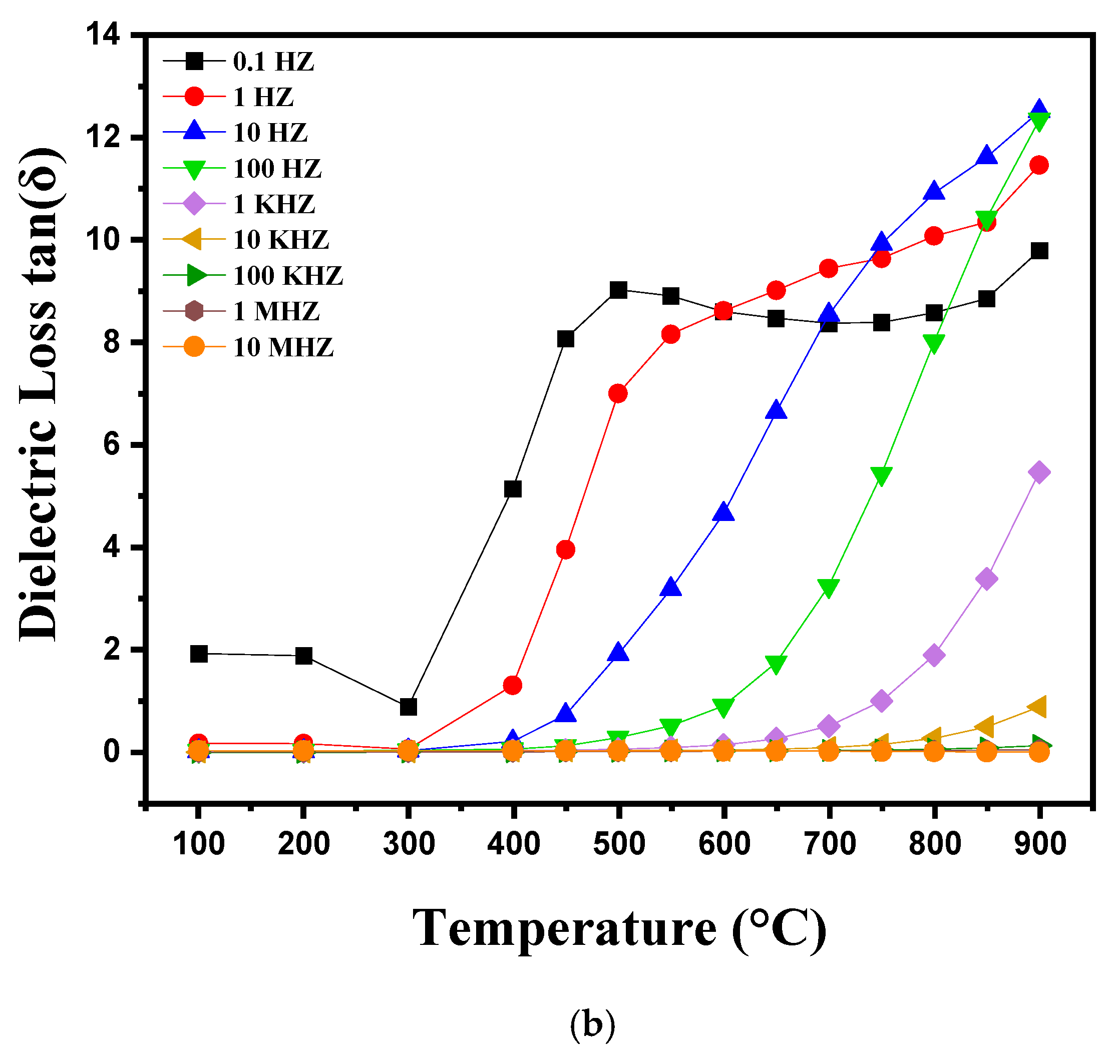
3.3. Dielectric Properties
3.3.1. Study of Dielectric Properties
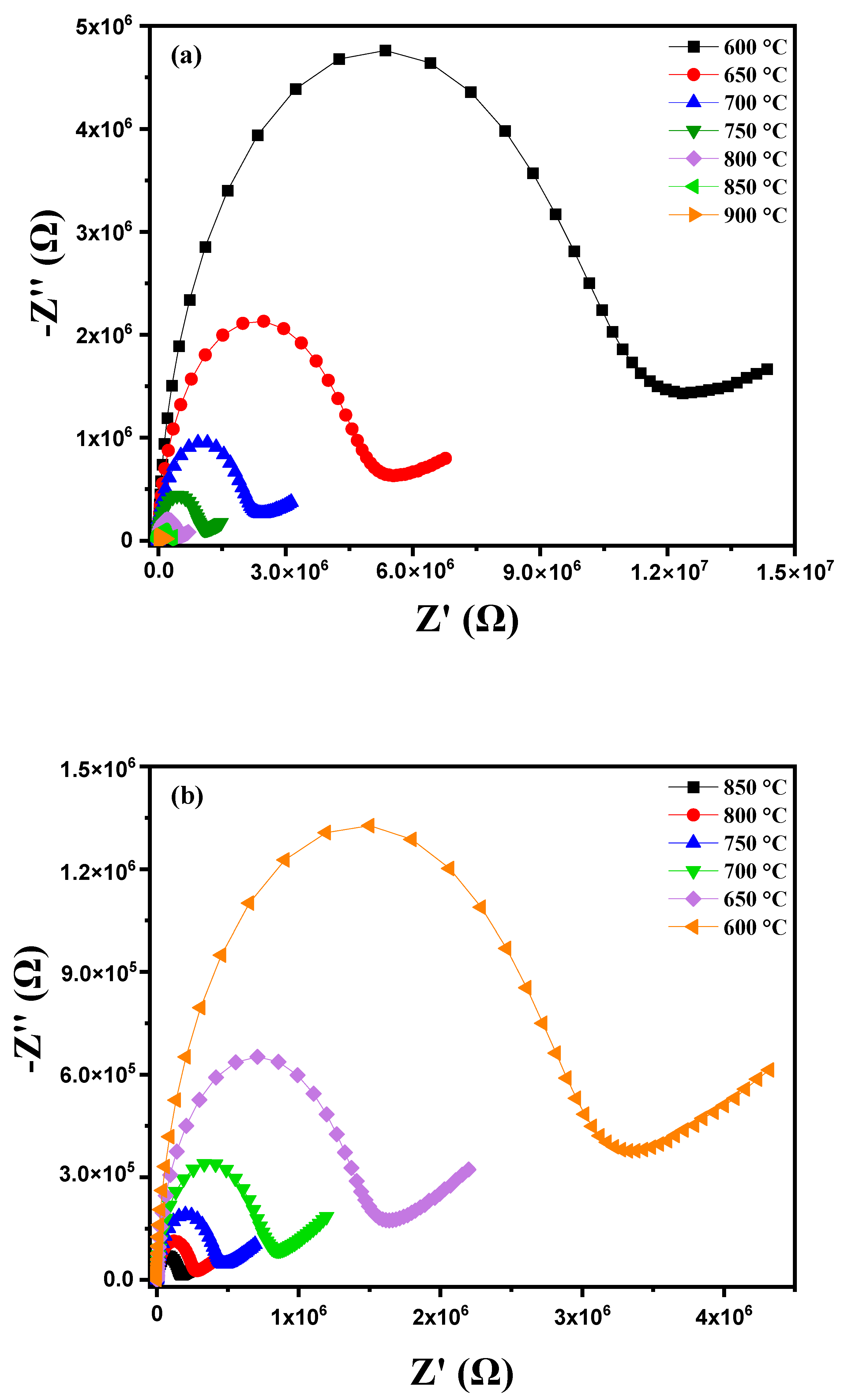
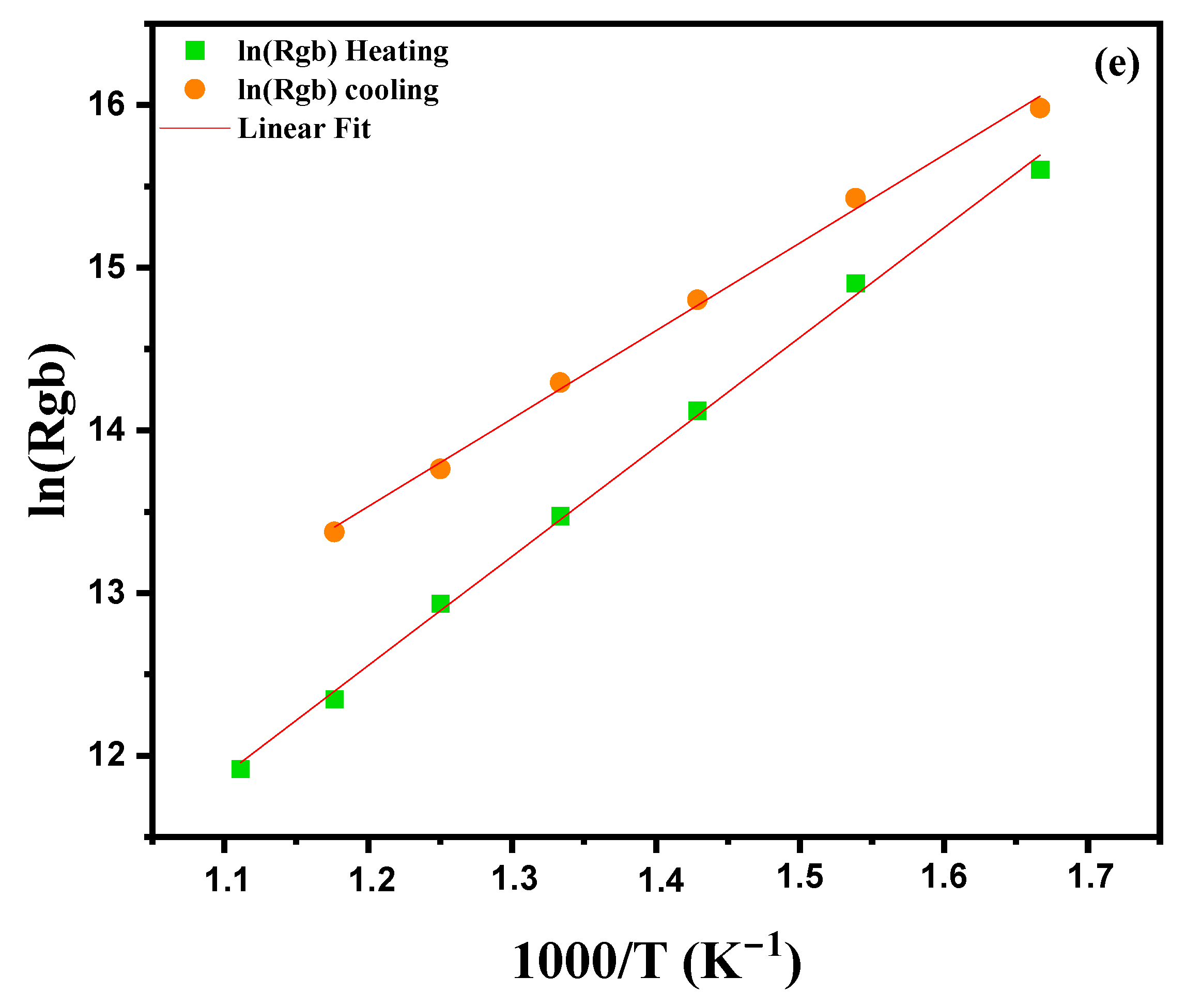
3.3.2. Complex Impedance Analysis (CIA)
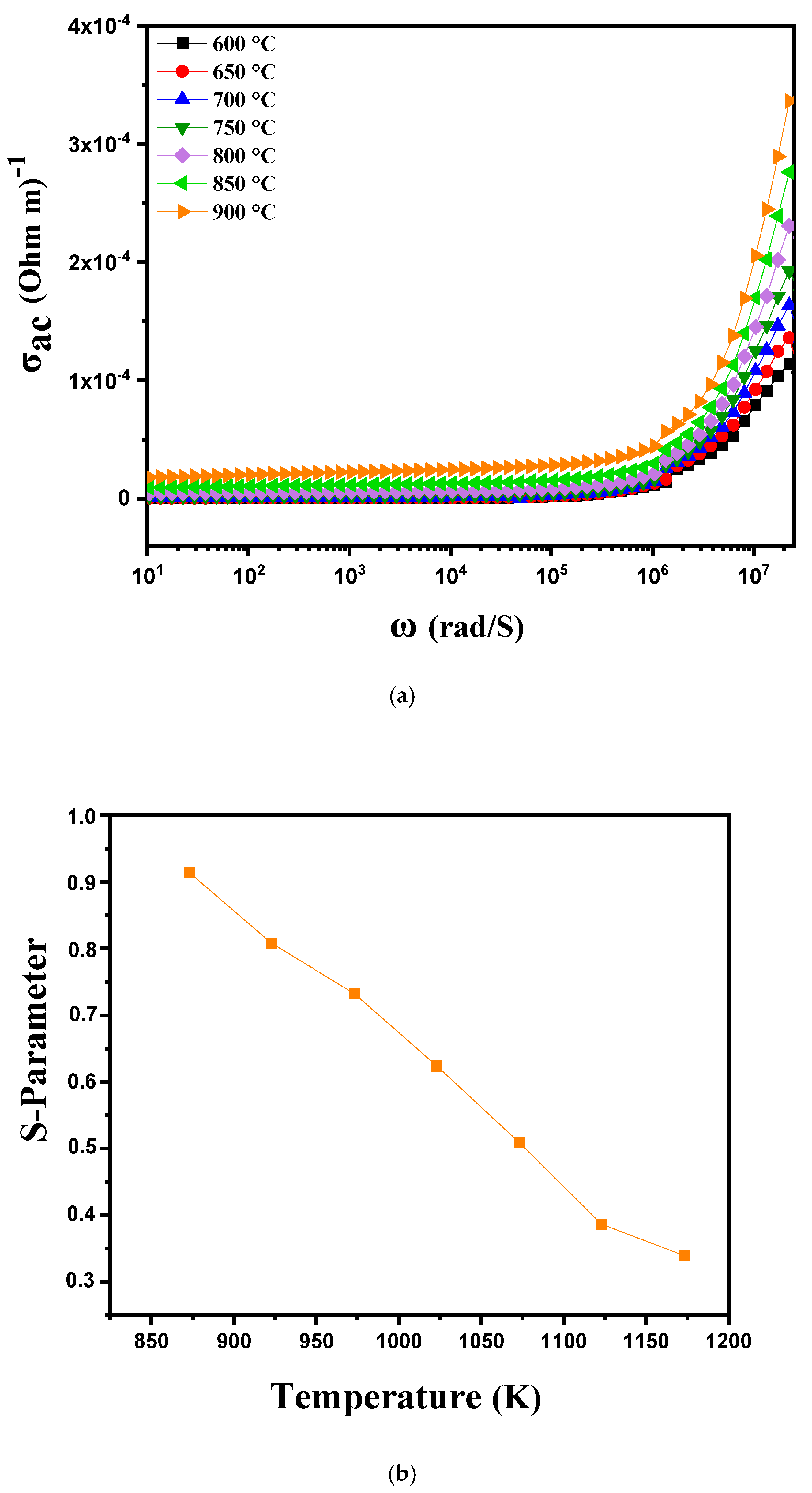
3.3.3. Conductivity Study
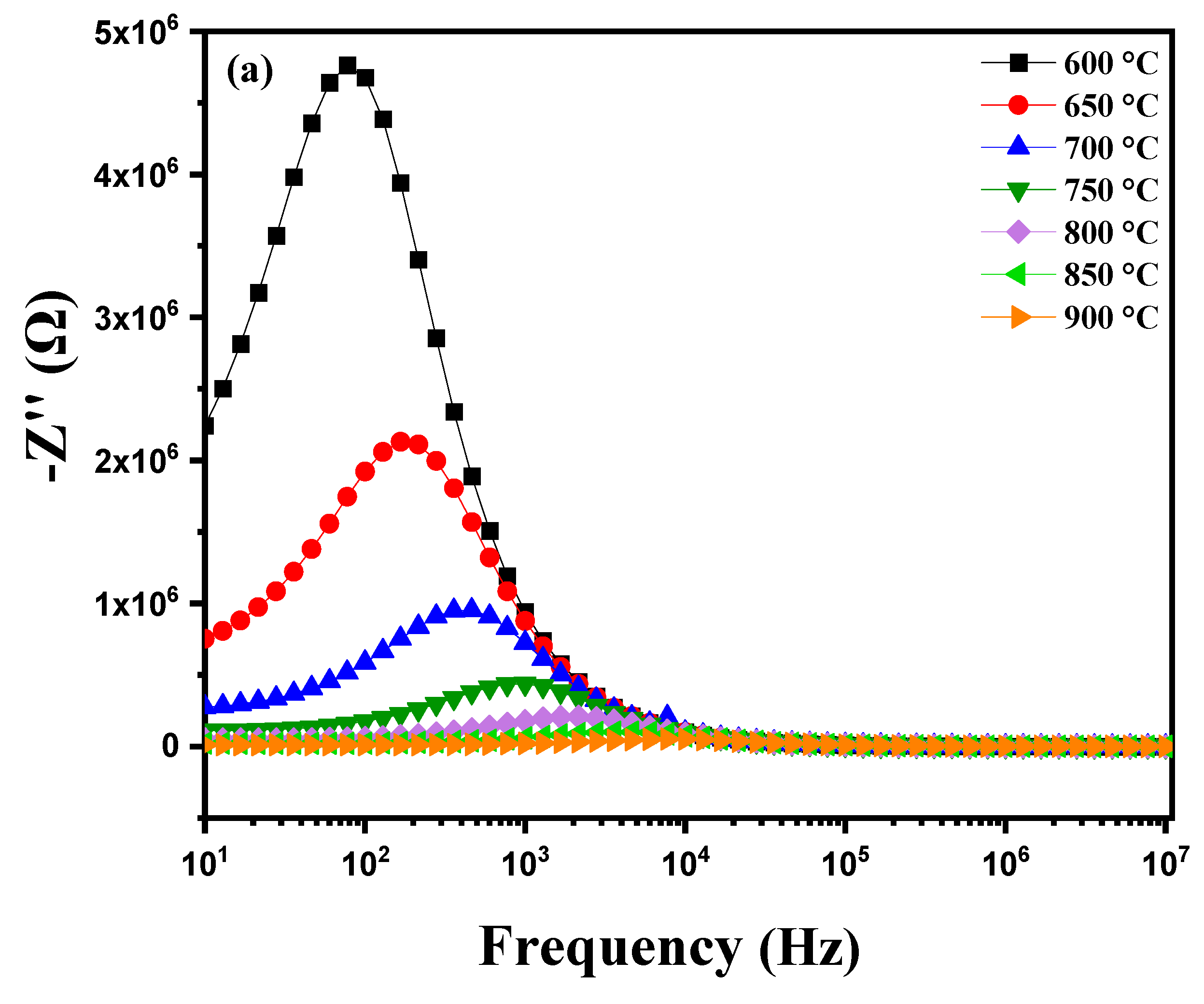
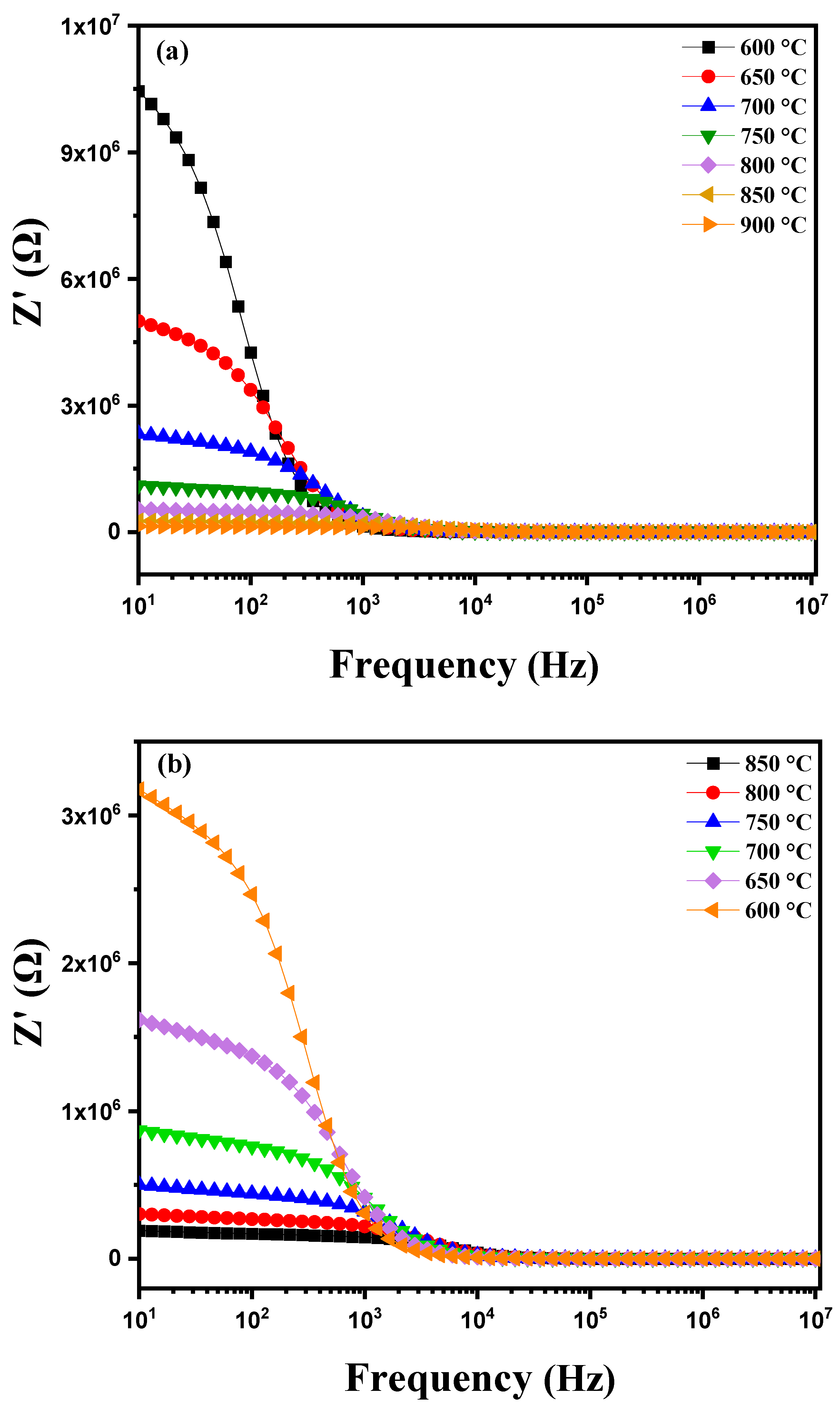
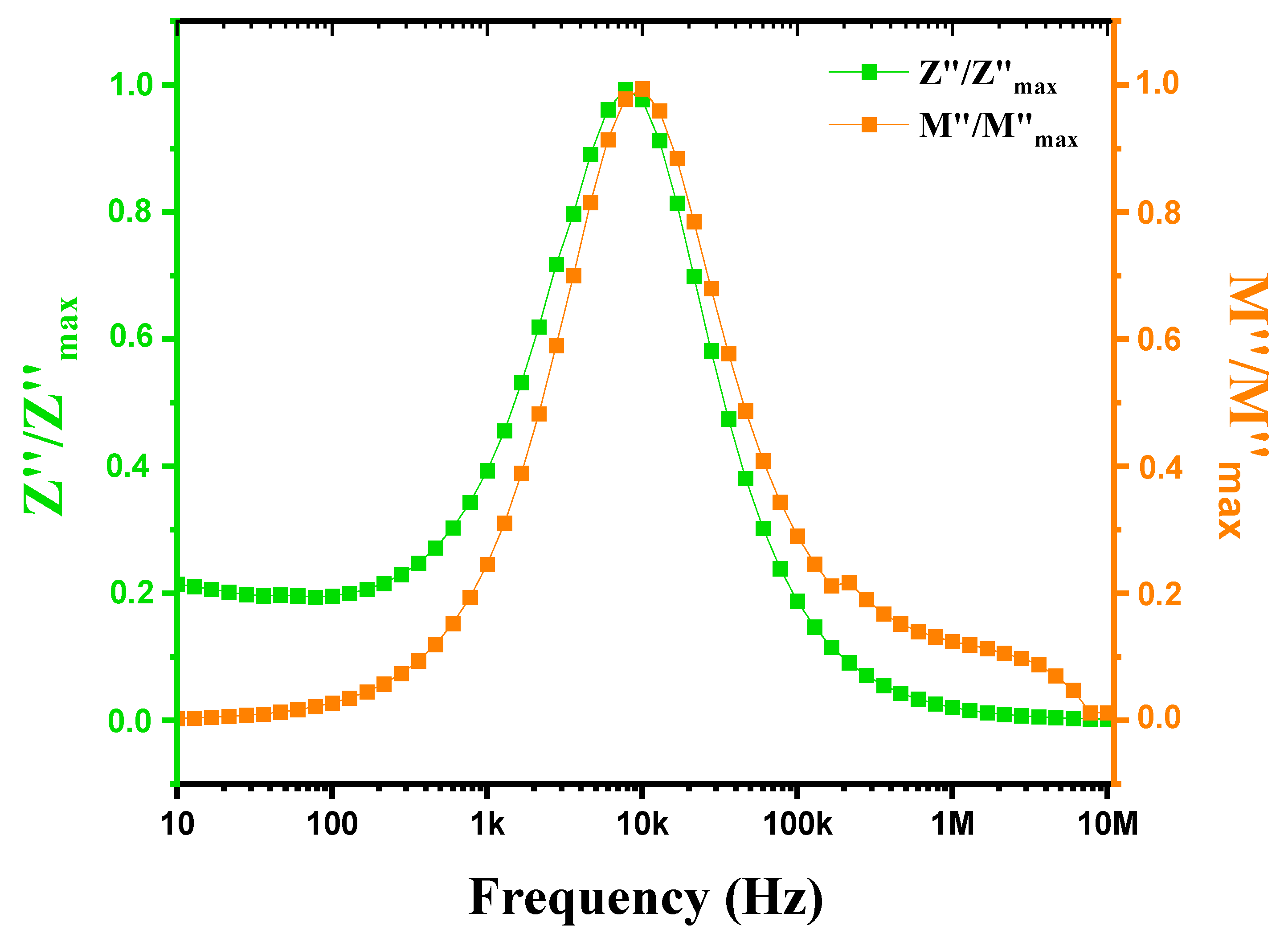
3.3.4. The Imaginary Spectrum and the Reality of Impedance
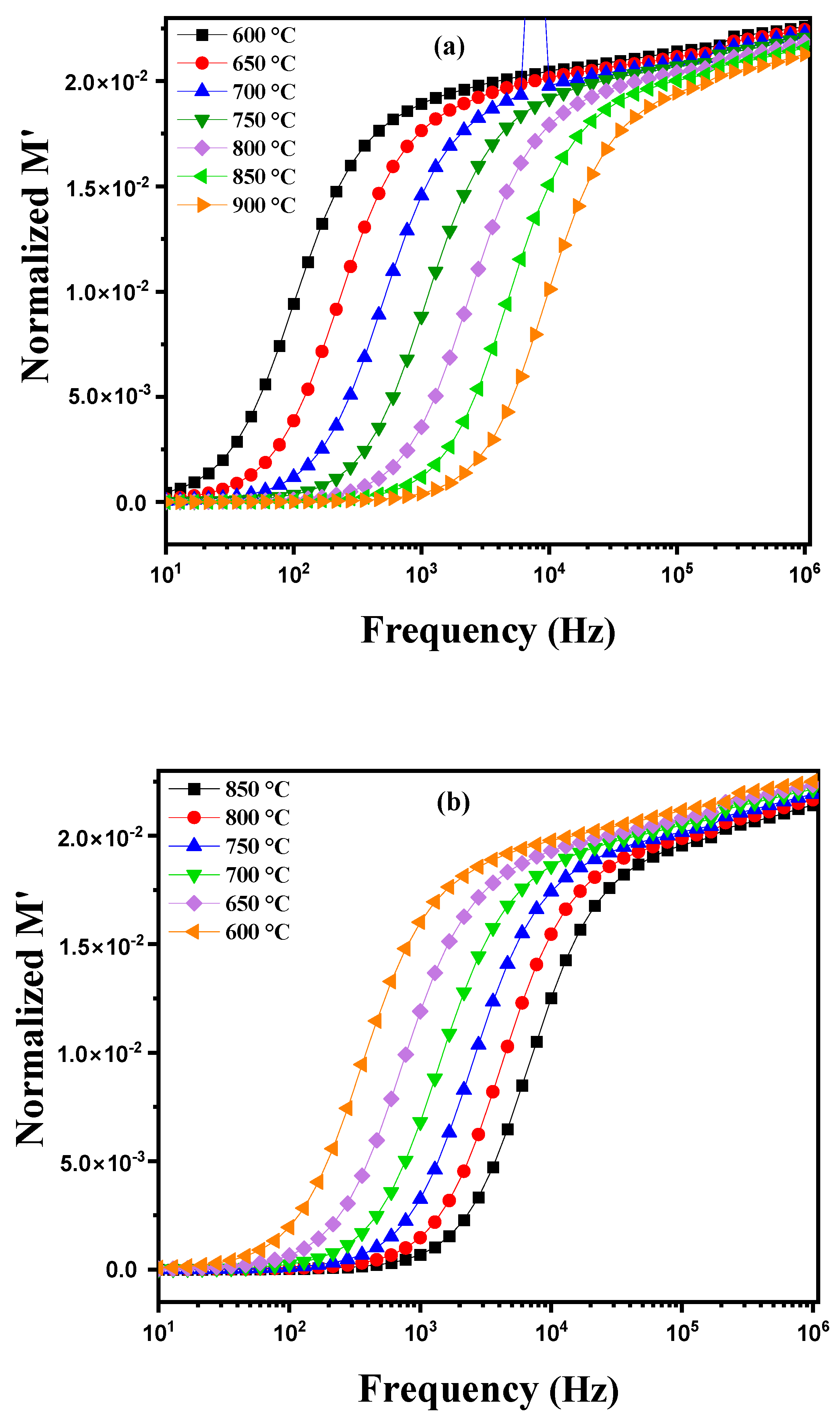
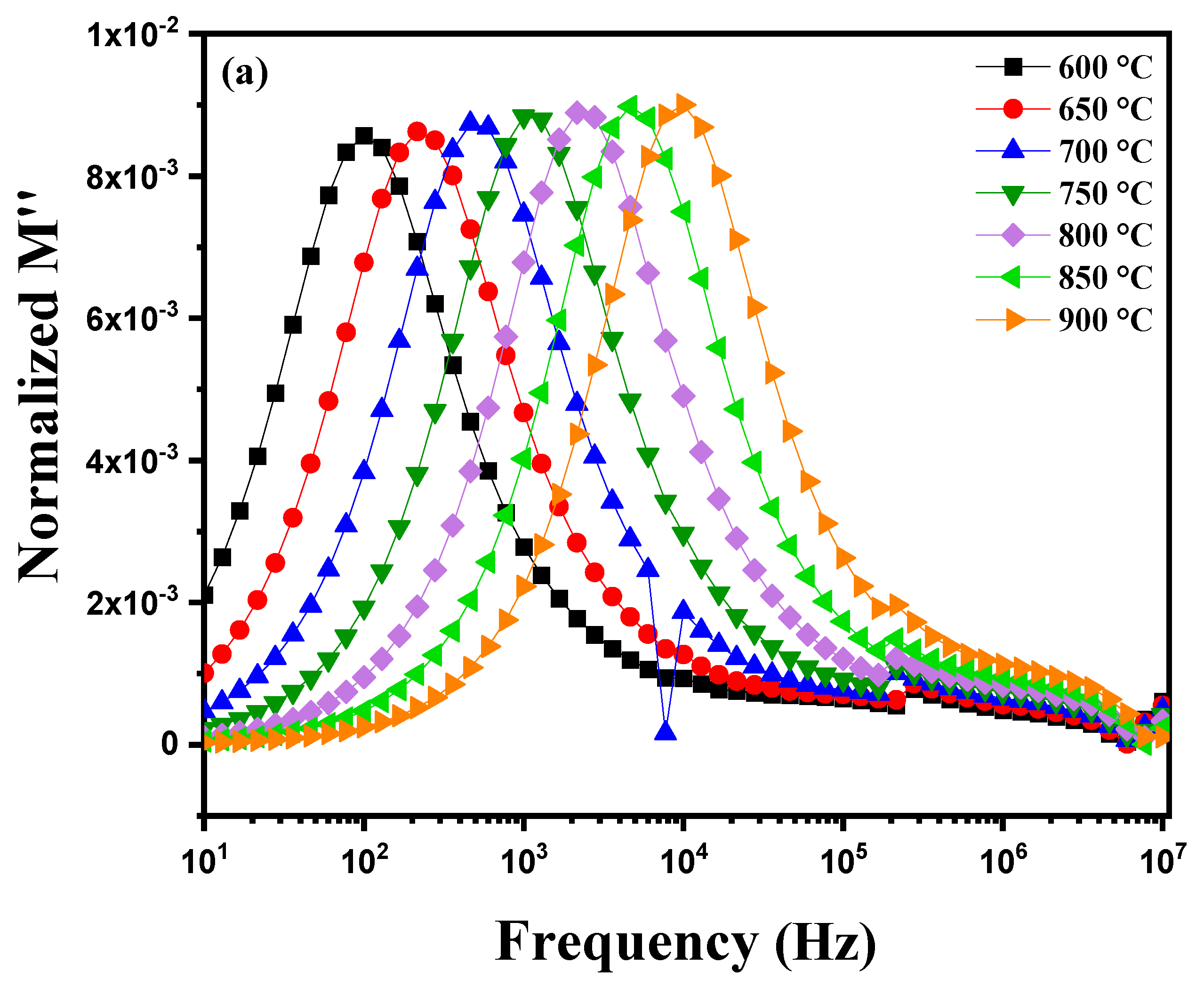
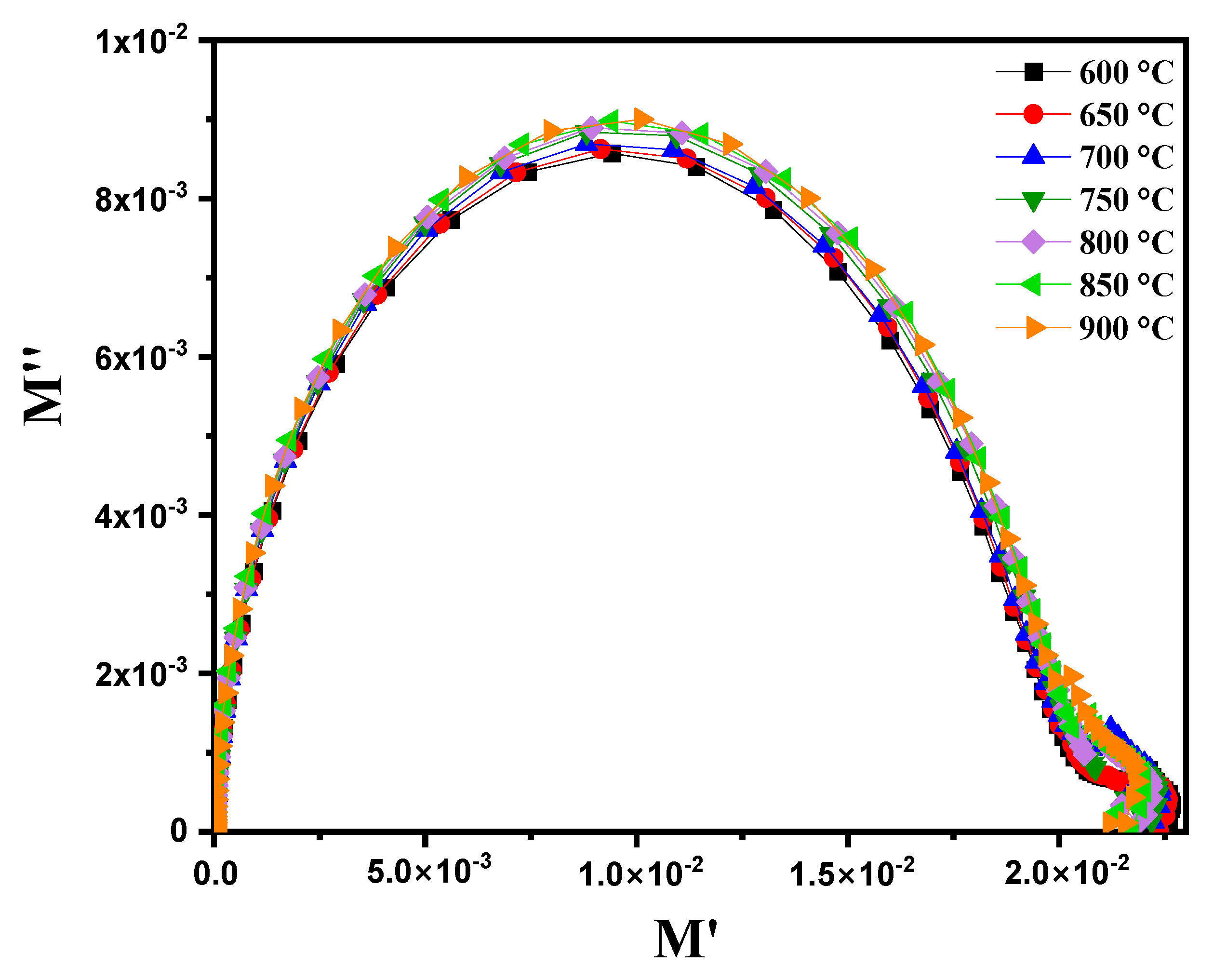
3.3.5. Modulus Spectroscopy Study
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abdelali, F.; Dine, S. L’effet de la Teneur en Fines du Sable Calcaire Concassé sur les Propriétés Mécaniques et la Durabilité du Mortier de Réparation Auto-Plaçant. Ph.D. Thesis, University of Ghardaïa, Ghardaia, Algeria, 2023. [Google Scholar]
- Djemal, R. Stabilisation d’un Sol Argileux Par un Nouveau Liant et une Fibre Naturelle; University of Guelma: Guelma, Algeria, 2024. [Google Scholar]
- Imane, Z.S. Contribution à L’étude d’un Enrichissement des Déchets Miniers: Cas des Stériles Francs du Gisement de Phosphate de Djebel Onk-Tébessa. Ph.D. Thesis, Université Badji Mokhtar–Annaba, Annaba, Algeria, 2023. [Google Scholar]
- Hamoudi, A. Étude du Comportement d’un Mélange de Marne et de Déchet de Laitier Cristallisé Dans les Corps de Chaussées. Ph.D. Thesis, Université Mouloud Mammeri TiziOuzou, Tizi Ouzou, Algeria, 2018. [Google Scholar]
- Zouai, K. Etude du Mode de Fabrication du Ciment et Effet des Matières Premières: Impact de L’utilisation du Ciment sur L’environnement. Ph.D. Thesis, Abdelkader BOUGARA; Université Hassiba Benbouali de Chlef, Ouled Fares, Algeria, 2011. [Google Scholar]
- Chanez, S. Valorisation des Débris de Bétons et de Briques en Construction Routière. Ph.D. Thesis, Université Mouloud Mammeri, Tizi Ouzou, Algeria, 2024. [Google Scholar]
- Julien, J.L.; Bourrié, G.; Bruand, A.; Feller, C.; Morlon, P.; van Oort, F.; Tessier, D. Histoire de trois concepts du sol mal maîtrisés: Le pH du sol, les cations échangeables et la capacité d’échange cationique. Étude Et Gest. Des Sols 2023, 30, 365–381. [Google Scholar]
- Martine, R.; Guerra, M.-F. Physico-Chimie des Matériaux Archéologiques et Culturels; Archives Contemporaines, Ed.; CEPAM: Nice, France, 2016. [Google Scholar]
- Suman, N.; Fortunato, E.; Martins, R. Green economy and waste management: An inevitable plan for materials science. Prog. Nat. Sci. Mater. Int. 2022, 32, 1–9. [Google Scholar]
- Ava, S.; Salimi, E. Low temperature preparation of diopside nanoparticles: In-vitro bioactivity and drug loading evaluation. Sci. Rep. 2023, 13, 16330. [Google Scholar]
- Benjamin, C. Etude Fondamentale de la Dissolution des Silicates Amorphes et Cristallins: Apport à la Robustesse des Modèles. Ph.D. Thesis, Université Grenoble Alpes, Grenoble, France, 2024. [Google Scholar]
- Diallo, B. Structural Study of Phase Separation Phenomena in Aluminosilicate and Borosilicate Oxide Glasses: Application to the Synthesis of Glass-Ceramics. Doctoral Thesis, Université d’Orléans, Orléans, France, 2018. [Google Scholar]
- Sherlin, J.; Swamiappan, S. Preparation and Characterization of Diopside-Wollastonite Composite for Orthopedic Application. Silicon 2024, 16, 1161–1171. [Google Scholar]
- Carlos, E.; Branquinho, R.; Kiazadeh, A.; Martins, J.; Barquinha, P.; Martins, R.; Fortunato, E. Boosting electrical performance of high-κ nanomultilayer dielectrics and electronic devices by combining solution combustion synthesis and UV irradiation. ACS Appl. Mater. Interfaces 2017, 9, 40428–40437. [Google Scholar] [CrossRef]
- Barquinha, P.; Pereira, L.; Gonçalves, G.; Martins, R.; Fortunato, E.; Kuscer, D.; Kosec, M.; Vilà, A.; Olziersky, A.; Morante, J.R. Low-temperature sputtered mixtures of high-κ and high bandgap dielectrics for GIZO TFTs. J. Soc. Inf. Disp. 2010, 18, 762–772. [Google Scholar] [CrossRef]
- Paramarta, V.; Taufik, A.; Salah, R. Better adsorption capacity of SnO2 nanoparticles with different graphene addition. J. Phys. Conf. Ser. 2016, 776, 012039. [Google Scholar] [CrossRef]
- Anfosso, M.; Gaggero, L.; Manfrinetti, P.; Vicini, S. Experimental protocols for the consolidation of synthetic carbonate rocks using diammonium phosphate (DAP). SSRN 2023, 5002591. [Google Scholar] [CrossRef]
- Ahlawat, R. Influence of multi-step annealing on the nanostructure and surface morphology of Y2O3:SiO2 powder. Ceram. Int. 2015, 41, 7345–7351. [Google Scholar] [CrossRef]
- Ahlawat, R. Preparation and effect of heat treatment on the Gd2O3:SiO2 nanocomposite. Mod. Phys. Lett. B 2015, 29, 1550046. [Google Scholar] [CrossRef]
- Goswami, B.; Rani, N.; Ahlawat, R. Structural and optical studies of Nd3⁺-doped Y2O3-SiO2 nanopowder. J. Alloy. Compd. 2018, 730, 450–457. [Google Scholar] [CrossRef]
- Bellucci, L.; Cassetta, M.; Skogby, H.; Nazzareni, S. Vibrational spectra of pure and Sc-doped diopside (CaMgSi2O6): Modeling and experiments. Phys. Chem. Chem. Phys. 2024, 26, 4029–4038. [Google Scholar] [CrossRef] [PubMed]
- Jiang, H.; Yuan, H.; Yu, Q.; Xie, J. The effect of SiO2 particle size on the crystallization behavior and space charge properties of SiO2/MMT/LDPE composites. Materials 2024, 17, 1605. [Google Scholar] [CrossRef]
- Joshi, J.; Kanchan, D.; Joshi, M.; Jethva, H.; Parikh, K. Spectroscopic studies of dielectric relaxation, complex impedance, and modulus of mixed-phase rod-shaped cobalt sulfide nanoparticles. Mater. Res. Bull. 2017, 93, 63–73. [Google Scholar] [CrossRef]
- Nofal, M.M.; Aziz, S.B.; Ghareeb, H.O.; Hadi, J.M.; Dannoun, E.M.A.; Al-Saeedi, S.I. Impedance and dielectric properties of PVC:NH4I solid polymer electrolytes (SPEs): Steps toward the fabrication of SPEs with high resistivity. Materials 2022, 15, 2143. [Google Scholar] [CrossRef]
- Mhamdi, A.; Mimouni, R.; Amlouk, A.; Amlouk, M.; Belgacem, S. Study of the effects of copper doping on the structural, optical and electrical properties of sputtered ZnO thin films. J. Alloy. Compd. 2014, 610, 250–257. [Google Scholar] [CrossRef]
- Udupa, K.S.; Rao, P.M.; Aithal, S.; Bhat, A.; Avasthi, D. Effect of heavy ion irradiation on dielectric constant and electrical conductivity of doped and undoped nonlinear substances. Bull. Mater. Sci. 1997, 20, 1069–1077. [Google Scholar] [CrossRef]
- Bochenek, D.; Niemiec, P.; Brzezińska, D.; Dercz, G.; Ziółkowski, G.; Jartych, E.; Grotel, J.; Suchanicz, J. Magnetoelectric properties of multiferroic composites based on BaTiO3 and nickel-zinc ferrite. Materials 2024, 17, 1905. [Google Scholar] [CrossRef]
- Arya, B.; Choudhary, R. Structural, dielectric and electrical properties of BaSnO3 and BaSeO3 modified Bi0.5Na0.5TiO3 ceramics. Ceram. Int. 2020, 46, 4222–4234. [Google Scholar] [CrossRef]
- Zhang, H.; Ye, X.; Xu, J.; Li, S.; Ma, X.; Xu, W.; Zhang, J. Effects of heat treatment on the electromagnetic wave absorption characteristics of ceramic particles resorcinol formaldehyde silicon dioxide. Materials 2024, 17, 2376. [Google Scholar] [CrossRef]
- Ashokkumar, M.; Muthukumaran, S. Electrical, dielectric, photoluminescent and magnetic properties of ZnO nanoparticles co-doped with Co and Cu. J. Magn. Magn. Mater. 2015, 374, 61–66. [Google Scholar] [CrossRef]
- Kashif, I.; Rahman, S.A.; Abdelghany, A.; El-Said, R. Dielectric behavior and PTCR effect in nanocrystallite PMN ferroelectric ceramics. Philos. Mag. A 2010, 90, 2115–2123. [Google Scholar] [CrossRef]
- Kinoshita, K.; Yamaji, A. Grainsize effects on dielectric properties in barium titanate ceramics. J. Appl. Phys. 1976, 47, 371–373. [Google Scholar] [CrossRef]
- Li, W.; Ma, Z.; Gao, L.; Wang, F. Preparation and electrical properties of La0.90Sr0.10TiO3+δ. Materials 2015, 8, 1176–1186. [Google Scholar] [CrossRef] [PubMed]
- Jakobsen, B.; Maggi, C.; Christensen, T.; Dyre, J.C. Study of mechanical shear and dielectric relaxation processes in two monoalcohols near the glass transition. J. Chem. Phys. 2008, 129, 184502. [Google Scholar] [CrossRef]
- Javed, M.; Khan, A.A.; Khisro, S.N.; Majeed, A.; Kazmi, J.; Bilkees, R.; Hussain, M.; Mohamed, M.A. Charge conduction mechanism and non-Debye type relaxation in the perovskite orthochromite LaCrO3. Mater. Chem. Phys. 2022, 290, 126522. [Google Scholar] [CrossRef]
- Almeida, G.N.; de Souza, R.N.; Lima, L.F.S.; Mohallem, N.D.S.; da Silva, E.P.; Silva, A.M.A. The influence of the synthesis method on the characteristics of BaTiO3. Materials 2023, 16, 3031. [Google Scholar] [CrossRef]
- Bendahhou, A.; Chourti, K.; Chaou, F.; Jalafi, I.; El Barkany, S.; Bahari, Z.; Abou-Salama, M. Structural, electrical, and dielectric study of the influence of 3.4% lanthanides (Ln3⁺ = Sm3⁺ and La3⁺) insertion at the A-site of the Ba0.95Ln0.034Ti0.99Zr0.01O3 perovskite. RSC Adv. 2022, 12, 33124–33141. [Google Scholar]
- Jebli, M.; Rayssi, C.; Dhahri, J.; Henda, M.B.; Belmabrouk, H.; Bajahzar, A. Structural and morphological studies, and temperature/frequency dependence of the electrical conductivity of Ba0.97La0.02Ti1−xNb4x/5O3 perovskite ceramics. RSC Adv. 2021, 11, 23664–23678. [Google Scholar] [CrossRef]
- Abouzari, M.S.; Berkemeier, F.; Schmitz, G.; Wilmer, D. Physical interpretation of constant phase element components. Solid State Ionics 2009, 180, 922–927. [Google Scholar] [CrossRef]
- Ali, H.; Karim, S.; Rafiq, M.; Maaz, K.; Rahman, A.U.; Nisar, A.; Ahmad, M. Electrical conduction mechanism in ZnS nanoparticles. J. Alloys Compd. 2014, 612, 64–68. [Google Scholar] [CrossRef]
- Chaari, M.; Belgacem, R.B.; Matoussi, A. Impedance analysis, dielectric relaxation, and modulus behavior of ZnO–Sn2O3 ceramics. J. Alloys Compd. 2017, 726, 49–56. [Google Scholar] [CrossRef]
- Jonscher, A.K. The “universal” dielectric response. Nature 1977, 267, 673–679. [Google Scholar] [CrossRef]
- Pradhan, D.K.; Behera, B.; Das, P.R. Studies on dielectric and electrical properties of a new type of electroactive ceramics with complex tungsten bronze structure. J. Mater. Sci. Mater. Electron. 2012, 23, 779–785. [Google Scholar] [CrossRef]
- Duta, L.; Grumezescu, V. Effect of doping on the electrical and dielectric properties of hydroxyapatite for medical applications: From powders to thin films. Materials 2024, 17, 640. [Google Scholar] [CrossRef]
- Funke, K. Jump relaxation in solid ionic conductors. Solid State Ionics 1988, 28, 100–107. [Google Scholar] [CrossRef]
- Elliott, S. Alternating current conduction in amorphous semiconductors based on chalcogenides and pnictures. Adv. Phys. 1987, 36, 135–217. [Google Scholar] [CrossRef]
- Shah, S.S.; Hayat, K.; Ali, S.; Rasool, K.; Iqbal, Y. Conduction mechanisms in lanthanum manganite nanofibers. Mater. Sci. Semicond. Process. 2019, 90, 65–71. [Google Scholar] [CrossRef]
- Guettiti, K.; Sekrafi, H.E.; Azizi, S.; Bouzidi, C.; Bouguila, N.; Khirouni, K.; Lamloumi, J. Study of the structural, dielectric, impedance and modulus properties of La0.8Pb0.2FeO3 doped with 25% Mg in site B. Phys. Condens. Matter 2023, 655, 414771. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, J.; Gharbi, O.; Vivier, V.; Gao, M.; Orazem, M.E. Electrochemical Impedance Spectroscopy. Nat. Methods Primers 2021, 1, 41. [Google Scholar] [CrossRef]
- Nassar, K.I.; Benamara, M.; Kechiche, L.; Teixeira, S.S.; Graça, M.P.F. Structural and electrical properties of bismuth and niobium doped LaNiO3 perovskite obtained by sol-gel route for future applications in electronic devices. Indian J. Phys. 2024, 98, 2745–2753. [Google Scholar] [CrossRef]
- Okazaki, Y. Oxide film characterization of implantable metals by electrochemical impedance spectroscopy. Materials 2019, 12, 3466. [Google Scholar] [CrossRef] [PubMed]
- Hosseini, S.; Ranjbar, K.; Dehmolaei, R.; Amirani, A. Fabrication of Al5083 surface composites reinforced with carbon nanotubes and cerium oxide nanoparticles by friction stir processing. J. Alloys Compd. 2015, 622, 725–733. [Google Scholar] [CrossRef]
- Badapanda, T.; Senthil, V.; Rout, S.K.; Cavalcante, L.S.; Simões, A.Z.; Sinha, T.P.; Panigrahi, S. Rietveld refinement, microstructure, conductivity and impedance properties of Ba[Zr0.25Ti0.75]O3 ceramic. Curr. Appl. Phys. 2011, 11, 1282–1293. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and absorption in dielectrics I. Characteristics of alternating current. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- Benamara, M.; Zahmouli, N.; Teixeira, S.S.; Graça, M.P.F.; Mir, L.E.; Valente, M.A. Electrical and magnetic studies of maghemite (γ-Fe2O3) prepared by sol-gel method. J. Electron. Mater. 2022, 51, 2698–2707. [Google Scholar] [CrossRef]
- Sambasiva Rao, K.; Murali Krishna, P.; Madhava Prasad, D. Effect of simultaneous substitution of Li⁺ and Ti4⁺ in Pb2KNb5O15 ceramics on structural, dielectric, modulus, impedance and conductivity properties. Phys. Status Solidi 2007, 244, 2267–2287. [Google Scholar] [CrossRef]
- Padmasree, K.; Kanchan, D.; Kulkarni, A. Impedance and modulus studies of solid electrolyte system 20CdI2–80[xAg2O–y(0.7V2O5–0.3B2O3)], where 1≤x/y≤3. Solid State Ion. 2006, 177, 475–482. [Google Scholar] [CrossRef]
- Chowdari, B.; Gopalakrishnan, R. AC conductivity analysis of vitreous silver iodomolybdate system. Solid State Ion. 1987, 23, 225–233. [Google Scholar] [CrossRef]
- Arora, M.; Arora, V.; Kaur, S.; Kaur, J.; Kumar, S.; Singh, A. Proof of non-Debye behavior of Pb0.76Sm0.24Ti0.76Fe0.24O3 ceramics by complex impedance spectroscopy. Mater. Today Proc. 2023, 80, 1079–1085. [Google Scholar] [CrossRef]
- Benyoussef, M.; Zannen, M.; Belhadi, J.; Manoun, B.; Dellis, J.-L.; Lahmar, A.; El Marssi, M. Complex impedance and Raman spectroscopy of Na0.5(Bi1−xDyx)0.5TiO3 ceramics. Ceram. Int. 2020, 46, 10979–10991. [Google Scholar] [CrossRef]
- Omari, L.H.; Moubah, R.; Boutahar, A.; Hajji, L.; El Ouatib, R. Analysis of electrical properties by complex impedance spectroscopy in solid solutions (PbTiO3)0.97–(LaFeO3)0.03 prepared by sol-gel technique. J. Electroceram. 2020, 44, 23–31. [Google Scholar] [CrossRef]
- Hossen, M.B.; Hossain, A.K.M.A. Complex impedance and electrical module studies of magnetic ceramic Ni0.27Cu0.10Zn0.63Fe2O4. J. Adv. Ceram. 2015, 4, 217–225. [Google Scholar] [CrossRef]





| Element/Oxide | Element [%] | Oxide [%] |
|---|---|---|
| Na/Na2O | 3.64 | 2.78 |
| Mg/MgO | 1.88 | 1.76 |
| Al/Al2O3 | 10.96 | 12.54 |
| Si/SiO2 | 59.74 | 68.12 |
| P/P2O5 | 0.148 | 0.169 |
| S/SO3 | 0.0755 | 0.0928 |
| K/K2O | 3.18 | 1.85 |
| Ca/CaO | 7.67 | 5.1 |
| Ti/TiO2 | 0.754 | 0.584 |
| V/V2O5 | 0.0442 | 0.0367 |
| Cr/Cr2O3 | 0.0132 | 0.0088 |
| Mn/MnO | 0.0735 | 0.0433 |
| Fe/Fe2O3 | 6.98 | 4.54 |
| Zn/ZnO | 0.0233 | 0.0126 |
| As/As2O3 | 0.296 | 0.149 |
| Rb/Rb2O | 0.027 | 0.0168 |
| Sr/SrO | 0.0479 | 0.0213 |
| Zr/ZrO2 | 0.0504 | 0.0264 |
| Nb/Nb2O5 | / | 0.0046 |
| Ba/BaO | 0.076 | 0.0387 |
| Cl | 4.25 | 2.08 |
| Sum | 99.929 | 99.974 |
| T(°C) | Rgb (Ω) | Cgb (F) | Qgb (F.sα1) | A | Rg (Ω) | Cg (F) | Qg (F.sα1) | β (°) |
|---|---|---|---|---|---|---|---|---|
| 600 °C | 5.974 × 106 | 1.004 × 10−9 | 0.169 × 10−9 | 0.1782 | 3.238 × 106 | 1.559 × 10−9 | 2.596 × 10−9 | 32.57 |
| 650 °C | 2.975 × 106 | 0.1925 × 10−9 | 0.2776 × 10−9 | 0.1817 | 1.575 × 106 | 1.671 × 10−9 | 3.136 × 10−9 | 36.14 |
| 700 °C | 1.359 × 106 | 0.1811 × 10−9 | 0.3606 × 10−9 | 0.1751 | 8.24606 × 105 | 1.81 4 × 10−9 | 3.826 × 10−9 | 33.25 |
| 750 °C | 7.10739 × 105 | 0.1784 × 10−9 | 0.397 × 10−9 | 0.2573 | 6.600315 × 105 | 1.964 × 10−9 | 4.266 × 10−9 | 30.45 |
| 800 °C | 4.15429 × 105 | 0.1679 × 10−9 | 0.4256 × 10−9 | 0.1454 | 3.6902 × 105 | 2.175 × 10−9 | 4.976 × 10−9 | 38.62 |
| 850 °C | 2.30314 × 105 | 0.1479 × 10−9 | 0.5643 × 10−9 | 0.3565 | 1.77127 × 105 | 2.419 × 10−9 | 5.1656 × 10−9 | 34.05 |
| 900 °C | 1.516 × 105 | 0.1388 × 10−9 | 0.669 × 10−9 | 0.3575 | 9.5970 × 104 | 2.675 × 10−9 | 6.163 × 10−9 | 32.38 |
| 850 °C | 6.439 × 105 | 0.1609 × 10−9 | 0.6365 × 10−9 | 0.1594 | 1.99689 × 105 | 2.714 × 10−9 | 6.103 × 10−9 | 34.88 |
| 800 °C | 9.491 × 105 | 0.1823 × 10−9 | 0.5312 × 10−9 | 0.1711 | 3.19745 × 105 | 2.516 × 10−9 | 5.393 × 10−9 | 37.47 |
| 750 °C | 1.612 × 106 | 0.1974 × 10−9 | 0.4025 × 10−9 | 0.2013 | 5.68456 × 105 | 3.227 × 10−9 | 4.553 × 10−9 | 31.59 |
| 700 °C | 2.684 × 106 | 1.164 × 10−9 | 0.3229 × 10−9 | 0.2288 | 9.98857 × 105 | 4.619 × 10−9 | 4.193 × 10−9 | 30.25 |
| 650 °C | 5.011 × 106 | 1.364 × 10−9 | 0.227 × 10−9 | 0.2333 | 1.747 × 106 | 6.033 × 10−9 | 3.733 × 10−9 | 30.15 |
| 600 °C | 8.562 × 106 | 1.564 × 10−9 | 0.2957 × 10−9 | 0.2394 | 3.775 × 106 | 8.049 × 10−9 | 2.803 × 10−9 | 30.64 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Riouchi, N.; Riouchi, O.; Lamrani, O.; Yahakoub, E.H.; Mansori, M.; Genorio, B.; Kolar, M.; Petrova, P.; El Barkany, S.; Abou-Salama, M.; et al. Development of Diopside-Modified Marl-Based Dielectric Composite for Microelectronics Applications. Nanomaterials 2025, 15, 668. https://doi.org/10.3390/nano15090668
Riouchi N, Riouchi O, Lamrani O, Yahakoub EH, Mansori M, Genorio B, Kolar M, Petrova P, El Barkany S, Abou-Salama M, et al. Development of Diopside-Modified Marl-Based Dielectric Composite for Microelectronics Applications. Nanomaterials. 2025; 15(9):668. https://doi.org/10.3390/nano15090668
Chicago/Turabian StyleRiouchi, Nassima, Oussama Riouchi, Othmane Lamrani, El Hassan Yahakoub, Mohammed Mansori, Boštjan Genorio, Mitja Kolar, Petranka Petrova, Soufian El Barkany, Mohamed Abou-Salama, and et al. 2025. "Development of Diopside-Modified Marl-Based Dielectric Composite for Microelectronics Applications" Nanomaterials 15, no. 9: 668. https://doi.org/10.3390/nano15090668
APA StyleRiouchi, N., Riouchi, O., Lamrani, O., Yahakoub, E. H., Mansori, M., Genorio, B., Kolar, M., Petrova, P., El Barkany, S., Abou-Salama, M., & Loutou, M. (2025). Development of Diopside-Modified Marl-Based Dielectric Composite for Microelectronics Applications. Nanomaterials, 15(9), 668. https://doi.org/10.3390/nano15090668









