Harnessing Magnetic Properties for Precision Thermal Control of Vortex Domain Walls in Constricted Nanowires
Abstract
1. Introduction
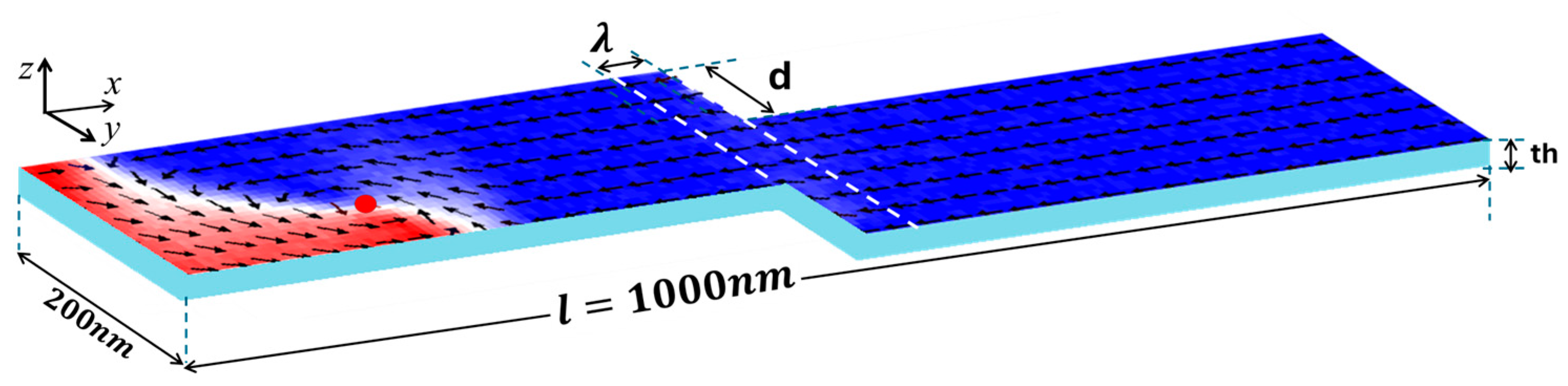
2. Theoretical Model
3. Results and Discussion
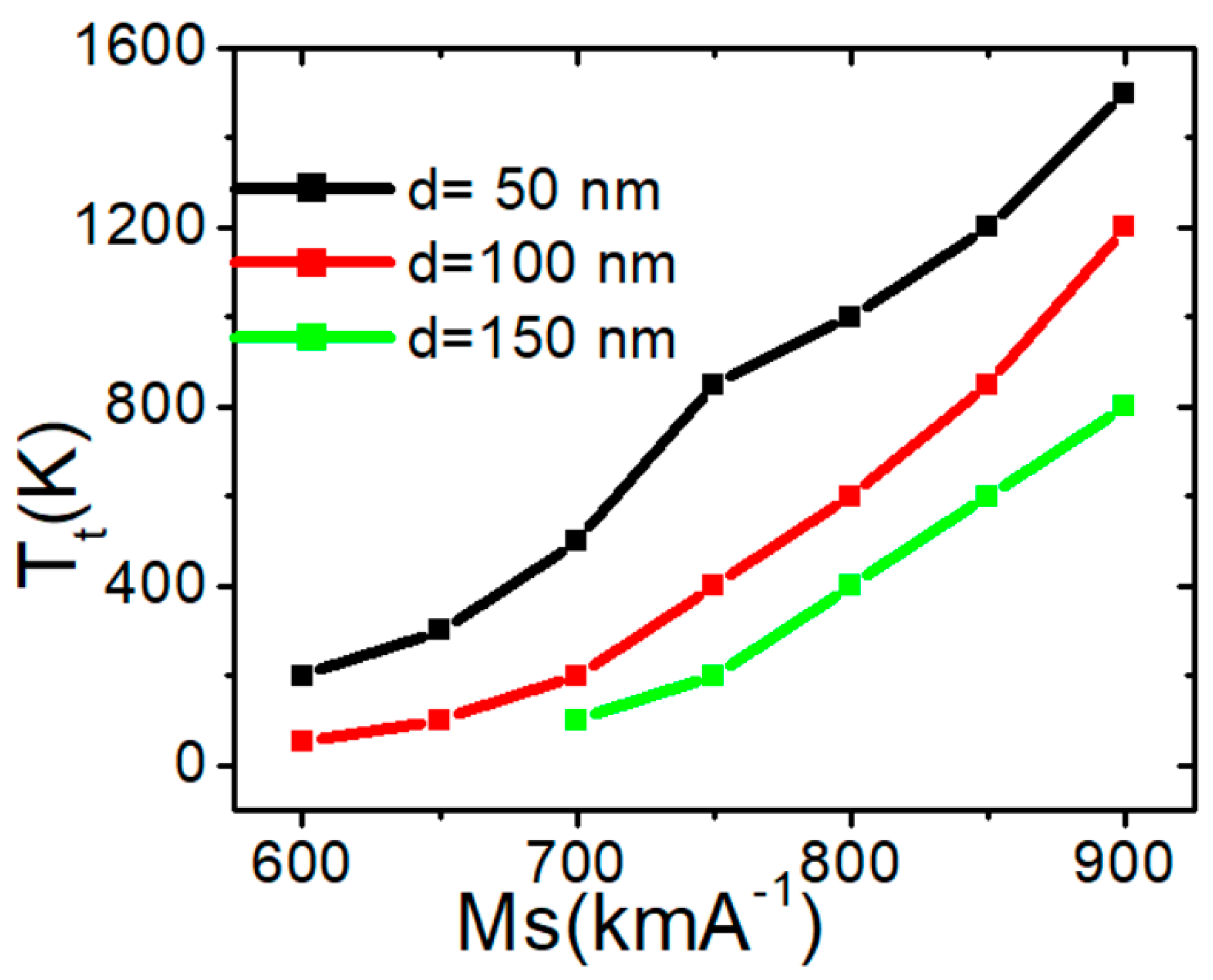
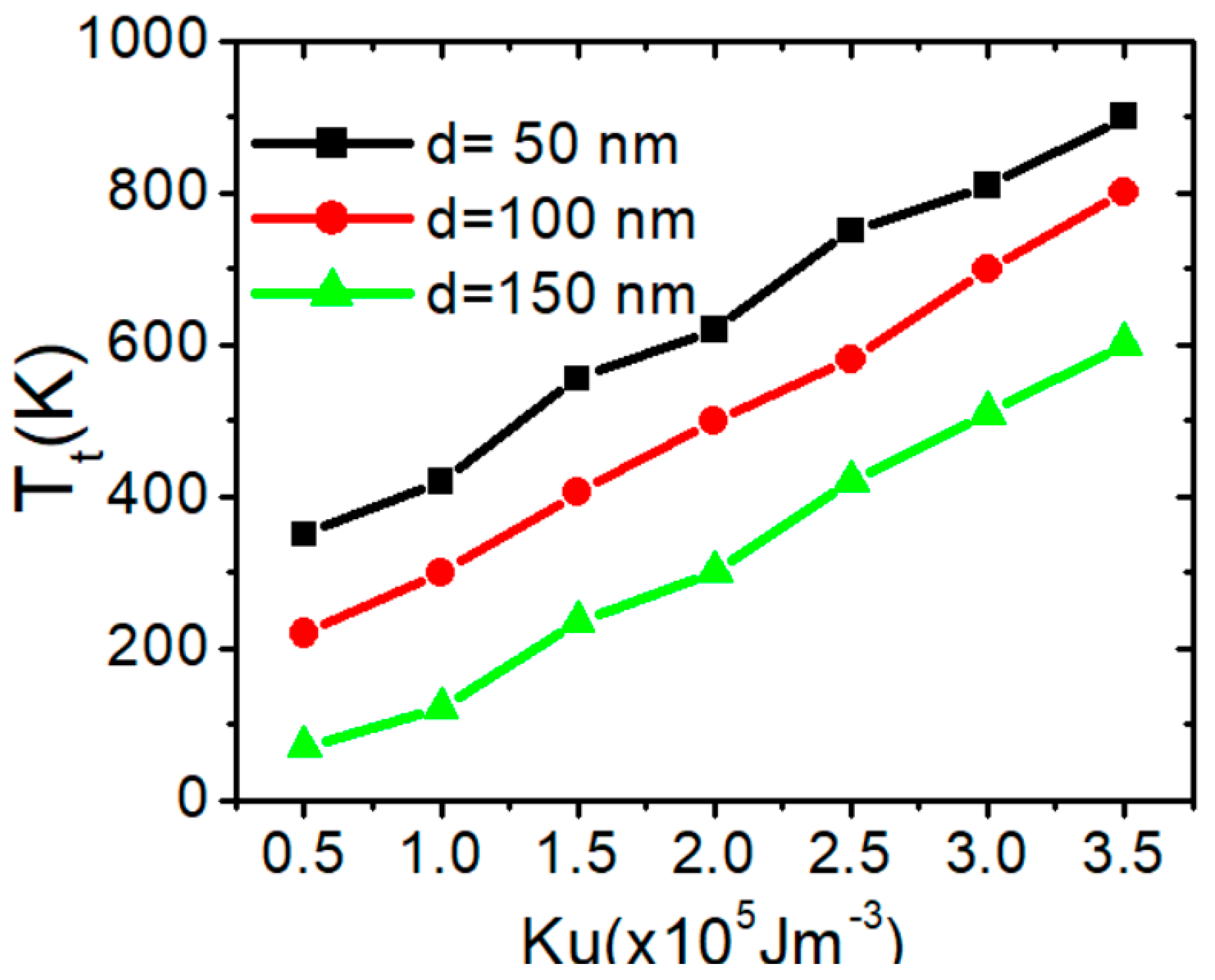
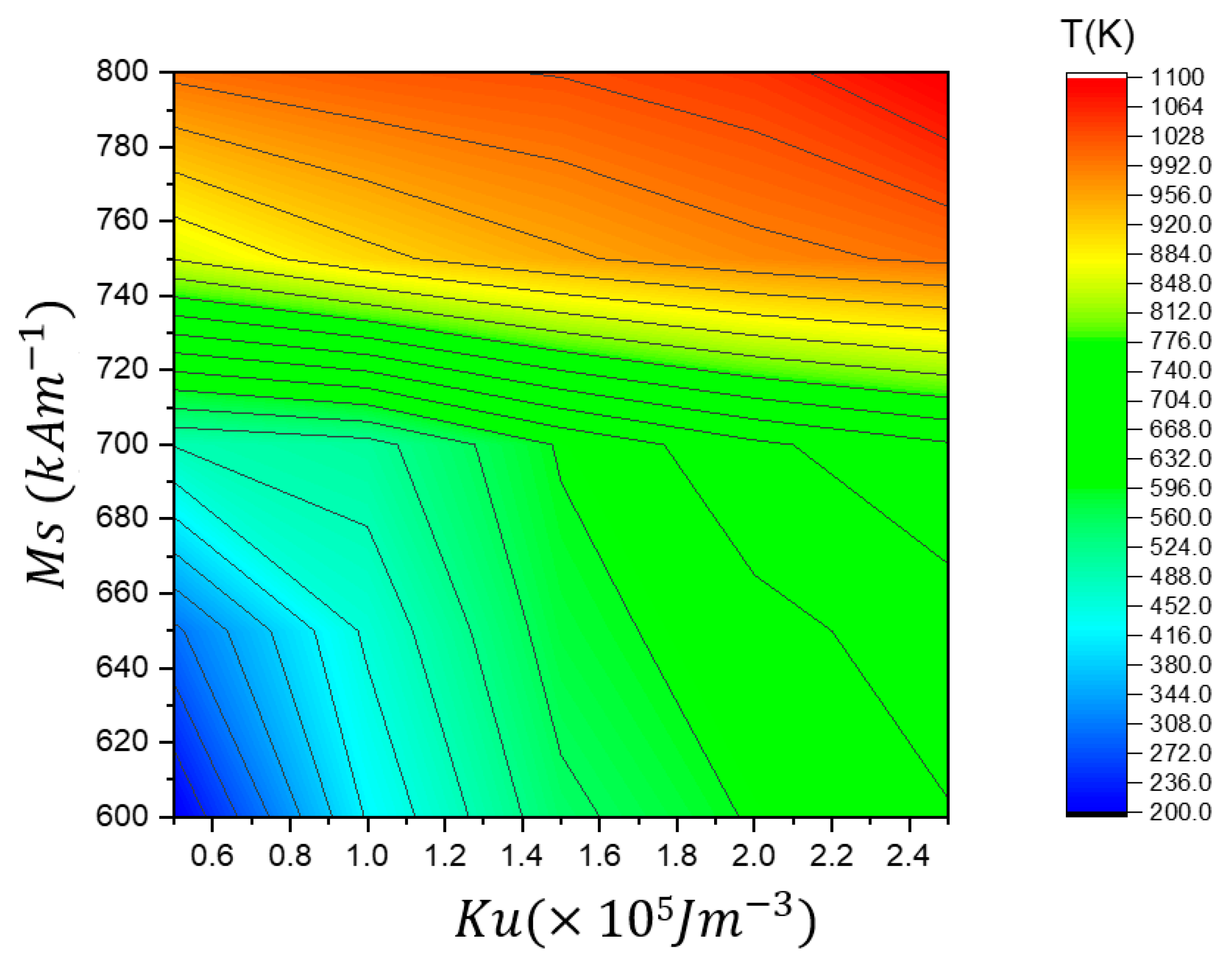
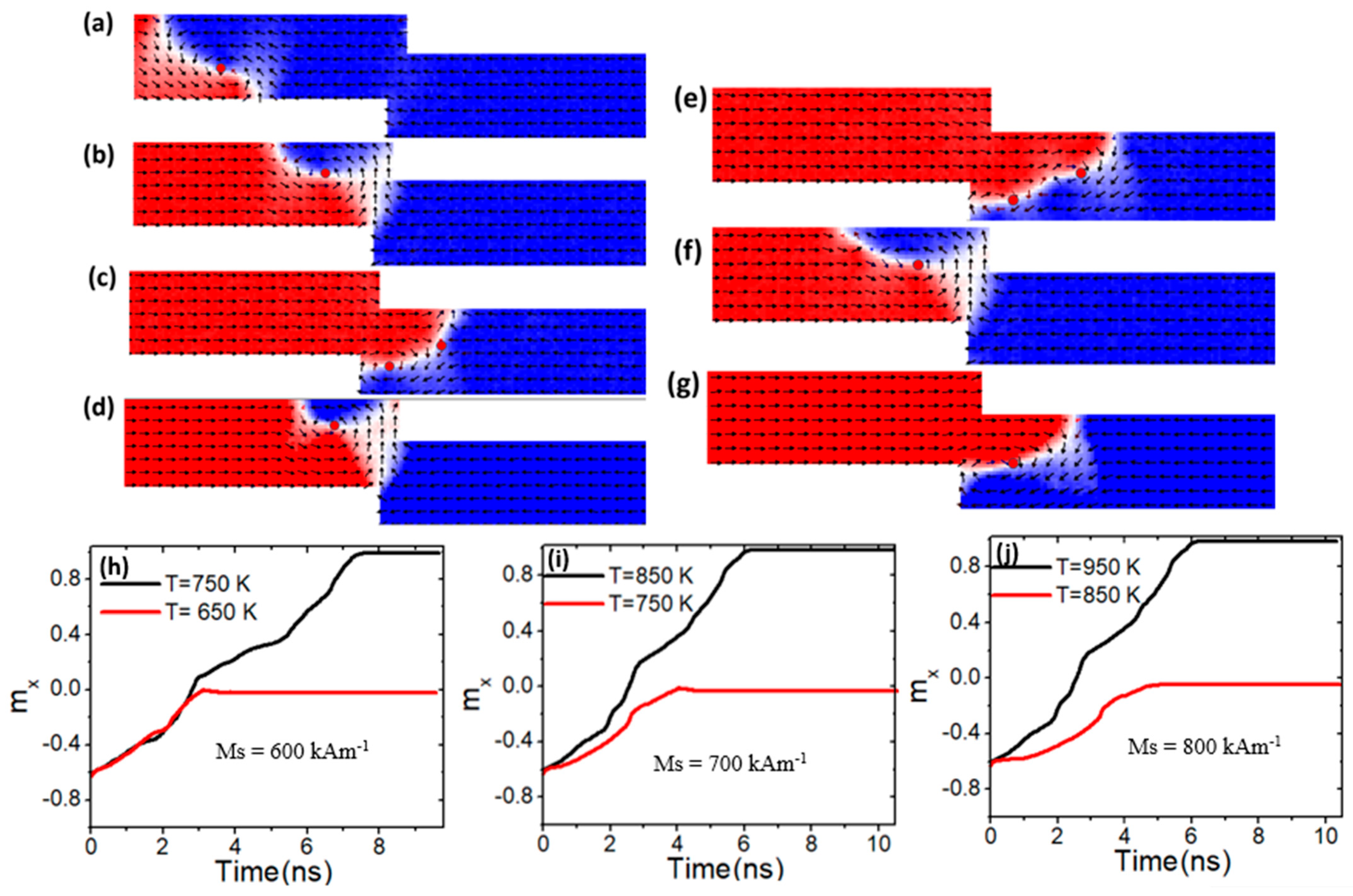
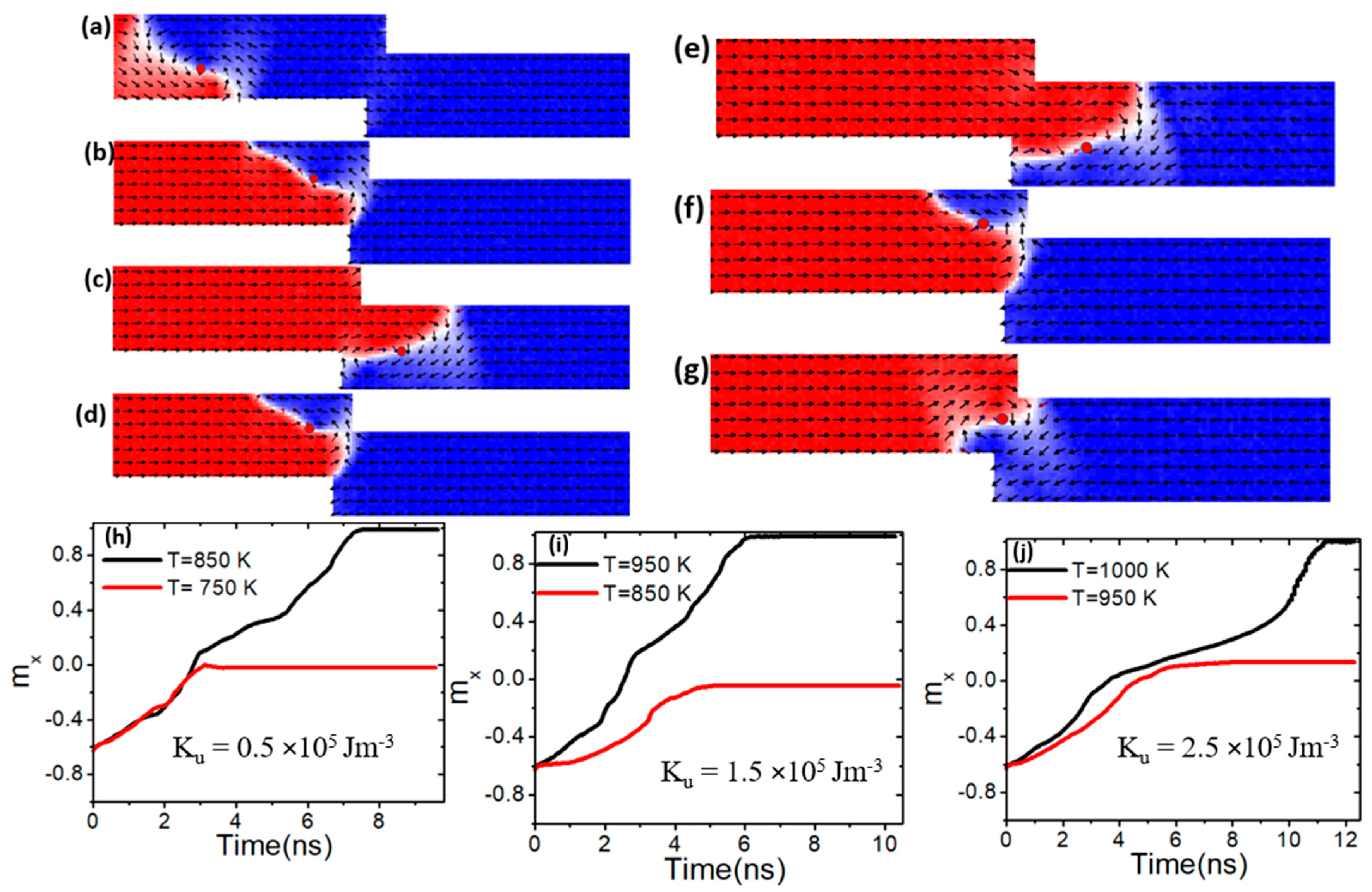
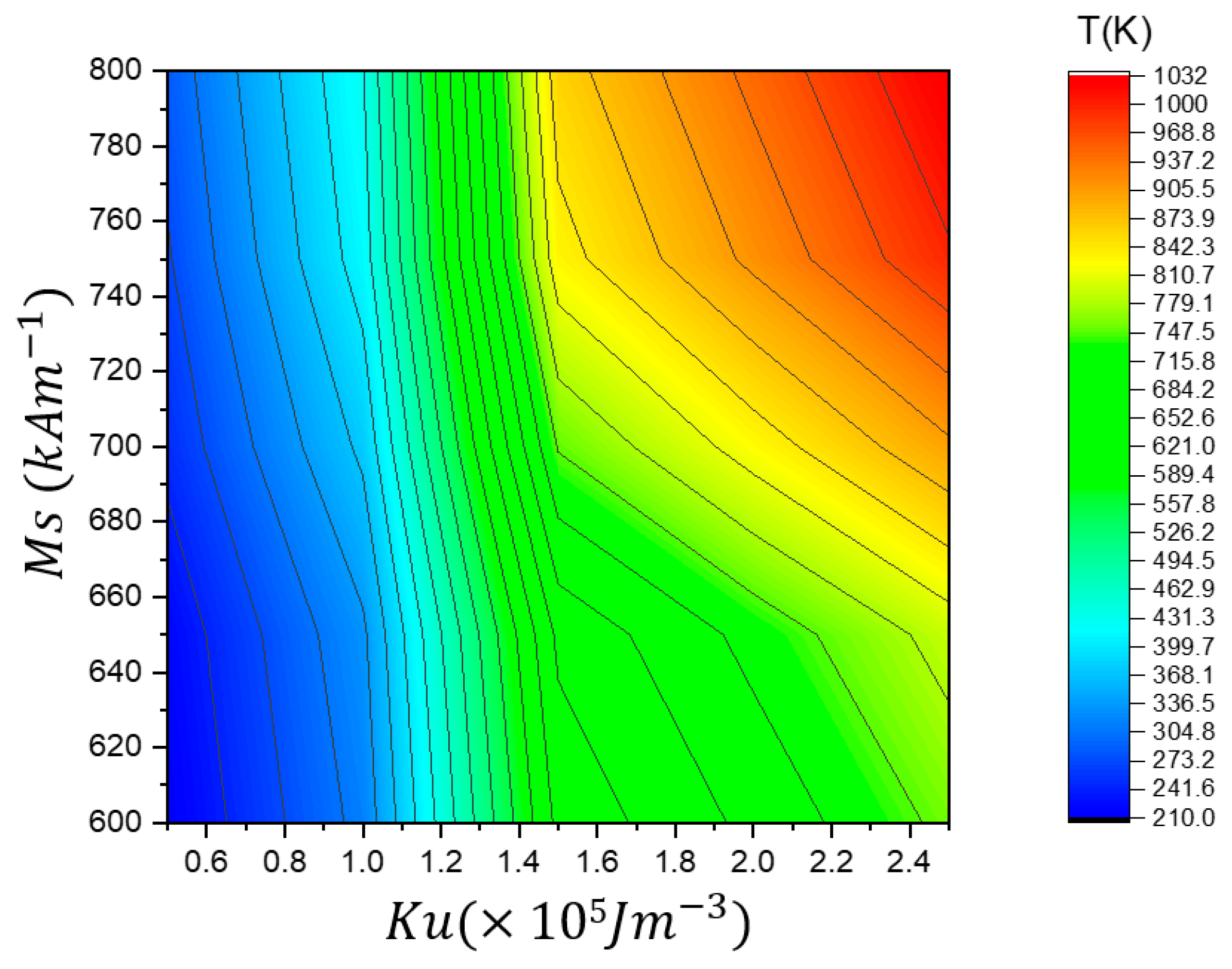
3.1. VW Thermal Structural Stability
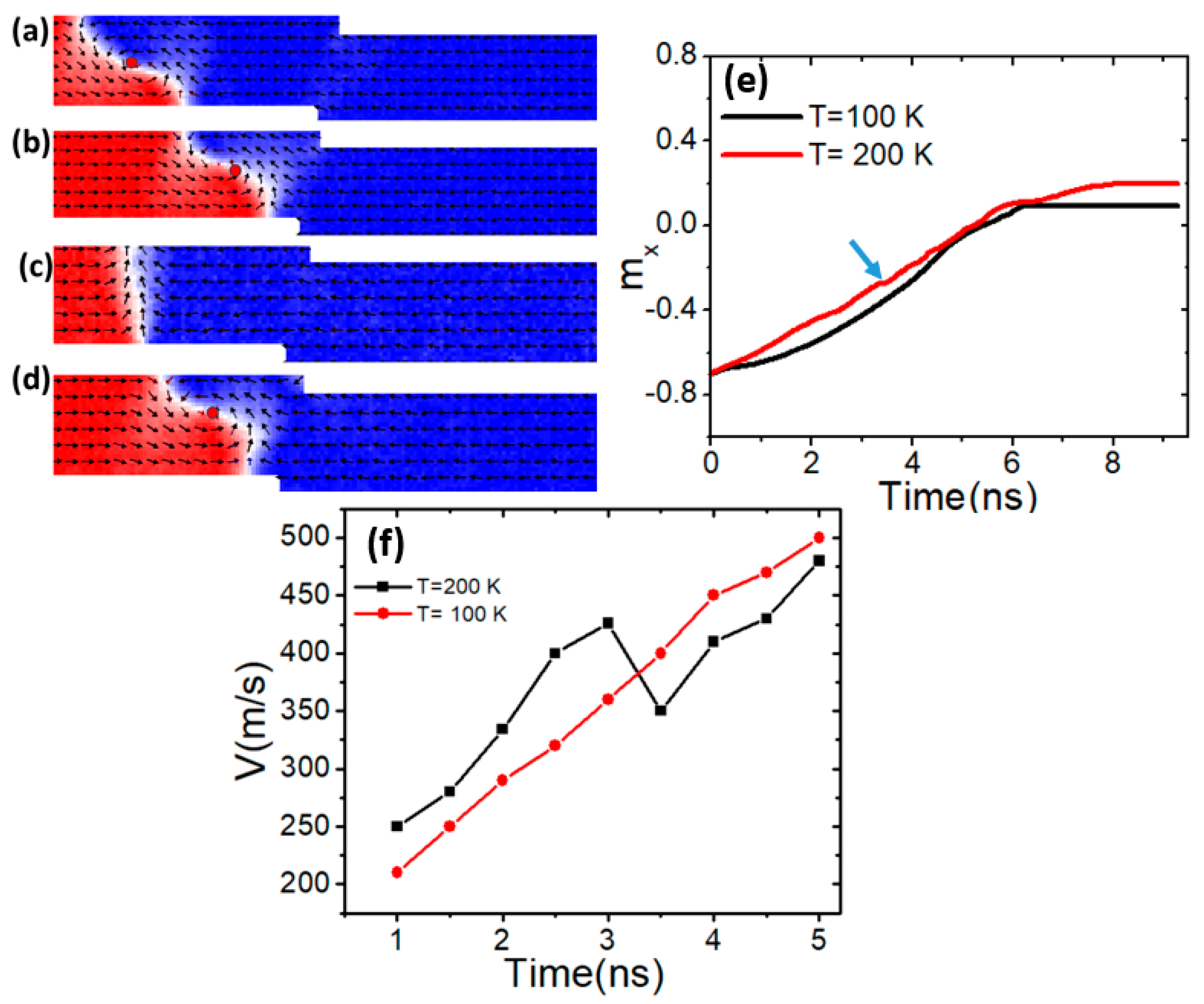
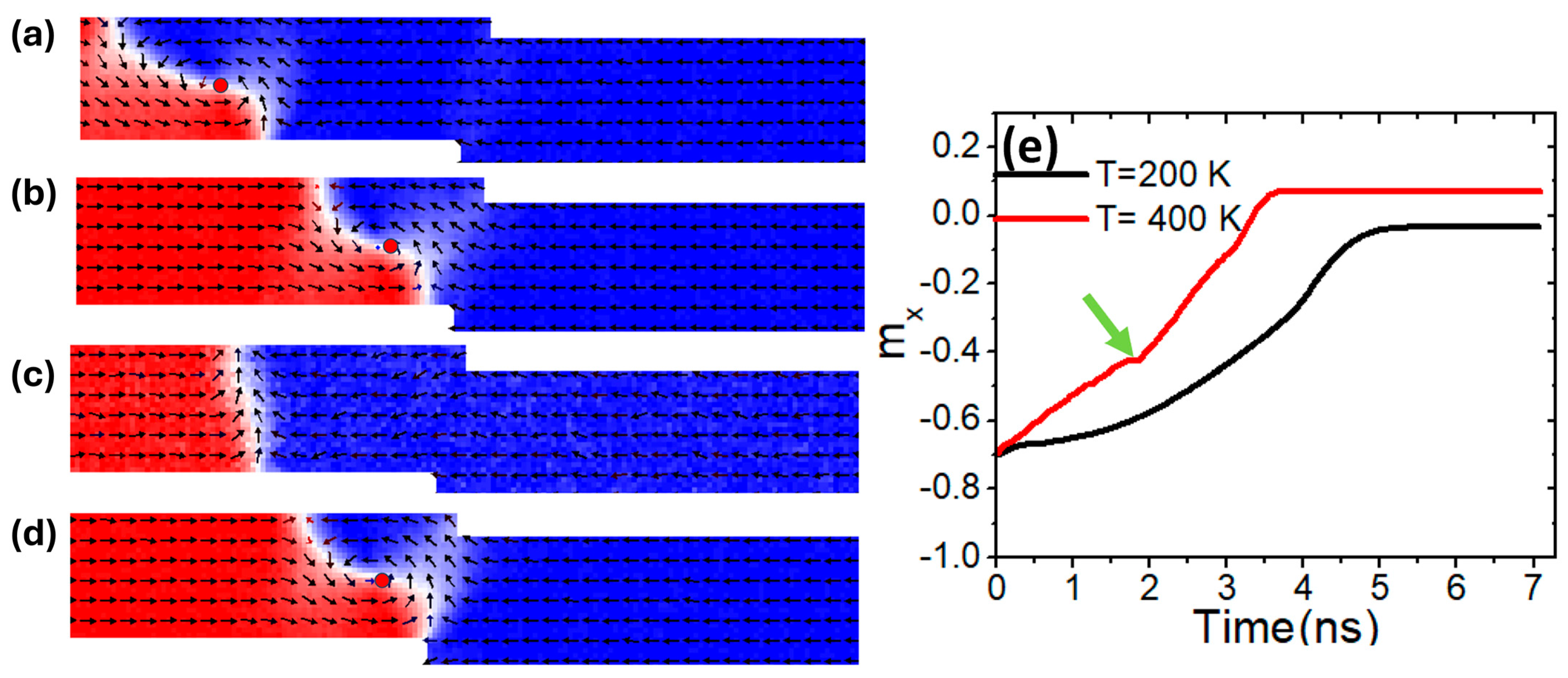
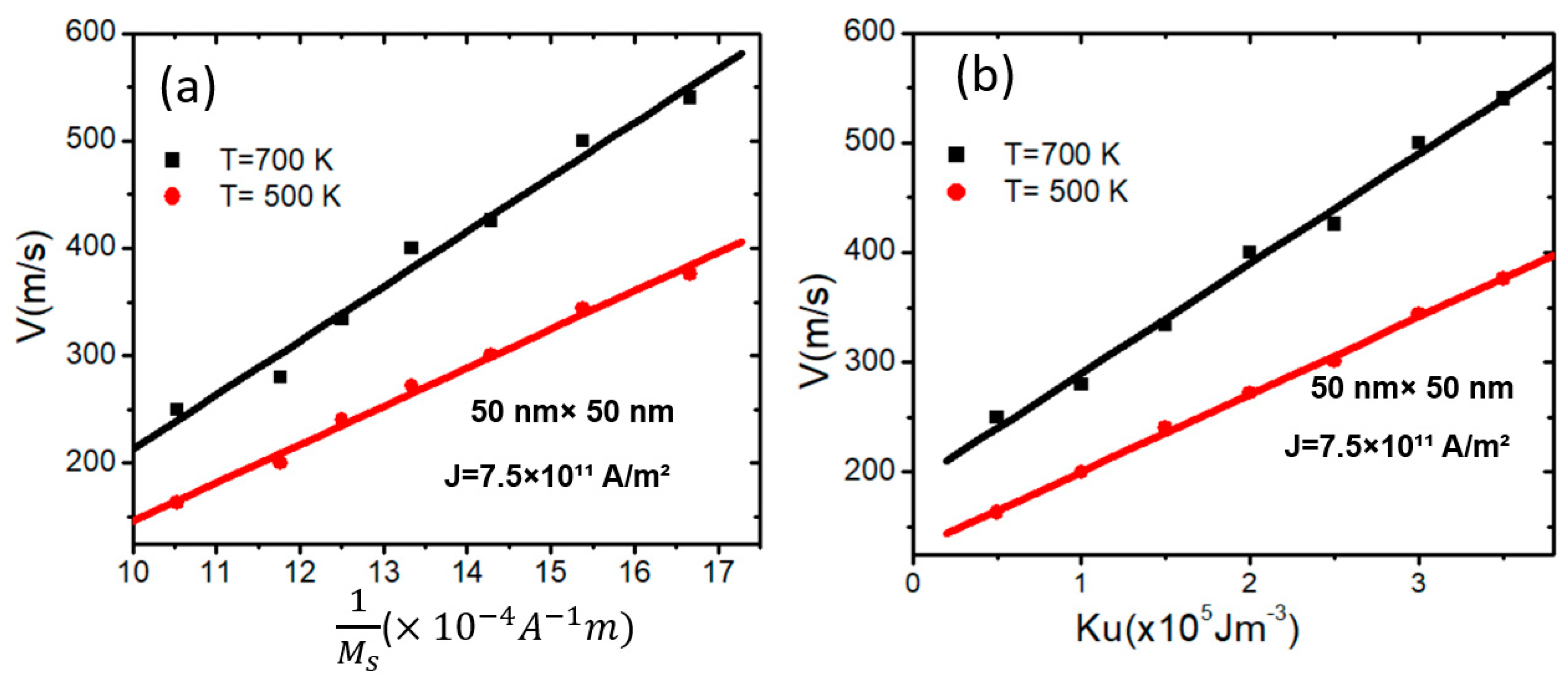
3.2. Thermal Effects on Vortex Domain Wall (VW) Dynamics
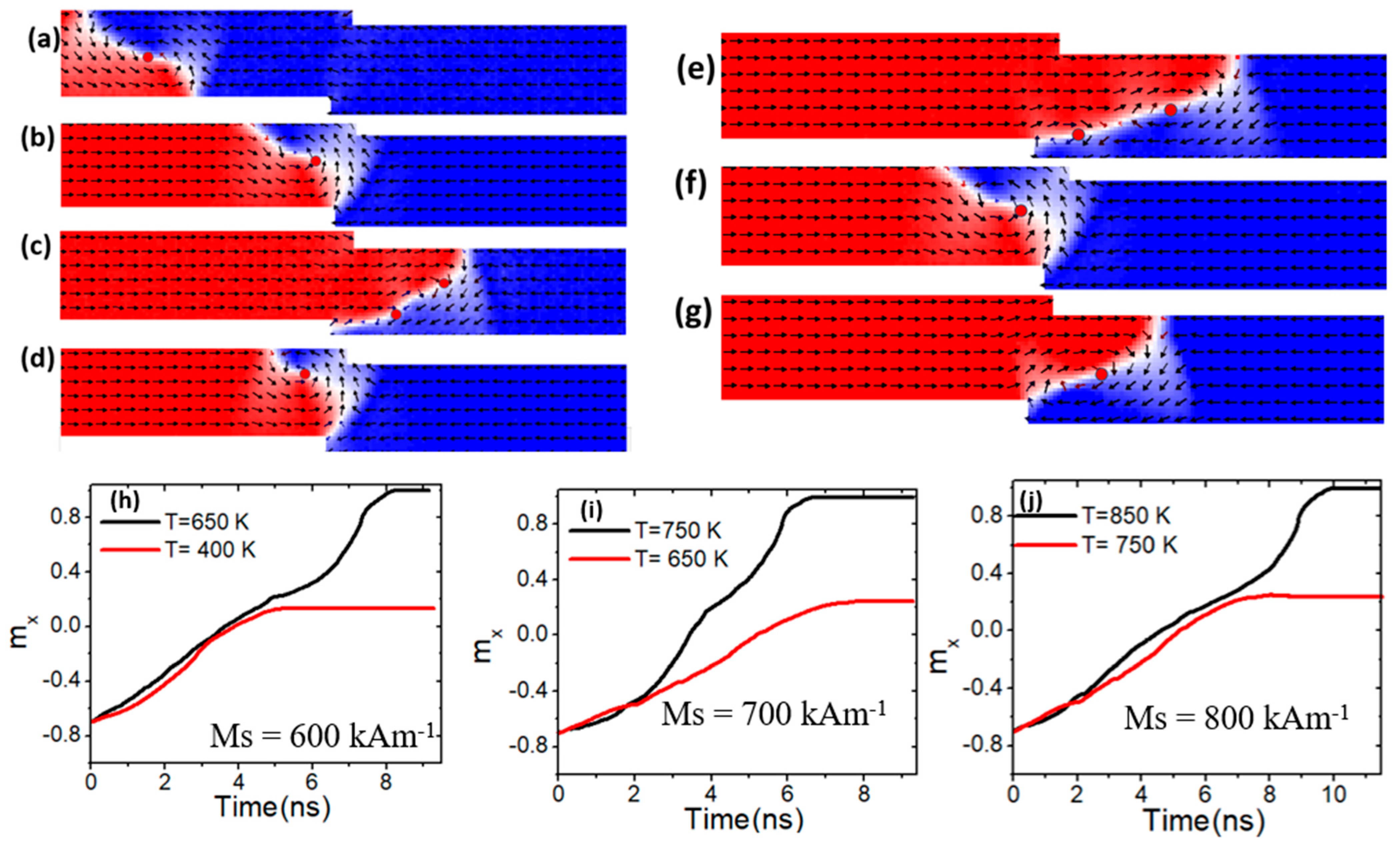
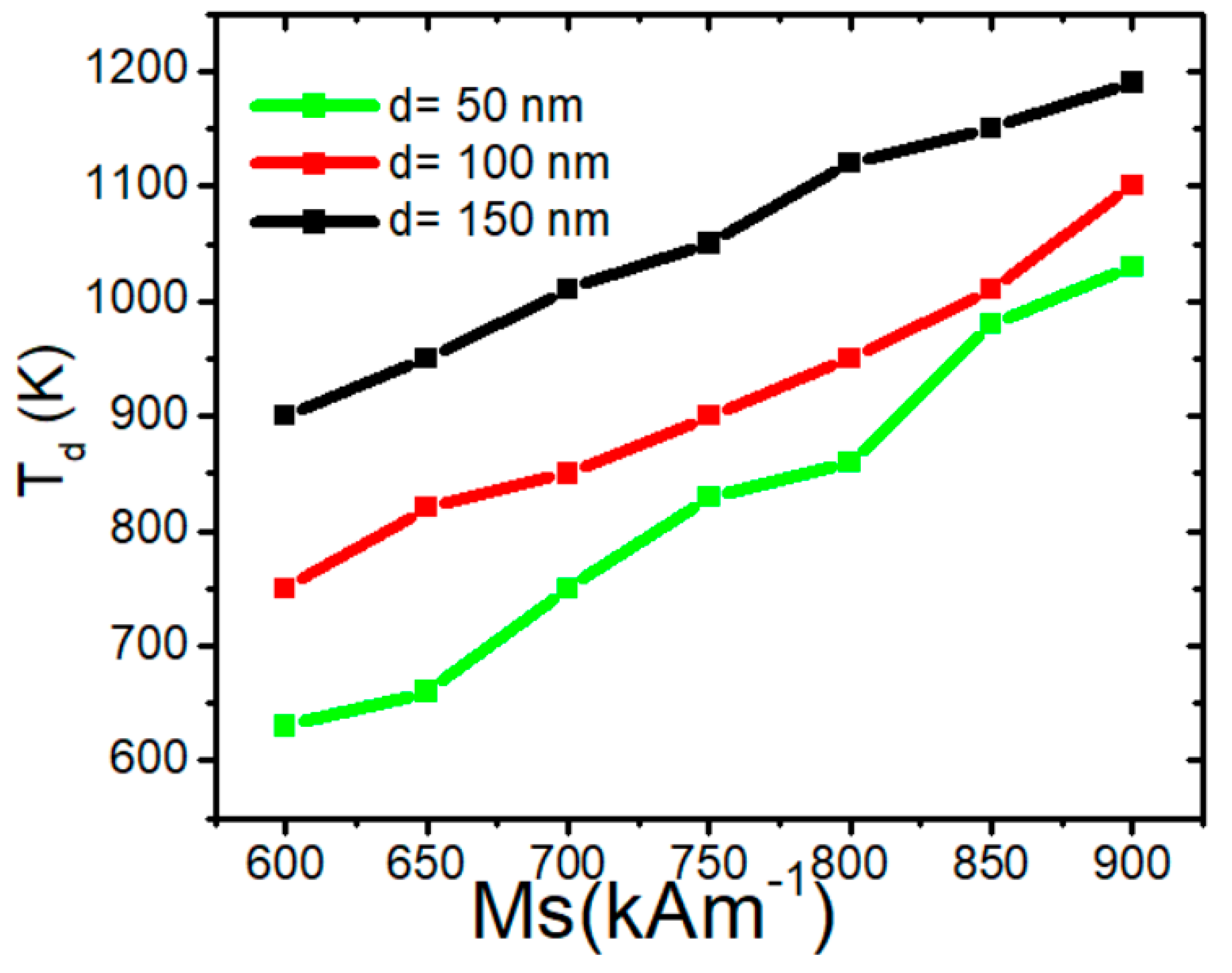
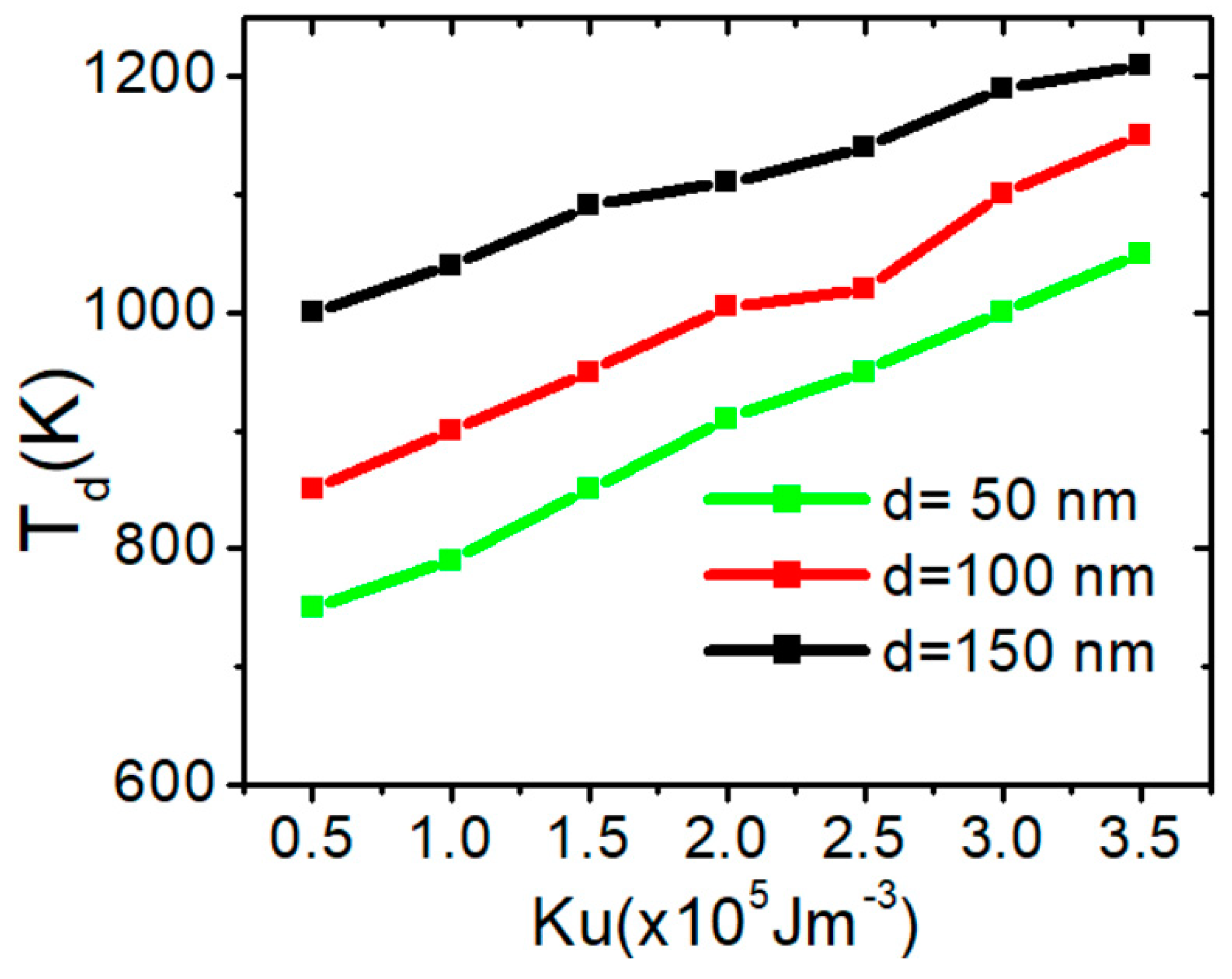
3.3. Thermal Control of VW Pinning and Depinning
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Parkin, S.S.P.; Hayashi, M.; Thomas, L. Magnetic Domain-Wall Racetrack Memory. Science 2008, 320, 190–194. [Google Scholar] [CrossRef] [PubMed]
- MacLaren, R.; Pearson, R.; MacNeil, A.; Douglas, R.H.; Salt, T.E.; Akimoto, M.; Swaroop, A.; Sowden, J.; Ali, R. Retinal repair by transplantation of photoreceptor precursors. Nature 2006, 444, 203–207. [Google Scholar] [CrossRef] [PubMed]
- Al Bahri, M.; Borie, B.; Jin, T.L.; Sbiaa, R.; Kläui, M.; Piramanayagam, S.N. Staggered magnetic nanowire devices for effective domain-wall pinning in racetrack memory. Phys. Rev. Appl. 2019, 11, 024023. [Google Scholar] [CrossRef]
- Hütten, A.; Sudfeld, D.; Ennen, I.; Reiss, G.; Wojczykowski, K.; Jutzi, P. Ferromagnetic FeCo nanoparticles for biotechnology. J. Magn. Magn. Mater. 2005, 293, 93–101. [Google Scholar] [CrossRef]
- Al Bahri, M. Geometrical Confinement of Vortex Domain Wall in Constricted Magnetic Nanowire with In-Plane Magnetic Anisotropy. IEEE Trans. Magn. 2021, 57, 1–7. [Google Scholar] [CrossRef]
- Bland, J.A.C.; Heinrich, B. Ultrathin Magnetic Structures I: Fundamentals of Nanomagnetism; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Tang, L.; Lee, K.; Lee, S.; Choi, J.; Lee, K.-J.; Lee, H. Thermal stability of vortex domain walls in stepped geometries. Nat. Mater. 2014, 13, 846–850. [Google Scholar] [CrossRef]
- Al Bahri, M. Controlling domain wall thermal stability switching in magnetic nanowires for storage memory nanodevices. J. Magn. Magn. Mater. 2021, 543, 168611. [Google Scholar] [CrossRef]
- Puebla, J.; Kim, J.; Kondou, K.; Otani, Y. Spintronic devices for energy-efficient data storage and energy harvesting. Commun. Mater. 2020, 1, 24. [Google Scholar] [CrossRef]
- Curcic, M.; Stoll, H.; Weigand, M.; Sackmann, V.; Juellig, P.; Kammerer, M.; Noske, M.; Sproll, M.; Van Waeyenberge, B. Magnetic vortex core reversal by rotating magnetic fields generated on micrometer length scales. Phys. Status Solidi B 2011, 248, 2331–2336. [Google Scholar] [CrossRef]
- Shaji, N.; Simmons, C.B.; Thalakulam, M.; Klein, L.J.; Qin, H.; Luo, H.; Savage, D.E.; Lagally, M.G.; Rimberg, A.J.; Joynt, R.; et al. Spin blockade and lifetime-enhanced transport in a few-electron Si/SiGe double quantum dot. Nat. Phys. 2008, 4, 540–544. [Google Scholar] [CrossRef]
- Al Bahri, M.; Sbiaa, R. Chirality-Dependent Dynamics and Pinning of Transverse Domain Wall in Constricted Nanowires. Phys. Status Solidi (A) 2024, 221, 202300906. [Google Scholar] [CrossRef]
- Ramirez, S.; Chan, K.; Hernandez, R.; Recinos, E.; Hernandez, E.; Salgado, R.; Khitun, A.G.; Garay, J.E.; Balandin, A.A. Thermal and magnetic properties of nanostructured densified ferrimagnetic composites with graphene-graphite fillers. Mater. Des. 2017, 118, 75–80. [Google Scholar] [CrossRef]
- Al Bahri, M.; Al Hinaai, M.; Al Balushi, R.; Al-Kamiyani, S. Enhancing the Thermal Stability of Skyrmion in Magnetic Nanowires for Nanoscale Data Storage. Nanomaterials 2024, 14, 1763. [Google Scholar] [CrossRef] [PubMed]
- Tanigawa, H.; Koyama, T.; Bartkowiak, M.; Kasai, S.; Kobayashi, K.; Ono, T.; Nakatani, Y. Dynamical Pinning of a Domain Wall in a Magnetic Nanowire Induced by Walker Breakdown. Phys. Rev. Lett. 2008, 101, 207203. [Google Scholar] [CrossRef]
- Beach, G.S.D.; Nistor, C.; Knutson, C.; Tsoi, M.; Erskine, J.L. Dynamics of field-driven domain-wall propagation in ferromagnetic nanowires. Nat. Mater. 2005, 4, 741–744. [Google Scholar] [CrossRef]
- Pawlak, A.; Gülpınar, G.; Erdem, R.; Ağartıoğlu, M. Domain wall pinning and depinning in stepped magnetic nanowires: A micromagnetic study. J. Magn. Magn. Mater. 2015, 395, 161–165. [Google Scholar] [CrossRef]
- Fukami, S.; Ieda, J.; Ohno, H. Thermal stability of a magnetic domain wall in nanowires. Phys. Rev. B 2015, 91, 235401. [Google Scholar] [CrossRef]
- Yang, S.-H.; Parkin, S.S.P.; Bonnell, D.A.; Johnson, A.T.C.; Samarth, N. Temperature and magnetic field effects on vortex domain wall motion in nanowires. J. Appl. Phys. 2009, 106, 033904. [Google Scholar] [CrossRef]
- Finocchio, G.; Carpentieri, M.; Azzerboni, B.; Torres, L.; Martinez, E.; Lopez-Diaz, L. Micromagnetic simulations of nanosecond magnetization reversal processes in magnetic nanopillar. J. Appl. Phys. 2006, 99, 2177049. [Google Scholar] [CrossRef]
- Thiaville, A.; Nakatani, Y. Domain-wall dynamics in nanowires and nanostrips. In Spin Dynamics in Confined Magnetic Structures III; Springer: Berlin/Heidelberg, Germany, 2006; Volume 1, p. 161. [Google Scholar] [CrossRef]
- Al Bahri, M.; Al-Kamiyani, S.; Al Habsi, A.M. Vortex Domain Wall Thermal Pinning and Depinning in a Constricted Magnetic Nanowire for Storage Memory Nanodevices. Nanomaterials 2024, 14, 1518. [Google Scholar] [CrossRef]
- Clark, B.K.; Abanin, D.A.; Sondhi, S.L. Magnetic domain walls in Confined geometries: Effect of temperature. Phys. Rev. Lett. 2011, 107, 087204. [Google Scholar] [CrossRef]
- Acharyya, M. Thermal stability of vortex domain walls in magnetic nanowires. J. Magn. Magn. Mater. 2013, 334, 11–15. [Google Scholar] [CrossRef][Green Version]
- Bogart, L.K.; Eastwood, D.S.; Atkinson, D. The effect of geometrical confinement and chirality on domain wall pinning behavior in planar nanowires. J. Appl. Phys. 2008, 104, 033904. [Google Scholar] [CrossRef]
- Filho, M.A.M.; Hsiao, C.-L.; dos Santos, R.B.; Hultman, L.; Birch, J.; Gueorguiev, G.K. Self-Induced Core–Shell InAlN Nanorods: Formation and Stability Unraveled by Ab Initio Simulations. ACS Nanosci. Au 2023, 3, 84–93. [Google Scholar] [CrossRef]
- Pokluda, J.; Černý, M.; Šob, M.; Umeno, Y. Ab initio calculations of mechanical properties: Methods and applications. Prog. Mater. Sci. 2015, 73, 127–158. [Google Scholar] [CrossRef]
- Donahue, M.; Porter, D.G. OOMMF User’s Guide; Version 1.0, Interagency Report NISTIR 6376; Technical 427 Report, Version 1.0; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1999. Available online: https://math.nist.gov/oommf/ (accessed on 10 October 2024).
- Martinez, E.; Lopez-Diaz, L.; Torres, L.; Tristan, C.; Alejos, O. Thermal effects in domain wall motion: Micromagnetic simulations and analytical model. Phys. Rev. B 2007, 75, 174409. [Google Scholar] [CrossRef]
- Gilbert, T.L. A phenomenological theory of damping in ferromagnetic materials. IEEE Trans. Magn. 2004, 40, 3443–3449. [Google Scholar] [CrossRef]
- Brown, W.F. Thermal Fluctuations of a Single-Domain Particle. Phys. Rev. B 1963, 130, 1677–1686. [Google Scholar] [CrossRef]
- Lloyd, J.C.; Smith, R.S. Structural and Magnetic Properties of Permalloy Films. J. Appl. Phys. 1959, 30, S274–S275. [Google Scholar] [CrossRef]
- Jeudy, V.; Pardo, R.D.; Torres, W.S.; Bustingorry, S.; Kolton, A.B. Pinning of domain walls in thin ferromagnetic films. Phys. Rev. B 2018, 98, 054406. [Google Scholar] [CrossRef]
- Gu, M.; Wiesner, K.; Rieper, E.; Vedral, V. Spin transfer torque in constricted magnetic nanowires. Nat. Commun. 2012, 3, 762. [Google Scholar] [CrossRef] [PubMed]
- Boulle, O.; Malinowski, G.; Kläui, M. Current-induced domain wall motion in nanoscale ferromagnetic elements. Mater. Sci. Eng. R Rep. 2011, 72, 159–187. [Google Scholar] [CrossRef]
- Kumar, D.; Parkin, S.S.P. Micromagnetic simulations of domain wall dynamics in the presence of pinning. J. Appl. Phys. 2013, 113, 133903. [Google Scholar]
- Bahri, M.; Al-Kamiyani, S. Thermal Effects on Domain Wall Stability at Magnetic Stepped Nanowire for Nanodevices Storage. Nanomaterials 2024, 14, 1202. [Google Scholar] [CrossRef]
- Hayashi, M.; Thomas, L.; Rettner, C.; Moriya, R.; Jiang, X.; Parkin, S.S. Dependence of Current and Field Driven Depinning of Domain Walls on Their Structure and Chirality in Permalloy Nanowires. Phys. Rev. Lett. 2006, 97, 207205. [Google Scholar] [CrossRef]
- Eastwood, D.S.; Bogart, L.K.; Atkinson, D. Scaling Behaviour of Chirality Dependent Domain Wall Pinning in Planar Nanowires. Acta Phys. Pol. A 2010, 118, 719–722. [Google Scholar] [CrossRef]
- Khvalkovskiy, A.V.; Cros, V.; Apalkov, D.; Nikitin, V.; Krounbi, M.; Zvezdin, K.A.; Anane, A.; Grollier, J.; Fert, A. Matching domain-wall configuration and spin-orbit torques for efficient domain-wall motion. Phys. Rev. B 2013, 87, 020402(R). [Google Scholar] [CrossRef]
- Boulle, O.; Kimling, J.; Warnicke, P.; Kläui, M.; Rüdiger, U.; Malinowski, G.; Swagten, H.J.M.; Koopmans, B.; Ulysse, C.; Faini, G. Nonadiabatic spin transfer torque in high anisotropy magnetic nanowires with narrow domain walls. Phys. Rev. Lett. 2008, 101, 216601. [Google Scholar] [CrossRef]
- Wu, Y.; Xu, X.; Zhang, D.; Li, X.; Yang, H.; Miao, J.; Jiang, Y. Current-induced domain wall motion in magnetic nanowires with different dimensions. Phys. Mech. Astron. 2012, 55, 2030–2032. [Google Scholar] [CrossRef]
- Yuan, H.Y.; Wang, X.R. Domain wall pinning in notched nanowire. Phys. Rev. B 2014, 89, 054423. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al Bahri, M.; Al-Kamiyani, S. Harnessing Magnetic Properties for Precision Thermal Control of Vortex Domain Walls in Constricted Nanowires. Nanomaterials 2025, 15, 372. https://doi.org/10.3390/nano15050372
Al Bahri M, Al-Kamiyani S. Harnessing Magnetic Properties for Precision Thermal Control of Vortex Domain Walls in Constricted Nanowires. Nanomaterials. 2025; 15(5):372. https://doi.org/10.3390/nano15050372
Chicago/Turabian StyleAl Bahri, Mohammed, and Salim Al-Kamiyani. 2025. "Harnessing Magnetic Properties for Precision Thermal Control of Vortex Domain Walls in Constricted Nanowires" Nanomaterials 15, no. 5: 372. https://doi.org/10.3390/nano15050372
APA StyleAl Bahri, M., & Al-Kamiyani, S. (2025). Harnessing Magnetic Properties for Precision Thermal Control of Vortex Domain Walls in Constricted Nanowires. Nanomaterials, 15(5), 372. https://doi.org/10.3390/nano15050372





