Tetrahedrite Nanocomposites for High Performance Thermoelectrics
Abstract
1. Introduction
2. Materials and Methods
3. Results
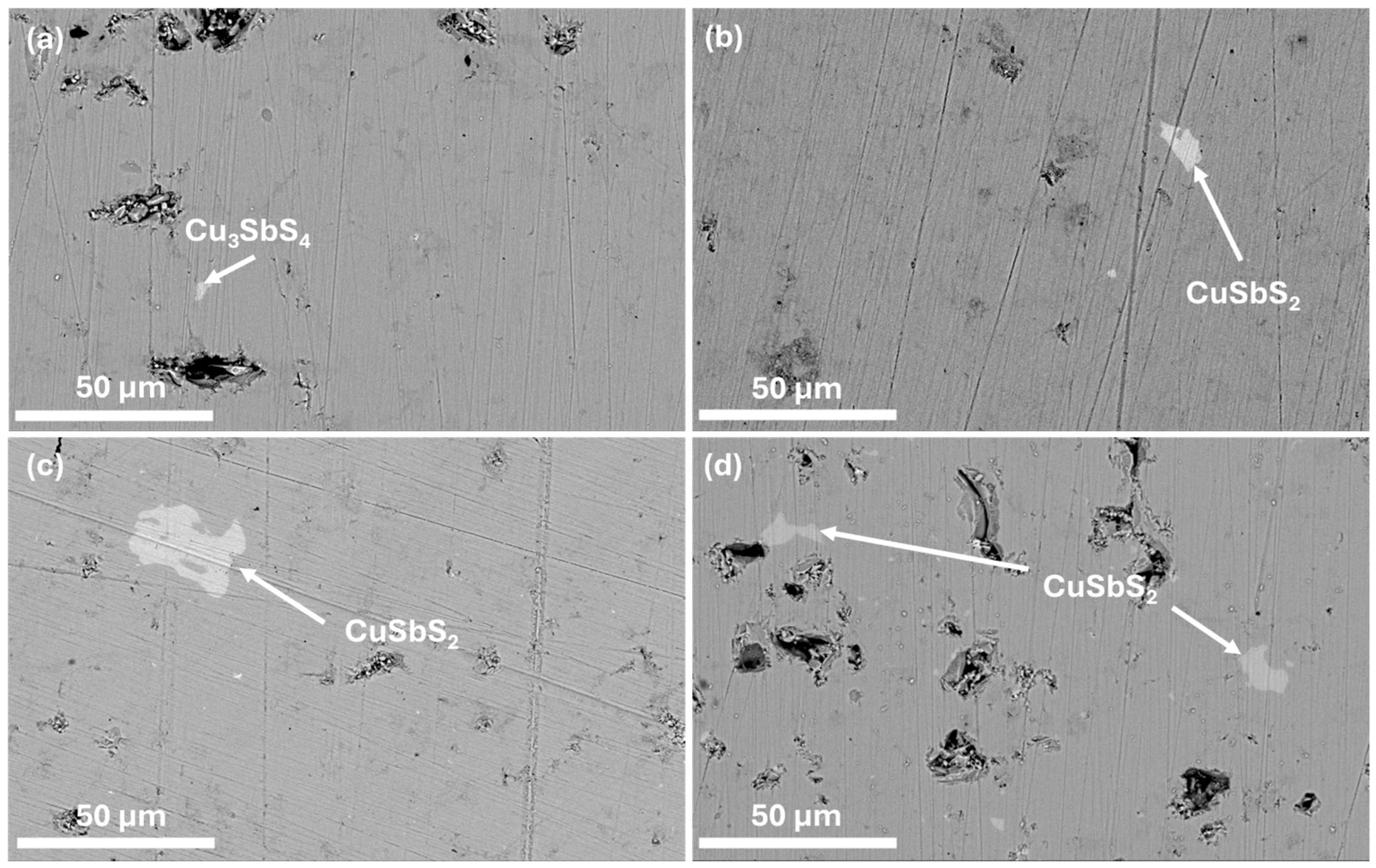
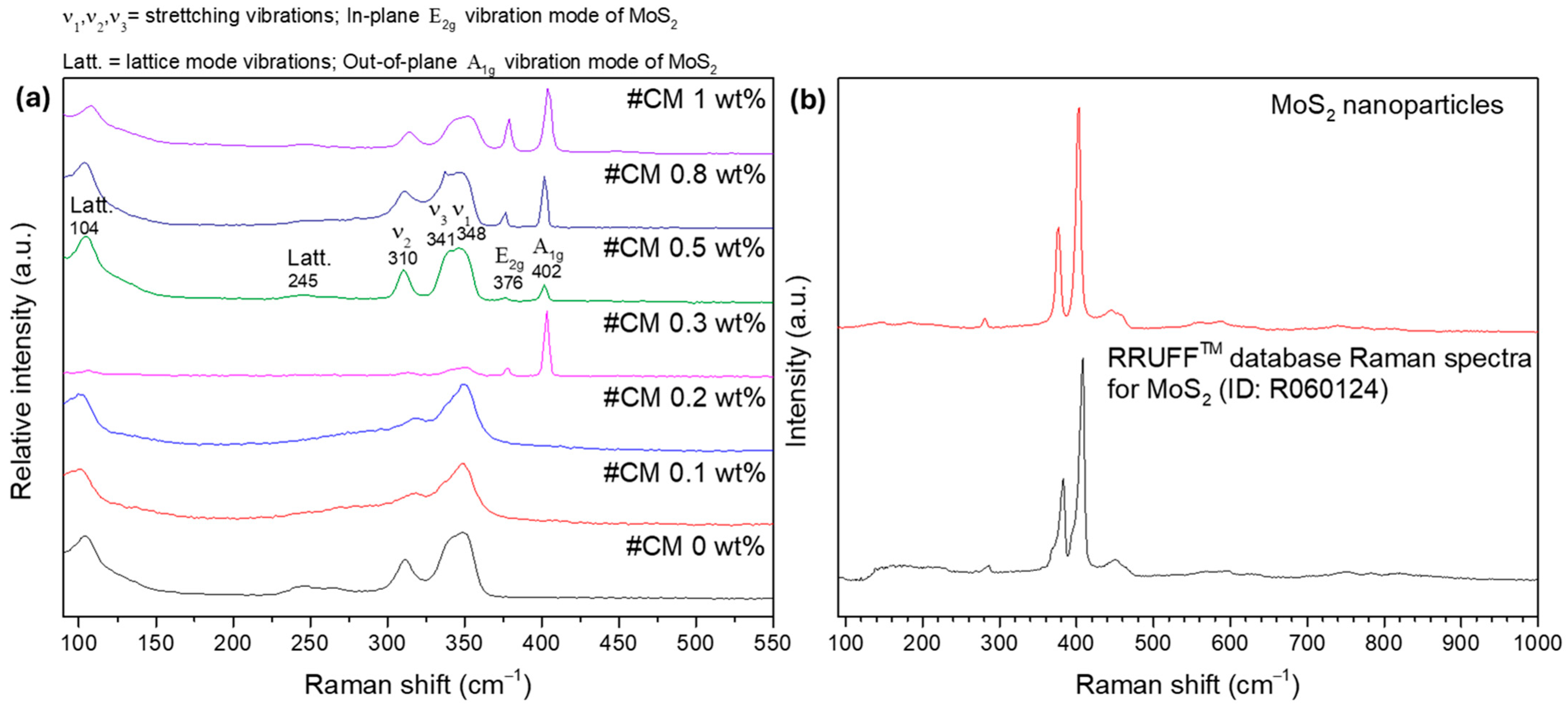
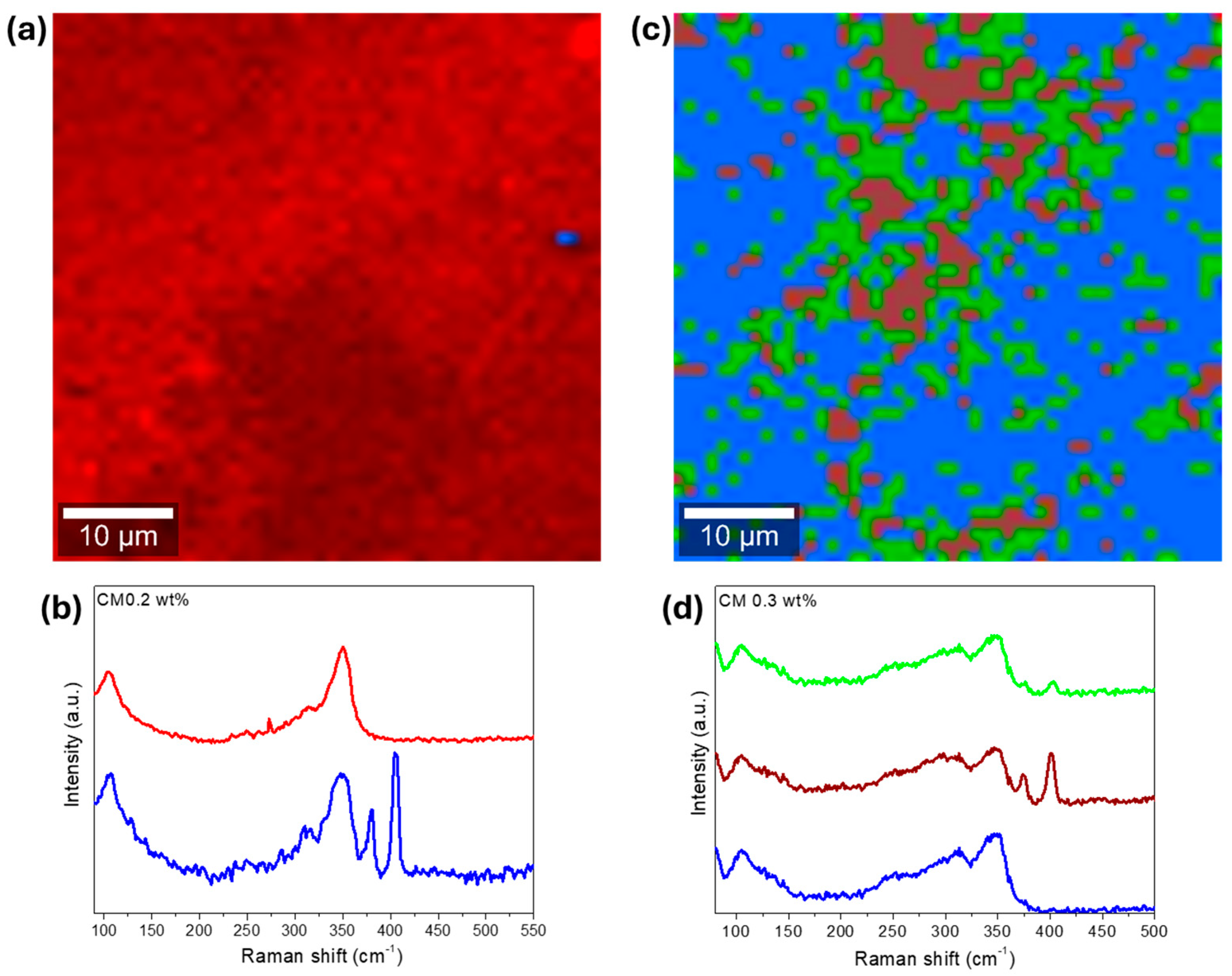
3.1. Crystall Structure and Phase Composition
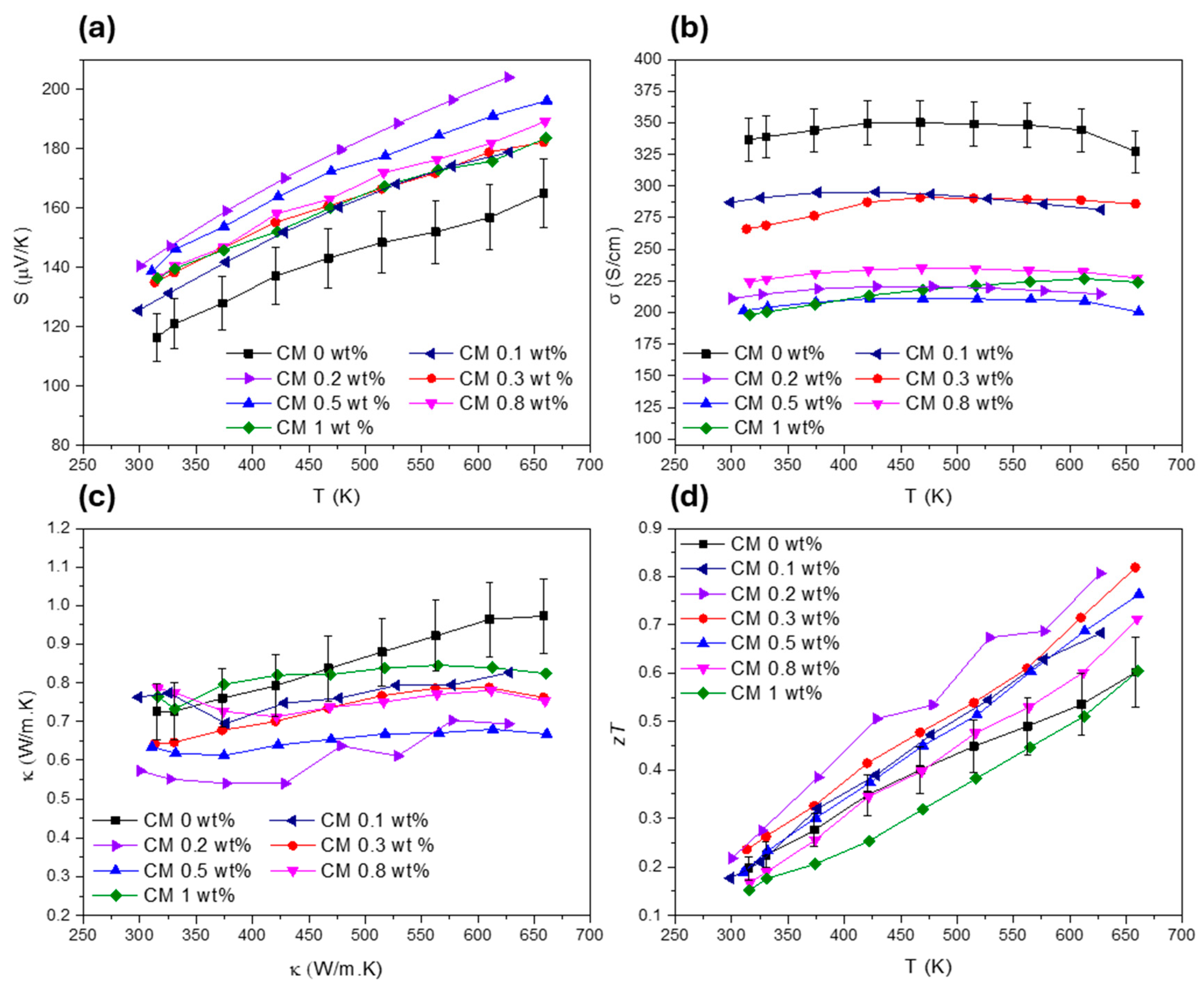
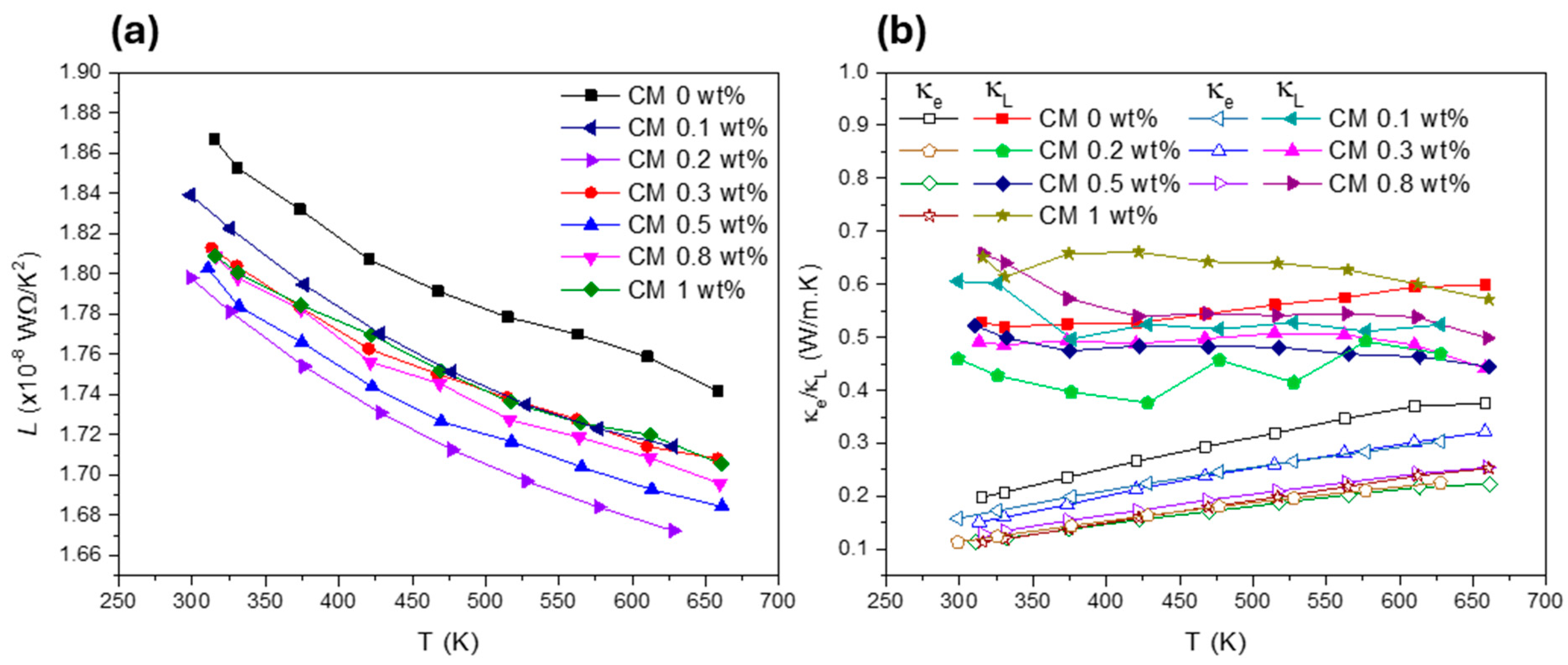
3.2. Transport Properties
4. Discussion
4.1. Lattice Thermal Conductivity Evaluation
4.2. Mobility Evaluation and Overall Performance
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Haras, M.; Skotnicki, T. Thermoelectricity for IoT—A Review. Nano Energy 2018, 54, 461–476. [Google Scholar] [CrossRef]
- dos Santos, A.D.; de Brito, S.C.; Martins, A.V.; Silva, F.F.; Morais, F. Thermoelectric Energy Harvesting on Rotation Machines for Wireless Sensor Network in Industry 4.0. In Proceedings of the 2021 14th IEEE International Conference on Industry Applications, INDUSCON 2021, São Paulo, Brazil, 15–18 August 2021; pp. 694–697. [Google Scholar] [CrossRef]
- Botnar, W.L.; Frederico, G.F. Industry 4.0 in the Electric Sector: Findings from a Systematic Review of the Literature. Electr. J. 2023, 36, 107337. [Google Scholar] [CrossRef]
- Singh, R.; Dogra, S.; Dixit, S.; Vatin, N.I.; Bhardwaj, R.; Sundramoorthy, A.K.; Perera, H.C.S.; Patole, S.P.; Mishra, R.K.; Arya, S. Advancements in Thermoelectric Materials for Efficient Waste Heat Recovery and Renewable Energy Generation. Hybrid Adv. 2024, 5, 100176. [Google Scholar] [CrossRef]
- Tyagi, K.; Gahtori, B.; Kumar, S.; Dhakate, S.R. Advances in Solar Thermoelectric and Photovoltaic-Thermoelectric Hybrid Systems for Power Generation. Sol. Energy 2023, 254, 195–212. [Google Scholar] [CrossRef]
- Twaha, S.; Zhu, J.; Yan, Y.; Li, B. A Comprehensive Review of Thermoelectric Technology: Materials, Applications, Modelling and Performance Improvement. Renew. Sustain. Energy Rev. 2016, 65, 698–726. [Google Scholar] [CrossRef]
- Chen, Y.; Hou, X.; Ma, C.; Dou, Y.; Wu, W. Review of Development Status of Bi2Te3-Based Semiconductor Thermoelectric Power Generation. Adv. Mater. Sci. Eng. 2018, 21, 1210562. [Google Scholar] [CrossRef]
- Ryu, B.; Chung, J.; Kumagai, M.; Mato, T.; Ando, Y.; Gunji, S.; Tanaka, A.; Yana, D.; Fujimoto, M.; Imai, Y.; et al. Best Thermoelectric Efficiency of Ever-Explored Materials. iScience 2023, 26, 106494. [Google Scholar] [CrossRef]
- Hatzikraniotis, E.; Polymeris, G.S.; Kyratsi, T.; Kyratsi, T. Thermal Conductivity in Thermoelectric Materials. In Novel Applications of Piezoelectric and Thermoelectric Materials; Intechopen: Rijeka, Croatia, 2022. [Google Scholar] [CrossRef]
- Mehdizadeh Dehkordi, A.; Zebarjadi, M.; He, J.; Tritt, T.M. Thermoelectric Power Factor: Enhancement Mechanisms and Strategies for Higher Performance Thermoelectric Materials. Mater. Sci. Eng. R Rep. 2015, 97, 1–22. [Google Scholar] [CrossRef]
- Snyder, G.J.; Toberer, E.S. Complex Thermoelectric Materials. Nat. Mater. 2008, 7, 105–114. [Google Scholar] [CrossRef]
- Sun, Y.; Liu, Y.; Li, R.; Li, Y.; Bai, S. Strategies to Improve the Thermoelectric Figure of Merit in Thermoelectric Functional Materials. Front. Chem. 2022, 10, 865281. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Z.; Hou, Y.; Liu, Y.; Hu, L.; Xie, W.; Shi, J.; Wei, J.; Xiong, R. High-Performance Bi0.4Sb1.6Te3 Alloy Prepared by a Low-Cost Method for Wearable Real-Time Power Supply and Local Cooling. Chem. Eng. J. 2024, 481, 148530. [Google Scholar] [CrossRef]
- Wu, X.; Wang, Z.; Liu, Y.; Sun, X.; Xu, Y.; Tian, Y.; Wang, B.; Sang, X.; Shi, J.; Xiong, R. Enhanced Performance of Bi2Te3-Based Thermoelectric Materials by Incorporating Bi2Fe4O9 Magnetic Nanoparticles. J. Alloys Compd. 2022, 904, 163933. [Google Scholar] [CrossRef]
- Li, J.F.; Liu, W.S.; Zhao, L.D.; Zhou, M. High-Performance Nanostructured Thermoelectric Materials. NPG Asia Mater. 2010, 2, 152–158. [Google Scholar] [CrossRef]
- Heremans, J.P.; Dresselhaus, M.S.; Bell, L.E.; Morelli, D.T. When Thermoelectrics Reached the Nanoscale. Nat. Nanotechnol. 2013, 8, 471–473. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Yan, X.; Chen, G.; Ren, Z. Recent Advances in Thermoelectric Nanocomposites. Nano Energy 2012, 1, 42–56. [Google Scholar] [CrossRef]
- Kanatzidis, M.G. Advances in Thermoelectrics: From Single Phases to Hierarchical Nanostructures and Back. MRS Bull. 2015, 40, 687–694. [Google Scholar] [CrossRef]
- Heremans, J.P.; Wiendlocha, B.; Chamoire, A.M. Resonant Levels in Bulk Thermoelectric Semiconductors. Energy Environ. Sci. 2012, 5, 5510–5530. [Google Scholar] [CrossRef]
- Liu, W.D.; Yin, L.C.; Li, L.; Yang, Q.; Wang, D.Z.; Li, M.; Shi, X.L.; Liu, Q.; Bai, Y.; Gentle, I.; et al. Grain Boundary Re-Crystallization and Sub-Nano Regions Leading to High Plateau Figure of Merit for Bi2Te3 Nanoflakes. Energy Environ. Sci. 2023, 16, 5123–5135. [Google Scholar] [CrossRef]
- Biswas, K.; He, J.; Blum, I.D.; Wu, C.I.; Hogan, T.P.; Seidman, D.N.; Dravid, V.P.; Kanatzidis, M.G. High-Performance Bulk Thermoelectrics with All-Scale Hierarchical Architectures. Nature 2012, 489, 414–418. [Google Scholar] [CrossRef]
- Wang, D.Z.; Liu, W.D.; Li, M.; Zheng, K.; Hu, H.; Yin, L.C.; Wang, Y.; Zhu, H.; Shi, X.L.; Yang, X.; et al. Hierarchical Architectural Structures Induce High Performance in N-Type GeTe-Based Thermoelectrics. Adv. Funct. Mater. 2023, 33, 2213040. [Google Scholar] [CrossRef]
- Wang, D.Z.; Liu, W.D.; Mao, Y.; Li, S.; Yin, L.C.; Wu, H.; Li, M.; Wang, Y.; Shi, X.L.; Yang, X.; et al. Decoupling Carrier-Phonon Scattering Boosts the Thermoelectric Performance of n-Type GeTe-Based Materials. J. Am. Chem. Soc. 2024, 146, 1681–1689. [Google Scholar] [CrossRef] [PubMed]
- Hu, Q.X.; Liu, W.D.; Zhang, L.; Sun, W.; Gao, H.; Shi, X.L.; Yang, Y.L.; Liu, Q.; Chen, Z.G. SWCNTs/Ag2Se Film with Superior Bending Resistance and Enhanced Thermoelectric Performance via in Situ Compositing. Chem. Eng. J. 2023, 457, 141024. [Google Scholar] [CrossRef]
- Zhao, L.D.; Lo, S.H.; Zhang, Y.; Sun, H.; Tan, G.; Uher, C.; Wolverton, C.; Dravid, V.P.; Kanatzidis, M.G. Ultralow Thermal Conductivity and High Thermoelectric Figure of Merit in SnSe Crystals. Nature 2014, 508, 373–377. [Google Scholar] [CrossRef] [PubMed]
- Caballero-Calero, O.; Ares, J.R.; Martín-González, M. Environmentally Friendly Thermoelectric Materials: High Performance from Inorganic Components with Low Toxicity and Abundance in the Earth. Adv. Sustain. Syst. 2021, 5, 2100095. [Google Scholar] [CrossRef]
- Lee, K.T.; Lee, D.S.; Chen, W.H.; Lin, Y.L.; Luo, D.; Park, Y.K.; Bandala, A. An Overview of Commercialization and Marketization of Thermoelectric Generators for Low-Temperature Waste Heat Recovery. iScience 2023, 26, 107874. [Google Scholar] [CrossRef] [PubMed]
- Li, K.; Garrison, G.; Zhu, Y.; Horne, R.; Petty, S. Cost Estimation of Thermoelectric Generators. In Proceedings of the PROCEEDINGS, 46th Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 16–18 February 2021; Stanford University: Stanford, CA, USA, 2021; Volume 46. [Google Scholar]
- Olaniyi, E.O.; Prause, G. Investment Analysis of Waste Heat Recovery System Installations on Ships’ Engines. J. Mar. Sci. Eng. 2020, 8, 811. [Google Scholar] [CrossRef]
- Charilaou, K.; Kyratsi, T.; Louca, L.S. Design of an Air-Cooled Thermoelectric Generator System through Modelling and Simulations, for Use in Cement Industries. Mater. Today Proc. 2020, 44, 3516–3524. [Google Scholar] [CrossRef]
- Yazawa, K.; Shakouri, A.; Hendricks, T.J. Thermoelectric Heat Recovery from Glass Melt Processes. Energy 2017, 118, 1035–1043. [Google Scholar] [CrossRef]
- Kuroki, T.; Kabeya, K.; Makino, K.; Kajihara, T.; Kaibe, H.; Hachiuma, H.; Matsuno, H.; Fujibayashi, A. Thermoelectric Generation Using Waste Heat in Steel Works. J. Electron. Mater. 2014, 43, 2405–2410. [Google Scholar] [CrossRef]
- Candolfi, C.; Bouyrie, Y.; Sassi, S.; Dauscher, A.; Lenoir, B. Tetrahedrites: Prospective Novel Thermoelectric Materials. In Thermoelectrics for Power Generation—A Look at Trends in the Technology; IntechOpen: London, UK, 2016; pp. 71–89. [Google Scholar]
- Chetty, R.; Bali, A.; Mallik, R.C. Tetrahedrites as Thermoelectric Materials: An Overview. J. Mater. Chem. C Mater. 2015, 3, 12364–12378. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Lopes, E.B. Towards the Use of Cu–S Based Synthetic Minerals for Thermoelectric Applications. Semiconductors 2019, 53, 1817–1824. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Lopes, E.B.; Monnier, J.; Bourgon, J.; Vaney, J.B.; Piarristeguy, A.; Pradel, A.; Lenoir, B.; Delaizir, G.; Pereira, M.F.C.; et al. Fast and Scalable Preparation of Tetrahedrite for Thermoelectrics via Glass Crystallization. J. Alloys Compd. 2016, 664, 209–217. [Google Scholar] [CrossRef]
- Lu, X.; Morelli, D.T.; Xia, Y.; Ozolins, V. Increasing the Thermoelectric Figure of Merit of Tetrahedrites by Co-Doping with Nickel and Zinc. Chem. Mater. 2015, 27, 408–413. [Google Scholar] [CrossRef]
- Weller, D.P.; Morelli, D.T. Tetrahedrite Thermoelectrics: From Fundamental Science to Facile Synthesis. Front. Electron. Mater. 2022, 2, 913280. [Google Scholar] [CrossRef]
- Shu, Z.; Shen, C.; Lu, A.; Gu, X. Chemical Composition and Crystal Structure of Kenoargentotetrahedrite-(Fe), Ag6Cu4Fe2Sb4S12, from the Bajiazi Pb-Zn Deposit, Liaoning, China. Crystals 2022, 12, 467. [Google Scholar] [CrossRef]
- Jonnson, N.E.; Cnq’ic, J.R.; Rrmsrror, J.D. Crystal Chemistry of Tetrahedrite. Am. Mineral. 1988, 73, 389–397. [Google Scholar]
- Gonçalves, A.P.; Lopes, E.B.; Monnier, J.; Alleno, E.; Godart, C.; Montemor, M.D.F.; Vaney, J.; Lenoir, B. Tetrahedrites for Low Cost and Sustainable Thermoelectrics. Solid State Phenom. 2017, 257, 135–138. [Google Scholar] [CrossRef]
- Yan, Y.; Li, N.; Wang, G.; Xiong, Q.; Fan, L.; Jiang, P.; Lu, X.; Wang, G.; Zhou, X. Achieving High Average Power Factor in Tetrahedrite Cu12Sb4S13 via Regulating Electron-Phonon Coupling Strength. Mater. Today Phys. 2022, 22, 100590. [Google Scholar] [CrossRef]
- Lu, X.; Morelli, D.T. Natural Mineral Tetrahedrite as a Direct Source of Thermoelectric Materials. Phys. Chem. Chem. Phys. 2013, 15, 5762–5766. [Google Scholar] [CrossRef]
- Levinský, P.; Ventrapati, P.K.; Dauscher, A.; Hejtmánek, J.; Candolfi, C.; Lenoir, P.B. Achieving High Thermoelectric Performance in Mixed Natural-Synthetic Tetrahedrites. ChemNanoMat 2022, 8, e202200364. [Google Scholar] [CrossRef]
- Levinsky, P.; Vaney, J.B.; Candolfi, C.; Dauscher, A.; Lenoir, B.; Hejtmánek, J. Electrical, Thermal, and Magnetic Characterization of Natural Tetrahedrites–Tennantites of Different Origin. J. Electron. Mater. 2016, 45, 1351–1357. [Google Scholar] [CrossRef]
- Dismukes, J.P.; Ekstrom, L.; Steigmeier, E.F.; Kudman, I.; Beers, D.S. Thermal and Electrical Properties of Heavily Doped Ge-Si Alloys up to 1300°K. J. Appl. Phys. 1964, 35, 2899–2907. [Google Scholar] [CrossRef]
- Kapera, K.; Koleżyński, A. First-Principles Study of Structural Disorder, Site Preference, Chemical Bonding and Transport Properties of Mg-Doped Tetrahedrite. Comput. Mater. Sci. 2022, 213, 111681. [Google Scholar] [CrossRef]
- Sun, F.; Dong, J.; Dey, S.; Wu, C.; Pan, Y. Enhanced Thermoelectric Performance of Cu12Sb4S13—δ Tetrahedrite via Nickel Doping. Sci. China Mater. 2018, 61, 1209–1217. [Google Scholar] [CrossRef]
- Rout, U.; Tippireddy, S.; Kumari, N.; Dasgupta, T.; Mallik, R.C. Effect of Ag-Addition on the Thermoelectric Properties of Cu12Sb4S13 Tetrahedrite. J. Appl. Phys. 2023, 134, 235102. [Google Scholar] [CrossRef]
- Sun, F.H.; Dong, J.; Tang, H.; Zhuang, H.L.; Li, J.F. ZnO-Nanoparticle-Dispersed Cu11.5Ni0.5Sb4S13−δ Tetrahedrite Composites with Enhanced Thermoelectric Performance. J. Electron. Mater. 2019, 48, 1840–1845. [Google Scholar] [CrossRef]
- Sun, F.H.; Dong, J.; Tang, H.; Shang, P.P.; Zhuang, H.L.; Hu, H.; Wu, C.F.; Pan, Y.; Li, J.F. Enhanced Performance of Thermoelectric Nanocomposites Based on Cu12Sb4S13 Tetrahedrite. Nano Energy 2019, 57, 835–841. [Google Scholar] [CrossRef]
- Hu, H.; Sun, F.H.; Dong, J.; Zhuang, H.L.; Cai, B.; Pei, J.; Li, J.F. Nanostructure Engineering and Performance Enhancement in Fe2O3-Dispersed Cu12Sb4S13 Thermoelectric Composites with Earth-Abundant Elements. ACS Appl. Mater. Interfaces 2020, 12, 17852–17860. [Google Scholar] [CrossRef]
- Slack, G.A. New Materials and Performance Limits for Thermoelectric Cooling. In CRC Handbook for Thermoelectrics; Rowe, D.M., Ed.; CRC: Boca Raton, FL, USA, 1995. [Google Scholar]
- Yang, B.; Wang, Q.; Guo, H.; Yang, X.; Yang, D. Nanoparticle-Modified Thermoelectric Tetrahedrite as an Effective Nucleating Agent for Sodium Acetate Trihydrate Based Phase Change Materials. Mater. Res. Express 2020, 7, 105504. [Google Scholar] [CrossRef]
- Sharief, P.; Dharmaiah, P.; Madavali, B.; Song, J.W.; Lee, J.K.; Lee, J.H.; Hong, S.J. Enhancing the Thermoelectric Properties through Hierarchical Structured Materials Fabricated through Successive Arrangement of Different Microstructure. J. Alloys Compd. 2022, 910, 164803. [Google Scholar] [CrossRef]
- Miler, M.; Mirtič, B. Accuracy and Precision of EDS Analysis for Identification of Metal-Bearing Minerals in Polished and Rough Particle Samples. Geologija 2013, 56, 5–17. [Google Scholar] [CrossRef]
- Thilagashanthi, T.; Gunasekaran, K.; Satyanarayanan, K.S. Microstructural Pore Analysis Using SEM and ImageJ on the Absorption of Treated Coconut Shell Aggregate. J. Clean. Prod. 2021, 324, 129217. [Google Scholar] [CrossRef]
- Haeri, M.; Haeri, M. ImageJ Plugin for Analysis of Porous Scaffolds Used in Tissue Engineering. J. Open Res. Softw. 2015, 3, e1. [Google Scholar] [CrossRef]
- Ohta, H.; Baba, T.; Shibata, H.; Waseda, Y. Evaluation of the Effective Sample Temperature in Thermal Diffusivity Measurements Using the Laser Flash Method. Int. J. Thermophys. 2002, 23, 1659–1668. [Google Scholar] [CrossRef]
- Natali, M.; Torre, L.; Rallini, M. Evaluation of the Thermal Diffusivity of Carbon/Phenolic Composites (CPCs) through Oxy-Acetylene Torch (OAT) Test—Part 1: Experimental Characterization and Preliminary Validation. Polymers 2024, 16, 577. [Google Scholar] [CrossRef]
- Kim, H.S.; Gibbs, Z.M.; Tang, Y.; Wang, H.; Snyder, G.J. Characterization of Lorenz Number with Seebeck Coefficient Measurement. APL Mater. 2015, 3, 041506. [Google Scholar] [CrossRef]
- Wang, H.; Bai, S.; Chen, L.; Cuenat, A.; Joshi, G.; Kleinke, H.; König, J.; Lee, H.W.; Martin, J.; Oh, M.-W.; et al. International Round-Robin Study on Thermoelectric Transport Properties of n-Type Half-Heusler from 300 K to 773 K. Available online: https://www.osti.gov/servlets/purl/1215580 (accessed on 20 October 2024).
- Ali, A.; Chiang, Y.W.; Santos, R.M. X-Ray Diffraction Techniques for Mineral Characterization: A Review for Engineers of the Fundamentals, Applications, and Research Directions. Minerals 2022, 12, 205. [Google Scholar] [CrossRef]
- Holland, T.J.B.; Redfern, S.A.T. Unit Cell Refinement from Powder Diffraction Data: The Use of Regression Diagnostics. Mineral. Mag. 1997, 61, 65–77. [Google Scholar] [CrossRef]
- Hassanzadeh-Tabrizi, S.A. Precise Calculation of Crystallite Size of Nanomaterials: A Review. J. Alloys Compd. 2023, 968, 171914. [Google Scholar] [CrossRef]
- Yang, T.Y.; Wang, Z.Y.; Yan, X.; Wang, C.Y.; Zhang, Y.X.; Ge, Z.H.; Feng, J. Excellent Thermoelectric Performance Realized in Copper Sulfide Magnetic Nanocomposites Via Modified Solid States Reaction. Adv. Sci. 2025, 2409494. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Yang, X.; Yan, X.; Shi, T.E.; Feng, J.; Ge, Z.H. One Stone Three Birds: Natural Mineral Enhancing Thermoelectric Properties in Cu2Se-Based Composites. J. Alloys Compd. 2024, 1002, 175222. [Google Scholar] [CrossRef]
- Ai, X.; Hou, D.; Liu, X.; Gu, S.; Wang, L.; Jiang, W. Enhanced Thermoelectric Performance of PbTe-Based Nanocomposites through Element Doping and SiC Nanoparticles Dispersion. Scr. Mater. 2020, 179, 86–91. [Google Scholar] [CrossRef]
- Ghosh, S.; Tippireddy, S.; Shankar, G.; Karati, A.; Rogl, G.; Rogl, P.; Bauer, E.; Malladi, S.R.K.; Murty, B.S.; Suwas, S.; et al. InSb Nanoparticles Dispersion in Yb-Filled Co4Sb12 Improves the Thermoelectric Performance. J. Alloys Compd. 2021, 880, 160532. [Google Scholar] [CrossRef]
- Pi, J.; Lee, G.; Kim, I. Thermal Stability, Mechanical Properties and Thermoelectric Performance of Cu11TrSb4S13 (Tr = Mn, Fe, Co, Ni, Cu, and Zn). J. Electron. Mater. 2019, 13, 2710–2718. [Google Scholar] [CrossRef]
- Coelho, R.; Symeou, E.; Kyratsi, T.; Gonçalves, A. Tetrahedrite Sintering Conditions: The Cu11Mn1Sb4S13 Case. Electron. Mater. 2020, 49, 5077–5083. [Google Scholar] [CrossRef]
- Chetty, R.; Prem Kumar, D.S.; Rogl, G.; Rogl, P.; Bauer, E.; Michor, H.; Suwas, S.; Puchegger, S.; Giester, G.; Mallik, R.C. Thermoelectric Properties of a Mn Substituted Synthetic Tetrahedrite. Phys. Chem. Chem. Phys. 2015, 17, 1716–1727. [Google Scholar] [CrossRef]
- Kim, S.Y.; Lee, G.E.; Kim, I.H. Charge Transport and Thermoelectric Properties of Mn-Doped Tetrahedrites Cu12-xMnxSb4S13. J. Korean Inst. Met. Mater. 2021, 59, 329–335. [Google Scholar] [CrossRef]
- Daniel, J.E.; Jesby, C.M.; Plass, K.E.; Anderson, M.E. Multinary Tetrahedrite (Cu12−x−YMxNySb4S13) Nanoparticles: Tailoring Thermal and Optical Properties with Copper-Site Dopants. Chem. Mater. 2024, 36, 3246–3258. [Google Scholar] [CrossRef]
- Humphry-Baker, S.A.; Schuh, C.A. Suppression of Grain Growth in Nanocrystalline Bi2Te3 through Oxide Particle Dispersions. J. Appl. Phys. 2014, 116, 173505. [Google Scholar] [CrossRef]
- Khodaei, M.; Yaghobizadeh, O.; Baharvandi, H.R.; Shahraki, A.A.; Mohammadi, H. The Effect of Nano-TiO2 Additions on the Densification and Mechanical Properties of SiC-Matrix Composite. Ceram. Int. 2020, 46, 6477–6483. [Google Scholar] [CrossRef]
- Kinzer, B.; Chandran, R.B. A Phase-Field Study on the Effects of Nanoparticles on Solidification and Grain Growth. JOM 2022, 76, 496–509. [Google Scholar] [CrossRef]
- Weygand, D.; Bréchet, Y.; Lépinoux, J. Inhibition of Grain Growth by Particle Distribution: Effect of Spatial Heterogeneities and of Particle Strength Dispersion. Mater. Sci. Eng. A 2000, 292, 34–39. [Google Scholar] [CrossRef]
- Disha, S.A.; Sahadat Hossain, M.; Habib, M.L.; Ahmed, S. Calculation of Crystallite Sizes of Pure and Metals Doped Hydroxyapatite Engaging Scherrer Method, Halder-Wagner Method, Williamson-Hall Model, and Size-Strain Plot. Results Mater. 2024, 21, 100496. [Google Scholar] [CrossRef]
- Gonçalves, A.P.; Lopes, E.B.; Montemor, M.F.; Monnier, J. Bertrand Lenoir Oxidation Studies of Cu12Sb3.9Bi0.1S10Se3 Tetrahedrite. Electron. Mater. 2018, 47, 2880–2889. [Google Scholar] [CrossRef]
- Zazakowny, K.; Kosonowski, A.; Lis, A.; Cherniushok, O.; Parashchuk, T.; Tobola, J.; Wojciechowski, K.T. Phase Analysis and Thermoelectric Properties of Cu-Rich Tetrahedrite Prepared by Solvothermal Synthesis. Materials 2022, 15, 849. [Google Scholar] [CrossRef]
- Lis, A.; Zazakowny, K.; Cherniushok, O.; Tobola, J.; Gajewska, M.; Parashchuk, T.; Wojciechowski, K.T. Nanostructured Cu12+xSb4S13 Tetrahedrites Prepared by Solvothermal Synthesis in 1-(2-Aminoethyl)Piperazine for Efficient Thermal Energy Harvesting. J. Alloys Compd. 2024, 977, 173337. [Google Scholar] [CrossRef]
- Kambale, K.R.; Mahajan, A.; Butee, S.P. Effect of Grain Size on the Properties of Ceramics. Metal. Powder Report. 2019, 74, 130–136. [Google Scholar] [CrossRef]
- Kaur, S.; Arora, M.; Kumar, S.; Malhi, P.S.; Singh, M.; Singh, A. Agreement in Experimental and Theoretically Obtained Electrocaloric Effect in Optimized Bi3+ doped PbZr0.52Ti0.48O3 material. J. Adv. Dielectr. 2022, 12, 2250003. [Google Scholar] [CrossRef]
- Krustok, J.; Raadik, T.; Kaupmees, R.; Ghisani, F.; Timmo, K.; Altosaar, M.; Mikli, V.; Grossberg, M. Broad-Band Photoluminescence of Donor-Acceptor Pairs in Tetrahedrite Cu10Cd2Sb4S13 microcrystals. J. Phys. D Appl. Phys. 2021, 54, 105102. [Google Scholar] [CrossRef]
- Kharbish, S.; Libowitzky, E.; Beran, A. The Effect of As-Sb Substitution in the Raman Spectra of Tetrahedrite-Tennantite and Pyrargyrite-Proustite Solid Solutions. Eur. J. Mineral. 2007, 19, 567–574. [Google Scholar] [CrossRef]
- McClary, S.A.; Balow, R.B.; Agrawal, R. Role of Annealing Atmosphere on the Crystal Structure and Composition of Tetrahedrite-Tennantite Alloy Nanoparticles. J. Mater. Chem. C Mater. 2018, 6, 10538–10546. [Google Scholar] [CrossRef]
- Apopei, A.I.; Damian, G.; Buzgar, N.; Buzatu, A.; Andráš, P.; Milovska, S. The Determination of the Sb/As Content in Natural Tetrahedrite–Tennantite and Bournonite–Seligmannite Solid Solution Series by Raman Spectroscopy. Miner. Mag. 2017, 81, 1439–1456. [Google Scholar] [CrossRef]
- Škácha, P.; Sejkora, J.; Plášil, J. Selenide Mineralization in the Příbram Uranium and Base-Metal District (Czech Republic). Minerals 2017, 7, 91. [Google Scholar] [CrossRef]
- Blanco, É.; Afanasiev, P.; Berhault, G.; Uzio, D.; Loridant, S. Resonance Raman Spectroscopy as a Probe of the Crystallite Size of MoS2 Nanoparticles. Comptes Rendus Chim. 2016, 19, 1310–1314. [Google Scholar] [CrossRef]
- Arora, A.K.; Rajalakshmi, M.; Ravindran, T.R.; Sivasubramanian, V. Raman Spectroscopy of Optical Phonon Confinement in Nanostructured Materials. J. Raman Spectrosc. 2007, 38, 604–617. [Google Scholar] [CrossRef]
- Sahoo, S.; Dhara, S.; Mahadevan, S.; Arora, A.K. Phonon Confinement in Stressed Silicon Nanocluster. J. Nanosci. Nanotechnol. 2009, 9, 5604–5607. [Google Scholar] [CrossRef][Green Version]
- Shi, W.; Zhang, X.; Li, X.L.; Qiao, X.F.; Wu, J.B.; Zhang, J.; Tan, P.H. Phonon Confinement Effect in Two-Dimensional Nanocrystallites of Monolayer MoS2 to Probe Phonon Dispersion Trends Away from Brillouin-Zone Center. Chin. Phys. Lett. 2016, 33, 057801. [Google Scholar] [CrossRef]
- Balandin, A.; Wang, K.L. Effect of Phonon Confinement on the Thermoelectric Figure of Merit of Quantum Wells. J. Appl. Phys. 1998, 84, 6149–6153. [Google Scholar] [CrossRef]
- Gouadec, G.; Bellot-Gurlet, L.; Baron, D.; Colomban, P. Raman Mapping for the Investigation of Nano-Phased Materials. In Springer Series in Optical Sciences; Springer: Berlin/Heidelberg, Germany, 2012; Volume 168, pp. 85–118. ISBN 9783642282515. [Google Scholar]
- Satyala, N.; Vashaee, D. The Effect of Crystallite Size on Thermoelectric Properties of Bulk Nanostructured Magnesium Silicide (Mg2Si) Compounds. Appl. Phys. Lett. 2012, 100, 073107. [Google Scholar] [CrossRef]
- Aishwarya, K.; Maruthasalamoorthy, S.; Thenmozhi, R.; Mani, J.; Anbalagan, G.; Nirmala, R.; Navaneethan, M.; Rangaswamy, N. Impact of Crystallite Size and Phase Boundaries on Magnetic and Thermoelectric Properties of Cu-Added BiFeO3. ACS Omega 2024, 9, 35088–35099. [Google Scholar] [CrossRef]
- Zebarjadi, M.; Esfarjani, K.; Shakouri, A.; Bian, Z.; Bahk, J.H.; Zeng, G.; Bowers, J.; Lu, H.; Zide, J.; Gossard, A. Effect of Nanoparticles on Electron and Thermoelectric Transport. J. Electron. Mater. 2009, 38, 954–959. [Google Scholar] [CrossRef]
- Callaway, J.; von Bakyer, H.C. Effect of Point Imperfections on Lattice Thermal Conductivity. Phys. Rev. 1960, 120, 954. [Google Scholar] [CrossRef]
- Schrade, M.; Finstad, T.G. Using the Callaway Model to Deduce Relevant Phonon Scattering Processes: The Importance of Phonon Dispersion. Phys. Status Solidi B Basic. Res. 2018, 255, 1800208. [Google Scholar] [CrossRef]
- Bjerg, L.; Iversen, B.B.; Madsen, G.K.H. Modeling the Thermal Conductivities of the Zinc Antimonides ZnSb and Zn4Sb3. Phys. Rev. B Condens. Matter Mater. Phys. 2014, 89, 024304. [Google Scholar] [CrossRef]
- Snyder, G.J.; Snyder, A.H.; Wood, M.; Gurunathan, R.; Snyder, B.H.; Niu, C. Weighted Mobility. Adv. Mater. 2020, 32, 2001537. [Google Scholar] [CrossRef]
- Coelho, R.; Lopes, E.B.; Gonçalves, A.P. Protective Coatings for Cu11Mn1Sb4S13 and Cu10.5Ni1.5Sb4S13 Tetrahedrites. J. Electron. Mater. 2021, 50, 467–477. [Google Scholar] [CrossRef]






| Sample# | a (Å) | Average Crystallite Size—D (nm) | |
|---|---|---|---|
| CM 0 wt% | |||
| CM 0.1 wt% | |||
| CM 0.2 wt% | |||
| CM 0.3 wt% | |||
| CM 0.5 wt% | |||
| CM 0.8 wt% | |||
| CM 1 wt% |
| #Sample | Nominal Composition | Analyzed Composition | Detected Secondary Phases |
|---|---|---|---|
| #CM 0 | Cu11Mn1Sb4S13 | Cu11.5±1.4Mn0.9±0.1Sb3.1±0.4S13.5±1.6 | Cu2.2±0.3Sb1.5±0.2S4.2±0.5 |
| #CM 0.1 | Cu11Mn1Sb4S13 | Cu10.4±1.3Mn1.1±0.1Sb2.9±0.3S14.6±1.8 | Cu1.1±0.1Sb0.7±0.1S2.2±0.3 |
| #CM 0.2 | Cu11Mn1Sb4S13 | Cu10.7±1.3Mn1.0±0.1Sb2.9±0.3S14.4±1.7 | Cu1.1±0.1Sb0.7±0.1S2.2±0.3 |
| #CM 0.3 | Cu11Mn1Sb4S13 | Cu11.7±1.4Mn0.9±0.1Sb2.7±0.3S13.7±1.6 | Cu1.1±0.1Sb0.8±0.1S2.1±0.3 |
| #Sample | Nominal Composition | Analyzed Composition | Detected Secondary Phases |
|---|---|---|---|
| #CM 0.5 | Cu11Mn1Sb4S13 | Cu11.7±1.4Mn1.0±0.1Sb3.0±0.4S13.3±1.6 | Cu1.1±0.1Sb0.8±0.1S2.1±0.3 |
| #CM 0.8 | Cu11Mn1Sb4S13 | Cu11.7±1.4Mn0.9±0.1Sb2.9±0.4S13.4±1.6 | Cu1.2±0.1Sb0.7±0.1S2.1±0.2Sb0.9±0.1O1.8±0.2 |
| #CM 1 | Cu11Mn1Sb4S13 | Cu11.2±1.3Mn1.0±0.1Sb2.8±0.3S14.0±1.7 | Cu1.2±0.1Sb0.7±0.1S2.1±0.3 |
| #NPs Powder | MoS2 | Mo1.1±0.1S1.9±0.2 | - |
| #Sample | Experimental Density (g/cm3) | Green Density | SEM Porosity (%) |
|---|---|---|---|
| CM 0 wt% | 97.24% | ||
| CM 0.1 wt% | 95.44% | ||
| CM 0.2 wt% | 94.93% | ||
| CM 0.3 wt% | 96.92% | ||
| CM 0.5 wt% | 96.45% | ||
| CM 0.8 wt% | 96.17% | ||
| CM 1 wt% | 96.59% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Coelho, R.; Moço, D.; Sá, A.I.d.; Luz, P.P.d.; Neves, F.; Cerqueira, M.d.F.; Lopes, E.B.; Brito, F.P.; Mangelis, P.; Kyratsi, T.; et al. Tetrahedrite Nanocomposites for High Performance Thermoelectrics. Nanomaterials 2025, 15, 351. https://doi.org/10.3390/nano15050351
Coelho R, Moço D, Sá AId, Luz PPd, Neves F, Cerqueira MdF, Lopes EB, Brito FP, Mangelis P, Kyratsi T, et al. Tetrahedrite Nanocomposites for High Performance Thermoelectrics. Nanomaterials. 2025; 15(5):351. https://doi.org/10.3390/nano15050351
Chicago/Turabian StyleCoelho, Rodrigo, Duarte Moço, Ana I. de Sá, Paulo P. da Luz, Filipe Neves, Maria de Fátima Cerqueira, Elsa B. Lopes, Francisco P. Brito, Panagiotis Mangelis, Theodora Kyratsi, and et al. 2025. "Tetrahedrite Nanocomposites for High Performance Thermoelectrics" Nanomaterials 15, no. 5: 351. https://doi.org/10.3390/nano15050351
APA StyleCoelho, R., Moço, D., Sá, A. I. d., Luz, P. P. d., Neves, F., Cerqueira, M. d. F., Lopes, E. B., Brito, F. P., Mangelis, P., Kyratsi, T., & Gonçalves, A. P. (2025). Tetrahedrite Nanocomposites for High Performance Thermoelectrics. Nanomaterials, 15(5), 351. https://doi.org/10.3390/nano15050351









