Synthesis and Optimization of Ni-Based Nano Metal–Organic Frameworks as a Superior Electrode Material for Supercapacitor
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
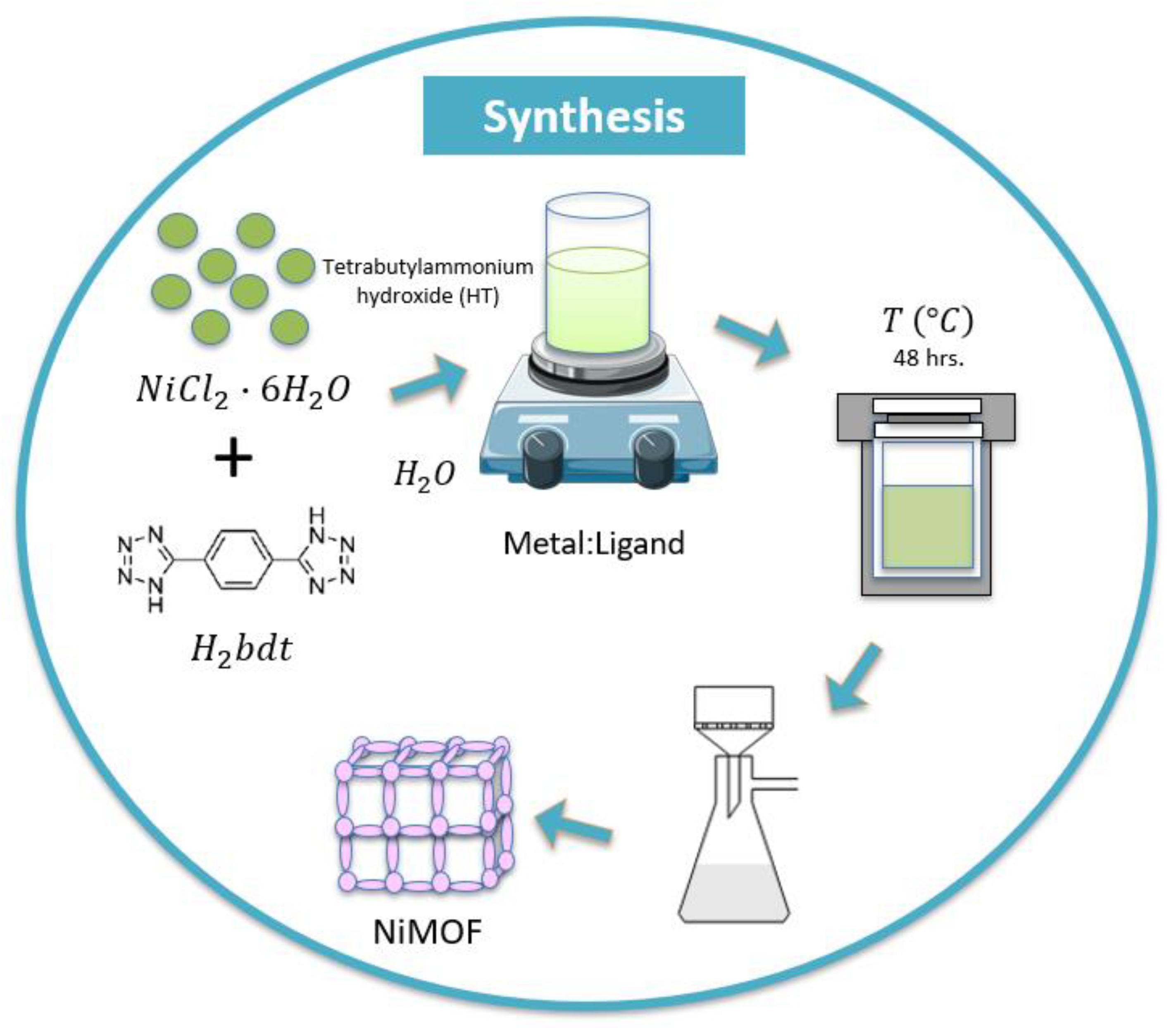
2.2. Synthesis Methods
2.2.1. Synthesis of Ligand
2.2.2. Synthesis of NiMOF
2.3. Structural, Microstructural, and Spectral Characterization
2.4. Electrochemical Characterization
2.4.1. Electrode Preparation
2.4.2. The Standard Three-Electrode Configuration
3. Results and Discussion
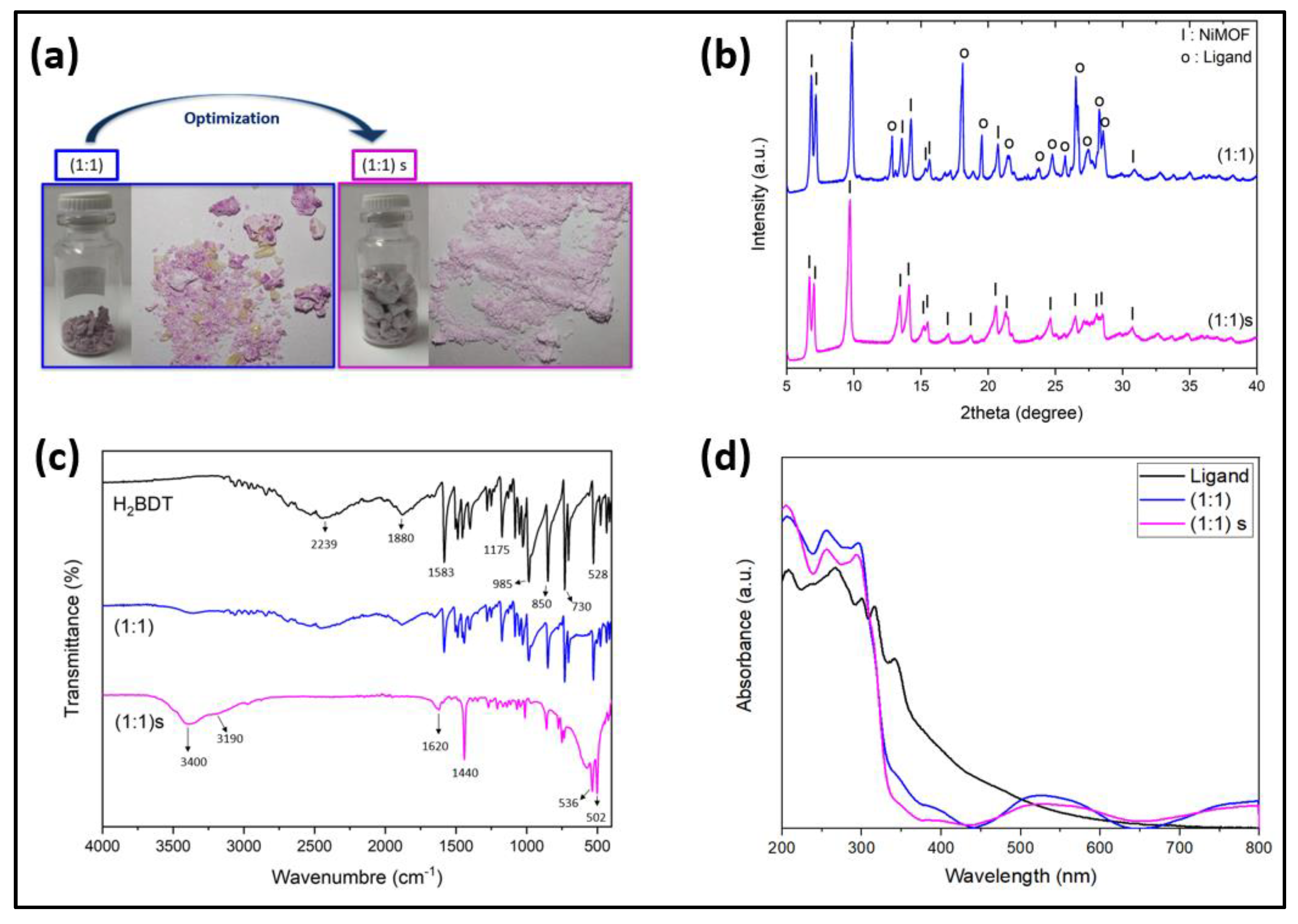
3.1. Characterization of Ligand
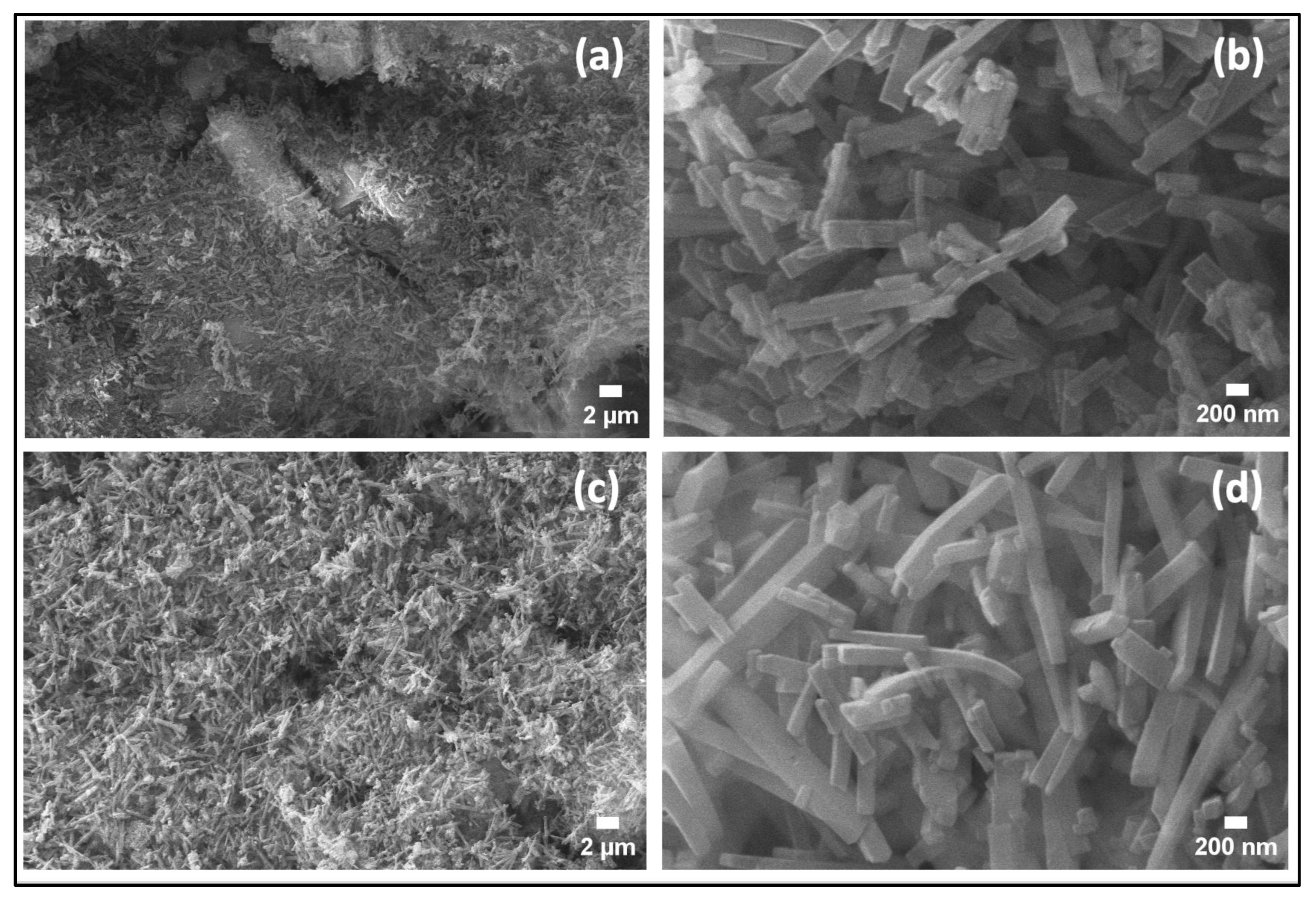
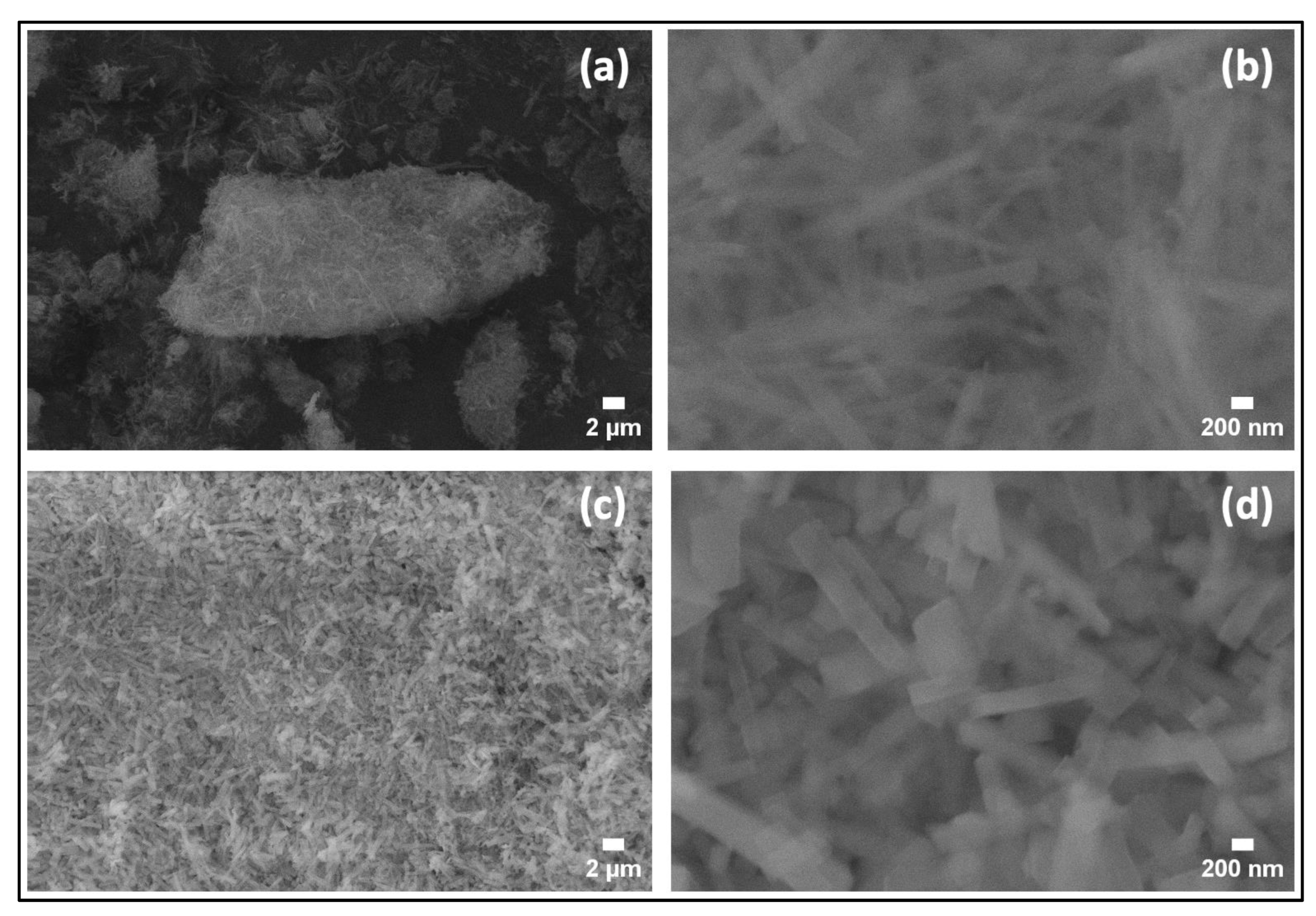
3.2. Structural Microstructural Characterization of Synthesized NiMOFs
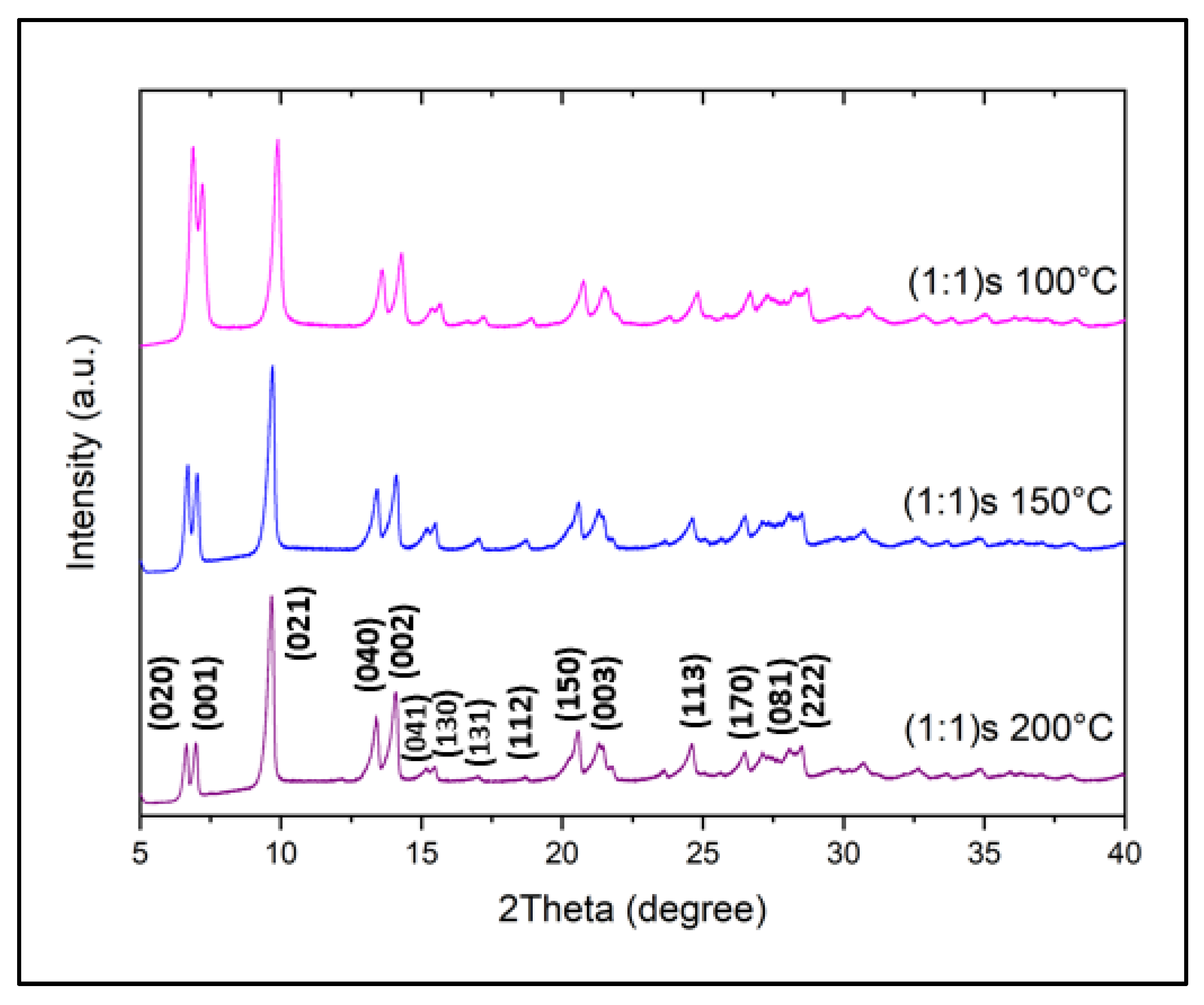
3.3. Effect of Temperature
3.4. Results Dielectric Spectroscopy
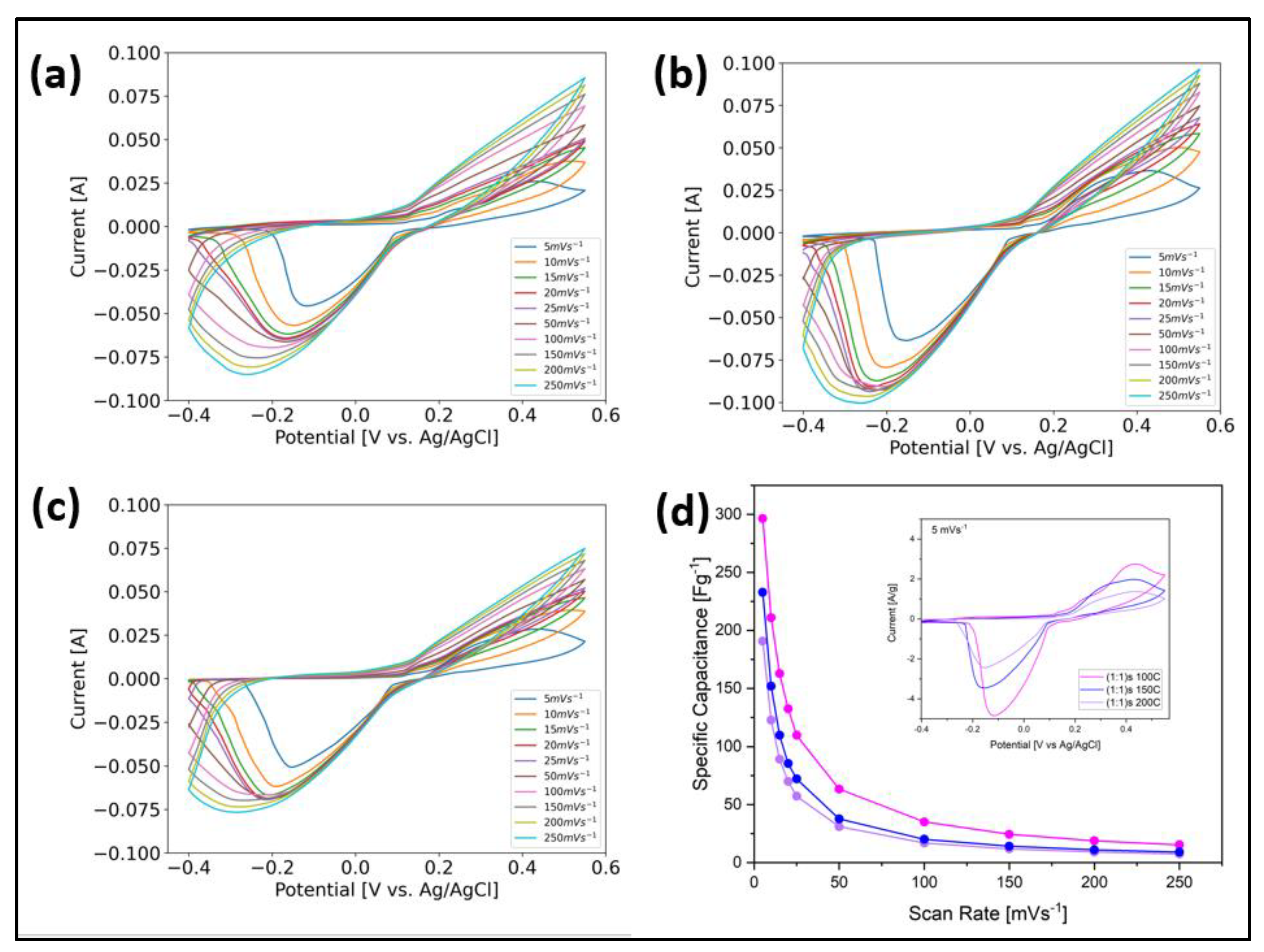
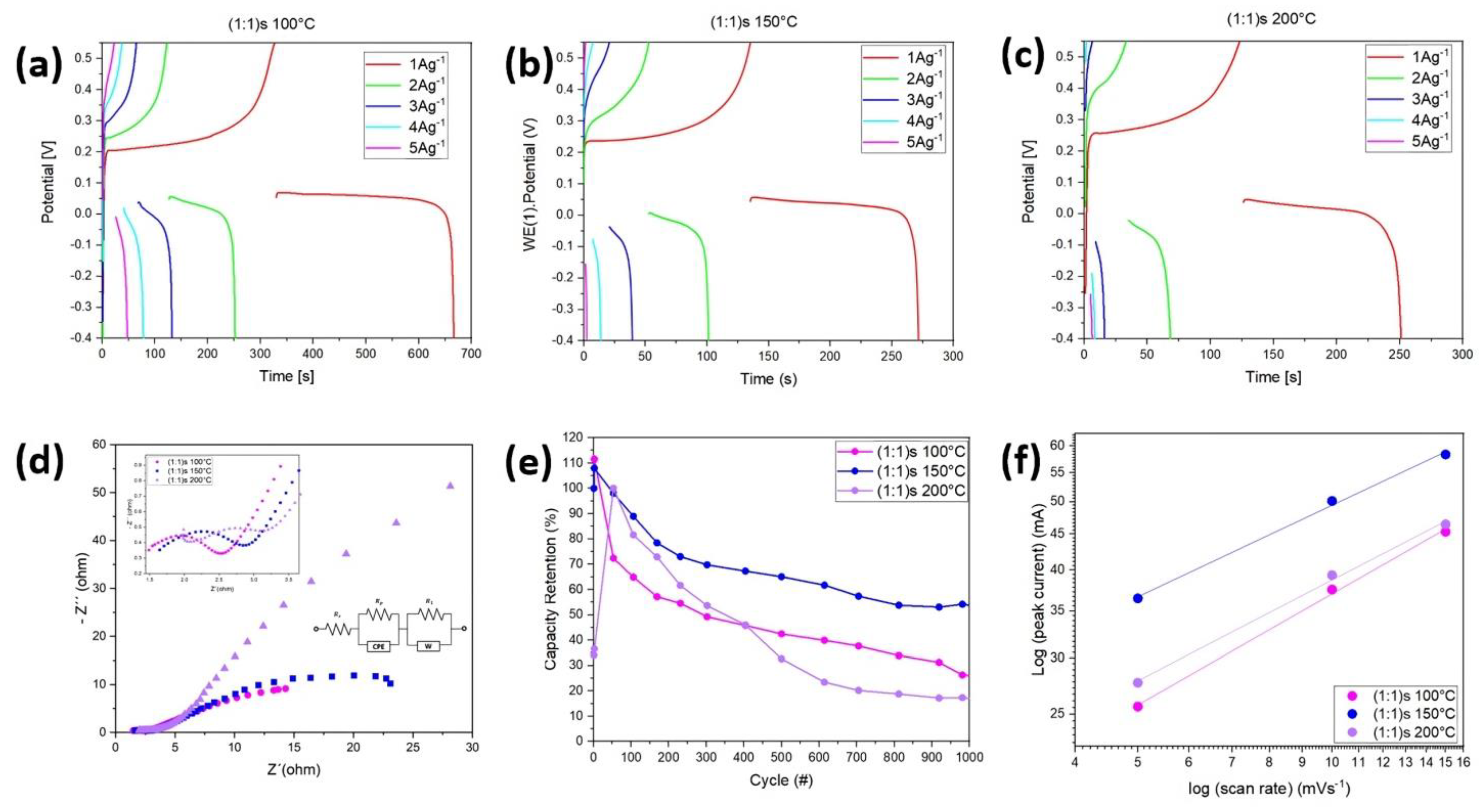
3.5. Results Electrochemical Characterization
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yaghi, O.M.; Li, H. Hydrothermal Synthesis of a Metal-Organic Framework Containing Large Rectangular Channels. J. Am. Chem. Soc. 1995, 117, 10401–10402. [Google Scholar] [CrossRef]
- Taddei, M.; Petit, C. Engineering metal–organic frameworks for adsorption-based gas separations: From process to atomic scale. Mol. Syst. Des. Eng. (R. Soc. Chem.) 2021, 6, 841–875. [Google Scholar] [CrossRef]
- Bavykina, A.; Kolobov, N.; Il Son Khan, J.; Bau, A.; Gascon, A.; Ramirez, J. Metal–Organic Frameworks in Heterogeneous Catalysis: Recent Progress, New Trends, and Future Perspectives. Chem. Rev. 2020, 120, 8468–8535. [Google Scholar] [CrossRef]
- Yin, H.-Q.; Yin, X.-B. Metal–Organic Frameworks with Multiple Luminescence Emissions: Designs and Applications. Acc. Chem. Res. 2020, 53, 485–495. [Google Scholar] [CrossRef]
- Yang, J.; Yang, Y. Metal–Organic Frameworks for Biomedical Applications. Small 2020, 16, 1906846. [Google Scholar] [CrossRef]
- Lawson, H.D.; Walton, S.P.; Chan, C. Metal–Organic Frameworks for Drug Delivery: A Design Perspective. ACS Appl. Mater. Interfaces 2021, 13, 7004–7020. [Google Scholar] [CrossRef]
- Chi-Durán, I.; Enríquez, J.; Manquian, C.; Wrighton-Araneda, K.; Cañon-Mancisidor, W.; Venegas-Yazig, D.; Herrera, F.; Dinesh Pratap, S. pH-Controlled Assembly of 3D and 2D Zinc-Based Metal-Organic Frameworks with Tetrazole Ligands. ACS Omega 2018, 3, 801–807. [Google Scholar] [CrossRef] [PubMed]
- Jia, M.; Xiong, W.; Yang, Z.; Cao, J.; Zhang, Y.; Xiang, Y.; Xu, H.; Song, P.; Xu, Z. Metal-organic frameworks and their derivatives-modified photoelectrodes for photoelectrochemical applications. Coord. Chem. Rev. 2021, 434, 213780. [Google Scholar] [CrossRef]
- Calbo, J.; Golomb, M.J.; Walsh, A. Redox-active metal–organic frameworks for energy conversion and storage. J. Mater. Chem. A (R. Soc. Chem. ) 2019, 7, 16571–16597. [Google Scholar] [CrossRef]
- Raza, H.; Bai, S.; Cheng, J.; Majumder, S.; Zhu, H.; Liu, Q.; Chen, G. Li-S Bateries: Challenges, Achievements and Opportunities. Electrochem. Energy Rev. 2023, 6, 29. [Google Scholar] [CrossRef]
- Li, C.; Zhang, L.; Chen, J.; Li, X.; Sun, J.; Zhu, J.; Wang, X.; Fu, Y.Y. Recent development and applications of electrical conductive MOFs. Nanoscale 2021, 13, 485–509. [Google Scholar] [CrossRef] [PubMed]
- Ma, T.; Li, H.; Ma, J.-G.; Cheng, P. Application of MOF-based materials in electrochemical sensing. Dalton Trans. 2020, 49, 17121–17129. [Google Scholar] [CrossRef] [PubMed]
- Raza, H.; Cheng, J.; Lin, C.; Majumder, S.; Zheng, G.; Chen, G. High-entropy stabilized oxides derived via a low-temperature template route for high-performance lithium-surfur batteries. EcoMat 2023, 5, e1234. [Google Scholar] [CrossRef]
- Sahoo, S.; Kumar, R.; Dhakal, G.; Shim, J. Recent advances in synthesis of metal-organic frameworks (MOFs)-derived metal oxides and its composites for electrochemical energy storage applications. J. Energy Storage 2023, 74, 109427. [Google Scholar] [CrossRef]
- Kuyuldar, S.; Genna, D.T.; Burda, C. On the potential for nanoscale metal–organic frameworks for energy applications. J. Mater. Chem. A (R. Soc. Chem. ) 2019, 7, 21545–21576. [Google Scholar] [CrossRef]
- Wang, K.-B.; Bi, R.; Wang, Z.-K.; Chu, Y.; Wu, H. Metal–organic frameworks with different spatial dimensions for supercapacitors. New J. Chem. (R. Soc. Chem. ) 2020, 44, 3147–3167. [Google Scholar] [CrossRef]
- Choi, K.M.; Jeong, H.M.; Park, J.H.; Zhang, Y.-B.; Kang, J.K.; Yaghi, O.M. Supercapacitors of Nanocrystalline Metal–Organic Frameworks. ACS Nano 2014, 8, 7451–7457. [Google Scholar] [CrossRef]
- Sheberla, D.; Bachman, J.C.; Elias, J.S.; Sun, C.-J.; Shao-Horn, Y.; Dincă, M. Conductive MOF electrodes for stable supercapacitors with high areal capacitance. Nat. Mater 2017, 16, 220–224. [Google Scholar] [CrossRef]
- Ouellette, W.; Darling, K.; Prosvirin, A.; Whitenack, K.; Dunbar, K.R.; Zubieta, J. Syntheses, structural characterization and properties of transition metal complexes of 5,5′-(1,4-phenylene)bis(1H-tetrazole) (H2bdt), 5′,5′′-(1,1′-biphenyl)-4,4′-diylbis(1H-tetrazole) (H2dbdt) and 5,5′,5′′-(1,3,5-phenylene)tris(1H- tetrazole) (H3btt). Dalton Trans. 2011, 40, 12288–12300. [Google Scholar] [CrossRef]
- Qiao, C.; Wei, Q.; Xia, Z.; Liang, J.; Chen, S. Pb(II) and Mn(II) Coordination Compounds Involving 5,5′-(1,4-Phenylene)bis(1H-tetrazole):Synthesis, Characterization, and Effect on Thermal Decomposition of Ammonium Perchlorate. Chin. J. Chem. 2011, 29, 724–730. [Google Scholar] [CrossRef]
- He, X.; An, B.L.; Li, M.X. 5,5´-(p-Phenylene)di-1H-tetrazole. Acta Crystallogr. Sect. E 2008, 64, o40. [Google Scholar] [CrossRef] [PubMed]
- Kim, B.K.; Sy, S.; Yu, A.; Zhang, J. Electrochemical Supercapacitors for Energy Storage and Conversion. In Handbook of Clean Energy Systems; Ltd John Wiley & Sons: Hoboken, NJ, USA, 2015; pp. 1–25. [Google Scholar]
- Vivas, L.; Jara, A.; Garcia-Garfido, J.M.; Serafini, D.; Singh, D.P. Facile Synthesis and Optimization of CrOOH/rGO-Based Electrode Material for a Highly Efficient Supercapacitor Device. ACS Omega 2022, 7, 42446–42455. [Google Scholar] [CrossRef] [PubMed]
- Chand, P.; Sharma, S. Supercapacitor and electrochemical techniques: A brief review. Results Chem. 2023, 5, 100885. [Google Scholar]
- Larkin, P. Infrared and Raman Spectroscopy, Principles and Spectral Interpretarion; Elsevier Science: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Vanaraj, R.; Vinodh, R.; Periyasamy, T.; Madhappan, S.; Babu, C.M.; Asrafali, S.P.; Haldhar, R.; Raorane, C.J.; Hwang, H.; Kim, H.-J.; et al. Capacitance Enhancement of Metal–Organic Framework (MOF) Materials by Their Morphology and Structural Formation. Energy Fuels 2022, 36, 4978–4991. [Google Scholar] [CrossRef]
- Kamath, T.N.; Ramesh; Vishnu, P. Synthesis of nickel hydroxide: Effect of precipitation conditions on phase selectivity and structural disorder. J. Power Sources 2006, 156, 655–661. [Google Scholar]
- Cotton, F.A.; Wilkinson, G. Advanced Inorganic Chemistry; A Wiley-Interscience Publication, John Willy & Sons, Inc.: New York, NY, USA; London, UK; Sydney, Australia; Toronto, ON, Canada; Geneva, Switzerland, 1972. [Google Scholar]
- Bonneviot, L.; Legendre, O.; Kermarec, M.; Daniéle, O.; Che, M. Characterization by UV-vis-NIR reflectance spectroscopy of the exchange sites of nickel on silica. J. Colloid Interface Sci. 1990, 134, 534–547. [Google Scholar] [CrossRef]
- Liu, W.; Migdisov, A.; Williams-Jones, A. The stability of aqueous nickel(II) chloride complexes in hydrothermal solutions: Results of UV–Visible spectroscopic experiments. Geochim. Et Cosmochim. Acta 2012, 94, 276–290. [Google Scholar] [CrossRef]
- Lee, K.J.; McCarthy, B.D.; Dempsey, J.L. On Decomposition, Degradation, and Voltammetric Deviation: The Electrochemist’s Field Guide to Identifying Precatalyst Transformation. Chem. Soc. Rev. 2019, 48, 2927–2945. [Google Scholar] [CrossRef]
- Schiavi, P.G.; Altimari, P.; Marzolo, F.; Rubino, A.; Zanoni, R.; Pagnanelli, F. Optimizing the structure of Ni–Ni(OH)2/NiO core-shell nanowire electrodes for application in pseudocapacitors: The influence of metallic core, Ni(OH)2/NiO ratio and nanowire length. J. Alloys Compd. 2021, 856, 157718. [Google Scholar] [CrossRef]
- Manikandan, M.R.; Cai, K.P.; Hu, Y.D.; Li, C.L.; Zheng, Y.P.; Liang, Y.F.; Song, H.R.; Shang, M.Y.; Shi, X.N.; Zhang, J.X.; et al. Influence of hydrothermal reaction time on the supercapacitor performance of Ni-MOF nanostructures. Appl. Phys. A 2021, 127, 421. [Google Scholar] [CrossRef]
- Akin, M.; Zhou, X. Recent advances in solid-state supercapacitors: From emerging materials to advanced applications. Int. J. Energy Res. 2022, 46, 10389–10452. [Google Scholar] [CrossRef]
- Brousse, T.; Bélanger, D.; Long, J.W. To Be or Not Be Pseudocapacitive? J. Electrochem. Soc. 2015, 165, A5185. [Google Scholar] [CrossRef]
- Gao, S.; Sui, Y.; Wei, F.; Qi, J.; Meng, Q.; He, Y. Facile synthesis of cuboid Ni-MOF for high-performance supercapacitors. J. Mater. Sci. 2018, 53, 6807–6818. [Google Scholar] [CrossRef]
- Shi, L.; Yang, W.; Zha, X.; Zeng, Q.; Tu, D.; Li, Y.; Yang, Y.; Xu, J.; Chen, F. In situ deposition of conducting polymer on metal organic frameworks for high performance hybrid supercapacitor electrode materials. J. Energy Storage 2022, 52, 104729. [Google Scholar] [CrossRef]
- Xu, J.; Yang, C.; Xue, Y.; Wang, C.; Cao, J.; Chen, Z. Facile synthesis of novel metal-organic nickel hydroxide nanorods for high performance supercapacitor. Electrochim. Acta 2016, 211, 595–602. [Google Scholar] [CrossRef]
- Jiang, D.; Wei, C.; Zhu, Z.; Xu, X.; Lu, M.; Wang, G. Preparation of Flower-like Nickel-Based Bimetallic Organic Framework Electrodes for High-Efficiency Hybrid Supercapacitors. Crystals 2021, 11, 1425. [Google Scholar] [CrossRef]
- Zhang, W.; Yin, H.; Yu, Z.; Jia, X.; Liang, J.; Li, G.; Li, Y.; Wang, K. Facile Synthesis of 4,4´-biphenyl Dicarboxylic Acid-Based Nickel Metal Organic Frameworks with a Tunable Pore Size towards High-Performance Supercapacitors. Nanomaterials 2022, 12, 2062. [Google Scholar] [CrossRef] [PubMed]
- Yan, Y.; Gu, P.; Zheng, S.; Zheng, M.; Pang, H.; Xue, H. Facile synthesis of an accordion-like Ni-MOF superstructure for high-performance flexible supercapacitors. J. Mater. Chem. A 2016, 4, 19078–19085. [Google Scholar] [CrossRef]
- Fox, M.A.; Akaba, R. Curve Crossing in the Cyclic Voltammetric Oxidation of 2-Phenylnorbornene. Evidence for an ECE Reaction Pathway. J. Am. Chem. Soc. 1983, 105, 3460–3463. [Google Scholar] [CrossRef]

| Sample | [pF] | [MΩ] | [µΩ cm−1] |
|---|---|---|---|
| (1:1) | 17.553 | 155.359 | 0.102 |
| (1:1)s 100°C | 39.402 | 53.538 | 0.322 |
| (1:1)s 150°C | 27.739 | 40.559 | 0.484 |
| (1:1)s 200°C | 12.454 | 196.122 | 0.101 |
| MOF | [F/g] | Window Potential [V] | Electrolyte | Ref. |
|---|---|---|---|---|
| Hierarchical NiMOFs | 1498.6 | 0.0–0.6 | 6M KOH | [33] |
| ) | 804 | 0.0–0.6 | 2M KOH | [36] |
| NiMOF (BTC) | 993 | −0.1–0.5 | 6M KOH | [37] |
| Ni-based MOF nanorods | 1698 | 0.0–0.6 | 6M KOH | [38] |
| NiMOF (PTA) | 579 | 0.0–0.6 | 2M KOH | [39] |
| nano Ni-BPDC-MOF | 488 | 0.0–0.6 | 3M KOH | [40] |
| 988 | 0.0–0.6 | 3M KOH | [41] | |
| NiMOF (1:1)s 100 °C | 606.62 | −0.4–0.55 | 2M KOH | This Work |
| NiMOF (1:1)s 150 °C | 307.33 | |||
| NiMOF (1:1)s 200 °C | 287.42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Manquian, C.; Navarrete, A.; Vivas, L.; Troncoso, L.; Singh, D.P. Synthesis and Optimization of Ni-Based Nano Metal–Organic Frameworks as a Superior Electrode Material for Supercapacitor. Nanomaterials 2024, 14, 353. https://doi.org/10.3390/nano14040353
Manquian C, Navarrete A, Vivas L, Troncoso L, Singh DP. Synthesis and Optimization of Ni-Based Nano Metal–Organic Frameworks as a Superior Electrode Material for Supercapacitor. Nanomaterials. 2024; 14(4):353. https://doi.org/10.3390/nano14040353
Chicago/Turabian StyleManquian, Carolina, Alberto Navarrete, Leonardo Vivas, Loreto Troncoso, and Dinesh Pratap Singh. 2024. "Synthesis and Optimization of Ni-Based Nano Metal–Organic Frameworks as a Superior Electrode Material for Supercapacitor" Nanomaterials 14, no. 4: 353. https://doi.org/10.3390/nano14040353
APA StyleManquian, C., Navarrete, A., Vivas, L., Troncoso, L., & Singh, D. P. (2024). Synthesis and Optimization of Ni-Based Nano Metal–Organic Frameworks as a Superior Electrode Material for Supercapacitor. Nanomaterials, 14(4), 353. https://doi.org/10.3390/nano14040353








