The Impact of Cavities in Different Thermal Applications of Nanofluids: A Review
Abstract
1. Introduction
1.1. Buongiorno Nanofluid Model
- The flow is incompressible;
- There is no chemical reaction between them;
- The effect of external force is neglected;
- The dilute mixture is less than one;
- There is no effect of viscous dissipation;
- No consideration is given to radiative heat transfer;
- Local thermal equilibrium between nanoparticles and clear fluid.
1.2. Tiwari and Das Nanofluid Model
- The fluid is laminar, Newtonian, incompressible, and unsteady.
- The nanoparticles are considered to have a homogeneous shape and size.
- The fluid phase and nanoparticles are thermally balanced and flow at the same rate.
- In comparison to other types of heat transmission, radiation heat transfer between sides is insignificant.
- Excluding for the density changes in the buoyancy force, which is dependent on the Boussinesq approximation, the thermophysical parameters of the nanofluid are considered to remain constant.
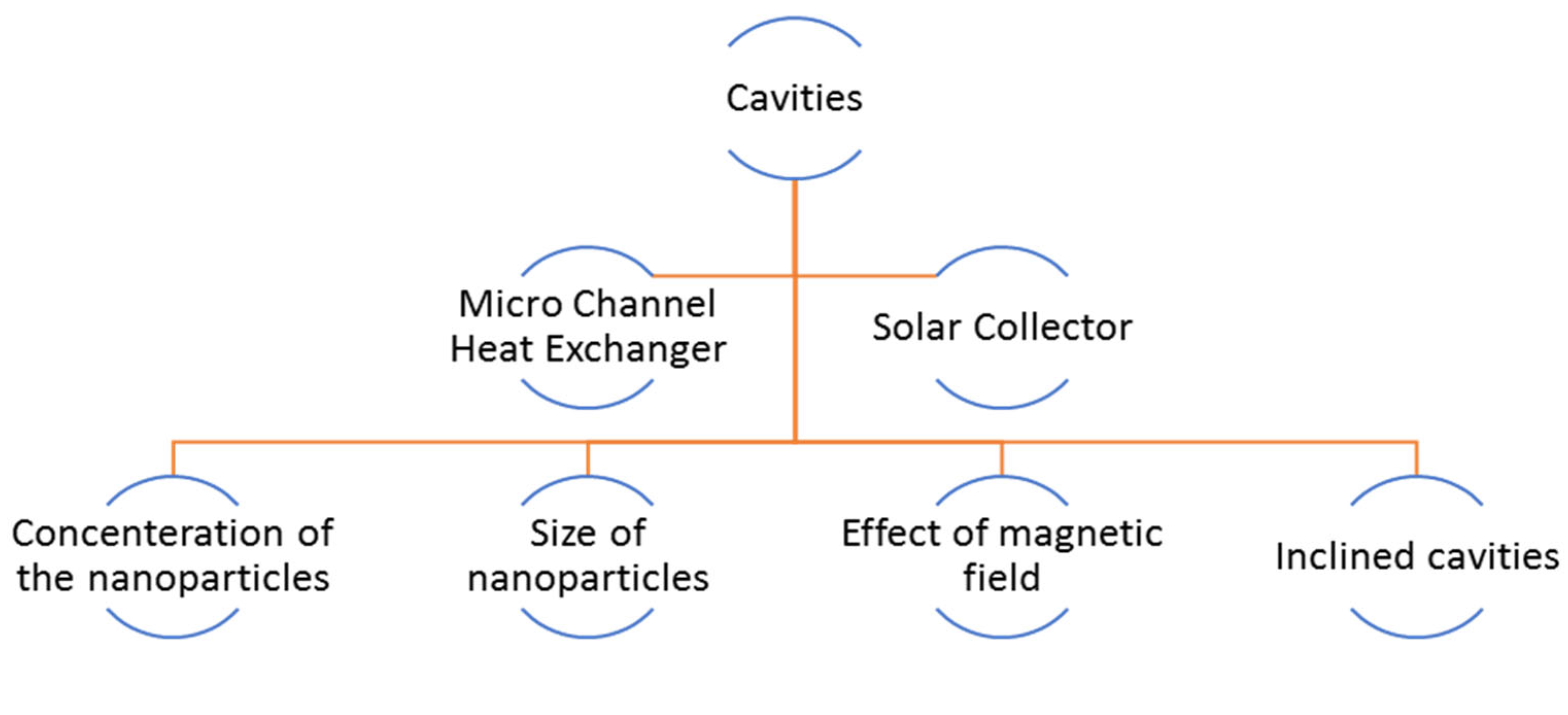
2. The Role of Cavities in Nanofluid Transport
2.1. Effect of the Nanoparticle Concentration in Cavities
2.2. Effect of the Nanoparticles in Cavities
2.3. Effect of the Cavities’ Inclination Angle
2.4. Effect of the Heater and Cooler Inside Cavities
2.5. Effect of the Magnetic Field in Cavities
3. Effect of Cavities in Microchannel Heat Exchangers
4. Effect of Cavities in Solar Collectors
5. Future Recommendations
- The internal nanofluids’ ability to generate heat convection and entropy depends on the cavity’s aspect ratio, so it is suggested that more research be focused on using inclined shape cavities in artificial neural networks.
- According to the literature, it is observed that the size of nanoparticles is very important in improving the heat-transfer rate; for smaller sizes, greater heat transfer is attained. After reviewing the many research articles it is recommended that a size of nanoparticles between 10 and 50 nm is more stable in base fluids in cavities at the specified temperature gradient. As a result, the most significant heat transmission may be obtained by making the diameter of the nanoparticles smaller in the cavity. It is suggested that research should focus on different shapes of nanoparticles smaller than 10 nm, with the same design of cavities in microchannel heat exchangers and solar collectors to improve performance.
- In the literature, it is reported that microchannel heat exchangers with circular cavities provide the highest performance. Even though circular cavities provide the best performance in micro heat exchangers, microchannel heat exchangers with square cavities have more applications in related industries, so different shapes of cavities, such as cubical, hexagonal, and conical, should be used in micro heat exchangers in the future.
- In solar collectors, different cavities such as square, rectangular, and triangular cavities are used, and the best results are observed with the use of these cavities, but there is less research focused on conical, hexa-conical, and other novel optimal cavity geometries, so it is recommended that in future research, more focus be given to these types of geometries.
6. Conclusions
- (a)
- In heat exchangers and solar collectors, the use of a specific shape and design of the cavity provides better results. The shape of the cavities depends on how they will be used, so it is important for thermal systems to have the right cavities.
- (b)
- In the literature, it is reported that L-shaped cavities are used in the cooling systems of nuclear, chemical, and electronic components and give suitable results.
- (c)
- It has been seen that the size of the channels in heat exchangers changes depending on what they are used for. In land-based systems, the smaller the channel, the better the results. This is because the smaller the channel, the smaller the hydraulic diameter, which is what makes the heat transfer work so well. This causes a big drop in pressure. When figuring out how to use microchannel heat exchangers in space, we can make the channels bigger to make them more effective. This is because the pressure drop across the system needs to be kept as low as possible to obtain the best heat-transfer rate.
- (d)
- Different shapes of cavities including square, circular, trapezoidal, rectangular, and others are used in microchannel heat exchangers. From the literature, it is observed that the circular cavities provide the best performance because they provide a high heat-transfer rate with low pumping power and are most efficient at low Reynolds numbers.
- (e)
- The use of nanofluids has been found to improve thermal performance in all the cavities studied. According to the experimental data, nanofluid use has been proven to be a dependable solution for enhancing thermal efficiency. The average thermal efficiency improvement using nanofluids is 12.90% for the hemispherical cavity, 5.84% for the cubical cavity, and 1.44% for the cylindrical cavity.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Rayleigh number | |
| Bejan number | |
| Thermal conductivity ratio of solid wall to pure fluid | |
| Velocity | |
| Nanoparticle volume fraction | |
| Density of the fluid | |
| Temperature of the fluid | |
| Stress tensor | |
| Brownian diffusion coefficient | |
| Thermal diffusion coefficient | |
| Pressure | |
| Specific heat | |
| Thermal conductivity | |
| Density of the nanoparticles | |
| Nanoparticle specific heat | |
| Time | |
| Dynamic viscosity | |
| Nanoparticle diameter | |
| Volumetric thermal expansion coefficient | |
| Boltzmann’s constant | |
| Laplacian operator | |
| Rectangular coordinate | |
| Momentum eddy diffusivity | |
| Energy eddy diffusivity | |
| Particle eddy diffusivity | |
| Friction factor | |
| Reynolds number | |
| Prandtl number | |
| Laminar sublayer | |
| Nusselt number | |
| Average total Nusselt number | |
| Solid block | |
| Richardson number | |
| Hartmann number | |
| Radius of the cylinder | |
| Nanoparticle | |
| Lewis number |
References
- Sheremet, M.; Pop, I.; Mahian, O. Natural convection in an inclined cavity with time-periodic temperature boundary conditions using nanofluids: Application in solar collectors. Int. J. Heat Mass Transf. 2018, 116, 751–761. [Google Scholar] [CrossRef]
- Afzal, F.; Mehmood, A.; Al Ghour, S.; Zafar, M.; Sakidin, H.; Gul, S. Characterization of Bipolar Vague Soft-Open Sets. J. Funct. Spaces 2022, 2022, 5964872. [Google Scholar] [CrossRef]
- Hussain, A.; Muthuvalu, M.S.; Faye, I.; Zafar, M.; Inc, M.; Afzal, F.; Iqbal, M.S. Numerical investigation of treated brain glioma model using a two-stage successive over-relaxation method. Comput. Biol. Med. 2023, 153, 106429. [Google Scholar] [CrossRef] [PubMed]
- Hussain, A.; Faye, I.; Muthuvalu, M.S.; Boon, T.T. Least Square QR Decomposition Method for Solving the Inverse Problem in Functional Near Infra-Red Spectroscopy. In Proceedings of the 2021 IEEE 19th Student Conference on Research and Development (SCOReD), Kota Kinabalu, Malaysia, 23–25 November 2021; pp. 362–366. [Google Scholar] [CrossRef]
- Abro, G.E.M.; Kakar, G.K.; Kumar, R.; Zafar, M. Maximum power point tracking using perturb & observe algorithm for hybrid energy generation. J. Indep. Stud. Res. Comput. 2020, 18. [Google Scholar] [CrossRef]
- Hussain, A.; Muthuvalu, M.S.; Faye, I.; Ali, M.K.M.; Lebelo, R.S. Numerical Study of Glioma Growth Model with Treatment Using the Two-Stage Gauss-Seidel Method. J. Phys. Conf. Ser. 2018, 1123, 012040. [Google Scholar] [CrossRef]
- Hussain, A.; Faye, I.; Muthuvalu, M.S. Performance analysis of successive over relaxation method for solving glioma growth model. AIP Conf. Proc. 2016, 1787, 020001. [Google Scholar] [CrossRef]
- Hussain, A.; Muthuvalu, M.S.; Faye, I. Numerical simulation of brain tumor growth model using two-stage Gauss-Seidel method. J. Fundam. Appl. Sci. 2018, 9, 227. [Google Scholar] [CrossRef]
- Wang, L.; Huang, C.; Yang, X.; Chai, Z.; Shi, B. Effects of temperature-dependent properties on natural convection of power-law nanofluids in rectangular cavities with sinusoidal temperature distribution. Int. J. Heat Mass Transf. 2018, 128, 688–699. [Google Scholar] [CrossRef]
- Mansour, M.; Mohamed, R.; Abd-Elaziz, M.; Ahmed, S.E. Numerical simulation of mixed convection flows in a square lid-driven cavity partially heated from below using nanofluid. Int. Commun. Heat Mass Transf. 2010, 37, 1504–1512. [Google Scholar] [CrossRef]
- Mansour, M.A.; Ahmed, S.E. Mixed Convection in Double Lid-Driven Enclosures Filled with Al2O3–Water Nanofluid. J. Thermophys. Heat Transf. 2013, 27, 707–718. [Google Scholar] [CrossRef]
- Azizul, F.M.; Alsabery, A.I.; Hashim, I. Heatlines visualisation of mixed convection flow in a wavy heated cavity filled with nanofluids and having an inner solid block. Int. J. Mech. Sci. 2020, 175, 105529. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Combined effects of double rotating cones and magnetic field on the mixed convection of nanofluid in a porous 3D U-bend. Int. Commun. Heat Mass Transf. 2020, 116, 104703. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F.; Afrand, M. Shape effects of TEG mounted ventilated cavities with alumina-water nanofluids on the performance features by using artificial neural networks. Eng. Anal. Bound. Elem. 2022, 140, 79–97. [Google Scholar] [CrossRef]
- Wu, S.-Y.; Xiao, L.; Cao, Y.; Li, Y.-R. Convection heat loss from cavity receiver in parabolic dish solar thermal power system: A review. Sol. Energy 2010, 84, 1342–1355. [Google Scholar] [CrossRef]
- Loni, R.; Asli-Ardeh, E.A.; Ghobadian, B.; Ahmadi, M.; Bellos, E. GMDH modeling and experimental investigation of thermal performance enhancement of hemispherical cavity receiver using MWCNT/oil nanofluid. Sol. Energy 2018, 171, 790–803. [Google Scholar] [CrossRef]
- Ye, M.; Du, J.; Wang, J.; Chen, L.; Varbanov, P.S.; Klemeš, J.J. Investigation on thermal performance of nanofluids in a microchannel with fan-shaped cavities and oval pin fins. Energy 2022, 260, 125000. [Google Scholar] [CrossRef]
- Li, F.; Zhu, W.; He, H. Field synergy analysis on flow and heat transfer characteristics of nanofluid in microchannel with non-uniform cavities configuration. Int. J. Heat Mass Transf. 2019, 144, 118617. [Google Scholar] [CrossRef]
- Al-Yaari, A.; Ching, D.L.C.; Sakidin, H.; Muthuvalu, M.S.; Zafar, M.; Alyousifi, Y.; Saeed, A.A.H.; Bilad, M.R. Thermophysical Properties of Nanofluid in Two-Phase Fluid Flow through a Porous Rectangular Medium for Enhanced Oil Recovery. Nanomaterials 2022, 12, 1011. [Google Scholar] [CrossRef]
- Zafar, M.; Sakidin, H.; Dzulkarnain, I.; Afzal, F. Numerical Investigations of Nano-fluid Flow in Square Porous Cavity: Buongiorno’s Mathematical Model. In Proceedings of the 6th International Conference on Fundamental and Applied Sciences; Springer: Berlin/Heidelberg, Germany, 2021; pp. 739–748. [Google Scholar] [CrossRef]
- Al-Yaari, A.; Sakidin, H.; Zainuddin, N.; Hashim, I. Unsteady Nanofluid Flow Over Exponentially Stretching Sheet with Vertical Throughflow. In Proceedings of the 6th International Conference on Fundamental and Applied Sciences; Springer: Berlin/Heidelberg, Germany, 2021; pp. 595–609. [Google Scholar]
- Al-Yaari, A.; Ching, D.L.C.; Sakidin, H.; Muthuvalu, M.S.; Zafar, M.; Alyousifi, Y.; Saeed, A.A.H.; Haruna, A. Optimum Volume Fraction and Inlet Temperature of an Ideal Nanoparticle for Enhanced Oil Recovery by Nanofluid Flooding in a Porous Medium. Processes 2023, 11, 401. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective Transport in Nanofluids. J. Heat Transfer. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Vajravelu, K. Nanofluid flow and heat transfer in a cavity with variable magnetic field. Appl. Math. Comput. 2016, 298, 272–282. [Google Scholar] [CrossRef]
- Armaghani, T.; Rashad, A.; Vahidifar, O.; Mishra, S.; Chamkha, A. Effects of discrete heat source location on heat transfer and entropy generation of nanofluid in an open inclined L-shaped cavity. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 1363–1377. [Google Scholar] [CrossRef]
- Rahman, M.; Alam, M.; Al-Salti, N.; Eltayeb, I. Hydromagnetic natural convective heat transfer flow in an isosceles trian-gular cavity filled with nanofluid using two-component nonhomogeneous model. Int. J. Therm. Sci. 2016, 107, 272–288. [Google Scholar] [CrossRef]
- Ebrahimi, D.; Yousefzadeh, S.; Akbari, O.A.; Montazerifar, F.; Rozati, S.A.; Nakhjavani, S.; Safaei, M.R. Mixed convection heat transfer of a nanofluid in a closed elbow-shaped cavity (CESC). J. Therm. Anal. Calorim. 2021, 144, 2295–2316. [Google Scholar] [CrossRef]
- Miroshnichenko, I.V.; Sheremet, M.A.; Oztop, H.F.; Al-Salem, K. MHD natural convection in a partially open trapezoidal cavity filled with a nanofluid. Int. J. Mech. Sci. 2016, 119, 294–302. [Google Scholar] [CrossRef]
- Safaei, M.R.; Karimipour, A.; Abdollahi, A.; Nguyen, T.K. The investigation of thermal radiation and free convection heat transfer mechanisms of nanofluid inside a shallow cavity by lattice Boltzmann method. Phys. A Stat. Mech. Its Appl. 2018, 509, 515–535. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Trîmbiţaş, R.; Groşan, T.; Pop, I. Natural convection of an alumina-water nanofluid inside an inclined wavy-walled cavity with a non-uniform heating using Tiwari and Das’ nanofluid model. Appl. Math. Mech. 2018, 39, 1425–1436. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, I.; Shenoy, A. Unsteady free convection in a porous open wavy cavity filled with a nanofluid using Buongiorno’s mathematical model. Int. Commun. Heat Mass Transf. 2015, 67, 66–72. [Google Scholar] [CrossRef]
- Sheremet, M.; Cimpean, D.; Pop, I. Free convection in a partially heated wavy porous cavity filled with a nanofluid under the effects of Brownian diffusion and thermophoresis. Appl. Therm. Eng. 2017, 113, 413–418. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Fluid-solid interaction of elastic-step type corrugation effects on the mixed convection of nanofluid in a vented cavity with magnetic field. Int. J. Mech. Sci. 2019, 152, 185–197. [Google Scholar] [CrossRef]
- Sheremet, M.; Oztop, H.; Pop, I. MHD natural convection in an inclined wavy cavity with corner heater filled with a nanofluid. J. Magn. Magn. Mater. 2016, 416, 37–47. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Mehryan, S.; Shafee, A.; Sheremet, M.A. Variable magnetic forces impact on magnetizable hybrid nanofluid heat transfer through a circular cavity. J. Mol. Liq. 2018, 277, 388–396. [Google Scholar] [CrossRef]
- Santra, A.K.; Sen, S.; Chakraborty, N. Study of heat transfer augmentation in a differentially heated square cavity using copper–water nanofluid. Int. J. Therm. Sci. 2008, 47, 1113–1122. [Google Scholar] [CrossRef]
- Hwang, K.S.; Lee, J.-H.; Jang, S.P. Buoyancy-driven heat transfer of water-based Al2O3 nanofluids in a rectangular cavity. Int. J. Heat Mass Transf. 2007, 50, 4003–4010. [Google Scholar] [CrossRef]
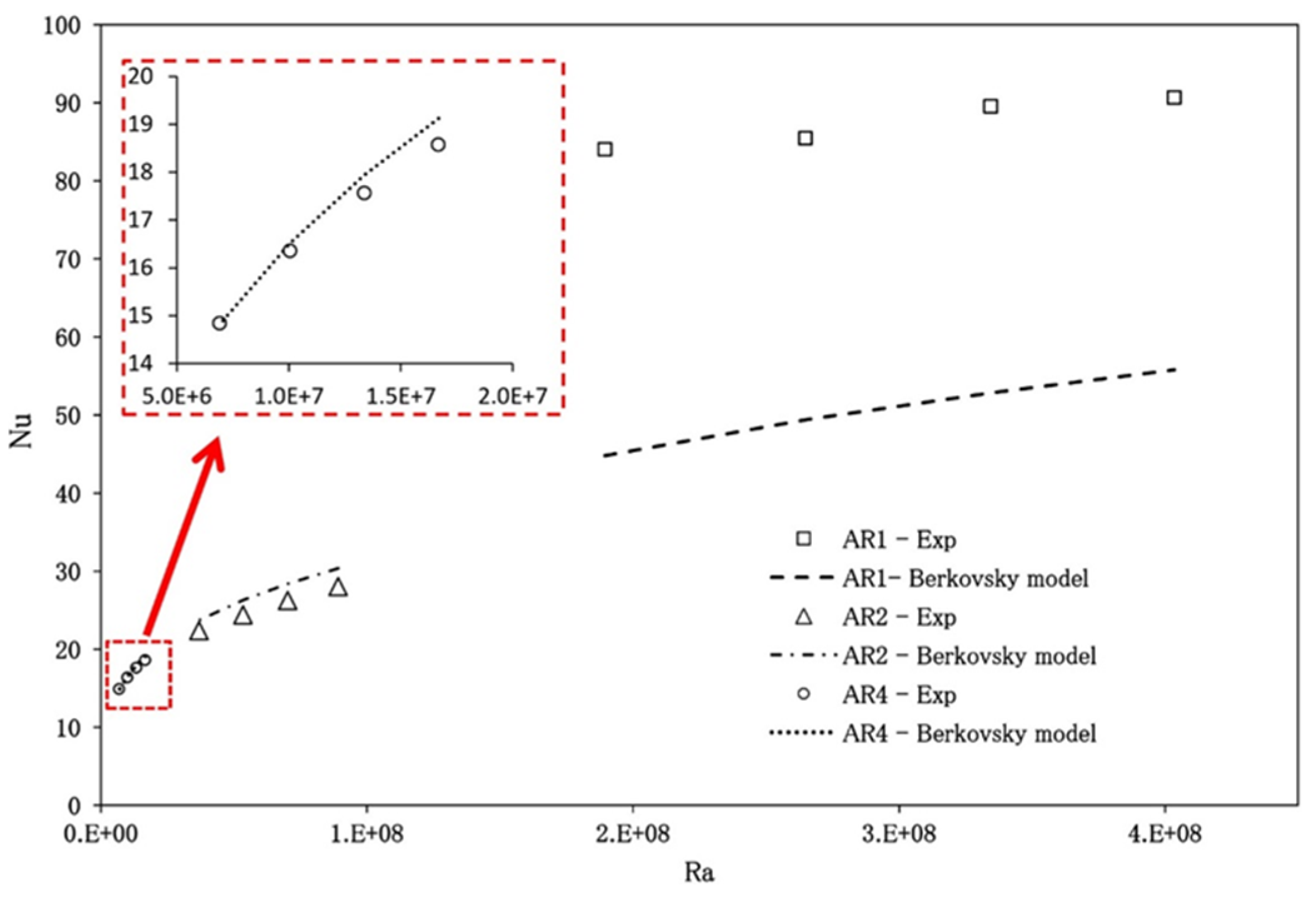
- Solomon, A.B.; van Rooyen, J.; Rencken, M.; Sharifpur, M.; Meyer, J.P. Experimental study on the influence of the aspect ratio of square cavity on natural convection heat transfer with Al2O3/Water nanofluids. Int. Commun. Heat Mass Transf. 2017, 88, 254–261. [Google Scholar] [CrossRef]
- Solomon, A.B.; Sharifpur, M.; Ottermann, T.; Grobler, C.; Joubert, M.; Meyer, J.P. Natural convection enhancement in a porous cavity with Al2O3-Ethylene glycol/water nanofluids. Int. J. Heat Mass Transf. 2017, 108, 1324–1334. [Google Scholar] [CrossRef]
- Iachachene, F.; Haddad, Z.; Oztop, H.F.; Abu-Nada, E. Melting of phase change materials in a trapezoidal cavity: Orientation and nanoparticles effects. J. Mol. Liq. 2019, 292, 110592. [Google Scholar] [CrossRef]
- Baïri, A. Experimental study on enhancement of free convective heat transfer in a tilted hemispherical enclosure by using Water-ZnO nanofluid saturated porous materials. Appl. Therm. Eng. 2019, 148, 992–998. [Google Scholar] [CrossRef]
- Heris, S.Z.; Pour, M.B.; Mahian, O.; Wongwises, S. A comparative experimental study on the natural convection heat transfer of different metal oxide nanopowders suspended in turbine oil inside an inclined cavity. Int. J. Heat Mass Transf. 2014, 73, 231–238. [Google Scholar] [CrossRef]
- Motlagh, S.Y.; Soltanipour, H. Natural convection of Al2O3-water nanofluid in an inclined cavity using Buongiorno’s two-phase model. Int. J. Therm. Sci. 2017, 111, 310–320. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Ismael, M.A.; Chamkha, A.J.; Hashim, I. Mixed convection of Al2O3-water nanofluid in a double lid-driven square cavity with a solid inner insert using Buongiorno’s two-phase model. Int. J. Heat Mass Transf. 2018, 119, 939–961. [Google Scholar] [CrossRef]
- Alsabery, A.; Sheremet, M.; Chamkha, A.; Hashim, I. Conjugate natural convection of Al2O3–water nanofluid in a square cavity with a concentric solid insert using Buongiorno’s two-phase model. Int. J. Mech. Sci. 2018, 136, 200–219. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Gedik, E.; Chamkha, A.J.; Hashim, I. Effects of two-phase nanofluid model and localized heat source/sink on natural convection in a square cavity with a solid circular cylinder. Comput. Methods Appl. Mech. Eng. 2018, 346, 952–981. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Grosan, T.; Pop, I. Natural Convection and Entropy Generation in a Square Cavity with Variable Temperature Side Walls Filled with a Nanofluid: Buongiorno’s Mathematical Model. Entropy 2017, 19, 337. [Google Scholar] [CrossRef]
- Garoosi, F.; Talebi, F. Numerical analysis of conjugate natural and mixed convection heat transfer of nanofluids in a square cavity using the two-phase method. Adv. Powder Technol. 2017, 28, 1668–1695. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Gedik, E.; Chamkha, A.J.; Hashim, I. Impacts of heated rotating inner cylinder and two-phase nanofluid model on entropy generation and mixed convection in a square cavity. Heat Mass Transf. 2019, 56, 321–338. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Armaghani, T.; Chamkha, A.J.; Hashim, I. Conjugate heat transfer of Al2O3–water nanofluid in a square cavity heated by a triangular thick wall using Buongiorno’s two-phase model. J. Therm. Anal. Calorim. 2018, 135, 161–176. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Mohebbi, R.; Chamkha, A.J.; Hashim, I. Impacts of magnetic field and non-homogeneous nanofluid model on convective heat transfer and entropy generation in a cavity with heated trapezoidal body. J. Therm. Anal. Calorim. 2019, 138, 1371–1394. [Google Scholar] [CrossRef]
- Astanina, M.S.; Riahi, M.K.; Abu-Nada, E.; Sheremet, M.A. Magnetohydrodynamic in partially heated square cavity with variable properties: Discrepancy in experimental and theoretical conductivity correlations. Int. J. Heat Mass Transf. 2018, 116, 532–548. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Armaghani, T.; Chamkha, A.J.; Sadiq, M.A.; Hashim, I. Effects of two-phase nanofluid model on convection in a double lid-driven cavity in the presence of a magnetic field. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 1272–1299. [Google Scholar] [CrossRef]
- Astanina, M.S.; Abu-Nada, E.; Sheremet, M.A. Combined Effects of Thermophoresis, Brownian Motion, and Nanofluid Variable Properties on CuO-Water Nanofluid Natural Convection in a Partially Heated Square Cavity. J. Heat Transf. 2018, 140, 082401. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Tayebi, T.; Chamkha, A.J.; Hashim, I. Effects of two-phase nanofluid model on natural convection in a square cavity in the presence of an adiabatic inner block and magnetic field. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 1613–1647. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Tayebi, T.; Chamkha, A.J.; Hashim, I. Effects of Non-Homogeneous Nanofluid Model on Natural Convection in a Square Cavity in the Presence of Conducting Solid Block and Corner Heater. Energies 2018, 11, 2507. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Roşca, N.C.; Roşca, A.V.; Pop, I. Mixed convection heat transfer in a square porous cavity filled with a nanofluid with suction/injection effect. Comput. Math. Appl. 2018, 76, 2665–2677. [Google Scholar] [CrossRef]
- Xu, D.; Hu, Y.; Li, D. A lattice Boltzmann investigation of two-phase natural convection of Cu-water nanofluid in a square cavity. Case Stud. Therm. Eng. 2018, 13, 100358. [Google Scholar] [CrossRef]
- Garoosi, F.; Jahanshaloo, L.; Rashidi, M.M.; Badakhsh, A.; Ali, M.E. Numerical simulation of natural convection of the nanofluid in heat exchangers using a Buongiorno model. Appl. Math. Comput. 2015, 254, 183–203. [Google Scholar] [CrossRef]
- Garoosi, F.; Bagheri, G.; Rashidi, M.M. Two phase simulation of natural convection and mixed convection of the nanofluid in a square cavity. Powder Technol. 2015, 275, 239–256. [Google Scholar] [CrossRef]
- Elshehabey, H.M.; Ahmed, S.E. MHD mixed convection in a lid-driven cavity filled by a nanofluid with sinusoidal temperature distribution on the both vertical walls using Buongiorno’s nanofluid model. Int. J. Heat Mass Transf. 2015, 88, 181–202. [Google Scholar] [CrossRef]
- Azimikivi, H.; Purmahmud, N.; Mirzaee, I. Rib shape and nanoparticle diameter effects on natural convection heat transfer at low turbulence two-phase flow of AL2O3-water nanofluid inside a square cavity: Based on Buongiorno’s two-phase model. Therm. Sci. Eng. Prog. 2020, 20, 100587. [Google Scholar] [CrossRef]
- Wang, L.; Yang, X.; Huang, C.; Chai, Z.; Shi, B. Hybrid lattice Boltzmann-TVD simulation of natural convection of nanofluids in a partially heated square cavity using Buongiorno’s model. Appl. Therm. Eng. 2018, 146, 318–327. [Google Scholar] [CrossRef]
- Groşan, T.; Revnic, C.; Pop, I.; Ingham, D. Free convection heat transfer in a square cavity filled with a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2015, 87, 36–41. [Google Scholar] [CrossRef]
- Quintino, A.; Ricci, E.; Habib, E.; Corcione, M. Natural convection from a pair of differentially-heated horizontal cylinders aligned side by side in a nanofluid-filled square enclosure. Energy Procedia 2017, 126, 26–33. [Google Scholar] [CrossRef]
- Xiong, Q.; Poor, H.Z.; Izadi, M.; Assareh, E. Natural heat exchange in inhomogeneous porous medium using linear and quadratic porosity distribution. Int. J. Therm. Sci. 2020, 161, 106731. [Google Scholar] [CrossRef]
- Mehryan, S.A.M.; Ghalambaz, M.; Izadi, M. Conjugate natural convection of nanofluids inside an enclosure filled by three layers of solid, porous medium and free nanofluid using Buongiorno’s and local thermal non-equilibrium models. J. Therm. Anal. Calorim. 2018, 135, 1047–1067. [Google Scholar] [CrossRef]
- Zahmatkesh, I.; Habibi, M.R. Natural and mixed convection of a nanofluid in porous cavities: Critical analysis using Buongiorno’s model. J. Theor. Appl. Mech. 2019, 57, 221–233. [Google Scholar] [CrossRef]
- Sadiq, M.A.; Alsabery, A.I.; Hashim, I. MHD Mixed Convection in a Lid-Driven Cavity with a Bottom Trapezoidal Body: Two-Phase Nanofluid Model. Energies 2018, 11, 2943. [Google Scholar] [CrossRef]
- Sekhar, B.C.; Kishan, N.; Haritha, C. Convection in Nanofluid-Filled Porous Cavity with Heat Absorption/Generation and Radiation. J. Thermophys. Heat Transf. 2017, 31, 549–562. [Google Scholar] [CrossRef]
- Motlagh, S.Y.; Taghizadeh, S.; Soltanipour, H. Natural convection heat transfer in an inclined square enclosure filled with a porous medium saturated by nanofluid using Buongiorno’s mathematical model. Adv. Powder Technol. 2016, 27, 2526–2540. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Sabour, M.; Pop, I. Free convection in a square cavity filled by a porous medium saturated by a nanofluid: Viscous dissipation and radiation effects. Eng. Sci. Technol. Int. J. 2016, 19, 1244–1253. [Google Scholar] [CrossRef]
- Pop, I.; Ghalambaz, M.; Sheremet, M. Free convection in a square porous cavity filled with a nanofluid using thermal non equilibrium and Buongiorno models. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 671–693. [Google Scholar] [CrossRef]
- Qi, C.; Tang, J.; Ding, Z.; Yan, Y.; Guo, L.; Ma, Y. Effects of rotation angle and metal foam on natural convection of nanofluids in a cavity under an adjustable magnetic field. Int. Commun. Heat Mass Transf. 2019, 109, 104349. [Google Scholar] [CrossRef]
- Manna, N.K.; Biswas, N.; Mandal, D.K.; Sarkar, U.; Öztop, H.F.; Abu-Hamdeh, N. Impacts of heater-cooler position and Lorentz force on heat transfer and entropy generation of hybrid nanofluid convection in quarter-circular cavity. Int. J. Numer. Methods Heat Fluid Flow 2022, 33, 1249–1286. [Google Scholar] [CrossRef]
- Aly, A.M.; Mohamed, E.M.; Alsedais, N. The magnetic field on a nanofluid flow within a finned cavity containing solid particles. Case Stud. Therm. Eng. 2021, 25, 100945. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Influence of magnetic field on nanofluid free convection in an open porous cavity by means of Lattice Boltzmann method. J. Mol. Liq. 2017, 234, 364–374. [Google Scholar] [CrossRef]
- Kefayati, G. Effect of a magnetic field on natural convection in an open cavity subjugated to water/alumina nanofluid using Lattice Boltzmann method. Int. Commun. Heat Mass Transf. 2013, 40, 67–77. [Google Scholar] [CrossRef]
- Ali, F.H.; Hamzah, H.K.; Egab, K.; Arıcı, M.; Shahsavar, A. Non-Newtonian nanofluid natural convection in a U-shaped cavity under magnetic field. Int. J. Mech. Sci. 2020, 186, 105887. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Magnetic field influence on nanofluid thermal radiation in a cavity with tilted elliptic inner cylinder. J. Mol. Liq. 2017, 229, 137–147. [Google Scholar] [CrossRef]
- Hatami, M.; Zhou, J.; Geng, J.; Jing, D. Variable magnetic field (VMF) effect on the heat transfer of a half-annulus cavity filled by Fe3O4-water nanofluid under constant heat flux. J. Magn. Magn. Mater. 2018, 451, 173–182. [Google Scholar] [CrossRef]
- Mliki, B.; Abbassi, M.A.; Omri, A.; Zeghmati, B. Augmentation of natural convective heat transfer in linearly heated cavity by utilizing nanofluids in the presence of magnetic field and uniform heat generation/absorption. Powder Technol. 2015, 284, 312–325. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Analysis of MHD mixed convection in a flexible walled and nanofluids filled lid-driven cavity with volumetric heat generation. Int. J. Mech. Sci. 2016, 118, 113–124. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Numerical investigation of nanofluid transportation in a curved cavity in existence of magnetic source. Chem. Phys. Lett. 2017, 667, 307–316. [Google Scholar] [CrossRef]
- Rashad, A.; Rashidi, M.; Lorenzini, G.; Ahmed, S.E.; Aly, A.M. Magnetic field and internal heat generation effects on the free convection in a rectangular cavity filled with a porous medium saturated with Cu–water nanofluid. Int. J. Heat Mass Transf. 2017, 104, 878–889. [Google Scholar] [CrossRef]
- Acharya, N.; Chamkha, A.J. On the magnetohydrodynamic Al2O3-water nanofluid flow through parallel fins enclosed inside a partially heated hexagonal cavity. Int. Commun. Heat Mass Transf. 2022, 132, 105885. [Google Scholar] [CrossRef]
- Sreedevi, P.; Reddy, P.S.; Rao, K.V.S. Effect of magnetic field and radiation on heat transfer analysis of nanofluid inside a square cavity filled with silver nanoparticles: Tiwari–Das model. Waves Random Complex Media 2021, 1–19. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D. Numerical approach for magnetic nanofluid flow in a porous cavity using CuO nanoparticles. Mater. Des. 2017, 120, 382–393. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Magnetic field influence on CuO–H2O nanofluid convective flow in a permeable cavity considering various shapes for nanoparticles. Int. J. Hydrogen Energy 2017, 42, 19611–19621. [Google Scholar] [CrossRef]
- Kherroubi, S.; Benkahla, Y.K.; Boutra, A.; Bensaci, A. Two- and three-dimensional comparative study of heat transfer and pressure drop characteristics of nanofluids flow through a ventilated cubic cavity (part II: Non-Newtonian nanofluids under the influence of a magnetic field). J. Therm. Anal. Calorim. 2020, 147, 1859–1886. [Google Scholar] [CrossRef]
- Sreedevi, P.; Reddy, P.S. Effect of magnetic field and thermal radiation on natural convection in a square cavity filled with TiO2 nanoparticles using Tiwari-Das nanofluid model. Alex. Eng. J. 2022, 61, 1529–1541. [Google Scholar] [CrossRef]
- Alnaqi, A.A.; Aghakhani, S.; Pordanjani, A.H.; Bakhtiari, R.; Asadi, A.; Tran, M.-D. Effects of magnetic field on the convective heat transfer rate and entropy generation of a nanofluid in an inclined square cavity equipped with a conductor fin: Considering the radiation effect. Int. J. Heat Mass Transf. 2018, 133, 256–267. [Google Scholar] [CrossRef]
- Afrand, M.; Pordanjani, A.H.; Aghakhani, S.; Oztop, H.F.; Abu-Hamdeh, N. Free convection and entropy generation of a nanofluid in a tilted triangular cavity exposed to a magnetic field with sinusoidal wall temperature distribution considering radiation effects. Int. Commun. Heat Mass Transf. 2020, 112, 104507. [Google Scholar] [CrossRef]
- Pordanjani, A.H.; Aghakhani, S.; Karimipour, A.; Afrand, M.; Goodarzi, M. Investigation of free convection heat transfer and entropy generation of nanofluid flow inside a cavity affected by magnetic field and thermal radiation. J. Therm. Anal. Calorim. 2019, 137, 997–1019. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Selimefendigil, F.; Oztop, H.F. Effects of a Rotating Cone on the Mixed Convection in a Double Lid-Driven 3D Porous Trapezoidal Nanofluid Filled Cavity under the Impact of Magnetic Field. Nanomaterials 2020, 10, 449. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Effects of conductive curved partition and magnetic field on natural convection and entropy generation in an inclined cavity filled with nanofluid. Phys. A Stat. Mech. Its Appl. 2019, 540, 123004. [Google Scholar] [CrossRef]
- Li, Z.; Hussein, A.K.; Younis, O.; Afrand, M.; Feng, S. Natural convection and entropy generation of a nanofluid around a circular baffle inside an inclined square cavity under thermal radiation and magnetic field effects. Int. Commun. Heat Mass Transf. 2020, 116, 104650. [Google Scholar] [CrossRef]
- Cho, C.-C. Mixed convection heat transfer and entropy generation of Cu-water nanofluid in wavy-wall lid-driven cavity in presence of inclined magnetic field. Int. J. Mech. Sci. 2018, 151, 703–714. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Mixed convection of nanofluids in a three dimensional cavity with two adiabatic inner rotating cylinders. Int. J. Heat Mass Transf. 2018, 117, 331–343. [Google Scholar] [CrossRef]
- Nimmagadda, R.; Reuven, R.; Asirvatham, L.G.; Wongwises, S. Thermal Management of Electronic Devices Using Gold and Carbon Nanofluids in a Lid-Driven Square Cavity under the Effect of Variety of Magnetic Fields. IEEE Trans. Compon. Packag. Manuf. Technol. 2020, 10, 1868–1878. [Google Scholar] [CrossRef]
- Kermani, E.; Dessiatoun, S.; Shooshtari, A.; Ohadi, M.M. Experimental investigation of heat transfer performance of a manifold microchannel heat sink for cooling of concentrated solar cells. In Proceedings of the 59th Electronic Components and Technology Conference, San Diego, CA, USA, 26–29 May 2009; pp. 453–459. [Google Scholar] [CrossRef]
- Tuckerman, D.; Pease, R. High-performance heat sinking for VLSI. IEEE Electron Device Lett. 1981, 2, 126–129. [Google Scholar] [CrossRef]
- Fattahi, M.; Vaferi, K.; Vajdi, M.; Moghanlou, F.S.; Namini, A.S.; Asl, M.S. Aluminum nitride as an alternative ceramic for fabrication of microchannel heat exchangers: A numerical study. Ceram. Int. 2020, 46, 11647–11657. [Google Scholar] [CrossRef]
- Huang, B.; Li, H.; Xia, S.; Xu, T. Experimental investigation of the flow and heat transfer performance in micro-channel heat exchangers with cavities. Int. J. Heat Mass Transf. 2020, 159, 120075. [Google Scholar] [CrossRef]
- Hou, T.; Chen, Y. Pressure drop and heat transfer performance of microchannel heat exchanger with different reentrant cavities. Chem. Eng. Process. Process Intensif. 2020, 153, 107931. [Google Scholar] [CrossRef]
- Li, H.; Li, Y.; Huang, B.; Xu, T. Numerical Investigation on the Optimum Thermal Design of the Shape and Geometric Parameters of Microchannel Heat Exchangers with Cavities. Micromachines 2020, 11, 721. [Google Scholar] [CrossRef] [PubMed]
- Pan, M.; Wang, H.; Zhong, Y.; Fang, T.; Zhong, X. Numerical simulation of the fluid flow and heat transfer characteristics of microchannel heat exchangers with different reentrant cavities. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4334–4348. [Google Scholar] [CrossRef]
- Huang, B.; Li, H.; Xu, T. Experimental Investigation of the Flow and Heat Transfer Characteristics in Microchannel Heat Exchangers with Reentrant Cavities. Micromachines 2020, 11, 403. [Google Scholar] [CrossRef]
- Pan, M.; Zhong, Y.; Xu, Y. Numerical investigation of fluid flow and heat transfer in a plate microchannel heat exchanger with isosceles trapezoid-shaped reentrant cavities in the sidewall. Chem. Eng. Process. Process Intensif. 2018, 131, 178–189. [Google Scholar] [CrossRef]
- Zhang, D.; Fu, L.; Guan, J.; Shen, C.; Tang, S. Investigation on the heat transfer and energy-saving performance of microchannel with cavities and extended surface. Int. J. Heat Mass Transf. 2022, 189, 122712. [Google Scholar] [CrossRef]
- Xia, G.; Chai, L.; Wang, H.; Zhou, M.; Cui, Z. Optimum thermal design of microchannel heat sink with triangular reentrant cavities. Appl. Therm. Eng. 2010, 31, 1208–1219. [Google Scholar] [CrossRef]
- Hou, T.; Chen, Y. Pressure Drop and Heat Transfer Performance of Microchannel Heat Exchanger with Circular Reentrant Cavities and Ribs. J. Heat Transf. 2020, 142, 042502. [Google Scholar] [CrossRef]
- Zhu, Q.; Jin, Y.; Chen, J.; Su, R.; Zhu, F.; Li, H.; Wan, J.; Zhang, H.; Sun, H.; Cui, Y.; et al. Computational study of rib shape and configuration for heat transfer and fluid flow characteristics of microchannel heat sinks with fan-shaped cavities. Appl. Therm. Eng. 2021, 195, 117171. [Google Scholar] [CrossRef]
- Naphon, P. Effect of wavy plate geometry configurations on the temperature and flow distributions. Int. Commun. Heat Mass Transf. 2009, 36, 942–946. [Google Scholar] [CrossRef]
- Hasan, M.I.; Rageb, A.; Yaghoubi, M.; Homayoni, H. Influence of channel geometry on the performance of a counter flow microchannel heat exchanger. Int. J. Therm. Sci. 2009, 48, 1607–1618. [Google Scholar] [CrossRef]
- Hejri, S.; Kamali, D.; Malekshah, E.H. An experimental/numerical hydrothermal-Second law analysis of a finned/tubular heat exchanger using Bhatnagar–Gross–Krook Lattice Boltzmann (BGKLBM) and rheological-thermal behavior of Fe2O3-water. Int. J. Numer. Methods Heat Fluid Flow 2020, 31, 2308–2329. [Google Scholar] [CrossRef]
- Malekshah, E.H.; Hussein, A.K.; Kolsi, L. Convective flow over heat dissipating fins for application of electronic package cooling using curved boundary scheme lattice Boltzmann method. Int. J. Numer. Methods Heat Fluid Flow 2022, 33, 1184–1202. [Google Scholar] [CrossRef]
- Fan, F.; Qi, C.; Tang, J.; Liu, Q.; Wang, X.; Yan, Y. A novel thermal efficiency analysis on the thermo-hydraulic performance of nanofluids in an improved heat exchange system under adjustable magnetic field. Appl. Therm. Eng. 2020, 179, 115688. [Google Scholar] [CrossRef]
- Ardalan, M.V.; Alizadeh, R.; Fattahi, A.; Rasi, N.A.; Doranehgard, M.H.; Karimi, N. Analysis of unsteady mixed convection of Cu–water nanofluid in an oscillatory, lid-driven enclosure using lattice Boltzmann method. J. Therm. Anal. Calorim. 2021, 145, 2045–2061. [Google Scholar] [CrossRef]
- Alizadeh, R.; Abad, J.M.N.; Ameri, A.; Mohebbi, M.R.; Mehdizadeh, A.; Zhao, D.; Karimi, N. A machine learning approach to the prediction of transport and thermodynamic processes in multiphysics systems—Heat transfer in a hybrid nanofluid flow in porous media. J. Taiwan Inst. Chem. Eng. 2021, 124, 290–306. [Google Scholar] [CrossRef]
- Olabi, A.; Wilberforce, T.; Sayed, E.T.; Elsaid, K.; Rahman, S.A.; Abdelkareem, M.A. Geometrical effect coupled with nanofluid on heat transfer enhancement in heat exchangers. Int. J. Thermofluids 2021, 10, 100072. [Google Scholar] [CrossRef]
- Ghalandari, M.; Maleki, A.; Haghighi, A.; Shadloo, M.S.; Nazari, M.A.; Tlili, I. Applications of nanofluids containing carbon nanotubes in solar energy systems: A review. J. Mol. Liq. 2020, 313, 113476. [Google Scholar] [CrossRef]
- Said, Z.; Hachicha, A.A.; Aberoumand, S.; Yousef, B.A.; Sayed, E.T.; Bellos, E. Recent advances on nanofluids for low to medium temperature solar collectors: Energy, exergy, economic analysis and environmental impact. Prog. Energy Combust. Sci. 2021, 84, 100898. [Google Scholar] [CrossRef]
- Al-Kayiem, H.H.; Yassen, T.A. On the natural convection heat transfer in a rectangular passage solar air heater. Sol. Energy 2015, 112, 310–318. [Google Scholar] [CrossRef]
- Akhbari, M.; Rahimi, A.; Hatamipour, M.S. Modeling and experimental study of a triangular channel solar air heater. Appl. Therm. Eng. 2020, 170, 114902. [Google Scholar] [CrossRef]
- Kumar, P.N.; Manokar, A.M.; Madhu, B.; Kabeel, A.; Arunkumar, T.; Panchal, H.; Sathyamurthy, R. Experimental investigation on the effect of water mass in triangular pyramid solar still integrated to inclined solar still. Groundw. Sustain. Dev. 2017, 5, 229–234. [Google Scholar] [CrossRef]
- Misra, R.; Singh, J.; Jain, S.K.; Faujdar, S.; Agrawal, M.; Mishra, A.; Goyal, P.K. Prediction of behavior of triangular solar air heater duct using V-down rib with multiple gaps and turbulence promoters as artificial roughness: A CFD analysis. Int. J. Heat Mass Transf. 2020, 162, 120376. [Google Scholar] [CrossRef]
- Nidhul, K.; Kumar, S.; Yadav, A.K.; Anish, S. Enhanced thermo-hydraulic performance in a V-ribbed triangular duct solar air heater: CFD and exergy analysis. Energy 2020, 200, 117448. [Google Scholar] [CrossRef]
- Cruz, J.M.; Hammond, G.P.; Reis, A.J. Thermal performance of a trapezoidal-shaped solar collector/energy store. Appl. Energy 2002, 73, 195–212. [Google Scholar] [CrossRef]
- Liu, H.; Jiang, L.; Wu, D.; Sun, W. Experiment and simulation study of a trapezoidal salt gradient solar pond. Sol. Energy 2015, 122, 1225–1234. [Google Scholar] [CrossRef]
- Larsen, S.F.; Altamirano, M.; Hernández, A. Heat loss of a trapezoidal cavity absorber for a linear Fresnel reflecting solar concentrator. Renew. Energy 2012, 39, 198–206. [Google Scholar] [CrossRef]
- Singh, P.L.; Sarviya, R.; Bhagoria, J. Thermal performance of linear Fresnel reflecting solar concentrator with trapezoidal cavity absorbers. Appl. Energy 2010, 87, 541–550. [Google Scholar] [CrossRef]
- Shetty, S.P.; Madhwesh, N.; Karanth, K.V. Numerical analysis of a solar air heater with circular perforated absorber plate. Sol. Energy 2021, 215, 416–433. [Google Scholar] [CrossRef]
- Qi, C.; Li, C.; Li, K.; Han, D. Natural convection of nanofluids in solar energy collectors based on a two-phase lattice Boltzmann model. J. Therm. Anal. Calorim. 2021, 147, 2417–2438. [Google Scholar] [CrossRef]
- Gómez-Villarejo, R.; Martín, E.I.; Sánchez-Coronilla, A.; Aguilar, T.; Gallardo, J.J.; Martínez-Merino, P.; Carrillo-Berdugo, I.; Alcántara, R.; Fernández-Lorenzo, C.; Navas, J. Towards the improvement of the global efficiency of concentrating solar power plants by using Pt-based nanofluids: The internal molecular structure effect. Appl. Energy 2018, 228, 2262–2274. [Google Scholar] [CrossRef]
- Aguilar, T.; Navas, J.; Sánchez-Coronilla, A.; Martín, E.I.; Gallardo, J.J.; Martínez-Merino, P.; Gómez-Villarejo, R.; Piñero, J.C.; Alcántara, R.; Fernández-Lorenzo, C. Investigation of enhanced thermal properties in NiO-based nanofluids for concentrating solar power appli-cations: A molecular dynamics and experimental analysis. Appl. Energy 2018, 211, 677–688. [Google Scholar] [CrossRef]
- Yasinskiy, A.; Navas, J.; Aguilar, T.; Alcántara, R.; Gallardo, J.J.; Sánchez-Coronilla, A.; Martín, E.I.; Santos, D.D.L.; Fernández-Lorenzo, C. Dramatically enhanced thermal properties for TiO2-based nanofluids for being used as heat transfer fluids in concentrating solar power plants. Renew. Energy 2018, 119, 809–819. [Google Scholar] [CrossRef]
- Martín, E.I.; Sánchez-Coronilla, A.; Navas, J.; Gómez-Villarejo, R.; Gallardo, J.J.; Alcántara, R.; Fernández-Lorenzo, C. Unraveling the role of the base fluid arrangement in metal-nanofluids used to enhance heat transfer in concentrating solar power plants. J. Mol. Liq. 2018, 252, 271–278. [Google Scholar] [CrossRef]
| Ref. | Cavity Geometry | Nanoparticles and Their Size (nm) | Concentration of Nanoparticles | Cavity Inclination Angle | Results |
|---|---|---|---|---|---|
| [25] | Square | Fe3O4, Ha = 0–10 | = 0.01–0.04% | - | Heat transfer increases with the increase in Lorentz force effect. |
| [26] | L-shaped | Ag | = 0.06% | 0, 30, 60, 90 | The inclination angle has a direct correlation to the amount of heat transferred. |
| [27] | Isosceles Triangular | Al2O3, dp = 10 nm, Ha = 0, 25, 50 | = 0.06% | As Ha and the angle of inclination of the magnetic field went up, the rate of heat transfer went down. | |
| [28] | Closed elbow-shaped | Cu | = 0–0.06% | - | Heat transfer increases due to high nanoparticle volume fraction. |
| [29] | Trapezoidal | CuO Ha = 0, 10, 50, 100 dp = 29 nm the angle inclination of magnetic field = 0– | = 0–0.04% | - | The heat transmission rate drops as rises and increases with a high nanoparticle volume fraction. |
| [30] | Shallow | Al2O3, dp = 10 nm | = 0–0.04% | - | Radiative heat transfer mixed with natural convection may impact the flow field and cause the rise in Nusselt number (Nu). Thermal radiation research is highly beneficial in the enrichment of heat-transfer rate. |
| [31] | Wavy-walled | Al2O3 | = 0–0.04% | /2 | Inclination angle and undulation number are non-monotonic functions of heat transmission and fluid flow. |
| [32] | Open wavy | - | - | - | The average Nusselt and Sherwood values can continuously be improved using wavy surface design parameters. |
| [33] | Porous wavy | - | - | - | Localized heat source affects nanofluid flow and heat transmission rate. |
| [34] | Vented | CuO, Ha = 0 and 40 dp = 29 nm, the magnetic field inclination angle = 0–/2 | = 0–0.03% | - | In the absence of MHD effect, the nanoparticles increase heat transfer up to 9–9.5%. |
| [35] | Inclined wavy | CuO, Ha= 0–100, the angle inclination of magnetic field = 0– | = 0–0.05% | Changing cavity inclination angle affects convective heat transfer. Heat transmission rate increases with nanoparticle volume fraction. | |
| [36] | Circular | MWCNT-Fe3O4/H2O, Ha = 0–50 | = 0–0.03% | - | Convective heat transfer is enhanced by ejecting hybrid nanoparticles into the host fluid. |
| [37] | Square | Copper | = 0–0.03% | - | Heat-transfer rate decreases with increasing solid volume fraction for a given Ra, but increases with increasing nanoparticle volume fraction. |
| [38] | Rectangular | Al2O3 | = 0.0–0.05% | - | Al2O3/H2O nanofluids are more stable than ordinary fluids in a heated rectangular chamber. |
| [39] | Rectangular | Al2O3 | - | - | Aspect ratio affects heat transfer coefficient and Nusselt number. |
| [40] | Porous square | Al2O3, dp = 30 nm | = 0.05–0.4% | - | The porous cavity increases the 10% heat-transfer rate with a 0.05% concentration of nanofluid volume fraction. |
| [41] | Trapezoidal | Paraffin wax, Graphine | Φ = 0.05 | - | Rearranging the direction of the trapezoidal cavity resulted in higher melting. |
| [42] | Hemispherical | Water-ZnO | - | - | The nanofluid saturated in the porous media improves natural convective heat transfer for the given problem. |
| [43] | Inclined cube | Al2O3, TiO2, CuO | - | 0, 45, 90 | Compared to the nanofluids, turbine oil has the maximum Nu anywhere at the inclination angle of the cavity. |
| Ref. | Numerical Method | Material of Nanoparticles | Range of Ra | Range of Le | Size of Nanoparticles | Nanoparticle Volume Fraction | Inclination Angle of Cavity/Magnetic Field | Range of Pr |
|---|---|---|---|---|---|---|---|---|
| [44] | FVM | Al2O3 | 33 nm | 4.623 | ||||
| [45] | FEM | Al2O3 | - | 33 nm | - | 4.623 | ||
| [46] | FDM | Al2O3 | 33 nm | - | 4.623 | |||
| [47] | FEM | Al2O3 | 33 nm | - | 4.623 | |||
| [48] | FDM | - | 1000 | - | - | - | 7.0 | |
| [49] | FVM | Cu, Al2O3, TiO2 | - | - | 4.623 | |||
| [50] | FEM | Al2O3 | 33 nm | - | 4.623 | |||
| [51] | FEM | Al2O3 | 33 nm | - | 4.623 | |||
| [52] | FEM | Al2O3 | 33 nm | - | 4.623 | |||
| [53] | FDM | Al2O3 | 15,267.8 | 47 nm | 6.51 | |||
| [54] | FEM | Al2O3 | - | 33 nm | - | 4.623 | ||
| [55] | FDM | CuO | 9460.61 | 29 nm | - | 6.53 | ||
| [56] | FDM | Al2O3 | 33 nm | 45° | 4.623 | |||
| [57] | FDM | Al2O3 | 33 nm | - | 4.623 | |||
| [58] | FDM | - | 1000 | - | - | - | 6.82 | |
| [59] | LBM | CuO | - | - | - | 6.2 | ||
| [60] | FVM | Cu, Al2O3, TiO2 | - | - | - | |||
| [61] | FVM | Cu, Al2O3, TiO2 | - | - | - | |||
| [62] | FVM | - | - | - | - | |||
| [63] | SIMULATION | Al2O3 | - | - | ||||
| [64] | Hybrid LBM & TVD | Al2O3 | - | - | - | |||
| [65] | FDM | Carbon Nanotubes | - | - | ||||
| [66] | FVM | CuO, Al2O3, TiO2 | - | - | - | - | ||
| [67] | FEM | - | - | - | - | - | 6.2 | |
| [68] | FEM | - | - | - | - | 6.2 | ||
| [69] | FVM | - | - | - | - | - | ||
| [70] | FEM | Al2O3 | - | 33 nm | 4.623 | |||
| [71] | FEM | - | - | - | - | - | ||
| [72] | FVM | Al2O3, CuO | - | 33 nm | 10 | |||
| [73] | FEM | - | 100 | 1000 | - | - | - | - |
| [74] | FEM | - | 100 | 1000 | - | - | - | - |
| Ref. | Cavities Geometry | Nanoparticles and Their Size (nm) | Hartmann Number | Cavity Inclination Angle | Results |
|---|---|---|---|---|---|
| [25] | Square | Fe3O4 | Ha = 0–10 | Heat transfer increases with the increase in Lorentz force. | |
| [77] | Finned | Cu | Ha = 0–50 | 0–90 | At 90 degrees highest heat transfer achieved and at 30 degrees lowest heat transfer achieved. |
| [78] | Porous open | Cu, dp = 29 nm | Ha = 0–60 | - | Heat transfer increases with increase in Darcy number. |
| [79] | Open | Al2O3 | Ha = 0–90 | - | has the largest particle effect at Ha = 30, and for Ra = at Ha = 60. |
| [29] | Trapezoidal | CuO, dp = 29 nm | Ha = 0, 10, 50, 100 | The heat transmission rate drops as rises and increases with high nanoparticle volume fraction. | |
| [80] | U-shaped | Fe2O3 | Ha = 0–30 | - | Influence of n and Ha on heat transport was studied. |
| [81] | Irregular cavity | Fe2O3, dp = 47 nm | Ha = 0–40 | /2 | Nusselt number rises with inclination angle, falls with Ha. |
| [82] | Half-annulus | Fe3O4 | Ha = 0, 20, 40, 80 | - | Due to Lorentz force from a greater magnetic field, low Eckert and Hartmann numbers decrease the Nusselt number. |
| [83] | Rectangular | Cu | Ha = 0–60 | - | For Ha values between 9 and 12, the heat transmission is not affected by the concentration of nanoparticles. |
| [34] | Vented | CuO, dp = 29 nm | Ha = 0 and 40 | /2 | In the absence and presence of a magnetic field, nanoparticles increase heat transmission by 9–9.5%. |
| [35] | Inclined wavy | CuO | Ha = 0–100 | Changing cavity tilt affects convective heat transmission. Heat transmission rate increases with nanoparticle volume fraction. | |
| [84] | Lid-driven | Cu | Ha = 0–50 | 0–90 | Average heat transmission increases 239.35% at Richardson number 100 vs. 1. |
| [85] | Curved | Fe3O4, dp = 47 nm | Ha = 0–60 | - | Temperature gradient reduces with enhancement of radiation influence. |
| [86] | Rectangular | Cu | Ha = 0–100 | 0–90 | The average Nusselt number rises with magnetic field inclination. |
| [87] | Hexagonal | Al2O3 | Ha = 0–100 | - | The analysis shows improved convection, velocity, and thermal results for Rayleigh number, but the opposite for Hartmann number and nanoparticle concentration. |
| [88] | Square | Ag | - | - | Silver nanoparticles dispersed in water increase heat transfer from 6.3% to 12.4%. |
| [89] | Porous cavity | Cu, dp = 47 nm | Ha = 0–40 | - | Radiation parameter increases heat transport, while Hartmann number decreases it. |
| [90] | Porous lid-driven | Cu, dp = 45 nm | Ha = 0–40 | - | Temperature gradient decreases with Ha and increases with Re. |
| [91] | Ventilated cube | ZnO | Ha = 100 | 0, 45, 90, 235 | In a magnetic field, = 45° offers the best heat-transfer rate, whatever the Reynolds number. |
| [92] | Square | TiO2 | - | - | Radiation parameter (R) increases heat transfer from hot wall to cold wall. |
| [93] | Inclined square | Al2O3, dp = 47 nm | Ha = 0–40 | - | Increasing Rayleigh and decreasing Hartmann increase the heat-transfer rate. For Hartmann number growing from 0 to 40, the Nusselt number drops up to 27%. |
| [94] | Tilted triangular | Al2O3, dp = 47 nm | Ha = 0, 20, 40 | 45 | The magnetic field angle does not affect heat transport, entropy generation, or Be. The 90-degree angle had the maximum transfer rate and entropy creation. |
| [95] | Rectangular | Al2O3, dp = 47 nm | Ha = 0, 30, 60 | 0–90 | Increasing the magnetic field angle decreases heat transfer and entropy formation and raises Bejan number. |
| [96] | Trapezoidal | Carbon Nanotube (CNT) | Ha = 0–50 | - | Magnetic field effects limited effective convection, although CNT particles increased the average Nu value by 84.3%. |
| [97] | Inclined | CuO, dp = 29 nm | 0–50 | 0–90 | Increasing Hartmann number from 0 to 50 reduces Nusselt number by 32% and 34% for water and nanofluid, respectively. |
| [98] | Inclined square | Al2O3, dp = 47 nm | Ha = 0–40 | 0–90 | An increase in Ha lowered heat transport and entropy by 45% and 35%, respectively. |
| [54] | Double lid-driven square | Al2O3, dp = 33 nm | Ha = 0–50 | 45 | A rise in Reynolds number or reduction of Hartmann number can increase the heat-transfer rate. |
| [99] | Wavy | Cu | Ha = 0–50 | 0–360 | Bejan number decreases when Hartmann number, irreversibility distribution ratio, and Richardson number rise. |
| [100] | Cubic | Cu, Al2O3, TiO2 | - | - | The Bejan number decreases with a higher Hartmann number, larger irreversibility ratio, and lower Richardson number. |
| [101] | Lid-driven | Au, SWCNT, dp of Au= 50 nm dp of SWCNT = 70 nm | Ha = 0–40 | - | Nanoparticles and nanofluid velocity affect heat-transfer efficiency. |
| Ref. | Cavity Geometry | Results |
|---|---|---|
| [125] | Rectangular | the best performance was achieved. |
| [126] | Triangular | the best performance was achieved. |
| [127] | Triangular pyramid | Inclination improves triangular pyramid solar still by 79.05 percent. |
| [128] | V-down ribs | Maximum heat-transfer rate is attained at roughness pitch at 45°. |
| [129] | V-rib triangular | Ribbed triangular duct solar air heater () is superior over various configurations of the ribbed rectangular duct solar air heater at higher mass flow rate. |
| [130] | Trapezoidal | Thermal stratification in the storage cavity affects energy savings. |
| [131] | Trapezoidal | Stability deteriorates with the temperature gradient. |
| [132] | Trapezoidal | The cavity was stable and convective. |
| [133] | Trapezoidal | Round pipe (multi-tube) receivers absorb more solar radiation than rectangular pipe receivers. |
| [134] | Circular | Circular geometry and vented absorber plates promote turbulence-induced heat transfer. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zafar, M.; Sakidin, H.; Sheremet, M.; Dzulkarnain, I.; Nazar, R.M.; Hussain, A.; Said, Z.; Afzal, F.; Al-Yaari, A.; Khan, M.S.; et al. The Impact of Cavities in Different Thermal Applications of Nanofluids: A Review. Nanomaterials 2023, 13, 1131. https://doi.org/10.3390/nano13061131
Zafar M, Sakidin H, Sheremet M, Dzulkarnain I, Nazar RM, Hussain A, Said Z, Afzal F, Al-Yaari A, Khan MS, et al. The Impact of Cavities in Different Thermal Applications of Nanofluids: A Review. Nanomaterials. 2023; 13(6):1131. https://doi.org/10.3390/nano13061131
Chicago/Turabian StyleZafar, Mudasar, Hamzah Sakidin, Mikhail Sheremet, Iskandar Dzulkarnain, Roslinda Mohd Nazar, Abida Hussain, Zafar Said, Farkhanda Afzal, Abdullah Al-Yaari, Muhammad Saad Khan, and et al. 2023. "The Impact of Cavities in Different Thermal Applications of Nanofluids: A Review" Nanomaterials 13, no. 6: 1131. https://doi.org/10.3390/nano13061131
APA StyleZafar, M., Sakidin, H., Sheremet, M., Dzulkarnain, I., Nazar, R. M., Hussain, A., Said, Z., Afzal, F., Al-Yaari, A., Khan, M. S., & Khan, J. A. (2023). The Impact of Cavities in Different Thermal Applications of Nanofluids: A Review. Nanomaterials, 13(6), 1131. https://doi.org/10.3390/nano13061131











