Gaussian Curvature Effects on Graphene Quantum Dots
Abstract
1. Introduction
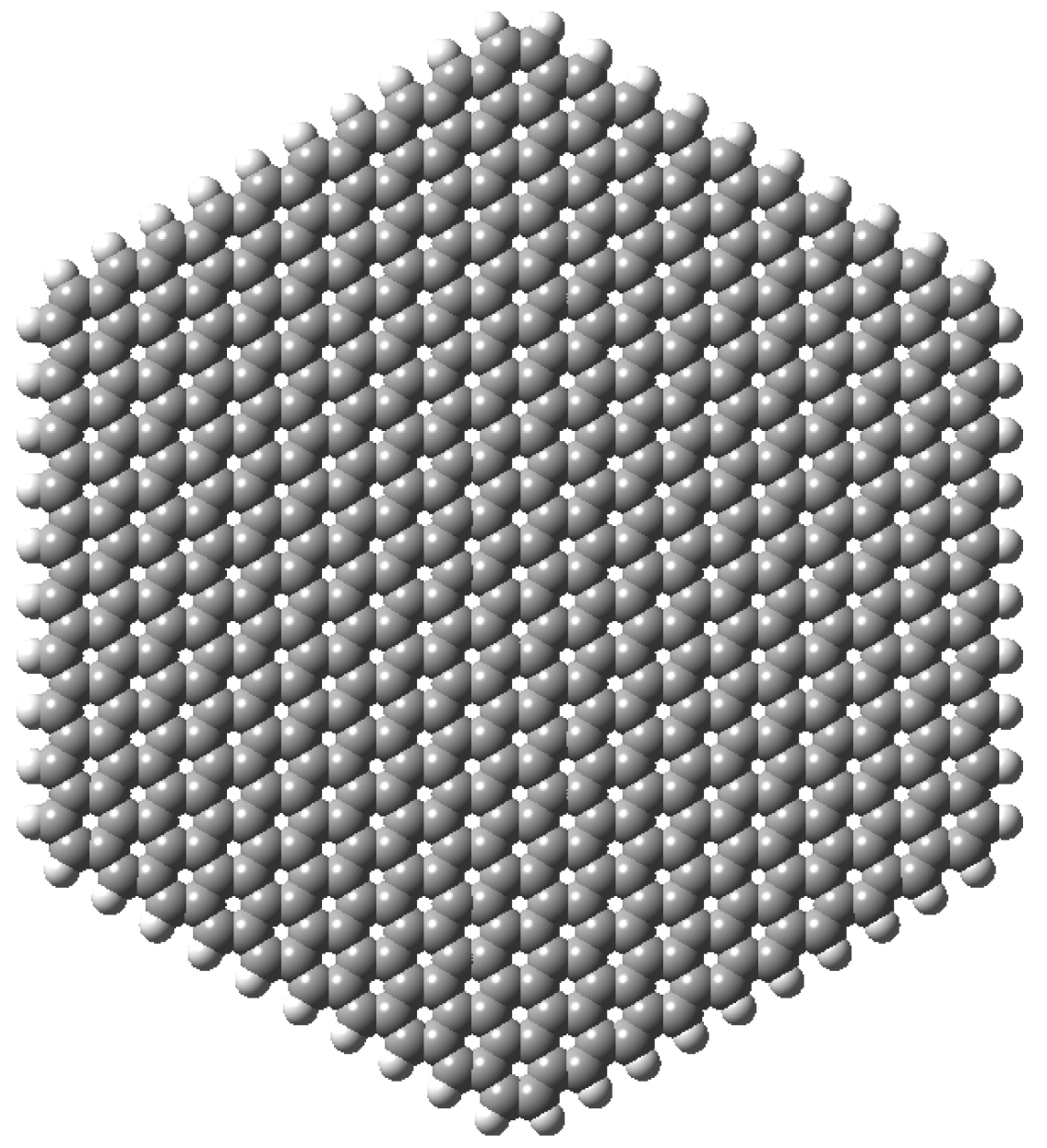
2. Materials and Methods
3. Results and Discussion
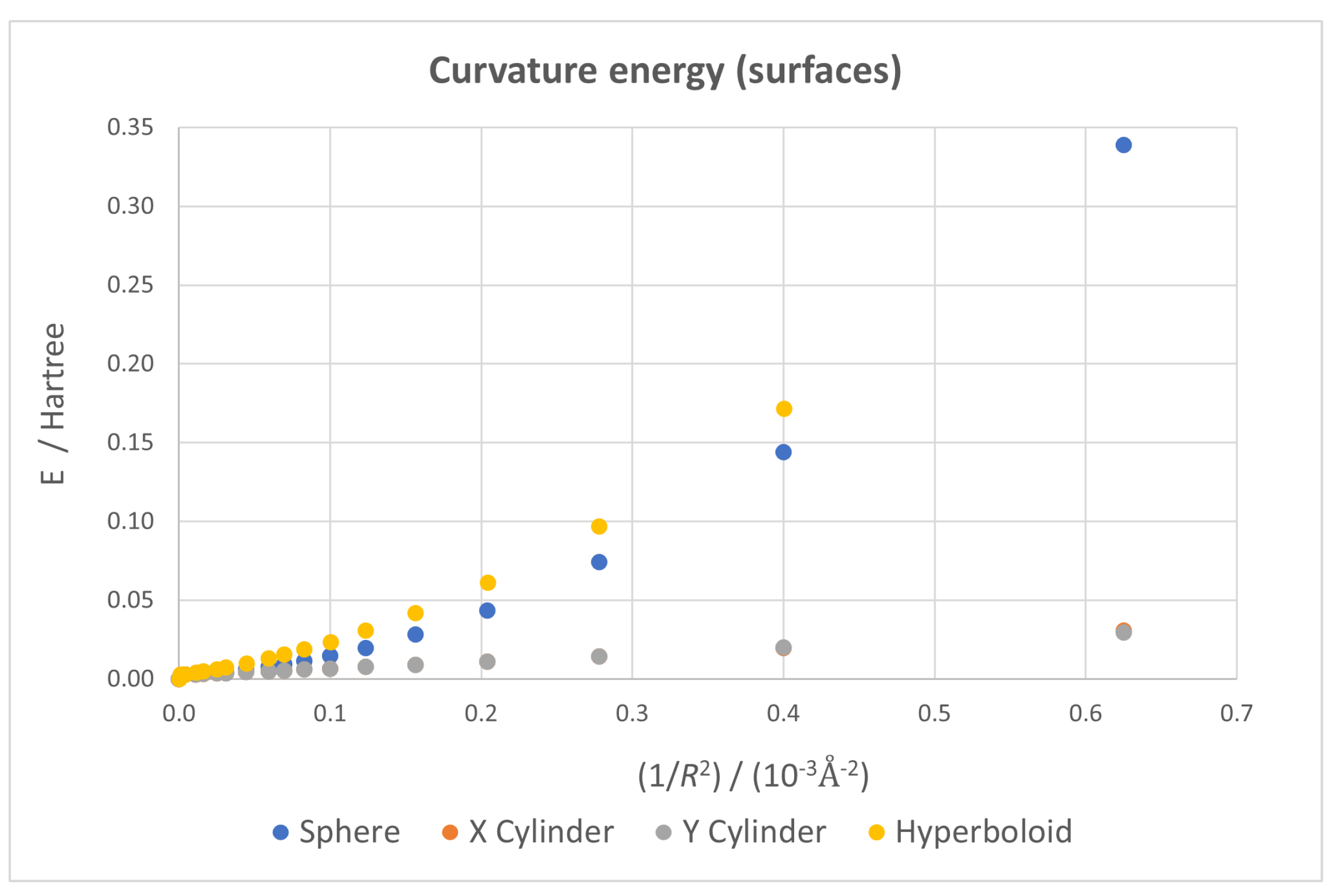
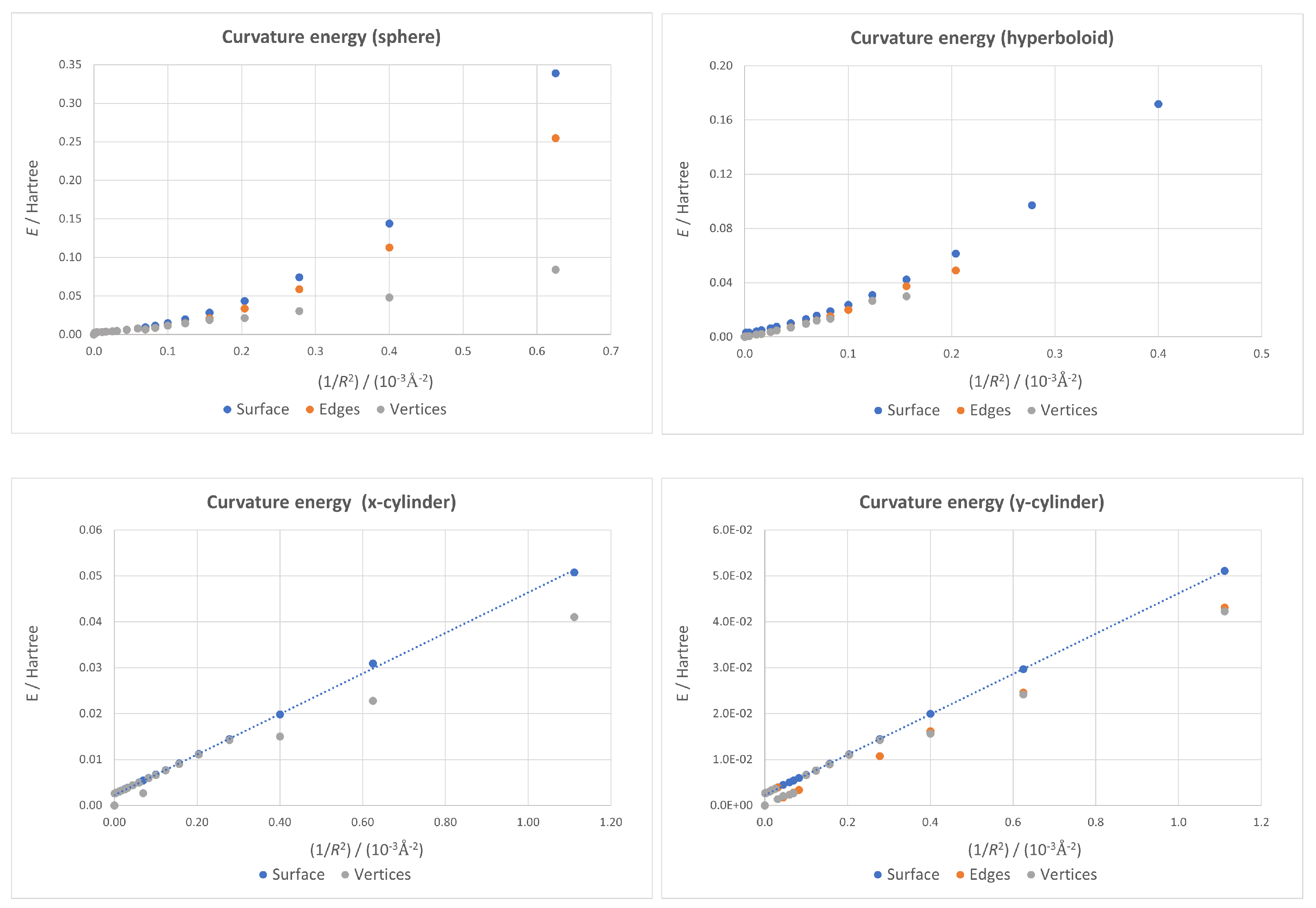
3.1. Curvature Energy
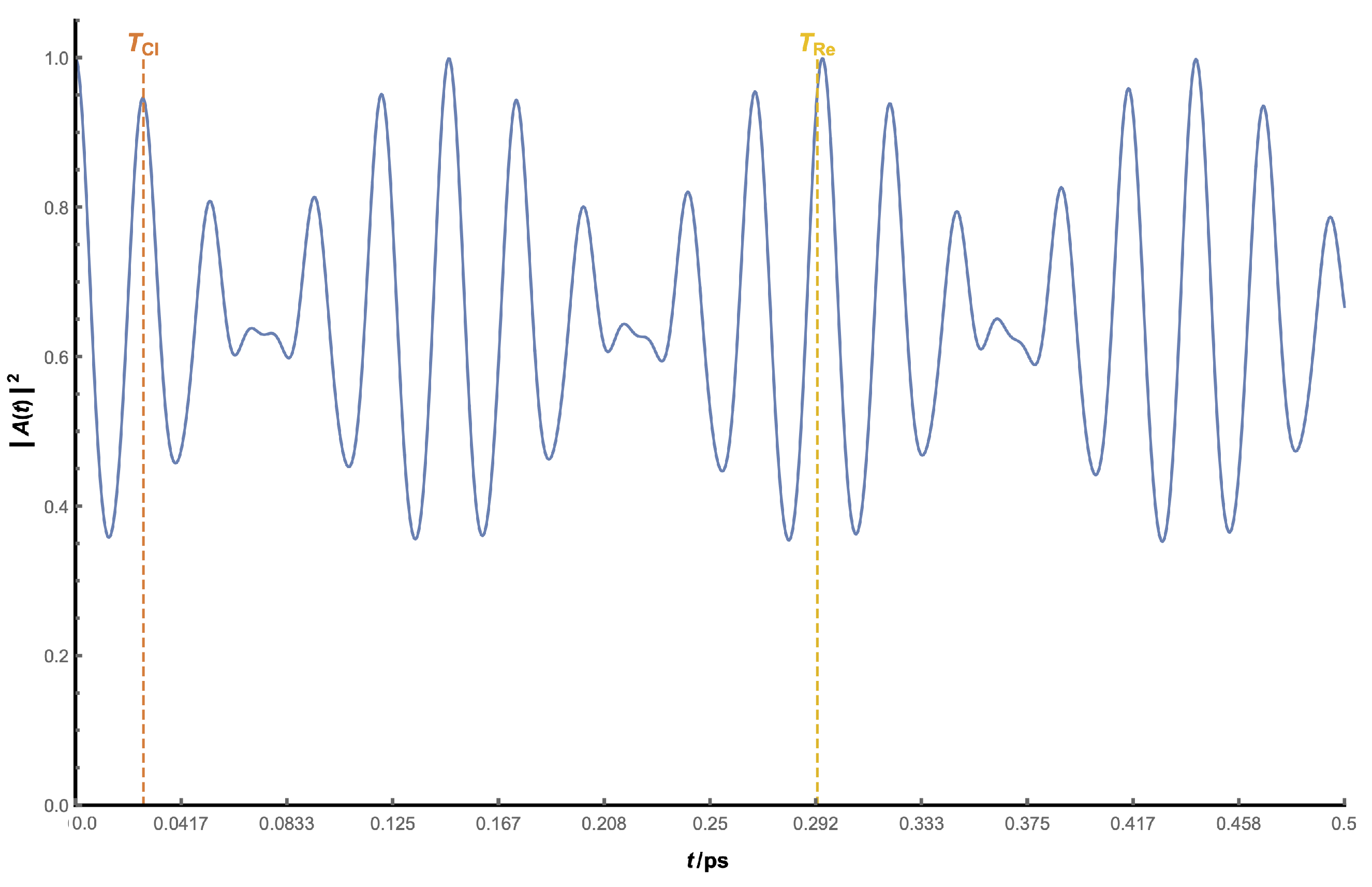
3.2. Regeneration Times
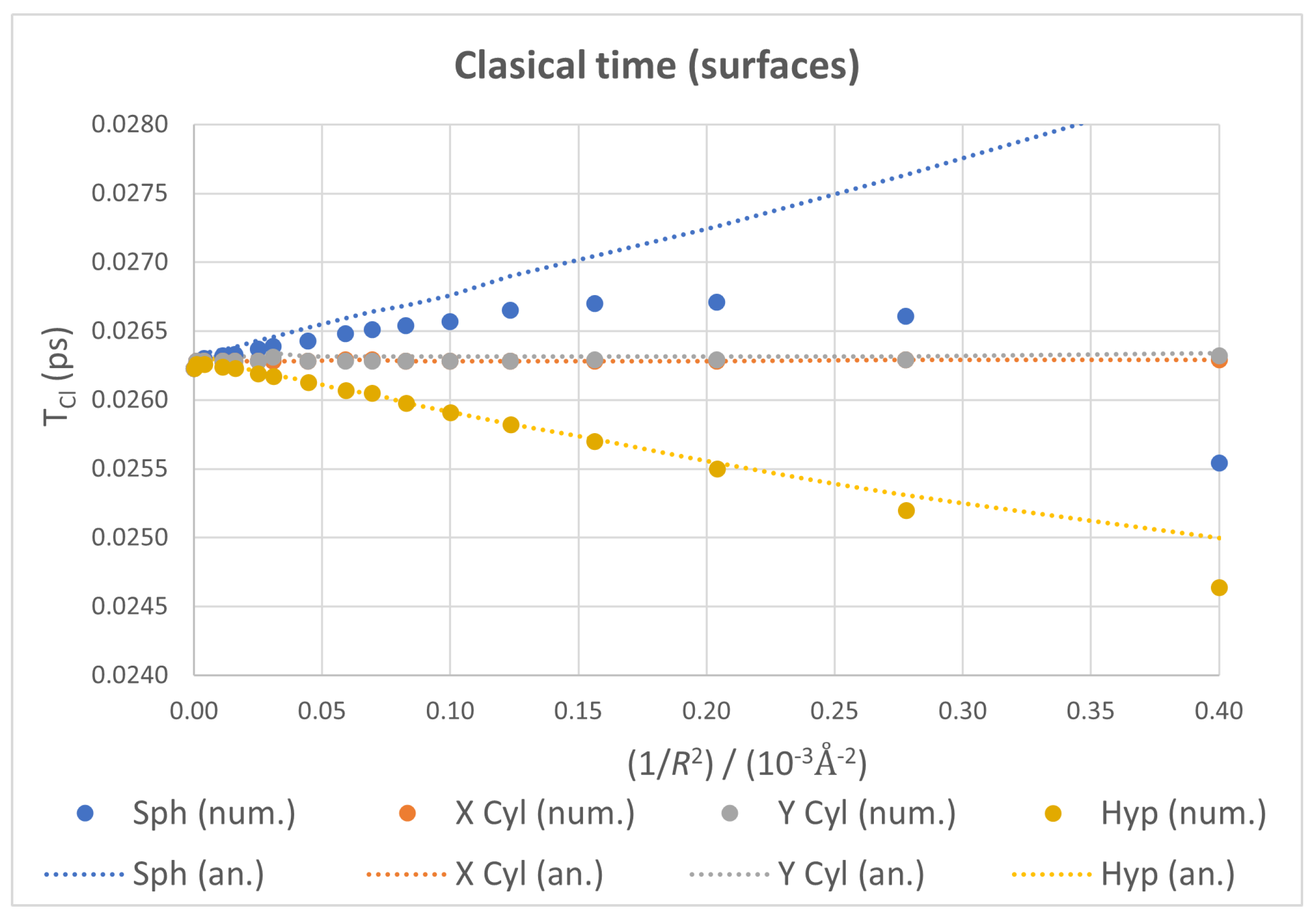
3.2.1. Classical Time
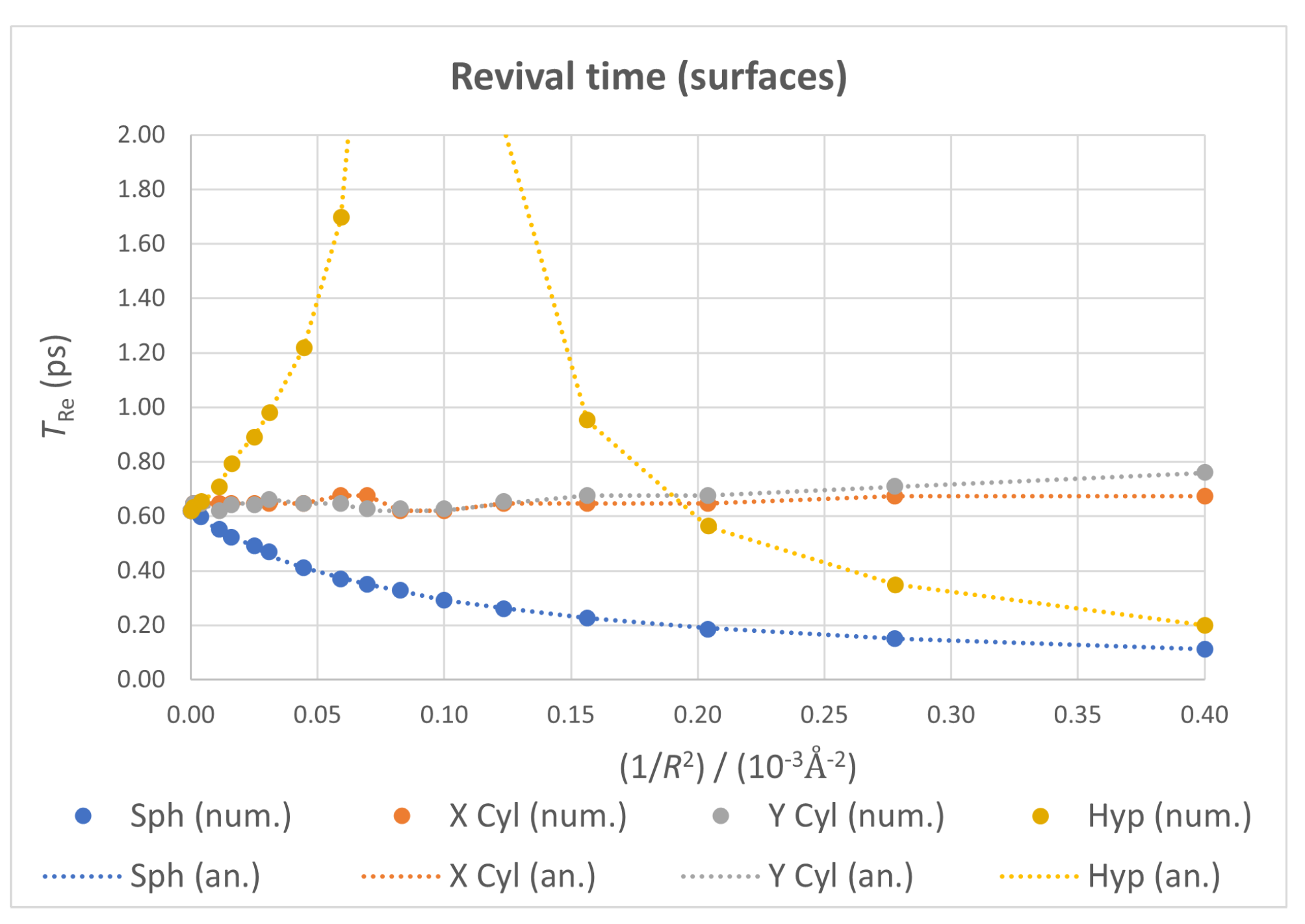
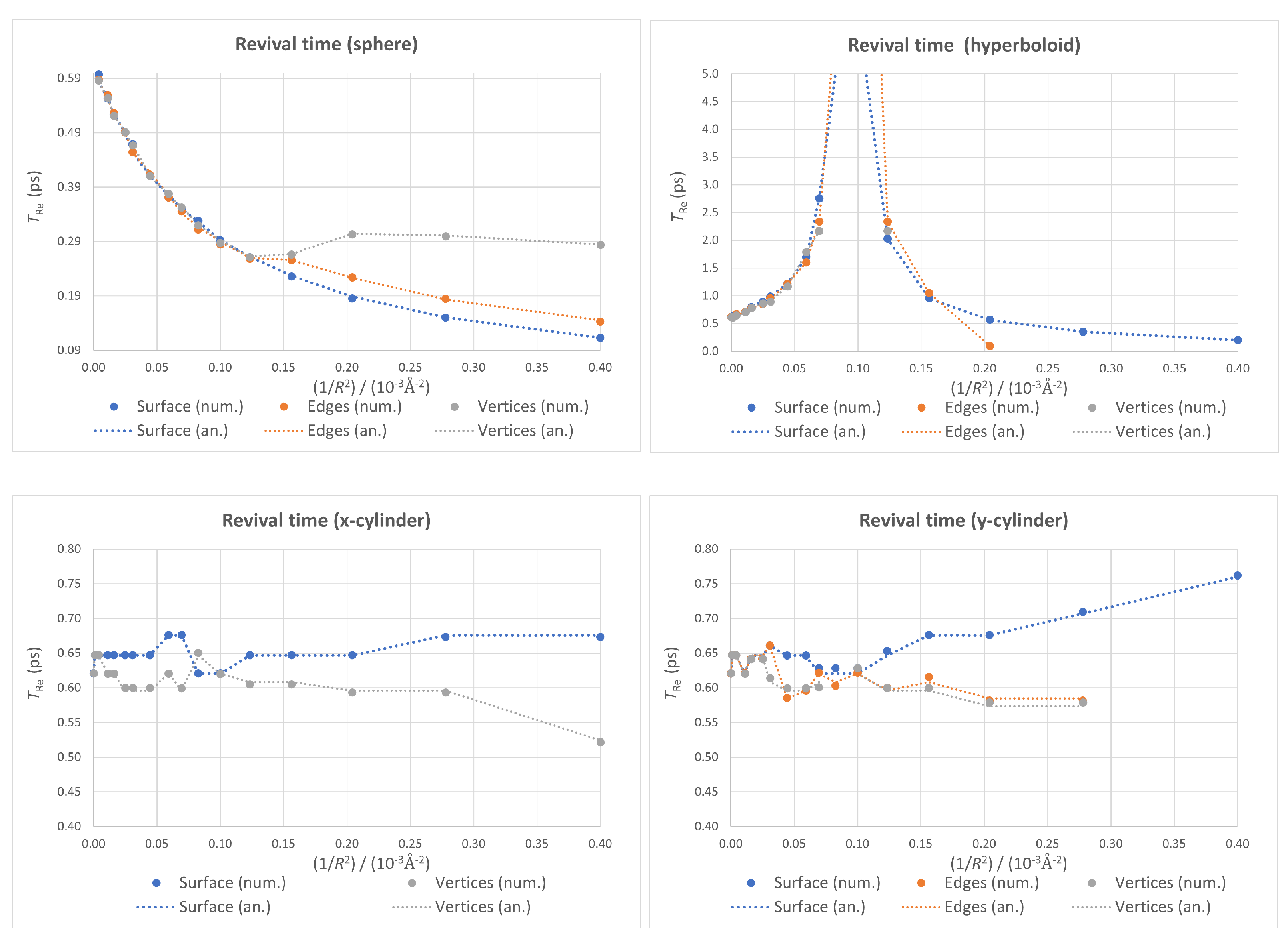
3.2.2. Revival Time
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DFT | Density Functional Theory |
| GGA | Generalized Gradient Approximation |
| LDA | Local Density Approximation |
| LUMO | Lower Unoccupied Molecular Orbital |
References
- Wallace, P.R. The Band Theory of Graphite. Phys. Rev. 1947, 71, 622–634. [Google Scholar] [CrossRef]
- Fradkin, E. Critical behavior of disordered degenerate semiconductors. II. Spectrum and transport properties in mean-field theory. Phys. Rev. B 1986, 33, 3263–3268. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Ming, P.; Li, J. Ab initio calculation of ideal strength and phonon instability of graphene under tension. Phys. Rev. B 2007, 76, 064120. [Google Scholar] [CrossRef]
- Jing, Y.; Sun, Y.; Niu, H.; Shen, J. Chirality and size dependent elastic properties of silicene nanoribbons under uniaxial tension. In Proceedings of the 13th International Conference on Fracture 2013, ICF 2013, Beijing, China, 16–21 June 2013; Volume 7, pp. 5663–5668. [Google Scholar]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Berman, D.; Erdemir, A.; Sumant, A.V. Reduced wear and friction enabled by graphene layers on sliding steel surfaces in dry nitrogen. Carbon 2013, 59, 167–175. [Google Scholar] [CrossRef]
- Berman, D.; Deshmukh, S.A.; Sankaranarayanan, S.K.; Erdemir, A.; Sumant, A.V. Extraordinary Macroscale Wear Resistance of One Atom Thick Graphene Layer. Adv. Funct. Mater. 2014, 24, 6640–6646. [Google Scholar] [CrossRef]
- Dimov, D.; Amit, I.; Gorrie, O.; Barnes, M.D.; Townsend, N.J.; Neves, A.I.; Withers, F.; Russo, S.; Craciun, M.F. Ultrahigh Performance Nanoengineered Graphene–Concrete Composites for Multifunctional Applications. Adv. Funct. Mater. 2018, 28, 1705183. [Google Scholar] [CrossRef]
- Lalwani, G.; Henslee, A.M.; Farshid, B.; Lin, L.; Kasper, F.K.; Qin, Y.X.; Mikos, A.G.; Sitharaman, B. Two-dimensional nanostructure-reinforced biodegradable polymeric nanocomposites for bone tissue engineering. Biomacromolecules 2013, 14, 900–909. [Google Scholar] [CrossRef]
- Priyadarsini, S.; Mohanty, S.; Mukherjee, S.; Basu, S.; Mishra, M. Graphene and graphene oxide as nanomaterials for medicine and biology application. J. Nanostructure Chem. 2018, 8, 123–137. [Google Scholar] [CrossRef]
- Hoseini-Ghahfarokhi, M.; Mirkiani, S.; Mozaffari, N.; Sadatlu, M.A.A.; Ghasemi, A.; Abbaspour, S.; Akbarian, M.; Farjadain, F.; Karimi, M. Applications of Graphene and Graphene Oxide in Smart Drug/Gene Delivery: Is the World Still Flat? Int. J. Nanomed. 2020, 15, 9469–9496. [Google Scholar] [CrossRef]
- Dahlan, N.A.; Thiha, A.; Ibrahim, F.; Milić, L.; Muniandy, S.; Jamaluddin, N.F.; Petrović, B.; Kojić, S.; Stojanović, G.M. Role of Nanomaterials in the Fabrication of bioNEMS/MEMS for Biomedical Applications and towards Pioneering Food Waste Utilisation. Nanomaterials 2022, 12, 4025. [Google Scholar] [CrossRef] [PubMed]
- Hill, E.W.; Vijayaragahvan, A.; Novoselov, K. Graphene sensors. IEEE Sens. J. 2011, 11, 3161–3170. [Google Scholar] [CrossRef]
- Lawal, A.T. Synthesis and utilisation of graphene for fabrication of electrochemical sensors. Talanta 2015, 131, 424–443. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.W.; Jeong, S.Y.; Kwon, Y.W.; Lee, J.U.; Cho, S.C.; Shin, B.S. Fabrication of laser-induced graphene-based multifunctional sensing platform for sweat ion and human motion monitoring. Sens. Actuators A Phys. 2022, 334, 113320. [Google Scholar] [CrossRef]
- Tan, C.; Wang, S.; Li, S.; Liu, X.; Wei, J.; Zhang, G.; Ye, H. Cancer Diagnosis Using Terahertz-Graphene-Metasurface-Based Biosensor with Dual-Resonance Response. Nanomaterials 2022, 12, 3889. [Google Scholar] [CrossRef] [PubMed]
- Huang, C.; Li, C.; Shi, G. Graphene based catalysts. Energy Environ. Sci. 2012, 5, 8848–8868. [Google Scholar] [CrossRef]
- Hu, H.; Xin, J.H.; Hu, H.; Wang, X.; Kong, Y. Metal-free graphene-based catalyst-insight into the catalytic activity: A short review. Appl. Catal. A Gen. 2015, 492, 1–9. [Google Scholar] [CrossRef]
- Yam, K.M.; Guo, N.; Jiang, Z.; Li, S.; Zhang, C. Graphene-based heterogeneous catalysis: Role of graphene. Catalysts 2020, 10, 53. [Google Scholar] [CrossRef]
- Askari, M.B.; Rozati, S.M.; Bartolomeo, A.D. Fabrication of Mn3O4-CeO2-rGO as Nanocatalyst for Electro-Oxidation of Methanol. Nanomaterials 2022, 12, 1187. [Google Scholar] [CrossRef]
- Stoller, M.D.; Park, S.; Yanwu, Z.; An, J.; Ruoff, R.S. Graphene-Based ultracapacitors. Nano Lett. 2008, 8, 3498–3502. [Google Scholar] [CrossRef]
- Hu, S.; Lozada-Hidalgo, M.; Wang, F.C.; Mishchenko, A.; Schedin, F.; Nair, R.R.; Hill, E.W.; Boukhvalov, D.W.; Katsnelson, M.I.; Dryfe, R.A.; et al. Proton transport through one-atom-thick crystals. Nature 2014, 516, 227–230. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Chen, W.; Zhang, S.; Wu, Z.; Wang, P.; Xu, Z.; Chen, H.; Yin, W.; Zhong, H.; Lin, S. 18.5cell. Nano Energy 2015, 16, 310–319. [Google Scholar] [CrossRef]
- Jo, J.W.; Lee, J.U.; Jo, W.H. Graphene-based electrodes for flexible electronics. Polym. Int. 2015, 64, 1676–1684. [Google Scholar] [CrossRef]
- Wang, L.; Zihlmann, S.; Baumgartner, A.; Overbeck, J.; Watanabe, K.; Taniguchi, T.; Makk, P.; Schönenberger, C. In Situ Strain Tuning in hBN-Encapsulated Graphene Electronic Devices. Nano Lett. 2019, 19, 4097–4102. [Google Scholar] [CrossRef]
- Gobbi, M.; Galanti, A.; Stoeckel, M.A.; Zyska, B.; Bonacchi, S.; Hecht, S.; Samorì, P. Graphene transistors for real-time monitoring molecular self-assembly dynamics. Nat. Commun. 2020, 11, 4731. [Google Scholar] [CrossRef] [PubMed]
- Lone, S.; Bhardwaj, A.; Pandit, A.K.; Gupta, S.; Mahajan, S. A Review of Graphene Nanoribbon Field-Effect Transistor Structures. J. Electron. Mater. 2021, 50, 3169–3186. [Google Scholar] [CrossRef]
- Wang, H.; Wang, H.S.; Ma, C.; Chen, L.; Jiang, C.; Chen, C.; Xie, X.; Li, A.P.; Wang, X. Graphene nanoribbons for quantum electronics. Nat. Rev. Phys. 2021, 3, 791–802. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, Y.; Zhang, P.; Wen, D. Physical Transient Photoresistive Variable Memory Based on Graphene Quantum Dots. Nanomaterials 2022, 12, 3976. [Google Scholar] [CrossRef]
- Bistritzer, R.; MacDonald, A.H. Moiré bands in twisted double-layer graphene. Proc. Natl. Acad. Sci. USA 2011, 108, 12233–12237. [Google Scholar] [CrossRef]
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef]
- Lu, X.; Stepanov, P.; Yang, W.; Xie, M.; Aamir, M.A.; Das, I.; Urgell, C.; Watanabe, K.; Taniguchi, T.; Zhang, G.; et al. Superconductors, orbital magnets and correlated states in magic-angle bilayer graphene. Nature 2019, 574, 653–657. [Google Scholar] [CrossRef] [PubMed]
- Räsänen, E.; Heller, E.J. Optimal control of quantum revival. Eur. Phys. J. B 2013, 86, 17. [Google Scholar] [CrossRef]
- Genest, V.X.; Vinet, L.; Zhedanov, A. Quantum spin chains with fractional revival. Ann. Phys. 2016, 371, 348–367. [Google Scholar] [CrossRef]
- Rosker, M.J.; Dantus, M.; Zewail, A.H. Femtosecond real-time probing of reactions. I. The technique. J. Chem. Phys. 1988, 89, 6113–6127. [Google Scholar] [CrossRef]
- Rose, T.S.; Rosker, M.J.; Zewail, A.H. Femtosecond real-time probing of reactions. IV. The reactions of alkali halides. J. Chem. Phys. 1989, 91, 7415–7436. [Google Scholar] [CrossRef]
- Bowman, R.; Dantus, M.; Zewail, A.H. Reprint of: Femtosecond transition-state spectroscopy of iodine: From strongly bound to repulsive surface dynamics. Chem. Phys. Lett. 2013, 589, 42–45. [Google Scholar] [CrossRef][Green Version]
- Hu, J.; Vanacore, G.M.; Cepellotti, A.; Marzari, N.; Zewail, A.H. Rippling ultrafast dynamics of suspended 2D monolayers, graphene. Proc. Natl. Acad. Sci. USA 2016, 113, 2016. [Google Scholar] [CrossRef]
- Fasolino, A.; Los, J.H.; Katsnelson, M.I. Intrinsic ripples in graphene. Nat. Mater. 2007, 6, 858–861. [Google Scholar] [CrossRef]
- Meyer, J.C.; Geim, A.K.; Katsnelson, M.I.; Novoselov, K.S.; Obergfell, D.; Roth, S.; Girit, C.; Zettl, A. On the roughness of single- and bi-layer graphene membranes. Solid State Commun. 2007, 143, 101–109. [Google Scholar] [CrossRef]
- Meyer, J.C.; Geim, A.K.; Katsnelson, M.I.; Novoselov, K.S.; Booth, T.J.; Roth, S. The structure of suspended graphene sheets. Nature 2007, 446, 60–63. [Google Scholar] [CrossRef]
- Ishigami, M.; Chen, J.H.; Cullen, W.G.; Fuhrer, M.S.; Williams, E.D. Atomic structure of graphene on SiO2. Nano Lett. 2007, 7, 1643–1648. [Google Scholar] [CrossRef] [PubMed]
- Geringer, V.; Liebmann, M.; Echtermeyer, T.; Runte, S.; Schmidt, M.; Rückamp, R.; Lemme, M.C.; Morgenstern, M. Intrinsic and extrinsic corrugation of monolayer graphene deposited on SiO2. Phys. Rev. Lett. 2009, 102, 076102. [Google Scholar] [CrossRef] [PubMed]
- Lundeberg, M.B.; Folk, J.A. Rippled graphene in an in-plane magnetic field: Effects of a random vector potential. Phys. Rev. Lett. 2010, 105, 146804. [Google Scholar] [CrossRef] [PubMed]
- Deng, S.; Berry, V. Wrinkled, rippled and crumpled graphene: An overview of formation mechanism, electronic properties, and applications. Mater. Today 2016, 19, 197–212. [Google Scholar] [CrossRef]
- Peierls, R. Quelques propriétés typiques des corps solides. Ann. L’Institut Henri Poincaré 1935, 5, 177–222. [Google Scholar]
- Peierls, R.; Barut, A.O. More Surprises in Theoretical Physics. Am. J. Phys. 1992, 60, 957–958. [Google Scholar] [CrossRef]
- Lee, S.H.; Chung, H.J.; Heo, J.; Yang, H.; Shin, J.; Chung, U.I.; Seo, S. Band gap opening by two-dimensional manifestation of peierls instability in graphene. ACS Nano 2011, 5, 2964–2969. [Google Scholar] [CrossRef]
- Cortijo, A.; Vozmediano, M.A. Effects of topological defects and local curvature on the electronic properties of planar graphene. Nucl. Phys. B 2007, 763, 293–308. [Google Scholar] [CrossRef]
- Stolyarova, E.; Stolyarov, D.; Bolotin, K.; Ryu, S.; Liu, L.; Rim, K.T.; Klima, M.; Hybertsen, M.; Pogorelsky, I.; Pavlishin, I.; et al. Observation of Graphene Bubbles and Effective Mass Transport under Graphene Films. Nano Lett. 2009, 9, 332–337. [Google Scholar] [CrossRef]
- Georgiou, T.; Britnell, L.; Blake, P.; Gorbachev, R.V.; Gholinia, A.; Geim, A.K.; Casiraghi, C.; Novoselov, K.S. Graphene bubbles with controllable curvature. Appl. Phys. Lett. 2011, 99, 2011–2014. [Google Scholar] [CrossRef]
- Leconte, N.; Kim, H.; Kim, H.J.; Ha, D.H.; Watanabe, K.; Taniguchi, T.; Jung, J.; Jung, S. Graphene bubbles and their role in graphene quantum transport. Nanoscale 2017, 9, 6041–6047. [Google Scholar] [CrossRef] [PubMed]
- Alfonsi, J. Small Crystal Models for the Electronic Properties of Carbon Nanotubes. Ph.D. Thesis, Università degli Studi di Padova, Padua, Italy, 2008. [Google Scholar]
- Jiao, L.; Zhang, L.; Wang, X.; Diankov, G.; Dai, H. Narrow graphene nanoribbons from carbon nanotubes. Nature 2009, 458, 877–880. [Google Scholar] [CrossRef] [PubMed]
- Kosynkin, D.V.; Higginbotham, A.L.; Sinitskii, A.; Lomeda, J.R.; Dimiev, A.; Price, B.K.; Tour, J.M. Longitudinal unzipping of carbon nanotubes to form graphene nanoribbons. Nature 2009, 458, 872–876. [Google Scholar] [CrossRef] [PubMed]
- Hedman, D. A Theoretical Study: The Connection between Stability of Single-Walled Carbon Nanotubes and Observed Products. Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2017. [Google Scholar] [CrossRef]
- Lin, C.T.; Lee, C.Y.; Chiu, H.T.; Chin, T.S. Graphene structure in carbon nanocones and nanodiscs. Langmuir 2007, 23, 12806–12810. [Google Scholar] [CrossRef] [PubMed]
- Ulloa, P.; Latgé, A.; Oliveira, L.E.; Pacheco, M. Cone-like graphene nanostructures: Electronic and optical properties. Nanoscale Res. Lett. 2013, 8, 384. [Google Scholar] [CrossRef]
- Ma, D.; Ding, H.; Wang, X.; Yang, N.; Zhang, X. The unexpected thermal conductivity from graphene disk, carbon nanocone to carbon nanotube. Int. J. Heat Mass Transf. 2017, 108, 940–944. [Google Scholar] [CrossRef]
- Shoyama, K.; Würthner, F. Synthesis of a Carbon Nanocone by Cascade Annulation. J. Am. Chem. Soc. 2019, 141, 13008–13012. [Google Scholar] [CrossRef]
- EL-Barbary, A.A.A.; Alkhateeb, M.A. DFT Study of Se-Doped Nanocones as Highly Efficient Hydrogen Storage Carrier. Graphene 2021, 10, 49–60. [Google Scholar] [CrossRef]
- Phillips, R.; Drabold, D.A.; Lenosky, T.; Adams, G.B.; Sankey, O.F. Electronic structure of schwarzite. Phys. Rev. B 1992, 46, 1941–1943. [Google Scholar] [CrossRef]
- Braun, E.; Lee, Y.; Moosavi, S.M.; Barthel, S.; Mercado, R.; Baburin, I.A.; Proserpio, D.M.; Smit, B. Generating carbon schwarzites via zeolite-templating. Proc. Natl. Acad. Sci. USA 2018, 115. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, F.Q.; Yu, J.; Gong, S.; Li, X.; Sun, Q. 2D carbon sheets with negative Gaussian curvature assembled from pentagonal carbon nanoflakes. Phys. Chem. Chem. Phys. 2018, 20, 9123–9129. [Google Scholar] [CrossRef] [PubMed]
- Miera, G.G.; Matsubara, S.; Kono, H.; Murakami, K.; Itami, K. Synthesis of octagon-containing molecular nanocarbons. Chem. Sci. 2022, 13, 1848–1868. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Zhu, Y.; Lan, D.; Pun, S.H.; Zhou, Z.; Wei, Z.; Wang, Y.; Lee, H.K.; Lin, C.; Wang, J.; et al. Charging a Negatively Curved Nanographene and Its Covalent Network. J. Am. Chem. Soc. 2021, 143, 5231–5238. [Google Scholar] [CrossRef] [PubMed]
- Shahsavari, R.; Sakhavand, N. Junction configuration-induced mechanisms govern elastic and inelastic deformations in hybrid carbon nanomaterials. Carbon 2015, 95, 699–709. [Google Scholar] [CrossRef]
- Morresi, T.; Binosi, D.; Simonucci, S.; Piergallini, R.; Roche, S.; Pugno, N.M.; Simone, T. Exploring event horizons and Hawking radiation through deformed graphene membranes. 2D Mater. 2020, 7, 041006. [Google Scholar] [CrossRef]
- Gallerati, A. Negative-curvature spacetime solutions for graphene. J. Phy. Condens. Matter 2021, 33, 135501. [Google Scholar] [CrossRef]
- de-la Huerta-Sainz, S.; Ballesteros, A.; Cordero, N.A. Quantum Revivals in Curved Graphene Nanoflakes. Nanomaterials 2022, 12, 1953. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, 864–871. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Cordero, N.A. Funcionales de Intercambio y de Correlación en la Teoría del Funcional de la Densidad: Aplicaciones a Átomos y a Moléculas Diatómicas. Ph.D. Thesis, Universidad de Valladolid, Valladolid, Spain, 1997. [Google Scholar]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian˜16, Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Hedin, L.; Lundqvist, B.I. Explicit local exchange-correlation potentials. J. Phys. Solid State Phys. 1971, 4, 2064–2083. [Google Scholar] [CrossRef]
- Vosko, S.H.; Wilk, L.; Nusair, M. Accurate spin-dependent electron liquid correlation energies for local spin density calculations: A critical analysis. Can. J. Phys. 1980, 58, 1200–1211. [Google Scholar] [CrossRef]
- Girifalco, L.A.; Hodak, M. Van der Waals binding energies in graphitic structures. Phys. Rev. B 2002, 65, 125404. [Google Scholar] [CrossRef]
- Hasegawa, M.; Nishidate, K. Semiempirical approach to the energetics of interlayer binding in graphite. Phys. Rev. B 2004, 70, 205431. [Google Scholar] [CrossRef]
- Birowska, M.; Milowska, K.; Majewski, J.A. Van Der Waals Density Functionals for Graphene Layers and Graphite. Acta Phys. Pol. A 2011, 120, 845–848. [Google Scholar] [CrossRef]
- Hod, O. Graphite and hexagonal boron-nitride have the same interlayer distance. Why? J. Chem. Theory Comput. 2012, 8, 1360–1369. [Google Scholar] [CrossRef]
- Torres-Rojas, R.M.; Contreras-Solorio, D.A.; Hernández, L.; Enciso, A. Band gap variation in bi, tri and few-layered 2D graphene/hBN heterostructures. Solid State Commun. 2022, 341, 114553. [Google Scholar] [CrossRef]
- Khantha, M.; Cordero, N.A.; Molina, L.M.; Alonso, J.A.; Girifalco, L.A. Interaction of lithium with graphene: An ab initio study. Phys. Rev. B 2004, 70, 125422. [Google Scholar] [CrossRef]
- Cordero, N.A.; Alonso, J.A. The interaction of sulfuric acid with graphene and formation of adsorbed crystals. Nanotechnology 2007, 18, 485705. [Google Scholar] [CrossRef]
- Khantha, M.; Cordero, N.A.; Alonso, J.A.; Cawkwell, M.; Girifalco, L.A. Interaction and concerted diffusion of lithium in a (5,5) carbon nanotube. Phys. Rev. B 2008, 78, 115430. [Google Scholar] [CrossRef]
- Cordero, N.A.; Alonso, J.A. Interaction of Surfactants Containing a Sulfuric Group with a (5,5) Carbon Nanotube. J. Phys. Chem. C 2010, 114, 17249–17256. [Google Scholar] [CrossRef]
- Ayala, I.G.; Cordero, N.A.; Alonso, J.A. Surfactant effect of sulfuric acid on the exfoliation of bilayer graphene. Phys. Rev. B 2011, 84, 165424. [Google Scholar] [CrossRef]
- Ayala, I.G.; Cordero, N.A. Interaction of sodium bisulfate with mono- and bi-layer graphene. J. Nanoparticle Res. 2012, 14, 1071. [Google Scholar] [CrossRef]
- Francl, M.M.; Pietro, W.J.; Hehre, W.J.; Binkley, J.S.; Gordon, M.S.; DeFrees, D.J.; Pople, J.A. Self-consistent molecular orbital methods. XXIII. A polarization-type basis set for second-row elements. J. Chem. Phys. 1982, 77, 3654–3665. [Google Scholar] [CrossRef]
- Ganguly, S.; Kabir, M.; Saha-Dasgupta, T. Magnetic and electronic crossovers in graphene nanoflakes. Phys. Rev. B 2017, 95, 174419. [Google Scholar] [CrossRef]
- Sun, Q.; Yao, X.; Gröning, O.; Eimre, K.; Pignedoli, C.A.; Müllen, K.; Narita, A.; Fasel, R.; Ruffieux, P. Coupled Spin States in Armchair Graphene Nanoribbons with Asymmetric Zigzag Edge Extensions. Nano Lett. 2020, 20, 6429–6436. [Google Scholar] [CrossRef] [PubMed]
- Dennington, R.; Keith, T.A.; Millam, J.M. GaussView, Version 6; Semichem Inc.: Shawnee Mission, KS, USA, 2019. [Google Scholar]
- Tibbetts, G.G. Why are carbon filaments tubular? J. Cryst. Growth 1984, 66, 632–638. [Google Scholar] [CrossRef]
- Kudin, K.N.; Scuseria, G.E.; Yakobson, B.I. C2F, BN, and C nanoshell elasticity from ab initio computations. Phys. Rev. B 2001, 64, 235406. [Google Scholar] [CrossRef]
- Arroyo, M.; Belytschko, T. Finite crystal elasticity of carbon nanotubes based on the exponential Cauchy-Born rule. Phys. Rev. B 2004, 69, 115415. [Google Scholar] [CrossRef]
- Lopez, M.; Rubio, A.; Alonso, J. Deformations and thermal stability of carbon nanotube ropes. IEEE Trans. Nanotechnol. 2004, 3, 230–236. [Google Scholar] [CrossRef]
- Lu, Q.; Arroyo, M.; Huang, R. Elastic bending modulus of monolayer graphene. J. Phys. Appl. Phys. 2009, 42, 102002. [Google Scholar] [CrossRef]
- Kürti, J.; Kresse, G.; Kuzmany, H. First-principles calculations of the radial breathing mode of single-wall carbon nanotubes. Phys. Rev. B 1998, 58, R8869–R8872. [Google Scholar] [CrossRef]
- Sánchez-Portal, D.; Artacho, E.; Soler, J.M.; Rubio, A.; Ordejón, P. Ab initio structural, elastic, and vibrational properties of carbon nanotubes. Phys. Rev. B 1999, 59, 12678–12688. [Google Scholar] [CrossRef]
- Wolfram. Mathematica, Version 10.3; Wolfram: Champaign, IL, USA, 2015. [Google Scholar]
- Robinett, R. Quantum wave packet revivals. Phys. Rep. 2004, 392, 1–119. [Google Scholar] [CrossRef]
- García, T.; Rodríguez-Bolívar, S.; Cordero, N.A.; Romera, E. Wavepacket revivals in monolayer and bilayer graphene rings. J. Phys. Condens. Matter 2013, 25, 235301. [Google Scholar] [CrossRef]
- García, T.; Cordero, N.A.; Romera, E. Zitterbewegung and quantum revivals in monolayer graphene quantum dots in magnetic fields. Phys. Rev. B 2014, 89, 075416. [Google Scholar] [CrossRef]
- Bolívar, J.C.; Cordero, N.A.; Nagy, Á.; Romera, E. Fidelity as a marker of topological phase transitions in 2D Dirac materials. Int. J. Quantum Chem. 2018, 118, e25674. [Google Scholar] [CrossRef]
- Masir, M.R.; Moldovan, D.; Peeters, F.M. Pseudo magnetic field in strained graphene: Revisited. Solid State Commun. 2013, 175–176, 76–82. [Google Scholar] [CrossRef]
- Kang, D.H.; Sun, H.; Luo, M.; Lu, K.; Chen, M.; Kim, Y.; Jung, Y.; Gao, X.; Parluhutan, S.J.; Ge, J.; et al. Pseudo-magnetic field-induced slow carrier dynamics in periodically strained graphene. Nat. Commun. 2021, 12, 5087. [Google Scholar] [CrossRef]
- Ornigotti, M.; Ornigotti, L.; Biancalana, F. Generation of half-integer harmonics and efficient THz-to-visible frequency conversion in strained graphene. APL Photonics 2021, 6, 060801. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
de-la-Huerta-Sainz, S.; Ballesteros, A.; Cordero, N.A. Gaussian Curvature Effects on Graphene Quantum Dots. Nanomaterials 2023, 13, 95. https://doi.org/10.3390/nano13010095
de-la-Huerta-Sainz S, Ballesteros A, Cordero NA. Gaussian Curvature Effects on Graphene Quantum Dots. Nanomaterials. 2023; 13(1):95. https://doi.org/10.3390/nano13010095
Chicago/Turabian Stylede-la-Huerta-Sainz, Sergio, Angel Ballesteros, and Nicolás A. Cordero. 2023. "Gaussian Curvature Effects on Graphene Quantum Dots" Nanomaterials 13, no. 1: 95. https://doi.org/10.3390/nano13010095
APA Stylede-la-Huerta-Sainz, S., Ballesteros, A., & Cordero, N. A. (2023). Gaussian Curvature Effects on Graphene Quantum Dots. Nanomaterials, 13(1), 95. https://doi.org/10.3390/nano13010095





