Broadband Optical Constants and Nonlinear Properties of SnS2 and SnSe2
Abstract
:1. Introduction
2. Results and Discussion
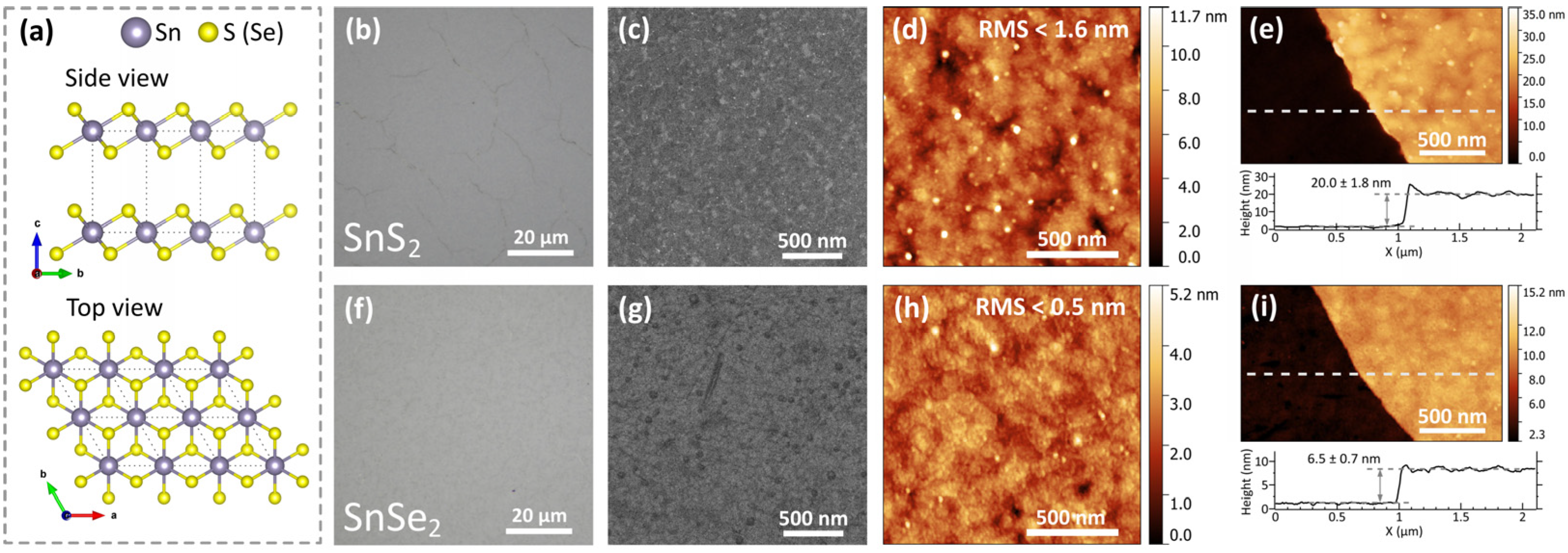
2.1. Surface and Structural Morphology Study
2.2. Analysis of the Crystal Structure and Raman Characterization
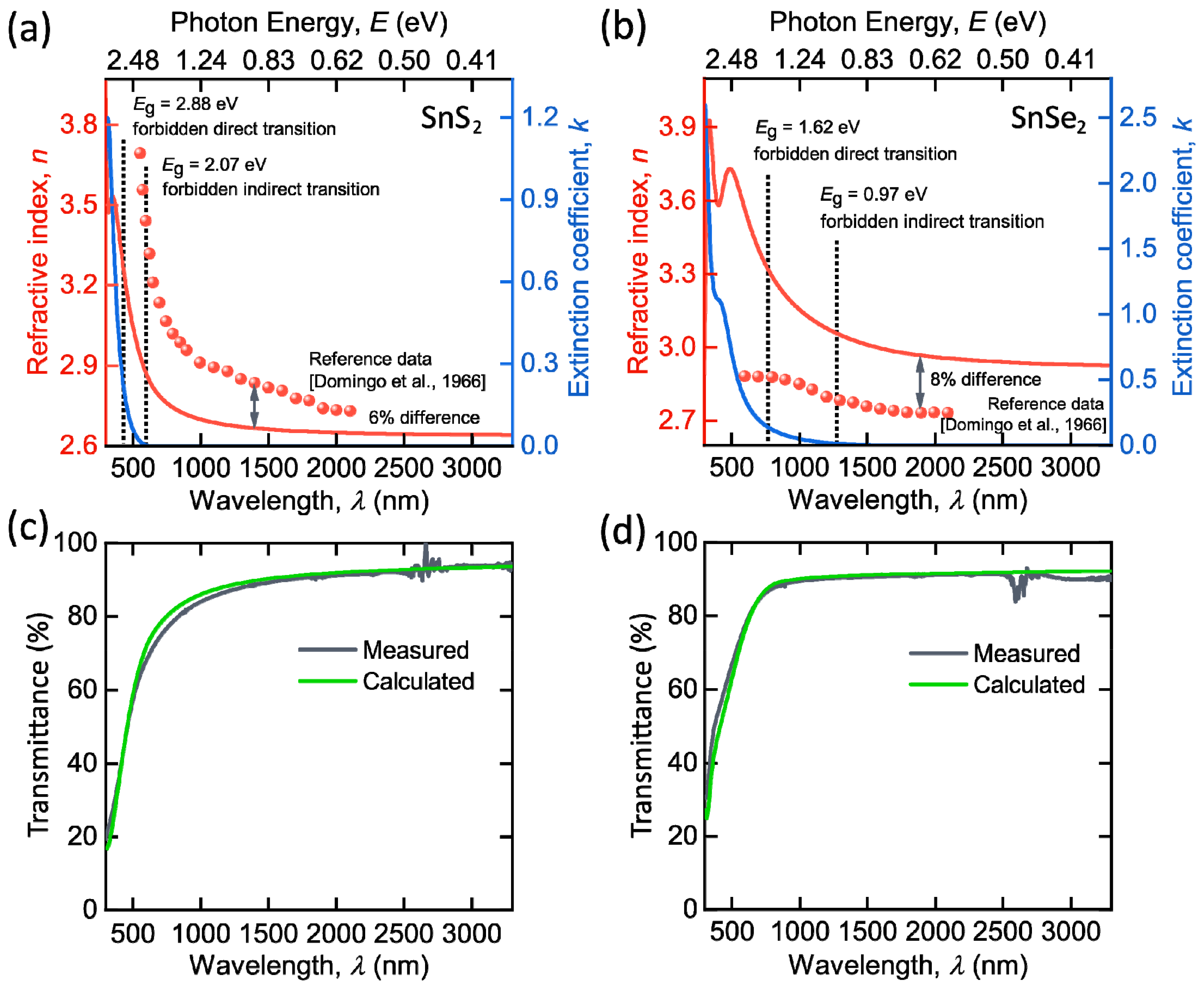
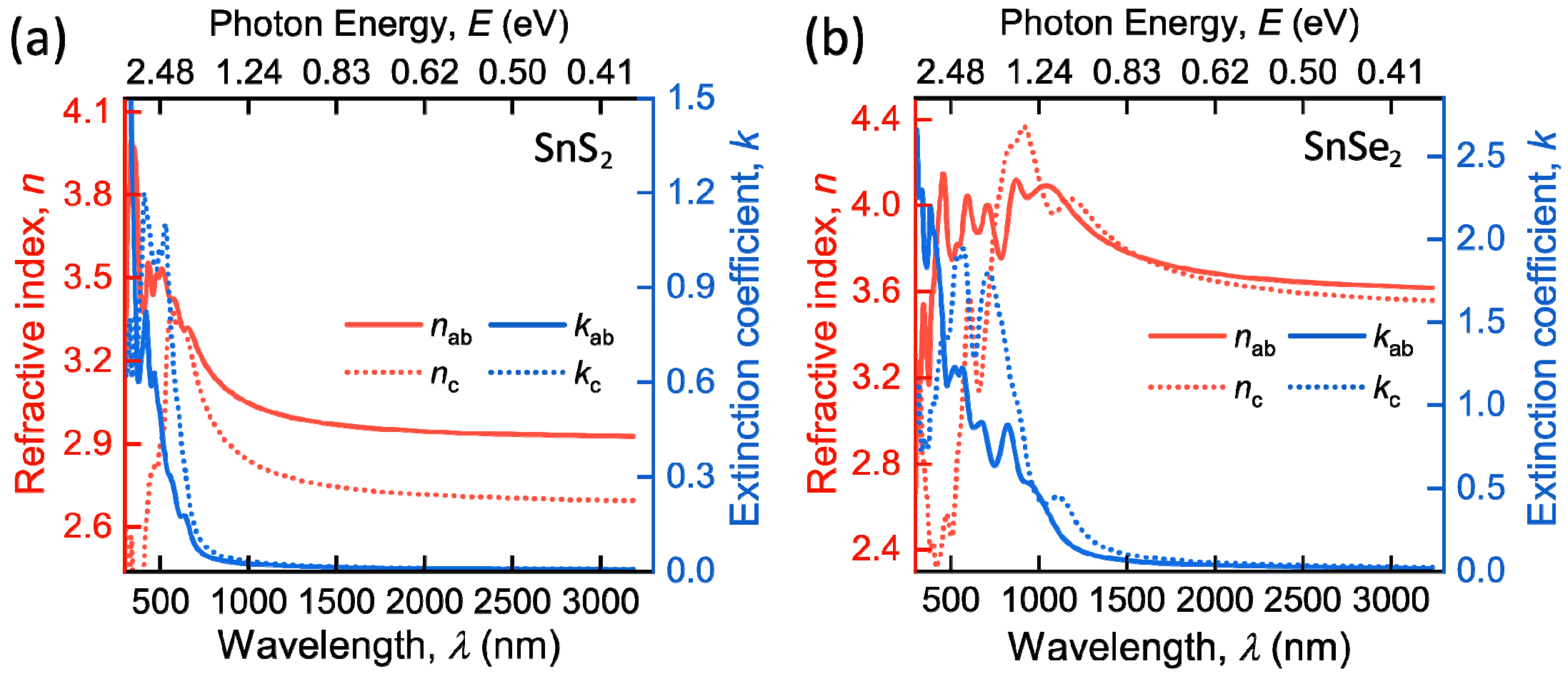
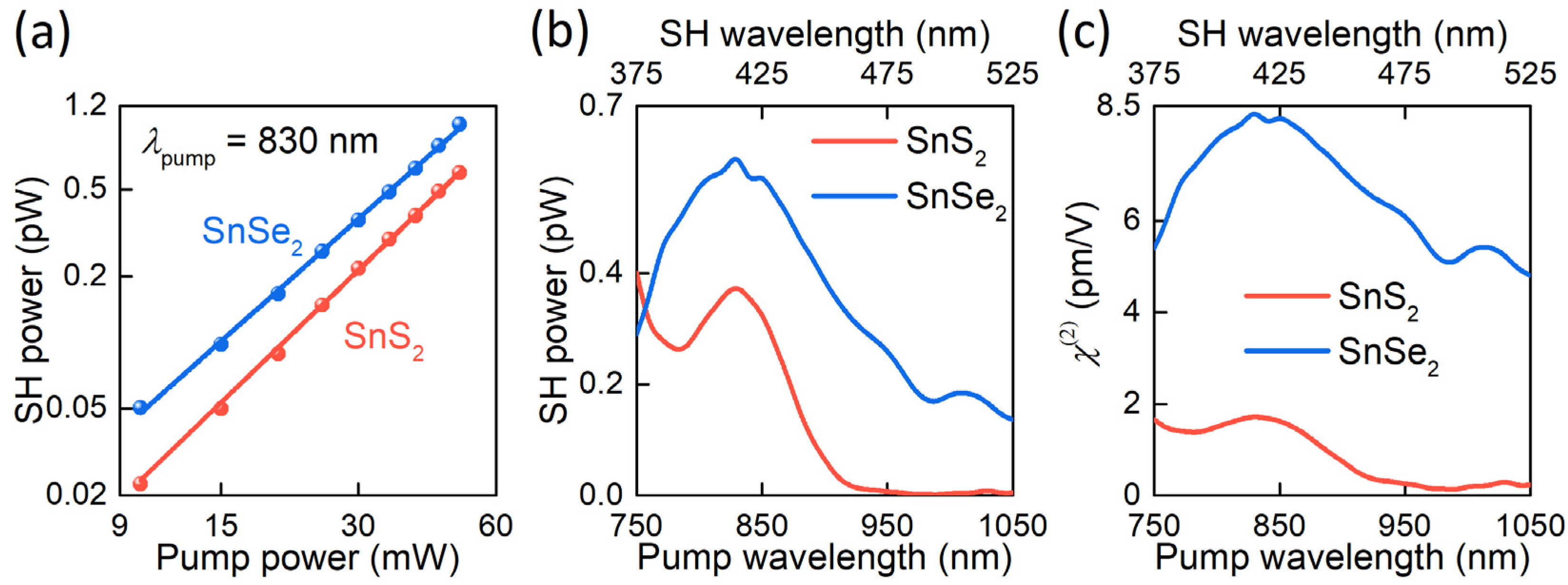
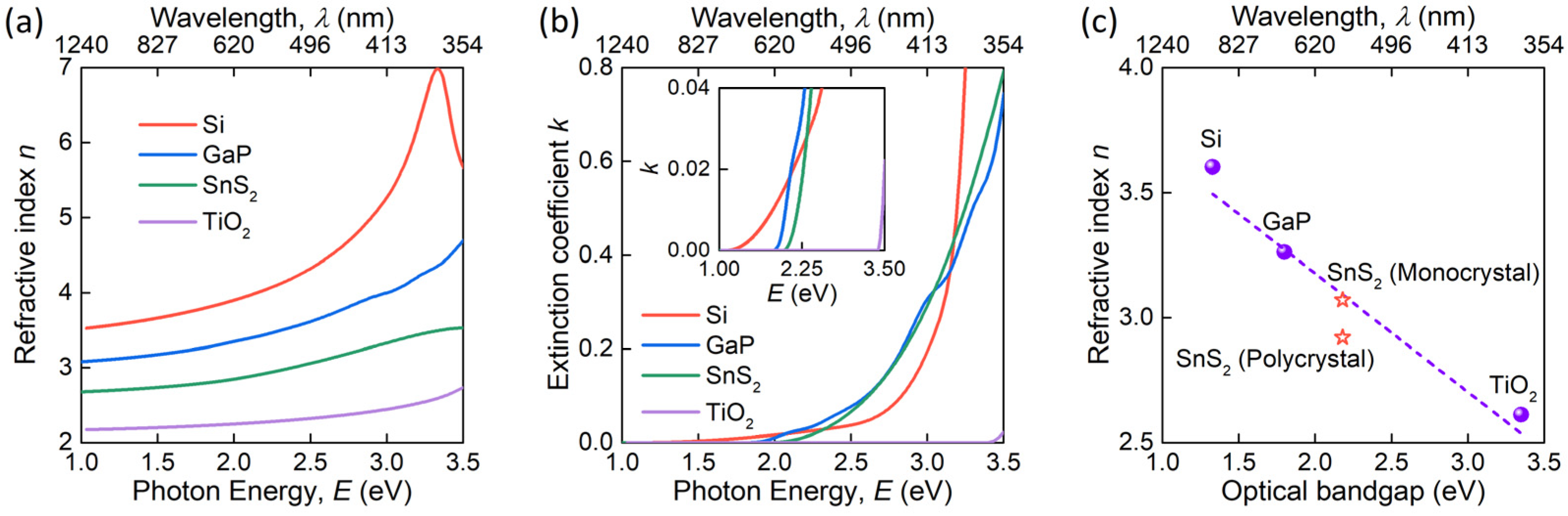
2.3. Optical Properties of SnS2 and SnSe2 Films
3. Materials and Methods
3.1. Materials
3.2. Surface Morphology Characterization
3.3. Crystal Structure Characterization
3.4. Raman Characterization
3.5. Ellipsometry Analysis
3.6. Optical Properties Characterization
3.7. First-Principle Calculations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| SnS2 | SnSe2 | |||
|---|---|---|---|---|
| λ (nm) | n | k | n | K |
| 300 | 3.8943 | 1.0436 | 2.8895 | 2.5984 |
| 350 | 3.5319 | 0.8434 | 3.8561 | 1.3915 |
| 400 | 3.3828 | 0.3664 | 3.5830 | 1.1143 |
| 450 | 3.1896 | 0.1537 | 3.6856 | 0.9836 |
| 500 | 3.0450 | 0.0599 | 3.7271 | 0.7105 |
| 550 | 2.9415 | 0.0180 | 3.6563 | 0.4900 |
| 600 | 2.8674 | 0.0021 | 3.5609 | 0.3480 |
| 650 | 2.8171 | 0.0000 | 3.4737 | 0.2566 |
| 700 | 2.7841 | 0.0000 | 3.4004 | 0.1950 |
| 750 | 2.7602 | 0.0000 | 3.3399 | 0.1515 |
| 800 | 2.7420 | 0.0000 | 3.2897 | 0.1195 |
| 850 | 2.7277 | 0.0000 | 3.2477 | 0.0952 |
| 900 | 2.7163 | 0.0000 | 3.2122 | 0.0762 |
| 1200 | 2.6782 | 0.0000 | 3.0787 | 0.0190 |
| 1500 | 2.6621 | 0.0000 | 3.0115 | 0.0021 |
| 1800 | 2.6537 | 0.0000 | 2.9751 | 0.0000 |
| 2100 | 2.6488 | 0.0000 | 3.6446 | 0.0000 |
| 2400 | 2.6456 | 0.0000 | 3.6001 | 0.0000 |
| 2700 | 2.6434 | 0.0000 | 3.5663 | 0.0000 |
| 3000 | 2.6419 | 0.0000 | 3.5400 | 0.0000 |
| 3300 | 2.6408 | 0.0000 | 3.5194 | 0.0000 |
References
- Mueller, T.; Xia, F.; Avouris, P. Graphene photodetectors for high-speed optical communications. Nat. Photonics 2010, 4, 297–301. [Google Scholar] [CrossRef] [Green Version]
- Zhang, K.; Feng, Y.; Wang, F.; Yang, Z.; Wang, J. Two dimensional hexagonal boron nitride (2D-hBN): Synthesis, properties and applications. J. Mater. Chem. C 2017, 5, 11992–12022. [Google Scholar] [CrossRef]
- Pi, L.; Li, L.; Liu, K.; Zhang, Q.; Li, H.; Zhai, T. Recent Progress on 2D Noble-Transition-Metal Dichalcogenides. Adv. Funct. Mater. 2019, 29, 1904932. [Google Scholar] [CrossRef]
- Yin, Z.; Li, H.; Li, H.; Jiang, L.; Shi, Y.; Sun, Y.; Lu, G.; Zhang, Q.; Chen, X.; Zhang, H. Single-Layer MoS2 Phototransistors. ACS Nano 2011, 6, 74–80. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mueller, T.; Malic, E. Exciton physics and device application of two-dimensional transition metal dichalcogenide semiconductors. npj 2D Mater. Appl. 2018, 2, 29. [Google Scholar] [CrossRef] [Green Version]
- Thakar, K.; Lodha, S. Optoelectronic and photonic devices based on transition metal dichalcogenides. Mater. Res. Express 2020, 7, 014002. [Google Scholar] [CrossRef]
- Manzeli, S.; Ovchinnikov, D.; Pasquier, D.; Yazyev, O.; Kis, A. 2D transition metal dichalcogenides. Nat. Rev. Mater. 2017, 2, 17033. [Google Scholar] [CrossRef]
- El-Sayed, M.; Ermolaev, G.; Voronin, K.; Romanov, R.; Tselikov, G.; Yakubovsky, D.; Doroshina, N.; Nemtsov, A.; Solovey, V.; Voronov, A.; et al. Optical Constants of Chemical Vapor Deposited Graphene for Photonic Applications. Nanomaterials 2021, 11, 1230. [Google Scholar] [CrossRef]
- Geim, A.K.; Grigorieva, I.V. Van der Waals heterostructures. Nature 2013, 499, 419–425. [Google Scholar] [CrossRef] [PubMed]
- Novoselov, K.S.; Mishchenko, A.; Carvalho, A.; Neto, A.H.C. 2D materials and van der Waals heterostructures. Science 2016, 353, aac9439. [Google Scholar] [CrossRef] [Green Version]
- Verre, R.; Baranov, D.G.; Munkhbat, B.; Cuadra, J.; Käll, M.; Shegai, T. Transition metal dichalcogenide nanodisks as high-index dielectric Mie nanoresonators. Nat. Nanotechnol. 2019, 14, 679–683. [Google Scholar] [CrossRef] [Green Version]
- Munkhbat, B.; Yankovich, A.B.; Baranov, D.G.; Verre, R.; Olsson, E.; Shegai, T.O. Transition metal dichalcogenide metamaterials with atomic precision. Nat. Commun. 2020, 11, 4604. [Google Scholar] [CrossRef] [PubMed]
- Mupparapu, R.; Steinert, M.; George, A.; Tang, Z.; Turchanin, A.; Pertsch, T.; Staude, I. Facile Resist-Free Nanopatterning of Monolayers of MoS2 by Focused Ion-Beam Milling. Adv. Mater. Interfaces 2020, 7, 2000858. [Google Scholar] [CrossRef]
- Castellanos-Gomez, A.; Barkelid, M.; Goossens, A.M.; Calado, V.E.; van der Zant, H.S.J.; Steele, G.A. Laser-Thinning of MoS2: On Demand Generation of a Single-Layer Semiconductor. Nano Lett. 2012, 12, 3187–3192. [Google Scholar] [CrossRef] [Green Version]
- Lin, H.; Xu, Z.-Q.; Cao, G.; Zhang, Y.; Zhou, J.; Wang, Z.; Wan, Z.; Liu, Z.; Loh, K.P.; Qiu, C.-W.; et al. Diffraction-limited imaging with monolayer 2D material-based ultrathin flat lenses. Light. Sci. Appl. 2020, 9, 137. [Google Scholar] [CrossRef]
- Mohan, V.B.; Lau, K.-T.; Hui, D.; Bhattacharyya, D. Graphene-based materials and their composites: A review on production, applications and product limitations. Compos. Part B Eng. 2018, 142, 200–220. [Google Scholar] [CrossRef]
- Castellanos-Gomez, A. Why all the fuss about 2D semiconductors? Nat. Photonics 2016, 10, 202–204. [Google Scholar] [CrossRef] [Green Version]
- Caldwell, J.D.; Aharonovich, I.; Cassabois, G.; Edgar, J.H.; Gil, B.; Basov, D.N. Photonics with hexagonal boron nitride. Nat. Rev. Mater. 2019, 4, 552–567. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mak, K.F.; Lee, C.; Hone, J.; Shan, J.; Heinz, T.F. Atomically Thin MoS2: A New Direct-Gap Semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [Green Version]
- Dean, C.R.; Young, A.; Meric, I.; Lee, C.; Wang, L.; Sorgenfrei, S.; Watanabe, K.; Taniguchi, T.; Kim, P.; Shepard, K.L.; et al. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 2010, 5, 722–726. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Mounet, N.; Gibertini, M.; Schwaller, P.; Campi, D.; Merkys, A.; Marrazzo, A.; Sohier, T.; Castelli, I.E.; Cepellotti, A.; Pizzi, G.; et al. Two-dimensional materials from high-throughput computational exfoliation of experimentally known compounds. Nat. Nanotechnol. 2018, 13, 246–252. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.; Xu, K.; Wang, Z.; Shifa, T.A.; Wang, Q.; Wang, F.; Jiang, C.; He, J. Designing the shape evolution of SnSe2 nanosheets and their optoelectronic properties. Nanoscale 2015, 7, 17375–17380. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Sutter, E.; Sadowski, J.; Cotlet, M.; Monti, O.L.; Racke, D.A.; Neupane, M.R.; Wickramaratne, D.; Lake, R.; Parkinson, B.A.; et al. Tin Disulfide—An Emerging Layered Metal Dichalcogenide Semiconductor: Materials Properties and Device Characteristics. ACS Nano 2014, 8, 10743–10755. [Google Scholar] [CrossRef] [PubMed]
- Domingo, G.; Itoga, R.S.; Kannewurf, C.R. Fundamental Optical Absorption in SnS2 and SnSe2. Phys. Rev. (Ser. I) 1966, 143, 536–541. [Google Scholar] [CrossRef]
- Burton, L.A.; Whittles, T.J.; Hesp, D.; Linhart, W.M.; Skelton, J.M.; Hou, B.; Webster, R.F.; O’Dowd, G.; Reece, C.; Cherns, D.; et al. Electronic and optical properties of single crystal SnS2: An earth-abundant disulfide photocatalyst. J. Mater. Chem. A 2015, 4, 1312–1318. [Google Scholar] [CrossRef] [Green Version]
- Bertrand, Y.; Leveque, G.; Raisin, C.; Levy, F. Optical properties of SnSe2 and SnS2. J. Phys. C Solid State Phys. 1979, 12, 2907–2916. [Google Scholar] [CrossRef]
- Bordas, J.; Robertson, J.; Jakobsson, A. Ultraviolet properties and band structure of SnS2, SnSe2, CdI2, PbI2, BiI3 and BiOI crystals. J. Phys. C Solid State Phys. 1978, 11, 2607–2621. [Google Scholar] [CrossRef]
- Mandalidis, S.; Kalomiros, J.; Kambas, K.; Anagnostopoulos, A.N. Optical investigation of SnS2 single crystals. J. Mater. Sci. 1996, 31, 5975–5978. [Google Scholar] [CrossRef]
- Song, H.S.; Li, S.L.; Gao, L.; Xu, Y.; Ueno, K.; Tang, J.; Cheng, Y.B.; Tsukagoshi, K. High-performance top-gated monolayer SnS2 field-effect transistors and their integrated logic circuits. Nanoscale 2013, 5, 9666–9670. [Google Scholar] [CrossRef] [PubMed]
- Su, G.; Hadjiev, V.; Loya, P.E.; Zhang, J.; Lei, S.; Maharjan, S.; Dong, P.; Ajayan, P.M.; Lou, J.; Peng, H. Chemical Vapor Deposition of Thin Crystals of Layered Semiconductor SnS2 for Fast Photodetection Application. Nano Lett. 2014, 15, 506–513. [Google Scholar] [CrossRef] [PubMed]
- Su, Y.; Ebrish, M.A.; Olson, E.J.; Koester, S.J. SnSe2 field-effect transistors with high drive current. Appl. Phys. Lett. 2013, 103, 263104. [Google Scholar] [CrossRef]
- Tan, F.; Qu, S.; Wu, J.; Liu, K.; Zhou, S.; Wang, Z. Preparation of SnS2 colloidal quantum dots and their application in organic/inorganic hybrid solar cells. Nanoscale Res. Lett. 2011, 6, 298. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bai, Y.; Zong, X.; Yu, H.; Chen, Z.-G.; Wang, L. Scalable Low-Cost SnS2 Nanosheets as Counter Electrode Building Blocks for Dye-Sensitized Solar Cells. Chem. Eur. J. 2014, 20, 8670–8676. [Google Scholar] [CrossRef]
- Biswas, R.; Dandu, M.; Prosad, A.; Das, S.; Menon, S.; Deka, J.; Majumdar, K.; Raghunathan, V. Strong near band-edge excited second-harmonic generation from multilayer 2H Tin diselenide. Sci. Rep. 2021, 11, 15017. [Google Scholar] [CrossRef]
- Yang, H.R.; Liu, X.M. Nonlinear optical response and applications of tin disulfide in the near- and mid-infrared. Appl. Phys. Lett. 2017, 110, 171106. [Google Scholar] [CrossRef]
- Lu, H.; Wang, Z.; Huang, Z.; Tao, J.; Xiong, H.; Qiu, W.; Guan, H.; Dong, H.; Dong, J.; Zhu, W.; et al. Resonance-assisted light–control–light characteristics of SnS2 on a microfiber knot resonator with fast response. Photonics Res. 2018, 6, 1137–1143. [Google Scholar] [CrossRef]
- Müller, M.; Zentel, R.; Maka, T.; Romanov, S.G.; Sotomayor Torres, C.M. Photonic Crystal Films with High Refractive Index Contrast. Adv. Mater. 2000, 12, 1499–1503. [Google Scholar] [CrossRef]
- Jin, Y.; Zhu, Y.; Yang, X.; Jiang, H.; Li, C. In situ synthesis of sulfide-coated polystyrene composites for the fabrication of photonic crystals. J. Colloid Interface Sci. 2006, 301, 130–136. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Zheng, Z.; Li, Y.; Zhao, Y.; Xu, L.; Deng, H.; Li, J. High performance tin diselenide photodetectors dependent on thickness: A vertical graphene sandwiched device and interfacial mechanism. Nanoscale 2019, 11, 13309–13317. [Google Scholar] [CrossRef]
- Fan, C.; Liu, Z.; Yuan, S.; Meng, X.; An, X.; Jing, Y.; Sun, C.; Zhang, Y.; Zhang, Z.; Wang, M.; et al. Enhanced Photodetection Performance of Photodetectors Based on Indium-Doped Tin Disulfide Few Layers. ACS Appl. Mater. Interfaces 2021, 13, 35889–35896. [Google Scholar] [CrossRef]
- Joseph, A.; Anjitha, C.; Aravind, A.; Aneesh, P. Structural, optical and magnetic properties of SnS2 nanoparticles and photo response characteristics of p-Si/n-SnS2 heterojunction diode. Appl. Surf. Sci. 2020, 528, 146977. [Google Scholar] [CrossRef]
- Smith, A.J.; Meek, P.E.; Liang, W.Y. Raman scattering studies of SnS2 and SnSe2. J. Phys. C Solid State Phys. 1977, 10, 1321–1323. [Google Scholar] [CrossRef]
- Mitchell, R.S.; Fujiki, Y.; Ishizawa, Y. Structural polytypism of SnS2. Nature 1974, 247, 537–538. [Google Scholar] [CrossRef]
- Gonzalez, J.M.; Oleynik, I.I. Layer-dependent properties of SnS2 and SnSe2 two-dimensional materials. Phys. Rev. B 2016, 94, 125443. [Google Scholar] [CrossRef] [Green Version]
- Lin, D.-Y.; Hsu, H.-P.; Tsai, C.-F.; Wang, C.-W.; Shih, Y.-T. Temperature Dependent Excitonic Transition Energy and Enhanced Electron-Phonon Coupling in Layered Ternary SnS2-xSex Semiconductors with Fully Tunable Stoichiometry. Molecules 2021, 26, 2184. [Google Scholar] [CrossRef] [PubMed]
- Lin, G.; Zheng, T.; Zhan, L.; Lu, J.; Huang, J.; Wang, H.; Zhou, Y.; Zhang, X.; Cai, W. Tunable structure and optical properties of single crystal SnS2 flakes. Appl. Phys. Express 2020, 13, 035504. [Google Scholar] [CrossRef]
- Shown, I.; Samireddi, S.; Chang, Y.-C.; Putikam, R.; Chang, P.-H.; Sabbah, A.; Fu, F.-Y.; Chen, W.-F.; Wu, C.-I.; Yu, T.-Y.; et al. Carbon-doped SnS2 nanostructure as a high-efficiency solar fuel catalyst under visible light. Nat. Commun. 2018, 9, 169. [Google Scholar] [CrossRef] [Green Version]
- Lee, N.; Lee, G.; Choi, H.; Park, H.; Choi, Y.; Seo, H.; Ju, H.; Kim, S.; Sul, O.; Lee, J.; et al. Layered deposition of SnS2 grown by atomic layer deposition and its transport properties. Nanotechnology 2019, 30, 405707. [Google Scholar] [CrossRef]
- Zhang, Y.; Shi, Y.; Wu, M.; Zhang, K.; Man, B.; Liu, M. Synthesis and Surface-Enhanced Raman Scattering of Ultrathin SnSe2 Nanoflakes by Chemical Vapor Deposition. Nanomaterials 2018, 8, 515. [Google Scholar] [CrossRef] [Green Version]
- Ermolaev, G.A.; Yakubovsky, D.I.; Stebunov, Y.V.; Arsenin, A.V.; Volkov, V.S. Spectral ellipsometry of monolayer transition metal dichalcogenides: Analysis of excitonic peaks in dispersion. J. Vac. Sci. Technol. B 2020, 38, 014002. [Google Scholar] [CrossRef]
- Ermolaev, G.; El-Sayed, M.; Yakubovsky, D.; Voronin, K.; Romanov, R.; Tatmyshevskiy, M.; Doroshina, N.; Nemtsov, A.; Voronov, A.; Novikov, S.; et al. Optical Constants and Structural Properties of Epitaxial MoS2 Monolayers. Nanomaterials 2021, 11, 1411. [Google Scholar] [CrossRef]
- Ermolaev, G.A.; Voronin, K.V.; Tatmyshevskiy, M.K.; Mazitov, A.B.; Slavich, A.S.; Yakubovsky, D.I.; Tselin, A.P.; Mironov, M.S.; Romanov, R.I.; Markeev, A.M.; et al. Broadband Optical Properties of Atomically Thin PtS2 and PtSe2. Nanomaterials 2021, 11, 3269. [Google Scholar] [CrossRef]
- Jellison, G.E.; Modine, F.A. Parameterization of the optical functions of amorphous materials in the interband region. Appl. Phys. Lett. 1996, 69, 371–373. [Google Scholar] [CrossRef]
- Ermolaev, G.A.; Stebunov, Y.V.; Vyshnevyy, A.A.; Tatarkin, D.E.; Yakubovsky, D.I.; Novikov, S.M.; Baranov, D.G.; Shegai, T.; Nikitin, A.Y.; Arsenin, A.V.; et al. Broadband optical properties of monolayer and bulk MoS2. npj 2D Mater. Appl. 2020, 4, 21. [Google Scholar] [CrossRef]
- Niu, Y.; Gonzalez-Abad, S.; Frisenda, R.; Marauhn, P.; Drüppel, M.; Gant, P.; Schmidt, R.; Taghavi, N.S.; Barcons, D.; Molina-Mendoza, A.J.; et al. Thickness-Dependent Differential Reflectance Spectra of Monolayer and Few-Layer MoS2, MoSe2, WS2 and WSe2. Nanomaterials 2018, 8, 725. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Passler, N.C.; Paarmann, A. Generalized 4 × 4 matrix formalism for light propagation in anisotropic stratified media: Study of surface phonon polaritons in polar dielectric heterostructures. J. Opt. Soc. Am. B 2017, 34, 2128–2139. [Google Scholar] [CrossRef] [Green Version]
- Ermolaev, G.A.; Tsapenko, A.P.; Volkov, V.S.; Anisimov, A.S.; Gladush, Y.G.; Nasibulin, A.G. Express determination of thickness and dielectric function of single-walled carbon nanotube films. Appl. Phys. Lett. 2020, 116, 231103. [Google Scholar] [CrossRef]
- Ermolaev, G.A.; Grudinin, D.V.; Stebunov, Y.V.; Voronin, K.V.; Kravets, V.G.; Duan, J.; Mazitov, A.B.; Tselikov, G.I.; Bylinkin, A.; Yakubovsky, D.I.; et al. Giant optical anisotropy in transition metal dichalcogenides for next-generation photonics. Nat. Commun. 2021, 12, 854. [Google Scholar] [CrossRef]
- Niu, K.; Chen, Q.; Sun, R.; Man, B.; Zhang, H. Passively Q-switched erbium-doped fiber laser based on SnS2 saturable absorber. Opt. Mater. Express 2017, 7, 3934–3943. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics; Elsevier: Amsterdam, The Netherlands, 2003; ISBN 9780121216825. [Google Scholar]
- Evlyukhin, A.B.; Novikov, S.M.; Zywietz, U.; Eriksen, R.L.; Reinhardt, C.; Bozhevolnyi, S.I.; Chichkov, B.N. Demonstration of Magnetic Dipole Resonances of Dielectric Nanospheres in the Visible Region. Nano Lett. 2012, 12, 3749–3755. [Google Scholar] [CrossRef]
- Baranov, D.G.; Zuev, D.A.; Lepeshov, S.I.; Kotov, O.V.; Krasnok, A.E.; Evlyukhin, A.B.; Chichkov, B.N. All-dielectric nanophotonics: The quest for better materials and fabrication techniques. Optica 2017, 4, 814–825. [Google Scholar] [CrossRef]
- Khmelevskaia, D.; Markina, D.I.; Fedorov, V.V.; Ermolaev, G.A.; Arsenin, A.V.; Volkov, V.S.; Goltaev, A.S.; Zadiranov, Y.M.; Tzibizov, I.A.; Pushkarev, A.P.; et al. Directly grown crystalline gallium phosphide on sapphire for nonlinear all-dielectric nanophotonics. Appl. Phys. Lett. 2021, 118, 201101. [Google Scholar] [CrossRef]
- Herzinger, C.M.; Johs, B.D.; McGahan, W.A.; Woollam, J.A.; Paulson, W.M. Ellipsometric determination of optical constants for silicon and thermally grown silicon dioxide via a multi-sample, multi-wavelength, multi-angle investigation. J. Appl. Phys. 1998, 83, 3323–3336. [Google Scholar] [CrossRef]
- Tiwald, T.E.; Schubert, M. Measurement of rutile TiO2 dielectric tensor from 0.148 to 33 μm using generalized ellipsometry. Opt. Diagn. Methods Inorg. Mater. II 2000, 4103, 19–29. [Google Scholar]
- Ermolaev, G.; Kushnir, S.E.; Sapoletova, N.A.; Napolskii, K.S. Titania Photonic Crystals with Precise Photonic Band Gap Position via Anodizing with Voltage versus Optical Path Length Modulation. Nanomaterials 2019, 9, 651. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Popkova, A.A.; Antropov, I.M.; Fröch, J.E.; Kim, S.; Aharonovich, I.; Bessonov, V.O.; Solntsev, A.S.; Fedyanin, A.A. Optical Third-Harmonic Generation in Hexagonal Boron Nitride Thin Films. ACS Photon 2021, 8, 824–831. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes forab initiototal-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Shishkin, M.; Kresse, G. Implementation and performance of the frequency-dependentGWmethod within the PAW framework. Phys. Rev. B 2006, 74, 035101. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ermolaev, G.A.; Yakubovsky, D.I.; El-Sayed, M.A.; Tatmyshevskiy, M.K.; Mazitov, A.B.; Popkova, A.A.; Antropov, I.M.; Bessonov, V.O.; Slavich, A.S.; Tselikov, G.I.; et al. Broadband Optical Constants and Nonlinear Properties of SnS2 and SnSe2. Nanomaterials 2022, 12, 141. https://doi.org/10.3390/nano12010141
Ermolaev GA, Yakubovsky DI, El-Sayed MA, Tatmyshevskiy MK, Mazitov AB, Popkova AA, Antropov IM, Bessonov VO, Slavich AS, Tselikov GI, et al. Broadband Optical Constants and Nonlinear Properties of SnS2 and SnSe2. Nanomaterials. 2022; 12(1):141. https://doi.org/10.3390/nano12010141
Chicago/Turabian StyleErmolaev, Georgy A., Dmitry I. Yakubovsky, Marwa A. El-Sayed, Mikhail K. Tatmyshevskiy, Arslan B. Mazitov, Anna A. Popkova, Ilya M. Antropov, Vladimir O. Bessonov, Aleksandr S. Slavich, Gleb I. Tselikov, and et al. 2022. "Broadband Optical Constants and Nonlinear Properties of SnS2 and SnSe2" Nanomaterials 12, no. 1: 141. https://doi.org/10.3390/nano12010141
APA StyleErmolaev, G. A., Yakubovsky, D. I., El-Sayed, M. A., Tatmyshevskiy, M. K., Mazitov, A. B., Popkova, A. A., Antropov, I. M., Bessonov, V. O., Slavich, A. S., Tselikov, G. I., Kruglov, I. A., Novikov, S. M., Vyshnevyy, A. A., Fedyanin, A. A., Arsenin, A. V., & Volkov, V. S. (2022). Broadband Optical Constants and Nonlinear Properties of SnS2 and SnSe2. Nanomaterials, 12(1), 141. https://doi.org/10.3390/nano12010141









