Spontaneous Zero-Field Cooling Exchange Bias in Ni–Co–Mn–Sn Metamagnetic Heusler Sputtered Film
Abstract
:1. Introduction
2. Materials and Methods
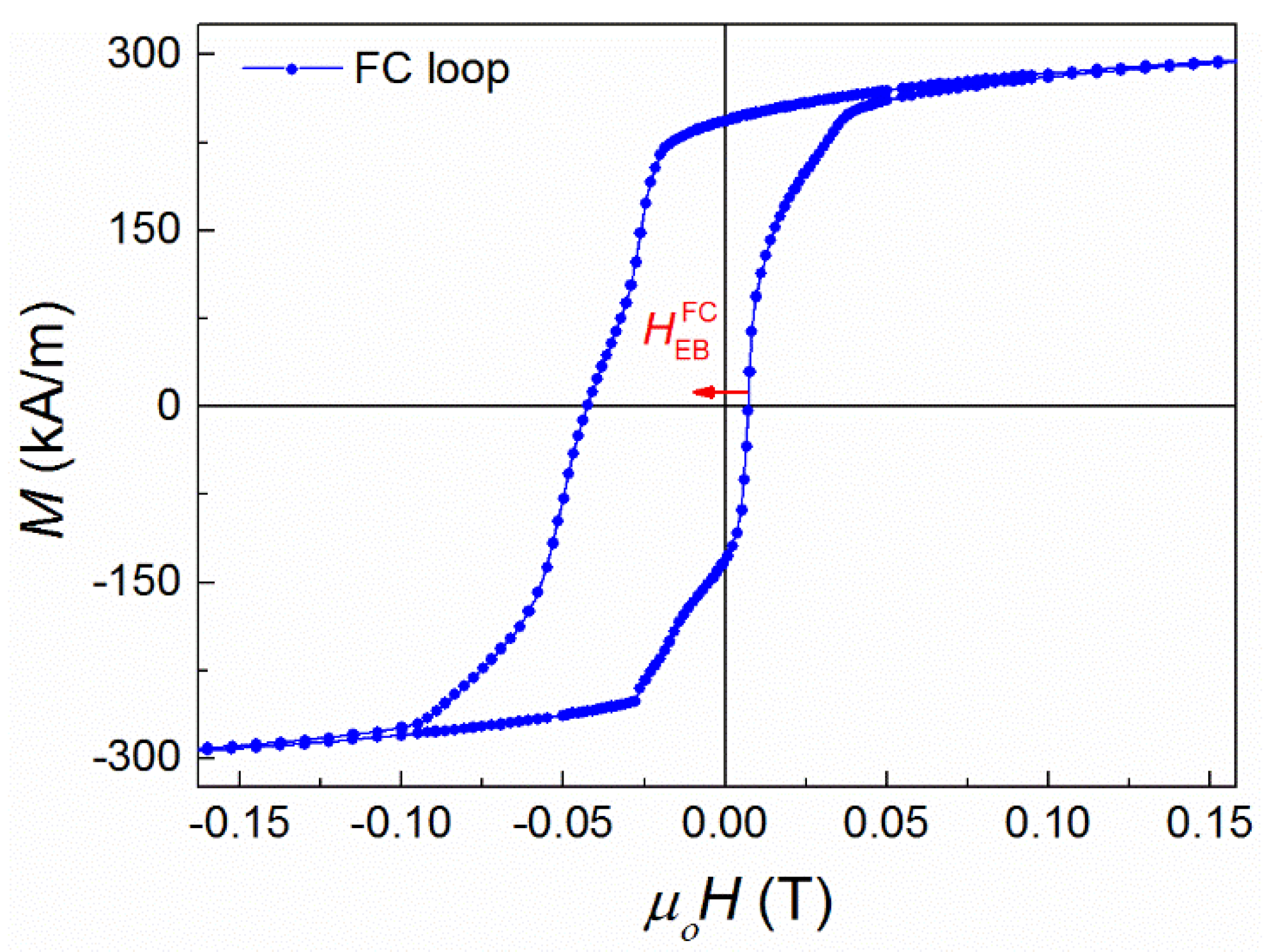
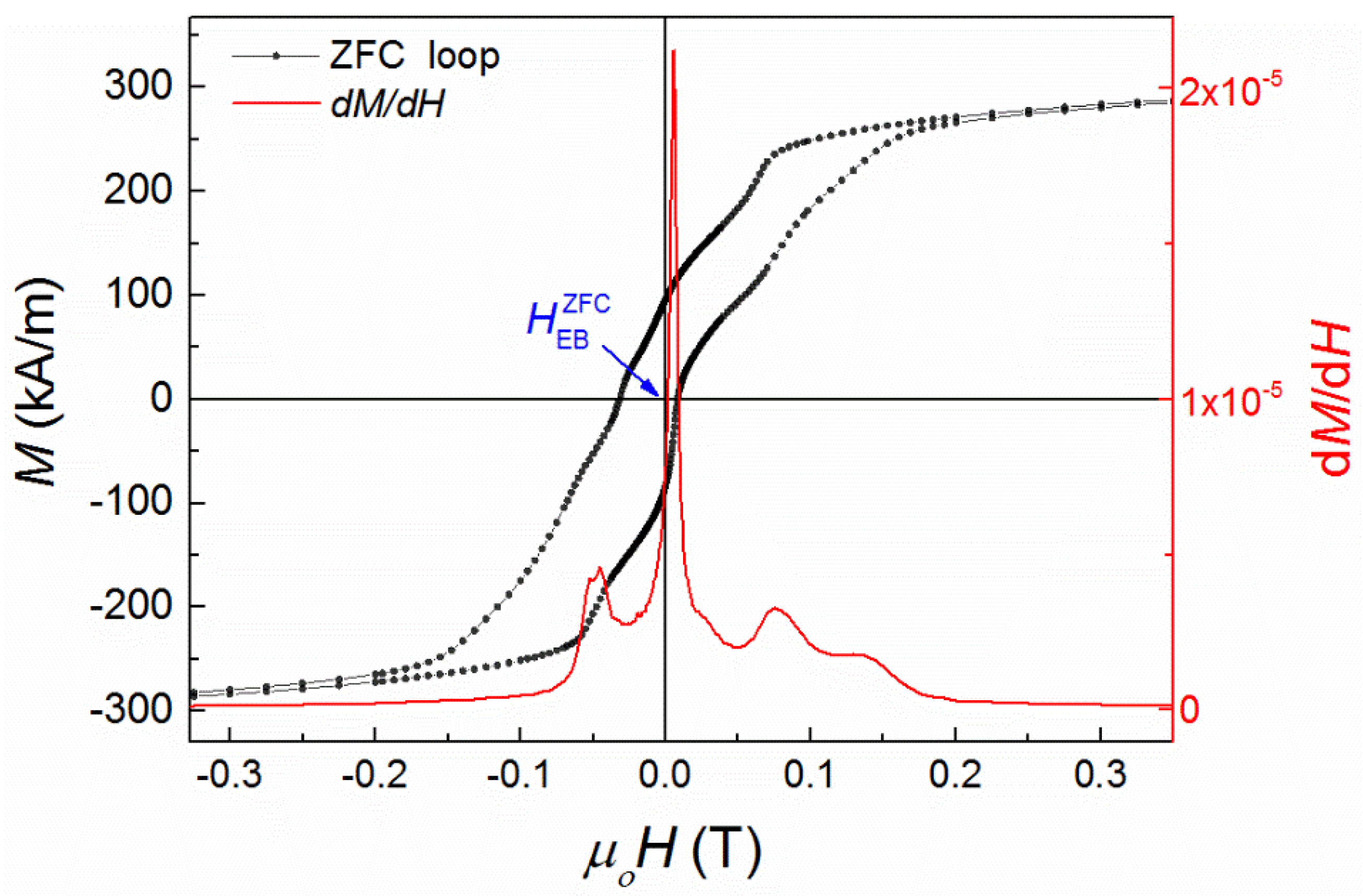
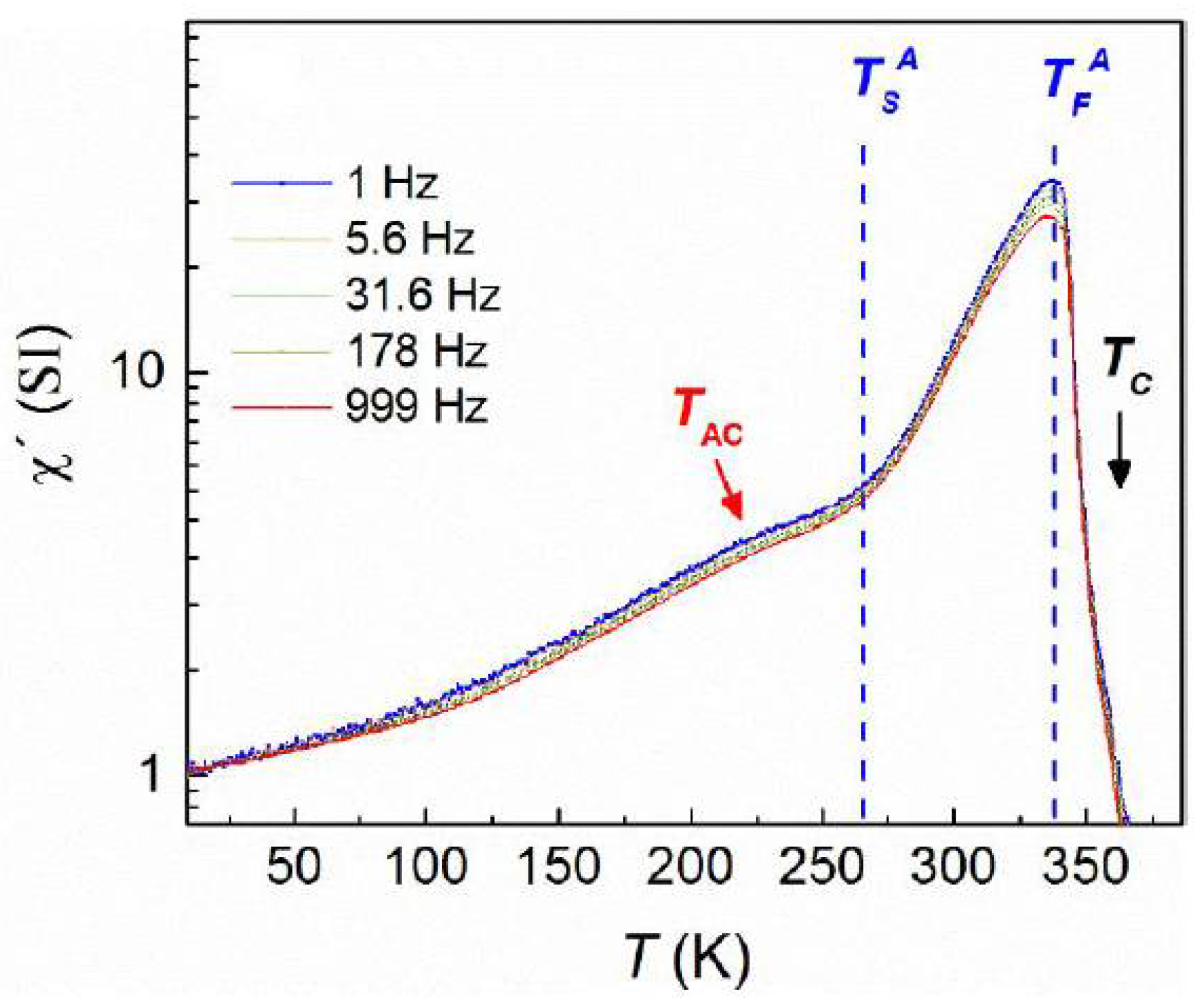
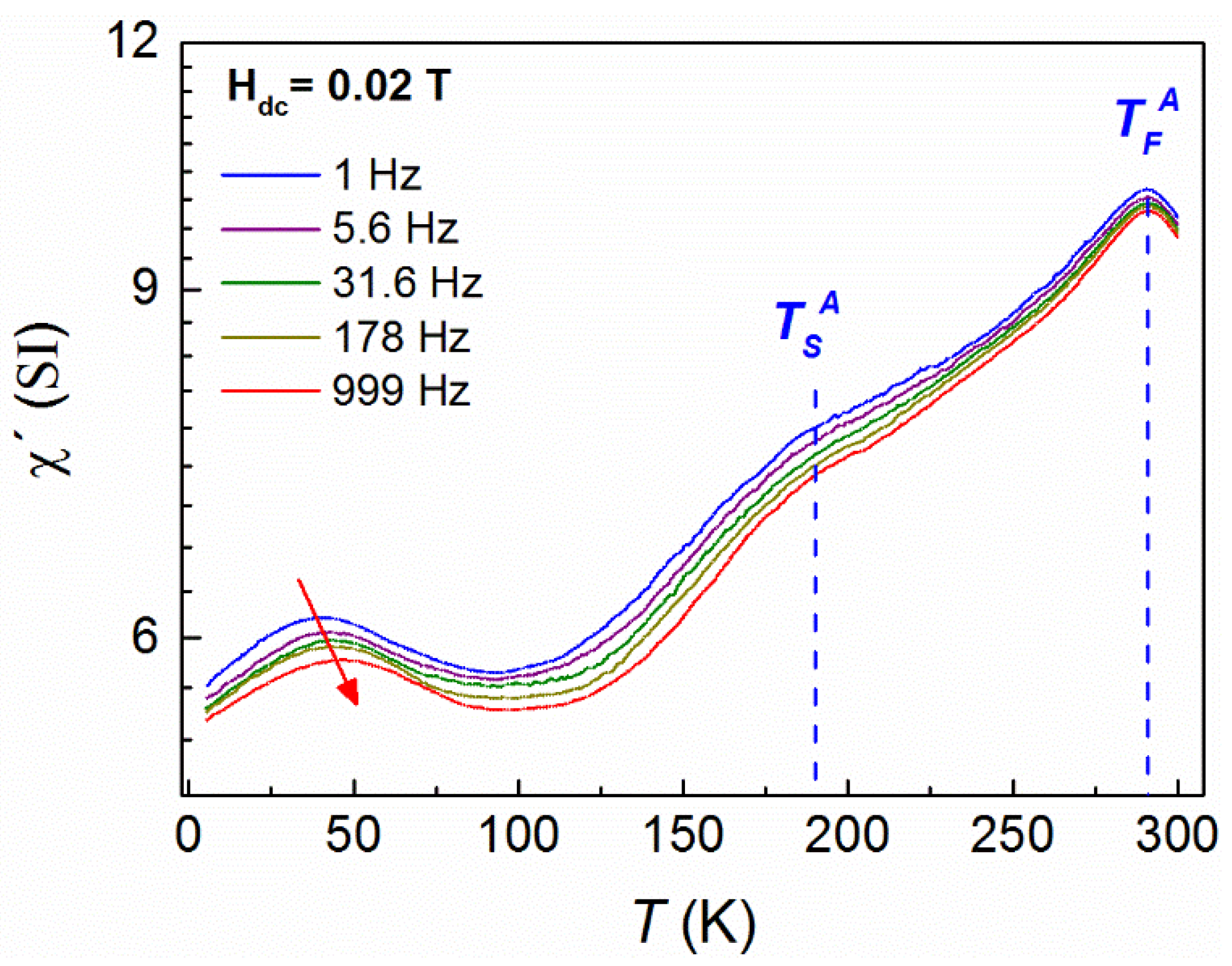
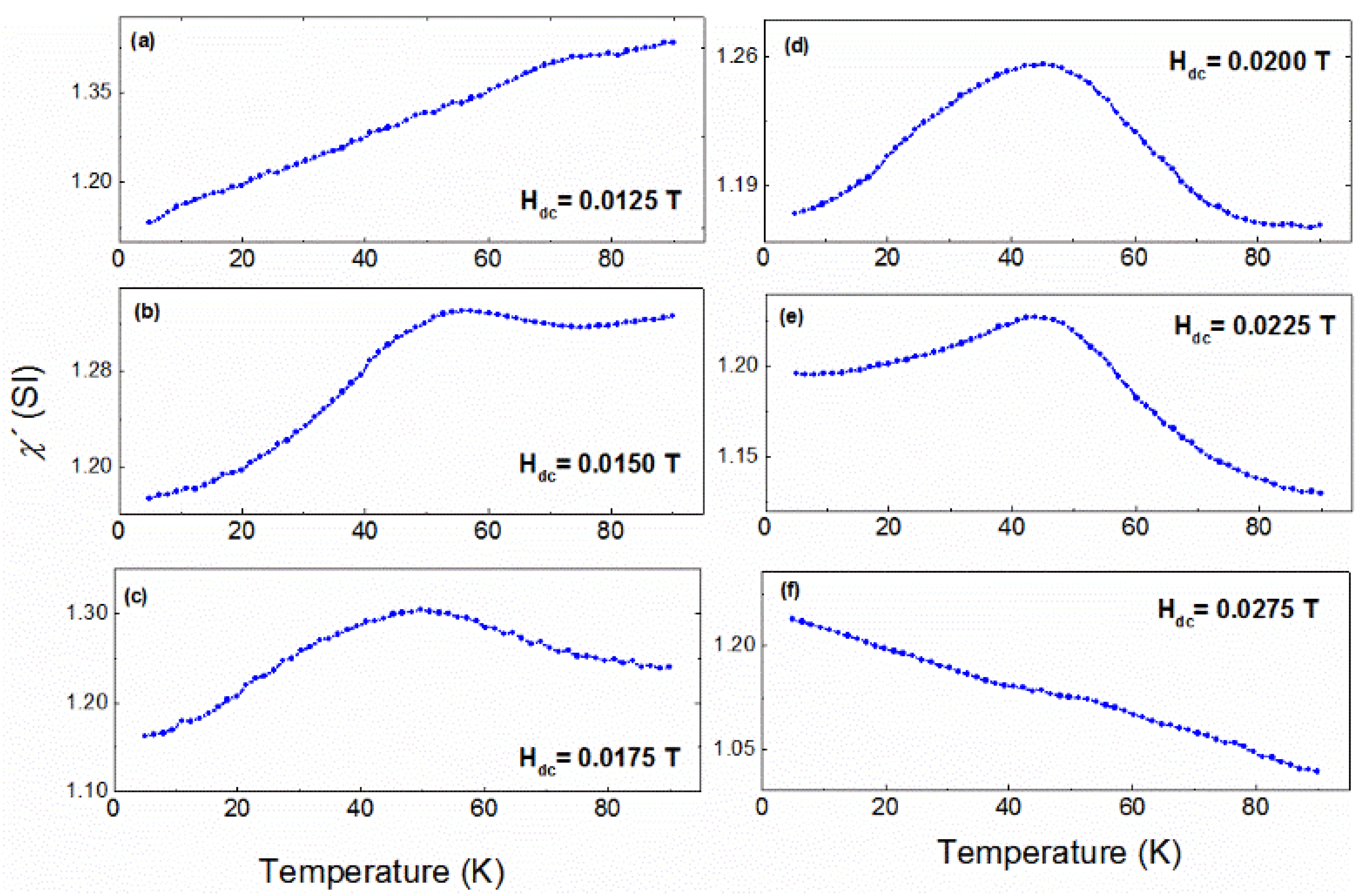
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gottschall, T.; Skokov, K.P.; Fries, M.; Taubel, A.; Radulov, I.; Scheibel, F.; Benke, D.; Riegg, S.; Gutfleisch, O. Making a Cool Choice: The Materials Library of Magnetic Refrigeration. Adv. Energy Mater. 2019, 9, 1901322. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Gottschall, T.; Skokov, K.P.; Moore, J.D.; Gutfleisch, O. Giant Magnetocaloric Effect Driven by Structural Transitions. Nat. Mater. 2012, 11, 620–626. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Tan, C.; Zhao, W.; Guo, E.; Tian, X. Computation-Guided Design of Ni–Mn–Sn Ferromagnetic Shape Memory Alloy with Giant Magnetocaloric Effect and Excellent Mechanical Properties and High Working Temperature via Multielement Doping. ACS Appl. Mater. Interfaces 2019, 11, 34827–34840. [Google Scholar] [CrossRef]
- Gràcia-Condal, A.; Gottschall, T.; Pfeuffer, L.; Gutfleisch, O.; Planes, A.; Mañosa, L. Multicaloric Effects in Metamagnetic Heusler Ni-Mn-In under Uniaxial Stress and Magnetic Field. Appl. Phys. Rev. 2020, 7, 041406. [Google Scholar] [CrossRef]
- Sokolovskiy, V.V.; Entel, P.; Buchelnikov, V.D.; Gruner, M.E. Achieving Large Magnetocaloric Effects in Co- and Cr-Substituted Heusler Alloys: Predictions from First-Principles and Monte Carlo Studies. Phys. Rev. B Condens. Matter Mater. Phys. 2015, 91, 220409. [Google Scholar] [CrossRef]
- Entel, P.; Siewert, M.; Gruner, M.E.; Herper, H.C.; Comtesse, D.; Arróyave, R.; Singh, N.; Talapatra, A.; Sokolovskiy, V.V.; Buchelnikov, V.D. Complex Magnetic Ordering as a Driving Mechanism of Multifunctional Properties of Heusler Alloys from First Principles. Eur. Phys. J. B 2013, 86, 65. [Google Scholar] [CrossRef]
- Comtesse, D.; Gruner, M.E.; Ogura, M.; Sokolovskiy, V.V.; Buchelnikov, V.D.; Grünebohm, A.; Arróyave, R.; Singh, N.; Gottschall, T.; Gutfleisch, O.; et al. First-Principles Calculation of the Instability Leading to Giant Inverse Magnetocaloric Effects. Phys. Rev. B 2014, 89, 184403. [Google Scholar] [CrossRef] [Green Version]
- Zhang, L.; Zhang, J.; Li, K.; Zhou, C.; Yao, Y.; Tan, T.T.; Wang, D.; Yang, S.; Li, S.; Carpenter, M.A. Glassy Magnetic Transitions and Accurate Estimation of Magnetocaloric Effect in Ni–Mn Heusler Alloys. ACS Appl. Mater. Interfaces 2020, 12, 43646–43652. [Google Scholar] [CrossRef] [PubMed]
- Lázpita, P.; Barandiarán, J.M.; Gutiérrez, J.; Feuchtwanger, J.; Chernenko, V.A.; Richard, M.L. Magnetic Moment and Chemical Order in Off-Stoichiometric Ni-Mn-Ga Ferromagnetic Shape Memory Alloys. New J. Phys. 2011, 13, 033039. [Google Scholar] [CrossRef]
- Richard, M.L.; Feuchtwanger, J.; Allen, S.M.; O’Handley, R.C.; Lázpita, P.; Barandiaran, J.M.; Gutierrez, J.; Ouladdiaf, B.; Mondelli, C.; Lograsso, T.; et al. Chemical Order in Off-Stoichiometric Ni-Mn-Ga Ferromagnetic Shape-Memory Alloys Studied with Neutron Diffraction. Philos. Mag. 2007, 87, 3437–3447. [Google Scholar] [CrossRef]
- Lázpita, P.; Barandiarán, J.M.; Gutiérrez, J.; Mondelli, C.; Sozinov, A.; Chernenko, V.A. Polarized Neutron Study of Ni-Mn-Ga Alloys: Site-Specific Spin Density Affected by Martensitic Transformation. Phys. Rev. Lett. 2017, 119, 155701. [Google Scholar] [CrossRef]
- Golub, V.; L’vov, V.A.; Salyuk, O.; Barandiaran, J.M.; Chernenko, V.A. Magnetism of Nanotwinned Martensite in Magnetic Shape Memory Alloys. J. Phys. Condens. Matter 2020, 32, 313001. [Google Scholar] [CrossRef]
- Golub, V.; Aseguinolaza, I.R.; Salyuk, O.; Popadiuk, D.; Sharay, I.; Fernández, R.; Alexandrakis, V.; Bunyaev, S.A.; Kakazei, G.N.; Barandiarán, J.M.; et al. Thickness Dependences of Structural and Magnetic Properties of Ni(Co)MnSn/MgO(001) Thin Films. J. Alloys Compd. 2021, 862, 158474. [Google Scholar] [CrossRef]
- Aksoy, S.; Acet, M.; Deen, P.P.; Mañosa, L.; Planes, A. Magnetic Correlations in Martensitic Ni-Mn-Based Heusler Shape-Memory Alloys: Neutron Polarization Analysis. Phys. Rev. B Condens. Matter Mater. Phys. 2009, 79, 212401. [Google Scholar] [CrossRef]
- El-Khatib, S.; Bhatti, K.P.; Srivastava, V.; James, R.D.; Leighton, C. Nanoscale Magnetic Phase Competition throughout the N I50-x C Ox M N40 S N10 Phase Diagram: Insights from Small-Angle Neutron Scattering. Phys. Rev. Mater. 2019, 3, 104413. [Google Scholar] [CrossRef]
- Meiklejohn, W.H.; Bean, C.P. New Magnetic Anisotropy. Phys. Rev. 1956, 102, 1413–1414. [Google Scholar] [CrossRef]
- Nogués, J.; Schuller, I.K. Exchange Bias. J. Magn. Magn. Mater. 1999, 192, 203–232. [Google Scholar] [CrossRef]
- Radu, F.; Zabel, H. Exchange Bias Effect of Ferro-/Antiferromagnetic Heterostructures. In Magnetic Heterostructures: Advances and Perspectives in Spinstructures and Spintransport; Zabel, H., Bader, S.D., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 97–184. [Google Scholar] [CrossRef]
- Ali, M.; Adie, P.; Marrows, C.H.; Greig, D.; Hickey, B.J.; Stamps, R.L. Exchange Bias Using a Spin Glass. Nat. Mater. 2007, 6, 70–75. [Google Scholar] [CrossRef]
- Shell, C.; Mn, M.; Salazar-Alvarez, G.; Sort, J.; Suriñach, S.; Baro, M.D.; Nogués, J. Synthesis and Size-Dependent Exchange Bias in Inverted Core−Shell MnO|Mn3O4 Nanoparticles. J. Am. Chem. Soc. 2007, 129, 9102–9108. [Google Scholar]
- Phan, M.H.; Alonso, J.; Khurshid, H.; Lampen-Kelley, P.; Chandra, S.; Repa, K.S.; Nemati, Z.; Das, R.; Iglesias, Ó.; Srikanth, H. Exchange Bias Effects in Iron Oxide-Based Nanoparticle Systems. Nanomaterials 2016, 6, 221. [Google Scholar] [CrossRef]
- Markovich, V.; Puzniak, R.; Mogilyansky, D.; Wu, X.; Suzuki, K.; Fita, I.; Wisniewski, A.; Chen, S.; Gorodetsky, G. Exchange Bias Effect in La0.2Ca0.8MnO3 Antiferromagnetic Nanoparticles with Two Ferromagnetic-like Contributions. J. Phys. Chem. C 2011, 115, 1582–1591. [Google Scholar] [CrossRef]
- Sharma, A.; Tripathi, J.; Tripathi, S.; Kumar, Y.; Ugochukwu, K.C.; Kumar, D.; Gupta, M.; Chaudhary, R.J. Exchange Bias in Co/CoO Thin Films Deposited onto Self-Assembled Nanosphere Arrays. J. Magn. Magn. Mater. 2020, 510, 166599. [Google Scholar] [CrossRef]
- Stamps, R.L. Mechanisms for Exchange Bias. J. Phys. D Appl. Phys. 2000, 33, R247. [Google Scholar] [CrossRef]
- Liu, K.; Baker, S.M.; Tuominen, M.; Russell, T.P.; Schuller, I.K. Tailoring Exchange Bias with Magnetic Nanostructures. Phys. Rev. B Condens. Matter Mater. Phys. 2001, 63, 604031–604034. [Google Scholar] [CrossRef] [Green Version]
- Parkin, S.S.P.; Roche, K.P.; Samant, M.G.; Rice, P.M.; Beyers, R.B.; Scheuerlein, R.E.; O’Sullivan, E.J.; Brown, S.L.; Bucchigano, J.; Abraham, D.W.; et al. Exchange-Biased Magnetic Tunnel Junctions and Application to Nonvolatile Magnetic Random Access Memory (Invited). J. Appl. Phys. 1999, 85, 5828–5833. [Google Scholar] [CrossRef]
- Parkin, S.S.P.; Kaiser, C.; Panchula, A.; Rice, P.M.; Hughes, B.; Samant, M.; Yang, S.H. Giant Tunnelling Magnetoresistance at Room Temperature with MgO (100) Tunnel Barriers. Nat. Mater. 2004, 3, 862–867. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, E.; Zhang, W.; Wong, P.K.J.; Xu, Z.; Hu, F.; Li, X.; Tang, J.; Wee, A.T.S.; Xu, F. Mechanical Strain Manipulation of Exchange Bias Field and Spin Dynamics in FeCo/IrMn Multilayers Grown on Flexible Substrates. ACS Appl. Mater. Interfaces 2019, 11, 8258–8265. [Google Scholar] [CrossRef]
- Huang, J.; Wang, H.; Wang, X.; Gao, X.; Liu, J.; Wang, H. Exchange Bias in a La0.67Sr0.33MnO3/NiO Heterointerface Integrated on a Flexible Mica Substrate. ACS Appl. Mater. Interfaces 2020, 12, 39920–39925. [Google Scholar] [CrossRef] [PubMed]
- Perzanowski, M.; Zarzycki, A.; Gregor-Pawlowski, J.; Marszalek, M. Magnetization Reversal Mechanism in Exchange-Biased Spring-like Thin-Film Composite. ACS Appl. Mater. Interfaces 2020, 12, 39926–39934. [Google Scholar] [CrossRef] [PubMed]
- You, W.; Che, R. Excellent NiO-Ni Nanoplate Microwave Absorber via Pinning Effect of Antiferromagnetic-Ferromagnetic Interface. ACS Appl. Mater. Interfaces 2018, 10, 15104–15111. [Google Scholar] [CrossRef]
- Khan, M.; Dubenko, I.; Stadler, S.; Ali, N. Exchange Bias Behavior in Ni-Mn-Sb Heusler Alloys. Appl. Phys. Lett. 2007, 91, 3–6. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Jing, C.; Chen, J.; Yuan, S.; Cao, S.; Zhang, J. Observation of Exchange Bias in the Martensitic State of Ni50Mn36Sn14 Heusler Alloy. Appl. Phys. Lett. 2007, 91, 1–4. [Google Scholar] [CrossRef]
- Khan, M.; Dubenko, I.; Stadler, S.; Ali, N. Exchange Bias in Bulk Mn Rich Ni-Mn-Sn Heusler Alloys. J. Appl. Phys. 2007, 102, 113914. [Google Scholar] [CrossRef] [Green Version]
- Wang, B.M.; Liu, Y.; Ren, P.; Xia, B.; Ruan, K.B.; Yi, J.B.; Ding, J.; Li, X.G.; Wang, L. Large Exchange Bias after Zero-Field Cooling from an Unmagnetized State. Phys. Rev. Lett. 2011, 106, 112505. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.M.; Liu, Y.; Xia, B.; Ren, P.; Wang, L. Large Exchange Bias Obtainable through Zero-Field Cooling from an Unmagnetized State in Ni-Mn-Sn Alloys. J. Appl. Phys. 2012, 111, 043912. [Google Scholar] [CrossRef]
- Liao, P.; Jing, C.; Wang, X.L.; Yang, Y.J.; Zheng, D.; Li, Z.; Kang, B.J.; Deng, D.M.; Cao, S.X.; Zhang, J.C.; et al. Strongly Enhanced Antiferromagnetism and Giant Spontaneous Exchange Bias in Ni50Mn36Co4Sn10 Heusler Alloy. Appl. Phys. Lett. 2014, 104, 092410. [Google Scholar] [CrossRef] [Green Version]
- Nayak, A.K.; Nicklas, M.; Chadov, S.; Shekhar, C.; Skourski, Y.; Winterlik, J.; Felser, C. Large Zero-Field Cooled Exchange-Bias in Bulk Mn2PtGa. Phys. Rev. Lett. 2013, 110, 127204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jia, L.; Shen, J.; Li, M.; Wang, X.; Ma, L.; Zhen, C.; Hou, D.; Liu, E.; Wang, W.; Wu, G. Tuning Antiferromagnetic Exchange Interaction for Spontaneous Exchange Bias in MnNiSnSi System. APL Mater. 2017, 5, 126105. [Google Scholar] [CrossRef]
- Alexandrakis, V.; Aseguinolaza, I.R.; Decker, P.; Salomon, S.; Barandiarán, J.M.; Ludwig, A.; Chernenko, V.A. Martensitic Transformation Hysteresis in Ni(Co)-Mn-Sn/MgO Metamagnetic Shape Memory Thin Films. Scr. Mater. 2018, 156, 101–104. [Google Scholar] [CrossRef]
- Marcano, N.; Gómez Sal, J.C.; Espeso, J.I.; Fernández Barquín, L.; Paulsen, C. Cluster-Glass Percolative Scenario in CeNi1−xCux Studied by Very Low-Temperature Ac Susceptibility and Dc Magnetization. Phys. Rev. B Condens. Matter Mater. Phys. 2007, 76, 224419. [Google Scholar] [CrossRef]
- Alonso, J.; Fdez-Gubieda, M.L.; Sarmiento, G.; Chaboy, J.; Boada, R.; García Prieto, A.; Haskel, D.; Laguna-Marco, M.A.; Lang, J.C.; Meneghini, C.; et al. Interfacial Magnetic Coupling between Fe Nanoparticles in Fe-Ag Granular Alloys. Nanotechnology 2012, 23, 025705. [Google Scholar] [CrossRef]
- Djurberg, C.; Svedlindh, P.; Nordblad, P. Dynamics of an Interacting Particle System: Evidence of Critical Slowing Down. Phys. Rev. Lett. 1997, 79, 5154–5157. [Google Scholar] [CrossRef] [Green Version]
- Stanley, H.E. Scaling, Universality, and Renormalization: Three Pillars of Modern Critical Phenomena. Rev. Mod. Phys. 1999, 71 (Suppl. S2), 358–366. [Google Scholar] [CrossRef] [Green Version]
- Hohenberg, P.C.; Halperin, B.I. Theory of Dynamic Critical Phenomena. Rev. Mod. Phys. 1977, 49, 435–479. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alexandrakis, V.; Rodríguez-Aseguinolaza, I.; Anastasakos-Paraskevopoulos, D.; Barandiarán, J.M.; Chernenko, V.; Porro, J.M. Spontaneous Zero-Field Cooling Exchange Bias in Ni–Co–Mn–Sn Metamagnetic Heusler Sputtered Film. Nanomaterials 2021, 11, 2188. https://doi.org/10.3390/nano11092188
Alexandrakis V, Rodríguez-Aseguinolaza I, Anastasakos-Paraskevopoulos D, Barandiarán JM, Chernenko V, Porro JM. Spontaneous Zero-Field Cooling Exchange Bias in Ni–Co–Mn–Sn Metamagnetic Heusler Sputtered Film. Nanomaterials. 2021; 11(9):2188. https://doi.org/10.3390/nano11092188
Chicago/Turabian StyleAlexandrakis, Vasileios, Iván Rodríguez-Aseguinolaza, Dimitrios Anastasakos-Paraskevopoulos, Jose Manuel Barandiarán, Volodymyr Chernenko, and Jose Maria Porro. 2021. "Spontaneous Zero-Field Cooling Exchange Bias in Ni–Co–Mn–Sn Metamagnetic Heusler Sputtered Film" Nanomaterials 11, no. 9: 2188. https://doi.org/10.3390/nano11092188
APA StyleAlexandrakis, V., Rodríguez-Aseguinolaza, I., Anastasakos-Paraskevopoulos, D., Barandiarán, J. M., Chernenko, V., & Porro, J. M. (2021). Spontaneous Zero-Field Cooling Exchange Bias in Ni–Co–Mn–Sn Metamagnetic Heusler Sputtered Film. Nanomaterials, 11(9), 2188. https://doi.org/10.3390/nano11092188






