Promising Bialkali Bismuthides Cs(Na, K)2Bi for High-Performance Nanoscale Electromechanical Devices: Prediction of Mechanical and Anisotropic Elastic Properties under Hydrostatic Tension and Compression and Tunable Auxetic Properties
Abstract
:1. Introduction
2. Computational Details
3. Results and Discussion
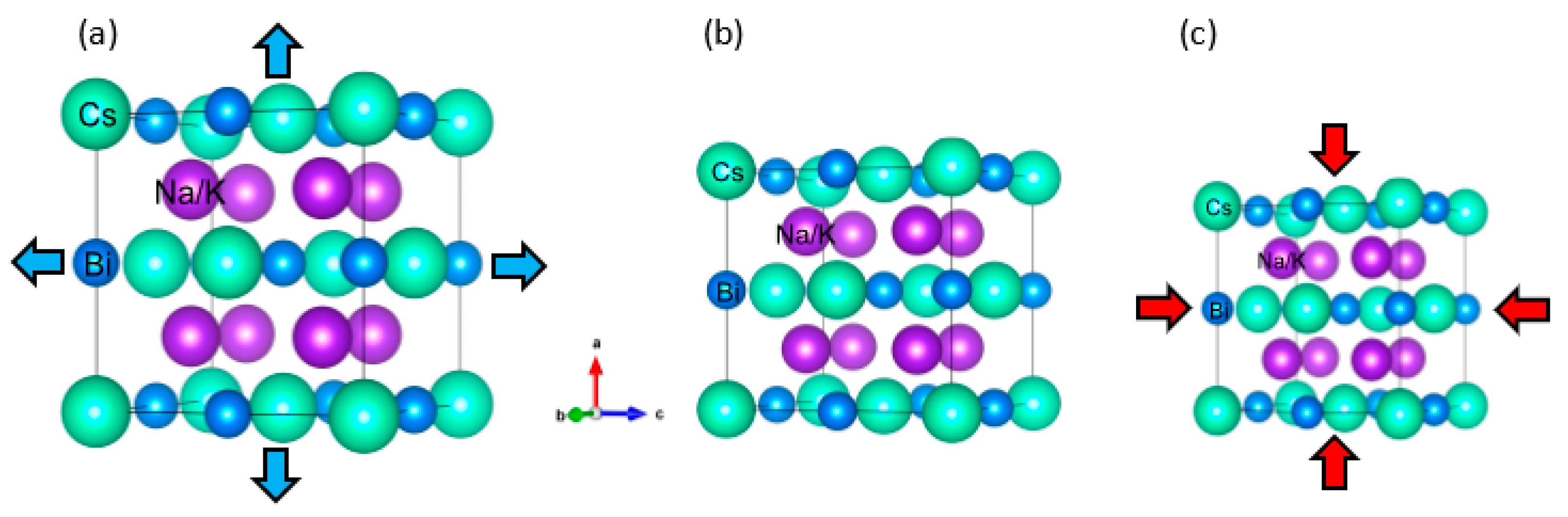
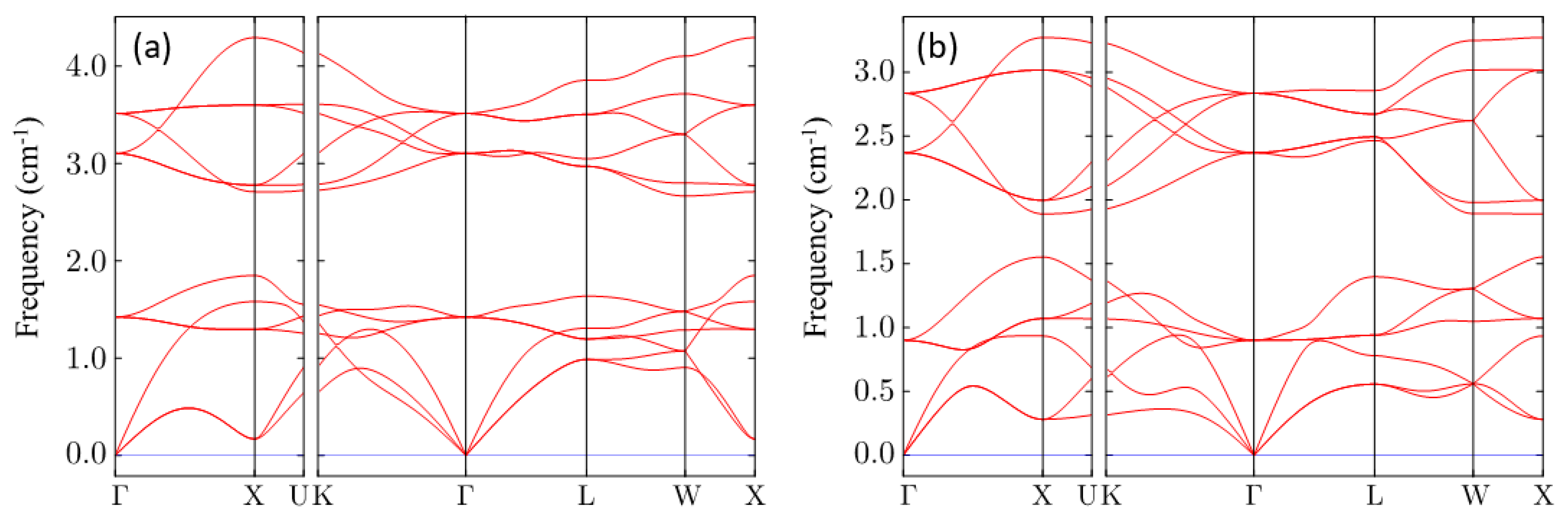
3.1. Structural Properties and Stability Conditions
3.2. Basic Mechanical Properties
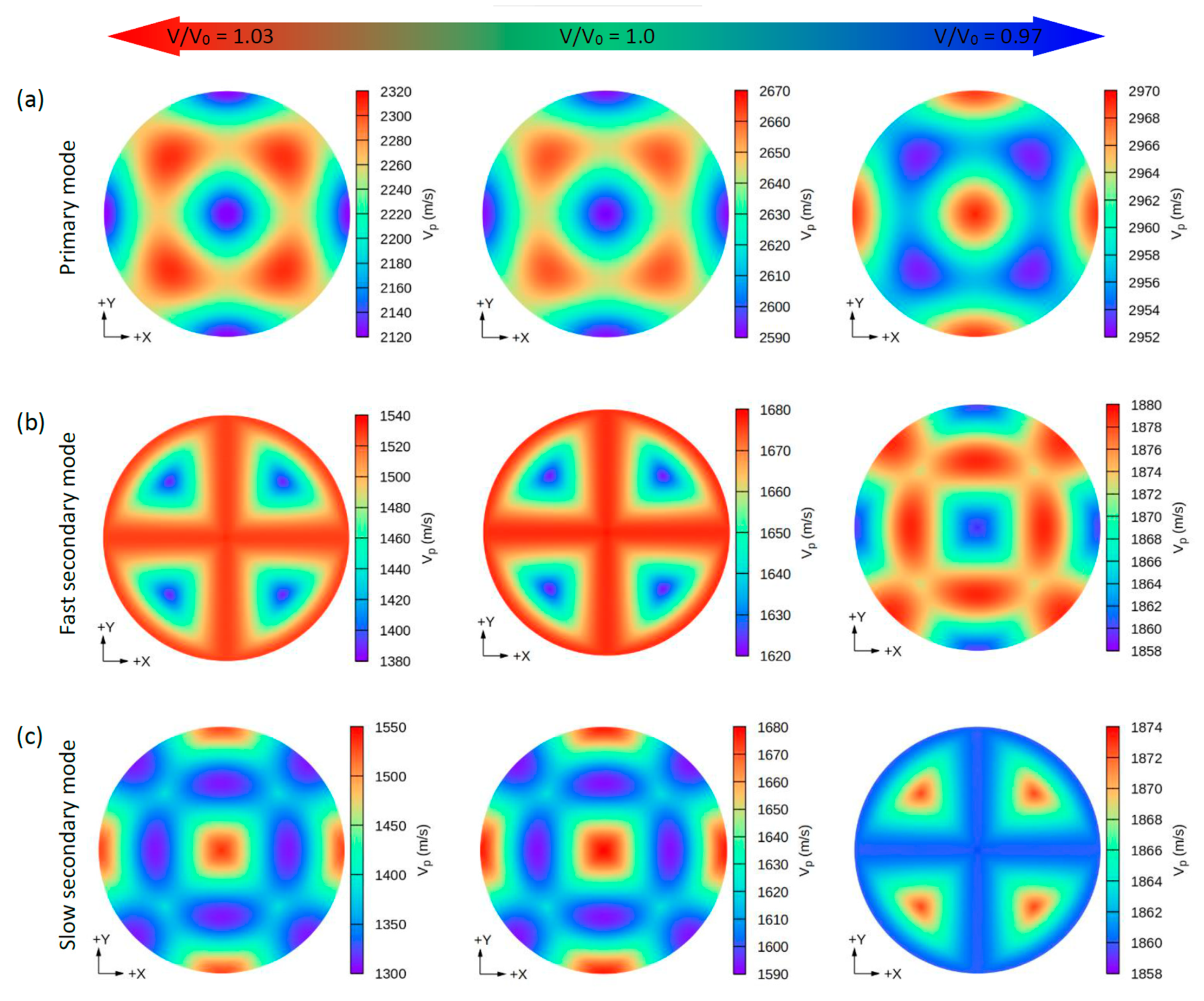
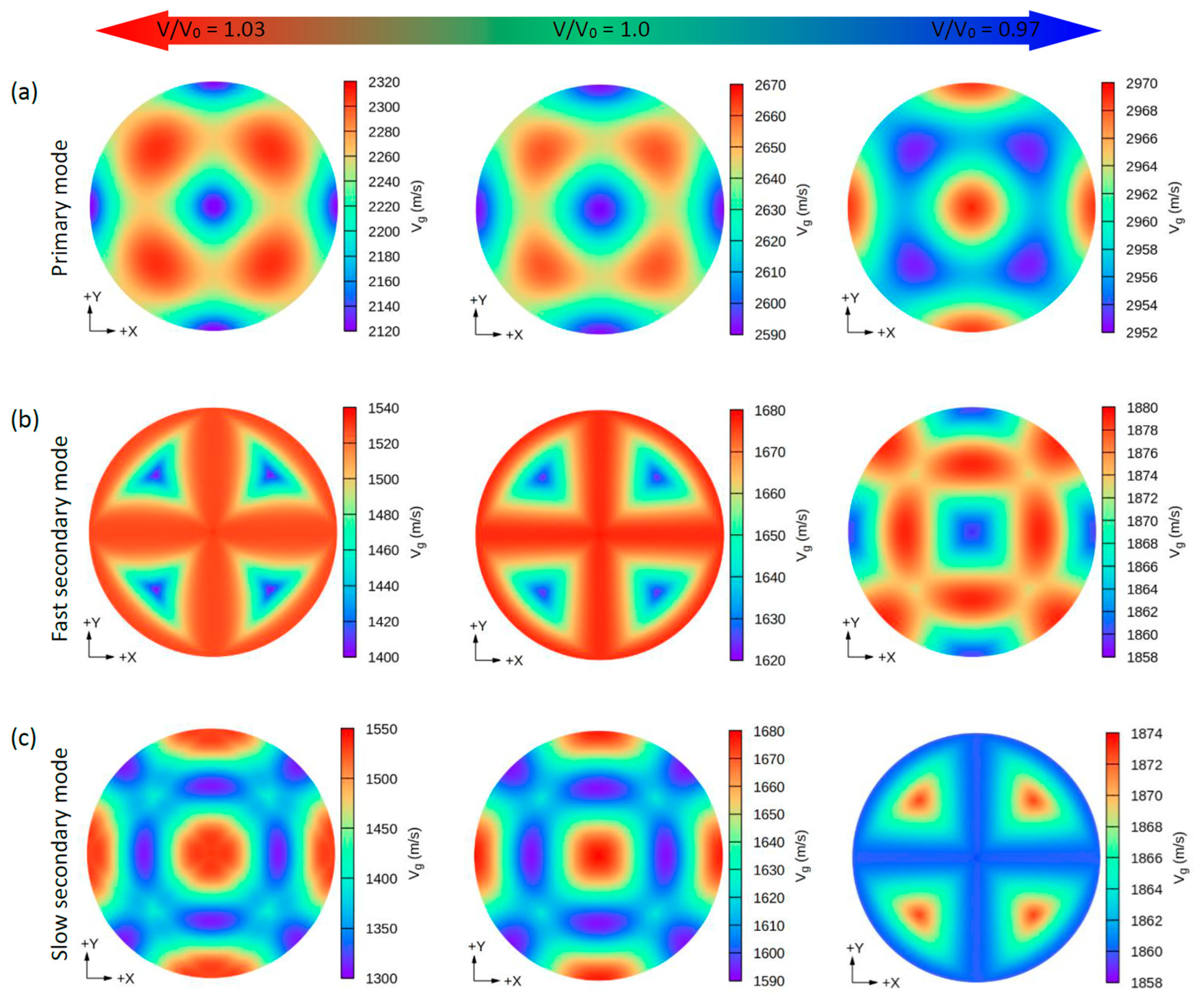
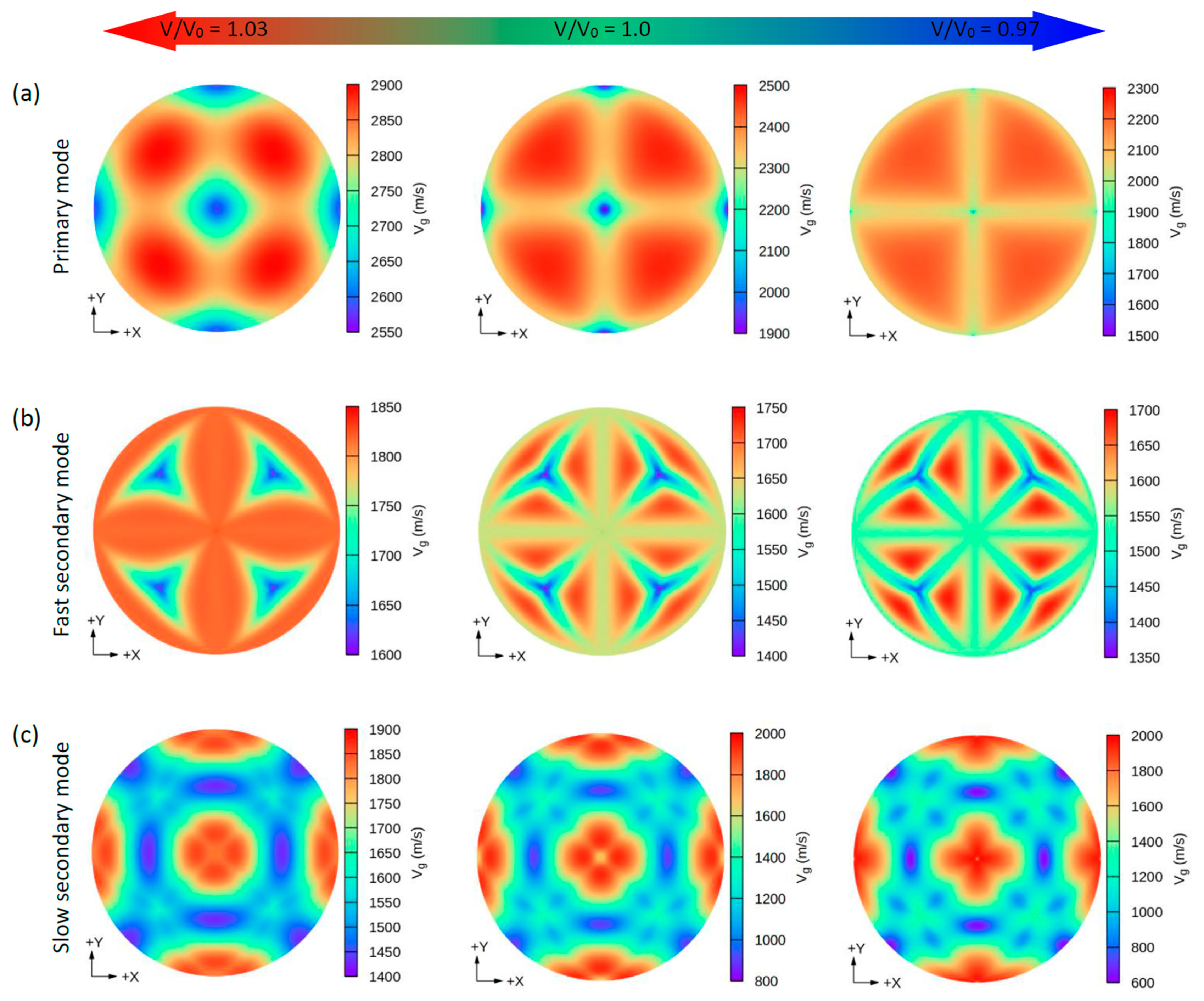
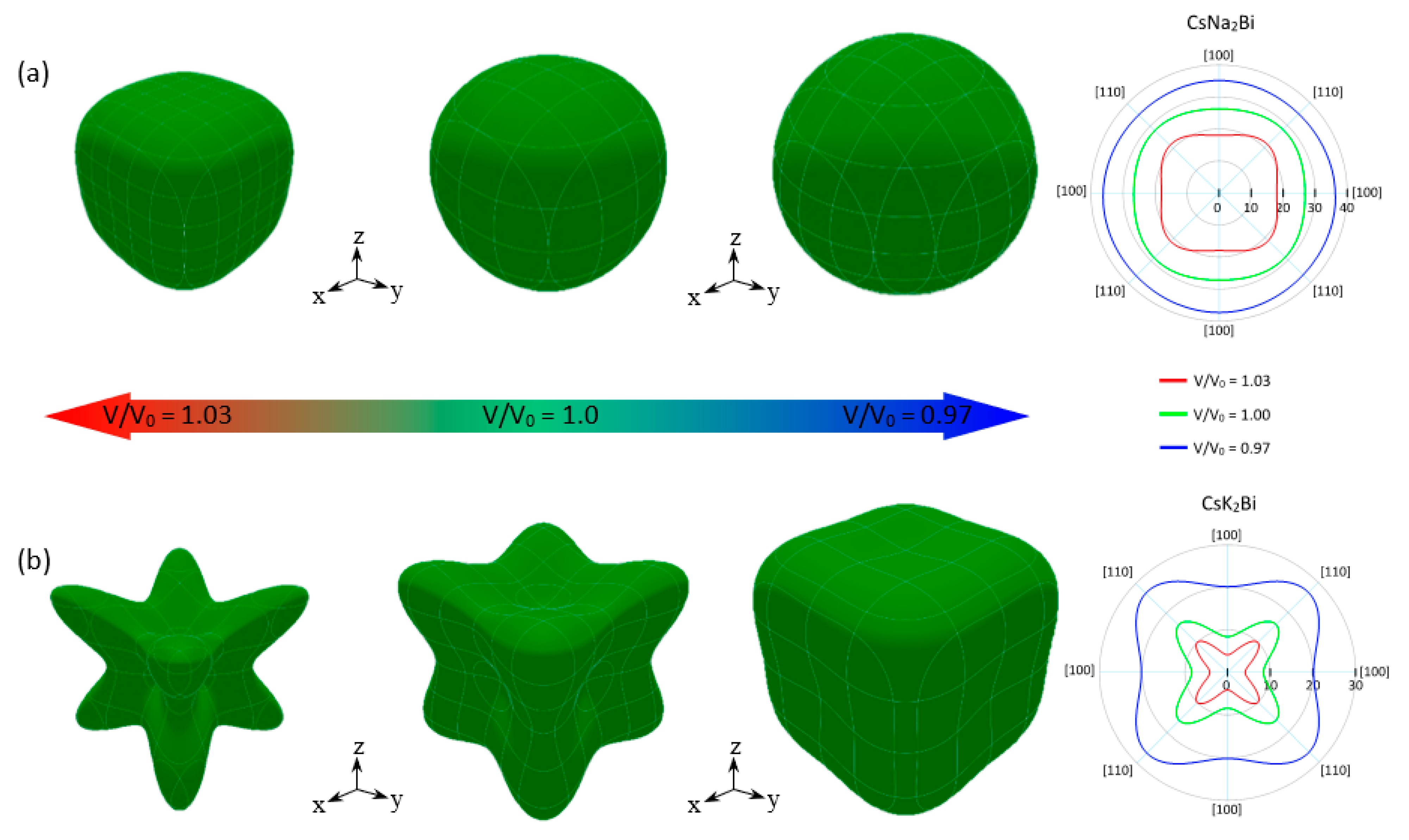
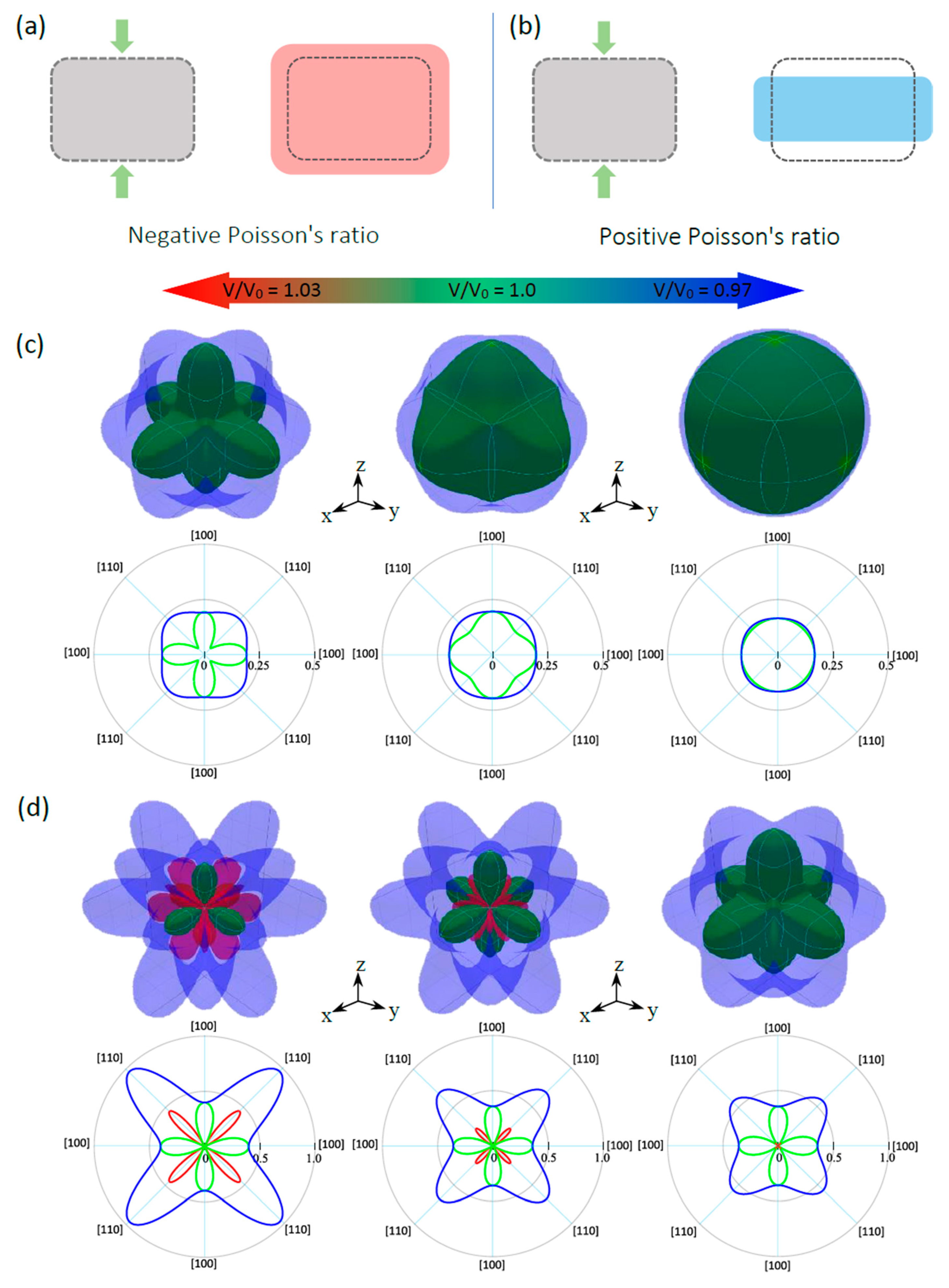
3.3. Elastic Anisotropy
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Spicer, W.E. Photoemissive, Photoconductive, and Optical Absorption Studies of Alkali-Antimony Compounds. Phys. Rev. 1958, 112, 114–122. [Google Scholar] [CrossRef]
- Sommer, A.H. New Photoemissive Cathodes of High Sensitivity. Rev. Sci. Instrum. 1955, 26, 725. [Google Scholar] [CrossRef]
- Spicer, W. The production of pairs in semiconductors by low energy electrons. J. Phys. Chem. Solids 1961, 22, 365–370. [Google Scholar] [CrossRef]
- Sommer, A.H. A new alkali antimonide photoemitter with high sensitivity to visible light. Appl. Phys. Lett. 1963, 3, 62–63. [Google Scholar] [CrossRef]
- Taft, E.A.; Philipp, H.R. Structure in the Energy Distribution of Photoelectrons fromK3Sb andCs3Sb. Phys. Rev. 1959, 115, 1583–1586. [Google Scholar] [CrossRef]
- Sklyadneva, I.Y.; Rusinov, I.P.; Heid, R.; Bohnen, K.-P.; Echenique, P.M.; Chulkov, E.V. Pressure-induced topological phases of KNa2Bi. Sci. Rep. 2016, 6, 24137. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yalameha, S.; Nourbakhsh, Z.; Vaez, A. Hydrostatic strain-induced topological phase of KNa2Sb. J. Magn. Magn. Mater. 2018, 468, 279–286. [Google Scholar] [CrossRef]
- Wang, Z.; Sun, Y.; Chen, X.-Q.; Franchini, C.; Xu, G.; Weng, H.; Dai, X.; Fang, Z. Dirac semimetal and topological phase transitions inA3Bi (A = Na, K, Rb). Phys. Rev. B 2012, 85, 195320. [Google Scholar] [CrossRef] [Green Version]
- Ebina, A.; Takahashi, T. Transmittance Spectra and Optical Constants of Alkali-Antimony CompoundsK3Sb, Na3Sb, and Na2KSb. Phys. Rev. B 1973, 7, 4712–4719. [Google Scholar] [CrossRef]
- Ghosh, C.K.; Varma, B.P. Preparation and study of properties of a few alkali antimonide photocathodes. J. Appl. Phys. 1978, 49, 4549–4553. [Google Scholar] [CrossRef] [Green Version]
- Tzolov, M.B.; Iliev, M.N. Raman scattering from monoalkali (Na-Sb and K-Sb), bialkali (Na-K-Sb) and multialkali (Na-K-Sb-Cs) photocathodes. Thin Solid Films 1992, 213, 99–102. [Google Scholar] [CrossRef]
- Gaowei, M.; Ding, Z.; Schubert, S.; Bhandari, H.B.; Sinsheimer, J.; Kuehn, J.; Nagarkar, V.V.; Marshall, M.S.J.; Walsh, J.; Muller, E.M.; et al. Synthesis and X-ray characterization of sputtered bi-alkali antimonide photocathodes. APL Mater. 2017, 5, 116104. [Google Scholar] [CrossRef]
- Kalarasse, L.; Bennecer, B.; Djeroud, S. Pressure effect on the electronic and optical properties of the alkali antimonide semiconductors Cs3Sb, KCs2Sb, CsK2Sb and K3Sb: Ab initio study. J. Phys. Chem. Solids 2010, 71, 1732–1741. [Google Scholar] [CrossRef]
- Amador, R.; Saßnick, H.-D.; Cocchi, C. Electronic structure and optical properties of Na2KSb and NaK2Sb from first-principles many-body theory. J. Phys. Condens. Matter 2021, 33, 365502. [Google Scholar] [CrossRef]
- Sharma, V.K.; Sreeparvathy, P.C.; Kanchana, V. Na2KSb: A promising thermoelectric material. AIP Conf. Proc. 2019, 2115, 030443. [Google Scholar] [CrossRef]
- Yalameha, S.; Nourbakhsh, Z.; Ramazani, A.; Vashaee, D. Highly stable full Heusler order Cs(Na, K)2Bi with diverse topological phases controlled by strain engineering. Mater. Sci. Eng. B 2021, 273, 115430. [Google Scholar] [CrossRef]
- Born, M. Thermodynamics of Crystals and Melting. J. Chem. Phys. 1939, 7, 591–603. [Google Scholar] [CrossRef]
- Ayuela, A.; Enkovaara, J.; Ullakko, K.; Nieminen, R.M. Structural properties of magnetic Heusler alloys. J. Phys. Condens. Matter 1999, 11, 2017–2026. [Google Scholar] [CrossRef] [Green Version]
- Karki, B.B.; Ackland, G.; Crain, J. Elastic instabilities in crystals fromab initiostress—Strain relations. J. Phys. Condens. Matter 1997, 9, 8579–8589. [Google Scholar] [CrossRef] [Green Version]
- Fine, M.; Brown, L.; Marcus, H. Elastic constants versus melting temperature in metals. Scr. Met. 1984, 18, 951–956. [Google Scholar] [CrossRef]
- Gilman, J.J. Electronic Basis of the Strength of Materials; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Wu, S.-C.; Fecher, G.H.; Naghavi, S.S.; Felser, C. Elastic properties and stability of Heusler compounds: Cubic Co2YZ compounds with L21 structure. J. Appl. Phys. 2019, 125, 082523. [Google Scholar] [CrossRef] [Green Version]
- Du, Z.; Zhou, M.; He, L.; Liu, H. Study on negative Poisson’s ratio of auxetic yarn under tension: Part 2—Experimental verification. Text. Res. J. 2015, 85, 768–774. [Google Scholar] [CrossRef]
- Mohsenizadeh, S.; Alipour, R.; Nejad, A.F.; Rad, M.S.; Ahmad, Z. Experimental Investigation on Energy Absorption of Auxetic Foam-filled Thin-walled Square Tubes under Quasi-static Loading. Procedia Manuf. 2015, 2, 331–336. [Google Scholar] [CrossRef] [Green Version]
- Scarpa, F.; Giacomin, J.; Zhang, Y.; Pastorino, P. Mechanical Performance of Auxetic Polyurethane Foam for Antivibration Glove Applications. Cell. Polym. 2005, 24, 253–268. [Google Scholar] [CrossRef] [Green Version]
- Ma, Z.-D.; Liu, Y.; Liu, X.; Sun, C.; Cui, Y. Ultralightweight Runflat Tires Based Upon Negative Poisson Ratio (NPR) Auxetic Structures. U.S. Patent US20110168313A1, 1 October 2013. [Google Scholar]
- Scarpa, F. Auxetic materials for bioprostheses [In the Spotlight]. IEEE Signal Process. Mag. 2008, 25, 126–128. [Google Scholar] [CrossRef]
- Lipsett, A.W.; Beltzer, A.I. Reexamination of dynamic problems of elasticity for negative Poisson’s ratio. J. Acoust. Soc. Am. 1988, 84, 2179–2186. [Google Scholar] [CrossRef]
- Alderson, A.; Rasburn, J.; Ameer-Beg, S.; Mullarkey, P.G.; Perrie, W.; Evans, K.E. An Auxetic Filter: A Tuneable Filter Displaying Enhanced Size Selectivity or Defouling Properties. Ind. Eng. Chem. Res. 2000, 39, 654–665. [Google Scholar] [CrossRef]
- Alderson, A.; Rasburn, J.; Evans, K.E.; Grima, J. Auxetic polymeric filters display enhanced de-fouling and pressure compensation properties. Membr. Technol. 2001, 2001, 6–8. [Google Scholar] [CrossRef]
- Javanbakht, M.; Adaei, M. Investigating the effect of elastic anisotropy on martensitic phase transformations at the nanoscale. Comput. Mater. Sci. 2019, 167, 168–182. [Google Scholar] [CrossRef]
- Lakes, R.; Elms, K. Indentability of Conventional and Negative Poisson’s Ratio Foams. J. Compos. Mater. 1993, 27, 1193–1202. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Van Vliet, K.J.; Zhu, T.; Yip, S.; Suresh, S. Atomistic mechanisms governing elastic limit and incipient plasticity in crystals. Nat. Cell Biol. 2002, 418, 307–310. [Google Scholar] [CrossRef] [PubMed]
- Niekiel, F.; Spiecker, E.; Bitzek, E. Influence of anisotropic elasticity on the mechanical properties of fivefold twinned nanowires. J. Mech. Phys. Solids 2015, 84, 358–379. [Google Scholar] [CrossRef]
- Blaha, P.; Schwarz, K.; Tran, F.; Laskowski, R.; Madsen, G.K.H.; Marks, L.D. WIEN2k: An APW+lo program for calculating the properties of solids. J. Chem. Phys. 2020, 152, 074101. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [Green Version]
- Blum, V.; Gehrke, R.; Hanke, F.; Havu, P.; Havu, V.; Ren, X.; Reuter, K.; Scheffler, M. Ab initio molecular simulations with numeric atom-centered orbitals. Comput. Phys. Commun. 2009, 180, 2175–2196. [Google Scholar] [CrossRef] [Green Version]
- Togo, A.; Tanaka, I. First principles phonon calculations in materials science. Scr. Mater. 2015, 108, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Jamal, M.; Bilal, M.; Ahmad, I.; Jalali-Asadabadi, S. IRelast package. J. Alloy. Compd. 2018, 735, 569–579. [Google Scholar] [CrossRef]
- Yalameha, S.; Nourbakhsh, Z.; Vashaee, D. ElATools: A tool for analyzing anisotropic elastic properties of the 2D and 3D materials. arXiv 2021, arXiv:2105.07279. [Google Scholar]
- Babiker, A.S.; Gao, G.; Yao, K. Half-metallicity and magnetism of Heusler alloys Co2 HfZ (Z = Al, Ga, Ge, Sn). J. Magn. Magn. Mater. 2017, 441, 356–360. [Google Scholar] [CrossRef]
- Akriche, A.; Bouafia, H.; Hiadsi, S.; Abidri, B.; Sahli, B.; Elchikh, M.; Timaoui, M.; Djebour, B. First-principles study of mechanical, exchange interactions and the robustness in Co2MnSi full Heusler compounds. J. Magn. Magn. Mater. 2017, 422, 13–19. [Google Scholar] [CrossRef]
- Tenelanda-Osorio, L.I.; Vélez, M.E. First principles study of the thermodynamic, mechanical and electronic properties of crystalline phases of Chromium Nitrides. J. Phys. Chem. Solids 2021, 148, 109692. [Google Scholar] [CrossRef]
- Yalameha, S.; Vaez, A. Ab-initio thermodynamic and elastic properties of AlNi and AlNi3 intermetallic compounds. Int. J. Mod. Phys. B 2018, 32, 1850129. [Google Scholar] [CrossRef]
- Yu, R.; Jiang, Y.; Zhou, R. First-principle studies of the stability, electronic and elastic properties of trigonal-type M2N (M = Cr, V, Nb and Ta). Solid State Commun. 2014, 186, 32–37. [Google Scholar] [CrossRef]
- Yalameha, S.; Vaez, A. Structural, electronic, elastic and thermodynamic properties of Al1-Z Ni (Z=Cr, V and x= 0, 0.125, 0.25) alloys: First-principle calculations. Comput. Condens. Matter 2019, 21, e00415. [Google Scholar] [CrossRef]
- Zhang, H.; Shang, S.-L.; Saal, J.; Saengdeejing, A.; Wang, Y.; Chen, L.-Q.; Liu, Z.-K. Enthalpies of formation of magnesium compounds from first-principles calculations. Intermetallics 2009, 17, 878–885. [Google Scholar] [CrossRef]
- Jain, A.; Ong, S.P.; Hautier, G.; Chen, W.; Richards, W.D.; Dacek, S.; Cholia, S.; Gunter, D.; Skinner, D.; Ceder, G.; et al. Commentary: The Materials Project: A materials genome approach to accelerating materials innovation. APL Mater. 2013, 1, 011002. [Google Scholar] [CrossRef] [Green Version]
- Born, M. On the stability of crystal lattices. I. In Mathematical Proceedings of the Cambridge Philosophical Society; Cambridge University Press: Cambridge, UK, 1940. [Google Scholar]
- Cowley, R.A. Soft Modes and Structural Phase Transitions. Integr. Ferroelectr. 2012, 133, 109–117. [Google Scholar] [CrossRef]
- Saeidi, P.; Kaviyani, M.H.S.; Yalameha, S. The structural and elastic properties of InSb1-xBix alloys. Comput. Condens. Matter 2019, 18, e00358. [Google Scholar] [CrossRef]
- Chung, D.H.; Buessem, W.R. The Voigt-Reuss-Hill (VRH) Approximation and the Elastic Moduli of Polycrystalline ZnO, TiO2 (Rutile), and α-Al2O3. J. Appl. Phys. 1968, 39, 2777–2782. [Google Scholar] [CrossRef]
- Bao, W.; Liu, D.; Li, P.; Duan, Y. Structural properties, elastic anisotropies and thermal conductivities of tetragonal LnB2C2 (Ln = Rare Earth) compounds from first-principles calculations. Ceram. Int. 2019, 45, 1857–1867. [Google Scholar] [CrossRef]
- Bao, W.; Liu, D.; Li, P.; Duan, Y. Elastic anisotropies and thermal properties of cubic TMIr (TM = Sc, Y, Lu, Ti, Zr and Hf): A DFT calculation. Mater. Res. Express 2019, 6, 086574. [Google Scholar] [CrossRef]
- Korozlu, N.; Colakoglu, K.; Deligoz, E.; Aydin, S. The elastic and mechanical properties of MB12 (M = Zr, Hf, Y, Lu) as a function of pressure. J. Alloy. Compd. 2013, 546, 157–164. [Google Scholar] [CrossRef]
- Kleinman, L. Deformation Potentials in Silicon. I. Uniaxial Strain. Phys. Rev. 1962, 128, 2614–2621. [Google Scholar] [CrossRef]
- Jaeken, J.W.; Cottenier, S. Solving the Christoffel equation: Phase and group velocities. Comput. Phys. Commun. 2016, 207, 445–451. [Google Scholar] [CrossRef] [Green Version]
- Baughman, R.H.; Shacklette, J.M.; Zakhidov, A.; Stafström, S. Negative Poisson’s ratios as a common feature of cubic metals. Nature 1998, 392, 362–365. [Google Scholar] [CrossRef]


| Properties | CsNa2Bi | CsK2Bi | ||||
|---|---|---|---|---|---|---|
| V/V0 = 1.03 (~0.76 GPa) | V/V0 = 1.0 | V/V0 = 0.97 (~1.0 GPa) | V/V0 = 1.03 (~0.72 GPa) | V/V0 = 1.0 | V/V0 = 0.97 (~1.0 GPa) | |
| C11 (GPa) | 19.82 | 29.58 | 38.81 | 8.80 | 14.05, 14 * | 25.88 |
| C12 (GPa) | 4.73 | 7.24 | 7.71 | 5.83 | 7.85, 6 * | 10.14 |
| C44 (GPa) | 10.26 | 12.38 | 15.23 | 9.09 | 10.25, 9 * | 12.73 |
| BV/BR/BVRH (GPa) | 9.7/9.7/9.7 | 14.7/14.7/14.7 | 18.1/18.1/18.1 | 6.8/6.8/6.8 | 9.9/9.9/9.9 | 15.4/15.4/15.4 |
| GV/GR/GVRH (GPa) | 9.1/8.9/9.0 | 11.9/11.9/11.9 | 38.6/38.6/38.6 | 6.0/2.9/4.5 | 7.39/5.32/6.36 | 10.9/10.2/10.5 |
| EV/ER/EVRH (GPa) | 20.9/20.6/20.7 | 28.1/28.1/28.1 | 35.9/35.9/35.9 | 14.0/7.8/10.9 | 17.7/13.5/15.6 | 26.2/25.1/25.6 |
| νv/νR/νVRH | 0.142/0.148/0.145 | 0.181/0.181/0.181 | 0.169/0.169/0.169 | 0.158/0.309/0.233 | 0.201/0.272/0.236 | 0.215/0.228/0.222 |
| B/GV/B/GR/B/GVRH | 1.06/1.08/1.07 | 1.23/1.23/1.23 | 1.17/1.17/1.17 | 1.12/2.29/1.51 | 1.34/1.86/1.55 | 1.42/1.50/1.46 |
| ξ | 0.446 | 0.454 | 0.392 | 1.111 | 0.930 | 0.665 |
| Proprieties | CsNa2Bi | CsK2Bi | ||||
|---|---|---|---|---|---|---|
| V/V0 = 1.03 | V/V0 = 1.0 | V/V0 = 0.97 | V/V0 = 1.03 | V/V0 = 1.0 | V/V0 = 0.97 | |
| (m/s) | 2307.4 | 2661.1 | 2968.6 | 2213.9 | 2470.7 | 2894.1 |
| (m/s) | 2121.3 | 2591.6 | 2952.7 | 1533.5 | 1906.5 | 2587.8 |
| (m/s) | 1526.6 | 1676.3 | 1878.8 | 1533.5 | 1628.8 | 1815.2 |
| (m/s) | 1386.0 | 1621.3 | 1859.9 | 1023.4 | 1194.0 | 1568.6 |
| (m/s) | 1526.6 | 1676.3 | 1872.5 | 1509.2 | 1628.8 | 1815.2 |
| (m/s) | 1308.7 | 1592.6 | 1859.9 | 619.4 | 895.0 | 1426.8 |
| (m/s) | 2307.4 | 2661.1 | 2968.6 | 2213.9 | 2470.7 | 2894.1 |
| (m/s) | 2121.3 | 2591.6 | 2952.7 | 1533.5 | 1906.5 | 2587.8 |
| (m/s) | 1526.6 | 1676.3 | 1878.8 | 1686.4 | 1717.5 | 1818.4 |
| (m/s) | 1401.9 | 1623.2 | 1859.9 | 1368.8 | 1401.2 | 1613.8 |
| (m/s) | 1530.4 | 1676.3 | 1872.6 | 1949.9 | 1932.2 | 1853.8 |
| (m/s) | 1308.7 | 1592.6 | 1859.9 | 619.4 | 895.0 | 1426.8 |
| Emax (GPa) | 22.80 | 28.99 | 36.26 | 29.95 | 22.87 | 18.87 |
| Emin (GPa) | 18.00 | 26.74 | 35.69 | 20.17 | 8.41 | 4.15 |
| νmax | 0.230 | 0.209 | 0.176 | 0.373 | 0.682 | 0.961 |
| νmin | 0.040 | 0.147 | 0.164 | 0.049 | −0.220 | −0.449 |
| AU | 0.1148 | 0.0126 | 0.0005 | 5.1503 | 1.9368 | 0.2837 |
| AZ | 1.36 | 1.11 | 0.98 | 6.12 | 3.30 | 1.61 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yalameha, S.; Nourbakhsh, Z.; Ramazani, A.; Vashaee, D. Promising Bialkali Bismuthides Cs(Na, K)2Bi for High-Performance Nanoscale Electromechanical Devices: Prediction of Mechanical and Anisotropic Elastic Properties under Hydrostatic Tension and Compression and Tunable Auxetic Properties. Nanomaterials 2021, 11, 2739. https://doi.org/10.3390/nano11102739
Yalameha S, Nourbakhsh Z, Ramazani A, Vashaee D. Promising Bialkali Bismuthides Cs(Na, K)2Bi for High-Performance Nanoscale Electromechanical Devices: Prediction of Mechanical and Anisotropic Elastic Properties under Hydrostatic Tension and Compression and Tunable Auxetic Properties. Nanomaterials. 2021; 11(10):2739. https://doi.org/10.3390/nano11102739
Chicago/Turabian StyleYalameha, Shahram, Zahra Nourbakhsh, Ali Ramazani, and Daryoosh Vashaee. 2021. "Promising Bialkali Bismuthides Cs(Na, K)2Bi for High-Performance Nanoscale Electromechanical Devices: Prediction of Mechanical and Anisotropic Elastic Properties under Hydrostatic Tension and Compression and Tunable Auxetic Properties" Nanomaterials 11, no. 10: 2739. https://doi.org/10.3390/nano11102739
APA StyleYalameha, S., Nourbakhsh, Z., Ramazani, A., & Vashaee, D. (2021). Promising Bialkali Bismuthides Cs(Na, K)2Bi for High-Performance Nanoscale Electromechanical Devices: Prediction of Mechanical and Anisotropic Elastic Properties under Hydrostatic Tension and Compression and Tunable Auxetic Properties. Nanomaterials, 11(10), 2739. https://doi.org/10.3390/nano11102739









