Role of Nanoimprint Lithography for Strongly Miniaturized Optical Spectrometers
Abstract
1. Introduction
2. Static FP Filter Arrays on Photodetector Arrays
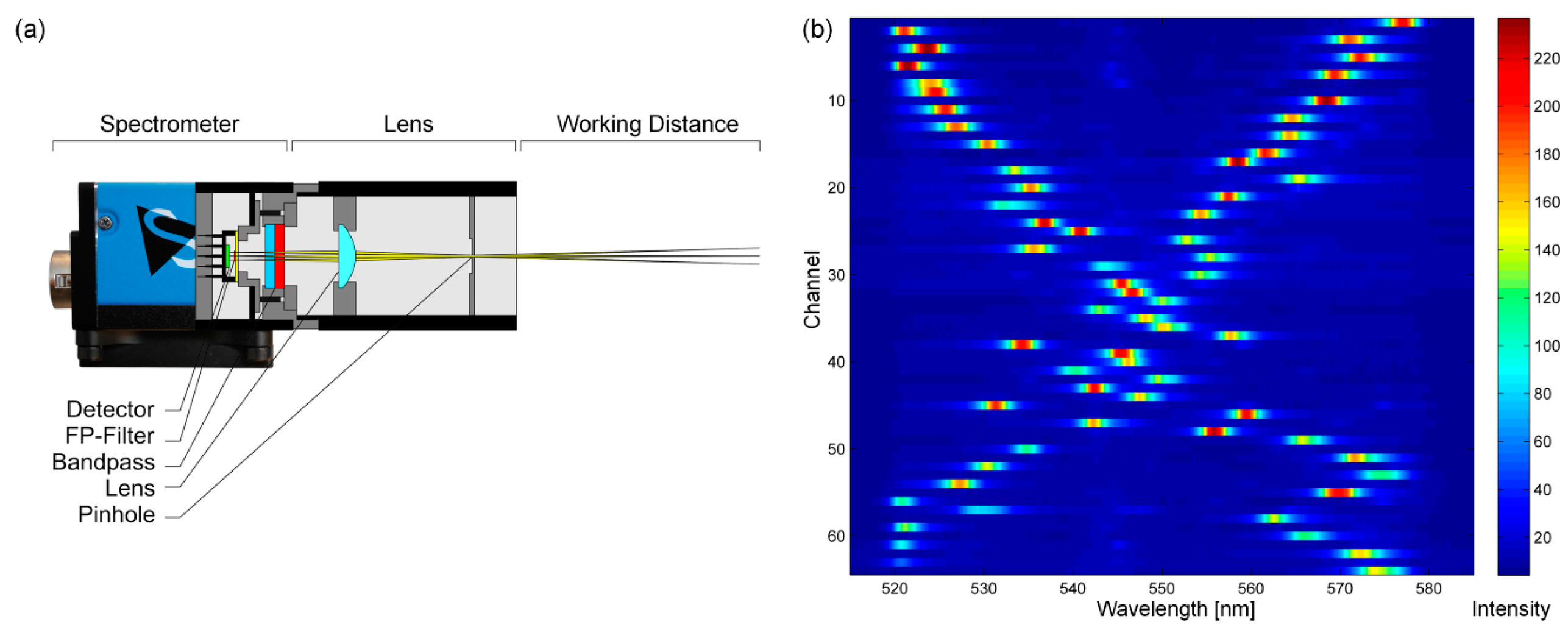
2.1. Microspectrometers
2.2. Nanospectrometers
3. Technological Fabrication of 3D Nanoimprint Templates by Digital Etching
4. Static FP Filter Array Fabrication in the VIS Spectral Range Demonstrating Single Nanoimprint over Three DBR Stacks of Different Heights
4.1. Nanomaterial and Geometric Issues of DBR Mirrors
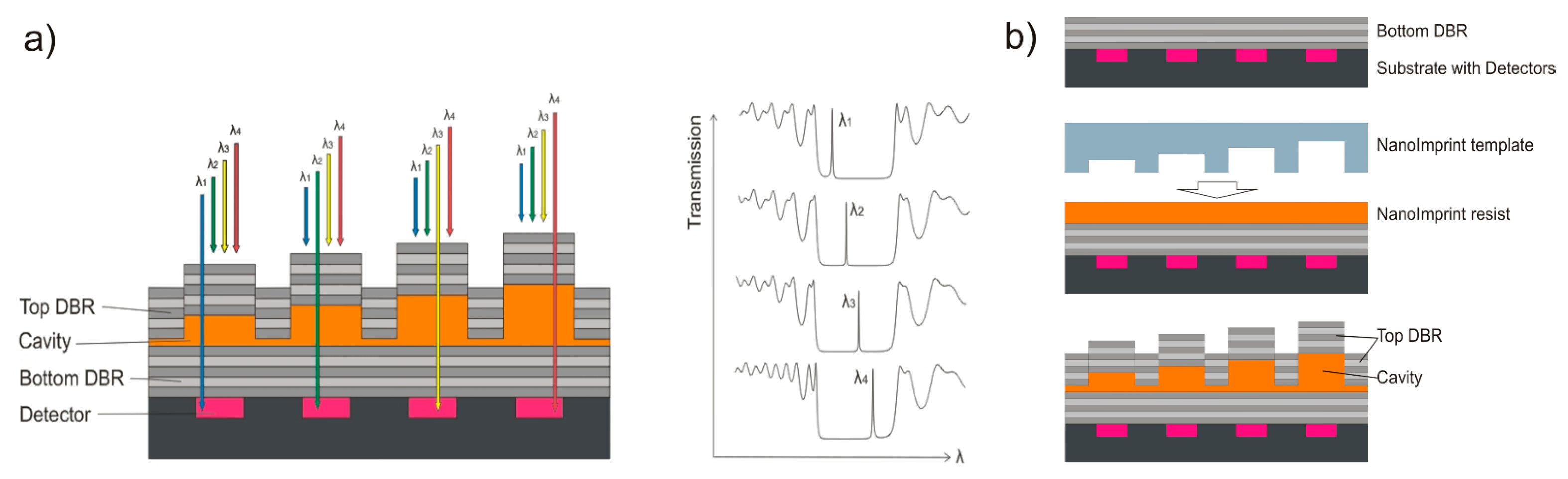
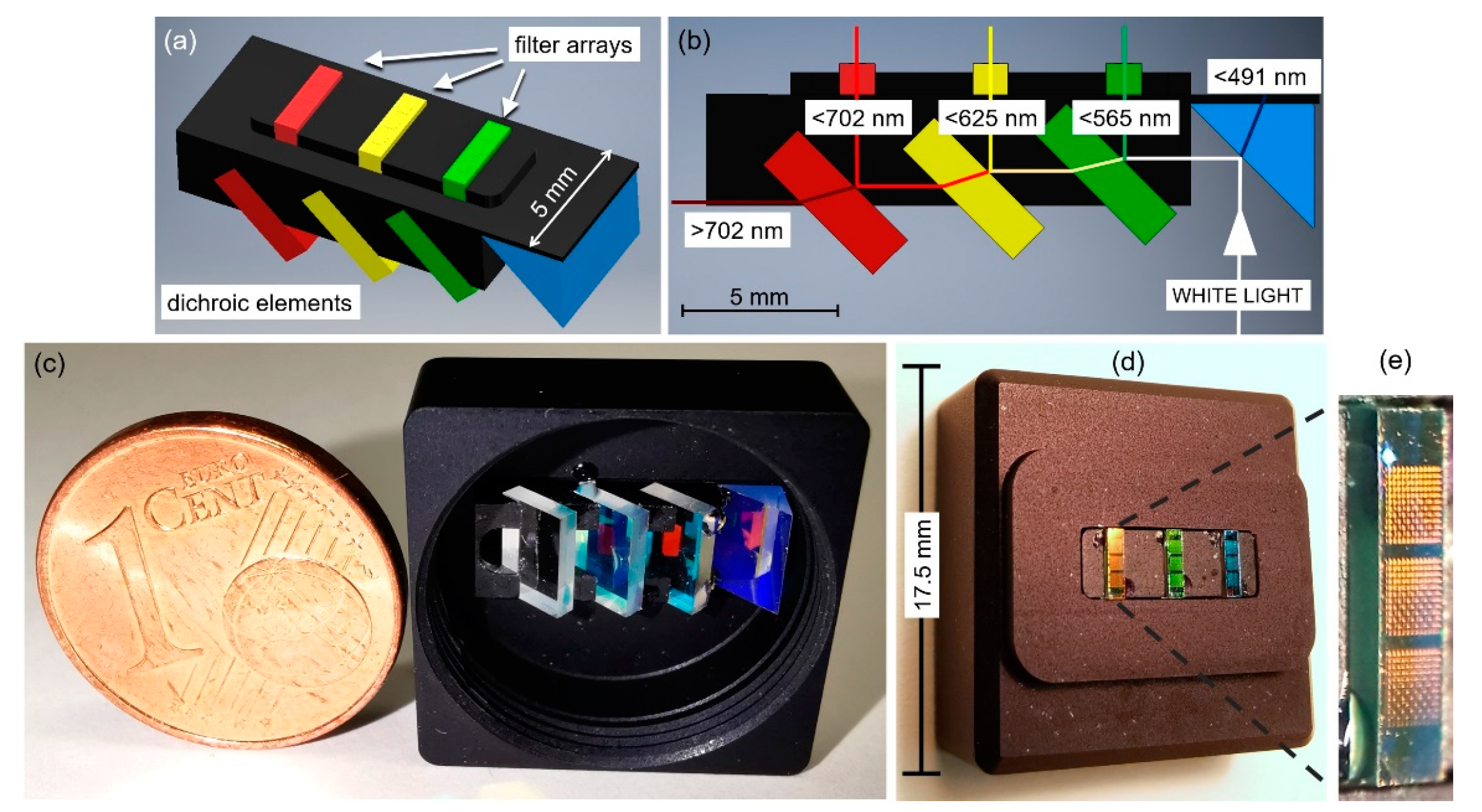
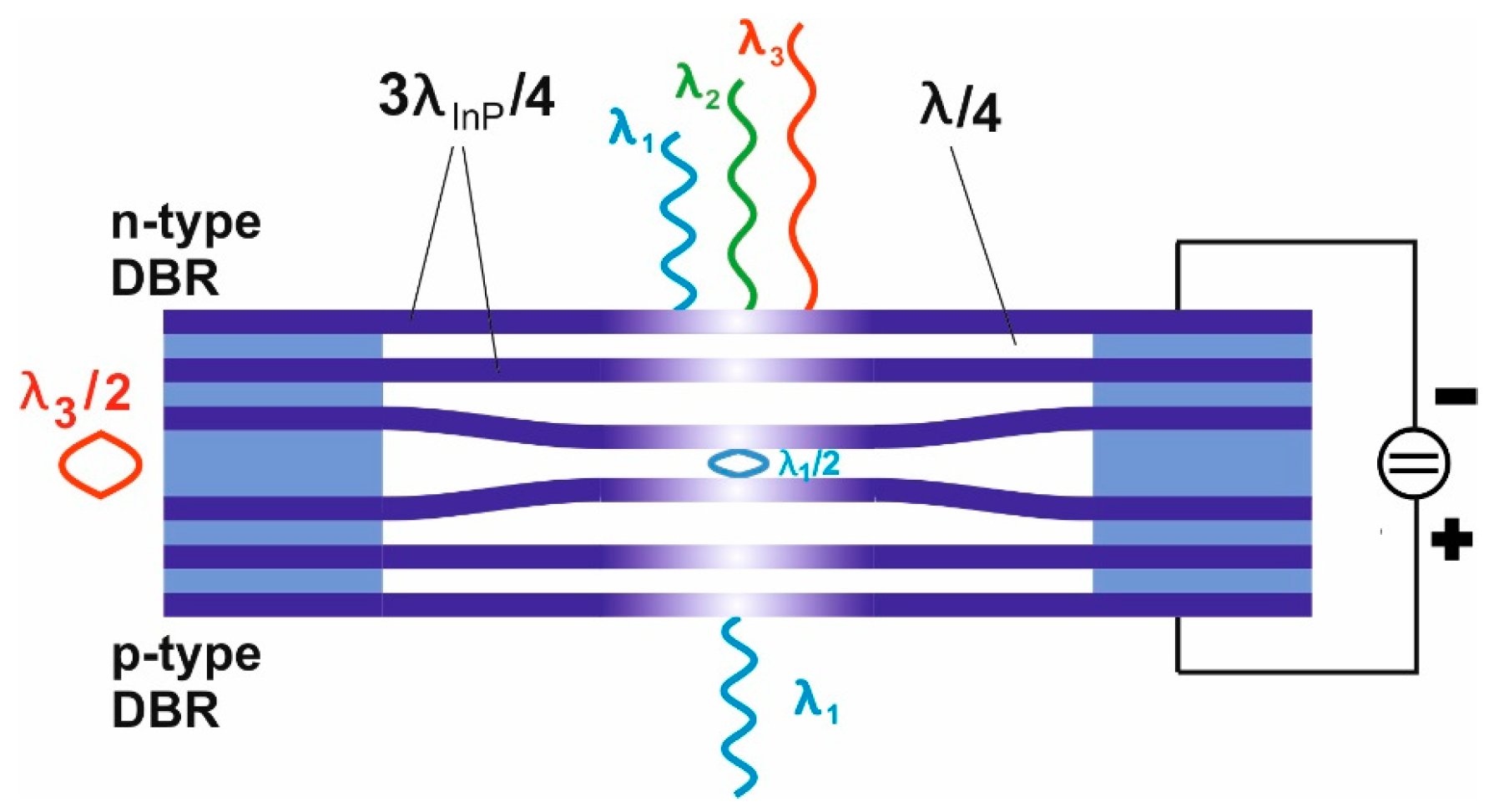
4.2. Fabrication Process of a FP Filter Array Combining Three Stopbands
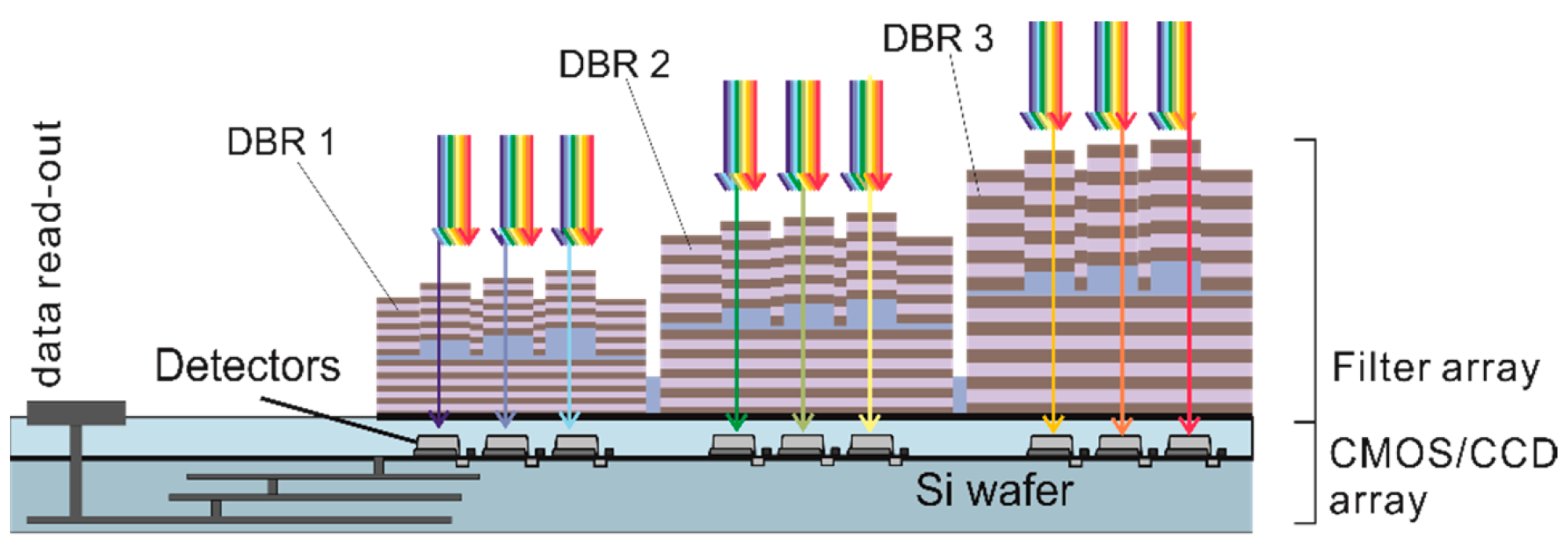
4.3. Lateral Arrangement of the FP Filters within the Array
5. Experimental Results of Static FP Filter Arrays in the VIS Range
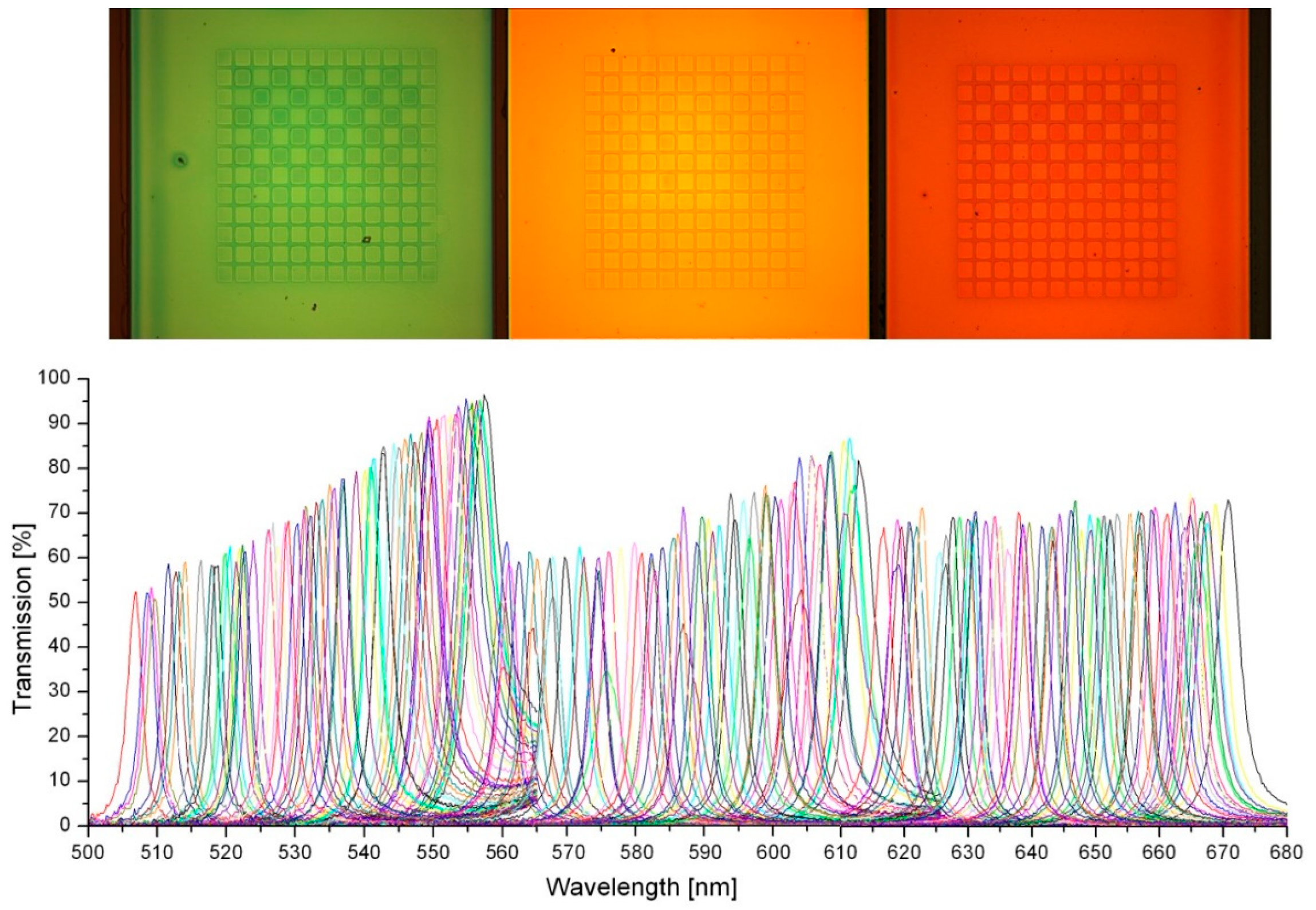
5.1. Transmission Spectra of Static FP Filter Arrays
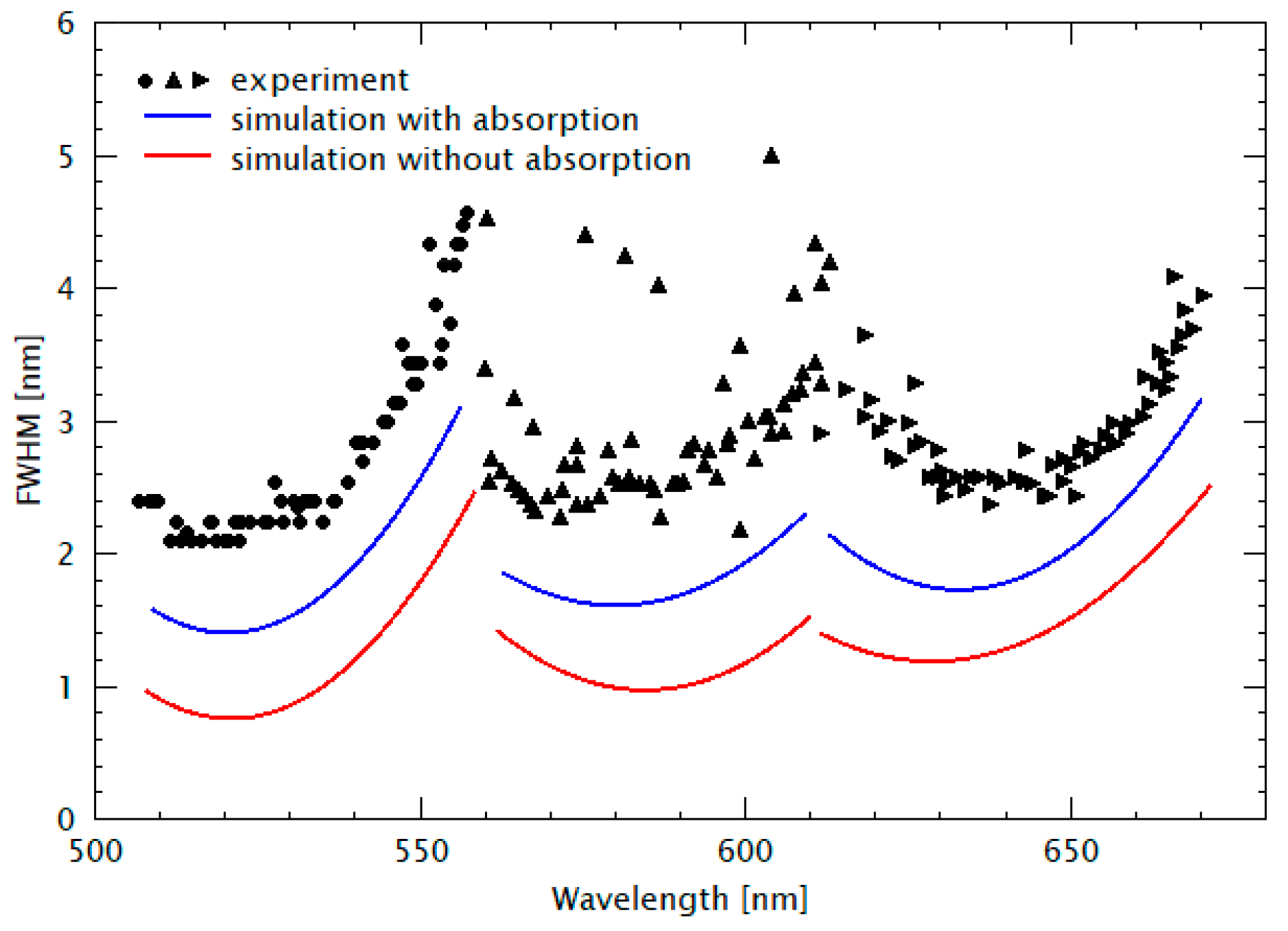
5.2. Experimental Linewidths
5.3. Discussion of the Linewidth Variation with Spectral Position
6. Laboratory Demonstrator of a Static FP Filter Array on a Detector Array with Telecentric Optics: Data Processing and Evaluation
7. Laboratory Demonstration of Efficiency Boosting by Spectral Preselection
8. FP Filter Arrays for the NIR: Fabrication and Characterization
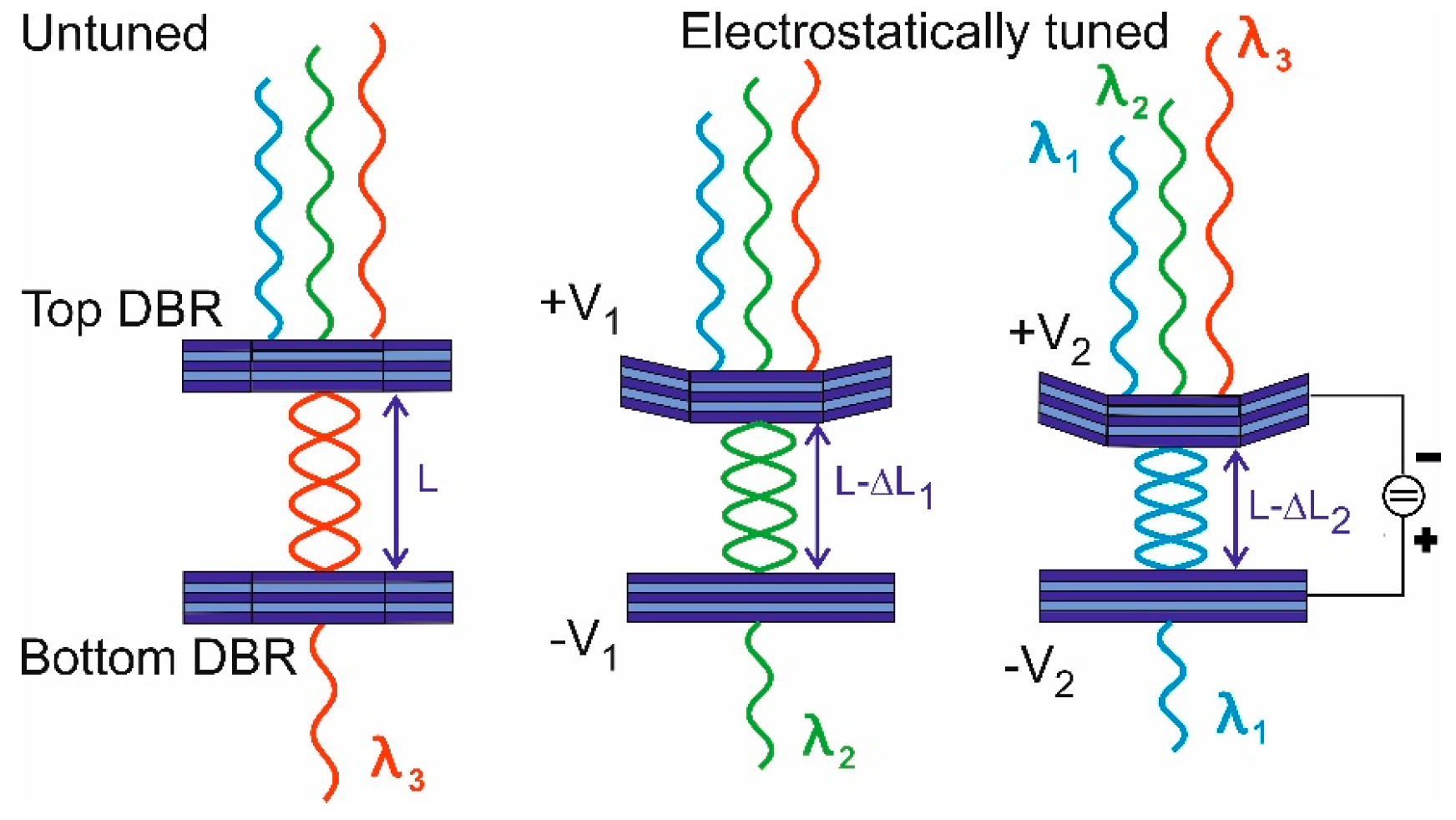
9. Fabrication and Characterization of MEMS Tunable FP Filters in the NIR Range
10. Estimation of Potential Space Requirement after Utmost Miniaturization
10.1. Static FP Filter Arrays to Cover a Spectral Span of 400 nm in the VIS Range
10.2. Static FP Filter Arrays to Cover a Spectral Span of 500 nm in the NIR Range
10.3. MEMS Tunable FP Filter Arrays to Cover a Spectral Span of 500 nm in the NIR Range
10.4. MEMS Tunable FP Filter Arrays to Cover a Spectral Span of 400 nm in the VIS Range
10.5. MEMS Tunable PC Filter to Cover a Spectral Span of 500 nm in the NIR Range
10.6. AWG to Cover a Spectral Span of 500 nm in the NIR Range
11. Resolution Limits of 3D Nanoimprint Lithography
12. Role of Nanoimprint for the Five Methodologies Compared
13. Conclusions
14. Patents
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lindon, J.C.; Tranter, G.E.; Koppenaal, D. Encyclopedia of Spectroscopy and Spectrometry, 3rd ed.; Elsevier Science: San Diego, CA, USA, 2016; ISBN 978-0-12-803224-4. [Google Scholar]
- Haken, H.; Wolf, H.C. Modern Methods of Optical Spectroscopy. In The Physics of Atoms and Quanta; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar] [CrossRef]
- Baeten, V.; Dardenne, P. Spectroscopy: Developments in Instrumentation and Analysis. Grasas Aceites 2002, 53, 45–63. [Google Scholar] [CrossRef]
- Tkachenko, N.V. Optical Spectroscopy: Methods and Instrumentations, 1st ed.; Elsevier: Amsterdam, The Netherlands, 2006; ISBN 978-0-444-52126-2. [Google Scholar]
- Dakin, J.P.; Chambers, P. Review of Methods of Optical Gas Detection by Direct Optical Spectroscopy, with Emphasis on Correlation Spectroscopy. In Optical Chemical Sensors. NATO Science Series II: Mathematics, Physics and Chemistry; Baldini, F., Chester, A., Homola, J., Martellucci, S., Eds.; Springer: Dordrecht, The Netherlands, 2006; Volume 224, pp. 457–477. ISBN 978-1-4020-4609-4. [Google Scholar]
- Hodgkinson, J.; Tatam, R.P. Optical Gas Sensing: A Review. Meas. Sci. Technol. 2013, 24, 012004. [Google Scholar] [CrossRef]
- Rolinger, L.; Rüdt, M.; Hubbuch, J. A Critical Review of Recent Trends, and a Future Perspective of Optical Spectroscopy as PAT in Biopharmaceutical Downstream Processing. Anal. Bioanal. Chem. 2020, 412, 2047–2064. [Google Scholar] [CrossRef]
- Neumann, W. Fundamentals of Dispersive Optical Spectroscopy Systems; Society of Photo-Optical Instrumentation Engineers (SPIE): Bellingham, WA, USA, 2013; PM242; ISBN 978-081-949-824-3. [Google Scholar]
- Appenzeller, I. Optical-Range Grating and Prism Spectrometers. In Introduction to Astronomical Spectroscopy (Cambridge Observing Handbooks for Research Astronomers); Cambridge University Press: Cambridge, UK, 2012; pp. 81–126. [Google Scholar] [CrossRef]
- Thorne, A.P. Dispersion and Resolving power: Prism Spectrographs. In Spectrophysics; Springer: Dordrecht, The Netherlands, 1988. [Google Scholar] [CrossRef]
- Kenda, A.; Frank, A.; Kraft, M.; Tortschanoff, A.; Sandner, T.; Schenk, H.; Scherf, W. Compact High-Speed Spectrometers Based on MEMS Devices with Large Amplitude In-Plane Actuators. Procedia Chem. 2009, 1, 556–559. [Google Scholar] [CrossRef]
- Tormen, M.; Lockhart, R.; Niedermann, P.; Overstolz, T.; Hoogerwerf, A.; Mayor, J.-M.; Pierer, J.; Bosshard, C.; Ischer, R.; Voirin, G.; et al. MEMS Tunable Grating Micro-spectrometer. In Proceedings of the International Conference on Space Optics—ICSO 2008, Toulouse, France, 14–18 October 2008; Volume 1056607. [Google Scholar] [CrossRef]
- Huang, J.; Wen, Q.; Nie, Q.; Chang, F.; Zhou, Y.; Wen, Z. Miniaturized NIR Spectrometer Based on Novel MOEMS Scanning Tilted Grating. Micromachines 2018, 9, 478. [Google Scholar] [CrossRef]
- Truxal, S.T.; Kurabayashi, K.; Tung, J.-C. Design of a MEMS Tunable Polymer Grating for Single Detector Spectroscopy. Int. J. Optomechatronics 2008, 2, 75–87. [Google Scholar] [CrossRef]
- Harmon, K. Interferometers: Fundamentals, Methods and Applications (Physics Research and Technology); Nova Science Publishers: Hauppauge, NY, USA, 2015; ISBN 978-1-63483-692-0. [Google Scholar]
- Hariharan, P. Basics of Interferometry; Academic Press: Boston, MA, USA, 1992; ISBN 978-012-325-218-0. [Google Scholar]
- Andersson, P.O.; Edwall, G.; Persson, A.; Thylén, L. Fiber Optic Mach-Zehnder Interferometer Based on Lithium Niobate Components. In Integrated Optics. Springer Series in Optical Sciences; Nolting, H.P.J., Ulrich, R., Eds.; Springer: Berlin/Heidelberg, Germany, 1985; Volume 48, pp. 26–28. ISBN 978-3-540-39452-5. [Google Scholar]
- Zetie, K.P.; Adams, S.F.; Tocknell, R.M. How Does a Mach-Zehnder Interferometer Work? Phys. Educ. 2000, 35, 46–48. [Google Scholar] [CrossRef]
- Russel, J.; Cohn, R. Mach Zehnder Interferometer; Book on Demand: Norderstedt, Germany, 2013; ISBN 978-551-267-842-8. [Google Scholar]
- Smit, M.K.; van Dam, C. PHASAR-Based WDM-Devices: Principles, Design and Applications. IEEE J. Sel. Top. Quantum Electron. 1996, 2, 236–250. [Google Scholar] [CrossRef]
- Kohtoku, M.; Sanjoh, H.; Oku, S.; Kadota, Y.; Yoshikuni, Y.; Shibata, Y. InP-Based 64-Channel Arrayed Waveguide Grating with 50 GHz Channel Spacing and up to −20dB Crosstalk. Electron. Lett. 1997, 33, 1786–1787. [Google Scholar] [CrossRef]
- Kohtoku, M.; Sanjoh, H.; Oku, S.; Kadota, Y.; Yoshikuni, Y. Polarization Independent Semiconductor Arrayed Waveguide Gratings Using a Deep-Ridge Waveguide Structure. IEICE Trans. Electron. 1998, E81-C, 1195–1204. [Google Scholar]
- Yoshikuni, Y. Semiconductor Arrayed Waveguide Gratings for Photonic Integrated Devices. IEEE J. Sel. Top. Quantum Electron. 2002, 8, 1102–1114. [Google Scholar] [CrossRef]
- Cvetojevic, N.; Jovanovic, N.; Bland-Hawthorn, J.; Haynes, R.; Lawrence, J. Miniature Spectrographs: Characterization of Arrayed Waveguide Gratings for Astronomy. In Proceedings of the SPIE Astronomical Telescopes + Instrumentation, San Diego, CA, USA, 27 June – 2 July 2010; Volume 7739, p. 77394H. [Google Scholar] [CrossRef]
- Seyringer, D.; Sagmeister, M.; Maese-Novo, A.; Eggeling, M.; Rank, R.; Muellner, P.; Hainberger, R.; Drexler, W.; Vlaskovic, M.; Zimmermann, h.; et al. Technological Verification of Size-Optimized 160-Channel Silicon Nitride-Based AWG-Spectrometer for Medical Applications. Appl. Phys. B 2019, 125, 88. [Google Scholar] [CrossRef]
- Muneeb, M.; Ruocco, A.; Malik, A.; Pathak, S.; Ryckeboer, E.; Sanchez, D.; Cerutti, L.; Rodriguez, J.B.; Tournié, E.; Bogaerts, W.; et al. Silicon-on-Insulator Shortwave Infrared Wavelength Meter with Integrated Photodiodes for on-Chip Laser Monitoring. Opt. Express 2014, 22, 27300–27308. [Google Scholar] [CrossRef] [PubMed]
- Correia, J.H.; Bartek, M.; Wolffenbuttel, R.F. High-Selectivity Single-Chip Spectrometer in Silicon for Operation in Visible Part of the Spectrum. IEEE Trans. Electron. Devices 2000, 47, 191–197. [Google Scholar] [CrossRef]
- Correia, J.H.; de Graaf, G.; Kong, S.H.; Bartek, M.; Wolffenbuttel, R.F. Single-Chip CMOS Optical Micro-Spectrometer. Sens. Actuators A Phys. 2000, 82, 191–197. [Google Scholar] [CrossRef]
- Wolffenbuttel, R.F. MEMS-Based Optical Mini- and Microspectrometers for the Visible and Infrared Spectral Range. J. Micromech. Microeng. 2005, 15, 145–152. [Google Scholar] [CrossRef]
- Wang, S.W.; Li, M.; Xia, C.S.; Wang, H.Q.; Chen, X.S.; Lu, W. 128 Channels of Integrated Filter Array Rapidly Fabricated by Using the Combinatorial Deposition Technique. Appl. Phys. B 2007, 88, 281–284. [Google Scholar] [CrossRef]
- Hillmer, H. Optisches Filter und Verfahren Zu Seiner Herstellung (Optical Filter and Its Fabrication Technology). Patent DE 10 2006 039 071, 19 April 2012. [Google Scholar]
- Albrecht, A.; Wang, X.; Mai, H.H.; Schotzko, T.; Memon, I.; Hornung, M.; Bartels, M.; Hillmer, H. High Vertical Resolution 3D Nano-Imprint Technology and Its Application in Optical Nanosensors. Nonlinear Opt. Quantum Opt. 2012, 43, 339–353. [Google Scholar]
- Wang, X.; Albrecht, A.; Mai, H.H.; Woidt, C.; Meinl, T.; Hornung, M.; Bartels, M.; Hillmer, H. High Resolution 3D Nanoimprint Technology: Template Fabrication, Application in Fabry–Pérot Filter-Array-Based Optical Nanospectrometers. Microelectron. Eng. 2013, 110, 44–51. [Google Scholar] [CrossRef]
- Mai, H.H.; Albrecht, A.; Woidt, C.; Wang, X.; Daneker, V.; Setyawati, O.; Woit, T.; Schultz, K.; Bartels, M.; Hillmer, H. 3D Nanoimprinted Fabry-Pérot Filter Arrays and Methodologies for Optical Characterization. Appl. Phys. B Lasers Opt. 2012, 107, 755–764. [Google Scholar] [CrossRef]
- Nguyen, D.T.; Ababtain, M.; Memon, I.; Ullah, A.; Istock, A.; Woidt, C.; Xie, W.; Lehmann, P.; Hillmer, H. 3D Nanoimprint for NIR Fabry-Pérot Filter Arrays: Fabrication, Characterization and Comparison of Different Cavity Designs. Appl. Nanosci. 2016, 6, 1127–1135. [Google Scholar] [CrossRef][Green Version]
- Shen, Y.; Istock, A.; Zaman, A.; Woidt, C.; Hillmer, H. Fabrication and Characterization of Multi-Stopband Fabry–Pérot Filter Array for Nanospectrometers in the VIS Range Using SCIL Nanoimprint Technology. Appl. Nanosci. 2018, 8, 1415–1425. [Google Scholar] [CrossRef]
- Larson, M.C.; Harris, J.S. Broadly-Tunable Resonant-Cavity Light-Emitting Diode. IEEE Photonics Technol. Lett. 1995, 7, 1267–1269. [Google Scholar] [CrossRef]
- Larson, M.C.; Pezeshki, B.; Harris, J.S. Vertical Coupled-Cavity Microinterferometer on GaAs with Deformable-Membrane Top Mirror. IEEE Photonics Technol. Lett. 1995, 7, 382–384. [Google Scholar] [CrossRef]
- Vail, E.C.; Wu, M.S.; Li, G.S.; Eng, L.; Chang-Hasnain, C.J. GaAs Micromachined Widely Tunable Fabry-Perot Filters. Electron. Lett. 1995, 31, 228–229. [Google Scholar] [CrossRef]
- Wu, M.S.; Vail, E.C.; Li, G.S.; Yuen, W.; Chang-Hasnain, C.J. Tunable Micromachined Vertical Cavity Surface Emitting Laser. Electron. Lett. 1995, 31, 1671–1672. [Google Scholar] [CrossRef]
- Spisser, A.; Ledantec, R.; Seassal, C.; Leclercq, J.L.; Benyattou, T.; Rondi, D.; Blondeau, R.; Guillot, G.; Viktorovitch, P. Highly Selective and Widely Tunable 1.55-mInP/Air-Gap Micromachined Fabry–Perot Filter for Optical Communications. IEEE Photonics Technol. Lett. 1998, 10, 1259–1261. [Google Scholar] [CrossRef]
- Tayebati, P.; Wang, P.; Azimi, M.; Maflah, L.; Vakhshoori, D. Microelectromechanical Tunable Filter with Stable Half Symmetric Cavity. Electron. Lett. 1998, 34, 1967–1968. [Google Scholar] [CrossRef]
- Peerlings, J.; Dehe, A.; Vogt, A.; Tilsch, M.; Hebeler, C.; Langenhan, F.; Meissner, P.; Hartnagel, H.L. Long Resonator Micromachined Tunable GaAs-AlAs Fabry-Perot Filter. IEEE Photonics Technol. Lett. 1997, 9, 1235–1237. [Google Scholar] [CrossRef]
- Streubel, K.; Rapp, S.; Andre, J.; Chitica, N. 1.26 µm Vertical Cavity Laser with Two InP/Air-Gap Reflectors. Electron. Lett. 1996, 32, 1369–1370. [Google Scholar] [CrossRef]
- Le Dantec, R.; Benyattou, T.; Guillot, G.; Spisser, G.; Seassal, C.; Leclercq, J.L.; Victorovitch, P.; Blondeau, R. Tunable Microcavity Based on InP-Air Bragg Mirrors. IEEE J. Sel. Top. Quantum Electron. 1999, 5, 111–114. [Google Scholar] [CrossRef]
- Hillmer, H.; Daleiden, J.; Prott, C.; Römer, F.; Irmer, S.; Rangelov, V.; Tarraf, A.; Schüler, S.; Strassner, M. Potential for Micromachined Actuation of Ultra-Wide Continuously Tunable Optoelectronic Devices. Appl. Phys. B 2002, 75, 3–13. [Google Scholar] [CrossRef]
- Daleiden, J.; Chitica, N.; Strassner, M.; Spisser, A.; Leclercq, J.L.; Victorovitch, P.; Rondi, D.; Goutain, E.; Peerlings, J.; Pfeiffer, J.; et al. Tunable InP/Air Gap Fabry Perot Filter for Wavelength Division Multiplex Fiber Optical Transmission. In Proceedings of the 11th International Conference on Indium Phosphide and Related Materials (IPRM’99), Davos, Switzerland, 16–20 May 1999; pp. 285–287. [Google Scholar] [CrossRef]
- Strassner, M.; Daleiden, J.; Chitica, N.; Keiper, D.; Stålnacke, B.; Greek, D.; Hjort, K. III-V Semiconductor Material for Tunable Fabry-Perrot Filters for Coarse and Dense WDM Systems. Sens. Actuators A Phys. 2000, 85, 249–255. [Google Scholar] [CrossRef]
- Chitica, N.; Strassner, M. Room-Temperature Operation of Photopumped Monolithic InP Vertical Cavity Laser with Two Air-Gap Bragg Reflectors. Appl. Phys. Lett. 2001, 78, 3935–3937. [Google Scholar] [CrossRef]
- Irmer, S.; Daleiden, J.; Rangelov, V.; Prott, C.; Römer, F.; Strassner, M.; Tarraf, A.; Hillmer, H. Ultralow Biased Widely Continuously Tunable Fabry-Perot Filter. IEEE Photonics Technol. Lett. 2003, 15, 434–436. [Google Scholar] [CrossRef]
- Daleiden, J.; Hillmer, H. Multiple Air-Gap Filters and Constricted Mesa Lasers—Material Processing Meets the Front of Optical Device Technology. Appl. Phys. B 2003, 76, 821–832. [Google Scholar] [CrossRef]
- Hillmer, H.; Daleiden, J.; Irmer, S.; Römer, F.; Prott, C.; Tarraf, A.; Strassner, M.; Ataro, E.; Scholz, T. Potential of Micromachined Photonics: Miniaturization, Scaling and Applications in Continuously Tunable Vertical Air-Cavity Filters. Photonics Fabr. Eur. 2003, 4947, 197–211. [Google Scholar] [CrossRef]
- Prott, C.; Römer, F.; Ataro, E.O.; Daleiden, J.; Irmer, S.; Tarraf, A.; Hillmer, H. Modeling of Ultrawidely Tunable Vertical Cavity Air-Gap Filters and VCSELs. IEEE J. Sel. Top. Quantum Electron. 2003, 9, 918–928. [Google Scholar] [CrossRef]
- Römer, F.; Prott, C.; Irmer, S.; Daleiden, J.; Tarraf, A.; Hillmer, H.; Strassner, M. Tuning Efficiency and Linewidth of Electrostatically Actuated Multiple Air-Gap Filters. Appl. Phys. Lett. 2003, 82, 176–178. [Google Scholar] [CrossRef]
- Hasse, A.; Irmer, S.; Daleiden, J.; Dharmarasu, N.; Hansmann, S.; Hillmer, H. Wide Continuous Tuning Range of 221nm by InP/Air-Gap Vertical-Cavity Filters. Electron. Lett. 2006, 42, 974–975. [Google Scholar] [CrossRef]
- Römer, F. Charakterisierung und Simulation Optischer Eigenschaften von Mikromechanisch Abstimmbaren Filterbauelementen (Characterization and Simulation of Optical Properties of Micromachined Tunable Filter Devices); Kassel University Press: Kassel, Germany, 2006; ISBN 978-3-89958-196-6. [Google Scholar]
- Williams, C.; Hong, N.; Julian, M.; Borg, S.; Kim, H.J. Tunable Mid-Wave Infrared Fabry-Perot Bandpass Filters Using Phase-Change GeSbTe. Opt. Express 2020, 28, 10583–10594. [Google Scholar] [CrossRef] [PubMed]
- Magnusson, R.; Wang, S.S. New Principle for Optical Filters. Appl. Phys. Lett. 1992, 61, 1022–1024. [Google Scholar] [CrossRef]
- Kanskar, M.; Paddon, P.; Pacradouni, V.; Morin, R.; Busch, A.; Young, J.F.; Johnson, S.R.; MacKenzie, J.; Tiedje, T. Observation of Leaky Slab Modes in an Air-Bridged Semiconductor Waveguide with a Two-Dimensional Photonic Lattice. Appl. Phys. Lett. 1997, 70, 1438–1440. [Google Scholar] [CrossRef]
- Hessel, O.; Oliner, A.A. A New Theory of Wood’s Anomalies on Optical Gratings. Appl. Opt. 1965, 4, 1275–1297. [Google Scholar] [CrossRef]
- Boutami, S.; Benbakir, B.; Letartre, X.; Leclercq, J.L.; Regreny, P.; Viktorovitch, P. Ultimate Vertical Fabry-Perot Cavity Based on Single-Layer Photonic Crystal Mirrors. Opt. Express 2007, 15, 12443–12449. [Google Scholar] [CrossRef] [PubMed]
- Boutami, S.; Bakir, B.B.; Hattori, H.; Letartre, X.; Leclercq, J.-L.; Rojo-Rome, P.; Garrigues, M.; Seassal, C.; Viktorovitch, P. Broadband and Compact 2-D Photonic Crystal Reflectors with Controllable Polarization Dependence. IEEE Photonics Technol. Lett. 2006, 18, 835–837. [Google Scholar] [CrossRef]
- Zamora, R.; Benes, M.; Kusserow, T.; Hillmer, H.; Akcakoca, U.; Witzigmann, B. Optical Characterization of Photonic Crystals as Polarizing Structures for Tunable Optical MEMS Devices. In Proceedings of the 16th International Conference on Optical MEMS and Nanophotonics, Istanbul, Turkey, 8–11 August 2011; pp. 83–84. [Google Scholar] [CrossRef]
- Kupec, J.; Akcakoca, U.; Witzigmann, B. Frequency Domain Analysis of Guided Resonances And polarization Selectivity in Photonic Crystal Membranes. J. Opt. Soc. Am. B 2011, 28, 69–78. [Google Scholar] [CrossRef]
- Kilic, O.; Digonnet, M.; Kino, G.; Solgaaard, O. Controlling Uncoupled Resonances in Photonic Crystals Through Breaking the Mirror Symmetry. Opt. Express 2008, 16, 13090–13103. [Google Scholar] [CrossRef]
- Kikuta, H.; Toyota, H.; Yu, W. Optical Elements with Subwavelength Structured Surfaces. Opt. Rev. 2003, 10, 63–73. [Google Scholar] [CrossRef]
- Oshita, M.; Takahashi, H.; Ajiki, Y.; Kan, T. Reconfigurable Surface Plasmon Resonance Photodetector with a MEMS Deformable Cantilever. ACS Photonics 2020, 7, 673–679. [Google Scholar] [CrossRef]
- Momeni, B.; Hosseini, E.S.; Askari, M.; Soltani, M.; Adibi, A. Integrated Photonic Crystal Spectrometers for Sensing Applications. Opt. Commun. 2009, 282, 3168–3171. [Google Scholar] [CrossRef]
- Hamamatsu Photonics K.K. Mini-Spectrometers. Available online: https://www.hamamatsu.com/eu/en/product/optical-sensors/spectrometers/mini-spectrometer/index.html (accessed on 29 November 2020).
- Suh, W.; Yanik, M.F.; Solgaard, O.; Fan, S. Displacement-Sensitive Photonic Crystal Structures Based on Guided Resonance in Photonic Crystal Slabs. Appl. Phys. Lett. 2003, 82, 1999. [Google Scholar] [CrossRef]
- Notomi, M.; Taniyama, H.; Mitsugi, S.; Kuramochi, E. Optomechanical Wavelength and Energy Conversion in High-Q Double-Layer Cavities of Photonic Crystal Slabs. Phys. Rev. Lett. 2006, 97, 023903. [Google Scholar] [CrossRef] [PubMed]
- Midolo, L.; van Veldhoven, P.J.; Dündar, M.A.; Nötzel, R.; Fiore, A. Electromechanical Wavelength Tuning of Double-Membrane Photonic Crystal Cavities. Appl. Phys. Lett. 2011, 98, 211120. [Google Scholar] [CrossRef]
- Liapis, A.C.; Gao, B.; Siddiqui, M.R.; Shi, Z.; Boyd, R.W. On-Chip Spectroscopy with Thermally Tuned High-Q Photonic Crystal Cavities. Appl. Phys. Lett. 2016, 108, 021105. [Google Scholar] [CrossRef]
- Zobenica, Ž.; van der Heijden, R.W.; Petruzzela, M.; Pagliano, F.; Leijssen, R.; Xia, T.; Midolo, L.; Cotrufo, M.; Cho, Y.J.; van Otten, F.W.; et al. Integrated Nano-Opto-Electro-Mechanical Sensor for Spectrometry and Nanometrology. Nat. Commun. 2017, 8, 2216. [Google Scholar] [CrossRef] [PubMed]
- Michel, A.; Ruprecht, R.; Harmening, M.; Bacher, W. Abformung von Mikrostrukturen auf Prozessierten Wafern (Molding of Microstructures on Processed Wafers). Ph.D. Thesis, Universitat Karlsruhe, Karlsruhe, Germany. Bericht des Kernforschungszentrums Karlsruhe KfK 5171.
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Nanoimprint Lithography. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. 1996, 14, 4129–4133. [Google Scholar] [CrossRef]
- Ji, R.; Hornung, M.; Verschuuren, M.A.; van de Laar, R.; van Eeckelen, J.; Plachetka, U.; Moeller, M.; Moormann, C. UV Enhanced Substrate Conformal Imprint Lithography (UV-SCIL) Technique for Photonic Crystals Patterning in LED Manufacturing. Microelectron. Eng. 2010, 87, 963–967. [Google Scholar] [CrossRef]
- Verschuuren, M.A.; Megens, M.; Ni, Y.; van Sprang, H.; Polman, A. Large Area Nanoimprint by Substrate Conformal Imprint Lithography (SCIL). Adv. Opt. Technol. 2017, 6, 243–264. [Google Scholar] [CrossRef]
- Larouche, S.; Martinu, L. OpenFilters: Open-Source Software for the Design, Optimization, and Synthesis of Optical Filters. Appl. Opt. 2008, 47, C219–C230. [Google Scholar] [CrossRef]
- Memon, I.; Shen, Y.; Khan, A.; Woidt, C.; Hillmer, H. Highly Uniform Residual Layers for Arrays of 3D Nanoimprinted Cavities in Fabry–Pérot Filter Array Based Nanospectrometers. Appl. Nanosci. 2015, 6, 599–606. [Google Scholar] [CrossRef]
- Emadi, A.; Wu, H.; de Graaf, G.; Wolffenbuttel, R. Design and Implementation of a Sub-nm Resolution Microspectrometer Based on a Linear-Variable Optical Filter. Opt. Express 2012, 20, 489. [Google Scholar] [CrossRef] [PubMed]
- Kobylinskiy, A.; Laue, B.; Förster, E.; Höfer, B.; Shen, Y.; Hillmer, H.; Brunner, R. Substantial Increase in Detection Efficiency for Filter Array-Based Spectral Sensors. Appl. Opt. 2020, 59, 2443–2451. [Google Scholar] [CrossRef] [PubMed]
- Abramson, A.R.; Tien, C.; Majumdar, A. Interface and Strain Effects on the Thermal Conductivity of Heterostructures: A Molecular Dynamics Study. J. Heat Transf. 2002, 124, 963–970. [Google Scholar] [CrossRef]
- Cheng, X.; Guo, L.J. A Combined-Nanoimprint-and-Photolithography Patterning Technique. Microelectron. Eng. 2004, 71, 277–282. [Google Scholar] [CrossRef]
- Kolli, V.R.; Woidt, C.; Hillmer, H. Residual-Layer-Free 3D Nanoimprint Using Hybrid Soft Templates. Microelectron. Eng. 2016, 149, 159–165. [Google Scholar] [CrossRef]
- Austin, M.D.; Ge, H.; Wei, W.; Li, M.; Yu, Z.; Wassermann, D.; Lyon, S.A.; Chou, S.Y. Fabrication of 5 nm Linewidth and 14 nm Pitch Features by Nanoimprint Lithography. Appl. Phys. Lett. 2004, 84, 5299–5301. [Google Scholar] [CrossRef]
- Hua, F.; Sun, Y.; Gaur, A.; Meitl, M.A.; Bilhaut, L.; Rotkina, L.; Wang, J.; Geil, P.; Shim, M.; Rogers, J.A.; et al. Polymer Imprint Lithography with Molecular-Scale Resolution. Nano Lett. 2004, 4, 2467–2471. [Google Scholar] [CrossRef]
- Yasuda, M.; Hirai, Y. Molecular Dynamics Simulations of Local Polymer Flow. In Proceedings of the 9th International Nanoimprint & Nanoprint Technology Conference, Copenhagen, Denmark, 13–15 October 2010; p. 32. [Google Scholar]
- Taga, A.; Yasuda, M.; Kawata, H.; Hirai, Y. Impact of Molecular Size on Resist Filling Process in Nanoimprint Lithography: Molecular Dynamics Study. J. Vac. Sci. Technol. B 2010, 28, C6M68. [Google Scholar] [CrossRef]
- Wallrabe, U.; Salle, V. LIGA Technology for R&D and Industrial Applications. In MEMS: A Practical Guide to Design, Analysis, and Applications; Korvink, J.G., Paul, O., Eds.; Springer: Berlin/Heidelberg, Germany, 2006; pp. 853–899. ISBN 978-3-540-21117-4. [Google Scholar]
- Müller, C.; Mohr, L. Microspectrometer Fabricated by the Liga Process. Microsyst. Technol. 1993, 18, 273–279. [Google Scholar] [CrossRef]
- Gibbon, M. Technologies for Photonic Integration. Ph.D. Thesis, University of Bath, Elsevier B.V., Amsterdam, The Netherlands, 1995. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hillmer, H.; Woidt, C.; Istock, A.; Kobylinskiy, A.; Nguyen, D.T.; Ahmed, N.; Brunner, R.; Kusserow, T. Role of Nanoimprint Lithography for Strongly Miniaturized Optical Spectrometers. Nanomaterials 2021, 11, 164. https://doi.org/10.3390/nano11010164
Hillmer H, Woidt C, Istock A, Kobylinskiy A, Nguyen DT, Ahmed N, Brunner R, Kusserow T. Role of Nanoimprint Lithography for Strongly Miniaturized Optical Spectrometers. Nanomaterials. 2021; 11(1):164. https://doi.org/10.3390/nano11010164
Chicago/Turabian StyleHillmer, Hartmut, Carsten Woidt, André Istock, Aliaksei Kobylinskiy, Duc Toan Nguyen, Naureen Ahmed, Robert Brunner, and Thomas Kusserow. 2021. "Role of Nanoimprint Lithography for Strongly Miniaturized Optical Spectrometers" Nanomaterials 11, no. 1: 164. https://doi.org/10.3390/nano11010164
APA StyleHillmer, H., Woidt, C., Istock, A., Kobylinskiy, A., Nguyen, D. T., Ahmed, N., Brunner, R., & Kusserow, T. (2021). Role of Nanoimprint Lithography for Strongly Miniaturized Optical Spectrometers. Nanomaterials, 11(1), 164. https://doi.org/10.3390/nano11010164








