A Critical Review on Metallic Glasses as Structural Materials for Cardiovascular Stent Applications
Abstract
1. Introduction
2. Conventional Metallic Glasses vs. Bulk Metallic Glasses
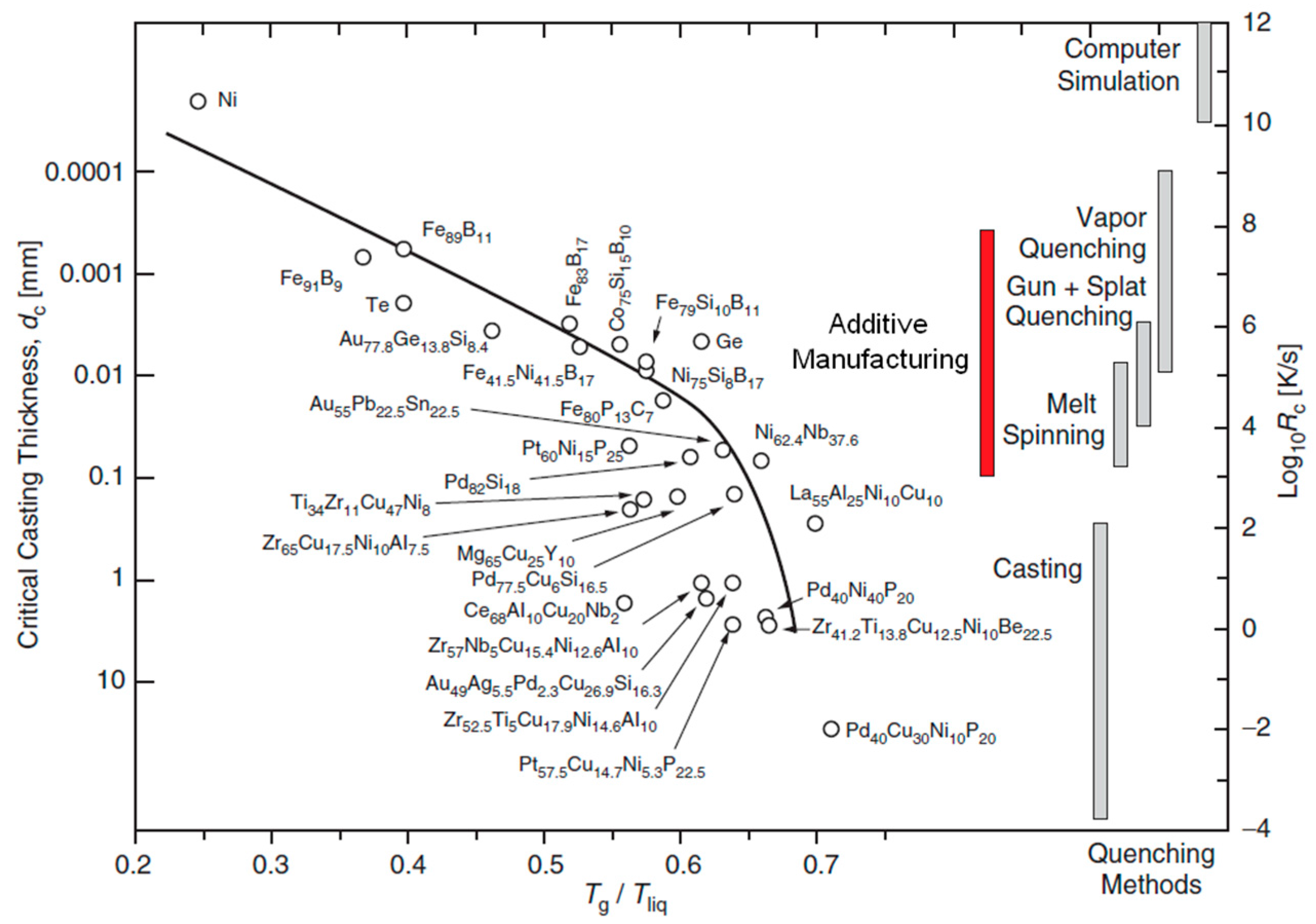
3. An Innovative Processing Route of Metallic Glasses: Additive Manufacturing
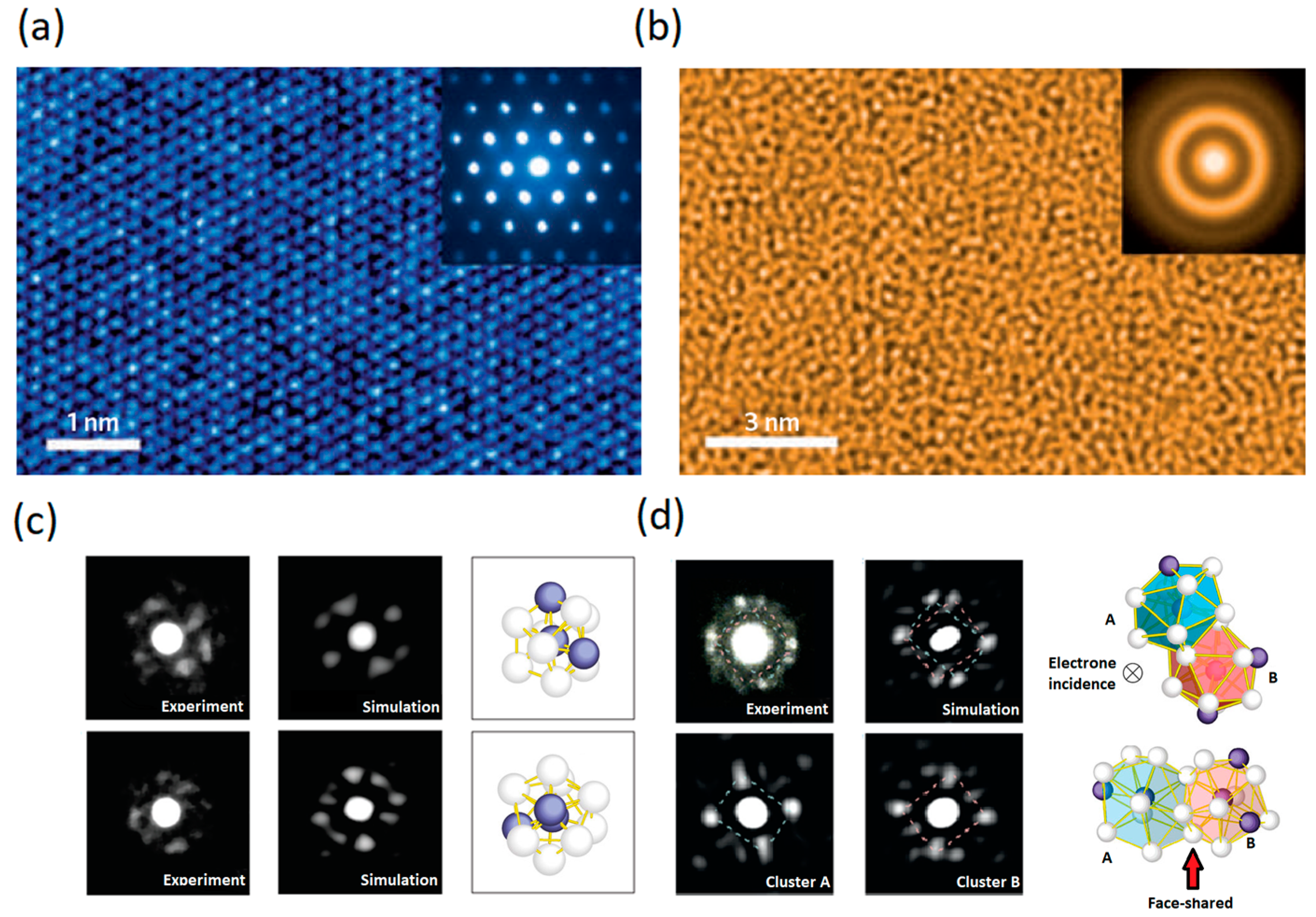
4. Structure of Metallic Glasses
5. Mechanical Properties
5.1. Strength Close to the Theoretical Limit
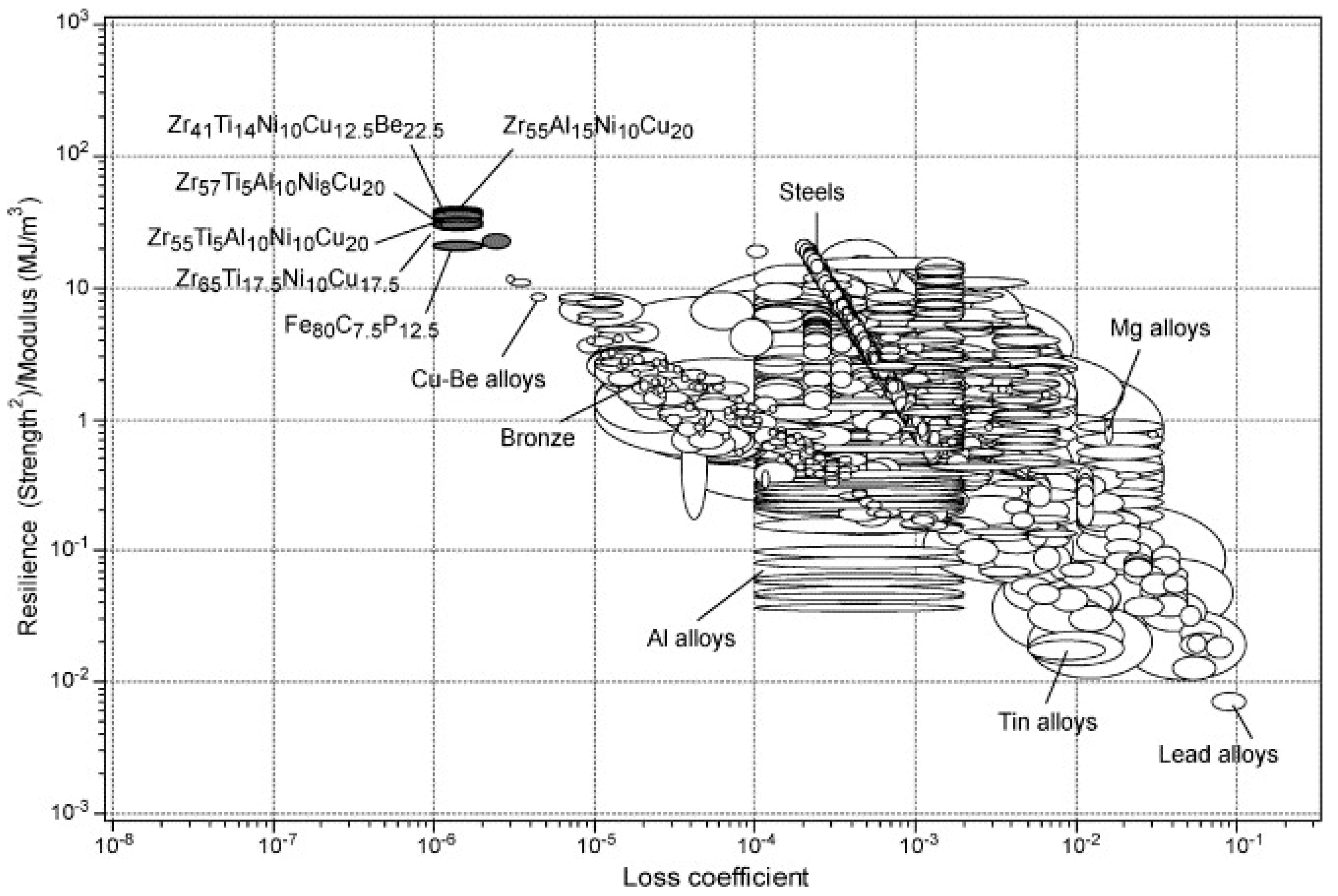
5.2. Elastic Properties: High Resilience
5.3. Plastic Properties: Severe Localized Plasticity
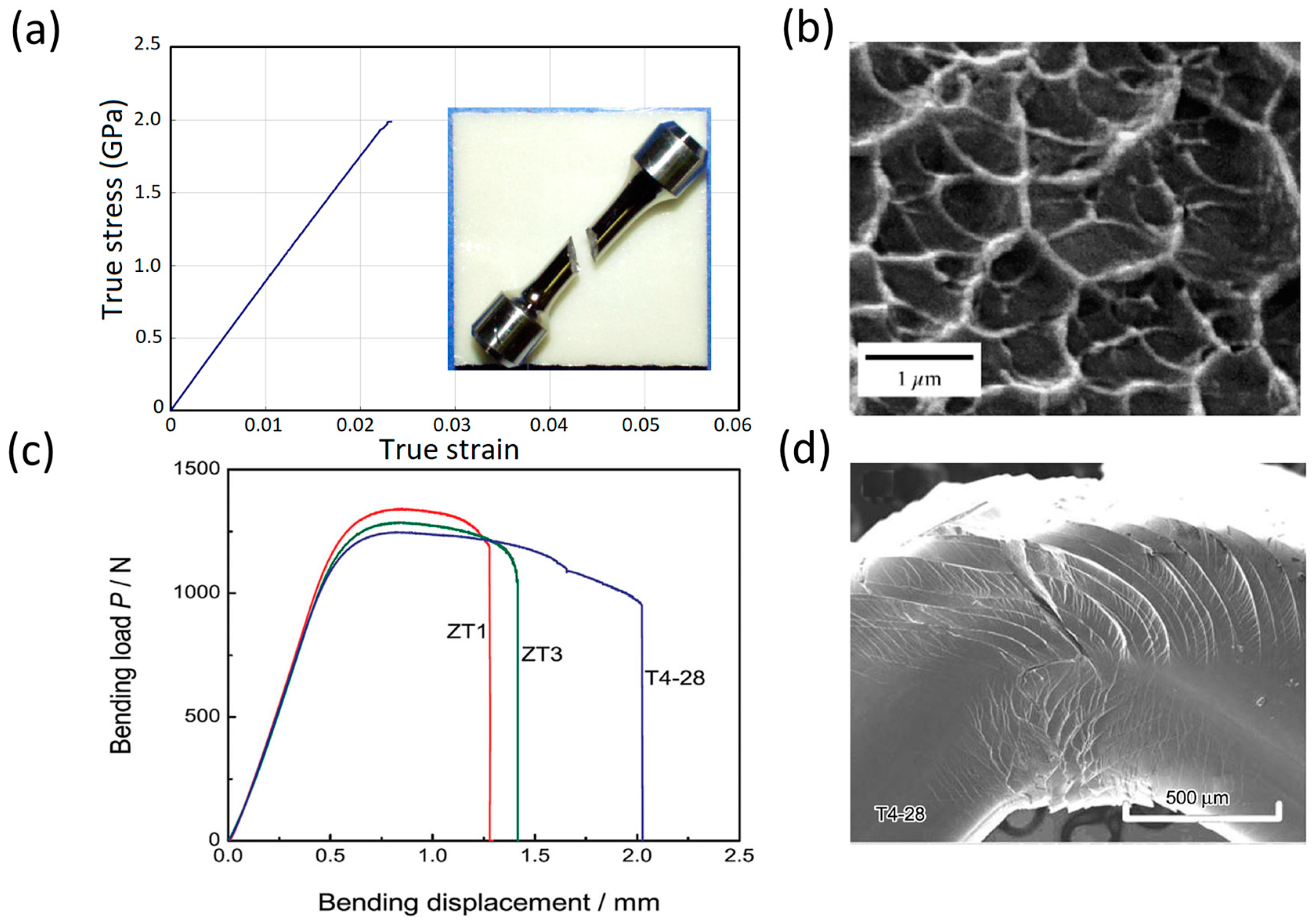
- The tensile fracture surface of MGs occurs along the plane with maximum resolved shear stress, i.e., at about 45° with the tensile loading axis, in contrast with 90° in typical brittle materials (see Figure 5a).
5.4. Fatigue Properties
5.5. Size Effects on Mechanical Properties
5.5.1. Size Effects on Yield Strength and Elastic Strain Limit
5.5.2. Size Effects on Ductility and Toughness
5.5.3. Size Effect on Fatigue Properties
5.5.4. Implications of Size Effects on Applications
6. Chemical Properties and Biocompatibility
7. Cardiovascular Stents and Their Required Mechanical Properties
8. Application of Metallic Glasses in Self-Expandable Stents
9. Summary
Author Contributions
Conflicts of Interest
References
- Wang, W.-H.; Dong, C.; Shek, C. Bulk metallic glasses. Mater. Sci. Eng. R Rep. 2004, 44, 45–89. [Google Scholar] [CrossRef]
- Groza, J.R.; Shackelford, J.F. Materials Processing Handbook; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Chen, M. A brief overview of bulk metallic glasses. NPG Asia Mater. 2011, 3, 82–90. [Google Scholar] [CrossRef]
- Greer, A.; Ma, E. Bulk metallic glasses: At the cutting edge of metals research. MRS Bull. 2007, 32, 611–619. [Google Scholar] [CrossRef]
- Greer, A.L. Metallic glasses… on the threshold. Mater. Today 2009, 12, 14–22. [Google Scholar] [CrossRef]
- Basu, J.; Ranganathan, S. Bulk metallic glasses: A new class of engineering materials. Sadhana 2003, 28, 783–798. [Google Scholar] [CrossRef]
- Khan, M.M.; Nemati, A.; Rahman, Z.U.; Shah, U.H.; Asgar, H.; Haider, W. Recent advancements in bulk metallic glasses and their applications: A review. Crit. Rev. Solid State Mater. Sci. 2017, 1–36. [Google Scholar] [CrossRef]
- Suryanarayana, C.; Inoue, A. Bulk Metallic Glasses; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Miller, M.K.; Liaw, P. Bulk Metallic Glasses: An Overview. Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Wang, W. Bulk metallic glasses with functional physical properties. Adv. Mater. 2009, 21, 4524–4544. [Google Scholar] [CrossRef]
- Kruzic, J.J. Bulk metallic glasses as structural materials: A review. Adv. Eng. Mater. 2016, 18, 1308–1331. [Google Scholar] [CrossRef]
- Schuh, C.A.; Hufnagel, T.C.; Ramamurty, U. Mechanical behavior of amorphous alloys. Acta Mater. 2007, 55, 4067–4109. [Google Scholar] [CrossRef]
- Chen, M. Mechanical behavior of metallic glasses: Microscopic understanding of strength and ductility. Annu. Rev. Mater. Res. 2008, 38, 445–469. [Google Scholar] [CrossRef]
- Greer, J.R.; De Hosson, J.T.M. Plasticity in small-sized metallic systems: Intrinsic versus extrinsic size effect. Prog. Mater. Sci. 2011, 56, 654–724. [Google Scholar] [CrossRef]
- Greer, A.; Cheng, Y.; Ma, E. Shear bands in metallic glasses. Mater. Sci. Eng. R Rep. 2013, 74, 71–132. [Google Scholar] [CrossRef]
- Takeuchi, S.; Edagawa, K. Atomistic simulation and modeling of localized shear deformation in metallic glasses. Prog. Mater. Sci. 2011, 56, 785–816. [Google Scholar] [CrossRef]
- Egami, T. Understanding the properties and structure of metallic glasses at the atomic level. JOM 2010, 62, 70–75. [Google Scholar] [CrossRef]
- Cheng, Y.; Ma, E. Atomic-level structure and structure–property relationship in metallic glasses. Prog. Mater. Sci. 2011, 56, 379–473. [Google Scholar] [CrossRef]
- Schroers, J.; Kumar, G.; Hodges, T.M.; Chan, S.; Kyriakides, T.R. Bulk metallic glasses for biomedical applications. JOM 2009, 61, 21–29. [Google Scholar] [CrossRef]
- Li, H.; Zheng, Y. Recent advances in bulk metallic glasses for biomedical applications. Acta Biomater. 2016, 36, 1–20. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.; Pu, C.; Fisher, R.K.; Mountain, D.J.; Gao, Y.; Liaw, P.K.; Zhang, W.; He, W. A Zr-based bulk metallic glass for future stent applications: Materials properties, finite element modeling, and in vitro human vascular cell response. Acta Biomater. 2015, 25, 356–368. [Google Scholar] [CrossRef] [PubMed]
- Faupel, F.; Frank, W.; Macht, M.-P.; Mehrer, H.; Naundorf, V.; Rätzke, K.; Schober, H.R.; Sharma, S.K.; Teichler, H. Diffusion in metallic glasses and supercooled melts. Rev. Modern Phys. 2003, 75, 237–280. [Google Scholar] [CrossRef]
- Busch, R. The thermophysical properties of bulk metallic glass-forming liquids. JOM 2000, 52, 39–42. [Google Scholar] [CrossRef]
- Klement, W.; Willens, R.; Duwez, P. Non-crystalline structure in solidified gold–silicon alloys. Nature 1960, 187, 869–870. [Google Scholar] [CrossRef]
- Li, Y.; Poon, S.; Shiflet, G.; Xu, J.; Kim, D.; Löffler, J. Formation of bulk metallic glasses and their composites. MRS Bull. 2007, 32, 624–628. [Google Scholar] [CrossRef]
- Chen, H. Thermodynamic considerations on the formation and stability of metallic glasses. Acta Metall. 1974, 22, 1505–1511. [Google Scholar] [CrossRef]
- Drehman, A.; Greer, A.; Turnbull, D. Bulk formation of a metallic glass: pd40Ni40P20. Appl. Phys. Lett. 1982, 41, 716–717. [Google Scholar] [CrossRef]
- Kui, H.; Greer, A.L.; Turnbull, D. Formation of bulk metallic glass by fluxing. Appl. Phys. Lett. 1984, 45, 615–616. [Google Scholar] [CrossRef]
- Inoue, A.; Zhang, T.; Masumoto, T. Zr–Al–Ni amorphous alloys with high glass transition temperature and significant supercooled liquid region. Mater. Trans. JIM 1990, 31, 177–183. [Google Scholar] [CrossRef]
- Inoue, A.; Zhang, T.; Masumoto, T. Al–La–Ni amorphous alloys with a wide supercooled liquid region. Mater. Trans. JIM 1989, 30, 965–972. [Google Scholar] [CrossRef]
- Inoue, A.; Nakamura, T.; Nishiyama, N.; Masumoto, T. Mg–Cu–Y bulk amorphous alloys with high tensile strength produced by a high-pressure die casting method. Mater. Trans. JIM 1992, 33, 937–945. [Google Scholar] [CrossRef]
- Inoue, A.; Zhang, T.; Nishiyama, N.; Ohba, K.; Masumoto, T. Preparation of 16 mm diameter rod of amorphous Zr65Al7. 5Ni10Cu17. 5 alloy. Mater. Trans. JIM 1993, 34, 1234–1237. [Google Scholar] [CrossRef]
- Inoue, A. Stabilization of metallic supercooled liquid and bulk amorphous alloys. Acta Mater. 2000, 48, 279–306. [Google Scholar] [CrossRef]
- Takeuchi, A.; Inoue, A. Classification of bulk metallic glasses by atomic size difference, heat of mixing and period of constituent elements and its application to characterization of the main alloying element. Mater. Trans. 2005, 46, 2817–2829. [Google Scholar] [CrossRef]
- De Boer, F.R.; Mattens, W.; Boom, R.; Miedema, A.; Niessen, A. Cohesion in Metals; North-Holland Press: Amsterdam, The Netherland, 1988. [Google Scholar]
- Luborsky, F. Amorphous Metallic Alloys; Butterworth and Co.: London, UK, 1983. [Google Scholar]
- Liu, Z.; Chen, W.; Carstensen, J.; Ketkaew, J.; Mota, R.M.O.; Guest, J.K.; Schroers, J. 3D metallic glass cellular structures. Acta Mater. 2016, 105, 35–43. [Google Scholar] [CrossRef]
- Ouyang, D.; Li, N.; Xing, W.; Zhang, J.; Liu, L. 3D printing of crack-free high strength Zr-based bulk metallic glass composite by selective laser melting. Intermetallics 2017, 90, 128–134. [Google Scholar] [CrossRef]
- Shen, Y.; Li, Y.; Chen, C.; Tsai, H.-L. 3D printing of large, complex metallic glass structures. Mater. Des. 2017, 117, 213–222. [Google Scholar] [CrossRef]
- Prashanth, K.G.; Scudino, S.; Chatterjee, R.P.; Salman, O.O.; Eckert, J. Additive manufacturing: Reproducibility of metallic parts. Technologies 2017, 5, 8. [Google Scholar] [CrossRef]
- Lu, Y.; Zhang, H.; Li, H.; Xu, H.; Huang, G.; Qin, Z.; Lu, X. Crystallization prediction on laser three-dimensional printing of Zr-based bulk metallic glass. J. Non-Cryst. Solids 2017, 461, 12–17. [Google Scholar] [CrossRef]
- Vilaro, T.; Kottman-Rexerodt, V.; Thomas, M.; Colin, C.; Bertrand, P.; Thivillon, L.; Abed, S.; Ji, V.; Aubry, P.; Peyre, P. Direct fabrication of a Ti-47Al-2Cr-2Nb alloy by selective laser melting and direct metal deposition processes. Adv. Mater. Res. 2010, 89–91, 586–591. [Google Scholar] [CrossRef]
- Li, X.; Roberts, M.; Liu, Y.; Kang, C.; Huang, H.; Sercombe, T. Effect of substrate temperature on the interface bond between support and substrate during selective laser melting of Al–Ni–Y–Co–La metallic glass. Mater. Des. (1980–2015) 2015, 65, 1–6. [Google Scholar] [CrossRef]
- Kim, J.; Kawamura, Y. Electron beam welding of the dissimilar Zr-based bulk metallic glass and Ti metal. Scr. Mater. 2007, 56, 709–712. [Google Scholar] [CrossRef]
- Jung, H.Y.; Choi, S.J.; Prashanth, K.G.; Stoica, M.; Scudino, S.; Yi, S.; Kühn, U.; Kim, D.H.; Kim, K.B.; Eckert, J. Fabrication of Fe-based bulk metallic glass by selective laser melting: A parameter study. Mater. Des. 2015, 86, 703–708. [Google Scholar] [CrossRef]
- Katakam, S.; Hwang, J.Y.; Paital, S.; Banerjee, R.; Vora, H.; Dahotre, N.B. In situ laser synthesis of Fe-based amorphous matrix composite coating on structural steel. Metall. Mater. Trans. A 2012, 43, 4957–4966. [Google Scholar] [CrossRef]
- Zhang, Y.; Lin, X.; Wei, L.; Liu, F.; Huang, W. Influence of powder size on the crystallization behavior during laser solid forming Zr55Cu30Al10Ni5 bulk amorphous alloy. Intermetallics 2016, 76, 1–9. [Google Scholar] [CrossRef]
- Sun, H.; Flores, K. Laser deposition of a cu-based metallic glass powder on a Zr-based glass substrate. J. Mater. Res. 2008, 23, 2692–2703. [Google Scholar] [CrossRef]
- Williams, E.; Lavery, N. Laser processing of bulk metallic glass: A review. J. Mater. Process. Technol. 2017, 247, 73–91. [Google Scholar] [CrossRef]
- Sun, H.; Flores, K. Microstructural analysis of a laser-processed Zr-based bulk metallic glass. Metall. Mater. Trans. A 2010, 41, 1752–1757. [Google Scholar] [CrossRef]
- Żrodowski, Ł.; Wysocki, B.; Wróblewski, R.; Kurzydłowski, K.J.; Święszkowski, W. The novel scanning strategy for fabrication metallic glasses by selective laser melting. Laser 2016, 20, 20. [Google Scholar]
- Pauly, S.; Löber, L.; Petters, R.; Stoica, M.; Scudino, S.; Kühn, U.; Eckert, J. Processing metallic glasses by selective laser melting. Mater. Today 2013, 16, 37–41. [Google Scholar] [CrossRef]
- Li, X.; Kang, C.; Huang, H.; Sercombe, T. The role of a low-energy–density re-scan in fabricating crack-free Al85Ni5Y6Co2Fe2 bulk metallic glass composites via selective laser melting. Mater. Des. 2014, 63, 407–411. [Google Scholar] [CrossRef]
- Li, X.; Kang, C.; Huang, H.; Zhang, L.; Sercombe, T.B. Selective laser melting of an Al86Ni6Y4.5Co2La1.5 metallic glass: Processing, microstructure evolution and mechanical properties. Mater. Sci. Eng. A 2014, 606, 370–379. [Google Scholar] [CrossRef]
- Li, X.; Roberts, M.; O’Keeffe, S.; Sercombe, T. Selective laser melting of zr-based bulk metallic glasses: Processing, microstructure and mechanical properties. Mater. Des. 2016, 112, 217–226. [Google Scholar] [CrossRef]
- Kawamura, Y.; Ohno, Y. Successful electron-beam welding of bulk metallic glass. Mater. Trans. 2001, 42, 2476–2478. [Google Scholar] [CrossRef]
- Prest, C.D.; Poole, J.C.; Stevick, J.; Waniuk, T.A. Layer-By-Layer Construction with Bulk Metallic Glasses. U.S Patent No 9, 044, 805, 9 May 2017. [Google Scholar]
- Drescher, P.; Seitz, H. Processability of an amorphous metal alloy powder by electron beam melting. Fachforum Rapid Technol. 2015, 2015, 670–673. [Google Scholar]
- Expertsvar. Nique Breakthrough in Bulk Metallic Glass Manufacturing. Available online: www.sciencedaily.com/releases/2012/11/121119114157.htm (accessed on 19 November 2017).
- Park, S.A.; Lee, S.J.; Lim, K.S.; Bae, I.H.; Lee, J.H.; Kim, W.D.; Jeong, M.H.; Park, J.-K. In vivo evaluation and characterization of a bio-absorbable drug-coated stent fabricated using a 3D-printing system. Mater. Lett. 2015, 141, 355–358. [Google Scholar] [CrossRef]
- Van Lith, R.; Baker, E.; Ware, H.; Yang, J.; Farsheed, A.C.; Sun, C.; Ameer, G. 3D-printing strong high-resolution antioxidant bioresorbable vascular stents. Adv. Mater. Technol. 2016, 1, 1600138. [Google Scholar] [CrossRef]
- Vukicevic, M.; Mosadegh, B.; Min, J.K.; Little, S.H. Cardiac 3D printing and its future directions. JACC Cardiovasc. Imaging 2017, 10, 171–184. [Google Scholar] [CrossRef] [PubMed]
- Ware, H.O.T.; Farsheed, A.C.; Baker, E.; Ameer, G.; Sun, C. Fabrication speed optimization for high-resolution 3D-printing of bioresorbable vascular scaffolds. Procedia CIRP 2017, 65, 131–138. [Google Scholar] [CrossRef]
- Chiu, J.-J.; Chien, S. Effects of disturbed flow on vascular endothelium: Pathophysiological basis and clinical perspectives. Physiol. Rev. 2011, 91, 327–387. [Google Scholar] [CrossRef] [PubMed]
- Gurbel, P.A.; Bliden, K.P.; Hiatt, B.L.; O’Connor, C.M. Clopidogrel for coronary stenting response variability, drug resistance, and the effect of pretreatment platelet reactivity. Circulation 2003, 107, 2908–2913. [Google Scholar] [CrossRef] [PubMed]
- Eisenstein, E.L.; Anstrom, K.J.; Kong, D.F.; Shaw, L.K.; Tuttle, R.H.; Mark, D.B.; Kramer, J.M.; Harrington, R.A.; Matchar, D.B.; Kandzari, D.E. Clopidogrel use and long-term clinical outcomes after drug-eluting stent implantation. JAMA 2007, 297, 159–168. [Google Scholar] [CrossRef] [PubMed]
- Taniuchi, M.; Kurz, H.I.; Lasala, J.M. Randomized comparison of ticlopidine and clopidogrel after intracoronary stent implantation in a broad patient population. Circulation 2001, 104, 539–543. [Google Scholar] [CrossRef] [PubMed]
- Gurbel, P.A.; Bliden, K.P.; Zaman, K.A.; Yoho, J.A.; Hayes, K.M.; Tantry, U.S. Clopidogrel loading with eptifibatide to arrest the reactivity of platelets. Circulation 2005, 111, 1153–1159. [Google Scholar] [CrossRef] [PubMed]
- Ma, E.; Zhang, Z. Amorphous alloys: Reflections from the glass maze. Nat. Mater. 2011, 10, 10–11. [Google Scholar] [CrossRef] [PubMed]
- Hirata, A.; Guan, P.; Fujita, T.; Hirotsu, Y.; Inoue, A.; Yavari, A.R.; Sakurai, T.; Chen, M. Direct observation of local atomic order in a metallic glass. Nat. Mater. 2011, 10, 28–33. [Google Scholar] [CrossRef] [PubMed]
- Yue, X.; Inoue, A.; Liu, C.-T.; Fan, C. The development of structure model in metallic glasses. Mater. Res. 2017, 20. [Google Scholar] [CrossRef][Green Version]
- Royall, C.P.; Williams, S.R. The role of local structure in dynamical arrest. Phys. Rep. 2015, 560, 1–75. [Google Scholar] [CrossRef]
- Jafary-Zadeh, M.; Tavakoli, R.; Koh, J.; Aitken, Z.; Zhang, Y.-W. Effect of chemical composition and affinity on the short-and medium-range order structures and mechanical properties of Zr-Ni-Al metallic glass. J. Non-Cryst. Solids 2017, 456, 68–75. [Google Scholar] [CrossRef]
- Frank, F.T.; Kasper, J. Complex alloy structures regarded as sphere packings. I. Definitions and basic principles. Acta Crystallogr. 1958, 11, 184–190. [Google Scholar] [CrossRef]
- Frank, F.T.; Kasper, J. Complex alloy structures regarded as sphere packings. II. Analysis and classification of representative structures. Acta Crystallogr. 1959, 12, 483–499. [Google Scholar] [CrossRef]
- Mattern, N.; Jóvári, P.; Kaban, I.; Gruner, S.; Elsner, A.; Kokotin, V.; Franz, H.; Beuneu, B.; Eckert, J. Short-range order of Cu–Zr metallic glasses. J. Alloys Compd. 2009, 485, 163–169. [Google Scholar] [CrossRef]
- Ding, J.; Cheng, Y.-Q.; Ma, E. Full icosahedra dominate local order in Cu64Zr34 metallic glass and supercooled liquid. Acta Mater. 2014, 69, 343–354. [Google Scholar] [CrossRef]
- Sha, Z.; Pei, Q. Ab initio study on the electronic origin of glass-forming ability in the binary Cu–Zr and the ternary Cu–Zr–Al (Ag) metallic glasses. J. Alloys Compd. 2015, 619, 16–19. [Google Scholar] [CrossRef]
- Sheng, H.; Cheng, Y.; Lee, P.; Shastri, S.; Ma, E. Atomic packing in multicomponent aluminum-based metallic glasses. Acta Mater. 2008, 56, 6264–6272. [Google Scholar] [CrossRef]
- Soklaski, R.; Nussinov, Z.; Markow, Z.; Kelton, K.; Yang, L. Connectivity of icosahedral network and a dramatically growing static length scale in Cu-Zr binary metallic glasses. Phys. Rev. B 2013, 87, 184203. [Google Scholar] [CrossRef]
- Tang, C.; Wong, C. A molecular dynamics simulation study of solid-like and liquid-like networks in Zr 46 Cu 46 Al 8 metallic glass. J. Non-Cryst. Solids 2015, 422, 39–45. [Google Scholar] [CrossRef]
- Gu, X.W.; Jafary-Zadeh, M.; Chen, D.Z.; Wu, Z.; Zhang, Y.-W.; Srolovitz, D.J.; Greer, J.R. Mechanisms of failure in nanoscale metallic glass. Nano Lett. 2014, 14, 5858–5864. [Google Scholar] [CrossRef] [PubMed]
- Jafary-Zadeh, M.; Tavakoli, R.; Srolovitz, D.J.; Zhang, Y.-W. Thermally induced failure mechanism transition and its correlation with short-range order evolution in metallic glasses. Extreme Mech. Lett. 2016, 9, 215–255. [Google Scholar] [CrossRef]
- Liontas, R.; Jafary-Zadeh, M.; Zeng, Q.; Zhang, Y.-W.; Mao, W.L.; Greer, J.R. Substantial tensile ductility in sputtered zr-ni-al nano-sized metallic glass. Acta Mater. 2016, 118, 270–285. [Google Scholar] [CrossRef]
- Stoica, M.; Van Steenberge, N.; Bednarčik, J.; Mattern, N.; Franz, H.; Eckert, J. Changes in short-range order of Zr 55 Cu 30 Al 10 Ni 5 and Zr 55 Cu 20 Al 10 Ni 10 Ti 5 BMGS upon annealing. J. Alloys Compd. 2010, 506, 85–87. [Google Scholar] [CrossRef]
- Xi, X.; Li, L.; Zhang, B.; Wang, W.; Wu, Y. Correlation of atomic cluster symmetry and glass-forming ability of metallic glass. Phys. Rev. Lett. 2007, 99, 095501. [Google Scholar] [CrossRef] [PubMed]
- Shan, Z.; Li, J.; Cheng, Y.; Minor, A.; Asif, S.S.; Warren, O.; Ma, E. Plastic flow and failure resistance of metallic glass: Insight from in situ compression of nanopillars. Phys. Rev. B 2008, 77, 155419. [Google Scholar] [CrossRef]
- Guo, H.; Yan, P.; Wang, Y.; Tan, J.; Zhang, Z.; Sui, M.; Ma, E. Tensile ductility and necking of metallic glass. Nat. Mater. 2007, 6, 735–739. [Google Scholar] [CrossRef] [PubMed]
- Hufnagel, T.; Ott, R.; Almer, J. Structural aspects of elastic deformation of a metallic glass. Phys. Rev. B 2006, 73, 064204. [Google Scholar] [CrossRef]
- Johnson, W.; Samwer, K. A universal criterion for plastic yielding of metallic glasses with a (t/t g) 2/3 temperature dependence. Phys. Rev. Lett. 2005, 95, 195501. [Google Scholar] [CrossRef] [PubMed]
- Ashby, M.; Greer, A. Metallic glasses as structural materials. Scr. Mater. 2006, 54, 321–326. [Google Scholar] [CrossRef]
- Bruck, H.A.; Rosakis, A.J.; Johnson, W.L. The dynamic compressive behavior of beryllium bearing bulk metallic glasses. J. Mater. Res. 1996, 11, 503–511. [Google Scholar] [CrossRef]
- Nishiyama, N.; Amiya, K.; Inoue, A. Novel applications of bulk metallic glass for industrial products. J. Non-Cryst. Solids 2007, 353, 3615–3621. [Google Scholar] [CrossRef]
- Lee, S.-W.; Jafary Zadeh, M.; Chen, D.Z.; Zhang, Y.-W.; Greer, J.R. Size effect suppresses brittle failure in hollow Cu60Zr40 metallic glass nanolattices deformed at cryogenic temperatures. Nano Lett. 2015, 15, 5673–5681. [Google Scholar] [CrossRef] [PubMed]
- Tian, L.; Cheng, Y.-Q.; Shan, Z.-W.; Li, J.; Wang, C.-C.; Han, X.-D.; Sun, J.; Ma, E. Approaching the ideal elastic limit of metallic glasses. Nat. Commun. 2012, 3, 609. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.H. The elastic properties, elastic models and elastic perspectives of metallic glasses. Prog. Mater. Sci. 2012, 57, 487–656. [Google Scholar] [CrossRef]
- Fecht, H. Defect-induced melting and solid-state amorphization. Nature 1992, 356, 133–135. [Google Scholar] [CrossRef]
- Limthongkul, P.; Jang, Y.-I.; Dudney, N.J.; Chiang, Y.-M. Electrochemically-driven solid-state amorphization in lithium-silicon alloys and implications for lithium storage. Acta Mater. 2003, 51, 1103–1113. [Google Scholar] [CrossRef]
- Rehn, L.; Okamoto, P.; Pearson, J.; Bhadra, R.; Grimsditch, M. Solid-state amorphization of zr 3 al: Evidence of an elastic instability and first-order phase transformation. Phys. Rev. Lett. 1987, 59, 2987. [Google Scholar] [CrossRef] [PubMed]
- Chukalkin, Y.G.; Goshchitskii, B. Radiation amorphization of orthoferrite YFEO3. Phys. Status Solidi A 2003, 200, R9–R11. [Google Scholar] [CrossRef]
- Shimizu, F.; Ogata, S.; Li, J. Theory of shear banding in metallic glasses and molecular dynamics calculations. Mater. Trans. 2007, 48, 2923–2927. [Google Scholar] [CrossRef]
- Li, Q.-K.; Li, M. Atomic scale characterization of shear bands in an amorphous metal. Appl. Phys. Lett. 2006, 88, 241903. [Google Scholar] [CrossRef]
- Li, Q.-K.; Li, M. Assessing the critical sizes for shear band formation in metallic glasses from molecular dynamics simulation. Appl. Phys. Lett. 2007, 91, 231905. [Google Scholar] [CrossRef]
- Shi, Y. Size-independent shear band formation in amorphous nanowires made from simulated casting. Appl. Phys. Lett. 2010, 96, 121909. [Google Scholar] [CrossRef]
- Shi, Y.; Falk, M.L. Stress-induced structural transformation and shear banding during simulated nanoindentation of a metallic glass. Acta Mater. 2007, 55, 4317–4324. [Google Scholar] [CrossRef]
- Xiao, Q.; Huang, L.; Shi, Y. Suppression of shear banding in amorphous zrcual nanopillars by irradiation. J. Appl. Phys. 2013, 113, 083514. [Google Scholar] [CrossRef]
- Spaepen, F. A microscopic mechanism for steady state inhomogeneous flow in metallic glasses. Acta Metall. 1977, 25, 407–415. [Google Scholar] [CrossRef]
- Wu, T.-W.; Spaepen, F. The relation between enbrittlement and structural relaxation of an amorphous metal. Philos. Mag. B 1990, 61, 739–750. [Google Scholar] [CrossRef]
- Argon, A. Plastic deformation in metallic glasses. Acta Metall. 1979, 27, 47–58. [Google Scholar] [CrossRef]
- Schuh, C.A.; Lund, A.C. Atomistic basis for the plastic yield criterion of metallic glass. Nat. Mater. 2003, 2, 449–452. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Greer, A. Thickness of shear bands in metallic glasses. Appl. Phys. Lett. 2006, 89, 071907. [Google Scholar] [CrossRef]
- He, Q.; Xu, J. Locating malleable bulk metallic glasses in Zr–Ti–Cu–Al alloys with calorimetric glass transition temperature as an indicator. J. Mater. Sci. Technol. 2012, 28, 1109–1122. [Google Scholar] [CrossRef]
- Schroers, J.; Johnson, W.L. Ductile bulk metallic glass. Phys. Rev. Lett. 2004, 93, 255506. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Dai, L.; Bai, Y.; Wei, B.; Eckert, J. Behavior of multiple shear bands in Zr-based bulk metallic glass. Mater. Chem. Phys. 2005, 93, 174–177. [Google Scholar] [CrossRef]
- Nieh, T.; Wadsworth, J.; Liu, C.; Ice, G. Plasticity in the Supercooled Liquid Region of Bulk Metallic Glasses; Lawrence Livermore National Laboratory (LLNL): Livermore, CA, USA, 2000. [Google Scholar]
- Song, S.; Jang, J.; Huang, J.; Nieh, T. Inhomogeneous to homogeneous transition in an au-based metallic glass and its deformation maps. Intermetallics 2010, 18, 702–709. [Google Scholar] [CrossRef]
- Kumar, G.; Desai, A.; Schroers, J. Bulk metallic glass: The smaller the better. Adv. Mater. 2011, 23, 461–476. [Google Scholar] [CrossRef] [PubMed]
- Jang, D.; Greer, J.R. Transition from a strong-yet-brittle to a stronger-and-ductile state by size reduction of metallic glasses. Nat. Mater. 2010, 9, 215–219. [Google Scholar] [CrossRef] [PubMed]
- Adibi, S.; Branicio, P.S.; Zhang, Y.-W.; Joshi, S.P. Composition and grain size effects on the structural and mechanical properties of cuzr nanoglasses. J. Appl. Phys. 2014, 116, 043522. [Google Scholar] [CrossRef]
- Adibi, S.; Sha, Z.-D.; Branicio, P.S.; Joshi, S.P.; Liu, Z.-S.; Zhang, Y.-W. A transition from localized shear banding to homogeneous superplastic flow in nanoglass. Appl. Phys. Lett. 2013, 103, 211905. [Google Scholar] [CrossRef]
- Ritter, Y.; Şopu, D.; Gleiter, H.; Albe, K. Structure, stability and mechanical properties of internal interfaces in cu 64 zr 36 nanoglasses studied by md simulations. Acta Mater. 2011, 59, 6588–6593. [Google Scholar] [CrossRef]
- Sha, Z.; He, L.; Pei, Q.; Liu, Z.; Zhang, Y.; Wang, T. The mechanical properties of a nanoglass/metallic glass/nanoglass sandwich structure. Scr. Mater. 2014, 83, 37–40. [Google Scholar] [CrossRef]
- Sha, Z.; He, L.; Pei, Q.; Pan, H.; Liu, Z.; Zhang, Y.; Wang, T. On the notch sensitivity of CuZr nanoglass. J. Appl. Phys. 2014, 115, 163507. [Google Scholar] [CrossRef]
- Fang, J.; Vainio, U.; Puff, W.; Wurschum, R.; Wang, X.; Wang, D.; Ghafari, M.; Jiang, F.; Sun, J.; Hahn, H. Atomic structure and structural stability of Sc75fe25 nanoglasses. Nano Lett. 2011, 12, 458–463. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.L.; Jiang, F.; Hahn, H.; Li, J.; Gleiter, H.; Sun, J.; Fang, J.X. Plasticity of a scandium-based nanoglass. Scr. Mater. 2015, 98, 40–43. [Google Scholar] [CrossRef]
- Dowling, N.E. Mechanical Behavior of Materials: Engineering Methods for Deformation, Fracture, and Fatigue; Pearson: London, UK, 2012. [Google Scholar]
- Flores, K.M.; Johnson, W.L.; Dauskardt, R.H. Fracture and fatigue behavior of a Zr–Ti–Nb ductile phase reinforced bulk metallic glass matrix composite. Scr. Mater. 2003, 49, 1181–1187. [Google Scholar] [CrossRef]
- Flores, K.M.; Dauskardt, R.H. Fracture and deformation of bulk metallic glasses and their composites. Intermetallics 2004, 12, 1025–1029. [Google Scholar] [CrossRef]
- Gilbert, C.; Ritchie, R.; Johnson, W. Fracture toughness and fatigue-crack propagation in a Zr–Ti–Ni–Cu–Be bulk metallic glass. Appl. Phys. Lett. 1997, 71, 476–478. [Google Scholar] [CrossRef]
- Kruzic, J. Understanding the problem of fatigue in bulk metallic glasses. Metall. Mater. Trans. A 2011, 42, 1516–1523. [Google Scholar] [CrossRef]
- Lavvafi, H.; Lewandowski, M.E.; Schwam, D.; Lewandowski, J.J. Effects of surface laser treatments on microstructure, tension, and fatigue behavior of aisi 316lvm biomedical wires. Mater. Sci. Eng. A 2017, 688, 101–113. [Google Scholar] [CrossRef]
- Huang, C.-K.; Lewandowski, J.J. Effects of changes in chemistry on flex bending fatigue behavior of al-based amorphous alloy ribbons. Metall. Mater. Trans. A 2012, 43, 2687–2696. [Google Scholar] [CrossRef]
- El-Shabasy, A.B.; Hassan, H.A.; Lewandowski, J.J. Effects of composition changes on strength, bend ductility, toughness, and flex-bending fatigue of iron-based metallic glass ribbons. Metall. Mater. Trans. A 2012, 43, 2697–2705. [Google Scholar] [CrossRef]
- Wang, G.; Liaw, P.; Peker, A.; Freels, M.; Peter, W.; Buchanan, R.; Brooks, C. Comparison of fatigue behavior of a bulk metallic glass and its composite. Intermetallics 2006, 14, 1091–1097. [Google Scholar] [CrossRef]
- Wang, G.; Liaw, P.; Peker, A.; Yang, B.; Benson, M.; Yuan, W.; Peter, W.; Huang, L.; Freels, M.; Buchanan, R. Fatigue behavior of Zr–Ti–Ni–Cu–Be bulk-metallic glasses. Intermetallics 2005, 13, 429–435. [Google Scholar] [CrossRef]
- Wang, G.; Liaw, P.; Peter, W.; Yang, B.; Yokoyama, Y.; Benson, M.; Green, B.; Kirkham, M.; White, S.; Saleh, T. Fatigue behavior of bulk-metallic glasses. Intermetallics 2004, 12, 885–892. [Google Scholar] [CrossRef]
- El-Shabasy, A.B.; Lewandowski, J.J. Fatigue coaxing experiments on a Zr-based bulk-metallic glass. Scr. Mater. 2010, 62, 481–484. [Google Scholar] [CrossRef]
- Sha, Z.; Qu, S.; Liu, Z.; Wang, T.; Gao, H. Cyclic deformation in metallic glasses. Nano Lett. 2015, 15, 7010–7015. [Google Scholar] [CrossRef] [PubMed]
- Sha, Z.; Wong, W.H.; Pei, Q.; Branicio, P.S.; Liu, Z.; Wang, T.; Guo, T.; Gao, H. Atomistic origin of size effects in fatigue behavior of metallic glasses. J. Mech. Phys. Solids 2017, 104, 84–95. [Google Scholar] [CrossRef]
- Ma, E. Tuning order in disorder. Nat. Mater. 2015, 14, 547–552. [Google Scholar] [CrossRef] [PubMed]
- Shimizu, F.; Ogata, S.; Li, J. Yield point of metallic glass. Acta Mater. 2006, 54, 4293–4298. [Google Scholar] [CrossRef]
- Chen, M.; Inoue, A.; Zhang, W.; Sakurai, T. Extraordinary plasticity of ductile bulk metallic glasses. Phys. Rev. Lett. 2006, 96, 245502. [Google Scholar] [CrossRef] [PubMed]
- Packard, C.; Witmer, L.; Schuh, C. Hardening of a metallic glass during cyclic loading in the elastic range. Appl. Phys. Lett. 2008, 92, 171911. [Google Scholar] [CrossRef]
- Ishihara, S.; McEvily, A. A coaxing effect in the small fatigue crack growth regime. Scr. Mater. 1999, 40, 617–622. [Google Scholar] [CrossRef]
- Lerch, B.; Draper, S.; Pereira, J.M. Conducting high-cycle fatigue strength step tests on gamma tial. Metall. Mater. Trans. A 2002, 33, 3871–3874. [Google Scholar] [CrossRef]
- Nicholas, T. Step loading for very high cycle fatigue. Fatigue Fract. Eng. Mater. Struct. 2002, 25, 861–869. [Google Scholar] [CrossRef]
- Xi, L.; Songlin, Z. Strengthening and damaging under low-amplitude loads below the fatigue limit. Int. J. Fatigue 2009, 31, 341–345. [Google Scholar] [CrossRef]
- Murakami, Y.; Tazunoki, Y.; Endo, T. Existence of the coaxing effect and effects of small artificial holes on fatigue strength of an aluminum alloy and 70–30 brass. Metall. Mater. Trans. A 1984, 15, 2029–2038. [Google Scholar] [CrossRef]
- Lewandowski, J.; Wang, W.; Greer, A. Intrinsic plasticity or brittleness of metallic glasses. Philos. Mag. Lett. 2005, 85, 77–87. [Google Scholar] [CrossRef]
- Lewandowski, J.J.; Seifi, M. Metal additive manufacturing: A review of mechanical properties. Ann. Rev. Mater. Res. 2016, 46, 151–186. [Google Scholar] [CrossRef]
- Seifi, M.; Salem, A.; Beuth, J.; Harrysson, O.; Lewandowski, J.J. Overview of materials qualification needs for metal additive manufacturing. JOM 2016, 68, 747–764. [Google Scholar] [CrossRef]
- Seifi, M.; Dahar, M.; Aman, R.; Harrysson, O.; Beuth, J.; Lewandowski, J.J. Evaluation of orientation dependence of fracture toughness and fatigue crack propagation behavior of As-deposited ARCAM EBM Ti-6Al-4V. JOM 2015, 67, 597–607. [Google Scholar] [CrossRef]
- Mower, T.M.; Long, M.J. Mechanical behavior of additive manufactured, powder-bed laser-fused materials. Mater. Sci. Eng. A 2016, 651, 198–213. [Google Scholar] [CrossRef]
- Kumar, G.P.; Cui, F.; Danpinid, A.; Su, B.; Hon, J.K.F.; Leo, H.L. Design and finite element-based fatigue prediction of a new self-expandable percutaneous mitral valve stent. Comput.-Aided Des. 2013, 45, 1153–1158. [Google Scholar] [CrossRef]
- Santos, H.; Auricchio, F.; Conti, M. Numerical Fatigue Life Assessment of Cardiovascular Stents: A Two-Scale Plasticity-Damage Model; IOP Publishing: Bristol, UK, 2013; p. 012031. [Google Scholar]
- Guerchais, R.; Scalet, G.; Constantinescu, A.; Auricchio, F. Micromechanical modeling for the probabilistic failure prediction of stents in high-cycle fatigue. Int. J. Fatigue 2016, 87, 405–417. [Google Scholar] [CrossRef]
- Wang, W. Elastic moduli and behaviors of metallic glasses. J. Non-Cryst. Solids 2005, 351, 1481–1485. [Google Scholar] [CrossRef]
- Liu, Y.H.; Wang, G.; Wang, R.J.; Pan, M.X.; Wang, W.H. Super plastic bulk metallic glasses at room temperature. Science 2007, 315, 1385–1388. [Google Scholar] [CrossRef] [PubMed]
- Yi, J.; Wang, W.H.; Lewandowski, J.J. Sample size and preparation effects on the tensile ductility of pd-based metallic glass nanowires. Acta Mater. 2015, 87, 1–7. [Google Scholar] [CrossRef]
- Yi, J.; Xia, X.X.; Zhao, D.Q.; Pan, M.X.; Bai, H.Y.; Wang, W.H. Micro-and nanoscale metallic glassy fibers. Adv. Eng. Mater. 2010, 12, 1117–1122. [Google Scholar] [CrossRef]
- Lewandowski, J.J.; Shazly, M.; Nouri, A.S. Intrinsic and extrinsic toughening of metallic glasses. Scr. Mater. 2006, 54, 337–341. [Google Scholar] [CrossRef]
- Vormelker, A.H.; Vatamanu, O.; Kecskes, L.; Lewandowski, J. Effects of test temperature and loading conditions on the tensile properties of a Zr-based bulk metallic glass. Metall. Mater. Trans. A 2008, 39, 1922–1934. [Google Scholar] [CrossRef]
- Lewandowski, J.J.; Lowhaphandu, P. Effects of hydrostatic pressure on the flow and fracture of a bulk amorphous metal. Philos. Mag. A 2002, 82, 3427–3441. [Google Scholar] [CrossRef]
- Xing, L.-Q.; Li, Y.; Ramesh, K.; Li, J.; Hufnagel, T. Enhanced plastic strain in Zr-based bulk amorphous alloys. Phys. Rev. B 2001, 64, 180201. [Google Scholar] [CrossRef]
- Das, J.; Tang, M.B.; Kim, K.B.; Theissmann, R.; Baier, F.; Wang, W.H.; Eckert, J. “Work-hardenable” ductile bulk metallic glass. Phys. Rev. Lett. 2005, 94, 205501. [Google Scholar] [CrossRef] [PubMed]
- Deng, Q.; Cheng, Y.; Yue, Y.; Zhang, L.; Zhang, Z.; Han, X.; Ma, E. Uniform tensile elongation in framed submicron metallic glass specimen in the limit of suppressed shear banding. Acta Mater. 2011, 59, 6511–6518. [Google Scholar] [CrossRef]
- Kuzmin, O.; Pei, Y.; Chen, C.; De Hosson, J.T.M. Intrinsic and extrinsic size effects in the deformation of metallic glass nanopillars. Acta Mater. 2012, 60, 889–898. [Google Scholar] [CrossRef]
- Volkert, C.; Donohue, A.; Spaepen, F. Effect of sample size on deformation in amorphous metals. J. Appl. Phys. 2008, 103, 083539. [Google Scholar] [CrossRef]
- Ghidelli, M.; Idrissi, H.; Gravier, S.; Blandin, J.-J.; Raskin, J.-P.; Schryvers, D.; Pardoen, T. Homogeneous flow and size dependent mechanical behavior in highly ductile Zr65Ni35 metallic glass films. Acta Mater. 2017, 131, 246–259. [Google Scholar] [CrossRef]
- Ghidelli, M.; Gravier, S.; Blandin, J.-J.; Djemia, P.; Mompiou, F.; Abadias, G.; Raskin, J.-P.; Pardoen, T. Extrinsic mechanical size effects in thin ZrNi metallic glass films. Acta Mater. 2015, 90, 232–241. [Google Scholar] [CrossRef]
- Ghidelli, M.; Gravier, S.; Blandin, J.-J.; Raskin, J.-P.; Lani, F.; Pardoen, T. Size-dependent failure mechanisms in ZrNi thin metallic glass films. Scr. Mater. 2014, 89, 9–12. [Google Scholar] [CrossRef]
- Adibi, S.; Branicio, P.S.; Liontas, R.; Chen, D.Z.; Greer, J.R.; Srolovitz, D.J.; Joshi, S.P. Surface roughness imparts tensile ductility to nanoscale metallic glasses. Extreme Mech. Lett. 2015, 5, 88–95. [Google Scholar] [CrossRef]
- Qu, R.; Calin, M.; Eckert, J.; Zhang, Z. Metallic glasses: Notch-insensitive materials. Scr. Mater. 2012, 66, 733–736. [Google Scholar] [CrossRef]
- Sha, Z.-D.; Pei, Q.-X.; Sorkin, V.; Branicio, P.S.; Zhang, Y.-W.; Gao, H. On the notch sensitivity of CuZr metallic glasses. Appl. Phys. Lett. 2013, 103, 081903. [Google Scholar] [CrossRef]
- Magagnosc, D.; Ehrbar, R.; Kumar, G.; He, M.; Schroers, J.; Gianola, D. Tunable tensile ductility in metallic glasses. Sci. Rep. 2013, 3, 1096. [Google Scholar] [CrossRef]
- Magagnosc, D.; Kumar, G.; Schroers, J.; Felfer, P.; Cairney, J.; Gianola, D. Effect of ion irradiation on tensile ductility, strength and fictive temperature in metallic glass nanowires. Acta Mater. 2014, 74, 165–182. [Google Scholar] [CrossRef]
- Chen, D.; Jang, D.; Guan, K.; An, Q.; Goddard, W., III; Greer, J. Nanometallic glasses: Size reduction brings ductility, surface state drives its extent. Nano Lett. 2013, 13, 4462–4468. [Google Scholar] [CrossRef] [PubMed]
- Murali, P.; Guo, T.F.; Zhang, Y.W.; Narasimhan, R.; Li, Y.; Gao, H.J. Atomic scale fluctuations govern brittle fracture and cavitation behavior in metallic glasses. Phys. Rev. Lett. 2011, 107, 215501. [Google Scholar] [CrossRef] [PubMed]
- Xi, X.; Zhao, D.; Pan, M.; Wang, W.; Wu, Y.; Lewandowski, J. Fracture of brittle metallic glasses: Brittleness or plasticity. Phys. Rev. Lett. 2005, 94, 125510. [Google Scholar] [CrossRef] [PubMed]
- Murali, P.; Zhang, Y.; Gao, H. On the characteristic length scales associated with plastic deformation in metallic glasses. Appl. Phys. Lett. 2012, 100, 201901. [Google Scholar] [CrossRef]
- Han, Z.; Wu, W.; Li, Y.; Wei, Y.; Gao, H. An instability index of shear band for plasticity in metallic glasses. Acta Mater. 2009, 57, 1367–1372. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, F.; Li, Y.; Chen, M. Deformation behavior of metallic glass thin films. J. Appl. Phys. 2012, 112, 063504. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, Q.-K.; Li, M. Processing dependence of mechanical properties of metallic glass nanowires. Appl. Phys. Lett. 2015, 106, 071905. [Google Scholar] [CrossRef]
- Hassan, H.A.; Kecskes, L.; Lewandowski, J. Effects of changes in test temperature and loading conditions on fracture toughness of a Zr-based bulk metallic glass. Metall. Mater. Trans. A 2008, 39, 2077–2085. [Google Scholar] [CrossRef]
- Lai, Y.; Lee, C.; Cheng, Y.; Chou, H.; Chen, H.; Du, X.; Chang, C.; Huang, J.C.; Jian, S.; Jang, J. Bulk and microscale compressive behavior of a Zr-based metallic glass. Scr. Mater. 2008, 58, 890–893. [Google Scholar] [CrossRef]
- Jang, D.; Maaß, R.; Wang, G.; Liaw, P.K.; Greer, J.R. Fatigue deformation of microsized metallic glasses. Scr. Mater. 2013, 68, 773–776. [Google Scholar] [CrossRef]
- Meagher, P.; O’Cearbhaill, E.D.; Byrne, J.H.; Browne, D.J. Bulk metallic glasses for implantable medical devices and surgical tools. Adv. Mater. 2016, 28, 5755–5762. [Google Scholar] [CrossRef] [PubMed]
- Raja, V.; Ranganathan, S. Effect of molybdenum and silicon on the electrochemical corrosion behavior of fenib metallic glasses. Corrosion 1988, 44, 263–270. [Google Scholar] [CrossRef]
- Jablonski, S.; Pantaleo, A.; Bauen, A.; Pearson, P.; Panoutsou, C.; Slade, R. The potential demand for bioenergy in residential heating applications (bio-heat) in the UK based on a market segment analysis. Biomass Bioenergy 2008, 32, 635–653. [Google Scholar] [CrossRef]
- Hua, N.; Chen, W.; Wang, Q.; Guo, Q.; Huang, Y.; Zhang, T. Tribocorrosion behaviors of a biodegradable mg 65 zn 30 ca 5 bulk metallic glass for potential biomedical implant applications. J. Alloys Compd. 2018, 745, 111–120. [Google Scholar] [CrossRef]
- Gu, X.-N.; Zheng, Y.-F. A review on magnesium alloys as biodegradable materials. Front. Mater. Sci. China 2010, 4, 111–115. [Google Scholar] [CrossRef]
- Sun, Y.; Huang, Y.; Fan, H.; Wang, Y.; Ning, Z.; Liu, F.; Feng, D.; Jin, X.; Shen, J.; Sun, J. In vitro and in vivo biocompatibility of an Ag-bearing Zr-based bulk metallic glass for potential medical use. J. Non-Cryst. Solids 2015, 419, 82–91. [Google Scholar] [CrossRef]
- Hua, N.; Huang, L.; He, W.; Pang, S.; Zhang, T. A Ni-free high-zirconium-based bulk metallic glass with enhanced plasticity and biocompatibility. J. Non-Cryst. Solids 2013, 376, 133–138. [Google Scholar] [CrossRef]
- Dambatta, M.; Izman, S.; Yahaya, B.; Lim, J.; Kurniawan, D. Mg-based bulk metallic glasses for biodegradable implant materials: A review on glass forming ability, mechanical properties, and biocompatibility. J. Non-Cryst. Solids 2015, 426, 110–115. [Google Scholar] [CrossRef]
- Wang, R.; Wang, Y.; Yang, J.; Sun, J.; Xiong, L. Influence of heat treatment on the mechanical properties, corrosion behavior, and biocompatibility of Zr56Al16Co28 bulk metallic glass. J. Non-Cryst. Solids 2015, 411, 45–52. [Google Scholar] [CrossRef]
- Nowosielski, R.; Bajorek, A.; Babilas, R. Corrosion behavior of bioresorbable Ca-Mg-Zn bulk metallic glasses. J. Non-Cryst. Solids 2016, 447, 126–133. [Google Scholar] [CrossRef]
- Shanahan, C.; Tofail, S.A.; Tiernan, P. Viscoelastic braided stent: Finite element modelling and validation of crimping behaviour. Mater. Des. 2017, 121, 143–153. [Google Scholar] [CrossRef]
- Rebelo, R.; Vila, N.; Fangueiro, R.; Carvalho, S.; Rana, S. Influence of design parameters on the mechanical behavior and porosity of braided fibrous stents. Mater. Des. 2015, 86, 237–247. [Google Scholar] [CrossRef]
- Azaouzi, M.; Lebaal, N.; Makradi, A.; Belouettar, S. Optimization based simulation of self-expanding nitinol stent. Mater. Des. 2013, 50, 917–928. [Google Scholar] [CrossRef]
- Azaouzi, M.; Makradi, A.; Belouettar, S. Deployment of a self-expanding stent inside an artery: A finite element analysis. Mater. Des. 2012, 41, 410–420. [Google Scholar] [CrossRef]
- Hsiao, H.-M.; Chiu, Y.-H.; Lee, K.-H.; Lin, C.-H. Computational modeling of effects of intravascular stent design on key mechanical and hemodynamic behavior. Comput.-Aided Des. 2012, 44, 757–765. [Google Scholar] [CrossRef]
- Brand, M.; Avrahami, I.; Einav, S.; Ryvkin, M. Numerical models of net-structure stents inserted into arteries. Comput. Biol. Med. 2014, 52, 102–110. [Google Scholar] [CrossRef] [PubMed]
- Jeong, W.; Han, M.H.; Rhee, K. The hemodynamic alterations induced by the vascular angular deformation in stent-assisted coiling of bifurcation aneurysms. Comput. Biol. Med. 2014, 53, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Fung, G.S.; Lam, S.; Cheng, S.W.; Chow, K. On stent-graft models in thoracic aortic endovascular repair: A computational investigation of the hemodynamic factors. Comput. Biol. Med. 2008, 38, 484–489. [Google Scholar] [CrossRef] [PubMed]
- Hajiali, Z.; Dabagh, M.; Debusschere, N.; De Beule, M.; Jalali, P. Tissue prolapse and stresses in stented coronary arteries: A computer model for multi-layer atherosclerotic plaque. Comput. Biol. Med. 2015, 66, 39–46. [Google Scholar] [CrossRef] [PubMed]
- Ovcharenko, E.; Klyshnikov, K.; Yuzhalin, A.; Savrasov, G.; Kokov, A.; Batranin, A.V.; Ganyukov, V.; Kudryavtseva, Y. Modeling of transcatheter aortic valve replacement: Patient specific vs general approaches based on finite element analysis. Comput. Biol. Med. 2016, 69, 29–36. [Google Scholar] [CrossRef] [PubMed]
- Kumar, G.P.; Cui, F.; Phang, H.Q.; Su, B.; Leo, H.L.; Hon, J.K.F. Design considerations and quantitative assessment for the development of percutaneous mitral valve stent. Med. Eng. Phys. 2014, 36, 882–888. [Google Scholar] [CrossRef] [PubMed]
- Kumar, G.P.; Mathew, L. Self-expanding aortic valve stent—material optimization. Comput. Biol. Med. 2012, 42, 1060–1063. [Google Scholar] [CrossRef] [PubMed]
- Kumar, G.V.P.; Mathew, L. New stent design for percutaneous aortic valve replacement. Cardiovasc. Revasc. Med. 2009, 10, 121–124. [Google Scholar] [CrossRef] [PubMed]
- Ismail, M.; Kumar, G.P.; Kabinejadian, F.; Nguyen, Y.N.; Cui, F.; Tay, E.L.W.; Leo, H.L. An experimental and computational study on the effect of caval valved stent oversizing. Cardiovasc. Eng. Technol. 2016, 7, 254–269. [Google Scholar] [CrossRef] [PubMed]
- Kumar, G.P.; Kabinejadian, F.; Liu, J.; Ho, P.; Leo, H.L.; Cui, F. Simulated bench testing to evaluate the mechanical performance of new carotid stents. Artif. Organs 2017, 41, 267–272. [Google Scholar] [CrossRef] [PubMed]
- Duerig, T.; Wholey, M. A comparison of balloon-and self-expanding stents. Minim. Invasive Ther. Allied Technol. 2002, 11, 173–178. [Google Scholar] [CrossRef] [PubMed]
- Cui, F.; Lee, H.; Lu, C.; Chai, P. Effects of balloon length and compliance on vascular stent expansion. Int. J. Appl. Mech. 2010, 2, 681–697. [Google Scholar] [CrossRef]
- Migliavacca, F.; Petrini, L.; Montanari, V.; Quagliana, I.; Auricchio, F.; Dubini, G. A predictive study of the mechanical behaviour of coronary stents by computer modelling. Med. Eng. Phys. 2005, 27, 13–18. [Google Scholar] [CrossRef] [PubMed]
- Etave, F.; Finet, G.; Boivin, M.; Boyer, J.-C.; Rioufol, G.; Thollet, G. Mechanical properties of coronary stents determined by using finite element analysis. J. Biomech. 2001, 34, 1065–1075. [Google Scholar] [CrossRef]
- Dumoulin, C.; Cochelin, B. Mechanical behaviour modelling of balloon-expandable stents. J. Biomech. 2000, 33, 1461–1470. [Google Scholar] [CrossRef]
- Lally, C.; Dolan, F.; Prendergast, P. Cardiovascular stent design and vessel stresses: A finite element analysis. J. Biomech. 2005, 38, 1574–1581. [Google Scholar] [CrossRef] [PubMed]
- Migliavacca, F.; Petrini, L.; Massarotti, P.; Schievano, S.; Auricchio, F.; Dubini, G. Stainless and shape memory alloy coronary stents: A computational study on the interaction with the vascular wall. Biomech. Model. Mechanobiol. 2004, 2, 205–217. [Google Scholar] [CrossRef] [PubMed]
- Hanawa, T. Materials for metallic stents. J. Artif. Organs 2009, 12, 73–79. [Google Scholar] [CrossRef] [PubMed]
- Niinomi, M. Recent metallic materials for biomedical applications. Metall. Mater. Trans. A 2002, 33, 477–486. [Google Scholar] [CrossRef]
- Thomann, U.I.; Uggowitzer, P.J. Wear–corrosion behavior of biocompatible austenitic stainless steels. Wear 2000, 239, 48–58. [Google Scholar] [CrossRef]
- Disegi, J.; Eschbach, L. Stainless steel in bone surgery. Injury 2000, 31, D2–D6. [Google Scholar] [CrossRef]
- Winters, G.L.; Nutt, M.J. Stainless Steels for Medical and Surgical Applications; Astm International Pittsburgh: West Conshohocken, PA, USA, 2003; Volume 1438. [Google Scholar]
- Yang, K.; Ren, Y. Nickel-free austenitic stainless steels for medical applications. Sci. Technol. Adv. Mater. 2010, 11, 014105. [Google Scholar] [CrossRef] [PubMed]
- Kounis, N.G.; Soufras, G.D. Coronary stent thrombosis: Beware of an allergic reaction and of kounis syndrome. Indian Heart J. 2014, 66, 153–155. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Morton, A.C.; Crossman, D.; Gunn, J. The influence of physical stent parameters upon restenosis. Pathol. Biol. 2004, 52, 196–205. [Google Scholar] [CrossRef] [PubMed]
- Köster, R.; Vieluf, D.; Kiehn, M.; Sommerauer, M.; Kähler, J.; Baldus, S.; Meinertz, T.; Hamm, C.W. Nickel and molybdenum contact allergies in patients with coronary in-stent restenosis. Lancet 2000, 356, 1895–1897. [Google Scholar] [CrossRef]
- Duerig, T.; Tolomeo, D.; Wholey, M. An overview of superelastic stent design. Minim. Invasive Ther. Allied Technol. 2000, 9, 235–246. [Google Scholar] [CrossRef] [PubMed]
- Kumar, G.P.; Cui, F. Stent design parameters and crimpability. Int. J. Cardiol. 2016, 223, 552–553. [Google Scholar] [CrossRef] [PubMed]
- Rapp, B. Nitinol for stents. Mater. Today 2004, 7, 13. [Google Scholar] [CrossRef]
- Dordoni, E.; Petrini, L.; Wu, W.; Migliavacca, F.; Dubini, G.; Pennati, G. Computational modeling to predict fatigue behavior of niti stents: What do we need? J. Funct. Biomater. 2015, 6, 299–317. [Google Scholar] [CrossRef] [PubMed]
- Ferraro, M.; Auricchio, F.; Boatti, E.; Scalet, G.; Conti, M.; Morganti, S.; Reali, A. An efficient finite element framework to assess flexibility performances of sma self-expandable carotid artery stents. J. Funct. Biomater. 2015, 6, 585–597. [Google Scholar] [CrossRef] [PubMed]
- Lörtscher, E. Wiring molecules into circuits. Nat. Nanotechnol. 2013, 8, 381–384. [Google Scholar] [CrossRef] [PubMed]
- Shayan, M.; Chen, Y.; Shridhar, P.; Kealey, C.P.; Chun, Y. In vitro study of a superhydrophilic thin film nitinol endograft that is electrostatically endothelialized in the catheter prior to the endovascular procedure. J. Funct. Biomater. 2016, 7, 31. [Google Scholar] [CrossRef] [PubMed]
- Kleinstreuer, C.; Li, Z.; Basciano, C.; Seelecke, S.; Farber, M.A. Computational mechanics of nitinol stent grafts. J. Biomech. 2008, 41, 2370–2378. [Google Scholar] [CrossRef] [PubMed]
- Robertson, S.; Pelton, A.; Ritchie, R. Mechanical fatigue and fracture of nitinol. Int. Mater. Rev. 2012, 57, 1–37. [Google Scholar] [CrossRef]
- Stoeckel, D.; Pelton, A.; Duerig, T. Self-expanding nitinol stents: Material and design considerations. Eur. Radiol. 2004, 14, 292–301. [Google Scholar] [CrossRef] [PubMed]
- Pelton, A. Nitinol fatigue: A review of microstructures and mechanisms. J. Mater. Eng. Perform. 2011, 20, 613–617. [Google Scholar] [CrossRef]
- Wu, M.H. Fabrication of nitinol materials and components. Mater. Sci. Forum 2002, 394–395, 285–292. [Google Scholar] [CrossRef]
- Duerig, T.; Pelton, A.; Stöckel, D. An overview of nitinol medical applications. Mater. Sci. Eng. A 1999, 273, 149–160. [Google Scholar] [CrossRef]
- Poncet, P.P. Nitinol medical device design considerations. Strain 2000, 2, 6. [Google Scholar]
- Elahinia, M.H.; Hashemi, M.; Tabesh, M.; Bhaduri, S.B. Manufacturing and processing of niti implants: A review. Prog. Mater Sci. 2012, 57, 911–946. [Google Scholar] [CrossRef]
- Smith, S.; Hodgson, E. Shape setting nitinol. In Proceedings of the Materials and Processes for Medical Devices Conference, Anaheim, CA, USA, 25–27 August 2004; pp. 266–270. [Google Scholar]
- Fuentes, J.G.; Gumpel, P.; Strittmatter, J. Phase change behavior of nitinol shape memory alloys. Adv. Eng. Mater. 2002, 4, 437–452. [Google Scholar] [CrossRef]
- Ye, J.; Mishra, R.K.; Pelton, A.R.; Minor, A.M. Direct observation of the niti martensitic phase transformation in nanoscale volumes. Acta Mater. 2010, 58, 490–498. [Google Scholar] [CrossRef]
- Gbur, J.L.; Lewandowski, J.J. Fatigue and fracture of wires and cables for biomedical applications. Int. Mater. Rev. 2016, 61, 231–314. [Google Scholar] [CrossRef]
- Praveen Kumar, G.; Jafary-Zadeh, M.; Tavakoli, R.; Cui, F. Feasibility of using bulk metallic glass for self-expandable stent applications. J. Biomed. Mater. Res. B Appl. Biomater. 2016, 105, 1874–1882. [Google Scholar] [CrossRef] [PubMed]
- Demanget, N.; Avril, S.; Badel, P.; Orgéas, L.; Geindreau, C.; Albertini, J.-N.; Favre, J.-P. Computational comparison of the bending behavior of aortic stent-grafts. J. Mech. Behav. Biomed. Mater. 2012, 5, 272–282. [Google Scholar] [CrossRef] [PubMed]
- Dean, M.R. Interventional Radiology—A Practical Guide; Radcliffe Medical Press: Oxford, NY, USA, 1996; Volume 19, p. 449. [Google Scholar]
- Pande, A.K.; Meier, B.; Urban, P.; Verin, V.; Moles, V.P.; Chappuis, F.; Mehan, V.K. Coronary angiography with four french catheters. Am. J. Cardiol. 1992, 70, 1085–1086. [Google Scholar] [CrossRef]
- Lawrence, E.; Turner, I. Materials for urinary catheters: A review of their history and development in the UK. Med. Eng. Phys. 2005, 27, 443–453. [Google Scholar] [CrossRef] [PubMed]
- Praveen Kumar, G.; Jafary-Zadeh, M.; Cui, F. Deployment of a bulk metallic glass-based self-expandable stent in a patient-specific descending aorta. ACS Biomater. Sci. Eng. 2016, 2, 1951–1958. [Google Scholar] [CrossRef]
- Zhao, H.Q.; Nikanorov, A.; Virmani, R.; Jones, R.; Pacheco, E.; Schwartz, L.B. Late stent expansion and neointimal proliferation of oversized nitinol stents in peripheral arteries. Cardiovasc. Intervent. Radiol. 2009, 32, 720–726. [Google Scholar] [CrossRef] [PubMed]


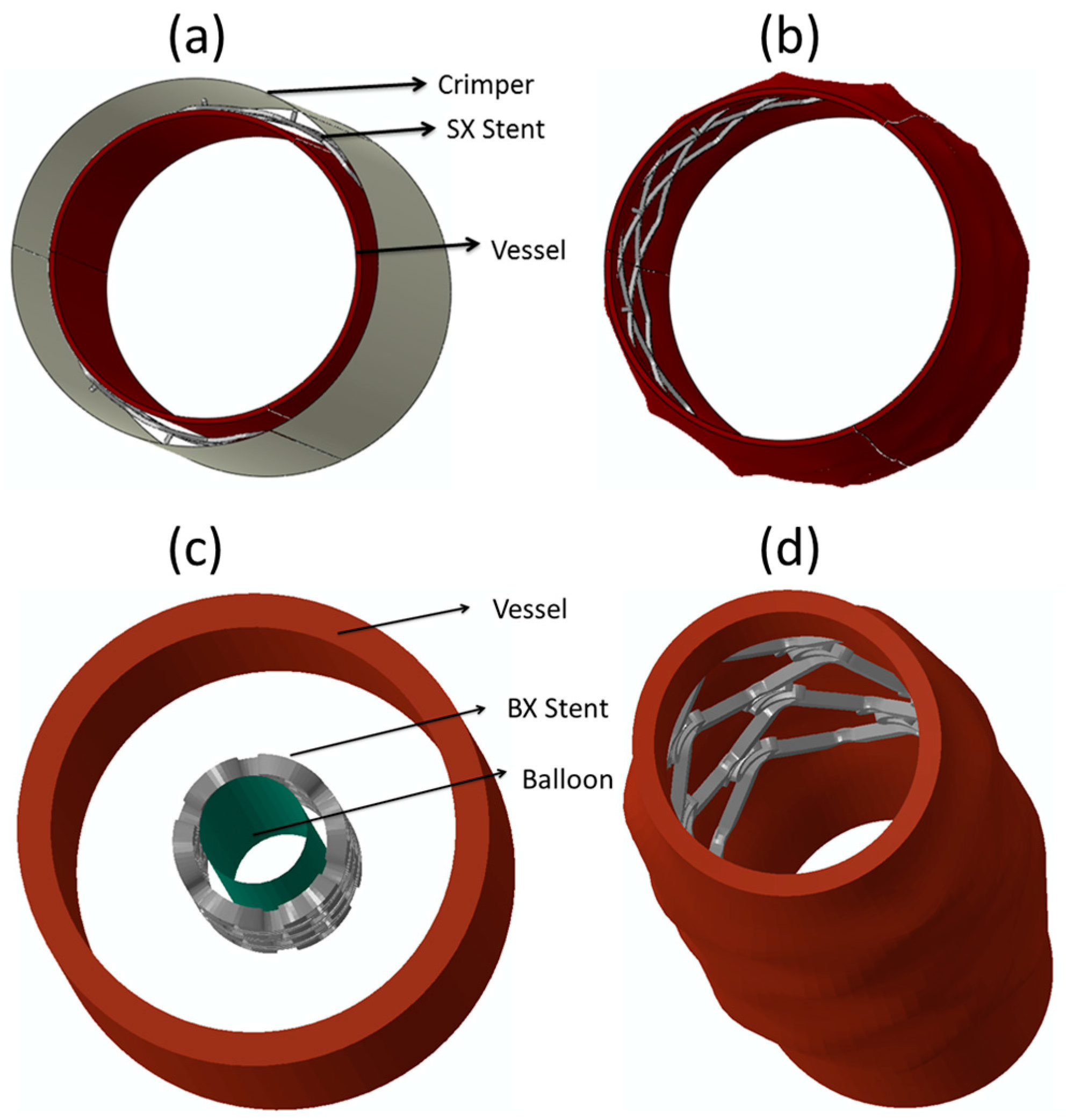
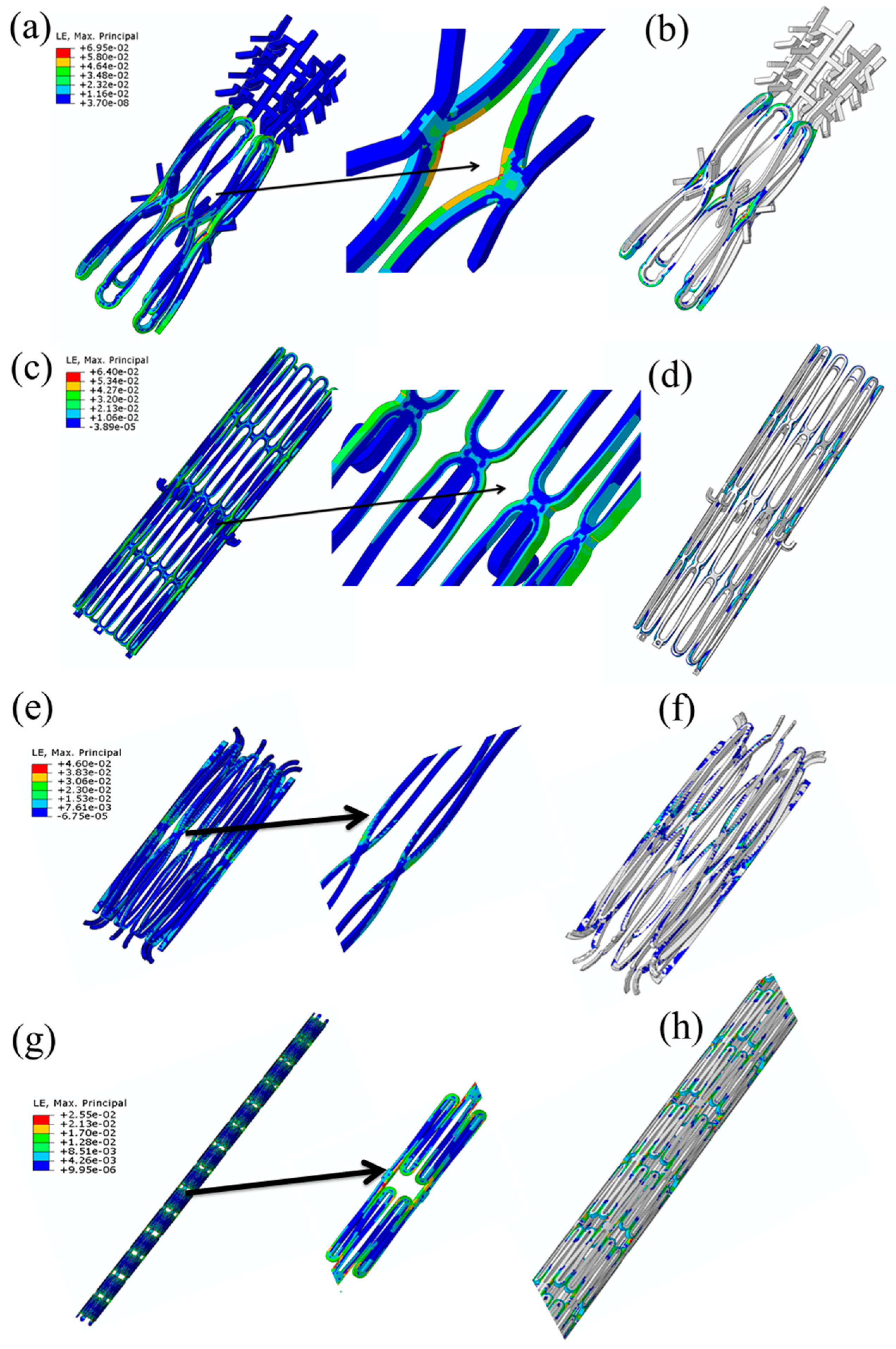
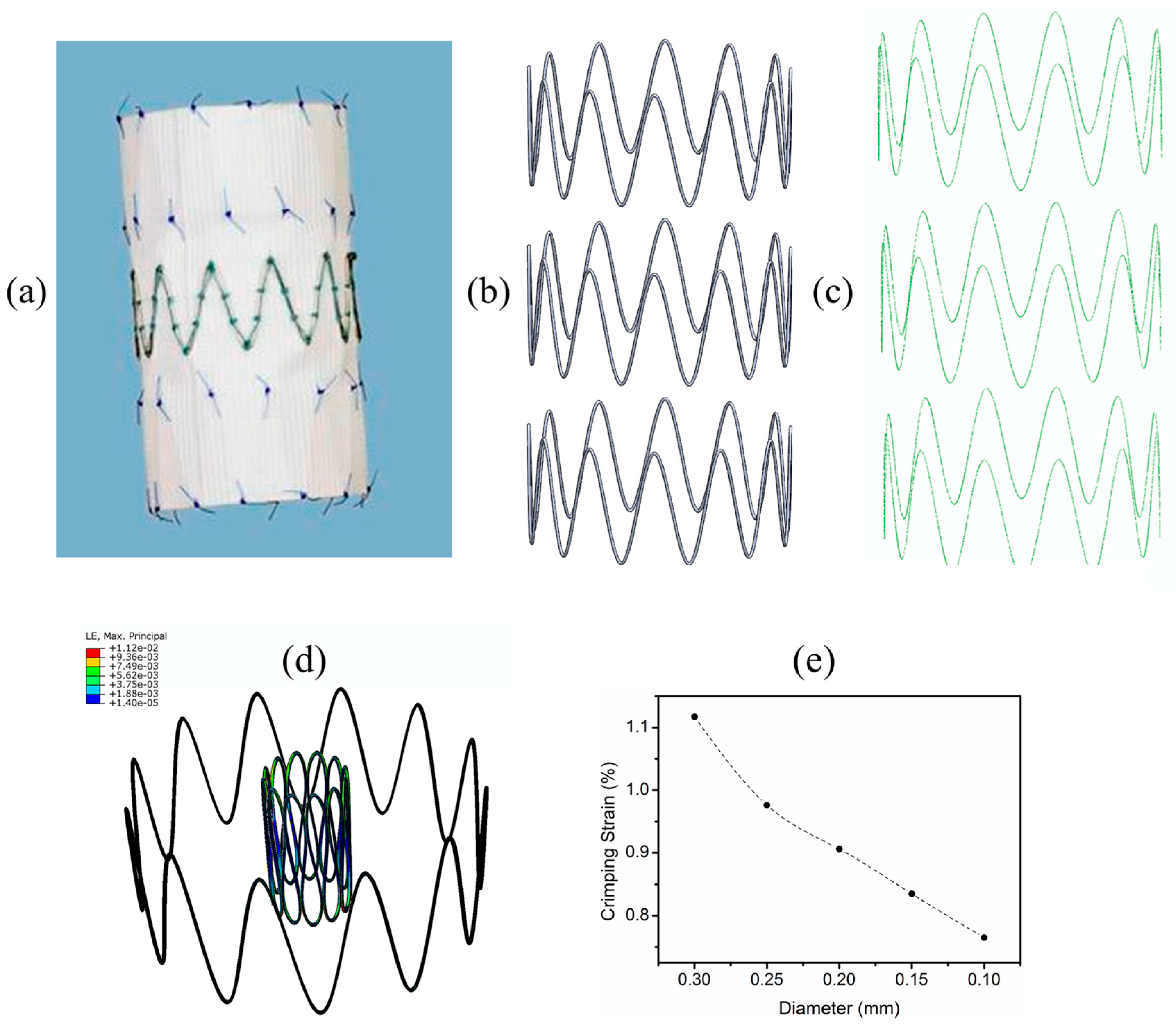

| Self-Expanding Stent | Balloon-Expanded Stent |
|---|---|
| Manufactured in expanded state | Manufactured in crimped state |
| Self-expansion due to stored elastic energy | Expansion using balloon inflation pressure |
| Expansion cannot be manually controlled | Expansion is a controlled process |
| No plastic deformation | Permanent plastic deformation |
| Used in bigger arteries and as valve replacement devices | Typically used smaller vessels like coronary arteries |
| Nitinol, shape-memory polymers, etc. | Stainless steel, Co-Cr, Platinum alloys, etc. |
| Nitinol | BMG |
|---|---|
| Temperature induced phase transformation | No phase transformation |
| Super elastic | Purely linear elastic |
| Crimping should be done only at a low temperature | Crimping can be done at any temperature |
| Temperature controlled recoil | Spring back-like recoil |
| Strut thickness based on application | Thinner struts giving superior strength |
| Difficulty in crimping with thicker struts | Thinner struts aid in better crimpability |
| Thicker struts may lead to restenosis | Thinner struts reduce rate of restenosis |
| Associated problems with phase transformation | No phase transformation related problems |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jafary-Zadeh, M.; Praveen Kumar, G.; Branicio, P.S.; Seifi, M.; Lewandowski, J.J.; Cui, F. A Critical Review on Metallic Glasses as Structural Materials for Cardiovascular Stent Applications. J. Funct. Biomater. 2018, 9, 19. https://doi.org/10.3390/jfb9010019
Jafary-Zadeh M, Praveen Kumar G, Branicio PS, Seifi M, Lewandowski JJ, Cui F. A Critical Review on Metallic Glasses as Structural Materials for Cardiovascular Stent Applications. Journal of Functional Biomaterials. 2018; 9(1):19. https://doi.org/10.3390/jfb9010019
Chicago/Turabian StyleJafary-Zadeh, Mehdi, Gideon Praveen Kumar, Paulo Sergio Branicio, Mohsen Seifi, John J. Lewandowski, and Fangsen Cui. 2018. "A Critical Review on Metallic Glasses as Structural Materials for Cardiovascular Stent Applications" Journal of Functional Biomaterials 9, no. 1: 19. https://doi.org/10.3390/jfb9010019
APA StyleJafary-Zadeh, M., Praveen Kumar, G., Branicio, P. S., Seifi, M., Lewandowski, J. J., & Cui, F. (2018). A Critical Review on Metallic Glasses as Structural Materials for Cardiovascular Stent Applications. Journal of Functional Biomaterials, 9(1), 19. https://doi.org/10.3390/jfb9010019






