Finite-Element Modeling of Viscoelastic Cells During High-Frequency Cyclic Strain
Abstract
:1. Introduction
2. Experimental Section
2.1. Generation of Cell Model Geometry
2.2. Assignment of Material Properties
2.3. Effect of Viscoelasticity
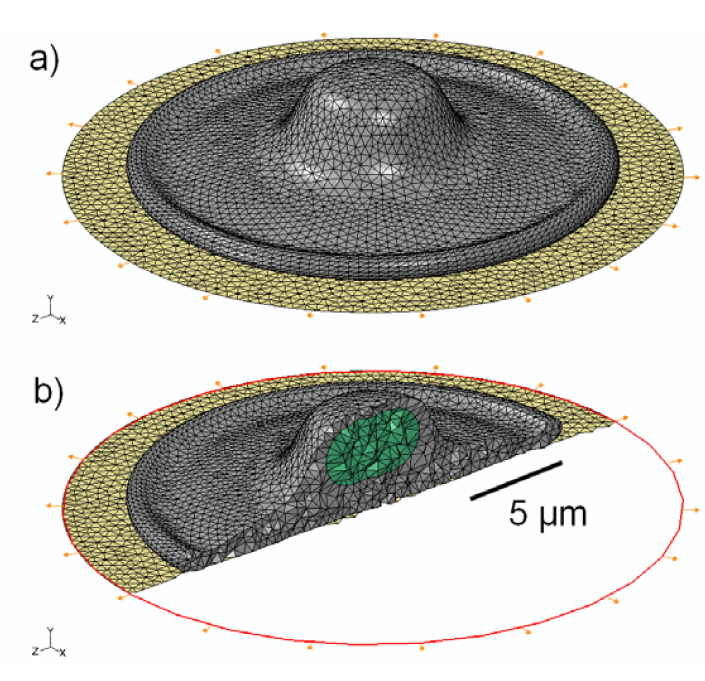
2.4. Effect of Cytoskeleton
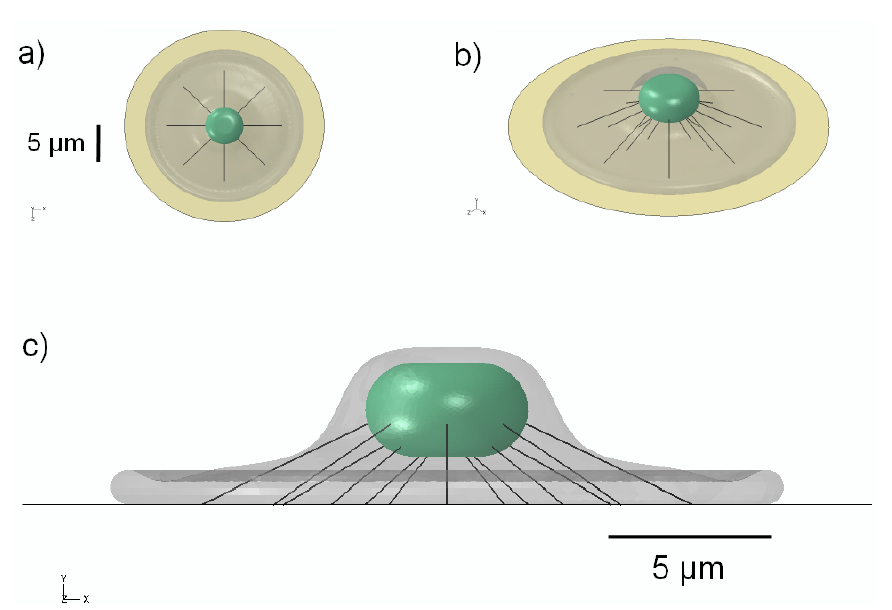
2.5. Finite-Element Modeling
3. Results and Discussion
3.1. 3D Model Geometry
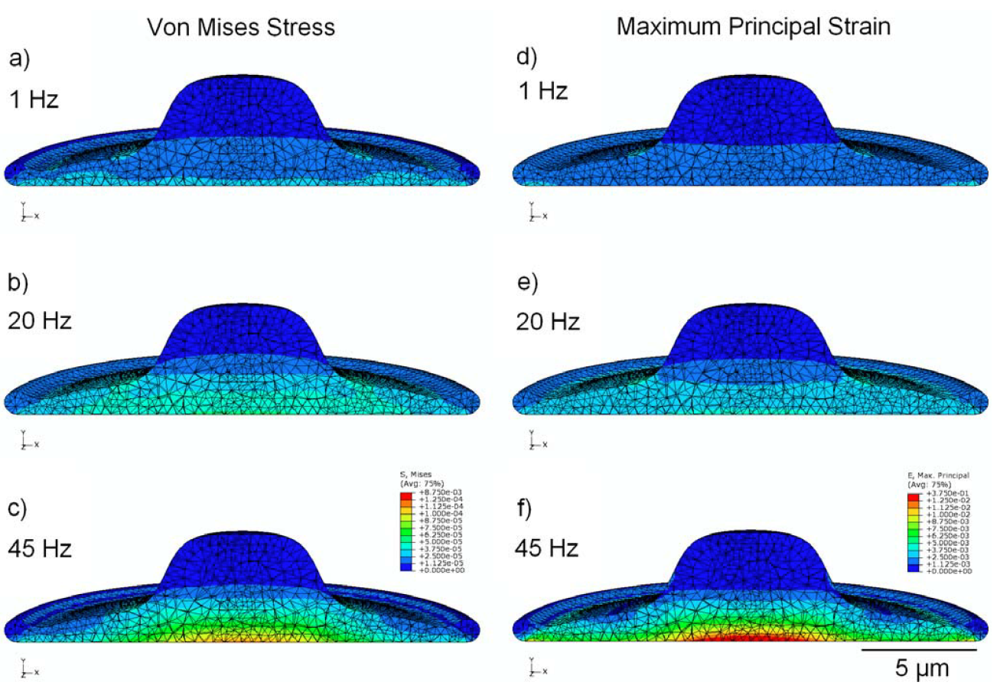
3.2. Von Mises Stress Distribution
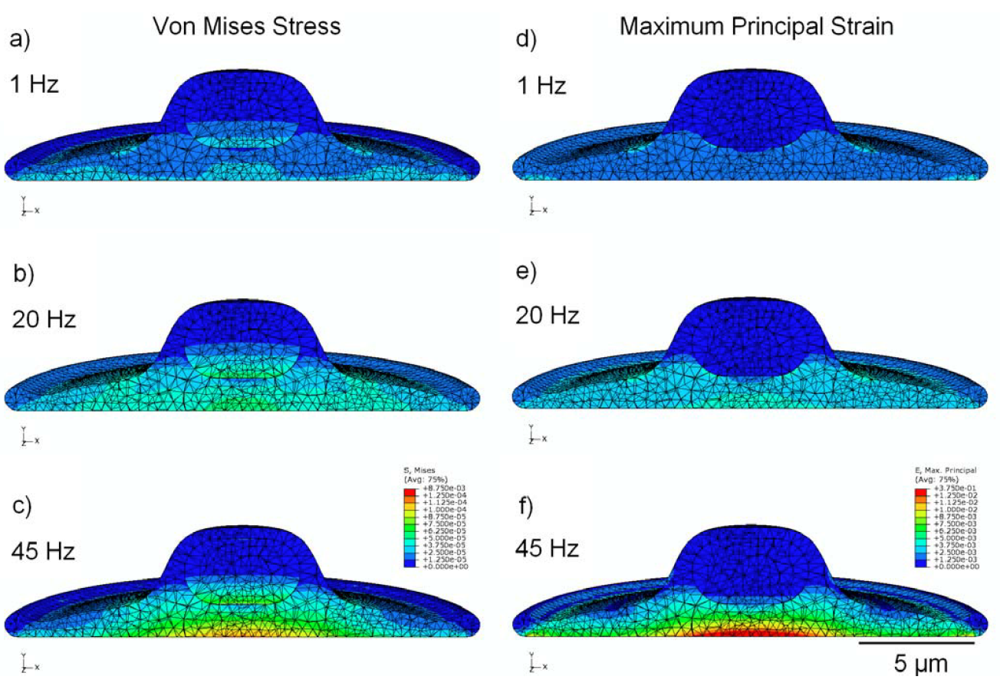
3.3. Strain Distribution
3.4. Effect of Viscoelasticity
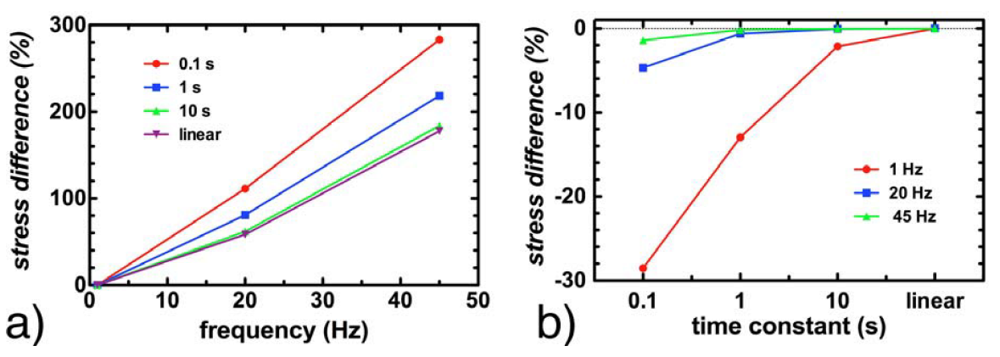
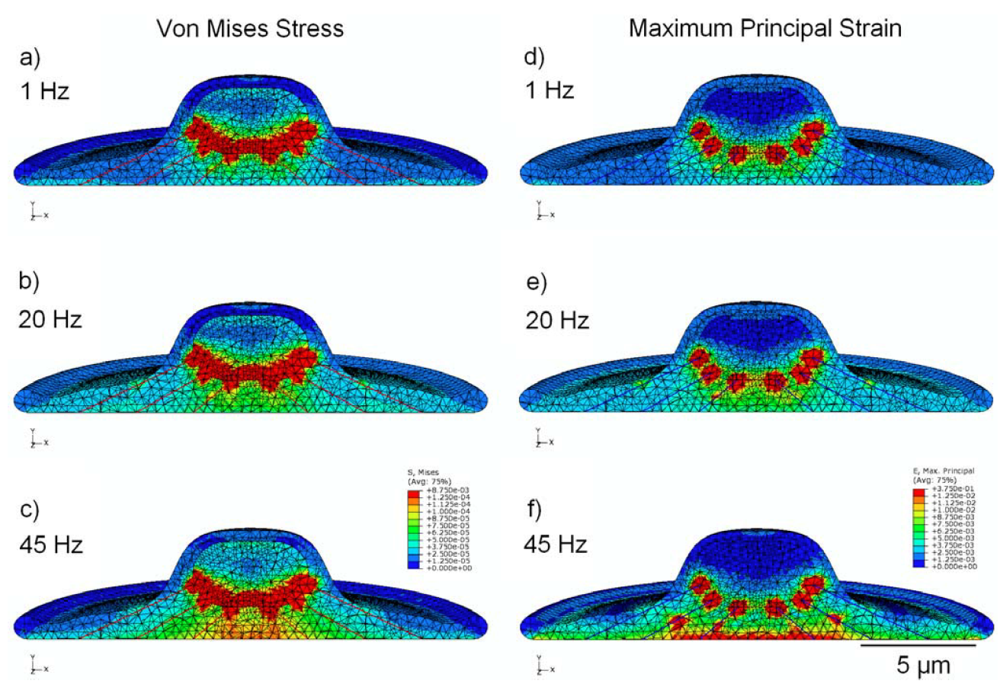
3.5. Effect of Cytoskeleton
4. Conclusions
Acknowledgments
References
- Hahn, C.; Schwartz, M.A. Mechanotransduction in vascular physiology and atherogenesis. Nat. Rev. Mol. Cell. Biol. 2009, 10, 53–62. [Google Scholar] [CrossRef]
- Jaalouk, D.E.; Lammerding, J. Mechanotransduction gone awry. Nat. Rev. Mol. Cell. Biol. 2009, 10, 63–73. [Google Scholar] [CrossRef]
- Huang, C.; Ogawa, R. Mechanotransduction in bone repair and regeneration. FASEB J. 2010, 24, 3625–3632. [Google Scholar] [CrossRef]
- Penley, N.J.; Schafer, C.P.; Bartoe, J.D. The international space station as a microgravity research platform. Acta Astronaut. 2002, 50, 691–696. [Google Scholar] [CrossRef]
- Rogers, M.J.; Hrovat, K.; Moskowitz, M.E. Effects of exercise equipment on the microgravity environment. Adv. Space. Res. 1999, 24, 1283–1287. [Google Scholar]
- Shi, Z.D.; Tarbell, J.M. Fluid flow mechanotransduction in vascular smooth muscle cells and fibroblasts. Ann. Biomed. Eng. 2011, 39, 1608–1619. [Google Scholar]
- Mazzag, B.; Barakat, A.I. The effect of noisy flow on endothelial cell mechanotransduction: A computational study. Ann. Biomed. Eng. 2011, 39, 911–921. [Google Scholar] [CrossRef]
- Ando, J.; Yamamoto, K. Effects of shear stress and stretch on endothelial function. Antioxid. Redox. Signal. 2011, 15, 1389–1403. [Google Scholar] [CrossRef]
- Willett, N.J.; Jr Long, R.C.; Maiellaro-Rafferty, K.; Sutliff, R.L.; Shafer, R.; Oshinski, J.N.; Giddens, D.P.; Guldberg, R.E.; Taylor, W.R. An in vivo murine model of low-magnitude oscillatory wall shear stress to address the molecular mechanisms of mechanotransduction—Brief report. Arterioscler. Thromb. Vasc. Biol. 2010, 30, 2099–2102. [Google Scholar]
- Ku, C.H.; Johnson, P.H.; Batten, P.; Sarathchandra, P.; Chambers, R.C.; Taylor, P.M.; Yacoub, M.H.; Chester, A.H. Collagen synthesis by mesenchymal stem cells and aortic valve interstitial cells in response to mechanical stretch. Cardiovasc. Res. 2006, 71, 548–556. [Google Scholar] [CrossRef]
- Butcher, J.T.; Nerem, R.M. Valvular endothelial cells regulate the phenotype of interstitial cells in co-culture: Effects of steady shear stress. Tissue Eng. 2006, 12, 905–915. [Google Scholar] [CrossRef]
- Birukova, A.A.; Fu, P.; Xing, J.; Yakubov, B.; Cokic, I.; Birukov, K.G. Mechanotransduction by GEF-H1 as a novel mechanism of ventilator-induced vascular endothelial permeability. Am. J. Physiol. Lung Cell. Mol. Physiol. 2010, 298, L837–L848. [Google Scholar] [CrossRef]
- Liu, M. Ventilator-induced lung injury and mechanotransduction: Why should we care? Crit. Care 2007, 11, 168–169. [Google Scholar] [CrossRef]
- Suki, B.; Ito, S.; Stamenovic, D.; Lutchen, K.R.; Ingenito, E.P. Biomechanics of the lung parenchyma: Critical roles of collagen and mechanical forces. J. Appl. Physiol. 2005, 98, 1892–1899. [Google Scholar]
- Weinbaum, S.; Duan, Y.; Satlin, L.M.; Wang, T.; Weinstein, A.M. Mechanotransduction in the renal tubule. Am. J. Physiol. Renal. Physiol. 2010, 299, F1220–F1236. [Google Scholar]
- Pecovnik-Balon, B. Cardiovascular calcification in patients with end-stage renal disease. Ther. Apher. Dial. 2005, 9, 208–210. [Google Scholar] [CrossRef]
- Suresh, S. Biomechanics and biophysics of cancer cells. Acta Mater. 2007, 55, 3989–4014. [Google Scholar] [CrossRef]
- Zeng, Y.; Sun, H.R.; Yu, C.; Lai, Y.; Liu, X.J.; Wu, J.; Chen, H.Q.; Liu, X.H. CXCR1 and CXCR2 are novel mechano-sensors mediating laminar shear stress-induced endothelial cell migration. Cytokine 2011, 53, 42–51. [Google Scholar] [CrossRef]
- Titushkin, I.A.; Shin, J.; Cho, M. A new perspective for stem-cell mechanobiology: Biomechanical control of stem-cell behavior and fate. Crit. Rev. Biomed. Eng. 2010, 38, 393–433. [Google Scholar] [CrossRef]
- Haudenschild, A.K.; Hsieh, A.H.; Kapila, S.; Lotz, J.C. Pressure and distortion regulate human mesenchymal stem cell gene expression. Ann. Biomed. Eng. 2009, 37, 492–502. [Google Scholar] [CrossRef]
- Kearney, E.M.; Prendergast, P.J.; Campbell, V.A. Mechanisms of strain-mediated mesenchymal stem cell apoptosis. J. Biomech. Eng. 2008, 130, 061004–061010. [Google Scholar] [CrossRef]
- Sarraf, C.E.; Otto, W.R.; Eastwood, M. In vitro mesenchymal stem cell differentiation after mechanical stimulation. Cell Prolif. 2011, 44, 99–108. [Google Scholar] [CrossRef]
- Galie, P.A.; Stegemann, J.P. Simultaneous application of interstitial flow and cyclic mechanical strain to a three-dimensional cell-seeded hydrogel. Tissue Eng. Part C Method. 2011, 17, 527–536. [Google Scholar] [CrossRef]
- Wolchok, J.C.; Brokopp, C.; Underwood, C.J.; Tresco, P.A. The effect of bioreactor induced vibrational stimulation on extracellular matrix production from human derived fibroblasts. Biomaterials 2009, 30, 327–335. [Google Scholar] [CrossRef]
- Pre, D.; Ceccarelli, G.; Benedetti, L.; Magenes, G.; Cusella de Angelis, M.G. Effects of low amplitude, high frequency vibrations on proliferation and differentiation of SAOS-2 human osteogenic cell line. Tissue Eng. Part C Method. 2009, 15, 669–679. [Google Scholar] [CrossRef]
- Schulz, R.M.; Wustneck, N.; van Donkelaar, C.C.; Shelton, J.C.; Bader, A. Development and validation of a novel bioreactor system for load- and perfusion-controlled tissue engineering of chondrocyte-constructs. Biotechnol. Bioeng. 2008, 101, 714–728. [Google Scholar] [CrossRef]
- Seguin, C.; McLachlan, J.M.; Norton, P.R.; Lagugne-Labarthet, F. Surface modification of poly(dimethylsiloxane) for microfluidic assay applications. Appl. Surf. Sci. 2010, 256, 2524–2531. [Google Scholar] [CrossRef]
- Forsyth, A.M.; Wan, J.; Ristenpart, W.D.; Stone, H.A. The dynamic behavior of chemically “Stiffened” red blood cells in microchannel flows. Microvasc. Res. 2010, 80, 37–43. [Google Scholar] [CrossRef]
- Kohles, S.S.; Neve, N.; Zimmerman, J.D.; Tretheway, D.C. Mechanical stress analysis of microfluidic environments designed for isolated biological cell investigations. J. Biomech. Eng. 2009, 131, 121006–121015. [Google Scholar] [CrossRef]
- Orr, D.E.; Burg, K.J. Design of a modular bioreactor to incorporate both perfusion flow and hydrostatic compression for tissue engineering applications. Ann. Biomed. Eng. 2008, 36, 1228–1241. [Google Scholar] [CrossRef]
- Maul, T.M.; Hamilton, D.W.; Nieponice, A.; Soletti, L.; Vorp, D.A. A new experimental system for the extended application of cyclic hydrostatic pressure to cell culture. J. Biomech. Eng. 2007, 129, 110–116. [Google Scholar]
- Wang, P.Y.; Chow, H.H.; Tsai, W.B.; Fang, H.W. Modulation of gene expression of rabbit chondrocytes by dynamic compression in polyurethane scaffolds with collagen gel encapsulation. J. Biomater. Appl. 2009, 23, 347–366. [Google Scholar]
- Lau, E.; Lee, W.D.; Li, J.; Xiao, A.; Davies, J.E.; Wu, Q.; Wang, L.; You, L. Effect of low-magnitude, high-frequency vibration on osteogenic differentiation of rat mesenchymal stromal cells. J. Orthop. Res. 2011, 29, 1075–1080. [Google Scholar]
- Lau, E.; Al-Dujaili, S.; Guenther, A.; Liu, D.; Wang, L.; You, L. Effect of low-magnitude, high-frequency vibration on osteocytes in the regulation of osteoclasts. Bone 2010, 46, 1508–1515. [Google Scholar] [CrossRef]
- Altman, G.H.; Lu, H.H.; Horan, R.L.; Calabro, T.; Ryder, D.; Kaplan, D.L.; Stark, P.; Martin, I.; Richmond, J.C.; Vunjak-Novakovic, G. Advanced bioreactor with controlled application of multi-dimensional strain for tissue engineering. J. Biomech. Eng. 2002, 124, 742–749. [Google Scholar]
- Wang, D.; Xie, Y.; Yuan, B.; Xu, J.; Gong, P.; Jiang, X. A stretching device for imaging real-time molecular dynamics of live cells adhering to elastic membranes on inverted microscopes during the entire process of the stretch. Integr. Biol. 2010, 2, 288–293. [Google Scholar] [CrossRef]
- Pang, Q.; Zu, J.W.; Siu, G.M.; Li, R.K. Design and development of a novel biostretch apparatus for tissue engineering. J. Biomech. Eng. 2010, 132, 014503–014506. [Google Scholar] [CrossRef]
- Zhang, L.; Kahn, C.J.; Chen, H.Q.; Tran, N.; Wang, X. Effect of uniaxial stretching on rat bone mesenchymal stem cell: Orientation and expressions of collagen types I and III and tenascin-C. Cell. Biol. Int. 2008, 32, 344–352. [Google Scholar] [CrossRef]
- Gerstmair, A.; Fois, G.; Innerbichler, S.; Dietl, P.; Felder, E. A device for simultaneous live cell imaging during uni-axial mechanical strain or compression. J. Appl. Physiol. 2009, 107, 613–620. [Google Scholar] [CrossRef]
- Bader, R.A.; Wagoner, K.L. Modulation of the response of rheumatoid arthritis synovial fibroblasts to proinflammatory stimulants with cyclic tensile strain. Cytokine 2010, 51, 35–41. [Google Scholar] [CrossRef]
- Doyle, A.M.; Nerem, R.M.; Ahsan, T. Human mesenchymal stem cells form multicellular structures in response to applied cyclic strain. Ann. Biomed. Eng. 2009, 37, 783–793. [Google Scholar] [CrossRef]
- Vande Geest, J.P.; Di Martino, E.S.; Vorp, D.A. An analysis of the complete strain field within flexercell membranes. J. Biomech. 2004, 37, 1923–1928. [Google Scholar] [CrossRef]
- Schaffer, J.L.; Rizen, M.; L'Italien, G.J.; Benbrahim, A.; Megerman, J.; Gerstenfeld, L.C.; Gray, M.L. Device for the application of a dynamic biaxially uniform and isotropic strain to a flexible cell culture membrane. J. Orthop. Res. 1994, 12, 709–719. [Google Scholar] [CrossRef]
- Park, J.S.; Chu, J.S.; Cheng, C.; Chen, F.; Chen, D.; Li, S. Differential effects of equiaxial and uniaxial strain on mesenchymal stem cells. Biotechnol. Bioeng. 2004, 88, 359–368. [Google Scholar] [CrossRef]
- Lee, A.A.; Delhaas, T.; Waldman, L.K.; MacKenna, D.A.; Villarreal, F.J.; McCulloch, A.D. An equibiaxial strain system for cultured cells. Am. J. Physiol. 1996, 271, C1400–1408. [Google Scholar]
- Hung, C.T.; Williams, J.L. A method for inducing equi-biaxial and uniform strains in elastomeric membranes used as cell substrates. J. Biomech. 1994, 27, 227–232. [Google Scholar]
- Ozcivici, E.; Luu, Y.K.; Adler, B.; Qin, Y.X.; Rubin, J.; Judex, S.; Rubin, C.T. Mechanical signals as anabolic agents in bone. Nat. Rev. Rheumatol. 2010, 6, 50–59. [Google Scholar]
- Judex, S.; Rubin, C.T. Is bone formation induced by high-frequency mechanical signals modulated by muscle activity? J. Musculoskelet. Neuronal Interact. 2010, 10, 3–11. [Google Scholar]
- Turner, C.H.; Warden, S.J.; Bellido, T.; Plotkin, L.I.; Kumar, N.; Jasiuk, I.; Danzig, J.; Robling, A.G. Mechanobiology of the skeleton. Sci. Signal. 2009, 2, 1–4. [Google Scholar]
- Sehmisch, S.; Galal, R.; Kolios, L.; Tezval, M.; Dullin, C.; Zimmer, S.; Stuermer, K.M.; Stuermer, E.K. Effects of low-magnitude, high-frequency mechanical stimulation in the rat osteopenia model. Osteoporos. Int. 2009, 20, 1999–2008. [Google Scholar] [CrossRef]
- Patel, M.J.; Chang, K.H.; Sykes, M.C.; Talish, R.; Rubin, C.; Jo, H. Low magnitude and high frequency mechanical loading prevents decreased bone formation responses of 2T3 preosteoblasts. J. Cell. Biochem. 2009, 106, 306–316. [Google Scholar] [CrossRef]
- Xie, L.; Rubin, C.; Judex, S. Enhancement of the adolescent murine musculoskeletal system using low-level mechanical vibrations. J. Appl. Physiol. 2008, 104, 1056–1062. [Google Scholar] [CrossRef]
- Rubin, C.T.; Capilla, E.; Luu, Y.K.; Busa, B.; Crawford, H.; Nolan, D.J.; Mittal, V.; Rosen, C.J.; Pessin, J.E.; Judex, S. Adipogenesis is inhibited by brief, daily exposure to high-frequency, extremely low-magnitude mechanical signals. Proc. Natl. Acad. Sci. USA 2007, 104, 17879–17884. [Google Scholar] [CrossRef]
- Judex, S.; Lei, X.; Han, D.; Rubin, C. Low-magnitude mechanical signals that stimulate bone formation in the ovariectomized rat are dependent on the applied frequency but not on the strain magnitude. J. Biomech. 2007, 40, 1333–1339. [Google Scholar] [CrossRef]
- Kloppel, T.; Wall, W.A. A novel two-layer, coupled finite element approach for modeling the nonlinear elastic and viscoelastic behavior of human erythrocytes. Biomech. Model. Mechanobiol. 2011, 10, 445–459. [Google Scholar] [CrossRef]
- Lavagnino, M.; Arnoczky, S.P.; Kepich, E.; Caballero, O.; Haut, R.C. A finite element model predicts the mechanotransduction response of tendon cells to cyclic tensile loading. Biomech. Model. Mechanobiol. 2008, 7, 405–416. [Google Scholar] [CrossRef]
- Gladilin, E.; Micoulet, A.; Hosseini, B.; Rohr, K.; Spatz, J.; Eils, R. 3D finite element analysis of uniaxial cell stretching: From image to insight. Phys. Biol. 2007, 4, 104–113. [Google Scholar] [CrossRef]
- McGarry, J.G.; Prendergast, P.J. A three-dimensional finite element model of an adherent eukaryotic cell. Eur. Cell. Mater. 2004, 7, 27–33. [Google Scholar]
- Miller, P.; Hu, L.; Wang, J. Finite element simulation of cell-substrate decohesion by laser-induced stress waves. J. Mech. Behav. Biomed. Mater. 2010, 3, 268–277. [Google Scholar] [CrossRef]
- Babalola, O.M.; Bonassar, L.J. Parametric finite element analysis of physical stimuli resulting from mechanical stimulation of tissue engineered cartilage. J. Biomech. Eng. 2009, 131, 061014–061020. [Google Scholar] [CrossRef]
- Kim, E.; Guilak, F.; Haider, M.A. The dynamic mechanical environment of the chondrocyte: A biphasic finite element model of cell-matrix interactions under cyclic compressive loading. J. Biomech. Eng. 2008, 130, 061009–061018. [Google Scholar] [CrossRef]
- Karcher, H.; Lammerding, J.; Huang, H.; Lee, R.T.; Kamm, R.D.; Kaazempur-Mofrad, M.R. A three-dimensional viscoelastic model for cell deformation with experimental verification. Biophys. J. 2003, 85, 3336–3349. [Google Scholar] [CrossRef]
- Donhauser, Z.J.; Jobs, W.B.; Binka, E.C. Mechanics of microtubules: Effects of protofilament orientation. Biophys. J. 2010, 99, 1668–1675. [Google Scholar] [CrossRef]
- Yang, N.; Wong, K.K.H.; De Bruyn, J.R.; Hutter, J.L. Frequency-dependent viscoelasticity measurement by atomic force microscopy. Meas. Sci. and Technol. 2009, 20. [Google Scholar] [CrossRef]
- Lulevich, V.; Zink, T.; Chen, H.Y.; Liu, F.T.; Liu, G.Y. Cell mechanics using atomic force microscopy-based single-cell compression. Langmuir 2006, 22, 8151–8155. [Google Scholar]
- Guhados, G.; Wan, W.; Hutter, J.L. Measurement of the elastic modulus of single bacterial cellulose fibers using atomic force microscopy. Langmuir 2005, 21, 6642–6646. [Google Scholar] [CrossRef]
- Zhao, R.; Wyss, K.; Simmons, C.A. Comparison of analytical and inverse finite element approaches to estimate cell viscoelastic properties by micropipette aspiration. J. Biomech. 2009, 42, 2768–2773. [Google Scholar] [CrossRef]
- Haider, M.A.; Guilak, F. An axisymmetric boundary integral model for assessing elastic cell properties in the micropipette aspiration contact problem. J. Biomech. Eng. 2002, 124, 586–595. [Google Scholar] [CrossRef]
- Bausch, A.R.; Ziemann, F.; Boulbitch, A.A.; Jacobson, K.; Sackmann, E. Local measurements of viscoelastic parameters of adherent cell surfaces by magnetic bead microrheometry. Biophys. J. 1998, 75, 2038–2049. [Google Scholar]
- Stops, A.J.; McMahon, L.A.; O'Mahoney, D.; Prendergast, P.J.; McHugh, P.E. A finite element prediction of strain on cells in a highly porous collagen-glycosaminoglycan scaffold. J. Biomech. Eng. 2008, 130, 061001–061011. [Google Scholar] [CrossRef]
- Wall, M.E.; Weinhold, P.S.; Siu, T.; Brown, T.D.; Banes, A.J. Comparison of cellular strain with applied substrate strain in vitro. J. Biomech. 2007, 40, 173–181. [Google Scholar] [CrossRef]
- Han, Y.; Cowin, S.C.; Schaffler, M.B.; Weinbaum, S. Mechanotransduction and strain amplification in osteocyte cell processes. Proc. Natl. Acad. Sci. USA 2004, 101, 16689–16694. [Google Scholar]
- Nguyen, B.V.; Wang, Q.G.; Kuiper, N.J.; El Haj, A.J.; Thomas, C.R.; Zhang, Z. Biomechanical properties of single chondrocytes and chondrons determined by micromanipulation and finite-element modelling. J. R. Soc. Interface 2010, 7, 1723–1733. [Google Scholar] [CrossRef]
- Peeters, E.A.; Bouten, C.V.; Oomens, C.W.; Bader, D.L.; Snoeckx, L.H.; Baaijens, F.P. Anisotropic, three-dimensional deformation of single attached cells under compression. Ann. Biomed. Eng. 2004, 32, 1443–1452. [Google Scholar] [CrossRef]
- Schneider, F.; Fellner, T.; Wilde, J.; Wallrabe, U. Mechanical properties of silicones for MEMS. J. Micromech. Microeng. 2008, 18. [Google Scholar] [CrossRef]
- Darling, E.M.; Topel, M.; Zauscher, S.; Vail, T.P.; Guilak, F. Viscoelastic properties of human mesenchymally-derived stem cells and primary osteoblasts, chondrocytes, and adipocytes. J. Biomech. 2008, 41, 454–464. [Google Scholar] [CrossRef]
- Cao, L.; Guilak, F.; Setton, L.A. Three-dimensional finite element modeling of pericellular matrix and cell mechanics in the nucleus pulposus of the intervertebral disk based on in situ morphology. Biomech. Model. Mechanobiol. 2010, 10, 1–10. [Google Scholar]
- Charras, G.T.; Horton, M.A. Determination of cellular strains by combined atomic force microscopy and finite element modeling. Biophys. J. 2002, 83, 858–879. [Google Scholar] [CrossRef]
- Or, M.; Kimmel, E. Modeling linear vibration of cell nucleus in low intensity ultrasound field. Ultrasound Med. Biol. 2009, 35, 1015–1025. [Google Scholar] [CrossRef]
- Wu, Z.Z.; Zhang, G.; Long, M.; Wang, H.B.; Song, G.B.; Cai, S.X. Comparison of the viscoelastic properties of normal hepatocytes and hepatocellular carcinoma cells under cytoskeletal perturbation. Biorheology 2000, 37, 279–290. [Google Scholar]
- Guilak, F.; Tedrow, J.R.; Burgkart, R. Viscoelastic properties of the cell nucleus. Biochem. Biophys. Res. Commun. 2000, 269, 781–786. [Google Scholar] [CrossRef]
- Jean, R.P.; Chen, C.S.; Spector, A.A. Finite-element analysis of the adhesion-cytoskeleton-nucleus mechanotransduction pathway during endothelial cell rounding: Axisymmetric model. J. Biomech. Eng. 2005, 127, 594–600. [Google Scholar] [CrossRef]
- Paul, R.; Heil, P.; Spatz, J.P.; Schwarz, U.S. Propagation of mechanical stress through the actin cytoskeleton toward focal adhesions: Model and experiment. Biophys. J. 2008, 94, 1470–1482. [Google Scholar] [CrossRef]
- Kumar, S.; Maxwell, I.Z.; Heisterkamp, A.; Polte, T.R.; Lele, T.P.; Salanga, M.; Mazur, E.; Ingber, D.E. Viscoelastic retraction of single living stress fibers and its impact on cell shape, cytoskeletal organization, and extracellular matrix mechanics. Biophys. J. 2006, 90, 3762–3773. [Google Scholar] [CrossRef]
- Bereiter-Hahn, J.; Karl, I.; Luers, H.; Voth, M. Mechanical basis of cell shape: Investigations with the scanning acoustic microscope. Biochem. Cell. Biol. 1995, 73, 337–348. [Google Scholar] [CrossRef]
- Dahl, K.N.; Ribeiro, A.J.; Lammerding, J. Nuclear shape, mechanics, and mechanotransduction. Circ. Res. 2008, 102, 1307–1318. [Google Scholar] [CrossRef]
- Wang, N. Structural basis of stress concentration in the cytoskeleton. Mol. Cell. Biomech. 2010, 7, 33–44. [Google Scholar]
© 2012 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Milner, J.S.; Grol, M.W.; Beaucage, K.L.; Dixon, S.J.; Holdsworth, D.W. Finite-Element Modeling of Viscoelastic Cells During High-Frequency Cyclic Strain. J. Funct. Biomater. 2012, 3, 209-224. https://doi.org/10.3390/jfb3010209
Milner JS, Grol MW, Beaucage KL, Dixon SJ, Holdsworth DW. Finite-Element Modeling of Viscoelastic Cells During High-Frequency Cyclic Strain. Journal of Functional Biomaterials. 2012; 3(1):209-224. https://doi.org/10.3390/jfb3010209
Chicago/Turabian StyleMilner, Jaques S., Matthew W. Grol, Kim L. Beaucage, S. Jeffrey Dixon, and David W. Holdsworth. 2012. "Finite-Element Modeling of Viscoelastic Cells During High-Frequency Cyclic Strain" Journal of Functional Biomaterials 3, no. 1: 209-224. https://doi.org/10.3390/jfb3010209
APA StyleMilner, J. S., Grol, M. W., Beaucage, K. L., Dixon, S. J., & Holdsworth, D. W. (2012). Finite-Element Modeling of Viscoelastic Cells During High-Frequency Cyclic Strain. Journal of Functional Biomaterials, 3(1), 209-224. https://doi.org/10.3390/jfb3010209




