Structure and Properties of High and Low Free Volume Polymers Studied by Molecular Dynamics Simulation
Abstract
1. Introduction
2. Modeling Details
- Preparation of a dense gas system from 500 monomers in a cubic computational cell with periodic boundary conditions.
- Obtaining of dense melt at a high temperature (800 K). Affine compression of the cells was performed simultaneously along three directions with a rate of 0.1 Å/ps. So far, their density will be 1.2 g/cm3 for polyetherimides and 0.9 g/cm3 for Si-substituted polytricyclonones. This density value is close to the experimental data given in Table 2.
- Random polymerization with the avoidance of cyclic formation and relaxation of the system within 1 ns. For this purpose, at each step of molecular dynamic calculation during this process, the end atoms of the monomer or already synthesized oligomer that were located closer than critical distance were connected via a harmonic potential and the forces arising at the same time begin to draw them together. One of the key parameters regulating the polymerization process is a critical distance which gradually increased from 4 Å at the beginning of polymerization process to 12 Å at the end. The polymerization process was considered successful if the degree of polymerization in the model exceeded the specified value (in this case it was more than 20). Upon completion of polymerization, new lists of valent communications for the synthesized polymeric systems were made. A high temperature (800 K) was chosen to increase the mobility of molecules and to increase the probability of approaching the unreacted ends of different molecules.
- The systems prepared at 800 K were allowed to slowly cool to 300 K at constant volume with a rate 1 K/ps.
- Then we used the NPT- simulation to relax the systems to a temperature of 300 K and atmospheric pressure, which was carried out for several hundred picoseconds until stationary parameters (density, contributions into the energy of the system valence bonds and valence and torsion angles, nonvalence Lennard–Jones (LJ) and Coulomb interactions) are achieved.
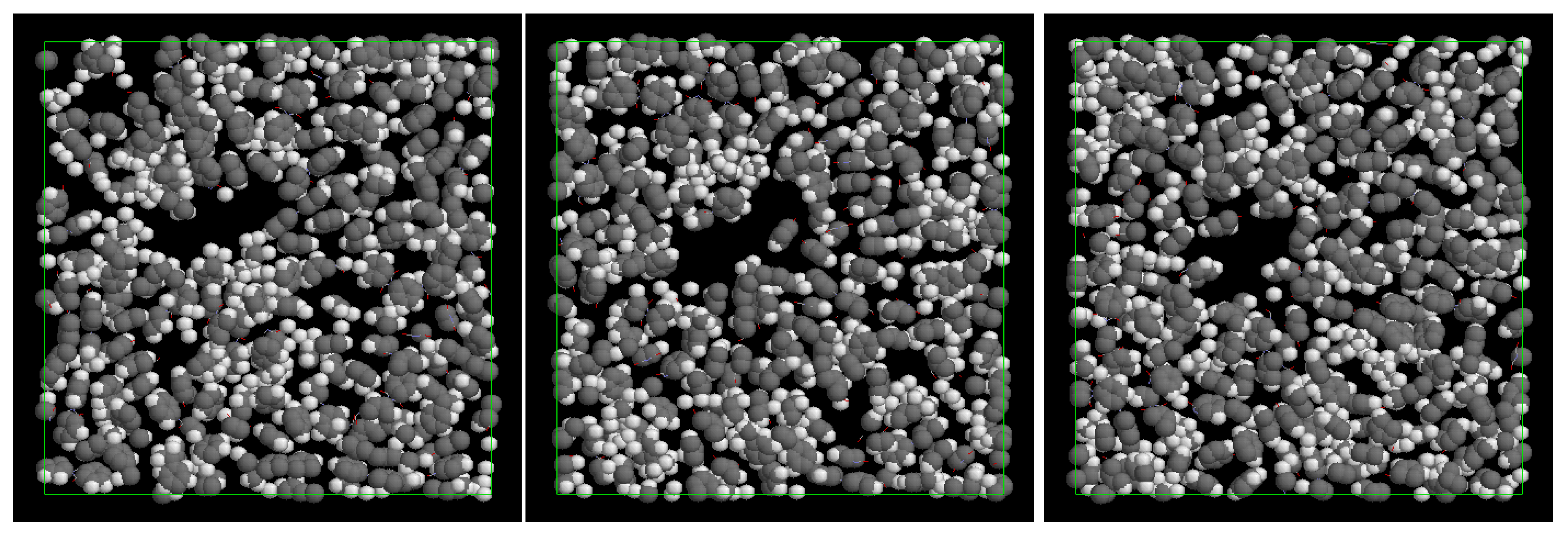
3. Description of Obtained Samples
4. Comparison of Free Volume in Low Permeability and Highly Permeable Polymers
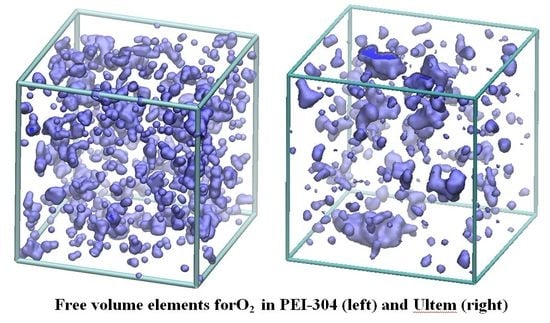
4.1. Visualization of Free Volume
4.2. Accessible Free Volume as a Function of Probe Size
5. Size Distribution of Free Volume
6. Analysis of the Surface of Free Volume Element
6.1. Procedures for Analysis of FVE
6.2. Results on FVE Surface Analysis
6.3. Nature of the Groups Forming the Surface of FVEs
7. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Theodorou, D.N. Principles of Molecular Simulation of Gas Transport in Polymers. In Materials Science of Membranes for Gas and Vapor Separation; Yampolskii, Y., Pinnau, I., Freeman, B.D., Eds.; Wiley: Chichester, UK, 2006; pp. 49–94. [Google Scholar]
- Neyertz, S. Gas transport in dense polymeric membranes, molecular dynamics simulations. Encycl. Membr. Sci. Technol. 2013, 1, 171–191. [Google Scholar]
- Hofmann, D.; Entrialgo-Castano, M.; Lerbret, A.; Heuchel, M.; Yampolskii, Y. Molecular Modeling Investigation of Free Volume Distributions in Stiff Chain Polymers with Conventional and Ultrahigh Free Volume: Comparison between Molecular Modeling and Positron Lifetime Studies. Macromolecules 2003, 36, 8528–8538. [Google Scholar] [CrossRef]
- Heuchel, M.; Fritsch, D.; Budd, P.M.; McKeown, N.B.; Hofmann, D. Atomistic packing model and free volume distribution of a polymer with intrinsic microporosity (PIM-1). J. Membr. Sci. 2008, 318, 84–99. [Google Scholar] [CrossRef]
- Heuchel, M.; Hofmann, D.; Pullumbi, P. Molecular Modeling of Small-Molecule Permeation in Polyimides and Its Correlation to Free-Volume Distributions. Macromolecules 2004, 37, 201–214. [Google Scholar] [CrossRef]
- Bisoi, S.; Manda, A.K.; Padmanabhan, V.; Banerjee, S. Aromatic polyamides containing trityl substituted triphenylamine: Gas transport properties and molecular dynamics simulations. J. Membr. Sci. 2017, 522, 77–90. [Google Scholar] [CrossRef]
- Tocci, E.; De Lorenzo, L.; Bernardo, P.; Clarizia, G.; Bazzarelli, F.; Mckeown, N.B.; Carta, M.; Malpass-Evans, R.; Friess, K.; Pilnacek, K.; et al. Molecular Modeling and Gas Permeation Properties of a Polymer of Intrinsic Microporosity Composed of Ethanoanthracene and Troöger’s Base Units. Macromolecules 2014, 47, 7900–7916. [Google Scholar] [CrossRef]
- Pinel, E.; Brown, D.; Bas, C.; Mercier, R.; Alberola, N.D.; Neyertz, S. Chemical Influence of the Dianhydride and the Diamine Structure on a Series of Copolyimides Studied by Molecular Dynamics Simulations. Macromolecules 2002, 35, 10198–10209. [Google Scholar] [CrossRef]
- Neyertz, S.; Douanne, A.; Brown, D. Effect of Interfacial Structure on Permeation Properties of Glassy Polymers. Macromolecules 2005, 38, 10286–10298. [Google Scholar] [CrossRef]
- Pandiyan, S.; Brown, D.; Neyertz, S.; van der Vegt, N.F.A. Carbon Dioxide Solubility in Three Fluorinated Polyimides Studied by Molecular Dynamics Simulations. Macromolecules 2010, 43, 2605–2621. [Google Scholar] [CrossRef]
- Spyriouni, T.; Boulougouris, G.C.; Theodorou, D.N. Prediction of Sorption of CO2 in Glassy Atactic Polystyrene at Elevated Pressures Through a New Computational Scheme. Macromolecules 2009, 42, 1759–1769. [Google Scholar] [CrossRef]
- Neyertz, S.; Brown, D. Oxygen Sorption in Glassy Polymers Studied at the Molecular Level. Macromolecules 2009, 42, 8521–8533. [Google Scholar] [CrossRef]
- Nazarychev, V.M.; Dobrovskiy, A.Y.; Larin, S.V.; Lyulin, A.V.; Lyulin, S.V. Simulating local mobility and mechanical properties of thermostable polyimides with different dianhydride fragments. J. Polym. Sci. Part B: Polym. Phys. 2018, 56, 375–382. [Google Scholar] [CrossRef]
- Lyulin, S.V.; Larin, S.V.; Nazarychev, V.M.; Fal’kovich, S.G.; Kenny, J.M. Multiscale computer simulation of polymer nanocomposites based on thermoplastics. Polym. Sci. Ser. C 2016, 58, 2–15. [Google Scholar] [CrossRef]
- Mazo, M.; Balabaev, N.; Alentiev, A.; Yampolskii, Y. Molecular Dynamics Simulation of Nanostructure of High Free Volume Polymers with SiMe3 Side Groups. Macromolecules 2018, 51, 1398–1408. [Google Scholar] [CrossRef]
- Barbari, T.A.; Koros, W.J.; Paul, D.R. Polymeric membranes based on bisphenol-A for gas separation. J. Membr. Sci. 1989, 42, 69–86. [Google Scholar] [CrossRef]
- Alentiev, A.Y.; Yampolskii, Y.P.; Rusanov, A.L.; Likhachev, D.; Kazakova, G.V.; Komarova, L.G.; Prigozhina, M.P. Transport Properties of Poly(ether imides). Polym. Sci. A 2003, 45, 933–939. [Google Scholar]
- Chirkov, S.V.; Kechekyan, A.S.; Belov, N.A.; Antonov, S.V.; Alentiev, A.Y. The influence of uniform deformation of Ultem-1000 polyetherimide films on their mechanical and gas transport characteristics. Petrol. Chem. 2016, 56, 1074–1084. [Google Scholar] [CrossRef]
- Alentiev, A.Y.; Belov, N.A.; Chirkov, S.V.; Yampolskii, Y.P. Gas diffusion characteristics as criteria of nonequilibrium state of amorphous glassy polymers. J. Membr. Sci. 2018, 547, 99–109. [Google Scholar] [CrossRef]
- Alentiev, A.Y.; Yampolskii, Y.P. Free volume model and tradeoff relations of gas permeability and selectivity in glassy polymers. J. Membr. Sci. 2000, 165, 201–216. [Google Scholar] [CrossRef]
- Ronova, I.A.; Alentiev, A.Y.; Chisca, S.; Sava, I.; Bruma, M.; Nikolaev, A.Y.; Belov, N.A.; Buzin, M.I. Change of microstructure of polyimide thin films under the action of supercritical carbon dioxide and its influence on the transport properties. Struct. Chem. 2014, 25, 301–310. [Google Scholar] [CrossRef]
- Chirkov, S.; Kechekian, A.; Belov, N.; Antonov, S.; Alentiev, A. Mechanical and gas permeation properties of uniformerly deformed films of polyetherimides. J. Butlerov Commun. 2016, 48, 54–59. [Google Scholar]
- Aliev, A.; Chalykh, A.; Gerasimov, V.; Balashova, E.; Alentiev, A.Y.; Yampolskii, Y.P.; Stepanenko, V. The Kinetics of Desorption of a Residual Solvent from Poly(ether imide). Polym. Sci. A 2002, 44, 603–608. [Google Scholar]
- Yampolskii, Y.P.; Alentiev, A.Y.; Bondarenko, G.; Kostina, Y.; Heuchel, M. Intermolecular Interactions: New Way to Govern Transport Properties of Membrane Materials. Ind. Eng. Chem. Res. 2010, 49, 12031–12037. [Google Scholar] [CrossRef]
- Gringolts, M.; Bermeshev, M.; Yampolskii, Y.P.; Starannikova, L.; Shantarovich, V.; Finkelshtein, E. New High Permeable Addition Poly(tricyclononenes) with Si(CH3)3 Side Groups. Synthesis, Gas Permeation Parameters, and Free Volume. Macromolecules 2010, 43, 7165–7172. [Google Scholar] [CrossRef]
- Chapala, P.; Bermeshev, M.; Starannikova, L.; Belov, N.; Ryzhikh, V.; Shantarovich, V.; Lakhtin, V.; Gavrilova, N.; Yampolskii, Y.; Finkelshtein, E. A Novel, Highly Gas-Permeable Polymer Representing a New Class of Silicon-Containing Polynorbornens as Efficient Membrane Materials. Macromolecules 2015, 48, 8055–8061. [Google Scholar] [CrossRef]
- Balabaev, N.K.; Mazo, M.A.; Lyulin, A.V.; Oleinik, E.F. Plastic Deformation of Glassy Polymethylene: Computer Aided Molecular Dynamic Simulation. Polym. Sci. Ser. A 2010, 52, 633–644. [Google Scholar] [CrossRef]
- Fried, J.R. Molecular simulation of gas and vapor transport in highly permeable polymers. In Materials Science of Membranes for Gas and Vapor Separation; Yampolskii, Y., Pinnau, I., Freeman, B.D., Eds.; Wiley: Chichester, UK, 2006; pp. 95–136. [Google Scholar]
- Glyakina, A.V.; Balabaev, N.K.; Galzitskaya, O.V. Mechanical unfolding of proteins L and G with constant force: Similarities and differences. J. Chem. Phys. 2009, 131, 045102. [Google Scholar] [CrossRef]
- Lyulin, A.V.; Balabaev, N.K.; Michels, M.A.J. Correlated Segmental Dynamics in Amorphous Atactic Polystyrene: A Molecular Dynamics Simulation Study. Macromolecules 2002, 35, 9595–9604. [Google Scholar] [CrossRef]
- Hill, J.R.; Sauer, J. Molecular Mechanics Potential for Silica and Zeolite Catalysts Based on ab Initio Calculations. 2. Aluminosilicates. J. Phys. Chem. 1995, 99, 9536–9550. [Google Scholar] [CrossRef]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids; Clarendon: Oxford, UK, 1987. [Google Scholar]
- Lemak, A.S.; Balabaev, N.K. Molecular dynamics simulation of polymer chain in solution by collisional dynamics method. J. Comput. Chem. 1996, 17, 1685–1695. [Google Scholar] [CrossRef]
- Lemak, A.S.; Balabaev, N.K. A comparison between collisional dynamics and Brownian dynamics. Mol. Simul. 1995, 15, 223–231. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Postma, J.P.M.; van Gunsteren, W.F.; Di Nola, A.; Haak, J.R. Molecular dynamics with coupling to an external bath. J. Chem. Phys. 1984, 81, 3684–3690. [Google Scholar] [CrossRef]
- Shantarovich, V.; Kevdina, I.; Yampolskii, Y.P.; Alentiev, A.Y. Positron annihilation lifetime study of high and low free volume glassy polymers: Effects of free volume sizes on the permeability and permselectivity. Macromolecules 2000, 33, 7453–7466. [Google Scholar] [CrossRef]
- Greenfield, M.L.; Theodorou, D.N. Geometric analysis of diffusion pathways in glassy and melt atactic polypropylene. Macromolecules 1993, 26, 5461–5472. [Google Scholar] [CrossRef]
- Teplyakov, V.; Meares, P. Correlation aspects of the selective gas permeabilities of polymeric materials and membranes. Gas Sep. Purif. 1990, 4, 66–73. [Google Scholar] [CrossRef]
- Mitternacht, S. FreeSASA: An open source C library for solvent accessible surface area calculations. F1000Res. 2016, 5, 189. [Google Scholar] [CrossRef]
- Medvedev, N.N. Voronoi-Delaunay Method in Structural Studies of Noncrystalline Systems; Nauka: Novosibirsk, Russia, 2000. (In Russian) [Google Scholar]
- Shaytan, A.K.; Xiao, H.; Armeev, G.A.; Gaykalova, D.A.; Komarova, G.A.; Wu, C.; Studitsky, V.M.; Landsman, D.; Panchenko, A.R. Structural interpretation of DNA–protein hydroxyl-radical footprinting experiments with high resolution using HYDROID. Nat. Protoc. 2018, 13, 2535–2556. [Google Scholar] [CrossRef]
- Mitchell, L.S.; Colwell, L.J. Analysis of nanobody paratopes reveals greater diversity than classical antibodies. Protein Eng. Des. Sel. 2018, 31, 267–275. [Google Scholar] [CrossRef]
- Mapes, N.J., Jr.; Rodriguez, C.; Chowriappa, P.; Dua, S. Residue Adjacency Matrix Based Feature Engineering for Predicting Cysteine Reactivity in Proteins. Comput. Struct. Biotechnol. J. 2019, 17, 90–100. [Google Scholar] [CrossRef]
- Breck, D.W. Zeolite Molecular Sieves: Structure, Chemistry and Uses; Wiley: New York, NY, USA, 1974; pp. 593–724. [Google Scholar]
- Poling, B.E.; Prausnitz, J.M.; O’Connell, J.P. The Properties of Gases and Liquids, 5th ed.; McGraw-Hill: New York, NY, USA, 2000. [Google Scholar]
- Hemalatha, P.; Ganesh, M.; Pei, M.M.; Jong, E.M.; Palaniohany, M.; Jang, H. Polyimides: Synthesis, characterization, and application to carbon dioxide adsorption. Comput. Inf. Sci. 2012, 341, 229–236. [Google Scholar]
- Yang, S.-Y. Advanced Polyimide Materials. Synthesis, Characterization and Applications; Elsevier: Amsterdam, The Netherlands, 2018; p. 304. [Google Scholar]










| Polymer | Formula of the Monomer |
|---|---|
| Ultem |  |
| PEI-304 |  |
| PTCNSi2v |  |
| PTCNSi2g |  |
| Parameter | Ultem [16,17,18,19] | PEI-304 [20,21,22,23,24] | PTCNSi2v [25] | PTCNSi2g [26] |
|---|---|---|---|---|
| Density, g/cm3 | 1.28–1.30 | 1.37 | 0.81 | 0.853 |
| FFV, % | 10.8–12.1 | 9.2–10.0 | 34.9 | 29.7 |
| Tg, °C | 215 | 180 | >370 | >340 |
| P(O2), Barrer | 0.40–0.88 | 0.68–0.84 | 2380 | 4750 |
| P(O2)/P(N2) | 6.4–7.6 | 8.4–11.7 | 1.9 | 1.8 |
| Polymer | Ave. Number of Repeat Units in One Chain | Mn (Da) | Mw (Da) | Polydispersity |
|---|---|---|---|---|
| Ultem | 25.6 ± 3.6 | 27,400 ± 6800 | 38,000 ± 12,000 | 1.36 ± 0.12 |
| PEI-304 | 30.5 ± 5.2 | 30,600 ± 5200 | 50,160 ± 11,000 | 1.65 ± 0.27 |
| Probe Radius Å | Ultem | PEI-304 | PTCNSi2v | PTCNSi2g |
|---|---|---|---|---|
| 0 | 4090 | 3830 | 5710 | 5330 |
| 0.53 | 1600 | 1365 | 2710 | 2900 |
| 1.07 | 407 | 257 | 1330 | 1607 |
| 1.445 | 144 | 62 | 816 | 1072 |
| 1.52 | 129 | 53 | 774 | 1027 |
| 1.485 | 121 | 48 | 749 | 1000 |
| 1.51 | 117 | 46 | 740 | 989 |
| Atom or Group | Number of Atoms in Repeat Unit | % of Total Atoms | Fraction (%) | ||
|---|---|---|---|---|---|
| Probe Radius < 1 Å | Probe Radius 1 ÷ 1.6 Å | Probe Radius 1.76 Å | |||
| –C(CH3)2– | 3 | 6.7 | 8–11 | 15–19 | 21 |
| Carbon in carbonyl group | 4 | 8.9 | 6–3 | 2 | 1.6 |
| CH group in benzene ring | 30 | 66.7 | 67–71 | 62–65 | 61 |
| Nitrogen | 2 | 4.4 | 1–2 | 0.3 | 0.25 |
| Oxygen in carbonyl group | 4 | 8.9 | 11–14 | 12–13 | 12 |
| Oxygen as connection group | 2 | 4.4 | 3–4 | 4 | 4 |
| Atom or Group | Number of Atoms in Repeat Unit | % of Total Atoms | Fraction (%) | ||
|---|---|---|---|---|---|
| Probe Radius < 1 Å | Probe Radius 1 ÷ 1.6 Å | Probe Radius 1.76 Å | |||
| –C(CH3)2– | 6 | 8.1 | 6–8 | ~9 | 9.6 |
| Carbon in carbonyl group | 4 | 5.4 | 2–3 | 2 | 2 |
| CH group in benzene ring | 48 | 64.9 | 65–70 | 60 | 60 |
| Nitrogen | 2 | 2.7 | 0.7–0.4 | 0.3 | 0.26 |
| Oxygen in carbonyl group | 4 | 5.4 | 7–9 | 8–9 | 8.4 |
| Oxygen as connection group | 4 | 5.4 | 4–5 | 4 | 4 |
| Fluorine | 6 | 8.1 | 8–12 | 14–16 | 16.3 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mazo, M.; Balabaev, N.; Alentiev, A.; Strelnikov, I.; Yampolskii, Y. Structure and Properties of High and Low Free Volume Polymers Studied by Molecular Dynamics Simulation. Computation 2019, 7, 27. https://doi.org/10.3390/computation7020027
Mazo M, Balabaev N, Alentiev A, Strelnikov I, Yampolskii Y. Structure and Properties of High and Low Free Volume Polymers Studied by Molecular Dynamics Simulation. Computation. 2019; 7(2):27. https://doi.org/10.3390/computation7020027
Chicago/Turabian StyleMazo, Mikhail, Nikolay Balabaev, Alexandre Alentiev, Ivan Strelnikov, and Yury Yampolskii. 2019. "Structure and Properties of High and Low Free Volume Polymers Studied by Molecular Dynamics Simulation" Computation 7, no. 2: 27. https://doi.org/10.3390/computation7020027
APA StyleMazo, M., Balabaev, N., Alentiev, A., Strelnikov, I., & Yampolskii, Y. (2019). Structure and Properties of High and Low Free Volume Polymers Studied by Molecular Dynamics Simulation. Computation, 7(2), 27. https://doi.org/10.3390/computation7020027