Dislocation Interactions with Hcp- and χ-Phase Particles in Tungsten: Molecular Dynamics Insights into Mechanical Strengthening Mechanisms
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
4. Conclusions
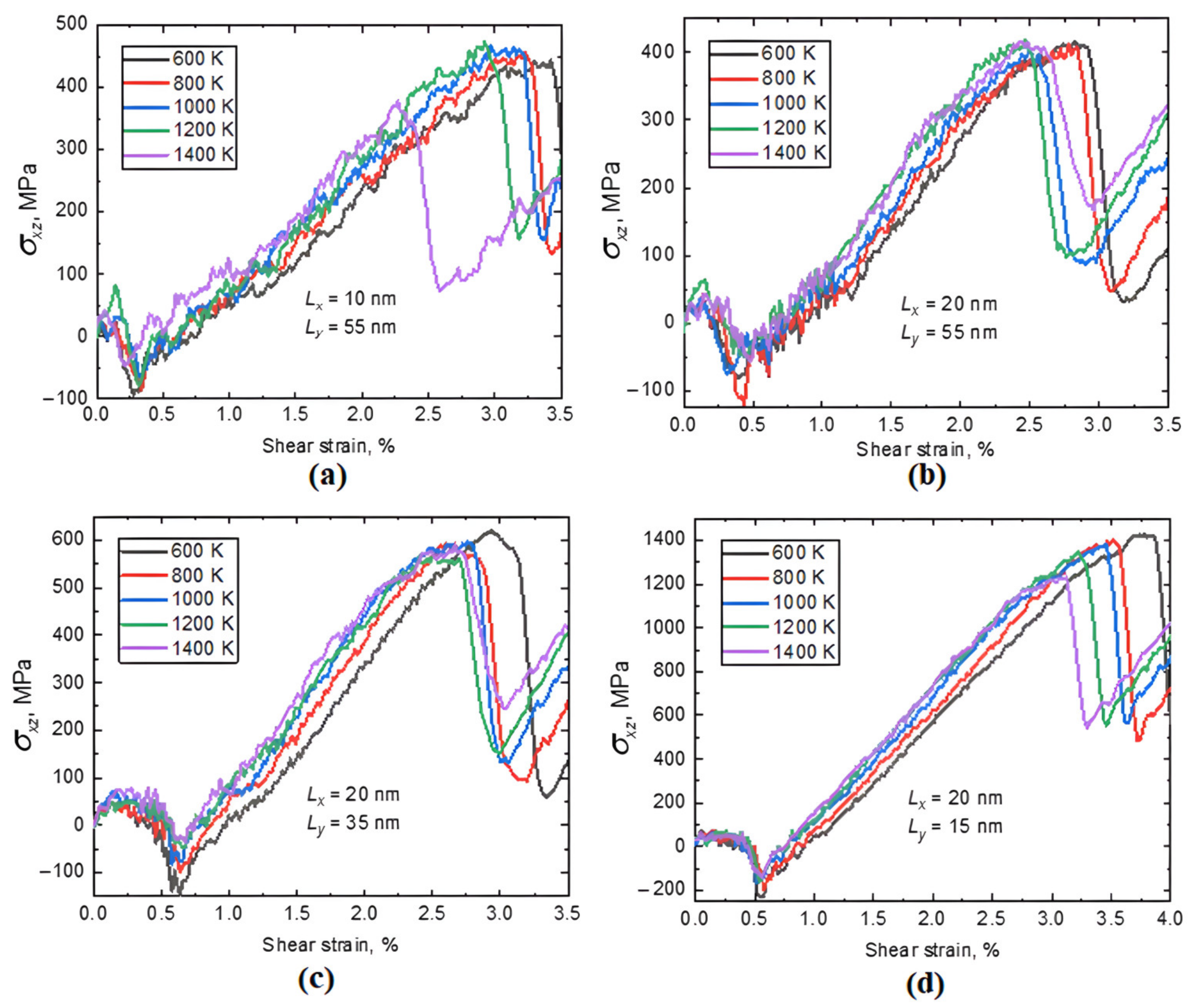
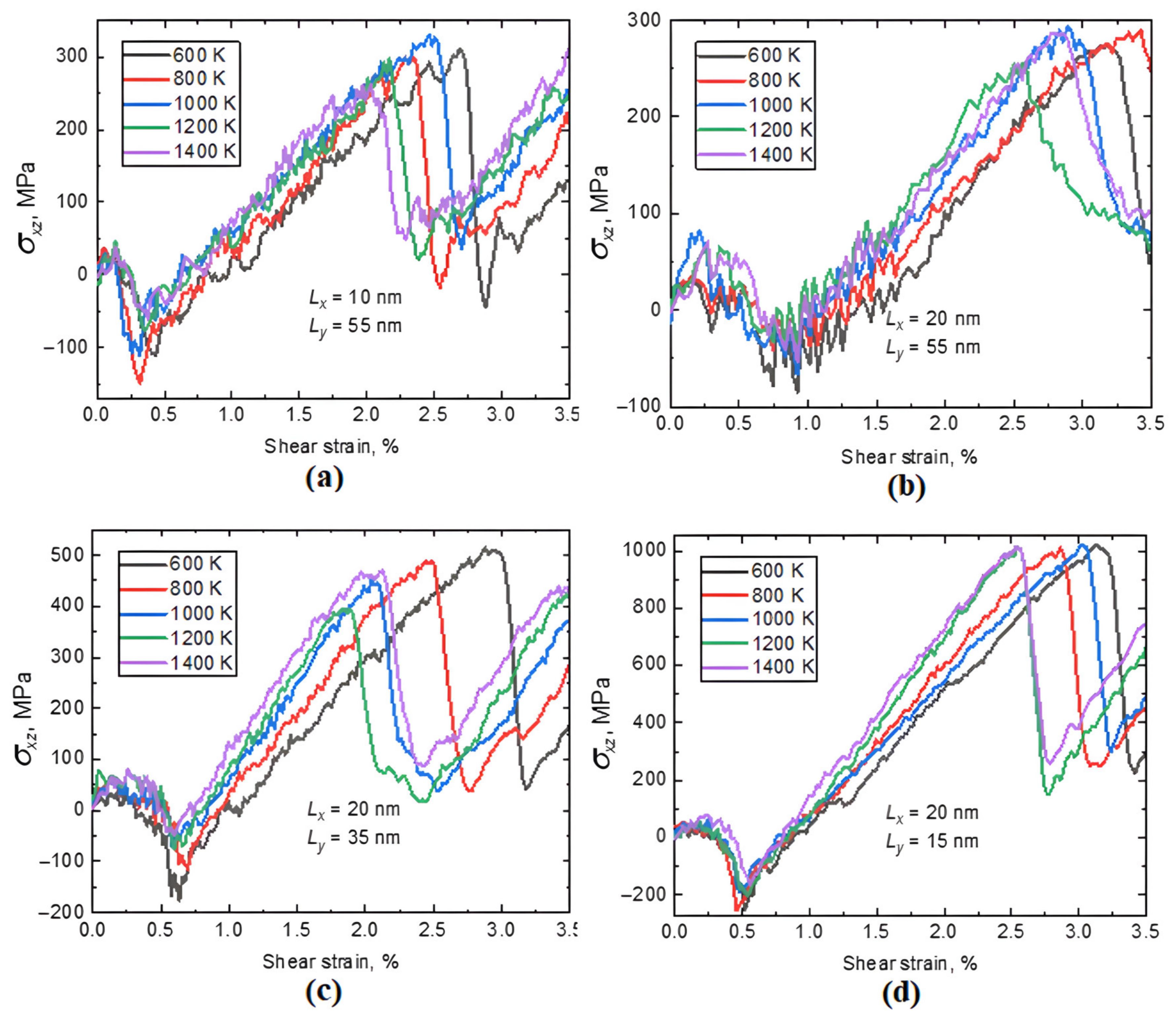
- Particle Shape and Size Effects: Cylindrical particles require approximately 20% higher critical shear stresses compared to spherical particles, indicating that particle shape significantly influences dislocation behaviour. The critical shear stress increases almost linearly with particle diameter.
- Temperature Effects: The influence of temperature on critical shear stress is more pronounced for χ-phase particles than for hcp-phase particles. Higher temperatures generally reduce the critical shear stress required for dislocation movement, highlighting the thermally activated nature of these interactions.
- Cell Size Effects: Reducing the cell size along the y-axis increases the critical shear stress, demonstrating the importance of spatial constraints on dislocation dynamics.
- Material Composition: The study confirms that hcp-phase particles, composed entirely of Re atoms, present stronger obstacles to dislocations than χ-phase particles, which contain a mix of W and Re atoms. This is attributed to the greater local lattice distortion around hcp-phase particles.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Knaster, J.; Moeslang, A.; Muroga, T. Materials research for fusion. Nat. Phys. 2016, 12, 424–434. [Google Scholar] [CrossRef]
- Riesch, J.; Feichtmayer, A.; Coenen, J.; Curzadd, B.; Gietl, H.; Hoeschen, T.; Manhard, A.; Schwarz-Selinger, T.; Neu, R. Irradiation effects in tungsten—From surface effects to bulk mechanical properties. Nucl. Mater. Energy 2021, 30, 101093. [Google Scholar] [CrossRef]
- Terentyev, D.; Zinovev, A.; Khvan, T.; You, J.-H.; Van Steenberge, N.; Zhurkin, E.E. Irradiation embrittlement in pure chromium and chromium-tungsten alloy in a view of their potential application for fusion plasma facing components. J. Nucl. Mater. 2021, 554, 153086. [Google Scholar] [CrossRef]
- Terentyev, D.; Rieth, M.; Pintsuk, G.; Riesch, J.; Von Müller, A.; Antusch, S.; Mergia, K.; Gaganidze, E.; Schneider, H.C.; Wirtz, M.; et al. Recent progress in the assessment of irradiation effects for in-vessel fusion materials: Tungsten and copper alloys. Nucl. Fusion 2021, 62, 026045. [Google Scholar] [CrossRef]
- Li, Y.-H.; Ma, F.-F.; Yue, F.-Y.; Ren, Q.-Y.; Zhou, H.-B.; Hong, L. Radiation-induced precipitation of transmutation elements rhenium/osmium and their effects on hydrogen behavior in tungsten. Prog. Nat. Sci. Mater. Int. 2019, 29, 285–294. [Google Scholar] [CrossRef]
- Yokoyama, T.; Ozawa, M. Production of Low Activity Rhenium by Transmuting Tungsten Metal in Fast Reactors with Moderator. Int. J. At. Nucl. Phys. 2019, 4, 12. [Google Scholar] [CrossRef]
- Osetsky, Y. Strengthening of tungsten by coherent rhenium precipitates formed during low fluence irradiation. Tungsten 2022, 4, 20–27. [Google Scholar] [CrossRef]
- Liu, M.; Cowley, J. Particle/dislocation interactions in a dispersion strengthened tungsten alloy at ultrahigh temperatures. Scr. Metall. Mater. 1993, 28, 307–312. [Google Scholar] [CrossRef]
- Mihajlović, A.; Jovanović, M.; Nešić, O. Interaction of dislocations with precipitates in a Cu–Be–Ni–Zr alloy. Met. Technol. 1979, 6, 190–193. [Google Scholar] [CrossRef]
- Jarlöv, A.; Ji, W.; Babicheva, R.; Tian, Y.; Hu, Z.; Seet, H.L.; Tan, L.; Liu, F.; Liu, Y.; Nai, S.M.L.; et al. Tailoring short-range order and dislocation evolution in Cr–Co–Ni medium-entropy alloys: A molecular dynamics study. Mater. Des. 2024, 240, 112840. [Google Scholar] [CrossRef]
- Jarlöv, A.; Ji, W.-M.; Zhu, Z.; Tian, Y.; Babicheva, R.; An, R.; Seet, H.; Nai, M.L.S.; Zhou, K. Molecular dynamics study on the strengthening mechanisms of Cr-Fe-Co-Ni high-entropy alloys based on the generalized stacking fault energy. J. Alloys Compd. 2022, 905, 164137. [Google Scholar] [CrossRef]
- Lee, H.; Shim, J.; Wirth, B. Molecular Dynamics Simulation of Dislocation-Obstacle Interactions in Irradiated Materials. Key Eng. Mater. 2007, 345–346, 947–950. [Google Scholar] [CrossRef]
- Wan, C.-F.; Sun, L.-G.; Qin, H.-L.; Bi, Z.-N.; Li, D.-F. A Molecular Dynamics Study on the Dislocation-Precipitate Interaction in a Nickel Based Superalloy during the Tensile Deformation. Materials 2023, 16, 6140. [Google Scholar] [CrossRef] [PubMed]
- Yazdani, S.; Mesbah, M.; Vitry, V. Molecular Dynamics Simulation of the Interaction between Dislocations and Iron–Vanadium Precipitates in Alpha Iron: Effect of Chemical Composition. Crystals 2023, 13, 1247. [Google Scholar] [CrossRef]
- Kazakov, A.; Babicheva, R.; Zinovev, A.; Terentyev, D.; Zhou, K.; Korznikova, E.; Dmitriev, S. Interaction of edge dislocations with voids in tungsten. Tungsten 2024, 6, 633–646. [Google Scholar] [CrossRef]
- Li, L.; Wang, H.; Xu, K.; Li, B.; Jin, S.; Li, X.-C.; Shu, X.; Liang, L.; Lu, G.-H. Atomic Simulations of the Interaction between a Dislocation Loop and Vacancy-Type Defects in Tungsten. Metals 2022, 12, 368. [Google Scholar] [CrossRef]
- Bonny, G.; Bakaev, A.; Terentyev, D. Assessment of hardening due to non-coherent precipitates in tungsten-rhenium alloys at the atomic scale. Sci. Rep. 2019, 9, 16215. [Google Scholar] [CrossRef] [PubMed]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comp. Phys. Comm. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Bonny, G.; Bakaev, A.; Terentyev, D.; Mastrikov, Y. Interatomic potential to study plastic deformation in tungsten-rhenium alloys. J. Appl. Phys. 2017, 121, 165107. [Google Scholar] [CrossRef]
- Kosarev, I.; Shcherbinin, S.; Kistanov, A.; Babicheva, R.; Korznikova, E.; Dmitriev, S. An approach to evaluate the accuracy of interatomic potentials as applied to tungsten. Comp. Mater. Sci. 2024, 231, 112597. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO—The Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]
- Fukuda, M.; Yabuuchi, K.; Nogami, S.; Hasegawa, A.; Tanaka, T. Microstructural development of tungsten and tungsten–rhenium alloys due to neutron irradiation in HFIR. J. Nucl. Mater. 2014, 455, 460–463. [Google Scholar] [CrossRef]
- Nemoto, Y.; Hasegawa, A.; Satou, M.; Abe, K. Microstructural development of neutron irradiated W–Re alloys. J. Nucl. Mater. 2000, 283–287, 1144–1147. [Google Scholar] [CrossRef]
- Tanno, T.; Hasegawa, A.; Fujiwara, M.; He, J.C.; Nogami, S.; Satou, M.; Shishido, T.; Abe, K. Precipitation of solid transmutation elements in irradiated tungsten alloys. Mater. Trans. 2008, 49, 2259–2264. [Google Scholar] [CrossRef]
- Hwang, T.; Hasegawa, A.; Tomura, K.; Ebisawa, N.; Toyama, T.; Nagai, Y.; Fukuda, M.; Miyazawa, T.; Tanaka, T.; Nogami, S. Effect of neutron irradiation on rhenium cluster formation in tungsten and tungsten-rhenium alloys. J. Nucl. Mater. 2018, 507, 78–86. [Google Scholar] [CrossRef]
- Fukuda, M.; Tanno, T.; Nogami, S.; Hasegawa, A. Effects of Re content and fabrication process on microstructural changes and hardening in neutron irradiated tungsten. Mater. Trans. 2012, 53, 2145–2150. [Google Scholar] [CrossRef]
- Tanno, T.; Fukuda, M.; Nogami, S.; Hasegawa, A. Microstructure Development in Neutron Irradiated Tungsten Alloys. Mater. Trans. 2011, 52, 1447–1451. [Google Scholar] [CrossRef]
- Morkina, A.Y.; Babicheva, R.I.; Korznikova, E.A.; Enikeev, N.A.; Edalati, K.; Dmitriev, S.V. A Molecular Dynamics Simulation to Shed Light on the Mechanical Alloying of an Al-Zr Alloy Induced by Severe Plastic Deformation. Metals 2023, 13, 1595. [Google Scholar] [CrossRef]
- Peng, Q.; Huang, Z.; Chen, G.; Zhang, Y.; Zhang, X.; Chen, X.-J.; Hu, Z. Effect of Strain Rate, Temperature, Vacancy, and Microcracks on Mechanical Properties of 8-16-4 Graphyne. Nanomaterials 2024, 14, 556. [Google Scholar] [CrossRef] [PubMed]
- Bacon, D.J.; Kocks, U.F.; Scattergood, R.O. The effect of dislocation self-interaction on the Orowan stress. Philos. Mag. 1973, 28, 1241–1263. [Google Scholar] [CrossRef]
- Haghighat, H.S.M.; Lucas, G.; Schäublin, R. State of a pressurized helium bubble in iron. Europhys. Lett. 2009, 85, 60008. [Google Scholar] [CrossRef]

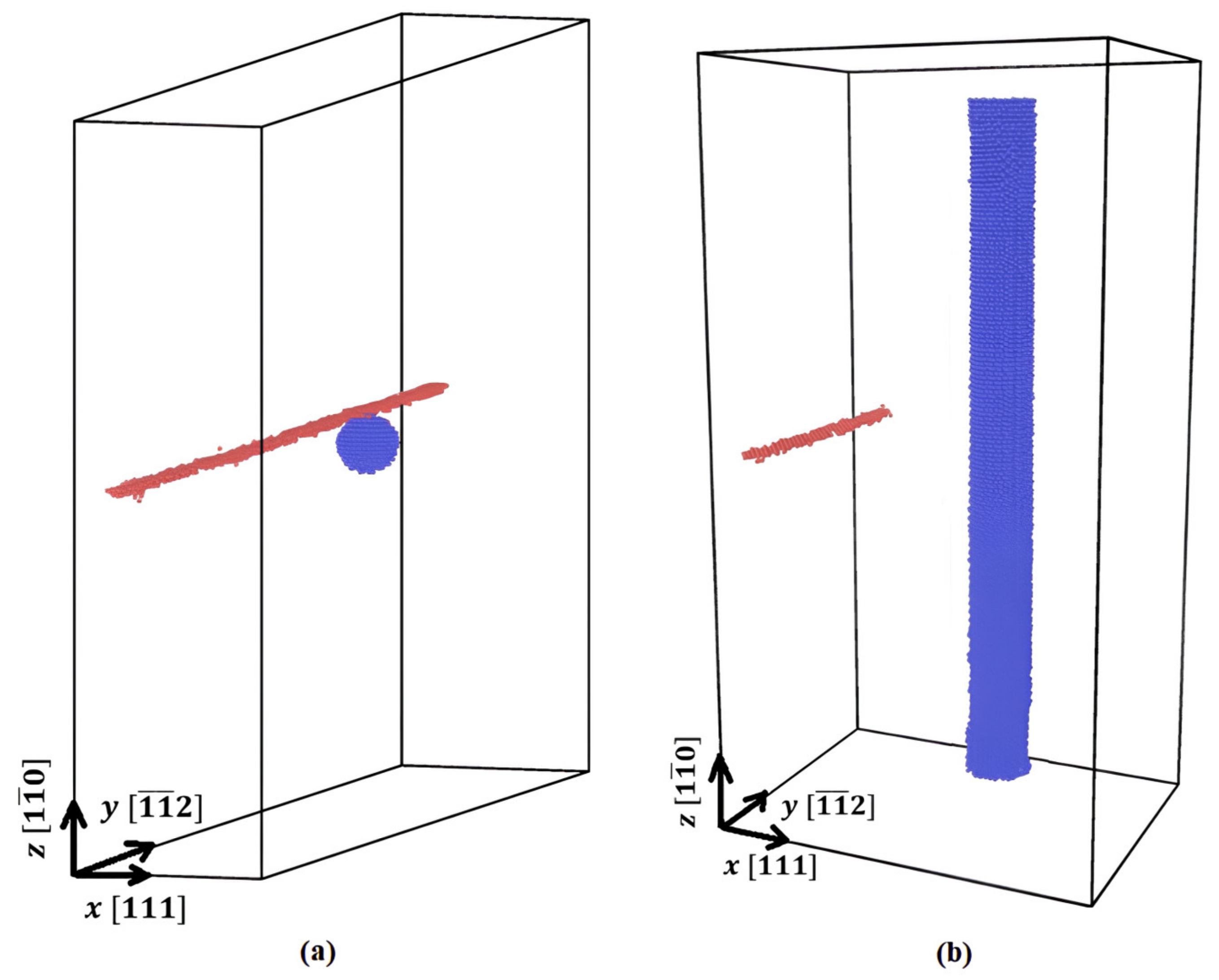

| NO | Lx, nm | Ly, nm | D, nm |
|---|---|---|---|
| Spherical particle | |||
| 1 | 10 | 55 | 3 |
| 2 | 20 | 55 | 3 |
| 3 | 20 | 55 | 5 |
| Cylindrical particle | |||
| 4 | 10 | 55 | 1 |
| 5 | 20 | 55 | 1 |
| 6 | 10 | 55 | 2 |
| 7 | 20 | 55 | 2 |
| 8 | 10 | 55 | 3 |
| 9 | 20 | 55 | 3 |
| 10 | 20 | 35 | 3 |
| 11 | 20 | 15 | 3 |
| NO | Lx, nm | Ly, nm | D, nm |
|---|---|---|---|
| Spherical particle | |||
| 1 | 10 | 55 | 3 |
| 2 | 20 | 55 | 3 |
| Cylindrical particle | |||
| 3 | 10 | 55 | 1 |
| 4 | 20 | 55 | 1 |
| 5 | 10 | 55 | 2 |
| 6 | 20 | 55 | 2 |
| 7 | 10 | 55 | 3 |
| 8 | 20 | 55 | 3 |
| 9 | 20 | 35 | 3 |
| 10 | 20 | 15 | 3 |
| NO | , MPa | ||||
|---|---|---|---|---|---|
| 600 K | 800 K | 1000 K | 1200 K | 1400 K | |
| Spherical particle | |||||
| 1 | 387 | 357 | 369 | 348 | 345 |
| 2 | 368 | 380 | 343 | 355 | 343 |
| 3 | 483 | 461 | 482 | 441 | 460 |
| Cylindrical particle | |||||
| 4 | 166 | 160 | 177 | 145 | 171 |
| 5 | 181 | 183 | 187 | 154 | 169 |
| 6 | 293 | 294 | 258 | 269 | 257 |
| 7 | 271 | 239 | 303 | 252 | 305 |
| 8 | 443 | 456 | 464 | 472 | 377 |
| 9 | 416 | 412 | 396 | 418 | 416 |
| 10 | 619 | 590 | 596 | 566 | 588 |
| 11 | 1424 | 1402 | 1381 | 1348 | 1230 |
| NO | , MPa | ||||
|---|---|---|---|---|---|
| 600 K | 800 K | 1000 K | 1200 K | 1400 K | |
| Spherical particle | |||||
| 1 | 336 | 332 | 267 | 328 | 228 |
| 2 | 293 | 289 | 293 | 303 | 289 |
| Cylindrical particle | |||||
| 3 | 144 | 114 | 117 | 128 | 105 |
| 4 | 137 | 131 | 149 | 144 | 147 |
| 5 | 237 | 267 | 245 | 257 | 249 |
| 6 | 248 | 241 | 261 | 253 | 233 |
| 7 | 308 | 299 | 330 | 297 | 261 |
| 8 | 274 | 287 | 292 | 254 | 286 |
| 9 | 516 | 487 | 455 | 393 | 469 |
| 10 | 1015 | 1010 | 1020 | 1011 | 1008 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sharapova, Y.R.; Kazakov, A.M.; Babicheva, R.I.; Semenov, A.S.; Izosimov, A.A.; Korznikova, E.A. Dislocation Interactions with Hcp- and χ-Phase Particles in Tungsten: Molecular Dynamics Insights into Mechanical Strengthening Mechanisms. Computation 2024, 12, 168. https://doi.org/10.3390/computation12080168
Sharapova YR, Kazakov AM, Babicheva RI, Semenov AS, Izosimov AA, Korznikova EA. Dislocation Interactions with Hcp- and χ-Phase Particles in Tungsten: Molecular Dynamics Insights into Mechanical Strengthening Mechanisms. Computation. 2024; 12(8):168. https://doi.org/10.3390/computation12080168
Chicago/Turabian StyleSharapova, Yu. R., A. M. Kazakov, R. I. Babicheva, A. S. Semenov, A. A. Izosimov, and E. A. Korznikova. 2024. "Dislocation Interactions with Hcp- and χ-Phase Particles in Tungsten: Molecular Dynamics Insights into Mechanical Strengthening Mechanisms" Computation 12, no. 8: 168. https://doi.org/10.3390/computation12080168
APA StyleSharapova, Y. R., Kazakov, A. M., Babicheva, R. I., Semenov, A. S., Izosimov, A. A., & Korznikova, E. A. (2024). Dislocation Interactions with Hcp- and χ-Phase Particles in Tungsten: Molecular Dynamics Insights into Mechanical Strengthening Mechanisms. Computation, 12(8), 168. https://doi.org/10.3390/computation12080168









