Stability Evaluations of Unlined Horseshoe Tunnels Based on Extreme Learning Neural Network
Abstract
:1. Introduction
2. Problem Statement
3. Method of Analysis
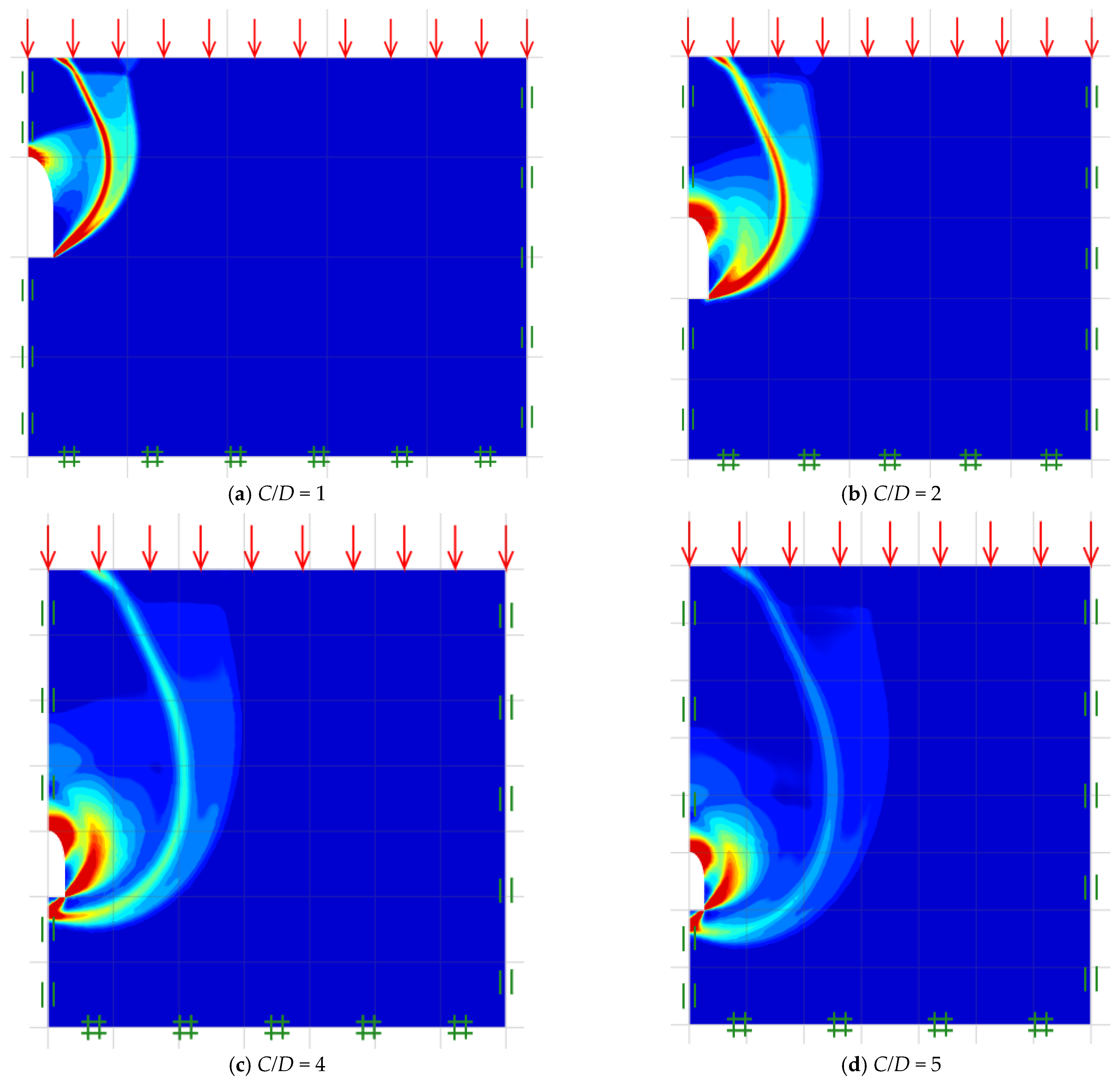
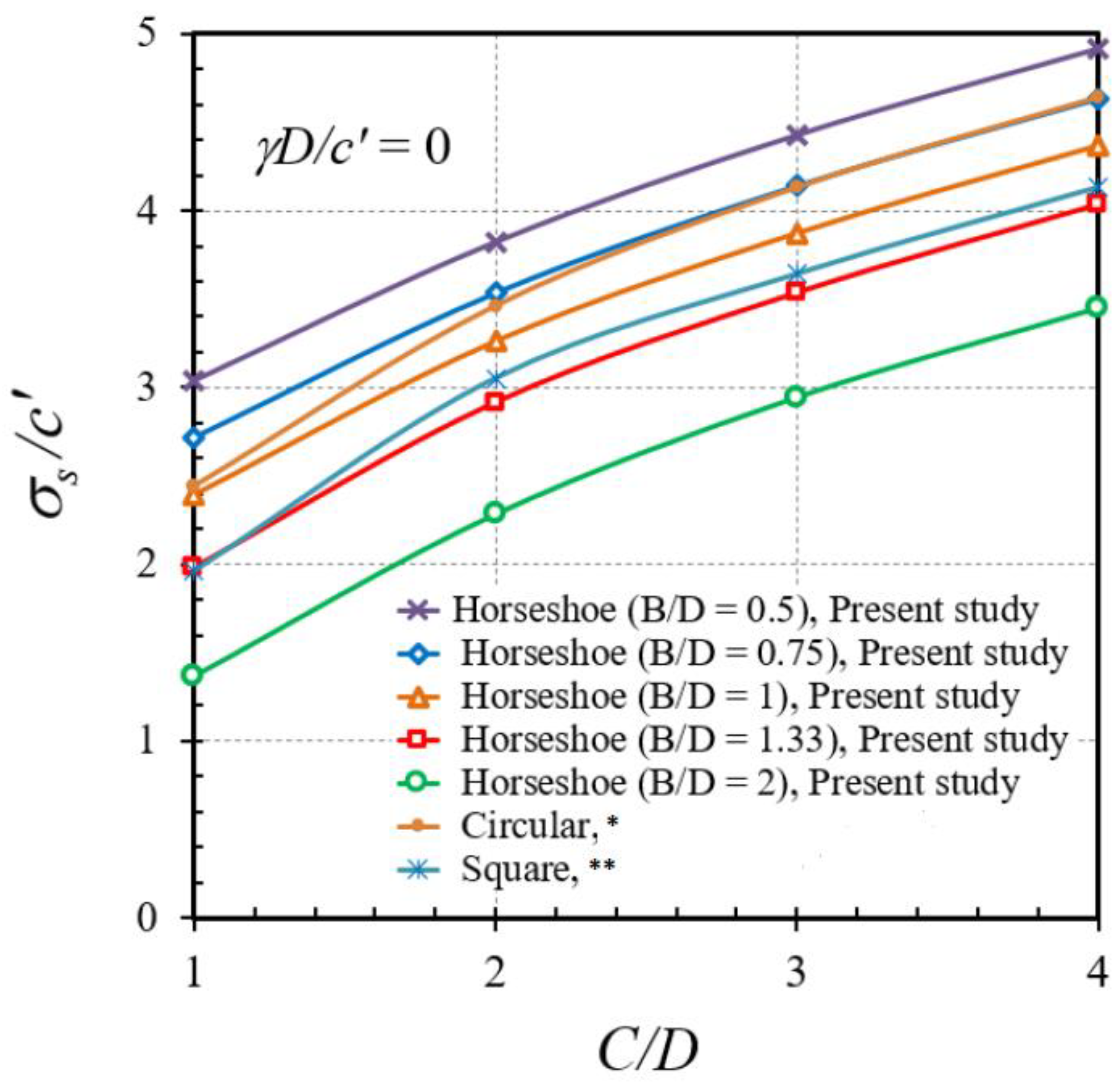
4. Results and Discussion
5. Proposed Predictive Models
5.1. Multiple Linear Regression
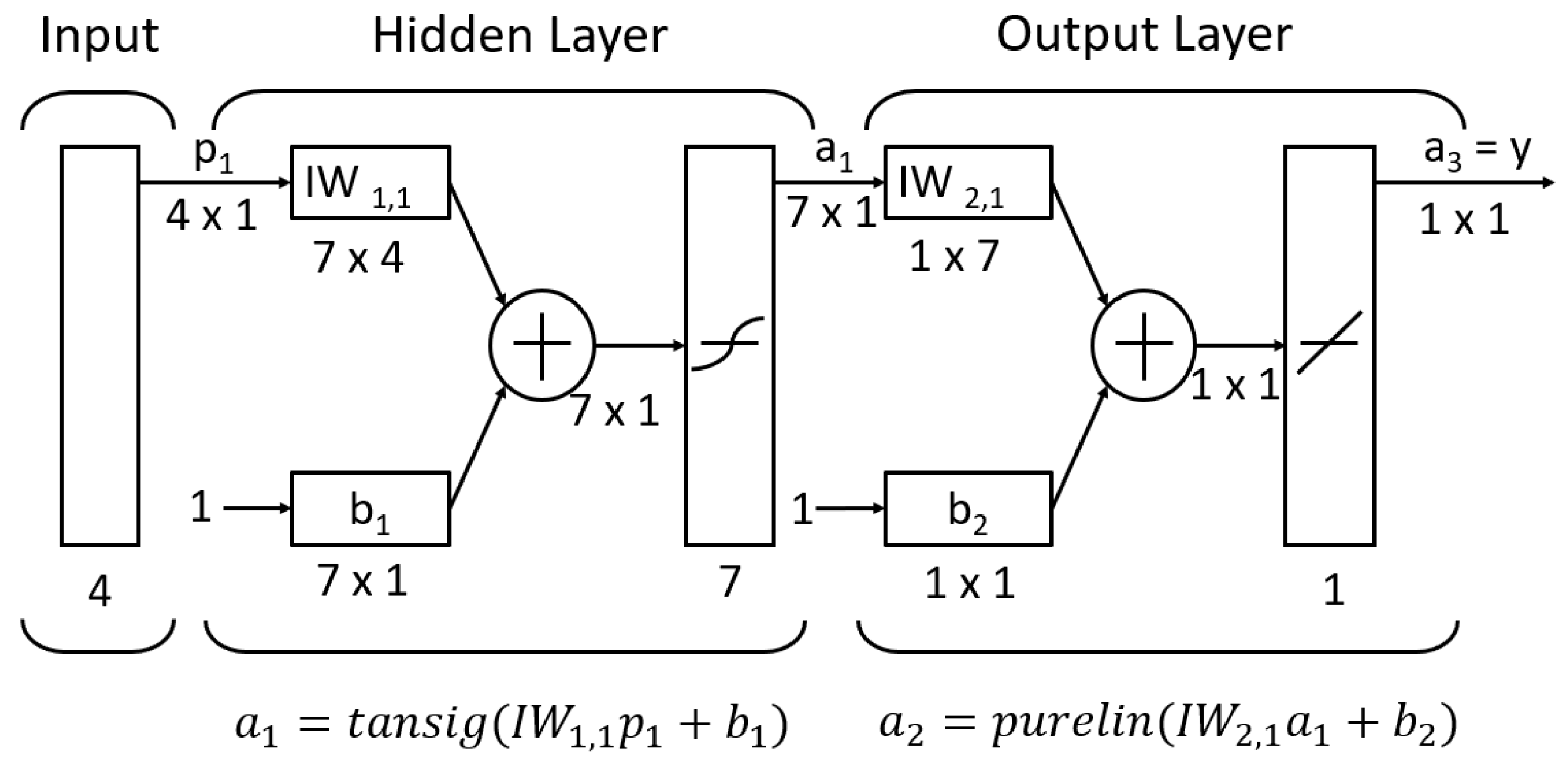
5.2. Artificial Neural Network (ANN)
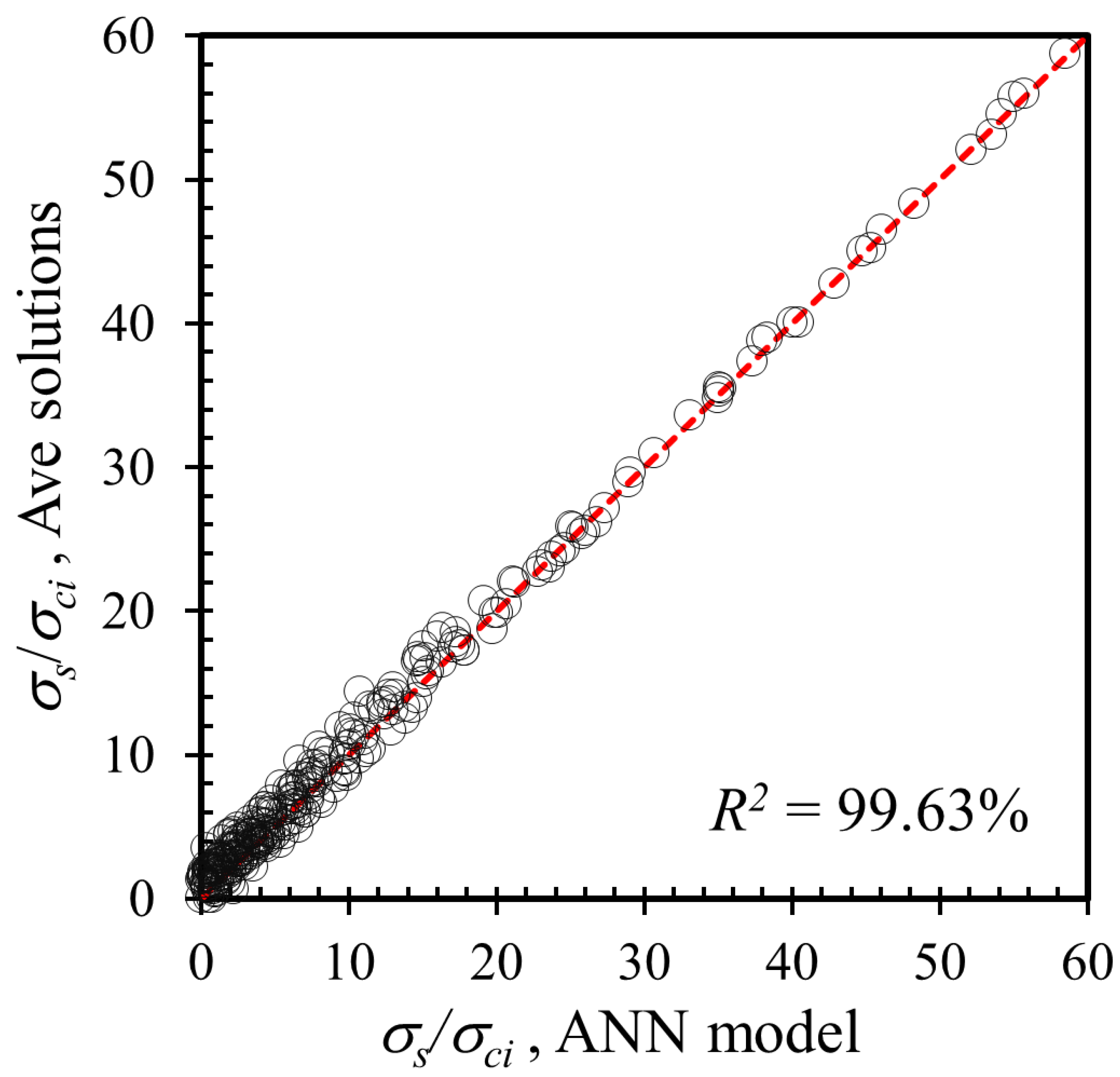
5.3. Cross-Validation and Performance Measures
5.4. Predictive Equations
5.4.1. Multiple Linear Regression
5.4.2. Artificial Neural Network
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sloan, S.; Assadi, A. Undrained stability of a plane strain heading. Can. Geotech. J. 1994, 31, 443–450. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S.; Yingchaloenkitkhajorn, K. Undrained face stability of tunnels in Bangkok subsoils. Int. J. Geotech. Eng. 2017, 11, 262–277. [Google Scholar] [CrossRef]
- Mair, R.J. Centrifugal Modelling of Tunnel Construction in Soft Clay. Ph.D. Thesis, Cambridge University, Cambridge, UK, 1979. [Google Scholar] [CrossRef]
- Shiau, J.; Al-Asadi, F. Three-Dimensional Analysis of Circular Tunnel Headings Using Broms and Bennermark’s Original Stability Number. Int. J. Geomech. 2020, 20, 06020015. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Lower bound stability analysis of plane strain headings in Hoek-Brown rock masses. Tunn. Undergr. Space Technol. 2019, 84, 99–112. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Stability of Retained Soils Behind Underground Walls with an Opening Using Lower Bound Limit Analysis and Second-Order Cone Programming. Geotech. Geol. Eng. 2019, 37, 1609–1625. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Design equations for undrained stability of opening in underground walls. Tunn. Undergr. Space Technol. 2017, 70, 214–220. [Google Scholar] [CrossRef]
- Fraldi, M.; Guarracino, F. Limit analysis of collapse mechanisms in cavities and tunnels according to the Hoek–Brown failure criterion. Int. J. Rock Mech. Min. Sci. 2009, 46, 665–673. [Google Scholar] [CrossRef]
- Davis, E.H.; Gunn, M.J.; Mair, R.J.; Seneviratine, H.N. The stability of shallow tunnels and underground openings in cohesive material. Geotechnique 1980, 30, 397–416. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Lower bound solutions for undrained face stability of plane strain tunnel headings in anisotropic and non-homogeneous clays. Comput. Geotech. 2019, 112, 204–217. [Google Scholar] [CrossRef]
- Ukritchon, B.; Yingchaloenkitkhajorn, K.; Keawsawasvong, S. Three-dimensional undrained tunnel face stability in clay with a linearly increasing shear strength with depth. Comput. Geotech. 2017, 88, 146–151. [Google Scholar] [CrossRef]
- Drucker, D.C.; Prager, W.; Greenberg, H.J. Extended limit design theorems for continuous media. Q. Appl. Math. 1952, 9, 381–389. [Google Scholar] [CrossRef] [Green Version]
- Chen, W.F.; Liu, X.L. Limit Analysis in Soil Mechanics; Elsevier: Amsterdam, The Netherlands, 1990. [Google Scholar]
- Sloan, S. Geotechnical stability analysis. Géotechnique 2013, 63, 531–571. [Google Scholar] [CrossRef] [Green Version]
- Sloan, S.W.; Assadi, A. Undrained stability of a square tunnel whose strength increases linearly with depth. Comput. Geotech. 1991, 12, 321–346. [Google Scholar] [CrossRef]
- Wilson, D.W.; Abbo, A.J.; Sloan, S.W.; Lyamin, A.V. Undrained stability of a circular tunnel where the shear strength increases linearly with depth. Can. Geotech. J. 2011, 48, 1328–1342. [Google Scholar] [CrossRef]
- Abbo, A.J.; Wilson, D.W.; Sloan, S.; Lyamin, A. Undrained stability of wide rectangular tunnels. Comput. Geotech. 2013, 53, 46–59. [Google Scholar] [CrossRef]
- Wilson, D.W.; Abbo, A.J.; Sloan, S.W. Undrained stability of tall tunnels. In Proceedings of the 14th International Conference of International Association for Computer Methods and Recent Advances in Geomechanics, Kyoto, Japan, 24 September 2014. [Google Scholar]
- Yamamoto, K.; Lyamin, A.; Wilson, D.W.; Sloan, S.; Abbo, A. Stability of a circular tunnel in cohesive-frictional soil subjected to surcharge loading. Comput. Geotech. 2011, 38, 504–514. [Google Scholar] [CrossRef]
- Yamamoto, K.; Lyamin, A.; Wilson, D.W.; Sloan, S.; Abbo, A. Stability of a single tunnel in cohesive–frictional soil subjected to surcharge loading. Can. Geotech. J. 2011, 48, 1841–1854. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Undrained stability of plane strain active trapdoors in anisotropic and non-homogeneous clays. Tunn. Undergr. Space Technol. 2021, 107, 103628. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Design equation for stability of shallow unlined circular tunnels in Hoek-Brown rock masses. Bull. Eng. Geol. Environ. 2020, 79, 4167–4190. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Likitlersuang, S. Undrained stability of active trapdoors in two-layered clays. Undergr. Space 2021, 6, 446–454. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Shiau, J. Stability of active trapdoors in axisymmetry. Undergr. Space 2022, 7, 50–57. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Stability of unlined square tunnels in Hoek-Brown rock masses based on lower bound analysis. Comput. Geotech. 2019, 105, 249–264. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Undrained Stability of Unlined Square Tunnels in Clays with Linearly Increasing Anisotropic Shear Strength. Geotech. Geol. Eng. 2020, 38, 897–915. [Google Scholar] [CrossRef]
- Yang, F.; Zhang, J.; Yang, J.; Zhao, L.; Zheng, X. Stability analysis of unlined elliptical tunnel using finite element upper-bound method with rigid translatory moving elements. Tunn. Undergr. Space Technol. 2015, 50, 13–22. [Google Scholar] [CrossRef]
- Yang, F.; Sun, X.; Zheng, X.; Yang, J. Stability analysis of a deep buried elliptical tunnel in cohesive–frictional (c–ϕ) soils with a nonassociated flow rule. Can. Geotech. J. 2017, 54, 736–741. [Google Scholar] [CrossRef]
- Yang, F.; Sun, X.; Zou, J.; Zheng, X. Analysis of an elliptical tunnel affected by surcharge loading. Proc. Inst. Civ. Eng.-Geotech. Eng. 2019, 172, 312–319. [Google Scholar] [CrossRef]
- Zhang, J.; Yang, J.; Yang, F.; Zhang, X.; Zheng, X. Upper-Bound Solution for Stability Number of Elliptical Tunnel in Cohesionless Soils. Int. J. Geomech. 2017, 17, 06016011. [Google Scholar] [CrossRef]
- Dutta, P.; Bhattacharya, P. Determination of internal pressure for the stability of dual elliptical tunnels in soft clay. Geomech. Geoengin. 2021, 16, 67–79. [Google Scholar] [CrossRef]
- Di, Q.; Li, P.; Zhang, M.; Guo, C.; Wang, F.; Wei, Y. Evaluation of Tunnel Face Stability Subjected to Seismic Load Based on the Non-associated Flow Rule. KSCE J. Civ. Eng. 2022, 26, 2478–2489. [Google Scholar] [CrossRef]
- Zhang, M.; Di, Q.; Li, P.; Wei, Y.; Wang, F. Influence of non-associated flow rule on face stability for tunnels in cohesive–frictional soils. Tunn. Undergr. Space Technol. 2022, 121, 104320. [Google Scholar] [CrossRef]
- Zheng, H.; Li, P.; Ma, G.; Zhang, Q. Experimental investigation of mechanical characteristics for linings of twins tunnels with asymmetric cross-section. Tunn. Undergr. Space Technol. 2022, 119, 104209. [Google Scholar] [CrossRef]
- Li, Y.; Zhou, G.; Tang, C.; Wang, S.; Wang, K.; Wang, T. Influence of undercrossing tunnel excavation on the settlement of a metro station in Dalian. Bull. Eng. Geol. Environ. 2021, 80, 4673–4687. [Google Scholar] [CrossRef]
- He, Z.; Li, C.; He, Q.; Liu, Y.; Chen, J. Numerical Parametric Study of Countermeasures to Alleviate the Tunnel Excavation Effects on an Existing Tunnel in a Shallow-Buried Environment near a Slope. Appl. Sci. 2020, 10, 608. [Google Scholar] [CrossRef] [Green Version]
- Ng, C.W.W.; Wang, R.; Boonyarak, T. A comparative study of the different responses of circular and horseshoe-shaped tunnels to an advancing tunnel underneath. Geotech. Lett. 2016, 6, 168–175. [Google Scholar] [CrossRef]
- Zhang, J.; Feng, T.; Yang, J.; Yang, F.; Gao, Y. Upper-bound stability analysis of dual unlined horseshoe-shaped tunnels subjected to gravity. Comput. Geotech. 2018, 97, 103–110. [Google Scholar] [CrossRef]
- Bhattacharya, P.; Sriharsha, P. Stability of Horseshoe Tunnel in Cohesive-Frictional Soil. Int. J. Geomech. 2020, 20, 06020021. [Google Scholar] [CrossRef]
- Li, A.; Khoo, S.; Lyamin, A.; Wang, Y. Rock slope stability analyses using extreme learning neural network and terminal steepest descent algorithm. Autom. Constr. 2016, 65, 42–50. [Google Scholar] [CrossRef]
- Li, A.-J.; Lim, K.; Fatty, A. Stability evaluations of three-layered soil slopes based on extreme learning neural network. J. Chin. Inst. Eng. 2020, 43, 628–637. [Google Scholar] [CrossRef]
- Qian, Z.; Li, A.; Chen, W.; Lyamin, A.; Jiang, J. An artificial neural network approach to inhomogeneous soil slope stability predictions based on limit analysis methods. Soils Found. 2019, 59, 556–569. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Seehavong, S.; Ngamkhanong, C. Application of artificial neural networks for predicting the stability of rectangular tunnel in Hoek-Brown rock masses. Front. Built Environ. 2022, 8, 837745. [Google Scholar] [CrossRef]
- Butterfield, R. Dimensional analysis for geotechnical engineering. Géotechnique 1999, 49, 357–366. [Google Scholar] [CrossRef]
- Krabbenhoft, K.; Lyamin, A.; Krabbenhoft, J. Optum Computational Engineering (OptumG2 Version G2 2020_2020.08.17). Available online: https://www.optumce.com (accessed on 1 July 2020).
- Ukritchon, B.; Keawsawasvong, S. Error in Ito and Matsui’s limit equilibrium solution of lateral force on a row of stabilizing piles. J. Geotech. Geoenviron. Eng. 2017, 143, 02817004. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. A new design equation for drained stability of conical slopes in cohesive-frictional soils. J. Rock Mech. Geotech. Eng. 2018, 10, 358–366. [Google Scholar] [CrossRef]
- Ukritchon, B.; Wongtoythong, P.; Keawsawasvong, S. New design equation for undrained pullout capacity of suction caissons considering combined effects of caisson aspect ratio, adhesion factor at interface, and linearly increasing strength. Appl. Ocean Res. 2018, 75, 1–14. [Google Scholar] [CrossRef]
- Ukritchon, B.; Keawsawasvong, S. Design equations of uplift capacity of circular piles in sands. Appl. Ocean. Res. 2019, 90, 101844. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Undrained basal stability of braced circular excavations in non-homogeneous clays with linear increase of strength with depth. Comput. Geotech. 2019, 115, 103180. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Ukritchon, B. Undrained stability of a spherical cavity in cohesive soils using finite element limit analysis. J. Rock Mech. Geotech. Eng. 2019, 11, 1274–1285. [Google Scholar] [CrossRef]
- Ukritchon, B.; Yoang, S.; Keawsawasvong, S. Three-dimensional stability analysis of the collapse pressure on flexible pavements over rectangular trapdoors. Transp. Geotech. 2019, 21, 100277. [Google Scholar] [CrossRef]
- Ukritchon, B.; Yoang, S.; Keawsawasvong, S. Undrained stability of unsupported rectangular excavations in non-homogeneous clays. Comput. Geotech. 2020, 117, 103281. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Lai, V.Q. End Bearing Capacity Factor for Annular Foundations Embedded in Clay Considering the Effect of the Adhesion Factor. Int. J. Geosynth. Ground Eng. 2021, 7, 15. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Thongchom, C.; Likitlersuang, S. Bearing Capacity of Strip Footing on Hoek-Brown Rock Mass Subjected to Eccentric and Inclined Loading. Transp. Infrastruct. Geotechnol. 2021, 8, 189–202. [Google Scholar] [CrossRef]
- Yodsomjai, W.; Keawsawasvong, S.; Likitlersuang, S. Stability of Unsupported Conical Slopes in Hoek-Brown Rock Masses. Transp. Infrastruct. Geotechnol. 2021, 8, 279–295. [Google Scholar] [CrossRef]
- Yodsomjai, W.; Keawsawasvong, S.; Senjuntichai, T. Undrained Stability of Unsupported Conical Slopes in Anisotropic Clays Based on Anisotropic Undrained Shear Failure Criterion. Transp. Infrastruct. Geotechnol. 2021, 8, 557–568. [Google Scholar] [CrossRef]
- Keawsawasvong, S.; Yoonirundorn, K.; Senjuntichai, T. Pullout Capacity Factor for Cylindrical Suction Caissons in Anisotropic Clays Based on Anisotropic Undrained Shear Failure Criterion. Transp. Infrastruct. Geotechnol. 2021, 8, 629–644. [Google Scholar] [CrossRef]
- Ciria, H.; Peraire, J.; Bonet, J. Mesh adaptive computation of upper and lower bounds in limit analysis. Int. J. Numer. Methods Eng. 2008, 75, 899–944. [Google Scholar] [CrossRef]


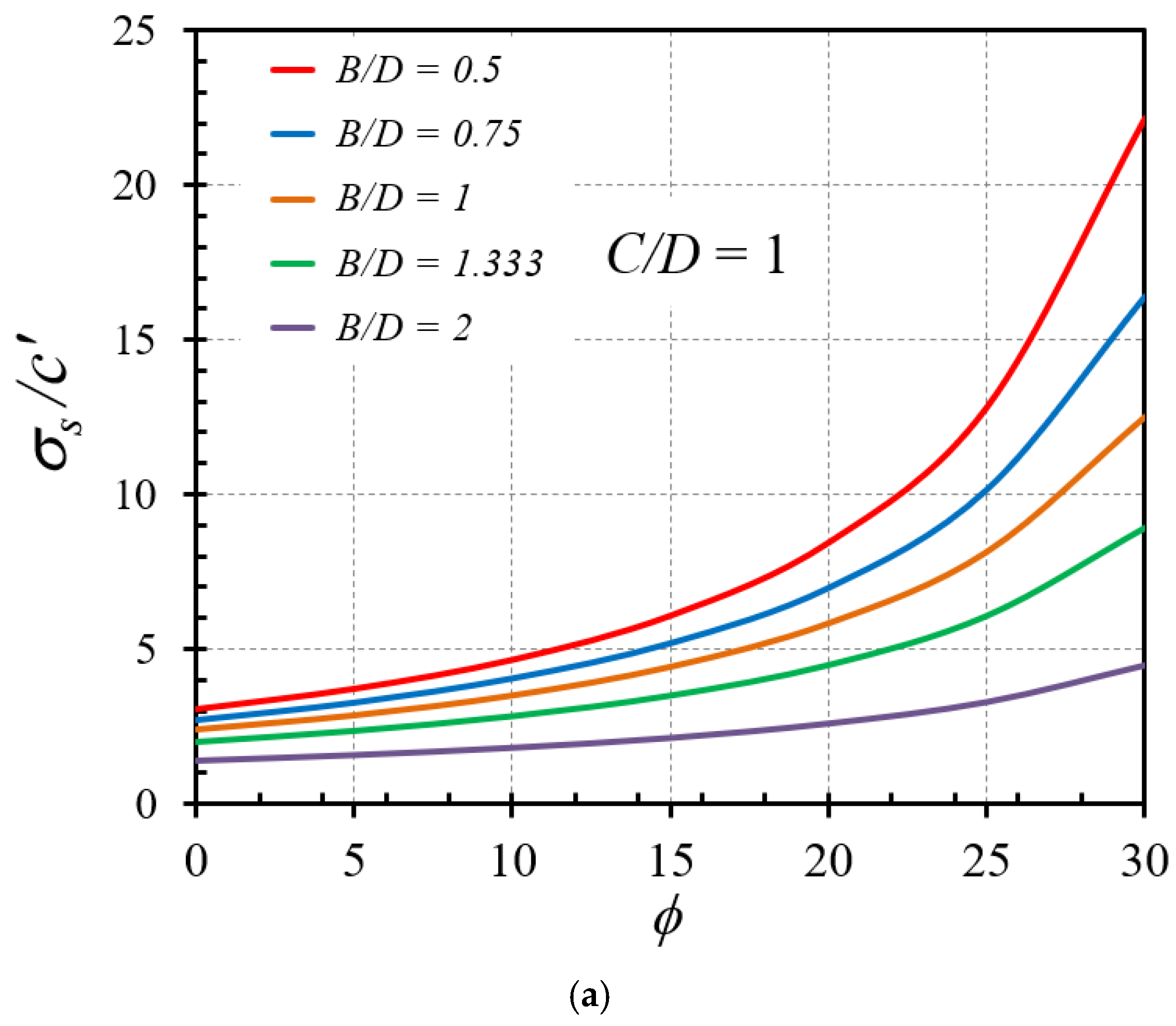

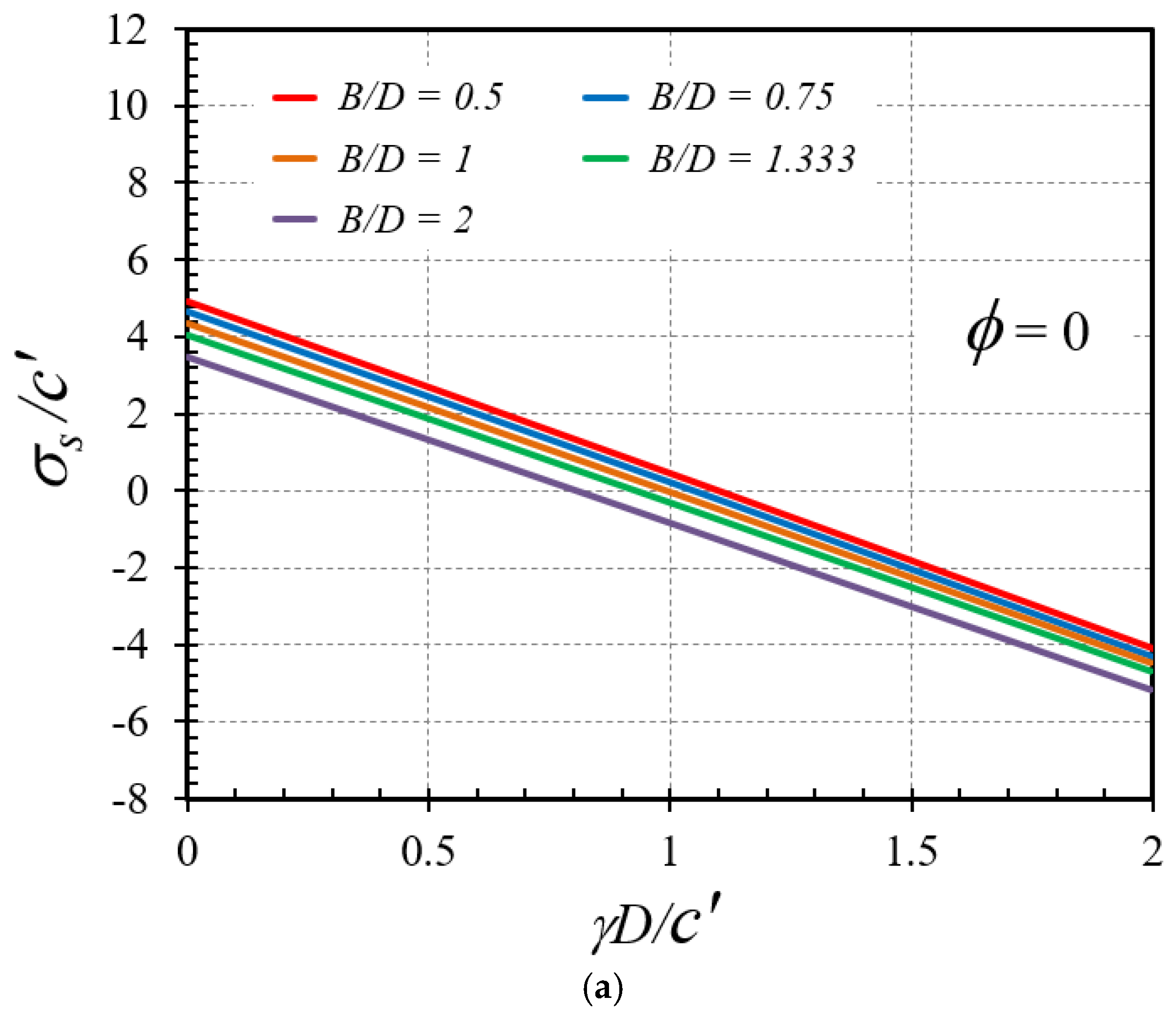

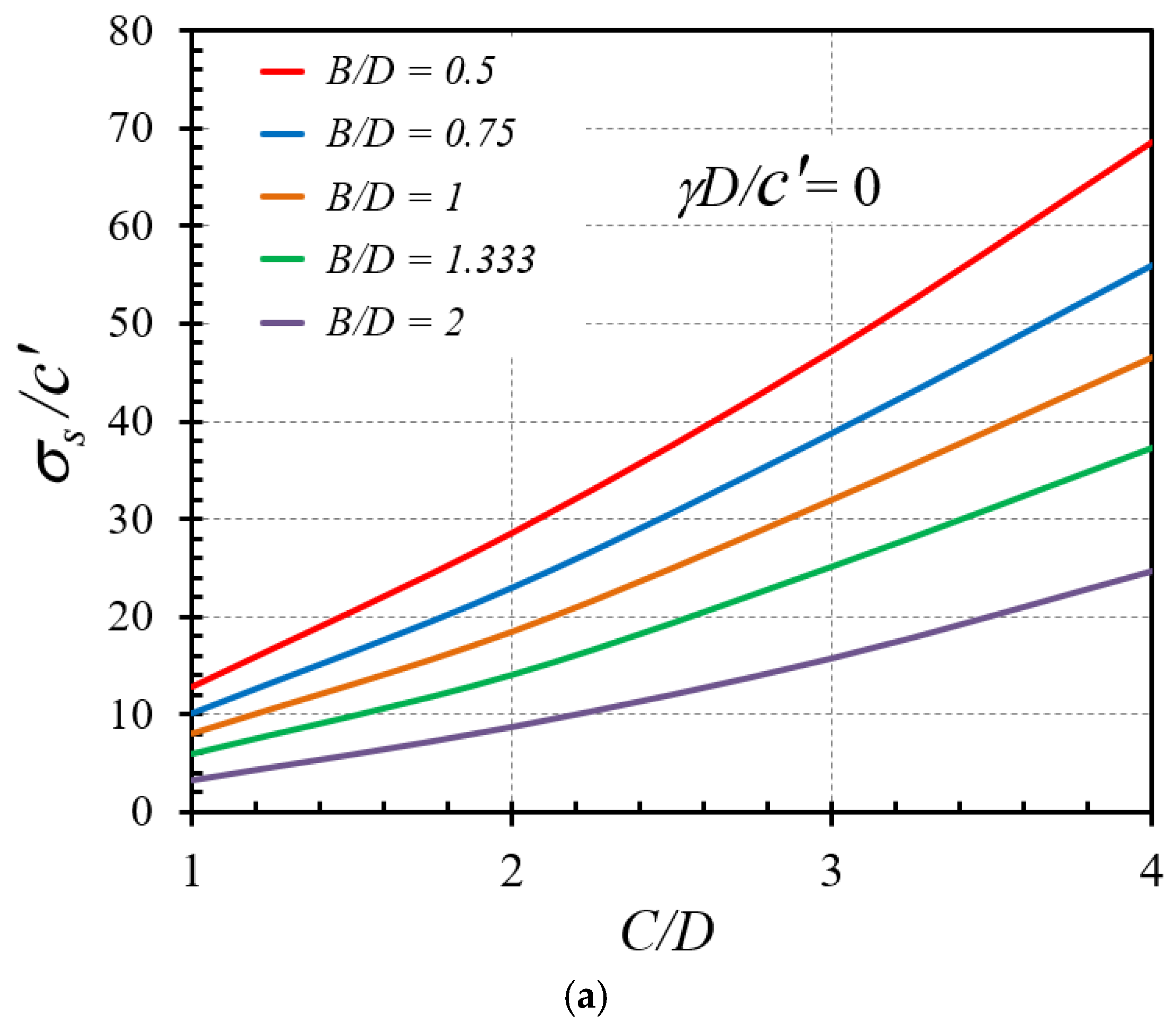
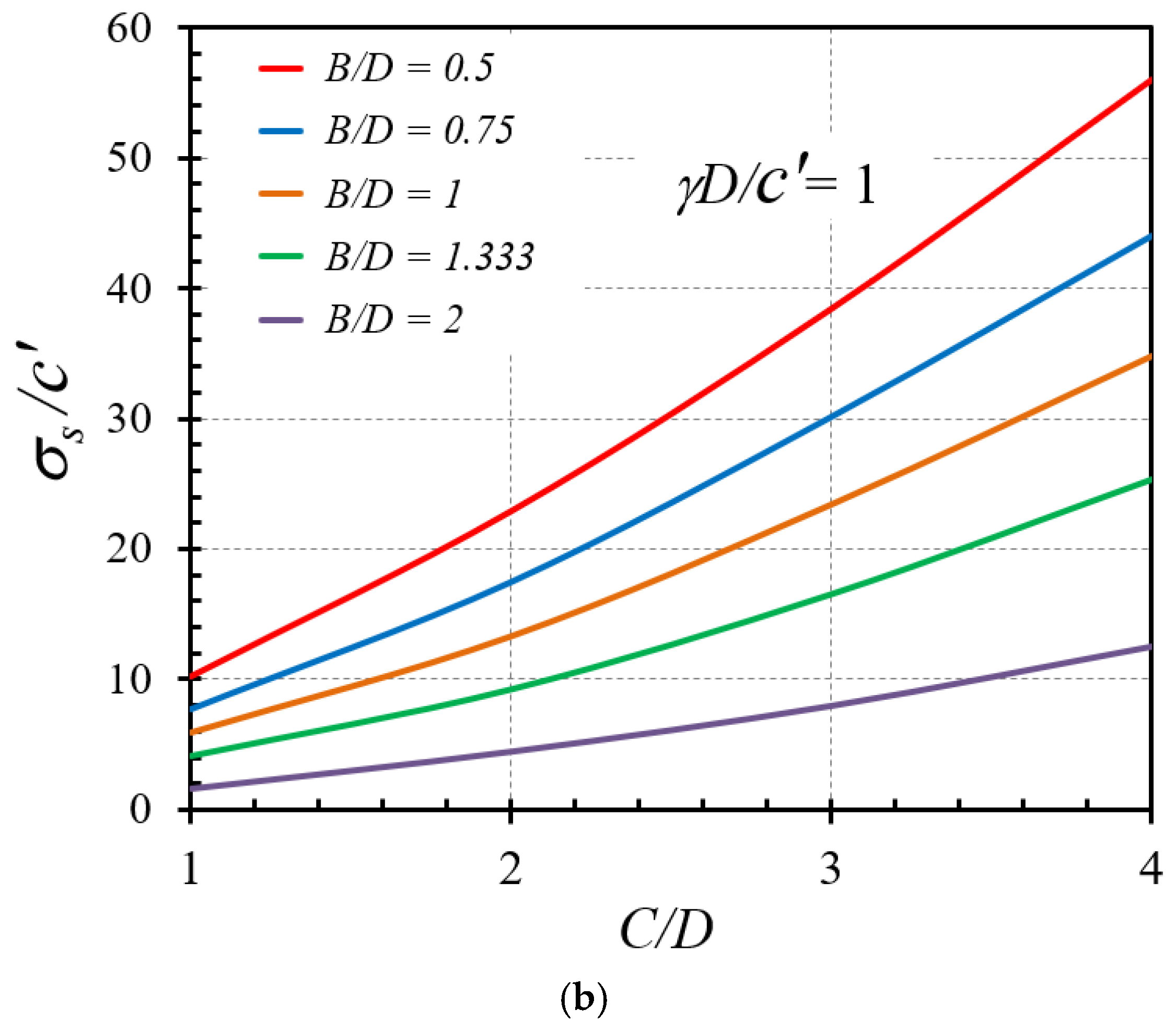


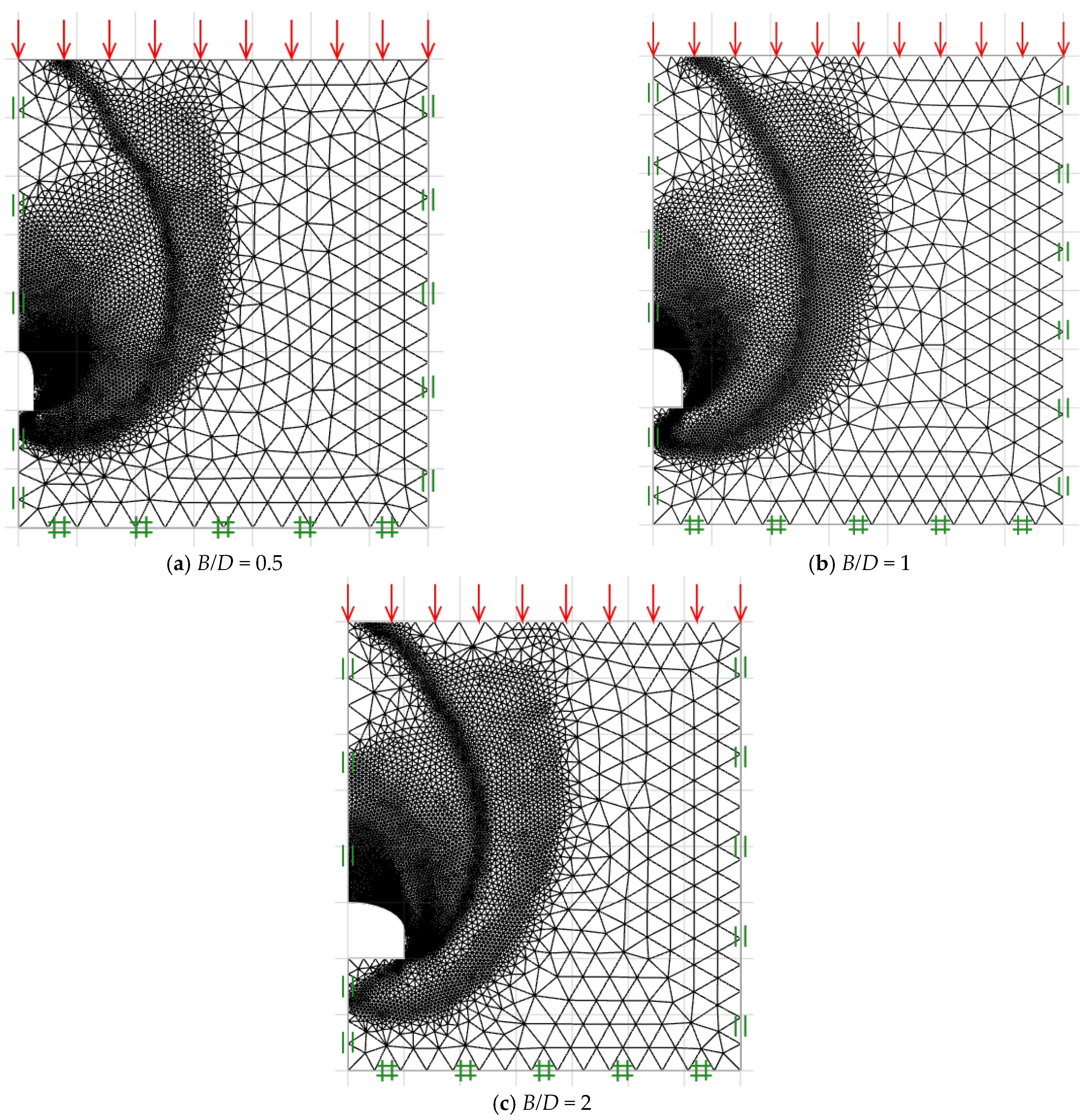
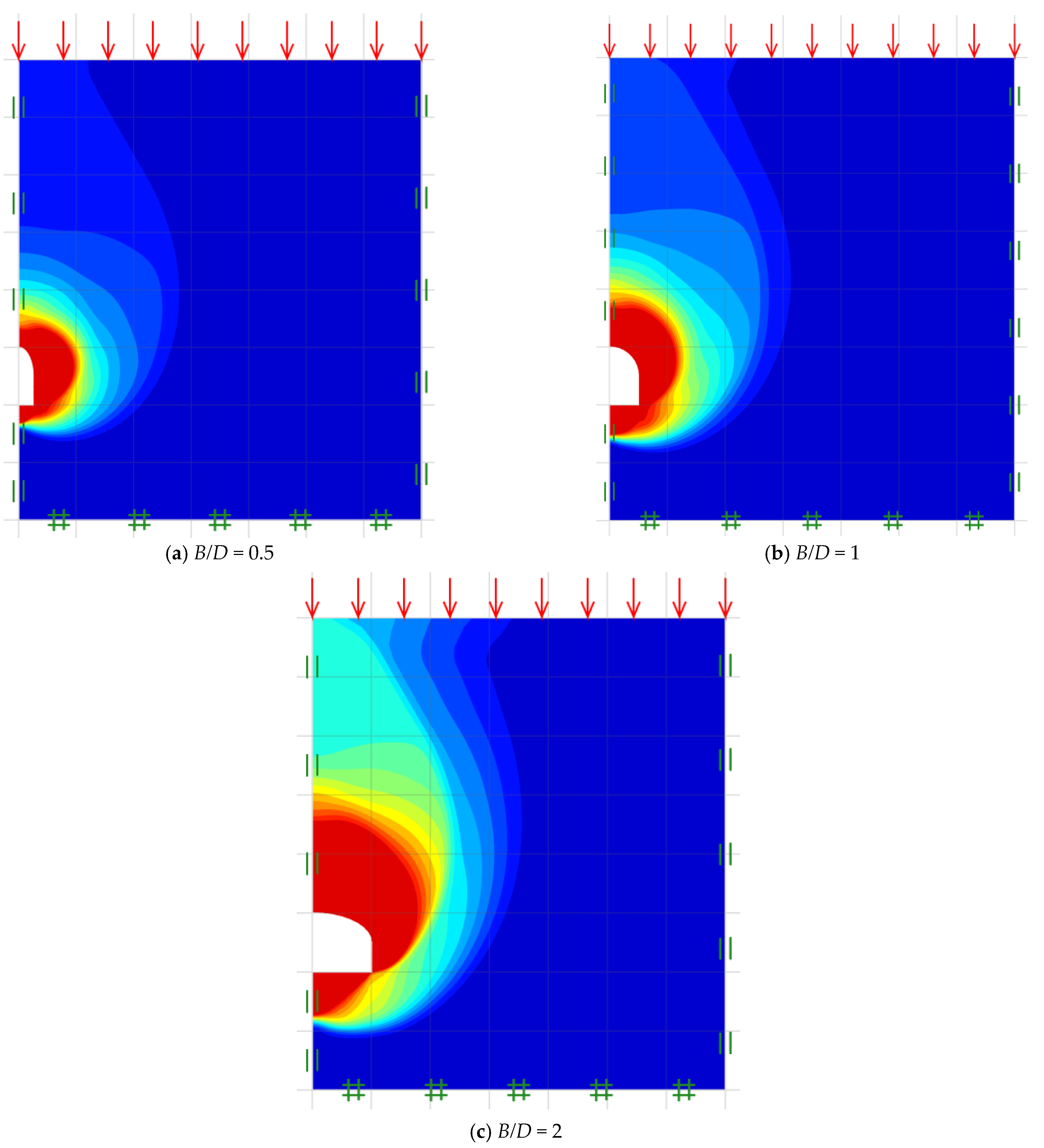
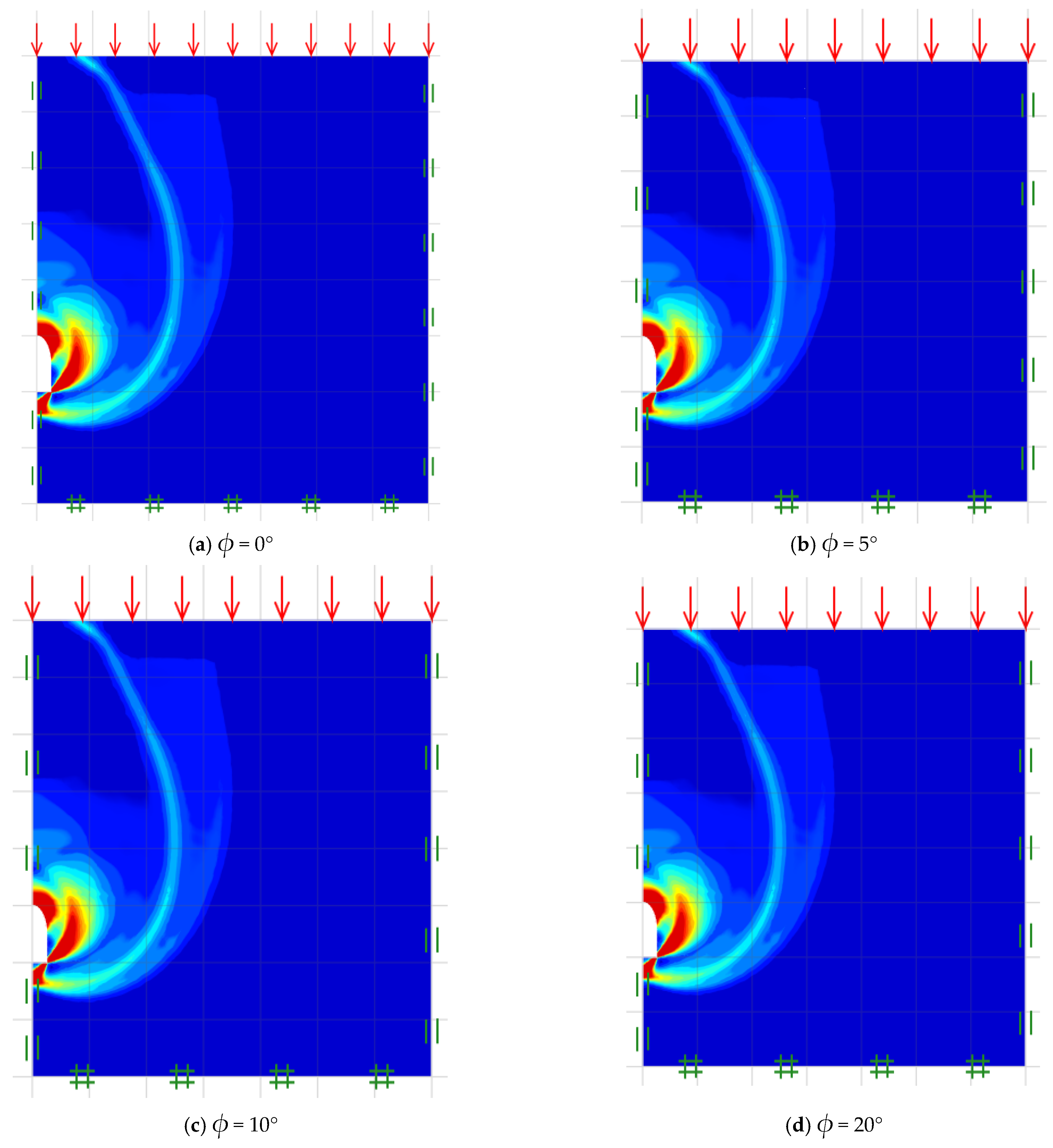
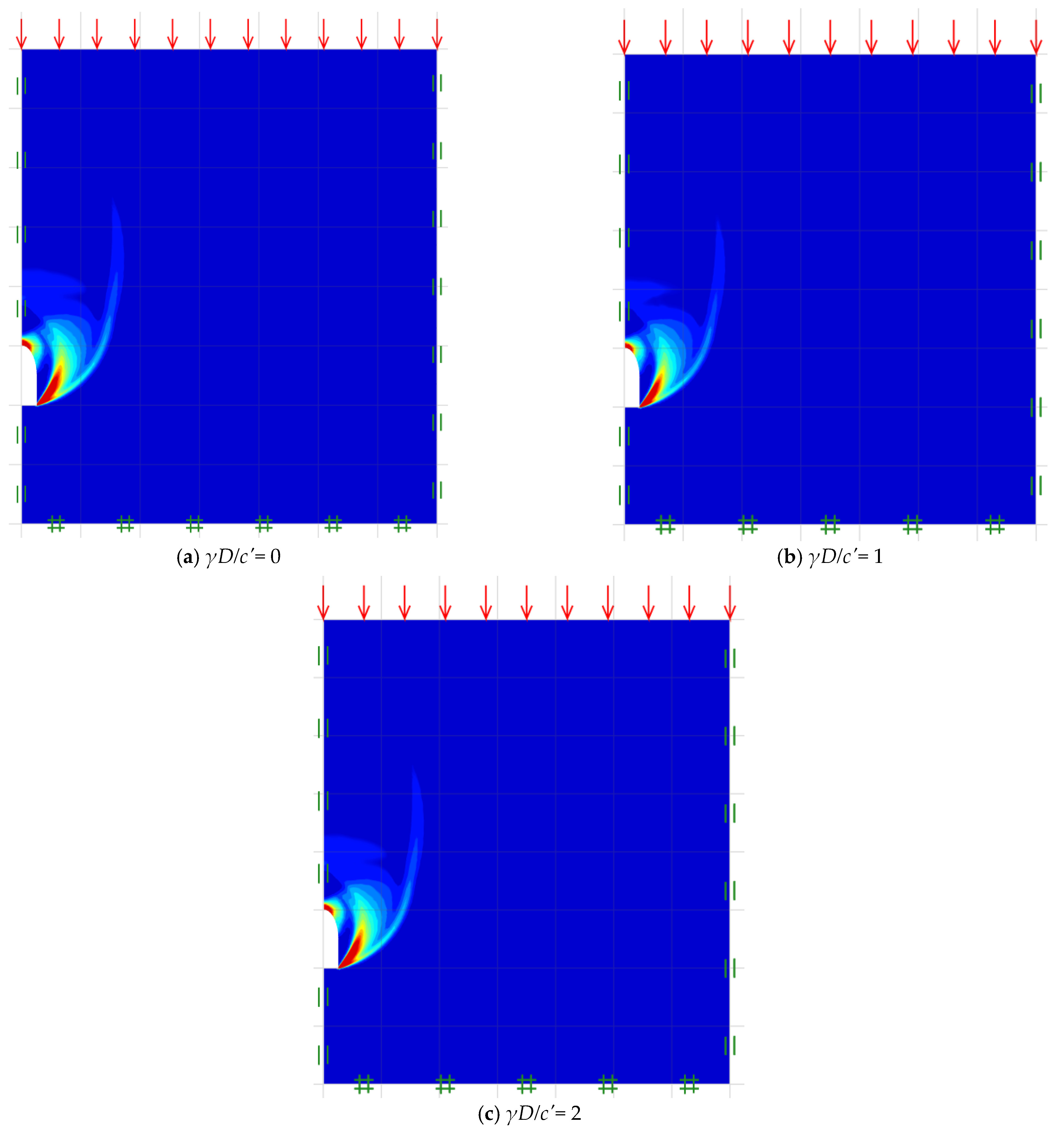


| Input Parameters | Values | Average |
|---|---|---|
| C/D | 1, 2, 3, 4 | 2.5 |
| B/D | 0.5, 0.75, 1, 1.33, 2 | 1.116 |
| γD/c′ | 0, 1, 2 | 1 |
| ϕ | 0°, 5°, 10°, 15°, 20°, 25°, 30° | 15° |
| γD/c′ | B/D | ϕ | C/D = 1 | C/D = 2 | C/D = 3 | C/D = 4 |
|---|---|---|---|---|---|---|
| 0 | 0.5 | 0 | 3.04 | 3.821 | 4.425 | 4.912 |
| 5 | 3.7005 | 4.92 | 5.934 | 6.797 | ||
| 10 | 4.6385 | 6.6645 | 8.4965 | 10.08 | ||
| 15 | 6.07 | 9.689 | 13.2485 | 16.448 | ||
| 20 | 8.4375 | 15.551 | 23.156 | 30.5665 | ||
| 25 | 12.818 | 28.57 | 47.2085 | 68.587 | ||
| 30 | 22.1785 | 62.7385 | 121.257 | 200.599 | ||
| 0.75 | 0 | 2.714 | 3.533 | 4.1405 | 4.635 | |
| 5 | 3.277 | 4.4865 | 5.4775 | 6.3235 | ||
| 10 | 4.061 | 5.9455 | 7.6755 | 9.2275 | ||
| 15 | 5.1965 | 8.4315 | 11.664 | 14.677 | ||
| 20 | 6.9845 | 13.068 | 19.8125 | 26.3335 | ||
| 25 | 10.156 | 22.9915 | 38.7855 | 55.9345 | ||
| 30 | 16.391 | 47.763 | 92.0795 | 151.6985 | ||
| 1 | 0 | 2.3925 | 3.2595 | 3.8705 | 4.3655 | |
| 5 | 2.8495 | 4.0845 | 5.0485 | 5.8775 | ||
| 10 | 3.4925 | 5.3325 | 6.9585 | 8.4395 | ||
| 15 | 4.4195 | 7.3585 | 10.3445 | 13.159 | ||
| 20 | 5.828 | 10.9975 | 17.052 | 22.913 | ||
| 25 | 8.136 | 18.521 | 31.9775 | 46.489 | ||
| 30 | 12.5025 | 36.556 | 72.2805 | 117.8115 | ||
| 1.33 | 0 | 1.982 | 2.915 | 3.5345 | 4.0335 | |
| 5 | 2.3405 | 3.606 | 4.5365 | 5.3415 | ||
| 10 | 2.815 | 4.6055 | 6.1085 | 7.4955 | ||
| 15 | 3.482 | 6.184 | 8.802 | 11.4065 | ||
| 20 | 4.4695 | 8.8815 | 13.938 | 19.237 | ||
| 25 | 6.0595 | 14.107 | 25.141 | 37.231 | ||
| 30 | 8.9065 | 25.7225 | 53.0995 | 87.328 | ||
| 2 | 0 | 1.369 | 2.2835 | 2.9445 | 3.449 | |
| 5 | 1.5525 | 2.7525 | 3.683 | 4.4385 | ||
| 10 | 1.7905 | 3.431 | 4.7755 | 5.9915 | ||
| 15 | 2.1115 | 4.4345 | 6.5385 | 8.6405 | ||
| 20 | 2.582 | 6.0205 | 9.6315 | 13.69 | ||
| 25 | 3.2825 | 8.746 | 15.778 | 24.7005 | ||
| 30 | 4.4815 | 14.1115 | 30.125 | 52.294 | ||
| 1 | 0.5 | 0 | 1.6035 | 1.3725 | 0.9705 | 0.4465 |
| 5 | 2.1705 | 2.2555 | 2.1225 | 1.835 | ||
| 10 | 2.9795 | 3.674 | 4.1425 | 4.369 | ||
| 15 | 4.214 | 6.2065 | 8.074 | 9.568 | ||
| 20 | 6.2895 | 11.287 | 16.6635 | 21.7955 | ||
| 25 | 10.181 | 22.8725 | 38.4145 | 56.004 | ||
| 30 | 18.692 | 54.527 | 106.835 | 178.696 | ||
| 0.75 | 0 | 1.3515 | 1.1175 | 0.7105 | 0.212 | |
| 5 | 1.82 | 1.867 | 1.7055 | 1.3975 | ||
| 10 | 2.464 | 3.0375 | 3.385 | 3.537 | ||
| 15 | 3.441 | 5.051 | 6.5905 | 7.864 | ||
| 20 | 4.9785 | 8.9385 | 13.39 | 17.761 | ||
| 25 | 7.7505 | 17.4825 | 30.127 | 43.9715 | ||
| 30 | 13.338 | 39.78 | 79.378 | 131.659 | ||
| 1 | 0 | 1.097 | 0.8765 | 0.4795 | −0.0275 | |
| 5 | 1.4865 | 1.508 | 1.32 | 0.9905 | ||
| 10 | 2.0195 | 2.47 | 2.7155 | 2.7855 | ||
| 15 | 2.7885 | 4.072 | 5.293 | 6.33 | ||
| 20 | 3.9495 | 7.0305 | 10.638 | 14.327 | ||
| 25 | 5.918 | 13.3085 | 23.4265 | 34.809 | ||
| 30 | 9.6975 | 28.8355 | 59.689 | 99.0255 | ||
| 1.33 | 0 | 0.778 | 0.5785 | 0.177 | −0.3265 | |
| 5 | 1.062 | 1.083 | 0.8585 | 0.4975 | ||
| 10 | 1.442 | 1.824 | 1.9455 | 1.899 | ||
| 15 | 1.98 | 3.01 | 3.8705 | 4.602 | ||
| 20 | 2.7975 | 5.096 | 7.6895 | 10.503 | ||
| 25 | 4.123 | 9.2305 | 16.528 | 25.319 | ||
| 30 | 6.469 | 18.8115 | 40.5245 | 69.0425 | ||
| 2 | 0 | 0.2685 | 0.0575 | −0.3515 | −0.8585 | |
| 5 | 0.398 | 0.3715 | 0.089 | −0.321 | ||
| 10 | 0.563 | 0.8185 | 0.757 | 0.5335 | ||
| 15 | 0.791 | 1.491 | 1.8475 | 2.077 | ||
| 20 | 1.126 | 2.5825 | 3.833 | 5.2025 | ||
| 25 | 1.646 | 4.4895 | 8.004 | 12.5485 | ||
| 30 | 2.529 | 8.361 | 18.2025 | 32.8185 | ||
| 2 | 0.5 | 0 | 0.1295 | −1.1115 | −2.534 | −4.1 |
| 5 | 0.611 | −0.449 | −1.7415 | −3.213 | ||
| 10 | 1.2895 | 0.623 | −0.352 | −1.642 | ||
| 15 | 2.329 | 2.612 | 2.5495 | 2.0485 | ||
| 20 | 4.0955 | 6.7755 | 9.487 | 11.888 | ||
| 25 | 7.4755 | 16.735 | 28.35 | 41.356 | ||
| 30 | 15.0435 | 45.0135 | 90.282 | 152.242 | ||
| 0.75 | 0 | −0.082 | −1.331 | −2.742 | −4.3085 | |
| 5 | 0.314 | −0.7865 | −2.1095 | −3.6025 | ||
| 10 | 0.8565 | 0.059 | −1.0265 | −2.401 | ||
| 15 | 1.657 | 1.575 | 1.189 | 0.3865 | ||
| 20 | 2.9525 | 4.6405 | 6.3395 | 7.8025 | ||
| 25 | 5.2945 | 11.7125 | 20.225 | 29.791 | ||
| 30 | 10.152 | 30.906 | 63.938 | 107.4515 | ||
| 1 | 0 | −0.284 | −1.542 | −2.949 | −4.4905 | |
| 5 | 0.0455 | −1.1025 | −2.4485 | −3.962 | ||
| 10 | 0.48 | −0.4305 | −1.623 | −3.086 | ||
| 15 | 1.0965 | 0.7045 | 0.0185 | −1.068 | ||
| 20 | 2.05 | 2.9205 | 3.7815 | 4.333 | ||
| 25 | 3.663 | 7.844 | 13.6145 | 20.4685 | ||
| 30 | 6.8415 | 20.625 | 44.0205 | 75.6995 | ||
| 1.33 | 0 | −0.515 | −1.8005 | −3.215 | −4.731 | |
| 5 | −0.273 | −1.469 | −2.8585 | −4.3985 | ||
| 10 | 0.0325 | −0.9895 | −2.304 | −3.875 | ||
| 15 | 0.4605 | −0.2175 | −1.257 | −2.684 | ||
| 20 | 1.0885 | 1.189 | 1.099 | 0.6395 | ||
| 25 | 2.1385 | 4.16 | 7.137 | 10.6765 | ||
| 30 | 3.9975 | 11.468 | 25.024 | 45.0415 | ||
| 2 | 0 | −0.9135 | −2.2385 | −3.6855 | −5.2045 | |
| 5 | −0.798 | −2.0605 | −3.528 | −5.122 | ||
| 10 | −0.671 | −1.8255 | −3.326 | −5.0805 | ||
| 15 | −0.534 | −1.489 | −3.0155 | −3.6745 | ||
| 20 | −0.3395 | −0.953 | −2.371 | −2.5215 | ||
| 25 | −0.0315 | 0.039 | −0.6185 | −1.811 | ||
| 30 | 0.5055 | 2.216 | 4.7235 | 8.757 |
| Methodology | R2 | Mean Absolute Error (MAE) | Root Mean Squared Error (RMSE) |
|---|---|---|---|
| Multi Linear Regression | 0.6616 | 11.8803 | 19.0166 |
| Artificial Neural Network (ANN) | 0.9963 | 1.4897 | 2.1889 |
| Hidden Layer Neurons (i) | Hidden Layer Bias (b1) | Hidden Weight IW1 | ||||||
|---|---|---|---|---|---|---|---|---|
| C/D (j = 1) | B/D (j = 2) | γD/c′ (j = 3) | ϕ (j = 4) | |||||
| 1 | −0.6618 | −0.20694 | −0.02904 | −0.07786 | 0.152957 | |||
| 2 | −1.35951 | 1.084979 | −0.31878 | −0.31652 | 1.316469 | |||
| 3 | −0.63364 | −0.76094 | 0.280919 | −0.00101 | 0.12054 | |||
| 4 | −5.65279 | 0.979189 | −1.15515 | 0.439291 | 3.511314 | |||
| 5 | −1.57382 | 1.605169 | 0.001409 | 0.82818 | −0.74447 | |||
| 6 | −4.5357 | 0.616293 | −0.29021 | −0.42419 | 2.613965 | |||
| 7 | −0.75008 | −1.03205 | 0.711475 | 0.377549 | 0.08785 | |||
| Output layer node (k) | Output layer bias (b2) | Output weight IW2 | ||||||
| i = 1 | i = 2 | i = 3 | i = 4 | i = 5 | i = 6 | i = 7 | ||
| 1 | 2.844085 | 0.207948 | −1.28044 | 0.623847 | −3.51562 | −1.838293 | −2.60675 | 0.89989 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jearsiripongkul, T.; Keawsawasvong, S.; Banyong, R.; Seehavong, S.; Sangjinda, K.; Thongchom, C.; Chavda, J.T.; Ngamkhanong, C. Stability Evaluations of Unlined Horseshoe Tunnels Based on Extreme Learning Neural Network. Computation 2022, 10, 81. https://doi.org/10.3390/computation10060081
Jearsiripongkul T, Keawsawasvong S, Banyong R, Seehavong S, Sangjinda K, Thongchom C, Chavda JT, Ngamkhanong C. Stability Evaluations of Unlined Horseshoe Tunnels Based on Extreme Learning Neural Network. Computation. 2022; 10(6):81. https://doi.org/10.3390/computation10060081
Chicago/Turabian StyleJearsiripongkul, Thira, Suraparb Keawsawasvong, Rungkhun Banyong, Sorawit Seehavong, Kongtawan Sangjinda, Chanachai Thongchom, Jitesh T. Chavda, and Chayut Ngamkhanong. 2022. "Stability Evaluations of Unlined Horseshoe Tunnels Based on Extreme Learning Neural Network" Computation 10, no. 6: 81. https://doi.org/10.3390/computation10060081
APA StyleJearsiripongkul, T., Keawsawasvong, S., Banyong, R., Seehavong, S., Sangjinda, K., Thongchom, C., Chavda, J. T., & Ngamkhanong, C. (2022). Stability Evaluations of Unlined Horseshoe Tunnels Based on Extreme Learning Neural Network. Computation, 10(6), 81. https://doi.org/10.3390/computation10060081










