Natural Convection Flow over a Vertical Permeable Circular Cone with Uniform Surface Heat Flux in Temperature-Dependent Viscosity with Three-Fold Solutions within the Boundary Layer
Abstract
1. Introduction
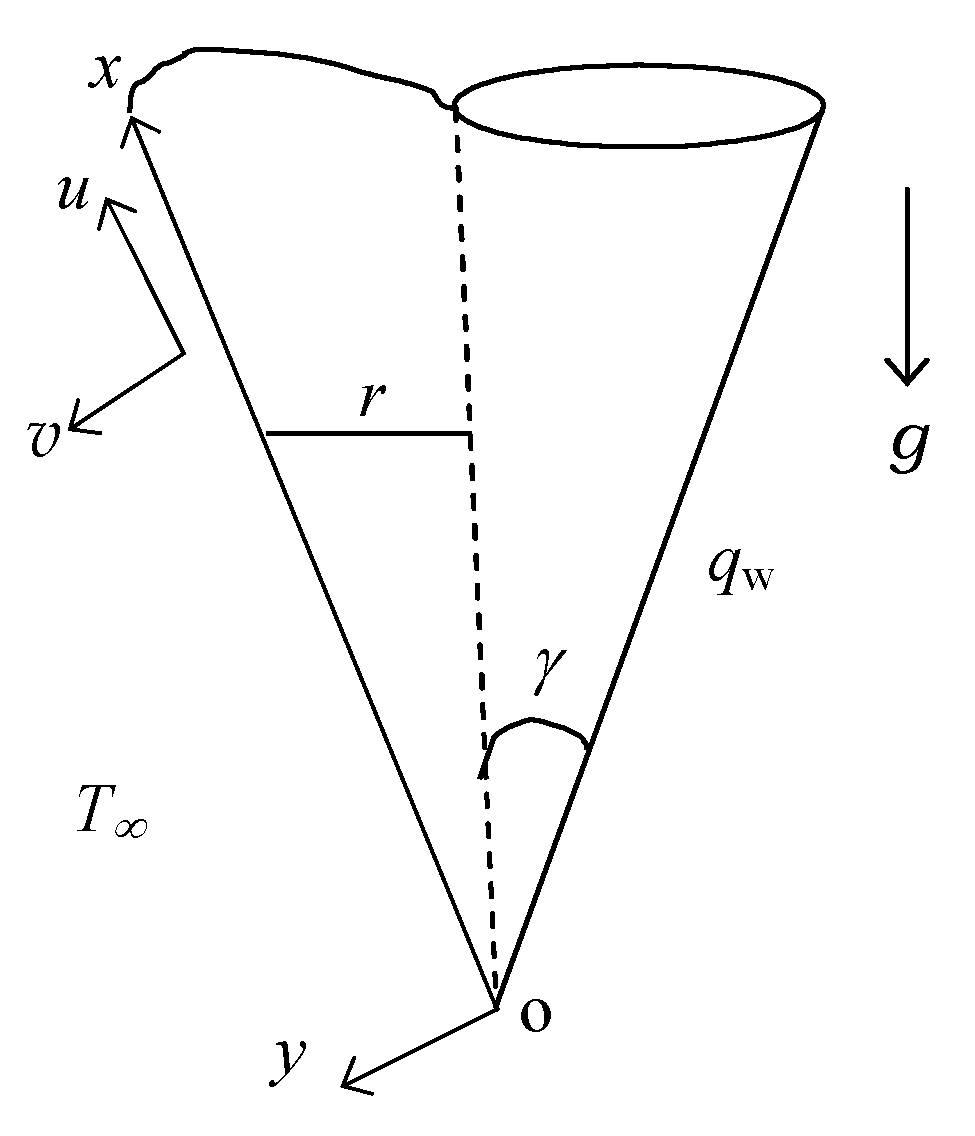
2. Formulation of the Problem
2.1. Solution for Small ξ (ξ << 1)
2.2. Solution for Large ξ
3. Results and Discussion
3.1. Computational Methods
3.2. Overview of Numerical Analyses
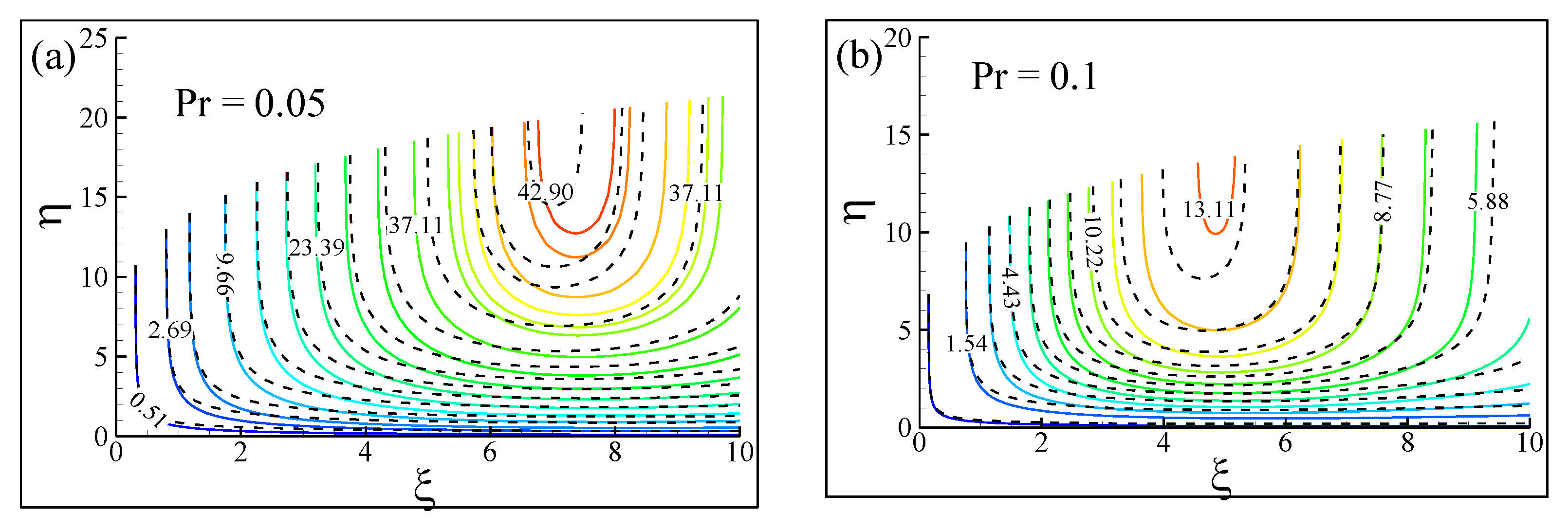
3.3. Comparing Finite Difference Solutions with Perturbation and Asymptotic Solutions
3.3.1. Validation and Comparison at Fixed Pr
3.3.2. Comparison at Variable Pr
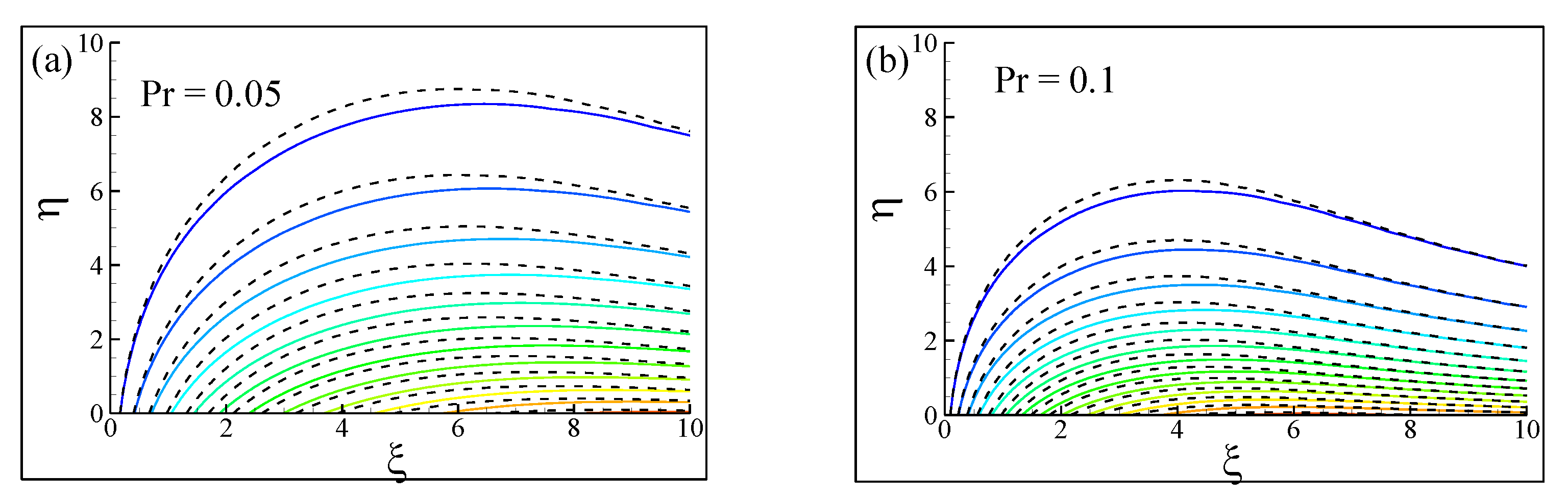
3.4. Development of Streamlines
3.5. Isothermal Behavior within the Boundary Layer
3.6. Impact of ε, η, and ξ Fluid Characteristics at Fixed Pr
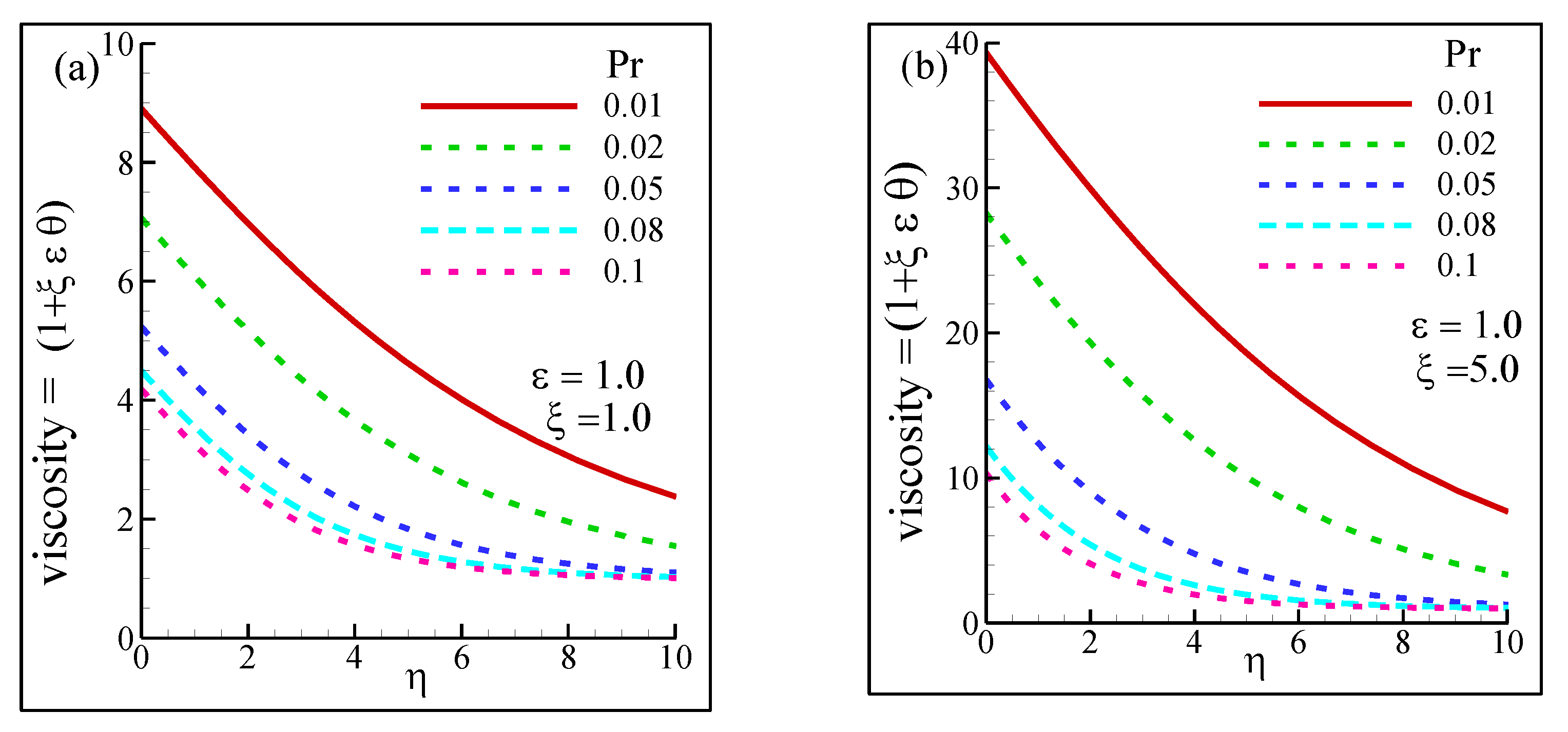
3.6.1. Influence on Viscosity Distribution
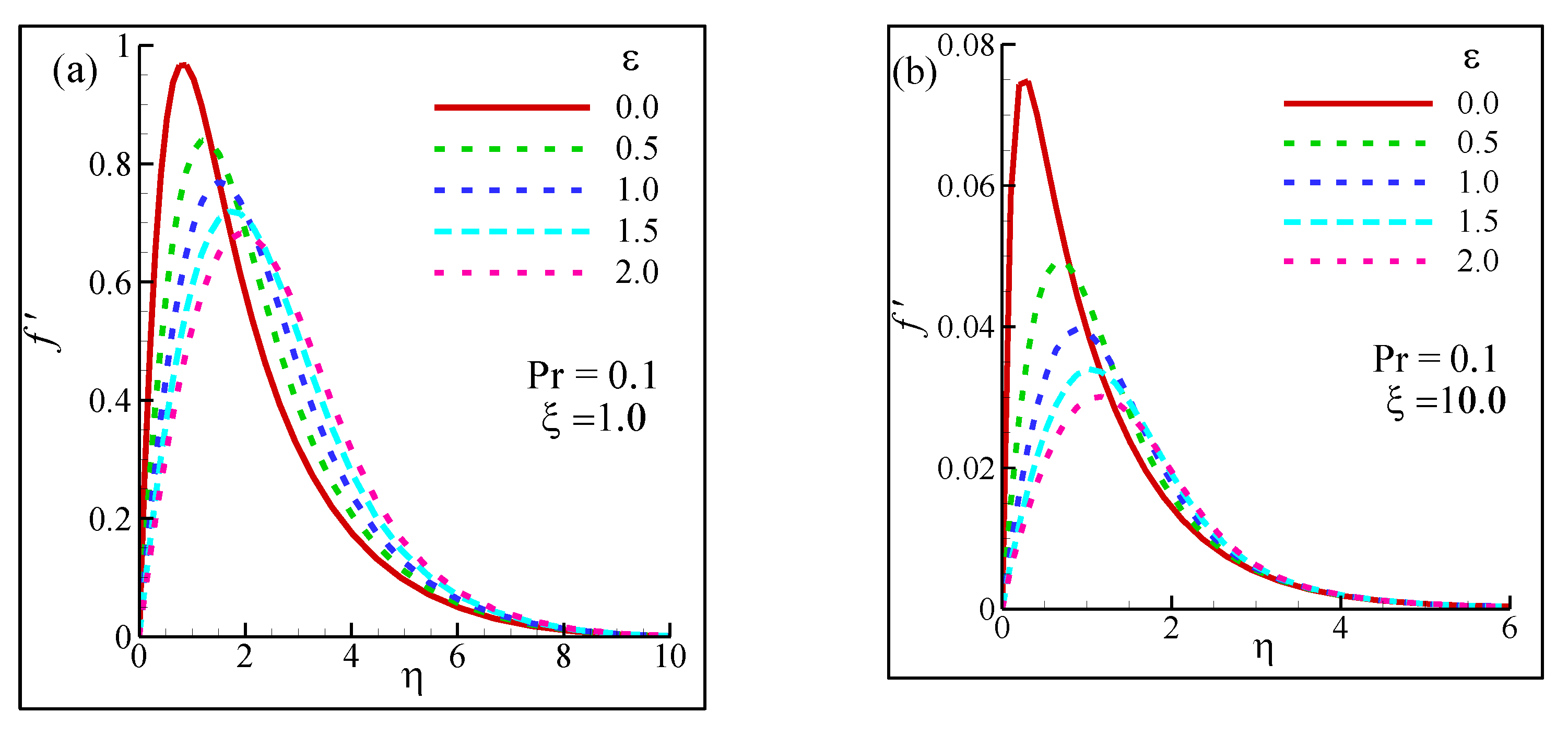
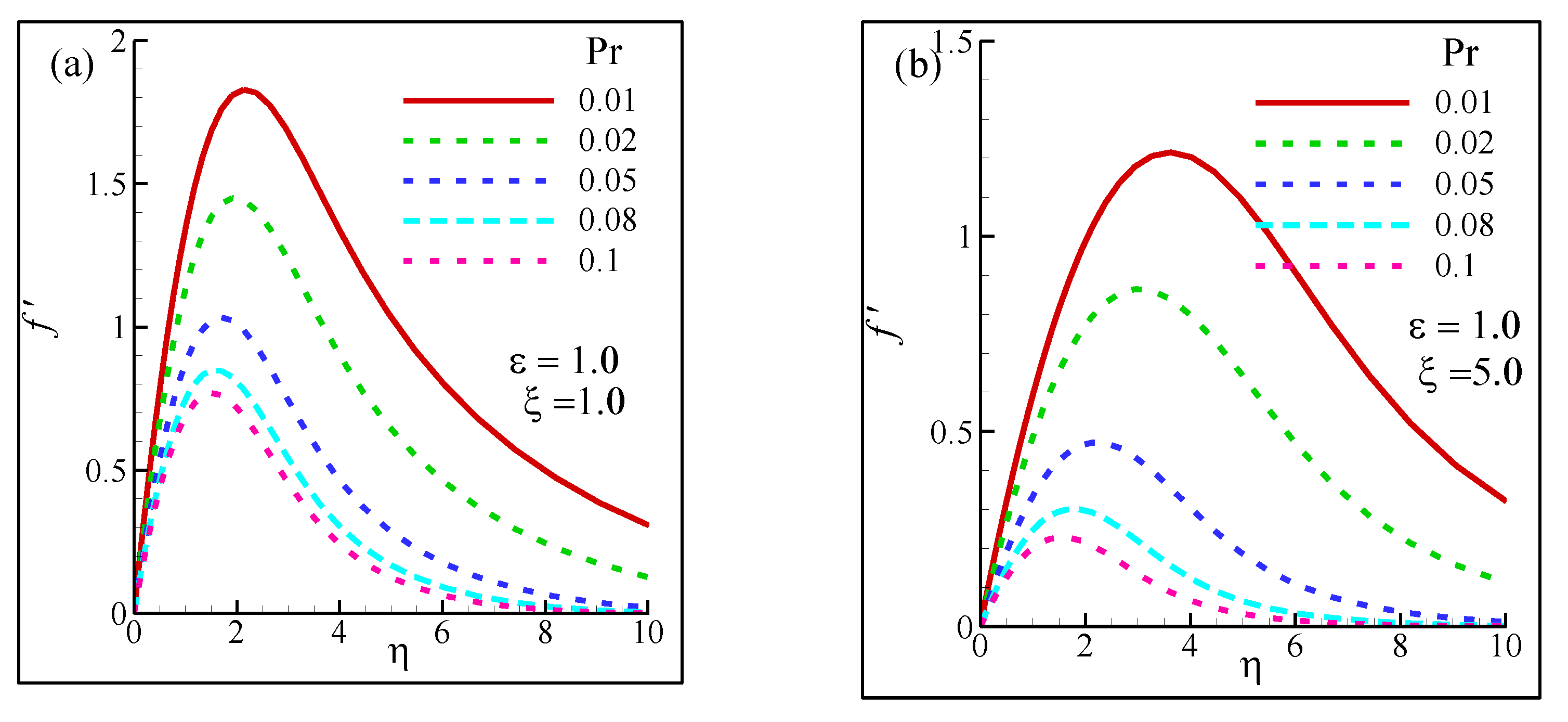
3.6.2. Effect on Velocity Distribution (f′)
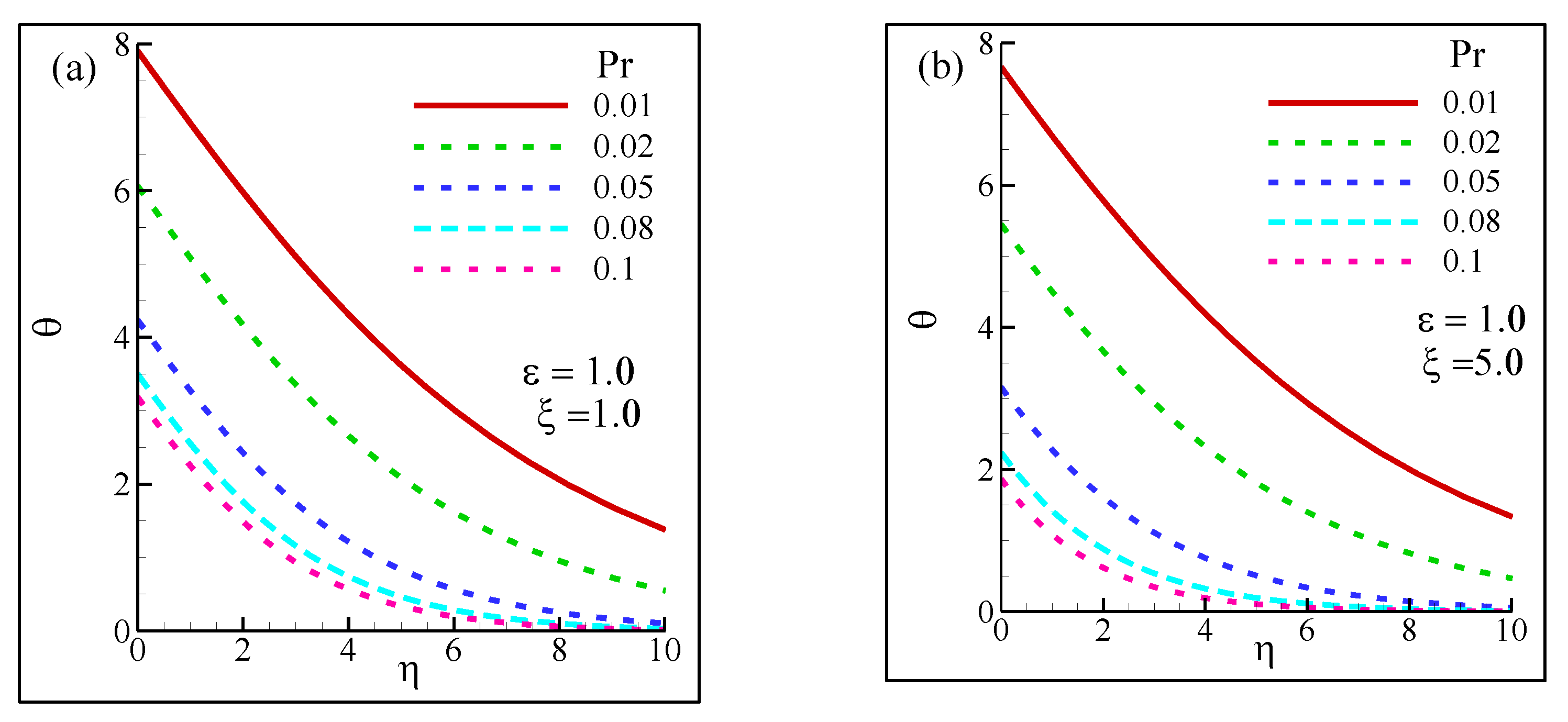
3.6.3. Changes in Temperature Distribution
3.7. Impact of ε, η, and ξ Fluid Characteristics at Variable Pr
3.7.1. Impact on Viscosity Distribution
3.7.2. Changes in the Velocity Distribution
3.7.3. Effect on Temperature Distribution
4. Conclusions
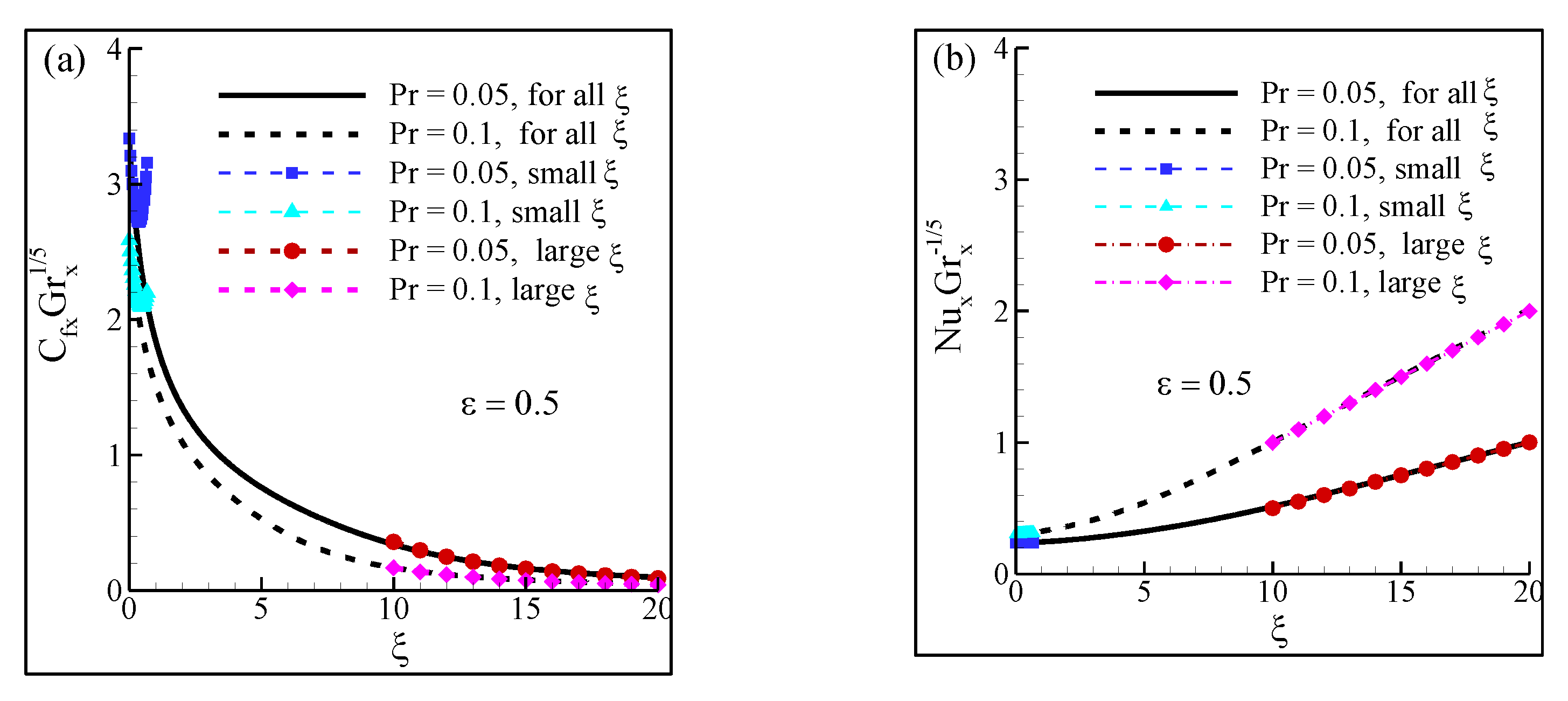
- Increasing the suction parameter (ξ) leads to decreasing shear stress (local skin-friction coefficient) and increasing the rate of heat transfer (local Nusselt number). The increasing and decreasing characteristics could be attributed to the temperature difference of the fluid within the boundary layer, which requires balancing the physical difference.
- As the viscosity-variation parameter (ε) increases, the local skin-friction coefficient decreases concurrently due to the effect of viscosity. On the other hand, increasing the rate of heat transfer as a function of ε does not remain consistent due to the effect of ξ. A small ξ rate of heat transfer decreases as ε increases, but the opposite behavior is observed with a large ξ, which indicates the superiority of the suction parameter over viscosity.
- If Pr increases, the local skin-friction coefficient decreases, and the rate of heat transfer increases rapidly due to the changes in the physical characteristics of the fluid and its viscosity.
- At ε ≠ 0, as η increases, viscosity decreases rapidly and heads towards 0 due to the dominance of η. However, at fixed ε and variable Pr, the curves corresponding to viscosity values exhibit the lowest value at a much later stage, indicating the high viscous characteristics of fluids with low Pr number.
- At any value of ε, at η ∈ [0,1], velocity increases the maximum at one point and then sharply plummets towards static condition at η > 6. However, a large suction parameter (ξ = 10) significantly lowers the peak values regardless of ε or types of fluids.
- An increased value of ε leads to the highest local maximum of temperature distribution in the absence of η and at a fixed Pr number. However, at η ≠ 0, the temperature starts to decrease gradually. The large suction parameter (ξ = 10) also suggestively lowers the peak values regardless of ε or types of fluids. Meanwhile, at the variable Pr number, the local maxima of temperature distribution get marginally affected at a low Pr number (<0.05).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| C | Specific heat |
| Cf | Skin-friction |
| f | Dimensionless stream function |
| Gr | Grashof number |
| k | Thermal conductivity |
| Nu | Nusselt number |
| Pr | Prandtl number |
| q | Heat flux |
| r | Radius of the cone |
| u | Velocity component in the x-direction |
| v | Velocity component in the y-direction |
| x | Coordinate along a cone ray |
| y | Coordinate normal to cone surface |
| g | Acceleration due to gravity |
| T | Temperature |
| V | Transpiration velocity |
| Greek Symbols | |
| β | Thermal expansion coefficient |
| Viscosity-variation parameter | |
| γ | Cone apex half-angle |
| Summation | |
| Dimensionless temperature function | |
| η | Pseudo-similarity variable |
| ξ | Dimensionless transpiration/suction parameter |
| Fluid density | |
| µ | Viscosity of the fluid |
| Stream function | |
| Subscripts | |
| fil | Film temperature |
| i | Sequence of term |
| p | Constant pressure or isobaric |
| x | Differentiation with respect to x |
| w | Surface |
| ∞ | Ambient state |
| Superscript | |
| ‘ | Differentiation with respect to |
| i | Number of iterations |
References
- Mojid, M.A.; Cho, H. Estimating the fully developed diffuse double layer thickness from the bulk electrical conductivity in clay. Appl. Clay Sci. 2006, 33, 278–286. [Google Scholar] [CrossRef]
- Zhang, N.; Wang, Z. Review of soil thermal conductivity and predictive models. Int. J. Therm. Sci. 2017, 117, 172–183. [Google Scholar] [CrossRef]
- Hasan, M.F.; Abuel-Naga, H.; Leong, E.-C. A modified series-parallel electrical resistivity model of saturated sand/clay mixture. Eng. Geol. 2021, 290, 106193. [Google Scholar] [CrossRef]
- Himika, T.A.; Hassan, S.; Hasan, M.F.; Molla, M.M. Lattice Boltzmann Simulation of MHD Rayleigh–Bénard Convection in Porous Media. Arab. J. Sci. Eng. 2020, 45, 9527–9547. [Google Scholar] [CrossRef]
- Simo-Tagne, M.; Ndukwu, M.C.; Zoulalian, A.; Bennamoun, L.; Kifani-Sahban, F.; Rogaume, Y. Numerical analysis and validation of a natural convection mix-mode solar dryer for drying red chilli under variable conditions. Ren. Energy 2020, 151, 659–673. [Google Scholar] [CrossRef]
- Pangavhane, D.R.; Sawhney, R.L.; Sarsavadia, P.N. Design, development and performance testing of a new natural convection solar dryer. Energy 2002, 27, 579–590. [Google Scholar] [CrossRef]
- Kumar, D.; Mahanta, P.; Kalita, P. Energy and exergy analysis of a natural convection dryer with and without sensible heat storage medium. J. Energy Stor. 2020, 29, 101481. [Google Scholar] [CrossRef]
- Calautit, J.K.; O’Connor, D.; Sofotasiou, P.; Hughes, B.R. CFD simulation and optimisation of a low energy ventilation and cooling system. Computation 2014, 3, 128–149. [Google Scholar] [CrossRef]
- Hireche, Z.; Himrane, N.; Nasseri, L.; Hamrioui, Y.; Ameziani, D.E. Analysis of Thermal Performances in a Ventilated Room Using LBM-MRT: Effect of a Porous Separation. Computation 2022, 10, 4. [Google Scholar] [CrossRef]
- Himika, T.A.; Hasan, M.F.; Molla, M.M. Lattice Boltzmann simulation of airflow and mixed convection in a general ward of hospital. J. Comp. Eng. 2016, 2016, 5405939. [Google Scholar] [CrossRef][Green Version]
- Hasan, M.F.; Himika, T.A.; Molla, M.M. Lattice Boltzmann simulation of airflow and heat transfer in a model ward of a hospital. J. Therm. Sci. Eng. Appl. 2017, 9, 011011. [Google Scholar] [CrossRef]
- Chen, L.; Zhao, J.; Yuan, Y.; Lee, J. Numerical Study on the Thermal Field and Heat Transfer Characteristics of a Hexagonal-Close-Packed Pebble Bed. Computation 2022, 10, 1. [Google Scholar] [CrossRef]
- Molla, M.M.; Nag, P.; Thohura, S.; Khan, A. A graphics process unit-based multiple-relaxation-time lattice Boltzmann simulation of non-Newtonian fluid flows in a backward facing step. Computation 2020, 8, 83. [Google Scholar] [CrossRef]
- Ahmed, N.S.; Badruddin, I.A.; Zainal, Z.A.; Khaleed, H.M.T.; Kanesan, J. Heat transfer in a conical cylinder with porous medium. Int. J. Heat Mass Transf. 2009, 52, 3070–3078. [Google Scholar] [CrossRef]
- Khan, T.Y.; Badruddin, I.A.; Quadir, G.A. Heat transfer in a conical porous cylinder with partial heating. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2016; p. 012211. [Google Scholar]
- Hanif, H.; Khan, I.; Shafie, S. Heat transfer exaggeration and entropy analysis in magneto-hybrid nanofluid flow over a vertical cone: A numerical study. J. Therm. Analys. Cal. 2020, 141, 2001–2017. [Google Scholar] [CrossRef]
- Rao, M.; Gangadhar, K.; Chamkha, A.J.; Surekha, P. Bioconvection in a convectional nanofluid flow containing gyrotactic microorganisms over an isothermal vertical cone embedded in a porous surface with chemical reactive species. Arab. J. Sci. Eng. 2021, 46, 2493–2503. [Google Scholar] [CrossRef]
- Rahman, A.; Molla, M.M.; Sarker, M.M.A. Natural convection flow along the vertical wavy cone in case of uniform surface heat flux where viscosity is an exponential function of temperature. Int. Comm. Heat Mass Transf. 2011, 38, 774–780. [Google Scholar] [CrossRef]
- Merk, H.J.; Prins, J.A. Thermal convection in laminary boundary layers I. Appl. Sci. Res. Sect. A 1953, 4, 11–24. [Google Scholar] [CrossRef]
- Merk, H.J.; Prins, J.A. Thermal convection in laminar boundary layers II. Appl. Sci. Res. Sect. A 1954, 4, 195–206. [Google Scholar] [CrossRef]
- Braun, W.H.; Ostrach, S.; Heighway, J.E. Free-convection similarity flows about two dimensional and axisymmetric bodies with closed lower ends. Int. J. Heat Mass Transf. 1961, 2, 121–135. [Google Scholar] [CrossRef]
- Hering, R.G.; Grosh, R.J. Laminar free convection from a non-isothermal cone. Int. J. Heat Mass Transf. 1962, 5, 1059–1068. [Google Scholar] [CrossRef]
- Hossain, M.A.; Paul, S.C. Free convection from a vertical permeable circular cone with non-uniform surface heat flux. Heat Mass Transf. 2001, 37, 167–173. [Google Scholar] [CrossRef]
- Hossain, M.A.; Paul, S.C. Free convection from a vertical permeable circular cone with non-uniform surface temperature. Acta Mech. 2001, 151, 103–114. [Google Scholar] [CrossRef]
- Hossain, M.A.; Paul, S.C.; Mandal, A.C. Natural convection flow along a vertical circular cone with uniform surface temperature and surface heat flux in a thermally stratified medium. Int. J. Num. Methods Heat Fluid Flow 2002, 12, 290–305. [Google Scholar] [CrossRef]
- Cebeci, T.; Bradshaw, P. Physical and Computational Aspects of Convective Heat Transfer; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Gary, J.; Kassoy, D.R.; Tadjeran, H.; Zebib, A. The effects of significant viscosity variation on convective heat transport in water-saturated porous media. J. Fluid Mech. 1982, 117, 233–249. [Google Scholar] [CrossRef]
- Mehta, K.N.; Sood, S. Transient free convection flow with temperature dependent viscosity in a fluid saturated porous medium. Int. J. Eng. Sci. 1992, 30, 1083–1087. [Google Scholar] [CrossRef]
- Ling, J.X.; Dybbs, A. Forced Convection over a Flat Plate Submersed in a Porous Medium: Variable Viscosity Case; American Society of Mechanical Engineers: New York, NY, USA, 1987. [Google Scholar]
- Thohura, S.; Rahman, A.; Molla, M.M.; Sarker, M.M. Effects of temperature dependent thermal conductivity on natural convection flow along a vertical wavy cone with heat flux. Proc. Eng. 2014, 90, 497–503. [Google Scholar] [CrossRef]
- Khan, M.; Salahuddin, T.; Altanji, M. A viscously dissipated Blasisus boundary layer flow with variable thermo-physical properties: An entropy generation study. Int. Comm. Heat Mass Transf. 2022, 131, 105873. [Google Scholar] [CrossRef]
- Gladys, T.; Reddy, G.V.R. Contributions of variable viscosity and thermal conductivity on the dynamics of non-Newtonian nanofluids flow past an accelerating vertical plate. Partial Diff. Eq. Appl. Math. 2022, 5, 100264. [Google Scholar] [CrossRef]
- Keller, H.B. Numerical methods in boundary-layer theory. Ann. Rev. Fluid Mech. 1978, 10, 417–433. [Google Scholar] [CrossRef]
- Kamran, A.; Azhar, E.; Akmal, N.; Mehmood, Z.; Iqbal, Z. Finite Difference Approach for Critical Value Analysis to Describe Jeffery–Hamel Flow toward an Inclined Channel with Microrotations. Arab. J. Sci. Eng. 2022, 1–8. [Google Scholar] [CrossRef]
- Reddy, Y.D.; Goud, B.S.; Chamkha, A.J.; Kumar, M.A. Influence of radiation and viscous dissipation on MHD heat transfer Casson nanofluid flow along a nonlinear stretching surface with chemical reaction. Heat Transfer. 2022, 1–17. [Google Scholar] [CrossRef]
- Vajravelu, K.; Kerehalli, V.P. Keller Box Method and Its Application; Walter de Gruyter GmbH & Co KG: De Gruyter, Germany, 2014; Volume 8. [Google Scholar]
- Molla, M.M.; Hossain, M.A. Radiation effect on mixed convection laminar flow along a vertical wavy surface. Int. J. Therm. Sci. 2007, 46, 926–935. [Google Scholar] [CrossRef]
- Charraudeau, J. Influence de gradients de proprietes physiques en convection forcee—Application au cas du tube. Int. J. Heat Mass Transf. 1975, 18, 87–95. [Google Scholar] [CrossRef]
- Butcher, J.C. Implicit Runge-Kutta processes. Math. Comput. 1964, 18, 50–64. [Google Scholar] [CrossRef]
- Nachtsheim, P.R.; Swigert, P. Statisfaction of Asymptotic Boundary Conditions in Numerical Solution of Systems of Nonlinear Equations of Boundary-Layer Type. No. NASA-TN-D-3004. 1965. Available online: https://ntrs.nasa.gov/api/citations/19650026350/downloads/19650026350.pdf (accessed on 18 March 2022).
- Akin, E.D. Object-Oriented Programming via Fortran 90/95; Cambridge University Press: Cambridge, UK, 2003; Volume 1. [Google Scholar]
- OriginPro, V. OriginLab Corporation; Northampton, MA, USA. 2016. Available online: https://www.originlab.com (accessed on 18 March 2022).
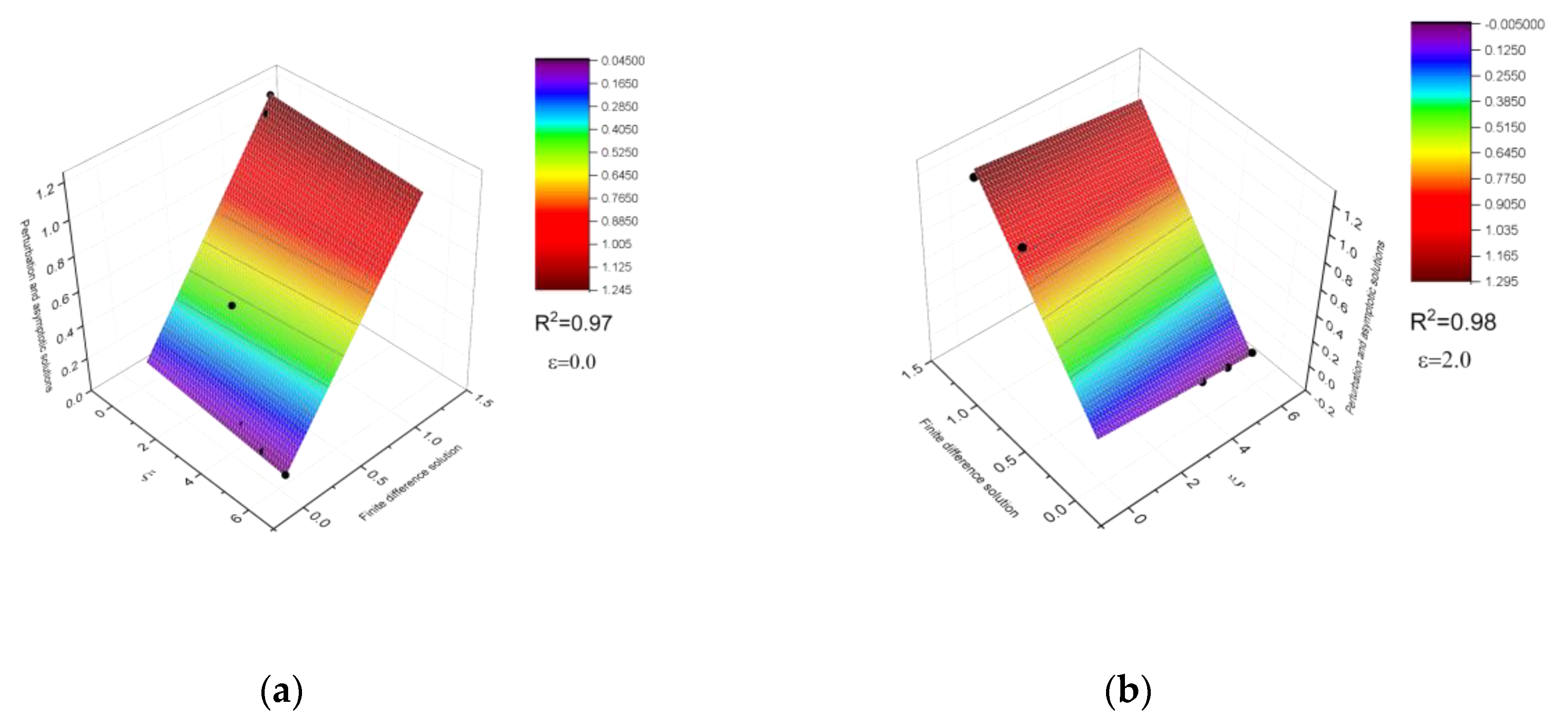
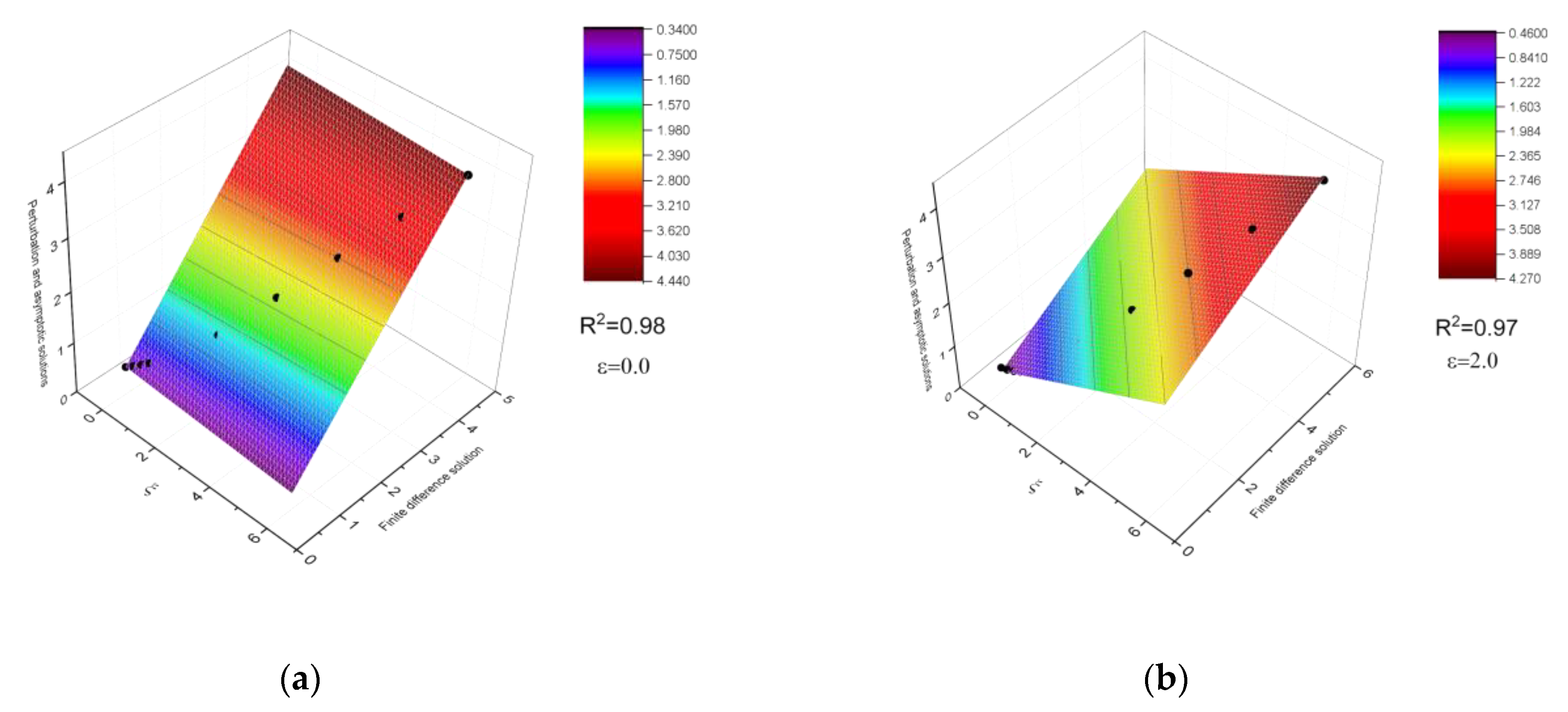


| ε = 0.0 | ε = 1.0 | ε = 0.0 | ε = 1.0 | |||||
|---|---|---|---|---|---|---|---|---|
| CfxGrx1/5 | NuxGrx−1/5 | |||||||
| ξ | Finite Diff. For All ξ | Small & Large ξ | Finite Diff. For All ξ | Small & Large ξ | Finite Diff. for All ξ | Small & Large ξ | Finite Diff. for All ξ | Small & Large ξ |
| 0.0 | 1.9905 | 1.9929 | 1.9905 | 1.9929 | 0.3821 | 0.3821 | 0.3821 | 0.3821 |
| 0.02 | 1.9919 | 1.9958 | 1.9319 | 1.9354 | 0.3838 | 0.3836 | 0.3823 | 0.3822 |
| 0.04 | 1.9939 | 1.9987 | 1.8766 | 1.8823 | 0.3855 | 0.3852 | 0.3826 | 0.3824 |
| 0.06 | 1.9969 | 2.0014 | 1.8256 | 1.8337 | 0.3871 | 0.3867 | 0.3828 | 0.3826 |
| 0.08 | 1.9999 | 2.0042 | 1.7778 | 1.7895 | 0.3887 | 0.3883 | 0.3829 | 0.3827 |
| 0.10 | 2.0028 | 2.0069 | 1.7330 | 1.7499 | 0.3903 | 0.3898 | 0.3833 | 0.3831 |
| 0.14 | 2.0028 | 2.0122 | 1.6909 | 1.6842 | 0.3934 | 0.3929 | 0.3836 | 0.3833 |
| 3.0 | 1.6938 | 0.3584 | - | 0.6893 | 0.6591 | - | ||
| 5.0 | 0.9349 | 0.9388 | 0.1657 | 0.1620 | 1.0185 | 1.0169 | 1.0119 | 1.0095 |
| 6.0 | 0.6741 | 0.6774 | 0.1189 | 0.1145 | 1.2099 | 1.2081 | 1.2077 | 1.2046 |
| 7.0 | 0.5020 | 0.5044 | 0.0898 | 0.0846 | 1.4064 | 1.4044 | 1.4069 | 1.4025 |
| 8.0 | 0.3064 | 0.3284 | 0.0709 | 0.0649 | 1.8044 | 1.6026 | 1.6082 | 1.6014 |
| 10.0 | 0.2488 | 0.2495 | 0.0496 | 0.0416 | 2.0042 | 2.0011 | 2.0163 | 2.0006 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasan, M.F.; Molla, M.M.; Kamrujjaman, M.; Siddiqa, S. Natural Convection Flow over a Vertical Permeable Circular Cone with Uniform Surface Heat Flux in Temperature-Dependent Viscosity with Three-Fold Solutions within the Boundary Layer. Computation 2022, 10, 60. https://doi.org/10.3390/computation10040060
Hasan MF, Molla MM, Kamrujjaman M, Siddiqa S. Natural Convection Flow over a Vertical Permeable Circular Cone with Uniform Surface Heat Flux in Temperature-Dependent Viscosity with Three-Fold Solutions within the Boundary Layer. Computation. 2022; 10(4):60. https://doi.org/10.3390/computation10040060
Chicago/Turabian StyleHasan, Md Farhad, Md. Mamun Molla, Md. Kamrujjaman, and Sadia Siddiqa. 2022. "Natural Convection Flow over a Vertical Permeable Circular Cone with Uniform Surface Heat Flux in Temperature-Dependent Viscosity with Three-Fold Solutions within the Boundary Layer" Computation 10, no. 4: 60. https://doi.org/10.3390/computation10040060
APA StyleHasan, M. F., Molla, M. M., Kamrujjaman, M., & Siddiqa, S. (2022). Natural Convection Flow over a Vertical Permeable Circular Cone with Uniform Surface Heat Flux in Temperature-Dependent Viscosity with Three-Fold Solutions within the Boundary Layer. Computation, 10(4), 60. https://doi.org/10.3390/computation10040060









