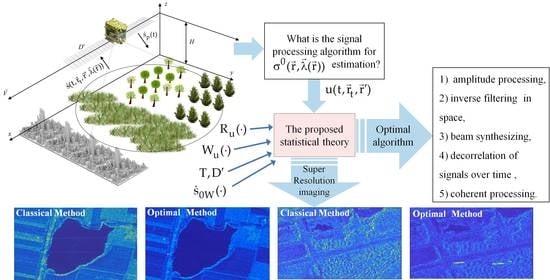
Statistical Theory of Optimal Stochastic Signals Processing in Multichannel Aerospace Imaging Radar Systems
Abstract
1. Introduction
2. Materials and Methods
2.1. Models of Signals, Noises and Observation Equation
2.2. Bases of Statistical Theory
3. Results
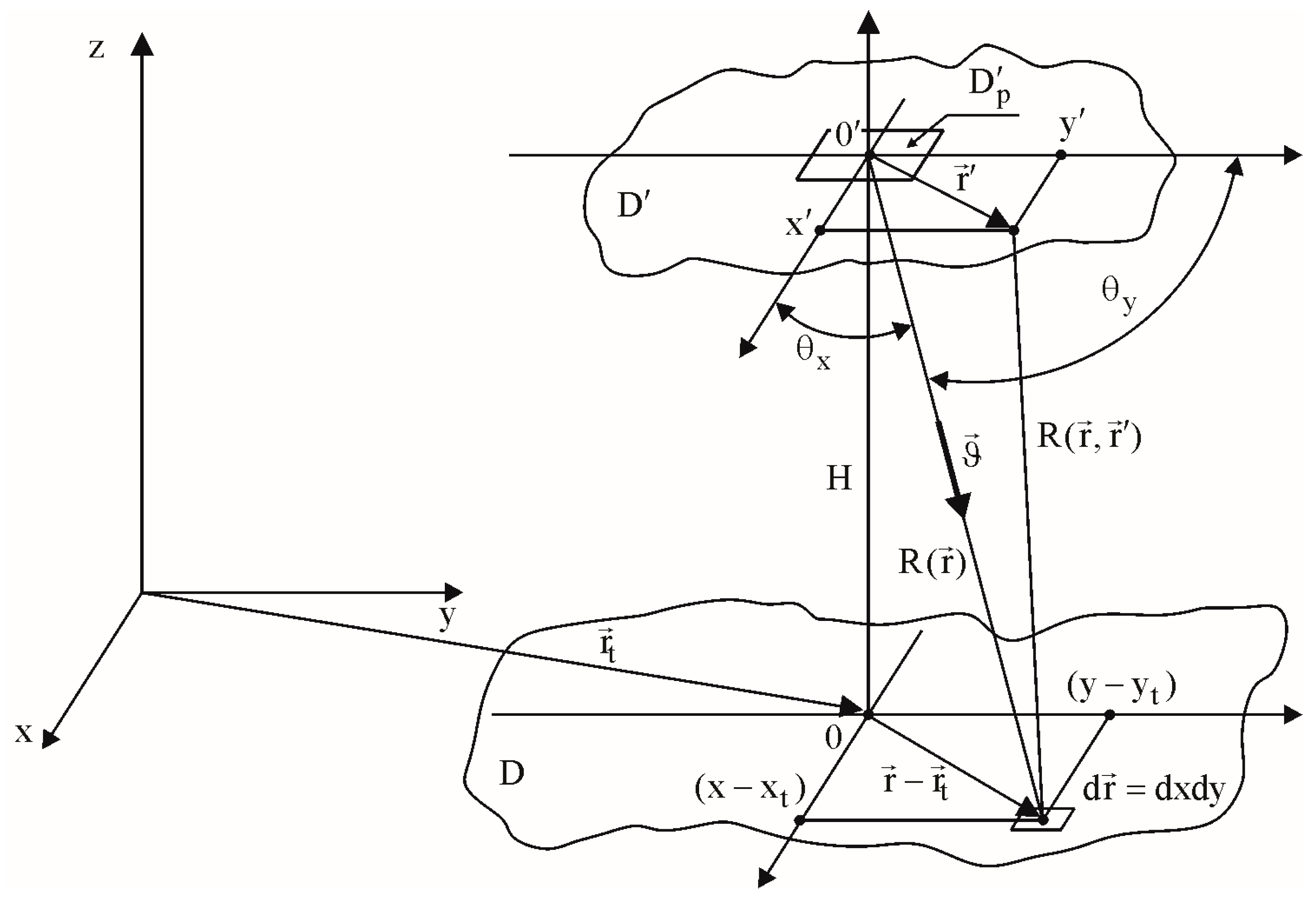
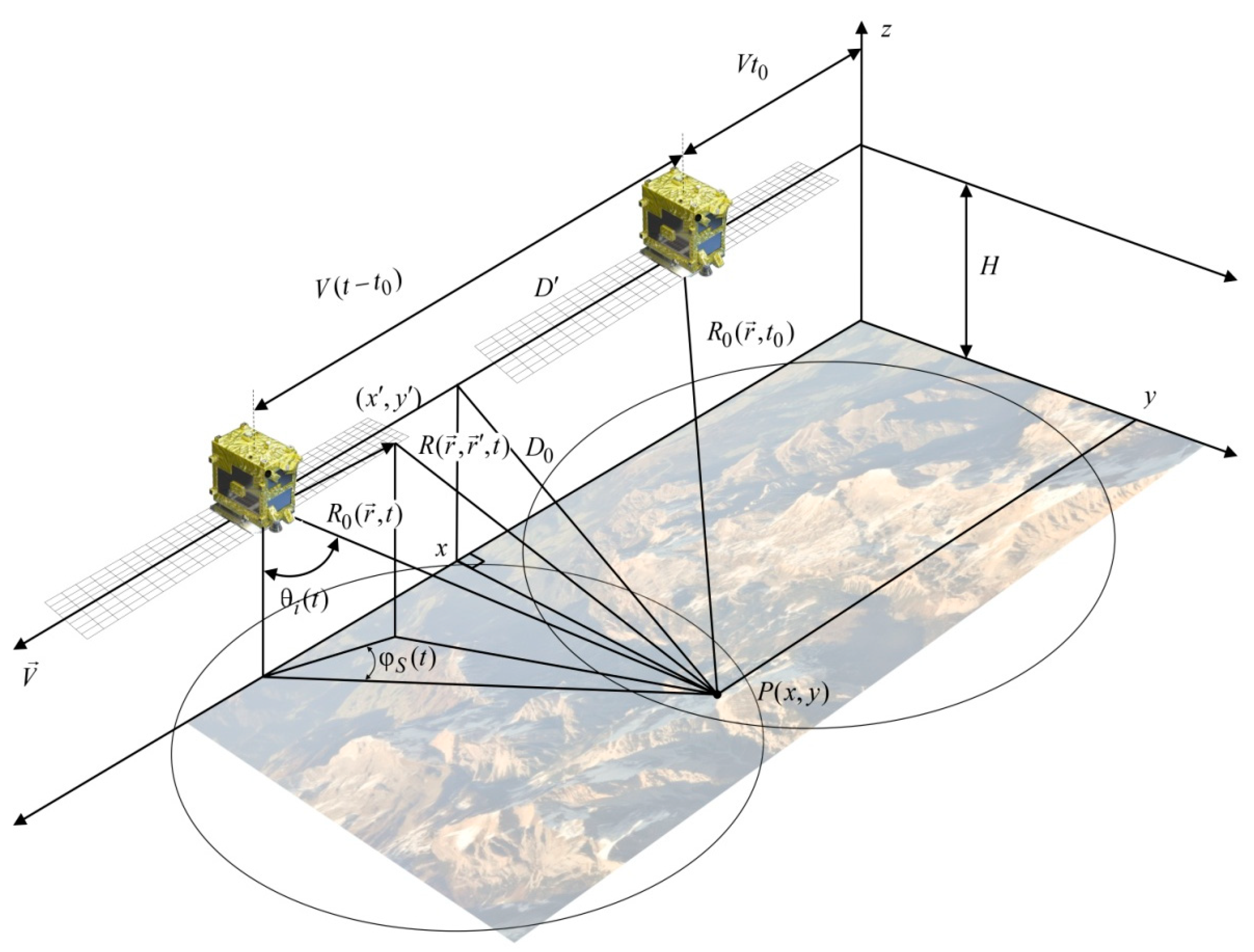
3.1. Geometry of the Surface Sensing
3.2. Problem Statement
3.3. Solution of the Optimization Problem
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kotel’nikov, V.A. The Theory of Optimum Noise Immunity; McGraw-Hill Book, Co.: New York, NY, USA, 1959. [Google Scholar]
- Middlton, D. Introduction to Statistical Communication Theory; Sovetskoye radio: Moscow, Russia, 1961–1962; Volume 1–2. (In Russian) [Google Scholar]
- Woodward, F.M. Probability Theory and Information Theory with Application to Radar; Pergamon: New York, NY, USA, 1964. [Google Scholar]
- Bakut, P.A.; Tartakovsky, G.P. Questions of the Statistical Theory of Radar; Sovetskoye radio: Moscow, Russia, 1963. (In Russian) [Google Scholar]
- Falkovich, S.E.; Ponomarev, V.I.; Shkvarko, Y.V. Optimal Reception of Space-Time Signals in Radio Channels with Scattering; Radio i svyaz: Moscow, Russia, 1989. (In Russian) [Google Scholar]
- Falkovich, S.E.; Kostenko, P.Y. Fundamentals of the Statistical Theory of Radio Engineering Systems: A Tutorial; Kharkiv Aviation Institute: Kharkov, Russia, 2005. (In Russian) [Google Scholar]
- Falkovich, S.E. Signal Parameters Estimation; Sovetskoye radio: Moscow, Russia, 1970. (In Russian) [Google Scholar]
- Volosyuk, V.K.; Kravchenko, V.F. Statistical Theory of Radio Engineering Systems for Remote Sensing and Radar; Fizmatlit: Moscow, Russia, 1988. (In Russian) [Google Scholar]
- Gutkin, L.S. Theory of Optimal Radio Reception Methods with Fluctuation Interference; Gosenergoizdat: Leningrad, Russia, 1961. (In Russian) [Google Scholar]
- Levin, B.R. Theoretical Foundations of Statistical Radio Engineering; Sovetskoye radio: Moscow, Russia, 1969. (In Russian) [Google Scholar]
- Shirman Ya, D.; Manzhos, V.N. Theory and Technique of Processing Radar Information Against the Background of Interference; Radio i svyaz: Moscow, Russia, 1981. (In Russian) [Google Scholar]
- Kremer, I. Spatial-Temporal Signal Processing; Moscow Izdatel Radio Sviaz: Moscow, Russia, 2004; 204p. [Google Scholar]
- Stratonovich, R. Selected Questions of Fluctuation Theory in Radio Engineering; Sov. Radio: Moscow, Russia, 1961. (In Russian) [Google Scholar]
- Stratonovich, R.L. On the theory of optimal non-linear filtration of random functions. Theory Prob. Appl. 1959, 4, 223–225. [Google Scholar]
- Stratonovich, R.L. Application of the Markov processes theory to optimum filtration of signals. Radio Eng. Electron. Phys. 1960, 5, 1–19. [Google Scholar]
- Tikhonov, V.I. Statistical Radio Engineering; Radio i svyaz: Moscow, Russia, 1982. (In Russian) [Google Scholar]
- Tikhonov, V.I. Optimal Signal Reception; Radio i svyaz: Moscow, Russia, 1983. (In Russian) [Google Scholar]
- Klyatskin, V.I. Stochastic Equations: Theory and Applications in Acoustics, Hydrodynamics, Magnetohydrodynamics, and Radiophysics, Volume 1: Basic Concepts, Exact Results, and Asymptotic Approximations. Understanding Complex Systems; Springer International Publishing: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Tan, S. Multiple Volume Scattering in Random Media and Periodic Structures with Applications in Microwave Remote Sensing and Wave Functional Materials. Ph.D. Thesis, University of Michigan, Ann Arbor, MI, USA, 2016. [Google Scholar]
- Tang, K.; Buckius, R.O. A statistical model of wave scattering from random rough surfaces. Int. J. Heat Mass Transf. 2001, 44, 4059–4073. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Liu, L.; Mackowski, D.W.; Cairns, B.; Videen, G. Multiple scattering by random particulate media: Exact 3D results. Opt. Express 2007, 15, 2822–2836. [Google Scholar] [CrossRef] [PubMed]
- Volosyuk, V.K.; Pavlikov, V.V.; Zhyla, S.S. Phenomenological Description of the Electromagnetic Field and Coherent Images in Radio Engineering and Optical Systems. In Proceedings of the 2018 IEEE 17th International Conference on Mathematical Methods in Electromagnetic Theory (MMET), Kyiv, Ukraine, 2–5 July 2018; pp. 302–305. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, K.; Shang, G. Surface Parameters Retrieval from Fully Bistatic Radar Scattering Data. Remote Sens. 2019, 11, 596. [Google Scholar] [CrossRef]
- Zeng, J.Y.; Chen, K.S.; Bi, H.Y.; Zhao, T.J.; Yang, X.F. A comprehensive analysis of rough soil surface scattering and emission predicted by AIEM with comparison to numerical simulations and experimental measurements. IEEE Trans. Geosci. Remote Sens. 2017, 55, 1696–1708. [Google Scholar] [CrossRef]
- Liao, T.H.; Tsang, L.; Huang, S.; Niamsuwan, N.; Jaruwatanadilok, S.; Kim, S.B.; Ren, H.; Chen, K.L. Co-polarized and cross-polarized backscattering from random rough soil surfaces from L-band to Ku-band using numerical solutions of Maxwell’s equations with near-field precondition. IEEE Trans. Geosci. Remote Sens. 2016, 54, 651–662. [Google Scholar] [CrossRef]
- TTeperik, V.; Archambault, A.; Marquier, F.; Greffet, J.J. Huygens-Fresnel principle for surface plasmons. Opt. Express 2009, 17, 17483–17490. [Google Scholar] [CrossRef] [PubMed]
- Chu, H.; Qi, J.; Wang, R.; Qiu, J. Generalized Rayleigh-Sommerfeld Diffraction Theory for Metasurface-Modulating Paraxial and Non-Paraxial Near-Field Pattern Estimation. IEEE Access 2019, 7, 57642–57650. [Google Scholar] [CrossRef]
- Klyatskin, V.I. Stochastic Equations Through the Eye of the Physicist; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Vasilenko, G.I.; Taratorin, A.M. Image Recovery; Radio i svyaz: Moscow, Russia, 1986. [Google Scholar]
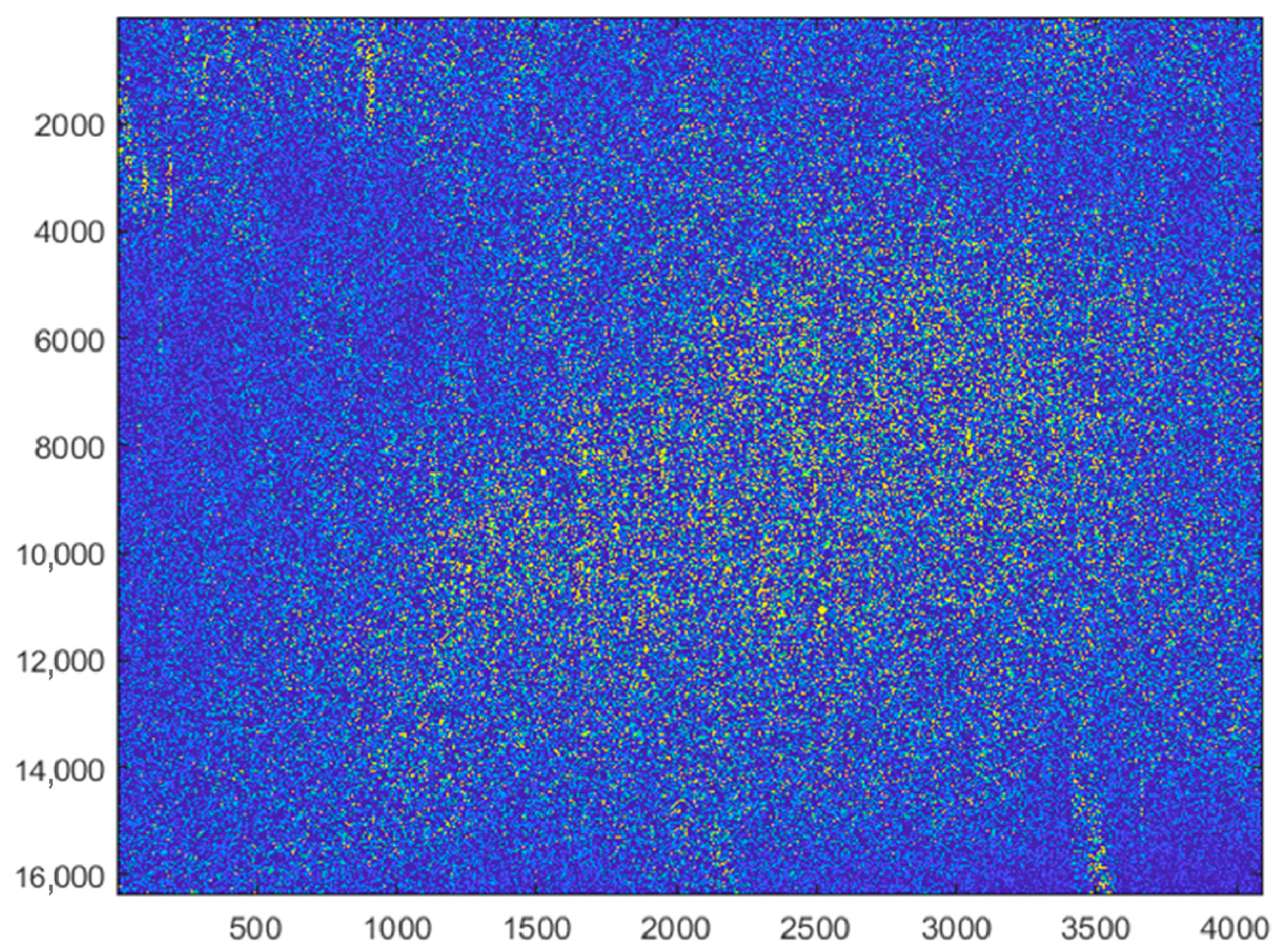

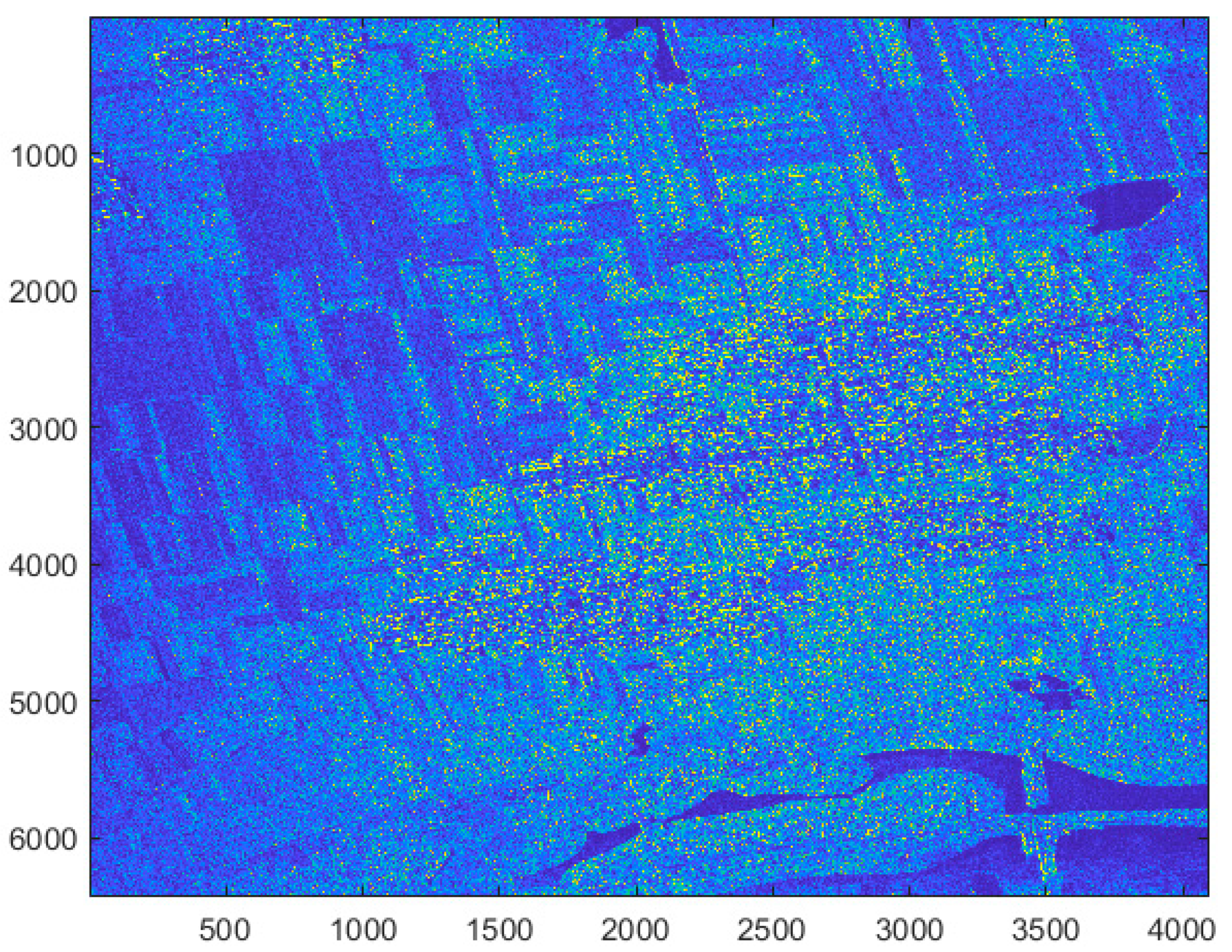

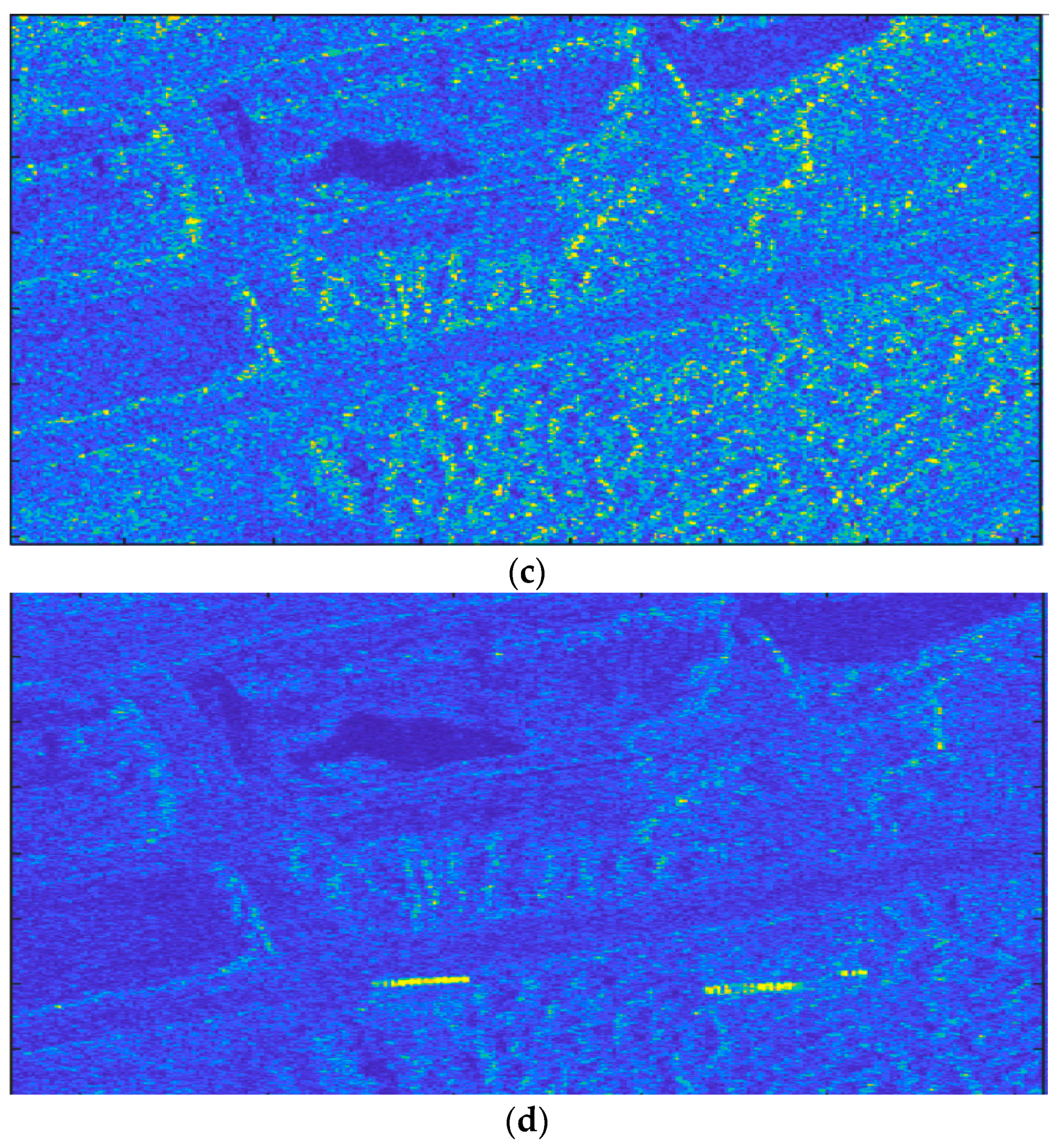
| Metrics | Coherent Processing without Decorrelation | The Proposed Optimal Method of Stochastic Signal Processing |
|---|---|---|
| MSE | 4.1259 × 103 | 4.0514 × 103 |
| PSNR | 11.9756 | 12.0547 |
| SSIM | 0.1245 | 0.1326 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Volosyuk, V.; Zhyla, S. Statistical Theory of Optimal Stochastic Signals Processing in Multichannel Aerospace Imaging Radar Systems. Computation 2022, 10, 224. https://doi.org/10.3390/computation10120224
Volosyuk V, Zhyla S. Statistical Theory of Optimal Stochastic Signals Processing in Multichannel Aerospace Imaging Radar Systems. Computation. 2022; 10(12):224. https://doi.org/10.3390/computation10120224
Chicago/Turabian StyleVolosyuk, Valeriy, and Semen Zhyla. 2022. "Statistical Theory of Optimal Stochastic Signals Processing in Multichannel Aerospace Imaging Radar Systems" Computation 10, no. 12: 224. https://doi.org/10.3390/computation10120224
APA StyleVolosyuk, V., & Zhyla, S. (2022). Statistical Theory of Optimal Stochastic Signals Processing in Multichannel Aerospace Imaging Radar Systems. Computation, 10(12), 224. https://doi.org/10.3390/computation10120224