Global Practical Output Tracking for a Class of Uncertain Inherently Time-Varying Delay Nonlinear Systems by Output Feedback
Abstract
1. Introduction
2. Useful Definition and Lemmas
- The dilation is defined byfor, with being called as the weights of the coordinate. For simplicity, we define dilation weight .
- A function is said to be homogeneous of degree if there is a real number, such that
- A vector fieldis said to be homogeneous of degree m if there is a real number, such that for
- A homogeneous p-norm is defined asfor a constant. For simplicity, we choose and write for .
- (i)
- is homogeneous of degree with being the homogeneous weight of.
- (ii)
- There is a constantsuch that. Moreover, if is positive definite,, for a constant.
3. Problem Statement and Main Results
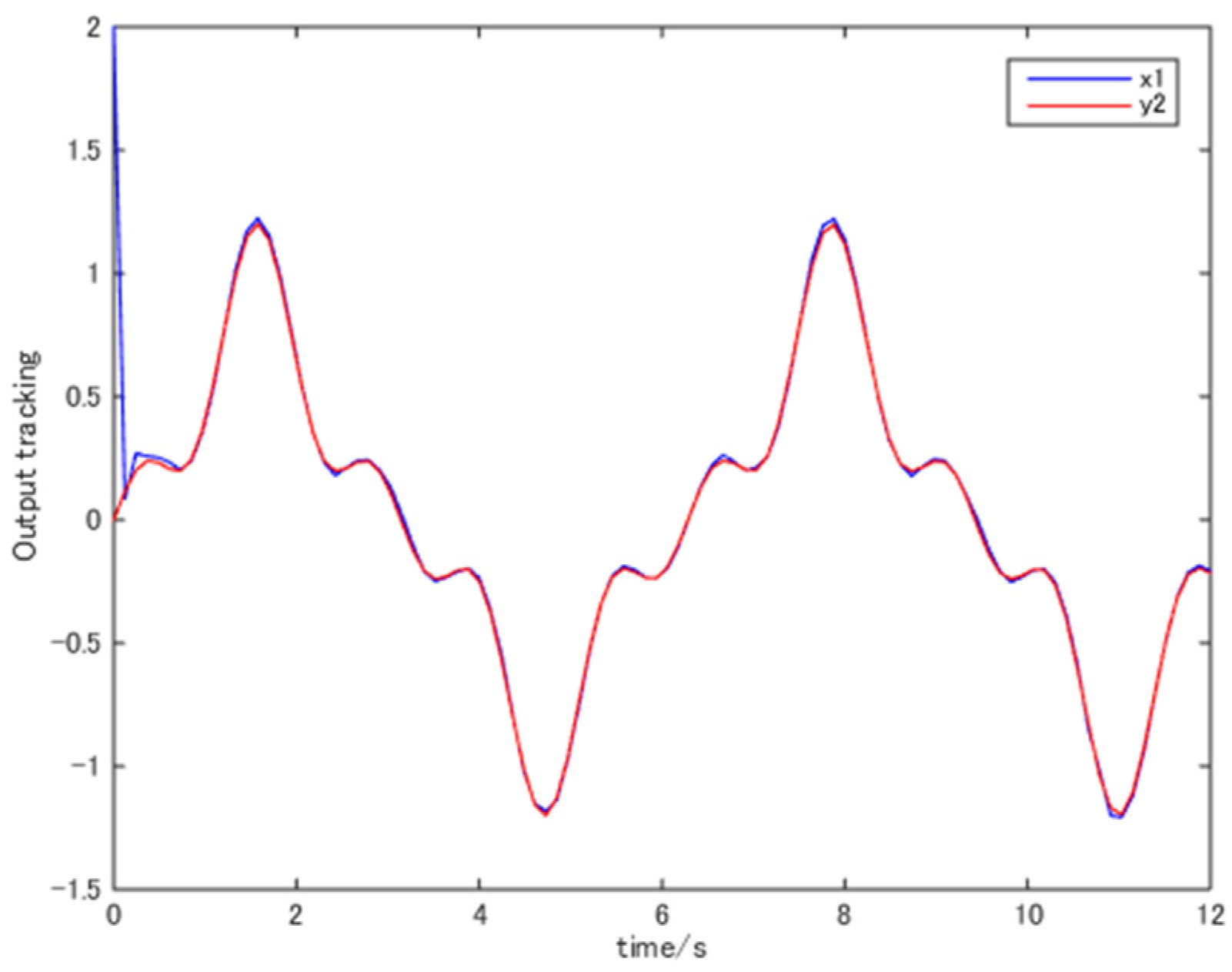
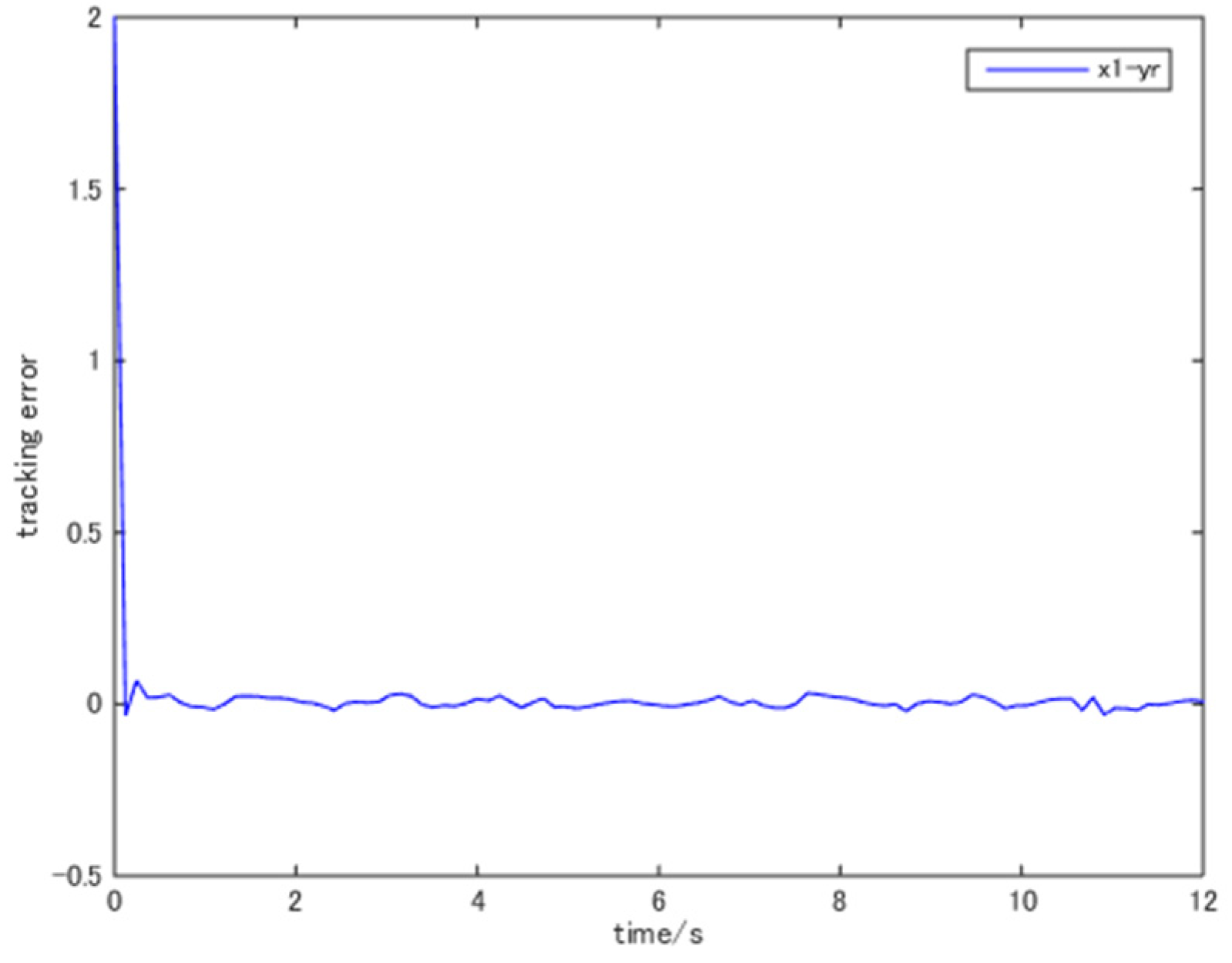
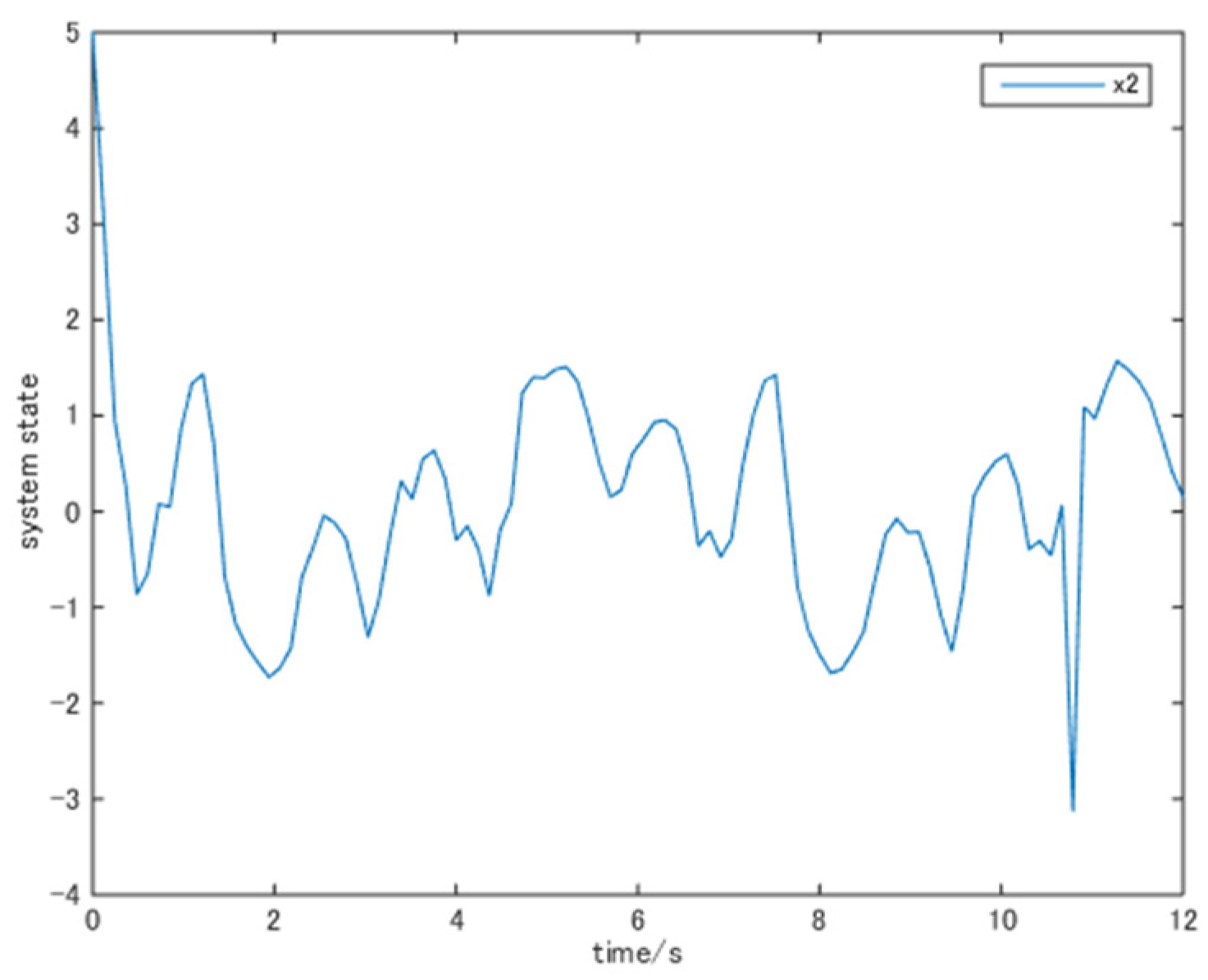
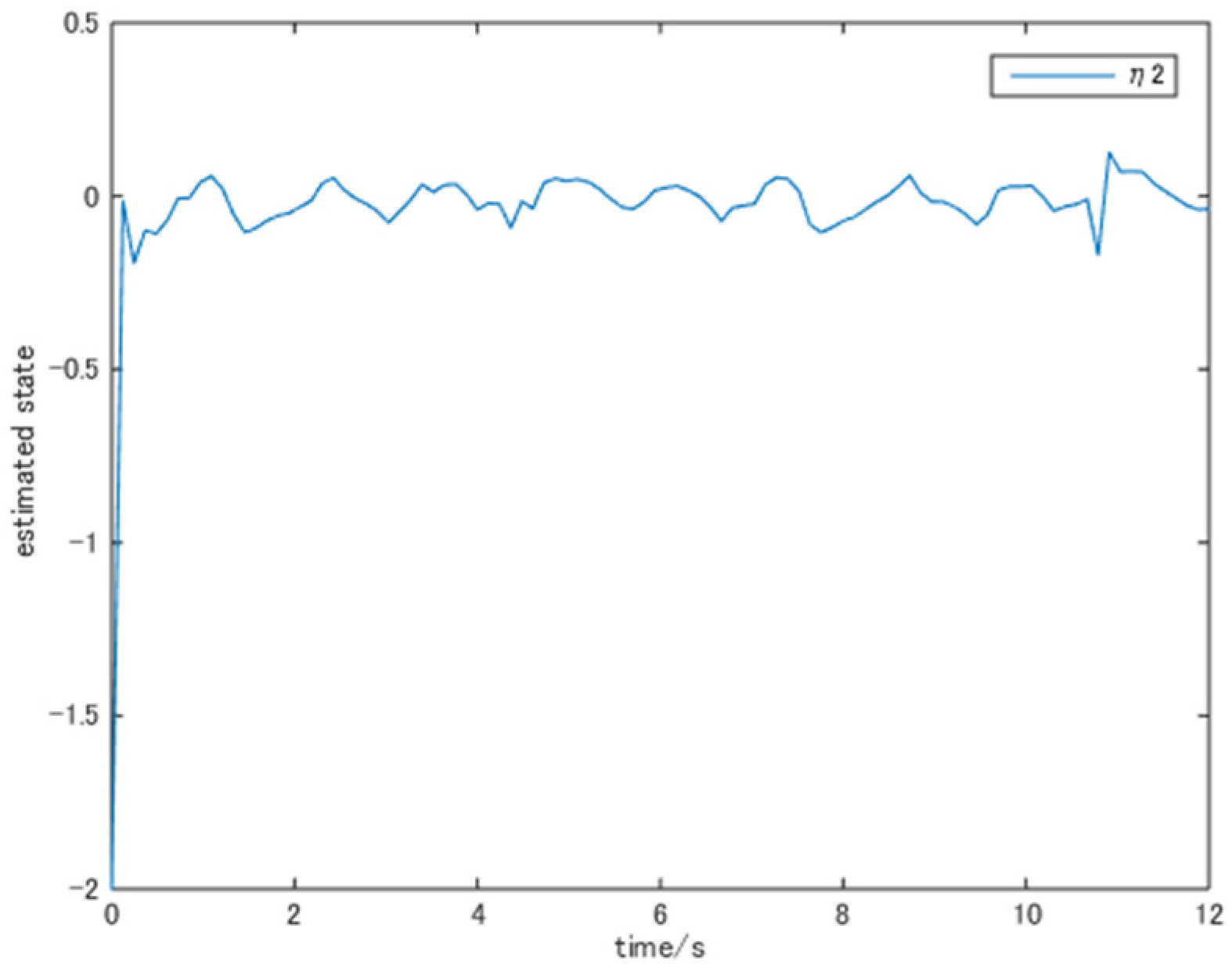
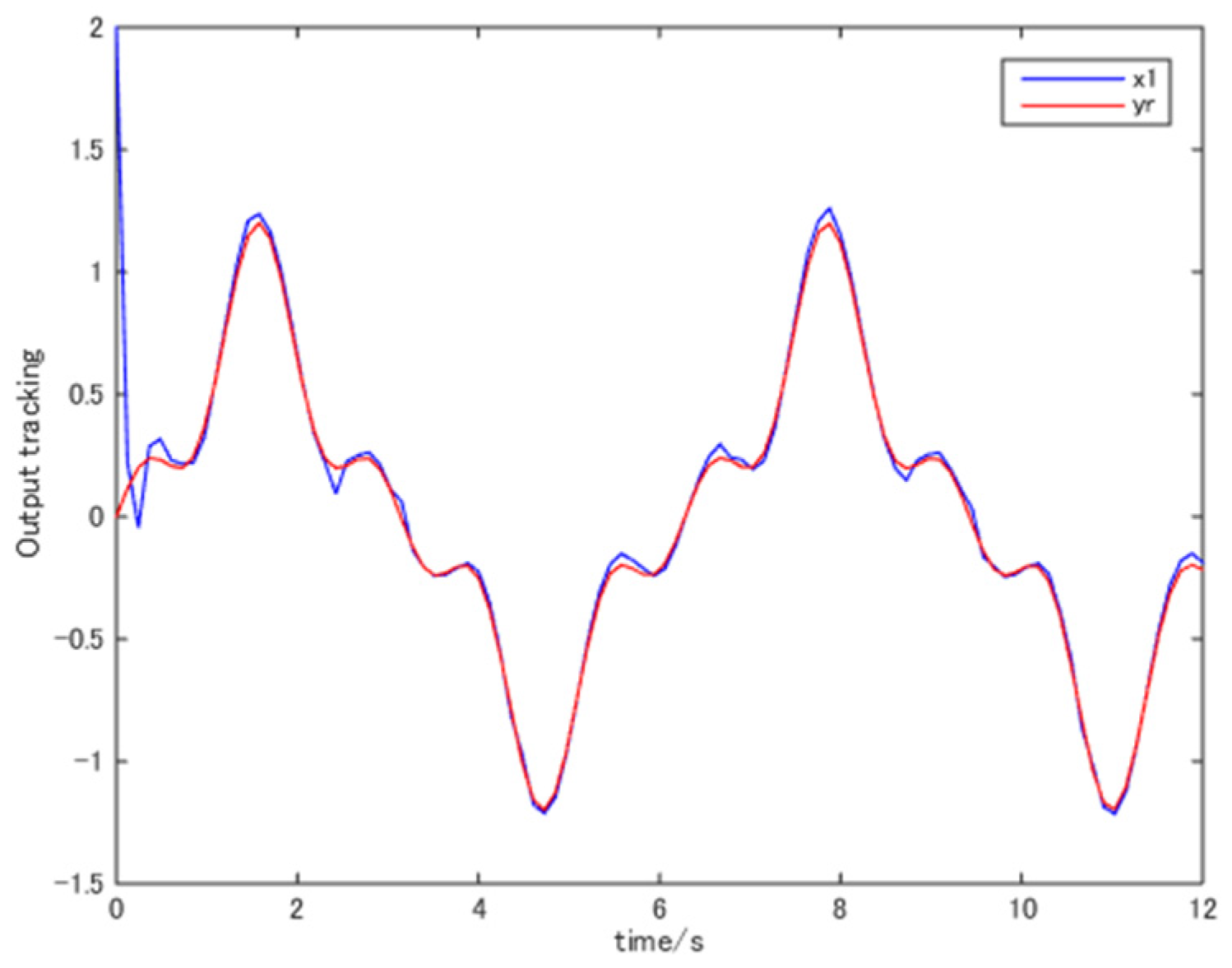
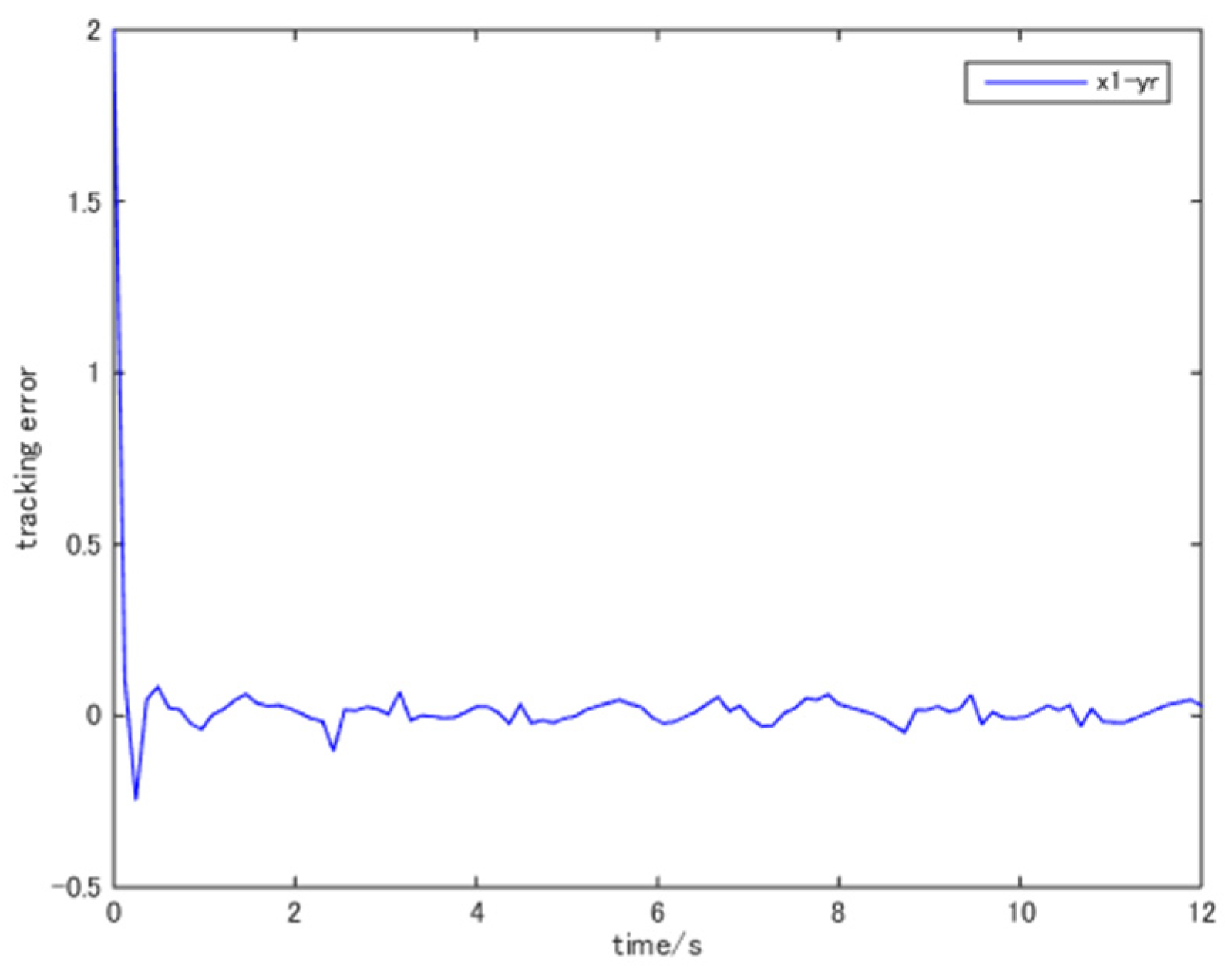
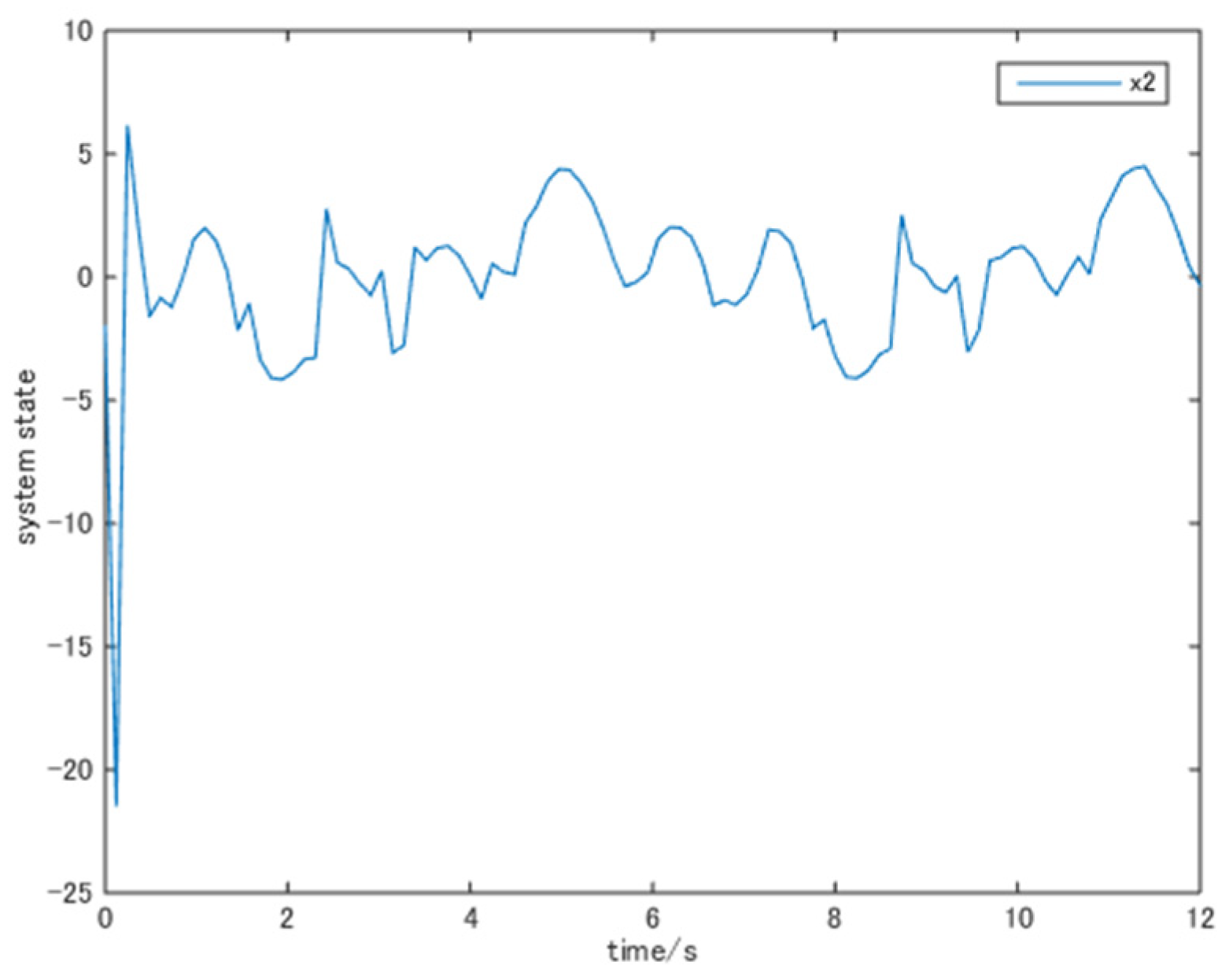
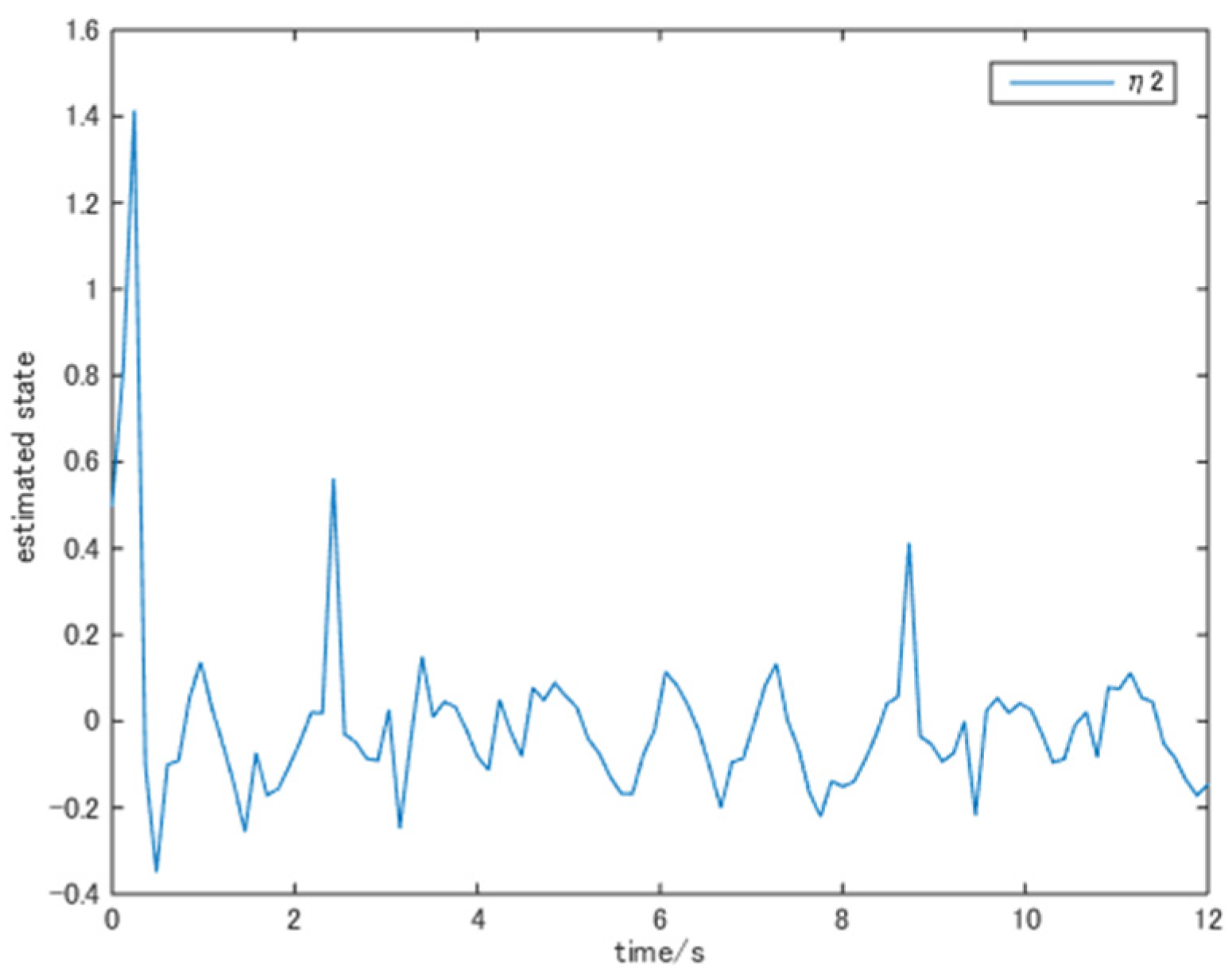
4. Example and Simulations
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qian, C.; Lin, W. Practical output tracking of nonlinear systems with uncontrollable unstable linearization. IEEE Trans. Autom. Control 2002, 47, 21–36. [Google Scholar] [CrossRef]
- Lin, W.; Pongvuthithum, R. Adaptive output tracking of inherently nonlinear systems with nonlinear parameterization. IEEE Trans. Autom. Control 2003, 48, 1737–1749. [Google Scholar] [CrossRef]
- Gong, Q.; Qian, C. Global practical output regulation of a class of nonlinear systems by output feedback. Automatica 2007, 43, 184–189. [Google Scholar] [CrossRef]
- Sun, Z.-Y.; Liu, Y.-G. Adaptive practical output tracking control for high-order nonlinear uncertain systems. Acta Autom. Sin. 2008, 34, 984–989. [Google Scholar] [CrossRef]
- Alimhan, K.; Inaba, H. Robust practical output tracking by output compensator for a class of uncertain inherently nonlinear systems. Int. J. Model. Identif. Control 2008, 4, 304–314. [Google Scholar] [CrossRef]
- Yan, X.; Liu, Y. Global practical tracking for high order uncertain nonlinear systems with unknown control directions. SIAM J. Control Optim. 2010, 48, 4453–4473. [Google Scholar] [CrossRef]
- Yan, X.; Liu, Y. Global practical tracking by output feedback for nonlinear systems with unknown growth rate. Sci. China Inform. Sci. 2011, 54, 2079–2090. [Google Scholar] [CrossRef]
- Zhai, J.; Fei, S. Global practical tracking control for a class of uncertain non-linear systems. IET Control Theory Appl. 2011, 5, 1343–1351. [Google Scholar] [CrossRef]
- Alimhan, K.; Otsuka, N.; Alimhan, K.; Otsuka, N. A note on practically output tracking control of nonlinear systems that may not be linearizable at the origin. In Communications in Computer and Information Science; Springer: Berlin/Heidelberg, Germany, 2011; Volume 256, pp. 17–25. [Google Scholar]
- Yan, X.; Liu, Y. The further result on global practical tracking for high-order uncertain nonlinear systems. J. Syst. Sci. Complex. 2012, 25, 227–237. [Google Scholar] [CrossRef]
- Zhai, J.; Qian, C. Global control of nonlinear systems with uncertain output function using homogeneous domination approach. Int. J. Robust. Nonlinear Control 2012, 22, 1543–1561. [Google Scholar] [CrossRef]
- Alimhan, K.; Otsuka, N.; Adamov, A.A.; Kalimoldayev, M.N. Global practical output tracking of inherently non-linear systems using continuously differentiable controllers. Math. Probl. Eng. 2015, 2015, 932097. [Google Scholar] [CrossRef]
- Alimhan, K.; Otsuka, N.; Kalimoldayev, M.N.; Adamov, A.A. Output Tracking Problem of Uncertain Nonlinear Systems with High-Order Nonlinearities. In Proceedings of the 2015 8th International Conference on Control and Automation, Jeju, Korea, 25–28 November 2015; pp. 1–4. [Google Scholar]
- Song, Z.; Zhai, J. Practical output tracking control for switched nonlinear systems: A dynamic gain based approach. Nonlinear Anal. Hybrid Syst. 2018, 30, 147–162. [Google Scholar] [CrossRef]
- Guo, L.-C. Practical tracking control for stochastic nonlinear systems with polynomial function growth conditions. Automatika 2019, 60, 443–450. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhai, J. Practical tracking control for a class of high-order switched nonlinear systems with quantized input. ISA Trans. 2020, 96, 218–227. [Google Scholar] [CrossRef] [PubMed]
- Qian, C. A homogeneous domination approach for global output feedback stabilization of a class of non-linear systems. In Proceedings of the American Control Conference, Portland, OR, USA, 8–10 June 2005; pp. 4708–4715. [Google Scholar]
- Sun, Z.; Liu, Y.; Xie, X. Global stabilization for a class of high-order time-delay nonlinear systems. Int. J. Innov. Comput. Inf. Control 2011, 7, 7119–7130. [Google Scholar]
- Sun, Z.; Xie, X.; Liu, Z. Global stabilization of high-order nonlinear systems with multiple time delays. Int. J. Control 2013, 86, 768–778. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, X.; Xie, X. Continuous global stabilization of high-order time-delay nonlinear systems. Int. J. Control 2013, 86, 994–1007. [Google Scholar] [CrossRef]
- Chai, L. Global Output Control for a Class of Inherently Higher-Order Nonlinear Time-Delay Systems Based on Homogeneous Domination Approach. Discret. Dyn. Nat. Soc. 2013, 2013, 180717. [Google Scholar] [CrossRef]
- Zhai, J. Global output feedback stabilization for a class of nonlinear time-varying delay systems. Appl. Math. Comput. 2014, 228, 606–614. [Google Scholar] [CrossRef]
- Zhang, N.; Zhang, E.; Gao, F. Global Stabilization of High-Order Time-Delay Nonlinear Systems under a Weaker Condition. In Abstract and Applied Analysis; Hindawi: London, UK, 2014; pp. 1–8. [Google Scholar] [CrossRef]
- Gao, F.; Wu, Y. Further results on global state feedback stabilization of high-order nonlinear systems with time-varying delays. ISA Trans. 2015, 55, 41–48. [Google Scholar] [CrossRef] [PubMed]
- Gao, F.; Wu, Y. Global stabilisation for a class of more general high-order time-delay nonlinear systems by output feedback. Int. J. Control 2015, 88, 1540–1553. [Google Scholar] [CrossRef]
- Gao, F.; Wu, Y.; Yuan, F. Global output feedback stabilisation of high-order nonlinear systems with multiple time-varying delays. Int. J. Syst. Sci. 2016, 47, 2382–2392. [Google Scholar] [CrossRef]
- Zhang, X.; Lin, W.; Lin, Y. Nonsmooth Feedback Control of Time-Delay Nonlinear Systems: A Dynamic Gain Based Approach. IEEE Trans. Autom. Control 2016, 62, 438–444. [Google Scholar] [CrossRef]
- Yan, X.; Song, X. Global Practical Tracking by Output Feedback for Nonlinear Systems with Unknown Growth Rate and Time Delay. Sci. World J. 2014, 2014, 713081. [Google Scholar] [CrossRef] [PubMed]
- Jia, X.; Xu, S.; Chen, J.; Li, Z.; Zou, Y. Global output feedback practical tracking for time-delay systems with uncertain polynomial growth rate. J. Frankl. Inst. 2015, 352, 5551–5568. [Google Scholar] [CrossRef]
- Jia, X.; Xu, S. Global practical tracking by output feedback for nonlinear time-delay systems with uncertain polynomial growth rate. In Proceedings of the 2015 34th Chinese Control Conference (CCC), Hangzhou, China, 28–30 July 2015; pp. 607–611. [Google Scholar]
- Jia, X.; Xu, S.; Ma, Q.; Qi, Z.; Zou, Y. Global practical tracking by output feedback for a class of non-linear time-delay systems. IMA J. Math. Control Inf. 2016, 33, 1067–1080. [Google Scholar] [CrossRef]
- Alimhan, K.; Otsuka, N.; Kalimoldayev, M.N.; Tasbolatuly, N. Output Tracking by State Feedback for High-Order Nonlinear Systems with Time-Delay. J. Theor. Appl. Inf. Technol. 2019, 97, 942–956. [Google Scholar]
- Alimhan, K.; Mamyrbayev, O.; Erdenova, A.; Akmetkalyeva, A. Global output tracking by state feedback for high-order nonlinear systems with time-varying delays. Cogent Eng. 2020, 7, 1711676. [Google Scholar] [CrossRef]
- Alimhan, K.; Mamyrbayev, O.; Abdenova, G.; Akmetkalyeva, A. Output Tracking Control for High-Order Nonlinear Systems with Time Delay via Output Feedback Design. Symmetry 2021, 13, 675. [Google Scholar] [CrossRef]
- Rosier, L. Homogeneous Lyapunov function for homogeneous continuous vector field. Syst. Control Lett. 1992, 19, 467–473. [Google Scholar] [CrossRef]
- Polendo, J.; Qian, C. A universal method for robust stabilization of nonlinear systems: Unification and extension of smooth and non-smooth approaches. In Proceedings of the 2006 American Control Conference, Minneapolis, MN, USA, 14–16 June 2006. [Google Scholar]
- Polendo, J.; Qian, C. A generalized homogeneous domination approach for global stabilization of inherently nonlinear systems via output feedback. Int. J. Robust Nonlinear Control 2007, 17, 605–629. [Google Scholar] [CrossRef]
- Yang, B.; Lin, W. Nonsmooth output feedback design with a dynamics gain for uncertain systems with strong nonlinearity. In Proceedings of the 46th IEEE Conference Decision Control, New Orieans, LA, USA, 12–14 December 2007; pp. 3495–3500. [Google Scholar]
- Khalil, H.K. Nonlinear Systems; Prentice-Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Lu, X.; Li, H.; Wang, C.; Zhang, X. Stability analysis of positive switched impulsive systems with delay on time scales. Int. J. Robust Nonlinear Control. 2020, 30, 6879–6890. [Google Scholar] [CrossRef]
- Li, Y.; Li, H.; Ding, X. Set stability of switched delayed logical networks with application to finite-field consensus. Automatica 2020, 113, 108768. [Google Scholar] [CrossRef]
- Zheng, Y.; Li, H.; Feng, J.E. State-feedback set stabilization of logical control networks with state-dependent delay. Sci. China Inf. Sci. 2021, 64, 169203. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alimhan, K.; Mamyrbayev, O.; Adamov, A.; Alisheva, S.; Oralbekova, D. Global Practical Output Tracking for a Class of Uncertain Inherently Time-Varying Delay Nonlinear Systems by Output Feedback. Computation 2022, 10, 187. https://doi.org/10.3390/computation10100187
Alimhan K, Mamyrbayev O, Adamov A, Alisheva S, Oralbekova D. Global Practical Output Tracking for a Class of Uncertain Inherently Time-Varying Delay Nonlinear Systems by Output Feedback. Computation. 2022; 10(10):187. https://doi.org/10.3390/computation10100187
Chicago/Turabian StyleAlimhan, Keylan, Orken Mamyrbayev, Abilmazhin Adamov, Sandugash Alisheva, and Dina Oralbekova. 2022. "Global Practical Output Tracking for a Class of Uncertain Inherently Time-Varying Delay Nonlinear Systems by Output Feedback" Computation 10, no. 10: 187. https://doi.org/10.3390/computation10100187
APA StyleAlimhan, K., Mamyrbayev, O., Adamov, A., Alisheva, S., & Oralbekova, D. (2022). Global Practical Output Tracking for a Class of Uncertain Inherently Time-Varying Delay Nonlinear Systems by Output Feedback. Computation, 10(10), 187. https://doi.org/10.3390/computation10100187






