Application of Large Eddy Simulation to Predict Underwater Noise of Marine Propulsors. Part 1: Cavitation Dynamics
Abstract
:1. Introduction
1.1. State of the Art
- Turbulence;
- Two-phase flow;
- Noise source description and propagation.
1.2. Contributions of Current Work
2. Methods
2.1. Methodology
2.1.1. Resolving Turbulence
2.1.2. Two-Phase Flow
- Viscous effects;
- Nonspherical bubble shape;
- Interface surface tension and sliding interfaces;
- Bubble interactions;
- Compressibility.
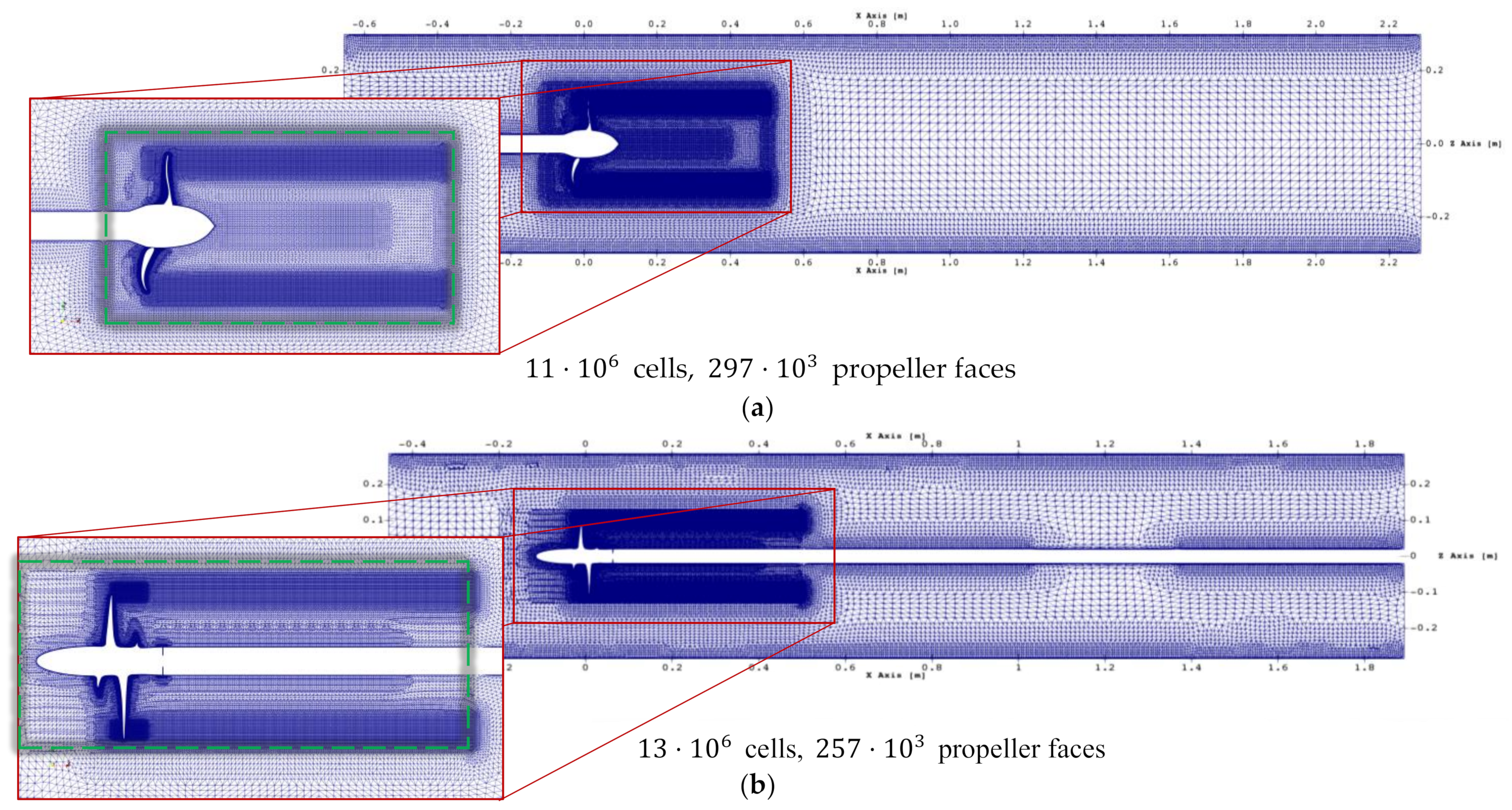
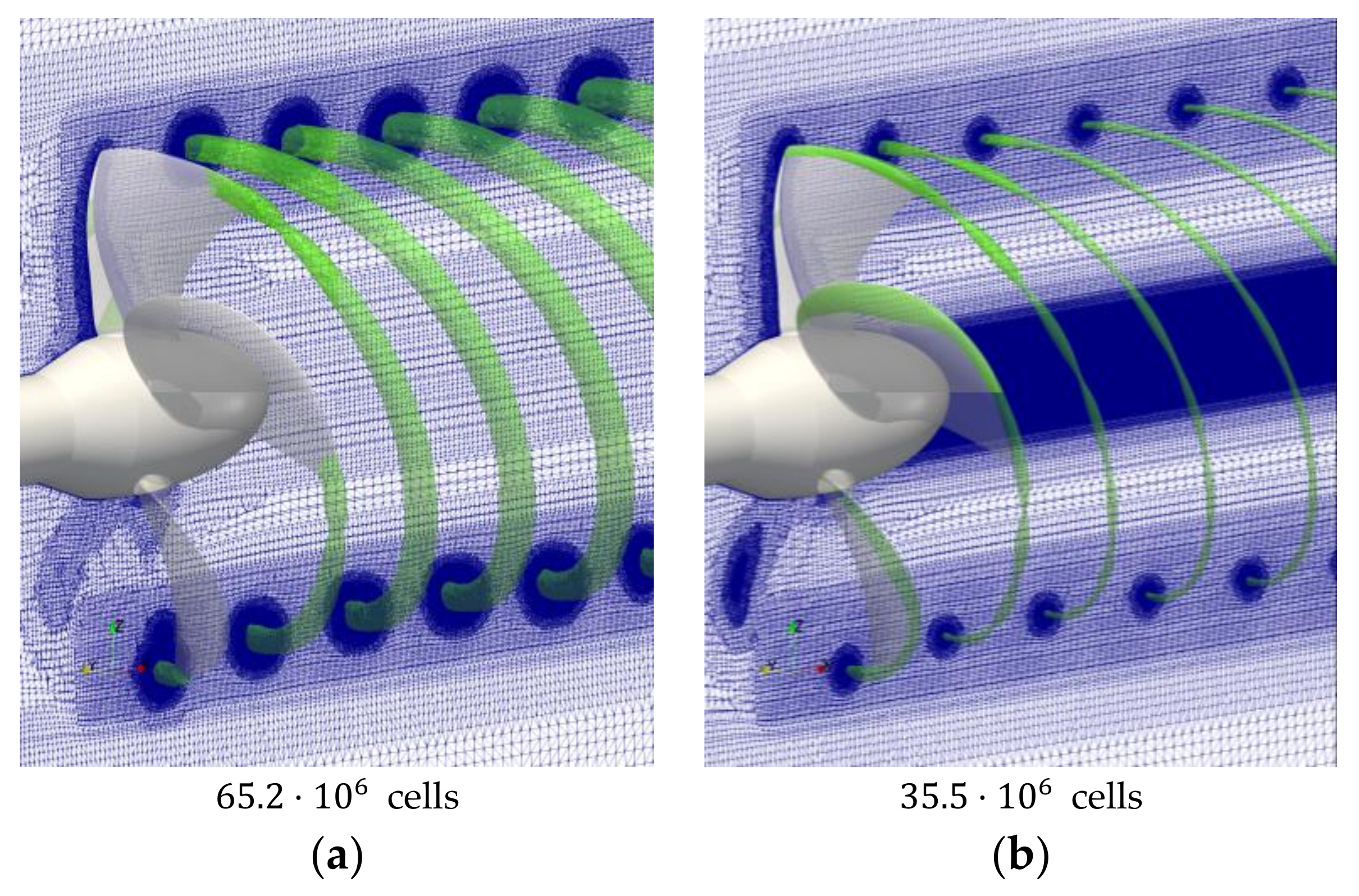
2.2. Numerical Setup
- To ensure that non-axisymmetric turbulence and cavity structures at the symmetry axis are resolved;
- In subsequent behind-ship condition setups, the non-axisymmetric inflow caused by the ship wakefield or inclined shaft lines and possible structural obstacles in the propeller slipstream, such as rudders or azimuthing propulsor housings, have to be considered.
3. Results
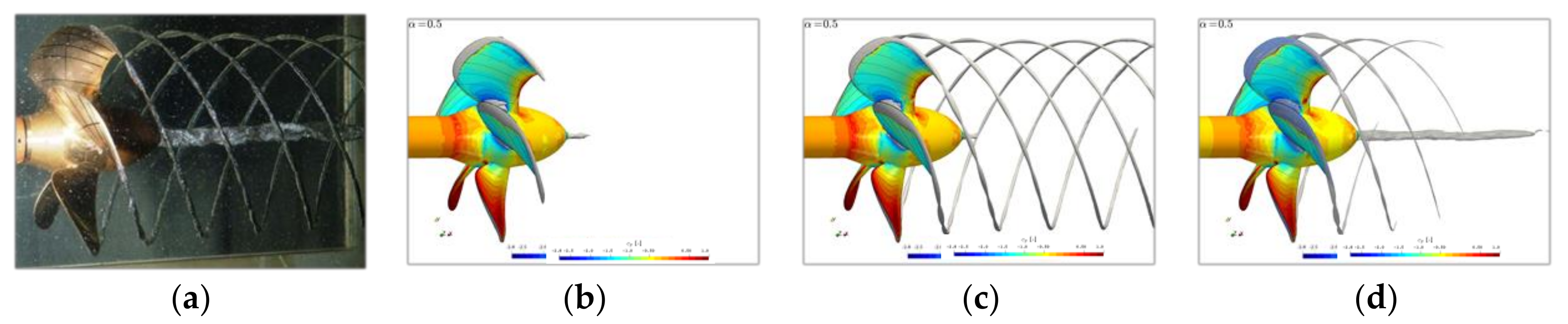
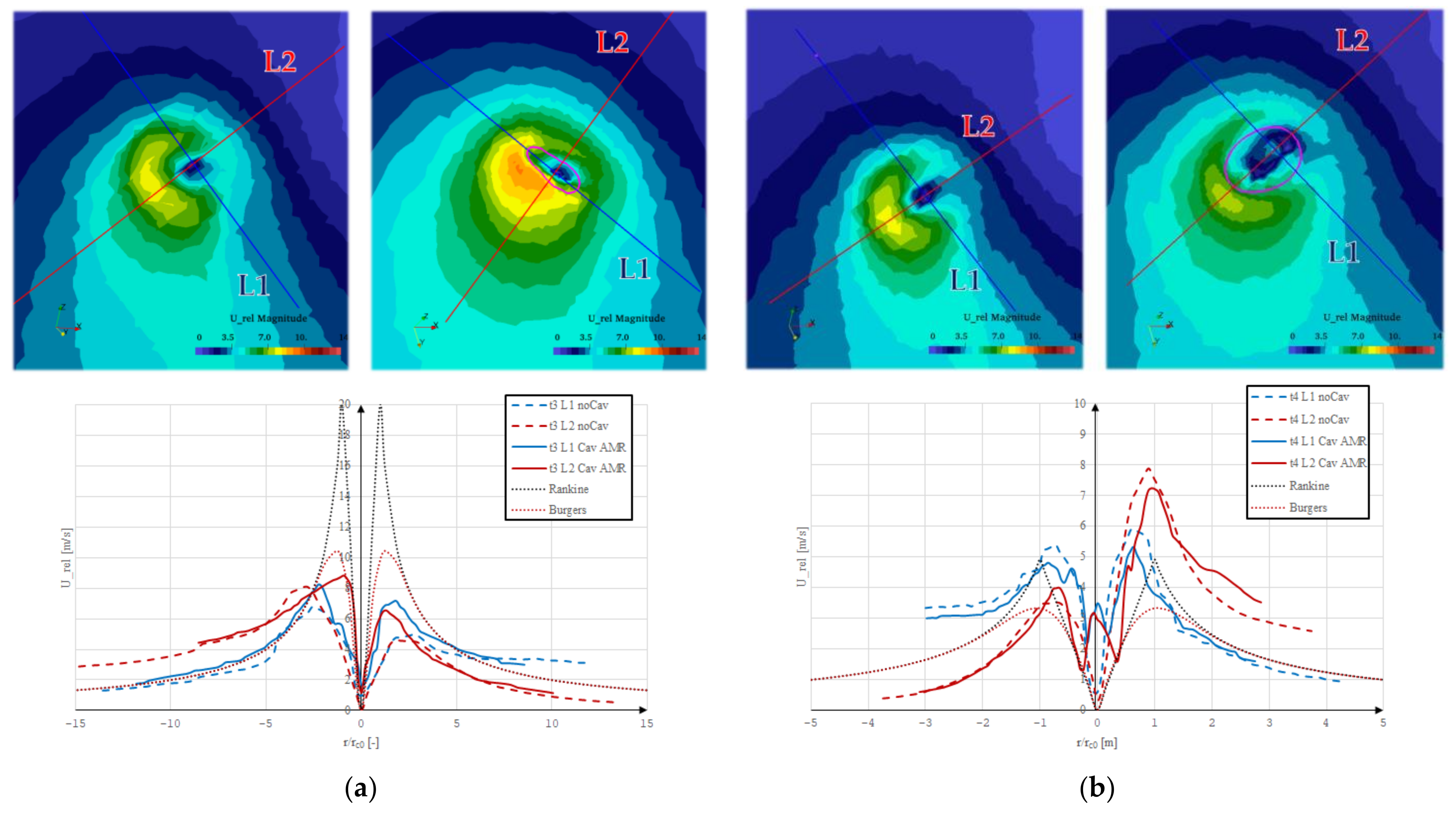
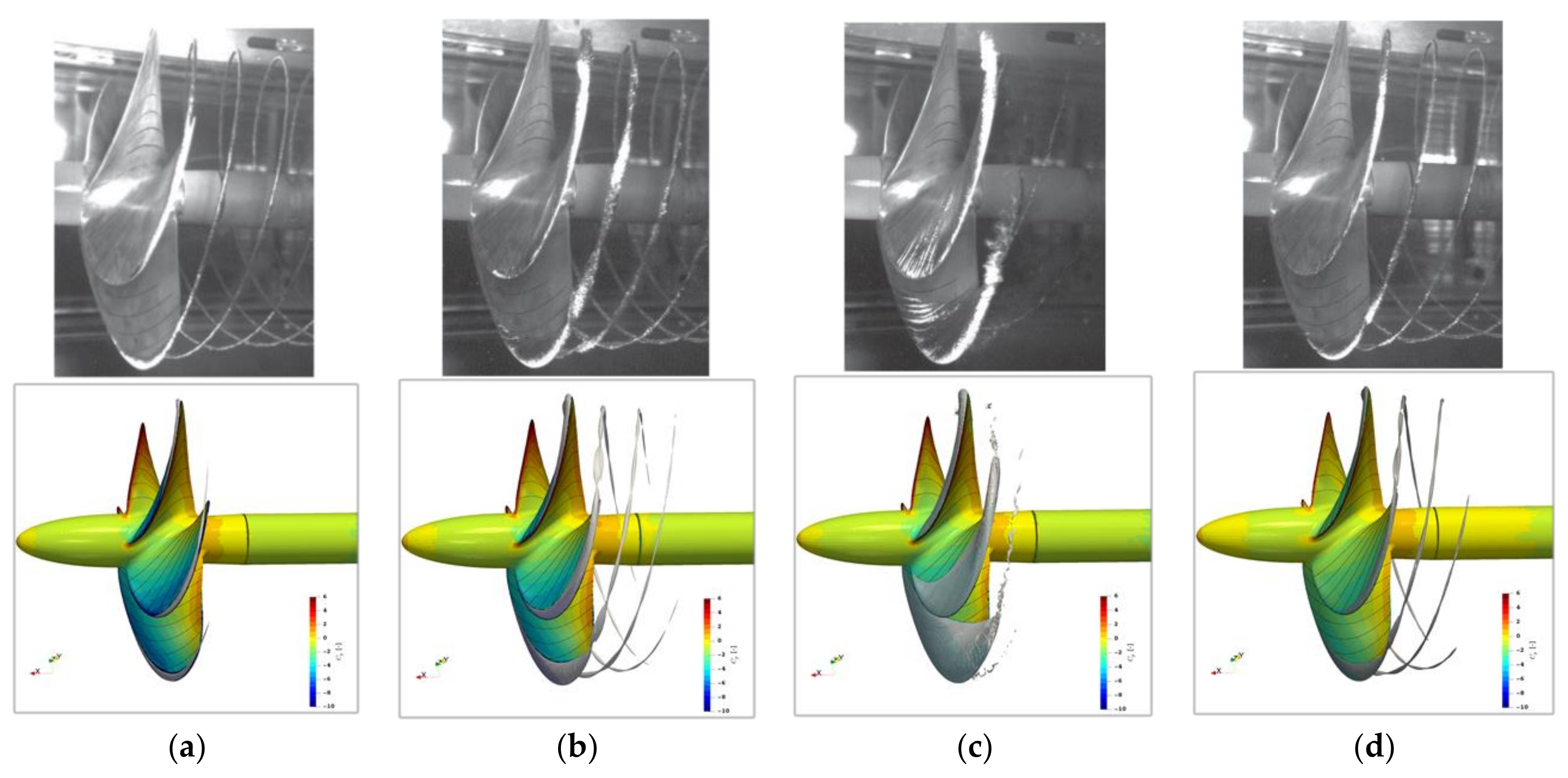
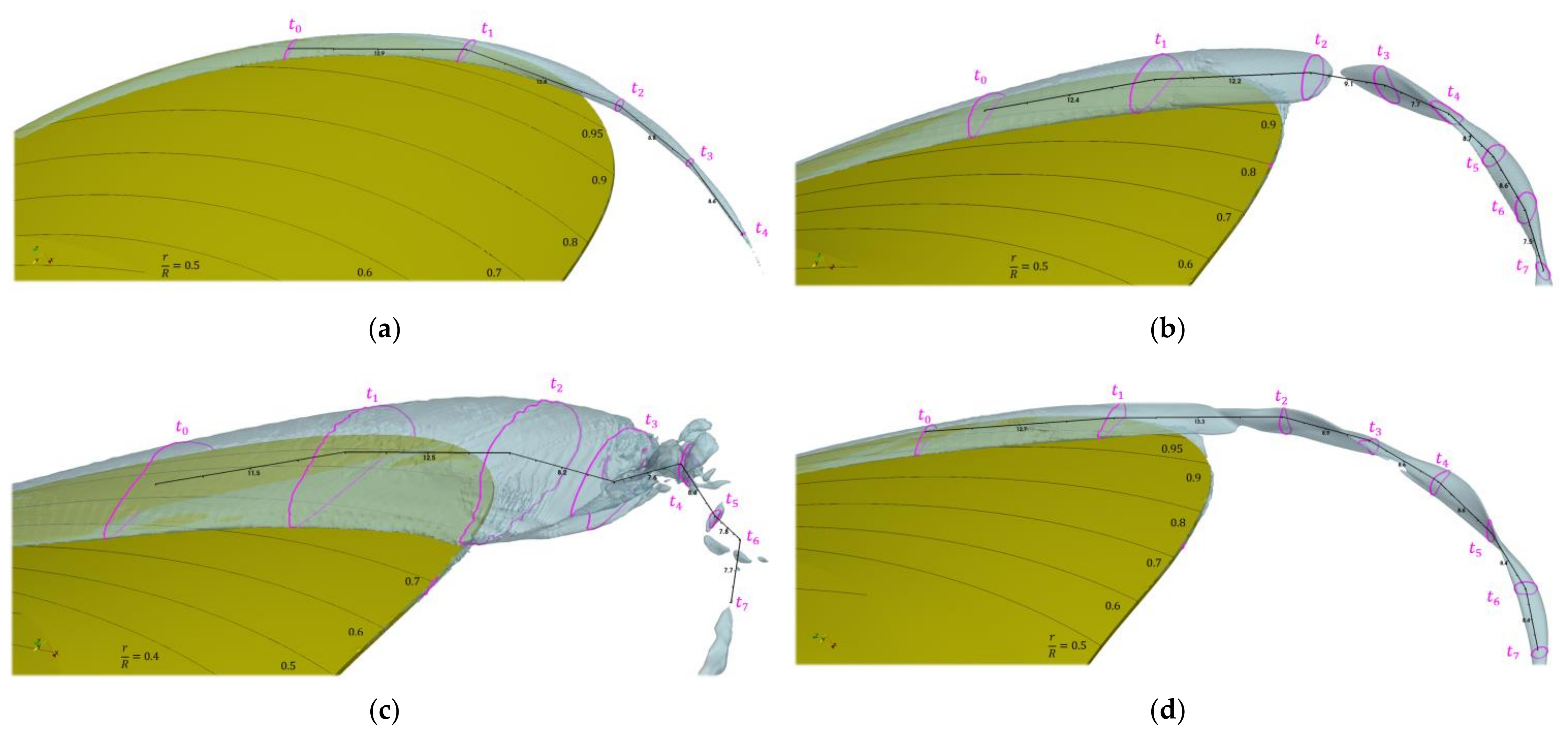
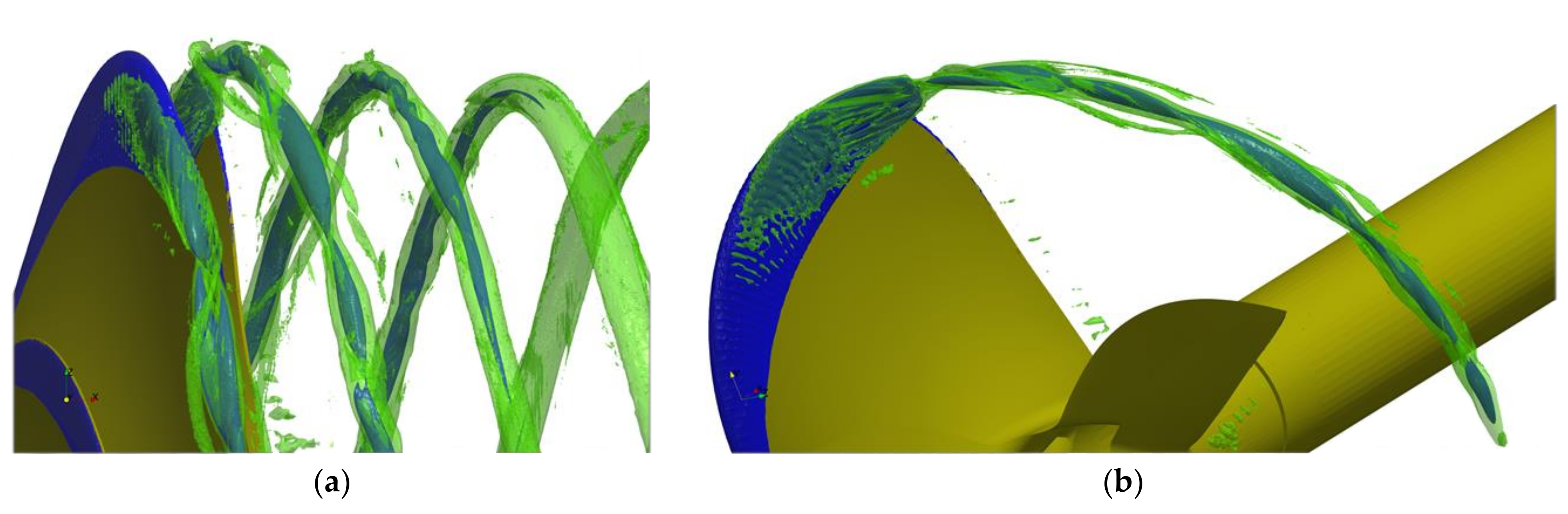
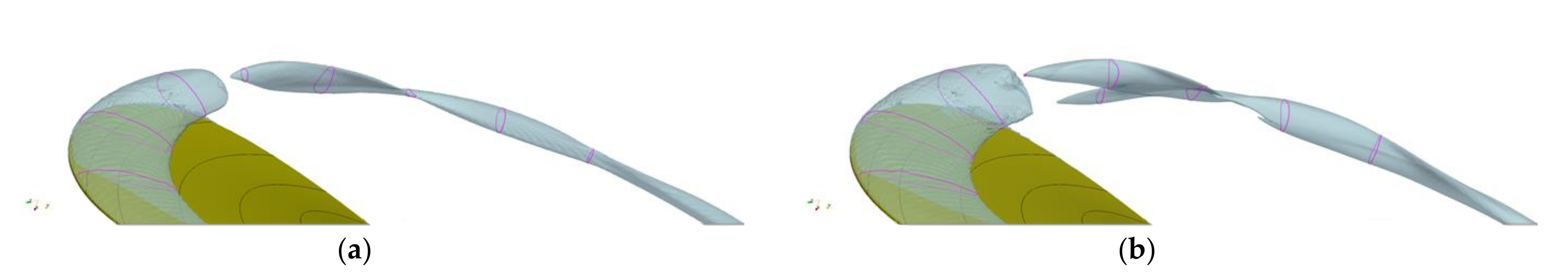
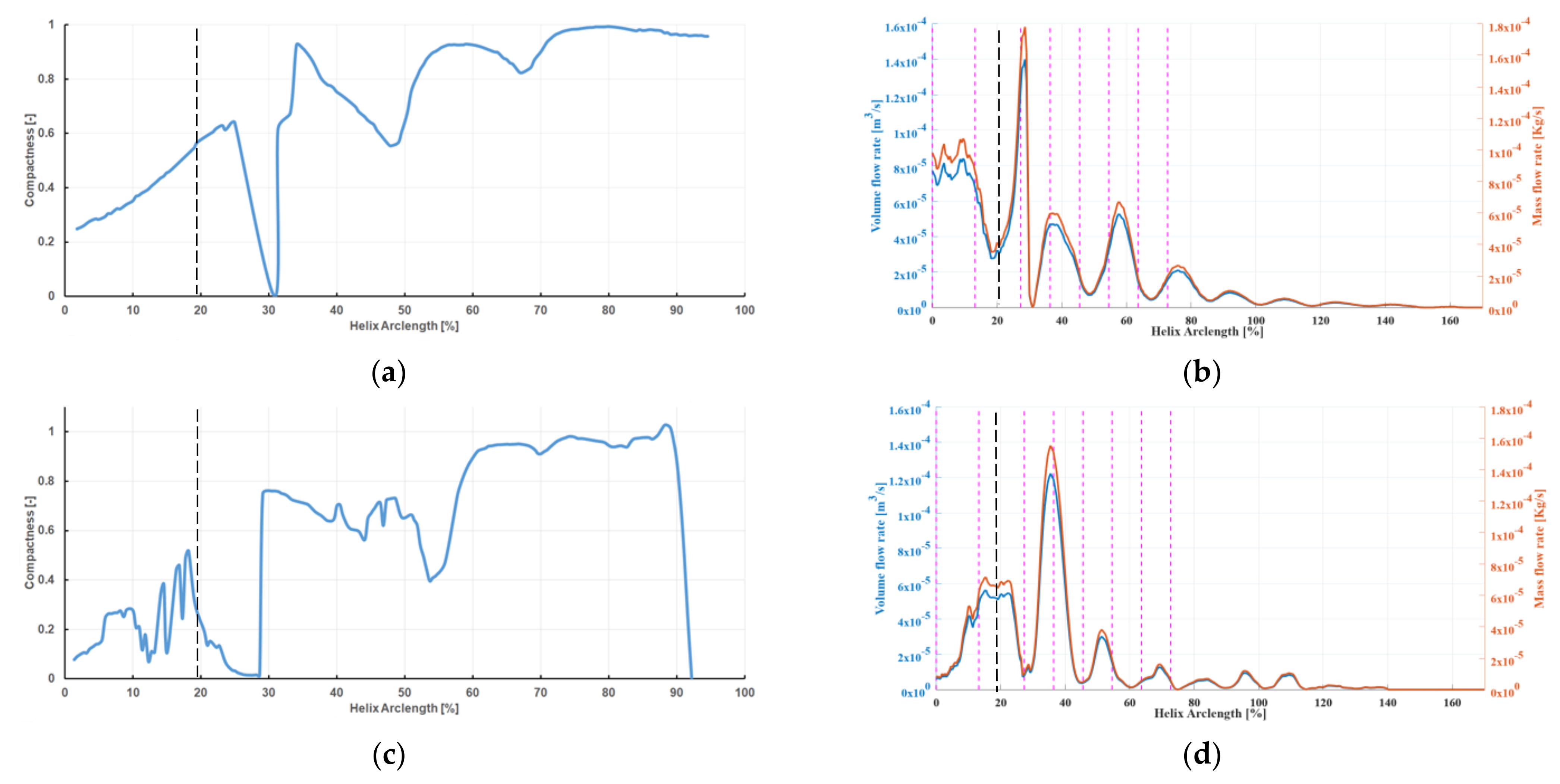
3.1. PPTC’11
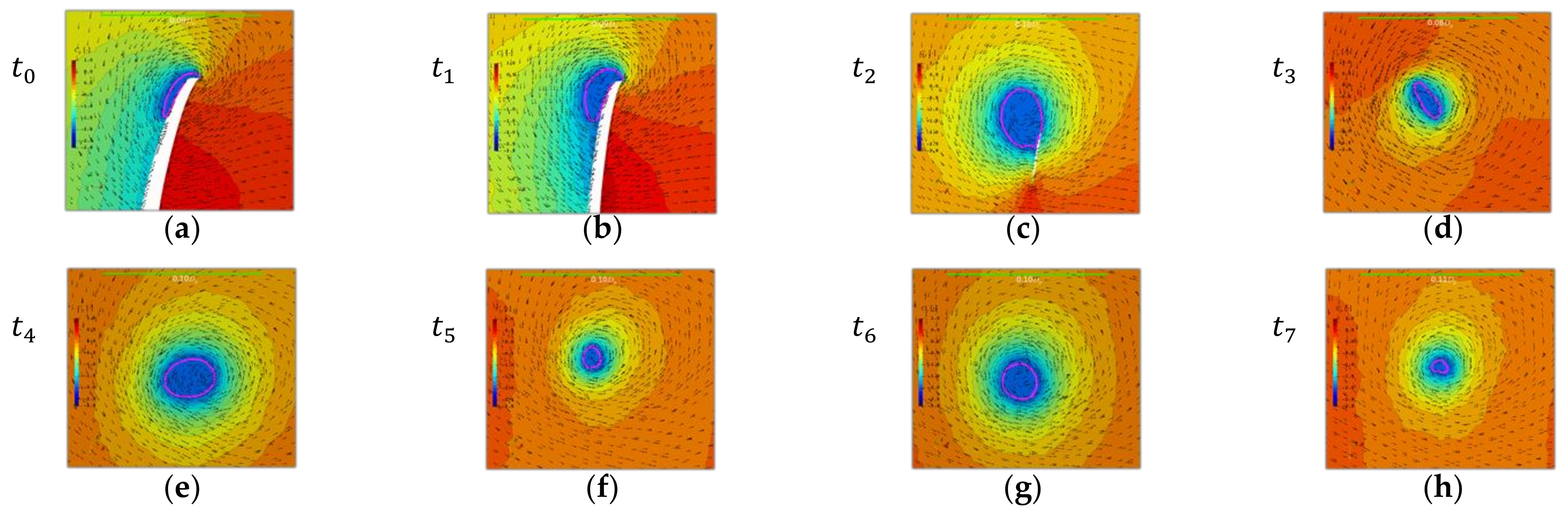
- The source of the formation of the cavity is the reduced pressure due to the accelerated flow (a–c), in accordance with Bernoulli’s principle;
- The assumed elliptical shape of the vortex is the result of the orientation of the detaching sheet along the blade surface;
- The conserved angular momentum along the running length maintains the cavity diameter, in accordance with Kelvin’s circulation theorem;
- The deformation of the ellipse to an energetically more favorable circular cross-sectional shape of the cavity takes place in the regions (d,e) vs. (f,g), as the surface tension is not considered in the Schnerr-Sauer model.
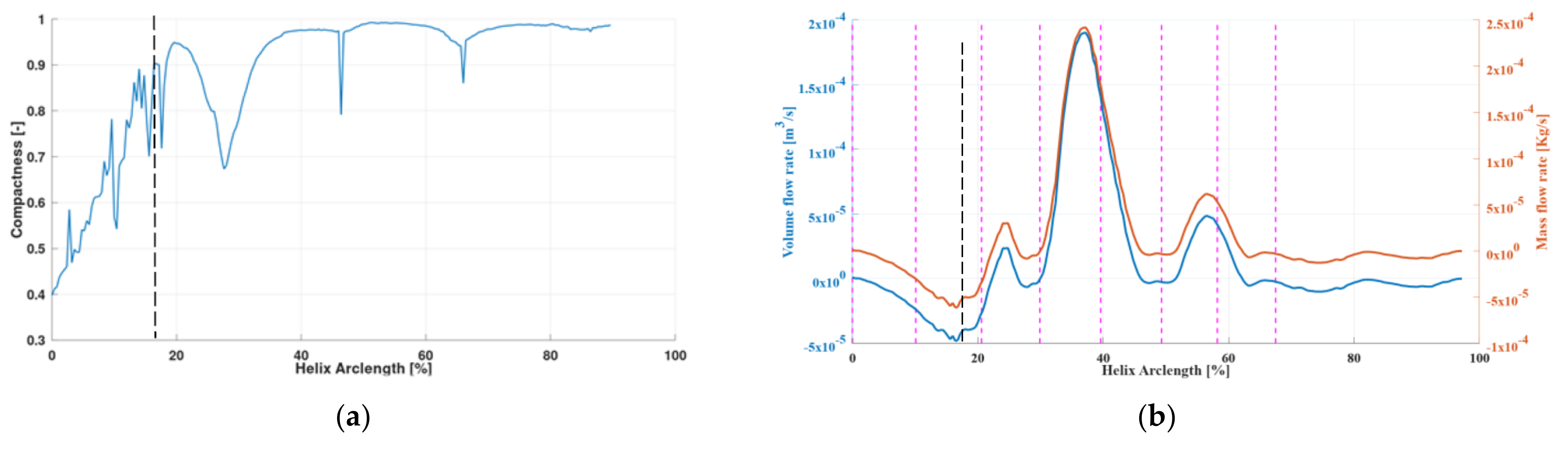
3.2. Newcastle Propeller Test Case
4. Discussion
4.1. Forces Prediction
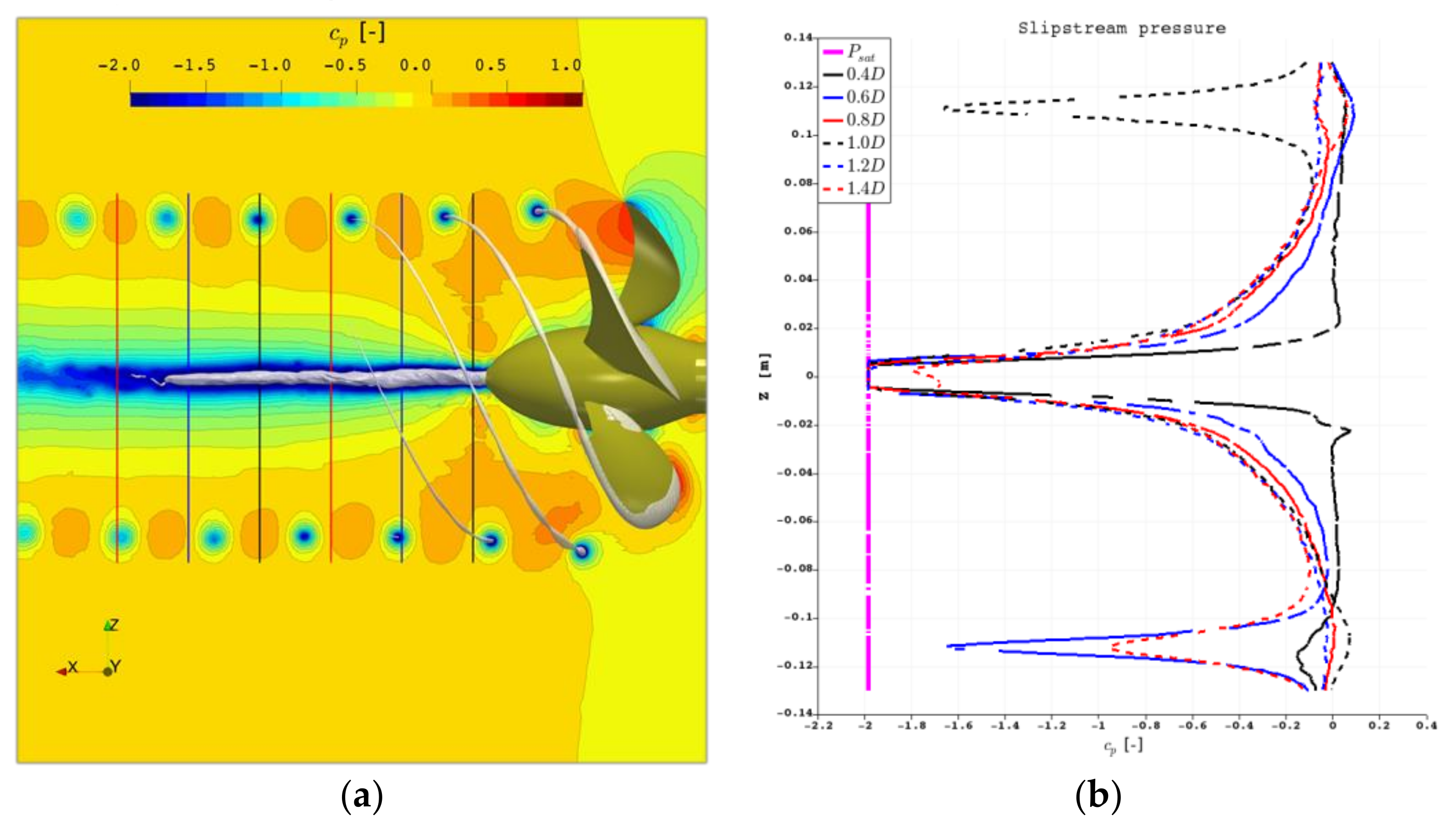
4.2. Noise Generation Mechanisms
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Van Wijngaarden, H.C.J. Prediction of Propeller-Induced Hull-Pressure Fluctuations; Maritime Research Institute Netherlands: Wageningen, The Netherlands, 2011. [Google Scholar]
- Berger, S. Numerical Analysis of Propeller-Induced Higher-Order Pressure Fluctuations on the Ship Hull; Schriftenreihe Schiffbau der Technischen Universität Hamburg-Harburg: Hamburg, Germany, 2018. [Google Scholar]
- Bosschers, J. Analysis of inertial waves on inviscid cavitating vortices in relation to lowfrequency radiated noise. In Proceedings of the Warwick Innovative Manufacturing Research Centre (WIMRC) Cavitation: Turbo-machinery and Medical Applications Forum, Coventry, UK, 7–9 July 2008. [Google Scholar]
- Asnaghi, A.; Bensow, R.E.; Svennberg, U. Comparative analysis of tip vortex flow using RANS and LES. In Proceedings of the VII International Conference on Computational Methods in Marine Engineering, Nantes, France, 15–17 May 2017. [Google Scholar]
- Bensow, R.E.; Liefvendahl, M. An acoustic analogy and scale-resolving flow simulation methodology for the prediction of propeller radiated noise. In Proceedings of the 31st Symposium on Naval Hydrodynamics, Monterey, CA, USA, 11–16 September 2016. [Google Scholar]
- Bensow, R.; Liefvendahl, M. Implicit and Explicit Subgrid Modeling in LES Applied to a Marine Propeller. Proceeding of the 38th fluid dynamics conference and exhibit, Siattle, DC, USA, 23–26 June 2008. [Google Scholar]
- Kimmerl, J.; Mertes, P.; Krasilnikov, V.; Koushan, K.; Savio, L.; Felli, M.; Abdel-Maksoud, M.; Göttsche, U.; Reichstein, N. Analysis Methods and Design Measures for the Reduction of Noise and Vibration Induced by Marine Propellers; Universitätsbibliothek der RWTH Aachen: Aachen, Germany, 2019. [Google Scholar]
- Asnaghi, R.E. Implicit Large Eddy Simulation of Tip Vortex on an Elliptical Foil. In Proceedings of the Fifth International Symposium on Marine Propulsion, Espoo, Finland, 12–15 June 2017. [Google Scholar]
- Tani, G.; Aktas, B.; Viviani, M.; Atlar, M. Two medium size cavitation tunnel hydro-acoustic benchmark experiment comparisons as part of a round robin test campaign. Ocean Eng. 2017, 138, 179–207. [Google Scholar] [CrossRef] [Green Version]
- Krasilnikov, V. CFD modelling of hydroacoustic performance of marine propellers: Predicting propeller cavitation. In Proceedings of the 22nd Numerical Towing Tank Symposium, Tomar, Portugal, 29 September–1 October 2019. [Google Scholar]
- Kuiper, G. Cavitation Inception on Ship Propeller Models. Ph.D. Thesis, TU Delft, Wageningen, The Netherlands, 1981. [Google Scholar]
- Viitanen, V.M.; Hynninen, A.; Sipilä, T.; Siikonen, T. DDES of Wetted and Cavitating Marine Propeller for CHA Underwater Noise Assessment. J. Mar. Sci. Eng. 2018, 6, 56. [Google Scholar] [CrossRef] [Green Version]
- Sagaut, P. Large Eddy Simulation for Incompressible Flows; Springer: New York, NY, USA, 2006. [Google Scholar]
- Arndt, R.E.A.; Arakeri, V.H.; Higuchi, H. Some observations of tip-vortex cavitation. J. Fluid Mech. 1991, 229, 169–289. [Google Scholar] [CrossRef]
- Felli, M.; Falchi, M. Propeller tip and hub vortex dynamics in the interaction with a rudder. Exp. Fluids 2011, 51, 1385–1402. [Google Scholar] [CrossRef] [Green Version]
- Zhang, G.; Khlifa, I.; Fezzaa, K.; Ge, M.; Coutier-Delgosha, O. Experimental investigation of internal two-phase flow structures and dynamics of quasi-stable sheet cavitation by fast synchrotron X-ray imaging. Phys. Fluids 2020, 32, 113310. [Google Scholar] [CrossRef]
- Van Terwisga, T.J.; Li, Z.R.; Fitzsimmons, P.A.; Foeth, E.J. Cavitation Erosion—A review of physical mechanisms and erosion risk models. In Proceedings of the 7th International Symposium on Cavitation, Ann Arbor, MI, USA, 16–20 August 2009. [Google Scholar]
- Sezen, S.; Atlar, M.; Fitzsimmons, P. Prediction of cavitating propeller underwater radiated noise using RANS & DES-based hybrid method. Ships Offshore Struct. 2021. [Google Scholar] [CrossRef]
- Yilmaz, N.; Atlar, M.; Fitzsimmons, P. An Improved Tip Vortex Cavitation Model for Propeller-Rudder Interaction. In Proceedings of the 10th International Cavitation Symposium, Baltimore, MD, USA, 13–16 May 2018. [Google Scholar]
- Viitanen, V.; Siikonen, T.; Sánchez-Caja, A. Cavitation on Model- and Full-Scale Marine Propellers: Steady and Transient Viscous Flow Simulations at Different Reynolds Numbers. J. Mar. Sci. Eng. 2020, 8, 141. [Google Scholar] [CrossRef] [Green Version]
- Bretschneider, H.; Bosschers, J.; Choi, G.H.; Ciappi, E.; Farabee, T.; Kawakita, C.; Tang, D. Specialist Committee on Hydrodynamic Noise. In Proceedings of the Final report and recommendations to the 27th ITTC, Copenhagen, Denmark, 31 August–5 September 2014. [Google Scholar]
- International Maritime Organization. Guidelines for the Reduction of Underwater Noise from Commercial Shipping to Address Adverse Impacts on Marine Life; International Maritime Organization: London, UK, 2014. [Google Scholar]
- DNV GL. Silent Class Notation; DNV GL AS: Bærum, Norwegen, 2010. [Google Scholar]
- Viitanen, V.; Siikonen, T.; Sánchez-Caja, A. Numerical Viscous Flow Simulations of Cavitating Propeller Flows at Different Reynolds Numbers. In Proceedings of the Sixth International Symposium on Marine Propulsors, Rome, Italy, 26–30 May 2019. [Google Scholar]
- Kimmerl, J.; Mertes, P.; Abdel-Maksoud, M. Turbulence Modelling Capabilities of ILES for Propeller Induced URN Prediction. In SNAME Maritime Convention; SNAME: Houston, TX, USA, 2020. [Google Scholar]
- Menter, F.R. Best Practice: Scale-Resolving Simulations in ANSYS CFD; ANSYS Germany GmbH: Nürnberg, Germany, 2015. [Google Scholar]
- Krasilnikov, V.; Abdel-Maksoud, M.; Göttsche, U.; Wang, Y.; Koushan, K.; Kimmerl, J.; Mertes, P.; Felli, M.; Reichstein, N.; Stück, R.; et al. Study on Analysis Methods for Marine Propeller Cavitation and the Radiated Noise; Hauptversammlung der Schiffbautechnischen Gesellschaft: Papenburg, Germany, 2019. [Google Scholar]
- SVA Potsdam GmbH. Potsdam Propeller Test Case (PPTC)—Cavitation Tests with the Model Propeller VP1304; Report 3753, Online; Schiffbau-Versuchsanstalt: Potsdam, Germany, 2011. [Google Scholar]
- Tani, G.; Viviani, M.; Felli, M.; Lafeber, F.H.; Lloyd, T.; Aktas, B.; Atlar, M.; Seol, H.; Hallander, J.; Sakamoto, N.; et al. Round Robin Test on Radiated Noise of a Cavitating Propeller. In Proceedings of the Sixth International Symposium on Marine Propulsors, Rome, Italy, 20 May 2019. [Google Scholar]



| Parameter | ||
|---|---|---|
| Unit | ||
| Value |
| Condition | C1 | C2 | C3 | C6 |
|---|---|---|---|---|
| [Hz] | 35 | 35 | 35 | 35 |
| Type | Step [°] | Q-Isovalue of with Axial Velocity | [-] | Effort (2 rot/100 CPUs) [d] |
|---|---|---|---|---|
| RANS | 10 |  | 1.38–550.49 | 0.1 |
| RANS | 1 |  | 0.13–72.38 | 0.32 |
| RANS | 0.1 |  | 0.01–7.33 | 3.4 |
| ILES | 1 |  | 0.13–66.20 | 0.4 |
| ILES | 0.1 | 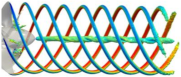 | 0.01–6.95 | 3.0 |
| ILES | 0.1 | 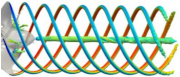 | 0.001–0.66 | 28.0 |
| Step [°] | Q-Isovalue of with Axial Velocity | [-] | Effort (2 rot/100 CPUs) [d] |
|---|---|---|---|
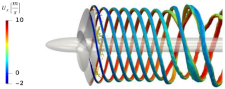 | 0.03–11.51 | 0.72 | |
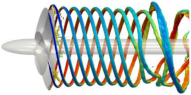 | 0.00–3.44 | 1.87 |
| Cell Count [ Cells] | ||
|---|---|---|
| Mesh | PPTC’11 | Newcastle |
| Initial mesh | ||
| Refinement step 1 | C1: C2: C3: C6: | |
| Refinement step 2 | - | |
| Investigation | [-] | Δ [%] | Δ [%] | |
|---|---|---|---|---|
| Experiment (Cav Off) | 0.381 | - | 0.976 | - |
| Experiment (Cav On) | 0.374 | - | 0.970 | - |
| RANS k-ω-SSTawt: tdiCoupled (Cav Off) | 0.381 | 0.1 | 1.008 | 3.3 |
| ILES: pimpleDyMFoam (Cav Off) | 0.396 | 4.1 | 0.995 | 1.9 |
| LES Sm..: interPhaseChangeDyMFoam (Cav Off) | 0.383 | 0.4 | 1.027 | 5.2 |
| ILES: interPhaseChangeDyMFoam (Cav On) | 0.429 | 14.9 | 0.995 | 2.6 |
| Investigation | [-] | Δ [%] | Δ [%] | |
|---|---|---|---|---|
| -Condition: C1, C2, C3 | ||||
| Experiment | 0.242 | - | 0.341 | - |
| RANS k-ω-SST: sdiCoupled (steady, Cav Off) | 0.243 | −0.6 | 0.340 | 0.3 |
| ILES: interPhaseChangeDyMFoam (Cav On) | 0.231 | 4.6 | 0.358 | 0.9 |
| -Condition: C6 | ||||
| Experiment | 0.190 | - | 0.284 | - |
| RANS k-kl-ω: interPhaseChangeDyMFoam (Cav Off) | 0.182 | −4.1 | 0.271 | −4.5 |
| ILES: interPhaseChangeDyMFoam (Cav On) | 0.204 | 7.2 | 0.318 | 11.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kimmerl, J.; Mertes, P.; Abdel-Maksoud, M. Application of Large Eddy Simulation to Predict Underwater Noise of Marine Propulsors. Part 1: Cavitation Dynamics. J. Mar. Sci. Eng. 2021, 9, 792. https://doi.org/10.3390/jmse9080792
Kimmerl J, Mertes P, Abdel-Maksoud M. Application of Large Eddy Simulation to Predict Underwater Noise of Marine Propulsors. Part 1: Cavitation Dynamics. Journal of Marine Science and Engineering. 2021; 9(8):792. https://doi.org/10.3390/jmse9080792
Chicago/Turabian StyleKimmerl, Julian, Paul Mertes, and Moustafa Abdel-Maksoud. 2021. "Application of Large Eddy Simulation to Predict Underwater Noise of Marine Propulsors. Part 1: Cavitation Dynamics" Journal of Marine Science and Engineering 9, no. 8: 792. https://doi.org/10.3390/jmse9080792
APA StyleKimmerl, J., Mertes, P., & Abdel-Maksoud, M. (2021). Application of Large Eddy Simulation to Predict Underwater Noise of Marine Propulsors. Part 1: Cavitation Dynamics. Journal of Marine Science and Engineering, 9(8), 792. https://doi.org/10.3390/jmse9080792







