Surface Crack Growth in Offshore Metallic Pipes under Cyclic Loads: A Literature Review
Abstract
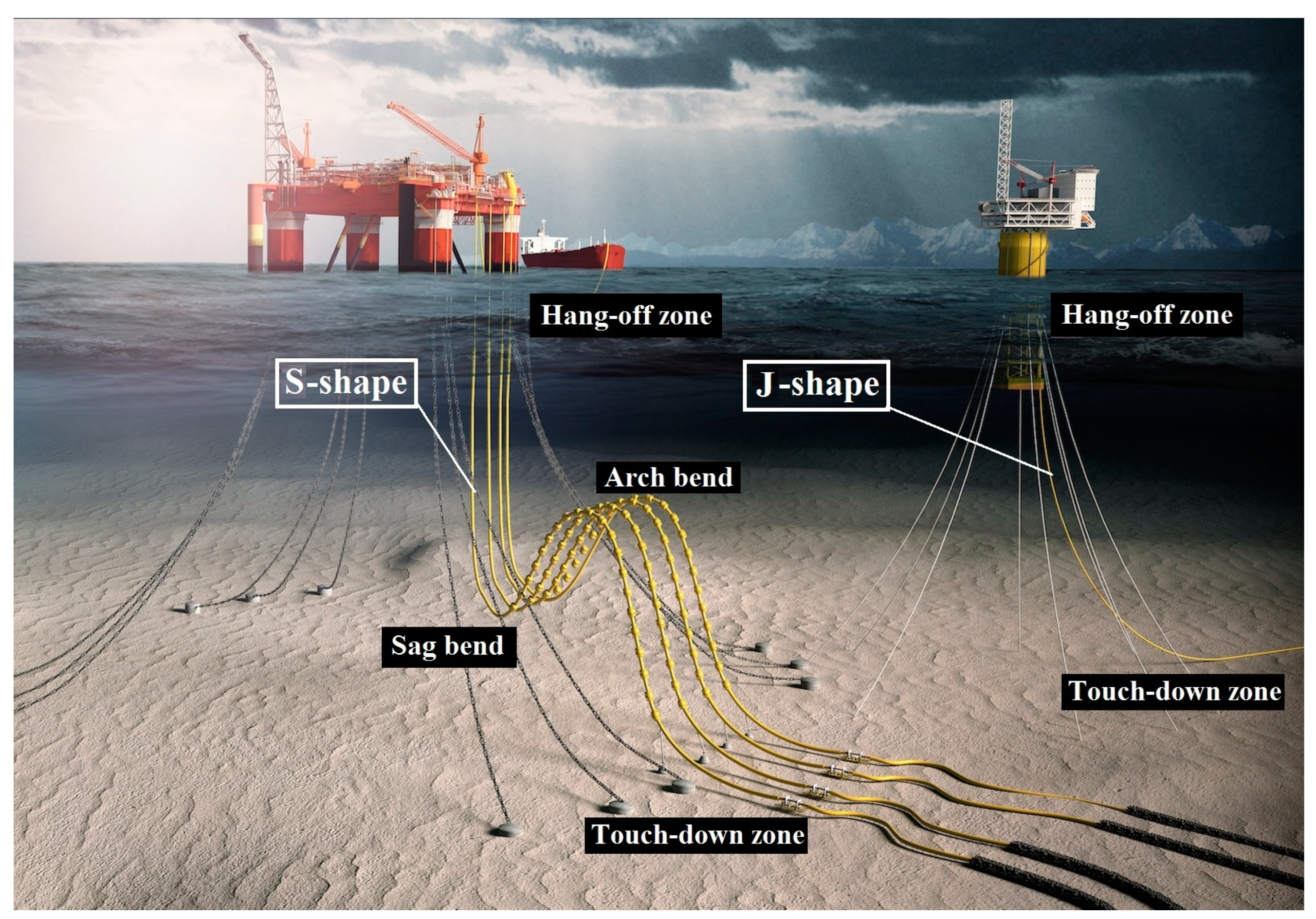
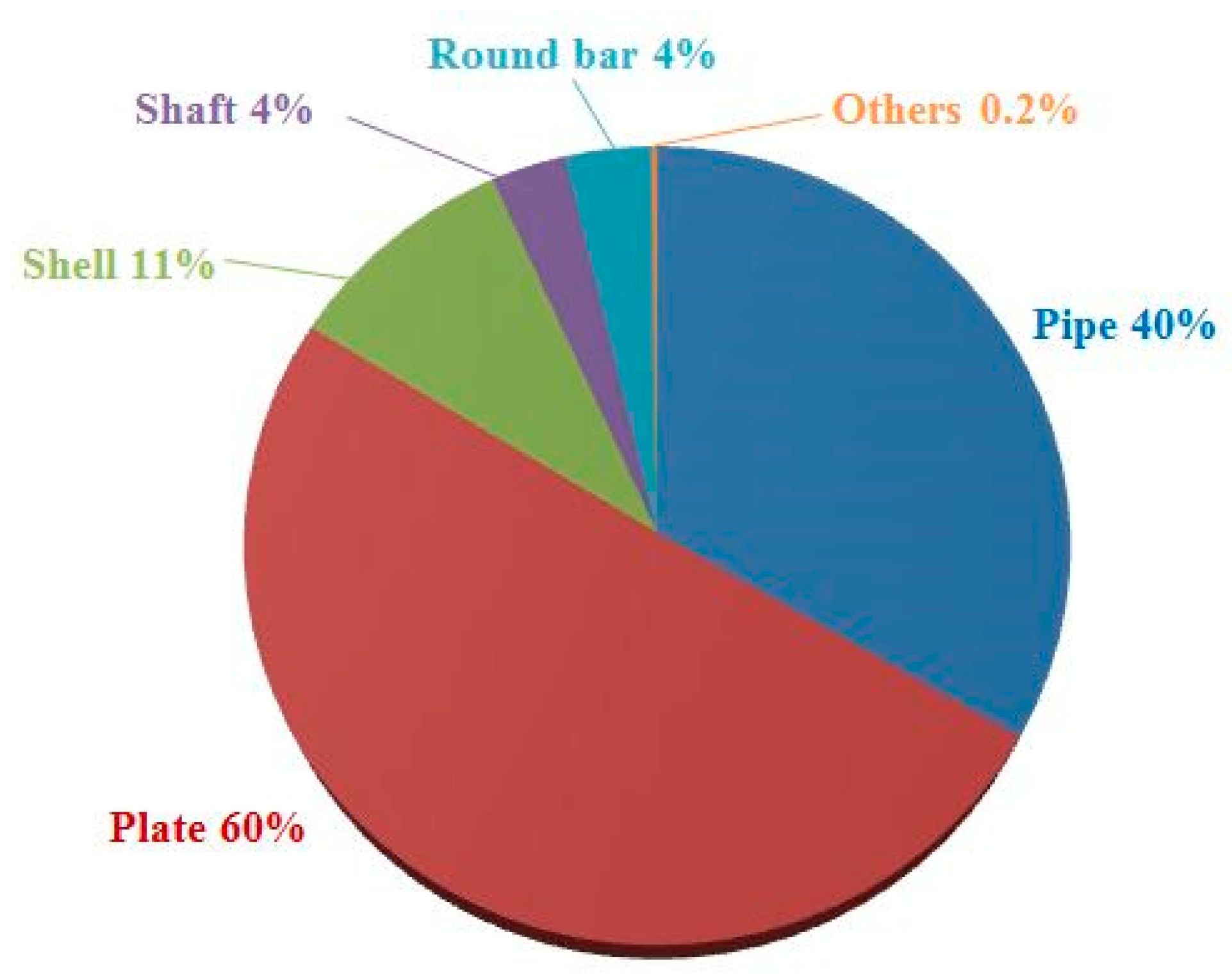
1. Introduction
2. Overview of the Research on Surface Crack Growth in Metallic Pipes
2.1. Experimental Research
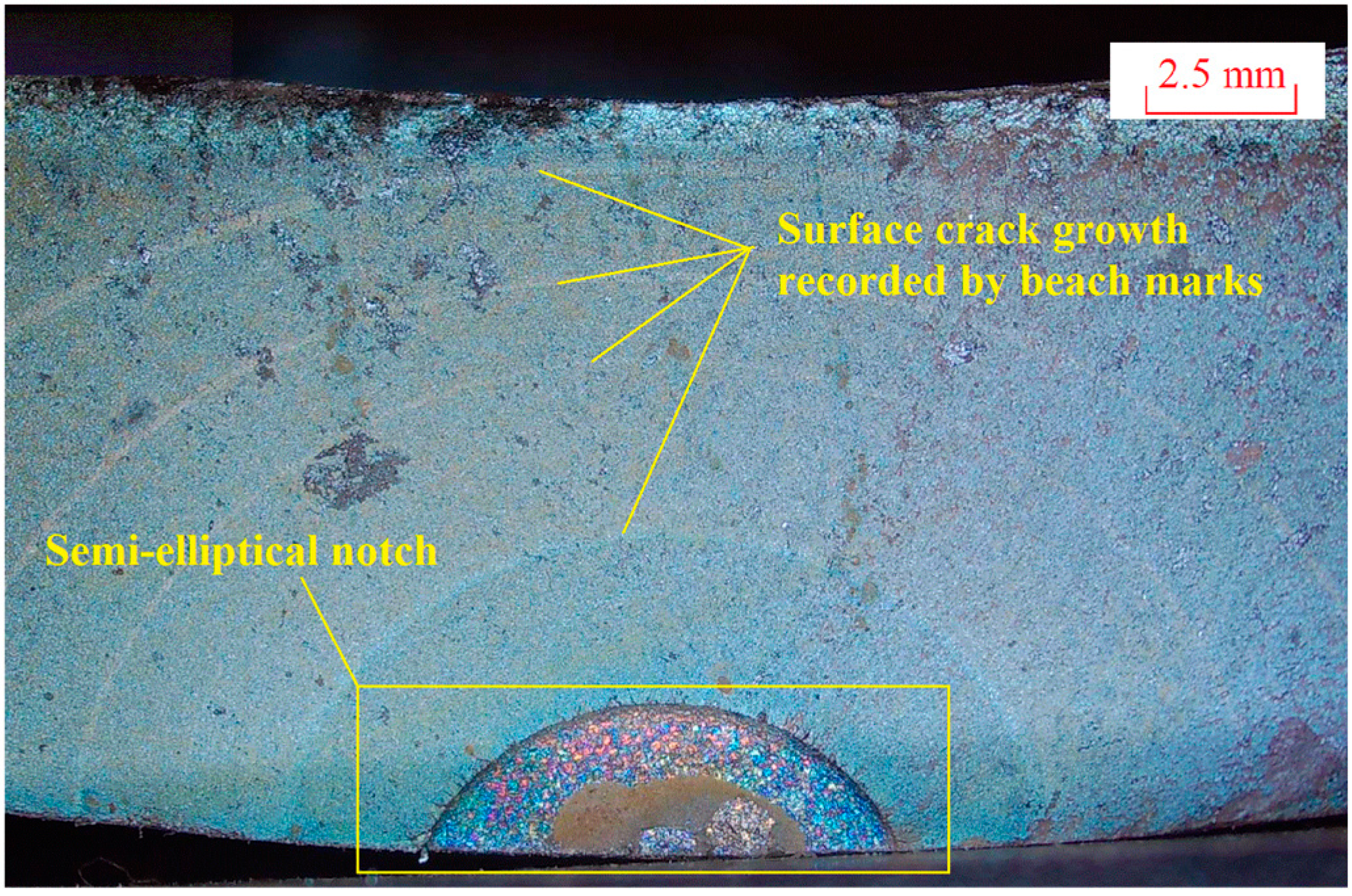
2.1.1. Experimental Procedures and Methods
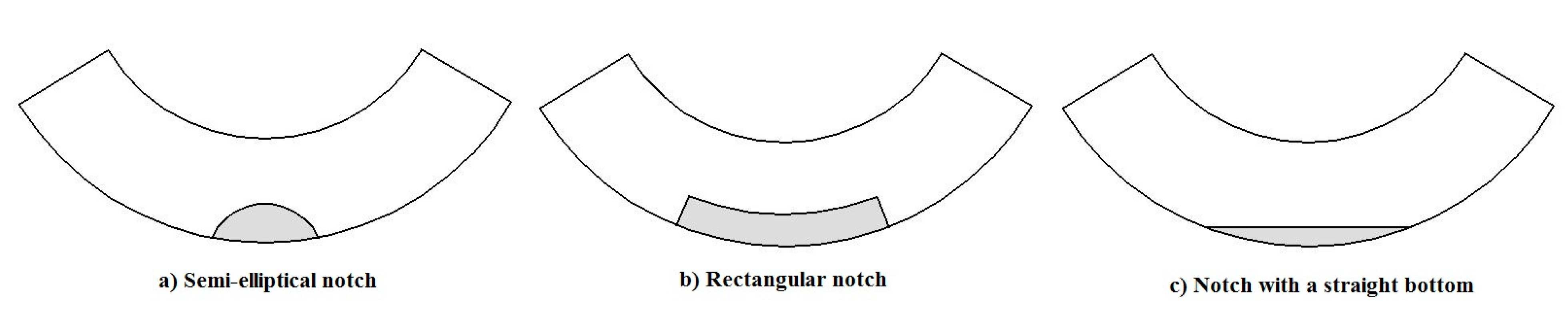
2.1.2. Crack Orientation and Load Cases
2.1.3. Load Ratio and the Paris Constants
2.2. Numerical Simulation of Predicting Surface Crack Growth in Metallic Pipes
2.2.1. FE modelling Strategy
2.2.2. Crack and Pipe Dimensions
2.2.3. Crack Propagation Evaluation
2.3. Analytical Methods of Predicting Surface Crack Growth in Metallic Pipes
2.3.1. The Foundation of Evaluating Surface Crack Growth
2.3.2. The Numerical Analysis Method: Newman–Raju’s Method
2.3.3. The Weight Function Method
- (i)
- Longitudinal internal surface crack in pipe subjected to internal pressure:
- (ii)
- Longitudinal external surface crack in pipe subjected to internal pressure:
- (iii)
- Circumferential surface crack in pipe subjected to bending:
3. Discussion
3.1. Configurations of Surface Cracks and Pipes
3.2. Environmental Influential Parameters
3.3. Girth Welding Effect
3.4. Numerical Simulation
3.5. Analytical Evaluation
4. Remaining Challenges
5. Possible Further Research Topics
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| A | crack size |
| a | crack depth of surface cracks |
| a/c | aspect ratio |
| b | plate width |
| C | Paris’ law constant |
| c | half crack length of surface cracks |
| D | external diameter of pipes |
| d | internal diameter of pipes |
| crack growth rate | |
| surface crack growth along the depth direction | |
| surface crack growth along the length direction | |
| F | boundary correction factor |
| H | bending correction factor |
| m | Paris’ law constant |
| p | pressure |
| pc | crack face pressure |
| Q | an approximation factor |
| t | thickness of the pipe wall |
| R | load ratio |
| Ri | inner radius of pipes |
| Rt | outer radius of pipes |
| S | nominal stress |
| Sb | bending stress |
| St | tension stress |
| the range of stress intensity factors | |
| the range of the SIF of the deepest point | |
| the range of the SIF of the surface point | |
| Mode-I stress intensity factor | |
| the SIF of a node along the surface crack front | |
| the increment of the surface crack size | |
| the eccentric angle of the crack |
References
- DNVGL. Riser fatigue. In Offshore Standard, DNV-RP-F204; DNVGL: Høvik, Norway, 2010. [Google Scholar]
- Li, Z.; Jiang, X.; Hopman, H. Numerical analysis on the SIF of internal surface cracks in steel pipes reinforced with CRS subjected to bending. Ships Offshore Struct. 2019, 1–14. [Google Scholar] [CrossRef]
- DNVGL. Environmental conditions and environmental loads. In Recommended Practice, DNV-RP-C205; DNVGL: Høvik, Norway, 2014. [Google Scholar]
- DNVGL. Dynamic risers. In Offshore Standard, DNV-OS-F201; DNVGL: Høvik, Norway, 2010. [Google Scholar]
- Richard, H.A.; Sander, M. Fatigue Crack Growth; Springer: Berlin, Germany, 2016. [Google Scholar]
- Li, Z.; Jiang, X.; Hopman, H.; Zhu, L.; Liu, Z.; Tang, W. Experimental investigation on FRP-reinforced surface cracked steel plates subjected to cyclic tension. Mech. Adv. Mater. Struct. 2020, 1–15. [Google Scholar] [CrossRef]
- DNVGL. Assessment of flaws in pipeline and riser girth welds. In DNV Recommended Practice, DNV-RP-F108; DNVGL: Høvik, Norway, 2017. [Google Scholar]
- Martins, C.; Higashi, E.; Silva, R. A parametric analysis of steel catenary risers: Fatigue behavior near the top. In Proceedings of the Tenth International Offshore and Polar Engineering Conference, Seattle, WA, USA, 28 May–2 June 2000; International Society of Offshore and Polar Engineers: Mountain View, CA, USA, 2000. [Google Scholar]
- Mansour, G. The impact of the second order vessel motion on the fatigue life of steel catenary risers. In Proceedings of the ASME 2004 23rd International Conference on Offshore Mechanics and Arctic Engineering, Vancouver, BC, Canada, 20–25 June 2004; American Society of Mechanical Engineers: New York, NY, USA, 2004; pp. 1177–1180. [Google Scholar]
- Norway, P.S.A. Riser Damage—Injuries and Incidents from the Petroleum Safety Authority Norway’s Codam Database; DNVGL: Høvik, Norway, 2018. [Google Scholar]
- Horn, A.M.; Lotsberg, I.; Orjaseater, O. The rationale for update of S-N curves for single sided girth welds forrisers and pipelines in DNV GL RP C-203 based on fatigue performance of more than 1700 full scale fatigue test results. In Proceedings of the ASME 2018 37th International, Conference on Ocean, Offshore and Arctic Engineering, Madrid, Spain, 17–22 June 2018. [Google Scholar]
- Li, Z.; Jiang, X.; Hopman, H.; Zhu, L.; Liu, Z. An investigation on the circumferential surface crack growth in steel pipes subjected to fatigue bending. Theor. Appl. Fract. Mech. 2020, 105, 102403. [Google Scholar] [CrossRef]
- Van Wee, B.; Banister, D. How to Write a Literature Review Paper? Transp. Rev. 2015, 36, 1–11. [Google Scholar] [CrossRef]
- Newman, J. A Review and Assessment of the Stress-Intensity Factors for Surface Cracks. In Part-Through Crack Fatigue Life Prediction; ASTM International: West Conshohocken, PA, USA, 2009; p. 16. [Google Scholar]
- Scott, P.M.; Thorpe, T.W. A Critical Review of Crack Tip Stress Intensity Factors for Semi-Elliptic Cracks. Fatigue Fract. Eng. Mater. Struct. 1981, 4, 291–309. [Google Scholar] [CrossRef]
- Parks, D. A Surface Crack Review: Elastic and Elastic-Plastic Behavior. In Surface-Crack Growth: Models, Experiments, and Structures; ASTM International: West Conshohocken, PA, USA, 2009; p. 9. [Google Scholar]
- Pang, H. A review of stress of intensity factors for semi-elliptical surface crack in a plate and fillet weld joint. Weld. Inst. Rpt. 1990, 426. (In Japanese). Available online: https://ci.nii.ac.jp/naid/10011466198/#cit (accessed on 2 April 2020).
- Brighenti, R.; Carpinteri, A. Surface cracks in fatigued structural components: A review. Fatigue Fract. Eng. Mater. Struct. 2013, 36, 1209–1222. [Google Scholar] [CrossRef]
- Branco, R.; Antunes, F.; Branco, R. A review on 3D-FE adaptive remeshing techniques for crack growth modelling. Eng. Fract. Mech. 2015, 141, 170–195. [Google Scholar] [CrossRef]
- Shimakawa, T.; Takahashi, H.; Doi, H.; Watashi, K.; Asada, Y. Creep-fatigue crack propagation tests and the development of an analytical evaluation method for surface cracked pipe. Nucl. Eng. Des. 1993, 139, 283–292. [Google Scholar] [CrossRef]
- Zhu, L.; Tao, X.; Cengdian, L. Fatigue strength and crack propagation life of in-service high pressure tubular reactor under residual stress. Int. J. Press. Vessel. Pip. 1998, 75, 871–877. [Google Scholar] [CrossRef]
- Yoo, Y.S.; Ando, K. Circumferential inner fatigue crack growth and penetration behaviour in pipe subjected to a bending moment. Fatigue Fract. Eng. Mater. Struct. 2000, 23, 1–8. [Google Scholar] [CrossRef]
- Singh, P.; Vaze, K.; Bhasin, V.; Kushwaha, H.; Gandhi, P.; Murthy, D.R. Crack initiation and growth behaviour of circumferentially cracked pipes under cyclic and monotonic loading. Int. J. Press. Vessel. Pip. 2003, 80, 629–640. [Google Scholar] [CrossRef]
- Arora, P.; Singh, P.; Bhasin, V.; Vaze, K.K.; Ghosh, A.; Pukazhendhi, D.; Gandhi, P.; Raghava, G. Predictions for fatigue crack growth life of cracked pipes and pipe welds using RMS SIF approach and experimental validation. Int. J. Press. Vessel. Pip. 2011, 88, 384–394. [Google Scholar] [CrossRef]
- Sahu, V.K.; Ray, P.K.; Verma, B.B. Experimental fatigue crack growth analysis and modelling in part-through circumferentially pre-cracked pipes under pure bending load. Fatigue Fract. Eng. Mater. Struct. 2017, 40, 1154–1163. [Google Scholar] [CrossRef]
- Shlyannikov, V.; Yarullin, R.; Ishtyryakov, I. Effect of temperature on the growth of fatigue surface cracks in aluminum alloys. Theor. Appl. Fract. Mech. 2018, 96, 758–767. [Google Scholar] [CrossRef]
- Diamantoudis, A.; Labeas, G. Stress intensity factors of semi-elliptical surface cracks in pressure vessels by global-local finite element methodology. Eng. Fract. Mech. 2005, 72, 1299–1312. [Google Scholar] [CrossRef]
- Oh, C.S.; Song, T.K.; Kim, Y.; Kim, J.S.; Jin, T.E. Approximate J estimates for axial part-through surface-cracked pipes. Fatigue Fract. Eng. Mater. Struct. 2007, 30, 1127–1139. [Google Scholar] [CrossRef]
- Meshii, T.; Tanaka, T.; Lu, K. T-Stress solutions for a semi-elliptical axial surface crack in a cylinder subjected to mode-I non-uniform stress distributions. Eng. Fract. Mech. 2010, 77, 2467–2478. [Google Scholar] [CrossRef]
- Li, C.; Yang, S. Stress intensity factors for high aspect ratio semi-elliptical internal surface cracks in pipes. Int. J. Press. Vessel. Pip. 2012, 96, 13–23. [Google Scholar] [CrossRef]
- Sharma, K.; Singh, I.; Mishra, B.; Maurya, S. Numerical simulation of semi-elliptical axial crack in pipe bend using xfem. J. Solid Mech. 2014, 6, 208–228. [Google Scholar]
- Carpinteri, A.; Brighenti, R.; Vantadori, S. Circumferentially notched pipe with an external surface crack under complex loading. Int. J. Mech. Sci. 2003, 45, 1929–1947. [Google Scholar] [CrossRef]
- Ligoria, S.A.; Knight, G.M.S.; Murthy, D.S.R. Three-dimensional Finite Element Analysis of a Semi-Elliptical Circumferential Surface Crack in a Carbon Steel Pipe Subjected to a Bending Moment. J. Strain Anal. Eng. Des. 2005, 40, 525–533. [Google Scholar] [CrossRef]
- KouPang, K.; Burdekin, F. Stress intensity factors for a wide range of long-deep semi-elliptical surface cracks, partly through-wall cracks and fully through-wall cracks in tubular members. Eng. Fract. Mech. 2006, 73, 1693–1710. [Google Scholar] [CrossRef]
- Shahani, A.R.; Habibi, S.E. Stress intensity factors in a hollow cylinder containing a circumferential semi-elliptical crack subjected to combined loading. Int. J. Fatigue 2007, 29, 128–140. [Google Scholar] [CrossRef]
- Mechab, B.; Serier, B.; Bouiadjra, B.A.B.; Kaddouri, K.; Feaugas, X. Linear and non-linear analyses for semi-elliptical surface cracks in pipes under bending. Int. J. Press. Vessel. Pip. 2011, 88, 57–63. [Google Scholar] [CrossRef]
- Dao, N.H.; Sellami, H. Stress intensity factors and fatigue growth of a surface crack in a drill pipe during rotary drilling operation. Eng. Fract. Mech. 2012, 96, 626–640. [Google Scholar] [CrossRef]
- Li, Z.; Jiang, X.; Liu, Z.; Hopman, H. Internal Surface Crack Growth in Offshore Rigid Pipes Reinforced With CFRP. In Proceedings of the ASME 2018 37th International Conference on Ocean, Offshore and Arctic Engineering, Madrid, Spain, 17–22 June 2018. [Google Scholar]
- ASTM. 647. Standard Test Method for Measurement of Fatigue Crack Growth Rates. In ASTM Annual Book of Standards; ASTM international: West Conshohocken, PA, USA, 2015; Volume 3. [Google Scholar]
- ASTM. 2899. Standard Test Method for Measurement of Initiation Toughness in Surface Cracks under Tension and Bending; ASTM international: West Conshohocken, USA, 2019; Volume 3. [Google Scholar]
- ASTM. 740-88: Standard Practice for Fracture Testing with Surface-Crack Tension Specimens. In ASTM Annual Book of Standards; ASTM international: West Conshohocken, PA, USA, 2016; Volume 3. [Google Scholar]
- Kim, S.; Kim, M.-H. Dynamic behaviors of conventional SCR and lazy-wave SCR for FPSOs in deepwater. Ocean Eng. 2015, 106, 396–414. [Google Scholar] [CrossRef]
- BSI. BS 7910: 2013+A1:2015; Guide to Methods for Assessing the Acceptability of Flaws in Metallic Structures; BSI Standards Limited: London, UK, 2015; ISBN 978-0-580-91721-9. [Google Scholar]
- Boiler, A.; Code, P.V. Section xi. Rules for Inservice Inspection of Nuclear Power Plant Components. In Companion Guide to the ASME Boiler and Pressure Vessel Code; Rao, K.R., Ed.; ASME Press: New York, NY, USA, 2012. [Google Scholar] [CrossRef]
- Corn, D. A study of cracking techniques for obtaining partial thickness cracks of pre-selected depths and shapes. Eng. Fract. Mech. 1971, 3, 45–52. [Google Scholar] [CrossRef]
- Branco, R.; Antunes, F.; Branco, R. Extent of the Surface Region in Notched Middle Cracked Tension Specimens. Key Eng. Mater. 2013, 560, 107–127. [Google Scholar] [CrossRef]
- Guinea, G.; Planas, J.; Elices, M. KI evaluation by the displacement extrapolation technique. Eng. Fract. Mech. 2000, 66, 243–255. [Google Scholar] [CrossRef]
- Lin, X.; Smith, R. Finite element modelling of fatigue crack growth of surface cracked plates. Eng. Fract. Mech. 1999, 63, 503–522. [Google Scholar] [CrossRef]
- Sedmak, A.; Savovic, N.; Pavisic, M. Esis Recommendations for Use of Finite Element Method in Fracture Mechanics; ECF9: Varna, Bulgaria, 1992. [Google Scholar]
- Pang, J.H.; Tsang, K.S.; Hoh, H.J. 3D stress intensity factors for weld toe semi-elliptical surface cracks using XFEM. Mar. Struct. 2016, 48, 1–14. [Google Scholar] [CrossRef]
- Heyder, M.; Kolk, K.; Kuhn, G. Numerical and experimental investigations of the influence of corner singularities on 3D fatigue crack propagation. Eng. Fract. Mech. 2005, 72, 2095–2105. [Google Scholar] [CrossRef]
- Predan, J.; Močilnik, V.; Gubeljak, N. Stress intensity factors for circumferential semi-elliptical surface cracks in a hollow cylinder subjected to pure torsion. Eng. Fract. Mech. 2013, 105, 152–168. [Google Scholar] [CrossRef]
- Lin, X.; Smith, R.A. Direct simulation of fatigue crack growth for arbitrary-shaped defects in pressure vessels. Proc. Inst. Mech. Eng. Part C: J. Mech. Eng. Sci. 1998, 213, 175–189. [Google Scholar] [CrossRef]
- Couroneau, N.; Royer, J. Simplified model for the fatigue growth analysis of surface cracks in round bars under mode I. Int. J. Fatigue 1998, 20, 711–718. [Google Scholar] [CrossRef]
- Paris, P.; Erdogan, F. A Critical Analysis of Crack Propagation Laws. J. Basic Eng. 1963, 85, 528–533. [Google Scholar] [CrossRef]
- Schijve, J. Fatigue of Structures and Materials; Springer Science & Business Media: Berlin, Germany, 2001. [Google Scholar]
- Newman, J.C., Jr.; Raju, I.S. Analysis of Surface Cracks in Finite Plates under Tension or Bending Loads; NASA-Langley: Hampton, VA, USA, 1979.
- Newman, J.C., Jr.; Raju, I.S. An empirical stress-intensity factor equation for the surface crack. Eng. Fract. Mech. 1981, 15, 185–192. [Google Scholar] [CrossRef]
- Petroski, H.; Achenbach, J. Computation of the weight function from a stress intensity factor. Eng. Fract. Mech. 1978, 10, 257–266. [Google Scholar] [CrossRef]
- Raju, I.S.; Newman, J.C., Jr. Stress-intensity factor influence coefficients for internal and external surface cracks in cylindrical vessels. J. Press. Vessel. 1982, 104, 37–49. [Google Scholar]
- Raju, I. Stress-intensity Factors for Circumferential Surface Cracks in Pipes and Rods under Tension and Bending Loads. In Fracture Mechanics: Seventeenth Volume; ASTM International: West Conshohocken, PA, USA, 2008; p. 789. [Google Scholar]
- Anderson, T.L. Development of stress intensity factor solutions for surface and embedded cracks in API579 (In Japanese). Welding Research Council Bulletin. 2002. Available online: https://ci.nii.ac.jp/naid/80015499214/ (accessed on 7 May 2020).
- Newman, J.C., Jr. Predicting Failure of Specimens with Either Surface Cracks or Corner Cracks at Holes; NASA-Langley: Hampton, VA, USA, 1976.
- Green, A.E.; Sneddon, I.N. The distribution of stress in the neighbourhood of a flat elliptical crack in an elastic solid. Math. Proc. Camb. Philos. Soc. 1950, 46, 159. [Google Scholar] [CrossRef]
- Russell, A. Application of Proof Testing to Assess k-Reactor Piping Integrity; Douglas United Nuclear, Inc.: Richland, WA, USA, 1970.
- Dedhia, D.; Harris, D. Improved influence functions for part-circumferential cracks in pipes. Circumferential Cracks Press. Vessel. Pip. 1983, 2, 35–48. [Google Scholar]
- Bergman, M. Stress Intensity Factors for Circumferential Surface Cracks in Pipes. Fatigue Fract. Eng. Mater. Struct. 1995, 18, 1155–1172. [Google Scholar] [CrossRef]
- Newman, J.C., Jr.; Raju, I.S. Stress-Intensity Factors for Internal Surface Cracks in Cylindrical Pressure Vessels. J. Press. Vessel. Technol. 1980, 102, 342–346. [Google Scholar] [CrossRef]
- Folias, E. On the effect of initial curvature on cracked flat sheets. Int. J. Fract. 1969, 5, 327–346. [Google Scholar] [CrossRef]
- Murakami, Y.; Keer, L.M. Stress Intensity Factors Handbook, Vol. 3. J. Appl. Mech. 1993, 60, 1063. [Google Scholar] [CrossRef]
- Green, D.; Knowles, J. The Treatment of Residual Stress in Fracture Assessment of Pressure Vessels. J. Press. Vessel. Technol. 1994, 116, 345–352. [Google Scholar] [CrossRef]
- API. API 579-1/ASME FFS-1: Fitness-for-Service; American Petroleum Institute: Houston, TX, USA, 2016. [Google Scholar]
- Lu, Z.; Liu, Y. Experimental investigation of random loading sequence effect on fatigue crack growth. Mater. Des. 2011, 32, 4773–4785. [Google Scholar] [CrossRef]
- Sumi, Y.; Inoue, T. Multi-scale modeling of fatigue crack propagation applied to random sequence of clustered loading. Mar. Struct. 2011, 24, 117–131. [Google Scholar] [CrossRef]
- Fassina, P.; Brunella, M.; Lazzari, L.; Re, G.; Vergani, L.; Sciuccati, A. Effect of hydrogen and low temperature on fatigue crack growth of pipeline steels. Eng. Fract. Mech. 2013, 103, 10–25. [Google Scholar] [CrossRef]
- Tognarelli, M.A.; Thodla, R.; Shademan, S. Fatigue Crack Growth Rate and Fracture Toughness of API5L X65 in Sweet Environments. In Proceedings of the ASME 2013 32nd International Conference on Ocean, Offshore and Arctic Engineering, Nantes, France, 9–14 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; p. V003T003A006. [Google Scholar]
- Fassina, P.; Brunella, F.; Lazzari, L.; Re, G.; Vergani, L.; Sciuccati, A. Fatigue Behavior Of Pipeline Steel Under Hydrogen Environment And Low Temperature. Procedia Eng. 2011, 10, 3345–3352. [Google Scholar] [CrossRef]
- Qian, G.; Zhou, C.; Hong, Y. A model to predict S–N curves for surface and subsurface crack initiations in different environmental media. Int. J. Fatigue 2015, 71, 35–44. [Google Scholar] [CrossRef]
- Qiang, B.; Li, Y.; Yao, C.; Wang, X. Through-thickness welding residual stress and its effect on stress intensity factors for semi-elliptical surface cracks in a butt-welded steel plate. Eng. Fract. Mech. 2018, 193, 17–31. [Google Scholar] [CrossRef]
- Kikuchi, M.; Wada, Y.; Shimizu, Y.; Li, Y. Crack growth analysis in a weld-heat-affected zone using S-version FEM. Int. J. Press. Vessel. Pip. 2012, 90, 2–8. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Methods Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Fish, J. The s-version of the finite element method. Comput. Struct. 1992, 43, 539–547. [Google Scholar] [CrossRef]
- Belytschko, T.; Gracie, R.; Ventura, G. A review of extended/generalized finite element methods for material modeling. Model. Simul. Mater. Sci. Eng. 2009, 17, 43001. [Google Scholar] [CrossRef]
- Kamaya, M. Growth evaluation of multiple interacting surface cracks. Part II: Growth evaluation of parallel cracks. Eng. Fract. Mech. 2008, 75, 1350–1366. [Google Scholar] [CrossRef]
- Akramin, M.R.M.; Shaari, M.; Ariffin, A.K.; Kikuchi, M.; Abdullah, S. Surface crack analysis under cyclic loads using probabilistic S-version finite element model. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 1851–1865. [Google Scholar] [CrossRef]
- ANSYS. Semi-Elliptical Crack. Defines a Semi-Elliptical Crack Based on an Internally Generated Mesh to Analyze Crack Fronts by Use of Geometric Parameters. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/corp/v191/wb_sim/ds_Crack_o_r.html (accessed on 7 May 2020).
- Fracture Analysis Consultants, Inc. Franc3d Abaqus Tutorial Version 7. Franc3D, Jan. 2017. Available online: http://www.franc3d.com/wp-content/uploads/2012/05/FRANC3D_V7_ABAQUS_Tutorial.pdf (accessed on 7 May 2020).
- Pachoud, A.J.; Manso, P.A.; Schleiss, A. Stress intensity factors for axial semi-elliptical surface cracks and embedded elliptical cracks at longitudinal butt welded joints of steel-lined pressure tunnels and shafts considering weld shape. Eng. Fract. Mech. 2017, 179, 93–119. [Google Scholar] [CrossRef]


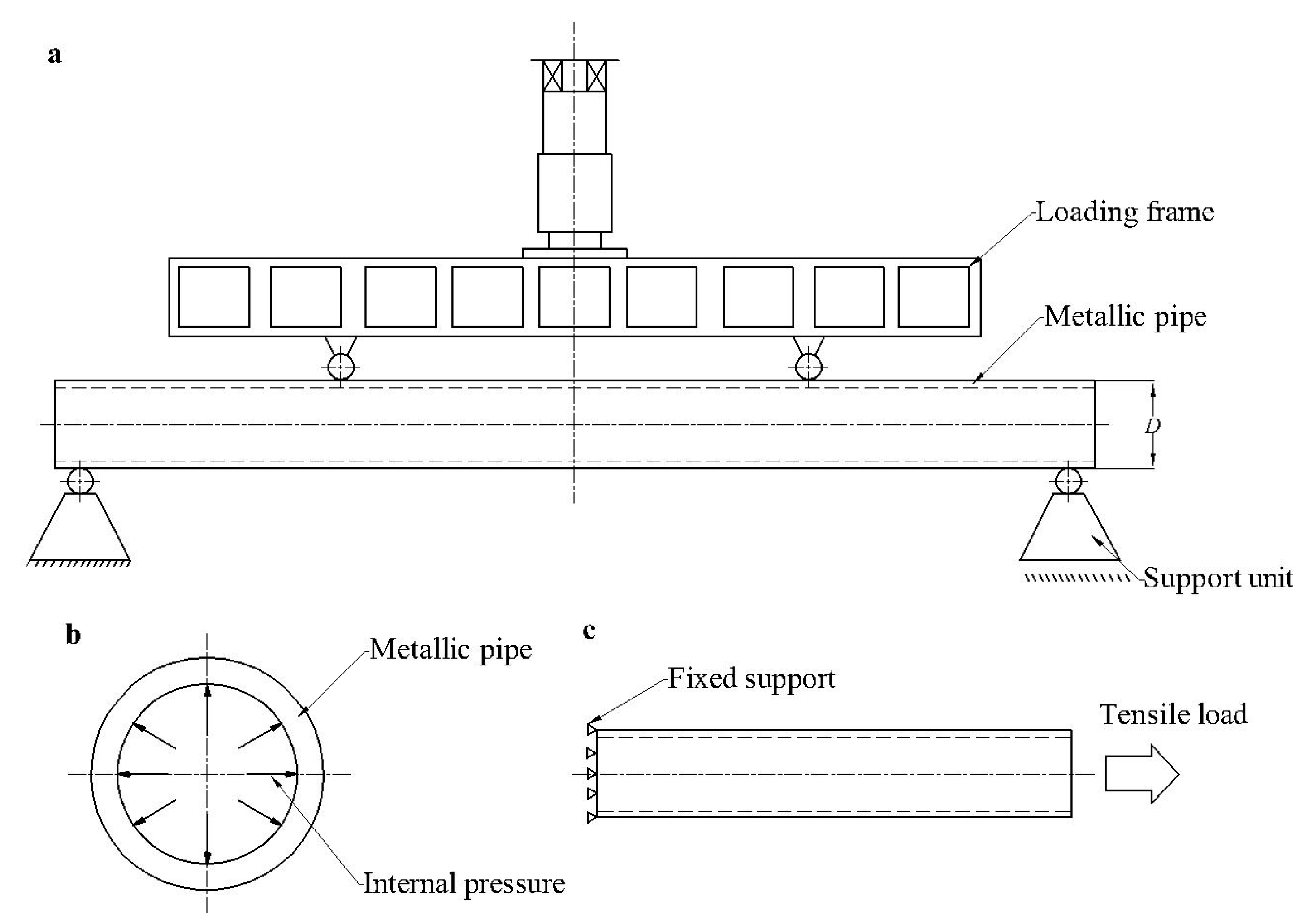
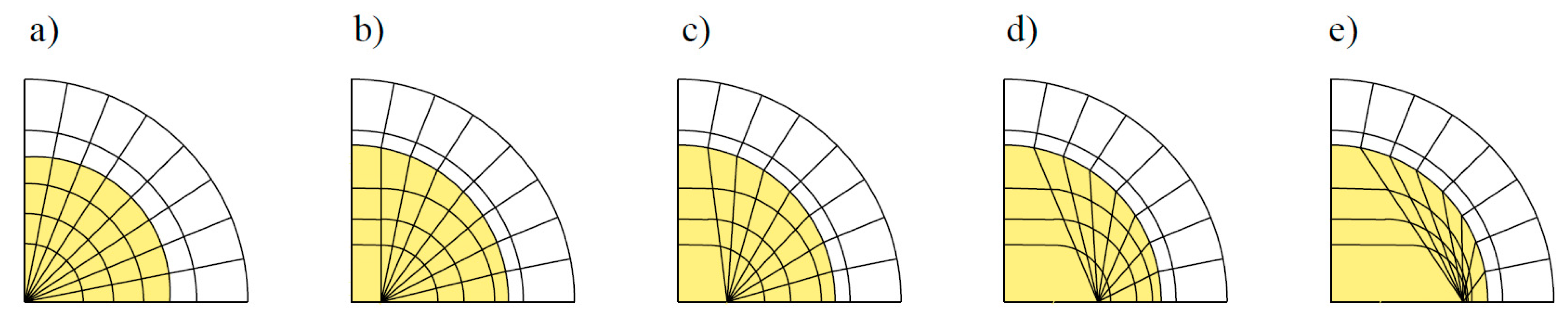

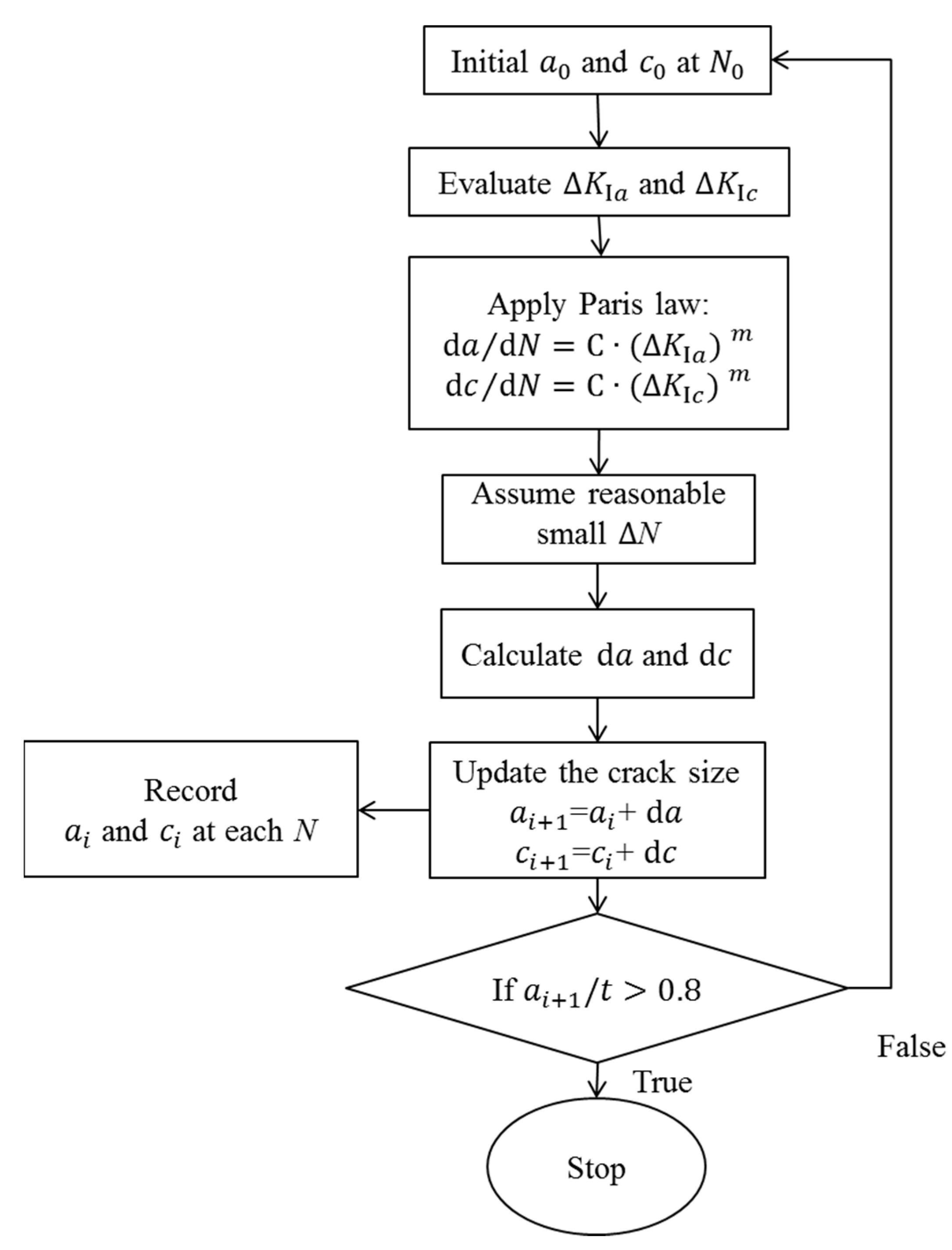
| Issue | Load | Crack Orientation | Crack Dimension | Pipe Dimension | Welding Effect | Environmental Effect |
|---|---|---|---|---|---|---|
| Experimental | + | + | ++ | + | / | + |
| Numerical | ++ | +++ | +++ | +++ | / | / |
| Analytical | + | + | + | / | + | / |
| Scenarios | Authors | Year | Material | Crack Shape | Load Type | Load Ratio | Crack Detection Method | Weld Effect |
|---|---|---|---|---|---|---|---|---|
| Circumferential external surface crack | Shimakawa, et al. [20] | 1993 | 304 Stainless steel | Semi-elliptical | Bending | \ | \ | \ |
| Longitudinal internal and external surface cracks | Zhu, et al. [21] | 1998 | AISI4340H II steel | Semi-elliptical | Internal pressure | 0 | BM | \ |
| Circumferential internal surface crack | Yoo and Ando [22] | 2000 | STS370 carbon steel | Semi-elliptical | Bending | 0.1 | BM | \ |
| Circumferential external surface crack | Singh, et al. [23] | 2003 | SA333 seamless steel | Rectangular machined notch propagated to semi-elliptical cracks | Bending | 0.1, 0.3, 0.5. | ACPD | \ |
| Circumferential external surface crack | Arora, et al. [24] | 2011 | Stainless SA312 type 304LN steel | Semi-elliptical | Bending | 0.1 | BM, ACPD | √ |
| Circumferential external surface crack | Sahu, et al. [25] | 2017 | Stainless steel TP316L | Notch with a straight line at the bottom propagated to semi-elliptical cracks | Bending | 0.1 | COD | \ |
| Circumferential external surface crack | Shlyannikov, et al. [26] | 2018 | Aluminium alloy | Semi-elliptical | Tension | 0.1 | BM | \ |
| Circumferential external surface crack | Li, et al. [12] | 2019 | API 5L X65 stainless steel pipe | Semi-elliptical | Bending | 0.1 | BM | \ |
| Scenario | Author | Year | Geometry and Pipe Material | Load Type | a/t Range | Rt/t Range | a/c Range | Simulation Method |
|---|---|---|---|---|---|---|---|---|
| Longitudinal internal surface crack | Diamantoudis and Labeas [27] | 2005 | Steel pressure vessel | Internal pressure | \ | 10, 14.29, 24.92 | [0.2,1.0] | FEM |
| Oh, et al. [28] | 2007 | Pipe | Internal pressure | 0.25, 0.5, 0.6, 0.75 | [5, 20] | 0.01 | FEM | |
| Meshii, et al. [29], | 2010 | Steel pipeline | Internal pressure | 0.2, 0.4, 0.5 | 5, 10 | [0.2,1.0] | FEM | |
| Li and Yang [30] | 2012 | Cast iron sewer pipe | Internal pressure | \ | \ | [1.0,) | FEM | |
| Sharma, et al. [31] | 2014 | Steel pipe bend | Internal pressure | \ | \ | \ | XFEM | |
| Circumferential external surface crack | Carpinteri, et al. [32] | 2003 | Pipe | Tension, bending | 0.2, 0.5, 0.8 | 1, 10 | 0.2, 0.6, 1.0 | FEM |
| Ligoria, et al. [33] | 2005 | Nuclear power plant piping | Bending | 0.24, 0.35, 0.53, 0.79 | 4, 6 | (0,0.5] | FEM | |
| Kou and Burdekin [34] | 2006 | Steel tubular | Tension | [0.8, 1) | 10, 15, 22.5 | [0.4,0.8] | FEM | |
| Shahani and Habibi [35] | 2007 | Metallic hollow cylinder | Combined tension, bending, and torsion | [0.2, 0.8] | \ | [0.2,1.2] | FEM | |
| Mechab, et al. [36] | 2011 | Steel pipeline | Bending | [0.2, 0.8] | [1, 80] | [0.6,0.8] | FEM | |
| Dao and Sellami [37] | 2012 | Steel pipe | Bending and tension | [0, 1] | 10 | 0.2, 0.5, 1.0 | FEM | |
| Predan et al. [52] | 2013 | High strength steel tubular | Torsion | [0.1, 0.5] | 2 | [0.1,1.0] | FEM | |
| Li et al. [12] | 2019 | Steel pipeline | Bending | [0.2, 0.8] | [2.5,12.5] | [0.2,1.0] | FEM | |
| Circumferential internal surface crack | Li et al. [38] | 2018 | Steel pipeline | Bending | (0, 1) | 4, 6.3 | (0.2,1.0] | FEM |
| Li et al. [12] | 2019 | Steel pipeline | Bending | [0.2, 0.8] | [2.5,12.5] | [0.2,1.0] | FEM |
| Method | Author | Scenarios | Year | Standard |
|---|---|---|---|---|
| Numerical analysis method | Newman Jr and Raju [58] | Circumferential surface cracks in plates subjected to bending | 1981 | BS 7910, DNVGL-RP-F108 |
| Raju and Newman Jr [60] | Longitudinal surface cracks in pipes subjected to internal pressure | 1982 | ||
| Raju and Newman [61] | Circumferential external surface cracks in pipe subjected to bending | 1986 | - | |
| Mechab, et al. [36] | Circumferential external surface crack in pipe subjected to bending | 2011 | - | |
| Li and Yang [30] | Longitudinal high aspect ratio surface crack in pipe subjected to internal pressure | 2012 | - | |
| Sahu, et al. [25] | Circumferential external surface cracks in pipe subjected to bending | 2017 | - | |
| Li et al. [12] | Circumferential surface cracks in pipe subjected to bending | 2019 | - | |
| Weight function method | Anderson [62] | Circumferential surface crack in pipe subjected to bending | 2002 | API 579-1/ASME FFS-1 |
| Longitudinal surface crack in pipe subjected to internal pressure |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Jiang, X.; Hopman, H. Surface Crack Growth in Offshore Metallic Pipes under Cyclic Loads: A Literature Review. J. Mar. Sci. Eng. 2020, 8, 339. https://doi.org/10.3390/jmse8050339
Li Z, Jiang X, Hopman H. Surface Crack Growth in Offshore Metallic Pipes under Cyclic Loads: A Literature Review. Journal of Marine Science and Engineering. 2020; 8(5):339. https://doi.org/10.3390/jmse8050339
Chicago/Turabian StyleLi, Zongchen, Xiaoli Jiang, and Hans Hopman. 2020. "Surface Crack Growth in Offshore Metallic Pipes under Cyclic Loads: A Literature Review" Journal of Marine Science and Engineering 8, no. 5: 339. https://doi.org/10.3390/jmse8050339
APA StyleLi, Z., Jiang, X., & Hopman, H. (2020). Surface Crack Growth in Offshore Metallic Pipes under Cyclic Loads: A Literature Review. Journal of Marine Science and Engineering, 8(5), 339. https://doi.org/10.3390/jmse8050339





