Effects of Salinity on Surface Lifetime of Large Individual Bubbles
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Conditions
2.2. Data Collection and Processing
3. Results
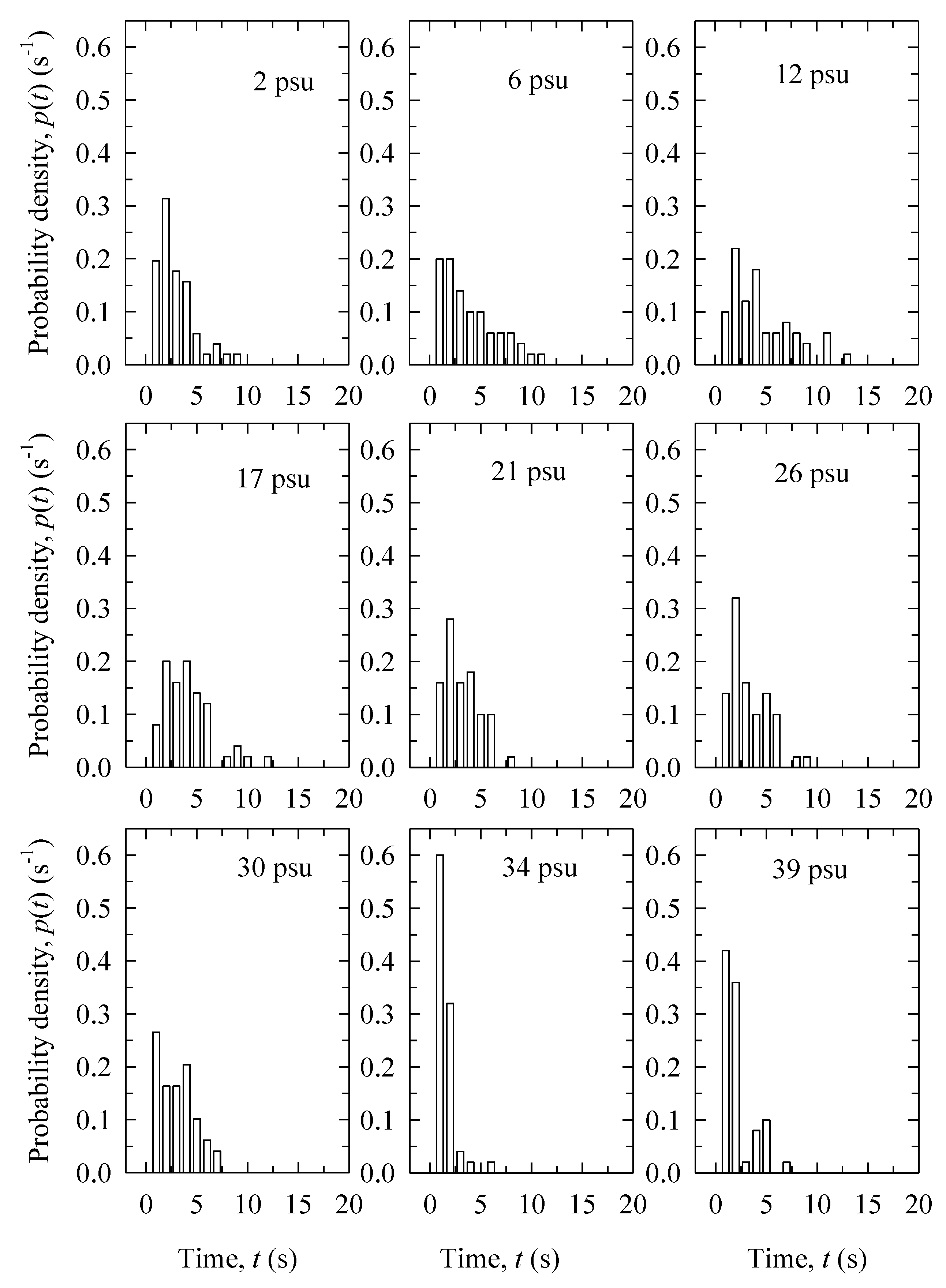
3.1. Bubble Surface Lifetime
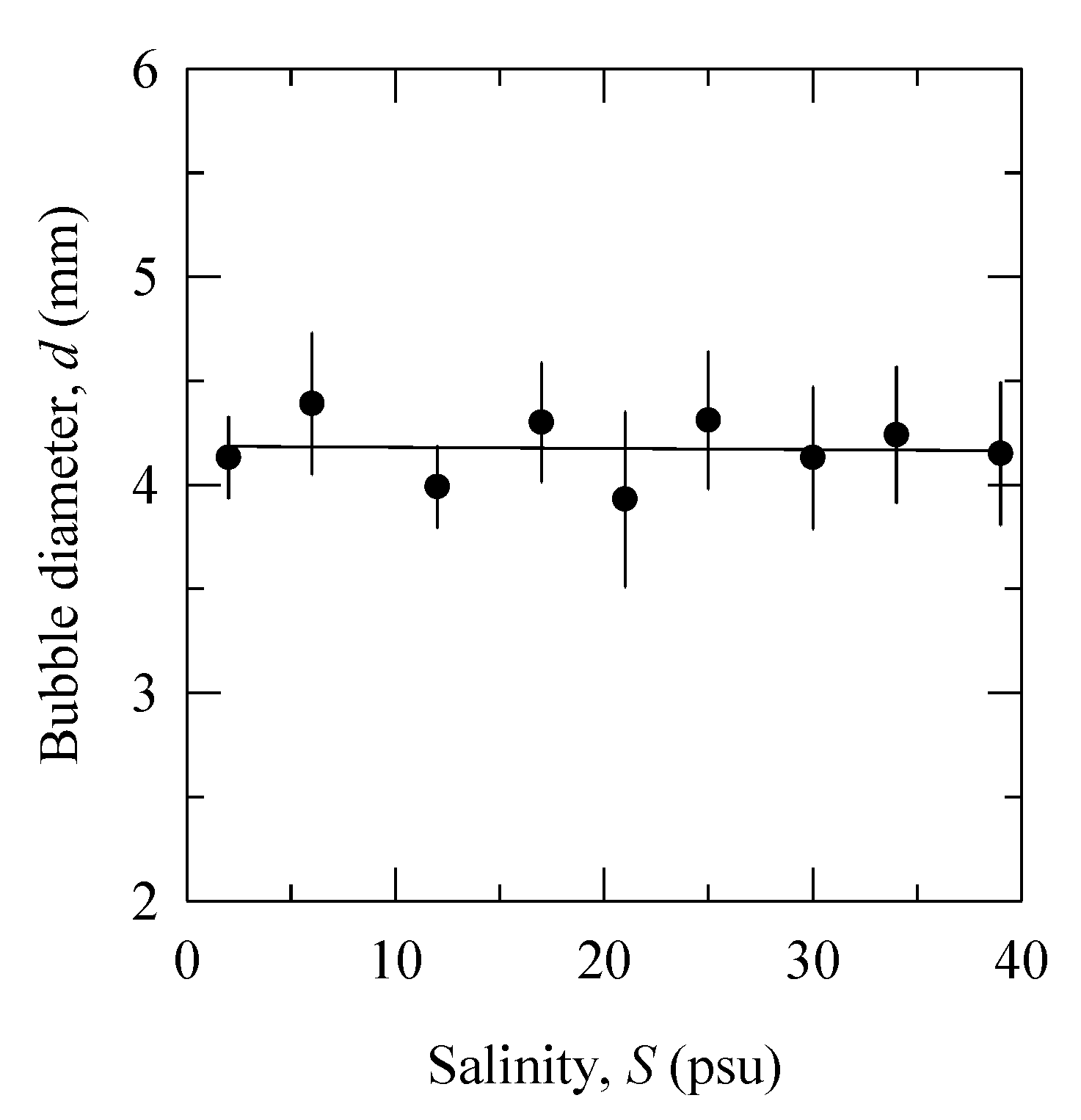
3.2. Bubble Size
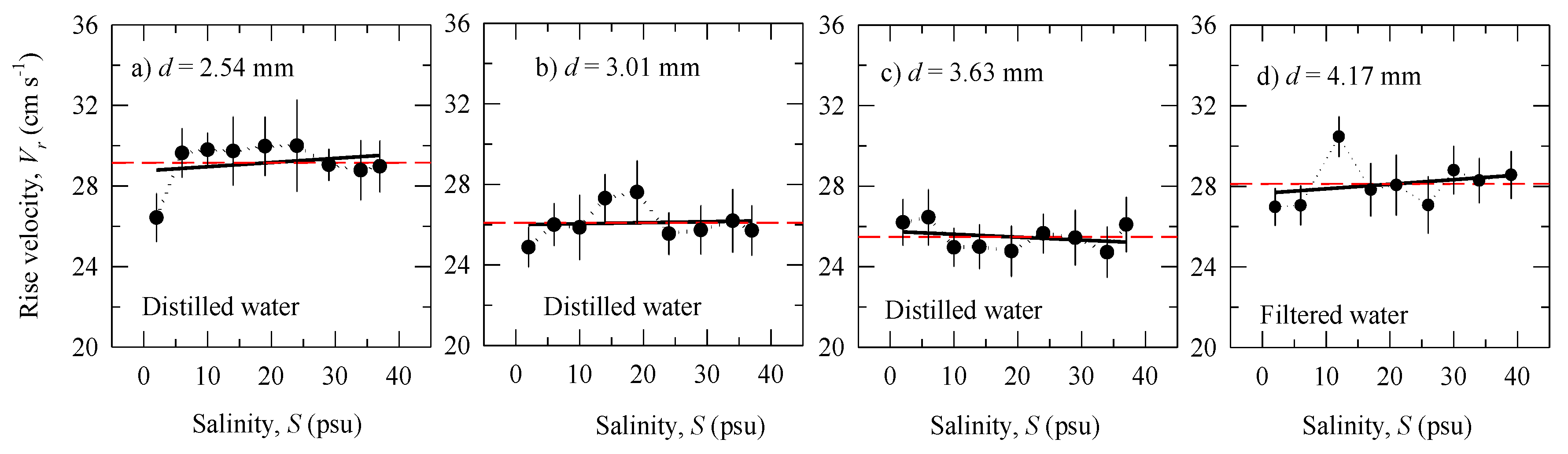
3.3. Bubble Rise Velocity
4. Discussion
4.1. Salinity Action during Experiments
4.2. Salinity Role for Bubble Stabilization
4.3. Salinity Effect on Large Bubble Sizes
5. Conclusions
- (1)
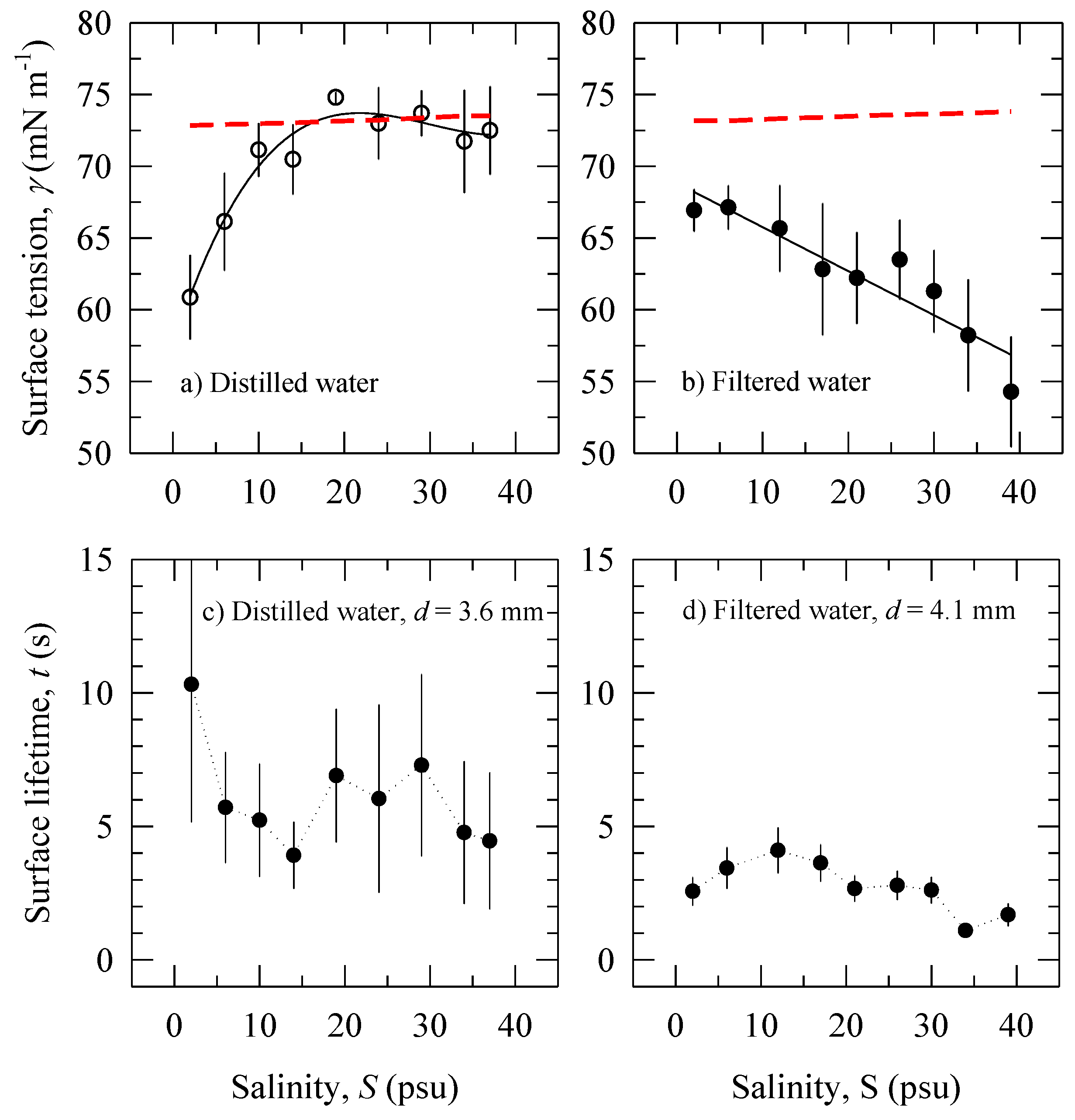
- Wide variations of the bubble surface lifetime t are measured with a range of 0.4–35 s. Changing water surface conditions after bubble bursting are most likely causing such wide variations; this effect is more pronounced in cleaner solutions (Figure 1c,d).
- (2)
- Even weakly contaminated solutions can stabilize bubbles at low S (Figure 1c). The anti-foaming action of S decreases bubble surface lifetime when surface tension approaches negative film pressure in a weakly contaminated water (Figure 1a,c). At a given level of contamination, bubbles are short-lived (less stable) at S > 30 psu (Figure 1c,d).
- (3)
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Formation of Individual Bubbles
Appendix B. Surface Tension Variations
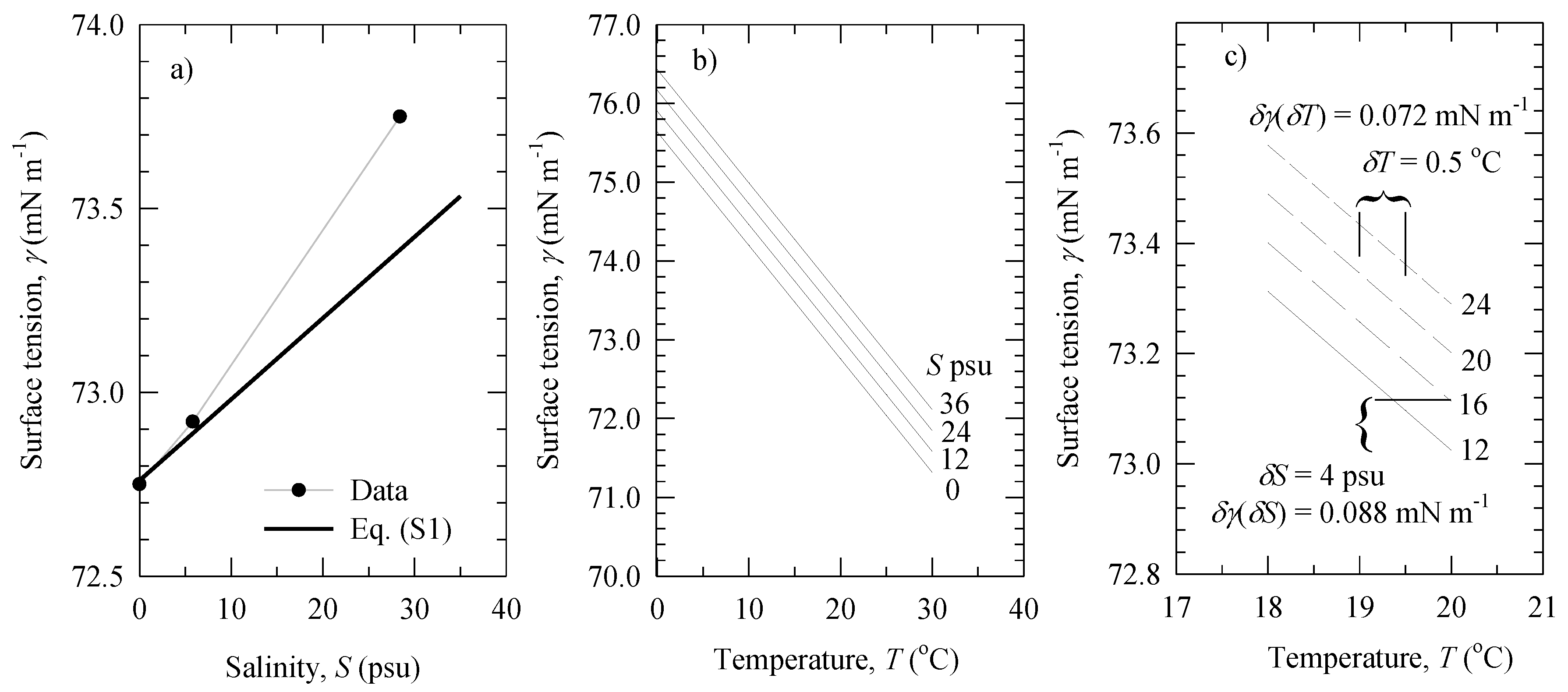
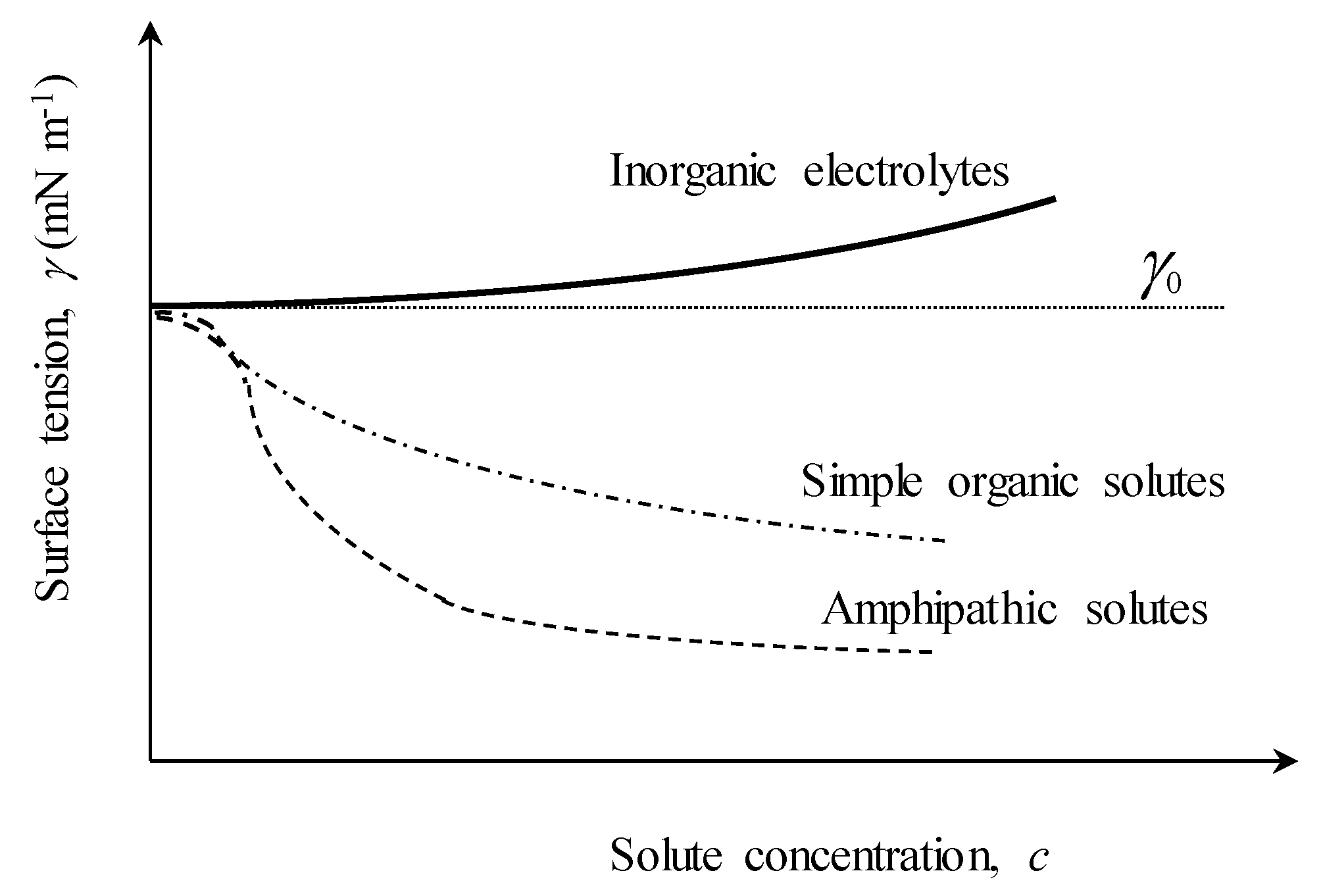
Appendix C. Stabilization of Air–Water Interfaces by Surface Films
- (1)
- An increase of γ above that of pure water γ0 (solid and dotted lines), typical for inorganic electrolytes (e.g., salts);
- (2)
- A decrease of γ progressively (dash-dot line), typical of simple, un-ionized organic compounds (e.g., alcohols); and
- (3)
- A decrease of γ until a saturation level is reached (dashed line), typical of surface-active materials (surfactants).
References
- Potter, H.; Smith, G.B.; Snow, C.M.; Dowgiallo, D.J.; Bobak, J.P.; Anguelova, M.D. Whitecap lifetime stages from infrared imagery with implications for microwave radiometric measurements of whitecap fraction. J. Geophys. Res. Oceans 2015, 120, 7521–7537. [Google Scholar] [CrossRef]
- Andreas, E.L.; Edson, J.B.; Monahan, E.C.; Rouault, M.P.; Smith, S.D. The spray contribution to the net evaporation from the sea: A review of recent progress. Bound. Layer Meteorol. 1995, 72, 3–52. [Google Scholar] [CrossRef]
- Melville, W.K. The role of surface-wave breaking in air-sea interaction. Annu. Rev. Fluid Mech. 1996, 28, 279–321. [Google Scholar] [CrossRef]
- Wanninkhof, R.; Asher, W.E.; Ho, D.T.; Sweeney, C.; McGillis, W.R. Advances in quantifying air-sea gas exchange and environmental forcing. Annu. Rev. Mar. Sci. 2009, 1, 213–244. [Google Scholar] [CrossRef] [PubMed]
- De Leeuw, G.; Andreas, E.L.; Anguelova, M.D.; Fairall, C.W.; Lewis, E.R.; O’Dowd, C.; Schulz, M.; Schwartz, S.E. Production flux of sea spray aerosol. Rev. Geophys. 2011, 49, RG2001. [Google Scholar] [CrossRef]
- Monahan, E.C.; O’Muircheartaigh, I.G. Whitecaps and the passive remote sensing of the ocean surface. Int. J. Remote Sens. 1986, 7, 627–642. [Google Scholar] [CrossRef]
- Salisbury, D.J.; Anguelova, M.D.; Brooks, I.M. On the variability of whitecap fraction using satellite-based observations. J. Geophys. Res. Oceans 2013, 118, 6201–6222. [Google Scholar] [CrossRef]
- Asher, W.E.; Karle, L.M.; Higgins, B.J. On the differences between bubble-mediated air-water transfer in freshwater and seawater. J. Mar. Res. 1997, 55, 813–845. [Google Scholar] [CrossRef]
- Deane, G.B.; Stokes, M.D. Scale dependence of bubble creation mechanisms in breaking waves. Nature 2002, 418, 839–844. [Google Scholar] [CrossRef] [PubMed]
- Lewis, E.R.; Schwartz, S.E. Sea Salt Aerosol Production: Mechanisms, Methods, Measurements and Models—A Critical Review; American Geophysical Union: Washington, DC, USA, 2004. [Google Scholar]
- Monahan, E.M.; Zietlow, C.R. Laboratory comparisons of fresh-water and salt-water whitecaps. J. Geophys. Res. 1969, 74, 6961–6966. [Google Scholar] [CrossRef]
- Peltzer, R.D.; Griffin, O.M. Stability of a three-dimensional foam layer in seawater. J. Geophys. Res. 1988, 93, 10804–10812. [Google Scholar] [CrossRef]
- Callaghan, A.H.; Deane, G.B.; Stokes, M.D.; Ward, B. Observed variation in the decay time of oceanic whitecap foam. J. Geophys. Res. 2012, 117, C09015. [Google Scholar] [CrossRef]
- Callaghan, A.H.; Deane, G.B.; Stokes, M.D. Two Regimes of Laboratory Whitecap Foam Decay: Bubble-Plume Controlled and Surfactant Stabilized. J. Phys. Oceanogr. 2013, 43, 1114–1126. [Google Scholar] [CrossRef]
- Scott, J.C. The role of salt in whitecap persistence. Deep Sea Res. 1975, 22, 653–657. [Google Scholar] [CrossRef]
- Burger, S.R.; Blanchard, D.C. The persistence of air bubbles at a seawater surface. J. Geophys. Res. 1983, 88, 7724–7726. [Google Scholar] [CrossRef]
- Struthwolf, M.; Blanchard, D.C. The residence time of air bubbles <400 µm diameter at the surface of distilled water and seawater. Tellus B 1984, 36B, 294–299. [Google Scholar]
- Zheng, Q.A.; Klemas, V.; Hsu, Y.-H.L. Laboratory measurements of water surface bubble life time. J. Geophys. Res. 1983, 88, 701–706. [Google Scholar] [CrossRef]
- Chattoraj, D.K.; Birdi, K.S. Adsorption and the Gibbs Surface Excess; Plenum Publishing Company: New York, NY, USA, 1984. [Google Scholar]
- Hiemenz, P.C.; Rajagopalan, R. Principles of Colloid and Surface Chemistry, 3rd ed.; Marcel Dekker, Inc.: New York, NY, USA, 1997. [Google Scholar]
- Asher, W.E.; Pankow, J.F. The interaction of mechanically generated turbulence and interfacial films with a liquid phase controlled gaslliquid transport process. Tellus B Chem. Phys. Meteorol. 1986, 38B, 305–318. [Google Scholar] [CrossRef]
- Anguelova, M.D.; Huq, P. Characteristics of bubble clouds at various wind speeds. J. Geophys. Res. 2012, 117, C03036. [Google Scholar] [CrossRef]
- Katsir, Y.; Marmur, A. Rate of bubble coalescence following quasi-static approach: Screening and neutralization of the electric double layer. Sci. Rep. 2014, 4, 4266. [Google Scholar] [CrossRef] [PubMed]
- Pounder, C. Sodium chloride and water temperature effects on bubbles. In Oceanic Whitecaps Their Role in Air-Sea Exchange Processes; Monahan, E.C., MacNiocaill, G., Eds.; Springer: Berlin, Germany, 1986; p. 278. [Google Scholar]
- Tomiyama, A.; Celata, G.P.; Hosokawa, S.; Yoshida, S. Terminal velocity of single bubbles in surface tension force dominant regime. Int. J. Multiph. Flow 2002, 28, 1497–1519. [Google Scholar] [CrossRef]
- Clift, R.; Grace, J.R.; Weber, M.E. Bubbles, Drops, and Particles; Academic Press: New York, NY, USA, 1978. [Google Scholar]
- Quinn, J.J.; Maldonado, M.; Gomez, C.O.; Finch, J.A. Experimental study on the shape-velocity relationship of an ellipsoidal bubble in inorganic salt solutions. Miner. Eng. 2014, 55, 5–10. [Google Scholar] [CrossRef]
- Thorpe, S.A. On the clouds of bubbles formed by breaking wind-waves in deep water, and their role in air-sea gas transfer. Philos. Trans. R. Soc. Lond. A 1982, A304, 155–210. [Google Scholar] [CrossRef]
- Garrett, W.D. Stabilization of air bubbles at the air-sea interface by surface-active material. Deep Sea Res. 1967, 14, 661–672. [Google Scholar] [CrossRef]
- Monahan, E.M. Comments on “Bubbles produced by breaking waves in fresh and salt water”. J. Phys. Oceanogr. 2001, 31, 1931–1932. [Google Scholar] [CrossRef]
- Soo, S.L. Fluid Dynamics of Multiphase Systems; Blaisdel Publishing Company: Waltham, MA, USA, 1967. [Google Scholar]
- Liu, H. Science and Engineering of Droplets: Fundamentals and Applications; William Andrew: Norwich, NY, USA, 1999. [Google Scholar]
- Leifer, I.; Judd, A.G. Oceanic methane layers: The hydrocarbon seep bubble deposition hypothesis. Terra Nova 2002, 14, 417–424. [Google Scholar] [CrossRef]
- Hardy, C.D.; Baylor, E.R. Surface tension reductions and urban wastes in the New York Bight. J. Geophys. Res. 1975, 80, 2696–2699. [Google Scholar] [CrossRef]
- Lewis, E.L.; Perkin, R.G. Salinity: Its definition and calculation. J. Geophys. Res. 1978, 83, 466–478. [Google Scholar] [CrossRef]
- Weast, R.C. (Ed.) Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 1986. [Google Scholar]
- Rosen, M.J. Surfactants and Interfacial Phenomena; John Wiley & Sons: New York, NY, USA, 1978. [Google Scholar]
- Couder, Y.; Chomaz, J.M.; Rabaud, M. On the hydrodynamics of soap films. Phys. D Nonlinear Phenom. 1989, 37, 384–405. [Google Scholar] [CrossRef]
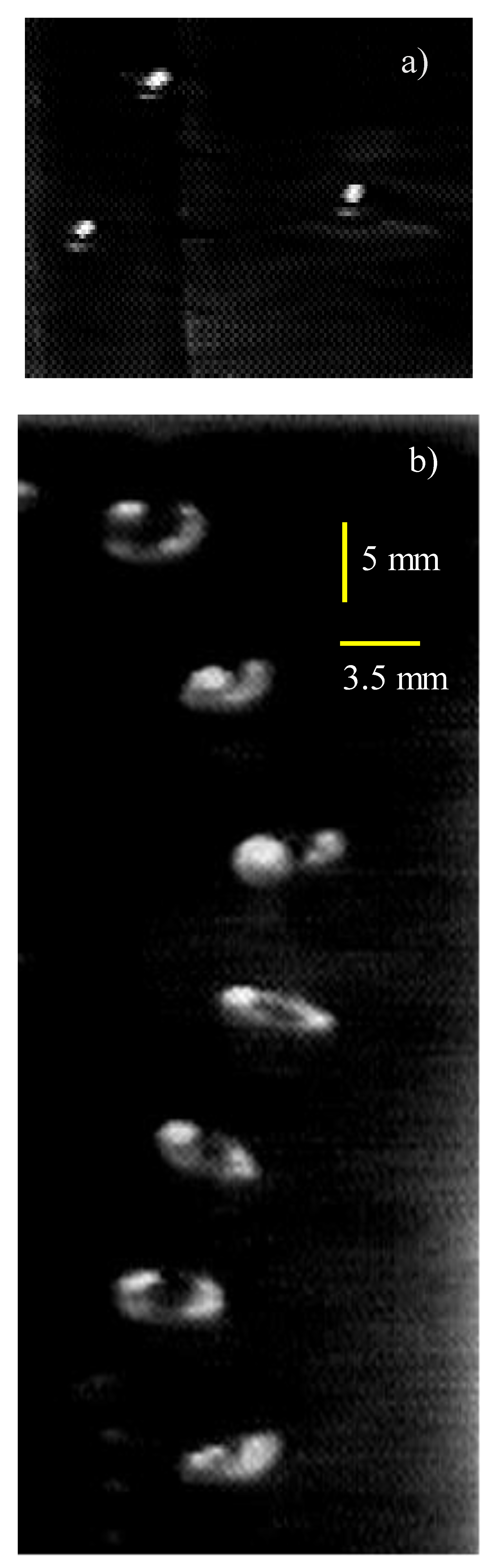
| Parameter | Values by Capillary Size | |||
|---|---|---|---|---|
| Capillary inner diameter, 2R (mm) | 0.5 | 1 | 1.15 | 1.5 |
| Predicted bubble diameter *, dpred (mm) | 2.81 | 3.54 | 3.7 | 4.04 |
| Measured bubble diameter **, d ± σd (mm) | 2.54 ± 0.3 | 3.01 ± 0.4 | 3.63 ± 0.5 | 4.17 ± 0.43 |
| Mean rise velocity Vr ± σV (cm s−1) | 29.2 ± 1.4 | 26.1 ± 1.8 | 25.5 ± 1.7 | 28.1 ± 1.6 |
| Flow rate, F (cm3 min−1) | 0.232 | 0.464 | 0.53 | 0.694 |
| Water type | Distilled | Distilled | Distilled | Filtered |
| Distilled Water Data Plotted in Figure 1a | Filtered Tap Water Data Plotted in Figure 1b | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| S psu | T °C | γ0 mN m−1 | γ mN m−1 | σγ mN m−1 | ∆γ mN m−1 | S psu | T °C | γ0 mN m−1 | γ mN m−1 | σγ mN m−1 | ∆γ mN m−1 |
| 2 | 19.7 | 72.85 | 60.87 | 4.08 | 11.98 | 2 | 17.4 | 73.18 | 66.92 | 2.03 | 6.26 |
| 6 | 19.9 | 72.91 | 66.15 | 4.75 | 6.76 | 6 | 18.2 | 73.15 | 67.13 | 2.14 | 6.02 |
| 10 | 20.1 | 72.97 | 71.14 | 2.59 | 1.83 | 12 | 17.9 | 73.33 | 65.66 | 4.17 | 7.67 |
| 14 | 20.3 | 73.03 | 70.47 | 4.14 | 2.56 | 17 | 18.1 | 73.41 | 62.82 | 6.41 | 10.59 |
| 19 | 20.3 | 73.14 | 74.79 | 0.40 | −1.65 | 21 | 18.1 | 73.50 | 62.21 | 4.46 | 11.29 |
| 24 | 20.5 | 73.22 | 72.98 | 3.49 | 0.24 | 26 | 18.2 | 73.59 | 63.49 | 3.86 | 10.10 |
| 29 | 20.2 | 73.37 | 73.69 | 2.20 | −0.32 | 30 | 18.5 | 73.64 | 61.28 | 4.01 | 12.36 |
| 34 | 20.1 | 73.50 | 71.74 | 5.00 | 1.76 | 34 | 18.7 | 73.70 | 58.21 | 5.42 | 15.49 |
| 37 | 20.5 | 73.51 | 72.49 | 4.27 | 1.02 | 39 | 18.7 | 73.81 | 54.28 | 5.36 | 19.53 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anguelova, M.D.; Huq, P. Effects of Salinity on Surface Lifetime of Large Individual Bubbles. J. Mar. Sci. Eng. 2017, 5, 41. https://doi.org/10.3390/jmse5030041
Anguelova MD, Huq P. Effects of Salinity on Surface Lifetime of Large Individual Bubbles. Journal of Marine Science and Engineering. 2017; 5(3):41. https://doi.org/10.3390/jmse5030041
Chicago/Turabian StyleAnguelova, Magdalena D., and Pablo Huq. 2017. "Effects of Salinity on Surface Lifetime of Large Individual Bubbles" Journal of Marine Science and Engineering 5, no. 3: 41. https://doi.org/10.3390/jmse5030041
APA StyleAnguelova, M. D., & Huq, P. (2017). Effects of Salinity on Surface Lifetime of Large Individual Bubbles. Journal of Marine Science and Engineering, 5(3), 41. https://doi.org/10.3390/jmse5030041





