Numerical Dynamic Response Analysis of a Ship Engine Room Explosion Simulation Using OpenFOAM
Abstract
1. Introduction
2. Materials and Methods
2.1. Theory
2.1.1. Explosion Process Equation
2.1.2. Simulation Method
2.2. Validation of Explosion Simulation
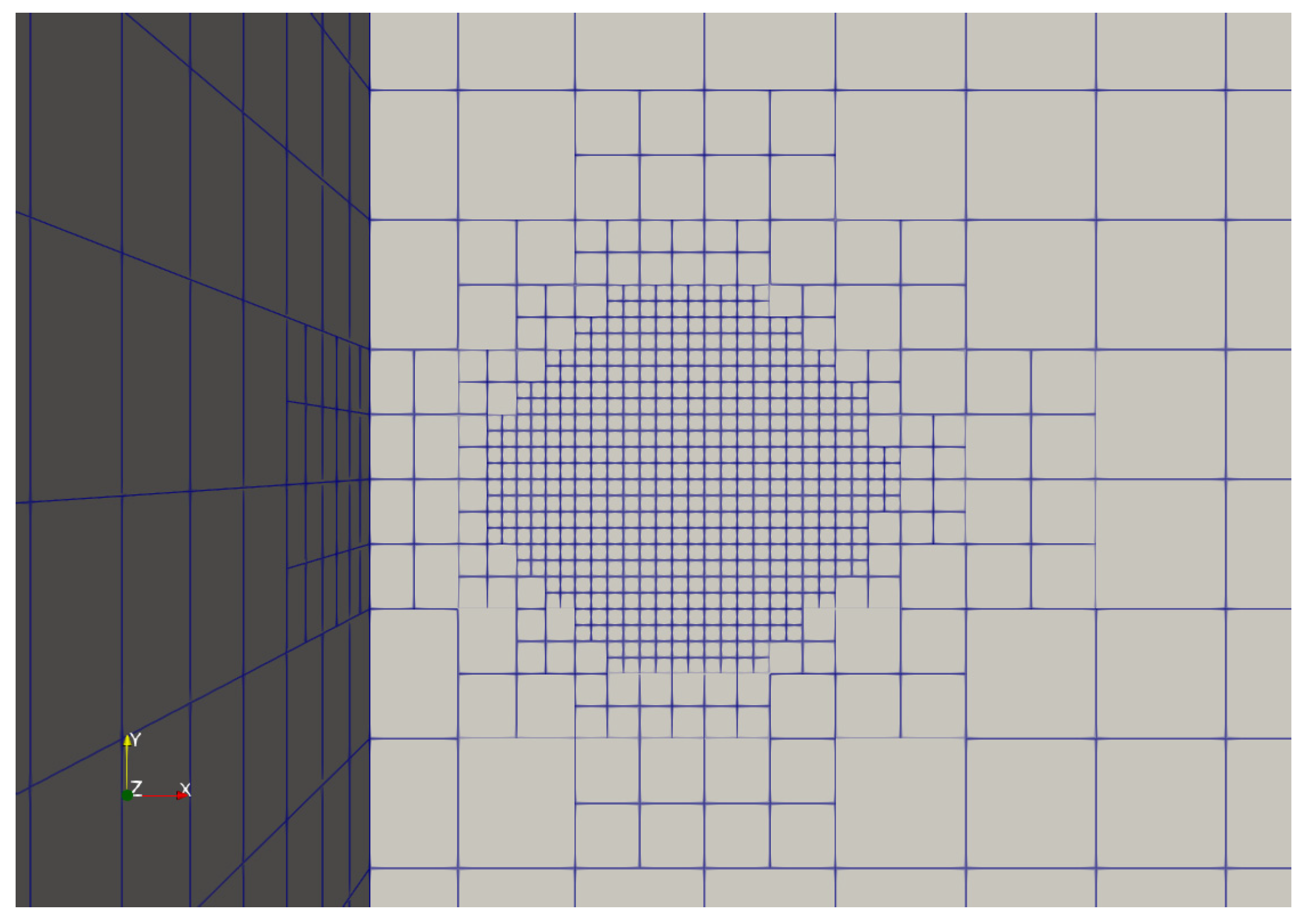
2.3. Configuration Settings
2.3.1. Ship Parameters
2.3.2. Boundary Conditions
3. Results
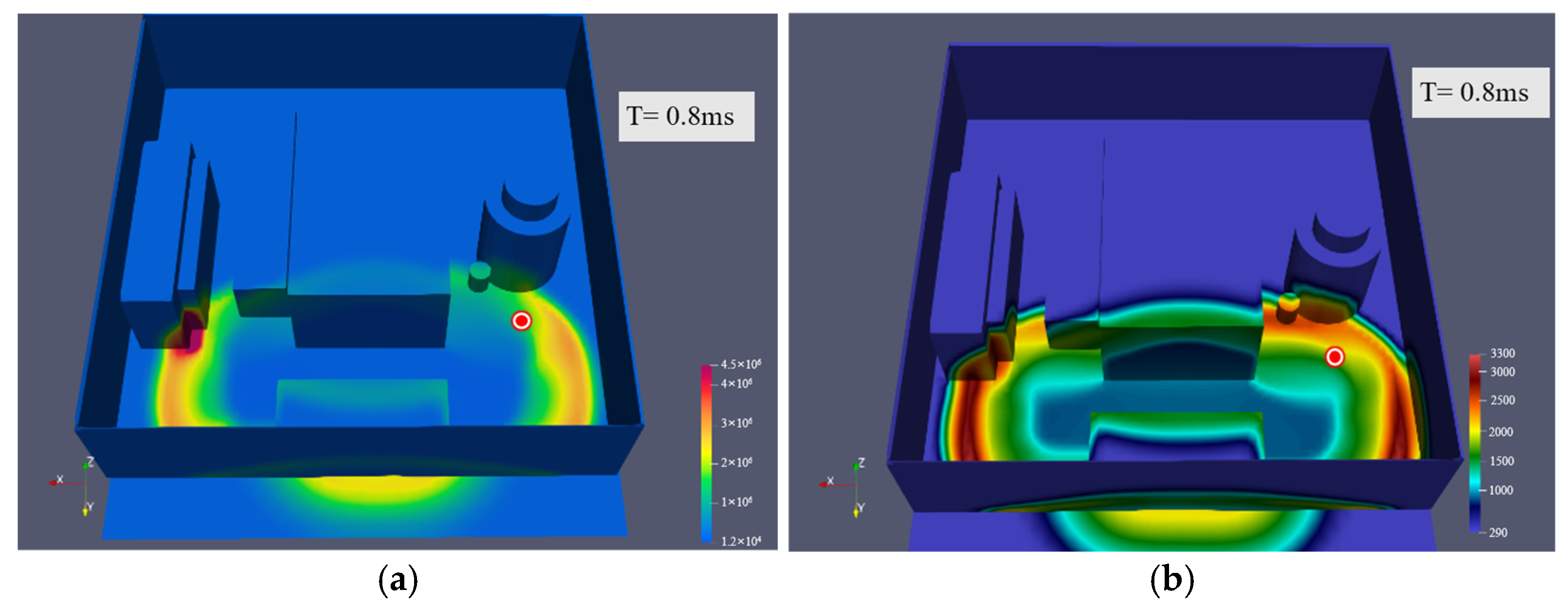
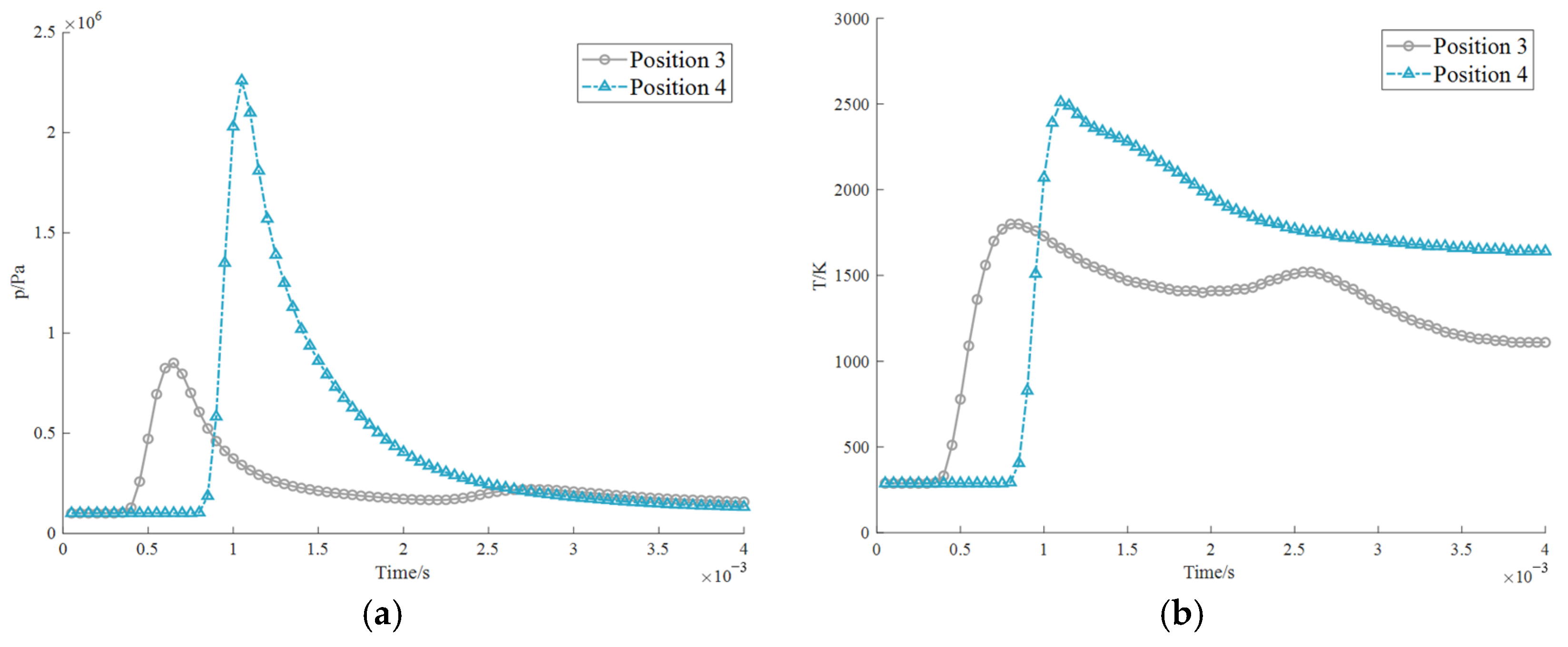
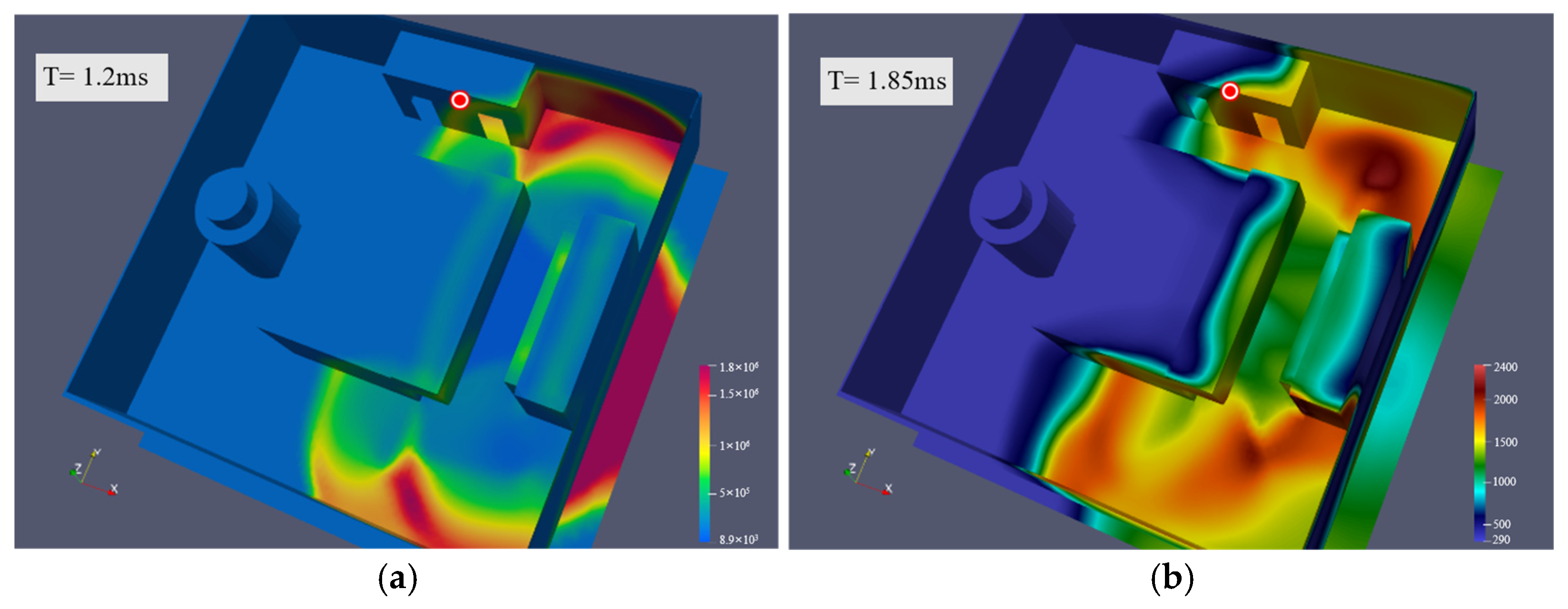
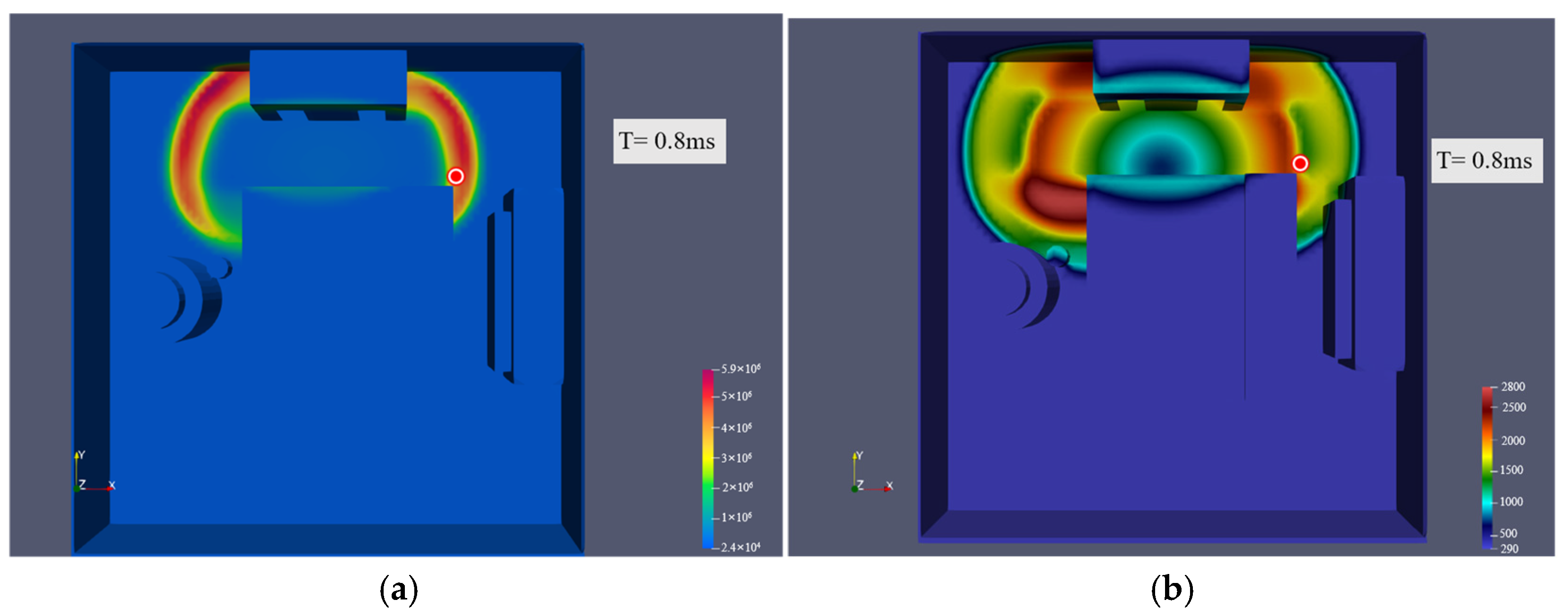
3.1. Effect of Explosion Location
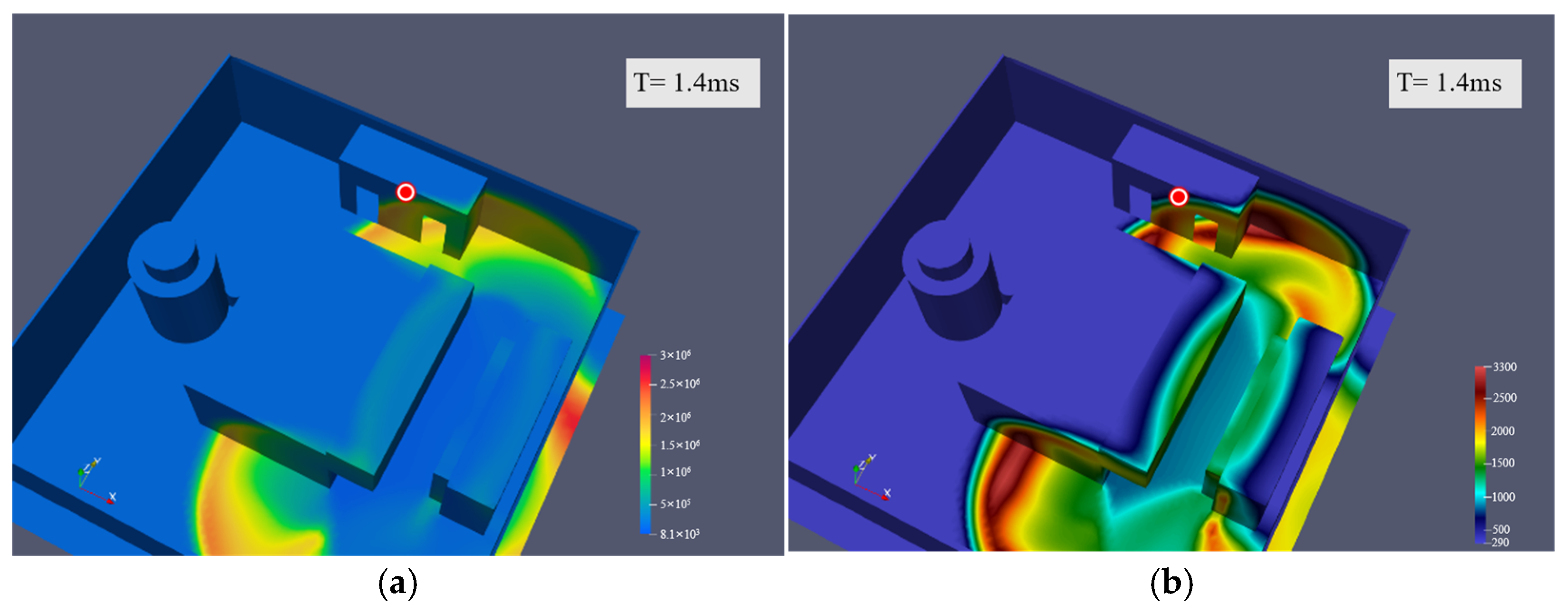
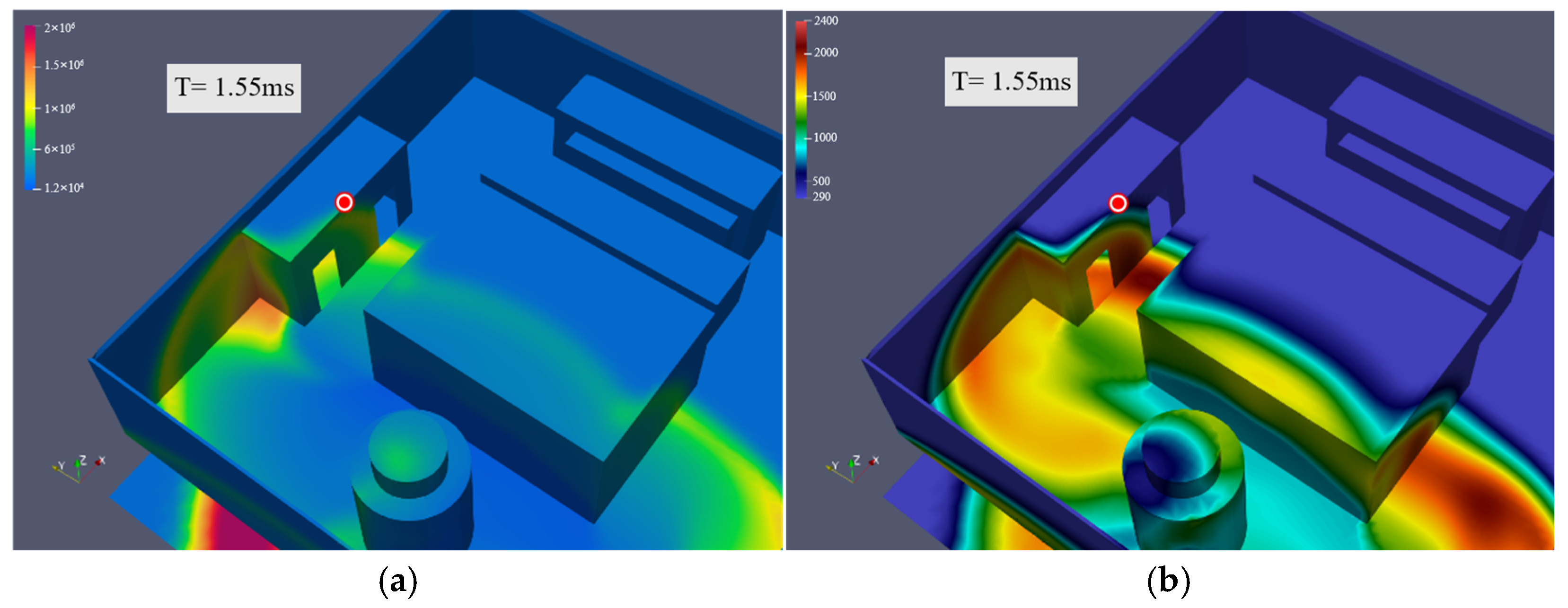
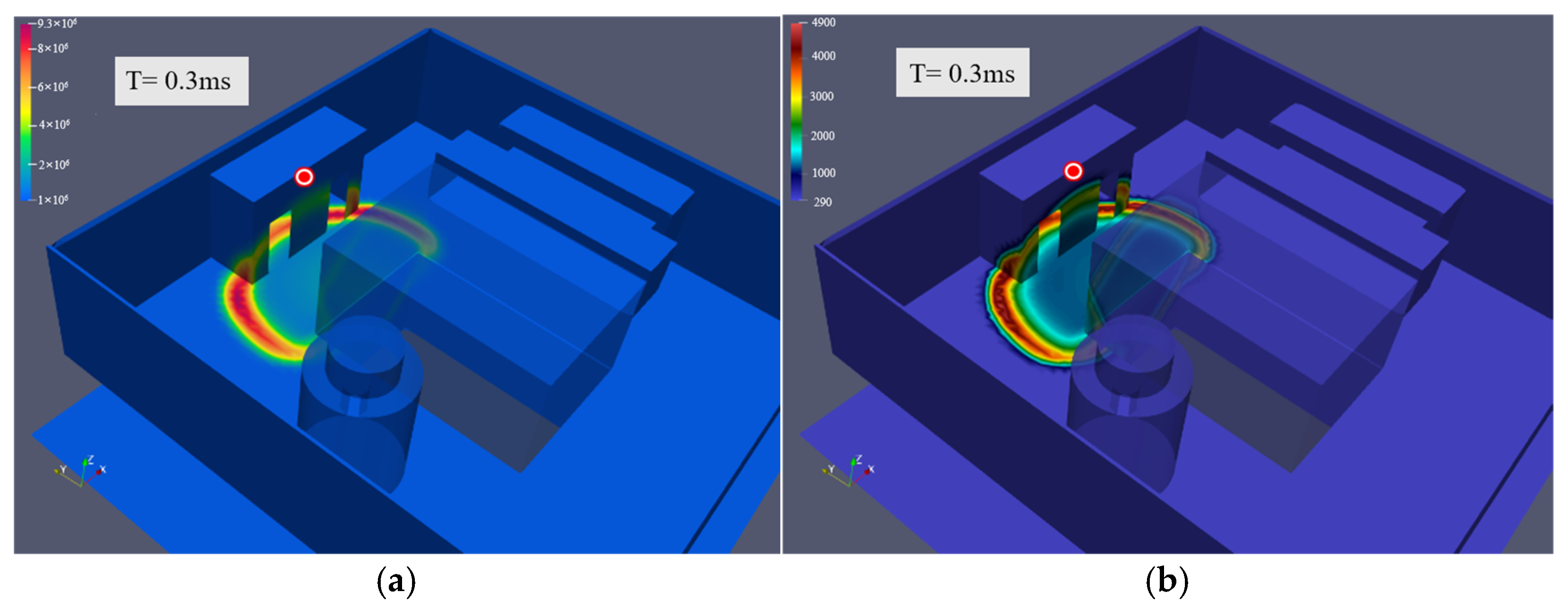
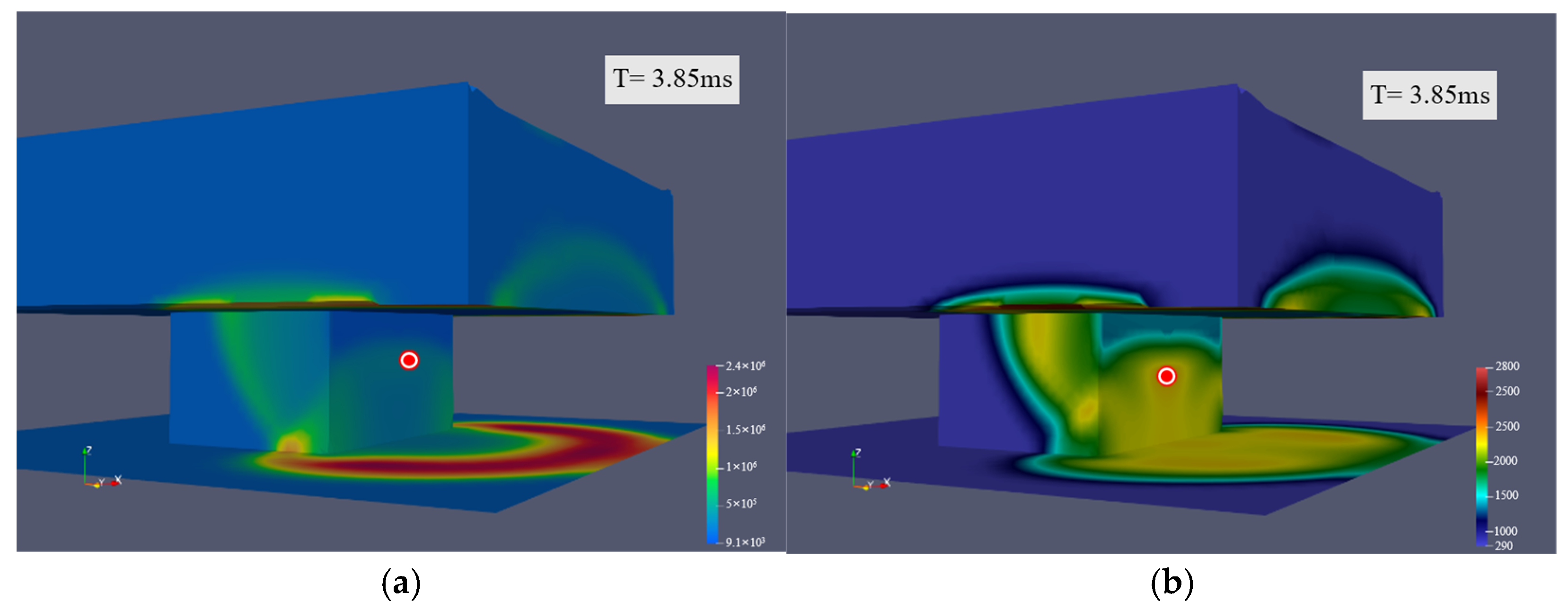
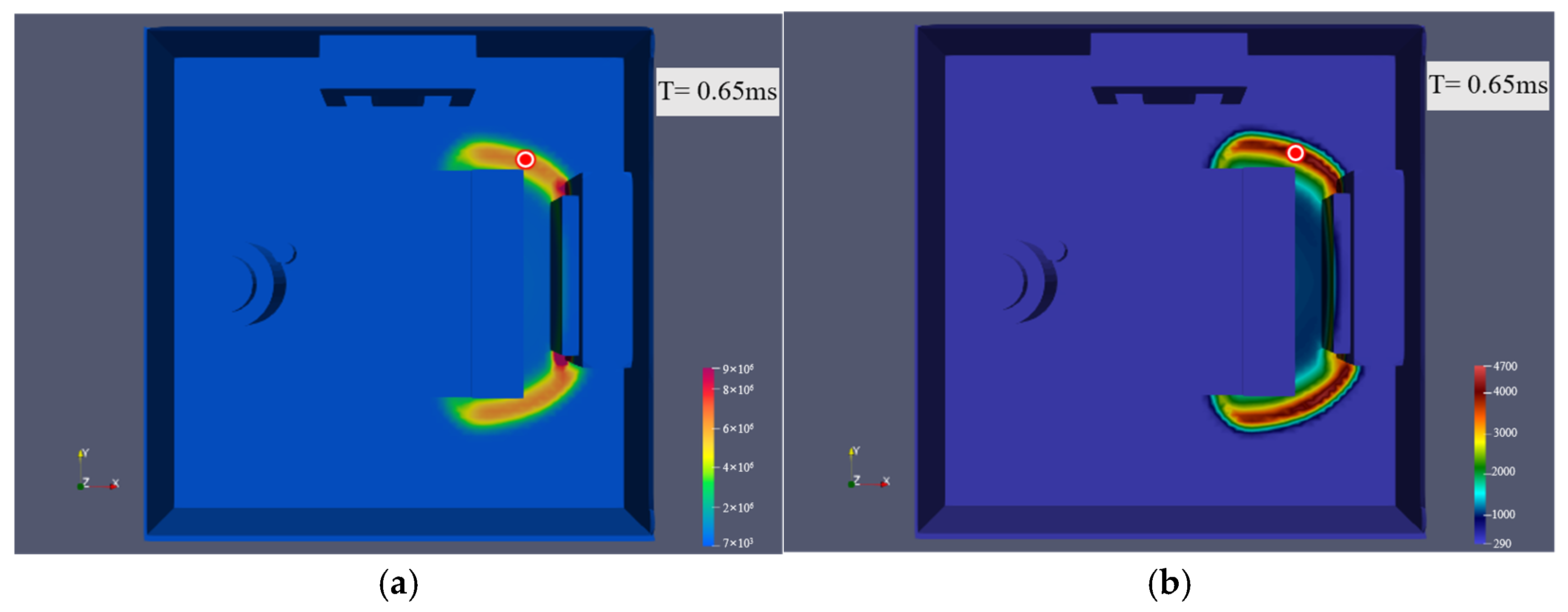
3.1.1. Boiler
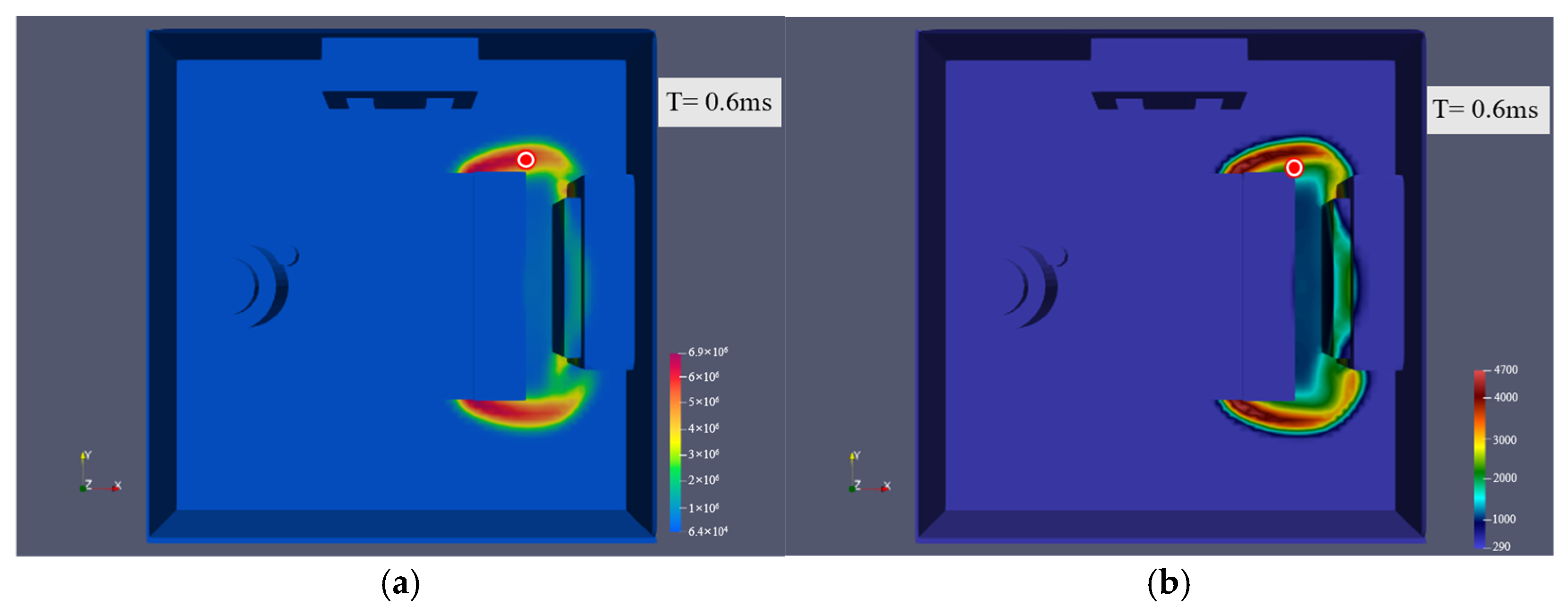
3.1.2. Centralized Control Room
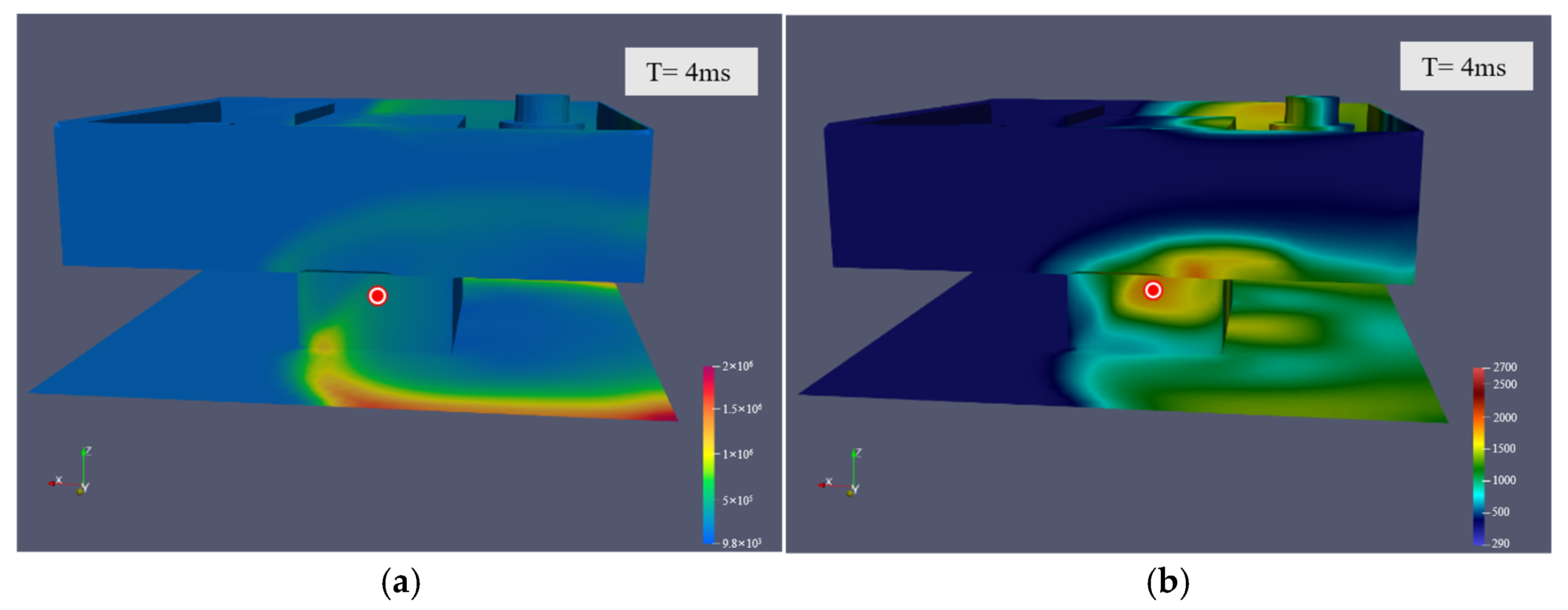
3.1.3. Lower Level of the Engine Compartment
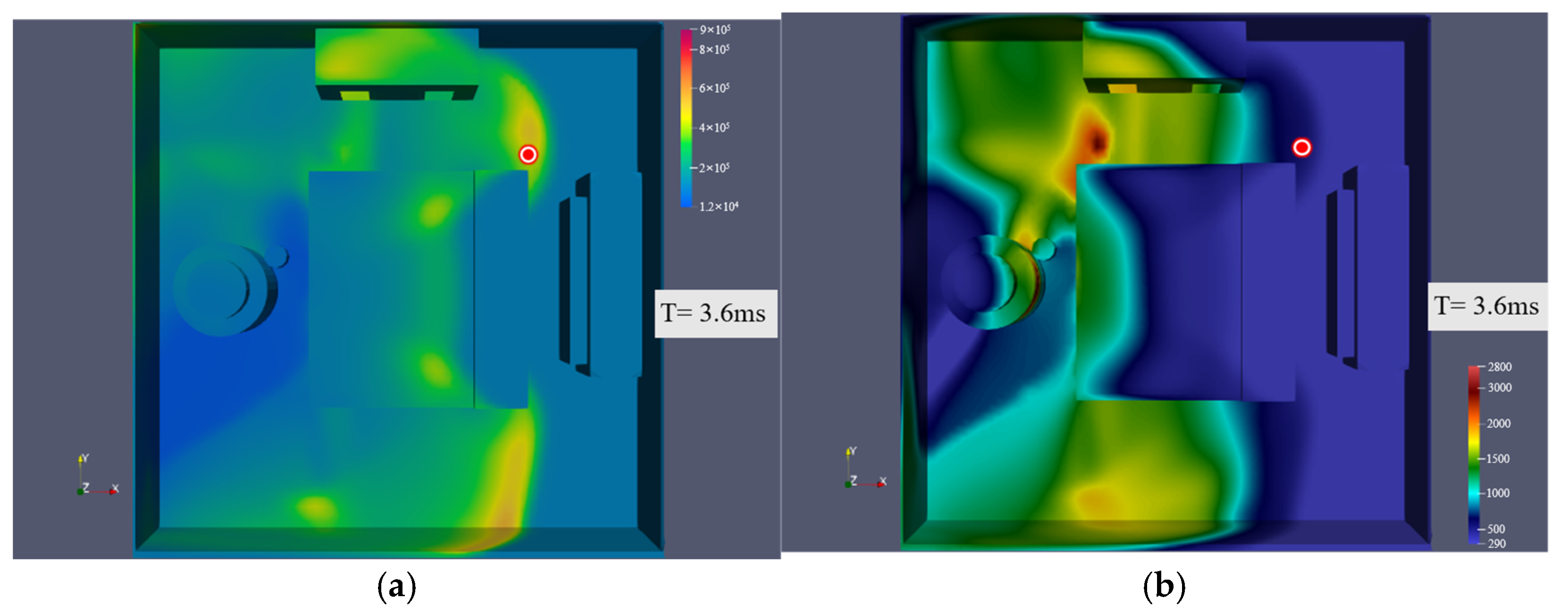
3.1.4. Intermediate
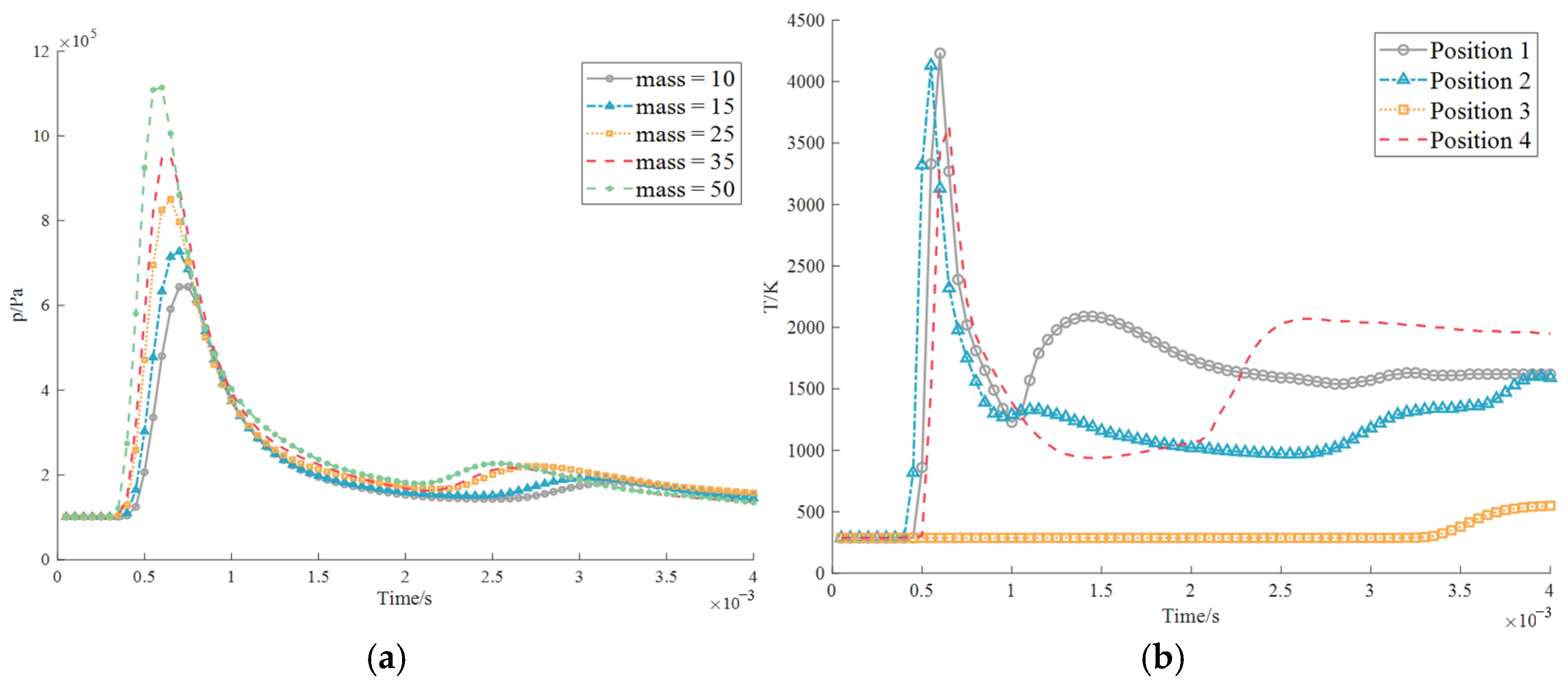
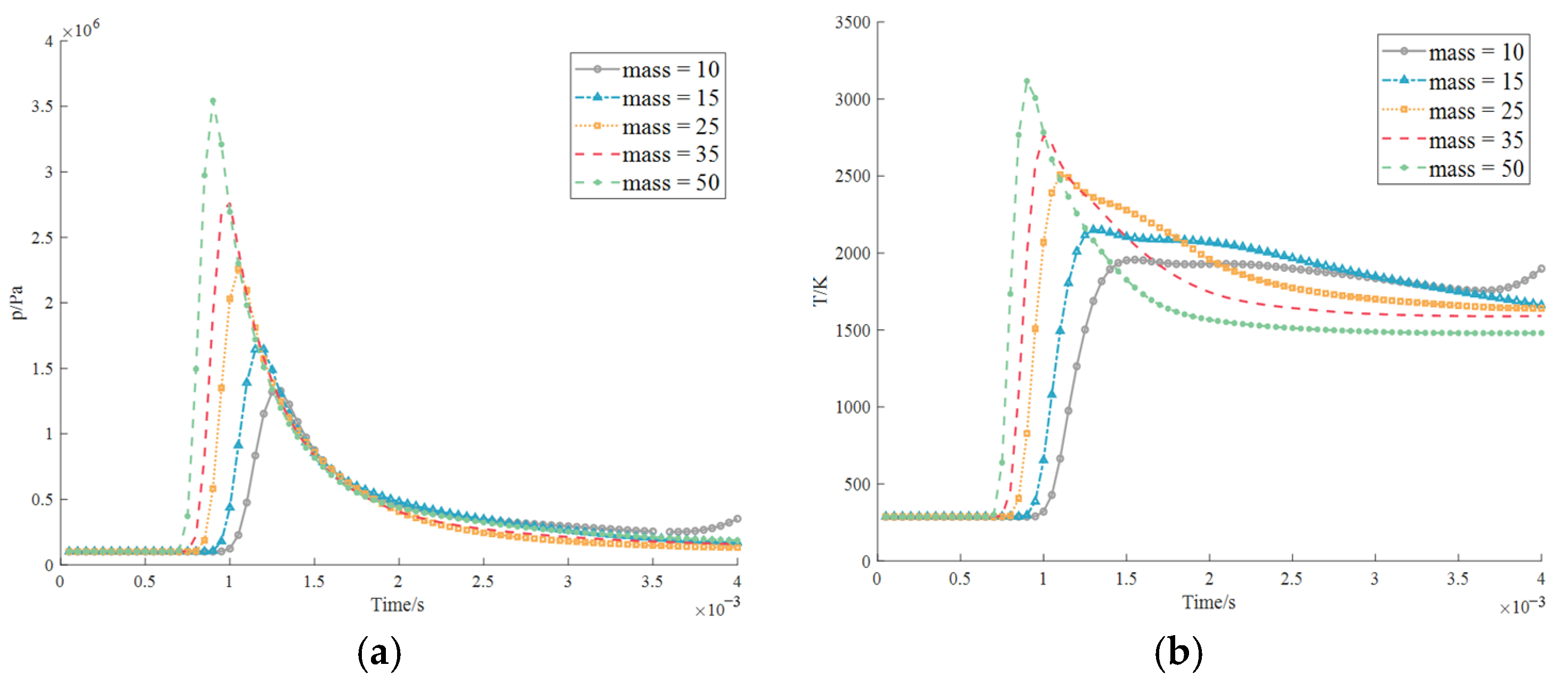
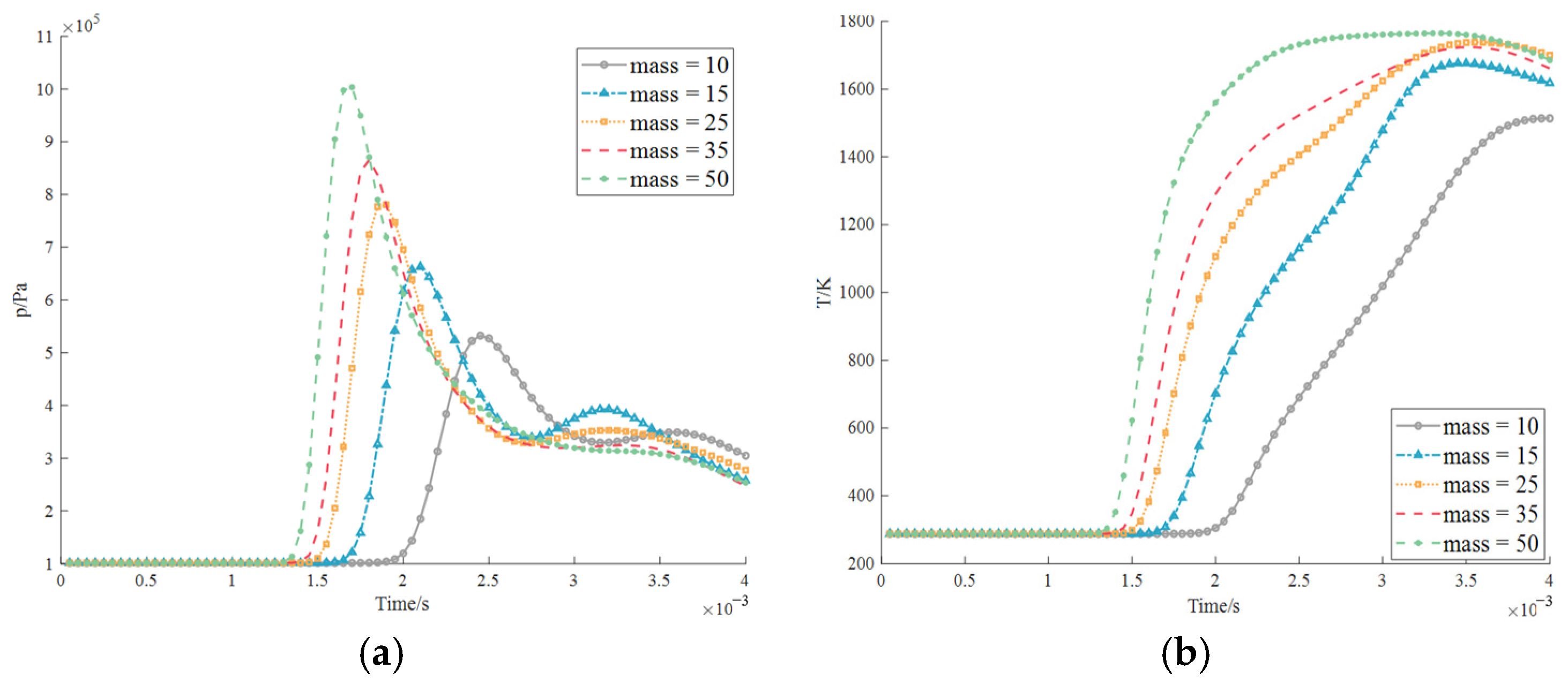
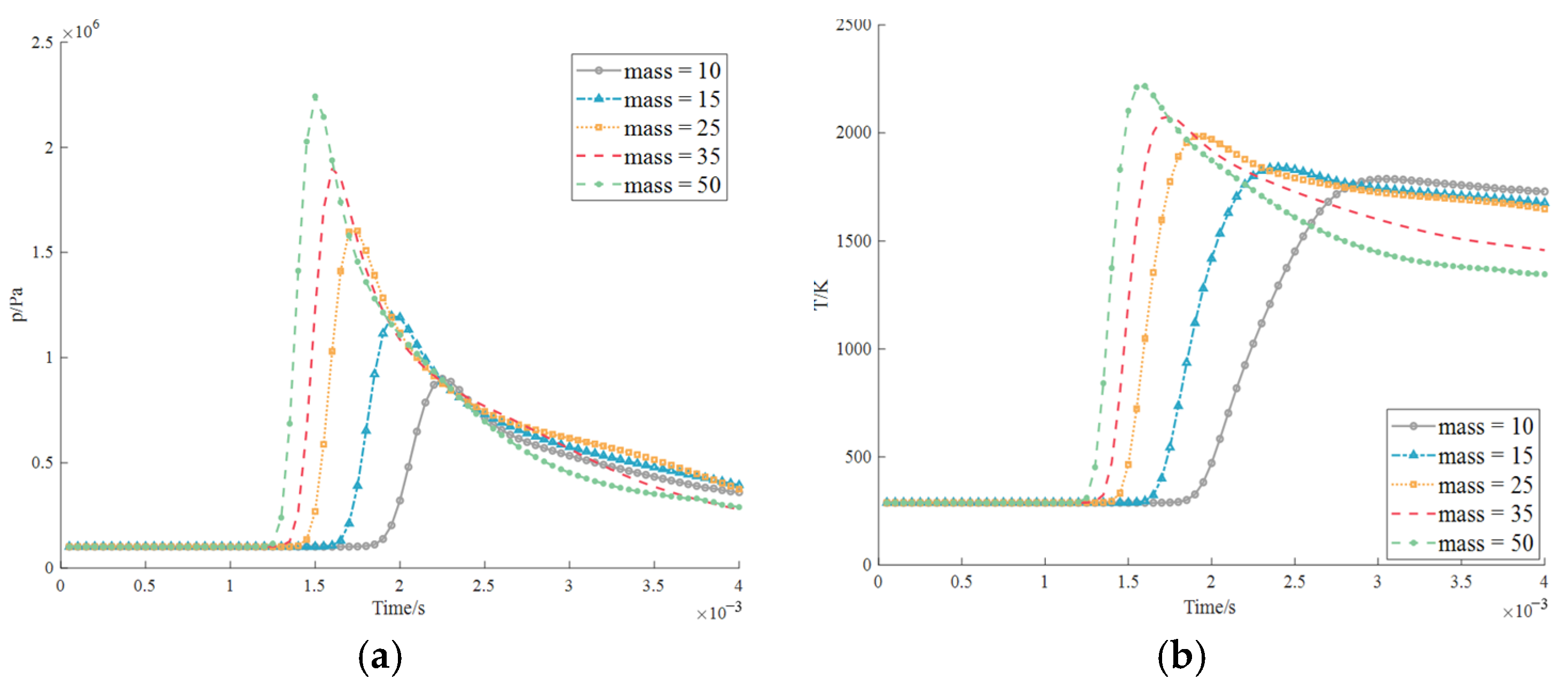
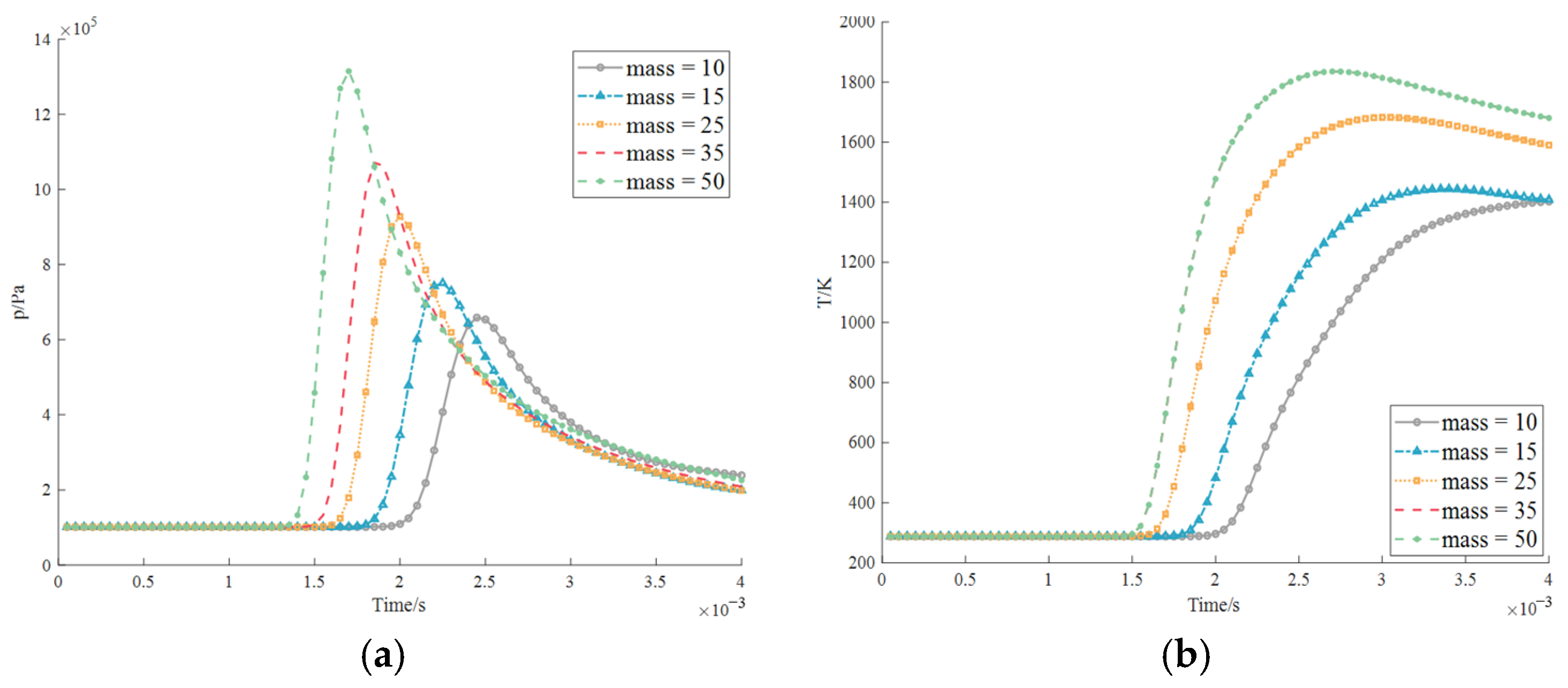
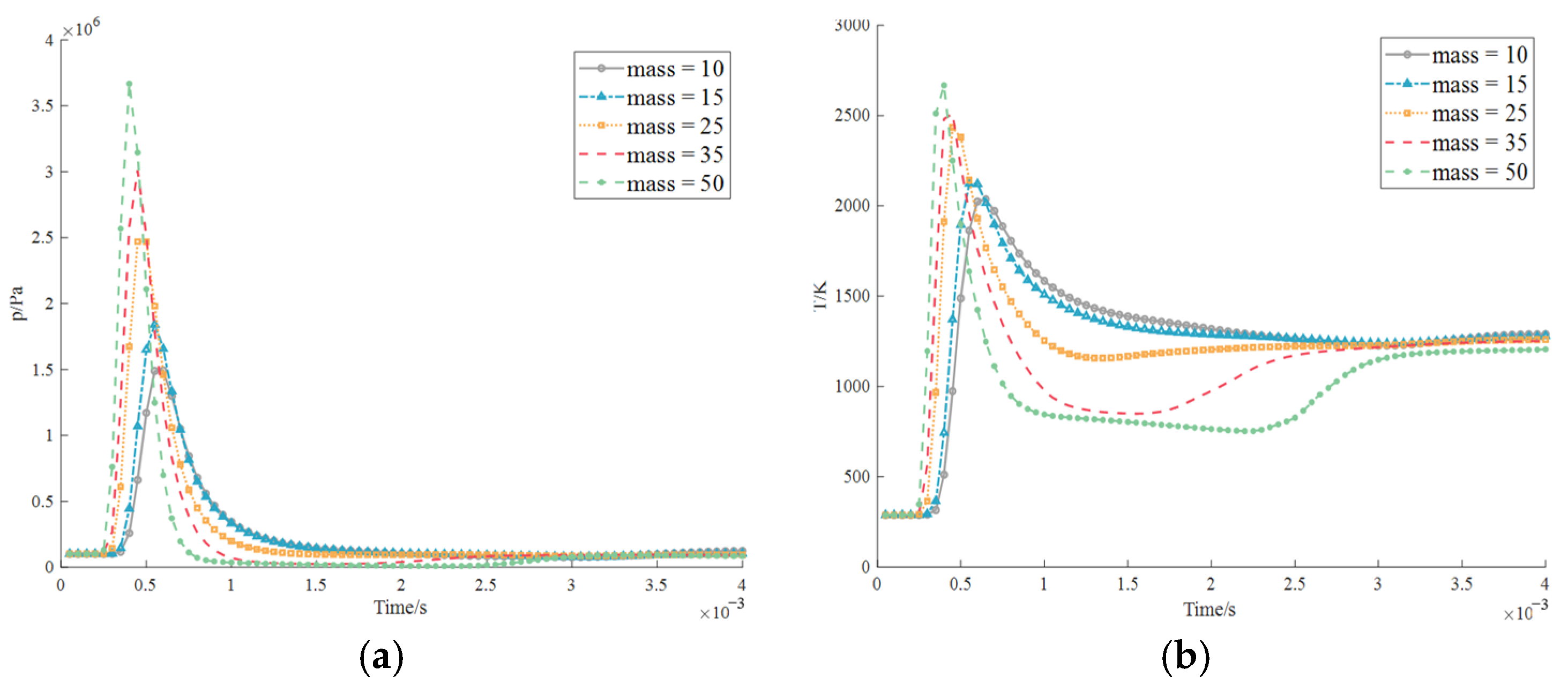
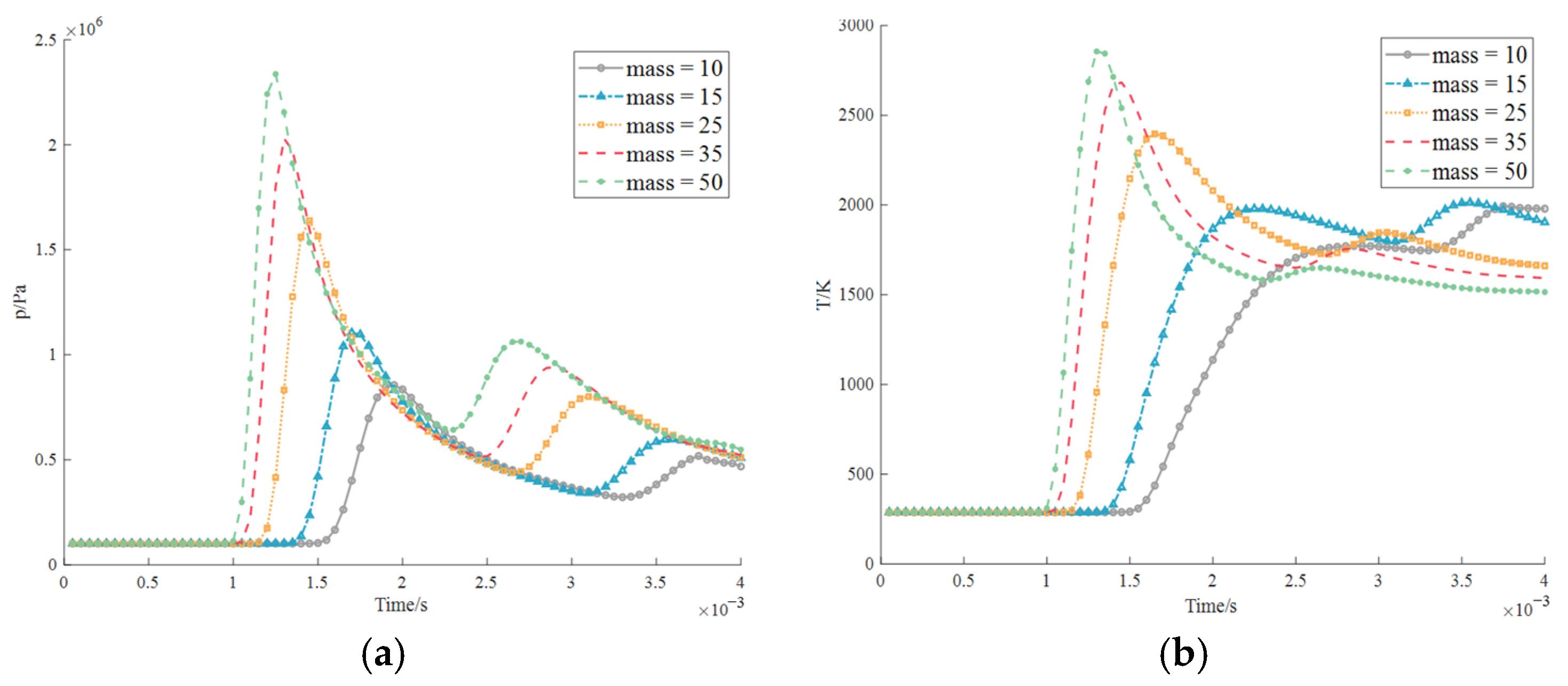
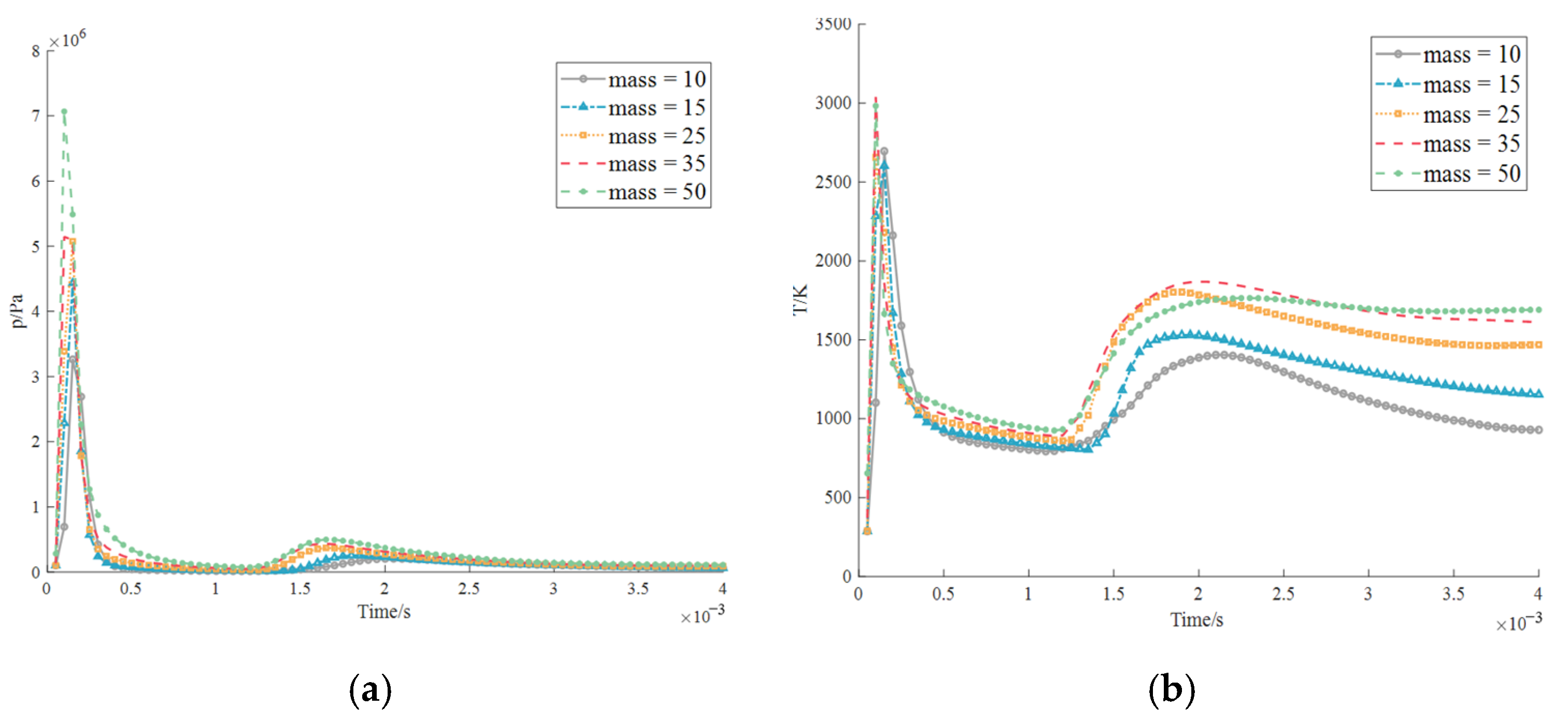
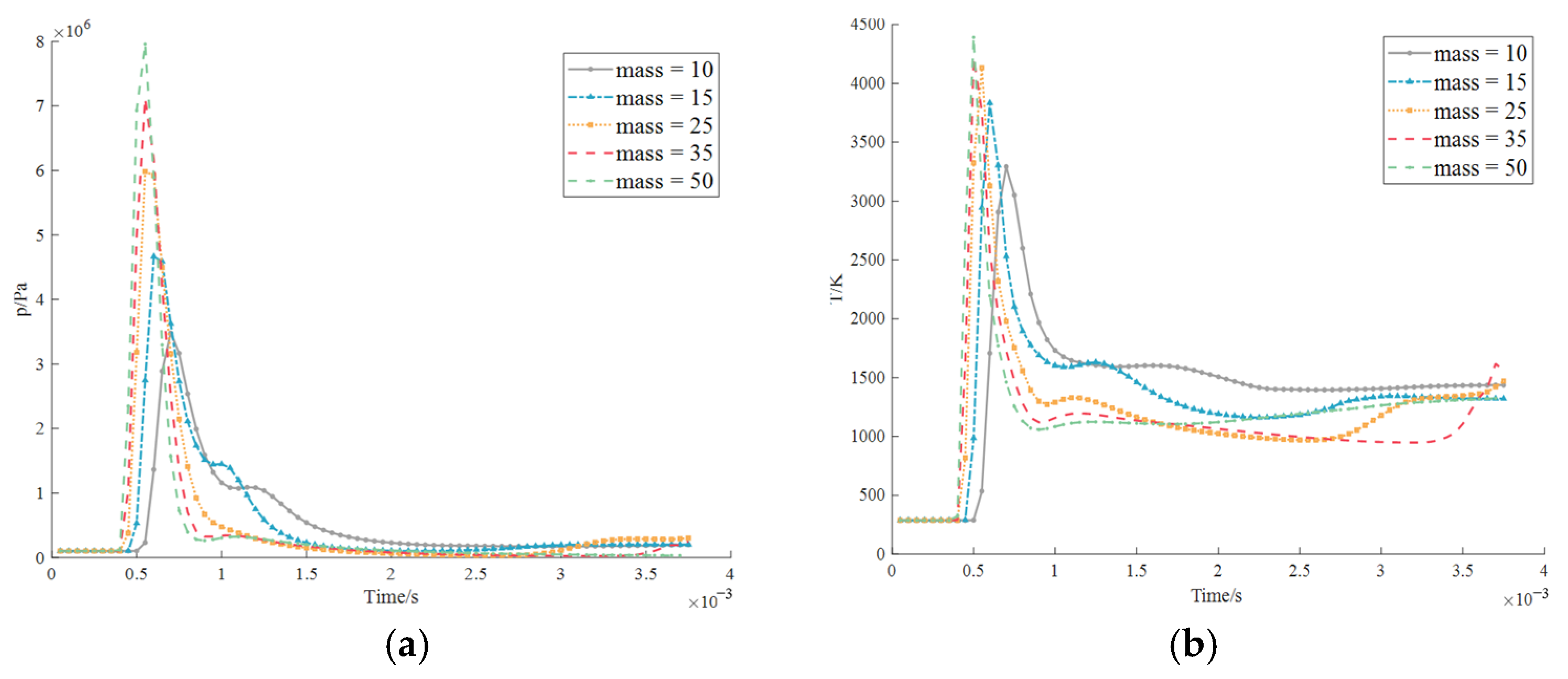
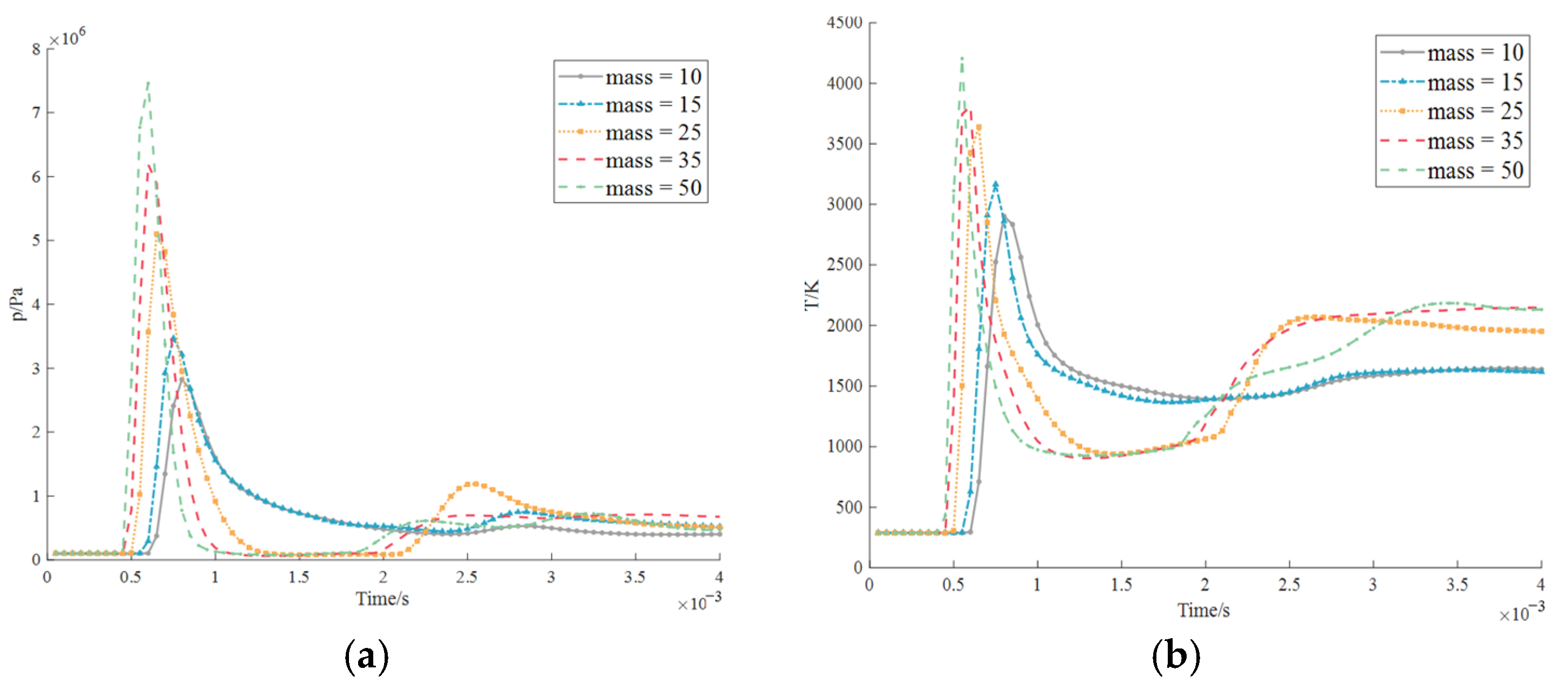
3.2. Impact of Explosion Size
3.2.1. Boiler
3.2.2. Centralized Control Room
3.2.3. Lower Level of the Engine Compartment
3.2.4. Intermediate
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| OpenFOAM | Open Field Operation and Manipulation |
| IMO | International Maritime Organization |
| WENO | Weighted Essentially Non-Oscillatory |
| JWL | Jones–Wilkins–Lee |
| TNT | Trinitrotoluene |
| LNG | Liquefied Natural Gas |
References
- International Maritime Organization (IMO). Global Integrated Shipping Information System (GISIS); IMO: London, UK, 2019. [Google Scholar]
- Sub-Committee on Ship Systems and Equipment, 10th Session (SSE 10), 4–8 March 2024. Available online: https://www.imo.org/en/MediaCentre/MeetingSummaries/Pages/SSE-10th-session.aspx (accessed on 1 April 2025).
- Wang, Y.; Bai, W.; Zhang, W.; Chen, S.; Zhao, Y. Optimal Course Tracking Control of USV with Input Dead Zone Based on Adaptive Fuzzy Dynamic Programing. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2024, 09596518241272850. [Google Scholar] [CrossRef]
- Rodríguez Castillo, C.A.; Yeter, B.; Li, S.; Brennan, F.; Collu, M. A Critical Review of Challenges and Opportunities for the Design and Operation of Offshore Structures Supporting Renewable Hydrogen Production, Storage, and Transport. Wind Energy Sci. 2024, 9, 533–554. [Google Scholar] [CrossRef]
- Talley, W.K. The Safety of Ferries: An Accident Injury Perspective. Marit. Policy Manag. 2002, 29, 331–338. [Google Scholar] [CrossRef]
- Wang, L.; Yang, Z. Bayesian Network Modelling and Analysis of Accident Severity in Waterborne Transportation: A Case Study in China. Reliab. Eng. Syst. Saf. 2018, 180, 277–289. [Google Scholar] [CrossRef]
- Franceschini, F.; Rossetto, S. Design for Quality: Selecting a Product’s Technical Features. Qual. Eng. 1997, 9, 681–688. [Google Scholar] [CrossRef]
- Cicek, K.; Celik, M. Application of Failure Modes and Effects Analysis to Main Engine Crankcase Explosion Failure On-Board Ship. Saf. Sci. 2013, 51, 6–10. [Google Scholar] [CrossRef]
- Chen, J.; Zhang, W.; Li, S.; Zhang, F.; Zhu, Y.; Huang, X. Identifying Critical Factors of Oil Spill in the Tanker Shipping Industry Worldwide. J. Clean. Prod. 2018, 180, 1–10. [Google Scholar] [CrossRef]
- Salem, A. Fire Engineering Tools Used in Consequence Analysis. Ships Offshore Struct. 2010, 5, 155–187. [Google Scholar] [CrossRef]
- Ugurlu, O.; Yildirim, U.; Yuksekyildiz, E. Marine Accident Analysis with GIS. In Proceedings of the NAV 2012 17th International Conference on Ships and Shipping Research, Naples, Italy, 17–19 October 2012. [Google Scholar]
- Tekin, A.; Namias, N.; O’Keeffe, T.; Pizano, L.; Lynn, M.; Prater-Varas, R.; Quintana, O.D.; Borges, L.; Ishii, M.; Lee, S.; et al. A Burn Mass Casualty Event Due to Boiler Room Explosion on a Cruise Ship: Preparedness and Outcomes. Am. Surg. 2005, 71, 210–215. [Google Scholar] [CrossRef]
- Talley, W.K.; Jin, D.; Kite-Powell, H. Determinants of the Severity of Cruise Vessel Accidents. Transp. Res. Part D Transp. Environ. 2008, 13, 86–94. [Google Scholar] [CrossRef]
- Baalisampang, T.; Abbassi, R.; Garaniya, V.; Khan, F.; Dadashzadeh, M. Review and Analysis of Fire and Explosion Accidents in Maritime Transportation. Ocean Eng. 2018, 158, 350–366. [Google Scholar] [CrossRef]
- Ming, F.R.; Zhang, A.M.; Xue, Y.Z.; Wang, S.P. Damage Characteristics of Ship Structures Subjected to Shockwaves of Underwater Contact Explosions. Ocean Eng. 2016, 117, 359–382. [Google Scholar] [CrossRef]
- Nurick, G.N.; Martin, J.B. Deformation of Thin Plates Subjected to Impulsive Loading—A Review: Part I: Theoretical Considerations. Int. J. Impact Eng. 1989, 8, 159–170. [Google Scholar] [CrossRef]
- Weibull, H.R.W. Pressures Recorded in Partially Closed Chambers at Explosion of Tnt Charges. Ann. N. Y. Acad. Sci. 1968, 152, 357–361. [Google Scholar] [CrossRef]
- Chen, Z.; Ren, H.; Ning, H.; Zhou, S.; Long, Z.; Huang, K. Analysis of Flow Field in a Blast Simulator Combined-Driven by Explosive Charge and Compressed Gas. Front. Earth Sci. 2023, 10, 1094415. [Google Scholar] [CrossRef]
- Liu, X.-D.; Osher, S.; Chan, T. Weighted Essentially Non-Oscillatory Schemes. J. Comput. Phys. 1994, 115, 200–212. [Google Scholar] [CrossRef]
- Jiang, G.-S.; Shu, C.-W. Efficient Implementation of Weighted ENO Schemes. J. Comput. Phys. 1996, 126, 202–228. [Google Scholar] [CrossRef]
- Shi, J.; Zhang, Y.-T.; Shu, C.-W. Resolution of High Order WENO Schemes for Complicated Flow Structures. J. Comput. Phys. 2003, 186, 690–696. [Google Scholar] [CrossRef]
- Stewart, J.B. Approximating a Free-Field Blast Environment in the Test Section of an Explosively Driven Conical Shock Tube. Shock Waves 2019, 29, 355–360. [Google Scholar] [CrossRef]
- Liu, Y.L.; Zhang, A.M.; Tian, Z.L.; Wang, S.P. Numerical Investigation on Global Responses of Surface Ship Subjected to Underwater Explosion in Waves. Ocean Eng. 2018, 161, 277–290. [Google Scholar] [CrossRef]
- Li, S.; Liang, Q.Q. Fibre-Based Modelling for Predicting the Progressive Collapse of Cylindrical Shells under Combined Axial Compression and Bending Moment. Eng. Struct. 2022, 272, 114988. [Google Scholar] [CrossRef]
- Shin, Y.S. Ship Shock Modeling and Simulation for Far-Field Underwater Explosion. Comput. Struct. 2004, 82, 2211–2219. [Google Scholar] [CrossRef]
- Kar, A.R.; Dutta, B.B. Risk Analysis Techniques for Improving Fire Safety Onboard Ships. In Proceedings of the 30th International Conference on Offshore Mechanics and Arctic Engineering, Rotterdam, The Netherlands, 19–24 June 2011; American Society of Mechanical Engineers Digital Collection, 31 October 2011. pp. 1–6. [Google Scholar]
- Li, J.; Huang, Z. Fire and Explosion Risk Analysis and Evaluation for LNG Ships. Procedia Eng. 2012, 45, 70–76. [Google Scholar] [CrossRef]
- Lutfi Tunçel, A.; Bal Beşikçi, E.; Akyuz, E.; Arslan, O. Safety Analysis of Fire and Explosion (F&E) Accidents Risk in Bulk Carrier Ships under Fuzzy Fault Tree Approach. Saf. Sci. 2023, 158, 105972. [Google Scholar] [CrossRef]
- Yang, H.Y.; Kim, D.K.; Cho, N.K.; Li, S. A Study on Hull Girder Longitudinal Strength Prediction by Adopting the Accurate Plate-Combination Model Based on Load-Shortening Curve; American Society of Mechanical Engineers: New York, NY, USA, 2024; Volume 87790, p. V002T02A064. [Google Scholar]
- Kong, X.; Zhou, H.; Zheng, C.; Liu, H.; Wu, W.; Guan, Z.; Dear, J.P. An Experimental Study on the Mitigation Effects of Fine Water Mist on Confined-Blast Loading and Dynamic Response of Steel Plates. Int. J. Impact Eng. 2019, 134, 103370. [Google Scholar] [CrossRef]
- Nubli, H.; Sohn, J.M. Procedure for Determining Design Accidental Loads in Liquified-Natural-Gas-Fuelled Ships under Explosion Using a Computational-Fluid-Dynamics-Based Simulation Approach. Ships Offshore Struct. 2022, 17, 2254–2271. [Google Scholar] [CrossRef]
- Smirnov, N.N.; Panfilov, I.I. Deflagration to Detonation Transition in Combustible Gas Mixtures. Combust. Flame 1995, 101, 91–100. [Google Scholar] [CrossRef]
- Kim, D.K.; Yang, H.Y.; Li, S.; Kim, S. An Updated Design Formula for Predicting the Compressive Strength of Plate: Elastic Buckling and Ultimate Compressive Strength. J. Mar. Sci. Eng. 2025, 13, 113. [Google Scholar] [CrossRef]
- Yang, A.; Liu, Y.; Gao, K.; Li, R.; Li, Q.; Li, S. Numerical Simulation of Gas Explosion with Non-Uniform Concentration Distribution by Using OpenFOAM. ACS Omega 2023, 8, 48798–48812. [Google Scholar] [CrossRef]
- Li, S.; Brennan, F. Digital Twin Enabled Structural Integrity Management: Critical Review and Framework Development. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2024, 238, 707–727. [Google Scholar] [CrossRef]
- Khan, R.U.; Yin, J.; Mustafa, F.S.; Liu, H. Risk Assessment and Decision Support for Sustainable Traffic Safety in Hong Kong Waters. IEEE Access 2020, 8, 72893–72909. [Google Scholar] [CrossRef]
- Uğurlu, Ö.; Köse, E.; Yıldırım, U.; Yüksekyıldız, E. Marine Accident Analysis for Collision and Grounding in Oil Tanker Using FTA Method. Marit. Policy Manag. 2015, 42, 163–185. [Google Scholar] [CrossRef]
- Arslan, O.; Er, I.D. SWOT Analysis for Safer Carriage of Bulk Liquid Chemicals in Tankers. J. Hazard. Mater. 2008, 154, 901–913. [Google Scholar] [CrossRef] [PubMed]
- Ellis, J. Analysis of Accidents and Incidents Occurring during Transport of Packaged Dangerous Goods by Sea. Saf. Sci. 2011, 49, 1231–1237. [Google Scholar] [CrossRef]
- Cao, X.; Lam, J.S.L. A Fast Reaction-Based Port Vulnerability Assessment: Case of Tianjin Port Explosion. Transp. Res. Part A Policy Pract. 2019, 128, 11–33. [Google Scholar] [CrossRef]
- Kim, D.K.; Wong, A.M.K.; Hwang, J.; Li, S.; Cho, N.-K. A Novel Formula for Predicting the Ultimate Compressive Strength of the Cylindrically Curved Plates. Int. J. Nav. Archit. Ocean Eng. 2024, 16, 100562. [Google Scholar] [CrossRef]
- Wu, C.; Huang, L. A New Accident Causation Model Based on Information Flow and Its Application in Tianjin Port Fire and Explosion Accident. Reliab. Eng. Syst. Saf. 2019, 182, 73–85. [Google Scholar] [CrossRef]
- Zhang, Y.; Jing, L.; Sun, C. Systems-Based Analysis of China-Tianjin Port Fire and Explosion: A Comparison of HFACS, AcciMap, and STAMP. J. Fail. Anal. Preven. 2018, 18, 1386–1400. [Google Scholar] [CrossRef]
- Bhardwaj, U.; Teixeira, A.P.; Guedes Soares, C.; Ariffin, A.K.; Singh, S.S. Evidence Based Risk Analysis of Fire and Explosion Accident Scenarios in FPSOs. Reliab. Eng. Syst. Saf. 2021, 215, 107904. [Google Scholar] [CrossRef]
- Kang, J.; Lv, K.; Sun, Y.; Li, M. Predictive Risk Assessment Framework for Leakage Accident of Offshore LNG Transfer System. Expert Syst. Appl. 2025, 271, 126580. [Google Scholar] [CrossRef]
- Heylmun, J.; Vonk, P.; Brewer, T. Blastfoam Theory and User Guide; Synthetik Applied Technologies: Austin, TX, USA, 2019. [Google Scholar]
- Gao, Y.; Yang, B.; Zhu, H.; Peng, G.; Zhang, Z.; Pan, G. Numerical Simulation of Flow Induced Vibration of Two Rigidly Connected Cylinders in Different Arrangements. Ships Offshore Struct. 2022, 17, 1142–1158. [Google Scholar] [CrossRef]
- Li, G.; Shi, D.; Wang, L.; Zhao, K.; Helal, W.M.K. Numerical Study on Structural Damage Characteristics under Long Pulse Width of Underwater Nuclear Explosions. Ships Offshore Struct. 2023, 18, 1746–1754. [Google Scholar] [CrossRef]
- Liu, K.; Lu, Y.; Wang, Z.; Wang, G. An Experimental, Numerical and Analytical Study on Deformation Mechanisms of Web Girders in a Collision or Grounding Incident. Ships Offshore Struct. 2019, 14, 839–852. [Google Scholar] [CrossRef]
- Zhang, S.; Guo, X.; Gan, X.; Gong, C.; Yang, W.; Li, C. Numerical study of detonation shock with partitioned fluid-structure interaction simulations. kqdlxxb 2022, 40, 163–172. [Google Scholar] [CrossRef]
- Shehu, E.; Lomazzi, L.; Giglio, M.; Manes, A. Computational Modeling of Confined Blast Waves with Focus on Interaction with Structures. IOP Conf. Ser. Mater. Sci. Eng. 2023, 1275, 012028. [Google Scholar] [CrossRef]
- Wu, J.; Sun, Z.; Jiang, Y.; Zhang, G.; Sun, T. Experimental and Numerical Study of Slamming Problem for a Trimaran Hull. Ships Offshore Struct. 2021, 16, 46–53. [Google Scholar] [CrossRef]
- Jeremić, R.; Bajić, Z. An Approach to Determining the TNT Equivalent of High Explosives. Sci. Tech. Rev. 2006, 56, 58–62. [Google Scholar]
- Shakor, Z.M.; AbdulRazak, A.A.; Sukkar, K.A. A Detailed Reaction Kinetic Model of Heavy Naphtha Reforming. Arab. J. Sci. Eng. 2020, 45, 7361–7370. [Google Scholar] [CrossRef]
- Salakhi, M.; Eghtesad, A.; Afshin, H. Heat and Mass Transfer Analysis and Optimization of Freeze Desalination Utilizing Cold Energy of LNG Leaving a Power Generation Cycle. Desalination 2022, 527, 115595. [Google Scholar] [CrossRef]
- UFC 3-340-02; Structures to Resist the Effects of Accidental Explosions. U.S. Department of Defense: Washington, DC, USA, 2008.
- Li, S.; Hu, Z.; Benson, S. Progressive Collapse Analysis of Ship Hull Girders Subjected to Extreme Cyclic Bending. Mar. Struct. 2020, 73, 102803. [Google Scholar] [CrossRef]
- Wu, C.; Zhang, G.; He, Y.; Liu, L.; Liu, J.; Yang, X. Dynamic Response of Blast Doors Enhanced by Enclosed-Space TNT Explosions: Experimental and Numerical Study. Def. Technol. 2025. [Google Scholar] [CrossRef]
- Yang, B.; Wang, D. Dynamic Ultimate Hull Girder Strength Analysis on a Container Ship under Impact Bending Moments. Int. J. Offshore Polar Eng. 2018, 28, 105–111. [Google Scholar] [CrossRef]
- Liu, H.-H.; Rutqvist, J.; Berryman, J.G. On the Relationship between Stress and Elastic Strain for Porous and Fractured Rock. Int. J. Rock Mech. Min. Sci. 2009, 46, 289–296. [Google Scholar] [CrossRef]
- Mohotti, D.; Wijesooriya, K.; Weckert, S. A Simplified Approach to Modelling Blasts in Computational Fluid Dynamics (CFD). Def. Technol. 2023, 23, 19–34. [Google Scholar] [CrossRef]
- Sun, J.; Wang, Y.; Tian, B.; Chen, Z. detonationFoam: An Open-Source Solver for Simulation of Gaseous Detonation Based on OpenFOAM. Comput. Phys. Commun. 2023, 292, 108859. [Google Scholar] [CrossRef]
- Gnedin, N.Y.; Semenov, V.A.; Kravtsov, A.V. Enforcing the Courant–Friedrichs–Lewy Condition in Explicitly Conservative Local Time Stepping Schemes. J. Comput. Phys. 2018, 359, 93–105. [Google Scholar] [CrossRef]
- Weng, Z.; Mével, R. Implementation of an OpenFOAM Solver for Shock and Detonation Simulation at High Pressure. Comput. Fluids 2023, 265, 106012. [Google Scholar] [CrossRef]
- De Moura, C.A.; Kubrusly, C.S. The Courant–Friedrichs–Lewy (Cfl) Condition. AMC 2013, 10, 45–90. [Google Scholar]
- Edri, I.; Savir, Z.; Feldgun, V.R.; Karinski, Y.S.; Yankelevsky, D.Z. On Blast Pressure Analysis Due to a Partially Confined Explosion: I. Experimental Studies. Int. J. Prot. Struct. 2011, 2, 1–20. [Google Scholar] [CrossRef]
- Kim, E.S. Fracture Analysis of Tube Boiler for Physical Explosion Accident. Forensic Sci. Int. 2017, 278, e1–e7. [Google Scholar] [CrossRef]
- Brode, H.L. Numerical Solutions of Spherical Blast Waves. J. Appl. Phys. 1955, 26, 766–775. [Google Scholar] [CrossRef]
- Brode, H.L. Blast Wave from a Spherical Charge. Phys. Fluids 1959, 2, 217–229. [Google Scholar] [CrossRef]
- Remennikov, A.M. Blast Resistant Consulting: A New Challenge for Structural Engineers. Aust. J. Struct. Eng. 2002, 4, 121–134. [Google Scholar] [CrossRef]
- Lee Perry, W.; Clements, B.; Ma, X.; Mang, J.T. Relating Microstructure, Temperature, and Chemistry to Explosive Ignition and Shock Sensitivity. Combust. Flame 2018, 190, 171–176. [Google Scholar] [CrossRef]
- Luo, Z.; Li, R.; Wang, T.; Cheng, F.; Liu, Y.; Yu, Z.; Fan, S.; Zhu, X. Explosion Pressure and Flame Characteristics of CO/CH4/Air Mixtures at Elevated Initial Temperatures. Fuel 2020, 268, 117377. [Google Scholar] [CrossRef]
- Jeong, B.; Lee, B.S.; Zhou, P. Quantitative Risk Assessment of Fuel Preparation Room Having High-Pressure Fuel Gas Supply System for LNG Fuelled Ship. Ocean Eng. 2017, 137, 450–468. [Google Scholar] [CrossRef]
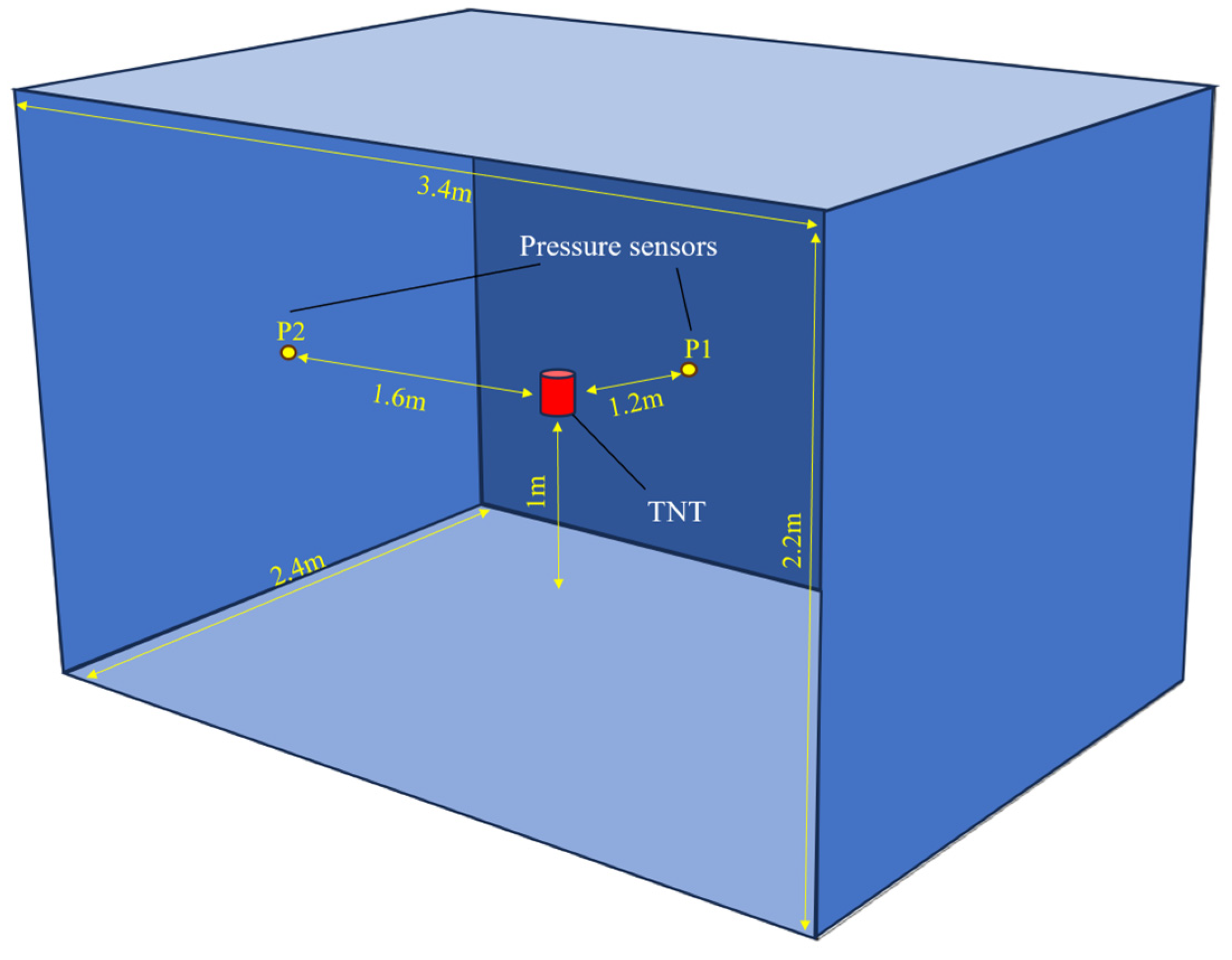
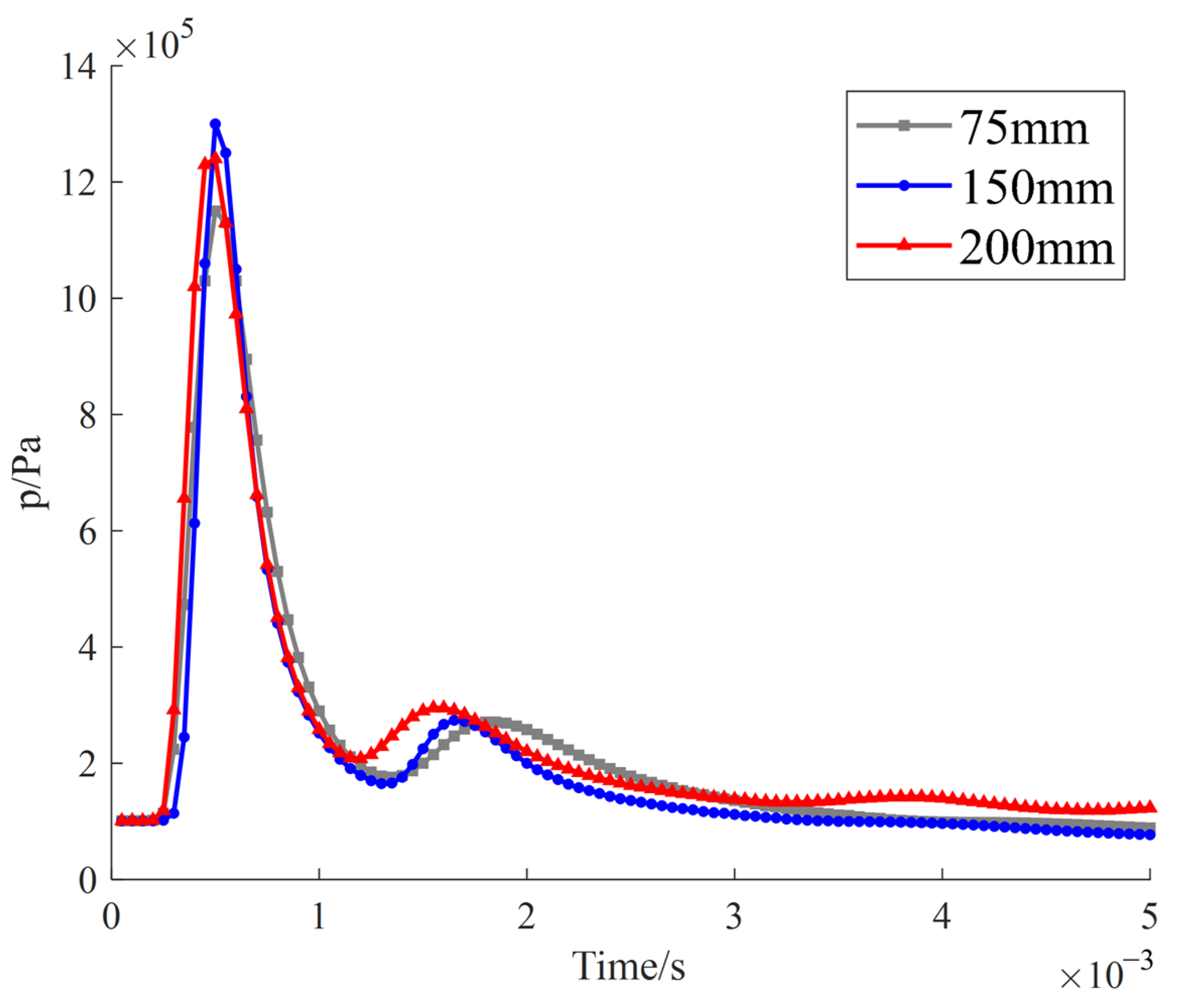
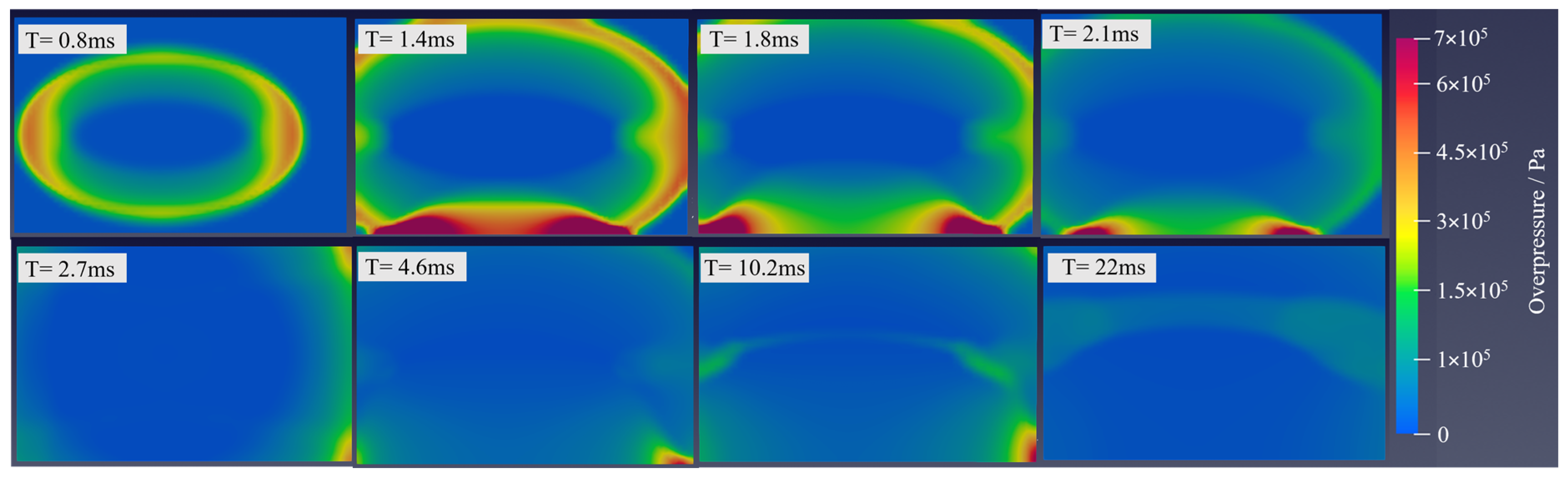

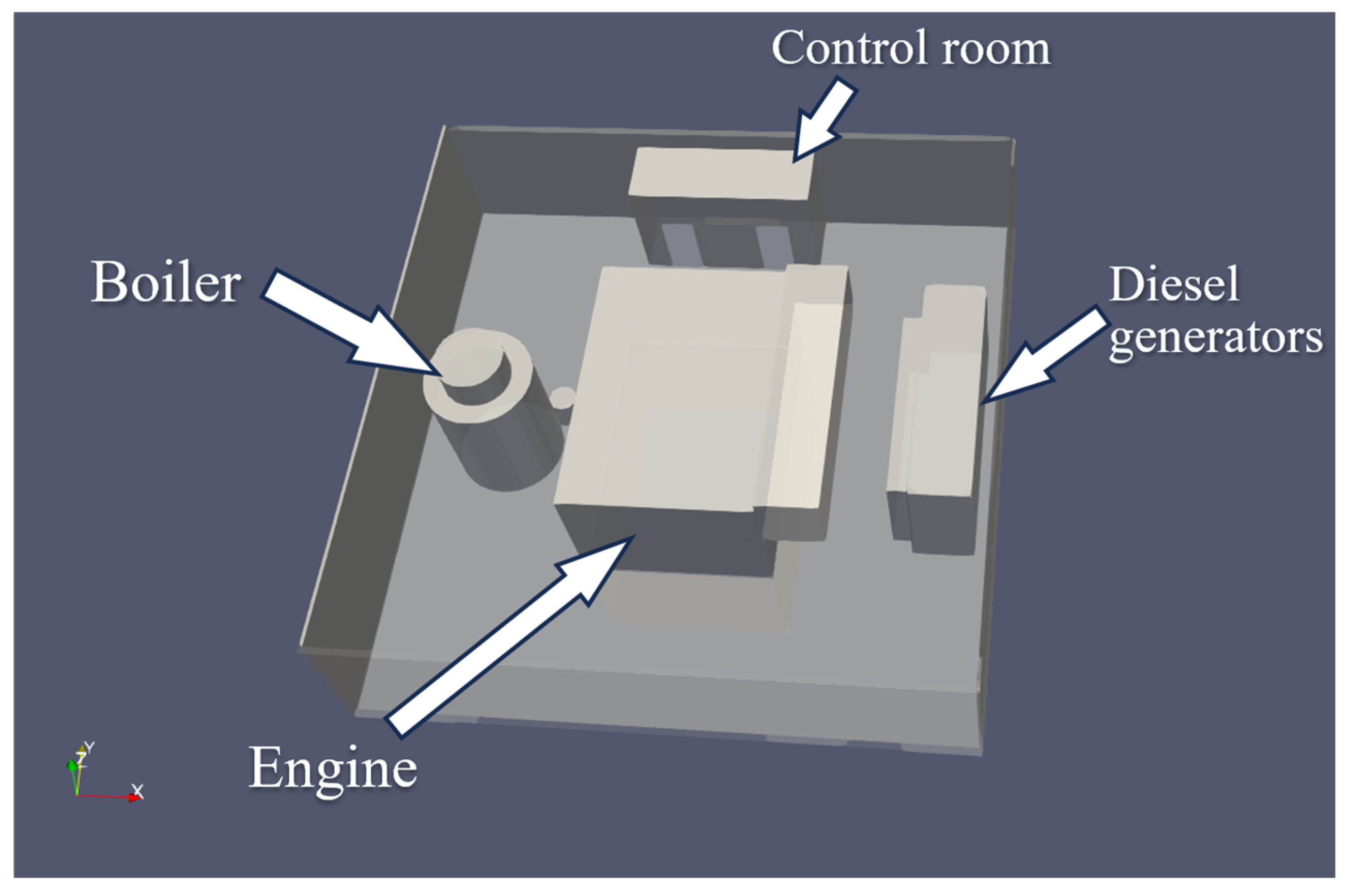
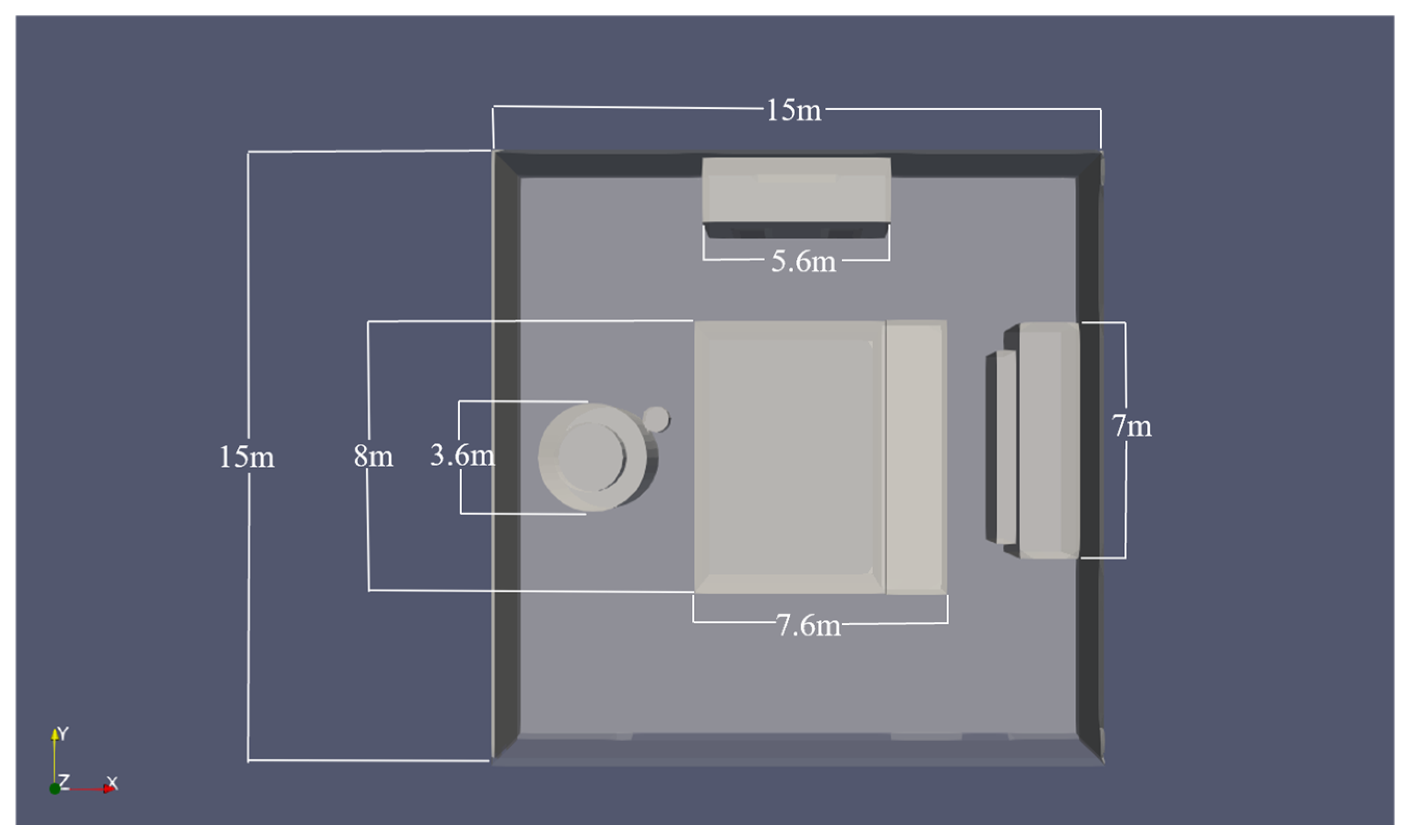
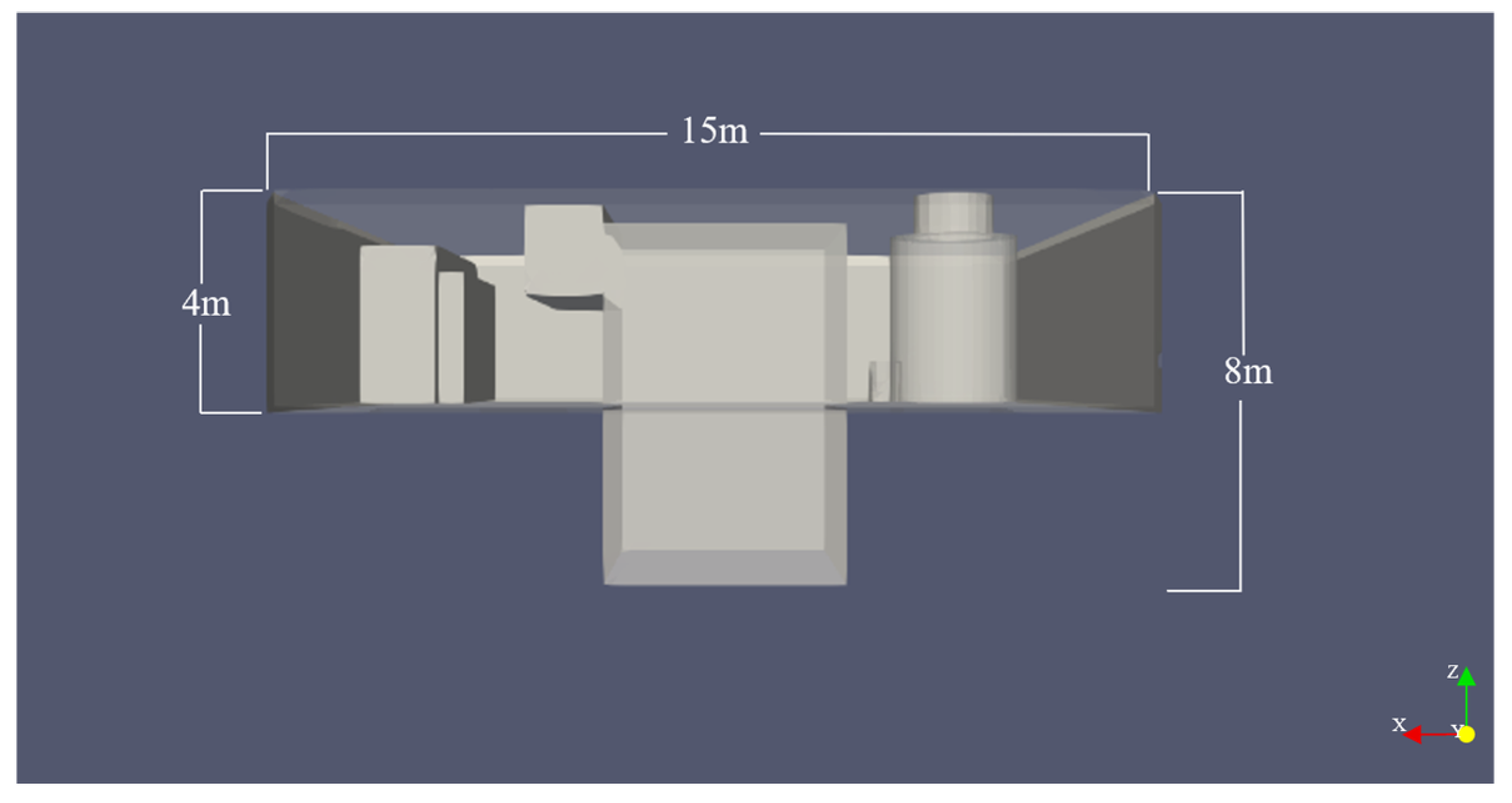
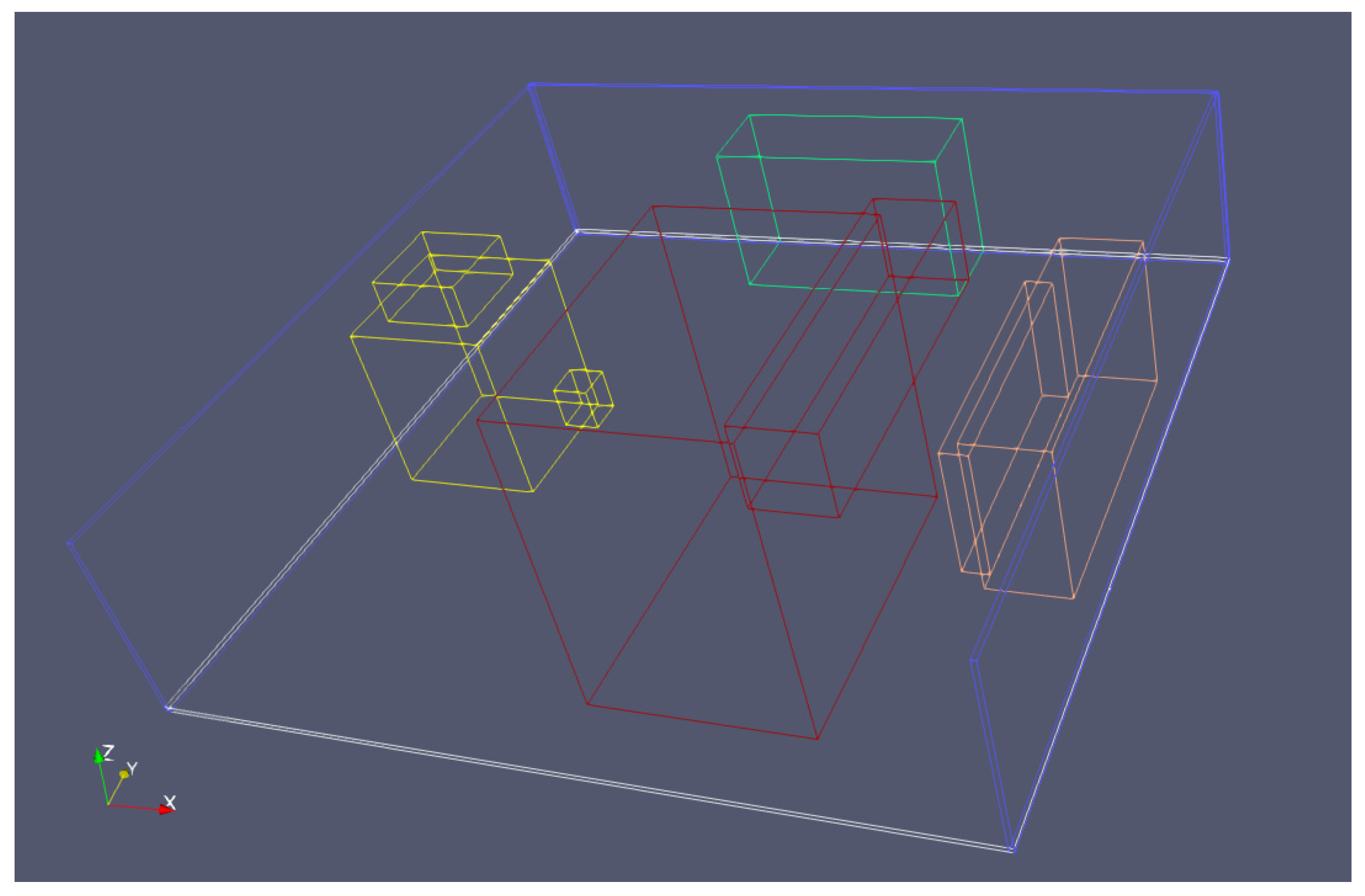
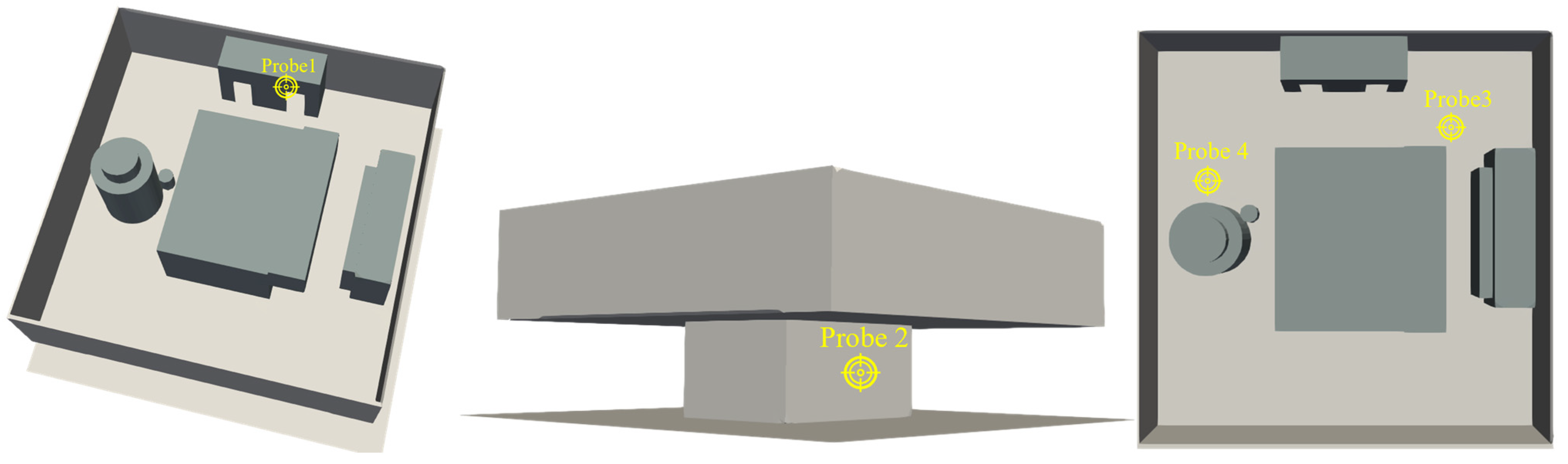












| Explosive Type | (kJ/kg) | |
|---|---|---|
| LPG | 46,100 | 10.199 |
| Naphtha | 42,800 | 9.469 |
| Diesel fuel | 42,700 | 9.447 |
| LNG | 55,500 | 12.279 |
| TNT | 4520 | 1.000 |
| Mesh Size | Points | Faces | Cells | Boundary Patches | Meshing Method |
|---|---|---|---|---|---|
| 0.15 m | 154,591 | 392,979 | 119,338 | 18 | BlockMesh; SnappyHexMesh; DynamicMesh |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miao, Z.; Zhao, Y.; Ye, B.; Chen, W. Numerical Dynamic Response Analysis of a Ship Engine Room Explosion Simulation Using OpenFOAM. J. Mar. Sci. Eng. 2025, 13, 1051. https://doi.org/10.3390/jmse13061051
Miao Z, Zhao Y, Ye B, Chen W. Numerical Dynamic Response Analysis of a Ship Engine Room Explosion Simulation Using OpenFOAM. Journal of Marine Science and Engineering. 2025; 13(6):1051. https://doi.org/10.3390/jmse13061051
Chicago/Turabian StyleMiao, Zeya, Yuechao Zhao, Baoyang Ye, and Wanzhou Chen. 2025. "Numerical Dynamic Response Analysis of a Ship Engine Room Explosion Simulation Using OpenFOAM" Journal of Marine Science and Engineering 13, no. 6: 1051. https://doi.org/10.3390/jmse13061051
APA StyleMiao, Z., Zhao, Y., Ye, B., & Chen, W. (2025). Numerical Dynamic Response Analysis of a Ship Engine Room Explosion Simulation Using OpenFOAM. Journal of Marine Science and Engineering, 13(6), 1051. https://doi.org/10.3390/jmse13061051






