The Impacts of Power Take-Off Surviving Strategies on the Extreme Load Estimations of Wave Energy Converters
Abstract
1. Introduction
2. Methodology
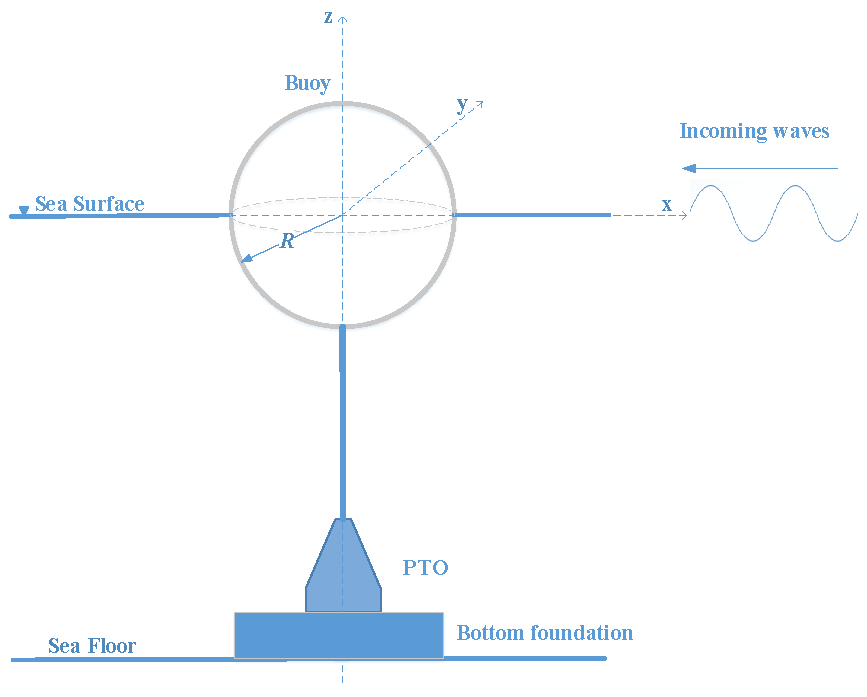
2.1. WEC Concept Description
2.2. Numerical Modeling of the WEC
2.3. PTO Parameter Settings in Surviving Conditions
2.4. Environmental Inputs
2.5. Extreme Design Load Condition Identification
3. Results and Discussion
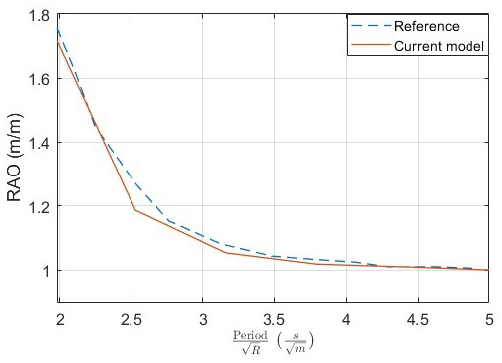
3.1. Model Verification
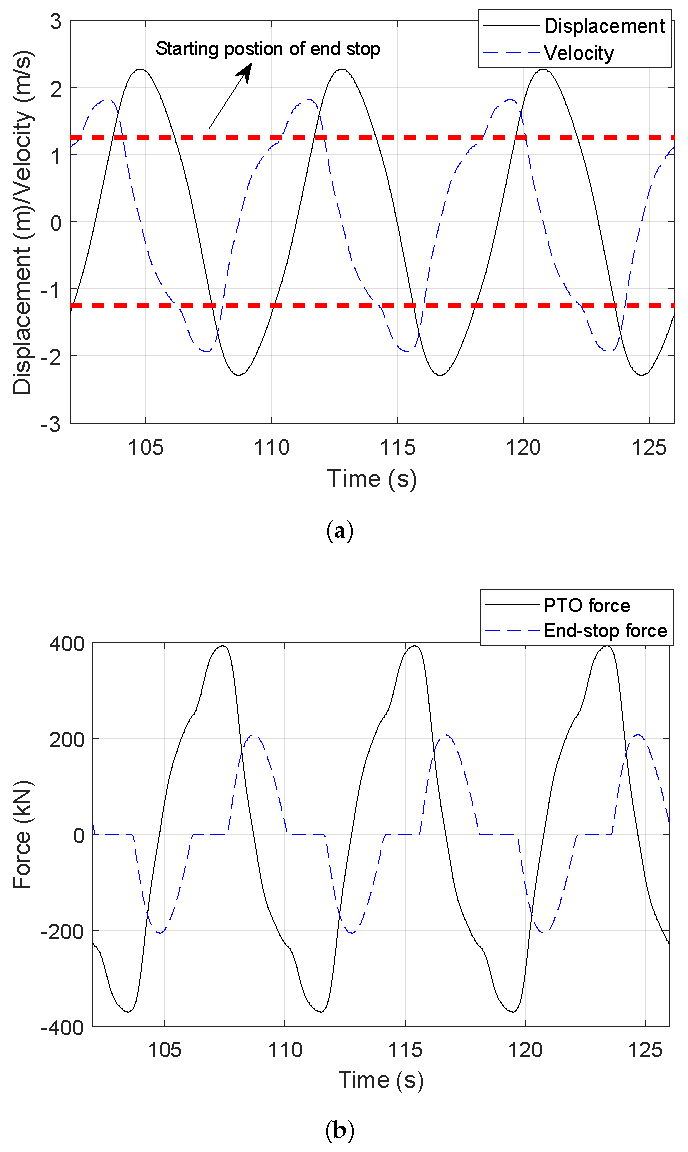
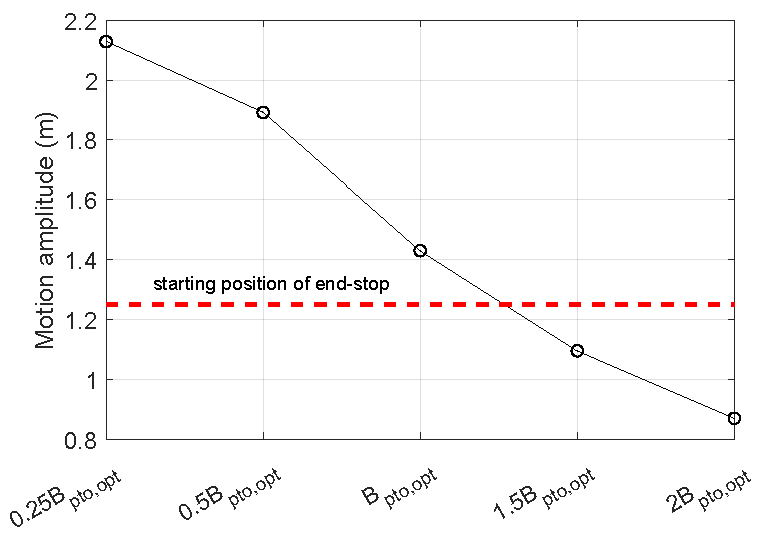
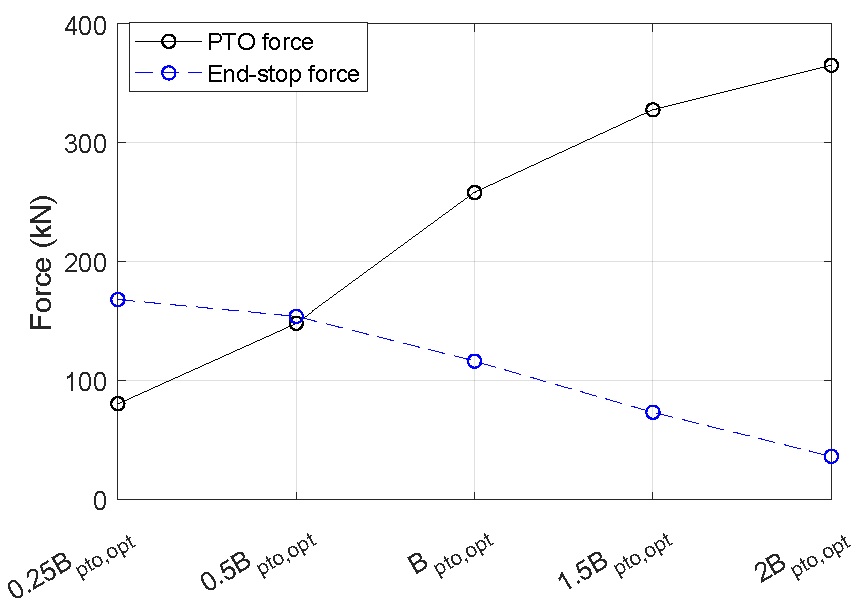
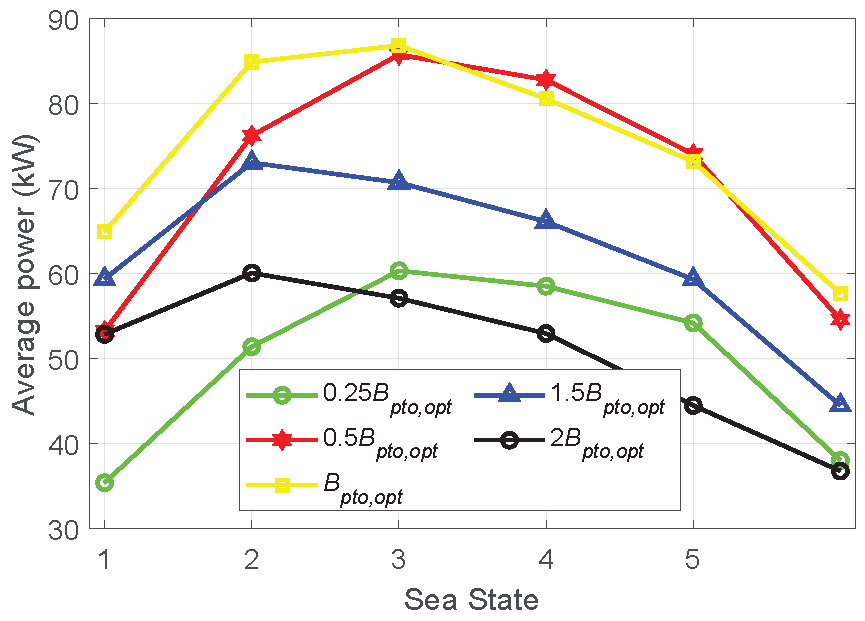
3.2. The Influence of PTO Parameters on the Loads
3.3. Extreme Load Prediction
3.4. Extreme Design Condition Identification
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature and Abbreviations
| M | Mass |
| Added mass | |
| z, and | Displacement, velocity and acceleration |
| t | Time |
| Excitation force | |
| PTO reaction force | |
| Hydrostatic force | |
| End-stop force | |
| Radiation impulse function | |
| PTO damping | |
| End-stop spring stiffness | |
| Stroke limit | |
| Optimal PTO damping | |
| Radiation damping | |
| Intrinsic reactance | |
| Hydrostatic stiffness | |
| Angular frequency | |
| CFD | Computational fluid dynamics |
| PCA | Principal component analysis |
| PTO | Power take-off |
| SPH | Smoothed particle hydrodynamics |
| WEC | Wave energy converter |
| WDRT | WEC design response toolbox |
References
- Journée, J.M.J.; Massie, W.W.; Huijsmans, R.H.M. Offshore Hydrodynamics; Delft University of Technology: Delft, The Netherlands, 2015. [Google Scholar]
- Yu, Y.H.; Van Rij, J.; Coe, R.; Lawson, M. Preliminary wave energy converters extreme load analysis. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering; American Society of Mechanical Engineers: St. John’s, NF, Canada, 2015; Volume 56574, p. V009T09A026. [Google Scholar]
- van Rij, J.; Yu, Y.H.; Guo, Y.; Coe, R.G. A wave energy converter design load case study. J. Mar. Sci. Eng. 2019, 7, 250. [Google Scholar] [CrossRef]
- Haselsteiner, A.F.; Coe, R.G.; Manuel, L.; Chai, W.; Leira, B.; Clarindo, G.; Soares, C.G.; Hannesdóttir, Á.; Dimitrov, N.; Sander, A.; et al. A benchmarking exercise for environmental contours. Ocean. Eng. 2021, 236, 109504. [Google Scholar] [CrossRef]
- Rafiee, A.; Fiévez, J. Numerical prediction of extreme loads on the CETO wave energy converter. In Proceedings of the 11th European Wave and Tidal Energy Conference, Nantes, France, 6–11 September 2015. Number 09A1-2. [Google Scholar]
- Katsidoniotaki, E.; Nilsson, E.; Rutgersson, A.; Engström, J.; Göteman, M. Response of point-absorbing wave energy conversion system in 50-years return period extreme focused waves. J. Mar. Sci. Eng. 2021, 9, 345. [Google Scholar] [CrossRef]
- Katsidoniotaki, E.; Shahroozi, Z.; Eskilsson, C.; Palm, J.; Engström, J.; Göteman, M. Validation of a CFD model for wave energy system dynamics in extreme waves. Ocean. Eng. 2023, 268, 113320. [Google Scholar] [CrossRef]
- Göteman, M.; Engström, J.; Eriksson, M.; Hann, M.; Ransley, E.; Greaves, D.; Leijon, M. Wave loads on a point-absorbing wave energy device in extreme waves. In Proceedings of the ISOPE International Ocean and Polar Engineering Conference, Kona, HI, USA, 21–26 June 2015; ISOPE: Mountain View, CA, USA, 2015; p. ISOPE–I. [Google Scholar]
- Parmeggiani, S.; Kofoed, J.P.; Friis-Madsen, E. Extreme loads on the mooring lines and survivability mode for the wave dragon wave energy converter. In Proceedings of the World Renewable Energy Congress 2011, Linköping, Sweden, 8–11 May 2011; Linköping University: Linköping, Sweden, 2011. [Google Scholar]
- Marrone, S.; Colagrossi, A.; Baudry, V.; Le Touzé, D. Extreme wave impacts on a wave energy converter: Load prediction through a SPH model. Coast. Eng. J. 2019, 61, 63–77. [Google Scholar] [CrossRef]
- Sirigu, S.A.; Bonfanti, M.; Begovic, E.; Bertorello, C.; Dafnakis, P.; Giorgi, G.; Bracco, G.; Mattiazzo, G. Experimental investigation of the mooring system of a wave energy converter in operating and extreme wave conditions. J. Mar. Sci. Eng. 2020, 8, 180. [Google Scholar] [CrossRef]
- Nguyen, P.T.; Manuel, L.; Coe, R.G. On the development of an efficient surrogate model for predicting long-term extreme loads on a wave energy converter. J. Offshore Mech. Arct. Eng. 2019, 141, 061103. [Google Scholar] [CrossRef]
- Tan, J.; Polinder, H.; Laguna, A.J.; Wellens, P.; Miedema, S.A. The Influence of Sizing of Wave Energy Converters on the Techno-Economic Performance. J. Mar. Sci. Eng. 2021, 9, 52. [Google Scholar] [CrossRef]
- Pecher, A. Handbook of Ocean Wave Energy; Springer Nature: Cham, Switzerland, 2017; Volume 7. [Google Scholar] [CrossRef]
- Tan, J.; Polinder, H.; Wellens, P.; Miedema, S. A feasibility study on downsizing of power take off system of wave energy converters. In Developments in Renewable Energies Offshore; CRC Press: Boca Raton, FL, USA, 2020; pp. 140–148. [Google Scholar]
- Ringwood, J.V.; Bacelli, G. Numerical optimal control of wave energy converters. IEEE Trans. Sust. Eng. 2014, 6, 294–302. [Google Scholar]
- Bacelli, G.; Coe, R.G. Comments on control of wave energy converters. IEEE Trans. Control Syst. Technol. 2020, 29, 478–481. [Google Scholar] [CrossRef]
- Said, H.A.; García-Violini, D.; Ringwood, J.V. Wave-to-grid (W2G) control of a wave energy converter. Energy Convers. Manag. X 2022, 14, 100190. [Google Scholar] [CrossRef]
- Windt, C.; Faedo, N.; Penalba, M.; Dias, F.; Ringwood, J.V. Reactive control of wave energy devices—The modelling paradox. Appl. Ocean. Res. 2021, 109, 102574. [Google Scholar] [CrossRef]
- Prado, M.; Polinder, H. Direct Drive Wave Energy Conversion Systems: An Introduction; Woodhead Publishing Limited: Sawston, UK, 2013; pp. 175–194. [Google Scholar] [CrossRef]
- Tan, J.; Wang, X.; Polinder, H.; Laguna, A.J.; Miedema, S.A. Downsizing the linear PM generator in wave energy conversion for improved economic feasibility. J. Mar. Sci. Eng. 2022, 10, 1316. [Google Scholar] [CrossRef]
- Tan, J.; Wang, X.; Jarquin Laguna, A.; Polinder, H.; Miedema, S. The Influence of Linear Permanent Magnet Generator Sizing on the Techno-Economic Performance of a Wave Energy Converter. In Proceedings of the 2021 13th International Symposium on Linear Drives for Industry Applications (LDIA), Wuhan, China, 1–3 July 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Yang, B.; Duan, J.; Chen, Y.; Wu, S.; Li, M.; Cao, P.; Jiang, L. A critical survey of power take-off systems based wave energy converters: Summaries, advances, and perspectives. Ocean. Eng. 2024, 298, 117149. [Google Scholar] [CrossRef]
- Bouhrim, H.; El Marjani, A.; Nechad, R.; Hajjout, I. Ocean Wave Energy Conversion: A Review. J. Mar. Sci. Eng. 2024, 12, 1922. [Google Scholar] [CrossRef]
- Tan, J.; Polinder, H.; Laguna, A.J.; Miedema, S. The application of the spectral domain modeling to the power take-off sizing of heaving wave energy converters. Appl. Ocean. Res. 2022, 122, 103110. [Google Scholar] [CrossRef]
- Silva, L.; Sergiienko, N.; Pesce, C.; Ding, B.; Cazzolato, B.; Morishita, H. Stochastic analysis of nonlinear wave energy converters via statistical linearization. Appl. Ocean. Res. 2020, 95, 102023. [Google Scholar] [CrossRef]
- Tan, J.; Tao, W.; Laguna, A.J.; Polinder, H.; Xing, Y.; Miedema, S. A spectral-domain wave-to-wire model of wave energy converters. Appl. Ocean. Res. 2023, 138, 103650. [Google Scholar] [CrossRef]
- Tan, J.; Laguna, A.J. Spectral-Domain Modelling of Wave Energy Converters as an Efficient Tool for Adjustment of PTO Model Parameters. In Proceedings of the European Wave and Tidal Energy Conference, Bilbao, Spain, 3–7 September 2023; Volume 15. [Google Scholar]
- Babarit, A.; Hals, J.; Muliawan, M.J.; Kurniawan, A.; Moan, T.; Krokstad, J. Numerical benchmarking study of a selection of wave energy converters. Renew. Energy 2012, 41, 44–63. [Google Scholar] [CrossRef]
- Lawson, M.; Yu, Y.H.; Ruehl, K.; Michelen, C. Development and Demonstration of the WEC-Sim Wave Energy Converter Simulation Tool. In Proceedings of the 2nd Marine Energy Technology Symposium, Seattle, WA, USA, 15–18 April 2014. [Google Scholar]
- Lawson, M.; Yu, Y.H.; Nelessen, A.; Ruehl, K.; Michelen, C. Implementing nonlinear buoyancy and excitation forces in the wec-sim wave energy converter modeling tool. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, San Francisco, CA, USA, 8–13 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014; Volume 45547, p. V09BT09A043. [Google Scholar]
- Cummins, W.; Iiuhl, W.; Uinm, A. The Impulse Response Function and Ship Motions; Massachusetts Institute of Technology: Cambridge, MA, USA, 1962. [Google Scholar]
- Penalba, M.; Kelly, T.; Ringwood, J. Using NEMOH for Modelling Wave Energy Converters: A Comparative Study with WAMIT. In Proceedings of the 12th European Wave and Tidal Energy Conference, Cork, Ireland, 27 August–1 September 2017; p. 10. [Google Scholar]
- Andersson, K. Application of the open source code Nemoh for modelling of added mass and damping in ship motion simulations. In Shipping and the Environment: Improving Environmental Performance in Marine Transportation; KTH Royal Institute of Technology: Stockholm, Sweden, 2018; pp. vii–viii. [Google Scholar]
- Giorgi, G.; Ringwood, J.V. Nonlinear Froude-Krylov and viscous drag representations for wave energy converters in the computation/fidelity continuum. Ocean. Eng. 2017, 141, 164–175. [Google Scholar] [CrossRef]
- Hals, J.; Falnes, J.; Moan, T. Constrained Optimal Control of a Heaving Buoy Wave-Energy Converter. J. Offshore Mech. Arct. Eng. 2010, 133, 011401. [Google Scholar] [CrossRef]
- Coe, R.G.; Michelen, C.; Eckert-Gallup, A.; Martin, N.; Yu, Y.H.; van Rij, J.; Quon, E.W.; Manuel, L.; Nguyen, P.; Esterly, T.; et al. WEC Design Response Toolbox (WDRT). Available online: http://wec-sim.github.io/WDRT (accessed on 15 May 2025).
- Lin, Z.; Liu, X.; Lotfian, S. Impacts of water depth increase on offshore floating wind turbine dynamics. Ocean. Eng. 2021, 224, 108697. [Google Scholar] [CrossRef]
- Campanile, A.; Piscopo, V.; Scamardella, A. Mooring design and selection for floating offshore wind turbines on intermediate and deep water depths. Ocean. Eng. 2018, 148, 349–360. [Google Scholar] [CrossRef]
- Huang, W.H.; Yang, R.Y. Water depth variation influence on the mooring line design for FOWT within shallow water region. J. Mar. Sci. Eng. 2021, 9, 409. [Google Scholar] [CrossRef]
- Coe, R.G.; Michelen, C. Comparison of Methods for Estimating Short-Term Extreme Response of Wave Energy Converters; Technical report; Sandia National Lab. (SNL-NM): Albuquerque, NM, USA, 2015. [Google Scholar]
- Nielsen, K. OES TASK 10-Numerical Modelling of Wave Energy Converters; 2024. IEA OES. Available online: http://www.ocean-energy-systems.org/ (accessed on 19 May 2025).
- Sheng, W.; Alcorn, R.; Lewis, T. Physical modelling of wave energy converters. Ocean. Eng. 2014, 84, 29–36. [Google Scholar] [CrossRef]
- Flinchum, M.D. Investigation of End-Stop Motion Constraint for a Wave Energy Converter; Universidade Federal do Rio de Janeiro: Rio de Janeiro, Brazil, 2018. [Google Scholar]
- Liu, Z.; Zhang, R.; Xiao, H.; Wang, X. Survey of the mechanisms of power take-off (PTO) devices of wave energy converters. Acta Mech. Sin. 2020, 36, 644–658. [Google Scholar] [CrossRef]
- Ransley, E.; Greaves, D.; Raby, A.; Simmonds, D.; Hann, M. Survivability of wave energy converters using CFD. Renew. Energy 2017, 109, 235–247. [Google Scholar] [CrossRef]
- Shahroozi, Z.; Göteman, M.; Engström, J. Experimental investigation of a point-absorber wave energy converter response in different wave-type representations of extreme sea states. Ocean Eng. 2022, 248, 110693. [Google Scholar] [CrossRef]
- Van Rij, J.; Yu, Y.H.; Coe, R.G. Design load analysis for wave energy converters. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering; American Society of Mechanical Engineers: New York, NY, USA, 2018; Volume 51319, p. V010T09A031. [Google Scholar]

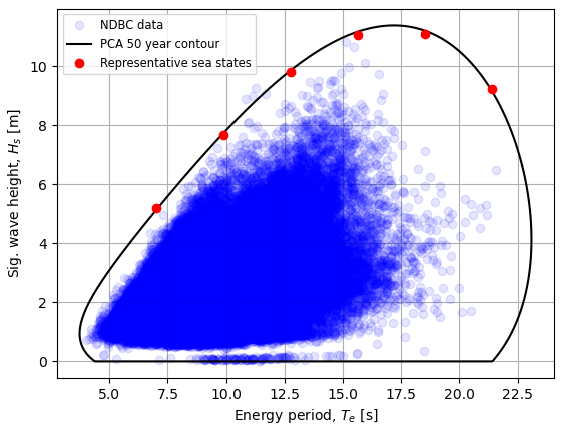


| Sea States | Sig. Wave Height (m) | Energy Period (s) |
|---|---|---|
| Sea State 1 | 5.21 | 7.00 |
| Sea State 2 | 7.66 | 9.88 |
| Sea State 3 | 9.68 | 12.76 |
| Sea State 4 | 10.94 | 15.64 |
| Sea State 5 | 11.04 | 18.52 |
| Sea State 6 | 9.23 | 21.40 |
| PTO Parameter | PTO Extreme Condition | End-Stop Extreme Condition |
|---|---|---|
| Sea State 5 | Sea State 6 | |
| Sea State 4 | Sea State 2 | |
| Sea State 3 | Sea State 6 | |
| Sea State 4 | Sea State 5 | |
| Sea State 4 | Sea State 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, J.; Zhang, Y.; Jain, A.; Lavidas, G. The Impacts of Power Take-Off Surviving Strategies on the Extreme Load Estimations of Wave Energy Converters. J. Mar. Sci. Eng. 2025, 13, 994. https://doi.org/10.3390/jmse13050994
Tan J, Zhang Y, Jain A, Lavidas G. The Impacts of Power Take-Off Surviving Strategies on the Extreme Load Estimations of Wave Energy Converters. Journal of Marine Science and Engineering. 2025; 13(5):994. https://doi.org/10.3390/jmse13050994
Chicago/Turabian StyleTan, Jian, Yufeng Zhang, Avni Jain, and George Lavidas. 2025. "The Impacts of Power Take-Off Surviving Strategies on the Extreme Load Estimations of Wave Energy Converters" Journal of Marine Science and Engineering 13, no. 5: 994. https://doi.org/10.3390/jmse13050994
APA StyleTan, J., Zhang, Y., Jain, A., & Lavidas, G. (2025). The Impacts of Power Take-Off Surviving Strategies on the Extreme Load Estimations of Wave Energy Converters. Journal of Marine Science and Engineering, 13(5), 994. https://doi.org/10.3390/jmse13050994







