Effect of Blade Number on Tip Vortex Cavitation of Propeller
Abstract
1. Introduction
2. Mathematical Model
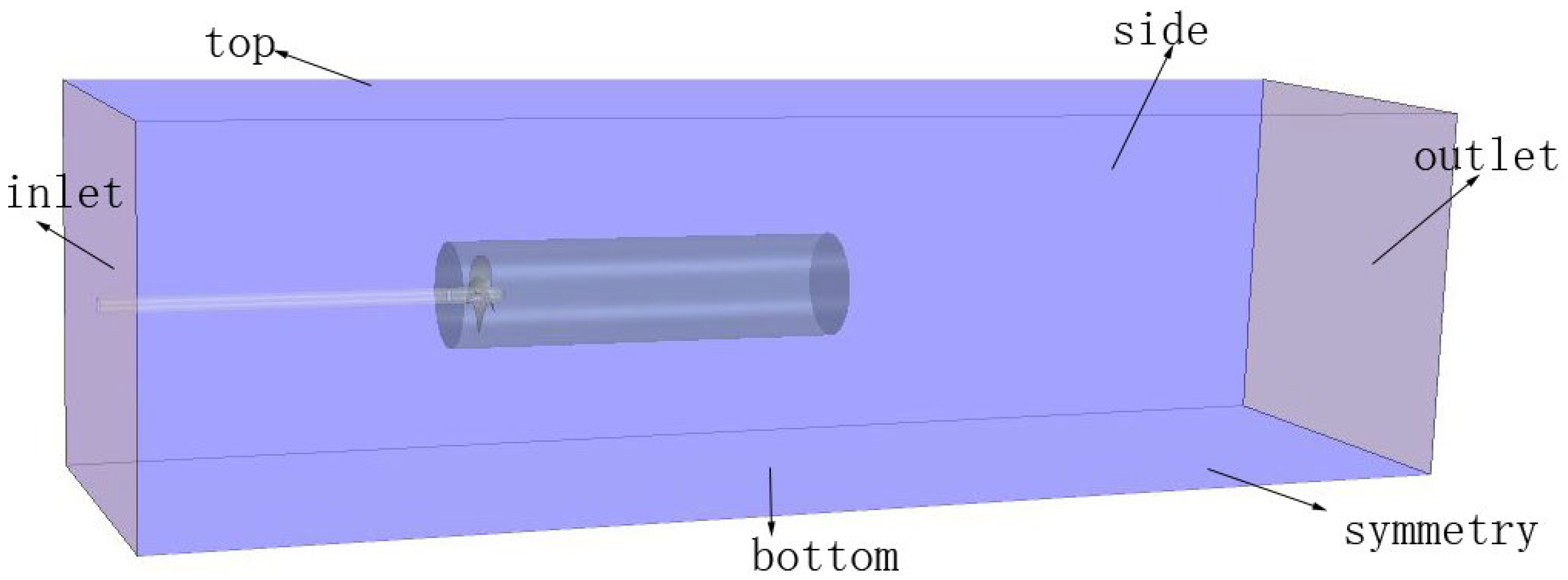
3. Computation Domain and Boundary Condition
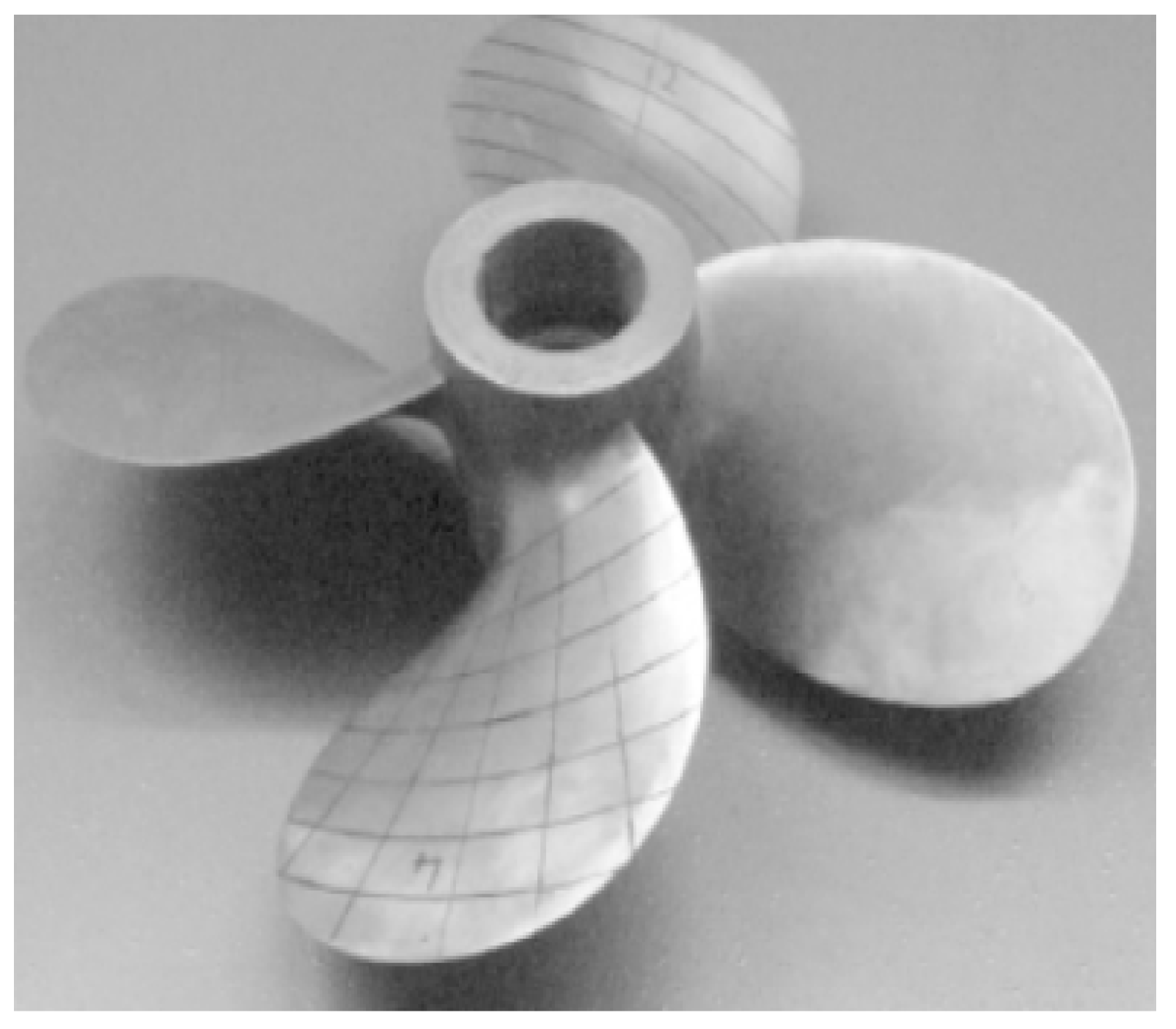
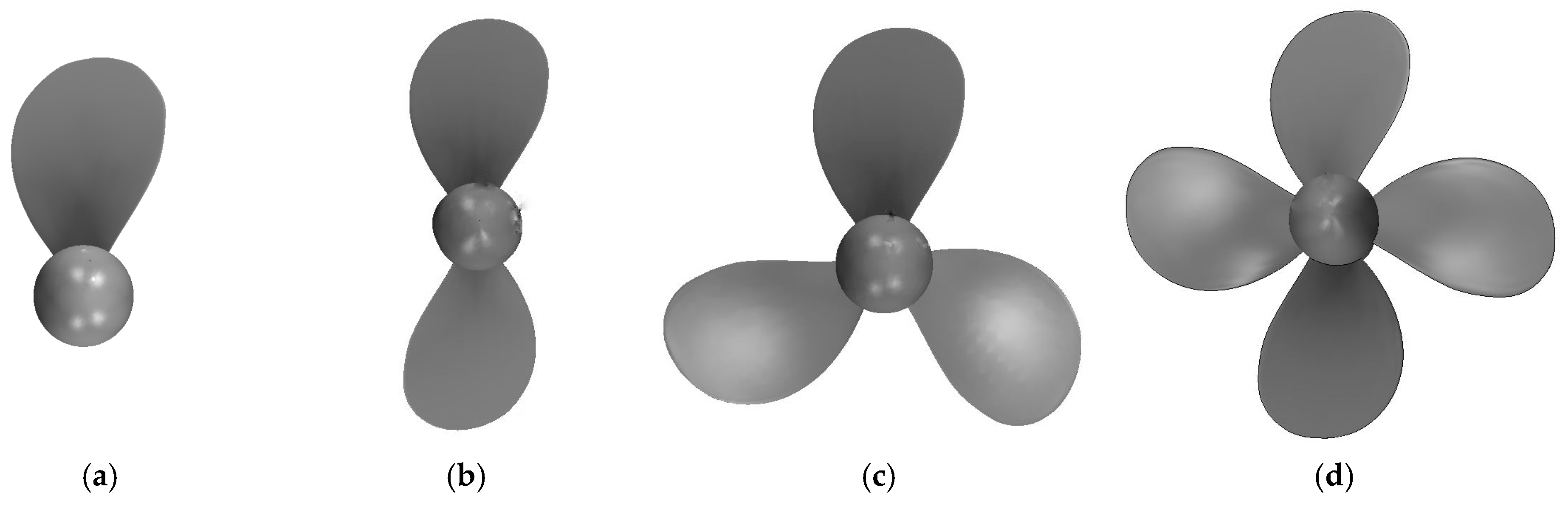
3.1. Propeller Parameters
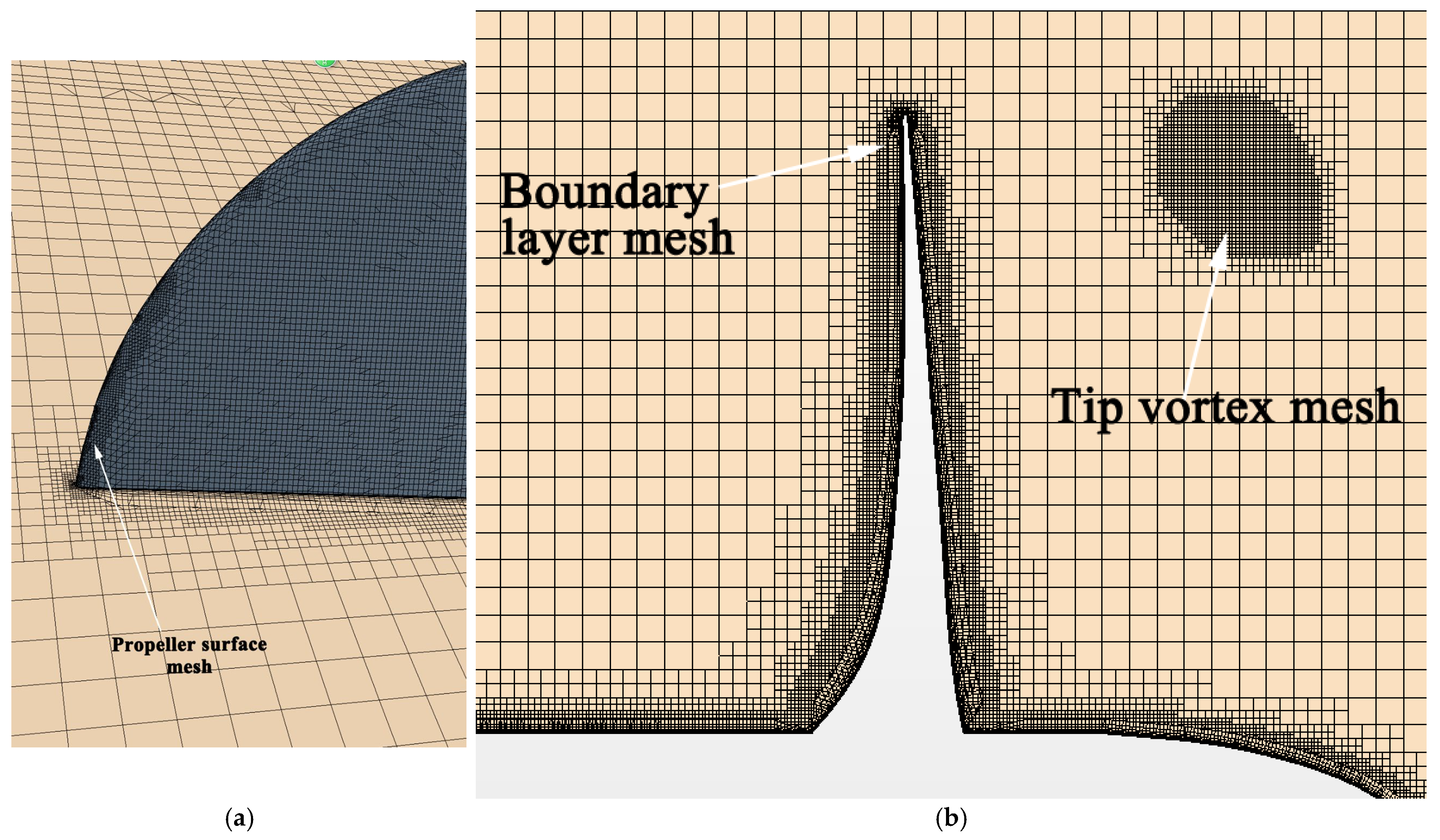
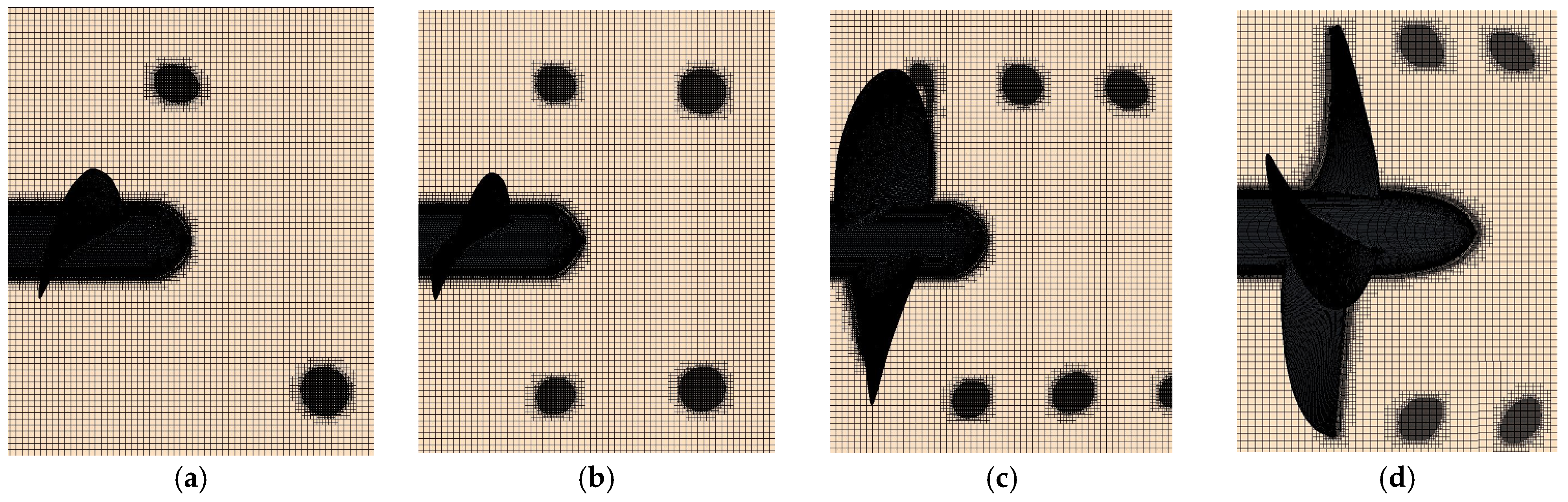
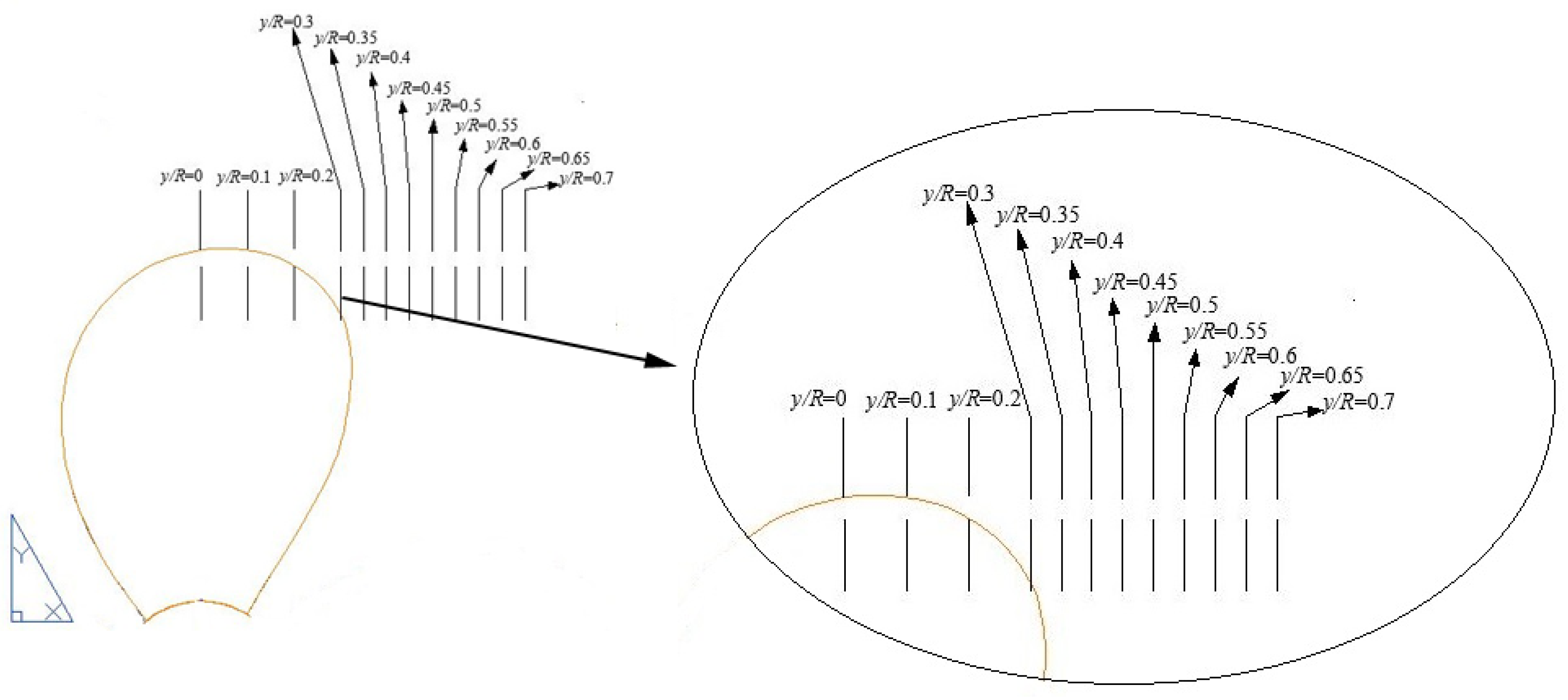
3.2. Meshing and Boundary Conditions
4. Results and Discussion
4.1. Hydrodynamic Performance
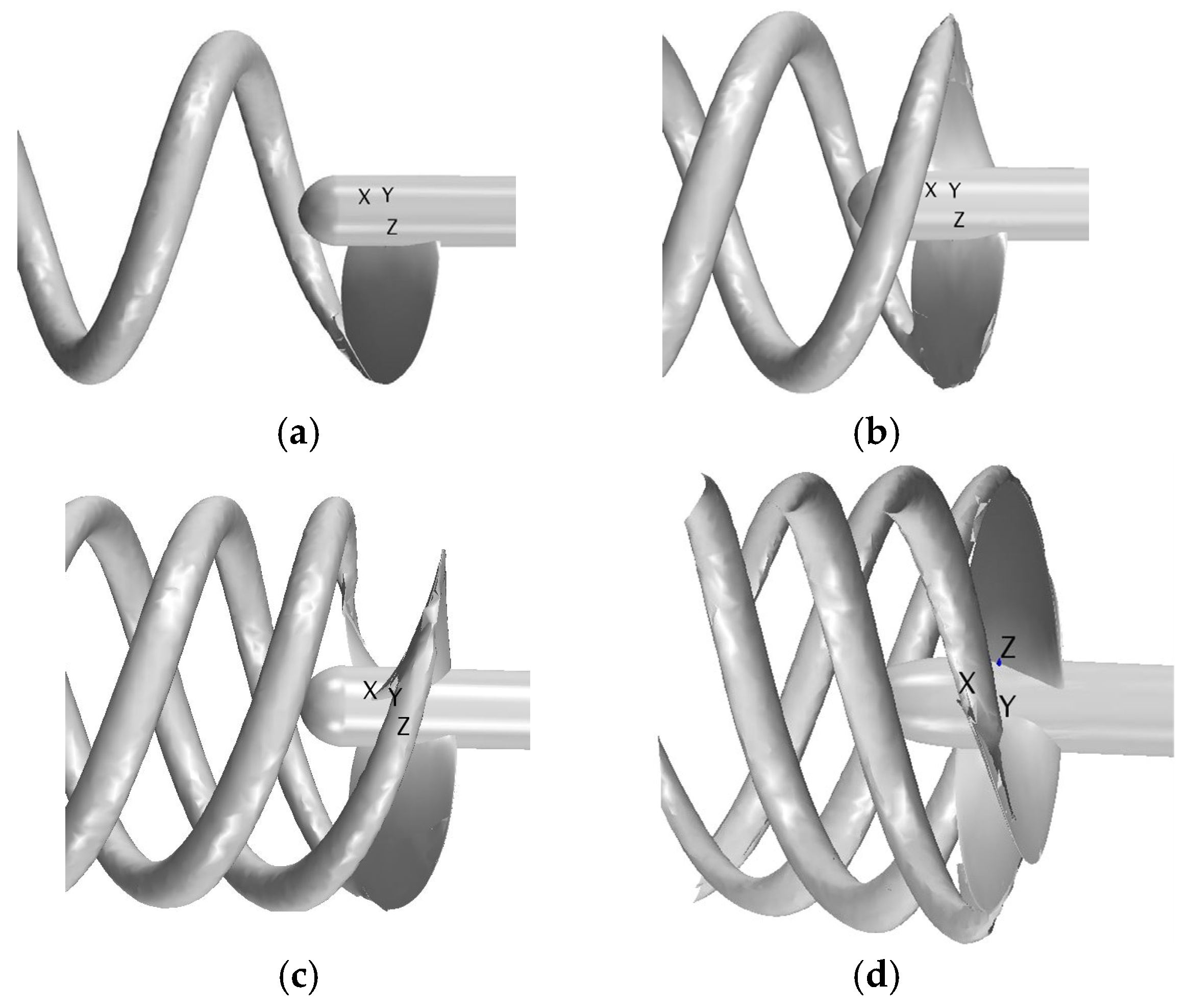
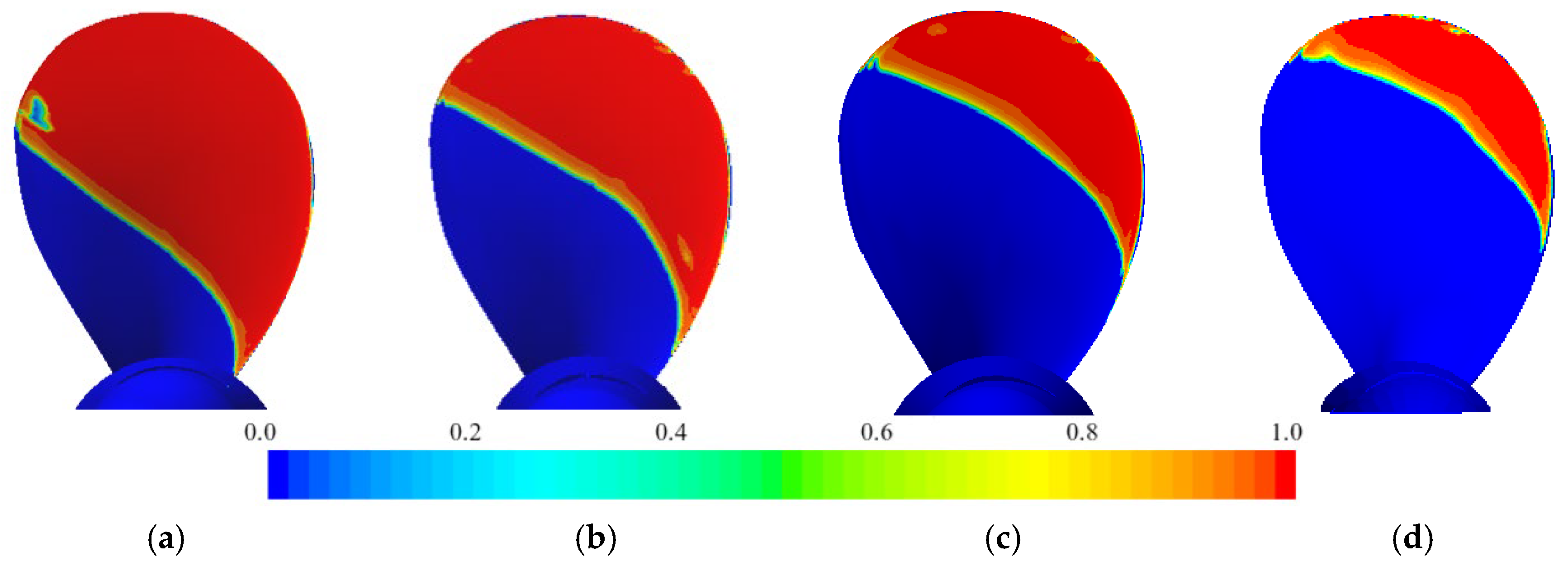
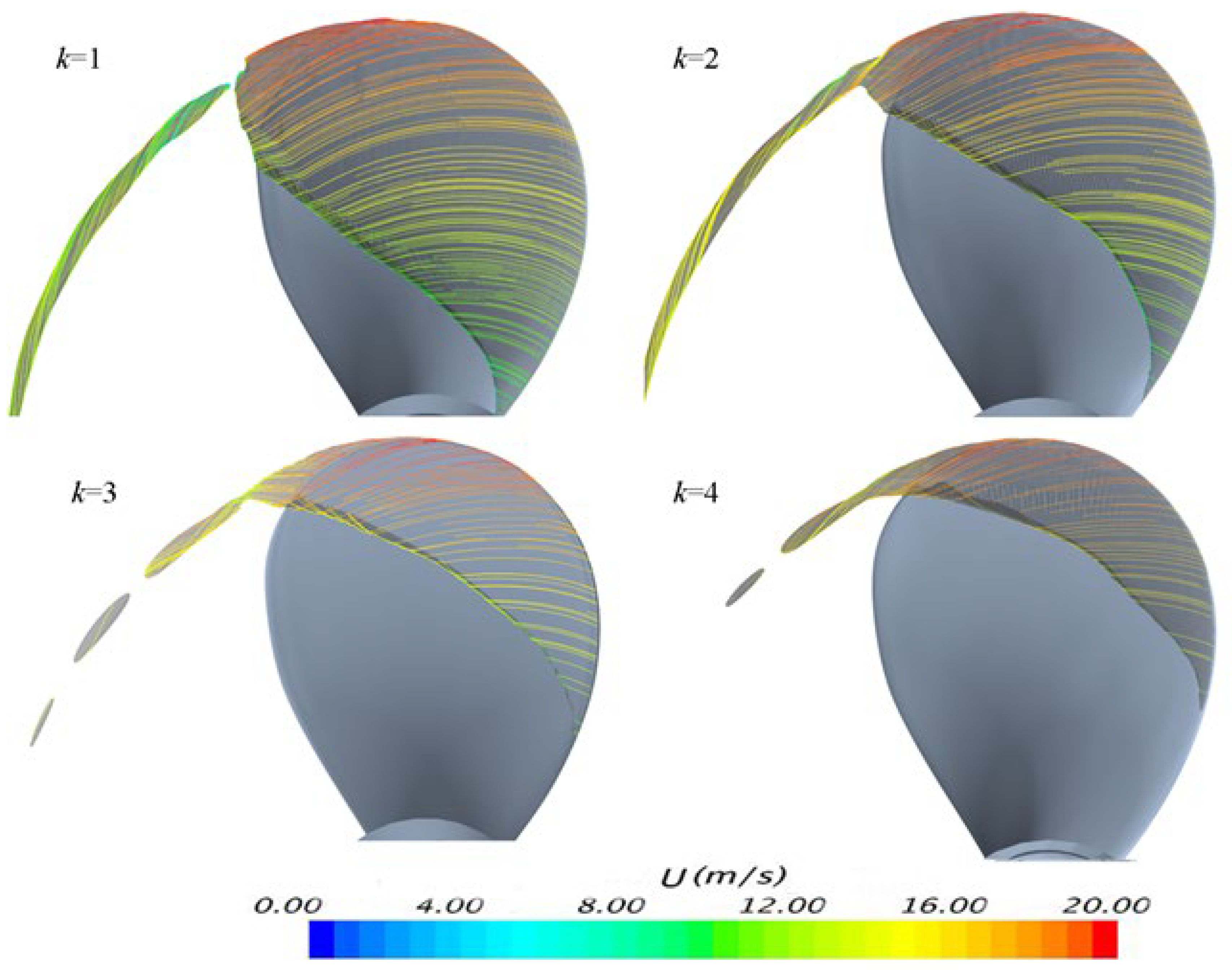
4.2. Cavitation Pattern
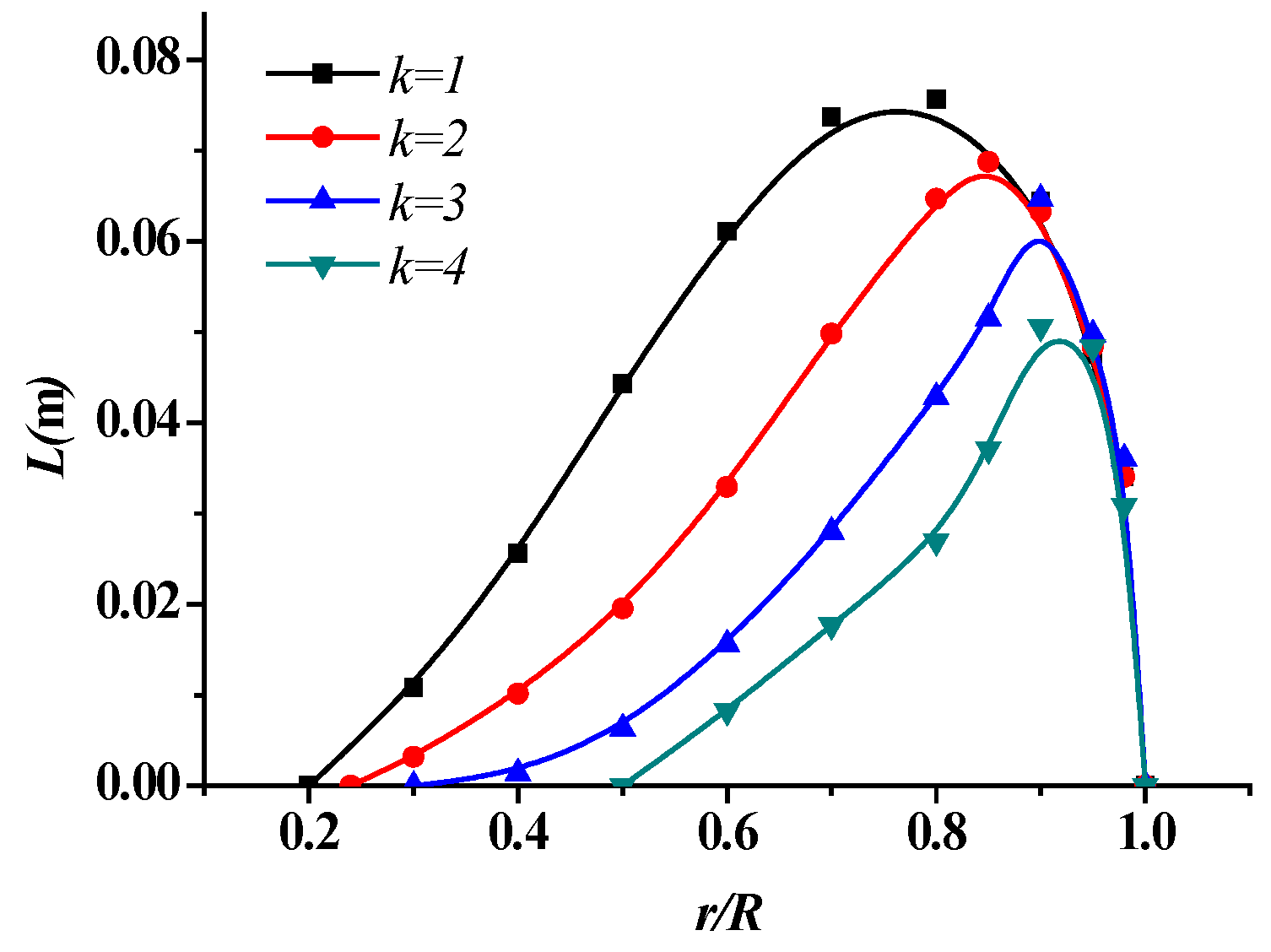
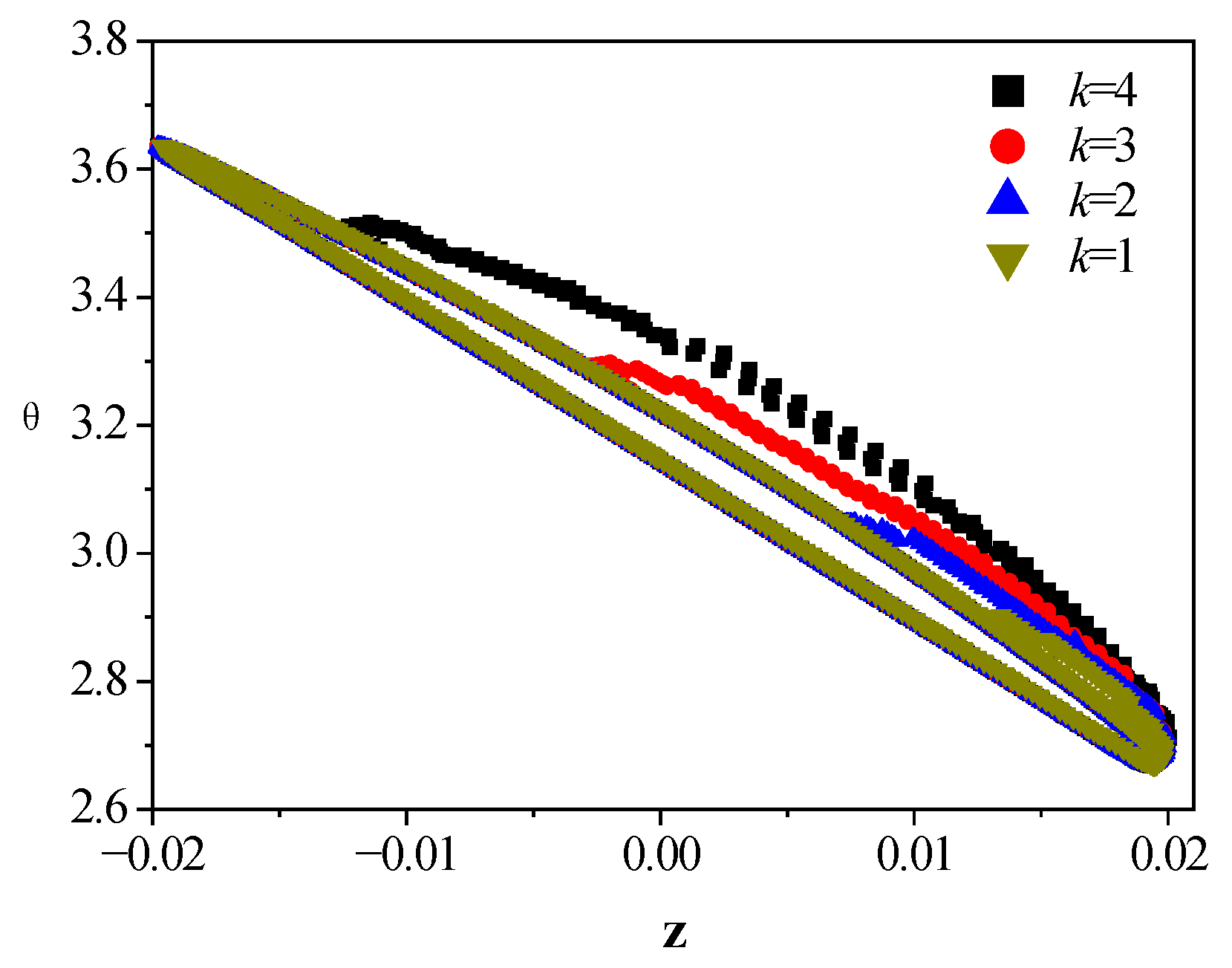
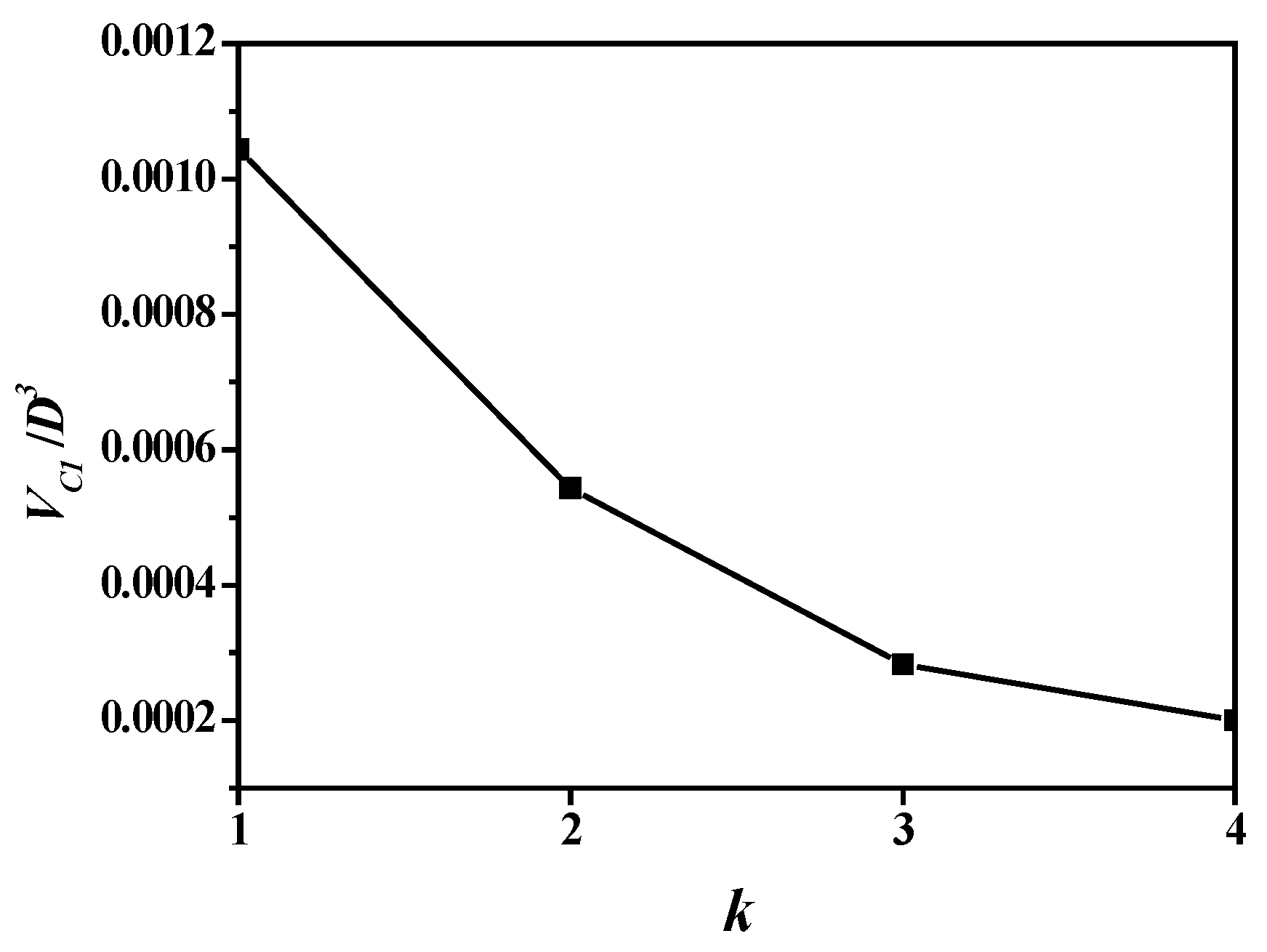
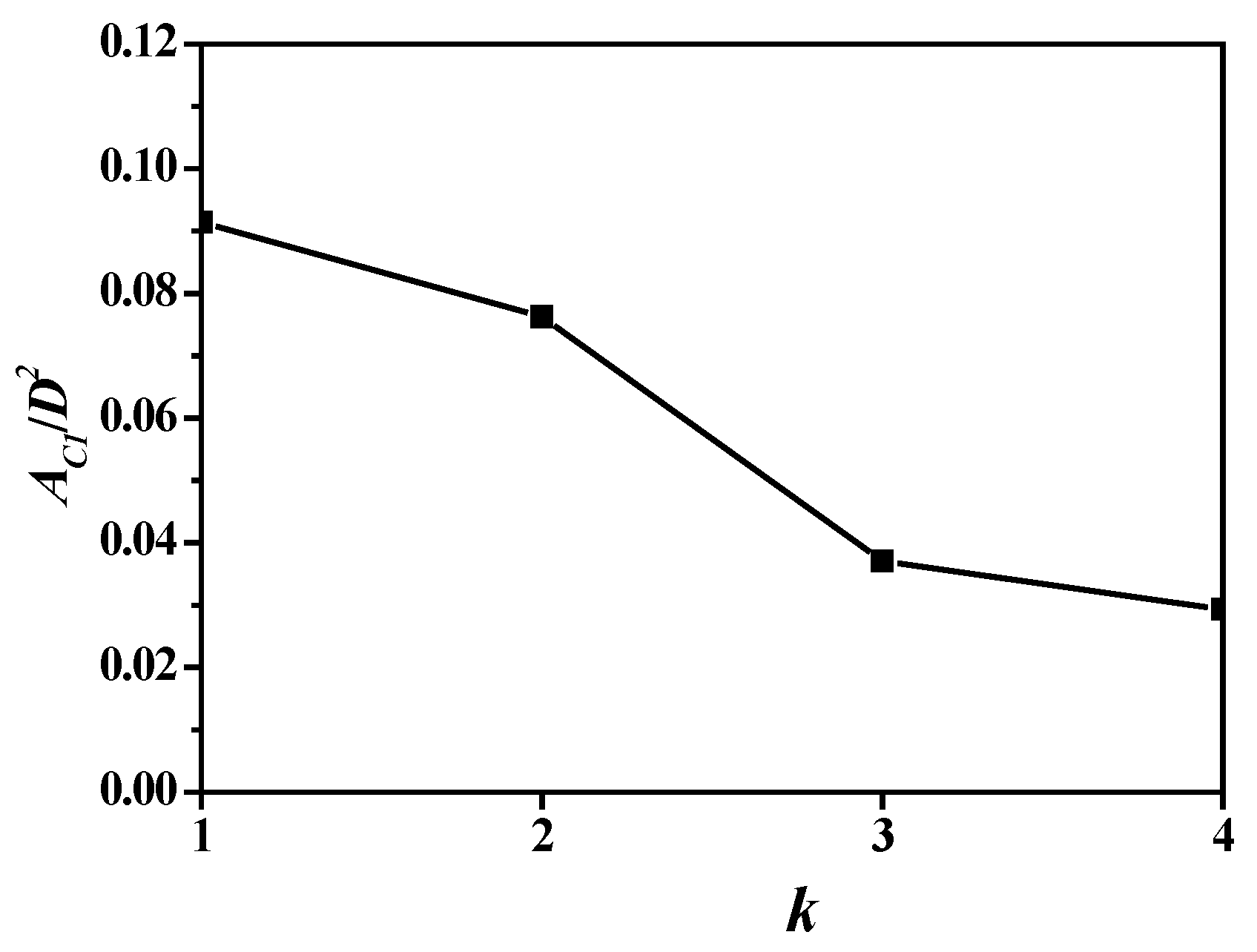
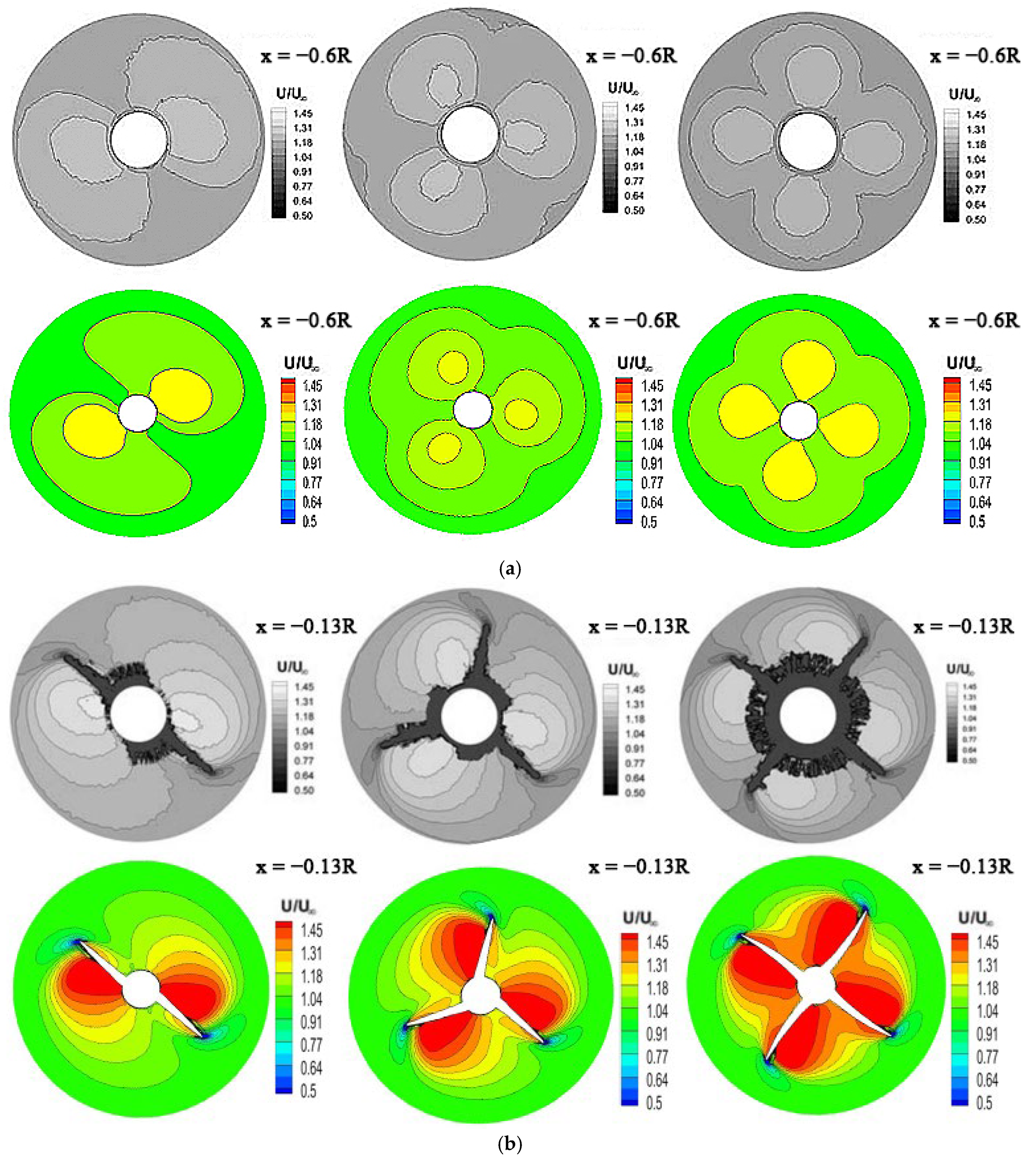
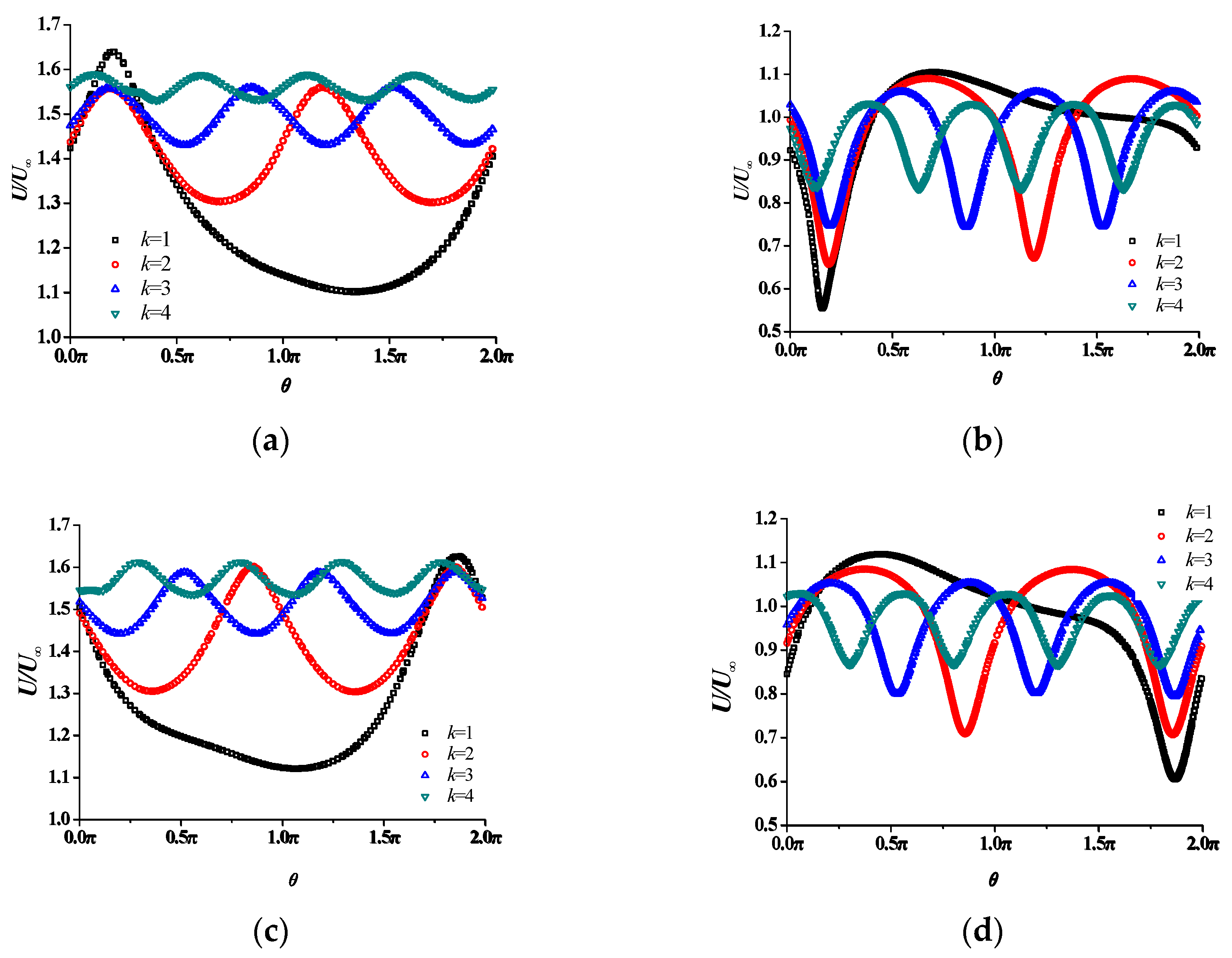
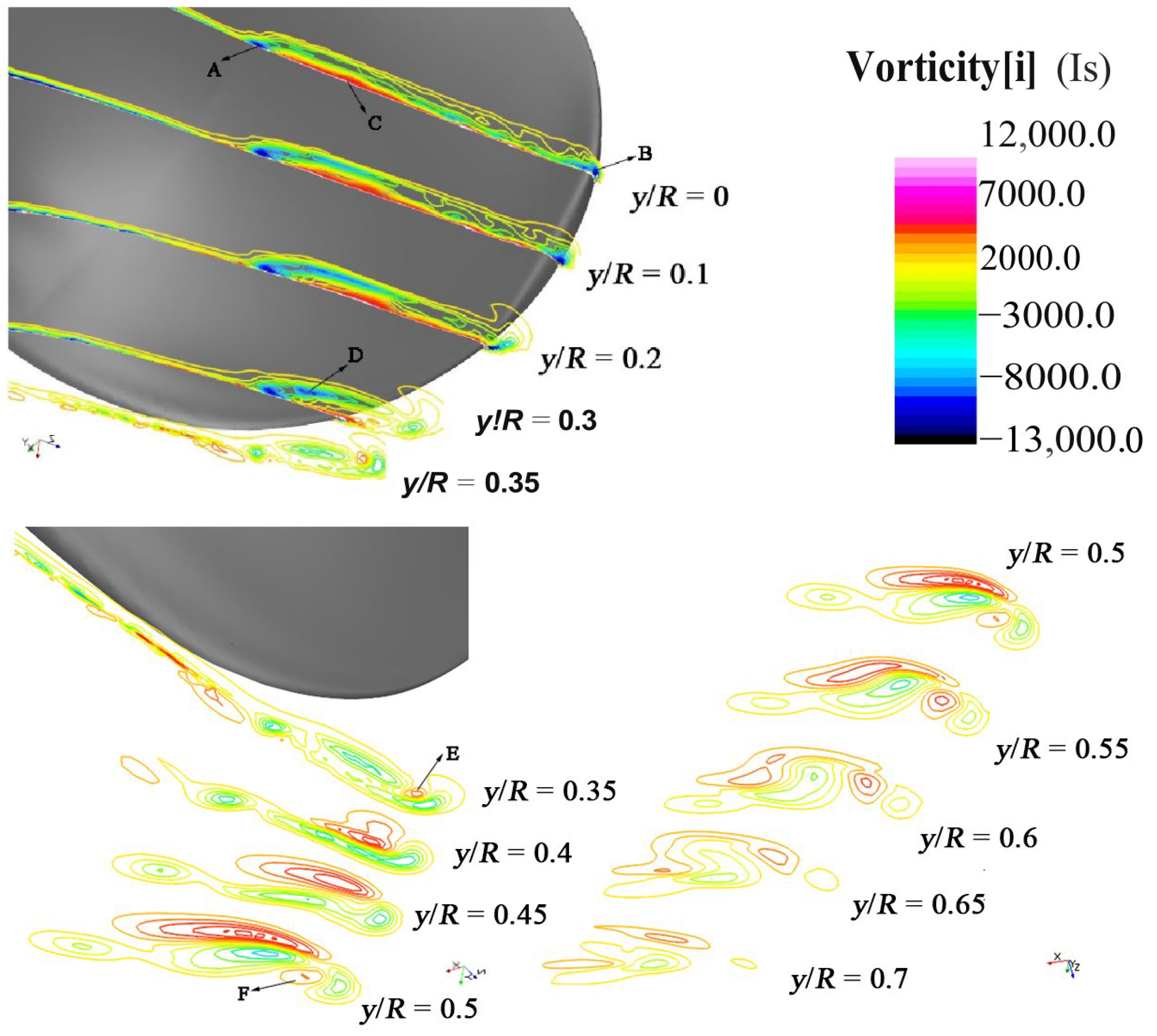
4.3. Flow-Field Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huse, E. Pressure Fluctuations on the Hull Induced by Cavitating Propellers; Technical Report 111, Norwegian Ship Model Experiment Tank; Trondheim University: Trondheim, Norway, 1972. [Google Scholar]
- Kinnas, S.A.; Hsin, C.-Y. Boundary element method for the analysis of the unsteady flow around extreme propeller geometries. AIAA J. 1992, 30, 688–696. [Google Scholar] [CrossRef]
- Fine, N.E.; Kinnas, S.A. A boundary element method for the analysis of the flow around 3-D cavitating hydrofoils. J. Ship Res. 1993, 37, 213–224. [Google Scholar] [CrossRef]
- Lee, H. Modeling of Unsteady Wake Alignment and Developed Tip Vortex Cavitation. Doctoral Dissertation, University of Texas at Austin, Austin, TX, USA, August 2002. [Google Scholar]
- Sharma, A. Numerical Modeling of a Hydrofoil or a Marine Propeller Undergoing Unsteady Motion via a Panel Method and RANS. Doctoral Dissertation, University of Texas at Austin, Austin, TX, USA, June 2011. [Google Scholar]
- Chang, Y.C.; Hu, C.N.; Tu, J.C.; Chow, Y.C. Experimental investigation and numerical prediction of cavitation incurred on propeller surfaces. J. Hydrodyn. 2010, 22, 722–727. [Google Scholar] [CrossRef]
- Sato, K.; Ohshima, A.; Egashira, H.; Takano, S. Numerical Prediction of Cavitation and Pressure Fluctuation Around Marine Propeller. In Proceedings of the 7th International Symposium on Cavitation, Ann Arbor, MI, USA, 16–20 August 2009. [Google Scholar]
- Feng, X.; Lu, J. Effects of balanced skew and biased skew on the cavitation characteristics and pressure fluctuations of the marine propeller. Ocean Eng. 2019, 184, 184–192. [Google Scholar] [CrossRef]
- Hu, J.; Zhang, W.; Wang, C.; Sun, S.; Guo, C. Impact of skew on propeller tip vortex cavitation. Ocean Eng. 2021, 220, 108479. [Google Scholar] [CrossRef]
- Jeong, S.; Hong, S.; Song, J.; Kwon, H.; Seol, H. Numerical method to determine the cavitation inception speed of a submarine propeller based on the noise obtained from bubble dynamics. Ocean Eng. 2021, 245, 110464. [Google Scholar] [CrossRef]
- Viitanen, V.; Sipilä, T.; Sánchez-Caja, A.; Siikonen, T. CFD predictions of unsteady cavitation for a marine propeller in oblique inflow. Ocean Eng. 2022, 266, 112596. [Google Scholar] [CrossRef]
- Li, M.; Hong, Y.; Si, B.; Ding, Y.; Tang, Z.; Wang, R.; He, X. Numerical and experimental study of the cavitation performance of a composite propeller. Phys. Fluids 2024, 36, 103303. [Google Scholar] [CrossRef]
- Zhu, W.; Li, Z.; Ding, R. Effect of pitch ratio on the cavitation of controllable pitch propeller. Ocean Eng. 2024, 293, 116692. [Google Scholar] [CrossRef]
- Li, M.M.; Hong, Y.; Zhang, H.L.; Wang, R.G.; He, X.D. The numerical research on cavitation performance of the composite propeller under uniform and non-uniform inflow. Ocean Eng. 2025, 322, 120399. [Google Scholar] [CrossRef]
- Muscari, R.; Di Mascio, A.; Verzicco, R. Modeling of vortex dynamics in the wake of a marine propeller. Comput. Fluids 2013, 73, 65–79. [Google Scholar] [CrossRef]
- Di Felice, F.; Felli, M.; Liefvendahl, M.; Svennberg, U. Numerical and experimental analysis of the wake behavior of a generic submarine propeller. Prism 2009, 1, 158. [Google Scholar]
- Bensow, R.E.; Bark, G. Implicit LES predictions of the cavitating flow on a propeller. J. Fluids Eng. 2010, 132, 041302. [Google Scholar] [CrossRef]
- Yilmaz, N.; Atlar, M.; Fitzsimmons, P.A. An Improved Tip Vortex Cavitation Model for Propeller-Rudder Interaction. In Proceedings of the 10th International Cavitation Symposium (CAV2018), Baltimore, MD, USA, 14–16 May 2018. [Google Scholar]
- Yimaz, N.; Atlar, M.; Khorasanchi, M. An improved Mesh Adaption and Refinement approach to Cavitation Simulation (MARCS) of propellers. Ocean Eng. 2019, 171, 139–150. [Google Scholar] [CrossRef]
- Nouri, N.M.; Mohammadi, S. A multi-objective approach for determining the number of blades on a NACA marine propeller. Brodogr. Teor. Praksa Brodogr. Pomor. Teh. 2016, 67, 15–32. [Google Scholar]
- Felli, M.; Guj, G.; Camussi, R. Effect of the number of blades on propeller wake evolution. Exp. Fluids 2008, 44, 409–418. [Google Scholar] [CrossRef]
- Zhu, Z.F. Numerical Study of Effect of the Number of Propeller Blades on Cavitating Flow. In Advanced Materials Research; Trans Tech Publications: Wollerau, Switzerland, 2013; Volume 716, pp. 739–743. [Google Scholar]
- Bakhtiari, M.; Ghassemi, H. 2019. Numerical analysis on effects of blade number on hydrodynamic performance of low-pitch marine cycloidal propeller. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2019, 234, 490–501. [Google Scholar]
- Schnerr, G.H.; Sauer, J. Physical and Numerical Modeling of Unsteady Cavitation Dynamics. In Proceedings of the Fourth International Conference on Multiphase Flow (ICMF), New Orleans, LA, USA, 27 May–1 June 2001. [Google Scholar]
- Salvatore, F.; Pereira, F.; Felli, M.; Calcagni, D.; Di Felice, F. Description of the INSEAN E779A Propeller Experimental Dataset (Version 1). Zenodo. INSEAN Tech. Rep., 2006–2085. Available online: https://doi.org/10.5281/zenodo.6077997 (accessed on 1 May 2015).


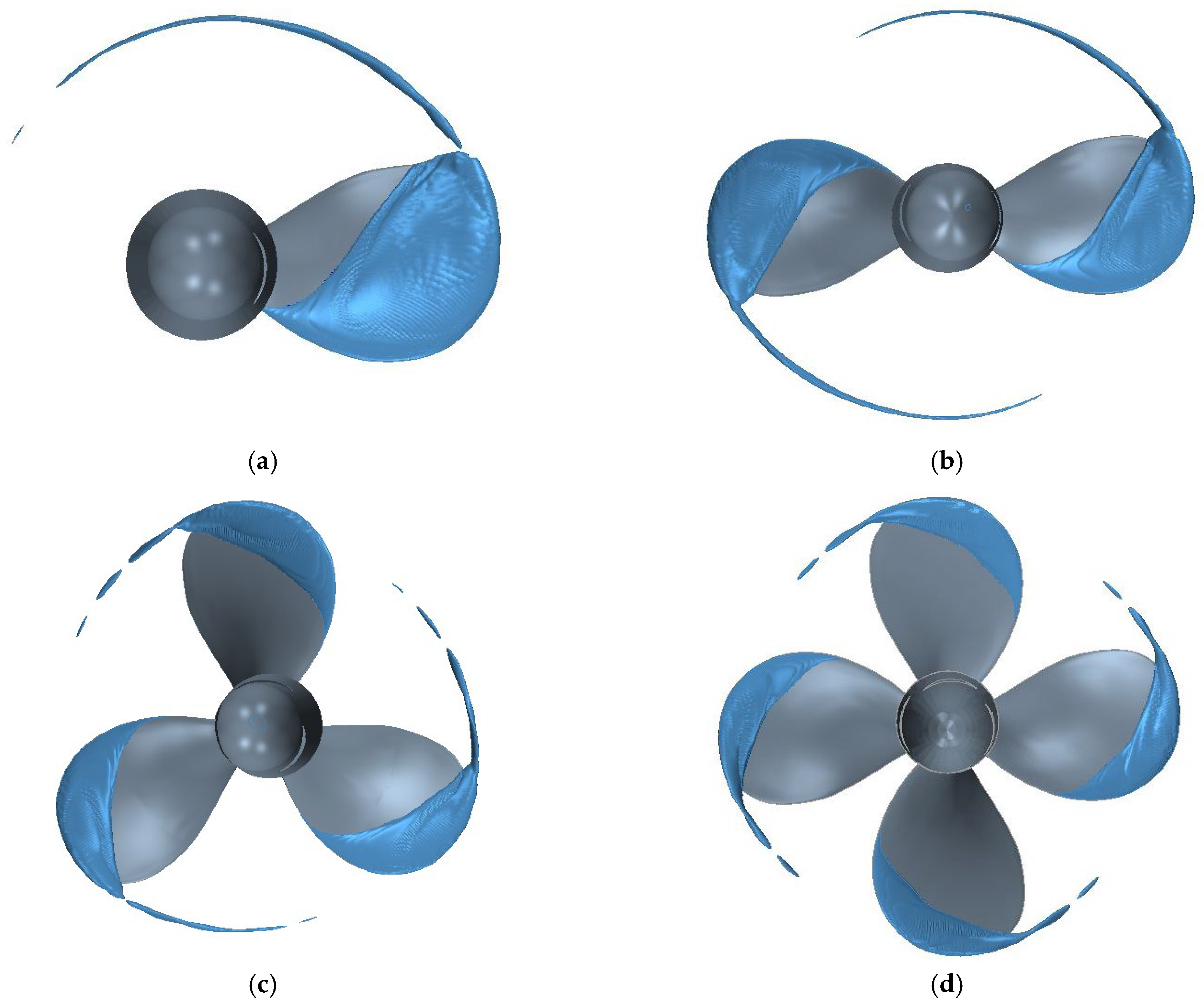



| Parameter | Value |
|---|---|
| Diameter D | 227 mm |
| Pitch ratio P | 1.1 |
| Disc ratio S | 0.689 |
| Hub diameter d | 45.53 mm |
| Rotational speed n | 25 rps |
| r/R | P/D | cb/D | xLE/D | tmax/D | xtmax/D | Rb/D |
|---|---|---|---|---|---|---|
| 0.2640 | 1.1118 | 0.2784 | 0.1604 | 0.0403 | 0.1763 | −0.0060 |
| 0.3520 | 1.1204 | 0.3080 | 0.1708 | 0.0312 | 0.1047 | −0.0107 |
| 0.4400 | 1.1202 | 0.3357 | 0.1802 | 0.0257 | 0.0867 | −0.0144 |
| 0.5280 | 1.1167 | 0.3600 | 0.1872 | 0.0212 | 0.0699 | −0.0179 |
| 0.6160 | 1.1147 | 0.3767 | 0.1897 | 0.0167 | 0.1035 | −0.0216 |
| 0.7040 | 1.1173 | 0.3784 | 0.1849 | 0.0129 | 0.0386 | −0.0250 |
| 0.7920 | 1.1174 | 0.3634 | 0.1695 | 0.0097 | 0.0166 | −0.0288 |
| 0.8800 | 1.1102 | 0.3170 | 0.1345 | 0.0063 | −0.0757 | −0.0327 |
| 0.9680 | 1.1179 | 0.1917 | 0.0597 | 0.0039 | −0.1884 | −0.0364 |
| 0.9900 | 1.1261 | 0.1157 | 0.0162 | 0.0033 | −0.4599 | −0.0376 |
| 0.9988 | 1.1261 | 0.0491 | −0.0207 | 0.0019 | −0.9726 | −0.0384 |
| Number of Blades k | Element Size | Number Before Spiral Tube Refinement | Number After Spiral Tube Refinement |
|---|---|---|---|
| 1 | 0.002D | 4.52 million | 13.52 million |
| 2 | 0.002D | 9.86 million | 17.19 million |
| 3 | 0.002D | 11.32 million | 19.52 million |
| 4 | 0.002D | 12.84 million | 22.02 million |
| Grids | Cells | KT | Error | RG | KQ | Error | RG |
|---|---|---|---|---|---|---|---|
| Fine | 30.21 M | 0.2270 | 0.5286% | 0.3655 | 0.4213 | 0.8070% | 0.3851 |
| Medium | 22.02 M | 0.2282 | 1.4461% | 0.4247 | 2.0956% | ||
| Coarse | 13.84 M | 0.2315 | -- | 0.4336 | -- |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Xiao, Y.; Fang, B.; Li, W.; Duan, C.; Zhang, W.; Hu, J. Effect of Blade Number on Tip Vortex Cavitation of Propeller. J. Mar. Sci. Eng. 2025, 13, 915. https://doi.org/10.3390/jmse13050915
Wang Y, Xiao Y, Fang B, Li W, Duan C, Zhang W, Hu J. Effect of Blade Number on Tip Vortex Cavitation of Propeller. Journal of Marine Science and Engineering. 2025; 13(5):915. https://doi.org/10.3390/jmse13050915
Chicago/Turabian StyleWang, Yanan, Yang Xiao, Bin Fang, Wen Li, Chuanzhi Duan, Weipeng Zhang, and Jian Hu. 2025. "Effect of Blade Number on Tip Vortex Cavitation of Propeller" Journal of Marine Science and Engineering 13, no. 5: 915. https://doi.org/10.3390/jmse13050915
APA StyleWang, Y., Xiao, Y., Fang, B., Li, W., Duan, C., Zhang, W., & Hu, J. (2025). Effect of Blade Number on Tip Vortex Cavitation of Propeller. Journal of Marine Science and Engineering, 13(5), 915. https://doi.org/10.3390/jmse13050915






