Experimental Study on Strength Characteristics of Overconsolidated Gassy Clay
Abstract
1. Introduction
2. Experimental Programs
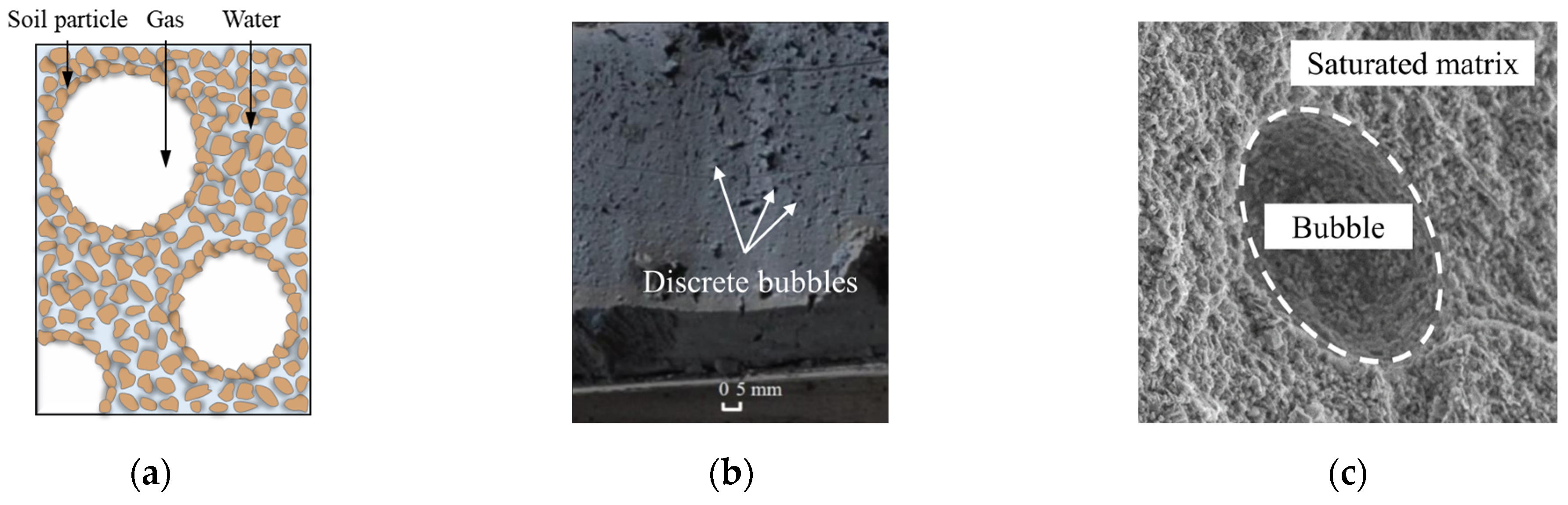
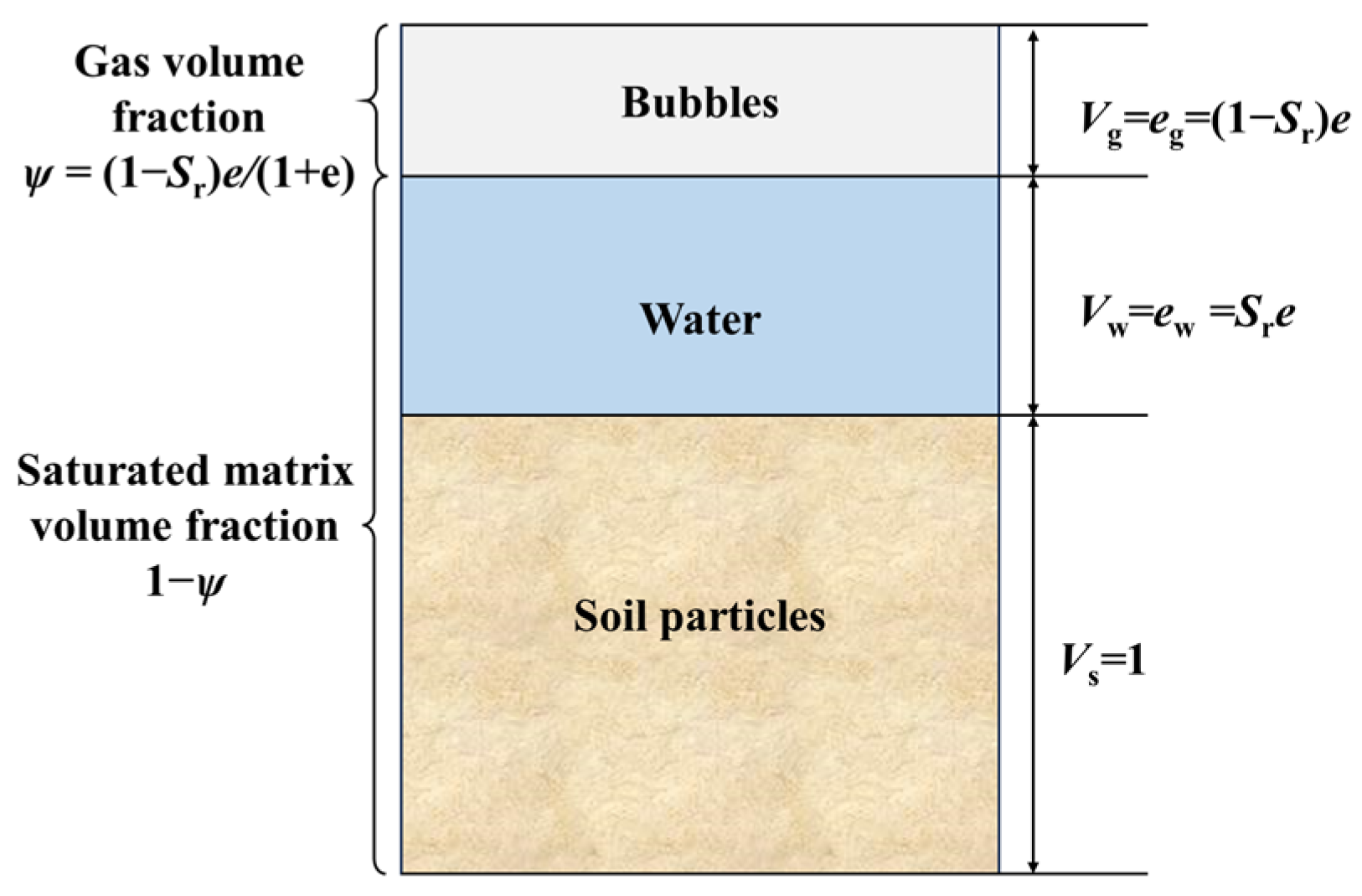
2.1. Testing Material and Preparation of Gassy Specimens
2.2. Scanning Electron Microscope Test


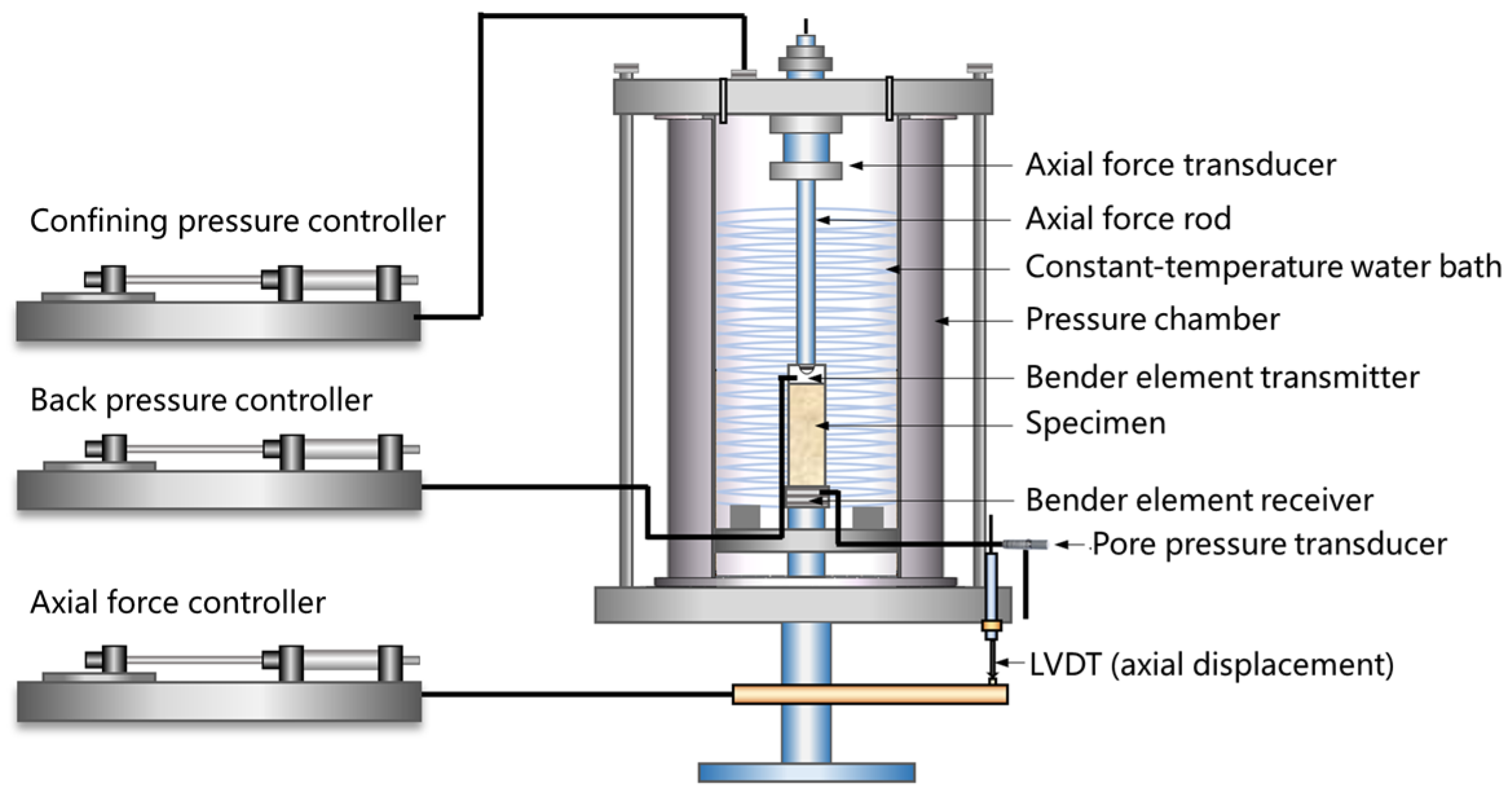
2.3. Consolidated-Undrained Triaxial Compression Test
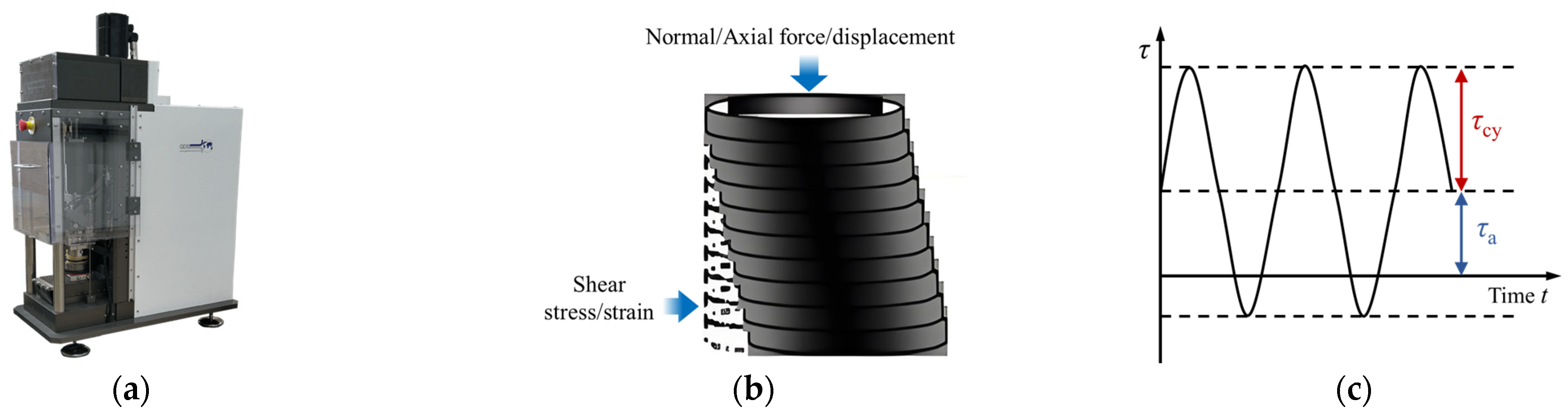
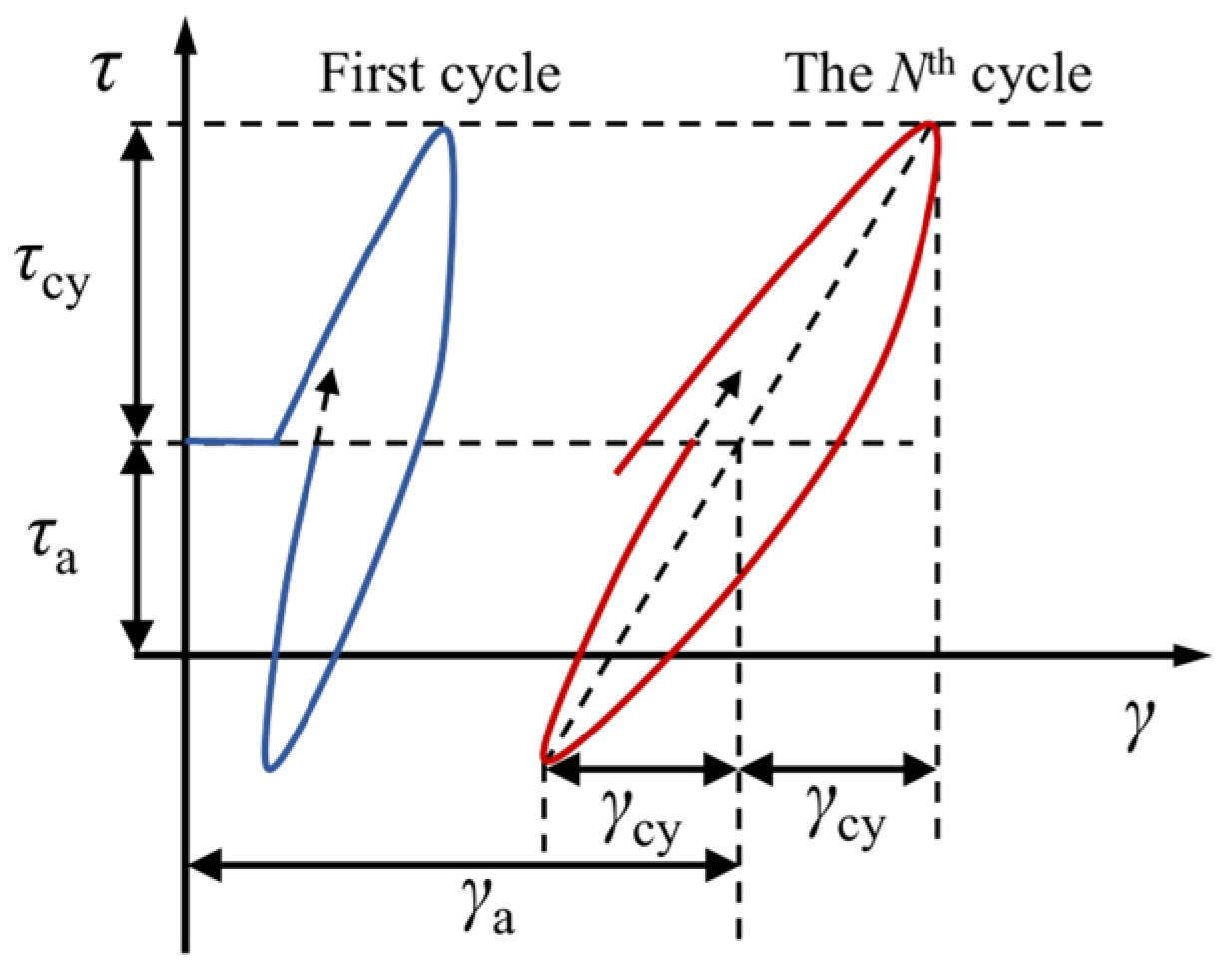
2.4. Cyclic Simple Shear Test
3. Experimental Results and Discussion
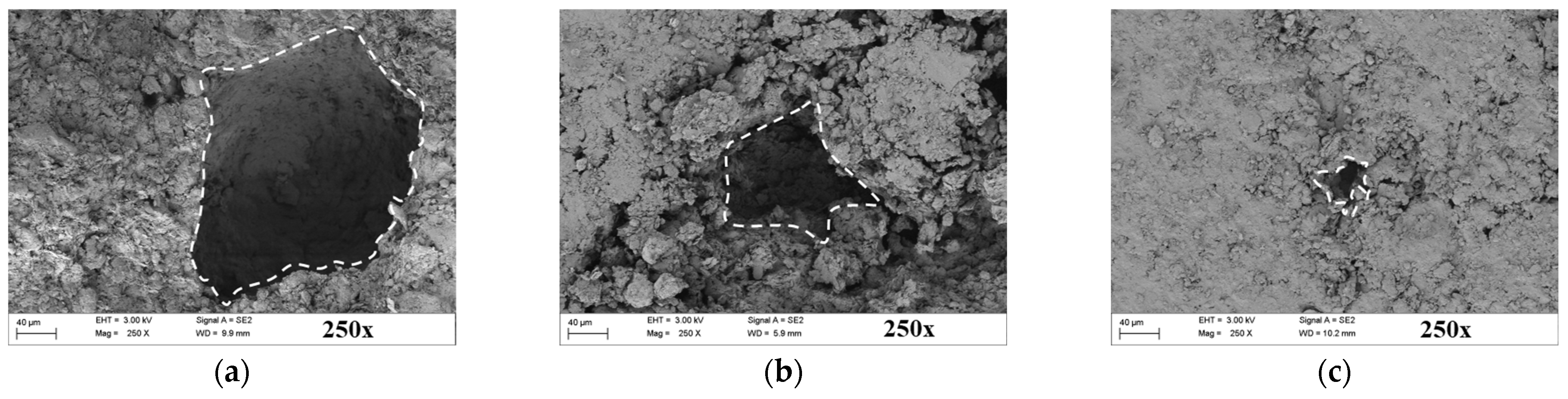
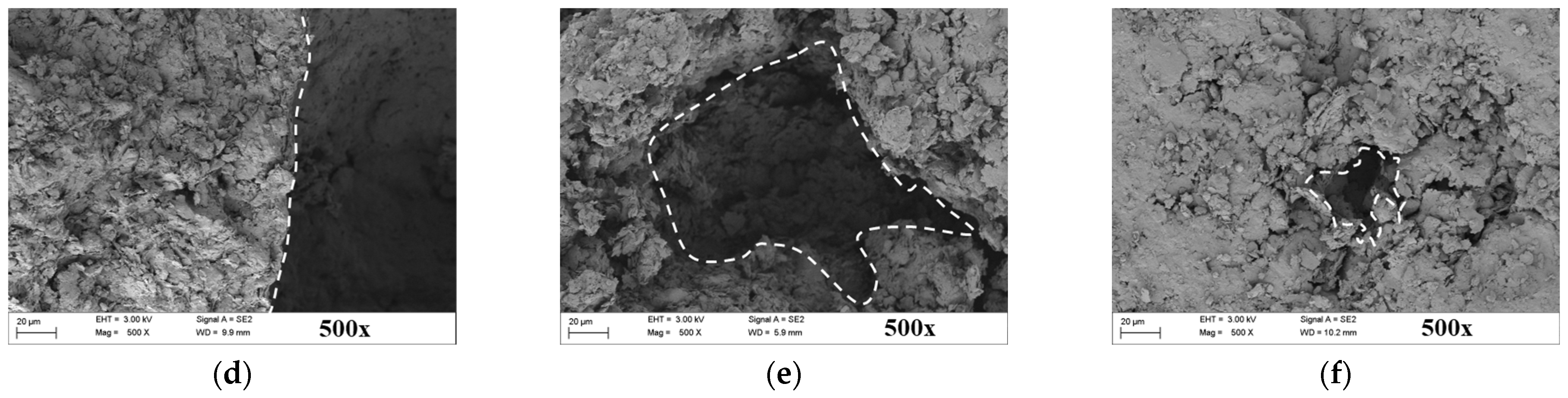
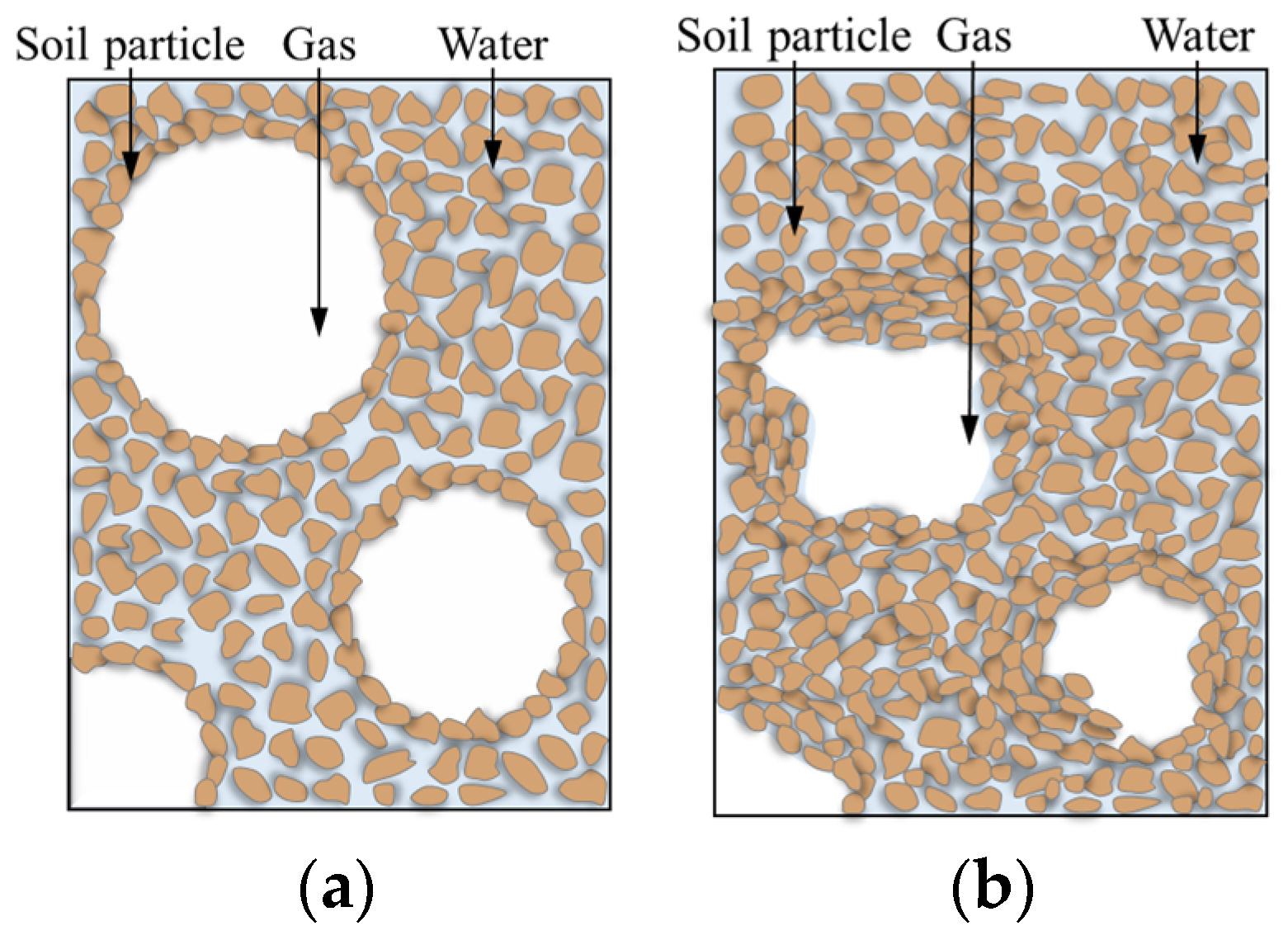
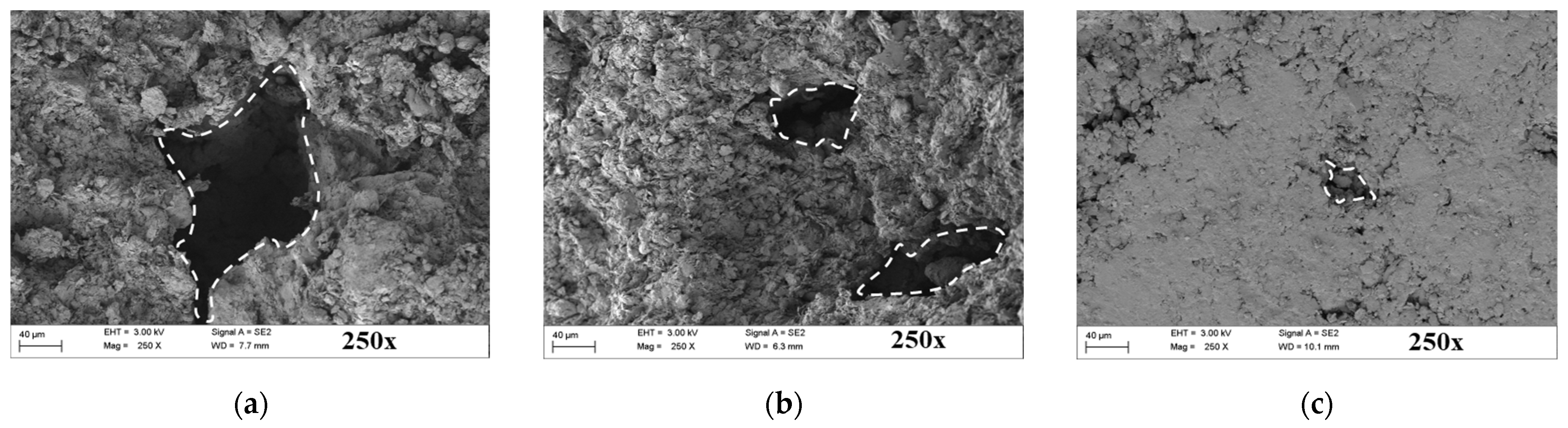
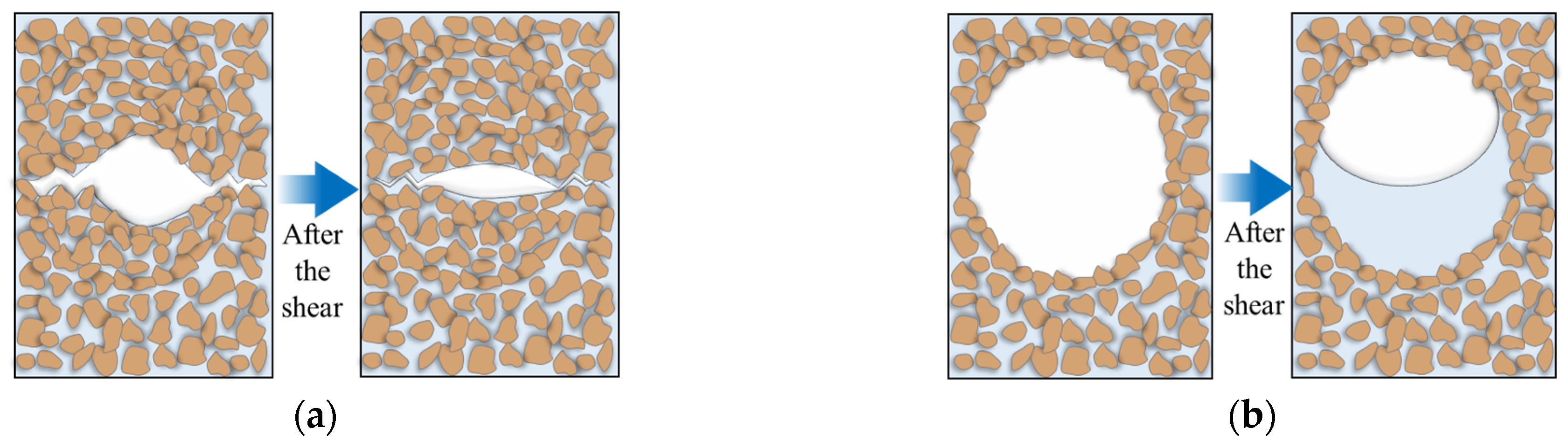
3.1. Micromorphological Characteristics
3.2. Consolidated Undrained Shear Characteristics
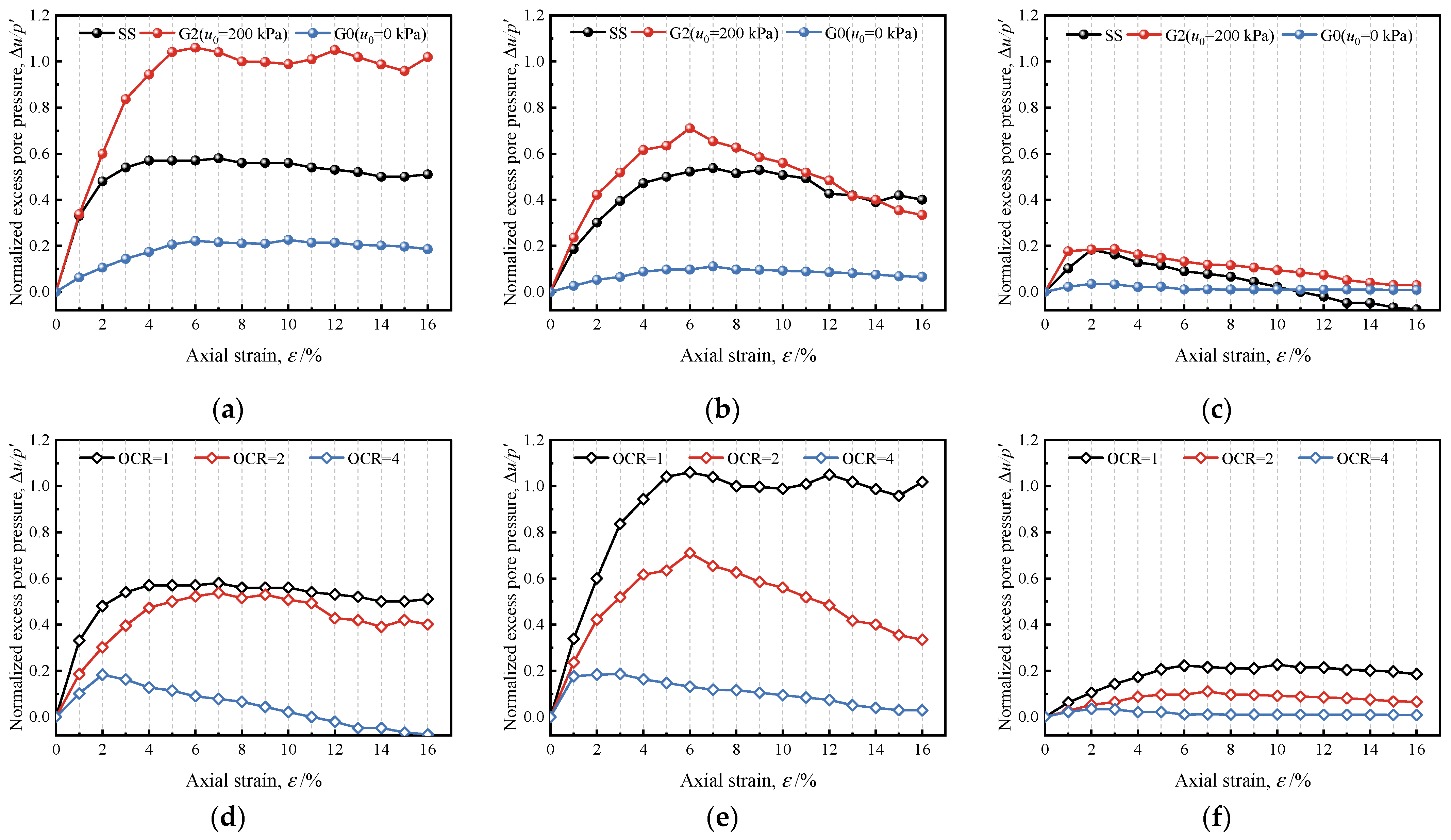
3.2.1. Excess Pore Pressure
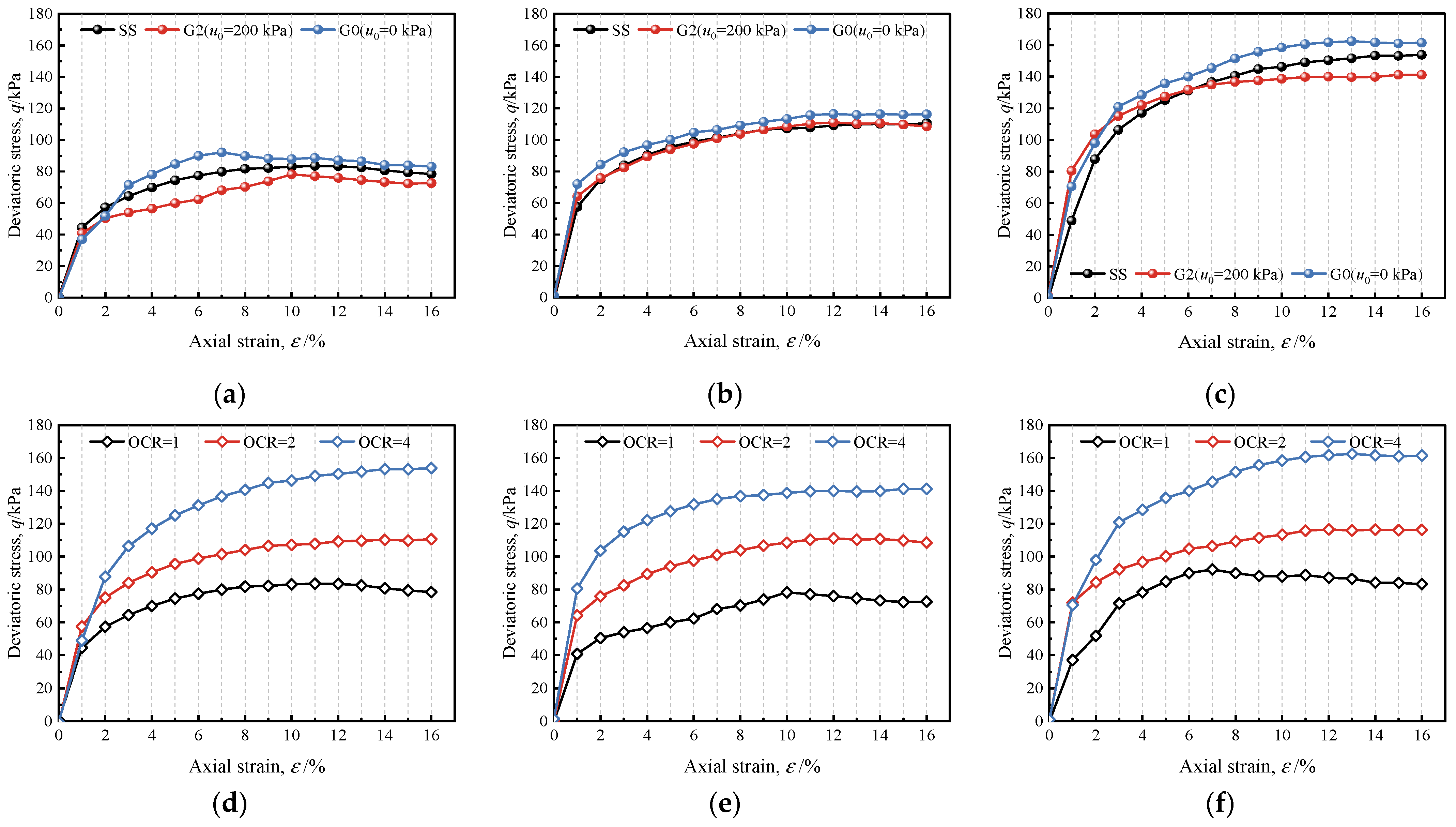
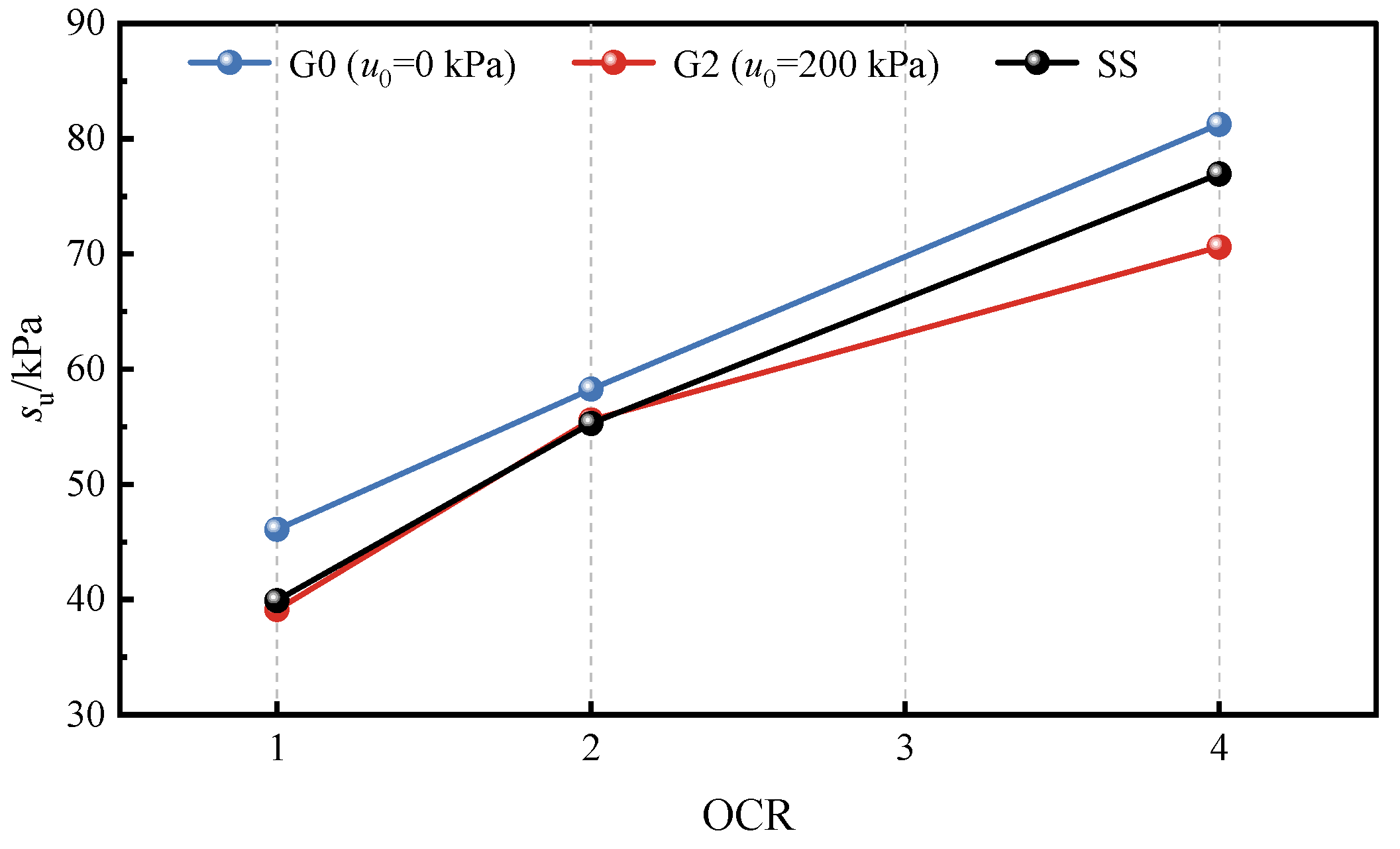
3.2.2. Stress–Strain Relationship
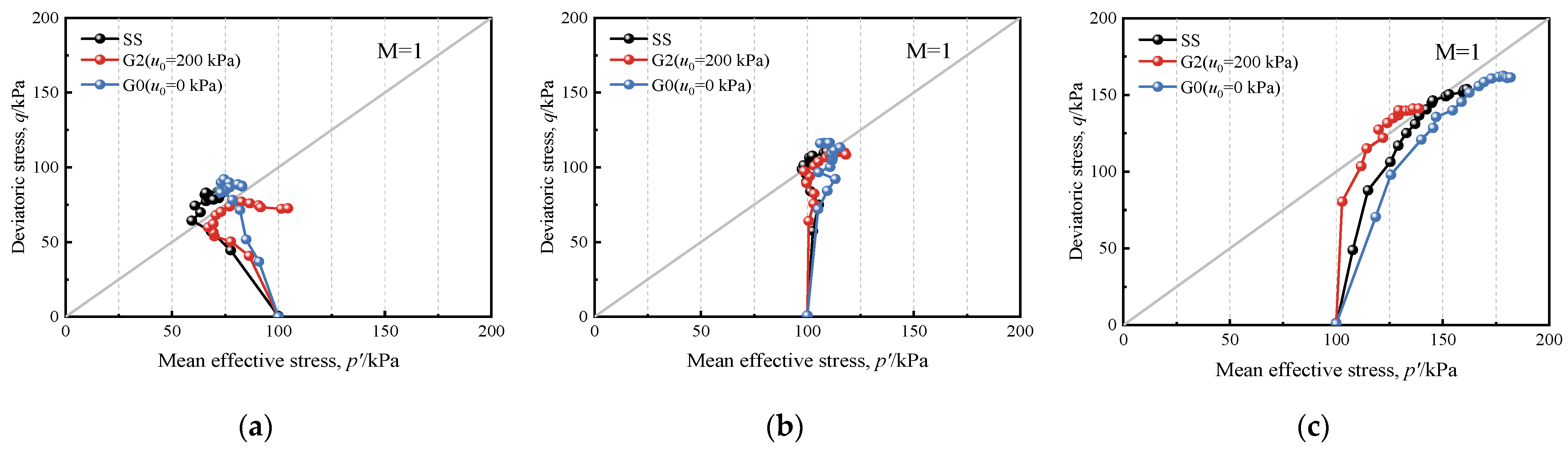
3.2.3. Stress Path
3.3. Cyclic Shear Characteristic
3.3.1. Dynamic Strain
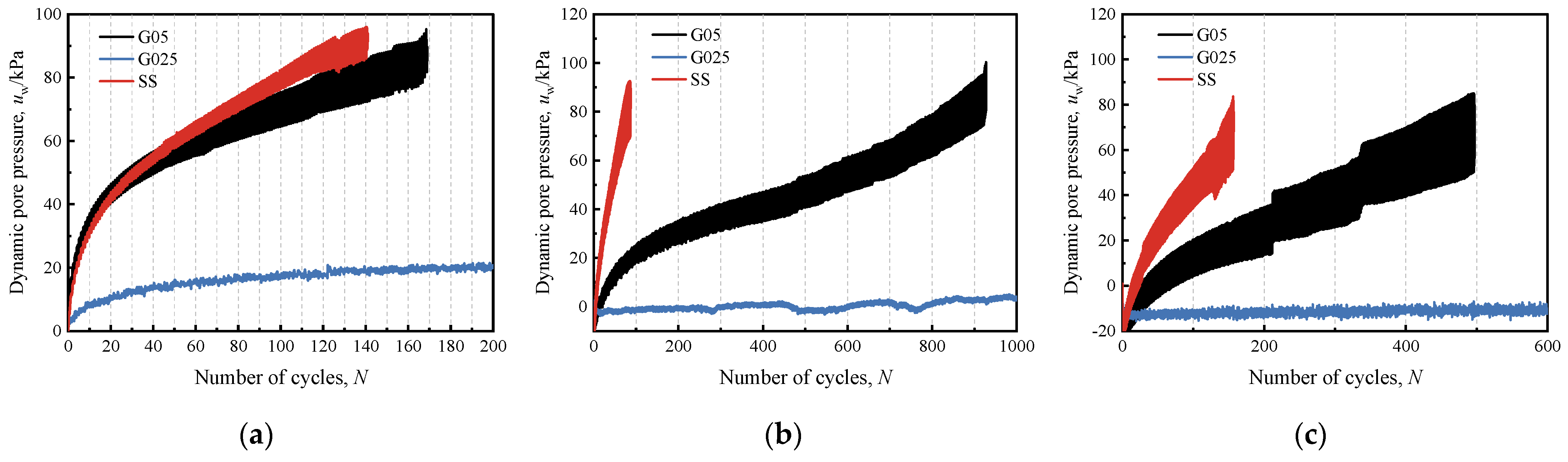
3.3.2. Dynamic Pore Pressure
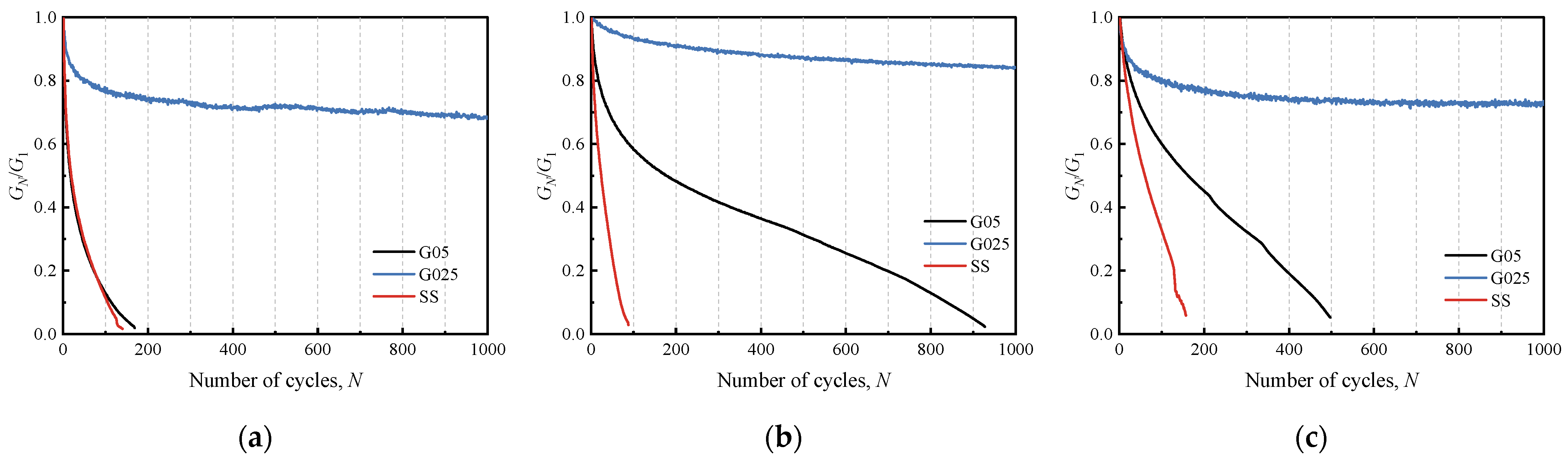
3.3.3. Stiffness Softening Coefficient
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guo, Z.; Liu, L.; Liu, Z.; Pan, Y.; Shen, X.; Lei, L. Experimental and numerical challenges in multiscale study on geomechanical and hydrological systems. Adv. Geo-Energy Res. 2025, 15, 95–98. [Google Scholar] [CrossRef]
- Guo, Z.; Gao, X.; Wu, H.; Liu, L.; Lei, L. Failure patterns in layered gas-storage systems. Adv. Geo-Energy Res. 2024, 12, 183–193. [Google Scholar] [CrossRef]
- Liu, T.; Yang, X.; Zhang, Y. A Review of Gassy Sediments: Mechanical Property, Disaster Simulation and In-Situ Test. Front. Earth Sci. 2022, 10, 15–35. [Google Scholar] [CrossRef]
- Kortekaas, S.; Sens, E.; Sarata, B. Shallow gas hazard linked to worldwide delta environments. In Frontiers in Offshore Geotechnics II; CRC Press: Boca Raton, FL, USA, 2010; pp. 221–225. [Google Scholar]
- Anderson, A.L.; Hampton, L.D. Acoustics of gas-bearing sediments I. Background. J. Acoust. Soc. Am. 1980, 67, 1865–1889. [Google Scholar] [CrossRef]
- Whelan, T.; Coleman, J.M.; Suhayda, J.N.; Roberts, H.H. Acoustical penetration and shear strength in gas-charged sediment. Mar. Geotechnol. 1977, 2, 147–159. [Google Scholar] [CrossRef]
- Puzrin, A.; Tront, J.; Schmid, A.; Hughes, J. Engineered use of microbial gas production to decrease primary consolidation settlement in clayey soils. Geotechnique 2011, 61, 785–794. [Google Scholar] [CrossRef]
- Chen, Z.; Lai, X.; Liao, L.; Huang, P. Submarine shallow gas detection method based on MIP-CPT technology: A case study in the Zhoushan sea area of the East China Sea. Acta Pet. Sin. 2016, 37, 8–16. [Google Scholar]
- Xie, C.Y. Shallow natural gas in riverside soft soils along the Yangtze River in Anhui Province and its impact on hydraulic structures. Water Resour. Hydropower Express 2000, 21, 6–12. [Google Scholar]
- Liu, L.; De Kock, T.; Wilkinson, J.; Cnudde, V.; Xiao, S.; Buchmann, C.; Uteau, D.; Peth, S.; Lorke, A. Methane bubble growth and migration in aquatic sediments observed by X-ray μCT. Environ. Sci. Technol. 2018, 52, 2007–2015. [Google Scholar] [CrossRef]
- Abegg, F.; Anderson, A.L. The acoustic turbid layer in muddy sediments of Eckernfoerde Bay, Western Baltic: Methane concentration, saturation and bubble characteristics. Mar. Geol. 1997, 137, 137–147. [Google Scholar] [CrossRef]
- Best, A.I.; Tuffin, M.D.J.; Dix, J.K.; Bull, J.M. Tidal height and frequency dependence of acoustic velocity and attenuation in shallow gassy marine sediments. J. Geophys. Res. Solid Earth 2004, 109, B08101. [Google Scholar] [CrossRef]
- Kim, G.Y.; Narantsetseg, B.; Kim, J.W.; Chun, J.H. Physical properties and micro-and macro-structures of gassy sediments in the inner shelf of SE Korea. Quat. Int. 2014, 344, 170–180. [Google Scholar] [CrossRef]
- Hong, Y.; Wang, L.; Yang, B.; Zhang, J. Stress-dilatancy behaviour of bubbled fine-grained sediments. Eng. Geol. 2019, 260, 105196. [Google Scholar] [CrossRef]
- Sobkowikz, J.C. The Mechanics of Gassy Sediments. Ph.D. Thesis, University of Alberta, Edmonton, AB, Canada, 1982. [Google Scholar]
- Grozic, J.; Nadim, F.; Kvalstad, T. On the undrained shear strength of gassy clays. Comput. Geotech. 2005, 32, 483–490. [Google Scholar] [CrossRef]
- Hong, Y.; Wang, X.; Wang, L.; Gao, Z. A state-dependent constitutive model for coarse-grained gassy soil and its application in slope instability modelling. Comput. Geotech. 2021, 129, 103847. [Google Scholar] [CrossRef]
- Sultan, N.; Garziglia, S. Mechanical behaviour of gas-charged fine sediments: Model formulation and calibration. Geotechnique 2014, 64, 851–864. [Google Scholar] [CrossRef]
- Hong, Y.; Wang, L.; Ng, C.W.; Yang, B. Effect of initial pore pressure on undrained shear behaviour of fine-grained gassy soil. Can. Geotech. J. 2017, 54, 1592–1600. [Google Scholar] [CrossRef]
- Wheeler, S.J. A conceptual model for soils containing large gas bubbles. Geotechnique 1988, 38, 389–397. [Google Scholar] [CrossRef]
- Thomas, S.D. The Consolidation Behaviour of Gassy Soil. Ph.D. Thesis, Oxford University, Oxford, UK, 1987. [Google Scholar]
- Ye, Z.B.; Li, J.R.; Ye, Q.Y.; Chen, X.X.; Zhou, W.T. Experimental study on consolidation settlement characteristics of fine-grained gassy soil. Found. Treat. 2022, 4, 472–478. [Google Scholar]
- Sills, G.C.; Wheeler, S.J.; Thomas, S.D.; Gardner, T.N. Behaviour of offshore soils containing gas bubbles. Geotechnique 1991, 41, 227–241. [Google Scholar] [CrossRef]
- Qin, A.; Sun, D.; Zhang, J. Semi-analytical solution to one-dimensional consolidation for viscoelastic unsaturated soils. Comput. Geotech. 2014, 62, 110–117. [Google Scholar] [CrossRef]
- Wang, L. On the consolidation and creep behaviour of layered viscoelastic gassy sediments. Eng. Geol. 2021, 293, 296–298. [Google Scholar] [CrossRef]
- Wheeler, S.J. The undrained shear strength of soils containing large gas bubbles. Geotechnique 1988, 38, 399–413. [Google Scholar] [CrossRef]
- Wheeler, S.J.; Sham, W.K.; Thomas, S.D. Gas pressure in unsaturated offshore soils. Can. Geotech. J. 1990, 27, 79–89. [Google Scholar] [CrossRef]
- Wheeler, S.J.; Gardner, T.N. Elastic moduli of soils containing large gas bubbles. Geotechnique 1989, 39, 333–342. [Google Scholar] [CrossRef]
- Liu, T.; Qing, C.; Zheng, J.; Ma, X.; Chen, J.; Liu, X. Study on the Mechanical Behavior of Fine-Grained Gassy Soil Under Different Stress Conditions. J. Mar. Sci. Eng. 2025, 13, 373. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, Y.; Cai, G.; Liang, Y.; Liu, T.; Chen, J. Study on the CPTu inversion methods for strength and consolidation parameters of gassy soil with different gas contents. Appl. Ocean. Res. 2024, 146, 146103960. [Google Scholar] [CrossRef]
- Hong, Y.; Chen, X.Y.; Wang, L.Z.; Yang, B.; Sanchez, M. Distinct Undrained Cyclic Behavior of Fine-Grained Gassy Soil at Various Initial Pore Water Pressures. J. Geotech. Geoenviron. Eng. 2021, 147, 06020027. [Google Scholar] [CrossRef]
- Gao, Z.; Cai, H.; Hong, Y.; Lu, D. A critical state constitutive model for gassy clay. Can. Geotech. J. 2022, 59, 1033–1045. [Google Scholar] [CrossRef]
- Cai, H.; Gao, Z.; Hong, Y.; Zhang, J. A bounding surface model for gassy clay. Comput. Geotech. 2023, 161, 105565. [Google Scholar] [CrossRef]
- Nie, Y.; Luan, M.T.; Tang, X.W.; Guo, Y.; Zhang, Z.D.; Song, Y.X. Study on shear characteristics of overconsolidated clay under monotonic and coupled cyclic loading. Rock Soil Mech. 2009, 30, 2616–2622. [Google Scholar]
- Bouriak, S.; Vanneste, M.; Saoutkine, A. Inferred gas hydrates and clay diapirs near the Storegga Slide on the southern edge of the Vøring Plateau, offshore Norway. Mar. Geol. 2000, 163, 125–148. [Google Scholar] [CrossRef]
- Maslin, M.; Mikkelsen, N.; Vilela, C.; Haq, B. Sea-level-and gas-hydrate-controlled catastrophic sediment failures of the Amazon Fan. Geology 1998, 12, 26–52. [Google Scholar] [CrossRef]
- Sultan, N.; De Gennaro, V.; Puech, A. Mechanical behaviour of gas-charged marine plastic sediments. Géotechnique 2012, 62, 751–766. [Google Scholar] [CrossRef]
- Zhang, R.; Lunne, T. Deepwater sample disturbance due to stress relief. Chin. J. Geotech. Eng. 2003, 25, 356–360. [Google Scholar]
- Hong, Y.; Zhang, J.F.; Wang, L.Z.; Liu, T. On evolving size and shape of gas bubble in marine clay under multi-stage loadings: Microcomputed tomography (μCT) characterization and cavity contraction analysis. Can. Geotech. J. 2020, 57, 1072–1091. [Google Scholar] [CrossRef]
- Lehane, B.M.; O’Loughlin, C.D.; Gaudin, C. Rate effects on penetrometer resistance in kaolin. Géotechnique 2009, 59, 41–52. [Google Scholar] [CrossRef]
- Tan, J. Research on Shear Characteristics of Soft Clay with Different Consolidation Degrees Under Static and Dynamic Loads. Master’s Thesis, Wenzhou University, Wenzhou, China, 2021. [Google Scholar] [CrossRef]
- Li, P.F.; Bi, Q.; Zhang, Y.H.; Yan, H.G.; Ren, B.; Tong, M. Research on the Cyclic Loading Characteristics of Silty Soil in the Sea Area of Eastern Guangdong. Mar. Sci. Bull. 2024, 43, 497–509. [Google Scholar]


| Parameter | Measured Value |
|---|---|
| Liquid limit: % | 40 |
| Plastic limit: % | 23 |
| Plasticity index | 17 |
| The angle of friction: ° | 25.4 |
| Coefficient of earth pressure at rest | 0.59 |
| Critical stress ratio | 1 |
| Sample Type | Zeolite Content (%) | Initial Pore Water Pressure (kPa) | OCR | Initial Mean Operative Stress (kPa) |
|---|---|---|---|---|
| Saturated (SS) | 0 | Saturation | 1, 2, 4 | 100 |
| Gassy (G0) | 20 | 0 | ||
| Gassy (G2) | 20 | 200 |
| Sample Type | Zeolite Content (%) | Dynamic Shear Stress Ratios (τcy/τmax) | OCR | Cyclic Shear Stress τcy (kPa) |
|---|---|---|---|---|
| Saturated (SS) | 0 | 0.5 | 1 | 14.6 |
| 2 | 24.8 | |||
| 4 | 34.9 | |||
| Gassy (G05) | 20 | 0.5 | 1 | 15.5 |
| 2 | 22.9 | |||
| 4 | 37.0 | |||
| Gassy (G025) | 20 | 0.25 | 1 | 7.8 |
| 2 | 11.5 | |||
| 4 | 18.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, T.; Zhu, L.; Zhang, Y.; Qing, C.; Zhan, Y.; Zhu, C.; Jia, J. Experimental Study on Strength Characteristics of Overconsolidated Gassy Clay. J. Mar. Sci. Eng. 2025, 13, 904. https://doi.org/10.3390/jmse13050904
Liu T, Zhu L, Zhang Y, Qing C, Zhan Y, Zhu C, Jia J. Experimental Study on Strength Characteristics of Overconsolidated Gassy Clay. Journal of Marine Science and Engineering. 2025; 13(5):904. https://doi.org/10.3390/jmse13050904
Chicago/Turabian StyleLiu, Tao, Longfei Zhu, Yan Zhang, Chengrong Qing, Yuanzhe Zhan, Chaonan Zhu, and Jiayang Jia. 2025. "Experimental Study on Strength Characteristics of Overconsolidated Gassy Clay" Journal of Marine Science and Engineering 13, no. 5: 904. https://doi.org/10.3390/jmse13050904
APA StyleLiu, T., Zhu, L., Zhang, Y., Qing, C., Zhan, Y., Zhu, C., & Jia, J. (2025). Experimental Study on Strength Characteristics of Overconsolidated Gassy Clay. Journal of Marine Science and Engineering, 13(5), 904. https://doi.org/10.3390/jmse13050904






