Compact Impedance Matching Circuit for Wideband Power Transfer in Janus Helmholtz Transducers
Abstract
1. Introduction
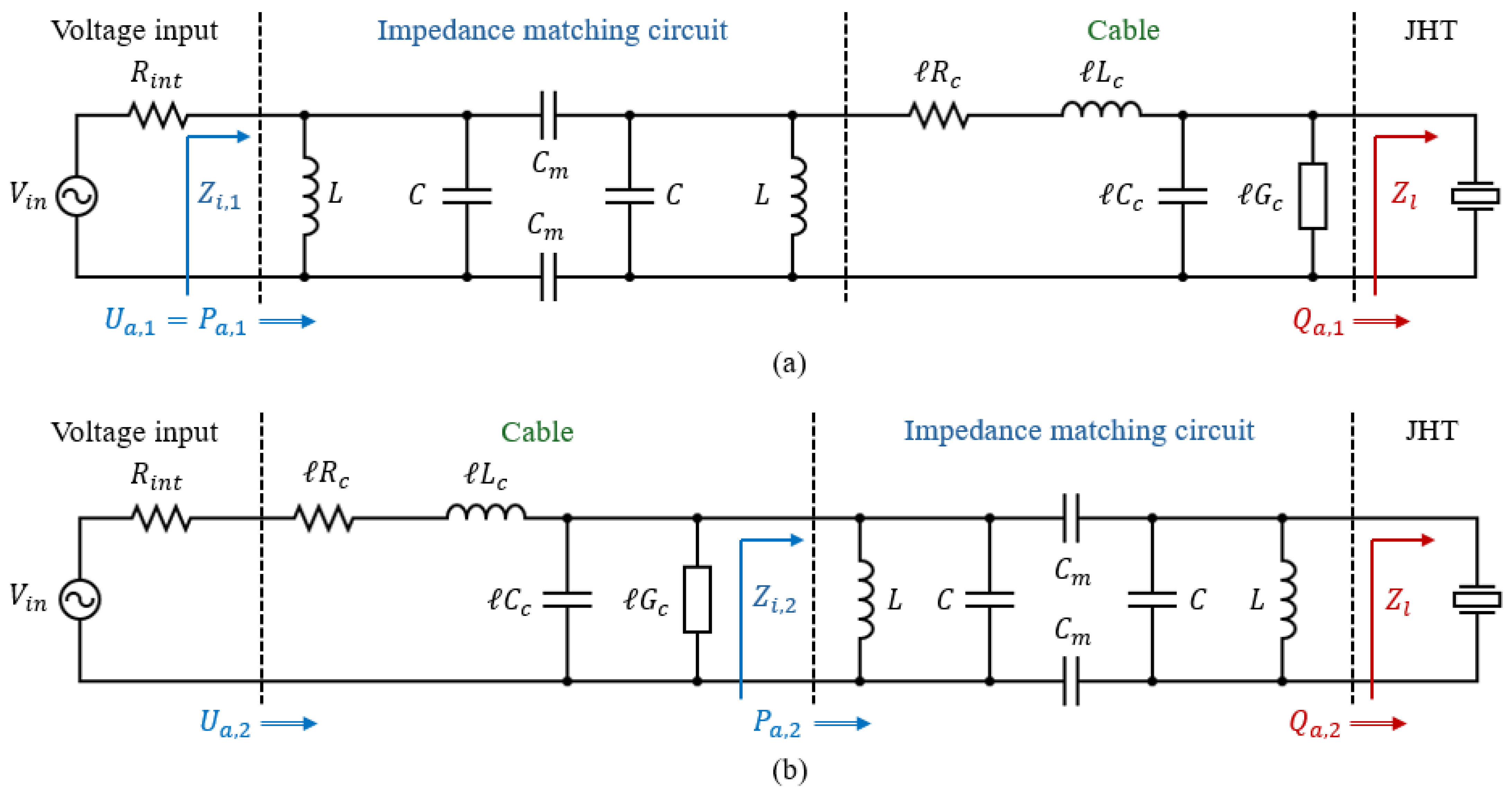
2. Impedance Matching Circuit
2.1. Impedances of the Driving System
2.2. Design of the Impedance Matching Circuit
3. Simulation Results
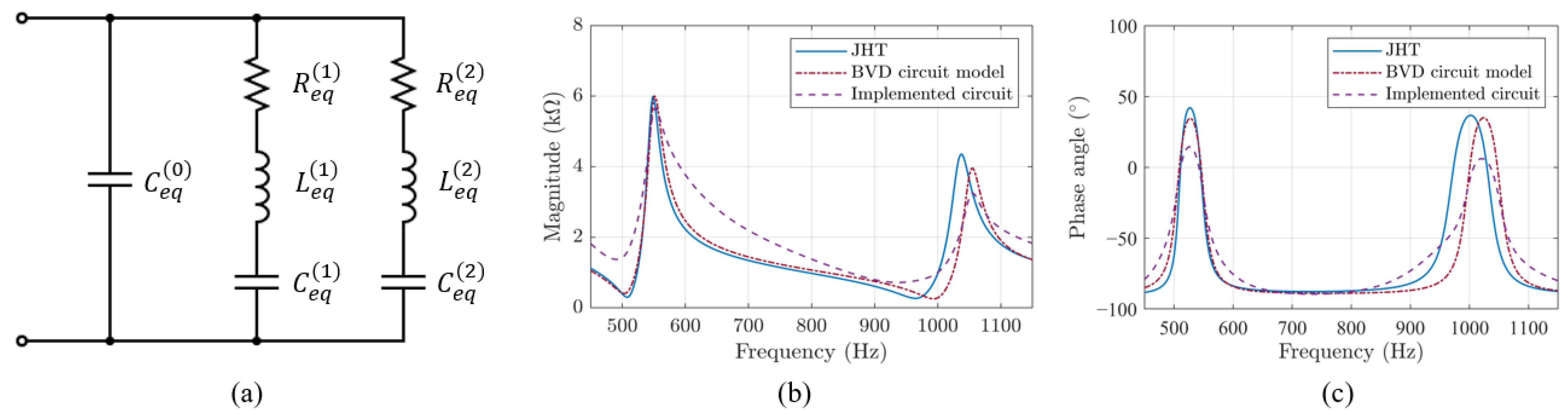
3.1. Design Details of the JHT and the Impedance Matching Circuit
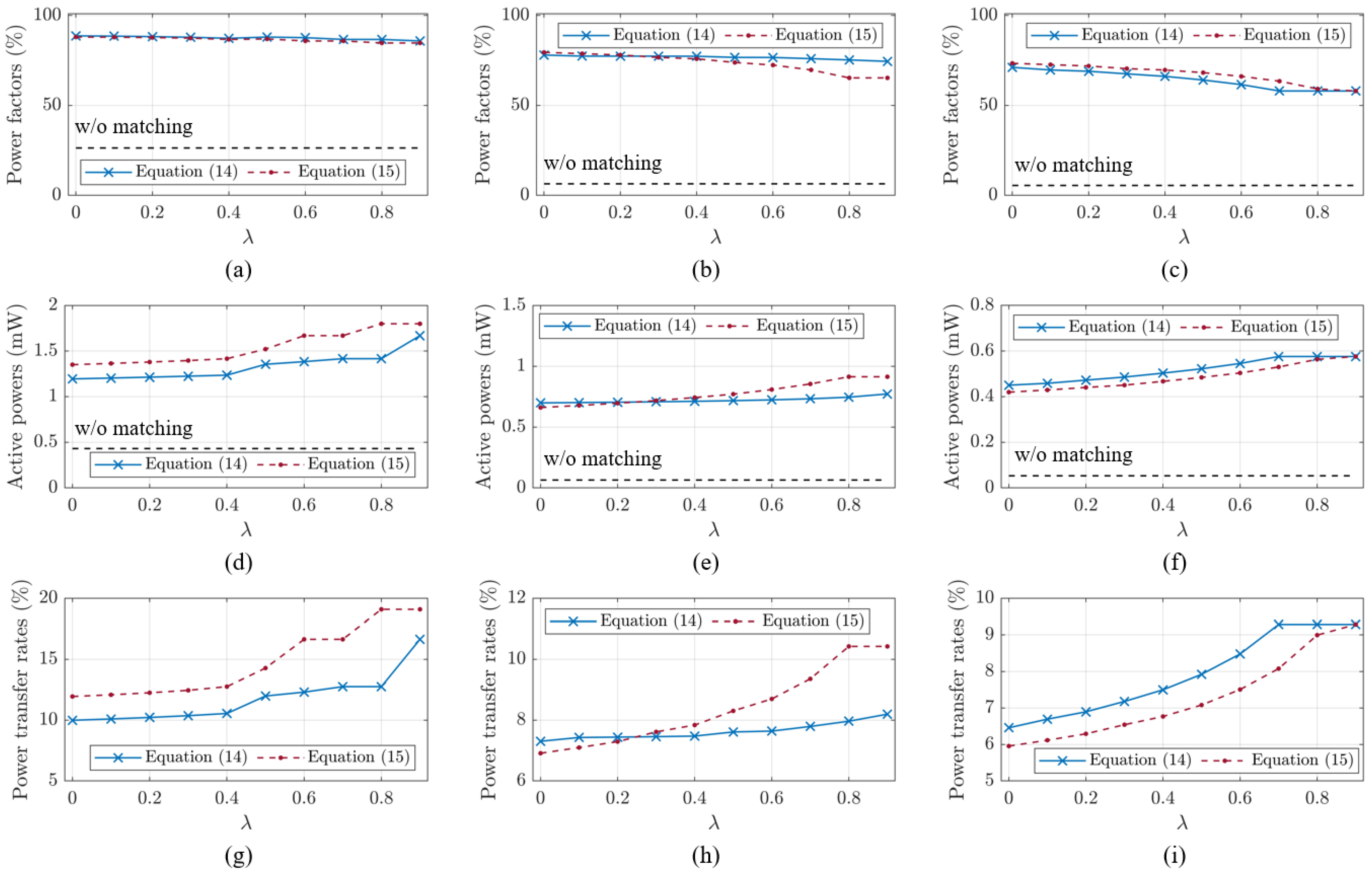
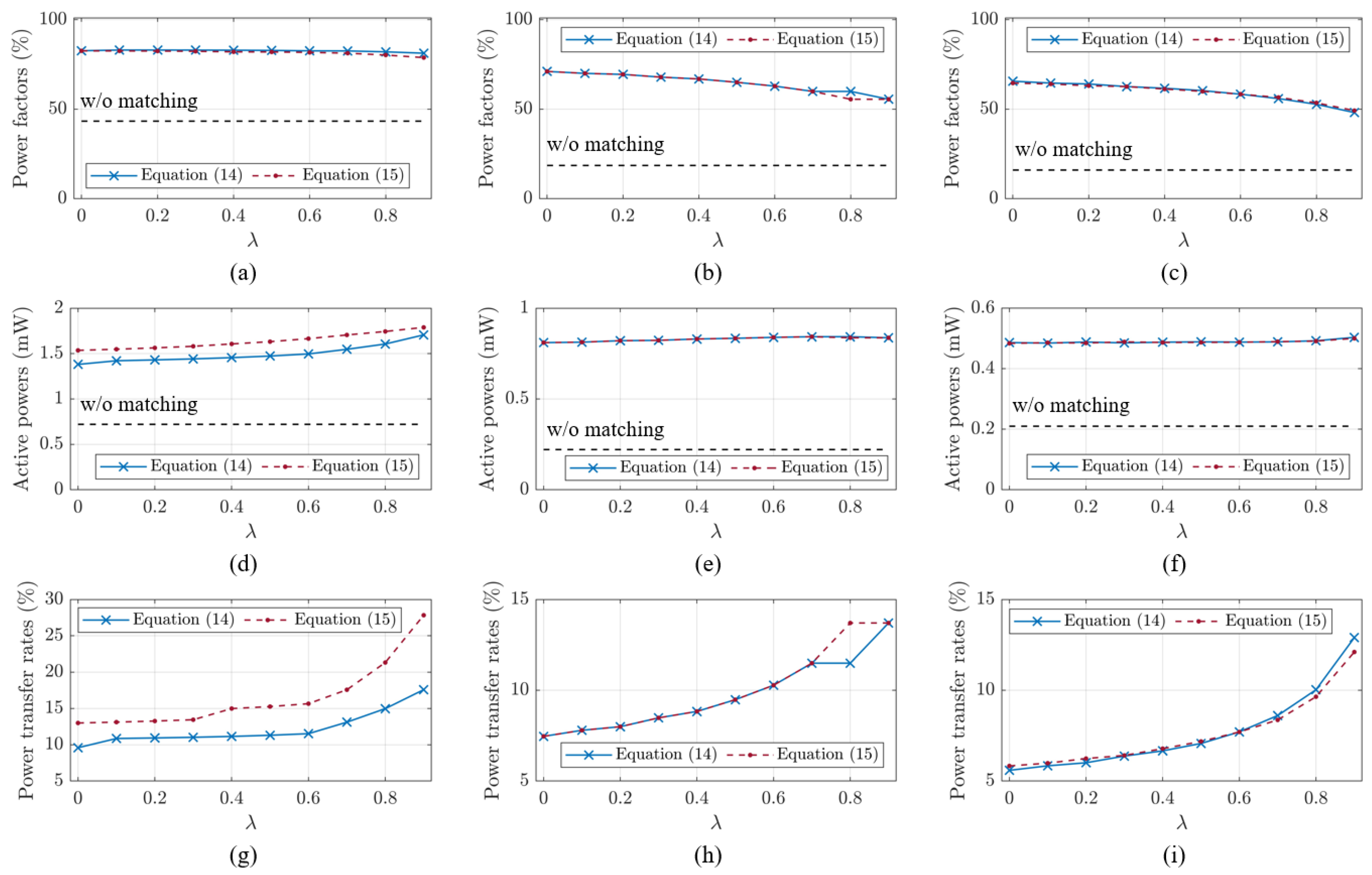
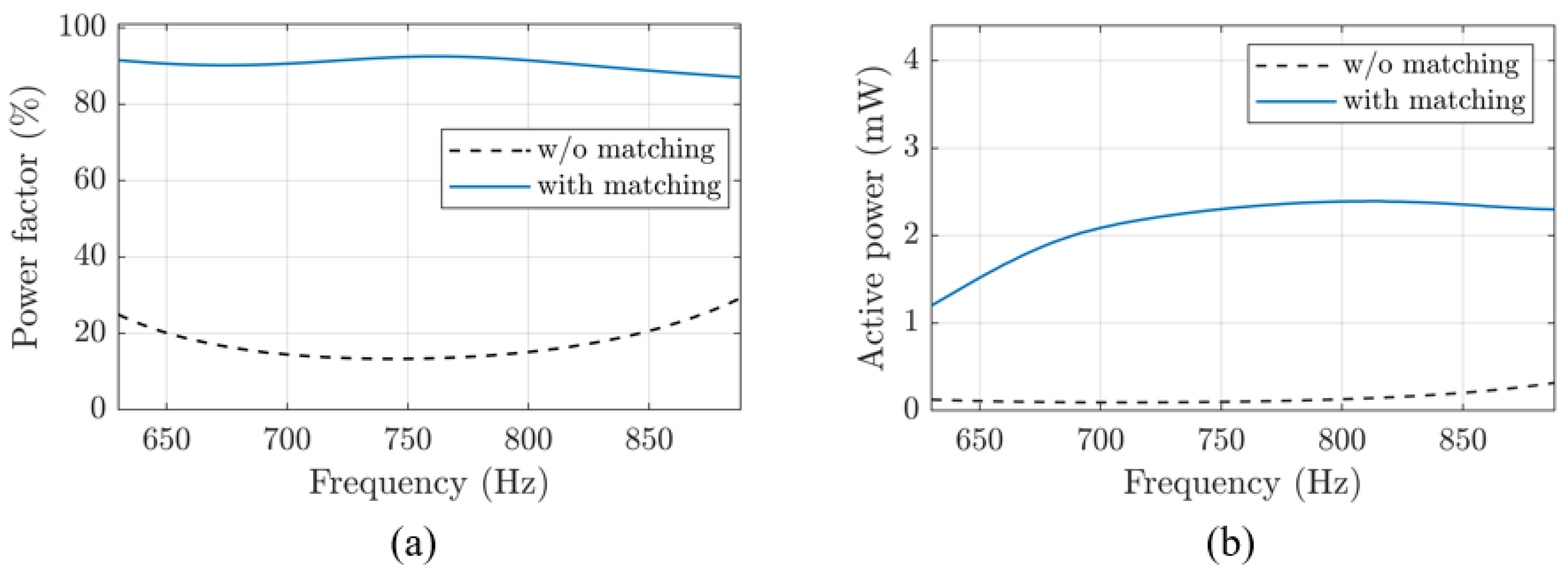
3.2. Evaluation of the Impedance Matching Circuit in Case I
3.3. Evaluation of the Impedance Matching Circuit in Case II
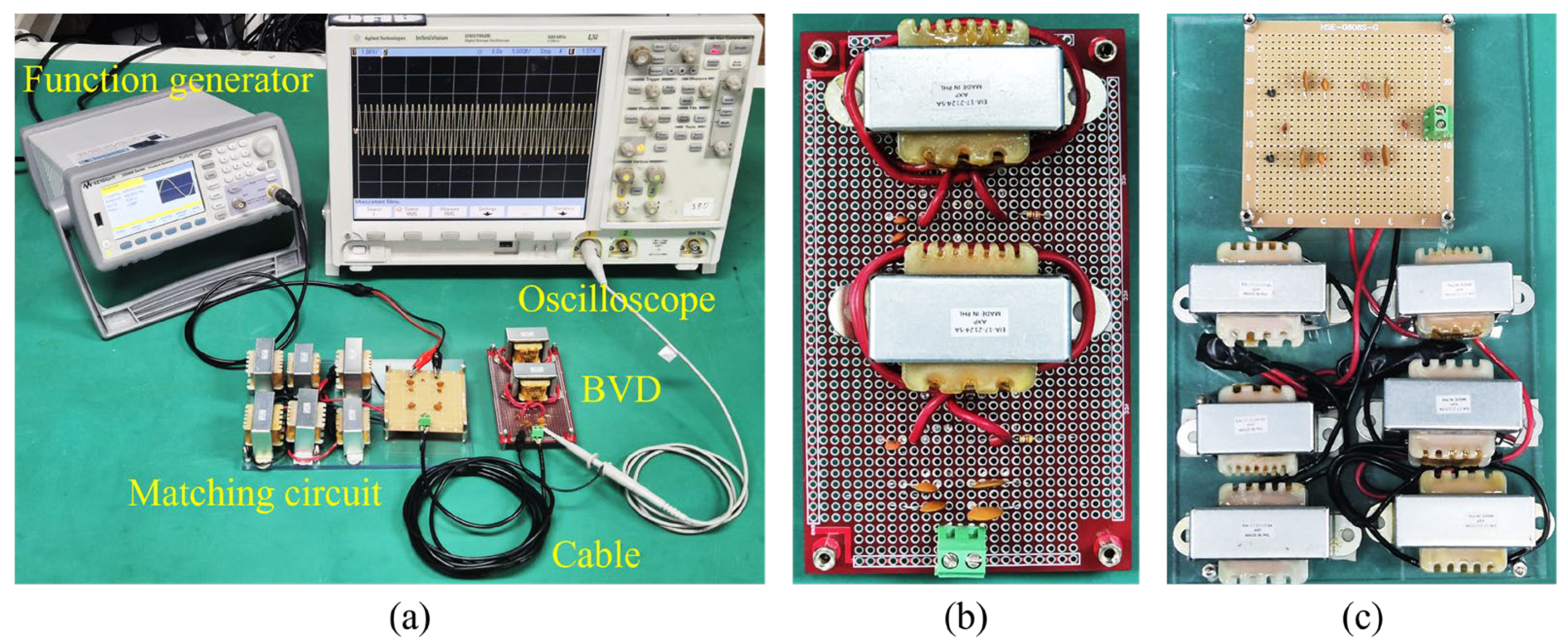
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| JHT | Janus Helmholtz Transducer |
| TVR | Transmission Voltage Response |
| KVL | Kirchhoff’s Voltage Law’ |
| PSO | Particle Swarm Optimization |
| SPICE | Simulation Program with Integrated Circuit Emphasis |
| BVD | Butterworth–Van Dyke |
Appendix A
Appendix B
Appendix C

| Material Properties | Unit | Value |
|---|---|---|
| Elastic modulus of the piezoelectric disc | m2/N | 15.5 × 10−12 |
| Density of the tail mass | kg/m3 | 4510 |
| Young’s modulus of the tail mass | pa | 110 × 109 |
| Density of the head mass | kg/m3 | 4500 |
| Young’s modulus of the head mass | pa | 116 × 109 |
| Young’s modulus of the cylindrical housing | pa | 70 × 109 |
| Parameters | Unit | Value |
|---|---|---|
| Resistance () | Ω | 107 × 10−3 |
| Inductance () | H | 321 × 10−9 |
| Capacitance () | F | 98.7 × 10−12 |
| Conductance () | S | 12.8 × 10−9 |
Appendix D
| Band | Type I | Type II | Type III | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Equation (14) | Equation (15) | Equation (14) | Equation (15) | Equation (14) | Equation (15) | ||||||||
| 0.0 | 7 | 1778 | 7 | 1704 | 49 | 1544 | 47 | 1541 | 44 | 2013 | 41 | 2178 | |
| 0.1 | 7 | 1769 | 7 | 1689 | 48 | 1506 | 47 | 1537 | 45 | 1949 | 42 | 2120 | |
| 0.2 | 7 | 1759 | 7 | 1671 | 48 | 1500 | 47 | 1532 | 47 | 1879 | 43 | 2056 | |
| 0.3 | 7 | 1747 | 7 | 1648 | 48 | 1492 | 47 | 1526 | 49 | 1800 | 45 | 1984 | |
| 0.4 | 7 | 1730 | 8 | 1619 | 49 | 1483 | 47 | 1519 | 51 | 1710 | 46 | 1903 | |
| 0.5 | 7 | 1708 | 8 | 1578 | 49 | 1471 | 48 | 1510 | 54 | 1607 | 49 | 1809 | |
| 0.6 | 7 | 1676 | 8 | 1522 | 50 | 1454 | 48 | 1498 | 58 | 1484 | 51 | 1698 | |
| 0.7 | 8 | 1623 | 9 | 1443 | 50 | 1430 | 49 | 1480 | 64 | 1332 | 55 | 1561 | |
| 0.8 | 8 | 1525 | 9 | 1323 | 52 | 1387 | 50 | 1452 | 73 | 1130 | 62 | 1380 | |
| 0.9 | 9 | 1317 | 11 | 1118 | 57 | 1270 | 52 | 1386 | 73 | 1130 | 62 | 1380 | |
| 0.0 | 8 | 1834 | 8 | 1769 | 44 | 2063 | 42 | 2187 | 39 | 3022 | 41 | 2895 | |
| 0.1 | 8 | 1830 | 8 | 1762 | 45 | 1989 | 43 | 2121 | 40 | 2922 | 42 | 2809 | |
| 0.2 | 8 | 1824 | 8 | 1752 | 47 | 1907 | 44 | 2048 | 42 | 2811 | 43 | 2715 | |
| 0.3 | 8 | 1817 | 8 | 1741 | 49 | 1815 | 46 | 1966 | 44 | 2689 | 45 | 2611 | |
| 0.4 | 8 | 1809 | 9 | 1728 | 49 | 1815 | 48 | 1873 | 46 | 2551 | 47 | 2494 | |
| 0.5 | 8 | 1799 | 9 | 1709 | 55 | 1587 | 50 | 1764 | 49 | 2392 | 50 | 2360 | |
| 0.6 | 8 | 1786 | 9 | 1684 | 74 | 877 | 53 | 1635 | 53 | 2206 | 53 | 2203 | |
| 0.7 | 8 | 1766 | 9 | 1645 | 74 | 877 | 53 | 1635 | 59 | 1977 | 58 | 2010 | |
| 0.8 | 8 | 1732 | 10 | 1573 | 84 | 758 | 66 | 1241 | 69 | 1678 | 66 | 1758 | |
| 0.9 | 9 | 1651 | 11 | 1389 | 84 | 758 | 89 | 716 | 69 | 1678 | 83 | 1380 | |
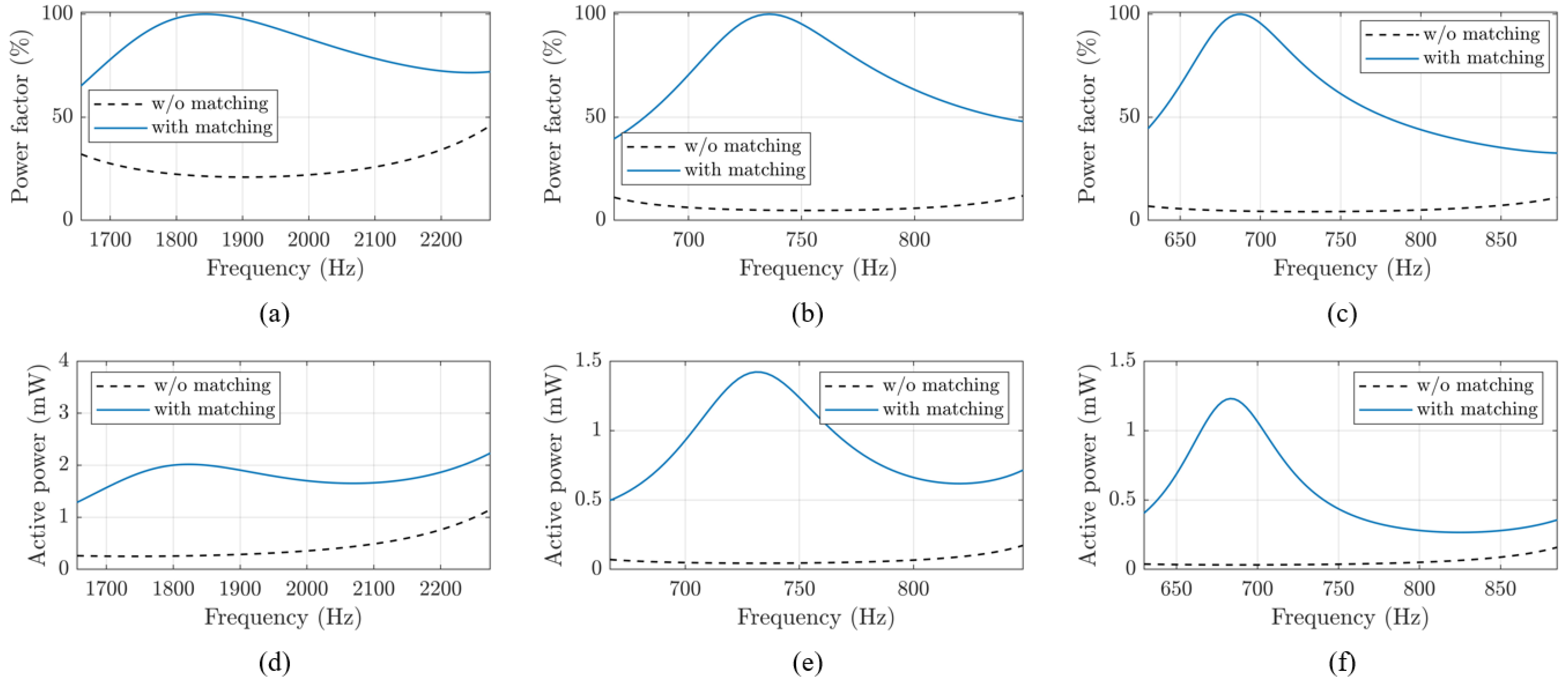

Appendix E
| Band | Type I | Type II | Type III | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Equation (14) | Equation (15) | Equation (14) | Equation (15) | Equation (14) | Equation (15) | ||||||||
| 0.0 | 7 | 1295 | 8 | 1190 | 45 | 1491 | 43 | 1578 | 45 | 1606 | 42 | 1732 | |
| 0.1 | 7 | 1279 | 8 | 1173 | 46 | 1485 | 44 | 1539 | 47 | 1561 | 43 | 1691 | |
| 0.2 | 7 | 1261 | 8 | 1153 | 46 | 1479 | 45 | 1497 | 48 | 1512 | 44 | 1646 | |
| 0.3 | 7 | 1240 | 8 | 1130 | 46 | 1471 | 47 | 1449 | 50 | 1455 | 46 | 1595 | |
| 0.4 | 7 | 1215 | 8 | 1096 | 46 | 1462 | 48 | 1395 | 52 | 1391 | 47 | 1537 | |
| 0.5 | 8 | 1185 | 9 | 1070 | 47 | 1450 | 51 | 1332 | 55 | 1315 | 49 | 1470 | |
| 0.6 | 8 | 1147 | 10 | 974 | 47 | 1436 | 53 | 1255 | 59 | 1223 | 52 | 1389 | |
| 0.7 | 8 | 1096 | 10 | 974 | 48 | 1416 | 57 | 1158 | 65 | 1104 | 56 | 1287 | |
| 0.8 | 8 | 1096 | 11 | 890 | 49 | 1387 | 64 | 1025 | 65 | 1104 | 63 | 1147 | |
| 0.9 | 10 | 974 | 11 | 890 | 50 | 1332 | 64 | 1025 | 65 | 1104 | 65 | 1104 | |
| 0.0 | 7 | 1358 | 9 | 1217 | 47 | 1547 | 47 | 1547 | 43 | 1962 | 45 | 1916 | |
| 0.1 | 8 | 1347 | 9 | 1201 | 49 | 1499 | 49 | 1499 | 45 | 1910 | 46 | 1869 | |
| 0.2 | 8 | 1334 | 9 | 1182 | 50 | 1445 | 50 | 1445 | 46 | 1852 | 48 | 1817 | |
| 0.3 | 8 | 1319 | 9 | 1160 | 53 | 1385 | 53 | 1385 | 49 | 1786 | 49 | 1759 | |
| 0.4 | 8 | 1300 | 10 | 1134 | 55 | 1315 | 55 | 1315 | 51 | 1712 | 52 | 1693 | |
| 0.5 | 8 | 1276 | 10 | 1103 | 59 | 1234 | 59 | 1234 | 54 | 1625 | 55 | 1617 | |
| 0.6 | 8 | 1245 | 10 | 1062 | 64 | 1134 | 64 | 1134 | 59 | 1522 | 59 | 1526 | |
| 0.7 | 9 | 1202 | 11 | 1007 | 72 | 1005 | 72 | 1005 | 66 | 1395 | 64 | 1414 | |
| 0.8 | 10 | 1135 | 13 | 924 | 72 | 1005 | 88 | 822 | 77 | 1231 | 74 | 1267 | |
| 0.9 | 11 | 1006 | 16 | 771 | 88 | 822 | 88 | 822 | 99 | 1023 | 93 | 1066 | |
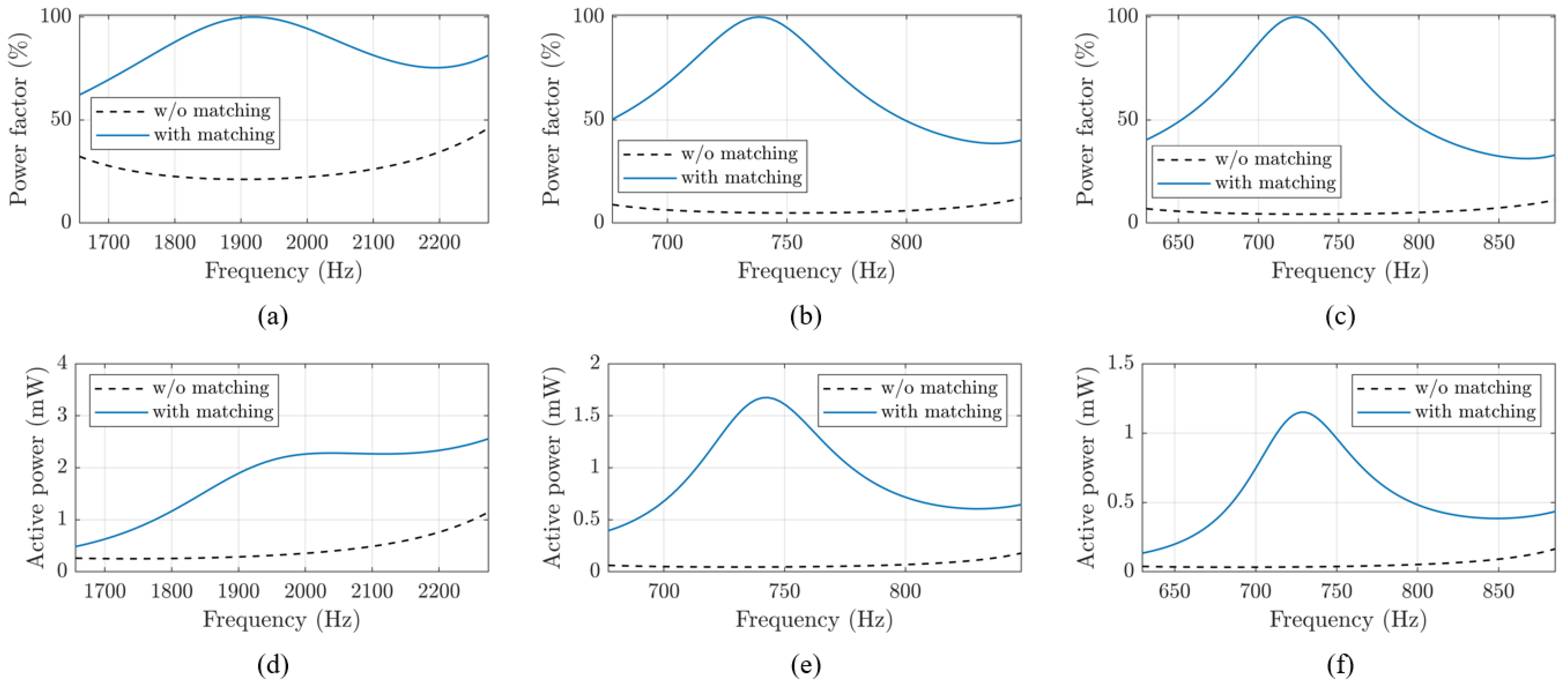
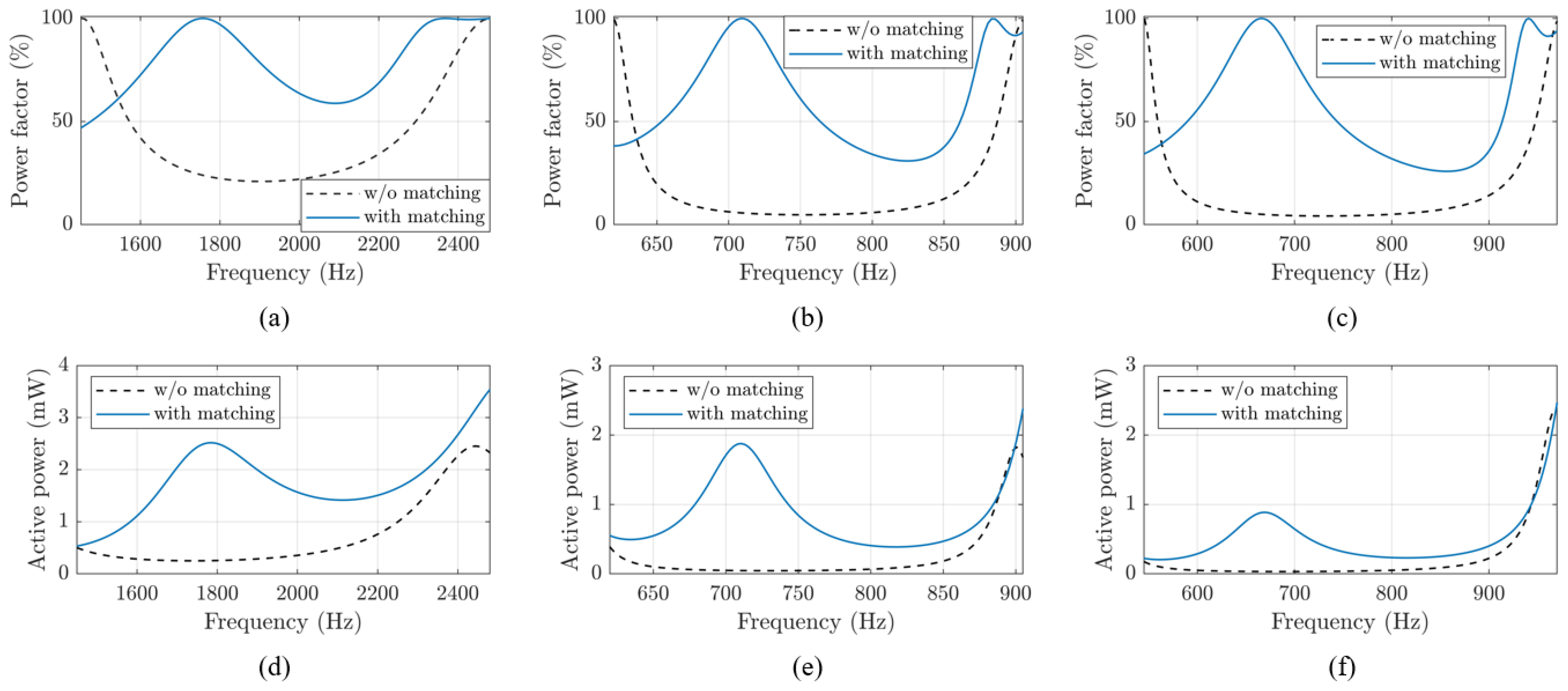
References
- Afzal, M.S.; Lim, Y.; Lee, S.; Yoon, H.; Roh, Y. Optimization and characterization of a wideband multimode Tonpilz transducer for underwater acoustical arrays. Sens. Actuat A-Phys. 2020, 307, 112001. [Google Scholar] [CrossRef]
- Abdullah, Z.; Nix, S.; Raja, M.A.Z.; Zammer, A. Design of wideband Tonpilz transducer for underwater SONAR applications with finite element model. Appl. Acoust. 2021, 183, 108293. [Google Scholar] [CrossRef]
- Pyo, S.; Afzal, M.S.; Lim, Y.; Lee, S.; Roh, Y. Design of a wideband Tonpilz transducer comprising non-uniform piezoceramic stacks with equivalent circuits. Sensors 2021, 21, 2680. [Google Scholar] [CrossRef] [PubMed]
- Bian, J.; Wang, Y.; Liu, Z.; Shen, M.; Zhao, H.; Sun, Y.; Zhu, J. Ultra-wideband underwater acoustic transducer with a gradient impedance matching layer. Appl. Acoust. 2021, 175, 107789. [Google Scholar] [CrossRef]
- Chen, W.; Ma, S.; Lai, X.; Wang, Z.; Zhao, H.; Zha, Q.; Chiu, Y.; Jin, Y. Design, fabrication, characterization, and simulation of AIN-based piezoelectric micromachined ultrasonic transducer for sonar imaging applications. Micromachines 2024, 15, 751. [Google Scholar]
- Gall, Y.L.; Boucher, D.; Lurton, X.; Bruneau, A.M. A 300 Hz Janus-Helmholtz transducer for ocean acoustic tomography. In Proceedings of the OCEANS ’93, Victoria, BC, Canada, 18–21 October 1993; pp. 278–281. [Google Scholar]
- Zhang, Y. A longitudinal-bending fluid-cavity coupled broadband underwater acoustic transducer. In Proceedings of the 2020 15th Symposium on Piezoelectricity, Acoustic Waves and Device Applications, Zhengzhou, China, 16–19 April 2021; pp. 390–393. [Google Scholar]
- Liu, W.; Mo, X.; Chai, Y.; Wu, T.; Pan, R. A distributed parameter model of the Janus-Helmholtz transducer. JASA 2024, 25, 681–694. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Mo, X.; Chai, Y.; Wu, T.; Zhang, X. Broadband Janus-Helmholtz transducer with various liquid cavity resonances. Appl. Acoust. 2024, 221, 110032. [Google Scholar] [CrossRef]
- Lee, J.; Lee, B.; Baek, K. Estimation of equivalent circuit parameters of underwater acoustic piezoelectric transducer for matching network design of sonar transmitter. J. KIMST 2009, 12, 282–289. [Google Scholar]
- Choi, J.; Mok, H. Simultaneous design of low-pass filter with impedance matching transformer for SONAR transducer using particle swarm optimization. Energies 2019, 12, 4646. [Google Scholar] [CrossRef]
- Manoj, G.; Ramesh, R.; Kundukulam, S.O. Impedance matching network of passive coupler with coaxial cable for airborne sonar applications. Adv. Mil. Technol. 2023, 18, 49–66. [Google Scholar] [CrossRef]
- Shin, C.; Lee, Y.; Ahn, B.; Yoon, H.; Kwon, B.; Kim, K.; Lee, J. Design and output control technique of sonar transmitter considering impedance variation of underwater acoustic transducer. J. ASK 2022, 41, 481–491. [Google Scholar]
- Zhou, H.; Huang, S.H.; Li, W. Electrical impedance matching between piezoelectric transducer and power amplifier. IEEE Sens. J. 2020, 20, 14273–14281. [Google Scholar] [CrossRef]
- Li, J.; Huang, W.; Shao, W.; Li, X.; Sen, Z.; Xu, J.; Shen, J.; Cui, Y. An electrical impedance matching method of dual-frequency transducers for ultrasound internal imaging. Measurement 2023, 221, 113413. [Google Scholar] [CrossRef]
- Tian, W.; Wang, L.; Yu, J.; Lv, Y.; Zhang, S.; Xu, Q.; Gao, B. A hybrid impedance matching network for underwater acoustic transducers. IEEE Trans. Power Electron. 2023, 38, 7622–7632. [Google Scholar] [CrossRef]
- Yu, J.; Tian, W.; Zhang, K.; Liao, S.; Xu, Q. An adaptive broadband active impedance matching network for underwater electroacoustic transduction system. IEEE Trans. Ind. Electron. 2023, 70, 5433–5443. [Google Scholar] [CrossRef]
- Bevilacqua, A.; Mazzanti, A. Doubly-tuned transformer networks: A tutorial. IEEE Trans. Circuits Syst. II 2021, 68, 550–555. [Google Scholar] [CrossRef]
- Bhagavatula, V.; Zhang, T.; Suvarna, A.R.; Rudell, J.C. An ultra-wideband IF millimeter-wave receiver with a 20 GHz channel bandwidth using gain-equalized transformers. IEEE J. Solid-State Circuits 2016, 51, 323–331. [Google Scholar] [CrossRef]
- Poole, C.; Darwazeh, I. Microwave Active Circuit Analysis and Design, 1st ed.; Academic Press: Cambridge, MA, USA, 2015; pp. 51–87. [Google Scholar]
- Irwin, J.D.; Nelms, R.M. Engineering Circuit Analysis, 10th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA; pp. 102–140.
- Mezura-Montes, E.; Coello Coello, C.A. Constraint-handling in nature-inspired numerical optimization: Past, present and future. Swarm Evol. Comput. 2011, 1, 173–194. [Google Scholar] [CrossRef]



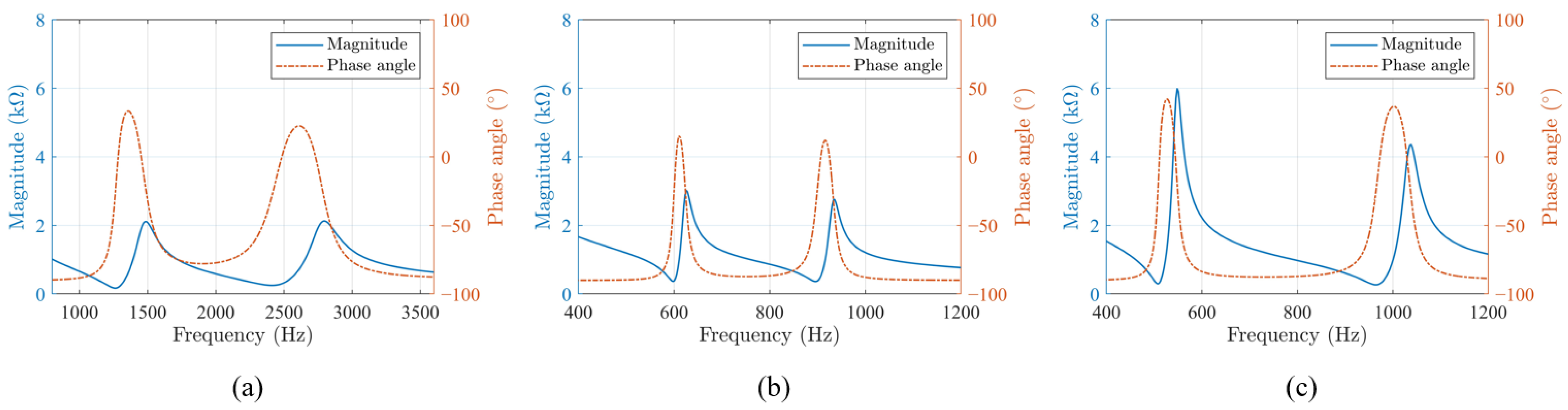
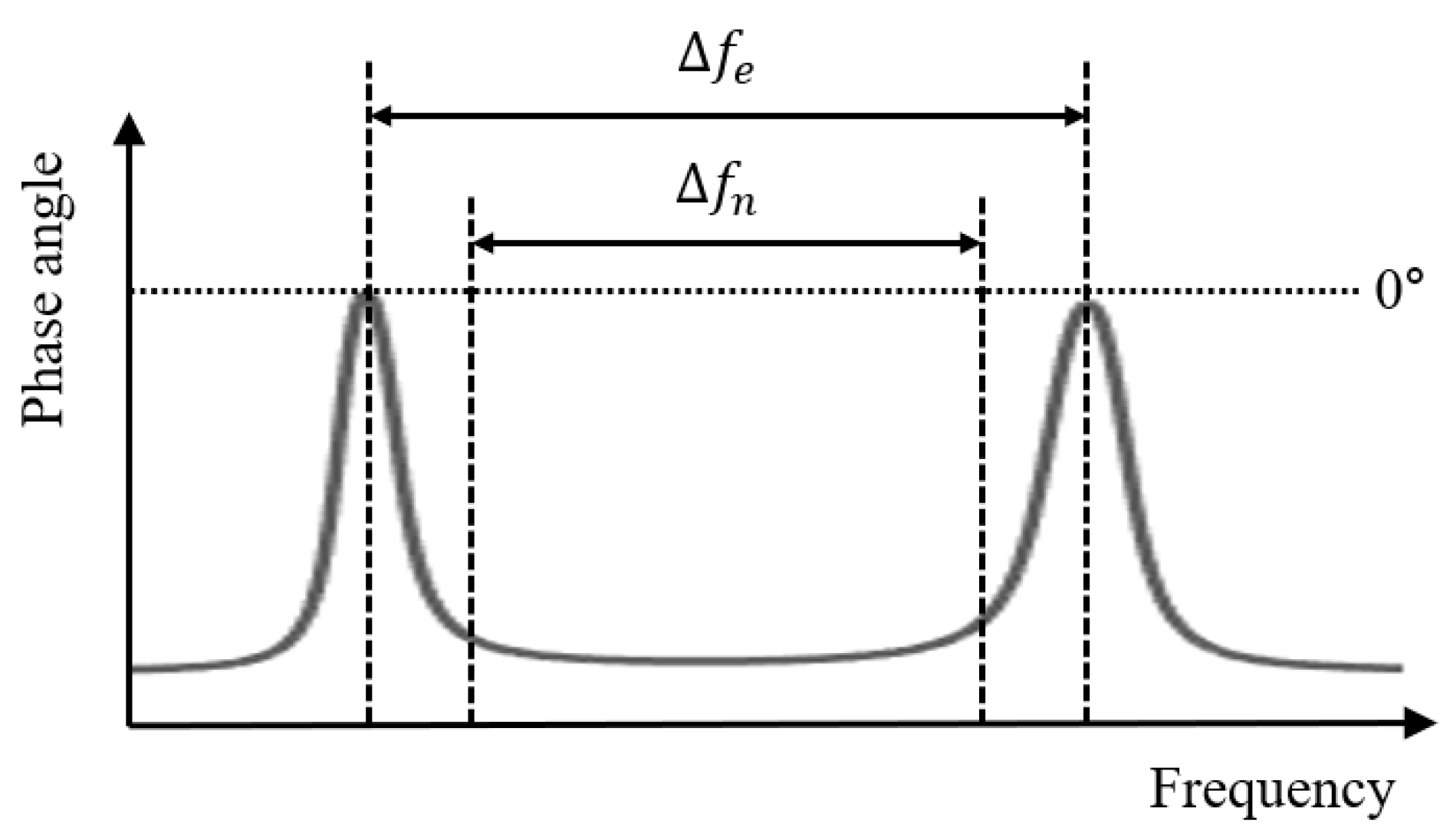
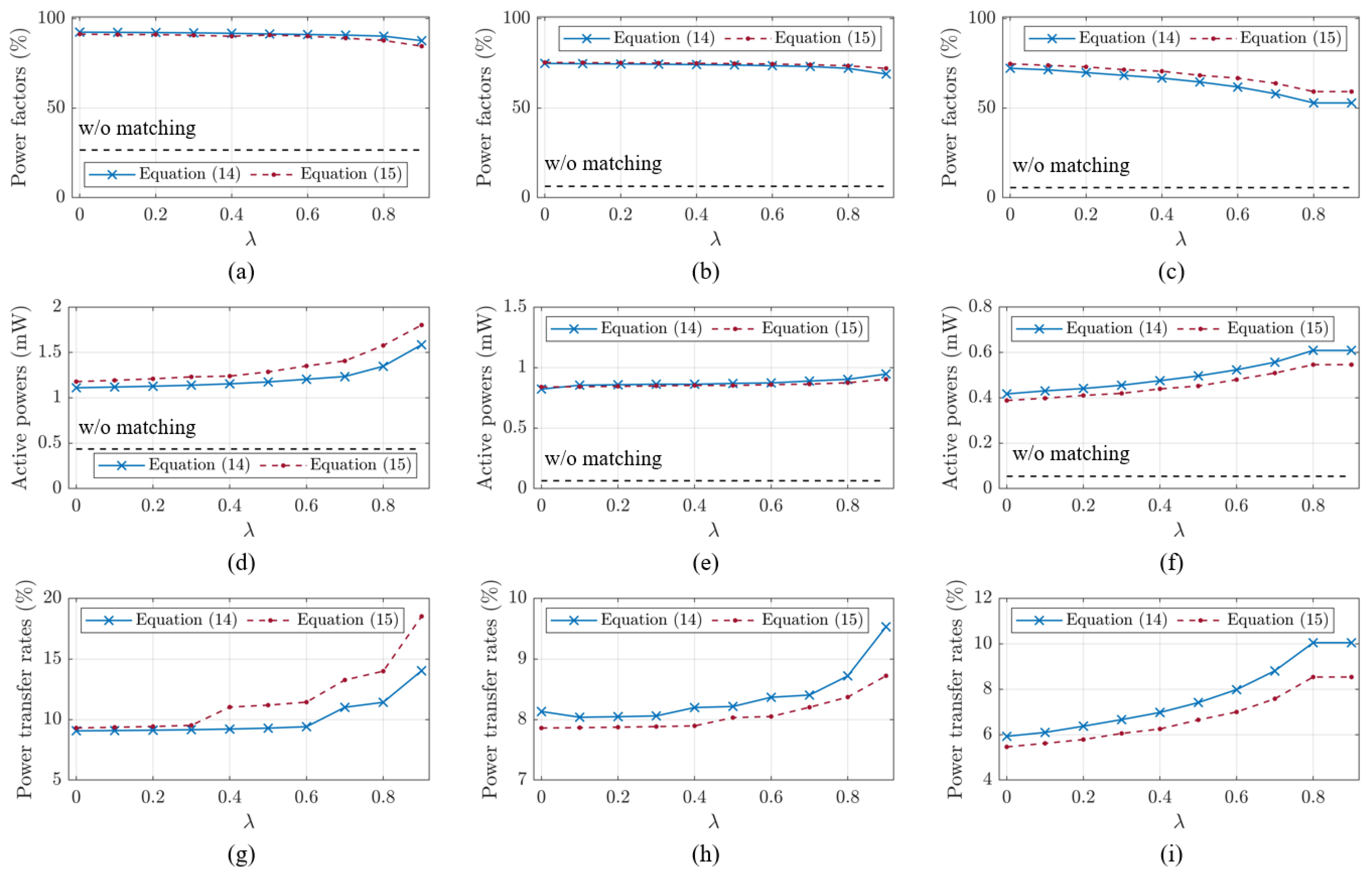
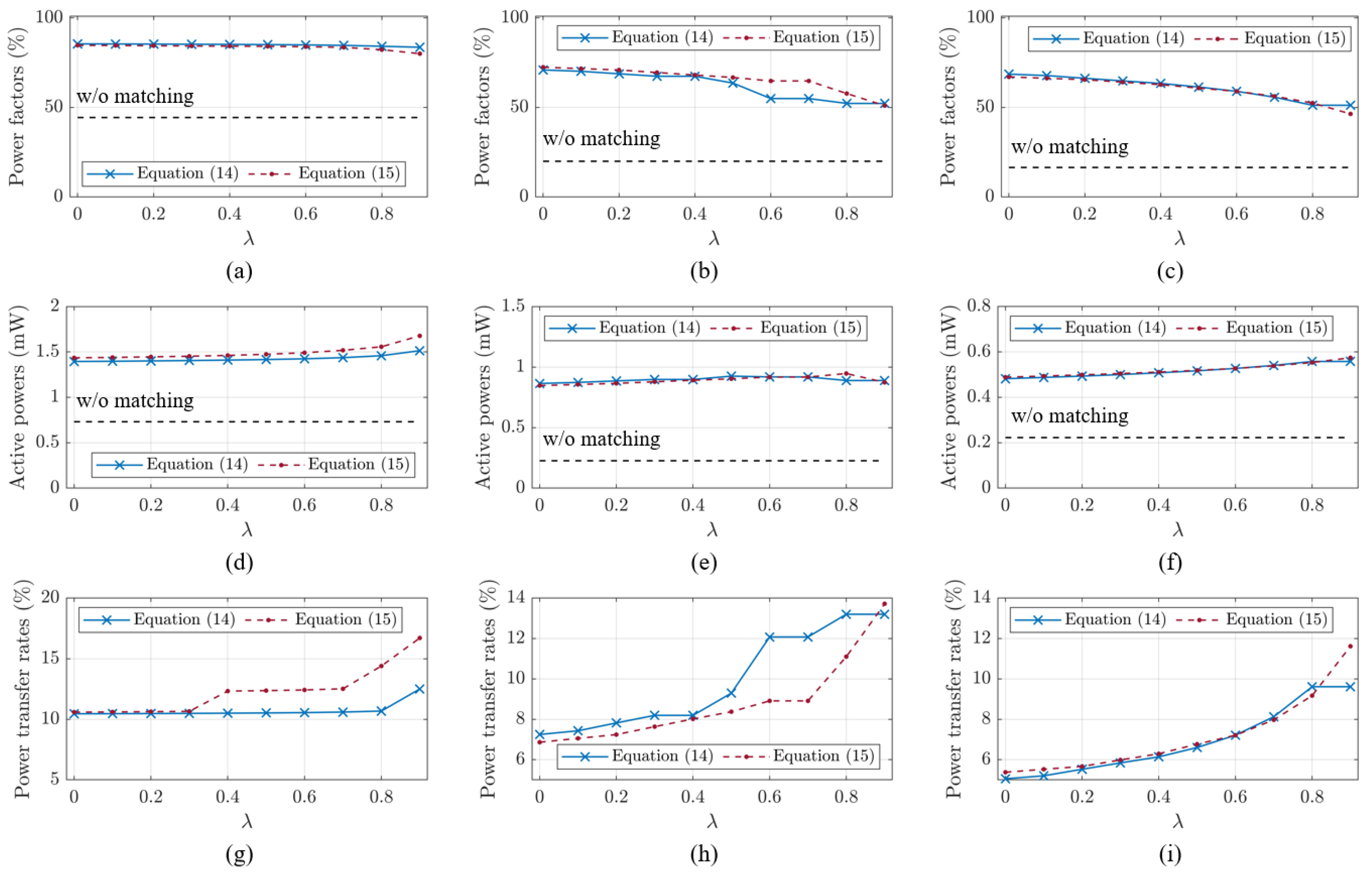


| Category | Passive Circuit [10,11] | Active Circuit [16,17] | Two-Resonant Circuit |
|---|---|---|---|
| Bandwidth | Narrow | Very wide | Wide |
| Design complexity | Low | High | Low |
| Implementation complexity | Low | High | Low |
| External device | Not required | Required | Not required |
| Structural Parameter | Type I [8] | Type II | Type III |
|---|---|---|---|
| Thickness of the tail mass () | 20 | 42 | 42 |
| Radius of the piezoelectric disc () | 28 | 94 | 61 |
| Thickness of the piezoelectric stack () | 150 | 767 | 285 |
| Radius of the head mass () | 95 | 160 | 160 |
| Thickness of the head mass (, ) | 15, 25 | 55, 25 | 55, 25 |
| Radius of the housing () | 97 | 162 | 162 |
| Thickness of the housing () | 15 | 38 | 43 |
| Length of the housing () | 150 | 423 | 456 |
| Band | Type I | Type II | Type III | |
|---|---|---|---|---|
| Frequency range | [1656, 2274] | [667, 848] | [630, 885] | |
| Bandwidth | 618 | 181 | 255 | |
| Frequency range | [1450, 2480] | [620, 905] | [545, 970] | |
| Bandwidth | 1030 | 285 | 425 | |
| Band | Type I | Type II | Type III | ||||
|---|---|---|---|---|---|---|---|
| Active Power | Power Transfer Rate | Active Power | Power Transfer Rate | Active Power | Power Transfer Rate | ||
| 0.0 | 1.17 | 9.3 | 0.82 | 8.1 | 0.41 | 5.9 | |
| 0.9 | 1.80 | 18.5 | 0.95 | 9.5 | 0.61 | 10.1 | |
| 0.0 | 1.43 | 10.5 | 0.84 | 6.8 | 0.48 | 5.3 | |
| 0.9 | 1.67 | 16.7 | 0.95 | 11.1 | 0.57 | 11.6 | |
| Band | Type I | Type II | Type III | ||||
|---|---|---|---|---|---|---|---|
| Active Power | Power Transfer Rate | Active Power | Power Transfer Rate | Active Power | Power Transfer Rate | ||
| 0.0 | 1.35 | 11.9 | 0.69 | 6.9 | 0.45 | 6.4 | |
| 0.9 | 1.80 | 19.1 | 0.91 | 10.4 | 0.58 | 9.3 | |
| 0.0 | 1.53 | 12.9 | 0.81 | 7.5 | 0.48 | 5.5 | |
| 0.9 | 1.79 | 27.8 | 0.84 | 13.7 | 0.50 | 13.0 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, K.; Yim, H.H.; Jeong, Y.; Lee, J.; Lee, C.H. Compact Impedance Matching Circuit for Wideband Power Transfer in Janus Helmholtz Transducers. J. Mar. Sci. Eng. 2025, 13, 898. https://doi.org/10.3390/jmse13050898
Lee K, Yim HH, Jeong Y, Lee J, Lee CH. Compact Impedance Matching Circuit for Wideband Power Transfer in Janus Helmholtz Transducers. Journal of Marine Science and Engineering. 2025; 13(5):898. https://doi.org/10.3390/jmse13050898
Chicago/Turabian StyleLee, Kibae, Hyun Hee Yim, Yoonsang Jeong, Jongkil Lee, and Chong Hyun Lee. 2025. "Compact Impedance Matching Circuit for Wideband Power Transfer in Janus Helmholtz Transducers" Journal of Marine Science and Engineering 13, no. 5: 898. https://doi.org/10.3390/jmse13050898
APA StyleLee, K., Yim, H. H., Jeong, Y., Lee, J., & Lee, C. H. (2025). Compact Impedance Matching Circuit for Wideband Power Transfer in Janus Helmholtz Transducers. Journal of Marine Science and Engineering, 13(5), 898. https://doi.org/10.3390/jmse13050898






