Investigating Morison Modeling of Viscous Forces by Steep Waves on Columns of a Fixed Floating Offshore Wind Turbine (FOWT) Using Computational Fluid Dynamics (CFD)
Abstract
1. Introduction
- Keulegan–Carpenter number (), where is the maximum relative velocity between the body and fluid, and T and are the oscillation period and amplitude, respectively.
- Reynolds number: , where is kinematic viscosity, or .
- Roughness number, , where k is the characteristic roughness size.
- Free-surface effects;
- Truncation, 3D effects;
- Interactions between columns;
- Non-planar velocities due to waves.
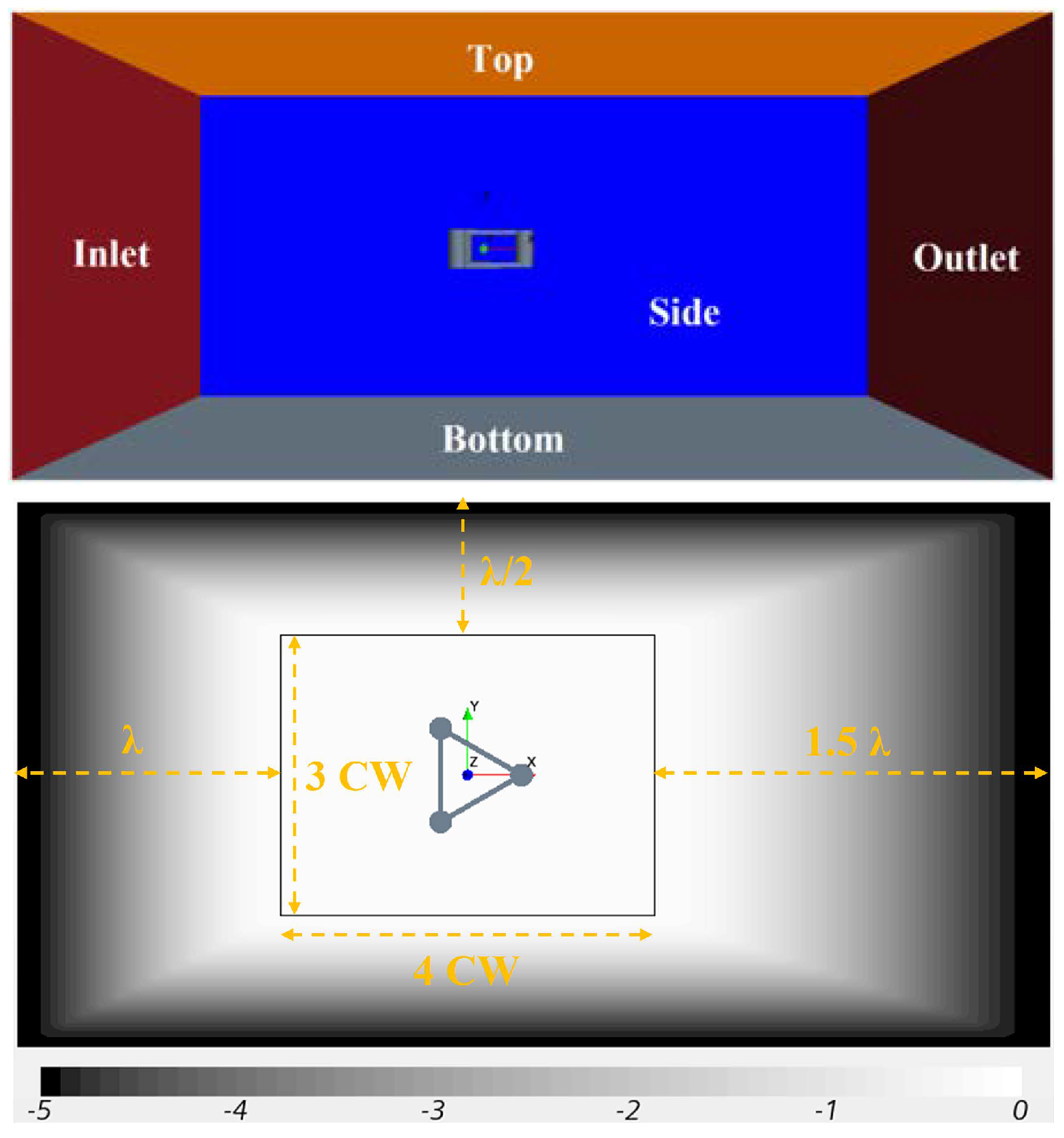
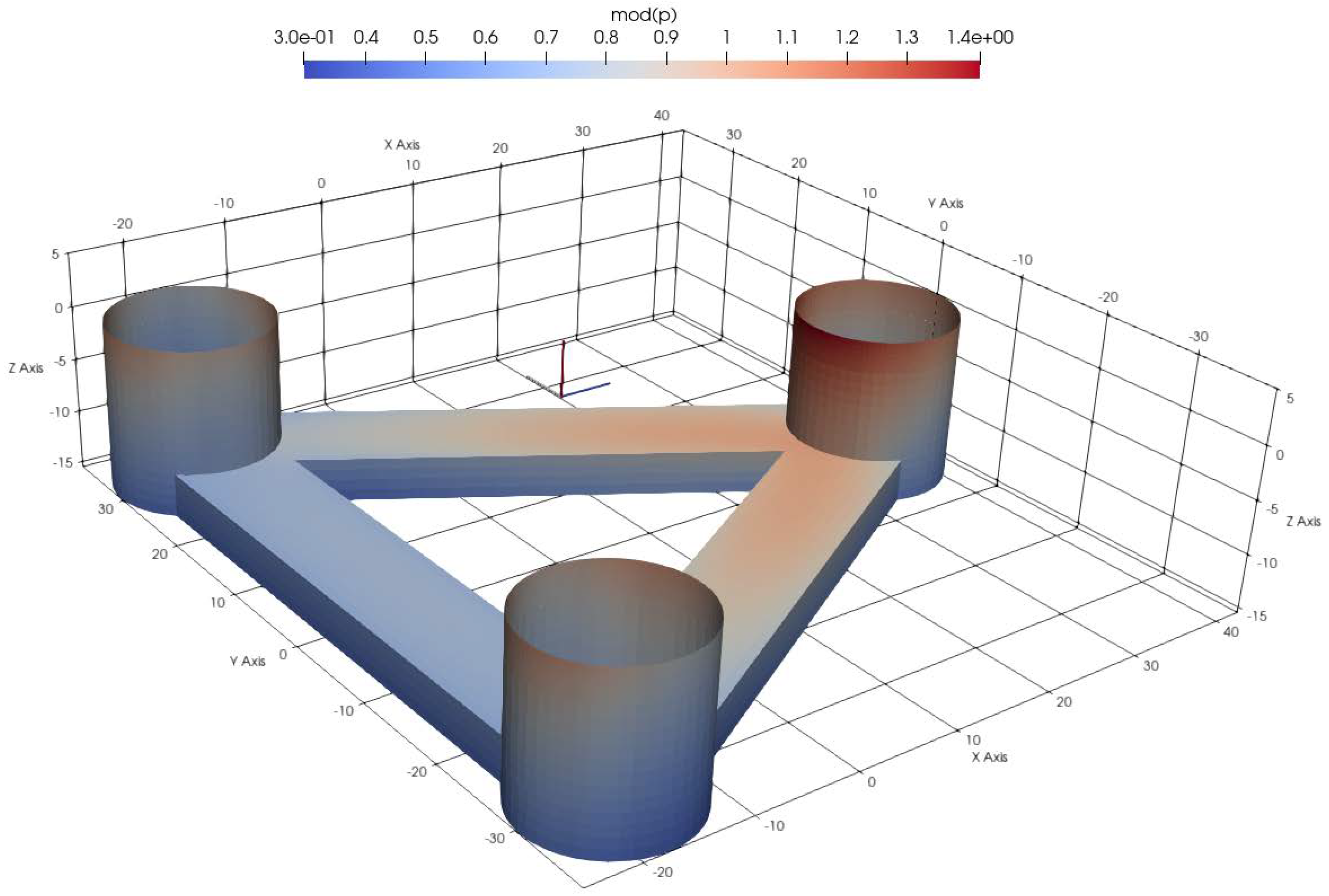
2. Problem Definition
2.1. CFD Numerical Setup
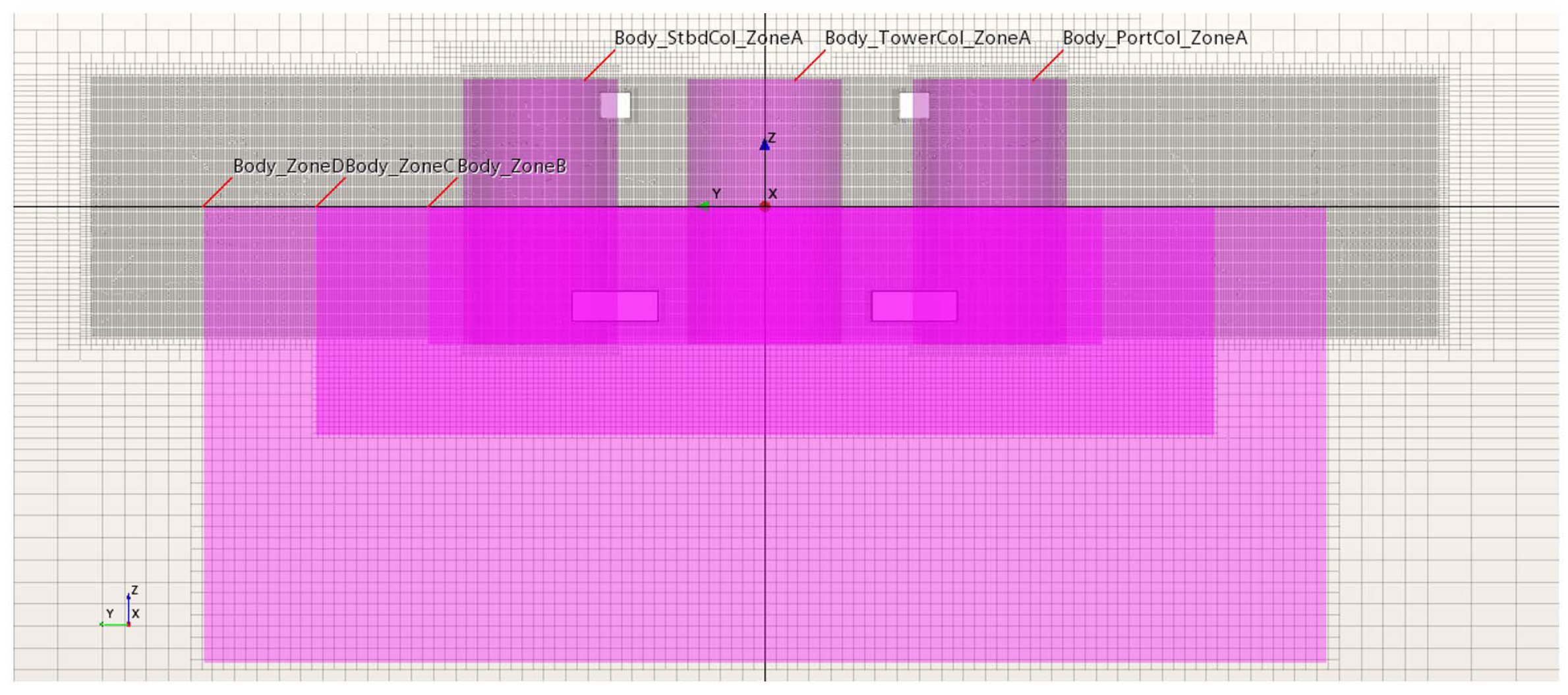
2.2. CFD Mesh
2.3. Force Calculation
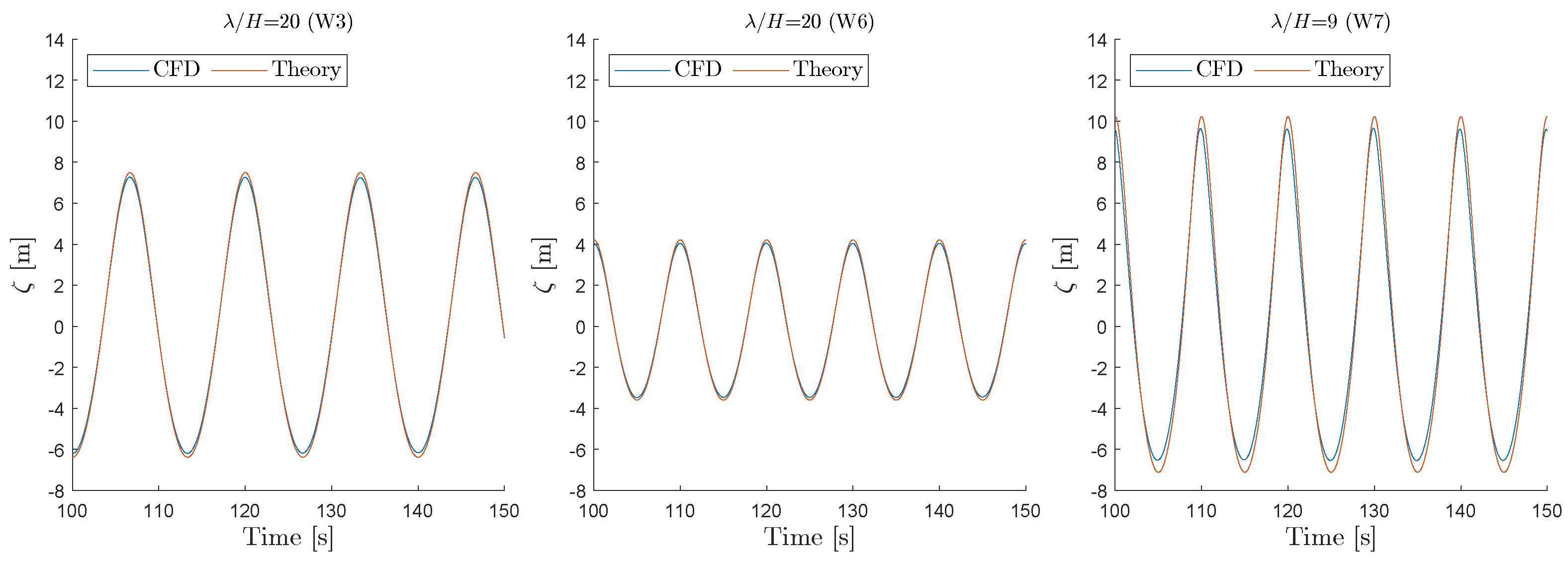
2.4. Wave Kinematics
2.5. Potential Flow Solution
3. Results and Discussions
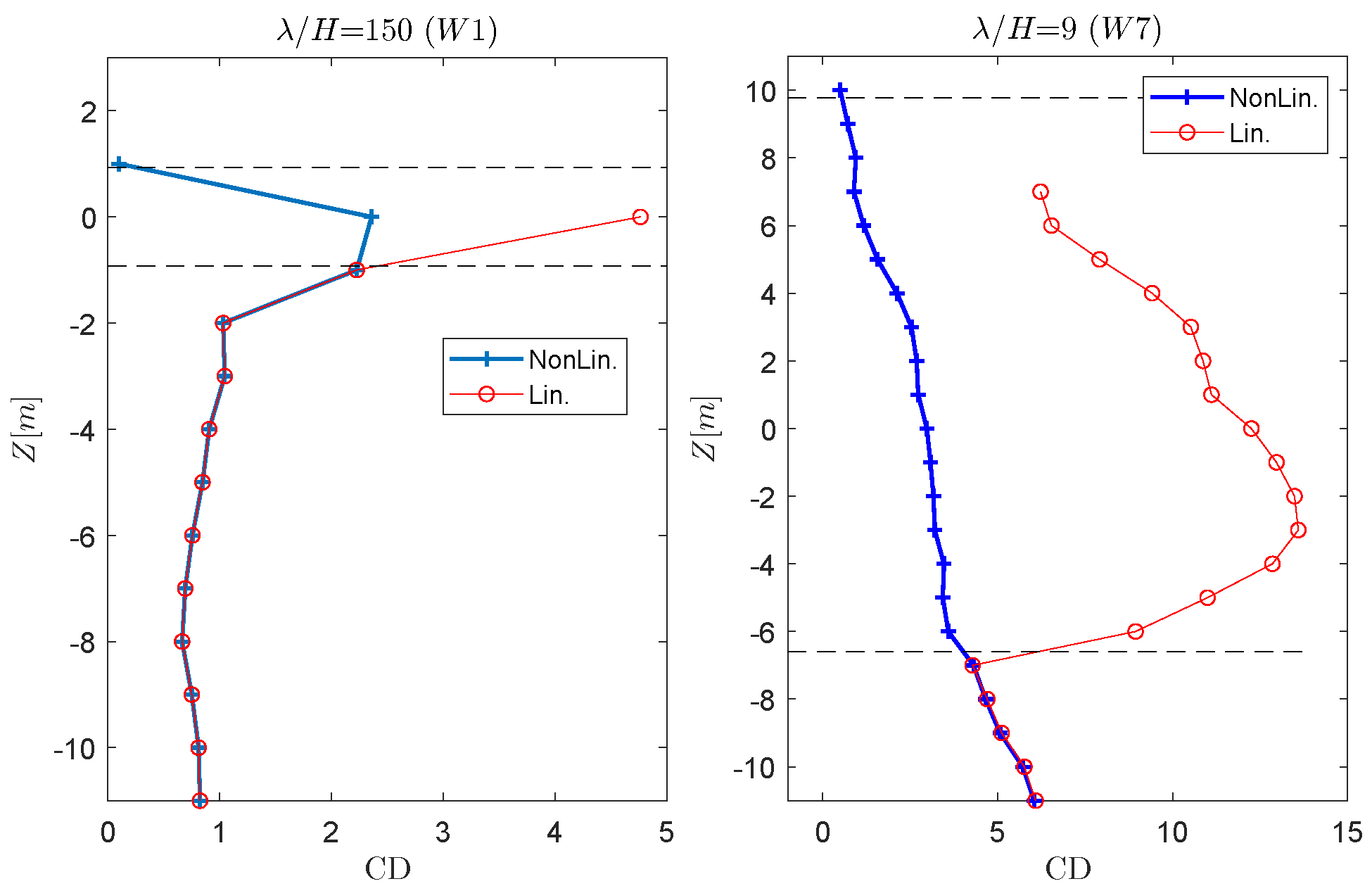
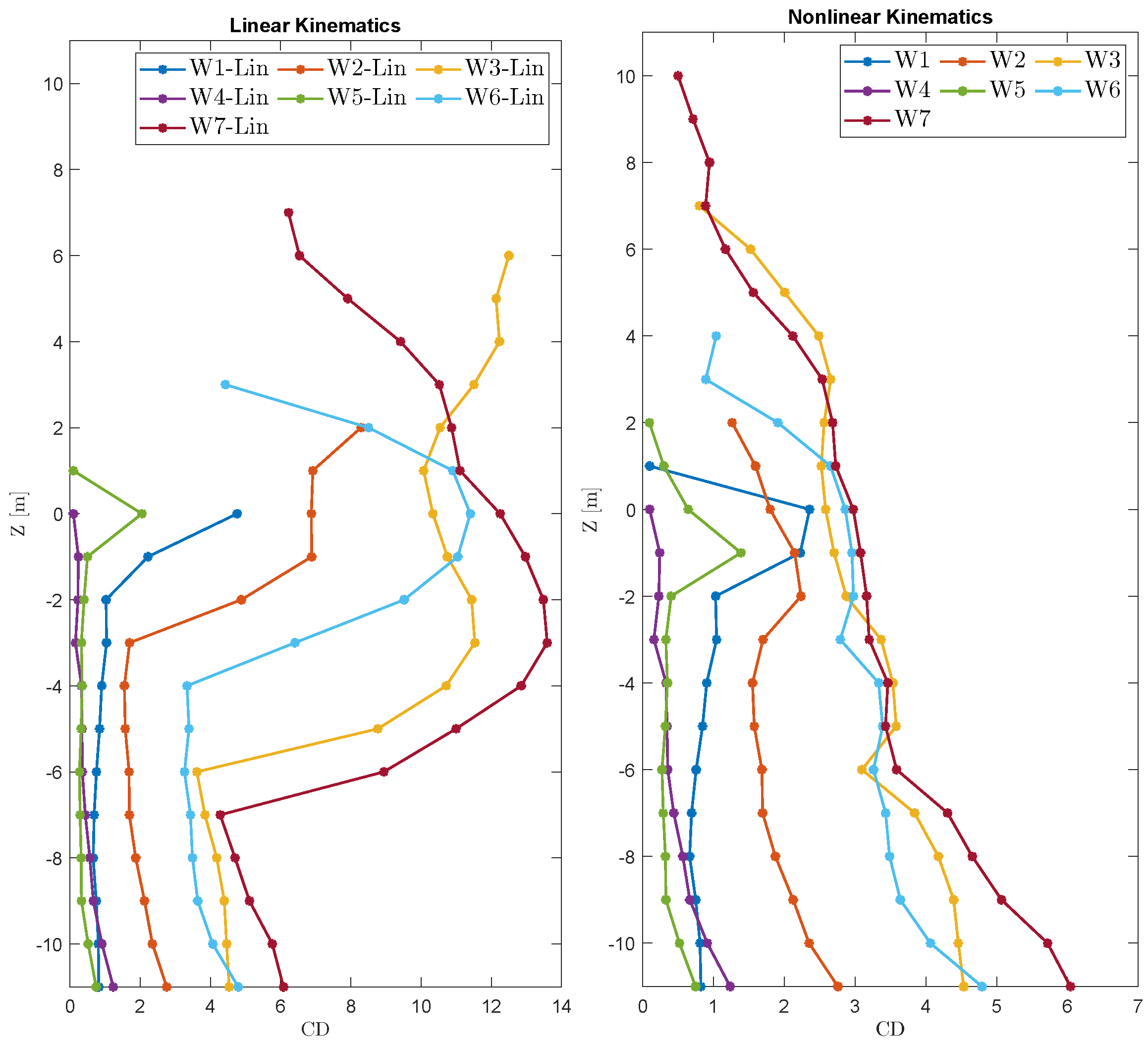
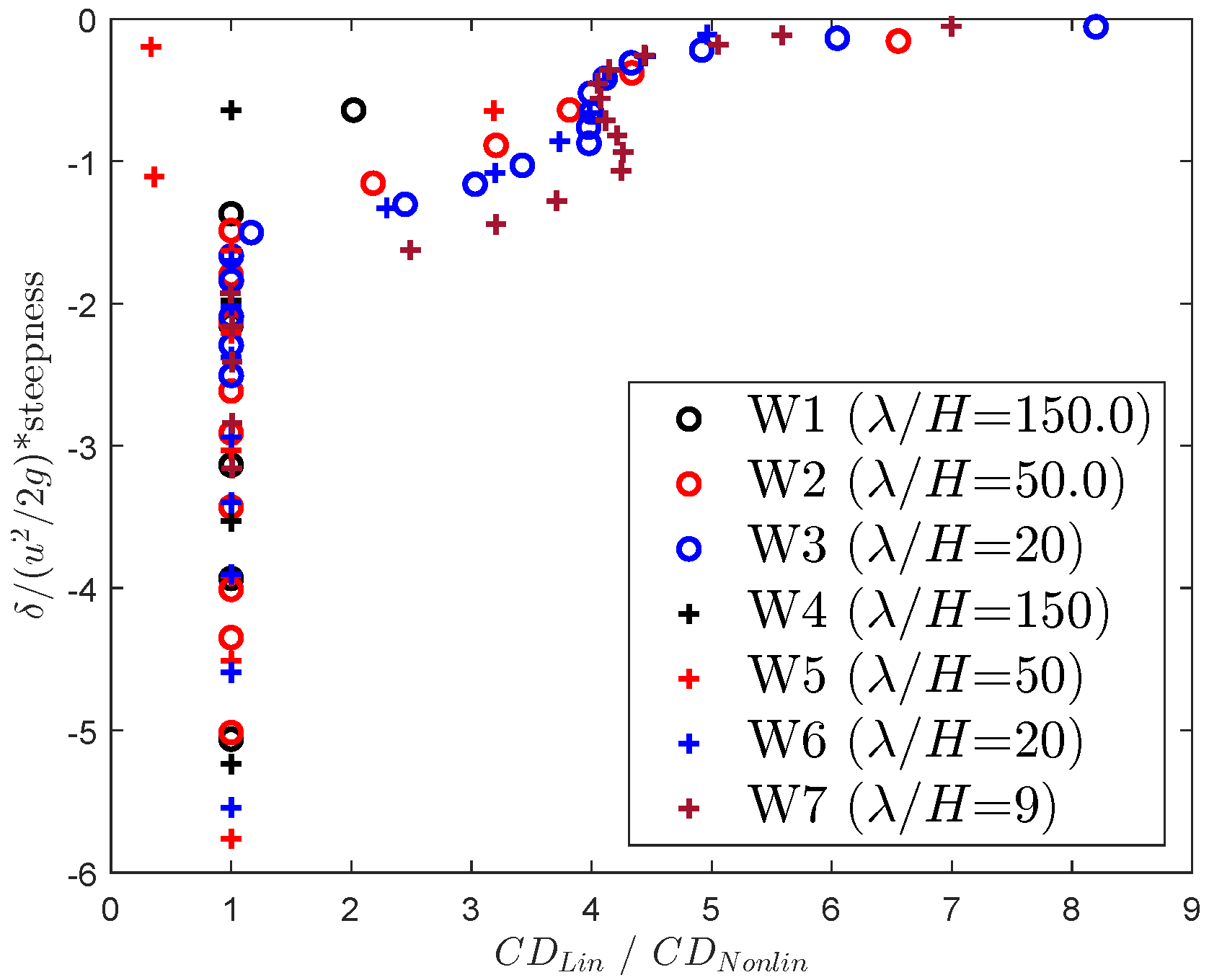
3.1. Drag Coefficients Along the Columns
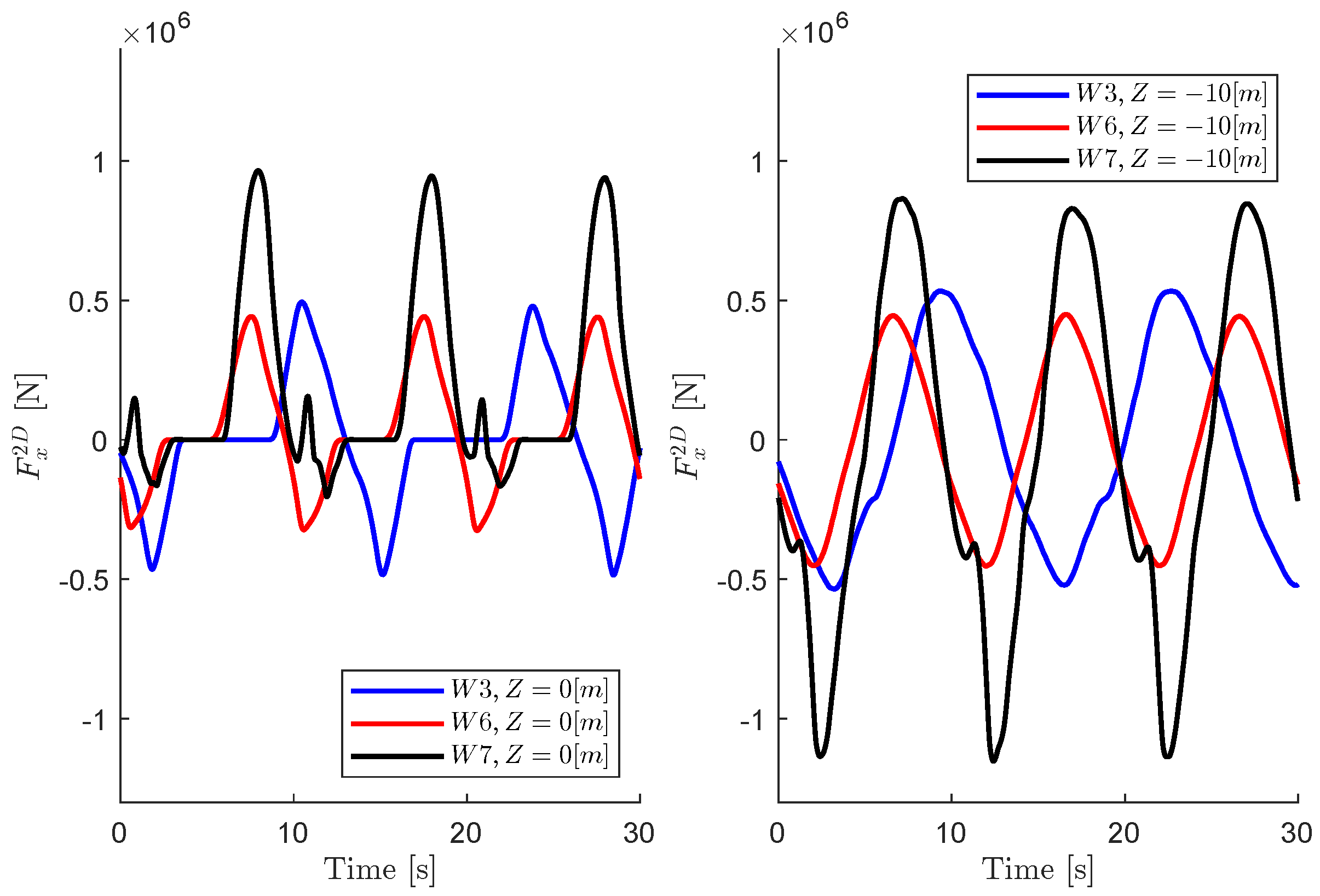
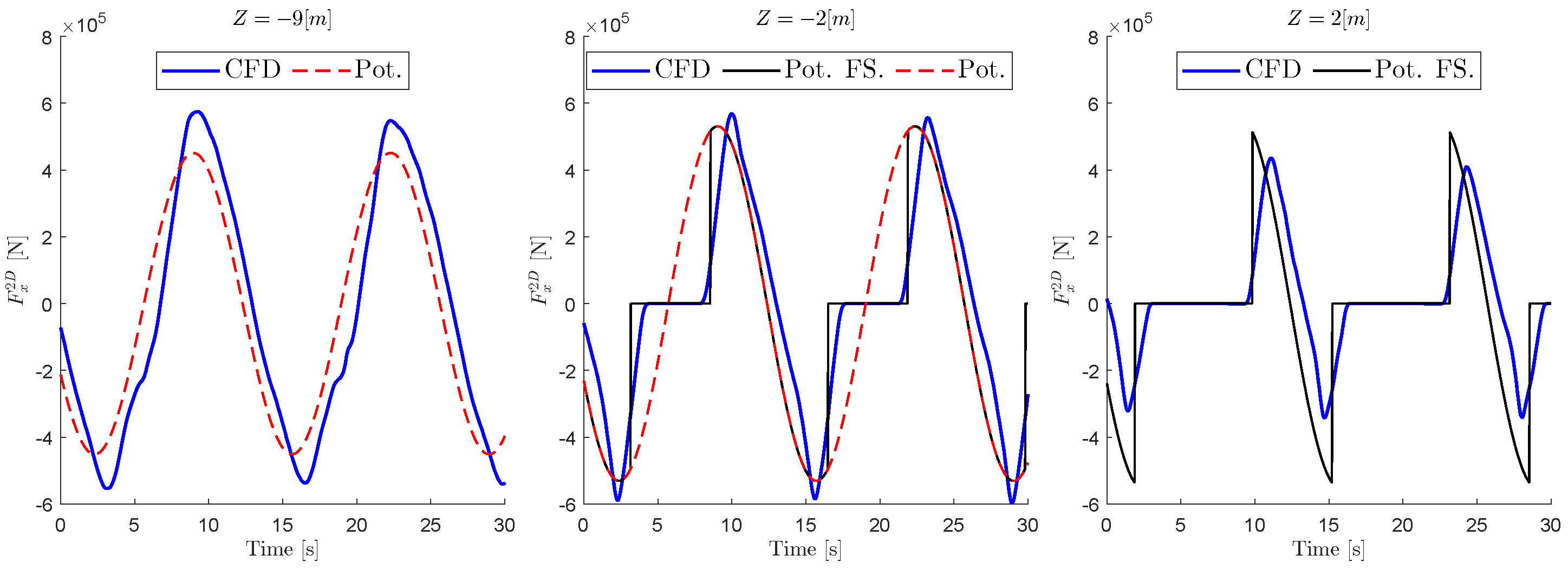
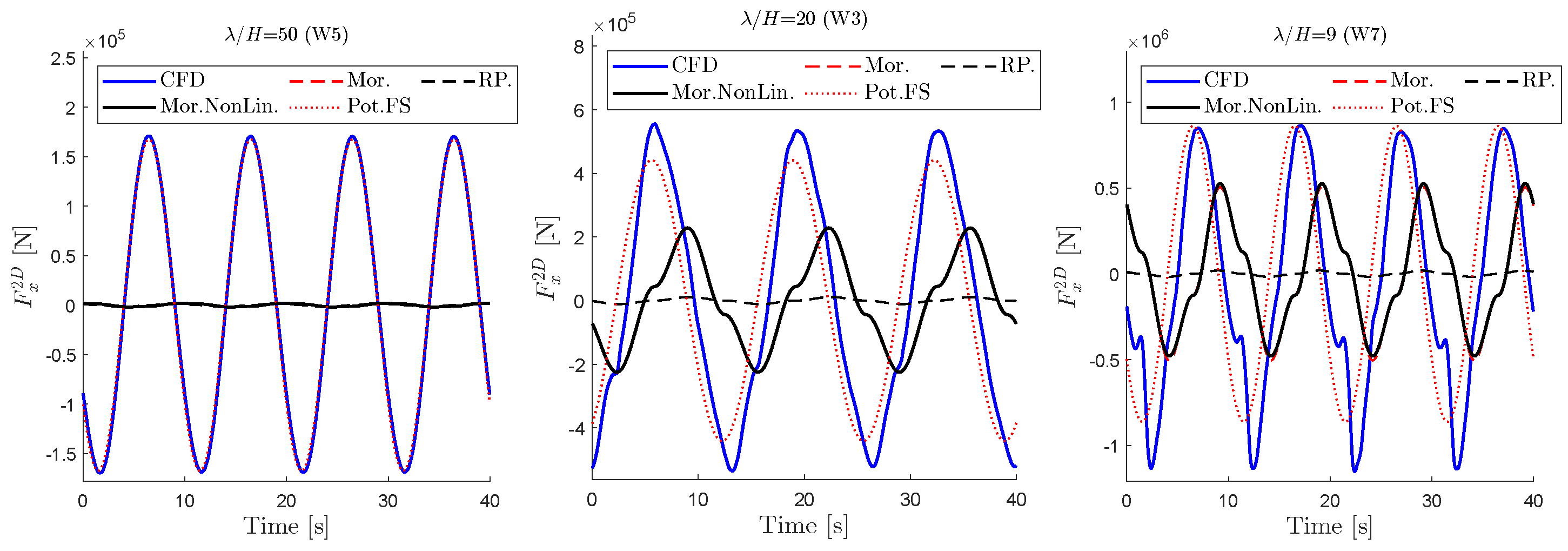
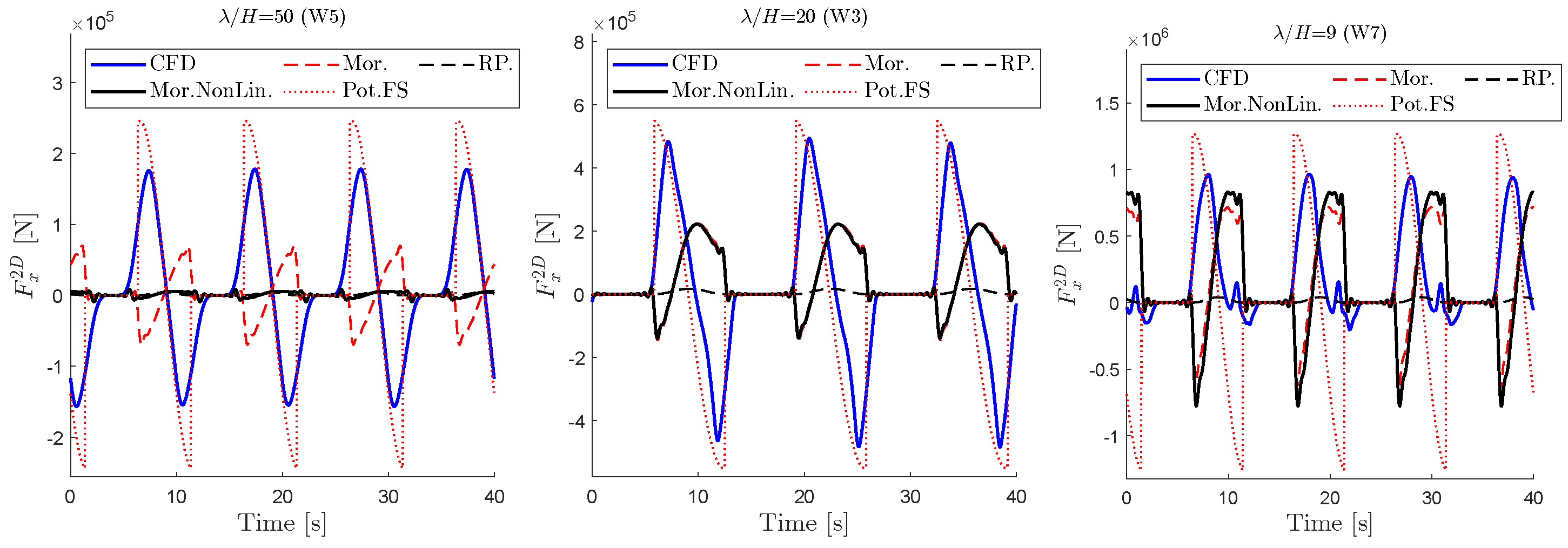
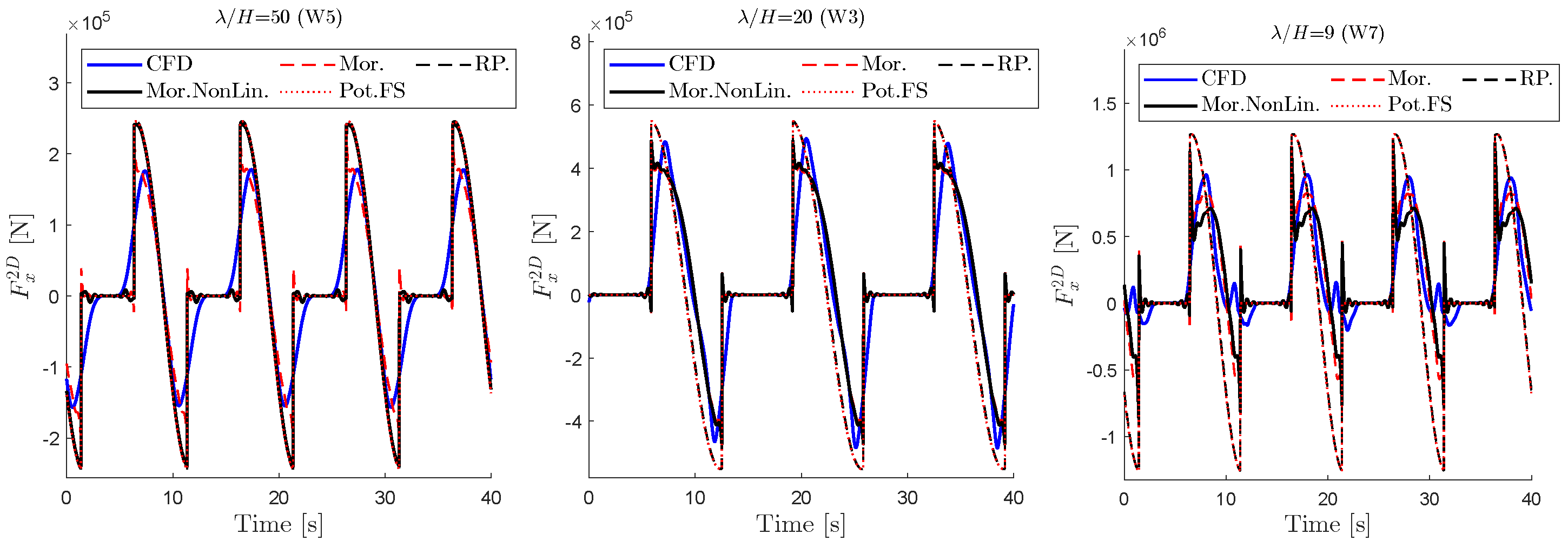
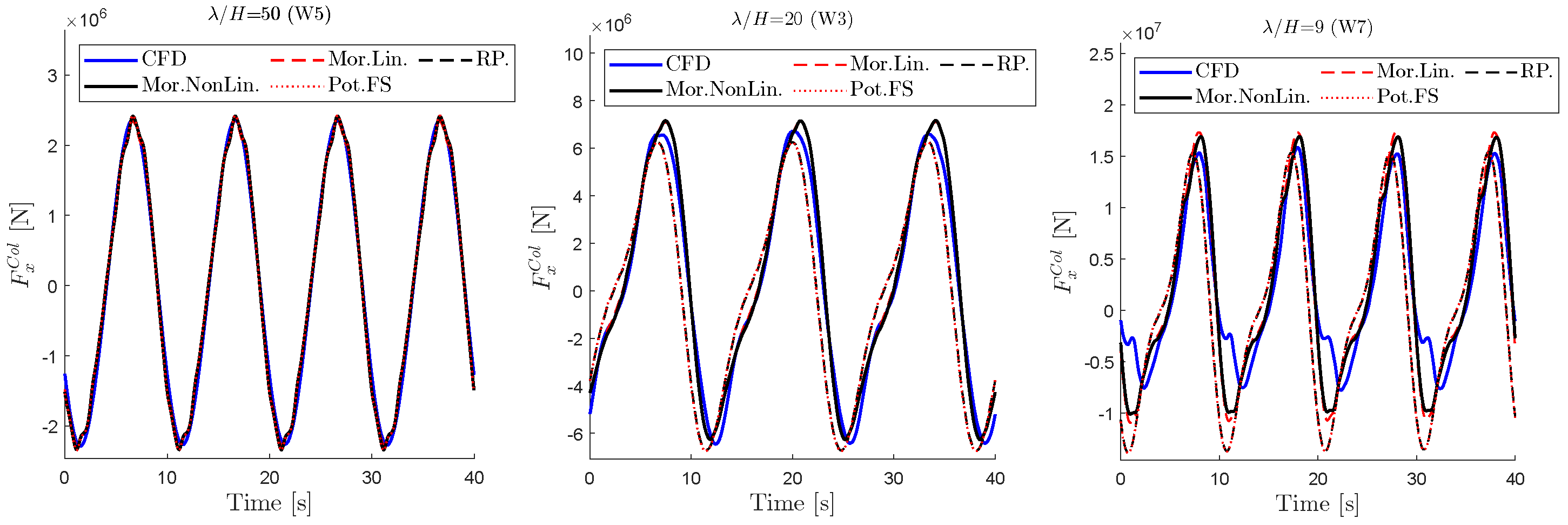
3.2. Reconstructed Forces
- Linear Diffraction (Pot.FS.): Linear diffraction forces calculated using potential flow theory and stretched up to instantaneous free surface.
- Linear Morison (Mor.): The drag coefficient is extracted using linear wave kinematics, and forces are calculated with linear wave kinematics.
- Nonlinear Morison (Mor. NonLin.): The drag coefficient is extracted using nonlinear wave kinematics, and nonlinear wave kinematics are used for calculating forces.
- Linear Morison with Recommended Practice drag coefficient [5] (RP): Linear wave kinematics and the recommended drag coefficient are used to calculate forces.
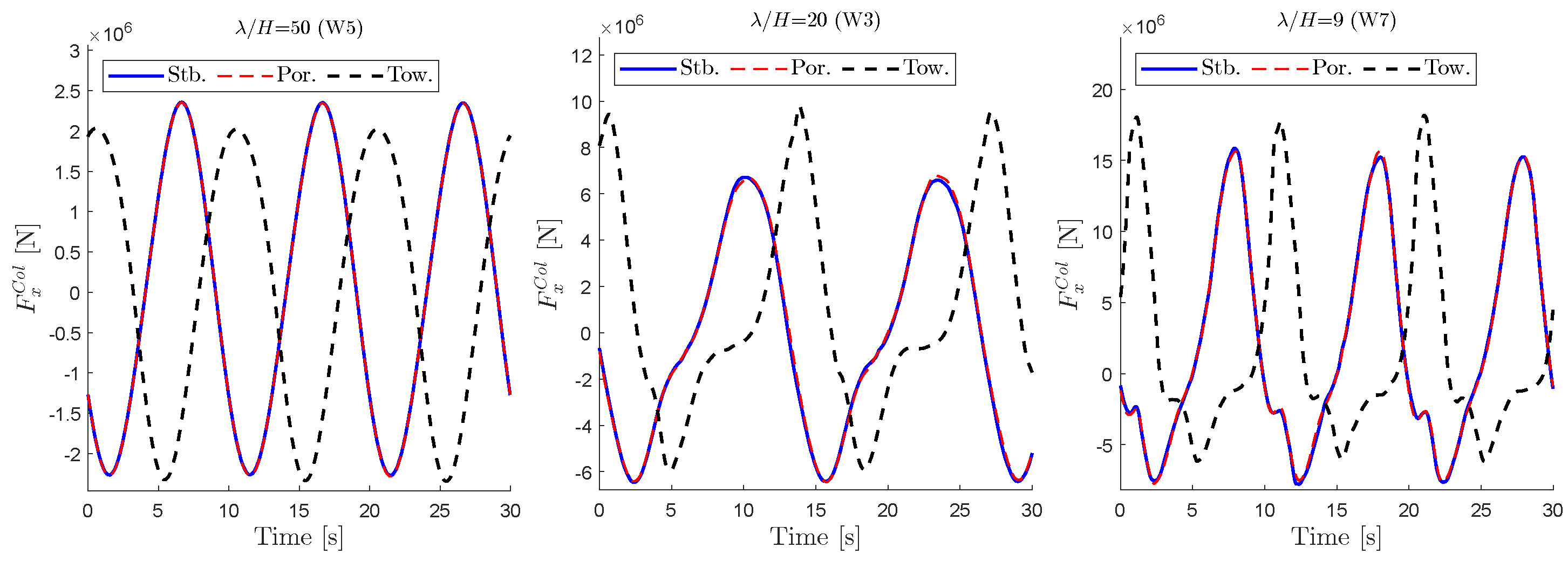
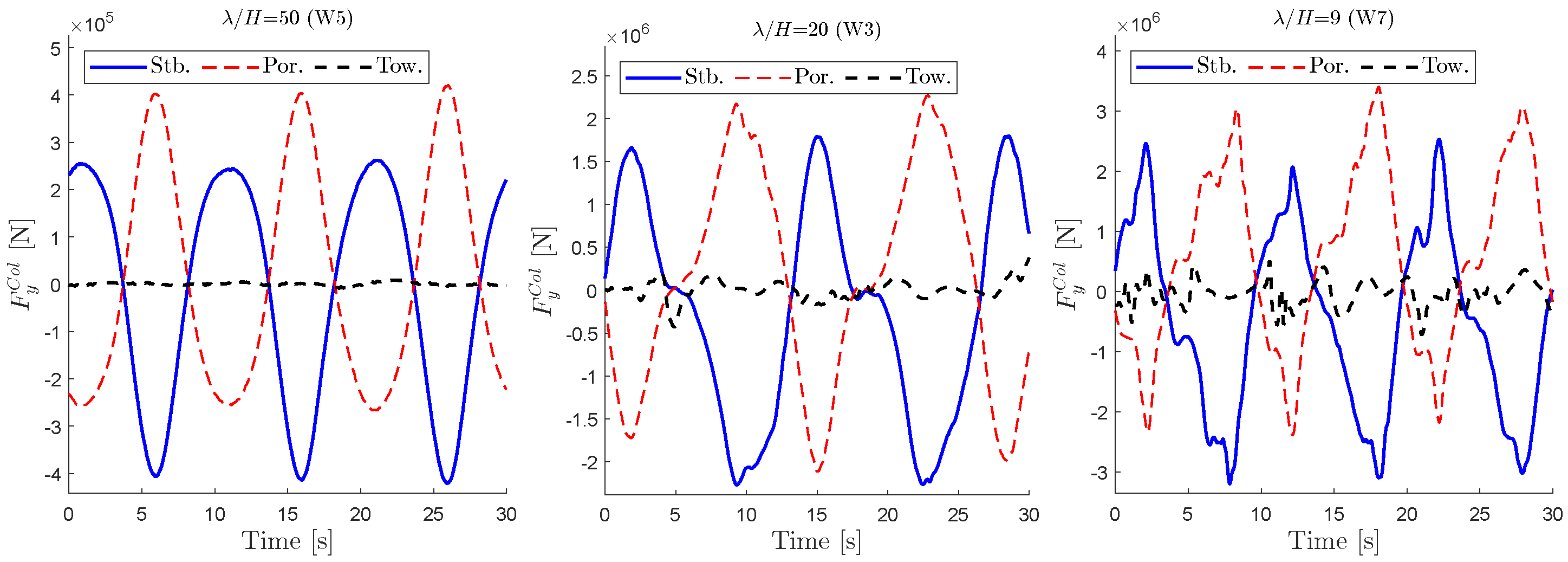
3.3. Interaction Effects
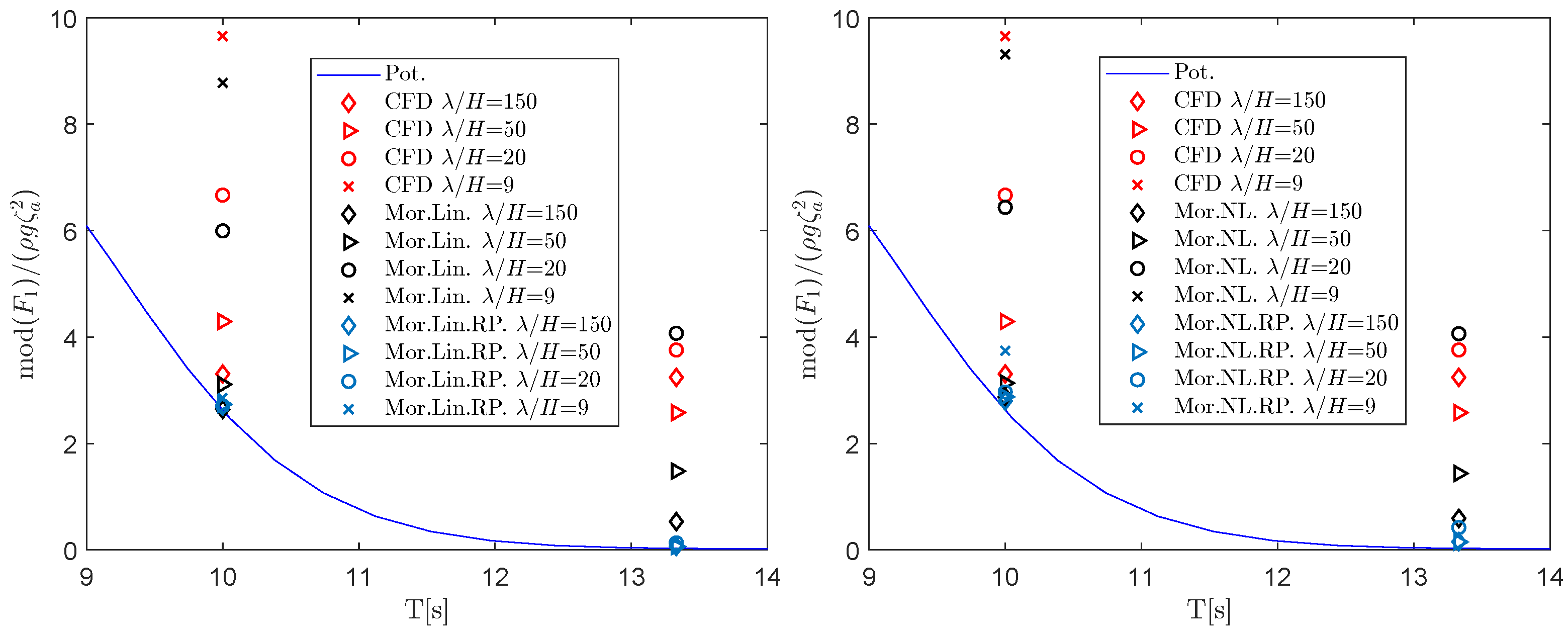
3.4. Drift Forces
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ommani, B.; Fonseca, N.; Stansberg, C.T. Simulation of Low Frequency Motions in Severe Seastates Accounting for Wave-Current Interaction Effects. In Proceedings of the International Conference on Ocean, Offshore and Arctic Engineering OMAE2017, Trondheim, Norway, 25–30 June 2017. [Google Scholar] [CrossRef]
- Wang, L.; Robertson, A.; Jonkman, J.; Yu, Y.H. OC6 phase I: Improvements to the OpenFAST predictions of nonlinear, low-frequency responses of a floating offshore wind turbine platform. Renew. Energy 2022, 187, 282–301. [Google Scholar] [CrossRef]
- Faltinsen, O.M. Sea Loads on Ships and Offshore Structures; Cambridge Ocean Technology Series; University Press: Cambridge, UK, 1990. [Google Scholar]
- Ormberg, H. SIMO-Theory Manual Version 4.0 Rev. 1; Technical Report MT51 F93-0184; SINTEF Ocean: Trondheim, Norway, 2012. [Google Scholar]
- DNV. DNV-RP-C205 Environmental Conditions and Environmental Loads; Technical Report DNV-RP-C205; DET NORSKE VERITAS: Høvik, Norway, 2014. [Google Scholar]
- Sarpkaya, T. In-Line and Transverse Forces on Cylinders in Oscillatory Flow at High Reynolds Numbers. J. Ship Res. 1977, 21, 200–216. [Google Scholar] [CrossRef]
- Bearman, P.W.; Downie, M.J.; Graham, J.M.R.; Obasaju, E.D. Forces on cylinders in viscous oscillatory flow at low Keulegan-Carpenter numbers. J. Fluid Mech. 1985, 154, 337–356. [Google Scholar] [CrossRef]
- Sarpkaya, T. Force on a circular cylinder in viscous oscillatory flow at low Keulegan—Carpenter numbers. J. Fluid Mech. 1986, 165, 61. [Google Scholar] [CrossRef]
- Chaplin, J.R. Loading on a cylinder in uniform oscillatory flow: Part I—Planar oscillatory flow. Appl. Ocean. Res. 1988, 10, 120–128. [Google Scholar] [CrossRef]
- Justesen, P. Hydrodynamic Forces on Large Cylinders in High Reynolds Number Oscillatory Flow. In Proceedings of the Behaviour of Offshore Structures ’88, Trondheim, Norway, 3 June 1988. [Google Scholar]
- Anaturk, A.R. An experimental investigation to measure hydrodynamic forces at small amplitudes and high frequencies. Appl. Ocean. Res. 1991, 13, 200–208. [Google Scholar] [CrossRef]
- Troesch, A.W.; Kim, S.K. Hydrodynamic forces acting on cylinders oscillating at small amplitudes. J. Fluids Struct. 1991, 5, 113–126. [Google Scholar] [CrossRef]
- Bearman, P.W.; Mackwood, P.R. Measurements of the Hydrodynamic Damping of Circular Cylinders. In Proceedings of the Behaviour of Offshore Structures ’92, London, UK, 7–10 July 1992; p. 405. [Google Scholar]
- Chaplin, J.R. Planar Oscillatory Flow Forces at High Reynolds Numbers. J. Offshore Mech. Arct. Eng. 1993, 115, 31–39. [Google Scholar] [CrossRef]
- Gao, Z.; Efthymiou, M.; Cheng, L.; Zhou, T.; Minguez, M.; Zhao, W. Hydrodynamic damping of a circular cylinder at low KC: Experiments and an associated model. Mar. Struct. 2020, 72, 102777. [Google Scholar] [CrossRef]
- Nakamura, M.; Hoshino, K.; Koterayama, W. Three-Dimensional Effects on Hydrodynamic Forces Acting on an Oscillating Finite-Length Circular Cylinder. Int. J. Offshore Polar Eng. 1992, 2, 6. [Google Scholar]
- Vengatesan, V.; Varyani, K.S.; Barltrop, N. An experimental investigation of hydrodynamic coefficients for a vertical truncated rectangular cylinder due to regular and random waves. Ocean. Eng. 2000, 27, 291–313. [Google Scholar] [CrossRef]
- Paulsen, B.T.; Bredmose, H.; Bingham, H.; Jacobsen, N. Forcing of a bottom-mounted circular cylinder by steep regular water waves at finite depth. J. Fluid Mech. 2014, 755, 1–34. [Google Scholar] [CrossRef]
- Clément, C.; Bozonnet, P.; Vinay, G.; Pagnier, P.; Nadal, A.B.; Réveillon, J. Evaluation of Morison approach with CFD modelling on a surface-piercing cylinder towards the investigation of FOWT Hydrodynamics. Ocean. Eng. 2022, 251, 111042. [Google Scholar] [CrossRef]
- Wang, L.; Robertson, A.; Jonkman, J.; Yu, Y.H.; Koop, A.; Borràs Nadal, A.; Li, H.; Bachynski-Polić, E.; Pinguet, R.; Shi, W.; et al. OC6 Phase Ib: Validation of the CFD predictions of difference-frequency wave excitation on a FOWT semisubmersible. Ocean. Eng. 2021, 241, 110026. [Google Scholar] [CrossRef]
- Sarpkaya, T. Structures of separation on a circular cylinder in periodic flow. J. Fluid Mech. 2006, 567, 281–297. [Google Scholar] [CrossRef]
- Kim, J.; O’Sullivan, J.; Read, A. Ringing Analysis of a Vertical Cylinder by Euler Overlay Method; American Society of Mechanical Engineers Digital Collection: New York, NY, USA, 2013; pp. 855–866. [Google Scholar] [CrossRef]
- Bouscasse, B.; Califano, A.; Choi, Y.M.; Haihua, X.; Kim, J.W.; Kim, Y.J.; Lee, S.H.; Lim, H.J.; Park, D.M.; Peric, M.; et al. Qualification Criteria and the Verification of Numerical Waves: Part 2: CFD-Based Numerical Wave Tank; American Society of Mechanical Engineers Digital Collection: New York, NY, USA, 2021. [Google Scholar] [CrossRef]
- Califano, A.; Berthelsen, P.A.; Magalhaes Duque Da Fonseca, N.M. Effect of body motion on the wave loads computed with CFD on the INO-WINDMOOR floater. J. Phys. Conf. Ser. 2023, 2626, 012034. [Google Scholar] [CrossRef]
- WAMIT. User Manual; Technical Report Version 7.4; WAMIT, Inc.: Chestnut Hill, MA, USA, 2020. [Google Scholar]
- Dadmarzi, F.H.; Califano, A.; Fonseca, N.; Berthelsen, P.A. Comparison of Morison Forces with CFD Modelling for a Surface Piercing Column of a FOWT. In Proceedings of the ASME 2022 International Offshore Wind Technical Conference IOWTC2022, Boston, MA, USA, 7–8 December 2022. [Google Scholar]
- Dean, R.G.; Dalrymple, R.A.; Hudspeth, R.T. Force Coefficients from Wave Project I and II Data Including Free Surface Effects. Soc. Pet. Eng. J. 1981, 21, 779–786. [Google Scholar] [CrossRef]
- Berthelsen, P.A.; Baarholm, R.; Pa´kozdi, C.; Stansberg, C.T.; Hassan, A.; Downie, M.; Incecik, A. Viscous Drift Forces and Responses on a Semisubmersible Platform in High Waves. In Proceedings of the Volume 1: Offshore Technology, Honolulu, HI, USA, 31 May–5 June 2009; pp. 469–478. [Google Scholar] [CrossRef]
- Kristiansen, T.; Faltinsen, O.M. Higher harmonic wave loads on a vertical cylinder in finite water depth. J. Fluid Mech. 2017. [Google Scholar] [CrossRef]
- Fonseca, N.; Stansberg, C.T. Wave Drift Forces and Low Frequency Damping on the Exwave Semi-Submersible. In Proceedings of the Volume 1: Offshore Technology, Trondheim, Norway, 25–30 June 2017; p. V001T01A085. [Google Scholar] [CrossRef]





| Article | -Range | -Range | |
|---|---|---|---|
| Sarpkaya (1977) [6] | 2–100 | 497–5260 | smooth, 1/100, 1/800 |
| Bearman et al. (1985) [7] | 0.2–10 | 196–1665 | smooth |
| Sarpkaya (1986) [8] | 0.4–25 | 1035–11,240 | smooth-1/100 |
| Chaplin (1988) [9] | 4–20 | 2000–28,000 | smooth |
| Justesen (1988) [10] | 1–20 | – | smooth, 1/50 |
| Anaturk (1991) [11] | 0.1–10 | 29,000–136,000 | smooth-1/50 |
| Troesch and Kim (1991) [12] | 0.1–1.0 | 23,200–48,600 | smooth |
| Bearman and Mackwood (1992) [13] | 0.1–3.0 | < | smooth, 1/50 |
| Chaplin (1993) [14] | 5–25 | – | |
| Gao et al. (2020) [15] | 0.04–5.0 | 20,950 | smooth |
| Platform Height | () | 31 m |
| Column Diameter | (D) | 15 m |
| Column-Column Distance | () | 61 m |
| Draft | 15.5 m | |
| Pontoon Width | 10 m | |
| Pontoon Height | 4 m | |
| Deck beams Width | 3.5 m |
| Wave | H [m] | T [s] | ||||
|---|---|---|---|---|---|---|
| ID | Nominal | |||||
| W1 | 1.85 | 13.33 | 150 | 18.50 | 0.38 | 1.69 |
| W2 | 5.55 | 13.33 | 50 | 18.50 | 1.13 | 1.69 |
| W3 | 13.88 | 13.33 | 20 | 18.50 | 2.77 | 1.69 |
| W4 | 1.04 | 10 | 150 | 10.41 | 0.21 | 2.25 |
| W5 | 3.12 | 10 | 50 | 10.41 | 0.62 | 2.25 |
| W6 | 7.81 | 10 | 20 | 10.41 | 1.55 | 2.25 |
| W7 | 17.35 | 10 | 9 | 10.41 | 3.17 | 2.25 |
| Base Size Ratio | Full Scale | Model Scale | |
|---|---|---|---|
| % | [m] | [m] | |
| Base | 100% | 3 | 0.075 |
| Free Surface | 2.7% | 0.08 | 0.002 |
| Columns | 2.7% | 0.08 | 0.002 |
| Pontoons | 5.3% | 0.16 | 0.004 |
| Deck beams | 10.7% | 0.32 | 0.008 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dadmarzi, F.H.; Ommani, B.; Califano, A.; Fonseca, N.; Berthelsen, P.A. Investigating Morison Modeling of Viscous Forces by Steep Waves on Columns of a Fixed Floating Offshore Wind Turbine (FOWT) Using Computational Fluid Dynamics (CFD). J. Mar. Sci. Eng. 2025, 13, 264. https://doi.org/10.3390/jmse13020264
Dadmarzi FH, Ommani B, Califano A, Fonseca N, Berthelsen PA. Investigating Morison Modeling of Viscous Forces by Steep Waves on Columns of a Fixed Floating Offshore Wind Turbine (FOWT) Using Computational Fluid Dynamics (CFD). Journal of Marine Science and Engineering. 2025; 13(2):264. https://doi.org/10.3390/jmse13020264
Chicago/Turabian StyleDadmarzi, Fatemeh Hoseini, Babak Ommani, Andrea Califano, Nuno Fonseca, and Petter Andreas Berthelsen. 2025. "Investigating Morison Modeling of Viscous Forces by Steep Waves on Columns of a Fixed Floating Offshore Wind Turbine (FOWT) Using Computational Fluid Dynamics (CFD)" Journal of Marine Science and Engineering 13, no. 2: 264. https://doi.org/10.3390/jmse13020264
APA StyleDadmarzi, F. H., Ommani, B., Califano, A., Fonseca, N., & Berthelsen, P. A. (2025). Investigating Morison Modeling of Viscous Forces by Steep Waves on Columns of a Fixed Floating Offshore Wind Turbine (FOWT) Using Computational Fluid Dynamics (CFD). Journal of Marine Science and Engineering, 13(2), 264. https://doi.org/10.3390/jmse13020264








