Sliding Mode Control for Variable-Speed Trajectory Tracking of Underactuated Vessels with TD3 Algorithm Optimization
Abstract
1. Introduction
2. Problem Formulation and Preliminaries
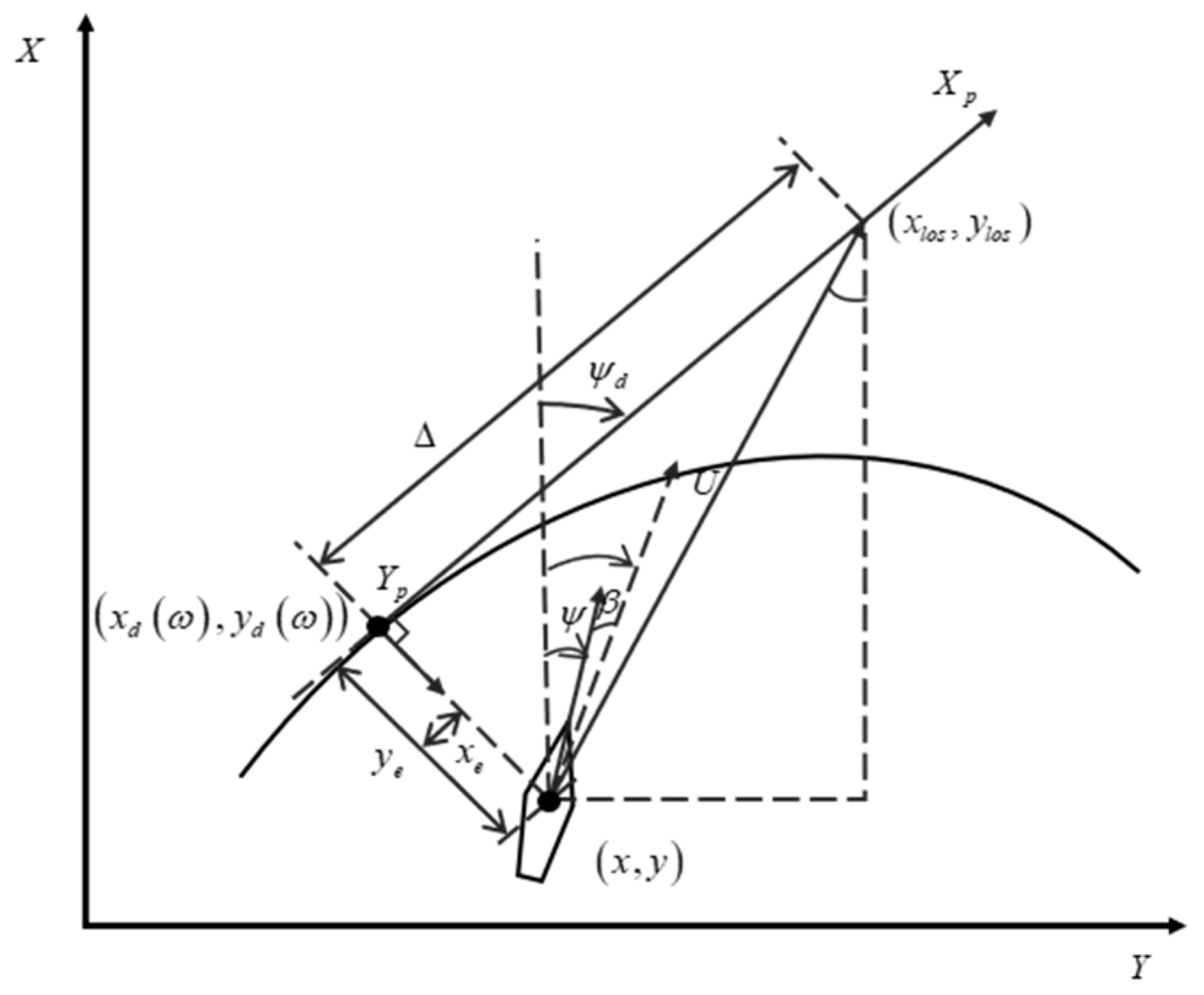
2.1. Problem Formulation
2.2. Preliminaries
3. Adaptive Sliding Mode Controller
3.1. Extended State Observer (ESO)
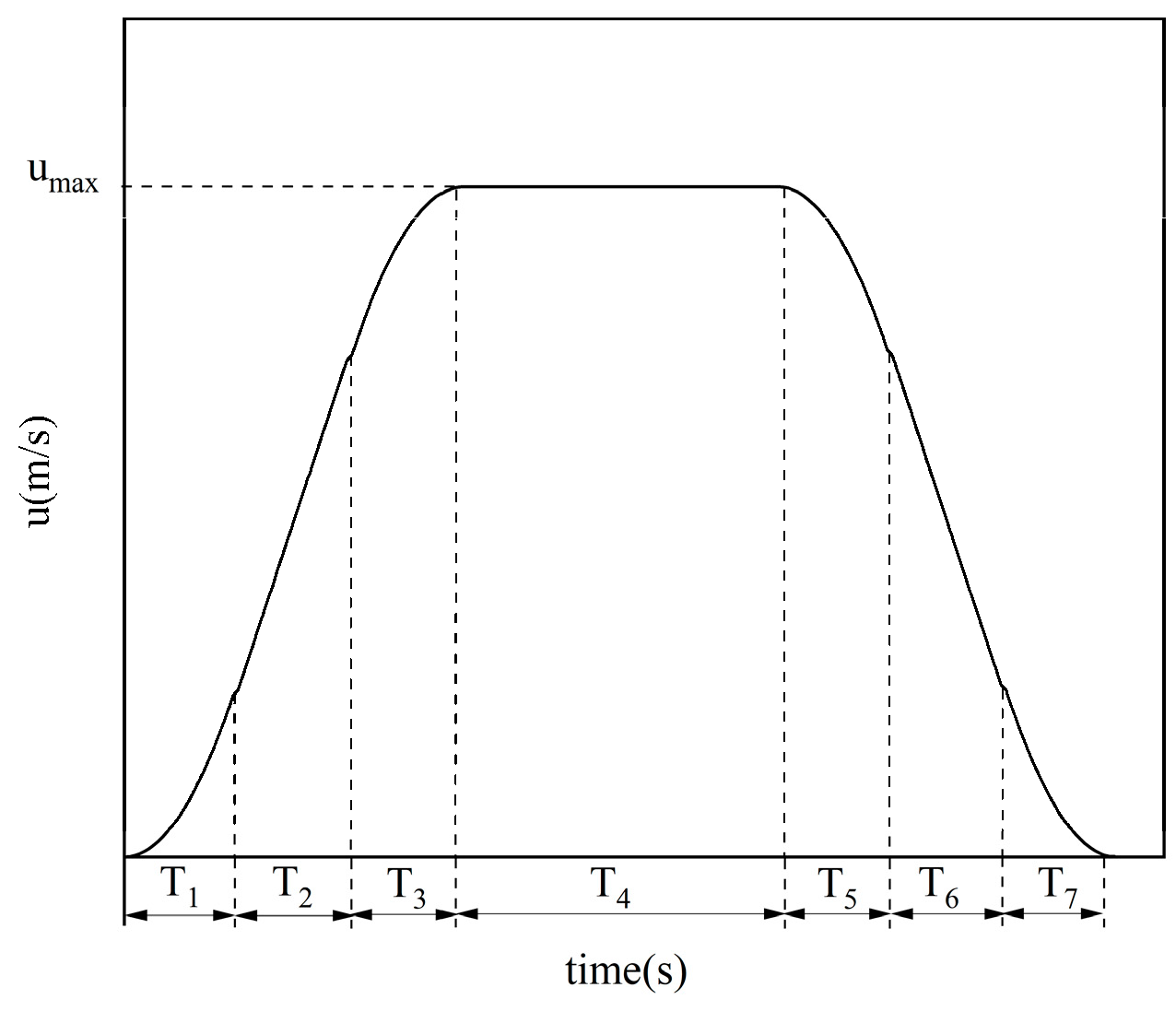
3.2. Heading and Speed Sliding Mode Control
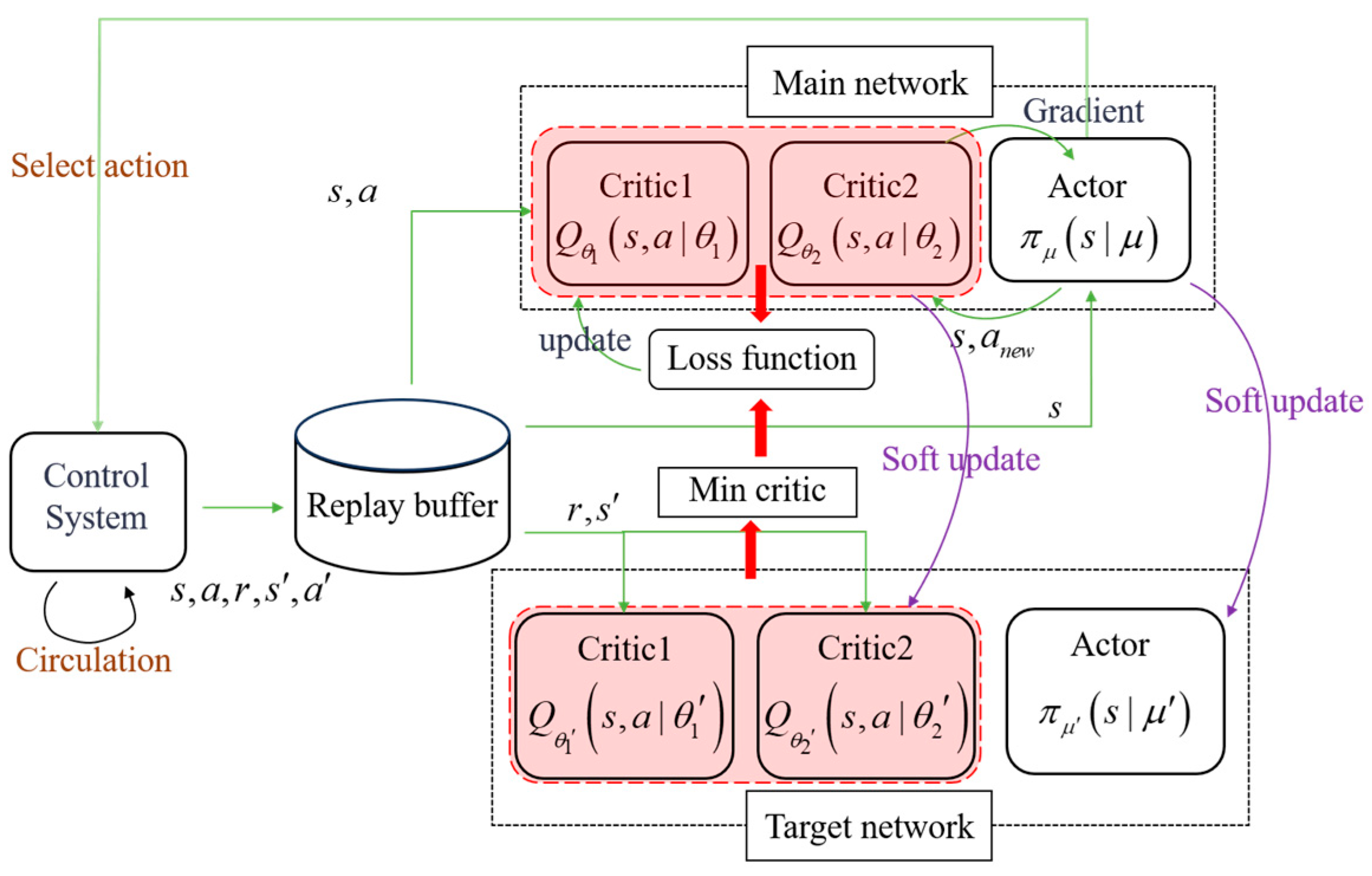
3.3. TD3 Algorithm Parameter Optimization
4. System Control Structure
5. Simulation Results
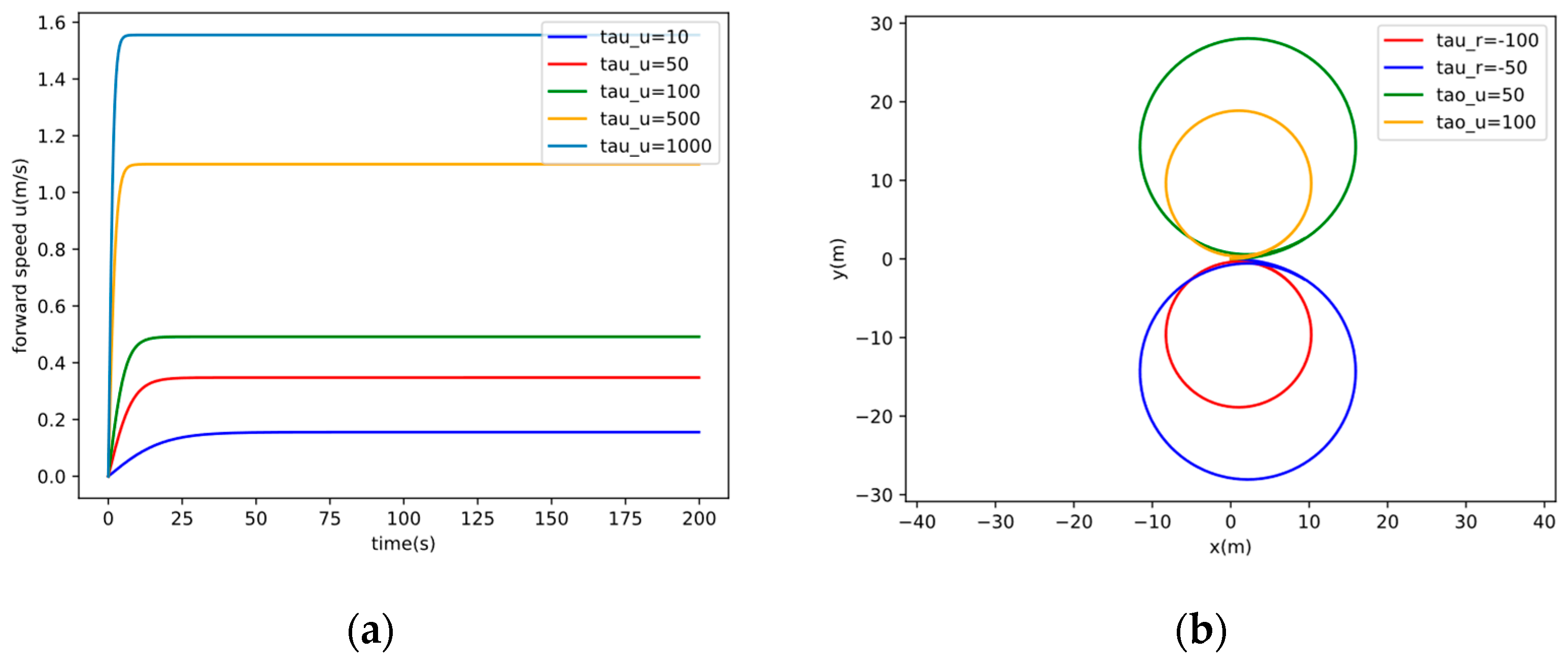
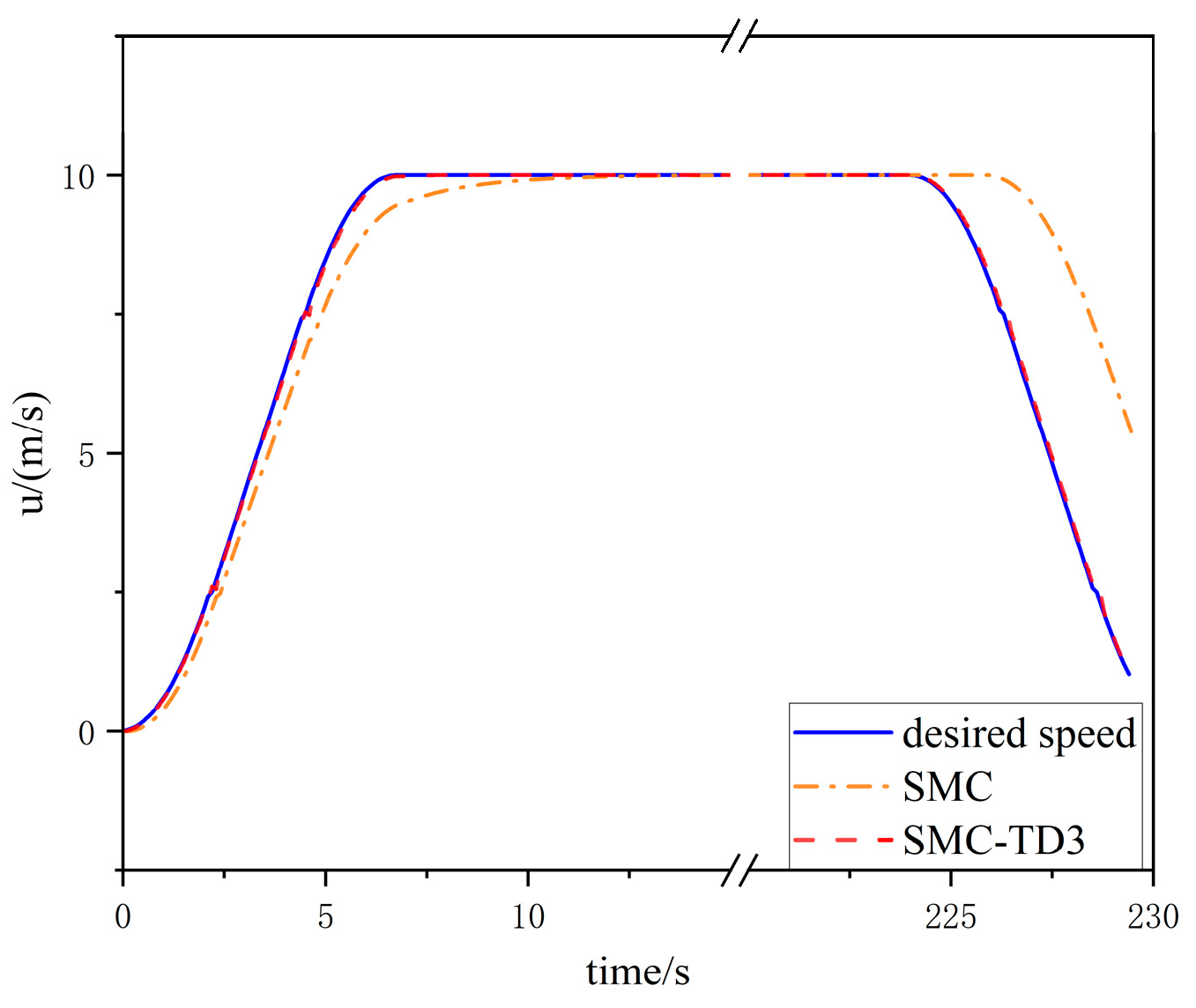
5.1. Maneuverability Analysis
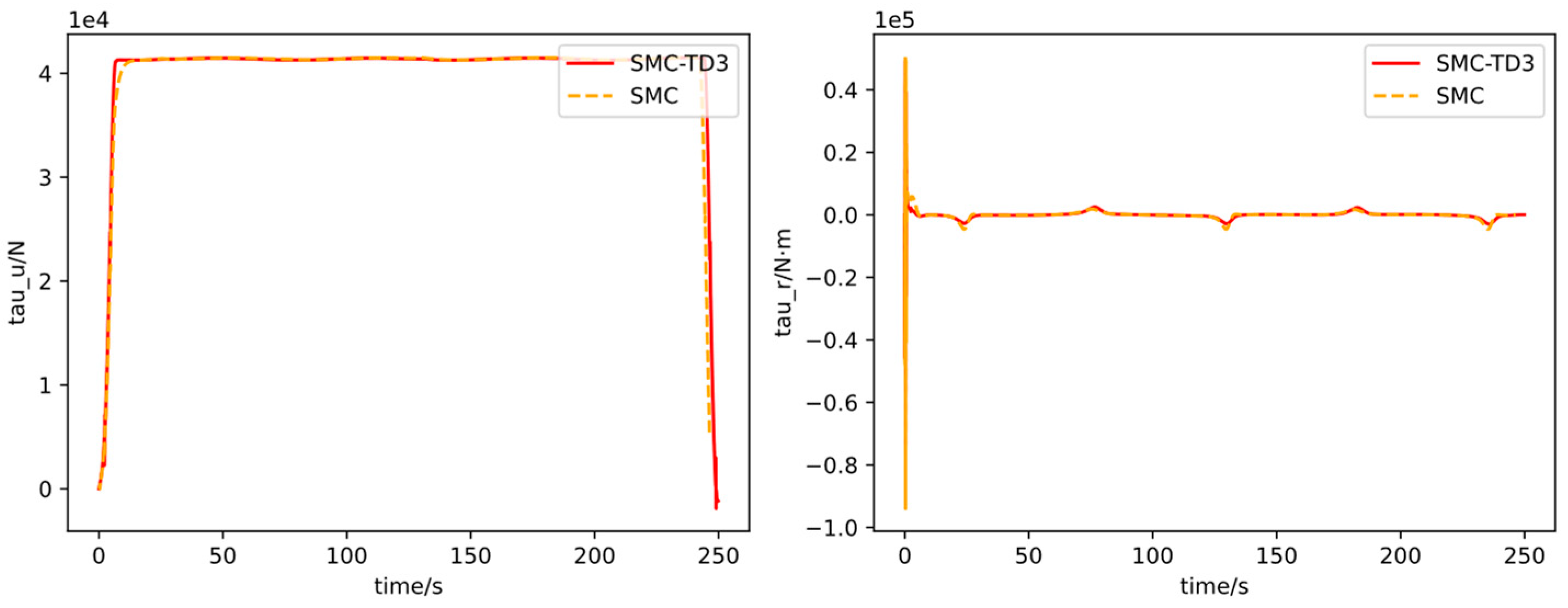
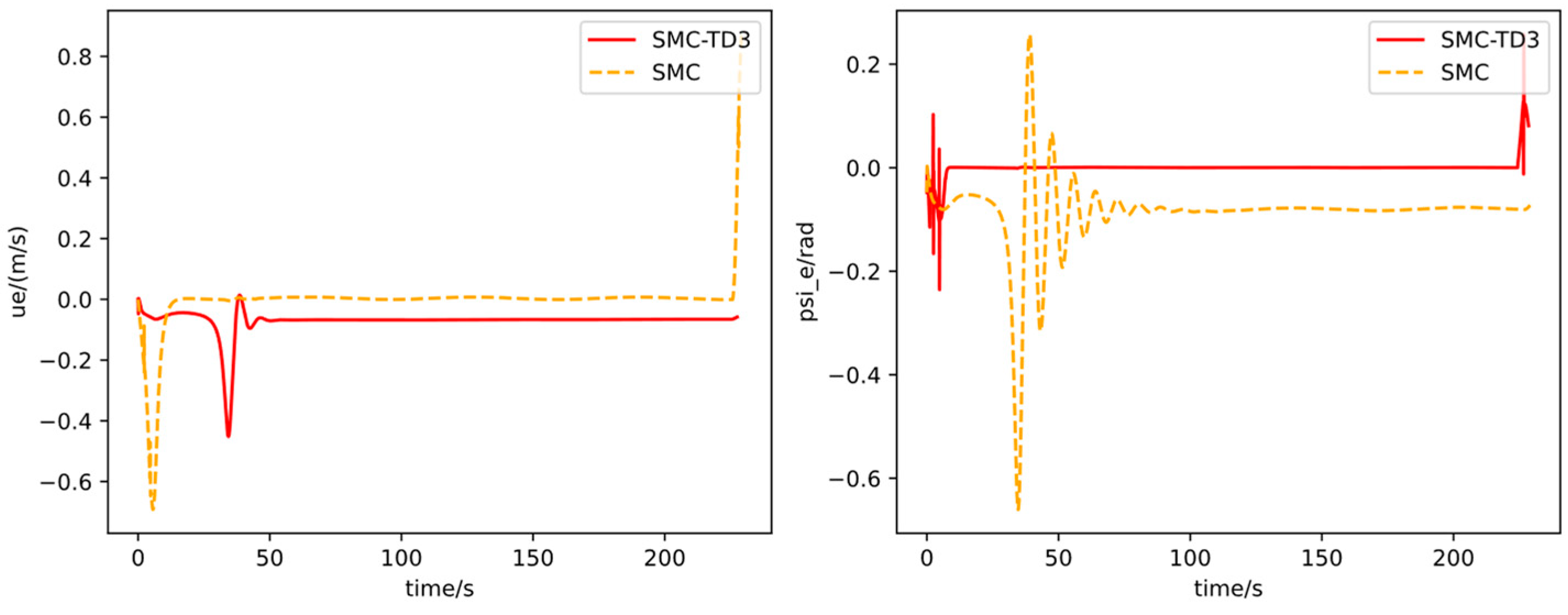
5.2. Sine Wave Trajectory Tracking Simulation
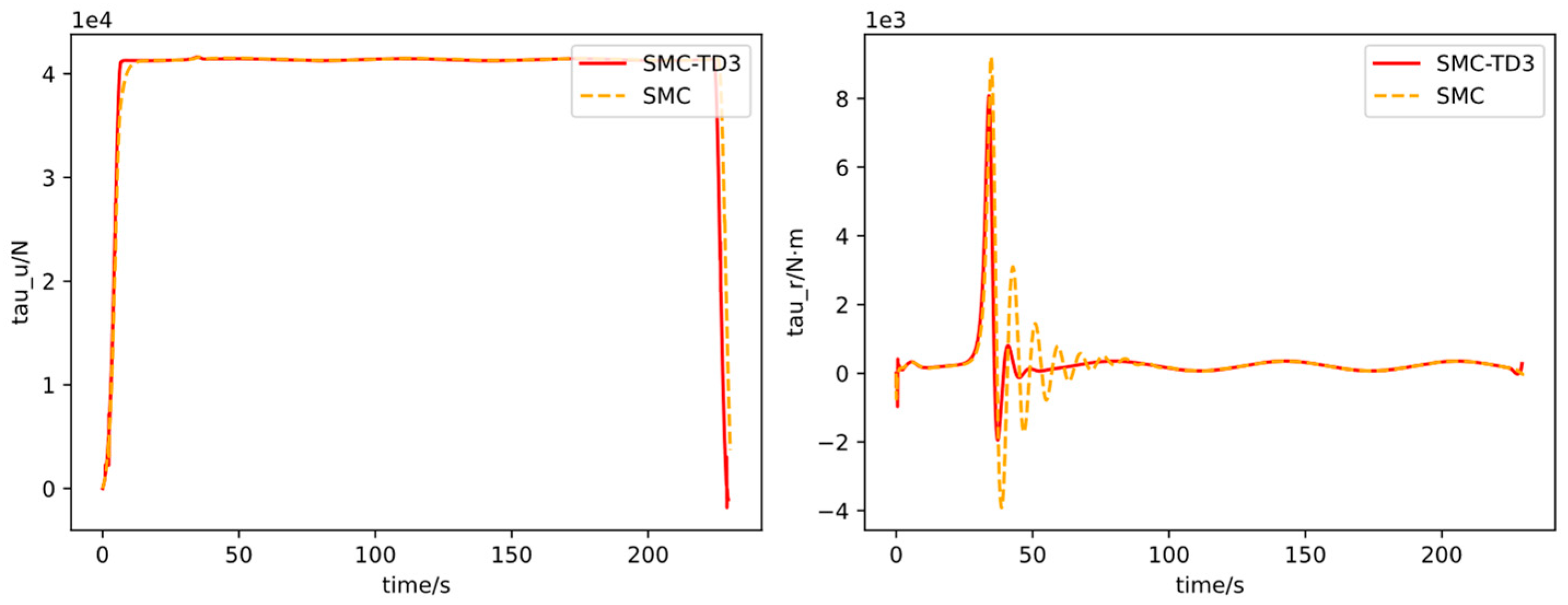
5.3. Circular Trajectory Tracking Simulation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, W.; Liao, Y.L.; Jiang, F.; Zhao, T.J. Development Review and Trend Analysis of Unmanned Surface Vehicles Technology. Unmanned Syst. Technol. 2019, 2, 1–9. [Google Scholar]
- Cong, X.; Zhao, C. PID control of uncertain nonlinear stochastic systems with state observer. Sci. China Inf. Sci. 2021, 64, 192201. [Google Scholar] [CrossRef]
- Liu, Z.Q.; Chi, R.H.; Huang, B.; Hou, Z.S. Finite-time PID control for nonlinear nonaffine systems. Sci. China Inf. Sci. 2024, 67, 212206. [Google Scholar] [CrossRef]
- Lyu, X.J.; Lin, Z.L. PID Control of Planar Nonlinear Uncertain Systems in the Presence of Actuator Saturation. IEEE/CAA J. Autom. Sin. 2022, 9, 90–98. [Google Scholar] [CrossRef]
- Liu, S.W.; Zuo, Y.; Li, T.S.; Wang, H.Q.; Gao, X.Y.; Xiao, Y. Adaptive fixed-time PID-based control of uncertain nonlinear systems and its application to unmanned surface vehicles. Int. J. Syst. Sci. 2024, 55, 2815–2824. [Google Scholar] [CrossRef]
- Zhao, C. Semiglobal stability of PID for uncertain nonaffine systems. Automatica 2024, 160, 111429. [Google Scholar] [CrossRef]
- Saleem, O.; Iqbal, J. Fuzzy-Immune-Regulated Adaptive Degree-of-Stability LQR for a Self-Balancing Robotic Mechanism: Design and HIL Realization. IEEE Robot. Autom. Lett. 2023, 8, 4577–4584. [Google Scholar] [CrossRef]
- Xin, G.Y.; Xin, S.T.; Cebe, O.; Pollayil, M.J.; Angelini, F.; Garabini, M.; Vijayakumar, S.; Mistry, M. Robust Footstep Planning and LQR Control for Dynamic Quadrupedal Locomotion. IEEE Robot. Autom. Lett. 2021, 6, 4488–4495. [Google Scholar] [CrossRef]
- Ali, N.; Ayaz, Y.; Iqbal, J. Collaborative Position Control of Pantograph Robot Using Particle Swarm Optimization. Int. J. Control Autom. Syst. 2022, 20, 198–207. [Google Scholar] [CrossRef]
- Choubey, C.; Ohri, J. Tuning of LQR-PID controller to control parallel manipulator. Neural Comput. Appl. 2022, 34, 3283–3297. [Google Scholar] [CrossRef]
- Yuan, Y.; Wang, Y.J.; Guo, L. Sliding-Mode-Observer-Based Time-Varying Formation Tracking for Multispacecrafts Subjected to Switching Topologies and Time-Delays. IEEE Trans. Automat. Control 2021, 66, 3848–3855. [Google Scholar] [CrossRef]
- Um, Y.-C.; Choi, H.-L. Integral γ-Sliding Mode Control for a Quadrotor with Uncertain Time-Varying Mass and External Disturbance. J. Electr. Eng. Technol. 2022, 17, 707–716. [Google Scholar] [CrossRef]
- Hou, H.Z.; Yu, X.H.; Fu, Z. Sliding-Mode Control of Uncertain Time-Varying Systems With State Delays: A Non-Negative Constraints Approach. IEEE Trans. Syst. Man Cybern Syst. 2022, 52, 1516–1524. [Google Scholar] [CrossRef]
- Weng, Y.P.; Wang, N. Finite-time observer-based model-free time-varying sliding-mode control of disturbed surface vessels. Ocean Eng. 2022, 251, 110866. [Google Scholar] [CrossRef]
- Davila, J.; Tranninger, M.; Fridman, L. Finite-Time State Observer for a Class of Linear Time-Varying Systems With Unknown Inputs. IEEE Trans. Autom. Control 2022, 67, 3149–3156. [Google Scholar] [CrossRef]
- Mao, Z.H.; Yan, X.-G.; Jiang, B.; Spurgeon, S.K. Sliding Mode Control of Nonlinear Systems With Input Distribution Uncertainties. IEEE Trans. Autom. Control 2023, 68, 6208–6215. [Google Scholar] [CrossRef]
- Zhou, B.; Ding, Y.; Zhang, K.-K.; Duan, G.-R. Prescribed time control based on the periodic delayed sliding mode surface without singularities. Sci. China Inf. Sci. 2024, 67, 172204. [Google Scholar] [CrossRef]
- Wang, J.; Wang, H.T.; Yan, H.C.; Wang, Y.Y.; Shen, H. Fuzzy H∞ Sliding Mode Control of Persistent Dwell-Time Switched Nonlinear Systems. IEEE Trans. Fuzzy Syst. 2022, 30, 5143–5151. [Google Scholar] [CrossRef]
- Gurumurthy, G.; Das, D.K. Terminal sliding mode disturbance observer based adaptive super twisting sliding mode controller design for a class of nonlinear systems. Eur. J. Control 2021, 57, 232–241. [Google Scholar] [CrossRef]
- Zhao, L.; Li, Z.J.; Li, H.B.; Liu, B. Backstepping integral sliding mode control for pneumatic manipulators via adaptive extended state observers. ISA Trans. 2024, 144, 374–384. [Google Scholar] [CrossRef] [PubMed]
- Ahmadi, K.; Asadi, D.; Merheb, A.; Nabavi-Chashmi, S.-Y.; Tutsoy, O. Active fault-tolerant control of quadrotor UAVs with nonlinear observer-based sliding mode control validated through hardware in the loop experiments. Control Eng. Pract. 2023, 137, 105557. [Google Scholar] [CrossRef]
- Herman, P. A Quasi-Velocity-Based Tracking Controller for a Class of Underactuated Marine Vehicles. Appl. Sci. 2022, 12, 8903. [Google Scholar] [CrossRef]
- Zhu, T.B.; Xiao, Y.J.; Zhang, H.; Pan, Y.F. Trajectory Tracking Control of USV Based on Exponential Global Fast Terminal Sliding Mode Control. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2024, 238, 47–58. [Google Scholar] [CrossRef]
- Wang, Y.; Du, Z.B. Trajectory Tracking Control for an Underactuated AUV via Nonsingular Fast Terminal Sliding Mode Approach. J. Mar. Sci. Eng. 2024, 12, 1442. [Google Scholar] [CrossRef]
- Xu, D.H.; Li, Z.L.; Xin, P.; Zhou, X.Q. The Non-Singular Terminal Sliding Mode Control of Underactuated Unmanned Surface Vessels Using Biologically Inspired Neural Network. J. Mar. Sci. Eng. 2024, 12, 112. [Google Scholar] [CrossRef]
- Wu, Z.W.; Peng, H.S.; Hu, B.; Feng, X.D. Trajectory Tracking of a Novel Underactuated AUV via Nonsingular Integral Terminal Sliding Mode Control. IEEE Access 2021, 9, 103407–103418. [Google Scholar] [CrossRef]
- Lei, Y.S.; Zhang, X.K. Ship trajectory tracking control based on adaptive fast non-singular integral terminal sliding mode. Ocean Eng. 2024, 311, 118975. [Google Scholar] [CrossRef]
- Sun, X.J.; Wang, G.F.; Fan, Y.S. Model Identification and Trajectory Tracking Control for Vector Propulsion Unmanned Surface Vehicles. Electronics 2019, 9, 22. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhang, M.J.; Yang, R.M.; Im, N. Adaptive Neural Finite-time Trajectory Tracking Control of MSVs Subject to Uncertainties. Int. J. Control Autom. Syst. 2021, 19, 2238–2250. [Google Scholar] [CrossRef]
- Zhang, C.J.; Wang, C.; Wei, Y.J.; Wang, J.Q. Neural-Based Command Filtered Backstepping Control for Trajectory Tracking of Underactuated Autonomous Surface Vehicles. IEEE Access 2020, 8, 42481–42490. [Google Scholar] [CrossRef]
- Endo, M.; Hsegawa, K. Passage planning system for small inland vessels based on standard paradigms and maneuvers of experts. In Proceedings of the International Conference on Marien Simulation and Ship Manoeuvrability, Kanazawa, Japan, 25–28 August 2003. [Google Scholar]
- Cai, Z.F. A Fast Realization Method of S-shaped Acceleration and Deceleration Control Curve for Stepper Motor Based on STM32. Inf. Technol. Informatiz. 2014, 27–29+33. [Google Scholar]
- Baraldo, S.; Valente, A. Smooth joint motion planning for high precision reconfigurable robot manipulators. In Proceeding of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Singapore, 29 May–3 June 2017. [Google Scholar] [CrossRef]
- Gao, Q.H.; Dong, J.C. Feedforward compensation design for observation error of extended state observer. J. Natl. Univ. Def. Technol. 2019, 41, 93–102. [Google Scholar]
- Sheng, Z.P. Adaptive Sliding Mode Control for Ship Motion; China Science Publishing & Media Ltd.: Beijing, China, 2019. [Google Scholar]
- Do, K.D.; Jiang, Z.J.; Pan, J. Robust adaptive path following of underactuated ships. Automatica 2004, 40, 929–944. [Google Scholar] [CrossRef]
- Jia, X.L.; Yang, T.S. Mathematical Models of Ship Motion: Mechanistic Modeling and Identification Modeling; Dalian Maritime University Press: Dalian, China, 1997. [Google Scholar]
- Zhou, Z.M.; Sheng, Z.Y.; Feng, W.S. Forecasting the Maneuverability of Multi-Purpose Cargo Ships. Ship Eng. 1983, 6, 21–29+36+4. [Google Scholar]


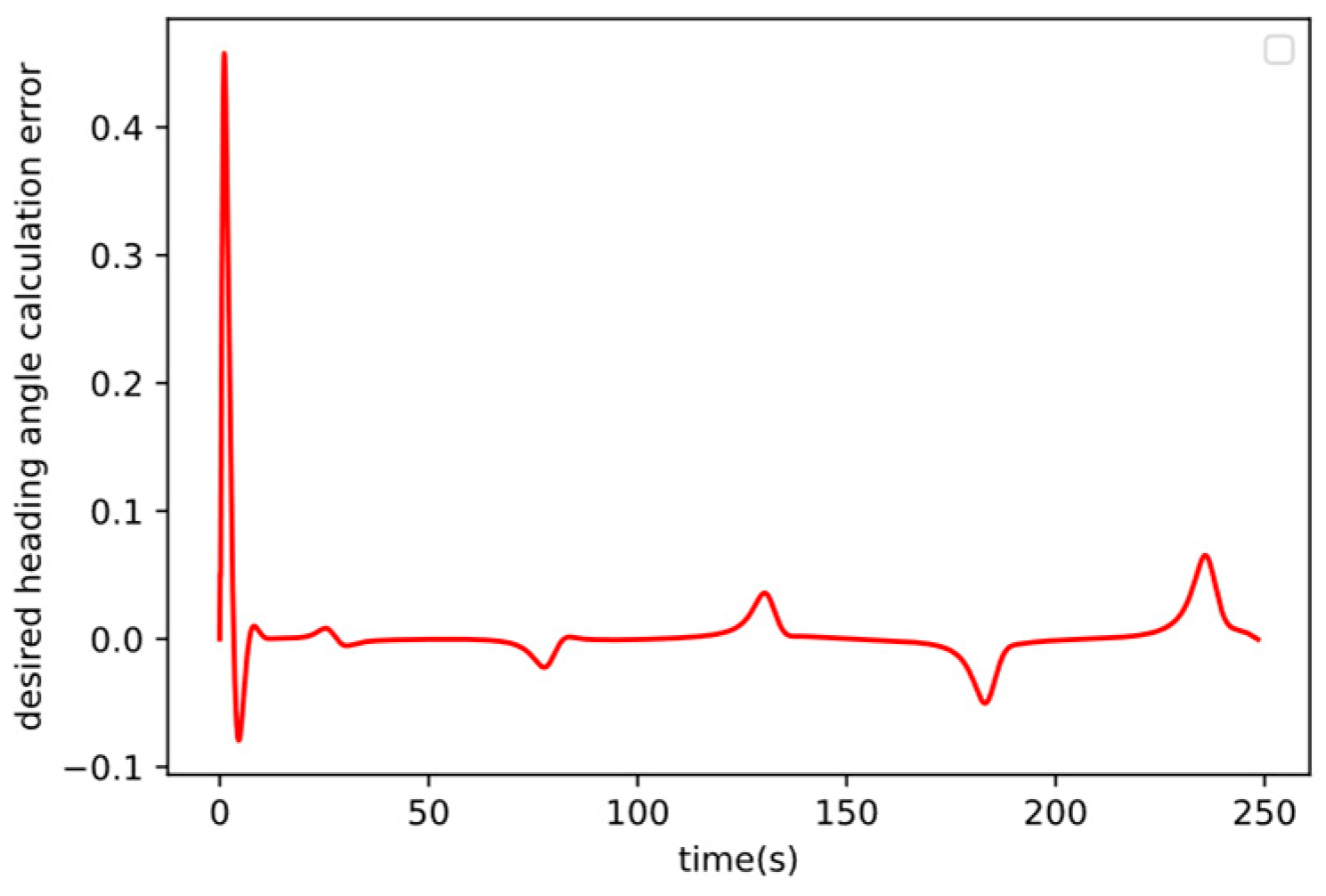
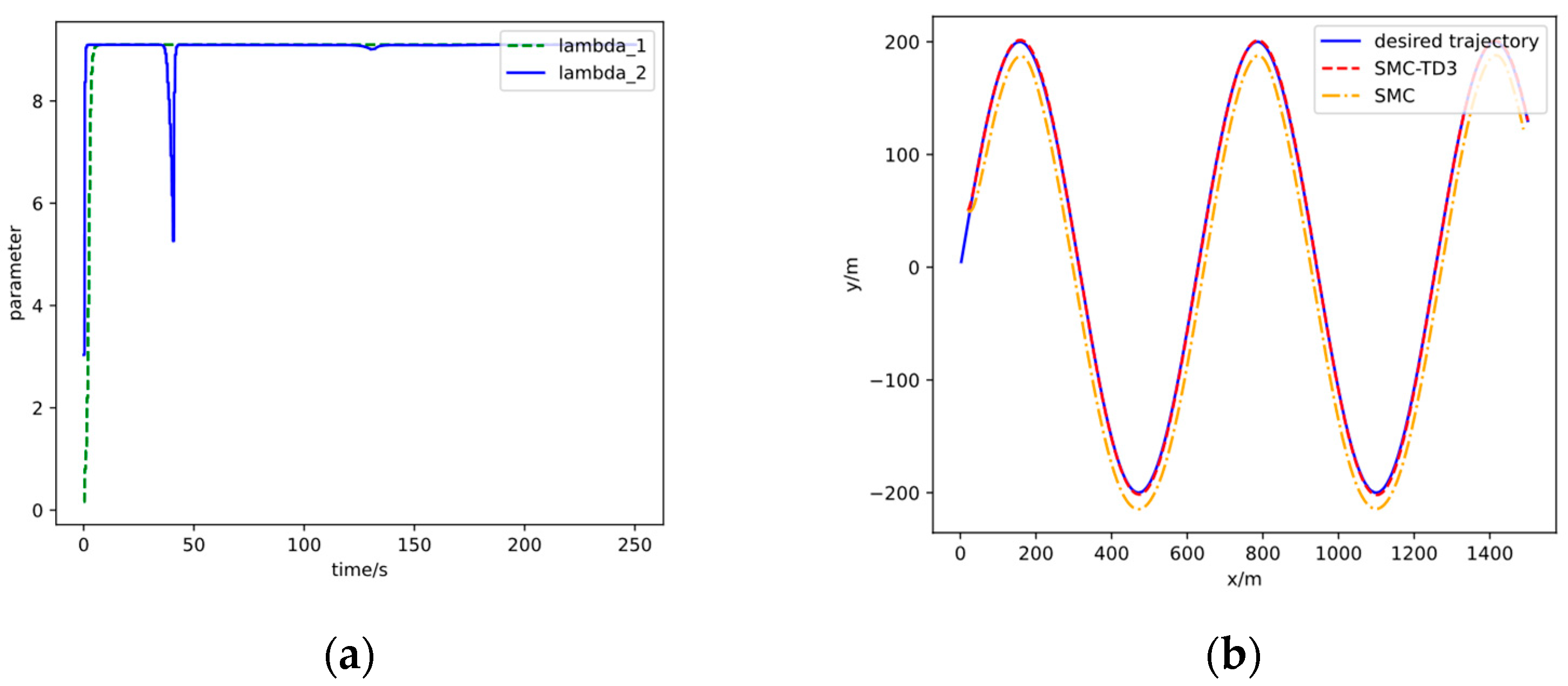
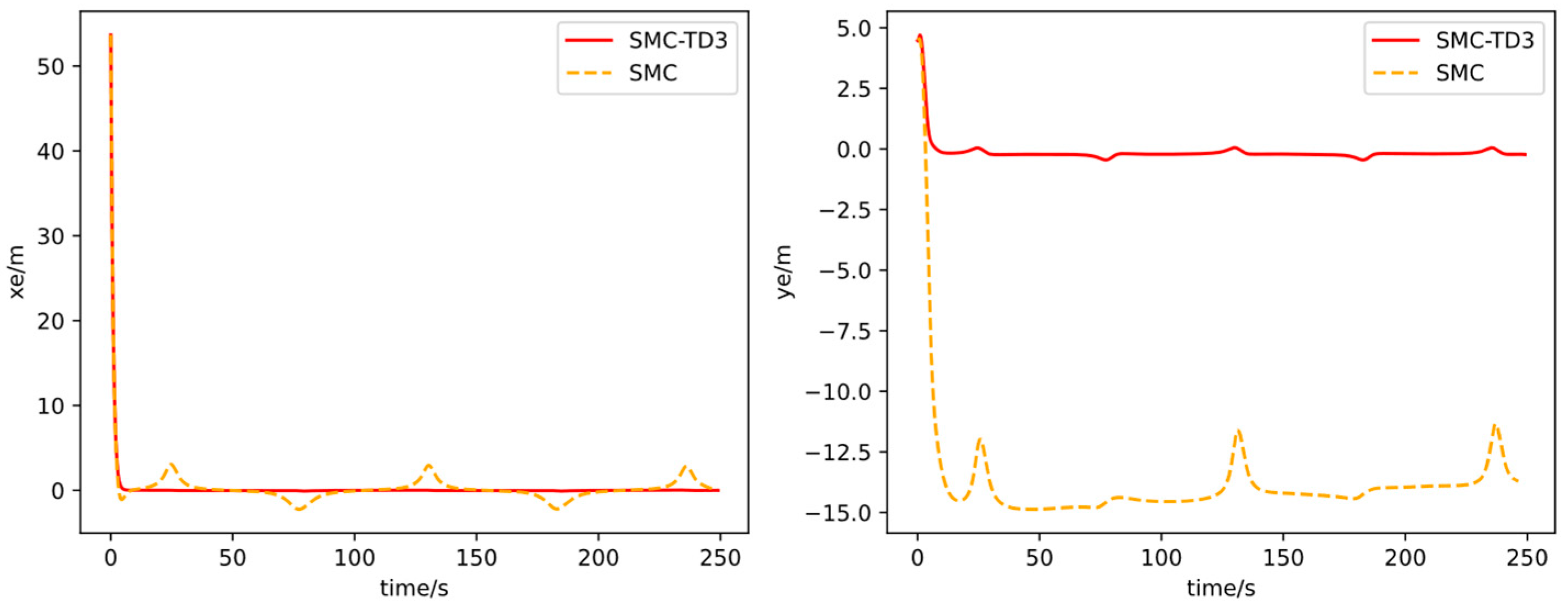

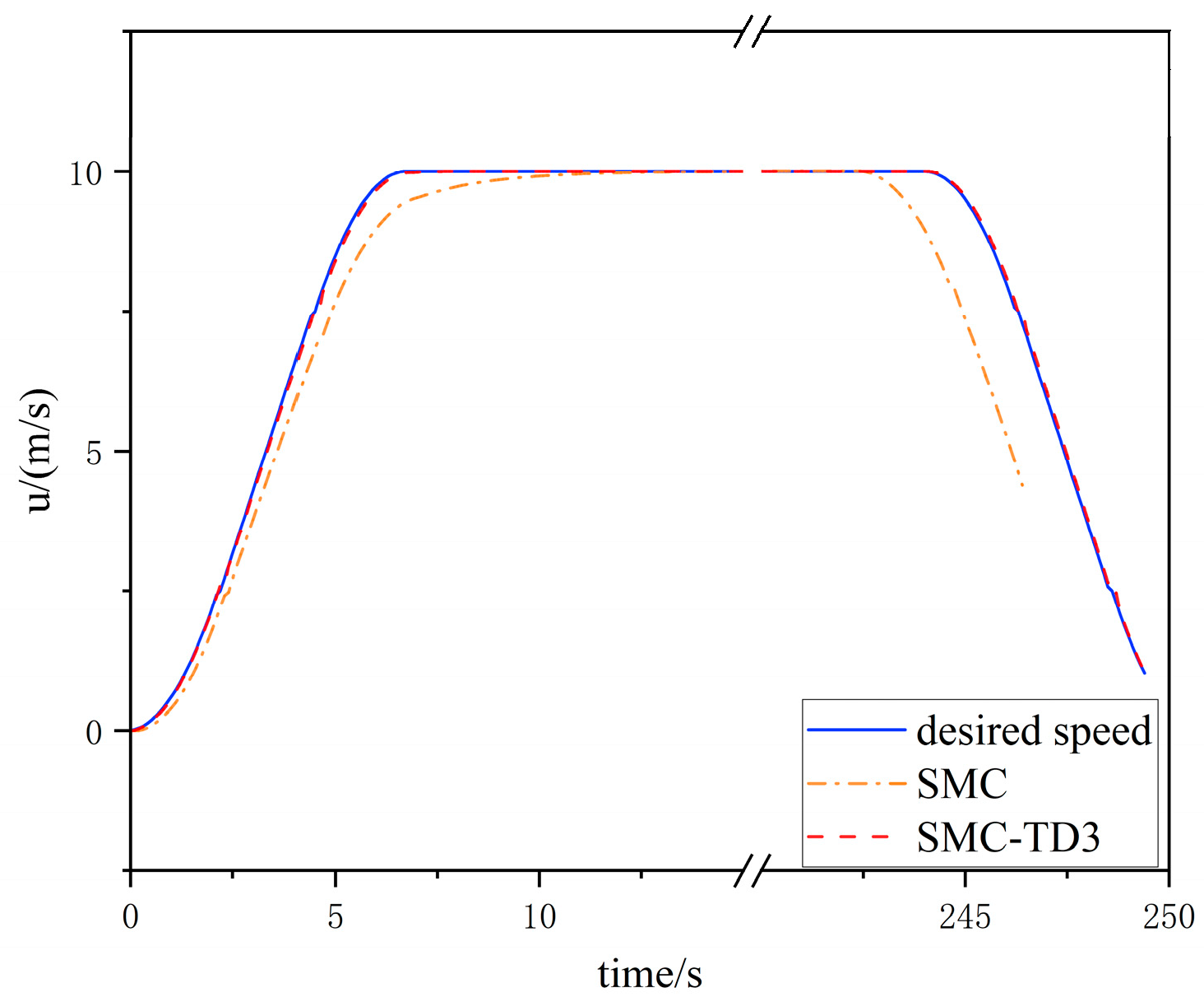


| Network | Hidden Layer | Neurons | Input | Output |
|---|---|---|---|---|
| Target actor network | 3 | 256 | 2 | 4 |
| Target critic network1 | 3 | 256 | 6 | 1 |
| Target critic network2 | 3 | 256 | 6 | 1 |
| Actor network | 3 | 256 | 2 | 4 |
| Critic network | 3 | 256 | 6 | 1 |
| Critic network | 3 | 256 | 6 | 1 |
| Parameter | Value | Parameter | Value | Parameter | Value | Parameter | Value |
|---|---|---|---|---|---|---|---|
| Displacement () | 1148 kg | 1170.58 | −0.2316 | −0.0496 | |||
| Length () | 7.201 m | 1865.66 | 0.06544 | −0.5987 | |||
| Width () | 2.507 m | 8472.23 | −0.5490 | −0.0624 | |||
| Draft () | 0.3 m | −413.58 | −0.2576 | ||||
| Block coefficient () | 0.2068 | −5.27 | −0.0377 | ||||
| Wetted surface area () | 8.07 | −834.71 | −0.0833 | ||||
| Water Density () | 1025 kg/ | 413.41 | −0.0380 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, S.; Zhang, G.; Wang, Q.; Li, Z. Sliding Mode Control for Variable-Speed Trajectory Tracking of Underactuated Vessels with TD3 Algorithm Optimization. J. Mar. Sci. Eng. 2025, 13, 99. https://doi.org/10.3390/jmse13010099
Zhu S, Zhang G, Wang Q, Li Z. Sliding Mode Control for Variable-Speed Trajectory Tracking of Underactuated Vessels with TD3 Algorithm Optimization. Journal of Marine Science and Engineering. 2025; 13(1):99. https://doi.org/10.3390/jmse13010099
Chicago/Turabian StyleZhu, Shiya, Gang Zhang, Qin Wang, and Zhengyu Li. 2025. "Sliding Mode Control for Variable-Speed Trajectory Tracking of Underactuated Vessels with TD3 Algorithm Optimization" Journal of Marine Science and Engineering 13, no. 1: 99. https://doi.org/10.3390/jmse13010099
APA StyleZhu, S., Zhang, G., Wang, Q., & Li, Z. (2025). Sliding Mode Control for Variable-Speed Trajectory Tracking of Underactuated Vessels with TD3 Algorithm Optimization. Journal of Marine Science and Engineering, 13(1), 99. https://doi.org/10.3390/jmse13010099





