Irreversible Thermodynamics of Seawater Evaporation †
Abstract
1. Introduction
Iris in her rainbow garment lifted water, bringing fresh supplies to the clouds.
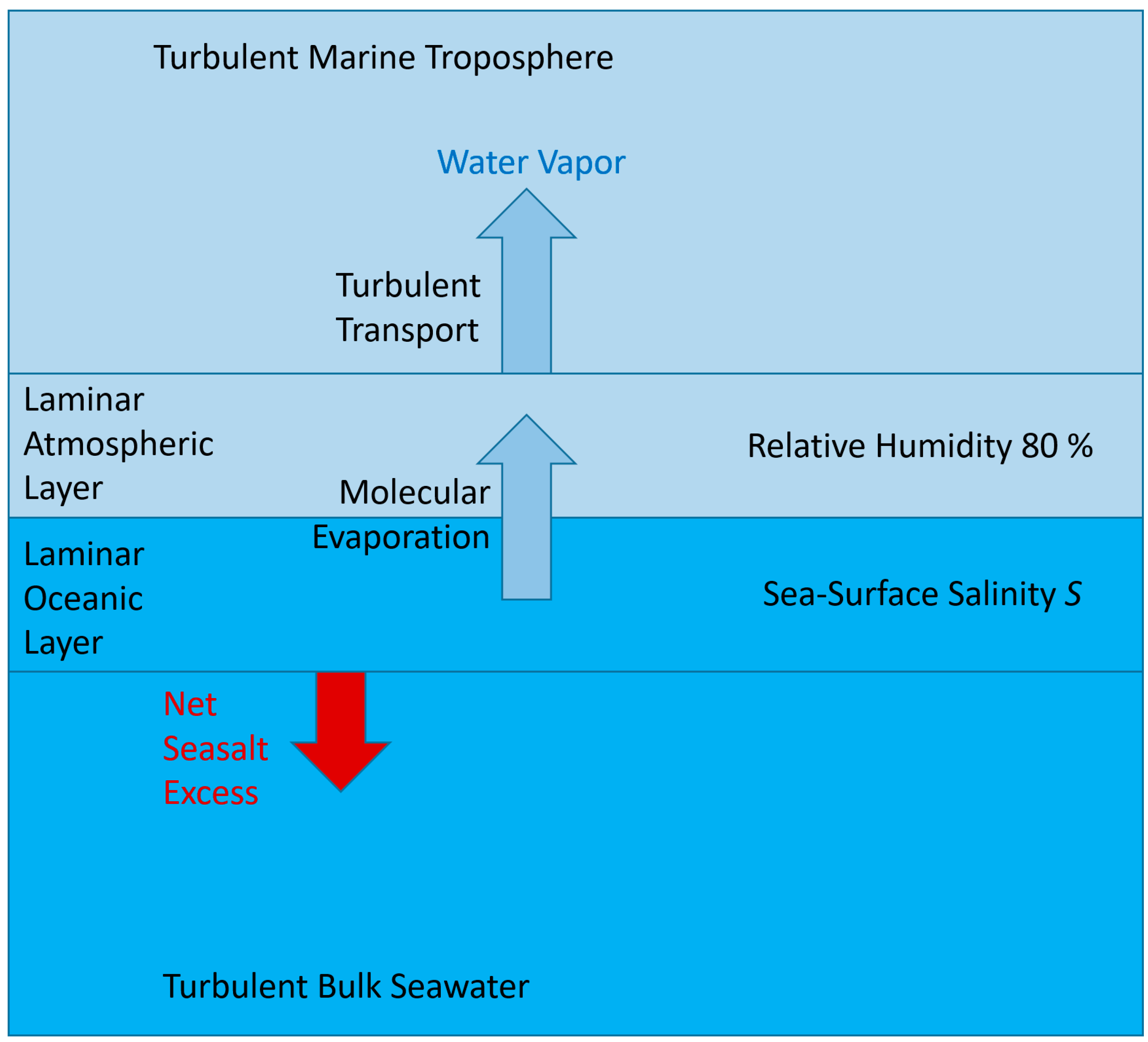
2. Irreversible Thermodynamics and Local Equilibrium
3. Onsager Force and Flux of Evaporation
4. Evaporation Enthalpy
5. Evaporation Entropy
6. Summary
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Quantity | Basic SI Unit |
| A | mass fraction of dry air in humid air, | |
| unit conversion constant | ||
| isobaric heat capacity | ||
| fugacity-based Dalton coefficient | ||
| humidity-based Dalton coefficient | ||
| partial pressure of water vapor of humid air | ||
| saturation vapor pressure of water | ||
| fugacity of water vapor in humid air | ||
| ideal gas fugacity of water vapor | ||
| fugacity of water in seawater | ||
| Gibbs energy (or free enthalpy), | ||
| specific Gibbs energy, Gibbs function | ||
| Gibbs energy of humid air | ||
| specific Gibbs energy of humid air | ||
| Gibbs energy of seawater | ||
| specific Gibbs energy of seawater | ||
| enthalpy, | ||
| specific enthalpy, | ||
| specific enthalpy of humid air | ||
| specific enthalpy of seawater | ||
| potential enthalpy | ||
| any Onsager flux | ||
| entropy flux | ||
| irreversible entropy flux | ||
| reversible entropy flux | ||
| evaporation mass flux of water | ||
| specific entropy of evaporation | ||
| specific latent heat of vaporization | ||
| specific latent heat of evaporation | ||
| vector of masses | ||
| mass | ||
| molar mass of dry air, | ||
| mass of dry air in humid air | ||
| mass of substance k | ||
| molar mass of sea salt, | ||
| mass of dissolved sea salt | ||
| mass of water vapor | ||
| molar mass of water, | ||
| mass of water solvent | ||
| entropy | ||
| entropy production | ||
| pressure | ||
| reference pressure, surface pressure | ||
| specific humidity, | ||
| saturation specific humidity | ||
| molar gas constant, | ||
| specific gas constant of water, | ||
| absolute salinity, | ||
| ITS-90 temperature | ||
| time | ||
| internal energy | ||
| specific internal energy, | ||
| volume | ||
| specific volume | ||
| vector of mass fractions | ||
| mass fraction of substance k, | ||
| mass flux driving force | ||
| any Onsager force | ||
| Onsager force vector of mass | ||
| Onsager force vector of internal energy | ||
| Onsager force vector of volume | ||
| mole fraction of water vapor in humid air | ||
| saturation mole fraction of water vapor in humid air | ||
| Onsager force of water evaporation | ||
| mole fraction of liquid water in seawater | ||
| laminar layer thickness | ||
| conservative temperature | ||
| potential temperature | ||
| Sverdrup’s diffusion coefficient (characteristic time) | ||
| specific entropy, | ||
| specific entropy of humid air | ||
| specific entropy of seawater | ||
| specific entropy of water vapor | ||
| specific entropy of liquid water | ||
| chemical potential | ||
| vector of chemical potentials | ||
| ideal gas chemical potential of water vapor | ||
| ideal-gas chemical potential of liquid water | ||
| chemical potential of substance k | ||
| chemical potential of water vapor in humid air | ||
| chemical potential of water in seawater | ||
| chemical potential difference of water | ||
| relative fugacity | 1 | |
| Onsager coefficient | ||
| Onsager coefficient of water evaporation |
| 1 | Halley (1687) [1]: p. 368. |
| 2 | Dalton (1789) [2]: p. 537. |
| 3 | Albrecht (1940) [8]: p. 36, 77. |
| 4 | Randall (2012) [9]: p. 176. |
| 5 | Albrecht (1940) [8]: p. 77. |
| 6 | Rapp (2014) [16]: p. 420. |
| 7 | Weller et al. (2022) [17]: p. E1968. |
| 8 | Planck (1948) [74]: p. 28, 29. |
References
- Halley, E. An estimate of the quantity of vapour raised out of the sea by the warmth of the sun; derived from an experiment shown before the Royal Society at one of their late meetings. Philos. Trans. 1687, 16, 366–370. [Google Scholar] [CrossRef]
- Dalton, J. Experimental Essays, on the Constitution of mixed GASES; on the Force of STEAM or VAPOUR from Water and other Liquids in different temperatures, both in a Torricellian Vacuum and in Air; on EVAPORATION.; and on the Expansion of GASES by Heat. Mem. Lit. Philos. Soc. Manch. 1798, 5, 535–602. Available online: https://www.biodiversitylibrary.org/part/308525 (accessed on 14 March 2023).
- IOC; SCOR; IAPSO. The International Thermodynamic Equation of Seawater—2010: Calculation and Use of Thermodynamic Properties; Intergovernmental Oceanographic Commission, Manuals and Guides No. 56; UNESCO (English): Paris, France, 2010; p. 196. Available online: http://www.TEOS-10.org (accessed on 26 November 2022).
- UNESCO. Algorithms for Computation of Fundamental Properties of Seawater, Unesco Technical Papers in Marine Science, 44, UNESCO, Paris, France. 1983. Available online: https://unesdoc.unesco.org/images/0005/000598/059832eb.pdf (accessed on 18 December 2023).
- Feistel, R. Thermodynamic properties of seawater, ice and humid air: TEOS-10, before and beyond. Ocean Sci. 2018, 14, 471–502. [Google Scholar] [CrossRef]
- Smythe-Wright, D.; Gould, W.J.; McDougall, T.J.; Sparnocchia, S.; Woodworth, P.L. IAPSO: Tales from the ocean frontier. Hist. Geo Space Sci. 2019, 10, 137–150. [Google Scholar] [CrossRef]
- Feistel, R.; Hellmuth, O. Relative Humidity: A Control Valve of the Steam Engine Climate. J. Hum. Earth Future 2021, 2, 140–182. [Google Scholar] [CrossRef]
- Albrecht, F. Untersuchungen über den Wärmehaushalt der Erdoberfläche in Verschiedenen Klimagebieten. Reichsamt für Wetterdienst, Wissenschaftliche Abhandlungen Bd. VIII, Nr. 2; Springer: Berlin/Heidelberg, Germany, 1940; Available online: https://www.springer.com/de/book/9783662425305 (accessed on 18 December 2023).
- Randall, D.A. Atmosphere, Clouds, and Climate; Princeton University Press: Princeton, NJ, USA, 2012. [Google Scholar]
- Josey, S.A.; Gulev, S.; Yu, L. Exchanges through the ocean surface. In Ocean Circulation and Climate. A 21st Century Perspective; Siedler, G., Griffies, S.M., Gould, J., Church, J.A., Eds.; Elsevier: Amsterdam, The Netherlands, 2013; pp. 115–140. [Google Scholar] [CrossRef]
- Rhein, M.; Rintoul, S.R.; Aoki, S.; Campos, E.; Chambers, D.; Feely, R.A.; Gulev, S.; Johnson, G.C.; Josey, S.A.; Kostianoy, A.; et al. (Eds.) Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2013. [Google Scholar]
- Cronin, M.F.; Gentemann, C.L.; Edson, J.; Ueki, I.; Bourassa, M.; Brown, S.; Clayson, C.A.; Fairall, C.W.; Farrar, J.T.; Gille, S.T.; et al. Air-sea fluxes with a focus on heat and momentum. Front. Mar. Sci. 2019, 6, 430. [Google Scholar] [CrossRef]
- Feistel, R. Salinity and relative humidity: Climatological relevance and metrological needs. Acta Imeko 2015, 4, 57–61. [Google Scholar] [CrossRef]
- Gorfer, M. Monitoring of Climate Change and Variability in Atmospheric Heat Content Based on Climate Records and Reanalyses; Wegener Center Scientific Report 94-2022; Wegener Center Verlag, University of Graz: Graz, Austria, 2022; Available online: https://unipub.uni-graz.at/obvugrhs/content/titleinfo/6751357 (accessed on 18 December 2023).
- Von Schuckmann, K.; Minère, A.; Gues, F.; Cuesta-Valero, F.J.; Kirchengast, G.; Adusumilli, S.; Straneo, F.; Ablain, M.; Allan, R.P.; Barker, P.; et al. Heat stored in the Earth system 1960–2020: Where does the energy go? Earth Syst. Sci. Data 2023, 15, 1675–1709. [Google Scholar] [CrossRef]
- Rapp, D. Assessing Climate Change—Temperatures. In Solar Radiation, and Heat Balance; Springer: Cham, Switzerland, 2014. [Google Scholar]
- Weller, R.A.; Lukas, R.; Potemra, J.; Plueddemann, A.J.; Fairall, C.; Bigorre, S. Ocean Reference Stations: Long-Term, Open-Ocean Observations of Surface Meteorology and Air–Sea Fluxes Are Essential Benchmarks. Cover. Bull. Am. Meteorol. Soc. 2022, 103, E1968–E1990. [Google Scholar] [CrossRef]
- Feistel, R.; Hellmuth, O. Thermodynamics of Evaporation from the Ocean Surface. Atmosphere 2023, 14, 560. [Google Scholar] [CrossRef]
- Bentamy, A.; Piollé, J.F.; Grouazel, A.; Danielson, R.; Gulev, S.; Paul, F.; Azelmat, H.; Mathieu, P.P.; von Schuckmann, K.; Sathyendranath, S.; et al. Review and assessment of latent and sensible heat flux accuracy over the global oceans. Remote Sens. Environ. 2017, 201, 196–218. [Google Scholar] [CrossRef]
- Hupfer, P.; Foken, T.; Panin, G. Existence and Structure of the Laminar Boundary Layer of the Atmosphere in the Near-Shore Zone of the Sea. Z. für Meteorol. 1975, 25, 94–102. [Google Scholar]
- Avery, K.R. Literature Search for Atmospheric Humidity Profile Models from the Sea Surface to 1000 Meters; NOAA Technical Memorandum EDS NODC-1; NOA: Silver Spring, MD, USA, 1972. [Google Scholar]
- Gao, Q.; Wang, S.; Yang, X. Estimation of Surface Air Specific Humidity and Air–Sea Latent Heat Flux Using FY-3C Microwave Observations. Remote Sens. 2019, 11, 466. [Google Scholar] [CrossRef]
- Foken, T.; Kitajgorodskij, S.A.; Kuznecov, O.A. On the Dynamics of the Molecular Boundary Layer Above the Sea. Bound.-Layer Meteorol. 1978, 15, 289–300. [Google Scholar] [CrossRef]
- Schmidt, W. Sonnenstrahlung und Verdunstung an freien Wasserflächen; ein Beitrag zum Wärmehaushalt des Weltmeeres und zum Wasserhaushalt der Erde. Ann. Der Hydrogr. Und Marit. Meteorol. 1915, 43, 11–178. [Google Scholar]
- Wüst, G. Die Verdunstung auf dem Meere. Veröffentlichungen des Instituts für Meereskunde an der Universität Berlin, Geogr. Naturwissenschaftliche Reihe 1920, 54, 1–95. [Google Scholar]
- Sverdrup, H.U. Das maritime Verdunstungsproblem. Ann. Hydrogr. Marit. Meteorol. 1936, 54, 41–47. [Google Scholar]
- Montgomery, R.B. Observations of Vertical Humidity Distribution Above the Ocean Surface and Their Relation to Evaporation. Pap. Phys. Oceanogr. Meteorol. 1940, 7, 2–30. [Google Scholar] [CrossRef]
- Sellers, W.D. Physical Climatology; University of Chicago Press: Chicago, IL, USA, 1965. [Google Scholar]
- Budyko, M.I. Der Wärmehaushalt der Erdoberfläche; Fachliche Mitteilungen der Inspektion Geophysikalischer Beratungsdienst der Bundeswehr im Luftwaffenamt; 1963; Volume 100, pp. 3–282. [Google Scholar]
- Debski, K. Continental Hydrology, Vol. II, Physics of Water, Atmospheric Precipitation and Evaporation; Scientific Publications Foreign Cooperation Center of the Central Institute for Scientific, Technical and Economic Information: Warsaw, Poland, 1966. [Google Scholar]
- Gill, A.E. Atmosphere-Ocean Dynamics; Academic Press: San Diego, CA, USA, 1982. [Google Scholar]
- Stewart, R.H. Introduction to Physical Oceanography; Texas A & M University: College Station, TX, USA, 2008. [Google Scholar] [CrossRef]
- Saunders, P.M. The temperature at the ocean–air interface. J. Atmos. Sci. 1967, 24, 269–273. [Google Scholar] [CrossRef]
- Schluessel, P.; Emery, W.J.; Grassl, H.; Mammen, T. On the bulk-skin temperature difference and its impact on satellite remote sensing of sea surface temperature. J. Geophys. Res. 1990, 95, 13341–13356. [Google Scholar] [CrossRef]
- Zülicke, C.; Hagen, E. Impact of the Skin Effect on the Near-Surface Temperature Profile. Phys. Chem. Earth 1998, 23, 531–535. [Google Scholar] [CrossRef]
- Katsaros, K. Evaporation and Humidity. In Encyclopedia of Ocean Sciences; Steele, J.H., Thorpe, A.S., Turekian, K.K., Eds.; Academic Press: San Diego, CA, USA, 2001; pp. 870–877. [Google Scholar] [CrossRef]
- Zülicke, C. Air–sea fluxes including the effect of the molecular skin layer. Deep Sea Res. II 2005, 52, 1220–1245. [Google Scholar] [CrossRef]
- Gibbs, J.W. On the Equilibrium of Heterogeneous Substances. Trans. Conn. Acad. Arts Sci. 1878, 108–520. [Google Scholar] [CrossRef]
- Feistel, R.; Wright, D.G.; Kretzschmar, H.-J.; Hagen, E.; Herrmann, S.; Span, R. Thermodynamic properties of sea air. Ocean Sci. 2010, 6, 91–141. [Google Scholar] [CrossRef]
- Feistel, R.; Wielgosz, R.; Bell, S.A.; Camões, M.F.; Cooper, J.R.; Dexter, P.; Dickson, A.G.; Fisicaro, P.; Harvey, A.H.; Heinonen, M.; et al. Metrological challenges for measurements of key climatological observables: Oceanic salinity and pH, and atmospheric humidity. Part 1—Overview. Metrologia 2016, 53, R1–R11. [Google Scholar] [CrossRef]
- Feistel, R. TEOS-10: A New International Oceanographic Standard for Seawater, Ice, Fluid Water, and Humid Air. Int. J. Thermophys. 2012, 33, 1335–1351. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifschitz, E.M. Hydrodynamik; Akademie: Berlin, Germany, 1966. [Google Scholar]
- Glansdorff, P.; Prigogine, I. Thermodynamic Theory of Structure, Stability and Fluctuations; Wiley-Interscience: London, UK; New York, NY, USA; Sydney, Australia; Toronto, ON, Canada, 1971. [Google Scholar]
- Subarew, D.N. Statistische Thermodynamik des Nichtgleichgewichts; Akademie-Verlag: Berlin, Germany, 1976. [Google Scholar]
- De Groot, S.R.; Mazur, P. Non-Equilibrium Thermodynamics; Dover Publications: New York, NY, USA, 1984. [Google Scholar]
- Kraus, E.B.; Businger, J.A. Atmosphere-Ocean Interaction; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- IOC-UNESCO Resolution XXV-7 International Thermodynamic Equation of Seawater (TEOS-10). In Proceedings of the Intergovernmental Oceanographic Commission, Twenty-Fifth Session of the Assembly, Paris, France, 16–25 June 2009; Available online: http://unesdoc.unesco.org/images/0018/001878/187890e.pdf (accessed on 20 December 2023).
- IUGG Resolution 4: Adoption of the International Thermodynamic Equation of Seawater–2010 (TEOS-10). In Proceedings of the International Union of Geodesy and Geophysics, XXV General Assembly, Melbourne, Australia, 27 June–7 July 2011; Available online: https://iugg.org/wp-content/uploads/2022/03/IUGG-Resolutions-XXV-GA-Melbourne-English.pdf (accessed on 20 December 2023).
- IAPWS AN6-16. Advisory Note No. 6: Relationship between Various IAPWS Documents and the International Thermodynamic Equation of Seawater—2010 (TEOS-10); The International Association for the Properties of Water and Steam: Dresden, Germany, September 2016; Available online: http://www.iapws.org (accessed on 18 December 2023).
- Wright, D.G.; Feistel, R.; Reissmann, J.H.; Miyagawa, K.; Jackett, D.R.; Wagner, W.; Overhoff, U.; Guder, C.; Feistel, A.; Marion, G.M. Numerical implementation and oceanographic application of the thermodynamic potentials of liquid water, water vapour, ice, seawater and humid air—Part 2: The library routines. Ocean Sci. 2010, 6, 695–718. [Google Scholar] [CrossRef]
- Feistel, R.; Hellmuth, O.; Lovell-Smith, J. Defining relative humidity in terms of water activity: III. Relations to dew-point and frost-point temperatures. Metrologia 2022, 59, 045013. [Google Scholar] [CrossRef]
- Feistel, R.; Lovell-Smith, J.W. Defining relative humidity in terms of water activity. Part 1: Definition. Metrologia 2017, 54, 566–576. [Google Scholar] [CrossRef]
- Falkenhagen, H.; Ebeling, W.; Hertz, G. Theorie der Elektrolyte; S. Hirzel Verlag: Leipzig, Germany, 1971. [Google Scholar]
- Feistel, R.; Ebeling, W. Physics of Self-Organization and Evolution; Wiley-VCH: Weinheim, Germany, 2011. [Google Scholar]
- Kirkaldy, J.S. Thermodynamics of Terrestrial Evolution. Biophys. J. 1965, 5, 965–979. [Google Scholar] [CrossRef] [PubMed]
- Landau, L.D.; Lifschitz, E.M. Statistische Physik; Akademie: Berlin, Germany, 1966. [Google Scholar]
- Feistel, R. Distinguishing between Clausius, Boltzmann and Pauling Entropies of Frozen Non-Equilibrium States. Entropy 2019, 21, 799. [Google Scholar] [CrossRef] [PubMed]
- Zivieri, R. Trends in the Second Law of Thermodynamics. Entropy 2023, 25, 1321. [Google Scholar] [CrossRef] [PubMed]
- Prigogine, I.; Wiaume, J.M. Biologie et thermodynamique des phénomènes irréversibles. Experientia 1946, 2, 451–453. [Google Scholar] [CrossRef]
- Planck, M. Vorlesungen über die Theorie der Wärmestrahlung; Johann Ambrosius Barth: Leipzig, Germany, 1906. [Google Scholar]
- Feistel, R.; Wagner, W. High-pressure thermodynamic Gibbs functions of ice and sea ice. J. Mar. Res. 2005, 63, 95–139. [Google Scholar] [CrossRef]
- Feistel, R.; Wagner, W. A new equation of state for H2O ice Ih. J. Phys. Chem. Ref. Data 2006, 35, 1021–1047. [Google Scholar] [CrossRef]
- Wagner, W.; Pruß, A. The IAPWS Formulation 1995 for the Thermodynamic Properties of Ordinary Water Substance for General and Scientific Use. J. Phys. Chem. Ref. Data 2002, 31, 387–535. [Google Scholar] [CrossRef]
- Feistel, R.; Wright, D.G.; Miyagawa, K.; Harvey, A.H.; Hruby, J.; Jackett, D.R.; McDougall, T.J.; Wagner, W. Mutually consistent thermodynamic potentials for fluid water, ice and seawater: A new standard for oceanography. Ocean Sci. 2008, 4, 275–291. [Google Scholar] [CrossRef]
- McDougall, T.J. Potential enthalpy: A conservative oceanic variable for evaluating heat content and heat fluxes. J. Phys. Oceanogr. 2003, 33, 945–963. [Google Scholar] [CrossRef]
- Graham, F.S.; McDougall, T.J. Quantifying the non-conservative production of Conservative Temperature, potential temperature and entropy. J. Phys. Oceanogr. 2013, 43, 838–862. [Google Scholar] [CrossRef]
- McDougall, T.J.; Barker, P.M.; Feistel, R.; Roquet, F. A Thermodynamic Potential of Seawater in Terms of Absolute Salinity, Conservative Temperature and in-situ Pressure. Ocean Sci. 2023, 19, 1719–1741. [Google Scholar] [CrossRef]
- Prigogine, I. Etude Thermodynamique des Phénomènes Irreversibles (These, Bruxelles 1945); Desoer: Liege, Belgium, 1947. [Google Scholar]
- Prigogine, I. Time, structure, and fluctuations (Nobel Lecture, 8 December 1977). Science 1978, 201, 777–785. [Google Scholar] [CrossRef]
- Fofonoff, N.P. Physical properties of sea water. In The Sea; Hill, M.N., Ed.; Wiley-Interscience: New York, NY, USA, 1962; Volume 1, pp. 3–28. [Google Scholar]
- Boltzmann, L. On the Relationship between the Second Main Theorem of Mechanical Heat Theory and the Probability Calculation with Respect to the Results about the Heat Equilibrium; Sitzb. d. Kaiserlichen Akademie der Wissenschaften, Mathematisch-Naturwissenschaftliche Klasse: Wien, Austria, 1877; LXXVI, Abt. II; pp. 373–435. [Google Scholar]
- Boltzmann, L. Vorlesung über Gastheorie, Band 1; Wiener Sitzungsberichte, Johann Ambrosius Barth: Leipzig, Germany, 1896. [Google Scholar]
- BIPM. The International System of Units (SI). 9th Edition of the SI Brochure, Available from the BIPM. 2019. Available online: https://www.bipm.org/en/publications/si-brochure (accessed on 18 December 2023).
- Planck, M. Wissenschaftliche Selbstbiographie; Johann Ambrosius Barth: Leipzig, Germany, 1948. [Google Scholar]
- Guggenheim, E.A. Thermodynamics; North-Holland: Amsterdam, The Netherlands, 1949. [Google Scholar]
- McDougall, T.J.; Feistel, R. What causes the adiabatic lapse rate? Deep Sea Res. I 2003, 50, 1523–1535. [Google Scholar] [CrossRef]
- Feistel, R.; Hagen, E. Thermodynamic Quantities in Oceanography. In The Oceans: Physical-Chemical Dynamics and Human Impact; Majumdar, S.K., Miller, E.W., Forbes, G.S., Schmalz, R.F., Panah, A.A., Eds.; The Pennsylvania Academy of Science: Scranton, PA, USA, 1994; pp. 1–13. [Google Scholar]
- Feistel, R.; Feistel, S. Die Ostsee als thermodynamisches System. In Irreversible Prozesse und Selbstorganisation; Schimansky-Geier, L., Malchow, H., Pöschel, T., Eds.; Logos: Berlin, Germany, 2006; pp. 81–98. [Google Scholar] [CrossRef]
- Feistel, R. Radiative entropy balance and vertical stability of a gray atmosphere. Eur. Phys. J. B 2011, 82, 197–206. [Google Scholar] [CrossRef]
- Doney, S.C. Irreversible Thermodynamic Coupling between Heat and Matter Fluxes across a Gas/Liquid Interface. J. Chem. Soc. Faraday Trans. 1994, 90, 1865–1874. [Google Scholar] [CrossRef]
- Prausnitz, J.M.; Lichtenthaler, R.N.; Gomes de Azevedo, E. Molecular Thermodynamics of Fluid-Phase Equilibria, 3rd ed.; Prentice Hall: Englewood Cliffs, NJ, USA, 1999. [Google Scholar]
- Millero, F.J.; Feistel, R.; Wright, D.G.; McDougall, T.J. The Composition of Standard Seawater and the Definition of the Reference-Composition Salinity Scale. Deep-Sea Res. I 2008, 55, 50–72. [Google Scholar] [CrossRef]
- Feistel, R.; Lovell-Smith, J.; Hellmuth, O. Virial Approximation of the TEOS-10 Equation for the Fugacity of Water in Humid Air. Int. J. Thermophys. 2015, 36, 44–68. [Google Scholar] [CrossRef]
- Baumgartner, A.; Reichel, E. The World Water Balance; Oldenbourg: München, Germany, 1975. [Google Scholar]
- Lovell-Smith, J.W.; Feistel, R.; Harvey, A.H.; Hellmuth, O.; Bell, S.A.; Heinonen, M.; Cooper, J.R. Metrological challenges for measurements of key climatological observables. Part 4: Atmospheric relative humidity. Metrologia 2016, 53, R39–R59. [Google Scholar] [CrossRef]
- Quasem, N.A.A.; Generous, M.M.; Qureshi, B.A.; Zubair, S.M. Thermodynamic and Thermophysical Properties of Saline Water; Models, Correlations and Data for Desalination and Relevant Applications; Springer: Cham, Switzerland, 2023. [Google Scholar]
- Ebeling, W.; Feistel, R. Physik der Selbstorganisation und Evolution; Akademie-Verlag: Berlin, Germany, 1982. [Google Scholar]
- Feistel, R. Entropy Flux and Entropy Production of Stationary Black-Body Radiation. J. Non-Equilib. Thermodyn. 2011, 36, 131–139. [Google Scholar] [CrossRef]
- Yan, Y.; Gan, Z.; Qi, Y. Entropy budget of the ocean system. Geophys. Res. Lett. 2004, 31, 1–4. [Google Scholar] [CrossRef]
- Gibbs, J.W. Graphical Methods in the Thermodynamics of Fluids. Trans. Conn. Acad. Arts Sci. 1873, 309–342. [Google Scholar]
- Jacobs, W.C. On the energy exchange between sea and atmosphere. J. Mar. Res. 1942, 5, 37–66. [Google Scholar]
- Jacobs, W. The Energy Exchange Between Sea and Atmosphere and Some of Its Consequences; Bulletin of the Scripps Institution of Oceanography of the University of California, La Jolla, California: Scripps Institution of Oceanography; University of California Press: Berkeley, CA, USA, 1951; Available online: https://books.google.de/books?id=cGkIvwEACAAJ (accessed on 8 January 2024).
- Sutton, O.G.; Simpson, G.C. Wind structure and evaporation in a turbulent atmosphere. Proc. R. Soc. London. Ser. A Contain. Pap. A Math. Phys. Character 1934, 146, 701–722. [Google Scholar] [CrossRef]
- Penman, H.L.; Keen, B.A. Natural evaporation from open water, bare soil and grass. Proc. R. Soc. London. Ser. A. Math. Phys. Sci. 1948, 193, 120–145. [Google Scholar] [CrossRef]
- Tomczak, G. 1939: Verdunstung freier Wasserflächen; Geophysikalischen Instituts der Universität: Leipzig, Germany, 1939; Volume XII, pp. 107–175. [Google Scholar]
- Brogmus, W. Zur Definition und Berechnung der Widerstands- und Verdunstungskoeffizienten bei nicht-adiabatischer Schichtung. Ann. Meteorol. 1958, 8, 225–233. [Google Scholar]
- Brogmus, W. Zur Theorie der Verdunstung der natürlichen Erdoberfläche; Einzelveröffentlichung; Deutscher Wetterdienst, Seewetteramt Hamburg: Hamburg, Germany, 1959; Volume 21. [Google Scholar]
- Dammann, W. Meteorologische Verdunstungsmessung, Näherungsformeln und die Verdunstung in Deutschland. Die Wasserwirtsch. 1965, 55, 315–321. [Google Scholar]
- Richter, D. Ein Beitrag zur Bestimmung der Verdunstung von freien Wasserflächen dargestellt am Beispiel des Stechlinsees; Akademie-Verlag: Berlin, Germany, 1969; Volume XI, 47p. [Google Scholar]
- Richter, D. Zur einheitlichen Berechnung der Wassertemperatur und der Verdunstung von freien Wasserflächen auf statistischer Grundlage; Akademie-Verlag: Berlin, Germany, 1977; Volume XVI, 38p. [Google Scholar]
- Richter, D. Ergebnisse einer statistischen Analyse der Daten aus dem Verdunstungskesselnetz der DDR; Akademie-Verlag: Berlin, Germany, 1978; Volume XVI, 35p. [Google Scholar]
- Richter, D. Das Langzeitverhalten von Niederschlag und Verdunstung und dessen Auswirkungen auf den Wasserhaushalt des Stechlinseegebietes; Selbstverlag. des Dt. Wetterdienstes: Offenbach am Main, Germany, 1997; Volume 201, 1267p. [Google Scholar]
- Richter, D.; Neubert, W.; Klämt, A. Temperatur und Wärmehaushalt des thermisch belasteten Stechlin- und Nehmitzsees; Akademie-Verlag: Berlin, Germany, 1979; Volume XVI, 43p. [Google Scholar]
- Kunz, M. Anwendung der Verdunstungstheorien in der Bewässerungsplanung bei zweckmäßig eingerichteten agrameteorologischen Stationen. Tropenlandwirt-J. Agric. Trop. Subtrop./Beiträge Trop. Landwirtsch. Veterinärmedizin 1972, 73, 7–22. [Google Scholar]
- Dyck, S.; Peschke, G. Grundlagen der Hydrologie; VEB Verlag für Bauwesen: Berlin, Germany, 1983; 388p. [Google Scholar]
- Vietinghoff, H. Die Verdunstung freier Wasserflächen–Grundlagen, Einflussfaktoren und Methoden der Ermittlung; UFO, Atelier für Gestaltung & Verlag GbR: Allensbach, Germany, 2000; 113p, Available online: http://www.gewaesserschutz-vietinghoff.de/medien/verd1.pdf (accessed on 28 December 2023).
- DWA. DWA-Regelwerk, Merkblatt DWA-M 504-1: Ermittlung der Verdunstung von Land- und Wasserflächen–Teil 1: Grundlagen, experimentelle Bestimmung der Landverdunstung, Gewässerverdunstung; Deutsche Vereinigung für Wasserwirtschaft, Abwasser und Abfall e.V. (DWA): Hennef, Germany, 2018. [Google Scholar]
- Monin, A.S.; Obuchow, A.M. Fundamentale Gesetzmäßigkeiten der turbulenten Vermischung in der bodennahen Schicht der Atmosphäre. In Sammelband zur Statistischen Theorie der Turbulenz; Kolmogorov, A.N., Obuchow, A.M., Jaglom, A.M., Monin, A.S., Goering, H., Eds.; Deutsche Akademie der Wissenschaften zu Berlin, Akademie-Verlag: Berlin, Germany, 1958; pp. 199–228. [Google Scholar]
- Monin, A.S.; Obukhov, A.M. 1990: Basic laws of turbulent mixing in the atmosphere near the ground. Tr. Akad. Nauk SSST Geofiz. Instituta 1954, 24, 163–187, Reprinted in Selected Papers on Turbulence in a Refractive Medium; Andreas, E.L., Ed.; SPIE Milestone Series; SPIE Optical Engineering Press: Bellingham, WA, USA, 1990; Volume MS 25, pp. 300–312. [Google Scholar]
- Foken, T. 50 Jahre Monin-Obukhov’sche Ähnlichkeitstheorie. Universität Bayreuth, Abt. Mikrometeorologie, Bayreuth, Deutschland. 2004. Available online: https://www.bayceer.uni-bayreuth.de/mm/de/pub/html/2569605_Fo.pdf (accessed on 28 December 2023).
- Pal Arya, S. Introduction to Micrometeorology; Academic Press, Inc.: San Diego, CA, USA, 1988; 303p. [Google Scholar]
- Foken, T. Turbulenter Energieaustausch zwischen Atmosphäre und Unterlage. Methoden, meßtechnische Realisierung sowie ihre Grenzen und Anwendungsmöglichkeiten; Berichte DWD; Selbstverlag des Deutschen Wetterdienstes: Offenbach, Germany, 1990; Volume 180, 287p. [Google Scholar]
- Schmugge, T.J.; André, J. Land Surface Evaporation. Measurement and Parameterization; Springer: New York, NY, USA, 1991; 424p. [Google Scholar]
- Garratt, J.R. The Atmospheric Boundary Layer; Cambridge Atmospheric and Space Science Series; Cambridge University Press: Cambridge, UK, 1992; 316p. [Google Scholar]
- Kaimal, J.C.; Finnigan, J.J. Atmospheric Boundary Layer Flows. Their Structure and Measurement; Oxford University Press: Oxford, UK, 1994; 289p. [Google Scholar]
- Stull, R.B. An Introduction to Boundary Layer Meteorology; Kluwer Academic Publishers: Dordrecht, Germany, 1997; 1670p. [Google Scholar]
- Etling, D. Theoretische Meteorologie. Eine Einführung, 3rd ed.; Springer: Berlin, Germany, 2010; 388p. [Google Scholar]
- Foken, T. Angewandte Meteorologie, 3rd ed.; Springer: Berlin, Germany, 2016; 394p. [Google Scholar]
- Emeis, S. Windenergie Meteorologie. Atmosphärenphysik für die Windenergieerzeugung, 2nd ed.; Springer: Berlin, Germany, 2022; 270p. [Google Scholar] [CrossRef]
- Foken, T.; Richter, S.H. Konzept der Parametrisierung des Austauschs von Energie und Beimengungen in der bodennahen Luftschicht. Abh. Meteor. Dienst. DDR 1991, 146, 7–13. [Google Scholar]
- Doms, G.; Förstner, J.; Heise, E.; Herzog, H.-J.; Mironov, D.; Raschendorfer, M.; Reinhardt, T.; Ritter, B.; Schrodin, R.; Schulz, J.-P.; et al. Part II: Physical Parameterizations (COSMO 5.00). A Description of the Nonhydrostatic Regional COSMO-Model; Schättler, U., Ed.; COSMO—Consortium for Small-Scale Modelling, Deutscher Wetterdienst: Offenbach, Germany, 2013; 156p, Available online: https://www.cosmo-model.org/content/model/cosmo/coreDocumentation/cosmo_physics_5.00.pdf (accessed on 28 December 2023).
- ECMWF-IFS. IFS Documentation CY47R3–Part IV Physical Processes. IFS Documentation CY47R3, 4, ECMWF. 2021. Available online: https://www.ecmwf.int/en/elibrary/81271-ifs-documentation-cy47r3-part-iv-physical-processes (accessed on 28 December 2023).
- Large, W.G.; Pond, S. Open ocean momentum flux measurements in moderate to strong winds. J. Phys. Oceanogr. 1981, 11, 324–336. [Google Scholar] [CrossRef]
- Large, W.G.; Pond, S. Sensible and latent heat flux measurements over the ocean. J. Phys. Oceanogr. 1982, 12, 464–482. [Google Scholar] [CrossRef]
- Large, W.G.; Danabasoglu, G.; Doney, S.C.; McWilliams, J.C. Sensitivity to surface forcing and boundary layer mixing in a global ocean model: Annual-mean climatology. J. Phys. Oceanogr. 1997, 27, 2418–2447. [Google Scholar] [CrossRef]
- Large, W.G.; Yeager, S.G. Diurnal to Decadal Global Forcing for Ocean and Sea-Ice Models: The Data Sets and Flux Climatologie; NCAR Technical Note, NCAR/TN-460+STR, Climate and Global Dynamics Division; National Center for Atmospheric Research: Boulder, Colorado, 2004; Available online: https://www.clivar.org/sites/default/files/documents/wgomd/Large%2526Yeager2004.pdf (accessed on 28 December 2023).
- Large, W.G.; Yeager, S.G. The global climatology of an interannually varying air–sea flux data set. Clim. Dyn. 2009, 33, 341–364. [Google Scholar] [CrossRef]
- Brodeau, L.; Barnier, B.; Gulev, S.K.; Woods, C. Climatologically significant effects of some approximations in the bulk parameterizations of turbulent air–sea fluxes. J. Phys. Oceanogr. 2017, 47, 5–28. [Google Scholar] [CrossRef]
- Webster, P.J.; Lukas, R. TOGA COARE: The Coupled Ocean–Atmosphere Response Experiment. Bull. Amer. Meteor. Soc. 1992, 73, 1377–1416. [Google Scholar] [CrossRef]
- Fairall, C.W.; Bradley, E.F.; Godfrey, J.S.; Wick, G.A.; Edson, J.B.; Young, G.S. Cool-skin and warm-layer effects on sea surface temperature. J. Geophys. Res. Oceans 1996a, 101, 1295–1308. [Google Scholar] [CrossRef]
- Fairall, C.W.; Bradley, E.F.; Rogers, D.P.; Edson, J.B.; Young, G.S. Bulk parameterization of air-sea fluxes for Tropical Ocean-Global Atmosphere Coupled–Ocean Atmosphere Response Experiment. J. Geophys. Res.: Oceans 1996b, 101, 3747–3764. [Google Scholar] [CrossRef]
- Fairall, C.W.; White, A.B.; Edson, J.B.; Hare, J.E. Integrated shipboard measurements of the marine boundary layer. J. Atmos. Ocean. Technol. 1997, 14, 338–359. [Google Scholar] [CrossRef]
- Fairall, C.W.; Bradley, E.F.; Hare, J.E.; Grachev, A.A.; Edson, J.B. Bulk parameterization of air–sea fluxes: Updates and verification for the COARE algorithm. J. Clim. 2003a, 16, 571–591. [Google Scholar] [CrossRef]
- Fairall, C.W.; Bradley, E.F.; Hare, J.E.; Grachev, A.A.; Edson, J.B. Session 3 Part 1: Air-Sea Fluxes and Interfacial Processes. 3.1 Bulk Parameterization of Air-Sea Fluxes: Updates and Verification for the COARE Algorithm. In Proceedings of the 12th Conference on Interactions of the Sea and Atmosphere, American Meteorological Society, Long Beach, CA, USA, 8 February 2003; 2003b. Available online: https://ams.confex.com/ams/annual2003/techprogram/session_14791.htm (accessed on 28 December 2023).
- Fairall, C.W.; Yang, M.; Bariteau, L.; Edson, J.B.; Helmig, D.; McGillis, W.; Pezoa, S.; Hare, J.E.; Huebert, B.; Blomquist, B. Implementation of the coupled ocean-atmosphere response experiment flux algorithm with CO2, dimethyl sulfide, and O3. J. Geophys. Res. Ocean. 2011, 116, C00F06. [Google Scholar] [CrossRef]
- Andreas, E.L. Session 3 Part 1: Air-Sea Fluxes and Interfacial Processes. 3.4 An Algorithm to predict the Turbulent Air-Sea Fluxes in High-Wind, Spray Conditions. In Proceedings of the 12th Conference on Interactions of the Sea and Atmosphere, American Meteorological Society, Long Beach, CA, USA, 8 February 2003; Available online: https://ams.confex.com/ams/annual2003/techprogram/session_14791.htm (accessed on 28 December 2023).
- Andreas, E.L.; Persson, P.; Ola, G.; Hare, J.E. A bulk turbulent air–sea flux algorithm for high-wind, spray conditions. J. Phys. Oceanogr. 2008, 38, 1581–1596. [Google Scholar] [CrossRef]
- Brunke, M.A.; Fairall, C.W.; Zeng, X.; Eymard, L.; Curry, J.A. Session 3 Part 1: Air-Sea Fluxes and Interfacial Processes. 3.2 The Performance of SEA Surface Turbulent Flux Algorithms over the Global Oceans. In Proceedings of the 12th Conference on Interactions of the Sea and Atmosphere, American Meteorological Society, Long Beach, CA, USA, 8 February 2003; Available online: https://ams.confex.com/ams/annual2003/techprogram/session_14791.htm (accessed on 28 December 2023).
- Zeng, X.; Zhang, Q.; Johnson, D.; Tao, W.K. Session 3 Part 1: Air-Sea Fluxes and Interfacial Processes. 3.5 Parameterization of Wind Gustiness for the Computation of Ocean Surface Fluxes at different Spatial Scales. In Proceedings of the 12th Conference on Interactions of the Sea and Atmosphere, American Meteorological Society, Long Beach, CA, USA, 8 February 2003; Available online: https://ams.confex.com/ams/annual2003/techprogram/session_14791.htm (accessed on 28 December 2023).
- Edson, J.B.; Jampana, V.; Weller, R.A.; Bigorre, S.P.; Plueddemann, A.J.; Fairall, C.W.; Miller, S.D.; Mahrt, L.; Vickers, D.; Hersbach, H. On the exchange of momentum over the open ocean. J. Phys. Oceanogr. 2013, 43, 1589–1610. [Google Scholar] [CrossRef]
- Yusup, Y.; Kayode, J.S.; Alkarkhi, A.F.M. Experimental data on the air-sea energy fluxes at the tropical coastal ocean in the southern South China Sea. Data Brief. 2018, 19, 1477–1481. [Google Scholar] [CrossRef]
- Yu, L. Global air-sea fluxes of heat, fresh water, and momentum: Energy budget closure and unanswered questions. Ann. Rev. Mar. Sci. 2019, 11, 227–248. [Google Scholar] [CrossRef]
- Dyer, A.J.; Hicks, B.B. Flux-gradient relationships in the constant flux layer. Q. J. R. Meteor. Soc. 1970, 96, 715–721. [Google Scholar] [CrossRef]
- Dyer, A.J. A review of flux-profile relationship. Bound.-Layer Meteorol. 1974, 7, 363–372. Available online: https://link.springer.com/article/10.1007/BF00240838 (accessed on 8 January 2024). [CrossRef]
- Paulson, C.A. The mathematical representation of wind speed and temperature profiles in the unstable atmospheric surface layer. J. Appl. Meteorol. 1970, 9, 857–861. [Google Scholar] [CrossRef]
- Businger, J.A.; Wyngaard, J.C.; Izumi, Y.; Bradley, E.F. Flux-profile relationships in the atmospheric surface layer. J. Atmos. Sci. 1971, 28, 181–189. [Google Scholar] [CrossRef]
- Kaimal, J.C.; Wyngaard, J.C.; Izumi, Y.; Coté, O.R. Spectral characteristics of suface layer turbulence. Q. J. R. Meteorol. Soc. 1971, 98, 563–589. [Google Scholar]
- Kaimal, J.C.; Wyngaard, J.C.; Haugen, D.A.; Coté, O.R.; Izumi, Y.; Caughey, S.J.; Readings, C.J. Turbulence structure in the convective boundary layer. J. Atmos. Sci. 1976, 33, 2152–2169, Reprinted in Selected Papers on Turbulence in a Refractive Medium; Andreas, E.L., Ed.; SPIE Milestone Series; SPIE Optical Engineering Press: Bellingham, WA, USA, 1990; Volume MS 25, pp. 353–370. [Google Scholar] [CrossRef]
- Skeib, G. Zur Definition universeller Funktionen für die Gradienten von Windgeschwindigkeit und Temperatur in der bodennahen Luftschicht. Z. Meteorol. 1980, 30, 23–32. [Google Scholar]
- Foken, T.; Skeib, G. Profile measurements in the atmospheric near-surface layer and the use of suitable universal functions for the determination of the turbulent energy exchange. Bound.-Layer Meteorol. 1983, 25, 55–62. [Google Scholar] [CrossRef]
- Skeib, G.; Richter, S.H. Praktische Anwendungen voll normierter universeller Funktionen für das turbulente Stromfeld in der bodennahen Luftschicht. Z. Meteorol. 1984, 34, 247–252. [Google Scholar]
- Holtslag, A.A.M. Surface Fluxes and Boundary Layer Scaling. Models and Applications; Scientific Report WR 87-2(FM); Koninklijk Nederlands Meteorologisch Instituut: De Bilt, The Netherlands, 1987. [Google Scholar]
- Högström, U. Non-dimensional wind and temperature profiles in the atmospheric surface layer: A re-evaluation. Bound.-Layer Meteorol. 1988, 42, 55–78. Available online: https://link.springer.com/article/10.1007/BF00119875 (accessed on 8 January 2024). [CrossRef]
- Foken, T. Die universelle Funktion nach Skeib–Grundlage für Maßstabsbetrachtungen in der atmosphärischen Bodenschicht. Z. Meteorol. 1991, 41, 1–7. [Google Scholar]
- Smith, S.D. Coefficients for sea surface wind stress, heat flux, and wind profiles as a function of wind speed and temperature. J. Geophys. Res. Ocean. 1988, 93, 15467–15472. [Google Scholar] [CrossRef]
- Miller, M.J.; Beljaars, A.C.M.; Palmer, T.N. The sensitivity of the ECMWF model to the parameterization of evaporation from the tropical oceans. J. Clim. 1992, 5, 418–434. [Google Scholar] [CrossRef]
- Beljaars, A.C.M. The parametrization of surface fluxes in large-scale models under free convection. Q. J. R. Meteor. Soc. 1995, 121, 255–270. [Google Scholar] [CrossRef]
- Liu, G.; Liu, Y.; Endo, S. Evaluation of surface flux parameterizations with long-term ARM observations. Mon. Wea. Rev. 2013, 141, 773–797. [Google Scholar] [CrossRef]
- Owen, P.R.; Thomson, W.R. Heat transfer across rough surfaces. J. Fluid. Mech. 1963, 15, 321–334. [Google Scholar] [CrossRef]
- Kondo, J. Air-sea bulk transfer coefficients in diabatic conditions. Bound.-Layer Meteorol. 1975, 9, 91–112. [Google Scholar] [CrossRef]
- Liu, W.T.; Katsaros, K.B.; Businger, J.A. Bulk parameterization of air-sea exchanges of heat and water vapor including the molecular constraints at the interface. J. Atmos. Sci. 1979, 36, 1722–1735. [Google Scholar] [CrossRef]
- Foken, T. Ein Energieaustauschmodell für den fühlbaren Wärmestrom in der Atmosphäre unter Berücksichtigung der molekularen Temperaturgrenzschicht. In Proceedings of the Tagung Transportprozesse in Turbulenten Strömungen, Eisenach, Germany, 20–24 December 1978; Akademie Verlag: Berlin, Germany, 1979a. Vorträge Heft 1. pp. 85–93. [Google Scholar]
- Foken, T. Vorschlag eines verbesserten Energieaustauschmodells mit Berücksichtigung der molekularen Grenzschicht der Atmosphäre. Z. Meteorol. 1979b, 29, 31–39. [Google Scholar]
- Foken, T. The parameterisation of the energy exchange across the air-sea interface. Dyn. Atmos. Ocean. 1984, 8, 297–305. [Google Scholar] [CrossRef]
- Foken, T. An operational model of the energy exchange across the air-sea interface. Z. Meteorol. 1986, 36, 354–359. [Google Scholar]
- Richter, S.H.; Skeib, G. Ein Verfahren zur Parametrisierung von Austauschprozessen in der bodennahen Luftschicht. Abh. Meteor. Dienst. DDR 1991, 146, 15–22. [Google Scholar]
- Brutsaert, W. A theory for local evaporation (or heat transfer) from rough and smooth surfaces at ground level. Water Resour. Res. 1975, 11, 543–550. [Google Scholar] [CrossRef]
- Soloviev, A.V.; Schlüssel, P. Parameterization of the cool skin of the ocean and of the air-ocean gas transfer on the basis of modeling surface renewal. J. Phys. Oceanogr. 1994, 24, 1339–1346. [Google Scholar] [CrossRef]
- Soloviev, A.; Schlüssel, P. Comments on “Air–sea gas transfer: Mechanisms and parameterization”. J. Phys. Oceanogr. 1998, 28, 1643–1645. [Google Scholar] [CrossRef]
- Clayson, C.A.; Fairall, C.W.; Curry, J.A. Evaluation of turbulent fluxes at the ocean surface using surface renewal theory. J. Geophys. Res. Oceans 1996, 101, 28503–28513. [Google Scholar] [CrossRef]
- Zappa, C.J.; Jessup, A.T.; Yeh, H. Skin layer recovery of free-surface wakes: Relationship to surface renewal and dependence on heat flux and background turbulence. J. Geophys. Res. Oceans 1998, 103, 21711–21722. [Google Scholar] [CrossRef]
- Mengistu, M.G.; Savage, M.J. Open water evaporation estimation for a small shallow reservoir in winter using surface renewal. J. Hydrol. 2010, 380, 27–35. [Google Scholar] [CrossRef]
- Horvath, I.R.; Chatterjee, S.G. A surface renewal model for unsteady-state mass transfer using the generalized Danckwerts age distribution function. R. Soc. Open Sci. 2018, 5, 172423. [Google Scholar] [CrossRef]
- Hu, Y.; Buttar, N.A.; Tanny, J.; Snyder, R.L.; Savage, M.J.; Lakhiar, I.A. Surface renewal application for estimating evapotranspiration: A review. Adv. Meteorol. 2018, 2018, 1690714. [Google Scholar] [CrossRef]
- Sverdrup, H.U. On the evaporation from the oceans. J. Mar. Res. 1937, 8, 3–14. [Google Scholar]
- Kondo, J.; Saigusa, N.; Sato, T. A parameterization of evaporation from bare soil surfaces. J. Appl. Met. Clim. 1990, 29, 385–389. [Google Scholar] [CrossRef]
- Ruprecht, E.; Simmer, C. Fluxes of latent heat over the oceans: Climatological studies and application of satellite observations. Dyn. Atmos. Ocean. 1991, 16, 111–121. [Google Scholar] [CrossRef]
- Zhang, G.J.; McPhaden, M.J. The relationship between sea surface temperature and latent heat flux in the equatorial Pacific. J. Clim. 1995, 8, 589–605. [Google Scholar] [CrossRef]
- Liu, X.; Xie, S.; Ghan, S.J. Evaluation of a new mixed-phase cloud microphysics parameterization with CAM3 single-column model and M–PACE observations. Geophys. Res. Lett. 2007, 34, L23712. [Google Scholar] [CrossRef]
- Yu, L. Global variations in oceanic evaporation (1958–2005): The role of the changing wind speed. J. Clim. 2007, 20, 5376–5390. [Google Scholar] [CrossRef]
- Rosenberg, A.M. Measuring and Modeling Oceanic Air-Sea Fluxes. M.S. thesis, University of Connecticut, Storrs, CT, USA, 2016. Available online: https://opencommons.uconn.edu/gs_theses/925 (accessed on 28 December 2023).
- Kumar, B.P.; Cronin, M.F.; Joseph, S.; Ravichandran, M.; Sureshkumar, N. Latent heat flux sensitivity to sea surface temperature: Regional perspectives. J. Clim. 2017, 30, 129–143. [Google Scholar] [CrossRef]
- Hogan, L. Air-Sea Fluxes in the Western Tropical Atlantic. Bachelor’s Thesis, Yale University, New Haven, CT, USA, 2020. [Google Scholar]
- Reeves Eyre, J.E.J.; Zeng, X.; Zhang, K. Ocean surface flux algorithm effects on earth system model energy and water cycles. Front. Mar. Sci. 2021, 8, 17. [Google Scholar] [CrossRef]
- Song, X.; Xie, X.; Qiu, B.; Cao, H.; Xie, S.-P.; Chen, Z.; Yu, W. Air-sea latent heat flux anomalies induced by oceanic submesoscale processes: An observational case study. Front. Mar. Sci. 2022, 9, 850207. [Google Scholar] [CrossRef]
- Kruspe, G. On moisture-flux parameterization. Bound.-Layer Meteorol. 1977, 11, 55–63. [Google Scholar] [CrossRef]
- Bunker, A.F. Computations of surface energy flux and annual air–sea interaction cycles of the North Atlantic Ocean. Mon. Wea. Rev. 1976, 104, 1122–1140. [Google Scholar] [CrossRef]
- Panin, G.N.; Nasonov, A.E.; Foken, T.; Lohse, H. On the parameterisation of evaporation and sensible heat exchange for shallow lakes. Theor. Appl. Climatol. 2006, 85, 123–129. [Google Scholar] [CrossRef]
- Babkin, V.I.; Evaporation from the Surface of the Globe. Encyclopedia of Life Support Systems (EOLSS). Hydrological Cycle–Vol. II, UNESCO-EOLSS. 2023. Available online: https://www.eolss.net/index.aspx (accessed on 28 December 2023).



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feistel, R.; Hellmuth, O. Irreversible Thermodynamics of Seawater Evaporation. J. Mar. Sci. Eng. 2024, 12, 166. https://doi.org/10.3390/jmse12010166
Feistel R, Hellmuth O. Irreversible Thermodynamics of Seawater Evaporation. Journal of Marine Science and Engineering. 2024; 12(1):166. https://doi.org/10.3390/jmse12010166
Chicago/Turabian StyleFeistel, Rainer, and Olaf Hellmuth. 2024. "Irreversible Thermodynamics of Seawater Evaporation" Journal of Marine Science and Engineering 12, no. 1: 166. https://doi.org/10.3390/jmse12010166
APA StyleFeistel, R., & Hellmuth, O. (2024). Irreversible Thermodynamics of Seawater Evaporation. Journal of Marine Science and Engineering, 12(1), 166. https://doi.org/10.3390/jmse12010166







