Modulation Effects of Internal-Wave Evolution on Acoustic Modal Intensity Fluctuations in a Shallow-Water Waveguide
Abstract
:1. Introduction
2. Theoretical Derivation of Modulation Effects in Modal Intensity Fluctuations
2.1. Stochastic Coupled Mode Equation and Its Dyson Series Solution
2.2. Modulation of Evolving ISWs on Modal Intensity Fluctuations
3. Sea-Trial Observation and Sound–Speed Reconstruction
3.1. Experiment Description
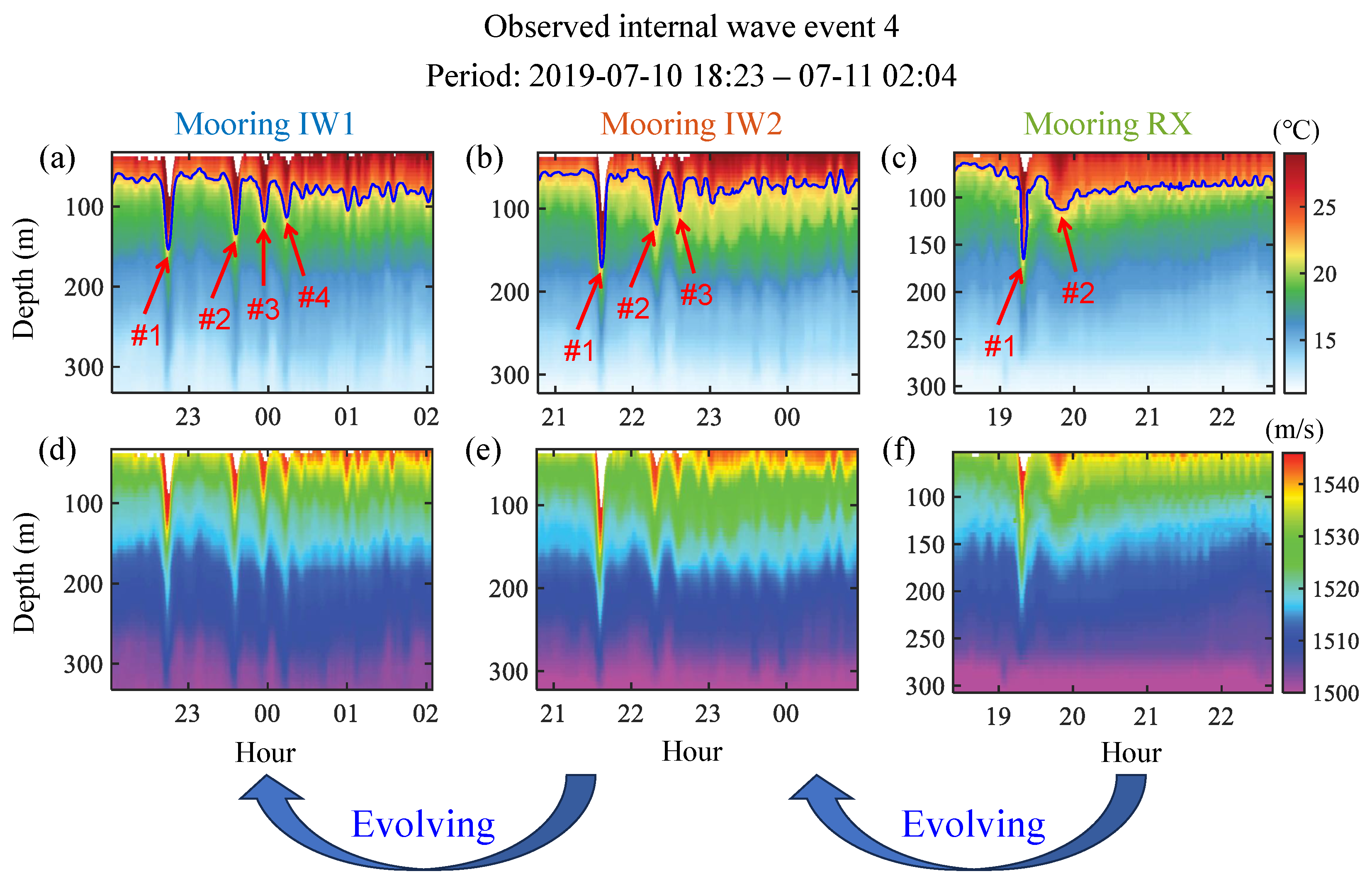
3.2. Oceanographic Observation
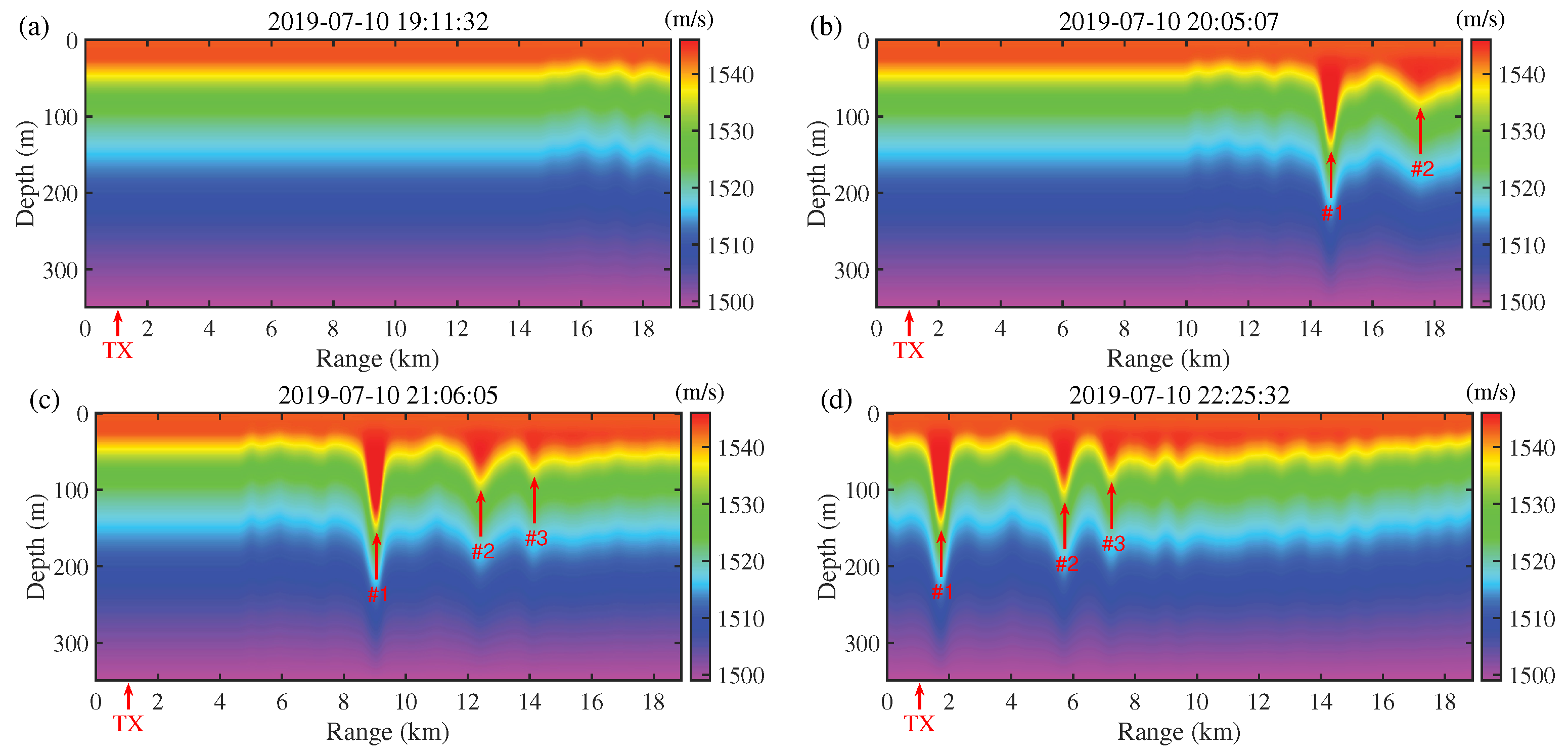
3.3. Reconstruction of Sound–Speed Fields
4. PE Simulation and Phenomenon Analysis of Modulation Effects
4.1. Configuration and Method
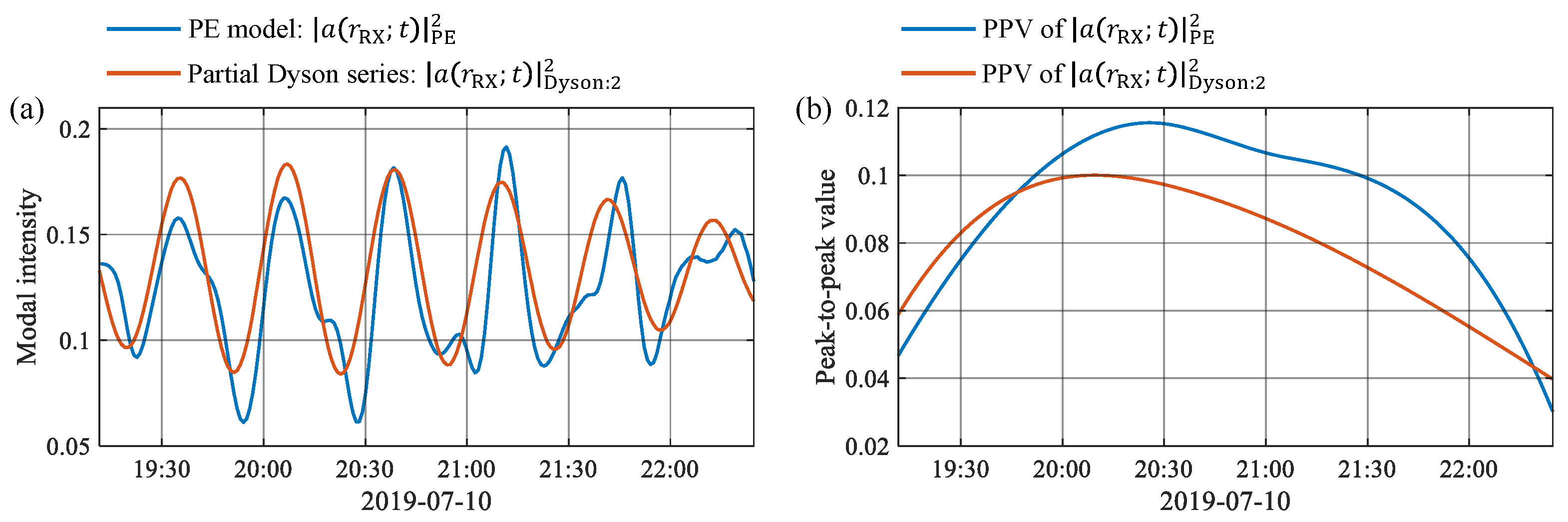
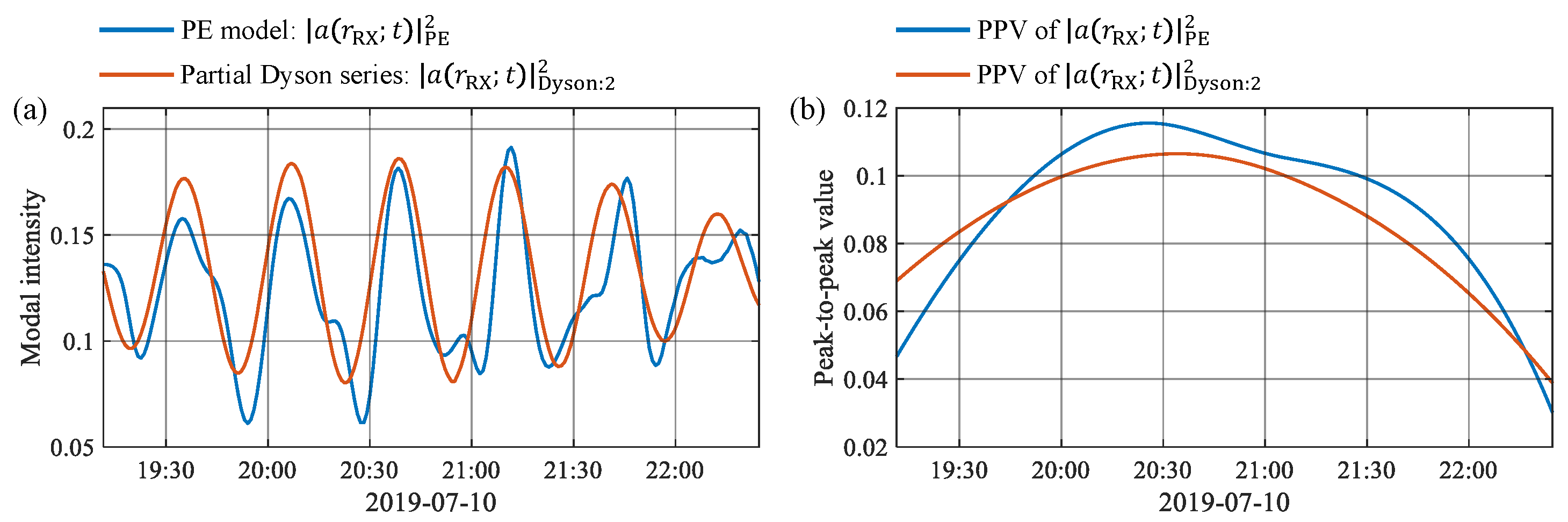
4.2. Simulation Results and Analysis
5. Mechanism Study of Modulation Effects Caused by Deformation and Dispersion
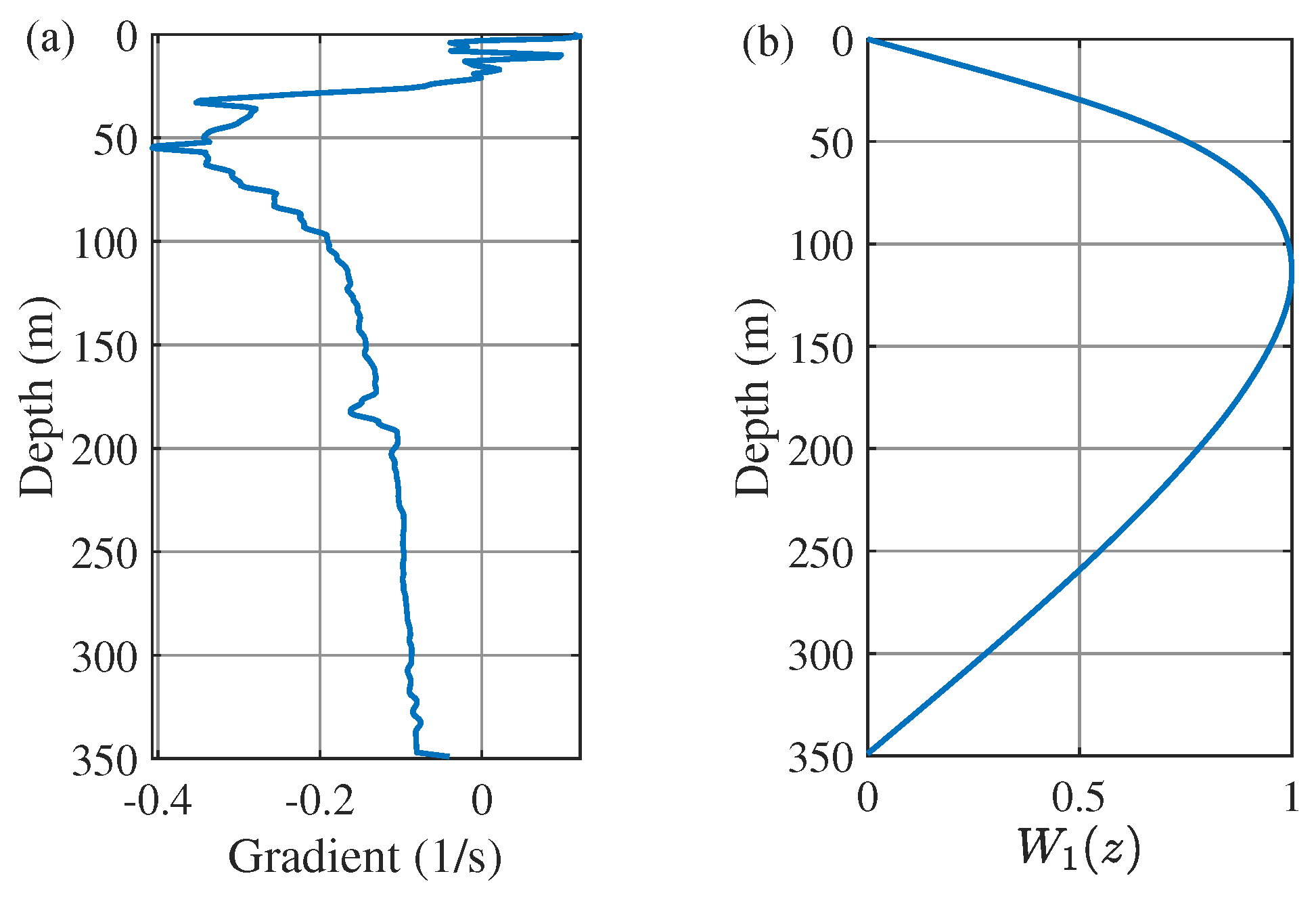
5.1. Study Approach
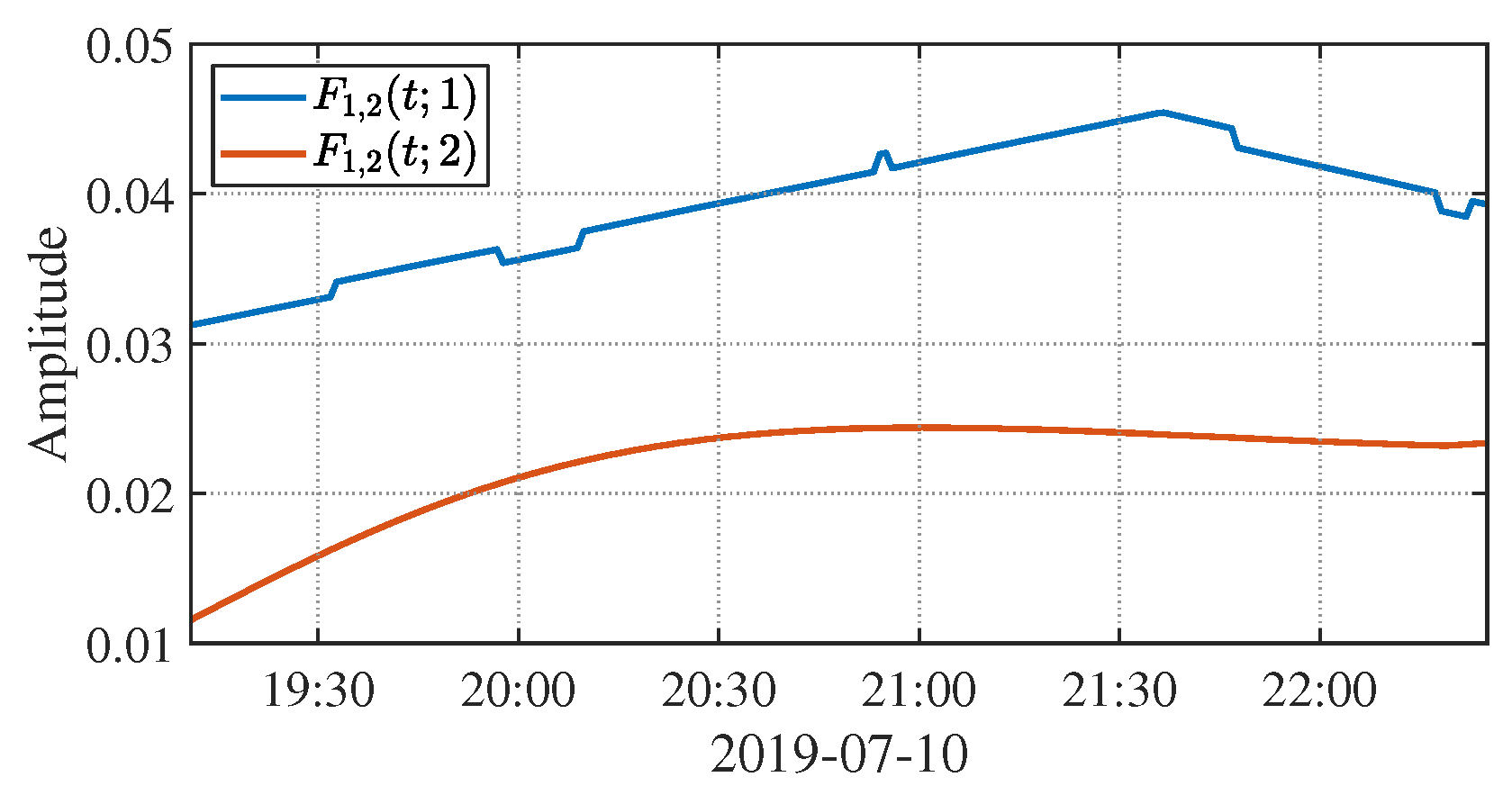
5.2. Modulation Effect Induced by Deformation
5.3. Modulation Effect Induced by Dispersion
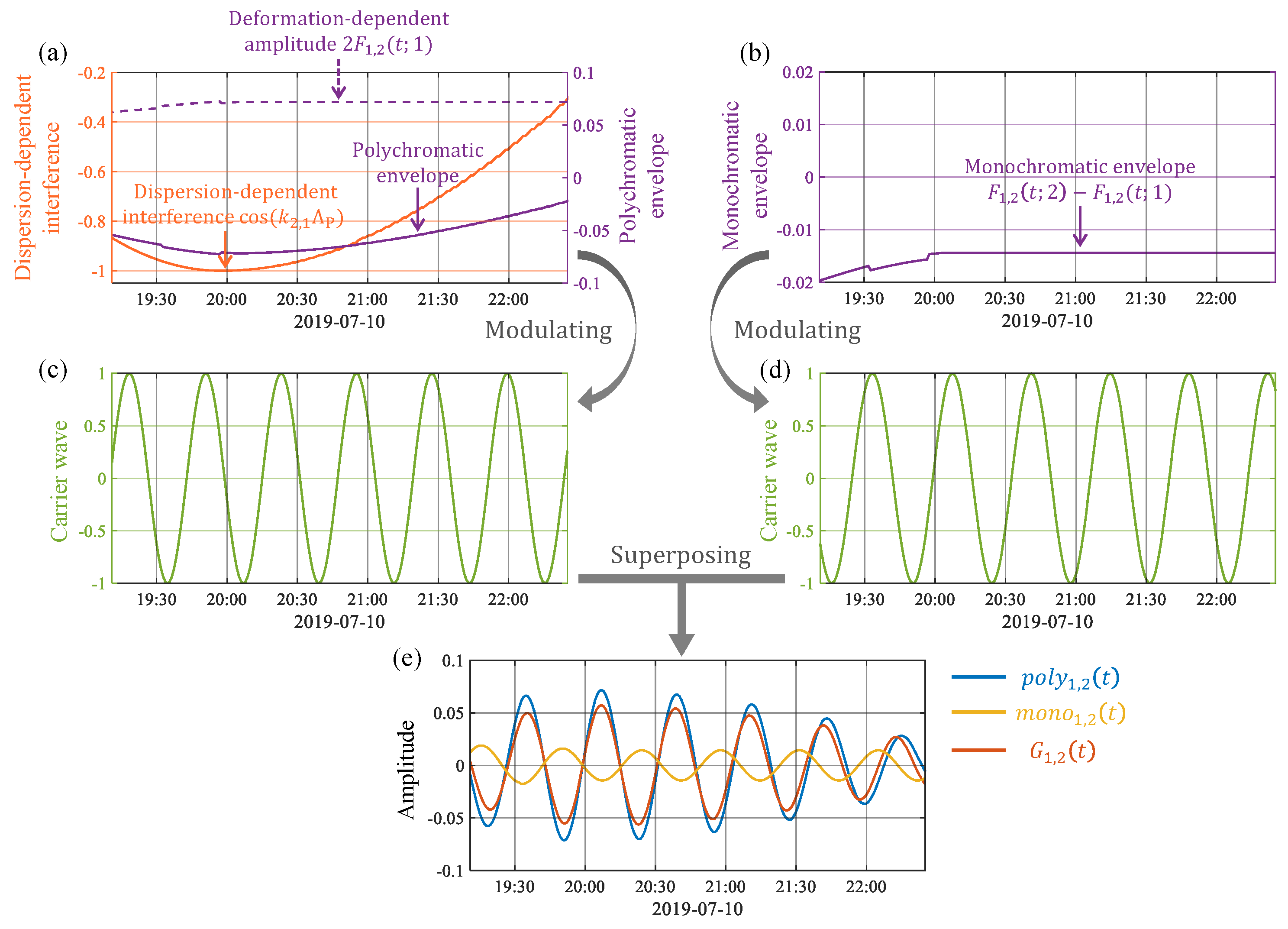
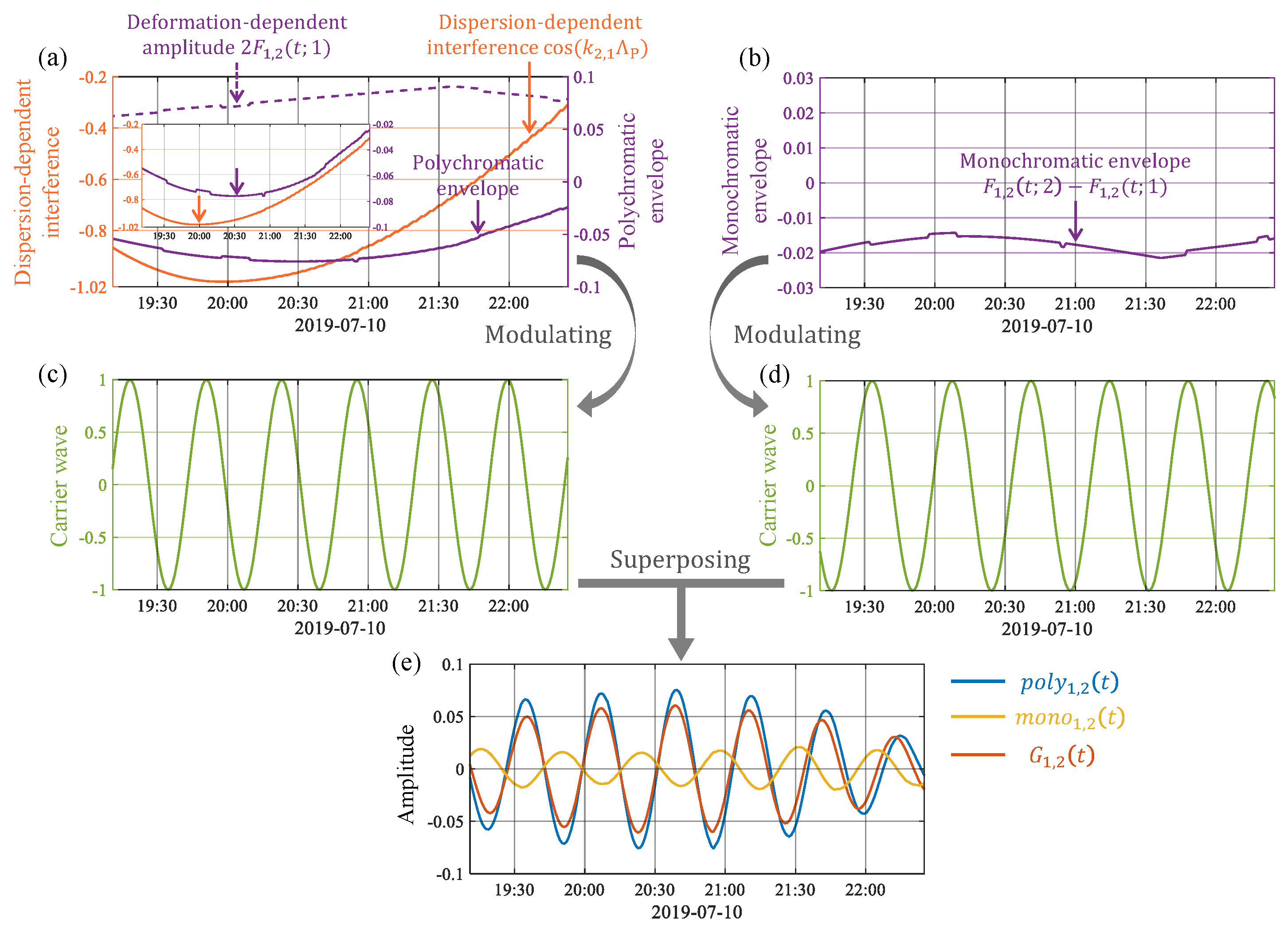
5.4. Modulation Effects Induced by Both Deformation and Dispersion
6. Conclusions and Discussions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CW | Continuous wave |
| DEPTS | Dispersive evolutionary propagated thermistor string |
| ISW | Internal solitary wave |
| LFM | Linear frequency-modulated |
| PE | Parabolic equation |
| PPV | Peak-to-peak value |
| SCS | South China Sea |
| SSP | Sound–speed profile |
| TP | Temperature-pressure |
| VLA | Vertical line array |
References
- Gerkema, T.; Zimmerman, J.T.F. An Introduction to Internal Waves. Lecture Notes, Royal Netherlands Institute for Sea Research. 2008. Available online: https://www.vliz.be/imisdocs/publications/ocrd/60/307760.pdf (accessed on 3 March 2023).
- Apel, J.R.; Badiey, M.; Chiu, C.S.; Finette, S.; Headrick, R.; Kemp, J.; Lynch, J.F.; Newhall, A.; Orr, M.H.; Pasewark, B.H.; et al. An overview of the 1995 SWARM shallow-water internal wave acoustic scattering experiment. IEEE J. Ocean. Eng. 1997, 22, 465–500. [Google Scholar] [CrossRef]
- Apel, J.R.; Ostrovsky, L.A.; Stepanyants, Y.A.; Lynch, J.F. Internal solitons in the ocean and their effect on underwater sound. J. Acoust. Soc. Am. 2007, 121, 695–722. [Google Scholar] [CrossRef]
- Colosi, J.A.; Duda, T.F.; Lin, Y.T.; Lynch, J.F.; Newhall, A.E.; Cornuelle, B.D. Observations of sound-speed fluctuations on the New Jersey continental shelf in the summer of 2006. J. Acoust. Soc. Am. 2012, 131, 1733–1748. [Google Scholar] [CrossRef] [PubMed]
- Simmons, H.; Chang, M.H.; Chang, Y.T.; Chao, S.Y.; Fringer, O.; Jackson, C.R.; Ko, D.S. Modeling and prediction of internal waves in the South China Sea. Oceanography 2011, 24, 88–99. [Google Scholar] [CrossRef]
- Farmer, D.M.; Alford, M.H.; Lien, R.C.; Yang, Y.J.; Chang, M.H.; Li, Q. From Luzon Strait to Dongsha Plateau: Stages in the life of an internal wave. Oceanography 2011, 24, 64–77. [Google Scholar] [CrossRef]
- Alford, M.H.; Peacock, T.; MacKinnon, J.A.; Nash, J.D.; Buijsman, M.C.; Centurioni, L.R.; Chao, S.Y.; Chang, M.H.; Farmer, D.M.; Fringer, O.B.; et al. The formation and fate of internal waves in the South China Sea. Nature 2015, 521, 65–69. [Google Scholar] [CrossRef]
- Rodriguez, O.C.; Jesus, S.; Stephan, Y.; Demoulin, X.; Porter, M.; Coelho, E. Nonlinear soliton interaction with acoustic signals: Focusing effects. J. Comput. Acoust. 2000, 8, 347–363. [Google Scholar] [CrossRef]
- Sagers, J.D. Predicting Acoustic Intensity Fluctuations Induced by Nonlinear Internal Waves in a Shallow Water Waveguide. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2012. [Google Scholar]
- Rouseff, D.; Turgut, A.; Wolf, S.N.; Finette, S.; Orr, M.H.; Pasewark, B.H.; Apel, J.R.; Badiey, M.; Chiu, C.S.; Headrick, R.H.; et al. Coherence of acoustic modes propagating through shallow water internal waves. J. Acoust. Soc. Am. 2002, 111, 1655–1666. [Google Scholar] [CrossRef] [PubMed]
- Zhou, J.X.; Zhang, X.Z.; Rogers, P.H. Resonant interaction of sound wave with internal solitons in the coastal zone. J. Acoust. Soc. Am. 1991, 90, 2042–2054. [Google Scholar] [CrossRef]
- Preisig, J.C.; Duda, T.F. Coupled acoustic mode propagation through continental-shelf internal solitary waves. IEEE J. Ocean. Eng. 1997, 22, 256–269. [Google Scholar] [CrossRef]
- Apel, J.R. A New Analytical Model for Internal Solitons in the Ocean. J. Phys. Oceanogr. 2003, 33, 2247–2269. [Google Scholar] [CrossRef]
- Frank, S.D.; Badiey, M.; Lynch, J.F.; Siegmann, W.L. Analysis and modeling of broadband airgun data influenced by nonlinear internal waves. J. Acoust. Soc. Am. 2004, 116, 3404–3422. [Google Scholar] [CrossRef]
- Colosi, J.A. Acoustic mode coupling induced by shallow water nonlinear internal waves: Sensitivity to environmental conditions and space-time scales of internal waves. J. Acoust. Soc. Am. 2008, 124, 1452–1464. [Google Scholar] [CrossRef]
- Dyson, F.J. The radiation theories of Tomonaga, Schwinger, and Feynman. Phys. Rev. 1949, 75, 486–502. [Google Scholar] [CrossRef]
- Dozier, L.B.; Tappert, F.D. Statistics of normal mode amplitudes in a random ocean. I. Theory. J. Acoust. Soc. Am. 1978, 63, 353–365. [Google Scholar] [CrossRef]
- Dozier, L.B.; Tappert, F.D. Statistics of normal mode amplitudes in a random ocean. II. Computations. J. Acoust. Soc. Am. 1978, 64, 533–547. [Google Scholar] [CrossRef]
- Jiang, Y.; Grigorev, V.; Katsnelson, B. Sound field fluctuations in shallow water in the presence of moving nonlinear internal waves. J. Mar. Sci. Eng. 2022, 10, 119. [Google Scholar] [CrossRef]
- Gao, F.; Hu, P.; Xu, F.; Li, Z.; Qin, J. Effects of Internal Waves on Acoustic Temporal Coherence in the South China Sea. J. Mar. Sci. Eng. 2023, 11, 374. [Google Scholar] [CrossRef]
- Jensen, F.B.; Kuperman, W.A.; Porter, M.B.; Schmidt, H. Computational Ocean Acoustics, 2nd ed.; Springer: New York, NY, USA, 2011. [Google Scholar]
- Finette, S.; Orr, M.H.; Turgut, A.; Apel, J.R.; Badiey, M.; Chiu, C.S.; Headrick, R.H.; Kemp, J.N.; Lynch, J.F.; Newhall, A.E.; et al. Acoustic field variability induced by time evolving internal wave fields. J. Acoust. Soc. Am. 2000, 108, 957–972. [Google Scholar] [CrossRef]
- Colosi, J.A. Sound Propagation through the Stochastic Ocean; Cambridge University Press: New York, NY, USA, 2016. [Google Scholar]
- Yang, Y.J.; Fang, Y.C.; Chang, M.H.; Ramp, S.R.; Kao, C.C.; Tang, T.Y. Observations of second baroclinic mode internal solitary waves on the continental slope of the northern South China Sea. J. Geophys. Res. Ocean. 2009, 114, C10003. [Google Scholar] [CrossRef]
- Weatherall, P.; Marks, K.M.; Jakobsson, M.; Schmitt, T.; Tani, S.; Arndt, J.E.; Rovere, M.; Chayes, D.; Ferrini, V.; Wigley, R. A new digital bathymetric model of the world’s oceans. Earth Space Sci. 2015, 2, 331–345. [Google Scholar] [CrossRef]
- Li, Q.; Sun, C.; Xie, L.; Huang, X. Reconstructions of time-evolving sound-speed fields perturbed by deformed and dispersive internal solitary waves in shallow water. Chin. Phys. B 2023. submitted. [Google Scholar]
- Chiu, L.Y.S.; Reeder, D.B.; Chang, Y.Y.; Chen, C.F.; Chiu, C.S.; Lynch, J.F. Enhanced acoustic mode coupling resulting from an internal solitary wave approaching the shelfbreak in the South China Sea. J. Acoust. Soc. Am. 2013, 133, 1306–1319. [Google Scholar] [CrossRef] [PubMed]
- Lee, D.; McDaniel, S.T. Ocean acoustic propagation by finite difference methods. Comput. Math. Appl. 1987, 14, 305–423. [Google Scholar]
- Porter, M.B. The KRAKEN Normal Mode Program; Technical Report SM-245; SACLANT Undersea Research Centre: La Spezia, Italy, 1991. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Q.; Sun, C.; Xie, L.; Huang, X. Modulation Effects of Internal-Wave Evolution on Acoustic Modal Intensity Fluctuations in a Shallow-Water Waveguide. J. Mar. Sci. Eng. 2023, 11, 1686. https://doi.org/10.3390/jmse11091686
Li Q, Sun C, Xie L, Huang X. Modulation Effects of Internal-Wave Evolution on Acoustic Modal Intensity Fluctuations in a Shallow-Water Waveguide. Journal of Marine Science and Engineering. 2023; 11(9):1686. https://doi.org/10.3390/jmse11091686
Chicago/Turabian StyleLi, Qinran, Chao Sun, Lei Xie, and Xiaodong Huang. 2023. "Modulation Effects of Internal-Wave Evolution on Acoustic Modal Intensity Fluctuations in a Shallow-Water Waveguide" Journal of Marine Science and Engineering 11, no. 9: 1686. https://doi.org/10.3390/jmse11091686
APA StyleLi, Q., Sun, C., Xie, L., & Huang, X. (2023). Modulation Effects of Internal-Wave Evolution on Acoustic Modal Intensity Fluctuations in a Shallow-Water Waveguide. Journal of Marine Science and Engineering, 11(9), 1686. https://doi.org/10.3390/jmse11091686







