Wave Characteristics over a Dual Porous Submerged Breakwater Using a Fully Nonlinear Numerical Wave Tank with a Porous Domain
Abstract
:1. Introduction
2. Methods
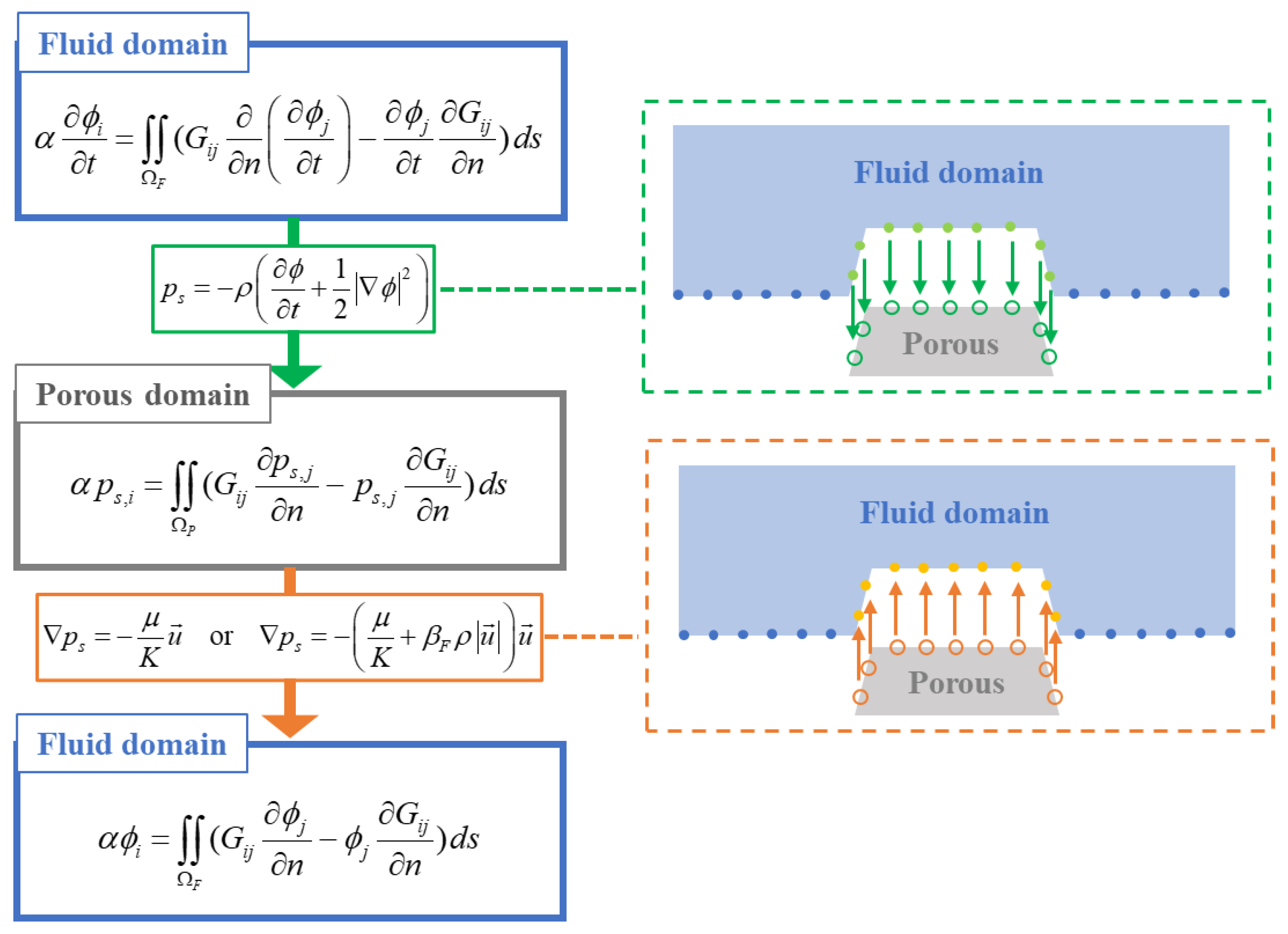
2.1. Fluid Domain
2.2. Porous Domain
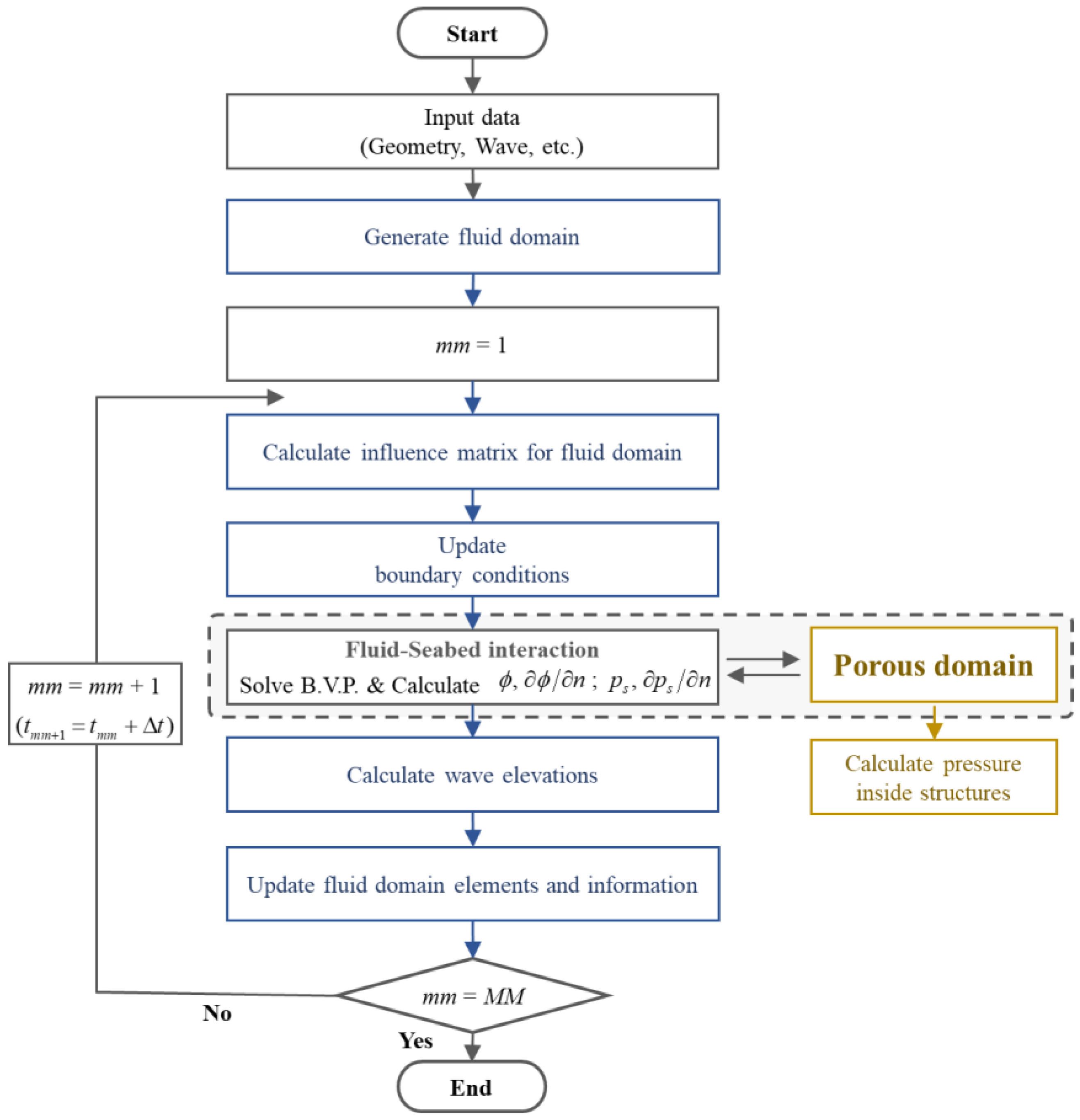
2.3. Numerical Calculation Process and Coupling of Fluid Domain and Porous Domain
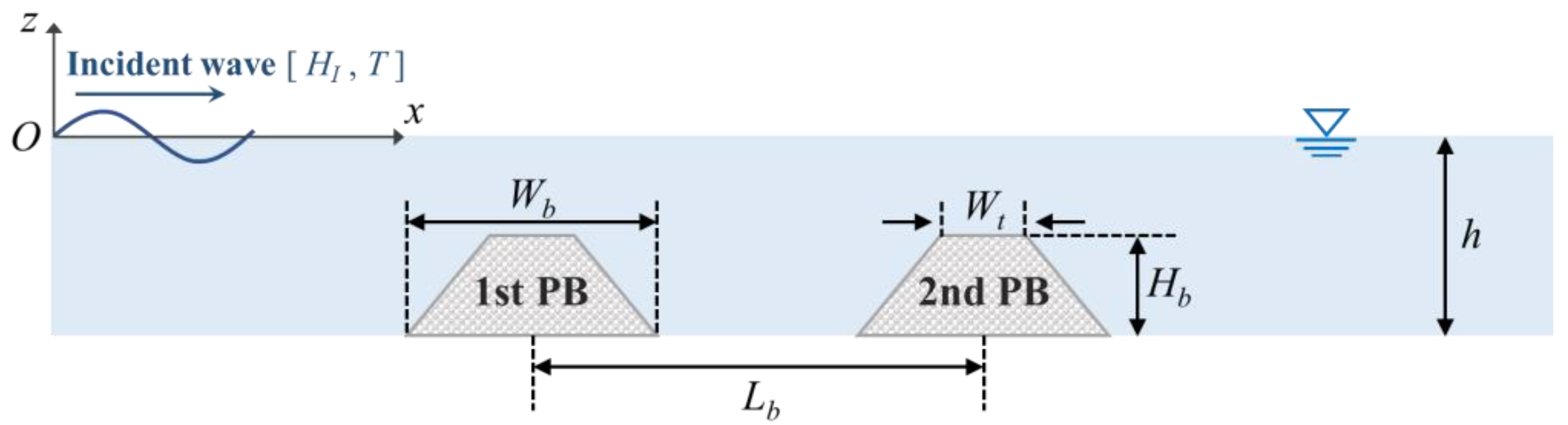
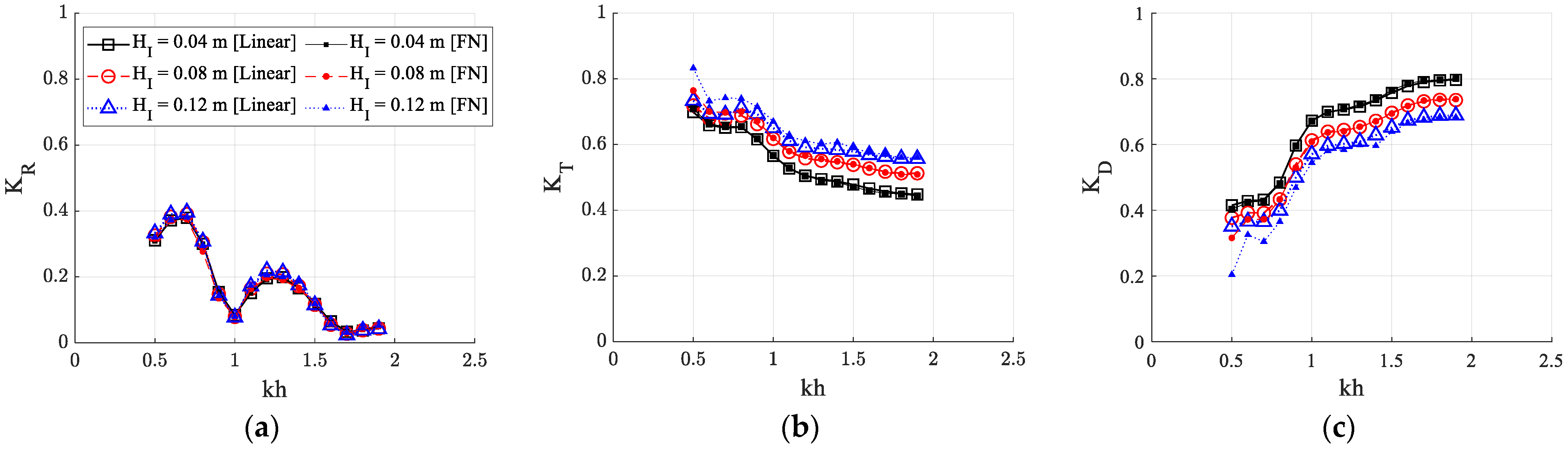
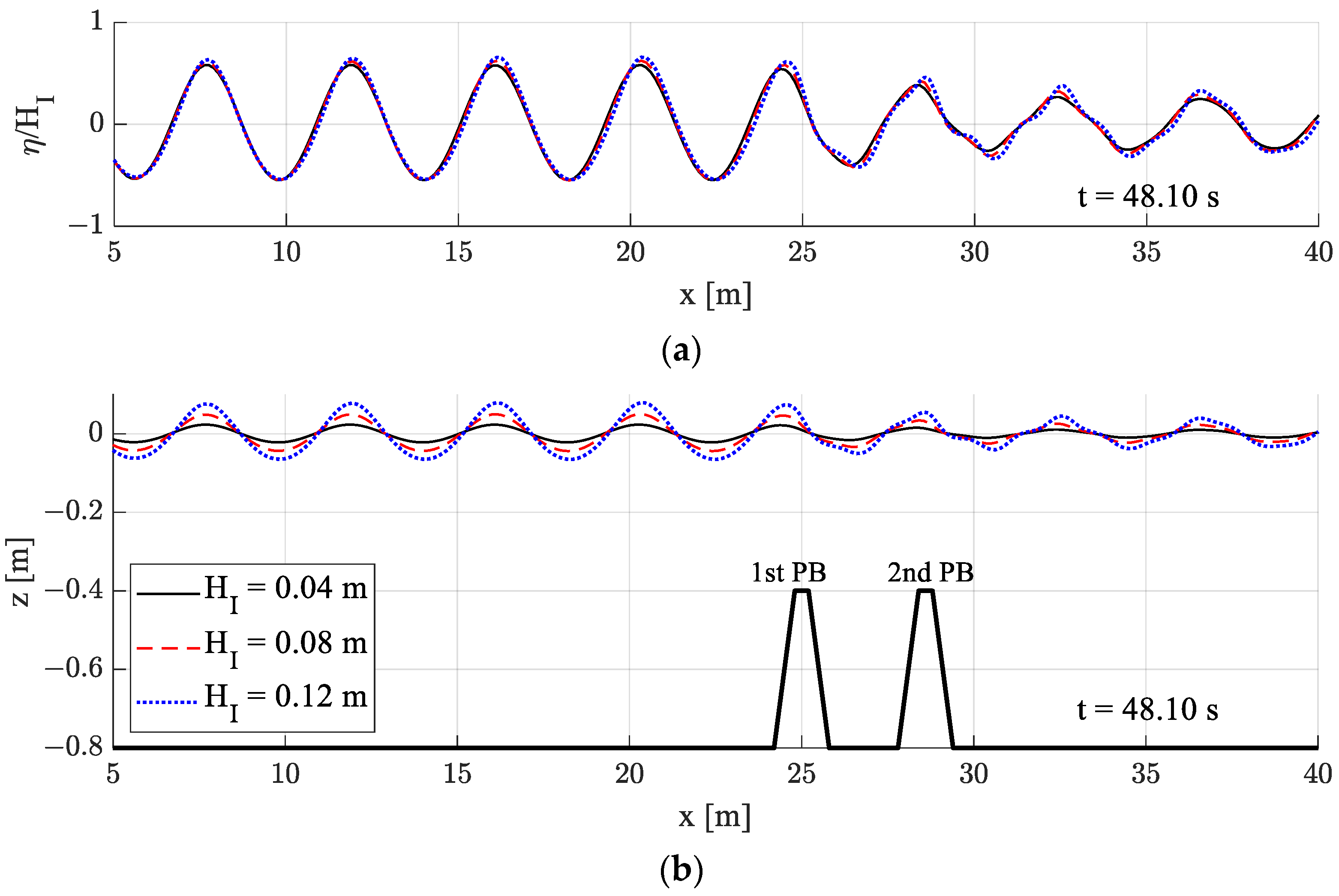
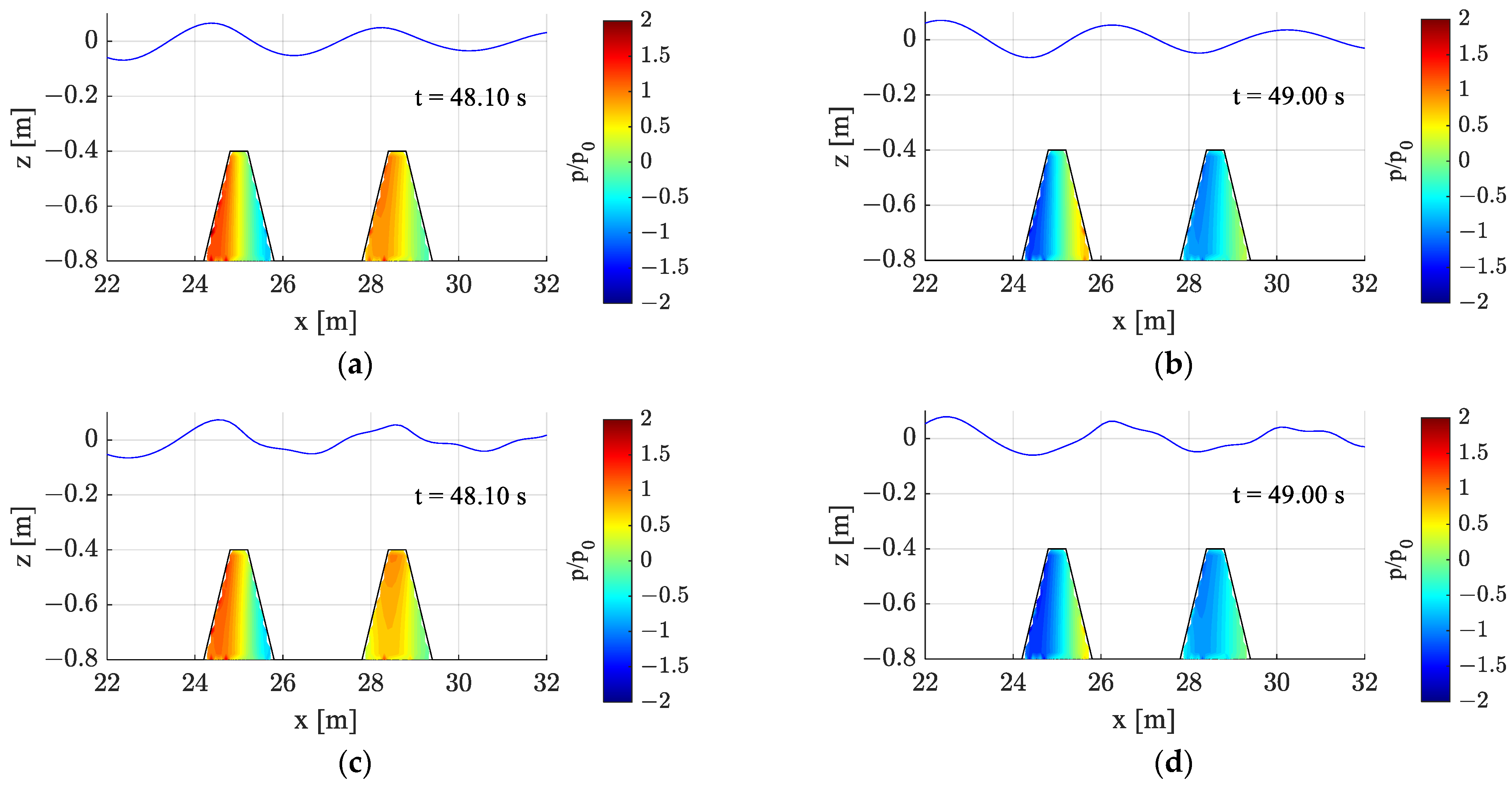
3. Numerical Results and Discussion
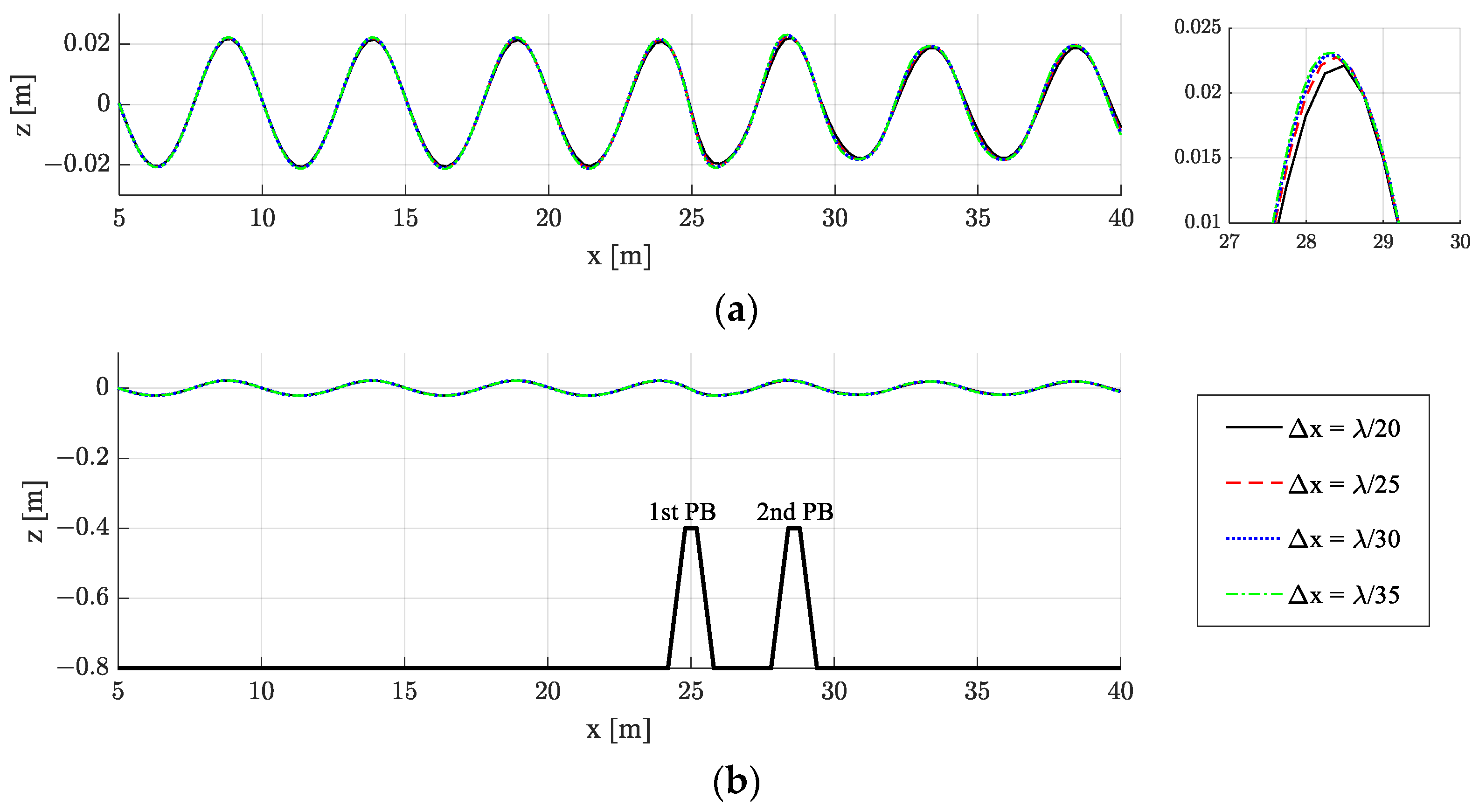
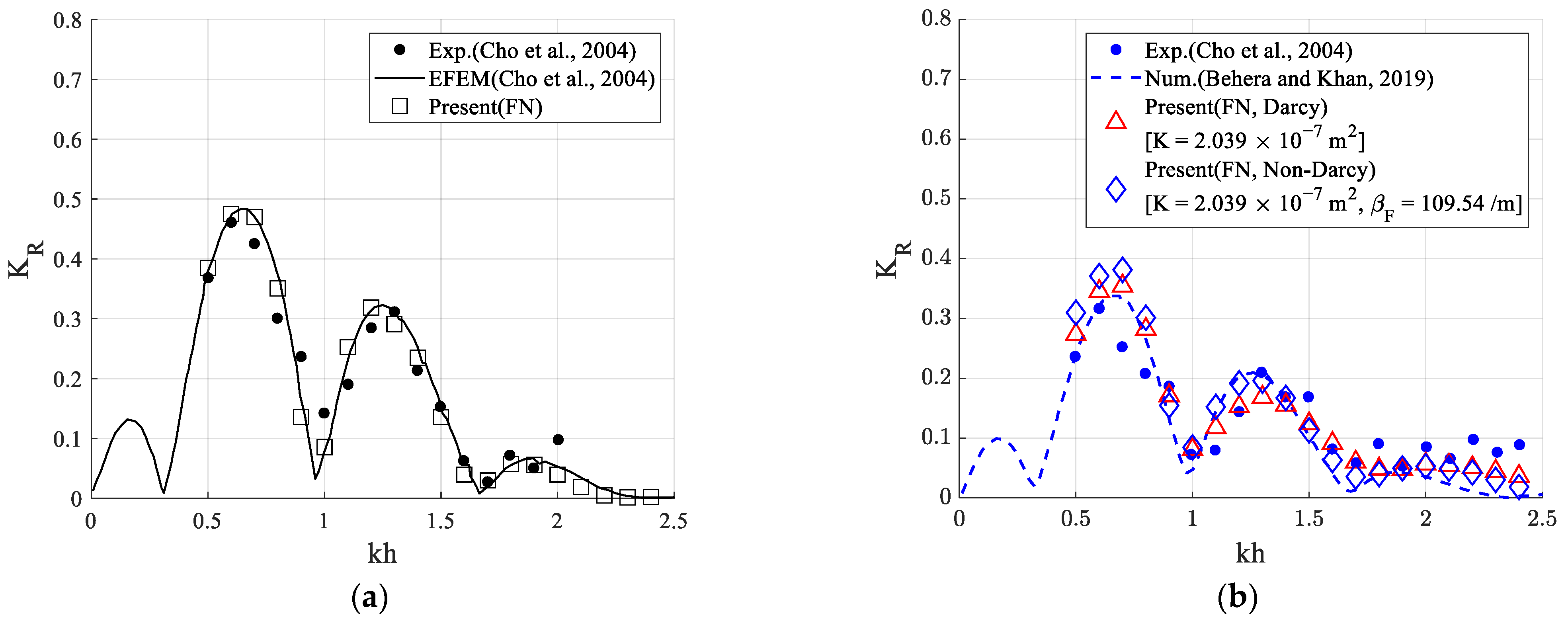
3.1. Validation of Numerical Results
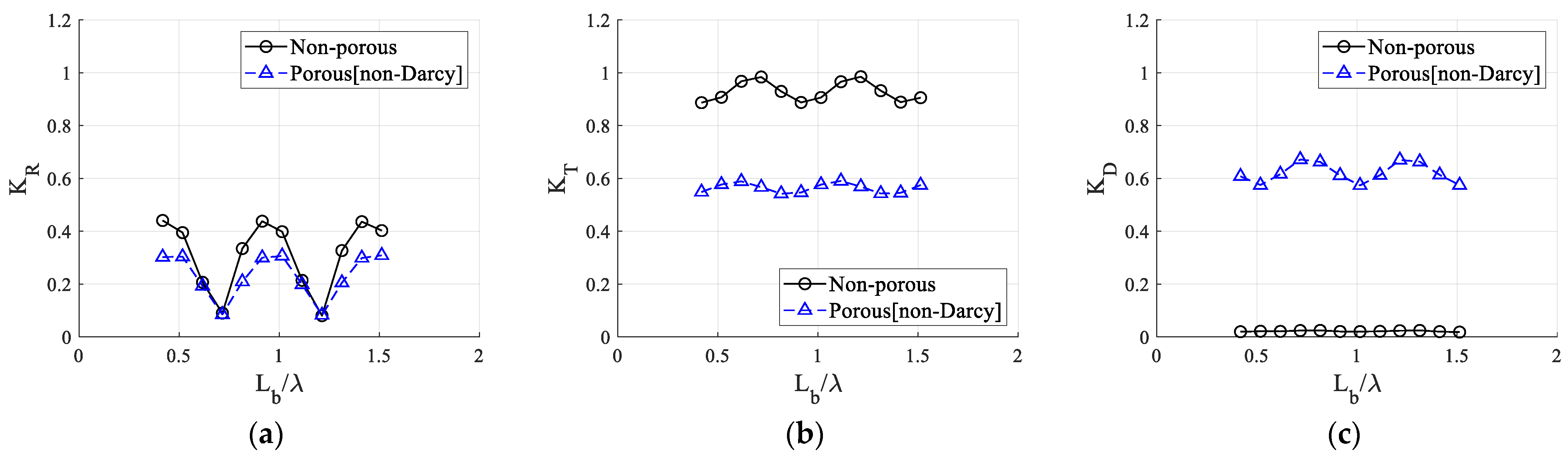
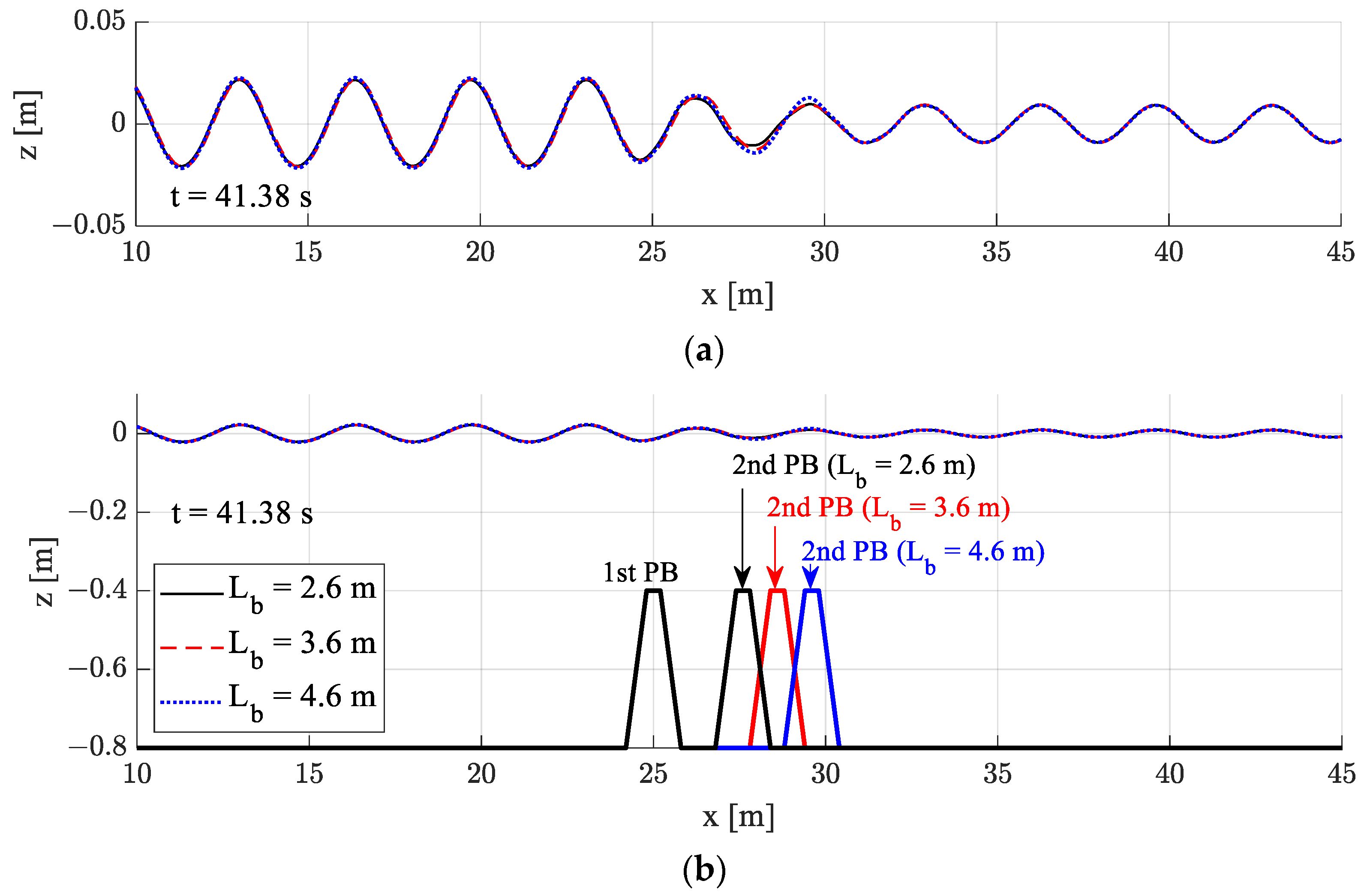
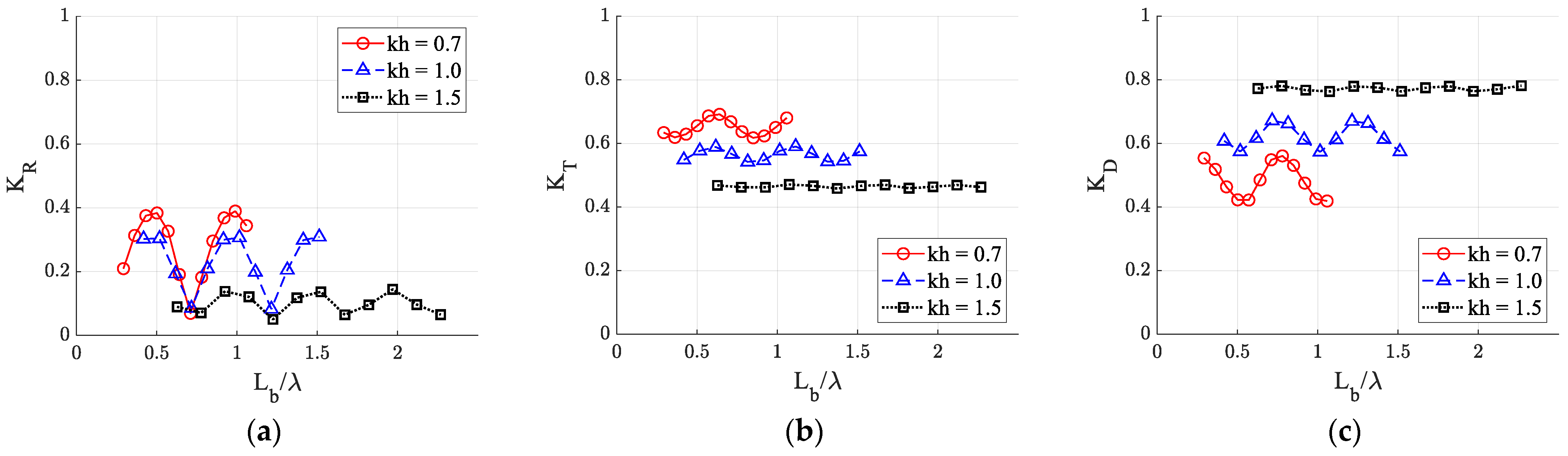
3.2. Comparison of Non-Porous and Porous Structures
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cuong, D.Q.; Tuan, T.Q. Wave hydrodynamics across steep platform reefs: A laboratory study. Civ. Eng. J. 2022, 8, 1739–1751. [Google Scholar] [CrossRef]
- Safari Ghaleh, R.; Aminoroayaie Yamini, O.; Mousavi, S.H.; Kavianpour, M.R. Numerical Modeling of Failure Mechanisms in Articulated Concrete Block Mattress as a Sustainable Coastal Protection Structure. Sustainability 2021, 13, 12794. [Google Scholar] [CrossRef]
- Srineash, V.K.; Murali, K. Effects of seaward slope on wave transmission of porous and non-porous reef breakwaters with smooth and stepped slopes. Coast. Struct. 2019, 2019, 275–285. [Google Scholar]
- Chyon, M.S.A.; Rahman, A.; Rahman, M.A. Comparative study on hydrodynamic performance of porous and non-porous submerged breakwater. Procedia Eng. 2017, 194, 203–210. [Google Scholar] [CrossRef]
- Mojtahedi, A.; Beiragh, M.S.; Farajpour, I.; Mohammadian, M. Investigation on hydrodynamic performance of an environmentally friendly pile breakwater. Ocean Eng. 2020, 217, 107942. [Google Scholar] [CrossRef]
- Widyastuti, I.; Thaha, M.A.; Lopa, R.T.; Hatta, M.P. Dam-break energy of porous structure for scour countermeasure at bridge abutment. Civ. Eng. J. 2022, 8, 3939–3951. [Google Scholar] [CrossRef]
- Mizutani, N.; Mostafa, A.M.; Iwata, K. Nonlinear regular waves, submerged breakwater and seabed dynamic interaction. Coast. Eng. 1998, 33, 177–202. [Google Scholar] [CrossRef]
- Mostafa, A.M.; Mizutani, N.; Iwata, K. Nonlinear wave, composite breakwater, and seabed dynamic interaction. J. Waterw. Port Coast. Ocean Eng. 1999, 236, 88–97. [Google Scholar] [CrossRef]
- Ranasinghe, R.S.; Sata, S.; Tajima, Y. Modeling of waves & currents around porous submerged breakwaters. Proc. Coast. Dyn. 2009, 2009, 1–13. [Google Scholar]
- Hieu, P.D.; Vinh, P.N. Numerical study of wave overtopping of a seawall supported by porous structures. Appl. Math. Model. 2012, 36, 2803–2813. [Google Scholar] [CrossRef]
- Lee, J.; Jeong, Y.M.; Kim, J.S.; Hur, D.S. Analysis of hydraulic characteristics according to the cross-section changes in submerged rigid vegetation. J. Ocean Eng. Technol. 2022, 36, 326–339. [Google Scholar] [CrossRef]
- Jeng, D.-S.; Ye, J.-H.; Zhang, J.-S.; Liu, P.L.-F. An integrated model for the wave-induced seabed response around marine structures: Model verifications and applications. Coast. Eng. 2013, 72, 1–19. [Google Scholar] [CrossRef]
- Ye, J.; Yu, D. ABAQUS-OlaFlow integrated numerical model for fluid-seabed-structure interaction. Mar. Struct. 2021, 78, 103016. [Google Scholar] [CrossRef]
- Dentale, F.; Donnarumma, G.; Carratelli, E.P. Numerical wave interaction with tetrapods breakwater. Int. J. Nav. Archit. Ocean Eng. 2014, 6, 800–812. [Google Scholar] [CrossRef]
- Zhang, T.; Zhao, Y.; Gan, Q.; Yuan, L.; Zhu, G.; Cai, Y.; Cao, B. Experimental investigation of Forchheimer coefficients for non-Darcy flow in conglomerate-confined aquifer. Geofluids 2018, 2018, 4209197. [Google Scholar] [CrossRef]
- Wang, D.X.; Dong, S.; Sun, J.W. Numerical modeling of the interactions between waves and a Jarlan-type caisson breakwater using OpenFOAM. Ocean Eng. 2019, 188, 106230. [Google Scholar] [CrossRef]
- Han, M.M.; Wang, C.M. Potential flow theory-based analytical and numerical modelling of porous and perforated breakwater: A review. Ocean Eng. 2022, 249, 110897. [Google Scholar] [CrossRef]
- Jeong, J.H.; Kim, J.H.; Lee, J.L. Analysis of wave transmission characteristics on the TTP submerged breakwater using a parabolic-type linear wave deformation model. J. Ocean Eng. Technol. 2021, 35, 82–90. [Google Scholar] [CrossRef]
- Behera, H.; Koley, S.; Sahoo, T. Wave transmission by partial porous structures in two-layer fluid. Eng. Anal. Bound. Elem. 2015, 58, 58–78. [Google Scholar] [CrossRef]
- Koley, S. Wave transmission through multilayered porous breakwater under regular and irregular incident waves. Eng. Anal. Bound. Elem. 2019, 108, 393–401. [Google Scholar] [CrossRef]
- Tabssum, S.; Kaligatla, R.B.; Sahoo, T. Gravity wave interaction with a porous breakwater in a two-layer ocean of varying depth. Ocean Eng. 2020, 196, 106816. [Google Scholar] [CrossRef]
- Tabssum, S.; Kaligatla, R.B.; Sahoo, T. Surface gravity wave interaction with a partial porous breakwater in the presence of bottom undulation. J. Eng. Mech. 2020, 146, 04020088. [Google Scholar] [CrossRef]
- Chen, K.H.; Chen, J.T.; Lin, S.Y.; Lee, Y.T. Dual boundary element analysis of normal incident wave passing a thin submerged breakwater with rigid, absorbing, and permeable boundaries. J. Waterw. Port Coast. Ocean Eng. 2004, 130, 179–190. [Google Scholar] [CrossRef]
- Han, M.M.; Wang, C.M. Modelling wide perforated breakwater with horizontal silts using Hybrid-BEM method. Ocean Eng. 2021, 222, 108630. [Google Scholar] [CrossRef]
- Duan, W.Y.; Liu, R.Z.; Chen, J.K.; Ma, S. Hydrodynamic analysis of floating breakwater with perforated structure based on the Taylor expansion boundary element method. Ocean Eng. 2020, 200, 107044. [Google Scholar] [CrossRef]
- Mizutani, N.; McDougal, W.; Mostafa, A. BEM-FEM combined analysis of nonlinear interaction between wave and submerged breakwater. Coast. Eng. 1997, 1996, 2377–2390. [Google Scholar]
- Cho, Y.S.; Lee, J.I.; Kim, Y.T. Experimental study of strong reflection of regular water waves over submerged breakwaters in tandem. Ocean Eng. 2004, 31, 1325–1335. [Google Scholar] [CrossRef]
- Jeon, C.H.; Cho, Y.S. Bragg reflection of sinusoidal waves due to trapezoidal submerged breakwaters. Ocean Eng. 2006, 33, 2067–2082. [Google Scholar] [CrossRef]
- Zhao, Y.; Liu, Y.; Li, H.; Chang, A. Oblique wave motion over multiple submerged porous bars near a vertical wall. J. Ocean Univ. China 2017, 16, 568–574. [Google Scholar] [CrossRef]
- Khan, M.B.M.; Behera, H. Oblique wave scattering by double porous structures. J. Phys. Conf. Ser. 2018, 1000, 012168. [Google Scholar] [CrossRef]
- Behera, H.; Khan, M.B.M. Numerical modeling for wave attenuation in double trapezoidal porous structures. Ocean Eng. 2019, 184, 91–106. [Google Scholar] [CrossRef]
- Jiang, L.; Zhang, J.; Tong, L.; Guo, Y.; He, R.; Sun, K. Wave motion and seabed response around a vertical structure sheltered by submerged breakwaters with Fabry-Pérot resonance. J. Mar. Sci. Eng. 2022, 10, 1797. [Google Scholar] [CrossRef]
- Zhao, E.; Dong, Y.; Tang, Y.; Xia, X. Performance of submerged semi-circular breakwater under solitary wave in consideration of porous media. Ocean Eng. 2021, 223, 108573. [Google Scholar] [CrossRef]
- Zhu, K.; Jiang, R.; Sun, Z.; Qin, H.; Cheng, Z.; Wang, Y.; Zhao, E. Numerical study on the effects of the multiple porous medium breakwaters on the propagation of the solitary wave. J. Mar. Sci. Eng. 2023, 11, 565. [Google Scholar] [CrossRef]
- Min, E.H.; Koo, W. Numerical simulation of wave propagation over structures on a porous seabed. J. Coast. Res. 2018, 85, 966–970. [Google Scholar] [CrossRef]
- Min, E.H.; Koo, W. Numerical analysis of nonlinear wave characteristics under porous sloped-seabed conditions. J. Coast. Res. 2021, 114, 116–120. [Google Scholar] [CrossRef]
- Chwang, A.T.; Chan, A.T. Interaction between porous media and wave motion. Annu. Rev. Fluid Mech. 1998, 30, 53–84. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S.; Cokelet, E. The deformation of steep surface waves on water-I. A numerical method of computation. Proc. R. Soc. Lond. A Math. Phys. Sci. 1976, 350, 1–26. [Google Scholar]
- Hou, X.; Shi, W.; Yang, T. A nonlinear flow model for the flow behavior of water inrush induced by the karst collapse column. RSC Adv. 2018, 8, 1656–1665. [Google Scholar] [CrossRef] [PubMed]
- Hong, S.Y.; Kim, M.H. Nonlinear wave forces on a stationary cylinder by HOBEM-NWT. In Proceedings of the 10th ISOPE International Ocean and Polar Engineering Conference, Seattle, WA, USA, 27 May–2 June 2000; Volume 3, pp. 214–220. [Google Scholar]
- Koo, W.C.; Kim, M.H. Fully nonlinear wave-body interaction with surface-piercing bodies. Ocean Eng. 2007, 34, 1000–1012. [Google Scholar] [CrossRef]
- Conzelmann, N.A.; Partl, M.N.; Clemens, F.J.; Müller, C.R.; Poulikakos, L.D. Effect of artificial aggregate shape on the porosity, tortuosity and permeability of their packings. Powder Technol. 2022, 397, 117019. [Google Scholar] [CrossRef]
- Sidiropoulou, M.G.; Moutsopoulos, K.N.; Tsihrintzis, V.A. Determination of Forchheimer equation coefficients a and b. Hydrol. Process. 2007, 21, 534–554. [Google Scholar] [CrossRef]






| Kh | Previous—Present [Darcy] | Previous—Present [Non-Darcy] |
|---|---|---|
| 0.6 | −6.93% | −14.79% |
| 1.2 | 24.87% | 5.64% |
| 1.3 | 17.83% | 4.20% |
| 1.4 | 6.56% | −0.50% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Min, E.-H.; Koo, W.; Kim, M.-H. Wave Characteristics over a Dual Porous Submerged Breakwater Using a Fully Nonlinear Numerical Wave Tank with a Porous Domain. J. Mar. Sci. Eng. 2023, 11, 1648. https://doi.org/10.3390/jmse11091648
Min E-H, Koo W, Kim M-H. Wave Characteristics over a Dual Porous Submerged Breakwater Using a Fully Nonlinear Numerical Wave Tank with a Porous Domain. Journal of Marine Science and Engineering. 2023; 11(9):1648. https://doi.org/10.3390/jmse11091648
Chicago/Turabian StyleMin, Eun-Hong, Weoncheol Koo, and Moo-Hyun Kim. 2023. "Wave Characteristics over a Dual Porous Submerged Breakwater Using a Fully Nonlinear Numerical Wave Tank with a Porous Domain" Journal of Marine Science and Engineering 11, no. 9: 1648. https://doi.org/10.3390/jmse11091648
APA StyleMin, E.-H., Koo, W., & Kim, M.-H. (2023). Wave Characteristics over a Dual Porous Submerged Breakwater Using a Fully Nonlinear Numerical Wave Tank with a Porous Domain. Journal of Marine Science and Engineering, 11(9), 1648. https://doi.org/10.3390/jmse11091648






