Distributed Event-Triggered Fixed-Time Leader–Follower Formation Tracking Control of Multiple Underwater Vehicles Based on an Adaptive Fixed-Time Observer
Abstract
:1. Introduction
- A novel AFxDO combined with an adaptive parameter estimation strategy was developed to eliminate the adverse effects of unknown time-varying external disturbances. Unlike existing disturbance observers, the proposed AFxDO guarantees the fixed-time stability of the observation errors, and the strict limitation of the prior information of the bound value of external disturbances was removed through the adaptive parameter estimation strategy.
- Together with the developed AFxDO, a distributed event-triggered fixed-time backstepping control strategy is proposed to solve the leader–follower formation control problem for MUVs subject to external disturbances. A nonlinear first-order filter was designed to avoid the “explosion of complexity” problem in conventional backstepping approach. The proposed formation tracking control methodology ensures that the formation tracking errors converge to an arbitrarily small neighborhood around the origin within a fixed time, independent of initial conditions. Additionally, the bandwidth resources are saved and the communication burdens are reduced by introducing an event-triggered mechanism.
2. Model Description and Preliminaries
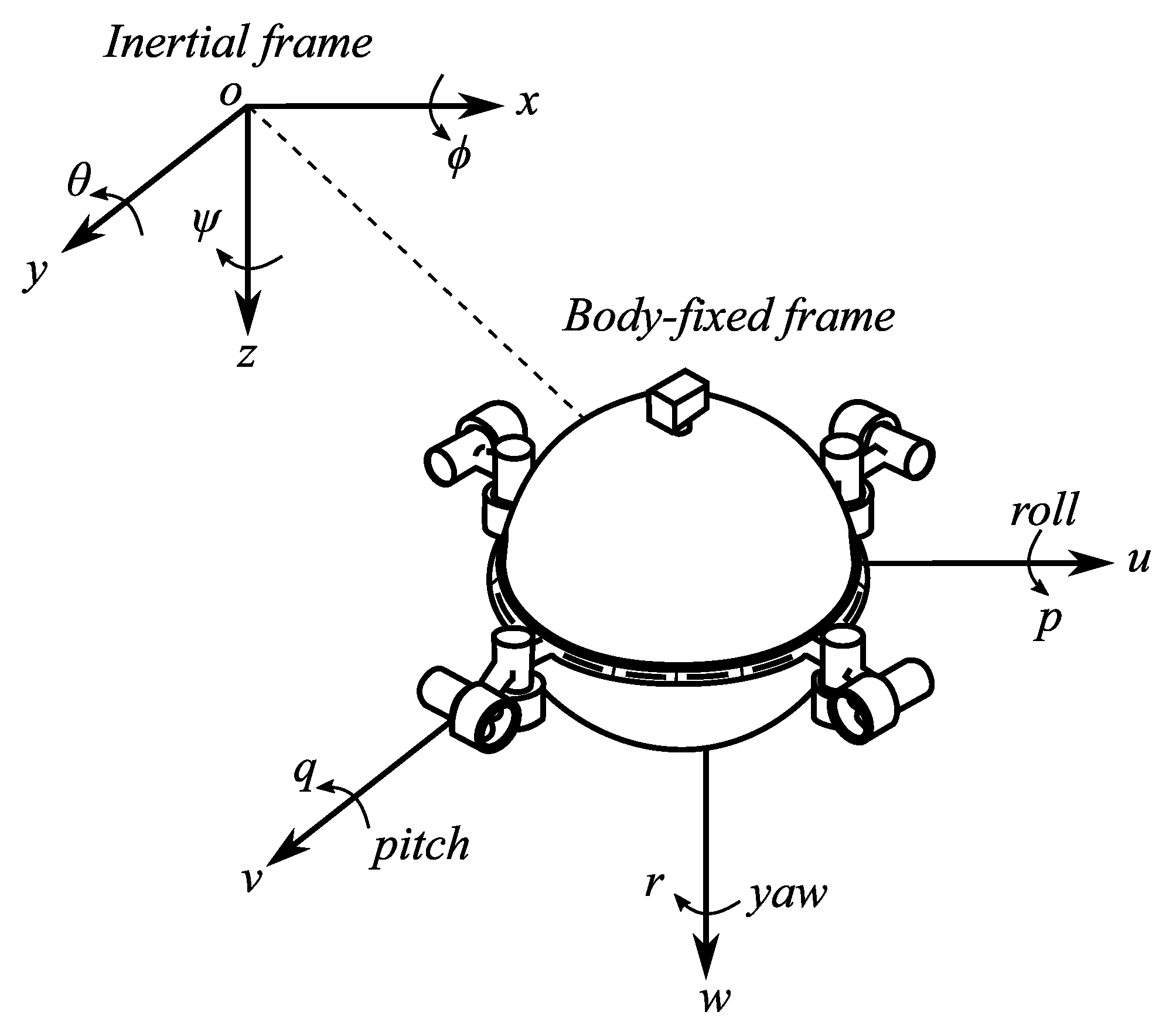
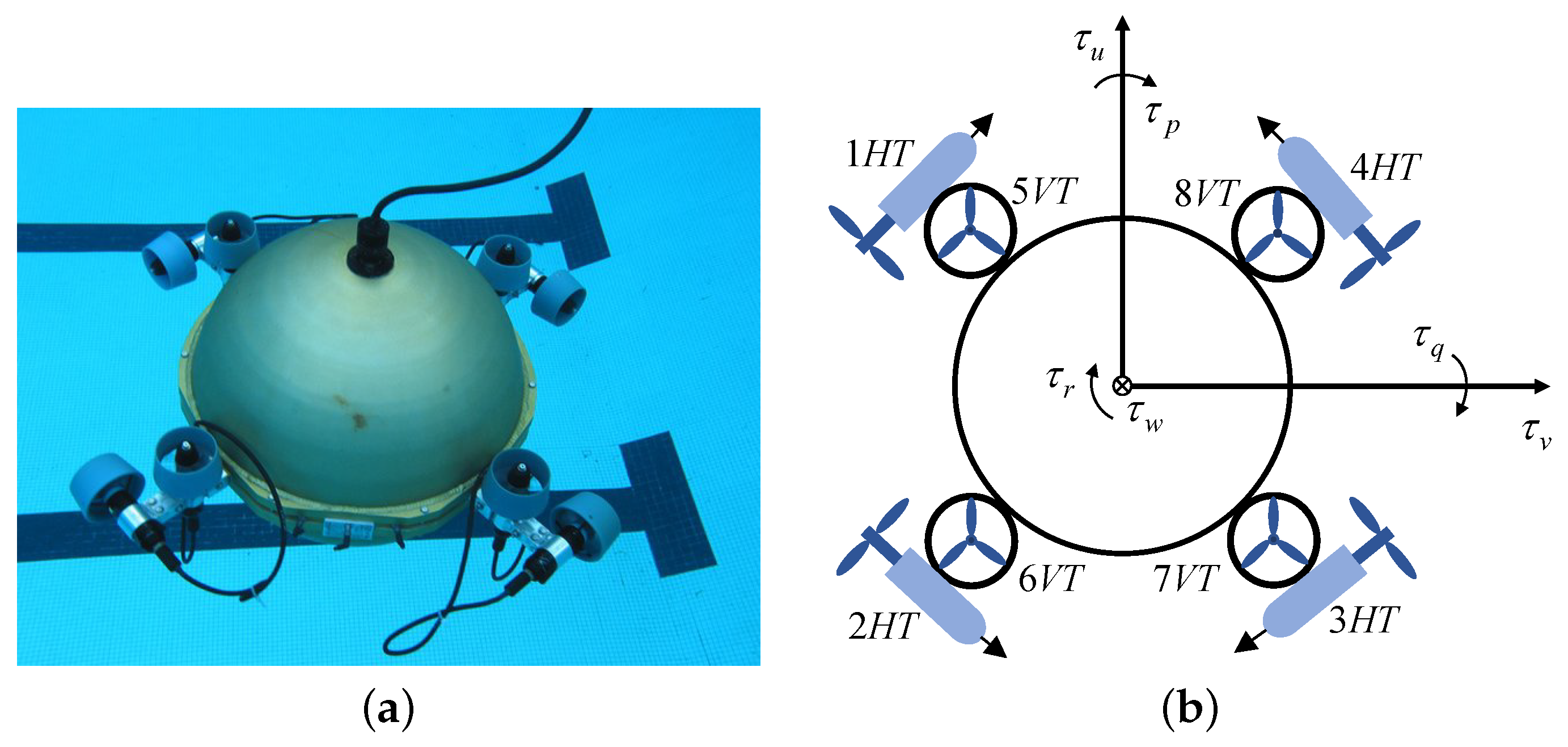
2.1. Modeling of Networked Underwater Vehicles
2.2. Notation and Related Lemmas
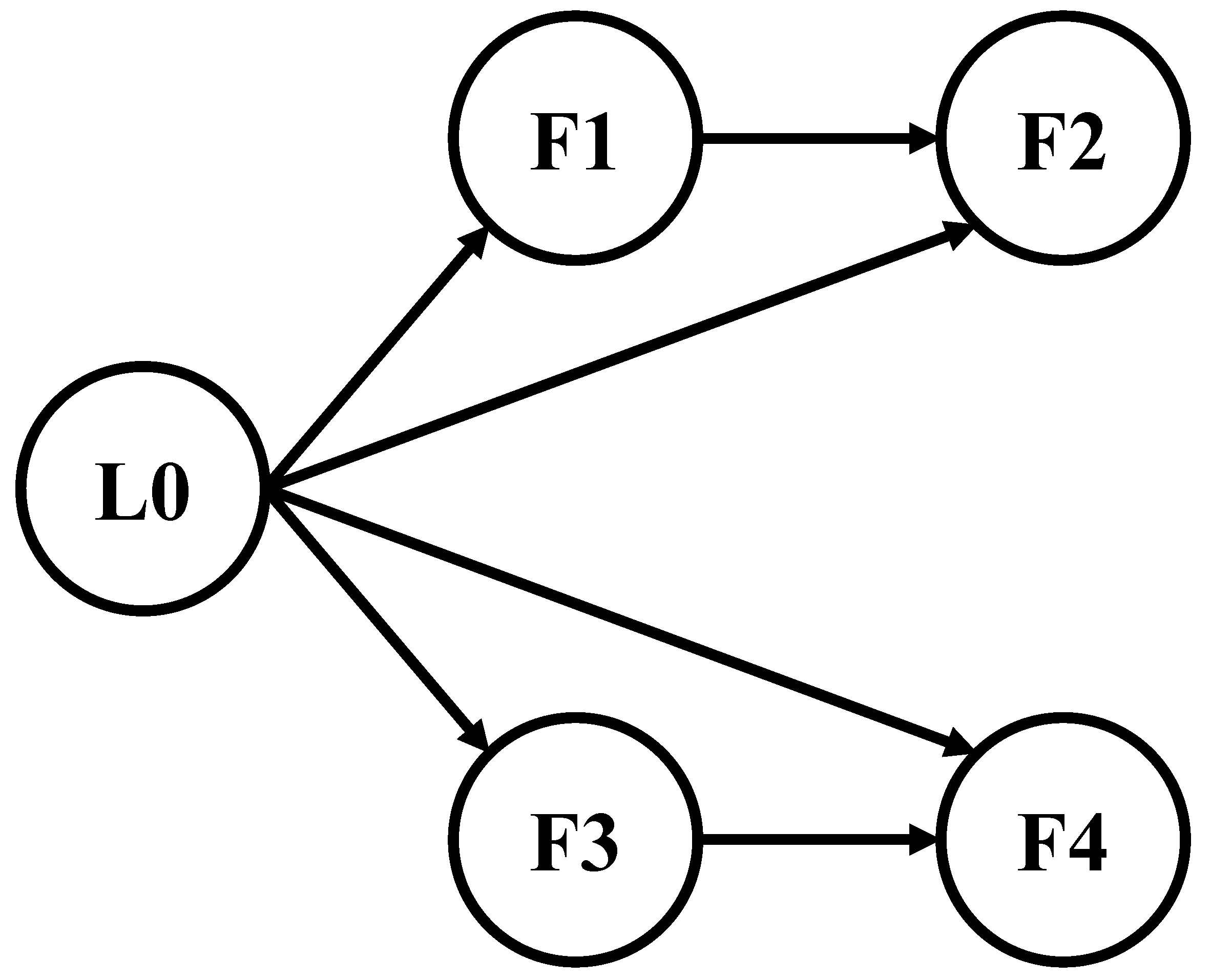
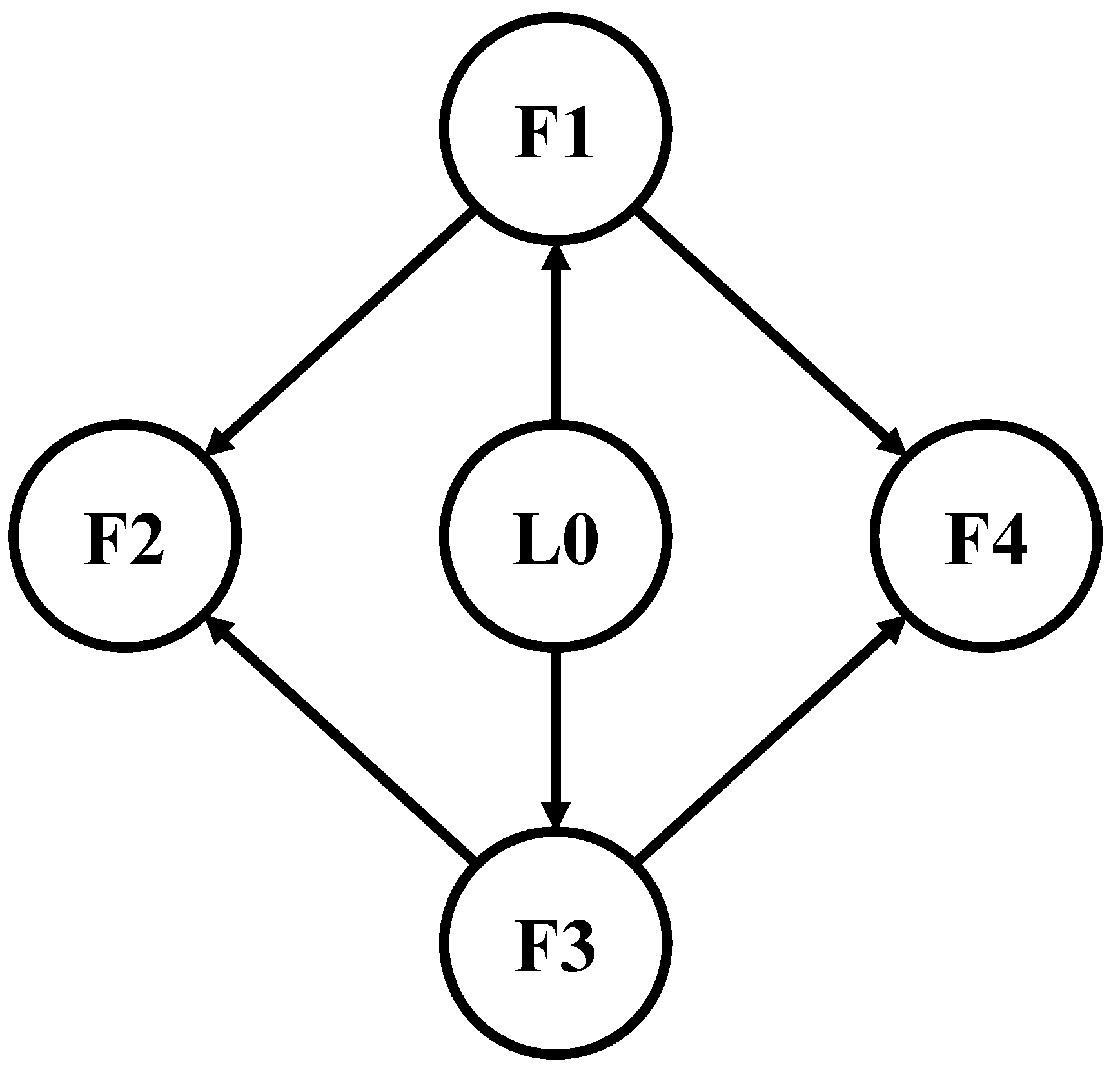
2.3. Basic Graph Theory
3. Main Results
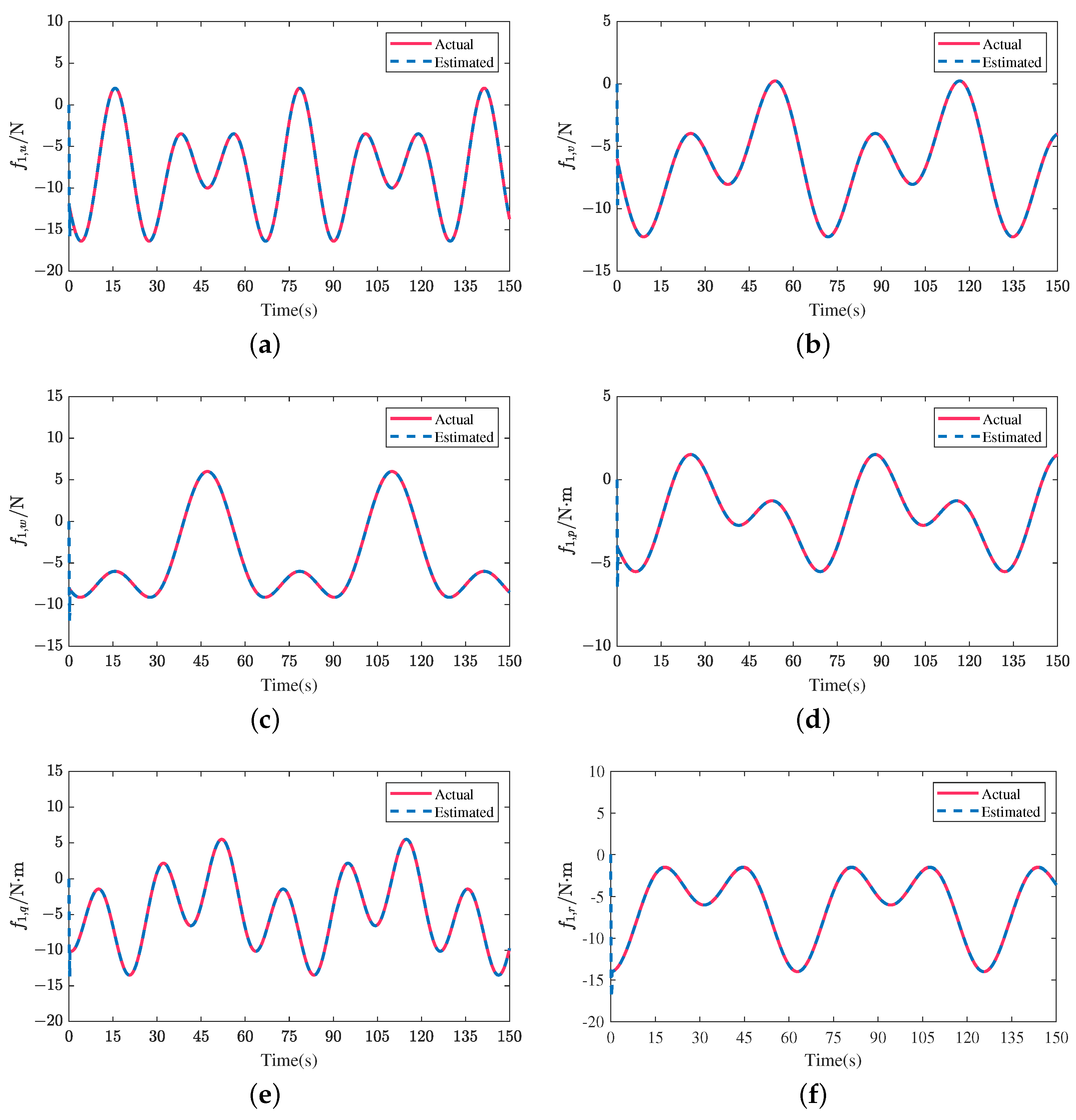
3.1. Design of Adaptive Fixed-Time Disturbance Observer
3.2. Design of Distributed Event-Triggered Fixed-Time Formation Tracking Controller
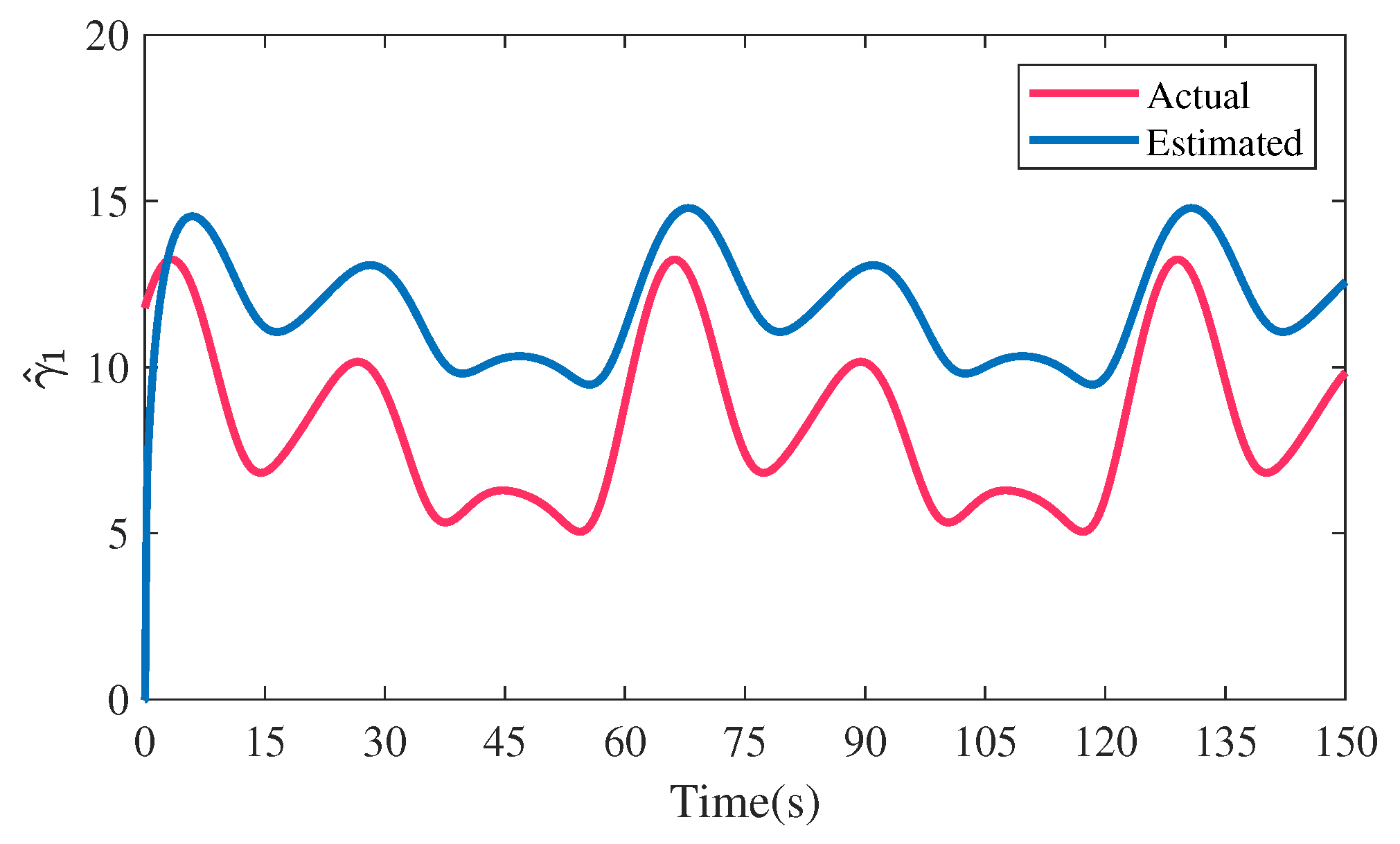
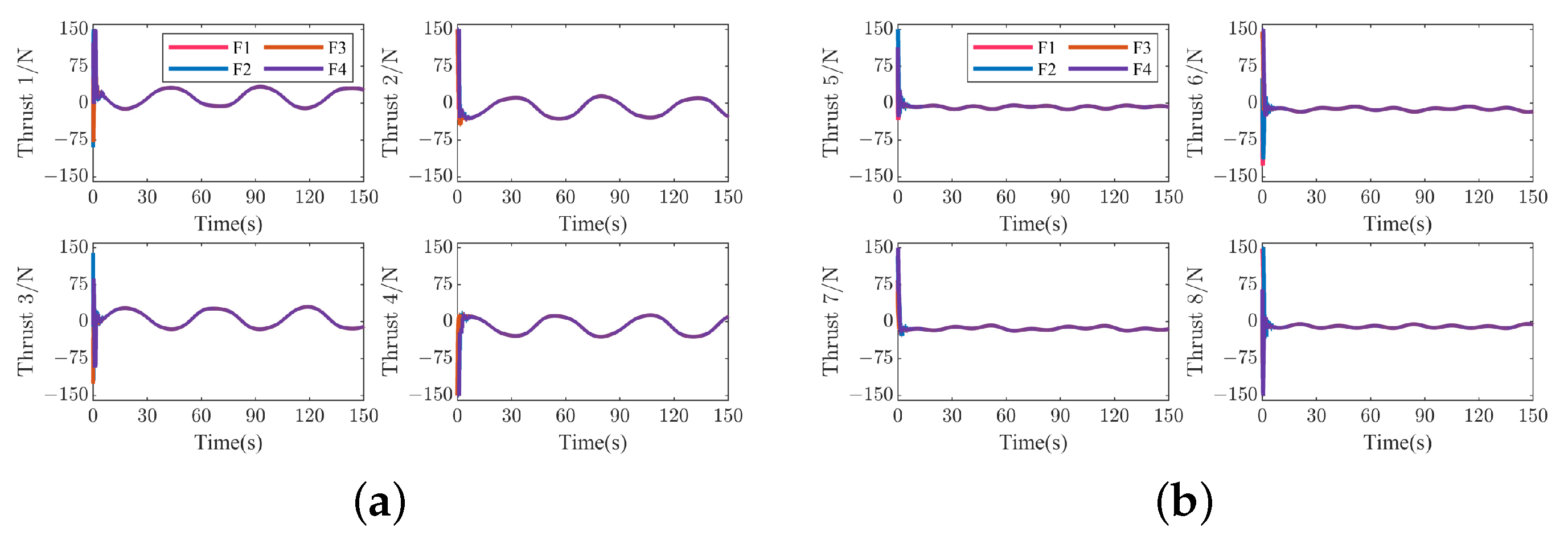
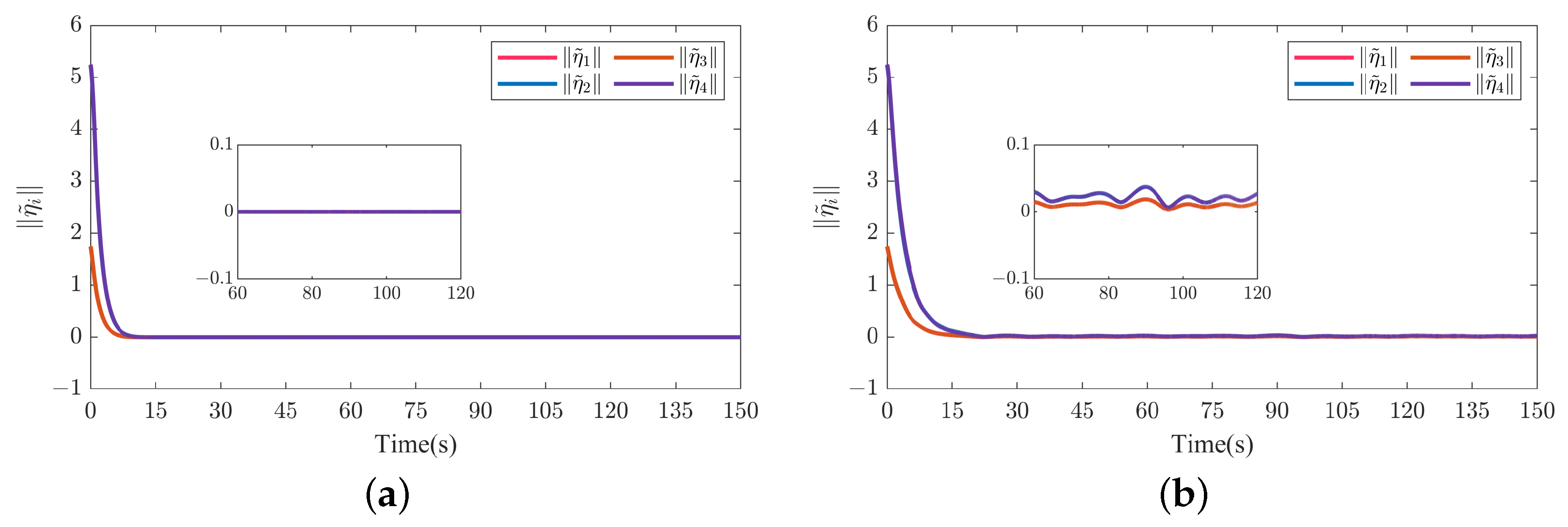
4. Simulation Results
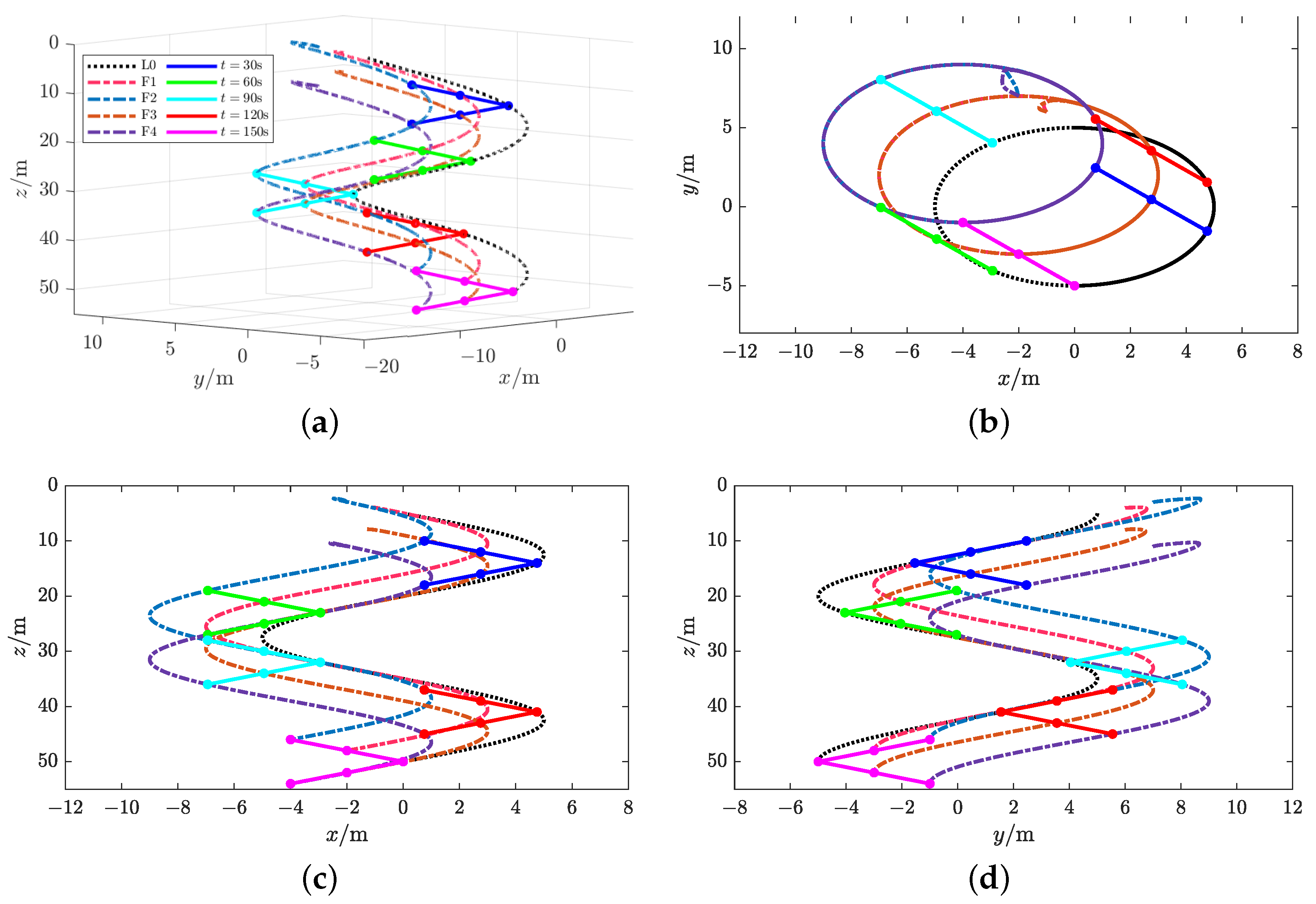
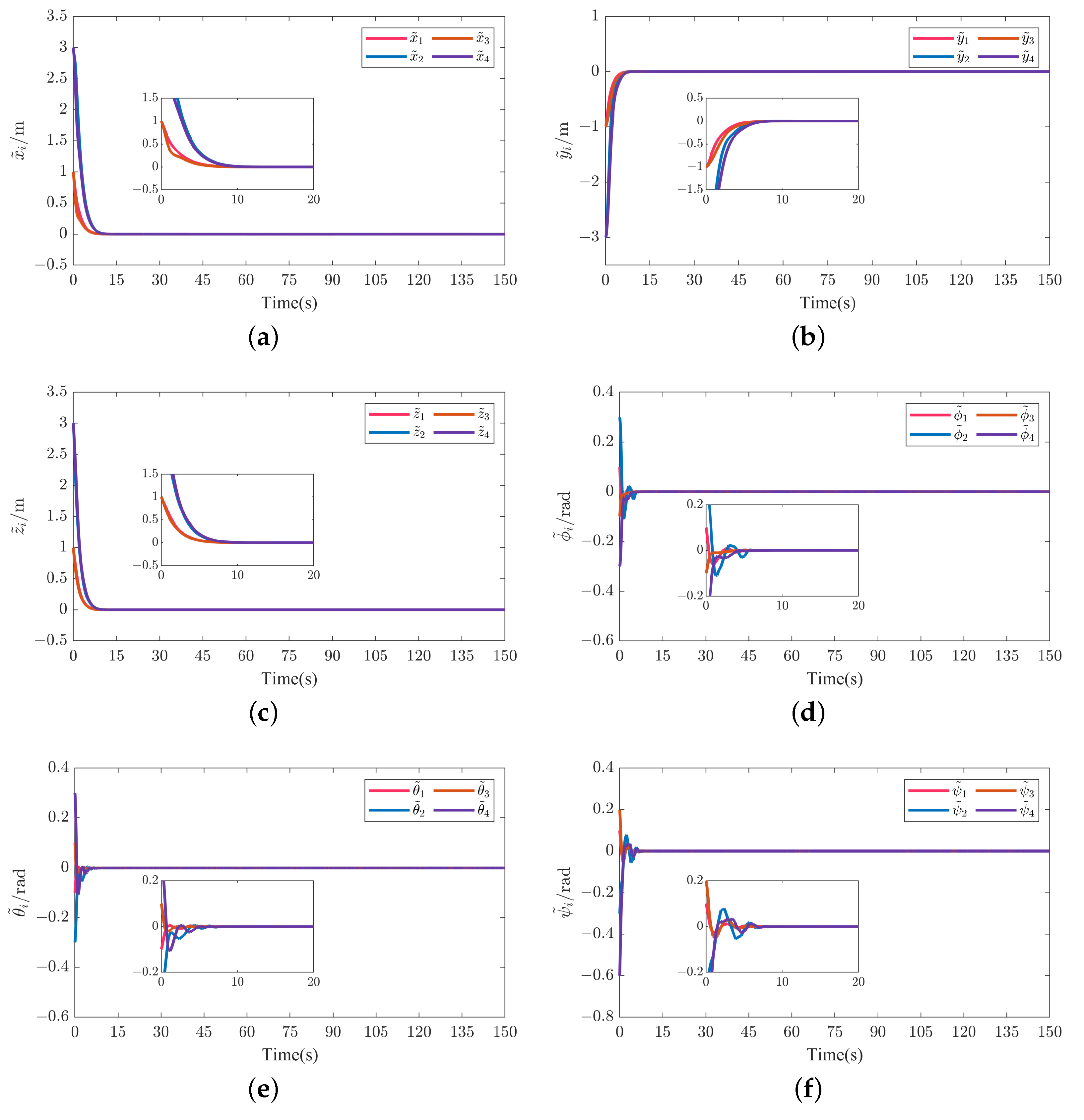
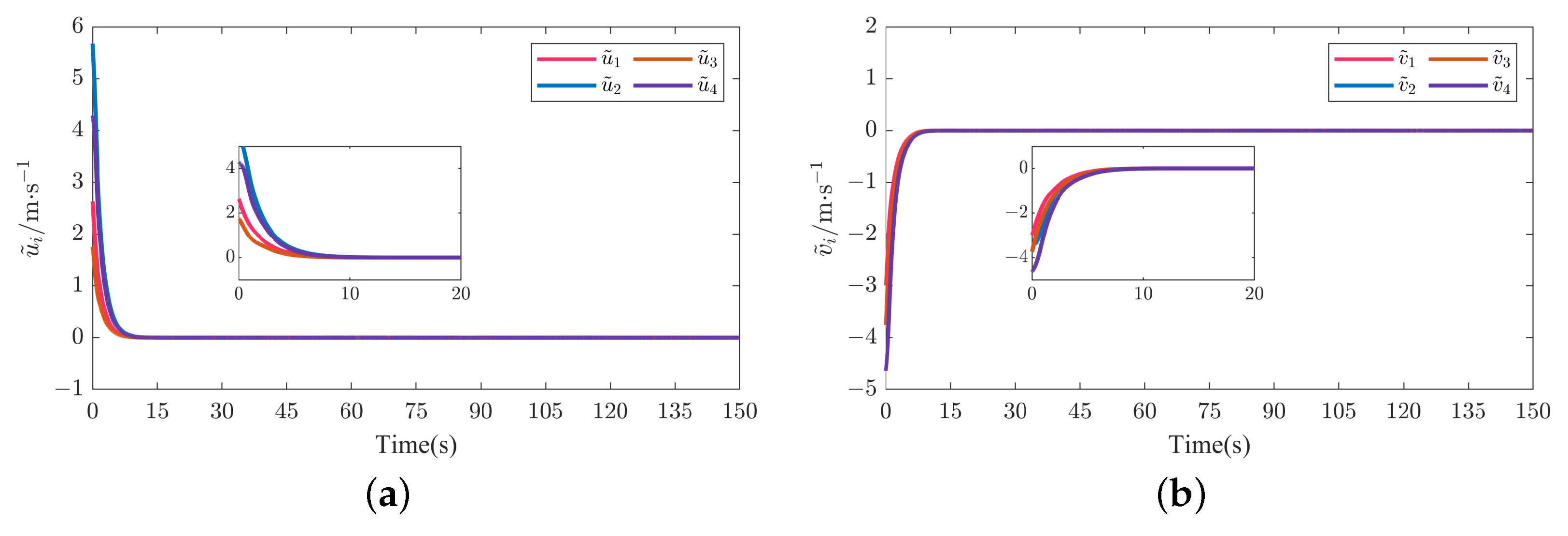
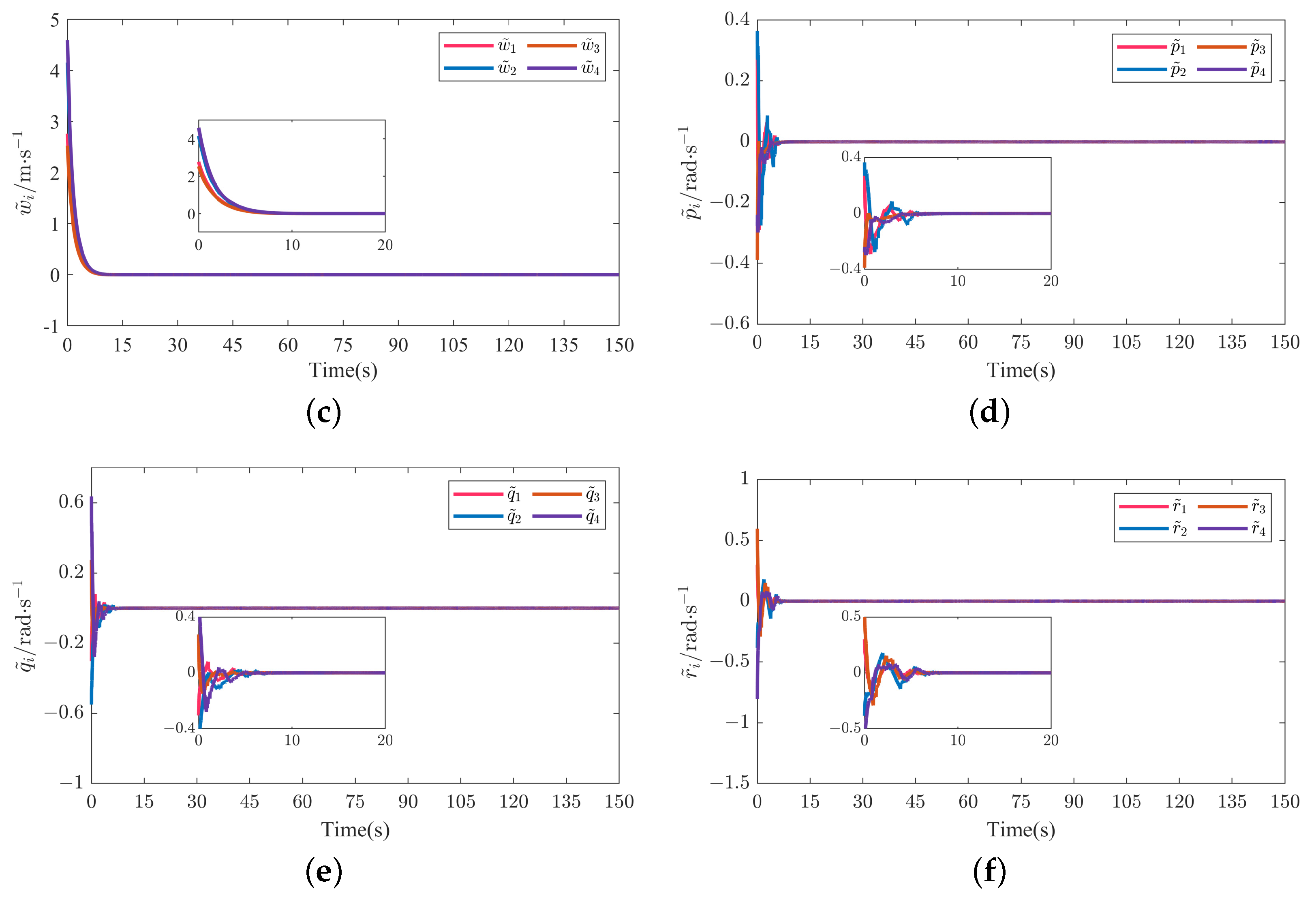
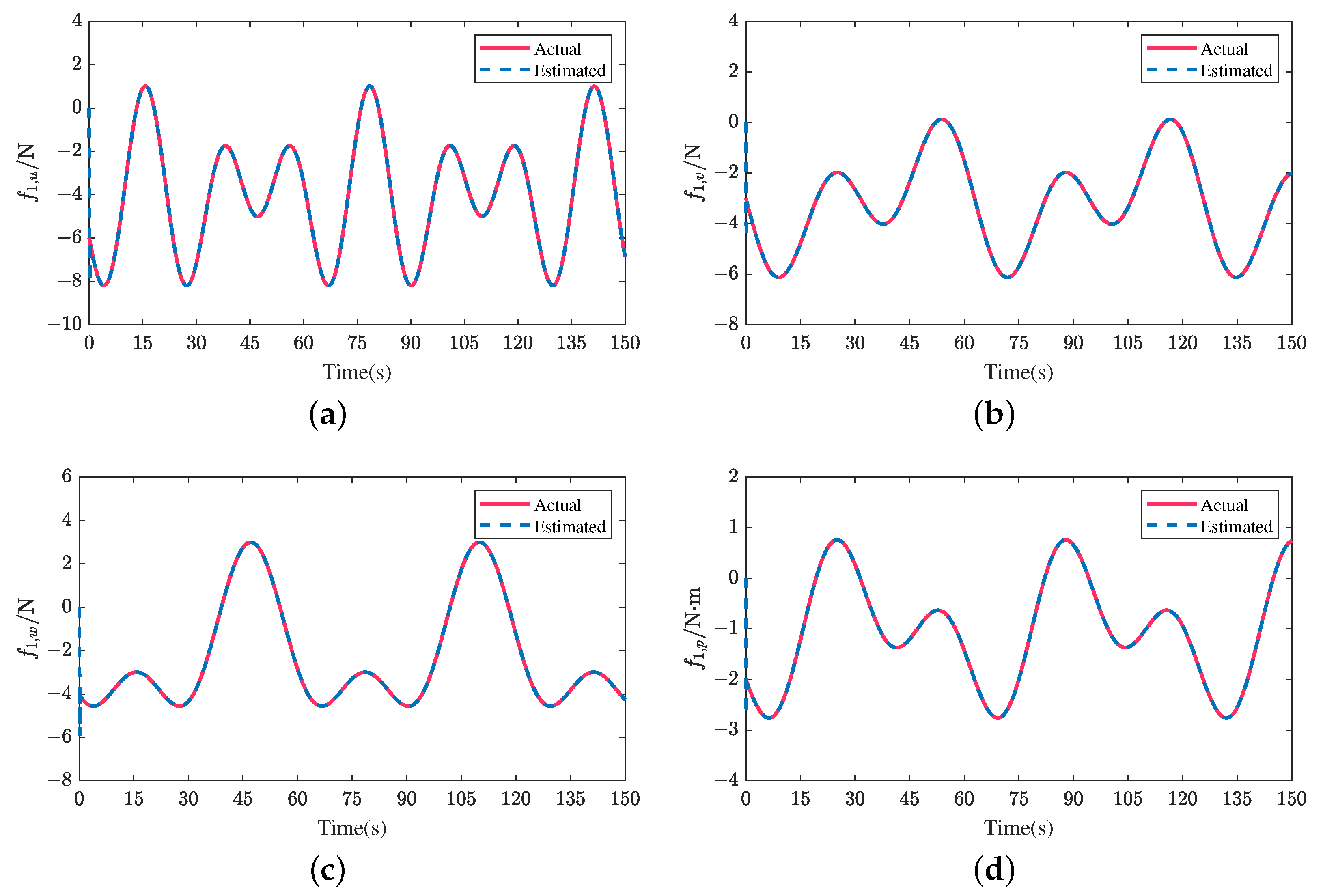
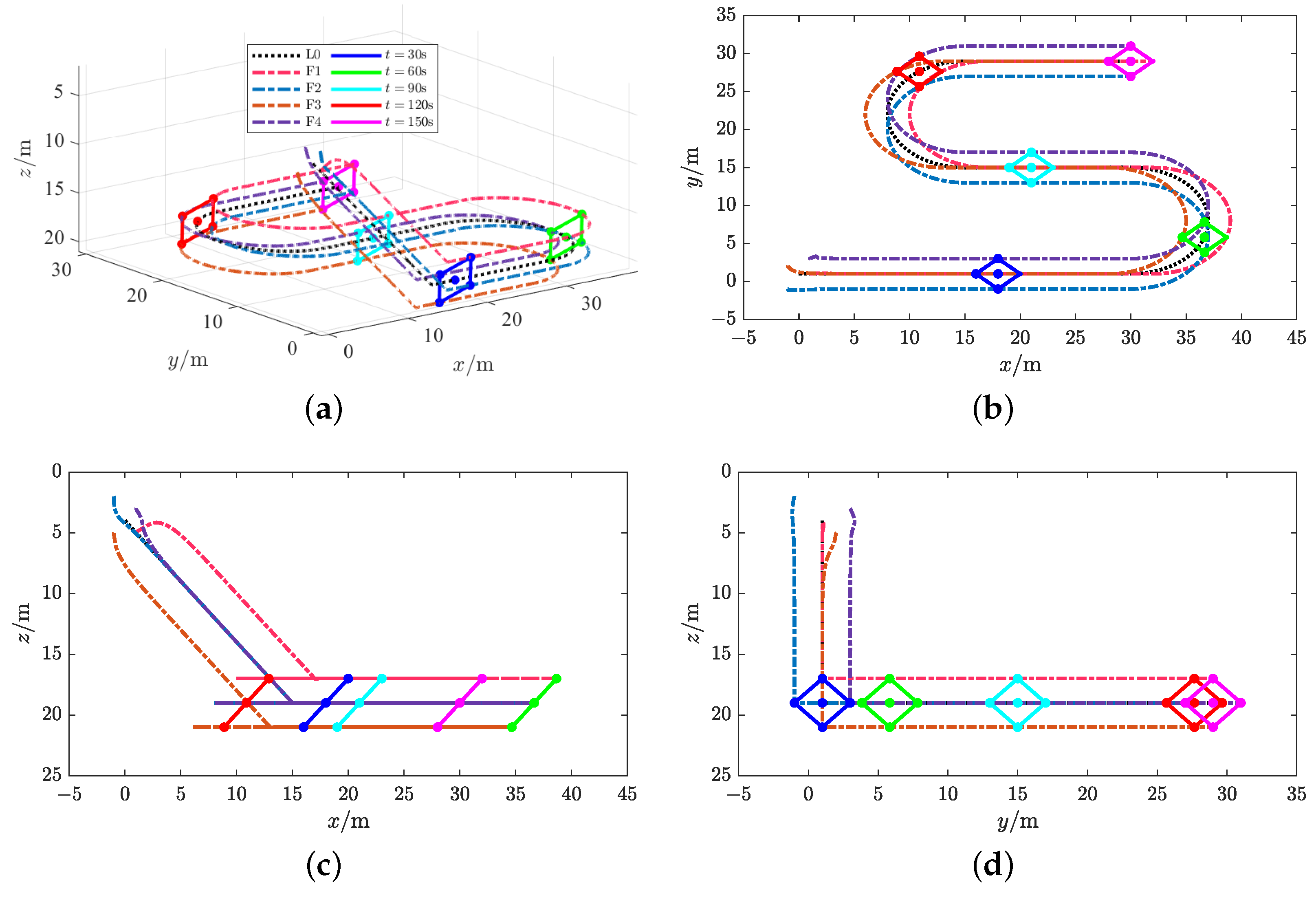
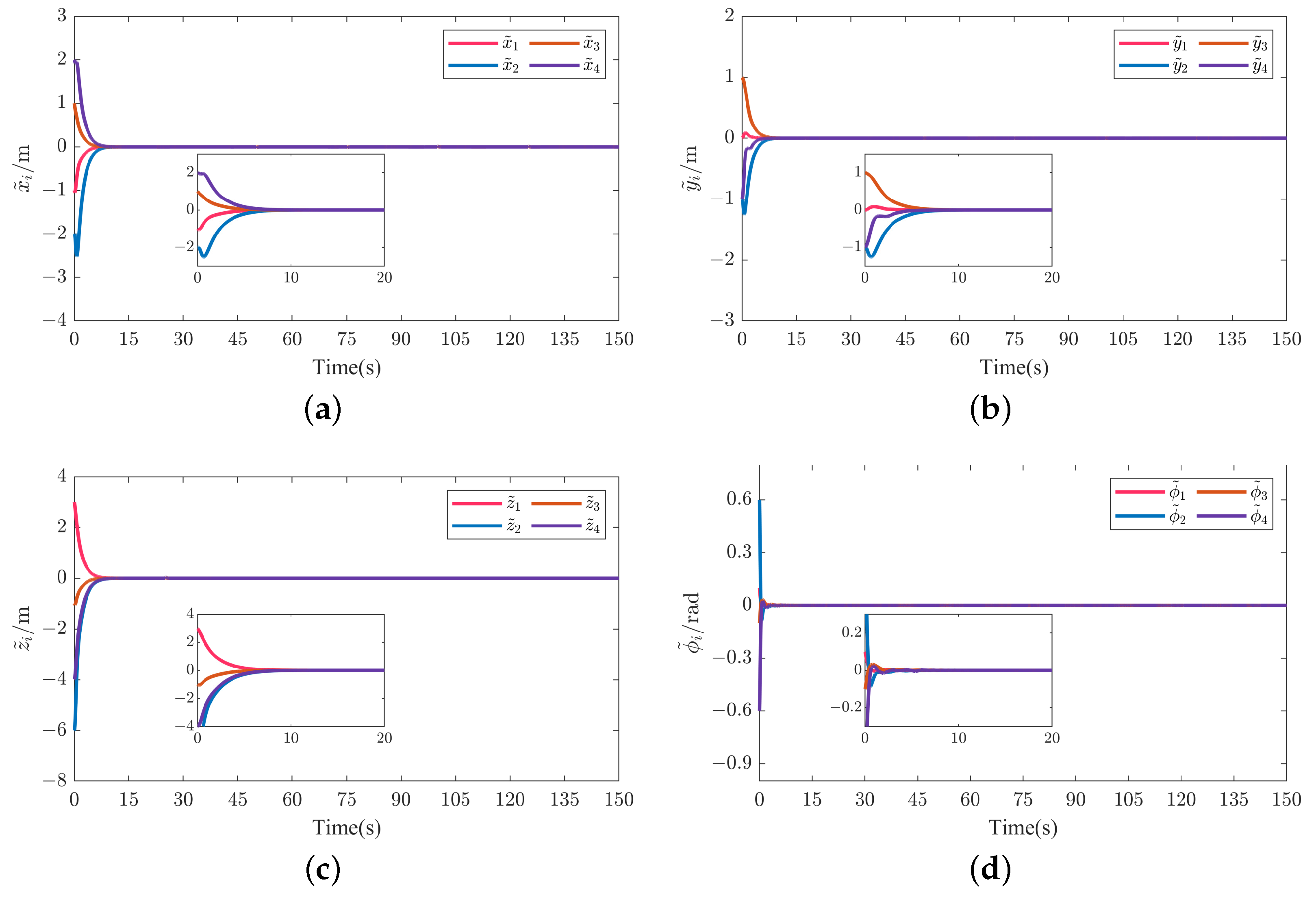
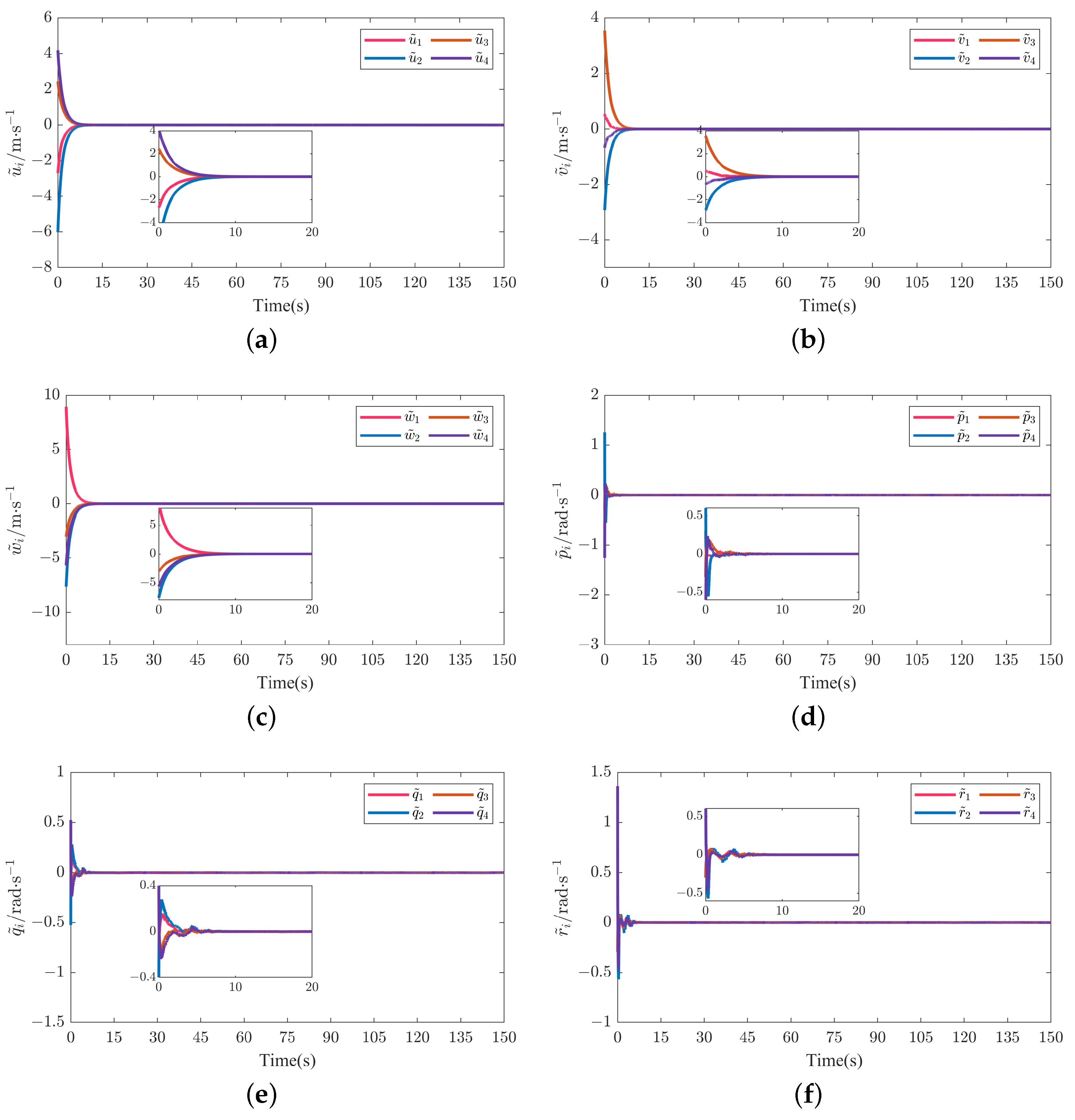
4.1. Scenario 1: Helical Trajectory Formation Tracking
4.2. Scenario 2: Dubins Trajectory Formation Tracking
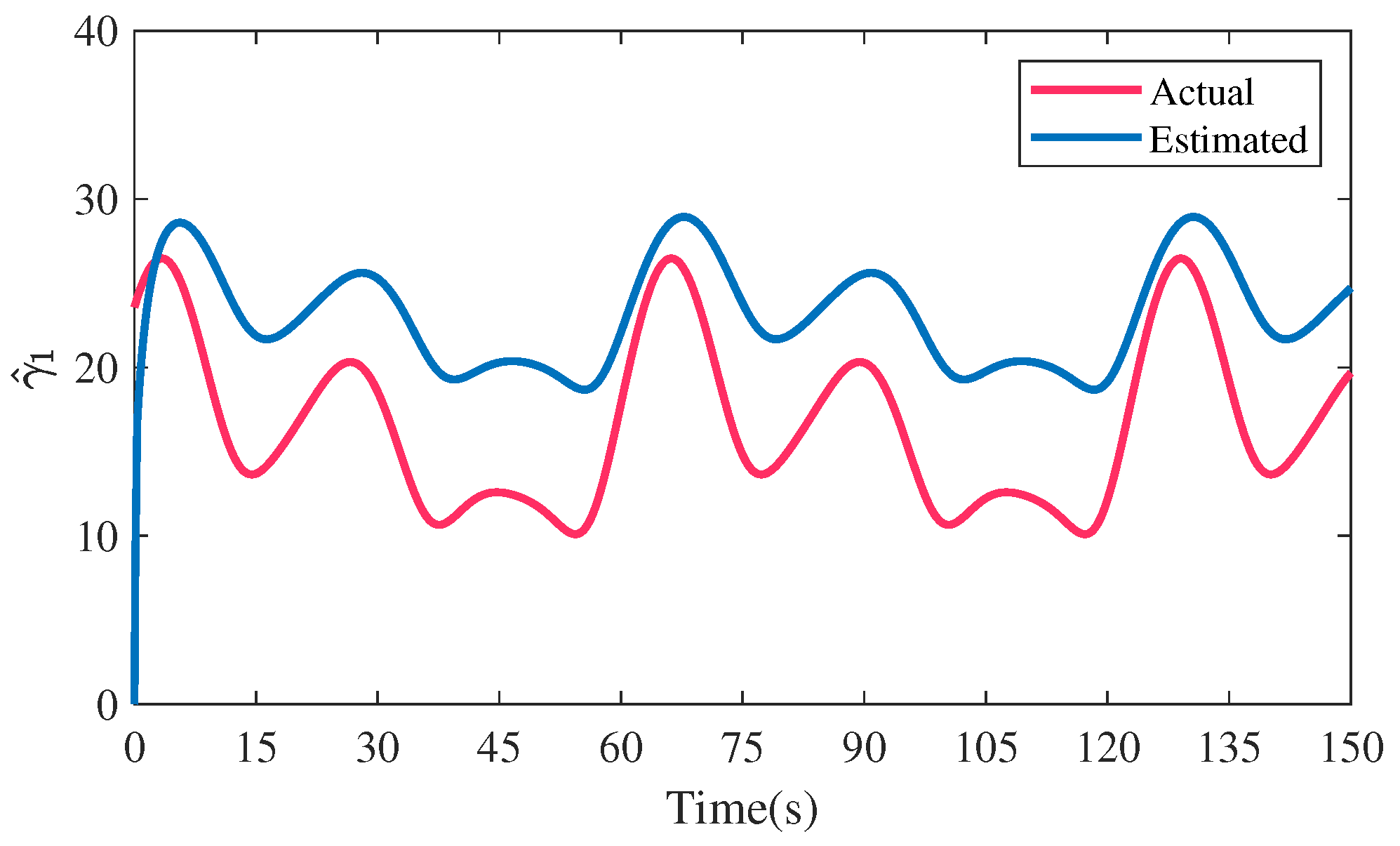
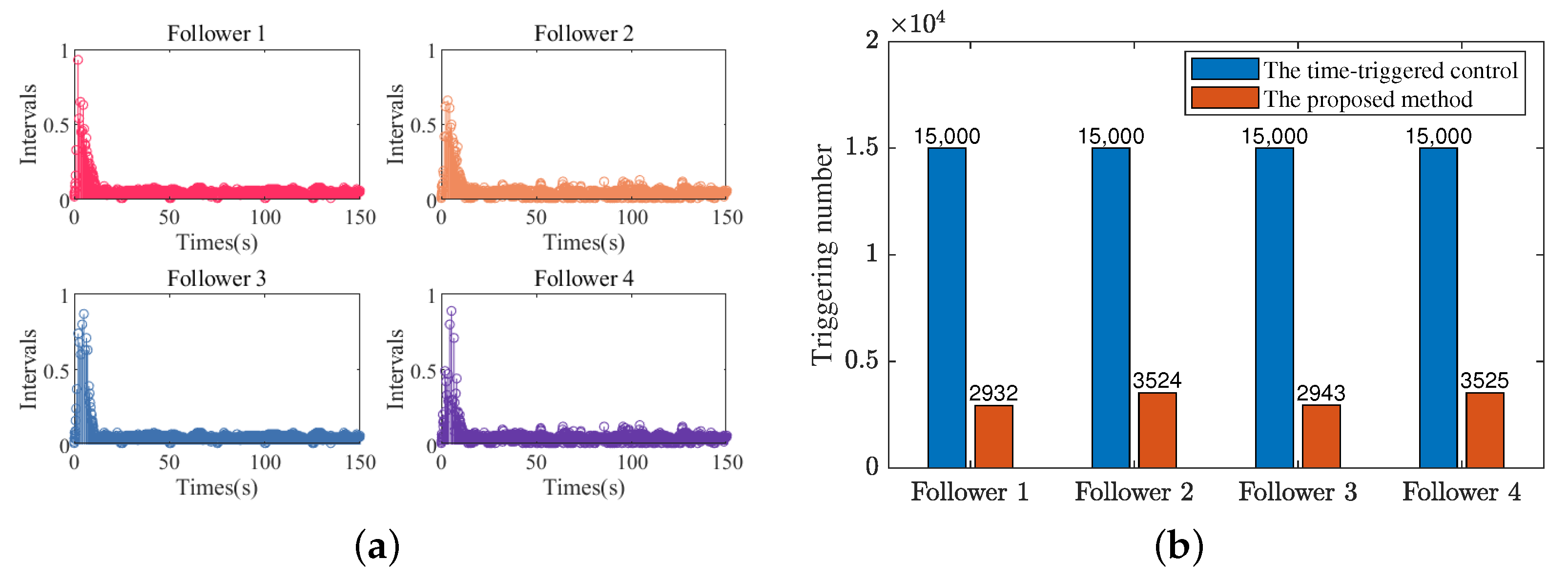
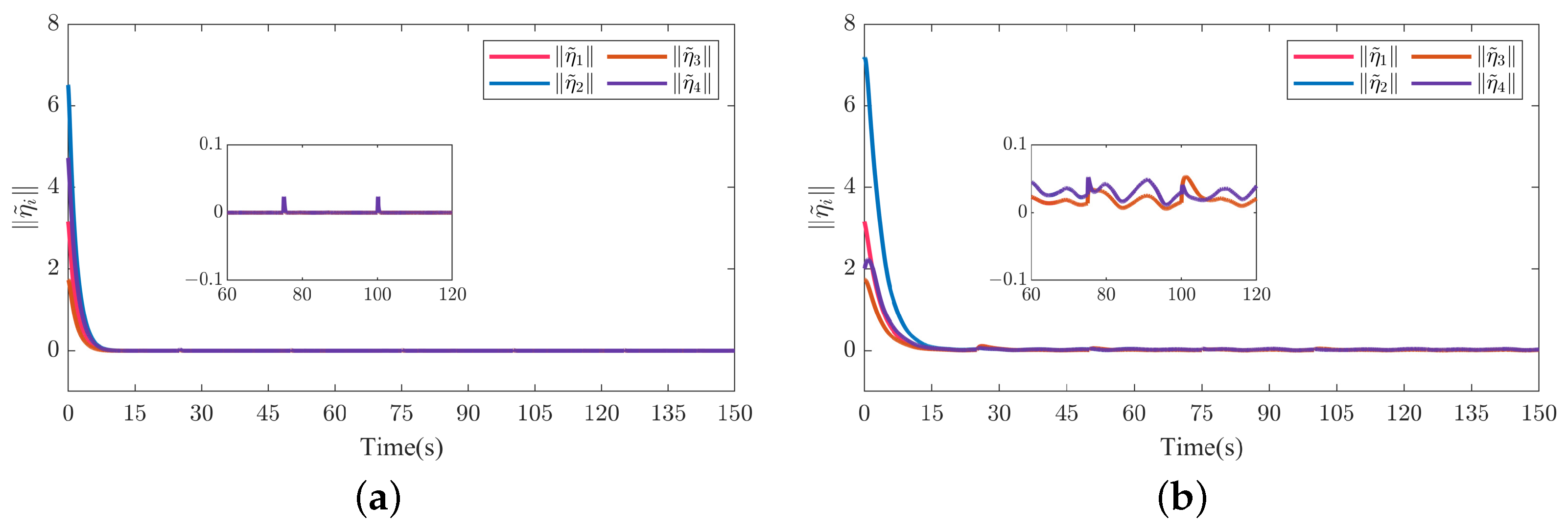
4.3. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sakthivel, R.; Parivallal, A.; Huy Tuan, N.; Manickavalli, S. Nonfragile control design for consensus of semi-Markov jumping multiagent systems with disturbances. Int. J. Adapt. Control Signal Process. 2021, 35, 1039–1061. [Google Scholar] [CrossRef]
- Sakthivel, R.; Manickavalli, S.; Parivallal, A.; Ren, Y. Observer-based bipartite consensus for uncertain Markovian-jumping multi-agent systems with actuator saturation. Eur. J. Control 2021, 61, 13–23. [Google Scholar] [CrossRef]
- Sahoo, A.; Dwivedy, S.K.; Robi, P. Advancements in the field of autonomous underwater vehicle. Ocean Eng. 2019, 181, 145–160. [Google Scholar] [CrossRef]
- Xiao, G.; Wang, T.; Luo, Y.; Yang, D. Analysis of port pollutant emission characteristics in United States based on multiscale geographically weighted regression. Front. Mar. Sci. 2023, 10, 1131948. [Google Scholar] [CrossRef]
- Qiao, L.; Zhang, W. Adaptive non-singular integral terminal sliding mode tracking control for autonomous underwater vehicles. IET Control Theory Appl. 2017, 11, 1293–1306. [Google Scholar] [CrossRef]
- Lu, J.; Wu, X.; Wu, Y. The Construction and Application of Dual-Objective Optimal Speed Model of Liners in a Changing Climate: Taking Yang Ming Route as an Example. J. Mar. Sci. Eng. 2023, 11, 157. [Google Scholar] [CrossRef]
- Qiao, L.; Zhang, W. Double-loop integral terminal sliding mode tracking control for UUVs with adaptive dynamic compensation of uncertainties and disturbances. IEEE J. Ocean. Eng. 2018, 44, 29–53. [Google Scholar] [CrossRef]
- An, S.; Wang, L.; He, Y. Robust fixed-time tracking control for underactuated AUVs based on fixed-time disturbance observer. Ocean Eng. 2022, 266, 112567. [Google Scholar] [CrossRef]
- Yang, H.; Wang, C.; Zhang, F. A decoupled controller design approach for formation control of autonomous underwater vehicles with time delays. IET Control Theory Appl. 2013, 7, 1950–1958. [Google Scholar] [CrossRef]
- Yan, T.; Xu, Z.; Yang, S.X. Consensus Formation Control for Multiple AUVSystems Using Distributed Bioinspired Sliding Mode Control. IEEE Trans. Intell. Veh. 2022, 8, 1081–1092. [Google Scholar] [CrossRef]
- Zhen, Q.; Wan, L.; Li, Y.; Jiang, D. Formation control of a multi-AUVs system based on virtual structure and artificial potential field on SE (3). Ocean Eng. 2022, 253, 111148. [Google Scholar] [CrossRef]
- Wang, N.; Li, H. Leader–follower formation control of surface vehicles: A fixed-time control approach. ISA Trans. 2020, 124, 356–364. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.H.; Yoo, S.J. Distributed event-triggered adaptive output-feedback formation tracking of uncertain underactuated underwater vehicles in three-dimensional space. Appl. Math. Comput. 2022, 424, 127046. [Google Scholar] [CrossRef]
- Wei, H.; Shen, C.; Shi, Y. Distributed Lyapunov-based model predictive formation tracking control for autonomous underwater vehicles subject to disturbances. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 5198–5208. [Google Scholar] [CrossRef]
- Mobayen, S.; Bayat, F.; ud Din, S.; Vu, M.T. Barrier function-based adaptive nonsingular terminal sliding mode control technique for a class of disturbed nonlinear systems. ISA Trans. 2023, 134, 481–496. [Google Scholar] [CrossRef]
- Ghaffari, V.; Mobayen, S.; ud Din, S.; Rojsiraphisal, T.; Vu, M.T. Robust tracking composite nonlinear feedback controller design for time-delay uncertain systems in the presence of input saturation. ISA Trans. 2022, 129, 88–99. [Google Scholar] [CrossRef] [PubMed]
- Chen, B.; Hu, J.; Zhao, Y.; Ghosh, B.K. Finite-time observer based tracking control of uncertain heterogeneous underwater vehicles using adaptive sliding mode approach. Neurocomputing 2022, 481, 322–332. [Google Scholar] [CrossRef]
- Su, Y.; Xue, H.; Liang, H.; Chen, D. Singularity avoidance adaptive output-feedback fixed-time consensus control for multiple autonomous underwater vehicles subject to nonlinearities. Int. J. Robust Nonlinear Control 2022, 32, 4401–4421. [Google Scholar] [CrossRef]
- Thanh, H.L.N.N.; Vu, M.T.; Nguyen, N.P.; Mung, N.X.; Hong, S.K. Finite-time stability of MIMO nonlinear systems based on robust adaptive sliding control: Methodology and application to stabilize chaotic motions. IEEE Access 2021, 9, 21759–21768. [Google Scholar] [CrossRef]
- Alattas, K.A.; Mobayen, S.; Din, S.U.; Asad, J.H.; Fekih, A.; Assawinchaichote, W.; Vu, M.T. Design of a non-singular adaptive integral-type finite time tracking control for nonlinear systems with external disturbances. IEEE Access 2021, 9, 102091–102103. [Google Scholar] [CrossRef]
- Mofid, O.; Amirkhani, S.; Din, S.u.; Mobayen, S.; Vu, M.T.; Assawinchaichote, W. Finite-time convergence of perturbed nonlinear systems using adaptive barrier-function nonsingular sliding mode control with experimental validation. J. Vib. Control 2023, 29, 3326–3339. [Google Scholar] [CrossRef]
- Rojsiraphisal, T.; Mobayen, S.; Asad, J.H.; Vu, M.T.; Chang, A.; Puangmalai, J. Fast terminal sliding control of underactuated robotic systems based on disturbance observer with experimental validation. Mathematics 2021, 9, 1935. [Google Scholar] [CrossRef]
- Alattas, K.A.; Vu, M.T.; Mofid, O.; El-Sousy, F.F.; Alanazi, A.K.; Awrejcewicz, J.; Mobayen, S. Adaptive nonsingular terminal sliding mode control for performance improvement of perturbed nonlinear systems. Mathematics 2022, 10, 1064. [Google Scholar] [CrossRef]
- Su, B.; Wang, H.b.; Wang, Y. Dynamic event-triggered formation control for AUVs with fixed-time integral sliding mode disturbance observer. Ocean Eng. 2021, 359, 109893. [Google Scholar] [CrossRef]
- Gao, Z.; Guo, G. Fixed-time sliding mode formation control of AUVs based on a disturbance observer. IEEE/CAA J. Autom. Sin. 2020, 7, 539–545. [Google Scholar] [CrossRef]
- Qin, H.; Si, J.; Wang, N.; Gao, L. Fast fixed-time nonsingular terminal sliding-mode formation control for autonomous underwater vehicles based on a disturbance observer. Ocean Eng. 2023, 270, 113423. [Google Scholar] [CrossRef]
- Thanh, H.L.N.N.; Vu, M.T.; Mung, N.X.; Nguyen, N.P.; Phuong, N.T. Perturbation observer-based robust control using a multiple sliding surfaces for nonlinear systems with influences of matched and unmatched uncertainties. Mathematics 2020, 8, 1371. [Google Scholar] [CrossRef]
- Thuyen, N.A.; Thanh, P.N.N.; Anh, H.P.H. Adaptive finite-time leader-follower formation control for multiple AUVs regarding uncertain dynamics and disturbances. Ocean Eng. 2023, 269, 113503. [Google Scholar] [CrossRef]
- Qin, H.; Si, J.; Wang, N.; Gao, L.; Shao, K. Disturbance Estimator-Based Nonsingular Fast Fuzzy Terminal Sliding-Mode Formation Control of Autonomous Underwater Vehicles. Int. J. Fuzzy Syst. 2023, 25, 395–406. [Google Scholar] [CrossRef]
- Meng, C.; Zhang, W.; Du, X. Finite-time extended state observer based collision-free leaderless formation control of multiple AUVs via event-triggered control. Ocean Eng. 2023, 268, 113605. [Google Scholar] [CrossRef]
- Wang, Y.; Li, H.; Guan, Y.; Chen, M. Continuous fixed-time formation control for AUVs using only position measurements. Ocean Eng. 2023, 269, 113476. [Google Scholar] [CrossRef]
- Xu, J.; Cui, Y.; Yan, Z.; Huang, F.; Du, X.; Wu, D. Event-triggered adaptive target tracking control for an underactuated autonomous underwater vehicle with actuator faults. J. Frankl. Inst. 2023, 360, 2867–2892. [Google Scholar] [CrossRef]
- Shi, Y.; Xie, W.; Zhang, G.; Dong, H.; Zhang, W. Event-Triggered Saturation-Tolerant Control for Autonomous Underwater Vehicles with Quantitative Transient Behaviors. IEEE Trans. Veh. Technol. 2023. early access. [Google Scholar] [CrossRef]
- Kim, J.H.; Yoo, S.J. Adaptive event-triggered control strategy for ensuring predefined three-dimensional tracking performance of uncertain nonlinear underactuated underwater vehicles. Mathematics 2021, 9, 137. [Google Scholar] [CrossRef]
- Wen, L.; Yu, S.; Zhao, Y.; Yan, Y. Adaptive dynamic event-triggered consensus control of multiple autonomous underwater vehicles. Int. J. Control 2023, 96, 746–756. [Google Scholar] [CrossRef]
- Kim, J.H.; Yoo, S.J. Distributed event-driven adaptive three-dimensional formation tracking of networked autonomous underwater vehicles with unknown nonlinearities. Ocean Eng. 2021, 233, 109069. [Google Scholar] [CrossRef]
- Fossen, T.I. Handbook of Marine Craft Hydrodynamics and Motion Control; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Ali, N.; Tawiah, I.; Zhang, W. Finite-time extended state observer based nonsingular fast terminal sliding mode control of autonomous underwater vehicles. Ocean Eng. 2020, 218, 108179. [Google Scholar] [CrossRef]
- Jin, Z.; Wang, C.; Liang, D.; Zuo, Z.; Liang, Z. Fixed-time leader-following consensus of multiple uncertain nonholonomic systems: An adaptive distributed observer approach. J. Frankl. Inst. 2022, 427, 6361–6391. [Google Scholar] [CrossRef]
- Xu, B.; Liang, Y.; Li, Y.X.; Hou, Z. Adaptive command filtered fixed-time control of nonlinear systems with input quantization. Appl. Math. Comput. 2022, 427, 127186. [Google Scholar] [CrossRef]
- Wang, F.; Lai, G. Fixed-time control design for nonlinear uncertain systems via adaptive method. Syst. Control Lett. 2020, 140, 104704. [Google Scholar] [CrossRef]
- Ding, F. Least squares parameter estimation and multi-innovation least squares methods for linear fitting problems from noisy data. J. Comput. Appl. Math. 2023, 426, 115107. [Google Scholar] [CrossRef]
- Ji, Y.; Jiang, A. Filtering-based accelerated estimation approach for generalized time-varying systems with disturbances and colored noises. IEEE Trans. Circuits Syst. II Express Briefs 2022, 70, 206–210. [Google Scholar] [CrossRef]
- Pan, J.; Zhang, H.; Guo, H.; Liu, S.; Liu, Y. Multivariable CAR-like system identification with multi-innovation gradient and least squares algorithms. Int. J. Control Autom. Syst. 2023, 21, 1455–1464. [Google Scholar] [CrossRef]
- Ding, F.; Liu, X.; Chen, H.; Yao, G. Hierarchical gradient based and hierarchical least squares based iterative parameter identification for CARARMA systems. Signal Process. 2014, 97, 31–39. [Google Scholar] [CrossRef]
- Ji, Y.; Liu, J.; Liu, H. An identification algorithm of generalized time-varying systems based on the Taylor series expansion and applied to a pH process. J. Process Control 2023, 128, 103007. [Google Scholar] [CrossRef]
- Li, M.; Liu, X. Iterative identification methods for a class of bilinear systems by using the particle filtering technique. Int. J. Adapt. Control Signal Process. 2021, 35, 2056–2074. [Google Scholar] [CrossRef]
- Xu, L. Parameter estimation for nonlinear functions related to system responses. Int. J. Control Autom. Syst. 2023, 21, 1780–1792. [Google Scholar] [CrossRef]
- Fan, Y.; Liu, X. Auxiliary model-based multi-innovation recursive identification algorithms for an input nonlinear controlled autoregressive moving average system with variable-gain nonlinearity. Int. J. Adapt. Control Signal Process. 2022, 36, 521–540. [Google Scholar] [CrossRef]
- Du, J.; Hu, X.; Krstić, M.; Sun, Y. Robust dynamic positioning of ships with disturbances under input saturation. Automatica 2016, 73, 207–214. [Google Scholar] [CrossRef]
- Gu, Y.; Zhu, Q.; Nouri, H. Identification and U-control of a state-space system with time-delay. Int. J. Adapt. Control Signal Process. 2022, 36, 138–154. [Google Scholar] [CrossRef]
- Ding, F.; Xu, L.; Zhang, X.; Zhou, Y. Filtered auxiliary model recursive generalized extended parameter estimation methods for Box–Jenkins systems by means of the filtering identification idea. Int. J. Robust Nonlinear Control 2023, 33, 5510–5535. [Google Scholar] [CrossRef]
- Wang, J.; Ji, Y.; Zhang, C. Iterative parameter and order identification for fractional-order nonlinear finite impulse response systems using the key term separation. Int. J. Adapt. Control Signal Process. 2021, 35, 1562–1577. [Google Scholar] [CrossRef]
- Xu, H.; Ding, F.; Champagne, B. Joint parameter and time-delay estimation for a class of nonlinear time-series models. IEEE Signal Process. Lett. 2022, 29, 947–951. [Google Scholar] [CrossRef]
- Ding, F.; Chen, T.; Iwai, Z. Adaptive digital control of Hammerstein nonlinear systems with limited output sampling. SIAM J. Control Optim. 2007, 45, 2257–2276. [Google Scholar] [CrossRef]
- Wang, J.; Ji, Y.; Zhang, X.; Xu, L. Two-stage gradient-based iterative algorithms for the fractional-order nonlinear systems by using the hierarchical identification principle. Int. J. Adapt. Control Signal Process. 2022, 36, 1778–1796. [Google Scholar] [CrossRef]
- Xu, L.; Chen, F.; Ding, F.; Alsaedi, A.; Hayat, T. Hierarchical recursive signal modeling for multifrequency signals based on discrete measured data. Int. J. Adapt. Control Signal Process. 2021, 35, 676–693. [Google Scholar] [CrossRef]
- Zhang, X.; Ding, F. Optimal adaptive filtering algorithm by using the fractional-order derivative. IEEE Signal Process. Lett. 2021, 29, 399–403. [Google Scholar] [CrossRef]
- Kang, Z.; Ji, Y.; Liu, X. Hierarchical recursive least squares algorithms for Hammerstein nonlinear autoregressive output-error systems. Int. J. Adapt. Control Signal Process. 2021, 35, 2276–2295. [Google Scholar] [CrossRef]
- Xu, L.; Ding, F. Separable synthesis gradient estimation methods and convergence analysis for multivariable systems. J. Comput. Appl. Math. 2023, 427, 115104. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
An, S.; Liu, Y.; Wang, X.; Fan, Z.; Zhang, Q.; He, Y.; Wang, L. Distributed Event-Triggered Fixed-Time Leader–Follower Formation Tracking Control of Multiple Underwater Vehicles Based on an Adaptive Fixed-Time Observer. J. Mar. Sci. Eng. 2023, 11, 1522. https://doi.org/10.3390/jmse11081522
An S, Liu Y, Wang X, Fan Z, Zhang Q, He Y, Wang L. Distributed Event-Triggered Fixed-Time Leader–Follower Formation Tracking Control of Multiple Underwater Vehicles Based on an Adaptive Fixed-Time Observer. Journal of Marine Science and Engineering. 2023; 11(8):1522. https://doi.org/10.3390/jmse11081522
Chicago/Turabian StyleAn, Shun, Yang Liu, Xiaoyuan Wang, Zhimin Fan, Qiang Zhang, Yan He, and Longjin Wang. 2023. "Distributed Event-Triggered Fixed-Time Leader–Follower Formation Tracking Control of Multiple Underwater Vehicles Based on an Adaptive Fixed-Time Observer" Journal of Marine Science and Engineering 11, no. 8: 1522. https://doi.org/10.3390/jmse11081522
APA StyleAn, S., Liu, Y., Wang, X., Fan, Z., Zhang, Q., He, Y., & Wang, L. (2023). Distributed Event-Triggered Fixed-Time Leader–Follower Formation Tracking Control of Multiple Underwater Vehicles Based on an Adaptive Fixed-Time Observer. Journal of Marine Science and Engineering, 11(8), 1522. https://doi.org/10.3390/jmse11081522







