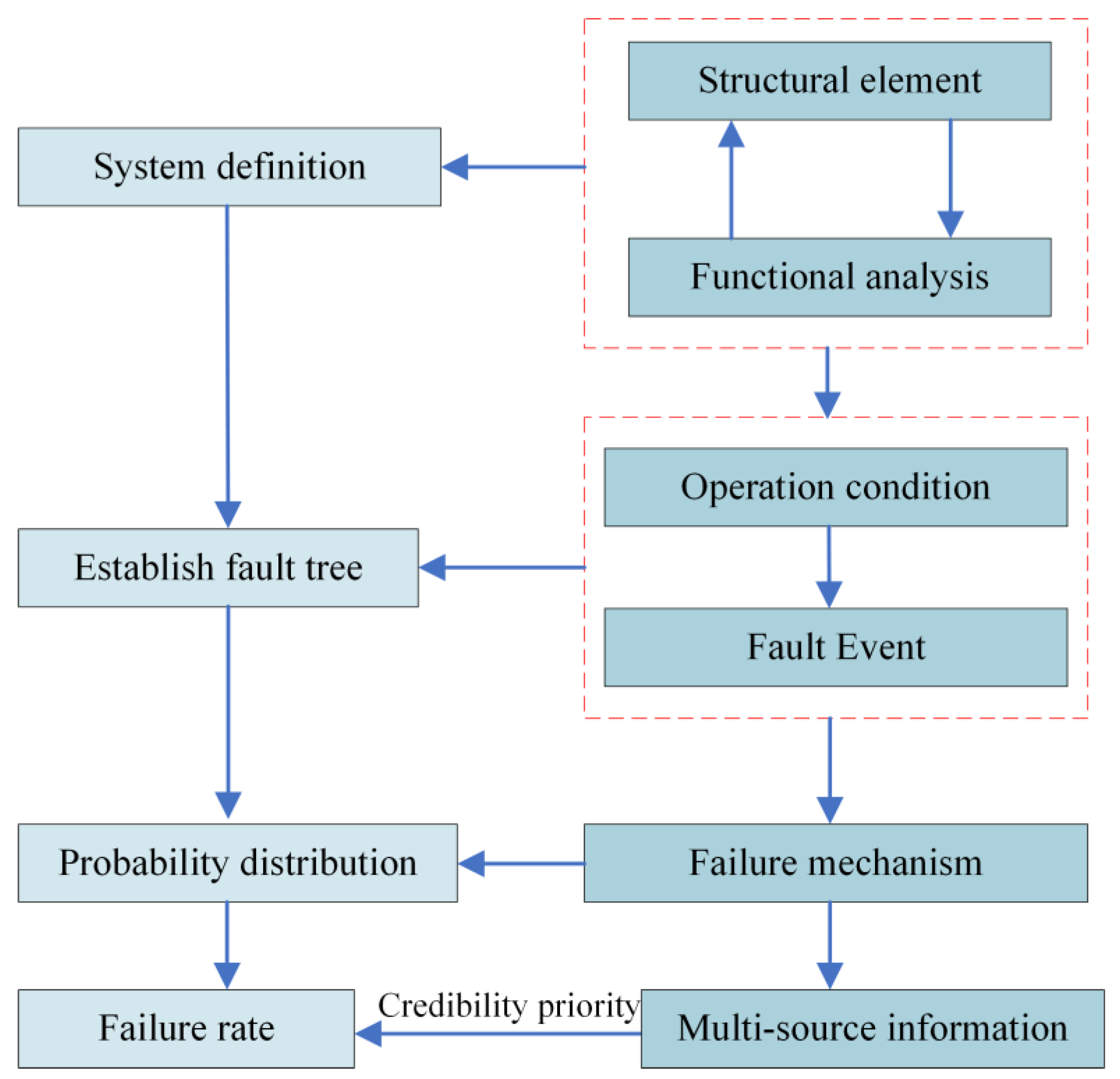
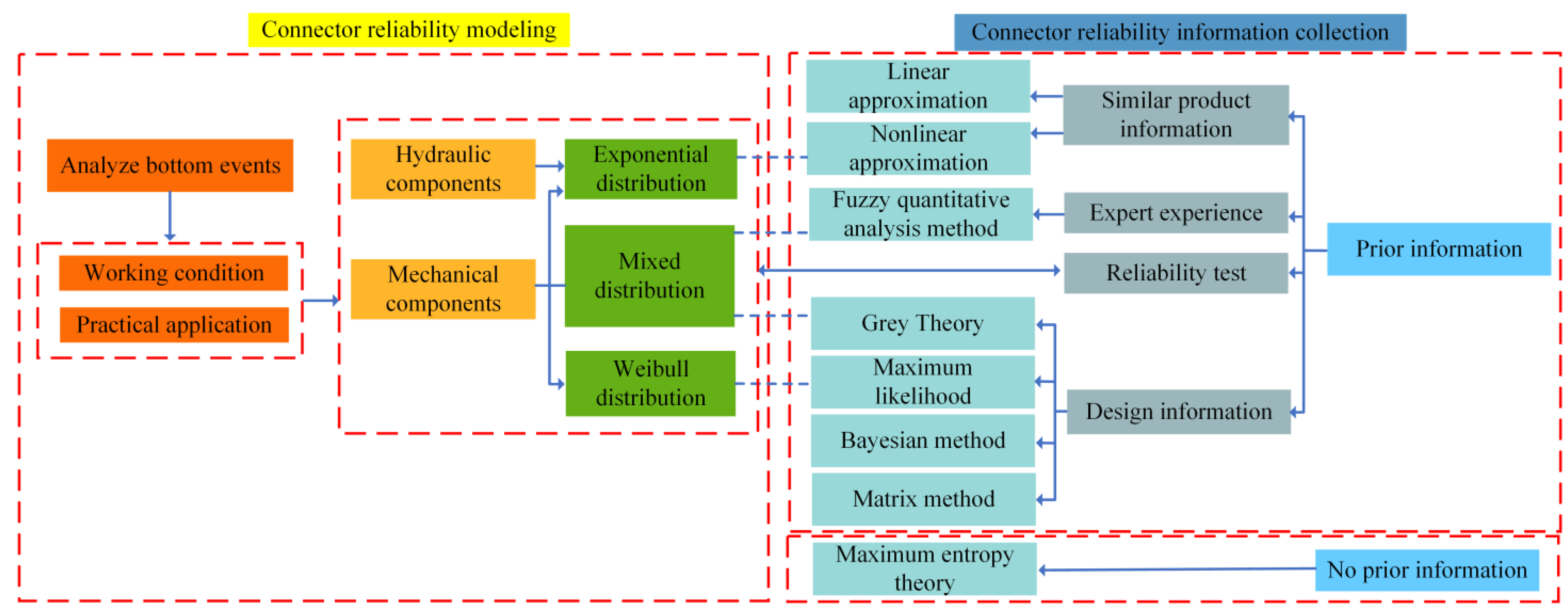
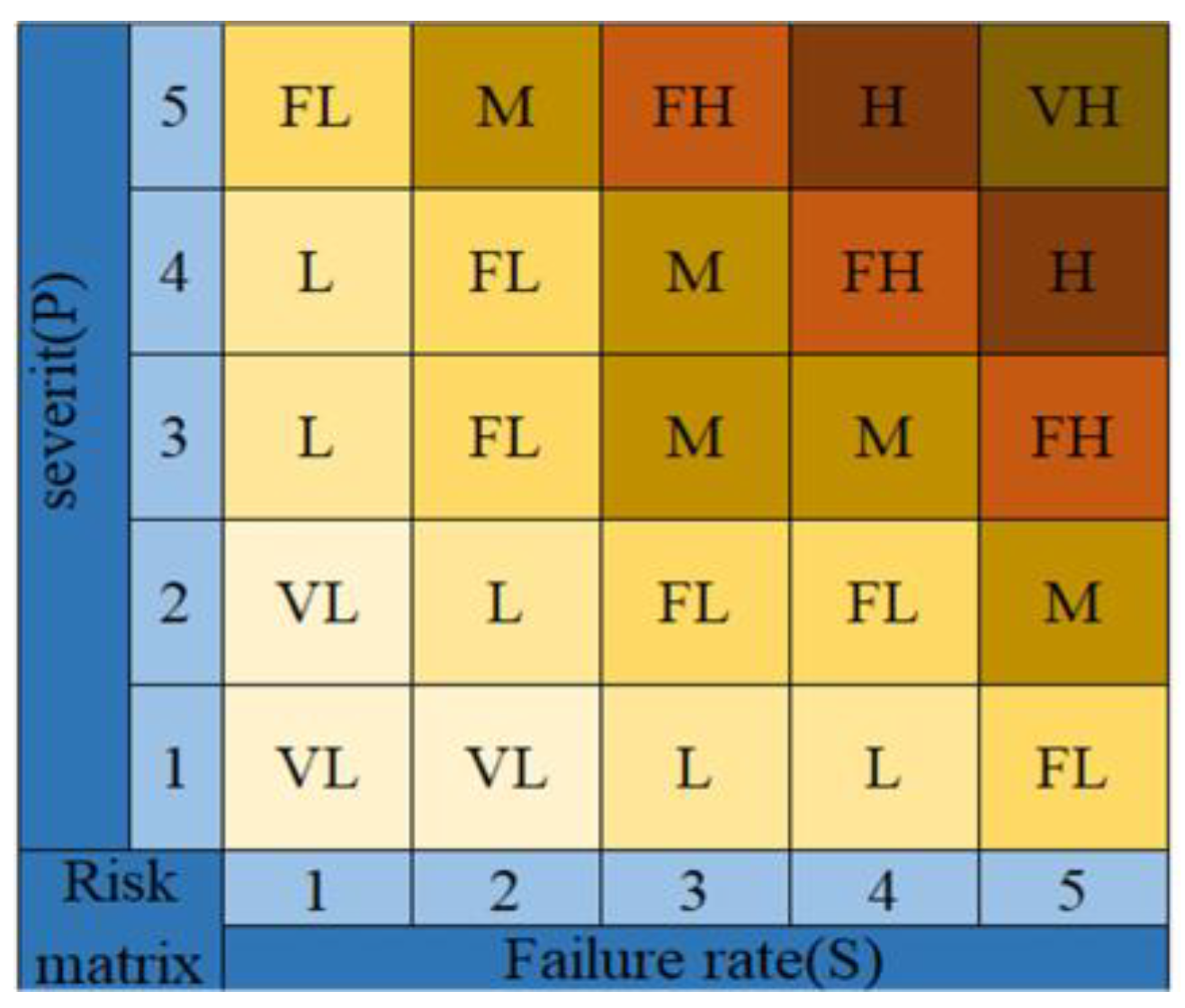
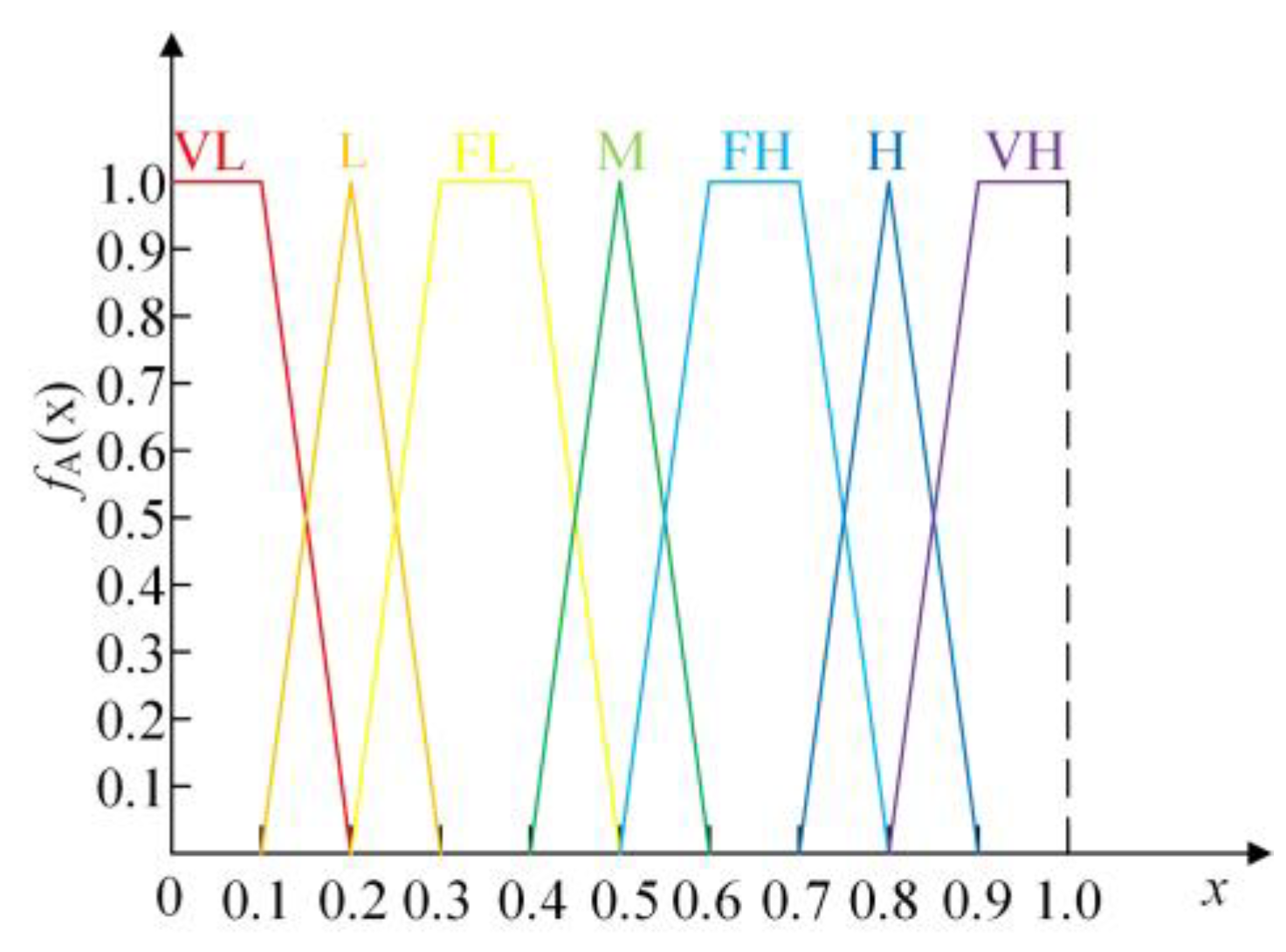
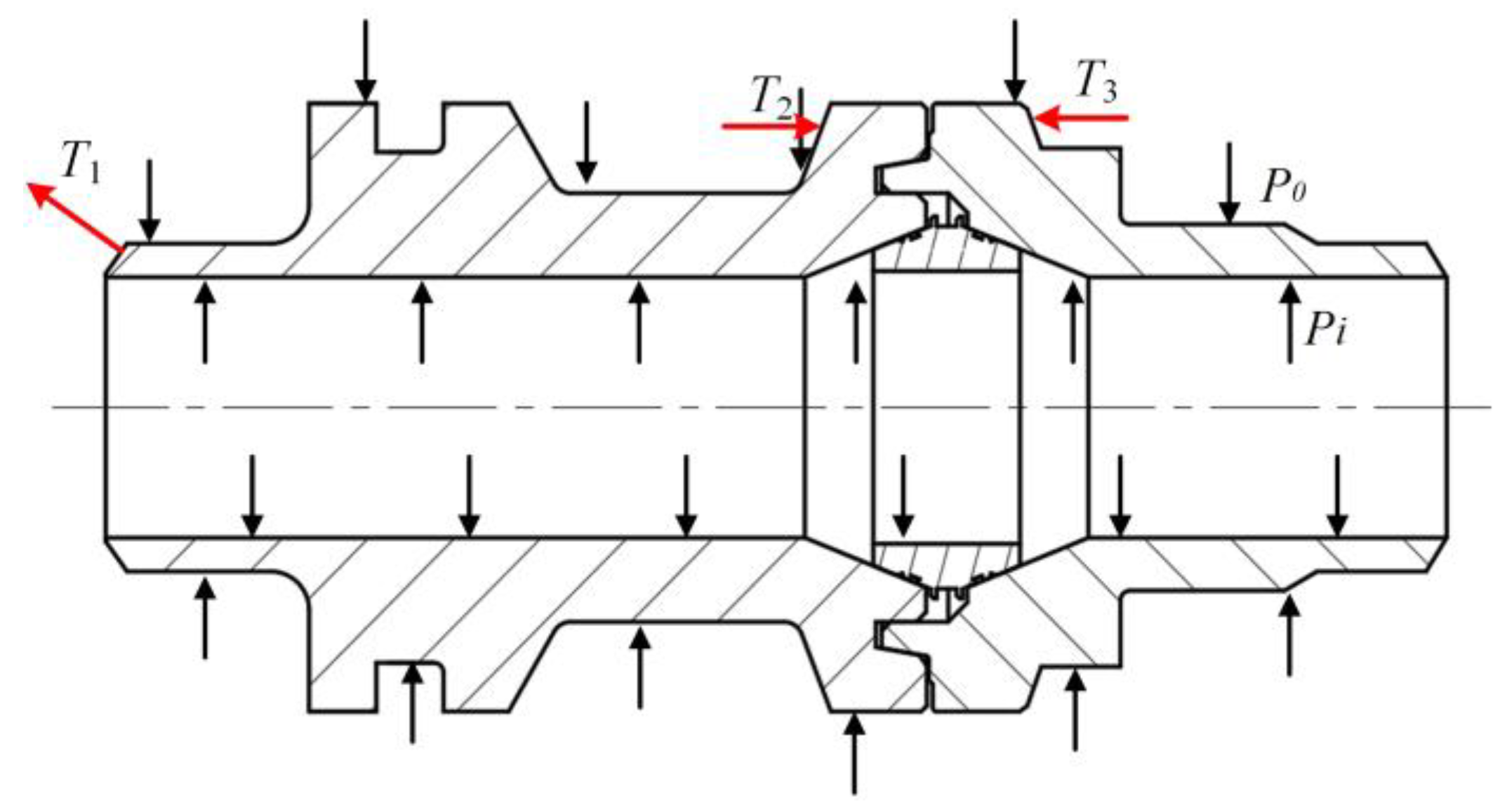
2.4.2. Reliability of Bottom Event under Expert Experience
Due to the limited availability of samples of the same type of connector, a risk assessment of different connector types can only serve as a reference and not be used for precise quantitative analysis using existing data. Expert scoring is an effective approach to resolve such issues [
35].
Since the level of expertise of invited experts may vary, this paper needs to weight the scores provided by them to reduce subjectivity. The weight of an expert can be comprehensively evaluated based on their job title, education, age, and years of experience in the industry. The lower the score given by an expert, the smaller their impact on the overall result. The factors for determining expert weights are shown in
Table 2 [
36].
Convert the expert’s resume into a score and add it up, so that the sum of all experts’ resumes is set to 1, and the weight of the
i-th expert can be normalized to:
In this paper, 7 connector experts were invited to assess the risk of horizontal clamp connector failure events, and their information is shown in the
Table 3.
Combined with Equation (6), the weights of the 7 experts can be obtained:
where
Ri is the weight value of the
ith expert.
For the fuzzification of event probabilities, only the degree of expression is needed, not the specifics, while the definition of the degree needs to be established. Fuzzy quantitative analysis methods represent the degree of membership of elements in a set with any value in the range of 0 to 1. When extended to the occurrence probability of basic events in engineering, it can be described using “very low (VL)”, “low (L)”, “relatively low (FL)”, “medium (M)”, “relatively high (FH)”, “high (H)”, and “very high (VH)”, using fuzzy numbers to express this language, and making their membership functions linearly related [
37,
38], as shown in
Figure 6. This paper chooses trapezoidal distribution to describe “very small”, “relatively small”, “relatively large”, and “very large”, and triangular distribution to describe “small”, “medium”, and “large”. The corresponding membership function graphs for these distributions are shown in
Figure 7.
The expression for this membership function is:
The λ-cuts of the membership functions represented by the expert fuzzy language are as follows:
where
l1,
l2, ……,
b1,
b2 represent the upper and lower limits of the
λ-cut sets.
Let
λ = (
v − 0.2)/0.1, then
v = 0.2 − 0.1
λ. Using the same method, the following formulas can be obtained:
Integrating the
λ-cuts of the expert opinions on the fuzzy language of a basic event yields the evaluation opinion and, thus, the average fuzzy number
W can be calculated as follows:
order
, then
,
.
Convert the above equation into a functional relationship using the membership function:
and using the left–right fuzzy ranking method, fuzzy numbers can be transformed into probability values. The fuzzy maximum set and fuzzy minimum set are defined as follows:
The left and right fuzzy numbers at the intersection of the fuzzy maximum set and fuzzy minimum set with the expert membership function are:
thus, the fuzzy possibility value
FPS(
W) is obtained as follows:
The fuzzy value is converted into fuzzy failure probability. The conversion equation of fuzzy possible value
FPS and fuzzy failure rate
FFR (failure probability) is:
where
k is the failure possibility coefficient when the maximum membership degree value is equal to 1. The expression for the failure possibility coefficient is:
where
ErM is the possible failure rate, obtained from reference values of top event statistical data or expert-provided empirical values.
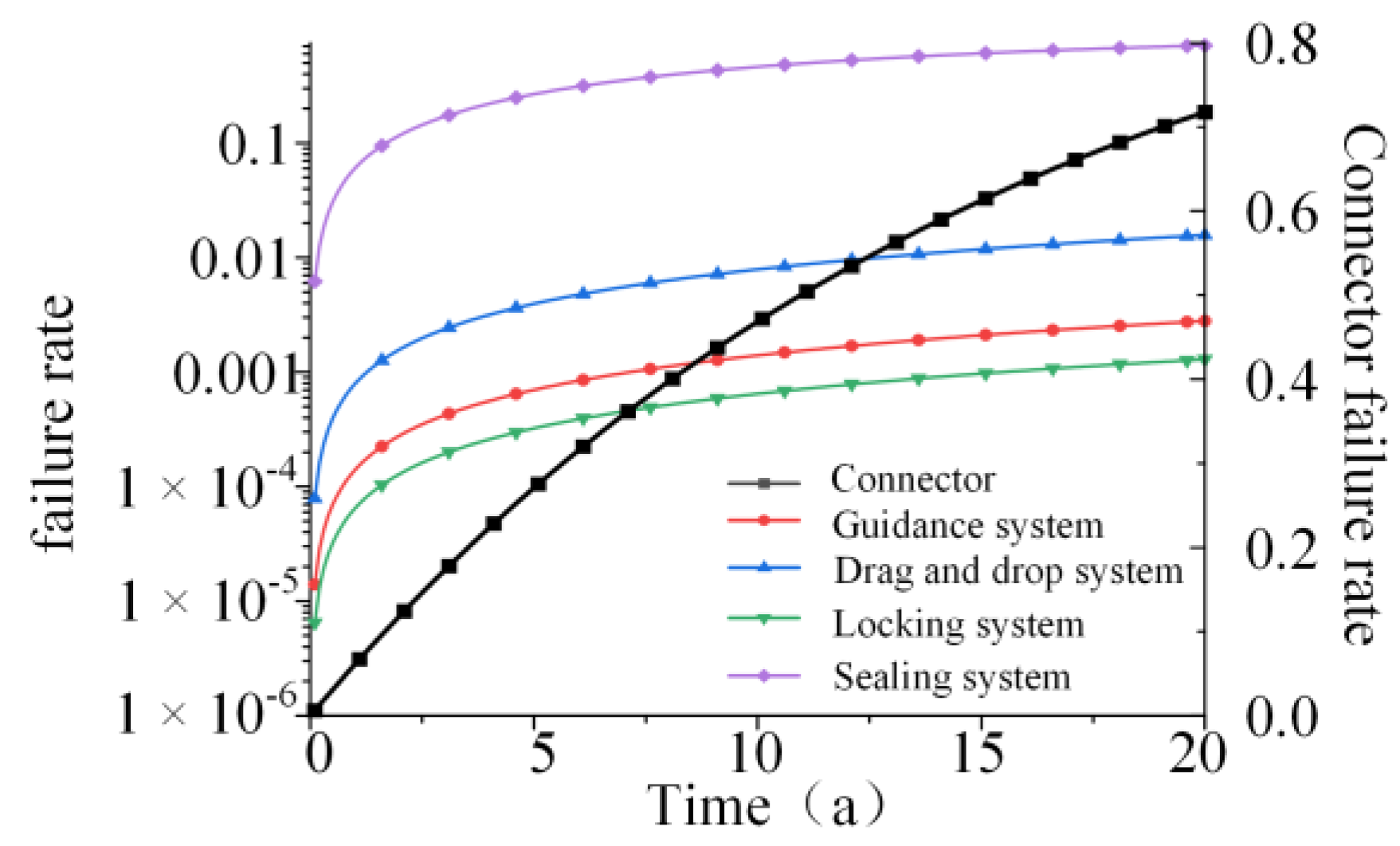
ErM values for M1, M2, M3, and M4 can be estimated based on the OREDA 2015 reliability database of connector failure samples [
39]. The
ErM values are 0.03 for M1, 0.03 for M2, 0.02 for M3, and 0.13 for M4.
The evaluation of each failure event by experts is shown in
Table 4.
2.4.3. Reliability of Bottom Events under Design Information
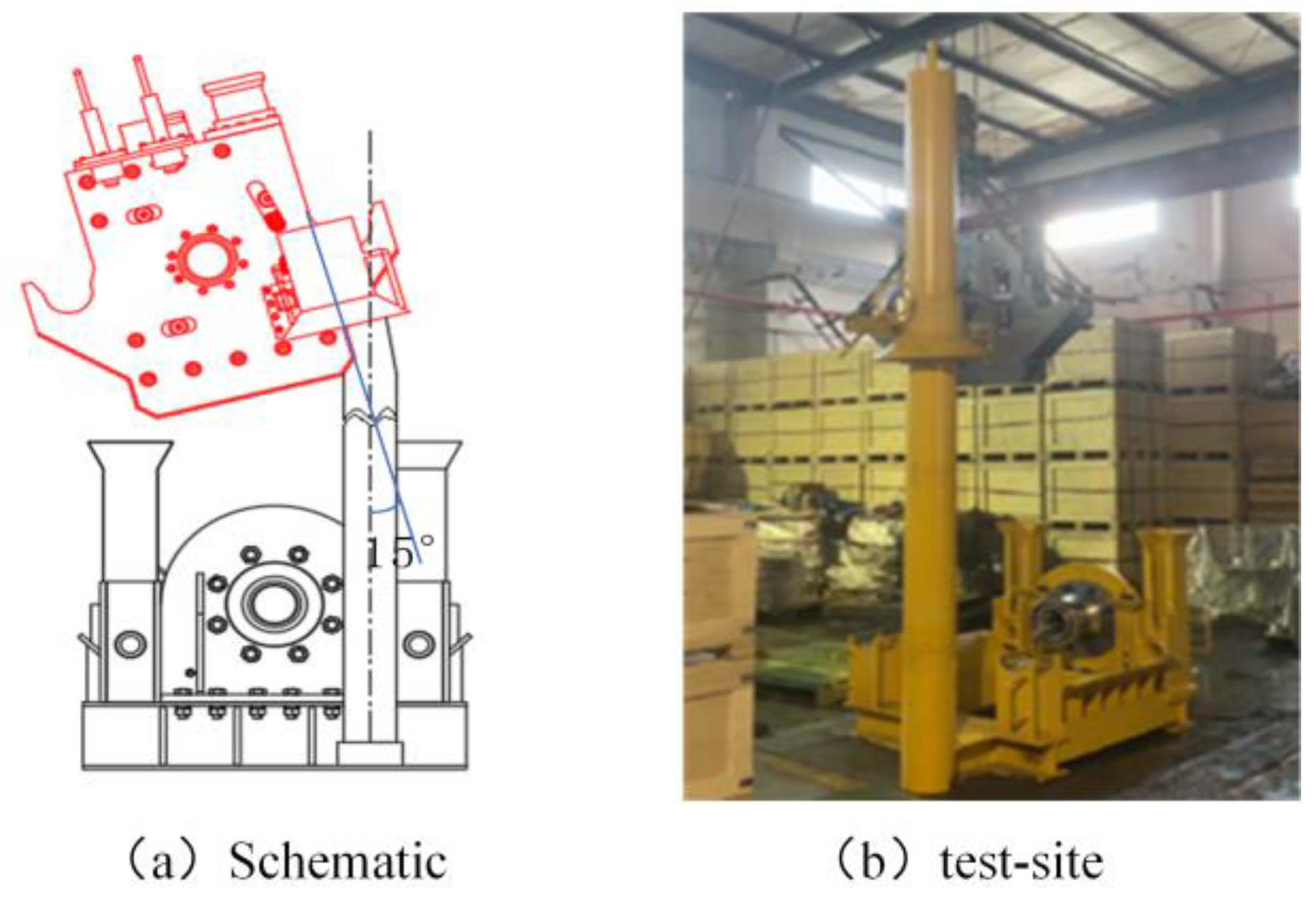
The reliability of the bottom events X43, X46, and X50 in the fault tree is time-variant reliability. The yield strength of the flange manufacturing material is 515 MPa, and according to the design information, its working process is subject to maximum stress values far below the yield strength. Although fatigue can occur during the process below the material yield strength, the difference between the two is too large. Therefore, the corrosion, which is the focus of attention, will win in the competition of failure mechanisms with fatigue and wear, and occur earlier than other mechanisms. Therefore, corrosion should be analyzed as the cause of pipeline rupture.
Expert experience is not applicable in predicting the development of failure events. Instead, the Weibull distribution can be utilized to describe the basic model of the corrosion of the connector sealing system, as it is a distribution form that describes cumulative wear failure. Reliability information of the sealing structure of the connector can be obtained by combining design information and predicting failure modes from the perspective of design reliability, and then fitting various parameters of the Weibull distribution.
- (a)
Corrosion Prediction Analysis
Figure 8 shows the stress state of the sealing structure in service. Sacrificial anodes are used to prevent external corrosion at the cross-joint pipe end. The corrosion rate of the material 12Cr2Mo1 in the fully immersed area is approximately 0.21 mm/a. This means that the flange corrosion rate is roughly 0.019 mm/a (95% corrosion protection rate) under cathodic protection [
40]. To prevent internal corrosion by cathodic protection, corrosion inhibitors are added to prevent the corrosive medium from contacting the inner wall and reacting with the corrosive medium. The corrosion rate for the internal corrosion depth d can be estimated using the empirical “de Waard & Williams” equation, which incorporates the effect of operating pressure and environmental temperature on the corrosion rate [
41]:
where
T(
t) represents the Kelvin temperature, and 0 °C is 273.15 Kelvin;
is the molar fraction of CO
2 in the gas phase, 0.02; Δ
poper is the operating pressure, 345 bar. Substituting the environmental conditions into Equation (18) yields the internal corrosion rate,
v(
t), of 1.25 mm/a.
To make the corrosion results closer to the actual working conditions during the service life of the connector, the corrosion degree is expected to be adjusted by considering the influence of the corrosive agent and the uncertainty of the model as factor [
42]:
where
Xac is the corrosion rate coefficient of cathodic protection, which is 0.6;
a(
t) is the distribution of corrosion inhibitor, which follows a beta (
a, 50%) distribution;
Xcorr is the uncertainty of the empirical corrosion rate model, which follows an
N (0.2, 20%) distribution.
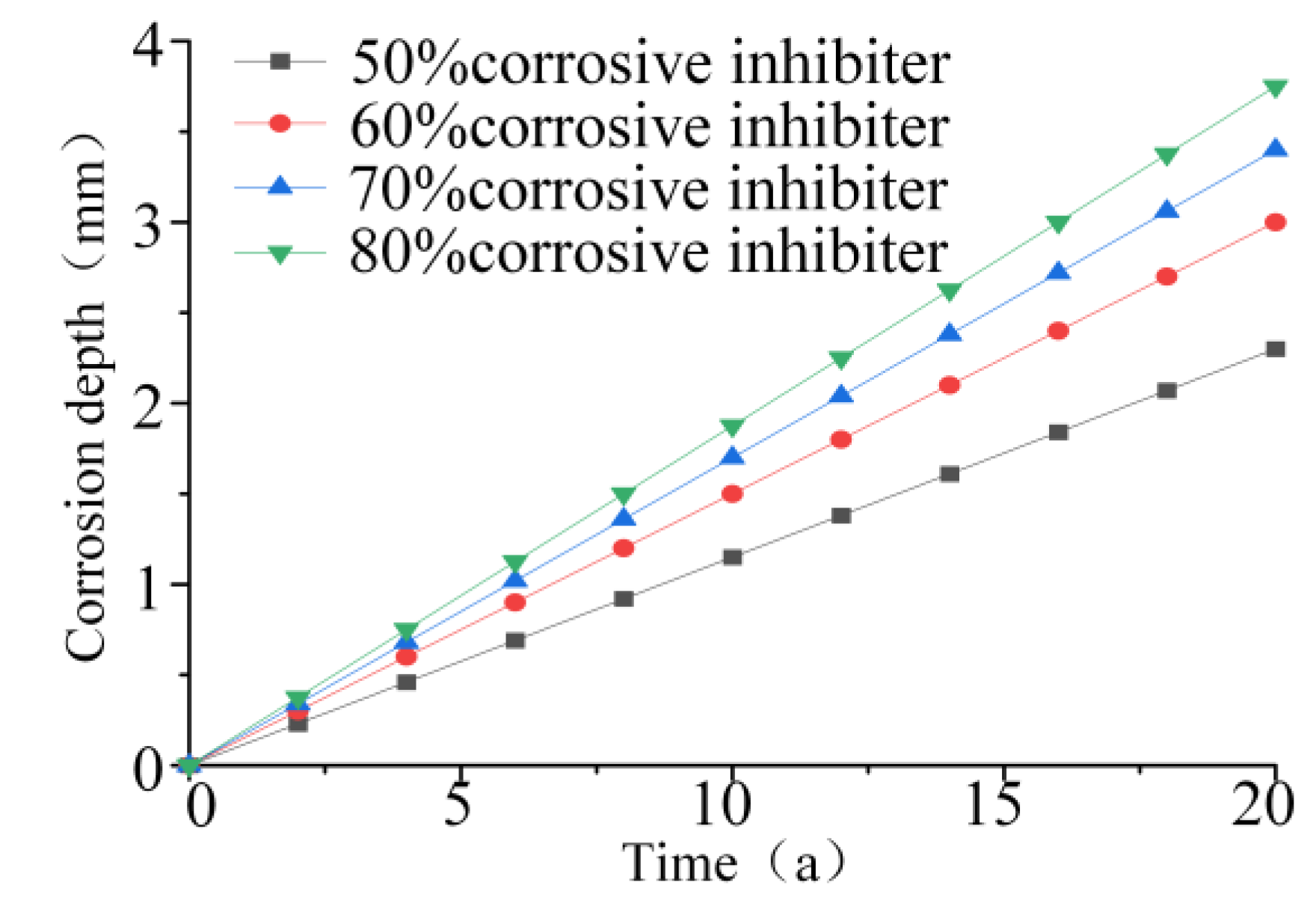
Combining Equations (18) and (19), the relationship between the utilization rate of the corrosion inhibitor and the expected corrosion and service life can be obtained, as shown in
Figure 9. In this paper, the maximum entropy theory is used to adopt a 50% utilization rate of the corrosion inhibitor.
Scholars have conducted extensive research and tests to prove that there is no correlation between corrosion depth
d and corrosion length
l [
43,
44,
45]. In actual working conditions, flange corrosion is mostly characterized by “6 o’clock” flat corrosion. According to Sakakibara’s research conclusions, when the longitudinal defect length
l is relatively large compared to the width defect
w, the influence of the width defect on the strength of the pipeline can be ignored [
46]. However, for a known defect depth, there are many factors that affect the defect length, and there is no good mathematical model to calculate it. Generally, statistical measurements are used to obtain data. Therefore, this paper refers to an empirical equation for defect length growth rate of 3 mm/a in marine engineering [
40].
The width
w growth rate in the defect area is calculated based on the empirical equation for length growth rate, with a length-to-width ratio of 0.2, i.e., the width
d is 0.6 mm/a. Then, the non-conservative Equation (20) proposed by Hopkins is used to correct the length and width growth rates, as they previously pointed out that the precise calculation of the cross-sectional area of the corrosion zone was overly conservative [
47]:
the 2/3 coefficient proportion is allocated to the defect length and width, which results in the parameter model of the defect area within a given time:
This paper mainly focuses on the corrosion degradation problem of the terminal flange. According to the relationship between the ultimate pressure equation and the wall thickness given by ASME-B31G, the general standard for evaluating the strength of the pipeline is the minimum wall thickness of the pipeline after corrosion. Therefore, the minimum wall thickness of the flange can be used as the inner and outer diameter of the pipeline for analysis, which results in more realistic results [
48]:
where
P is the carrying pressure in MPa,
σ is the average stress,
d is the inner diameter in mm;
is the average stress in MPa,
D is the outer diameter in mm, and
M is the expansion coefficient. The expansion coefficient
M can be expressed as:
By combining Equations (22) and (23), the maximum corrosion depth can be obtained:
- (b)
Finite Element Analysis
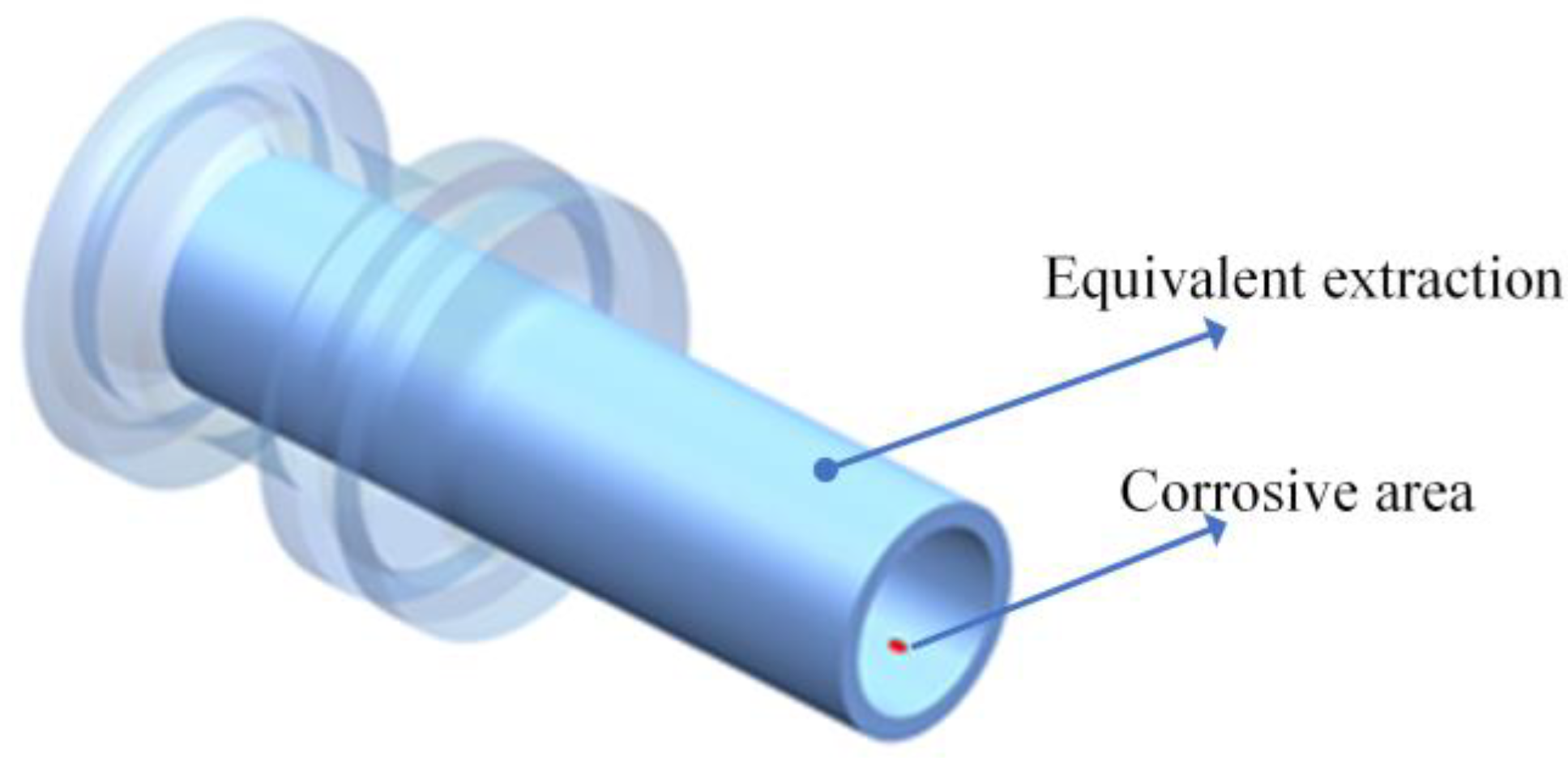
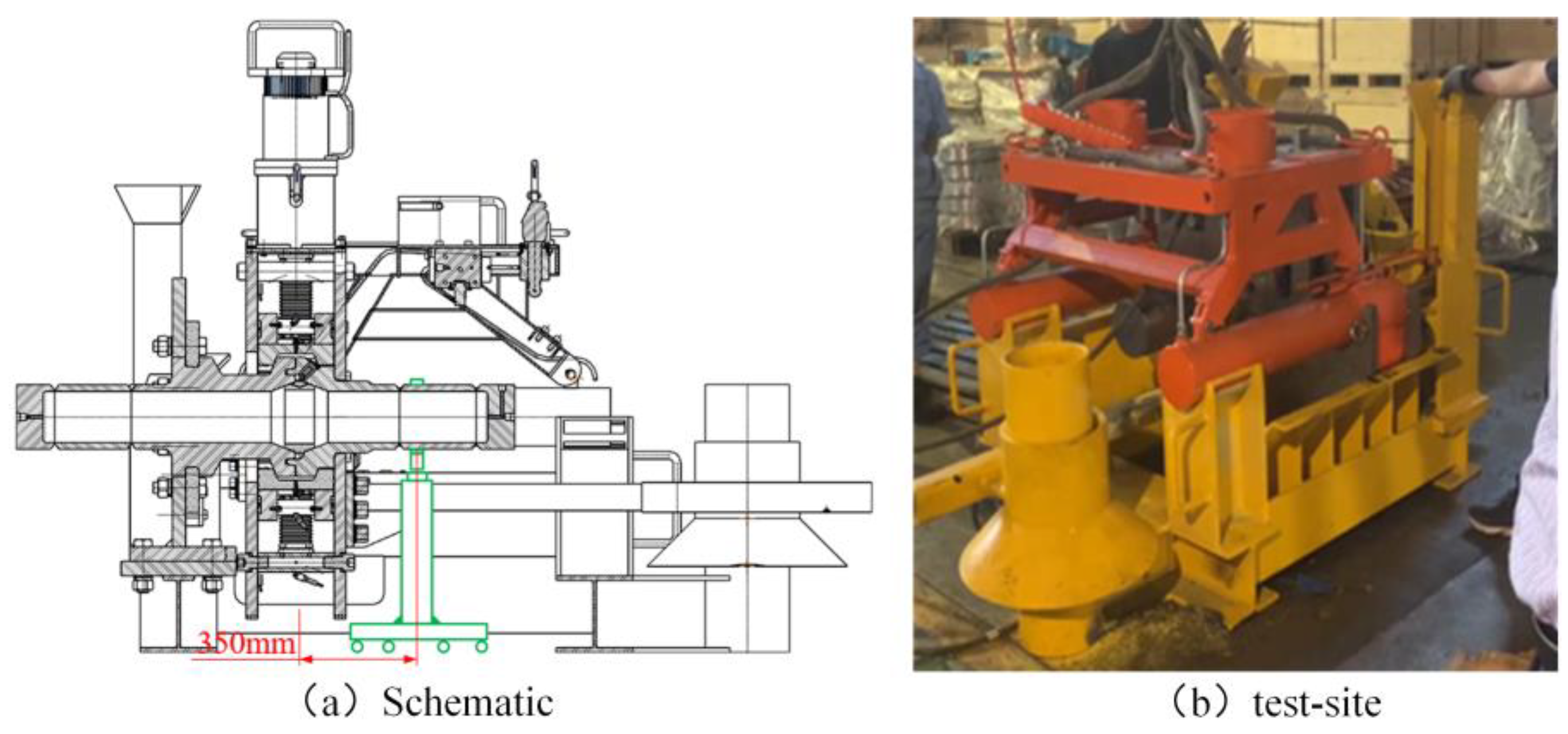
As shown in
Figure 10, the terminal flange is parametrically modeled and simplified into a solid part, and the equivalent length is 428 mm, the inner radius is 66.85 mm, and the outer radius is 84.15 mm, combined with the design information. As the corrosion on the flange is mostly in the form of long plateau corrosion, the internal corrosion point is equivalent to a semi-elliptical defect after parameterization, which is approximately equivalent to long plateau corrosion. The corrosion defect is located at the boundary where the wall thickness is the thinnest, as this is where the pipeline is weakest due to the welding of the cross-connection pipe, and also where corrosion is most likely to occur.
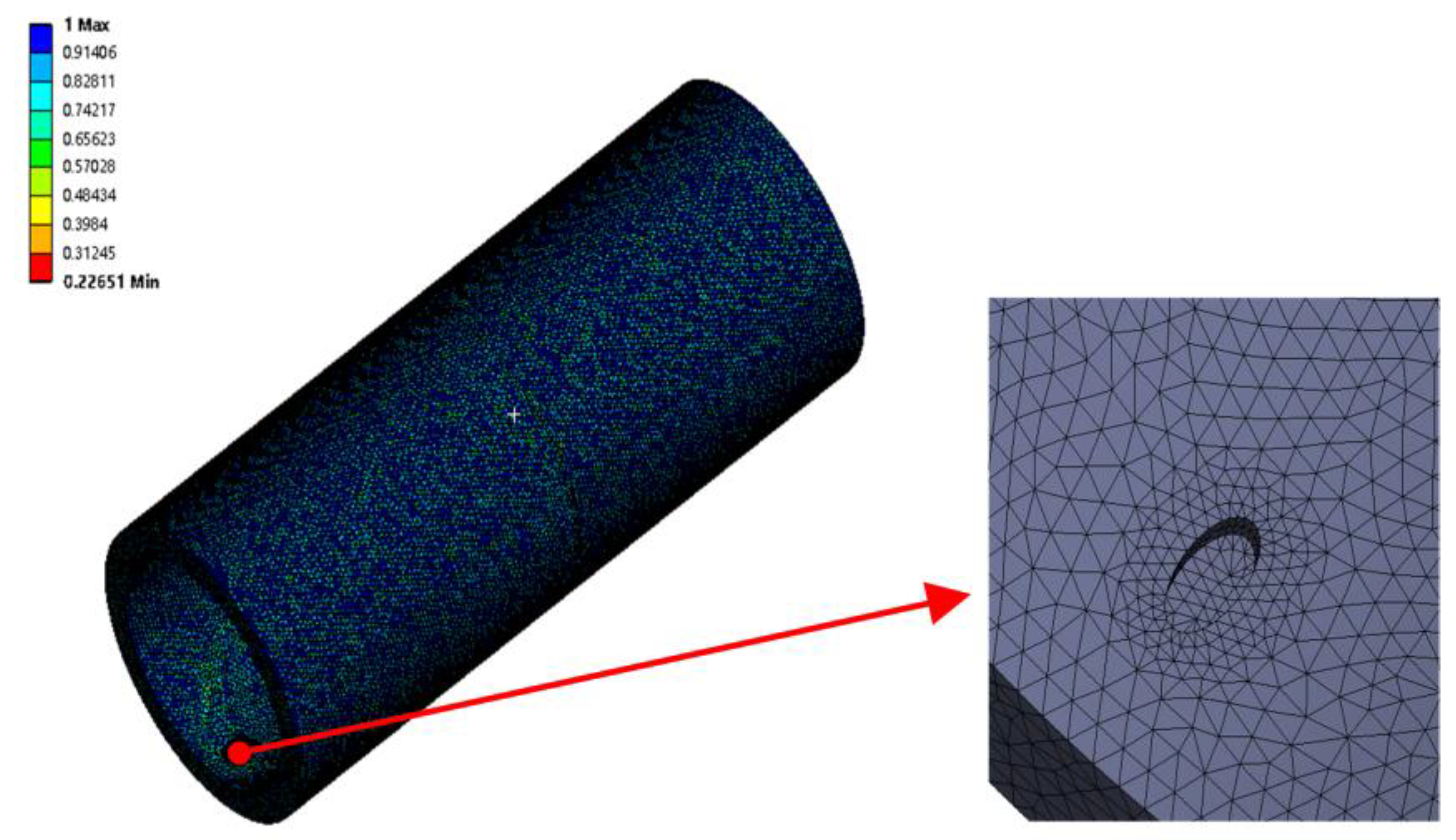
Finite element analysis of the seal structure with corrosion defects is performed using Ansys Workbench software. The Geometry module in the static structural module is selected to partition the model into meshes. The material properties of the equivalent model are as follows: the flange is made of 12Cr2Mo1, with an elastic modulus of 2.1 × 10
5 MPa, a Poisson’s ratio of 0.3, and a yield strength of 515 MPa. The meshing method is Hex Dominant, the mesh size is 3 mm, and the number of meshes after partitioning is 245,824. The mesh in the defect area is refined by 1 mm, as shown in
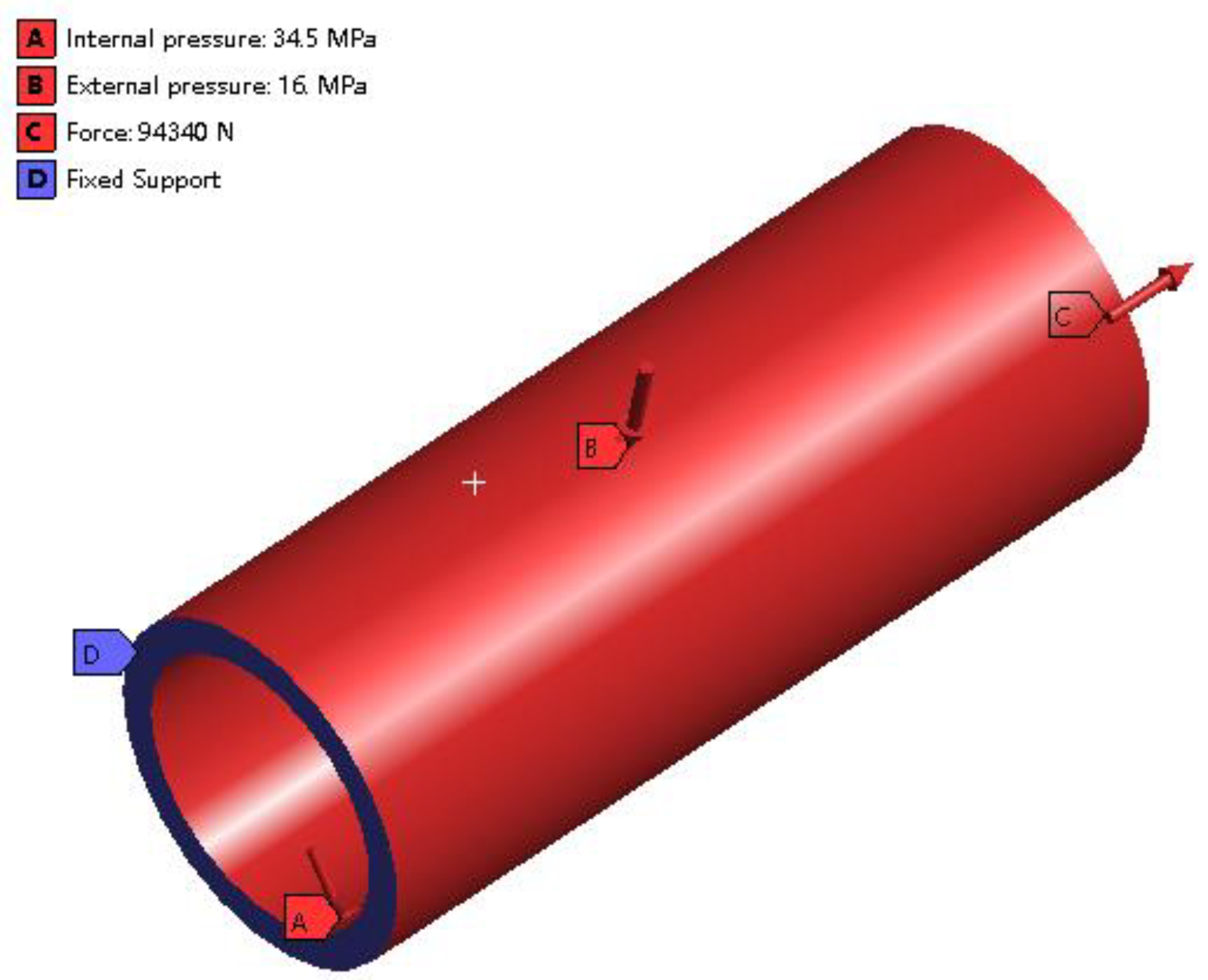
Figure 11. According to the connector design operating conditions, an internal pressure of 34.5 MPa is applied to the inner surface of the flange, an external pressure of 16 MPa is applied to the outer surface, and a cross-joint pipe acting load of 94,340 N is applied to the left end surface of the equivalent body, and a constraint is applied to the right end surface, as shown in
Figure 12.
According to the third strength theory, under the combined effect of the uniform reduction of the outer wall thickness of the flange and the defects caused by internal point corrosion, the sealing failure of the flange defect area occurs when the equivalent stress under internal and external pressure exceeds the yield strength of the material itself, so the maximum equivalent stress in the defect area should be analyzed.
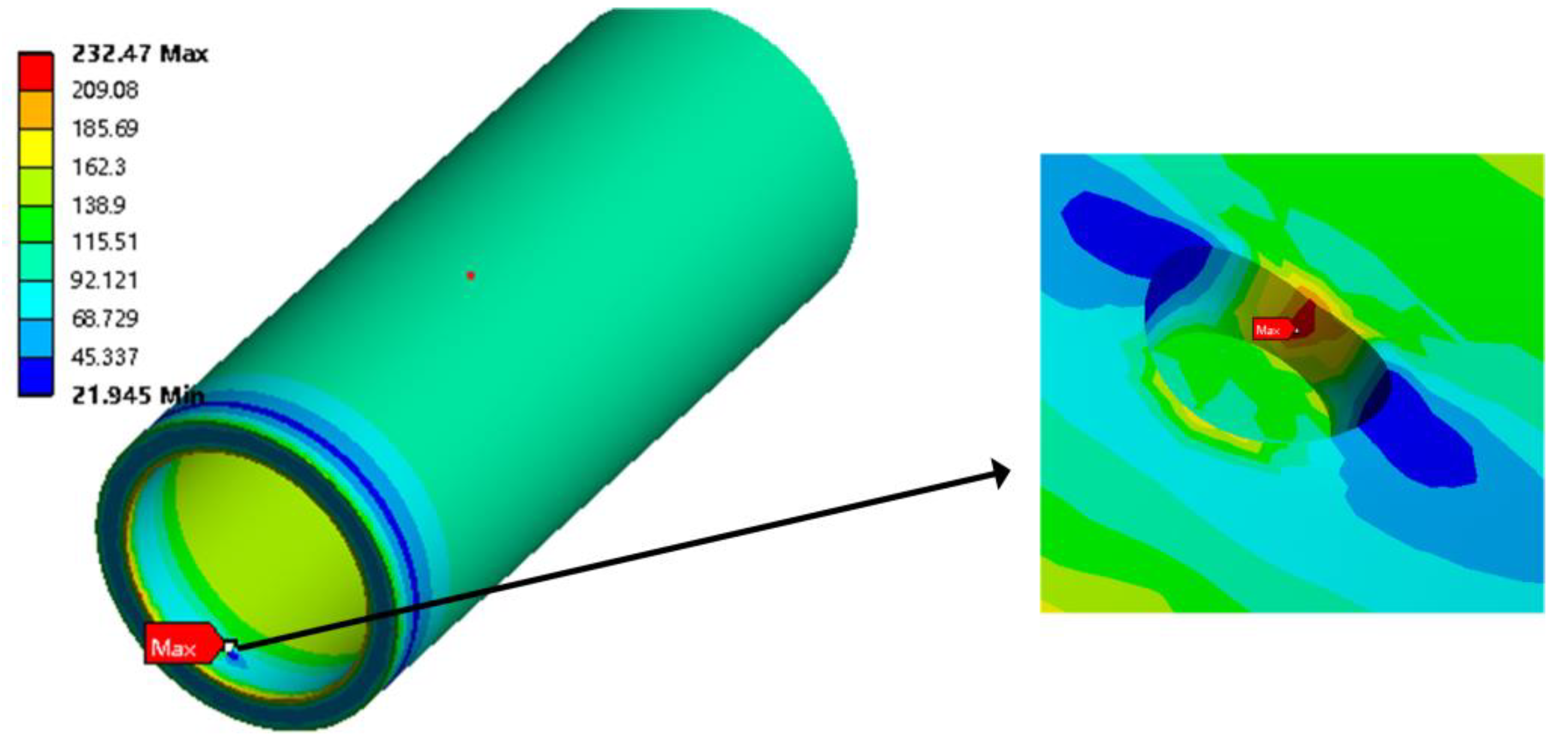
Equivalent stress and the maximum stress on the inner wall surface of the defect in the upper flange were selected as the solution results. As shown in
Figure 13, the maximum equivalent stress of the equivalent body is 232.47 MPa; when the defect depth is 0.5 mm, the length is 4 mm, and the width is 2 mm, the maximum equivalent stress of the defect area is 141.8 MPa.
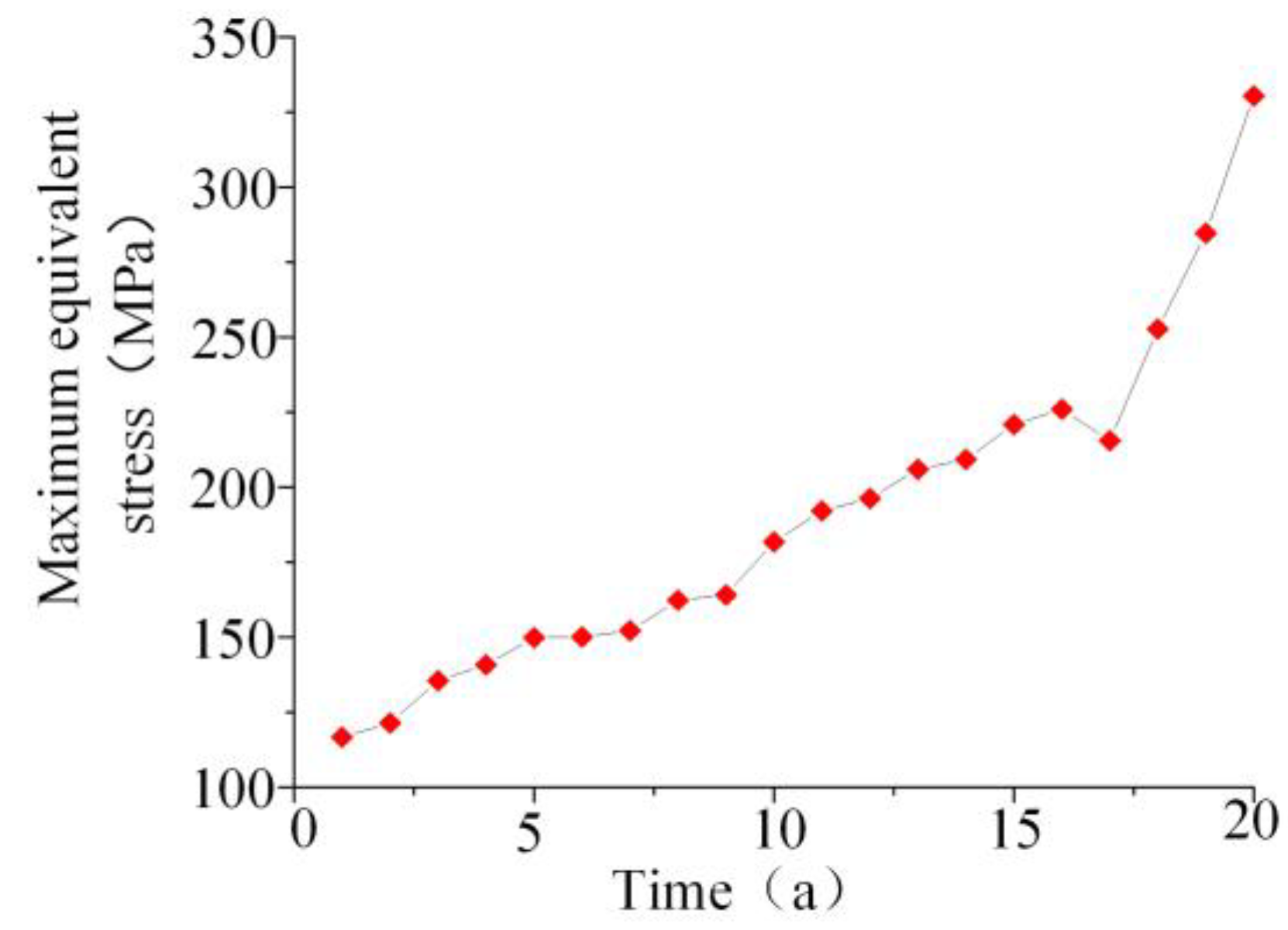
Load the Six Sigma analysis module in Ansys Workbench, select the Design of Experiments type of custom+Sampling option, predict the strength change over 20 years, and choose a sample size of 20 to obtain the equivalent stress of the corroded defect area as shown in
Figure 14:
Grey system theory is a method based on the analysis and prediction of a small amount of sample data, which can handle uncertain and incomplete information. Grey system theory is mainly used to analyze and predict systems that are difficult to model and analyze through traditional methods [
49]. The data from
Figure 14 show that the stress results are generally increasing, and the rate of increase in the last three years is significantly greater. The maximum stress is 116.7 MPa without defects, and the maximum stress in the defect area is 330.4 MPa after 20 years.
- (c)
Weibull Parameter Fitting
The unknown parameters
η,
β, and
γ of the Weibull distribution are estimated by fitting using the stress transformation of the connection flange combined with the grey prediction model GM (1,1) and transforming Equation (4):
Establish the GM (1,1) model, with the whitening differential equation:
where
a is the development coefficient and
b is the grey action amount. Solving the whitening differential equation and discretizing it gives the time response function of the GM (1,1) model:
and insert the equivalent stress values of different years from
Figure 13 into the Equation (28):
Generate the first-order accumulated data of the original data
x(0) to obtain Equation (29):
where
. Generate the mean of
X(1) near numbers for Equation (30)
After obtaining the sequence, use the least squares method to estimate the parameter variables of GM (1,1):
where
;
.
By substituting
η,
β, and
γ into Equation (25), the corrosion reliability function of the flange can be obtained. Combining with the reliability database OREDA [
39], which provides a welding failure rate of 3 × 10
−6, the mixed distribution of flange failure due to rupture can be obtained.