Investigation on the Erosion Characteristics of Liquid–Solid Two-Phase Flow in Tee Pipes Based on CFD-DEM
Abstract
:1. Introduction
2. Numerical Model
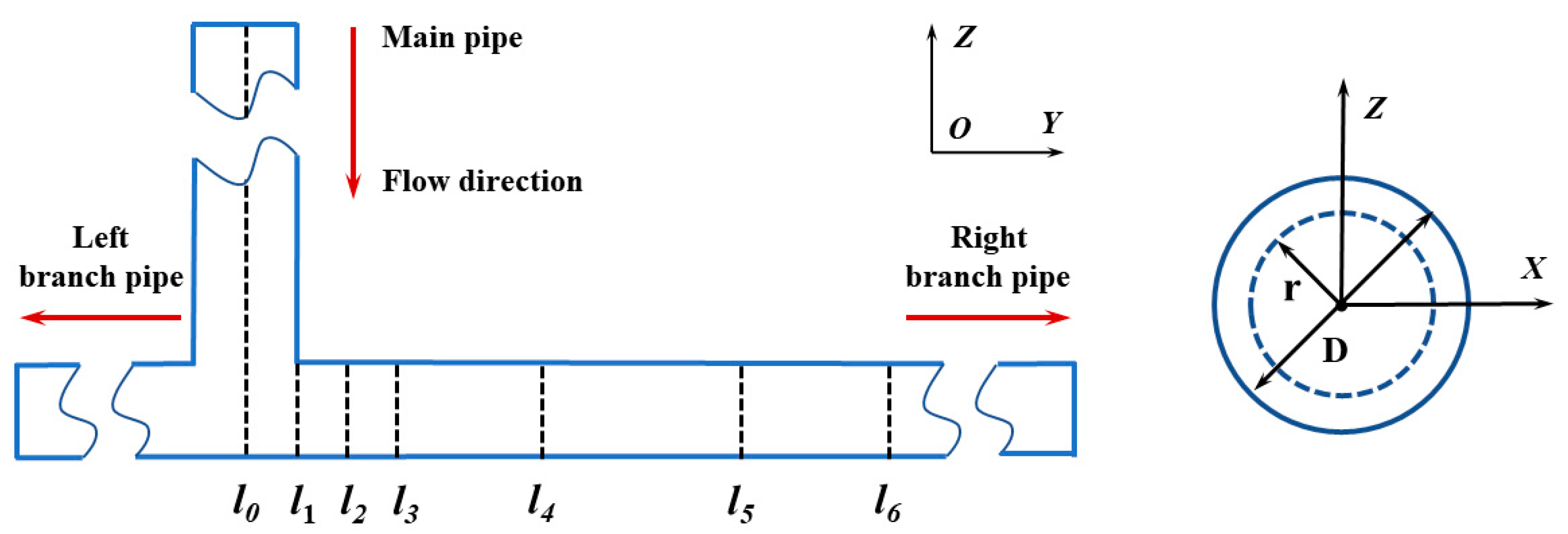
2.1. Tee Pipe Physical Model Establishment
2.2. Mathematical Equations
2.2.1. Governing Equations of Liquid Phase
2.2.2. Governing Equations of Solid Phase
2.2.3. The Program Interaction and Calculation Algorithm
2.3. Erosion Model
2.4. Numerical Simulation Verification of Vertical Pipe
2.5. Verification of Erosion Model
3. Geometric Model and Boundary Conditions
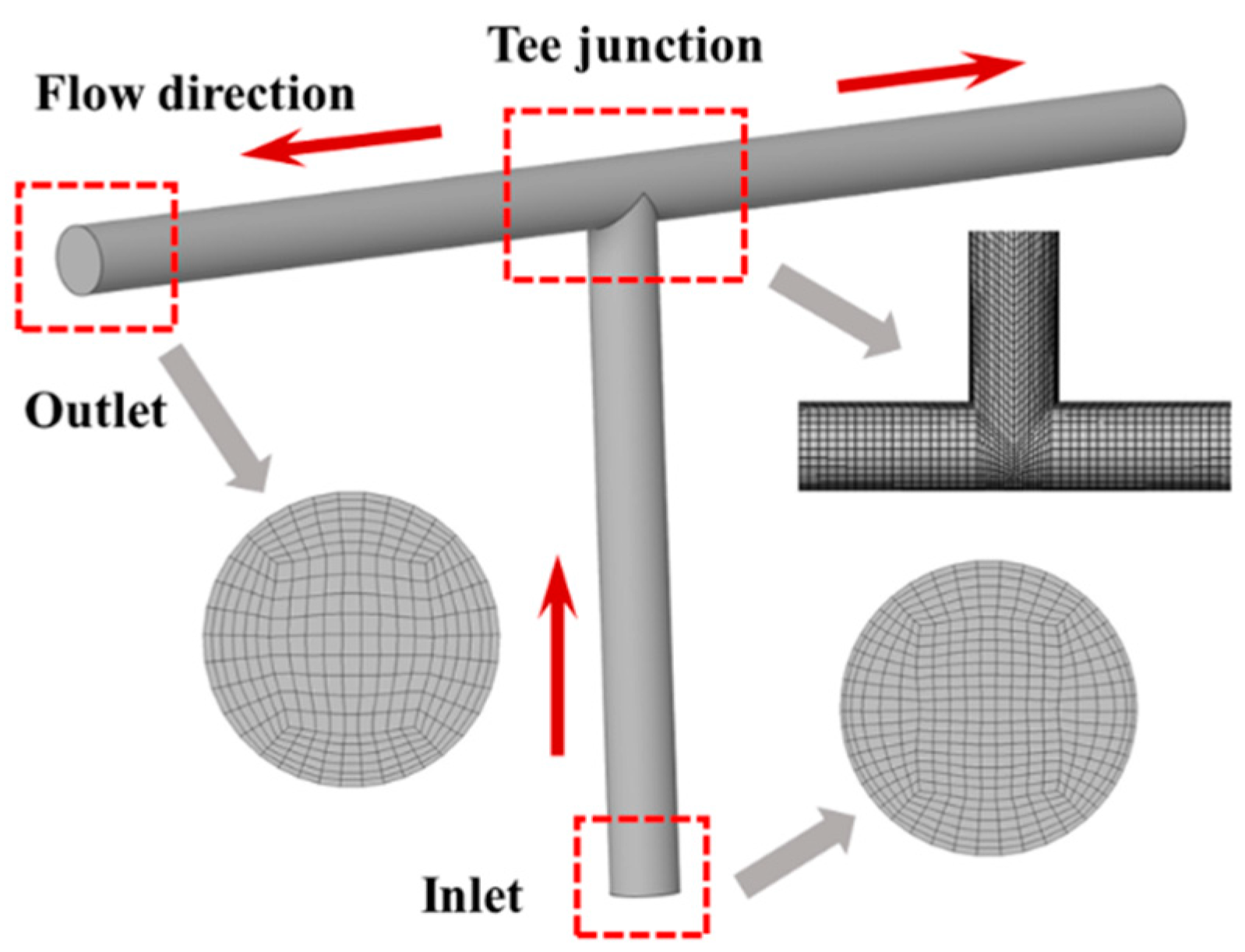
3.1. Numerical Model Establishment
3.2. Independence Verification of Mesh
3.3. Boundary Condition Settings
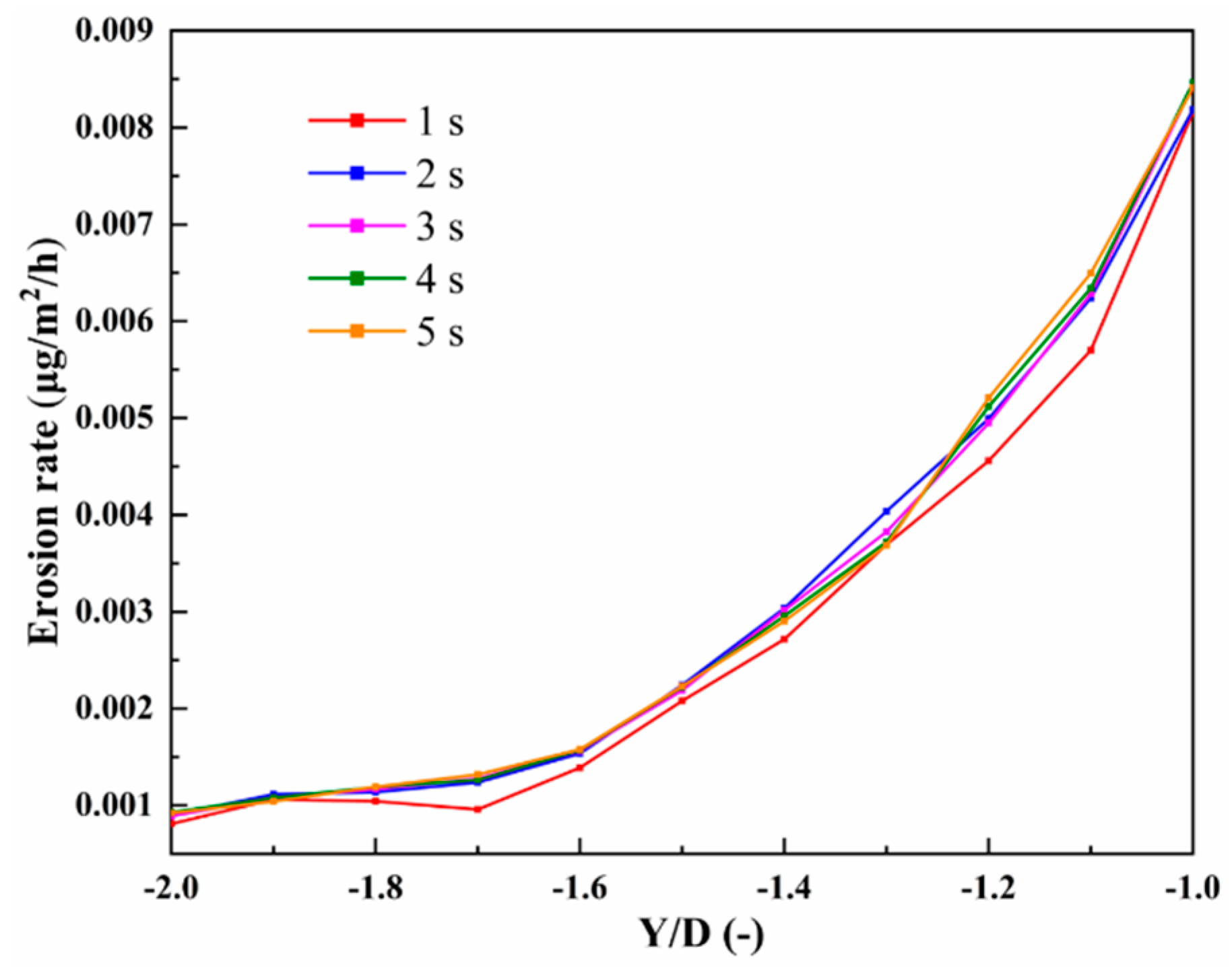
3.4. Independence Verification of Calculation Time
4. Results and Discussion
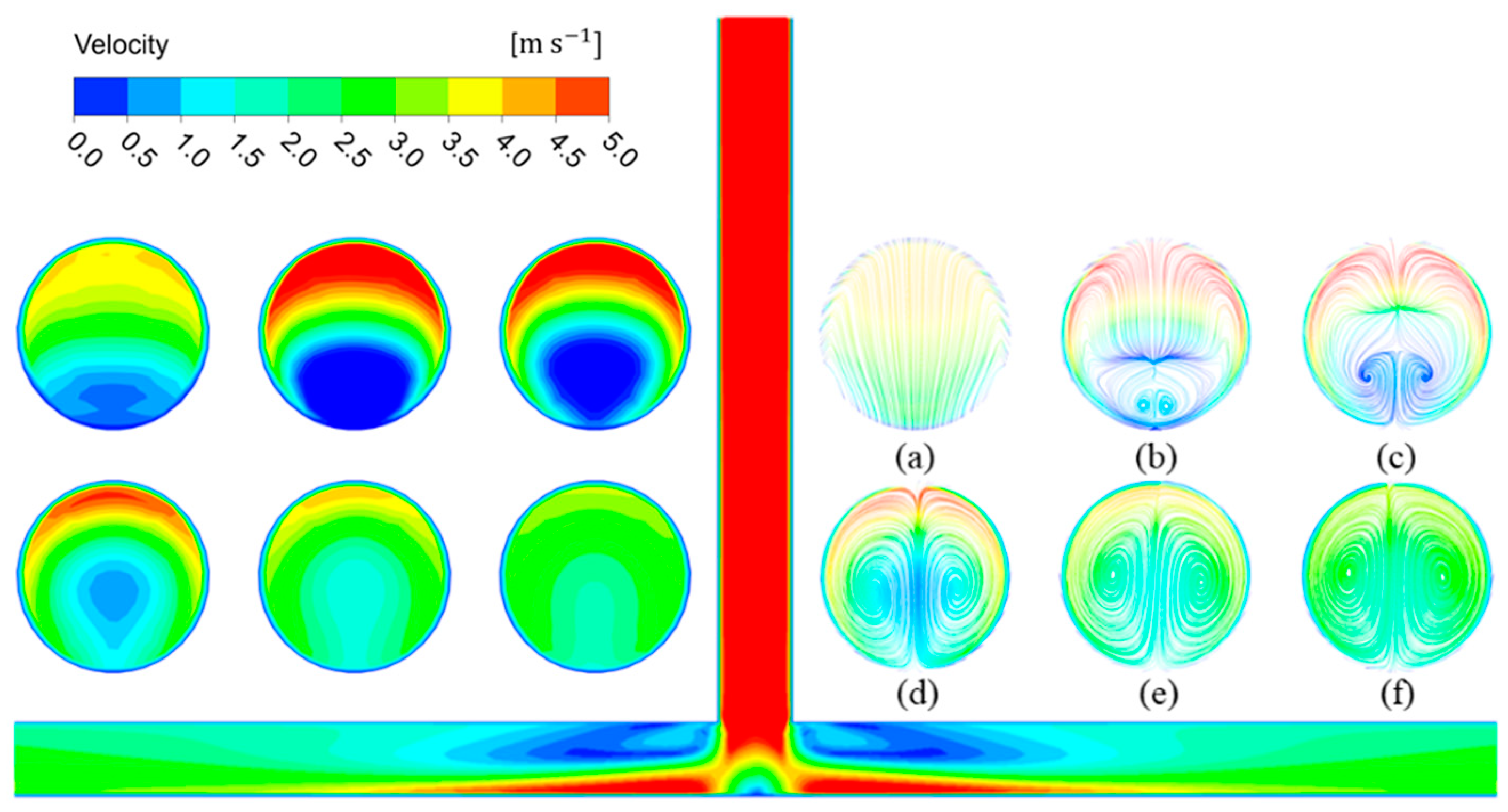
4.1. Distribution of Cross-Sectional Velocity
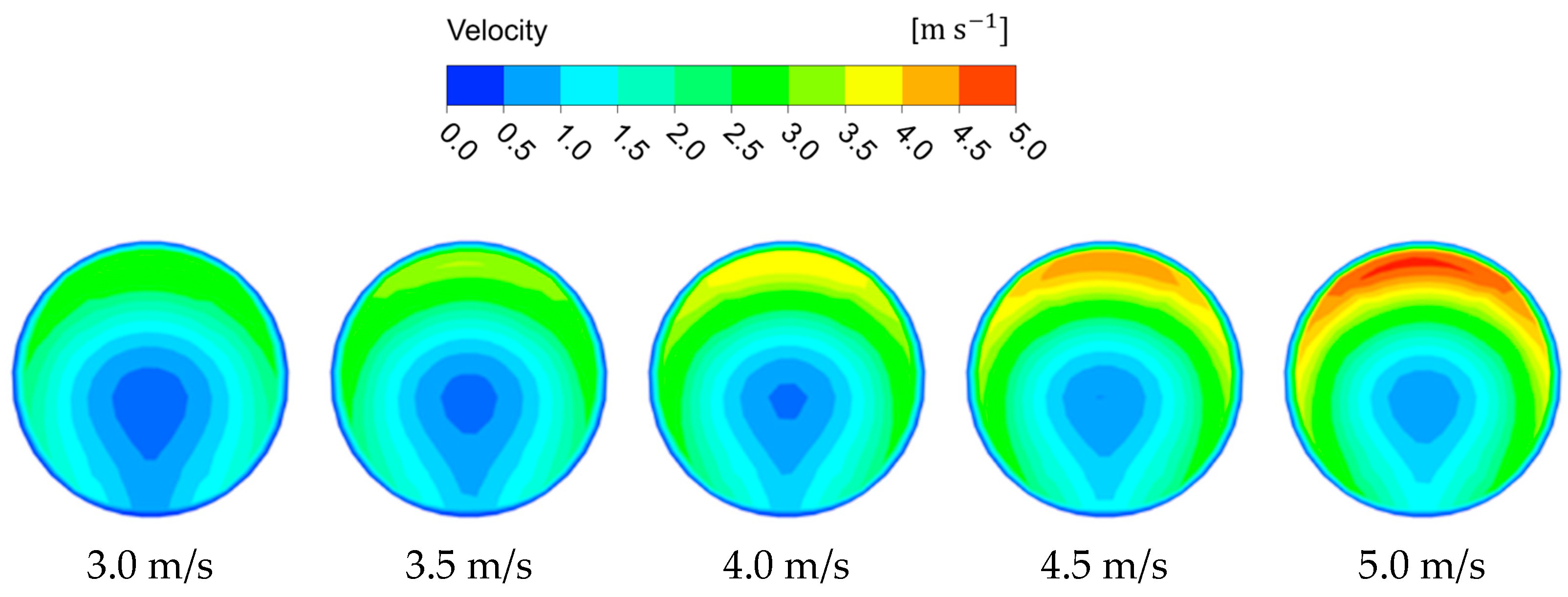
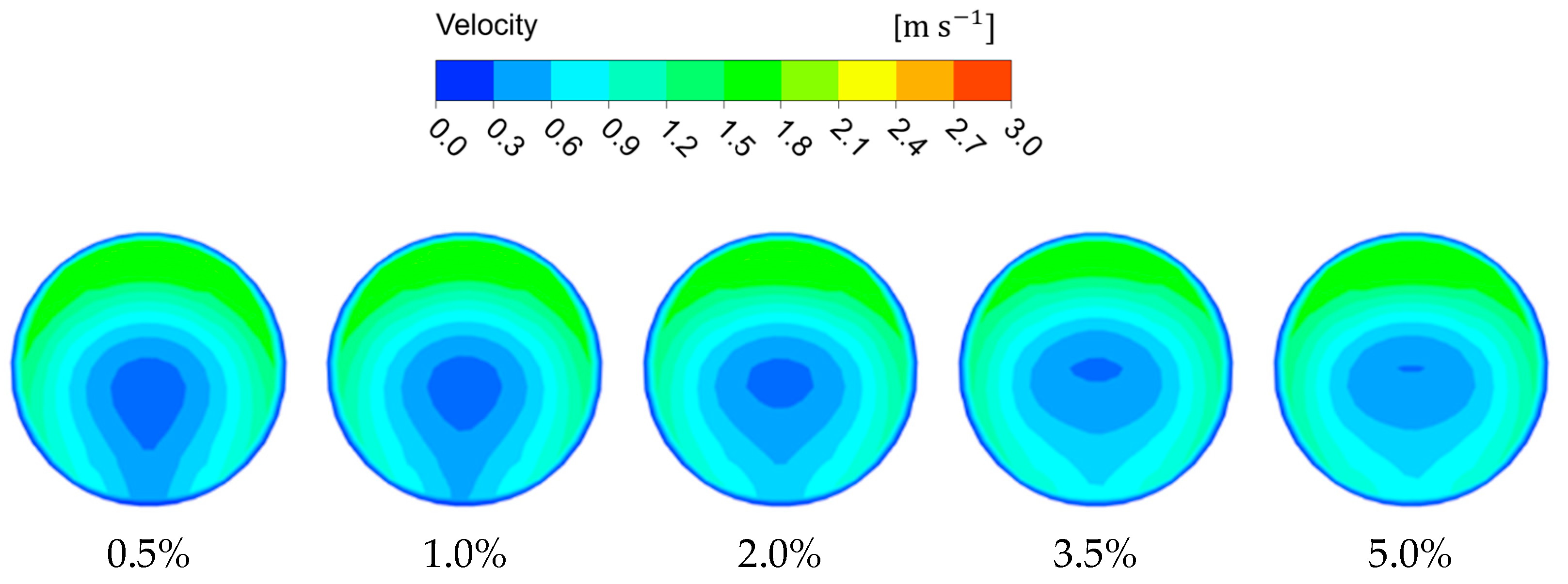
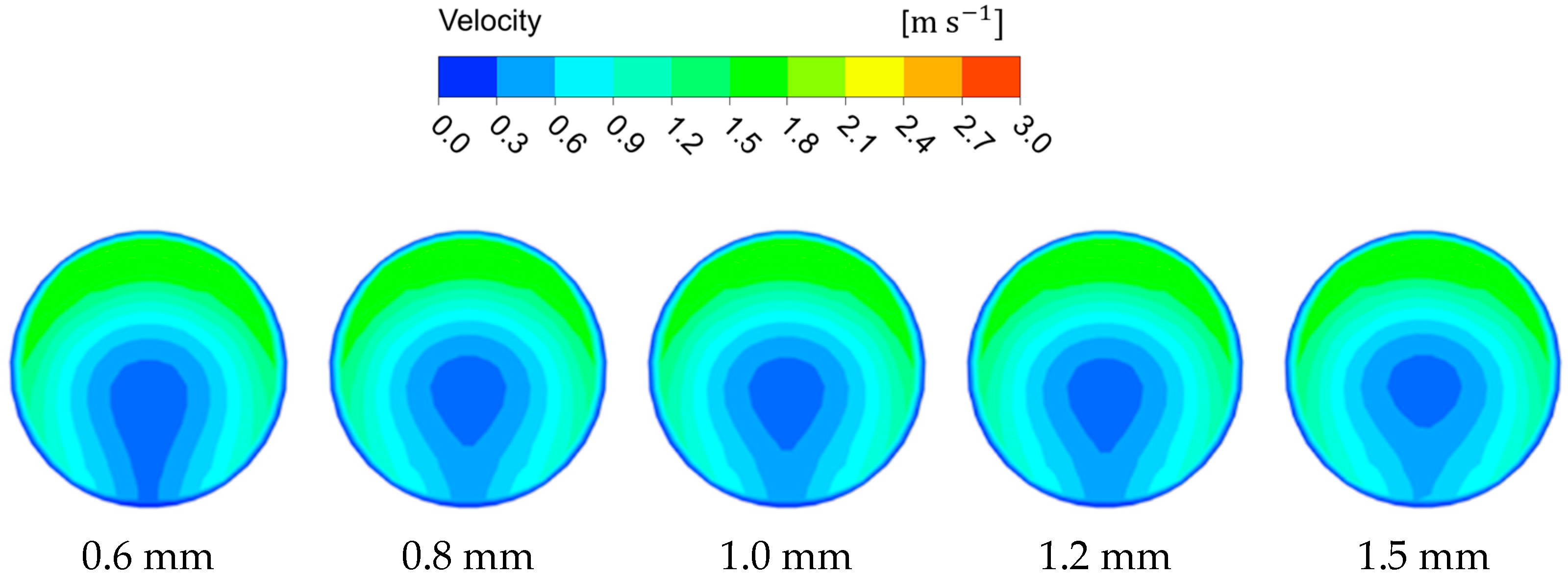
4.1.1. Cross-Sectional Velocity Profiles under Clean Water Conditions
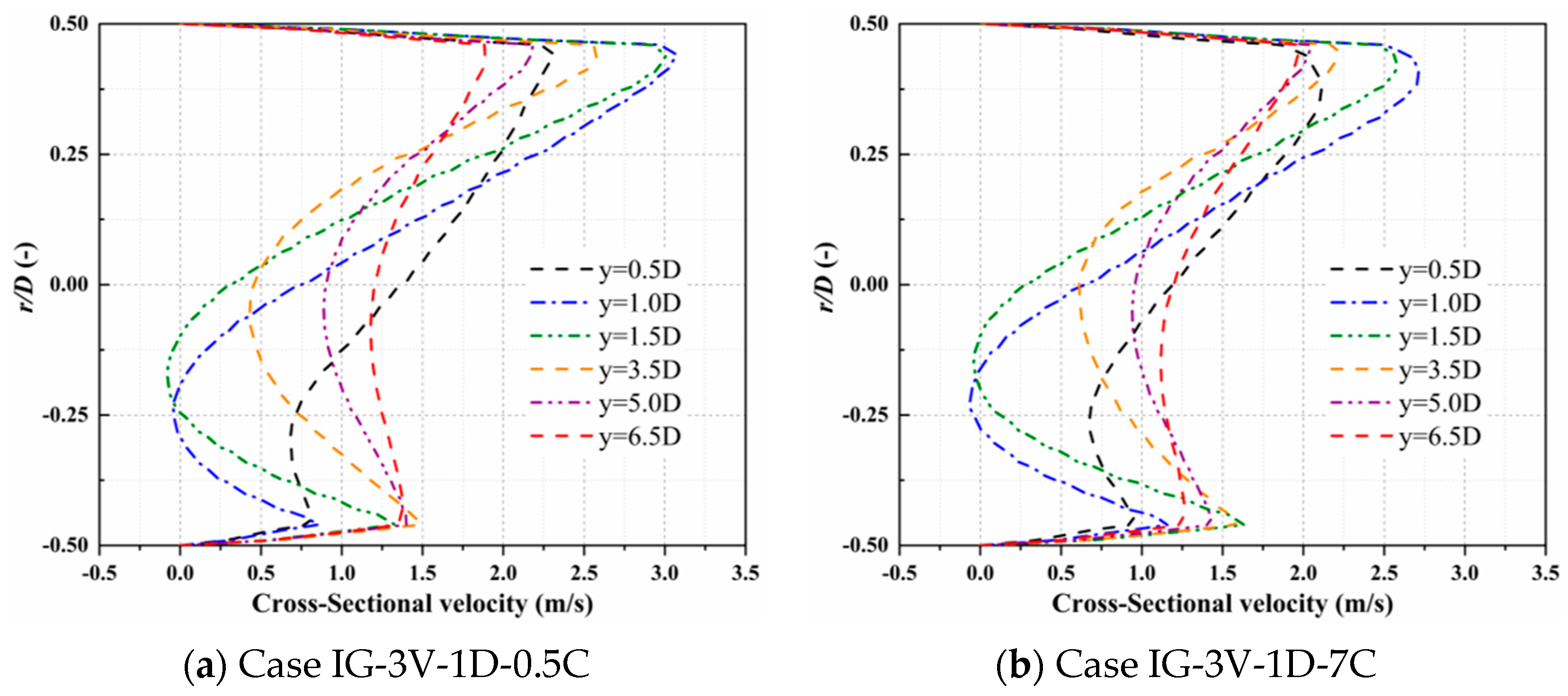
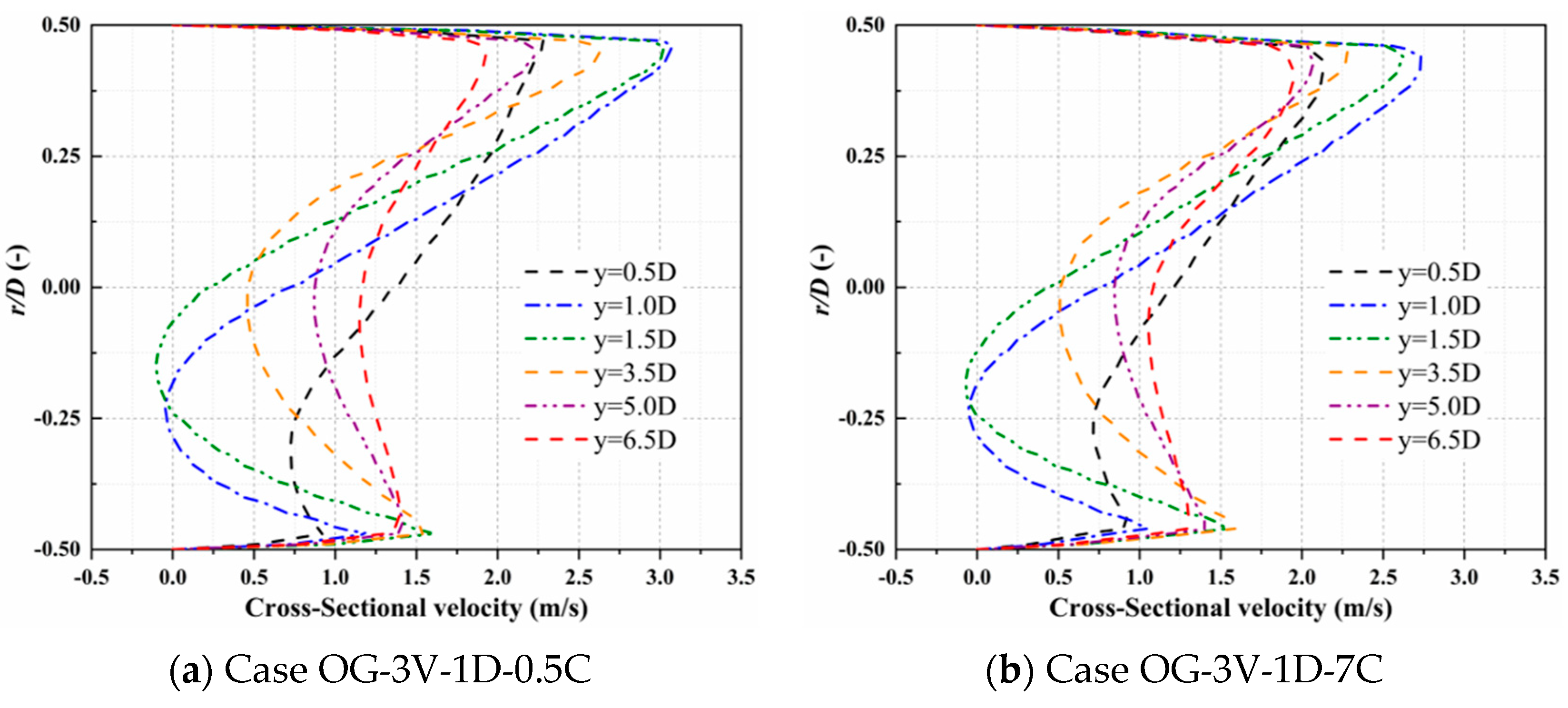
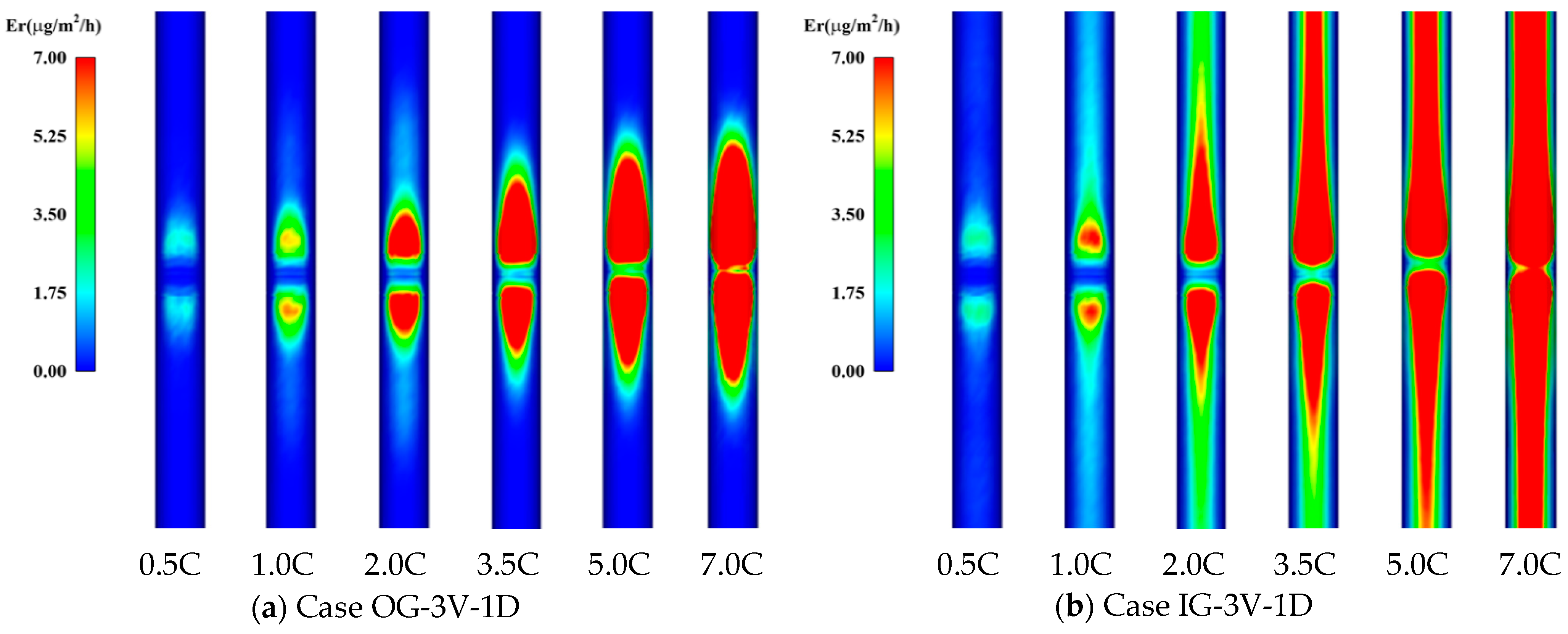
4.1.2. Cross-Sectional Velocity Profiles under Case IG-3V-1D and Case OG-3V-1D
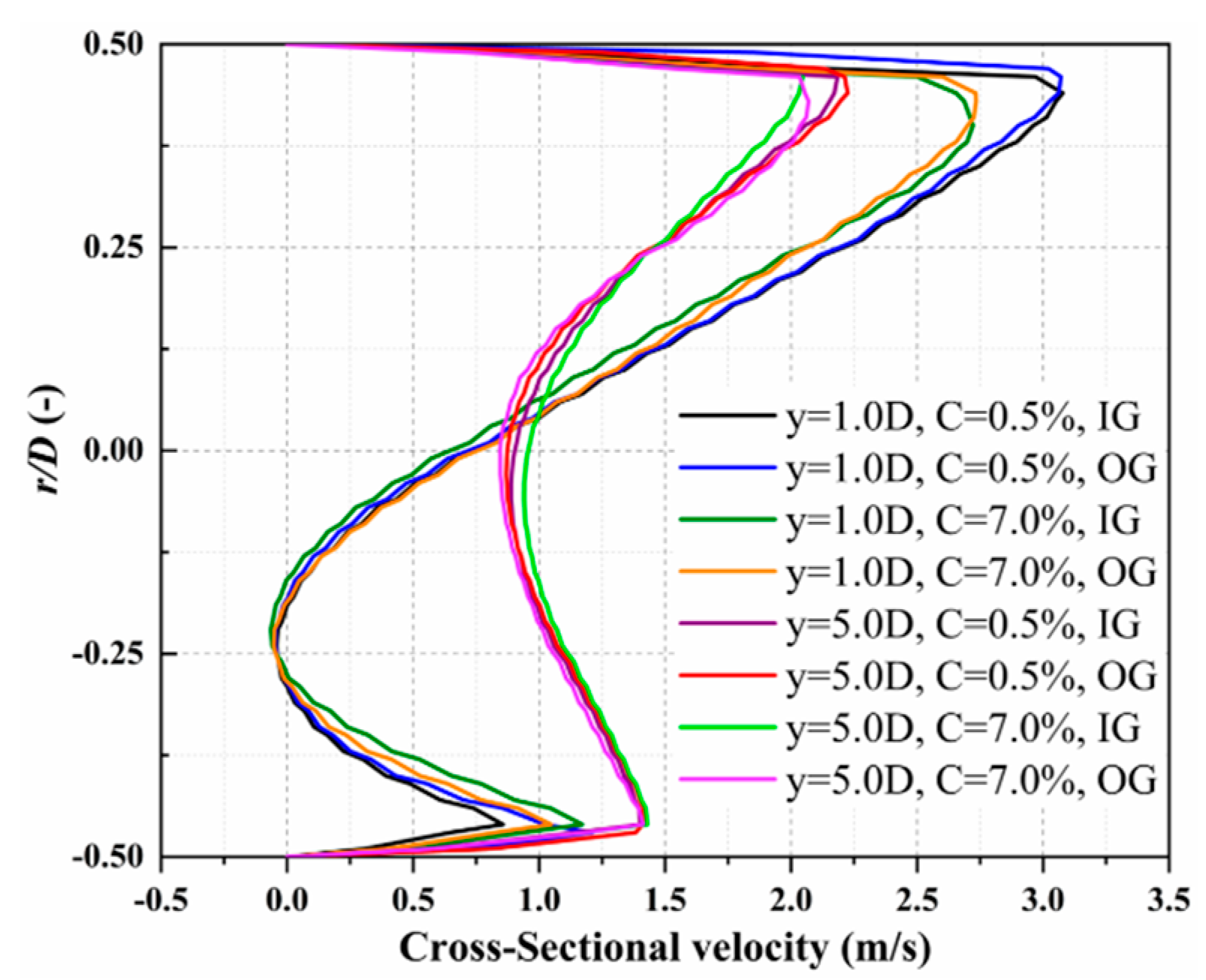
4.1.3. Cross-Sectional Velocity Profiles under Case 0.5C-1D and Case 3V-0.5C
4.2. Analysis of Tee Pipe Erosion
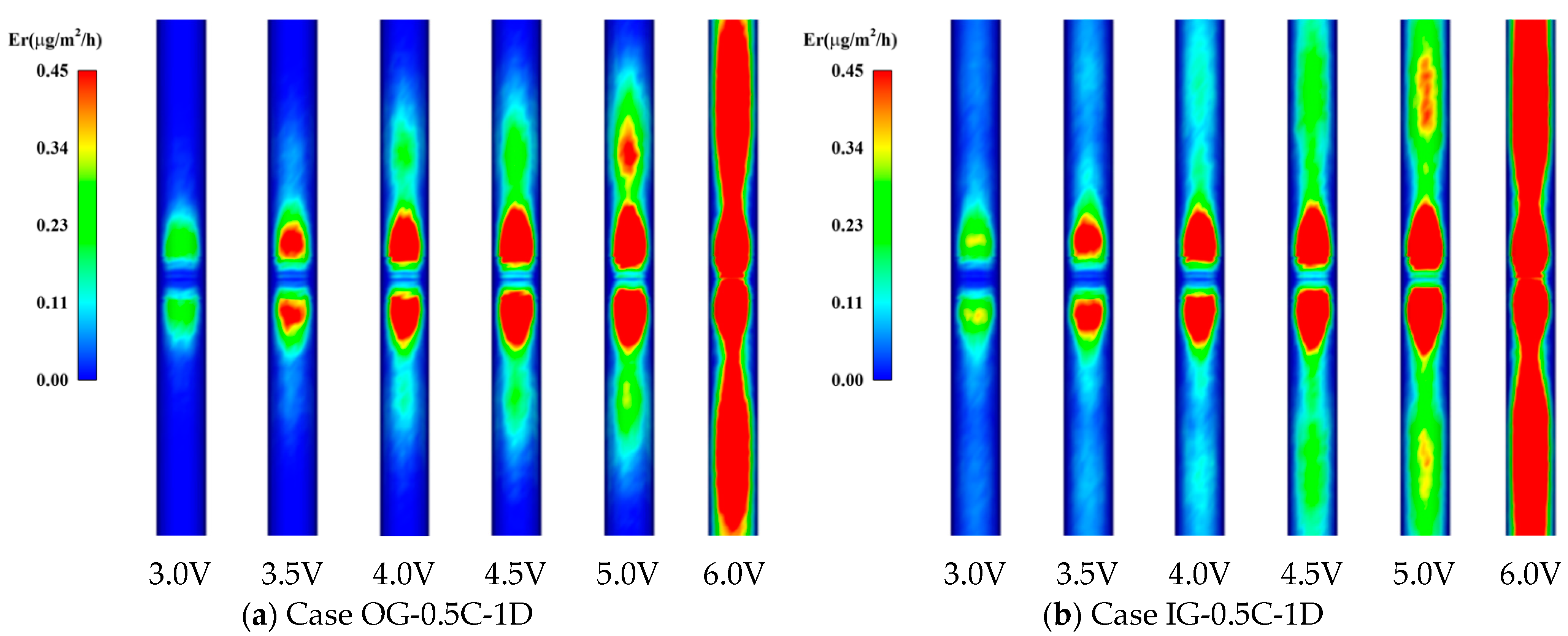
4.2.1. Effect of Inlet Velocity
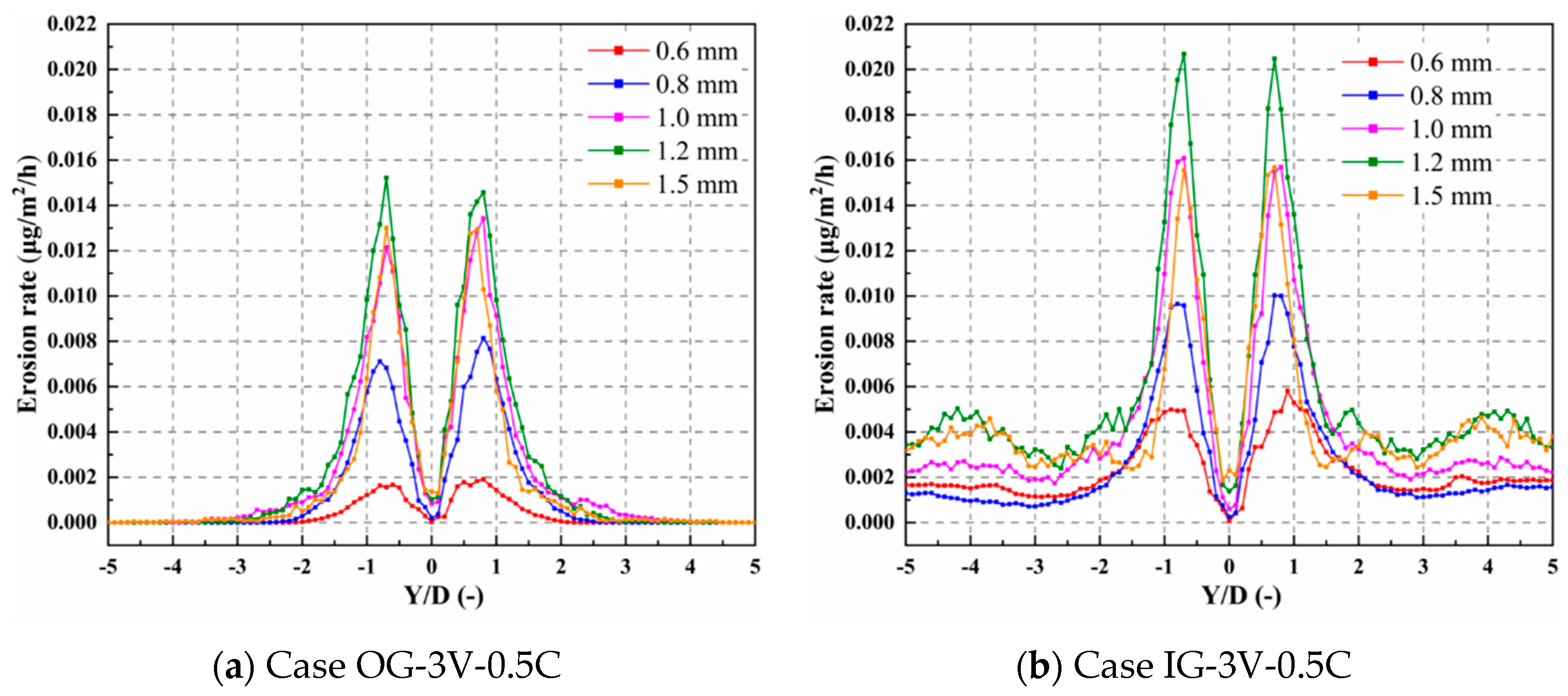
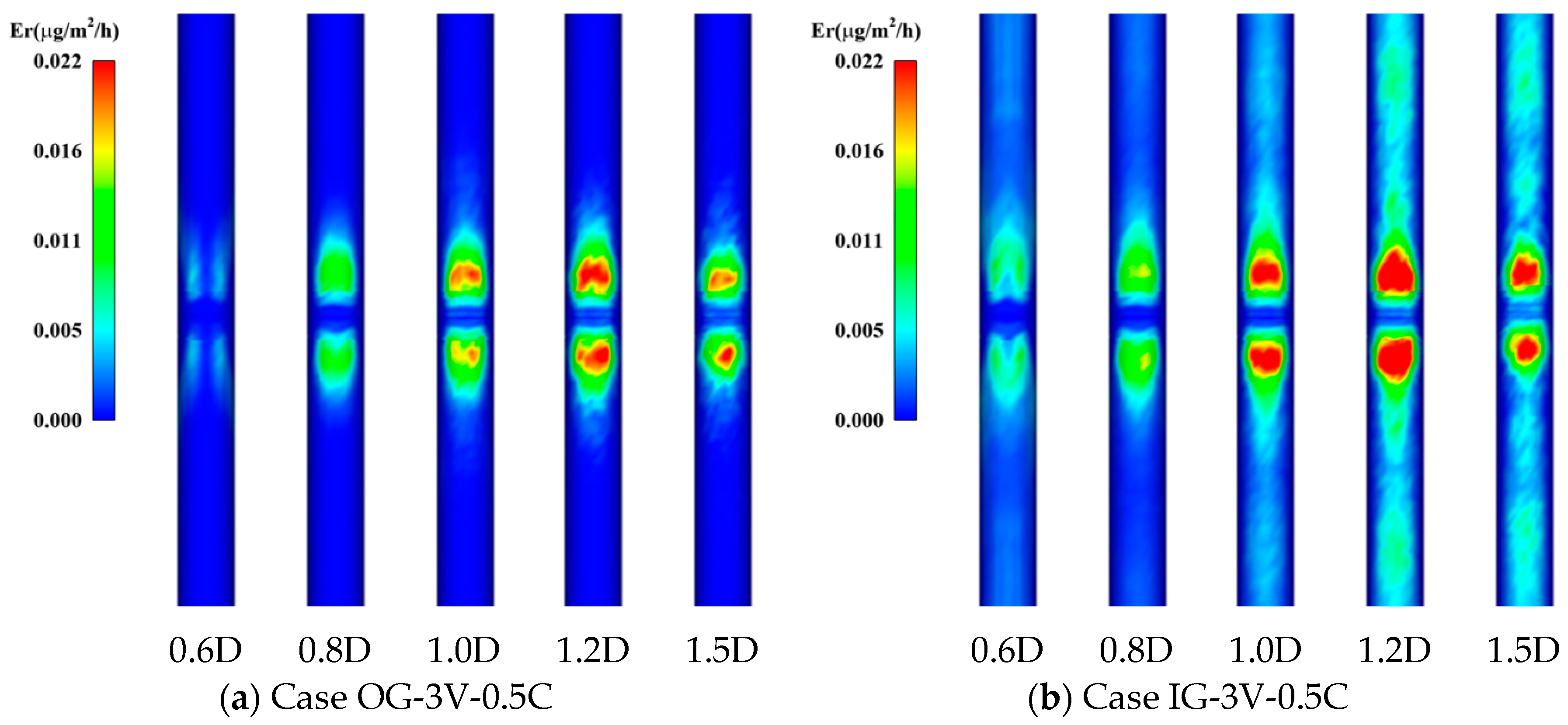
4.2.2. Effect of Particle Diameter
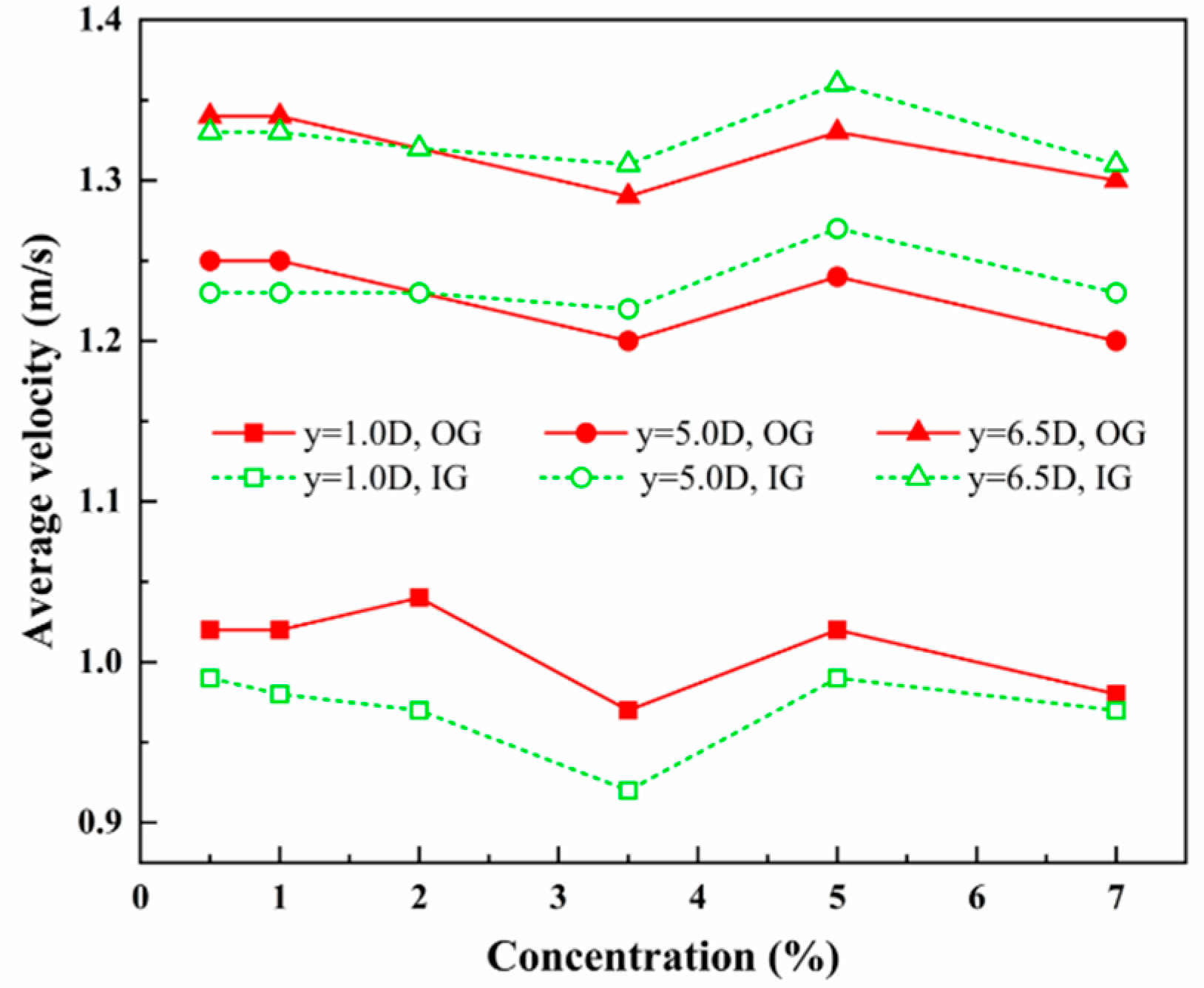
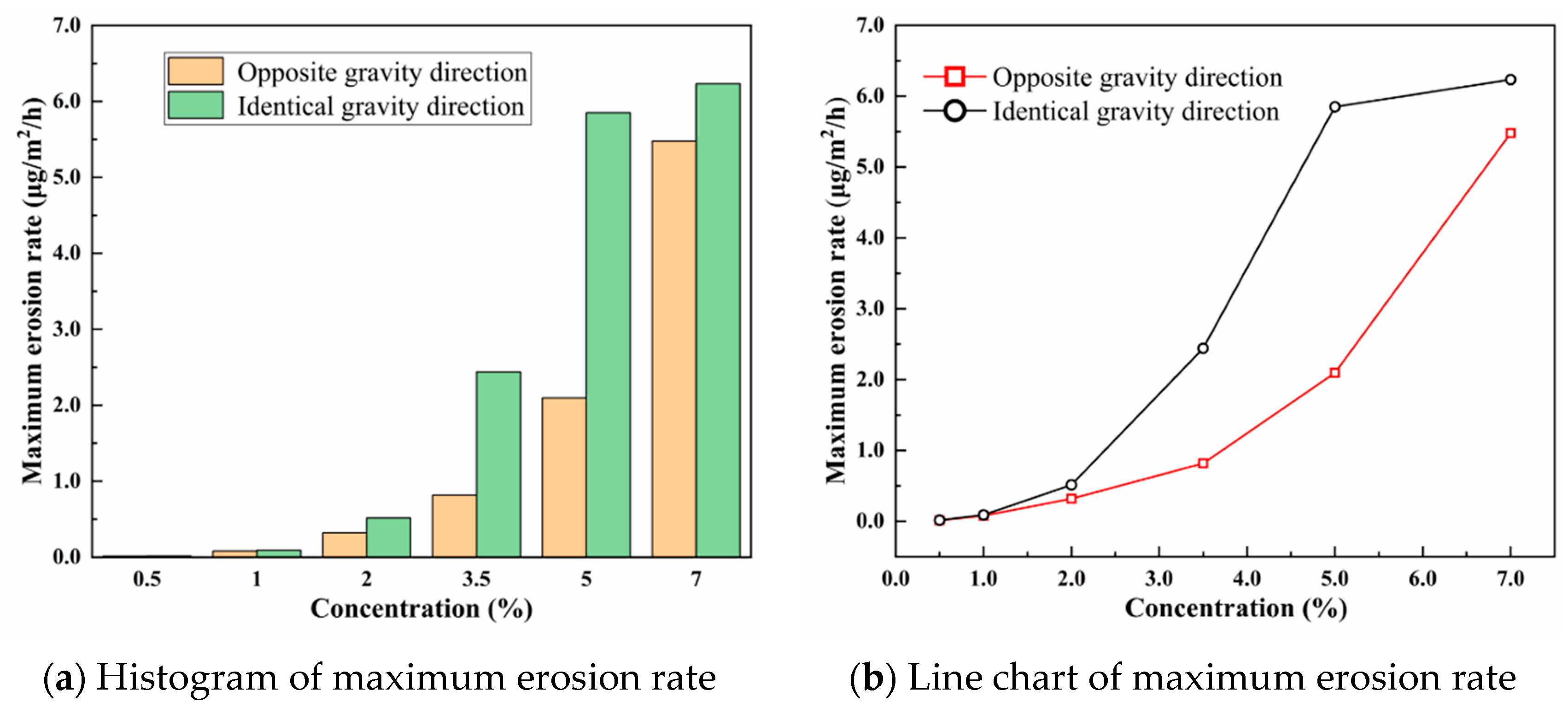
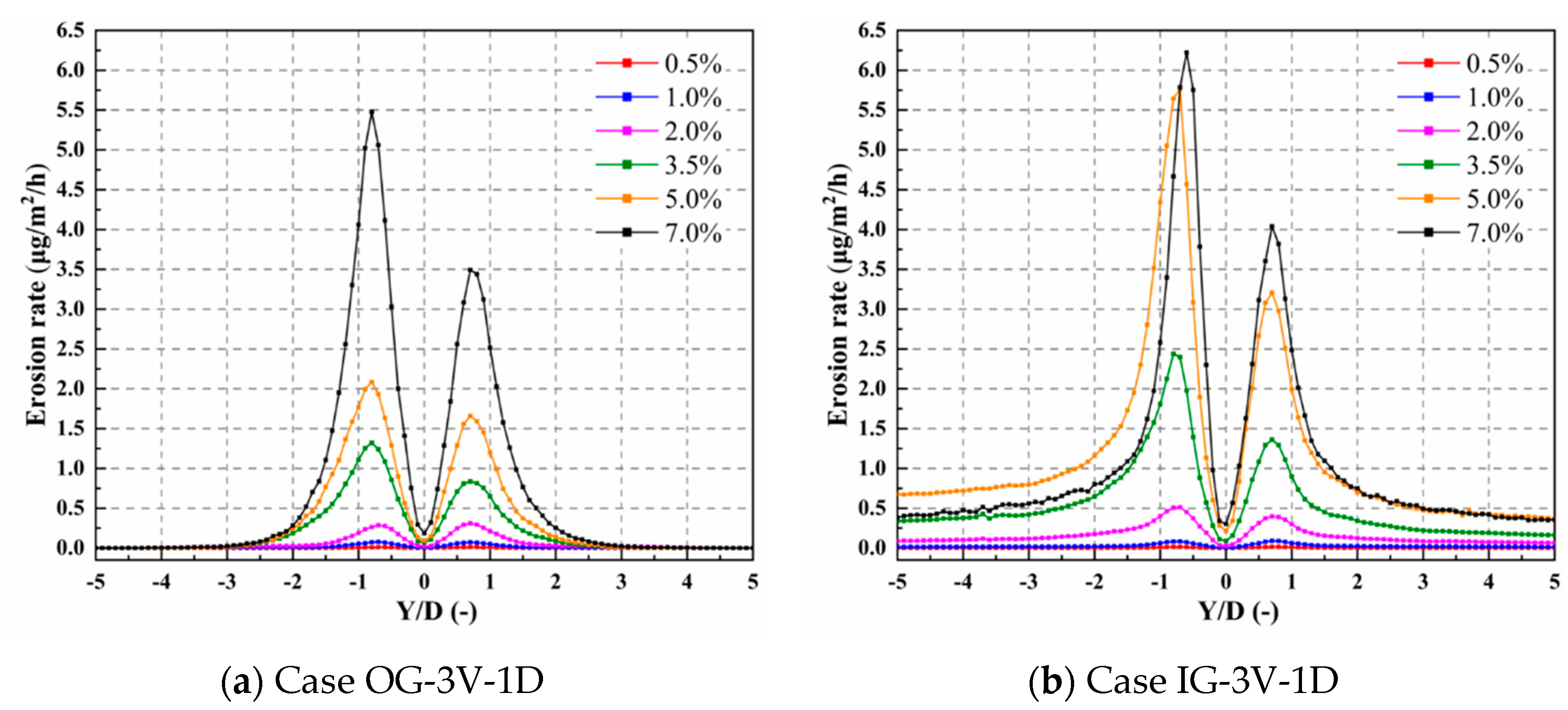
4.2.3. Effect of Particle Volume Concentration
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Finnie, I. Erosion of surfaces by solid particles. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Finnie, I. The Fundamental Mechanisms of the Erosive Wear of Ductile Metals by Solid Particles; Lawrence Berkeley National Laboratory: Berkeley, CA, USA, 1978. [Google Scholar]
- Bitter, J.G.A. A study of erosion phenomena part I. Wear 1963, 6, 5–21. [Google Scholar] [CrossRef]
- Bitter, J.G.A. A study of erosion phenomena: Part II. Wear 1963, 6, 169–190. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Oka, Y.I.; Okamura, K.; Yoshida, T. Practical estimation of erosion damage caused by solid particle impact: Part 1: Effects of impact parameters on a predictive equation. Wear 2005, 259, 95–101. [Google Scholar] [CrossRef]
- Oka, Y.I.; Yoshida, T. Practical estimation of erosion damage caused by solid particle impact: Part 2: Mechanical properties of materials directly associated with erosion damage. Wear 2005, 259, 102–109. [Google Scholar] [CrossRef]
- Chen, X.; McLaury, B.S.; Shirazi, S.A. Effects of applying a stochastic rebound model in erosion prediction of elbow and plugged tee. Fluids Eng. Div. Summer Meet. 2002, 36169, 247–254. [Google Scholar]
- Edwards, J.K.; McLaury, B.S.; Shirazi, S.A. Modeling solid particle erosion in elbows and plugged tees. J. Energy Resour. Technol. 2001, 123, 277–284. [Google Scholar] [CrossRef]
- Wang, K.; Li, X.; Wang, Y.; He, R. Numerical investigation of the erosion behavior in elbows of petroleum pipelines. Powder Technol. 2017, 314, 490–499. [Google Scholar] [CrossRef]
- Huang, Y.; Zhu, L.; Liao, H.; Zou, D.; Wang, J. Erosion of plugged tees in exhaust pipes through variously-sized cuttings. Appl. Math. Model. 2016, 40, 8708–8721. [Google Scholar] [CrossRef]
- Zamani, M.; Seddighi, S.; Nazif, H.R. Erosion of natural gas elbows due to rotating particles in turbulent gas-solid flow. J. Nat. Gas Sci. Eng. 2017, 40, 91–113. [Google Scholar] [CrossRef]
- Chen, X.; McLaury, B.S.; Shirazi, S.A. Numerical and experimental investigation of the relative erosion severity between plugged tees and elbows in dilute gas/solid two-phase flow. Wear 2006, 261, 715–729. [Google Scholar] [CrossRef]
- Farokhipour, A.; Mansoori, Z.; Rasteh, A.; Rasoulian, M.A.; Saffar-Avval, M.; Ahmadi, G. Study of erosion prediction of turbulent gas-solid flow in plugged tees via CFD-DEM. Powder Technol. 2019, 352, 136–150. [Google Scholar] [CrossRef]
- Liu, C.; Mao, J.; Yu, M. Analysis of Gas-Solid Two-Phase Flow and Erosion in a 90 Curved Duct. J.-Xi’an Jiaotong Univ. 1999, 33, 53–57. [Google Scholar]
- Yao, J.L.; Di, Q.F.; Wang, W.C.; Hu, Y.B. Abrasion of exhaust pipe by the high-speed gas with the cutting in air drilling. Drill. Prod. Technol. 2009, 32, 13–15. [Google Scholar]
- Duarte, C.A.R.; de Souza, F.J.; dos Santos, V.F. Numerical investigation of mass loading effects on elbow erosion. Powder Technol. 2015, 283, 593–606. [Google Scholar] [CrossRef]
- Zhang, J.X.; Bai, Y.Q.; Kang, J.; Wu, X. Failure analysis and erosion prediction of tee junction in fracturing operation. J. Loss Prev. Process Ind. 2017, 46, 94–107. [Google Scholar] [CrossRef]
- Charron, Y.; Whalley, P.B. Gas-liquid annular flow at a vertical tee junction-Part I. Flow separation. Int. J. Multiph. Flow 1995, 21, 569–589. [Google Scholar] [CrossRef]
- Brown, G.J. Erosion prediction in slurry pipeline tee-junctions. Appl. Math. Model. 2002, 26, 155–170. [Google Scholar] [CrossRef]
- Zhang, J.; Fan, J.; Wang, T.; Wang, X. Experimental study and numerical simulation of erosion wear on high-pressure pipe and tee. In ICPTT 2013: Trenchless Technology; American Society of Civil Engineers: Reston, VA, USA, 2013; pp. 927–934. [Google Scholar]
- Costa, N.P.; Maia, R.; Proenca, M.F.; Proença, M. Edge effects on the flow characteristics in a 90 deg tee junction. J. Fluids Eng. 2006, 128, 1204–1217. [Google Scholar] [CrossRef]
- Jin, H.U.; Zhang, H.; Zhang, J.; Niu, S.; Cai, W. Gas-solid Erosion Wear Characteristics of Two-phase Flow Tee Pipe: Erosion Wear of Two-phase Flow Tee Pipe. Mechanics 2021, 27, 193–200. [Google Scholar]
- Zhang, J.; Zhu, P.; Zhang, H.; Lv, L. The erosion wear mechanism of liquid-solid two-phase high-pressure manifold tee pipes. Rev. Int. Métodos Numéricos Cálculo Diseño Ing. 2021, 37, 1–14. [Google Scholar] [CrossRef]
- Alajbegović, A.; Assad, A.; Bonetto, F.; Lahey, R.T., Jr. Phase distribution and turbulence structure for solid/fluid upflow in a pipe. Int. J. Multiph. Flow 1994, 20, 453–479. [Google Scholar] [CrossRef]
- Zeng, L.; Zhang, G.A.; Guo, X.P. Erosion-corrosion at different locations of X65 carbon steel elbow. Corros. Sci. 2014, 85, 318–330. [Google Scholar] [CrossRef]
- Çengel, Y.A.; Cimbala, J.M. Fluid Mechanics: Fundamentals and Applications, 4th ed.; Mc Graw Hill: Noida, India, 2018. [Google Scholar]

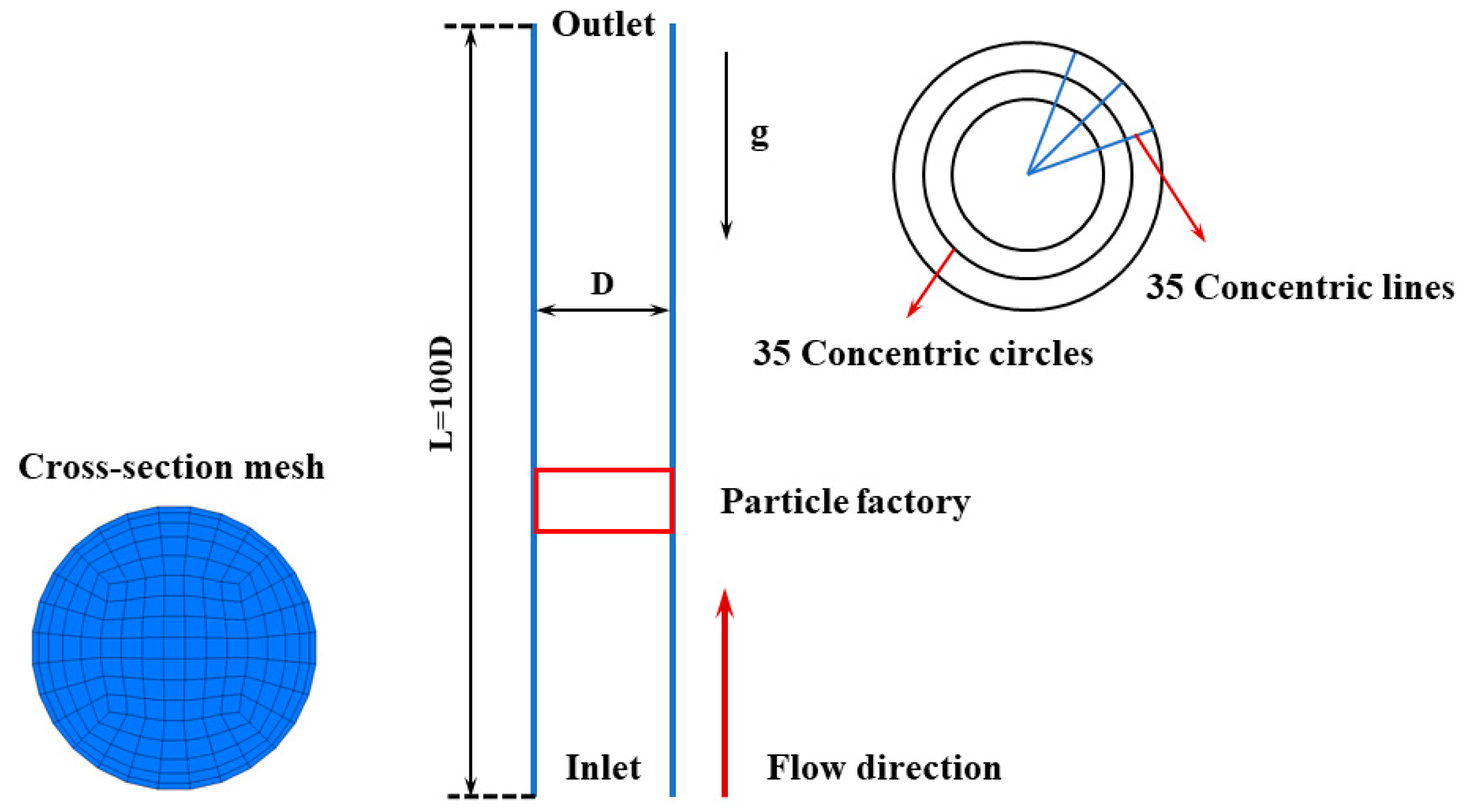
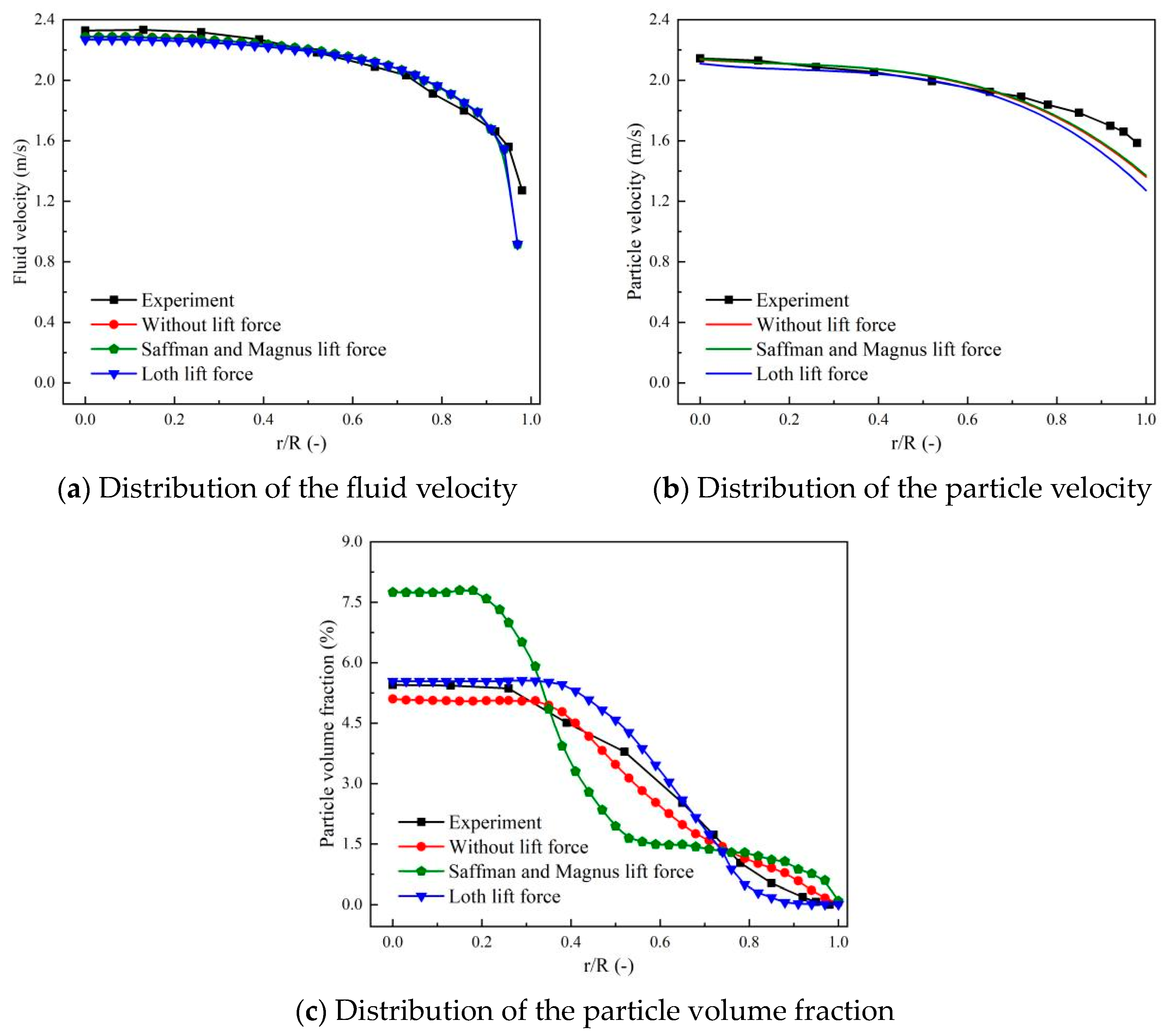
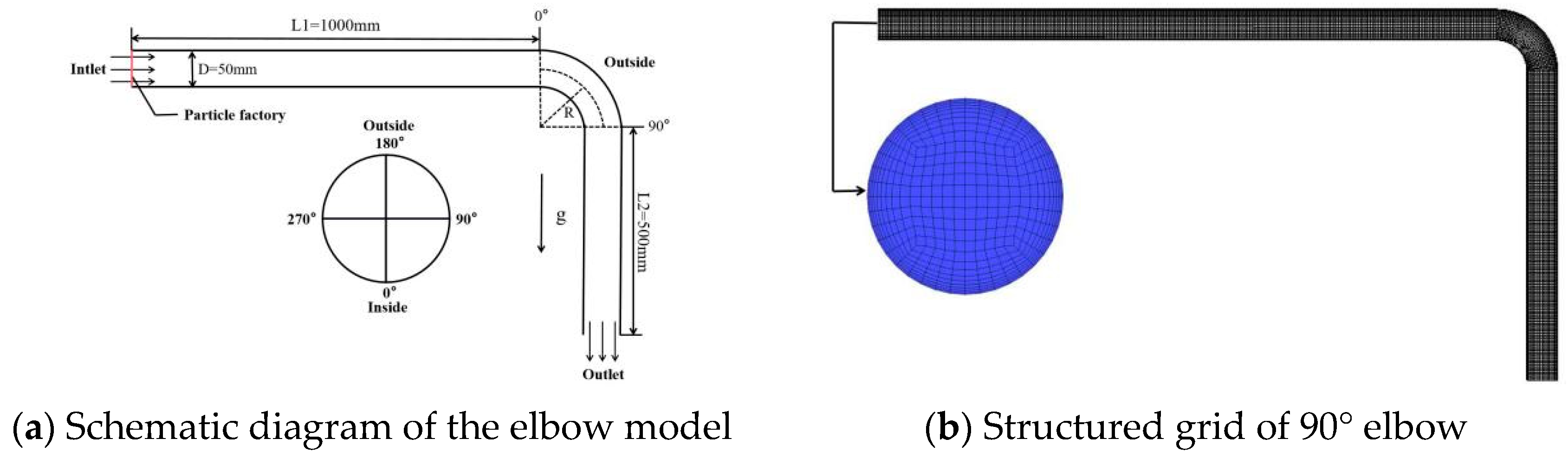
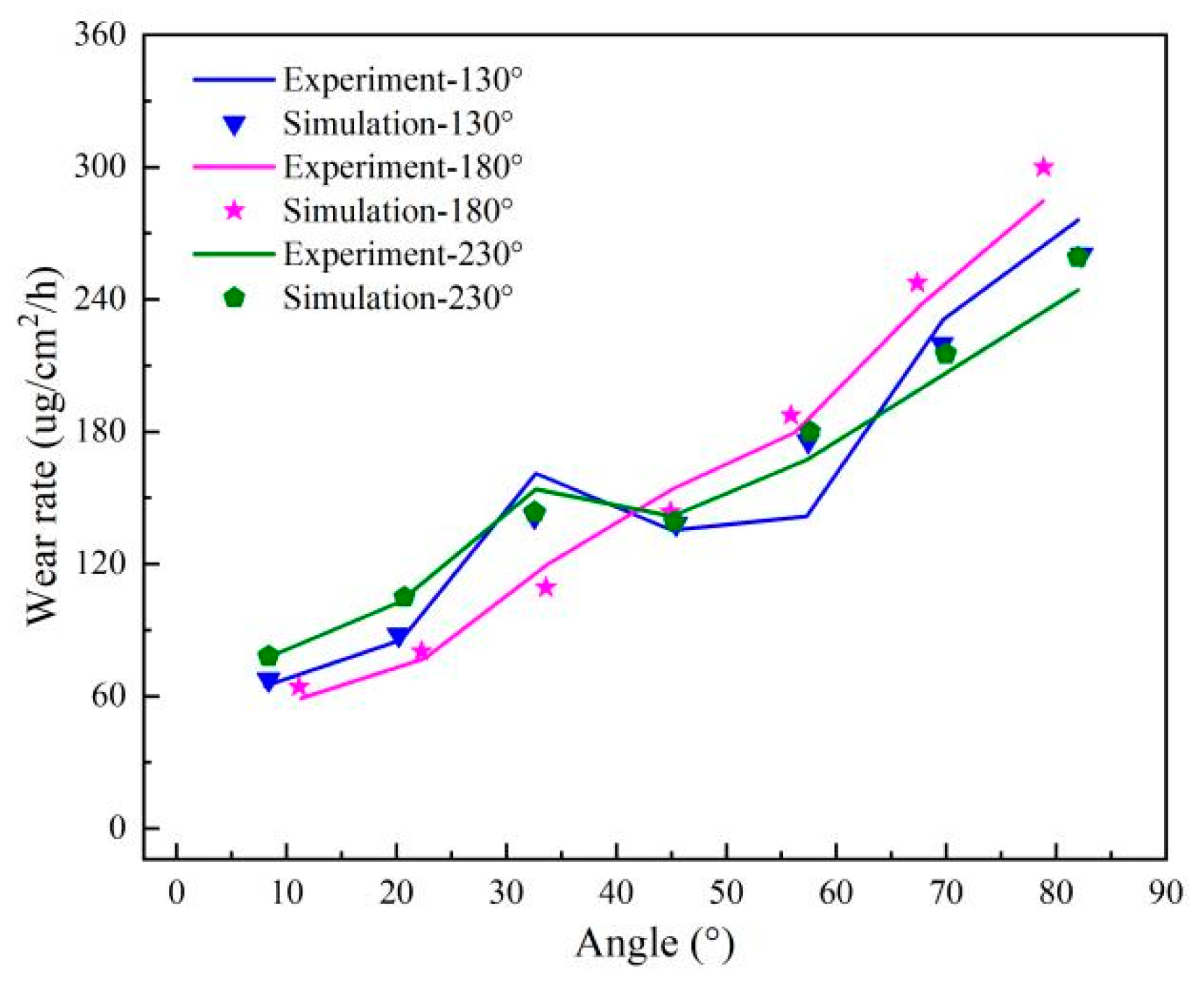







| Physical Quantity | Unit | Value | |
|---|---|---|---|
| Fluid | Density | kg/m3 | 998.2 |
| Inlet velocity | m/s | 1.888 | |
| Outlet pressure | atm | 1 | |
| Particle | Density | kg/m3 | 2450 |
| Particle diameter | mm | 2.36 | |
| Volume concentration | % | 2.33 | |
| Inlet velocity | m/s | 1.888 | |
| Poisson’s ratio | 0.3 | ||
| Young’s modulus | GPa | 10 | |
| Particle–particle restitution coefficient | 0.85 | ||
| Particle–particle static friction coefficient | 0.1 | ||
| Particle–particle rolling friction coefficient | 0.01 | ||
| Wall | Density | kg/m3 | 2150 |
| Poisson’s ratio | 0.3 | ||
| Young’s modulus | GPa | 260 | |
| Particle–wall restitution coefficient | 0.85 | ||
| Particle–wall static friction coefficient | 0.2 | ||
| Particle–wall rolling friction coefficient | 0.01 |
| Physical Quantity | Unit | Value | |
|---|---|---|---|
| Fluid | Density | kg/m3 | 998.2 |
| Inlet velocity | m/s | 4 | |
| Outflow | |||
| Particle | Density | kg/m3 | 2650 |
| Particle diameter | mm | 0.5 | |
| Mass flow rate | kg/s | 0.235 | |
| Inlet velocity | m/s | 4 | |
| Poisson’s ratio | 0.23 | ||
| Young’s modulus | GPa | 59 | |
| Particle–particle restitution coefficient | 0.9 | ||
| Wall | Density | kg/m3 | 8200 |
| Poisson’s ratio | 0.3 | ||
| Young’s modulus | GPa | 207 | |
| Particle–wall restitution coefficient | 0.8 | ||
| Particle–wall static friction coefficient | 0.2 |
| Scheme | Nodes | Elements | Fluid Velocity (m/s) | RMS Velocity (m/s) | Orthogonal Quality | Skewness |
|---|---|---|---|---|---|---|
| Coarse mesh | 73,264 | 77,782 | 1.59 | 0.1499 | 0.35 | 0.66 |
| Medium mesh | 106,814 | 113,068 | 1.66 | 0.1807 | 0.51 | 0.48 |
| Fine mesh | 134,276 | 141,886 | 1.65 | 0.1943 | 0.63 | 0.42 |
| Physical Quantity | Unit | Value | |
|---|---|---|---|
| Fluid | Inlet velocity | m/s | 3 |
| Turbulence intensity | % | 5 | |
| Hydraulic diameter | mm | 30 | |
| Particle | Density | kg/m3 | 2650 |
| Particle diameter | mm | 1 | |
| Poisson’s ratio | 0.17 | ||
| Particle incident velocity | m/s | 3 | |
| Particle–particle restitution coefficient | 0.95 | ||
| Particle–particle static friction coefficient | 0.005 | ||
| Particle–particle rolling friction coefficient | 0.4 | ||
| Wall | Poisson’s ratio | 0.3 | |
| Young’s modulus | GPa | 200 | |
| Particle–wall restitution coefficient | 0.737 | ||
| Particle–wall static friction coefficient | 0.2 | ||
| Particle–wall rolling friction coefficient | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, S.; Peng, G.; Yu, D.; Chang, H.; Wang, X. Investigation on the Erosion Characteristics of Liquid–Solid Two-Phase Flow in Tee Pipes Based on CFD-DEM. J. Mar. Sci. Eng. 2023, 11, 2231. https://doi.org/10.3390/jmse11122231
Hong S, Peng G, Yu D, Chang H, Wang X. Investigation on the Erosion Characteristics of Liquid–Solid Two-Phase Flow in Tee Pipes Based on CFD-DEM. Journal of Marine Science and Engineering. 2023; 11(12):2231. https://doi.org/10.3390/jmse11122231
Chicago/Turabian StyleHong, Shiming, Guangjie Peng, Dehui Yu, Hao Chang, and Xikun Wang. 2023. "Investigation on the Erosion Characteristics of Liquid–Solid Two-Phase Flow in Tee Pipes Based on CFD-DEM" Journal of Marine Science and Engineering 11, no. 12: 2231. https://doi.org/10.3390/jmse11122231
APA StyleHong, S., Peng, G., Yu, D., Chang, H., & Wang, X. (2023). Investigation on the Erosion Characteristics of Liquid–Solid Two-Phase Flow in Tee Pipes Based on CFD-DEM. Journal of Marine Science and Engineering, 11(12), 2231. https://doi.org/10.3390/jmse11122231







