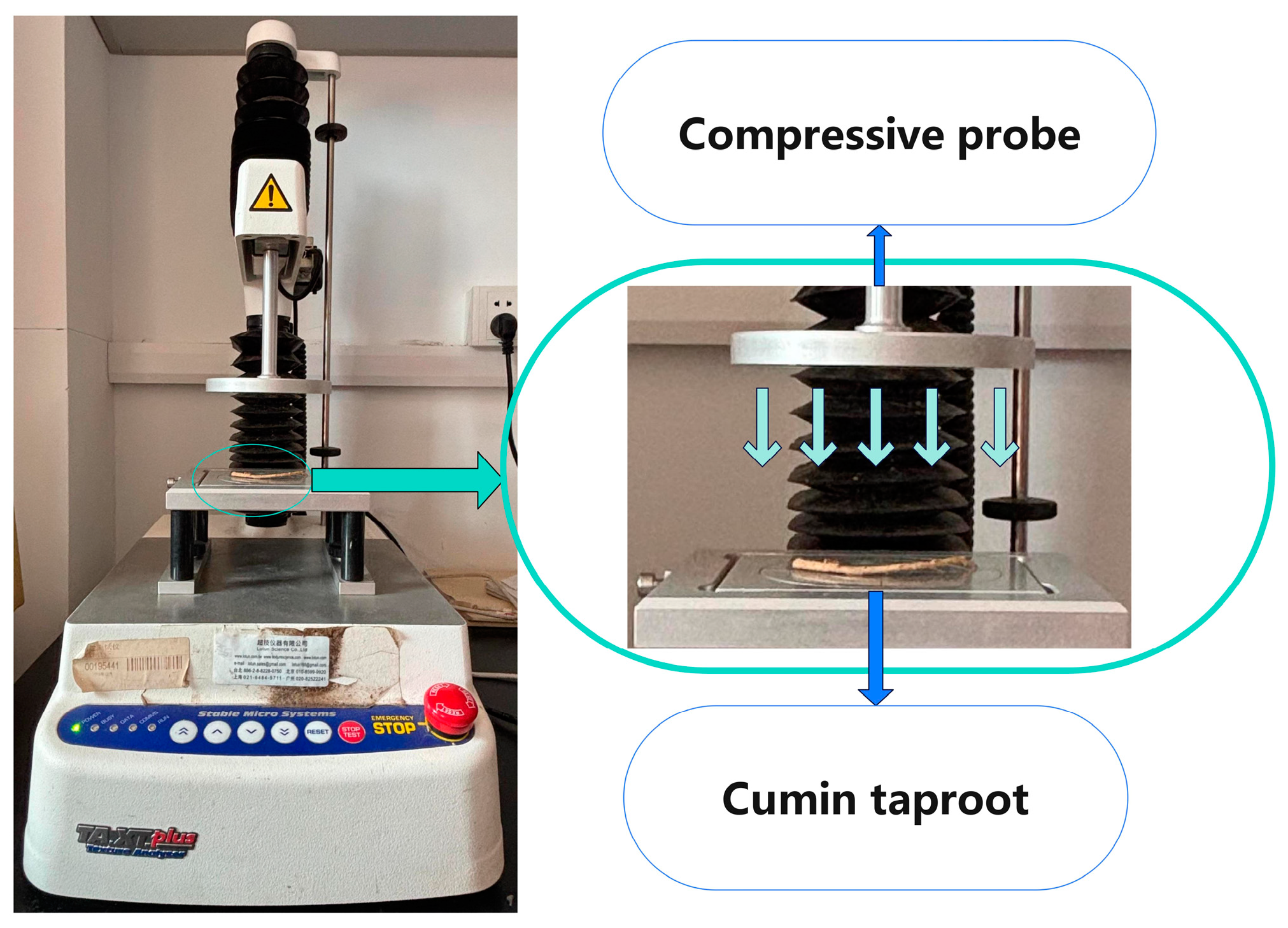
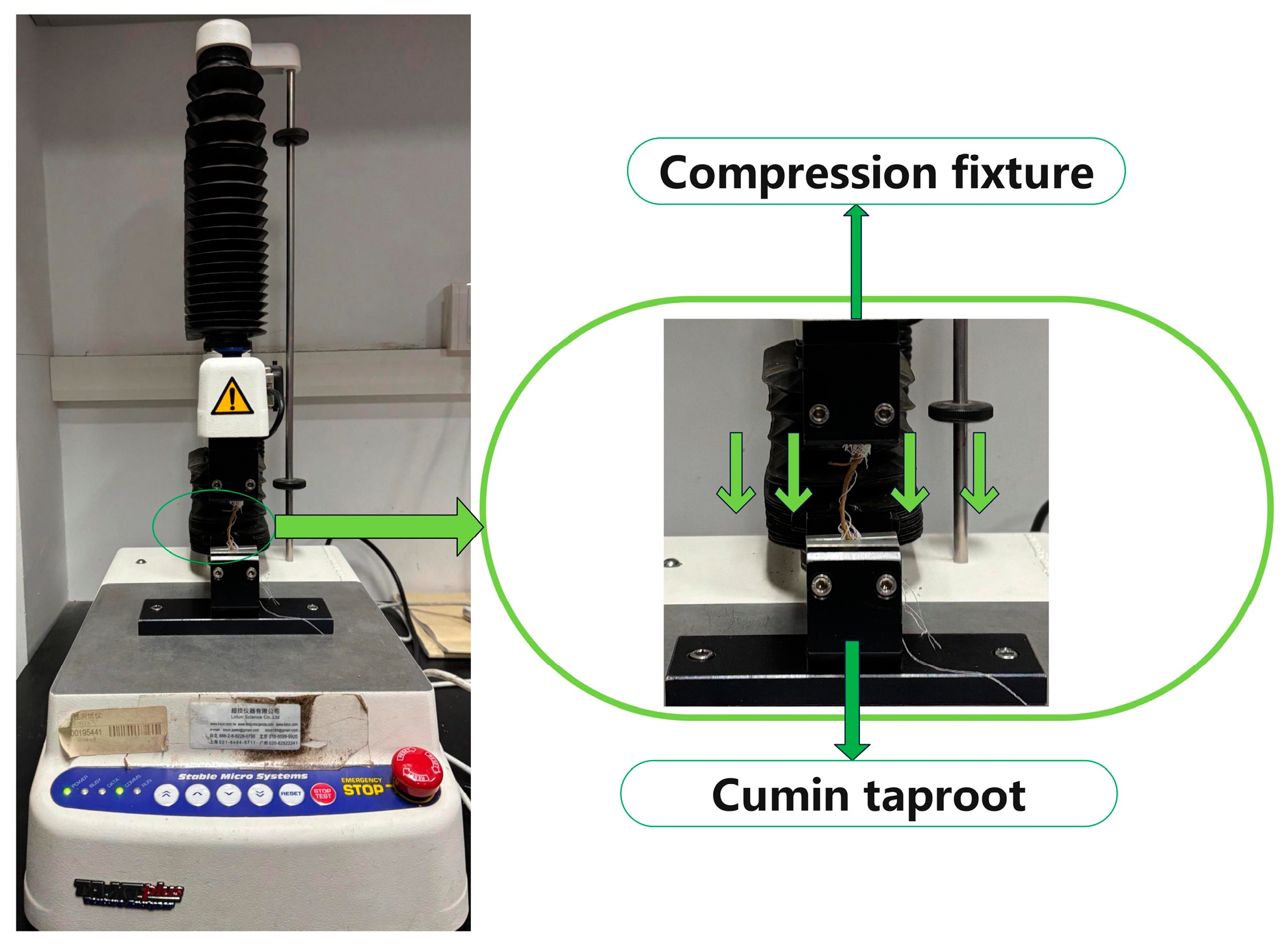
2.3. Discrete Element Method Analysis of the Taproot–Sandy Loam Soil Interaction Mechanism
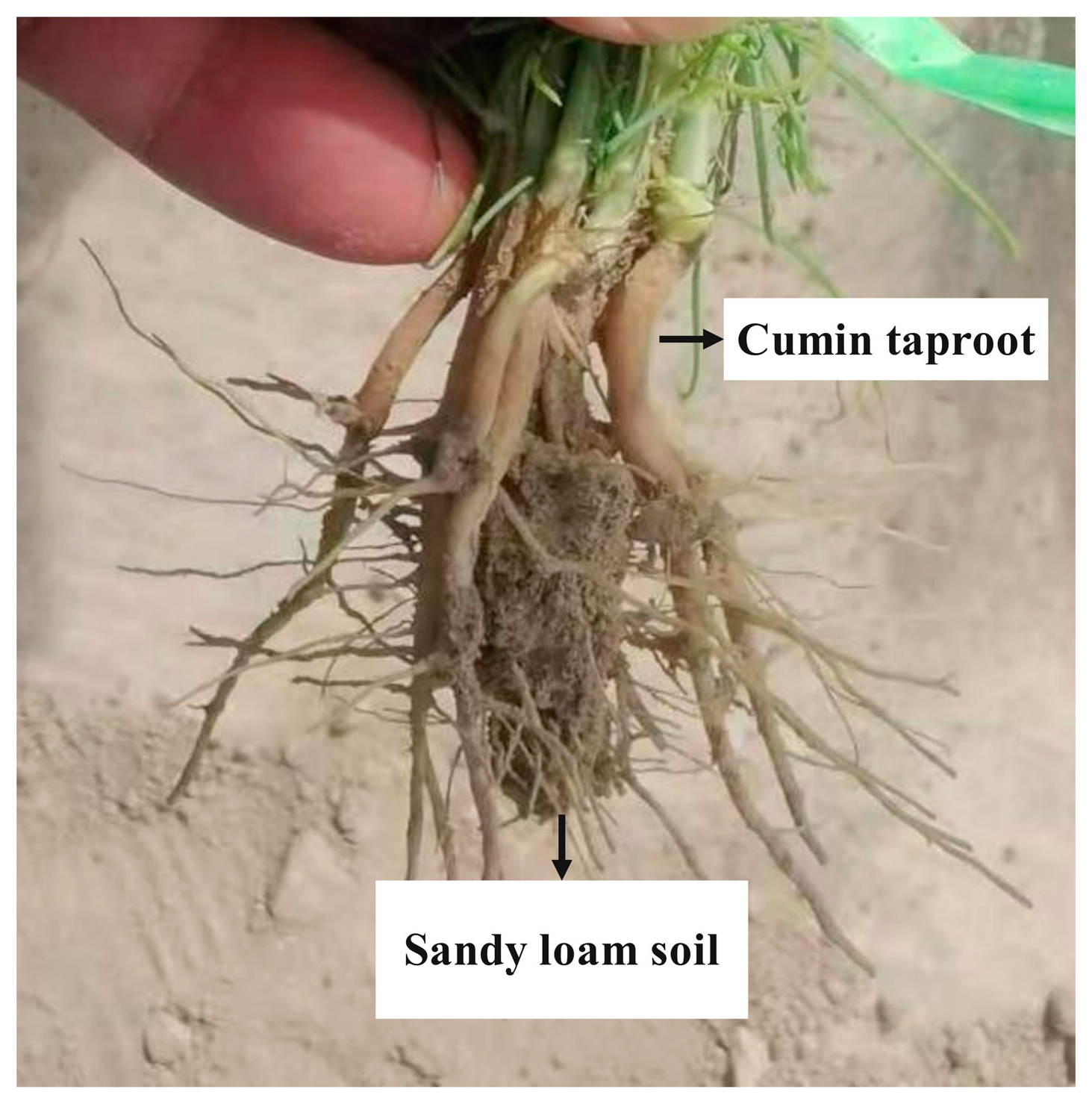
The root–soil complex of cumin is a versatile composite material structure, which is formed through the process of adsorption between cumin taproot and sandy loam soil particles, together with water and air. Due to the influence of the cumin taproot, the interparticle forces within the root–soil complex differ from those in bare soil. Specifically, the cohesive forces between soil particles and the adhesive forces between soil particles and the root are significantly enhanced within the complex, as illustrated in
Figure 3. Therefore, the adhesive forces need to be accorded careful consideration during the process of uprooting. The process entails overcoming the interparticle adhesive forces that end up destroying the cohesive bonds and bring about the changes in deformation and movement dynamics in the soil and the root.
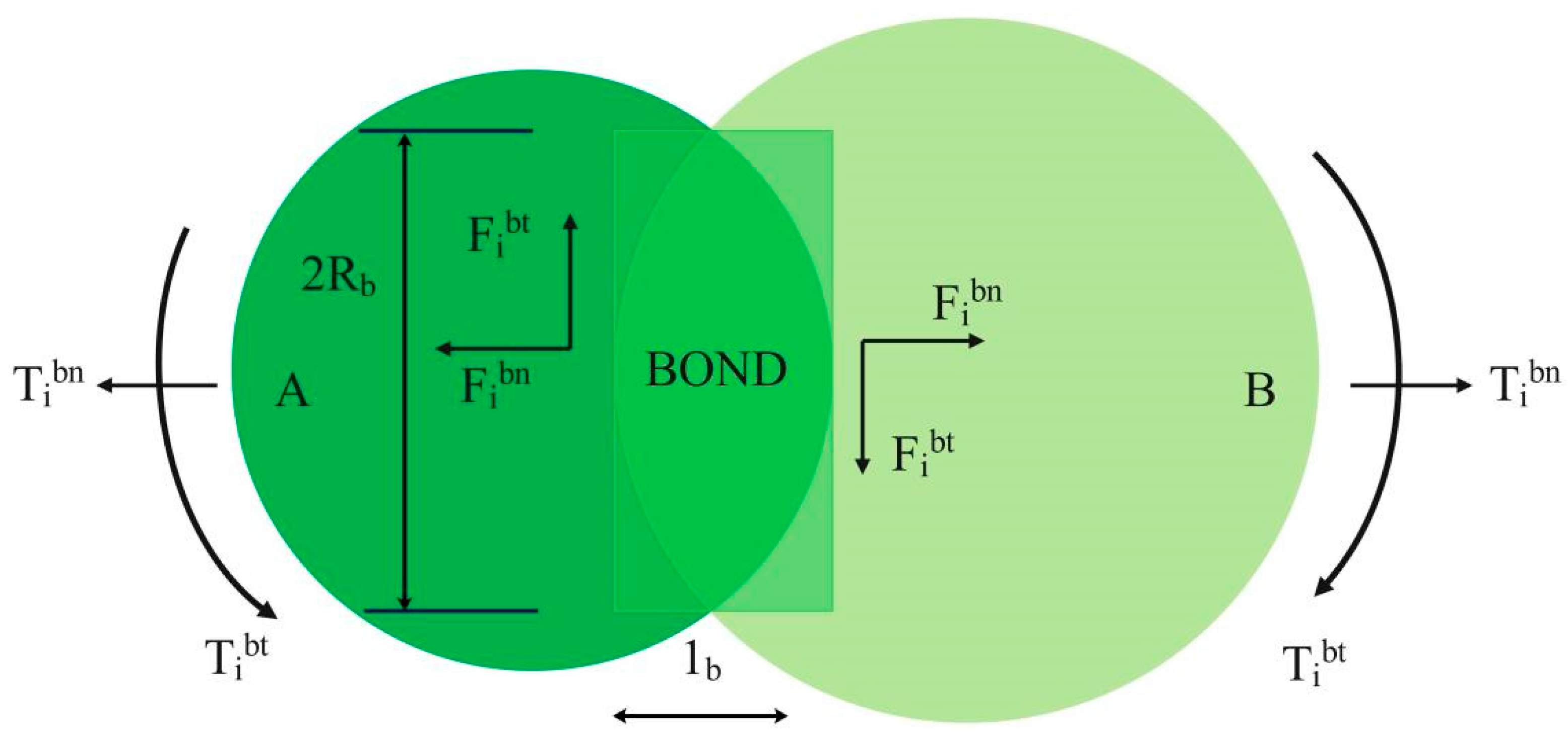
Internal friction force, cohesive force, and adhesive force are significant soil parameters that determine the resistance while performing field operations. The internal friction force is developed due to the relative motion of the soil particles and is a common force inherent in the soil matrix. Cohesive force is the intermolecular force between adjacent particles that is developed due to the liquid bridges between the soil particles. Adhesive force refers to the intermolecular forces that enable mutual adsorption between various materials. The Hertz–Mindlin theoretical contact model includes a range of contact models that can effectively investigate the interaction dynamics between soil particles of varying characteristics. The Hertz–Mindlin model with bonding V2 is particularly valuable in examining the bonding behavior of particles in soil within the root–soil complex, where the particles are held together by bonds that limit their normal and tangential forces, as illustrated in
Figure 15. The direction of the force determines the application of the forces on particles. If the normal and tangential forces reach beyond preset threshold values, the bonds will be broken, and interparticle forces can be determined using the typical Hertz–Mindlin contact model, as demonstrated in
Figure 16.
According to Newton’s Second Law, the dynamic equation of a soil particle is
In the formula, mi—the mass of the particle (), Δv—the increment in particle velocity (), Δω—the increment in particle angular velocity (), ΔT—the increment in time (), Ji—the polar moment of inertia of the particle (), Fib—the interparticle bonding force (), Fic—the interparticle contact force (), Fih—the liquid bridge force generated by the presence of water in the soil (), Tic—the contact torque (), and Tib—the bonding torque ().
Given the prominence of bonding interactions among soil particles within the root–soil composite, the analysis of interparticle bonding forces is of paramount importance. The mobilization of soil particles during cumin extraction induces deformation in both the soil particles and the bonding structures. The mathematical expressions for calculating the bonding force and bonding torque experienced by individual soil particles are presented as follows [
33].
In the formula, ΔFibn—increment of interparticle normal force (), ΔFibt—increment of interparticle tangential force (), ΔTibn—increment of interparticle torsional moment (), ΔTibt—increment of interparticle bending moment (), vn—interparticle relative normal velocity (), vt—interparticle relative tangential velocity (), ωn—interparticle relative normal angular velocity (), ωt—interparticle relative tangential angular velocity (), kn—normal stiffness (), kt—tangential stiffness (), Δt—time step (), Rb—bond radius (), Jb—moment of inertia of the bond cross-section (), and A—interparticle contact area ().
The maximum shear stress and normal stress under adhesive action can be explained by beam theory. If the shear stress and normal stress exceed a pre-selected critical stress value, the adhesive bond will break [
34], and the calculation formula is as follows:
In the formula, τc—shear stress (), τmax—maximum shear stress (), σc—normal stress (), and σmax—maximum normal stress ().
In the process of cumin uprooting, the cumin uprooting energy balance equation is established based on the quasi-static fracture energy balance theory:
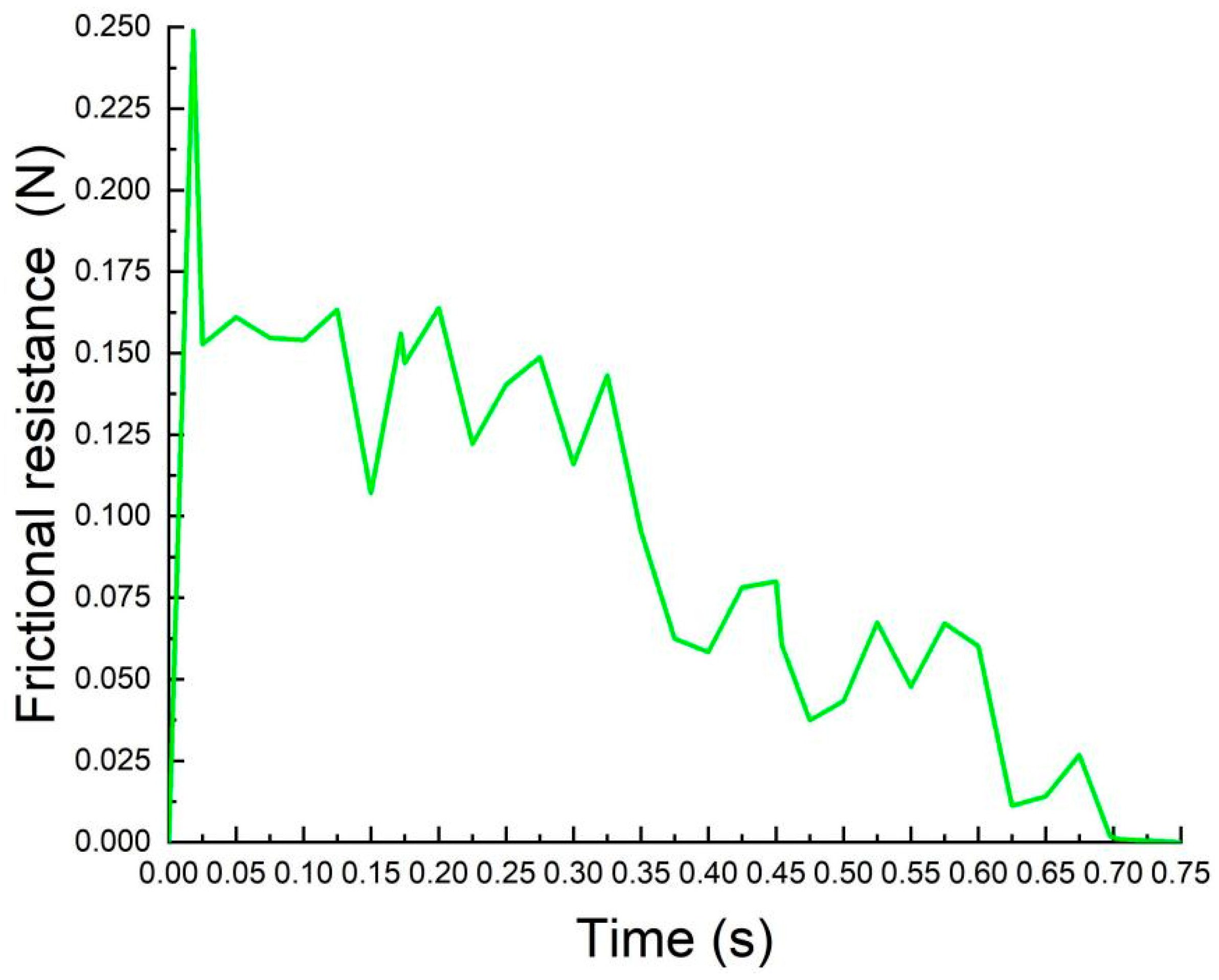
The less energy WP needed to uproot cumin from the soil, the more easily the cumin can be separated from the soil, hence making the processes of clamping, picking, and conveying easier. Equation (14) indicates that in order to reduce this energy WP need, one must minimize both the total of the soil surface energy and the inelastic strain energy included in the uprooting of cumin. These energies are mainly generated in the process of uprooting. The uprooting of cumin causes the links within the soil to break, leading to the breaking of the soil linked with the root–soil complex, at which point the cumin is pulled out from the soil. The mechanical analysis of soil particles shows that it is necessary to increase the velocity of soil particles to enable the breaking of these bonds. Hence, it is necessary to investigate the influence of soil particle velocity on the process of cumin uprooting.
Since cumin extraction occurs underground and is under the control of intricate interactions between the taproot and sandy loam soil, coupled with constraints on experimental conditions and measurement technology, it is hard to measure precisely the interactive dynamics between the taproot and sandy loam soil, along with the effect of the uprooting process on root–soil movement patterns through conventional methods that blend theoretical analysis and empirical experimentation. Thus, the investigation of the process of uprooting using simulation is of critical importance. The discrete element method (DEM) enables us to investigate root–soil movement dynamics and root–soil interaction on a micro-structural scale. Presently, researchers have utilized the DEM extensively to develop soil models with the aim of examining the interactions between soil and machinery during various operations like subsoiling, excavation, and shoveling. Laboratory tests on soil physical properties indicate that, when as a separate body composed of numerous tiny particles, there exists a definite adhesion force among soil particles. It is quite important for the interaction between the soil and equipment. The Hertz–Mindlin contact model with bonding V2 can simulate the bonding phenomenon between soil particles very well. It includes both normal and tangential contact forces that may result in the agglomeration and bonding of particles. Following bonding, bonds are formed at the interface of the particles, as indicated by
Figure 15, which causes the cohesion of small particles into large masses of material. As soon as the externally applied force is greater than the critical value of the bonding force, it will result in the crushing and fracturing of the material.
In order to achieve a correct root–soil composite DEM model, the DEM model parameters need to be calibrated separately for the root, the soil, and the root–soil contact model. The DEM has been widely used in the establishment of soil models, thus, there are a broad variety of relatively well-established research methodologies for calibrating the parameters of the soil DEM model [
35,
36,
37]. Based on the calibration techniques of soil DEM model parameters used by domestic and foreign researchers, the parameters of the soil DEM model can be obtained accurately.
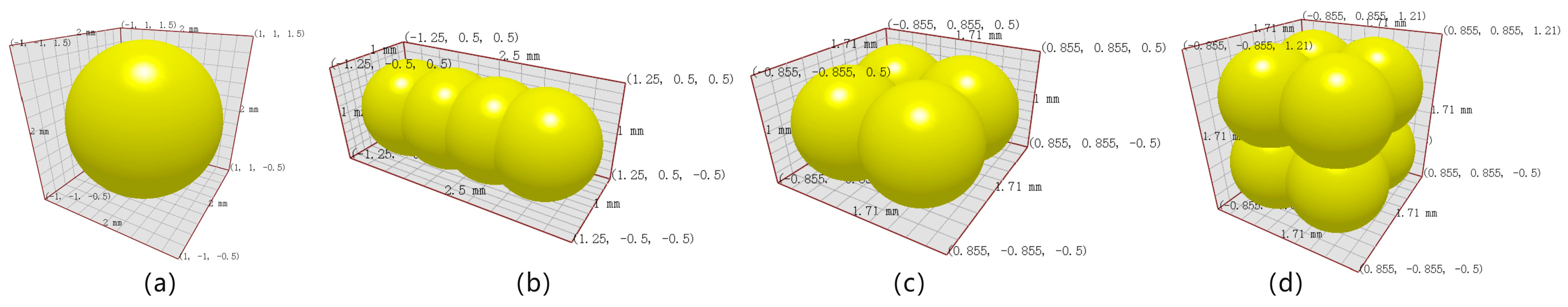
With the non-similar and random shapes of the soil particles, the clumped particle method is adopted in this research to develop four various soil particle models with different shapes as presented in
Figure 17; the discrete element method (DEM) parameters of Xinjiang sandy loam soil [
31,
32] are listed in
Table 1.
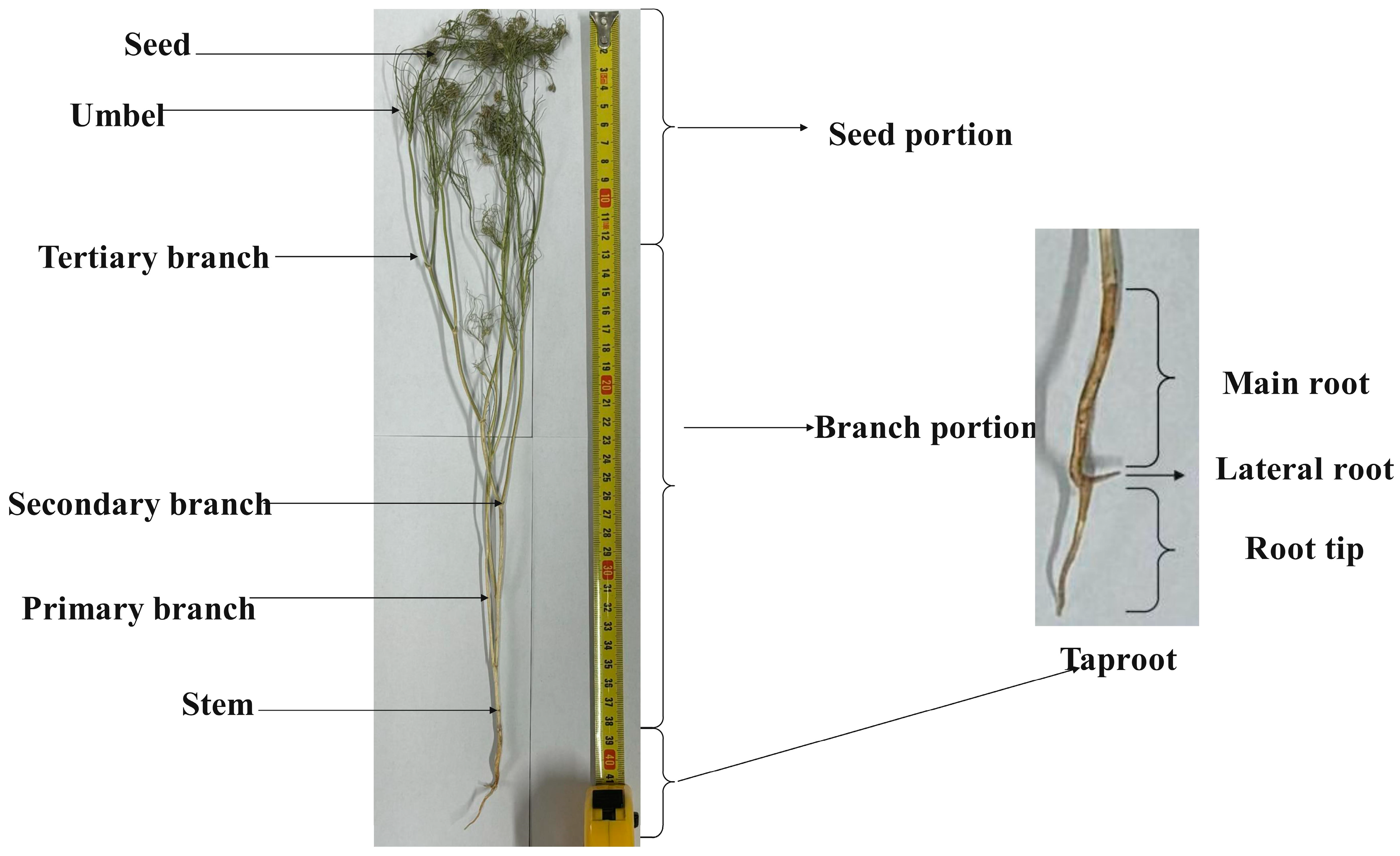
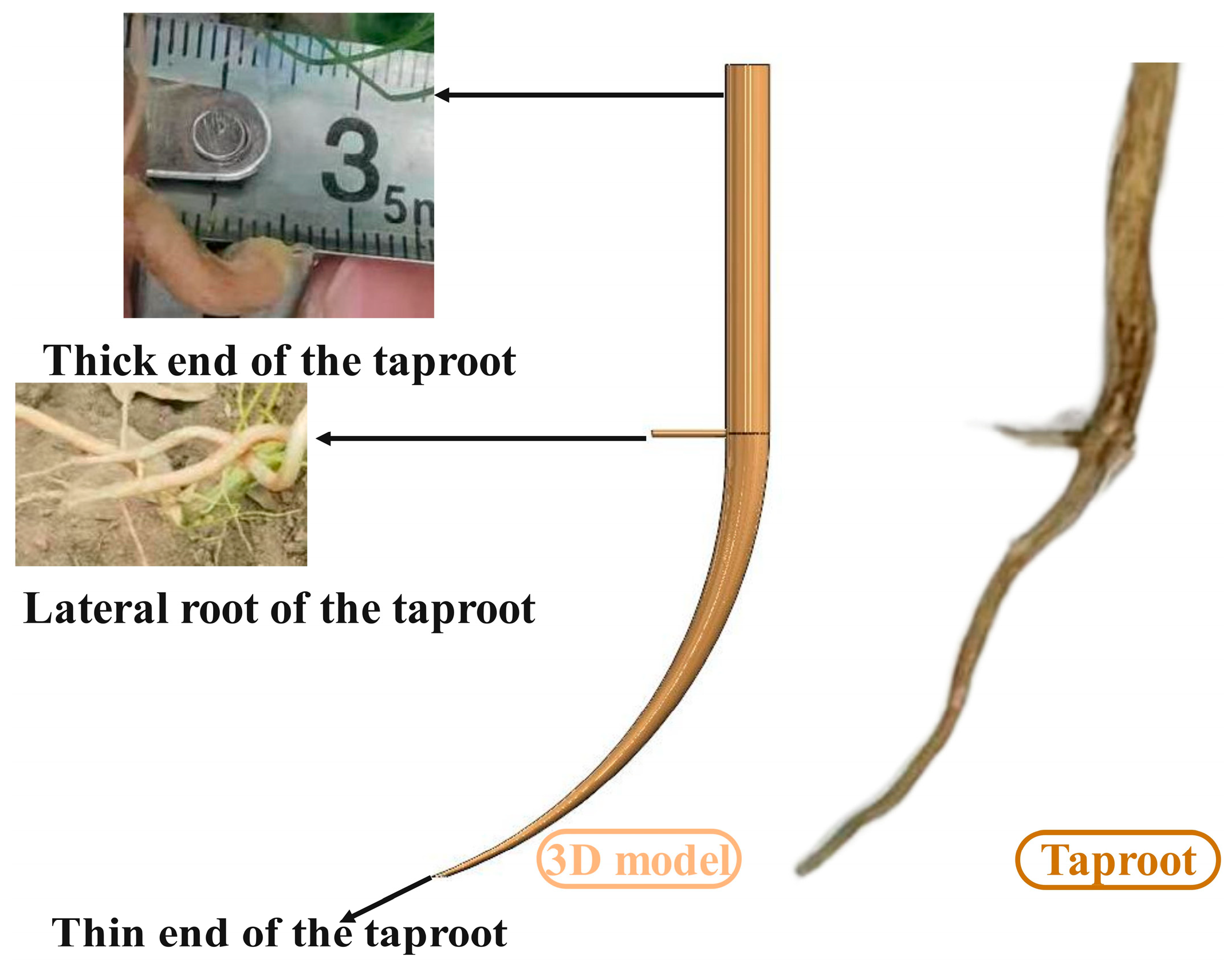
Statistical analysis of the dimensional parameters of mature, ready-to-harvest cumin taproots revealed them to be approximately slender, slightly curved, and tapered cylinders, predominantly featuring a single small lateral root. Specifically, the diameter of the thick end of the taproot is 3 ± 0.5 mm, the diameter of the thin end is 1 ± 0.5 mm, and the diameter of the lateral root is approximately 1 ± 0.5 mm. Consequently, the cumin taproot was treated as a slightly curved cylinder with a thick-end diameter of 3 mm, a thin-end diameter of 1 mm, and a depth of 55 mm. The cumin taproot 3D model was then created using SOLIDWORKS 2023 software based on these dimensions, as shown in
Figure 18. To verify the accuracy of the cumin taproot 3D model, MATLAB R2022a was employed to extract and compare the contour curves of both the 3D model and the actual taproots.
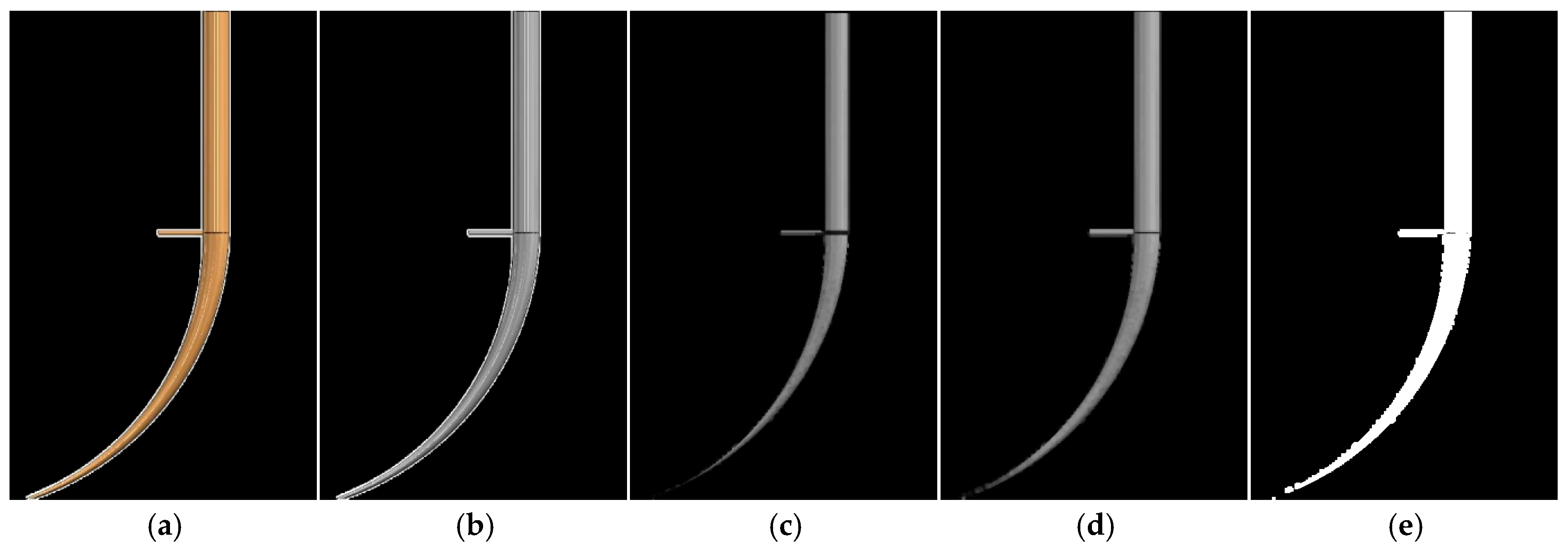
The images were first preprocessed, including grayscale conversion, erosion, dilation, and binarization, as shown in
Figure 19 and
Figure 20. Subsequently, the contours of the binarized images were extracted, and the coordinates of the points on the contours were obtained. Contour curves of the images were then plotted in
Figure 21, and scatter plots of the coordinates of the points on the contours were generated for comparative analysis.
Both the taproot profile curve and the taproot 3D model profile curve show a downward tendency from left to right, and there is a sharp decline for both curves at about X = 270 along the X-axis. There is a relatively stable vertical distance between the taproot profile curve and the taproot 3D model profile curve along most of their lengths, suggesting similar shapes with the same vertical displacement. In spite of the presence of minor local discrepancies in details, the two curves show a high level of similarity and precision, as manifested in their macro trend stability, coincidence of significant feature points, and comparatively stable vertical shift. This observation corroborates a fundamental agreement in their macroscopic structure, prevailing direction, and inherent characteristics.
Through the application of contact detection algorithms together with a cohesive contact model, EDEM software has the capability to calculate the forces on particles [
38]. The Hertz–Mindlin with JKR Cohesion model, hereinafter known as the JKR model, is one such cohesive contact model derived from the Hertz contact model. This model considers the effect of cohesive forces between particles in wet conditions on their motion and is particularly appropriate for simulating materials with high adhesion and agglomeration caused by forces such as electrostatic attraction and water, which is suitable for soil and agricultural products. Because of this, the JKR model was selected to represent the contact mechanics between the cumin taproots and sandy loam soil. The JKR model includes surface energy in particle interactions. When the root–soil particle interactions are modeled using the JKR contact model, it is observed from Equation (15) that the force required for detachment of the two types of particles is a function of the liquid surface tension and the wetting angle. Interparticle adhesive forces are significant to model, and surface energy is used by the JKR model for modeling these adhesive forces.
In the formula, Fp—the force required for separating the two particles (N), τ—wetting angle (), and Ri, Rj—particle radius ().
Parameter calibration of the established cumin taproot discrete element model (DEM) is crucial for its accurate construction [
39,
40,
41,
42,
43]. Intrinsic material properties and contact properties, such as elastic modulus and coefficient of friction, were directly measured using the direct measurement method. The final modeling parameters were determined by calibrating the model against real-world experiments using virtual simulations. The calibrated parameters for the cumin taproot DEM are listed in
Table 2.
Hertz–Mindlin with the bonding V2 contact model primarily includes seven bonding parameters: normal stiffness per unit area, normal range, shear stiffness per unit area, shear range, normal strength, shear strength, and bonded disk scale. Normal stiffness per unit area represents the bonding strength between sandy loam particles normal to the contact surface. Higher values imply a greater ability of the particles to resist compression and tension. Shear stiffness per unit area indicates the bonding strength between sandy loam particles parallel to the contact surface. Higher values signify a greater resistance of the particles to sliding. Stiffness parameters govern the extent of deformation within the elastic range of the bond. Range parameters define the limits of the bonding force. Strength parameters determine the critical threshold for bond breakage. The bonded disk scale influences the area of the bonding effect. Reliable bonding parameter settings employed in the DEM simulation are detailed in
Table 3.
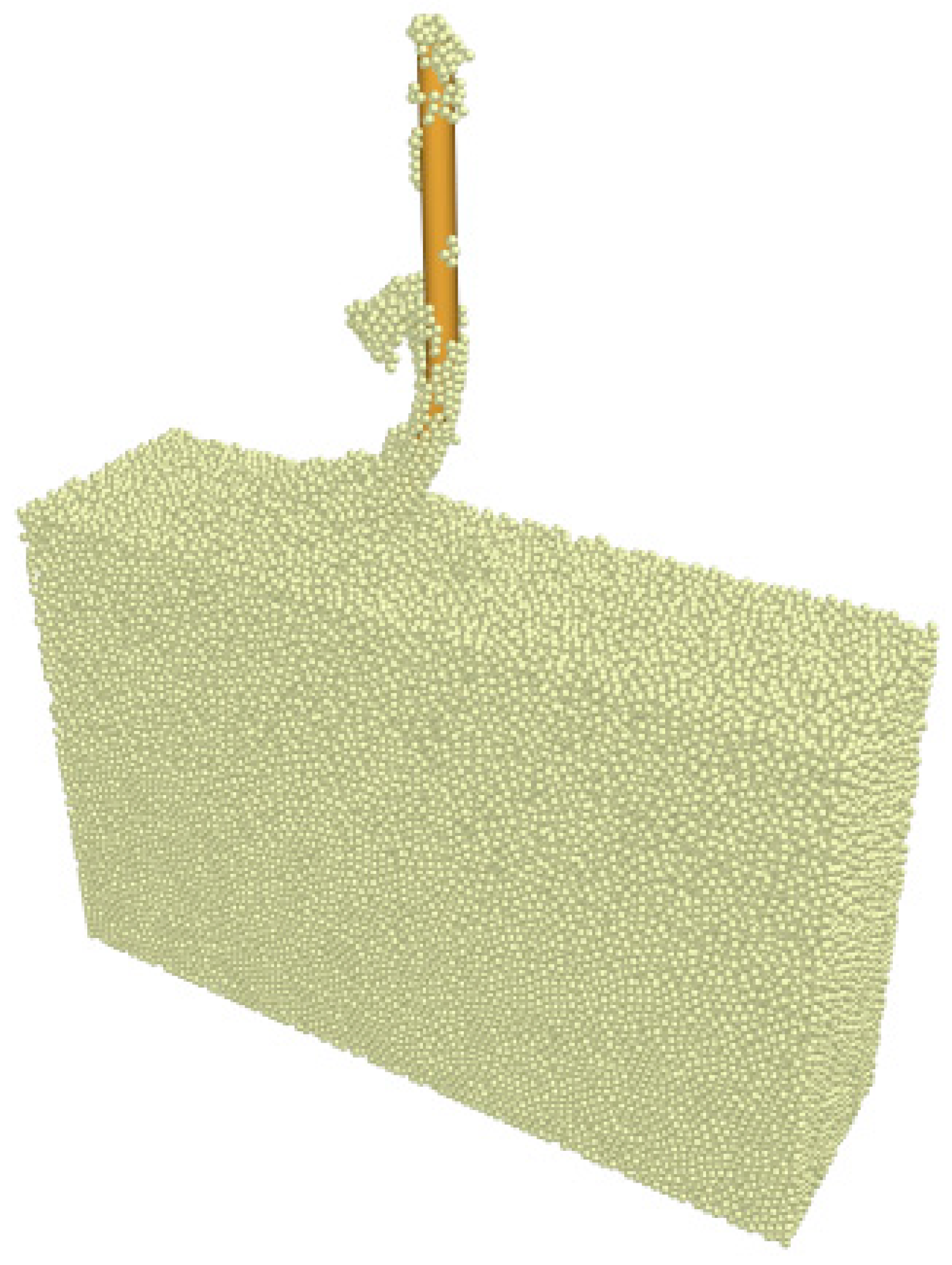
The composite DEM soil bin model for cumin taproot in sandy loam soil is illustrated in
Figure 22. Using EDEM 2024, forces and motion can be added to the cumin taproot, and the resultant combination of vertical and horizontal movement constitutes the extraction process. The extraction angle and speed are modified by adjusting the magnitudes of the vertical and horizontal velocities.
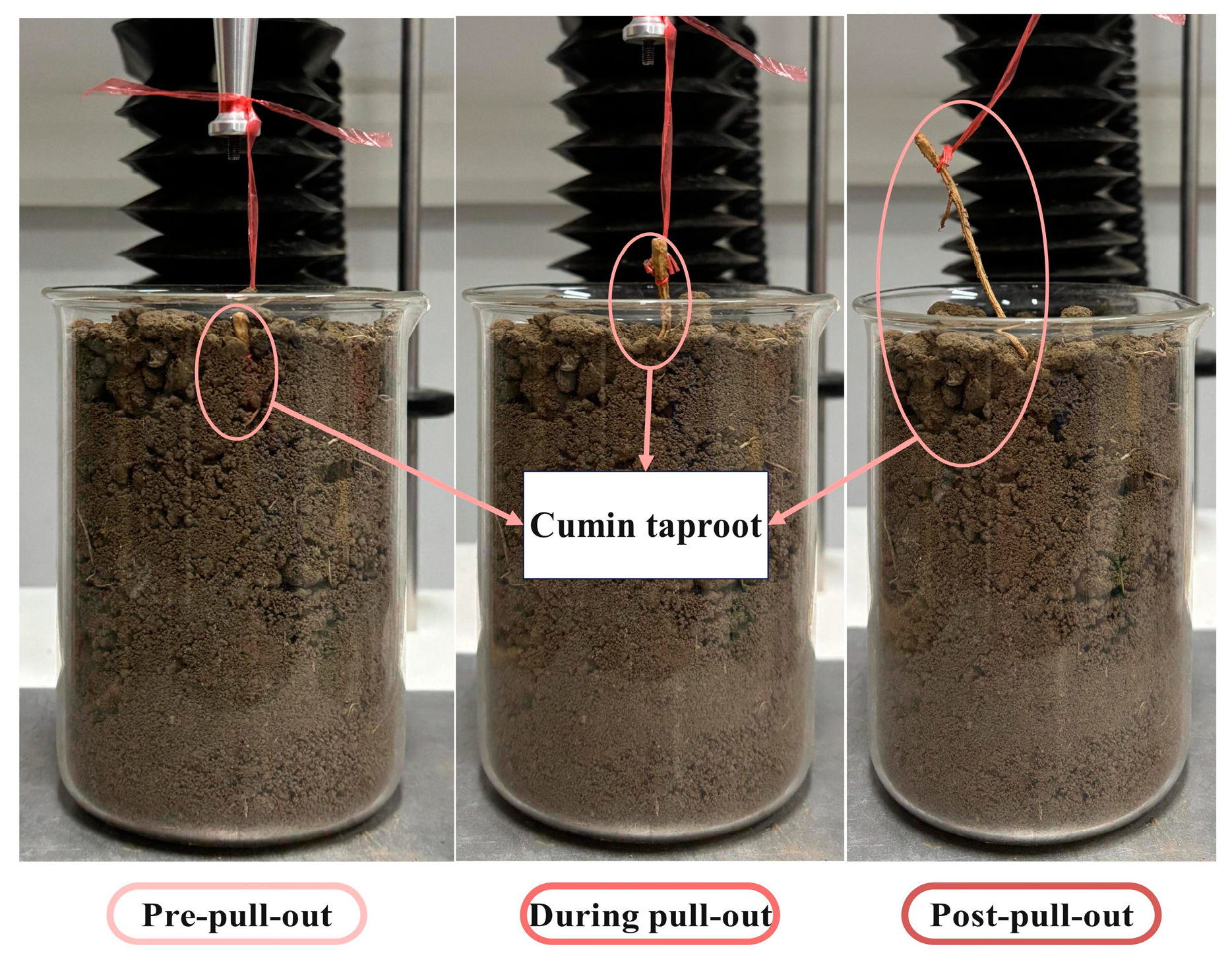
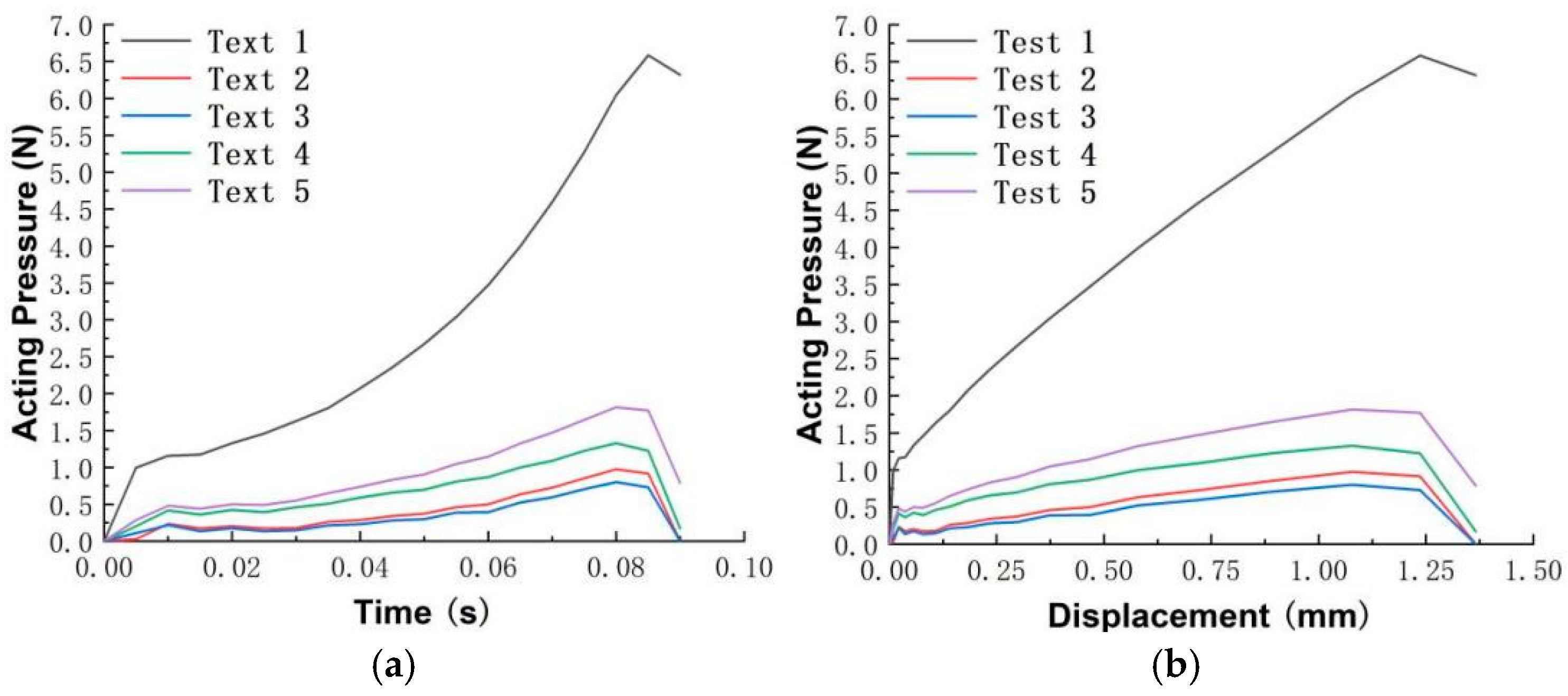
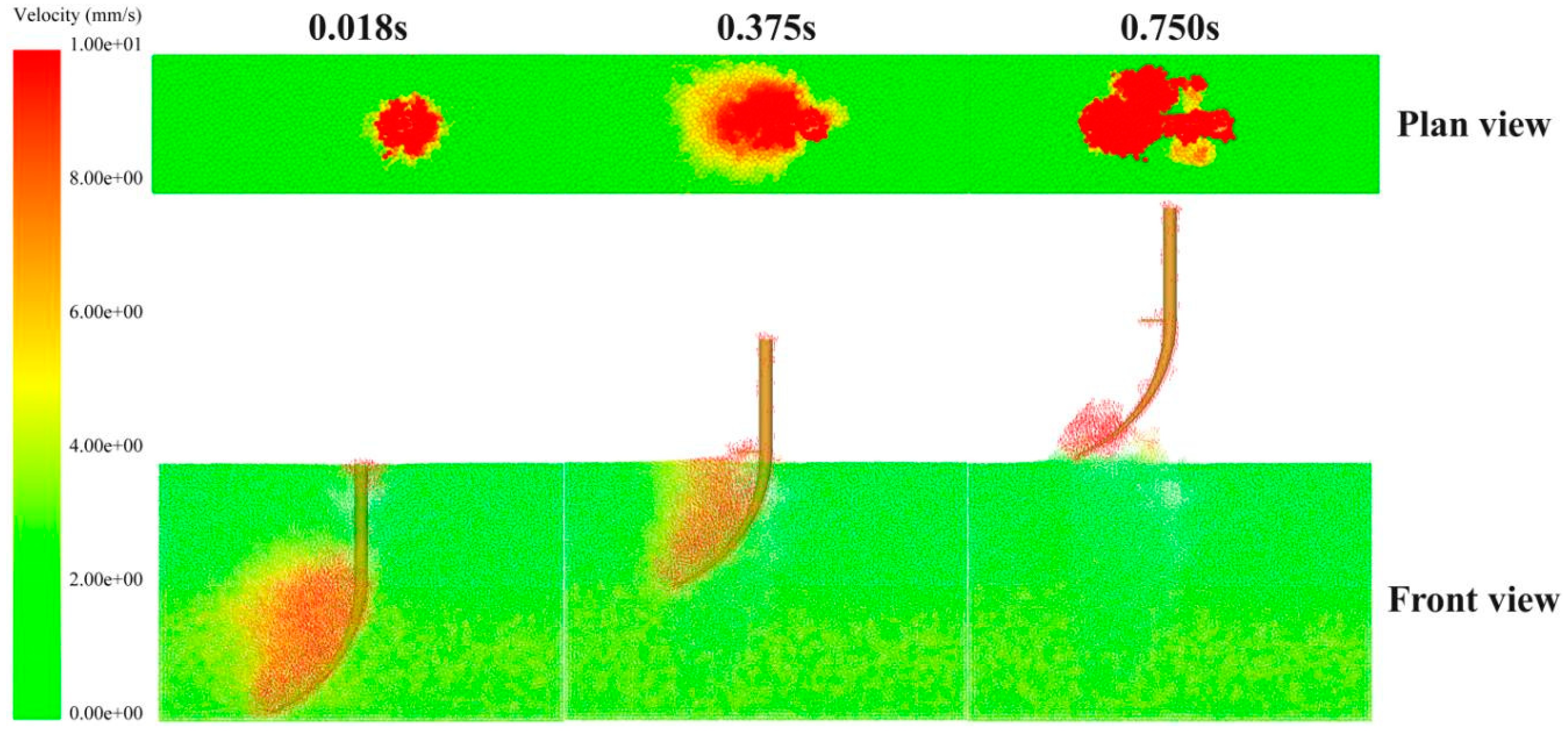
As shown in
Figure 23, an upward extraction force was applied to the cumin taproot until it detached from the soil surface. During the initial stage of the extraction process, due to the surface energy between the root and the soil, the soil moved with the root until the bond between soil particles fractured, ultimately achieving root–soil separation. However, some soil particles still adhered to the surface of the extracted cumin taproot. The entire extraction process is consistent with the actual field extraction of cumin.
In the cumin taproot–sandy loam composite DEM soil bin model, motion constraints were applied to the cumin taproot. In the ’Dynamics’ module, the extraction force and angle of the cumin taproot were set, and a ’Force Controller’ motion type was added. The extraction angle was set to , and the extraction speed was set to . In the solver, the time step was set to 20%, the total simulation time was set to 0.75 s, and the cell size in the solver mesh was set to .
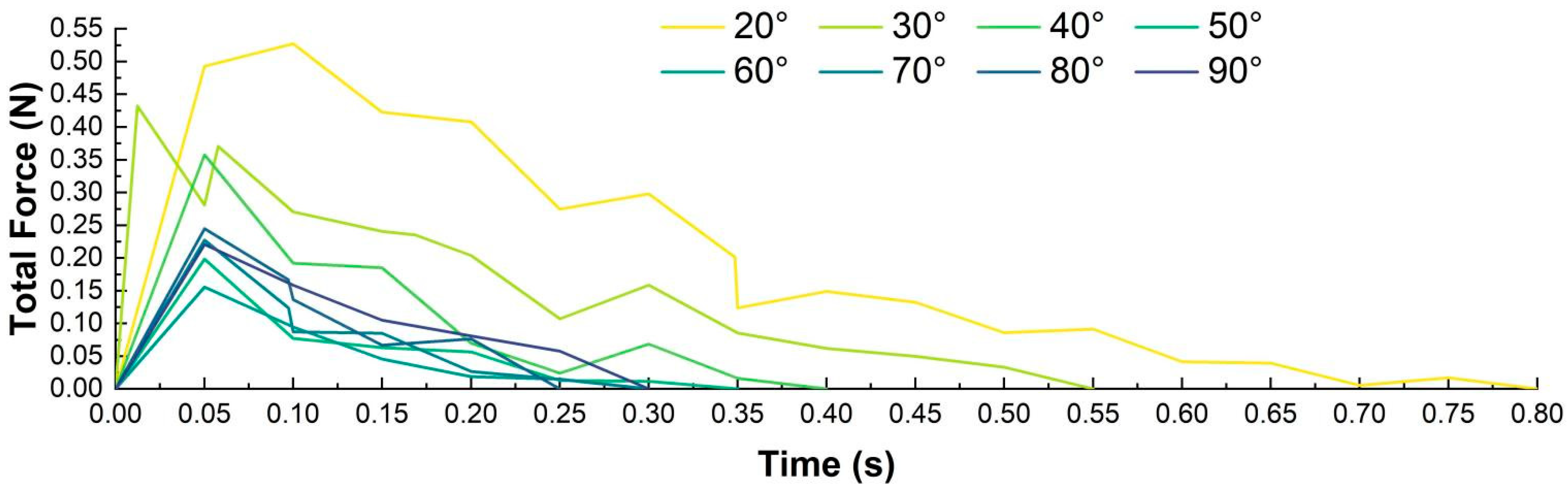
To investigate the variation pattern of the maximum pull-out force under different pull-out angles, a simulation experiment of cumin taproot pull-out was conducted with pull-out angles of , , , , , , , and . The pull-out linear velocity was set to 23.4 cm/s. In the solver, the time step was set to 20%, and the total simulation time was set such that the cell size in the solver grid was 3R min.