Design and Experiment of Opposed Roller-Type Picking Device for Chrysanthemum Indicum
Abstract
1. Introduction
2. Materials and Methods
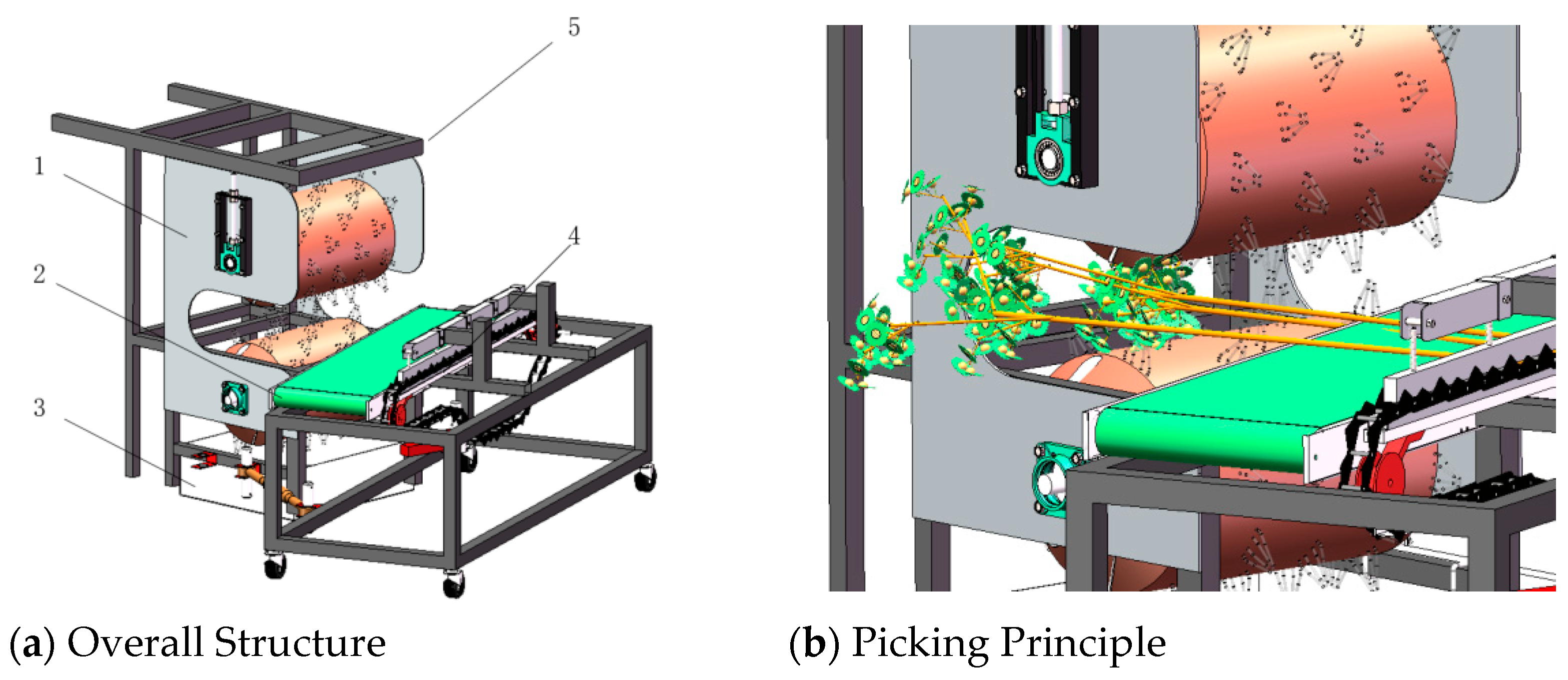
2.1. Overall Structure and Working Principle
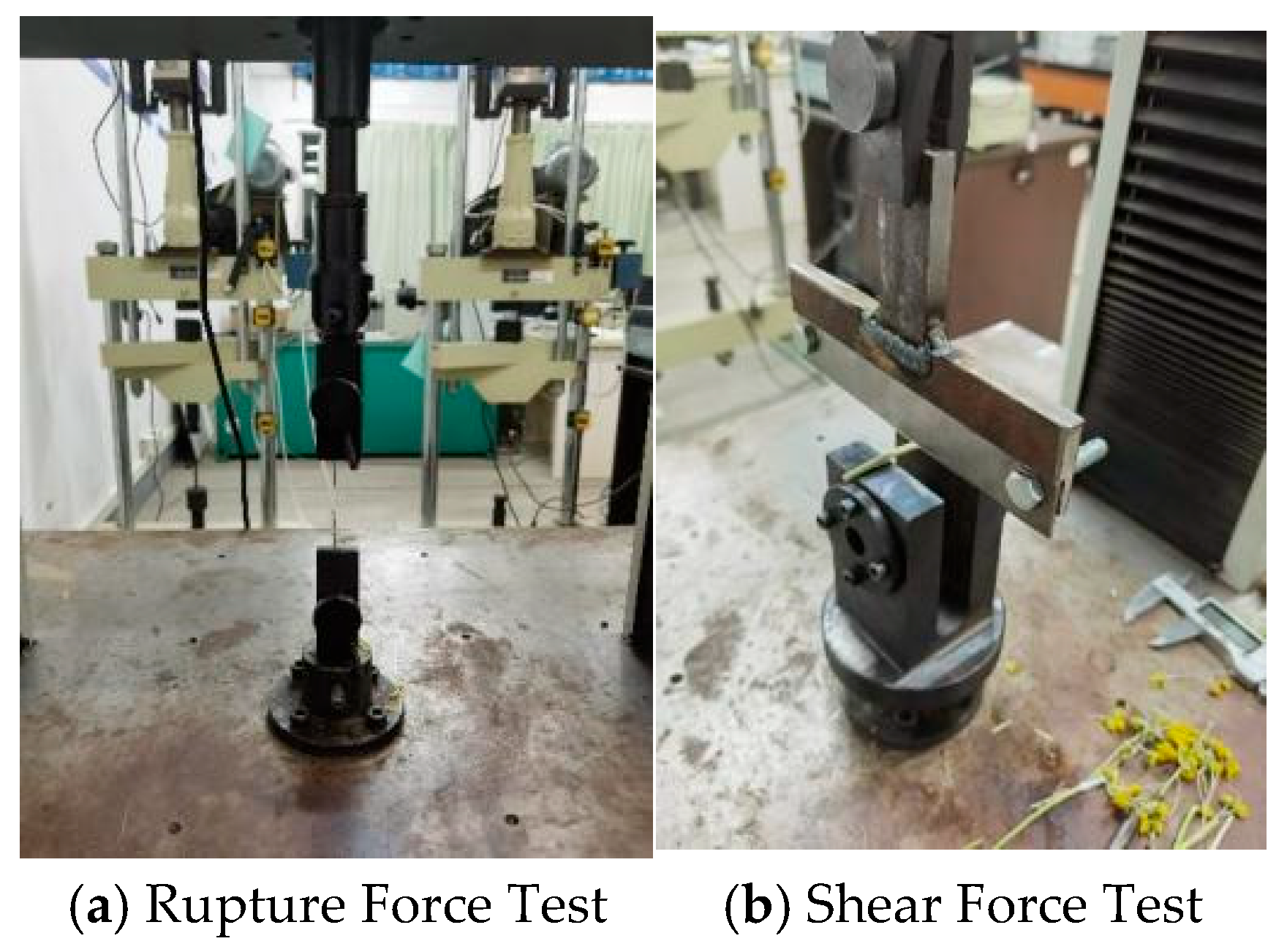
2.2. Harvesting Characteristics of Wild Chrysanthemums
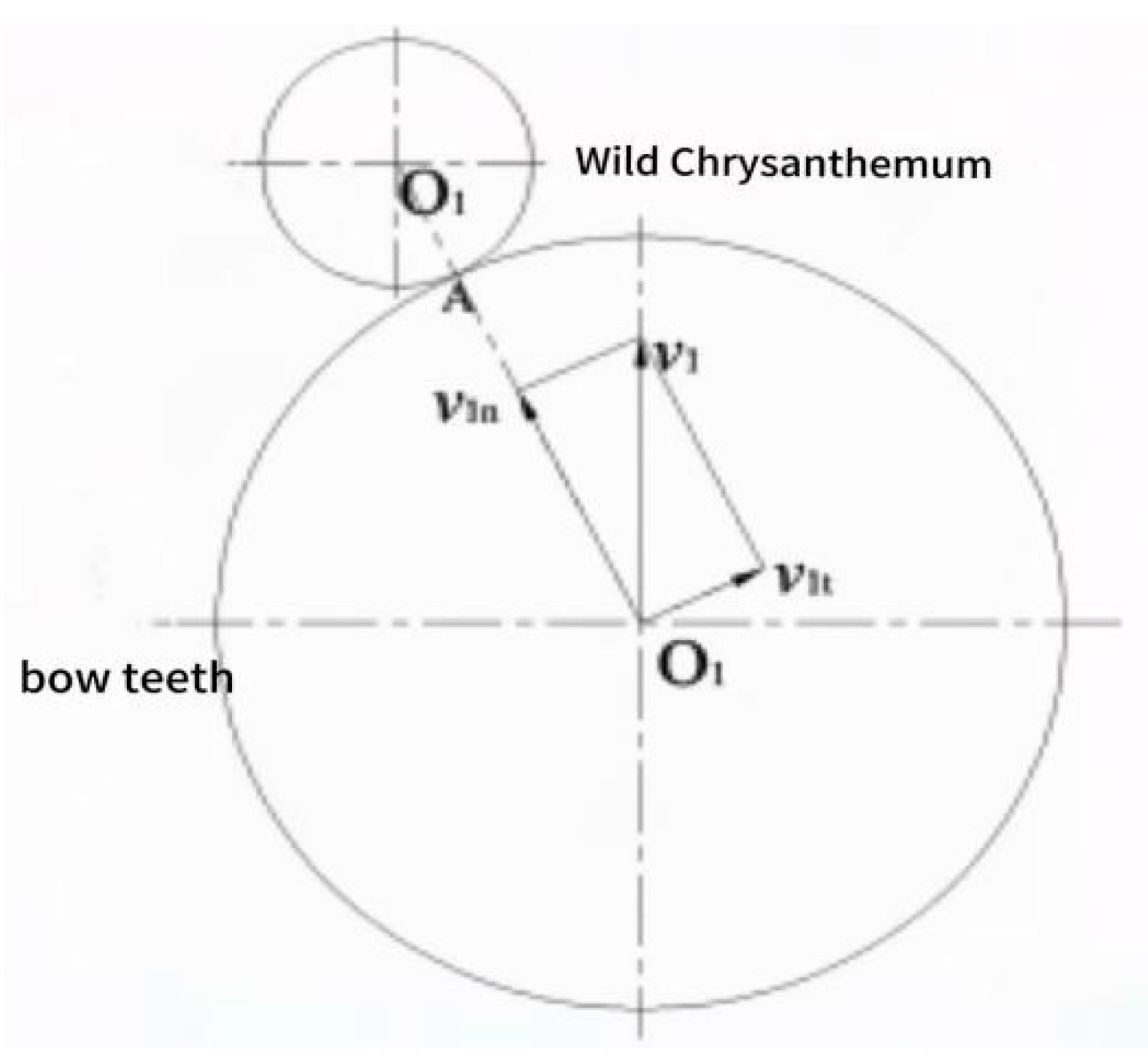
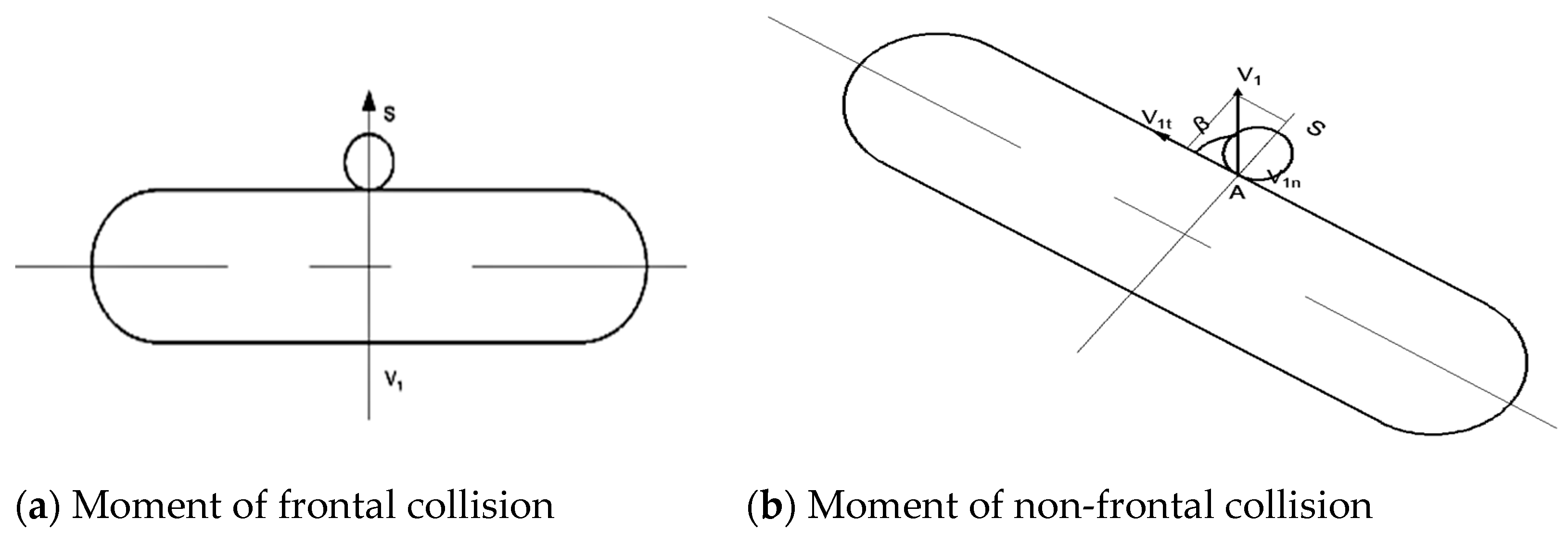
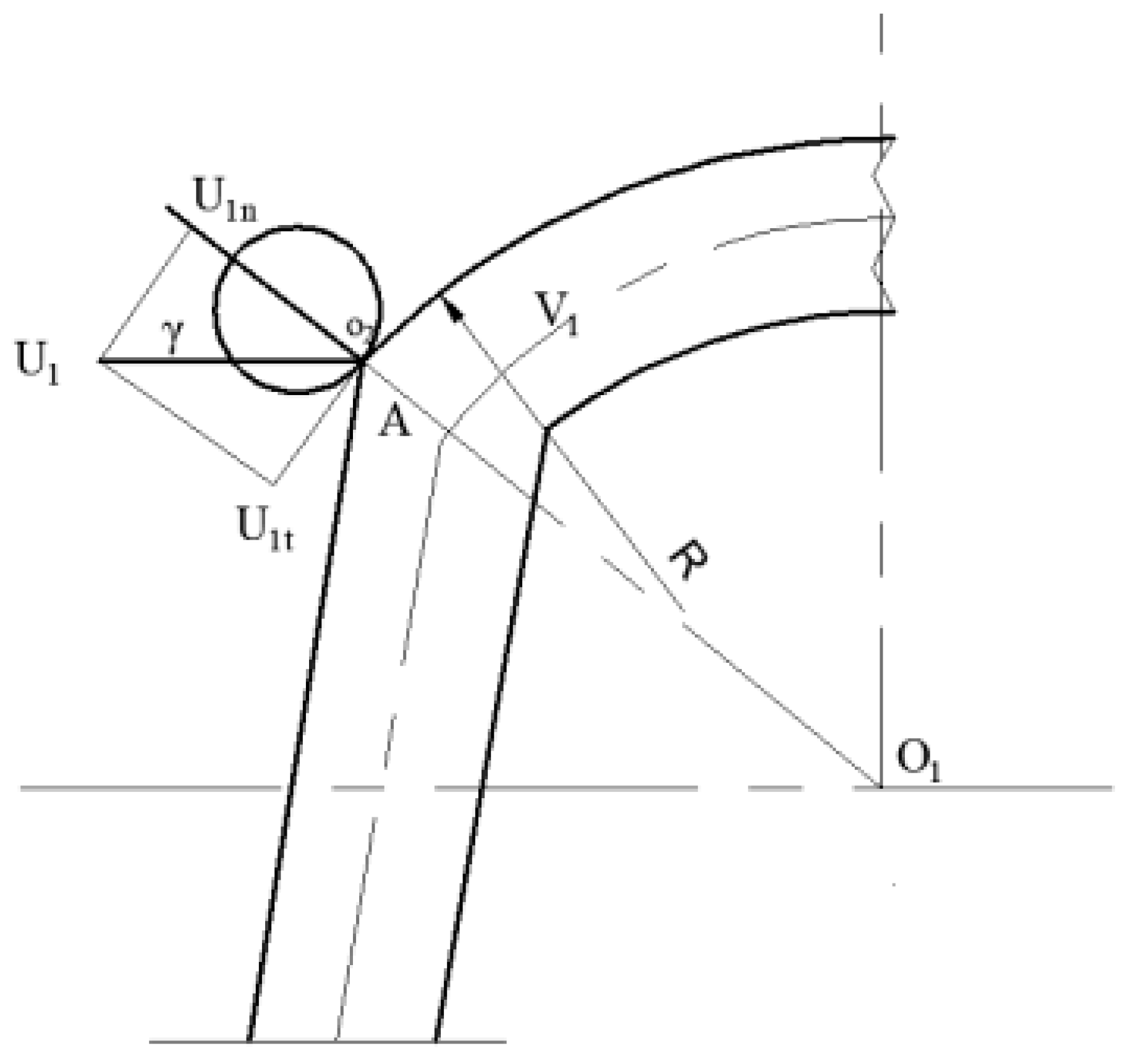
2.3. Dynamics Analysis of the Picking Process
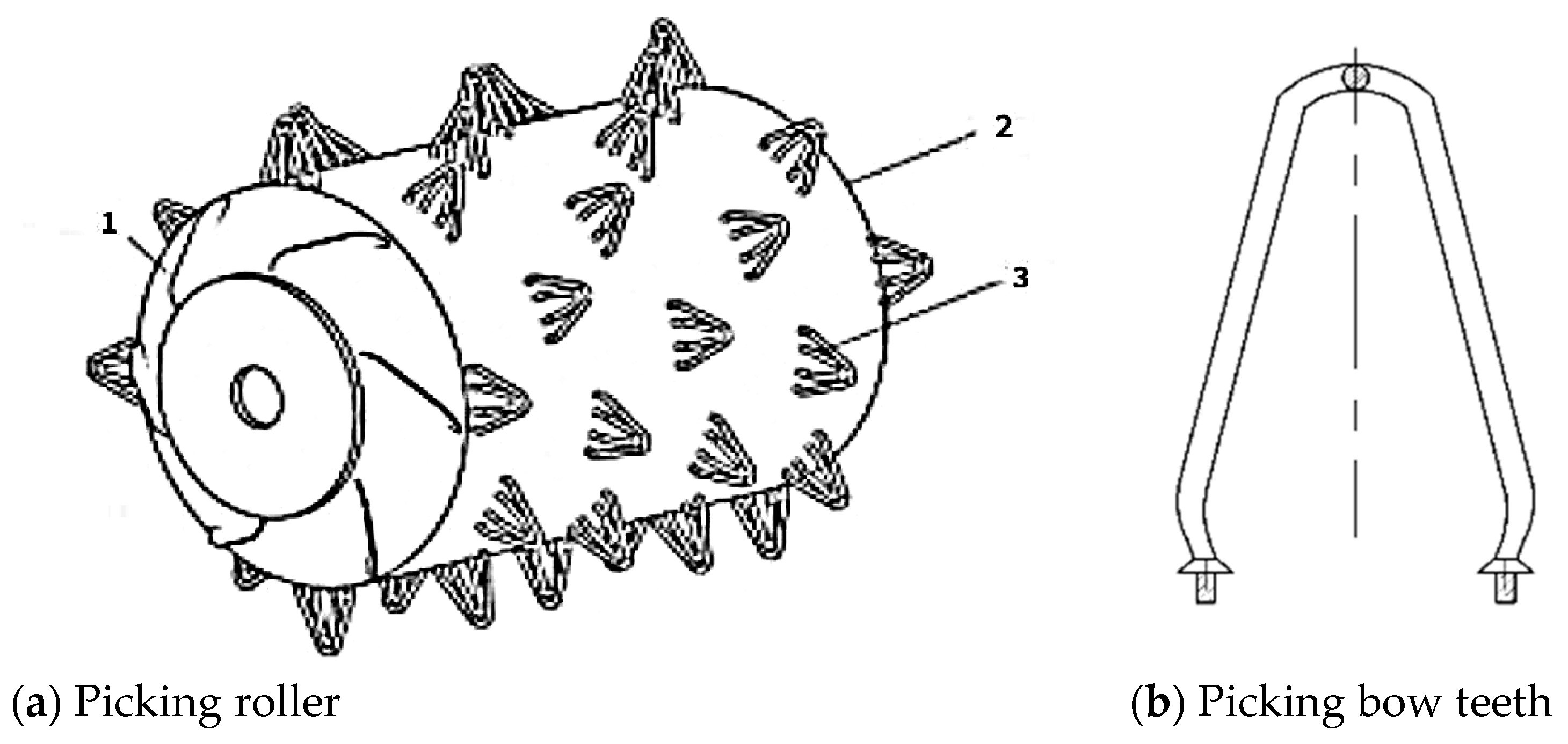
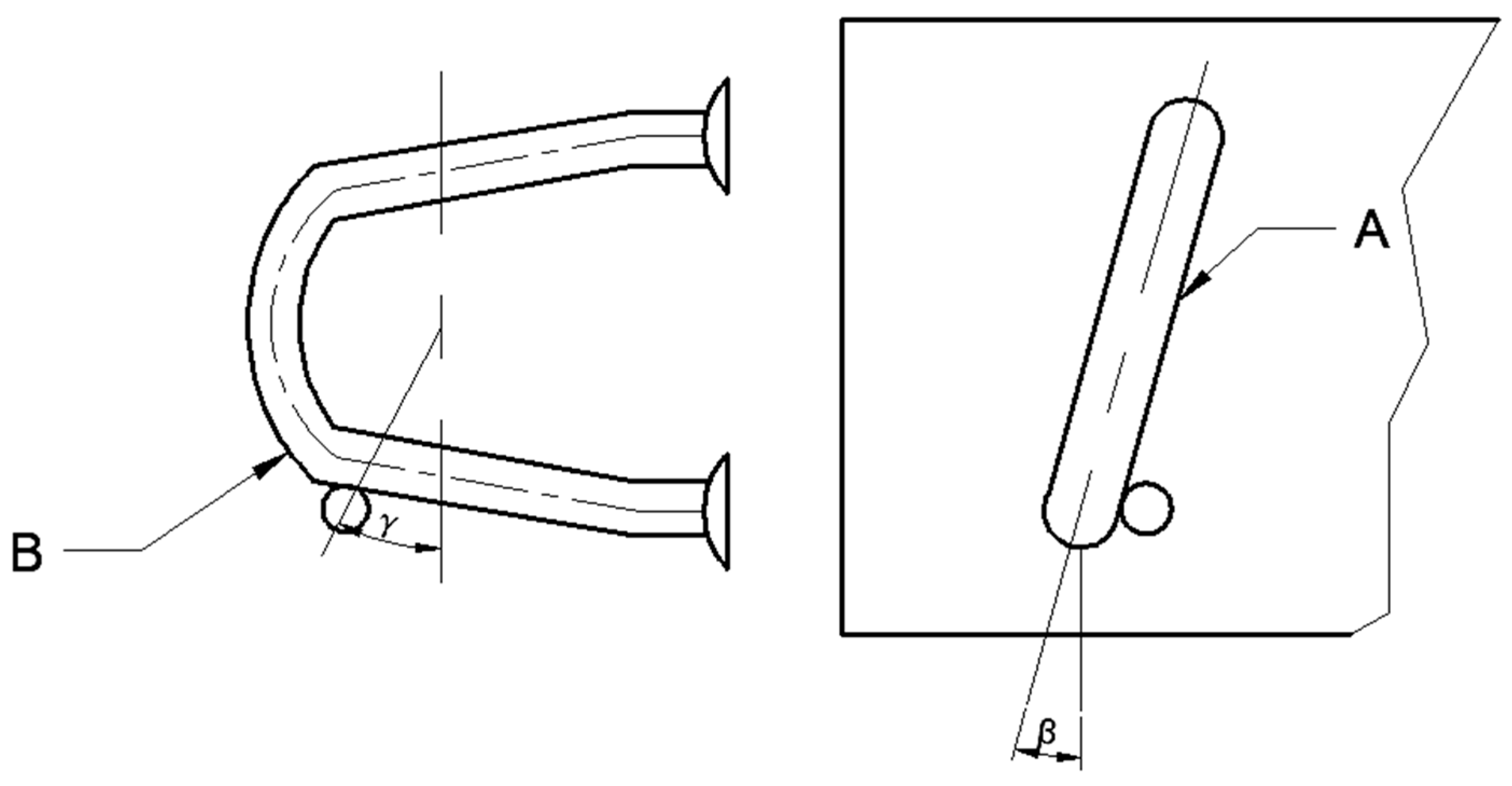
2.4. Structure Design of Picking Roller
2.5. Simulation Experiment
2.5.1. Simulation Model Establishment
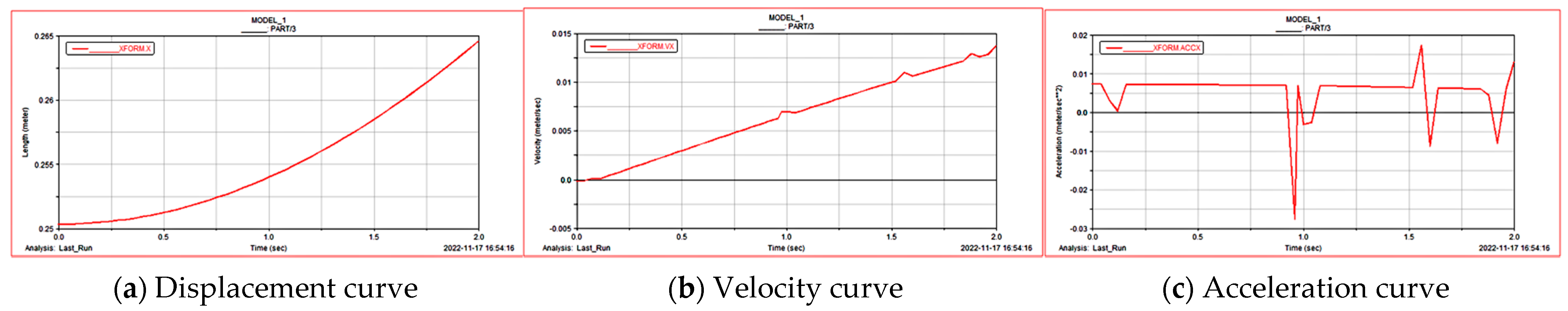
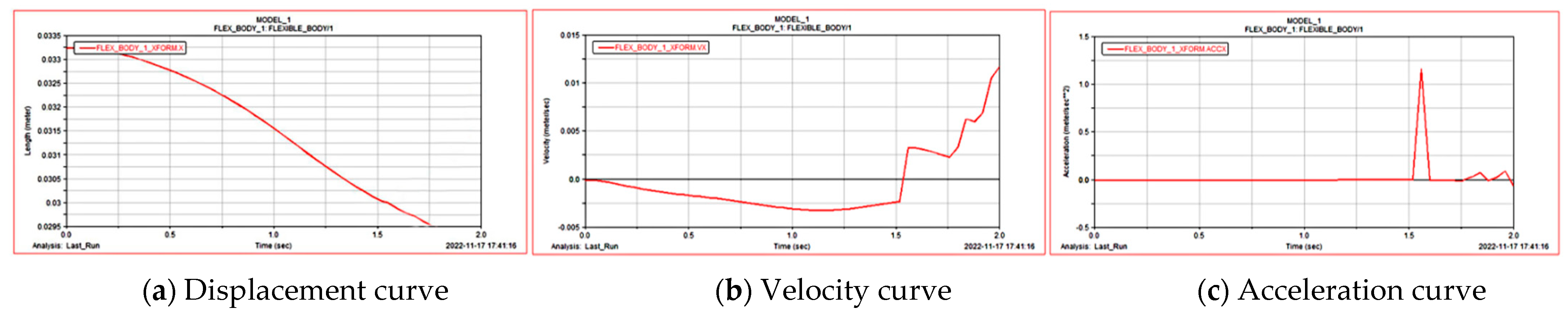
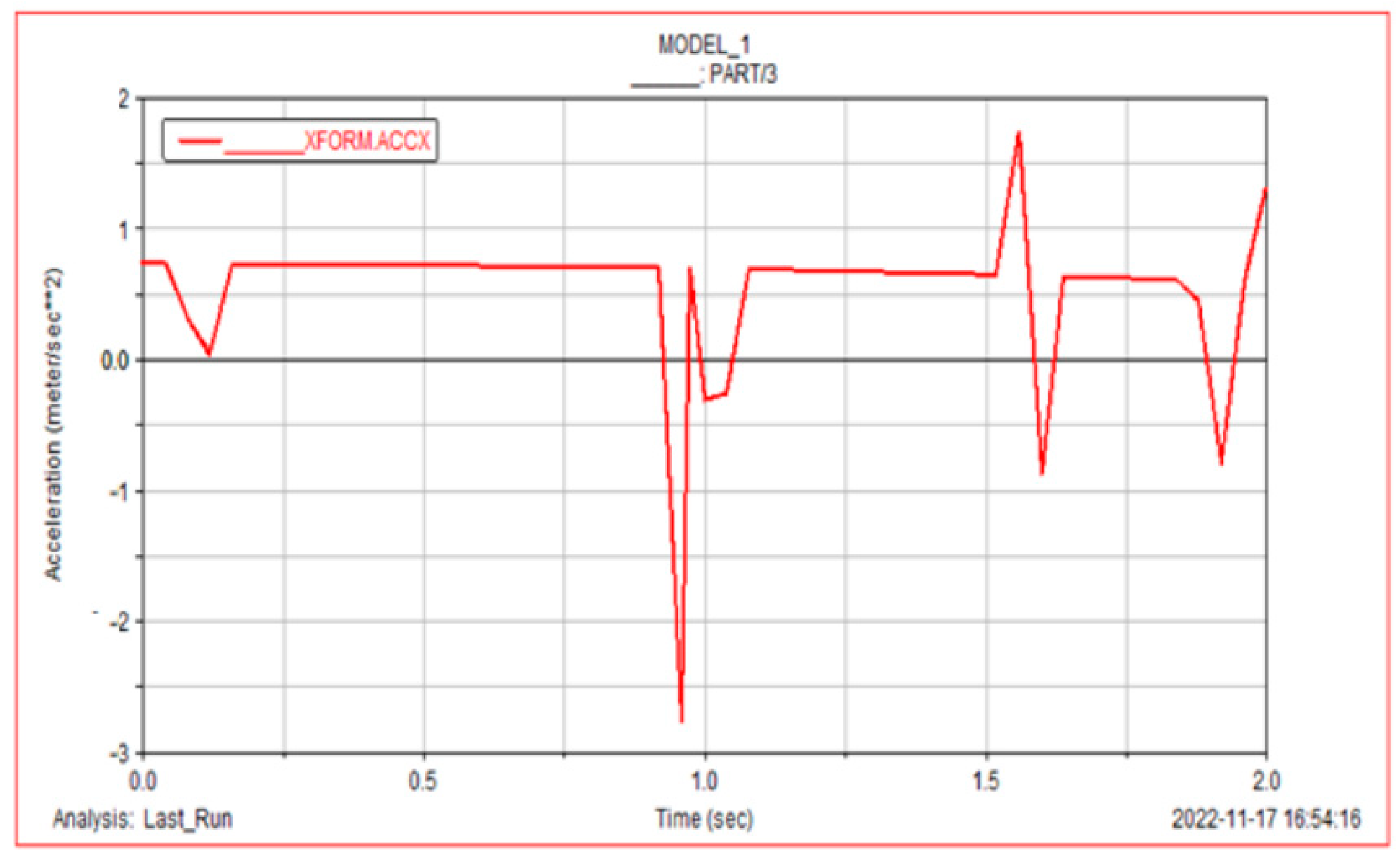
2.5.2. Simulation Analysis of Collision Process
2.5.3. Experiment of the Influence of Motion Parameters on Flower Picking Performance
2.5.4. Validation Test
2.6. Bench Test
3. Results and Discussion
3.1. Effects of Harvesting Characteristics of Wild Chrysanthemums
3.2. Effects of Motion Parameters on Flower Picking Performance
3.3. Effects of Bench Test
3.3.1. Analysis of Test Results
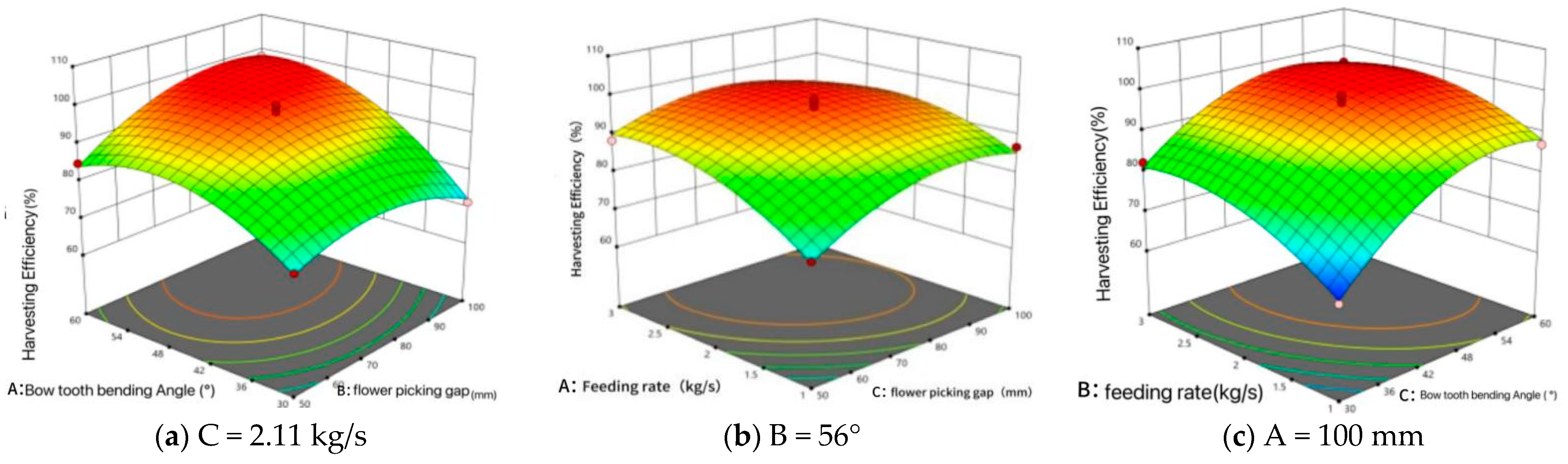
3.3.2. Response Surface Analysis
4. Conclusions
- (1)
- In response to the challenges of high labor demands and low efficiency associated with the manual harvesting of wild chrysanthemum in China, and the absence of dedicated mechanized harvesting equipment, an opposed roller picking device was designed, tailored to meet the specific agronomic requirements of wild chrysanthemum harvesting.
- (2)
- The key components of the opposed roller picking device for wild chrysanthemum were analyzed to ensure full-range harvesting coverage during the picking process, and the optimal harvesting tines were selected. To enhance the picking efficiency during the collision between the flowers and the tines on the rollers, the roller arrangement was carefully designed, and the motion dynamics during the collision process were analyzed. It was determined that the primary factors influencing the picking performance were the roller rotational speed, feeding speed, and collision angle. Based on these factors, a regression orthogonal experiment was designed using critical acceleration as the experimental index to establish a regression model. Under optimal collision force conditions, the feeding speed was determined to be 1.3 m/s, and the roller rotational speed was 3.73 rad/s.
- (3)
- A regression orthogonal experiment was conducted using the picking gap, roller bow tooth bending angle, and feeding rate as experimental factors, with the flower picking cleanliness rate as the experimental index. A regression model was established to describe the relationship between the experimental index and the influencing factors, thereby identifying the optimal range of experimental parameters. Bench tests further verified that, when the picking gap was set to 100 mm, the roller bow tooth bending angle to 56°, and the feeding rate to 2.1 kg/s, the picking rate reached 95.9%, effectively meeting the design requirements.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Yuan, H.; Lai, Z.; Guan, Y.; Zhang, Y.; Huang, Y. Pharmacology study of active constituents from chrysanthemum indicum. Chin. Arch. Tradit. Chin. Med. 2018, 36, 651–653. (In Chinese) [Google Scholar]
- Song, Y.; Xu, L.; Miu, J.; Xiao, P. Research progress in chrysanthemum indicum. Mod. Chin. Med. 2020, 22, 1751–1756, 1762. (In Chinese) [Google Scholar]
- Liu, S. Design and Experimental Study of Drum Type Wild Chrysanthemum Picking Device. Master’s Thesis, Northwest A&F University, Xianyang, China, 2023. (In Chinese). [Google Scholar]
- Wang, T. Design and Experimental Study of Stem Cutter for Wild Chrysanthemum. Master’s Thesis, Northwest A&F University, Xianyang, China, 2023. (In Chinese). [Google Scholar]
- Wang, R.; Yu, H.; Chen, A.; Li, A.; Gao, L.; Zheng, Z. Design and Test of Pneumatic Flipping Comb-type Chrysanthemum Picking Device. Trans. Chin. Soc. Agric. Mach. 2022, 53, 184–193. (In Chinese) [Google Scholar]
- Wang, R.; Zheng, Z.; Lu, X.; Cui, B.; Gao, L. Design and Test of Comb-teeth Reciprocating Chrysanthemum morifolium Picking Device. Trans. Chin. Soc. Agric. Mach. 2019, 50, 73–79, 97. (In Chinese) [Google Scholar]
- Ji, C.; Zhang, C.; Gu, B.; Fu, H.; Xie, D.; Guo, J. Design and experiment of shear-sucting mountain chrysanthemum picking machine. Trans. Chin. Soc. Agric. Mach. 2017, 48, 137–145. (In Chinese) [Google Scholar]
- Beier, K.; Ehlert, D. Methods for evaluation of picking performance of chamomile (Matricaria recutita L.) harvesters. J. Appl. Res. Med. Aromat. Plants 2014, 1, e1–e7. [Google Scholar]
- Kumhála, F.; Kavka, M.; Prošek, V. Capacitive throughput unit applied to stationary hop picking machine. Comput. Electron. Agric. 2013, 95, 92–97. [Google Scholar] [CrossRef]
- Zhai, D.; Zhai, W.; Zhai, Z. Hand-held Chrysanthemum Picker. CN201216069U, 8 April 2009. [Google Scholar]
- Cui, T.; Liu, J.; Zhang, D.; Shi, D. Flexible body simulation for corn stem based on ANSYS and ADAMS. Trans. Chin. Soc. Agric. Mach. 2012, 43 (Suppl. S1), 112–115. (In Chinese) [Google Scholar]
- Wang, F.; Li, F.; Zhang, P.; Zhang, L.; Wang, H. Design and experiment of double roller dried pepper pedicel removal device. Trans. Chin. Soc. Agric. Mach. 2024, 55, 191–199. (In Chinese) [Google Scholar]
- Lan, W. Research and experiment on harvesting straw during threshing before cutting. Fujian Agric. Mach. 1998, 39–43. (In Chinese) [Google Scholar]
- Pu, M.; Wu, J. Study on flexible sugarcane modeling based on ADAMS Software. J. Syst. Simul. 2009, 21, 1930–1932. (In Chinese) [Google Scholar]
- Sun, C.; Hu, C.; Hou, Q.; Pan, Z. Simulation of mechanical thinning flower actuator based on ADAMS. J. Agric. Mech. Res. 2015, 37, 70–73. (In Chinese) [Google Scholar]
- Chen, J. A Pilot Study About the Main Parameters of Hop Picking Machine. Master’s Thesis, Xin Jiang Agricultural University, Urumqi, China, 2011. (In Chinese). [Google Scholar]
- Zeng, H. Research on Hop’s Machine Pickability. Master’s Thesis, Xin Jiang Agricultural University, Urumqi, China, 2000. (In Chinese). [Google Scholar]
- Ehlert, D.; Adamek, R.; Giebel, A.; Horn, H.-J. Influence of comb parameters on picking properties for chamomile flowers (Matricaria recutita). Ind. Crops Prod. 2011, 33, 242–247. [Google Scholar] [CrossRef]
- Franke, R.; Schilcher, H. Relevance and Use of Chamomile (Matricaria recutita L.). Proceedings of the First International Symposium on Chamomile Research, Development and Production. Acta Hortic. 2007, 749, 29–43. [Google Scholar] [CrossRef]
- Lai, Q.; Yu, Q.; Dong, J. Dynamic analysis of rotary tiller gearbox based on EDEM, ADAMS and ANSYS. J. Intell. Fuzzy Syst. 2018, 36, 1153–1160. [Google Scholar] [CrossRef]
- Du, H.W.; Han, Z.Z. Design of Sweet Potato Vine Chopping and Returning Machine. Appl. Mech. Mater. 2014, 3064, 538. [Google Scholar] [CrossRef]
- Bajema, R.G.M.; Hyde, K. Peterson. Instrumentation design for dynamic axial compression of cylindrical tissue samples. Trans. ASAE 1998, 41, 747. [Google Scholar] [CrossRef]
- Derafshi, M.H. Design and Construction of a Pneumatic-thermal Machine for Controlling Colorado Potato Beetle (Leptinotarsa decemlineata). J. Appl. Sci. 2006, 6, 919–925. [Google Scholar]
- El Masry, G.; Cubero, S.; Molto, E.; Blasco, J. In-line sorting of irregular potatoes by using automated computer-based machine vision system. J. Food Eng. 2012, 112, 60–68. [Google Scholar] [CrossRef]





| Parameter Name | Unit | Range | Average Value | Standard Deviation |
|---|---|---|---|---|
| Flower Diameter | mm | 9.51–18.41 | 16.22 | 2.211 |
| Flower Stalk Diameter | mm | 0.95–1.98 | 1.40 | 0.276 |
| Flower Axis Diameter | mm | 1.12–2.84 | 2.96 | 0.656 |
| Small Branch Diameter | mm | 2.22–4.35 | 2.65 | 0.470 |
| Large Branch Diameter | mm | 2.26–5.04 | 3.70 | 0.277 |
| Flower Quality | g | 0.11–0.21 | 0.16 | 0.036 |
| Leaf Major Axis | mm | 8.73–16.17 | 13.0 | 5.123 |
| Leaf Minor Axis | mm | 4.57–8.32 | 6.40 | 4.126 |
| Flower Density | flowers/cm2 | 1.73–2.44 | 2.02 | 0.357 |
| Part | Maximum Rupture Force (N) | Maximum Shear Force (N) | Elastic Modulus (MPa) |
|---|---|---|---|
| Flower Stalk | 16.57 | 19.86 | 9.59 |
| Flower Axis | 49.00 | 20.65 | 10.17 |
| Small Branch | 25.80 | 29.99 | 10.54 |
| Parameter | Average Value |
|---|---|
| Poisson’s Ratio of Wild Chrysanthemum | 0.3 |
| Elastic Modulus of Flower Stem (MPa) | 10 |
| Coding | Factor | ||
|---|---|---|---|
| Critical Speed A (r/s) | Feeding Speed B (m/s) | Collision Angle C (°) | |
| 1 | 3.9632 | 1.5 | 90 |
| 0 | 3.20585 | 1.15 | 45 |
| −1 | 2.4485 | 0.8 | 0 |
| No. | Experiment Factors | Response Value | ||
|---|---|---|---|---|
| A | B | C | Critical Acceleration (m/s2) | |
| 1 | 1 | 0 | −1 | 3.657 |
| 2 | 1 | −1 | 0 | 3.705 |
| 3 | −1 | 0 | 1 | 1.802 |
| 4 | −1 | 1 | 0 | 3.341 |
| 5 | 0 | −1 | 1 | 2.515 |
| 6 | 0 | 0 | 0 | 3.568 |
| 7 | 0 | 1 | 1 | 2.716 |
| 8 | 0 | 0 | 0 | 3.403 |
| 9 | 0 | 0 | 0 | 3.601 |
| 10 | −1 | 0 | −1 | 2.802 |
| 11 | 1 | 1 | 0 | 3.912 |
| 12 | 0 | 0 | 0 | 3.666 |
| 13 | 0 | 0 | 0 | 3.543 |
| 14 | 0 | −1 | −1 | 3.515 |
| 15 | 1 | 0 | 0 | 2.057 |
| 16 | 0 | 1 | −1 | 3.716 |
| 17 | −1 | −1 | 0 | 2.701 |
| Coding | Factor | ||
|---|---|---|---|
| Picking Gap x1/(mm) | Bow Tooth Bending Angle x2/(°) | Feeding Rate x3/(kg·s−1) | |
| 1 | 100 | 60 | 3 |
| 0 | 75 | 45 | 2 |
| −1 | 50 | 30 | 1 |
| No. | Experiment Factors | R/% | ||
|---|---|---|---|---|
| A | B | C | ||
| 1 | 0 | 0 | 0 | 99.04 |
| 2 | 1 | 0 | 1 | 90.48 |
| 3 | 0 | −1 | −1 | 65.85 |
| 4 | 0 | 0 | 0 | 98.37 |
| 5 | 1 | 0 | −1 | 86.96 |
| 6 | −1 | 1 | 0 | 84.88 |
| 7 | 0 | 0 | 0 | 93.51 |
| 8 | −1 | 0 | −1 | 74.29 |
| 9 | 0 | 1 | −1 | 87.07 |
| 10 | 0 | 0 | 0 | 96.43 |
| 11 | 0 | 1 | 1 | 95.73 |
| 12 | −1 | −1 | 0 | 74.76 |
| 13 | 0 | 0 | 0 | 97.10 |
| 14 | 1 | 1 | 0 | 98.08 |
| 15 | 1 | −1 | 0 | 71.43 |
| 16 | −1 | 0 | 1 | 88.30 |
| 17 | 0 | −1 | 1 | 82.28 |
| Source of Variance | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| Model | 3.76 | 9 | 0.4175 | 4.86 | 0.0246 |
| A | 0.9863 | 1 | 0.9863 | 11.47 | 0.0117 |
| B | 0.2968 | 1 | 0.2968 | 3.45 | 0.1055 |
| C | 1.38 | 1 | 1.38 | 16.02 | 0.0052 |
| AB | 0.0002 | 1 | 0.0002 | 0.0024 | 0.9619 |
| AC | 0.065 | 1 | 0.065 | 0.7561 | 0.4134 |
| BC | 0.0072 | 1 | 0.0072 | 0.084 | 0.7803 |
| A2 | 0.4257 | 1 | 0.4257 | 4.95 | 0.0614 |
| B2 | 0.0147 | 1 | 0.0147 | 0.1706 | 0.692 |
| C2 | 0.5433 | 1 | 0.5433 | 6.32 | 0.0402 |
| Residual | 0.602 | 7 | 0.086 | ||
| Lack of Fit | 0.3985 | 3 | 0.1328 | 2.61 | 0.1883 |
| Pure Error | 0.2034 | 4 | 0.0509 | ||
| Total | 4.36 | 16 |
| Source of Variance | Sum of Squares | Degrees of Freedom | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| Model | 1717.11 | 9 | 190.79 | 45.6 | <0.0001 |
| A | 76.38 | 1 | 76.38 | 18.26 | 0.0037 |
| B | 637.96 | 1 | 637.96 | 152.47 | <0.0001 |
| C | 227.06 | 1 | 227.06 | 54.27 | 0.0002 |
| AB | 68.31 | 1 | 68.31 | 16.33 | 0.0049 |
| AC | 27.51 | 1 | 27.51 | 6.57 | 0.0373 |
| BC | 15.09 | 1 | 15.09 | 3.61 | 0.0993 |
| A2 | 159.97 | 1 | 159.97 | 38.23 | 0.0005 |
| B2 | 299.84 | 1 | 299.84 | 71.66 | <0.0001 |
| C2 | 137.7 | 1 | 137.7 | 32.91 | 0.0007 |
| Residual | 29.29 | 7 | 4.18 | ||
| Lack of Fit | 10.8 | 3 | 3.6 | 0.7783 | 0.5643 |
| Pure Error | 18.49 | 4 | 4.62 | ||
| Total | 1746.4 | 16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, Y.; Ma, Z.; Liu, S.; Liu, Z.; Yan, X.; Huang, Y. Design and Experiment of Opposed Roller-Type Picking Device for Chrysanthemum Indicum. Agriculture 2025, 15, 1147. https://doi.org/10.3390/agriculture15111147
Bai Y, Ma Z, Liu S, Liu Z, Yan X, Huang Y. Design and Experiment of Opposed Roller-Type Picking Device for Chrysanthemum Indicum. Agriculture. 2025; 15(11):1147. https://doi.org/10.3390/agriculture15111147
Chicago/Turabian StyleBai, Yiduo, Zhuanghong Ma, Suyuan Liu, Zhengdao Liu, Xiaoli Yan, and Yuxiang Huang. 2025. "Design and Experiment of Opposed Roller-Type Picking Device for Chrysanthemum Indicum" Agriculture 15, no. 11: 1147. https://doi.org/10.3390/agriculture15111147
APA StyleBai, Y., Ma, Z., Liu, S., Liu, Z., Yan, X., & Huang, Y. (2025). Design and Experiment of Opposed Roller-Type Picking Device for Chrysanthemum Indicum. Agriculture, 15(11), 1147. https://doi.org/10.3390/agriculture15111147






