A Universal Pharmacokinetic Model for Dexmedetomidine in Children and Adults
Abstract
1. Introduction
2. Methods
2.1. Data Sources
- Hannivoort Model: Hannivoort and colleagues [7] recruited 18 (9 male and 9 female) individuals 18–72 years old with BMI scores between 18 and 30 kg/m2. Dexmedetomidine was delivered using the Dyck model [22] targeting concentrations of 1, 2, 3, 4, 6, and 8 ng/mL after an initial infusion of 6 µg/kg/h for 10 s. Each step was maintained for 30 min. Blood samples for dexmedetomidine assay were obtained at 2 minutes after the initial drug infusion, before each increase in target concentration and at 2, 5, 10, 20, 60, and 120 min after the drug infusion stopped. A large local database [23] was used to sample 18 individuals representative of the demographics in the Hannivoort population. Simulated predicted concentrations in these 18 individuals given 2 mcg/kg loading dose over 10 min followed by infusion 1 mcg/kg for 2 h were used to develop the universal model.
- Potts Model: Potts and colleagues [12] recruited 45 children (22 males and 23 females) after cardiac surgery. Dexmedetomidine was administered (1–4 µg/kg) over 10 min. Three to four blood samples were obtained in the first 30 min after infusion. Samples were obtained at 1–2, 3–4, and 6–10 h thereafter. These data were pooled with two other PK studies (n = 34) of dexmedetomidine [24,25]. These studies are summarised in Supplementary Materials Table S1.
- Cortinez Model: Cortinez and colleagues [16] recruited 20 obese (BMI >35 kg/m2) and 20 non-obese individuals (BMI 18.5–30 kg/m2, 18–60 years old), undergoing elective laparoscopic surgery. Dexmedetomidine 0.5 µg/kg was given to all participants for 10 min. Subsequently, participants were randomised to two infusion regimens: 0.25 or 0.5 µg/kg/h. Doses were based on total body weight (TBW). Blood samples were obtained at 2, 5, 10, 15, 20, 30, 45, 60, 90, and 120 min during dexmedetomidine infusion and at 0, 2, 5, 10, 20, 30, 60, 90, 120, 240, and 360 min after the infusion was stopped.
- Rolle Model: This study enrolled 40 adults (age 18 to 60 years, weights 47 to 126 kg, BMI 18–49 kg/m2) scheduled for abdominal laparoscopic surgery [17]. Dexmedetomidine bolus of 0.5 mcg/kg over 10 min was followed by an infusion of 0.5 mcg/kg/h. Venous blood samples were drawn at 0, 5, 10, 20, 30, 45, 60 min after the start of dexmedetomidine administration and thereafter every 30 min during anaesthesia maintenance. Once dexmedetomidine infusion was stopped at the end of surgery, samples were drawn at the end of dexmedetomidine infusion, and then 5, 10, 20, 30, 60, 90, 120, 240, 360 min, with a last sample between 720 and 1200 min.
- Talke Model: Talke and colleagues recruited 10 healthy individuals (21–36 years old and 52–89 kg) [26]. Dexmedetomidine 4 µg/mL was administered for 15 min to target a plasma concentration of 0.3 ng/ml. Blood samples were obtained at 1, 2, 3, 4, 5, 7.5, 10, and 15 min during drug infusion and 15, 30, and 60 min after the end of the infusion.
2.2. Hannivoort Model Performance in Children Older Than 1 Year
2.3. Pooled Data Analysis
2.3.1. Pharmacokinetic Analyses
2.3.2. Covariate Analysis for Age and Size
2.3.3. Model Selection
2.4. Model Simulation
3. Results
4. Discussion
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Anderson, B.J.; Bagshaw, O. Practicalities of total intravenous anesthesia and target-controlled infusion in children. Anesthesiology 2019, 131, 164–185. [Google Scholar] [CrossRef] [PubMed]
- Schnider, T.W.; Minto, C.F.; Gambus, P.L.; Andresen, C.; Goodale, D.B.; Shafer, S.L.; Youngs, E.J. The influence of method of administration and covariates on the pharmacokinetics of propofol in adult volunteers. Anesthesiology 1998, 88, 1170–1182. [Google Scholar] [CrossRef] [PubMed]
- Gray, J.M.; Kenny, G.N. Development of the technology for ‘Diprifusor’ TCI systems. Anaesthesia 1998, 53 (Suppl 1), 22–27. [Google Scholar] [CrossRef]
- Minto, C.F.; Schnider, T.W.; Egan, T.D.; Youngs, E.; Lemmens, H.J.; Gambus, P.L.; Billard, V.; Hoke, J.F.; Moore, K.H.; Hermann, D.J.; et al. Influence of age and gender on the pharmacokinetics and pharmacodynamics of remifentanil. I. Model development. Anesthesiology 1997, 86, 10–23. [Google Scholar] [CrossRef] [PubMed]
- Gepts, E.; Shafer, S.L.; Camu, F.; Stanski, D.R.; Woestenborghs, R.; Van Peer, A.; Heykants, J.J. Linearity of pharmacokinetics and model estimation of sufentanil. Anesthesiology 1995, 83, 1194–1204. [Google Scholar] [CrossRef] [PubMed]
- Maitre, P.O.; Ausems, M.E.; Vozeh, S.; Stanski, D.R. Evaluating the accuracy of using population pharmacokinetic data to predict plasma concentrations of alfentanil. Anesthesiology 1988, 68, 59–67. [Google Scholar] [CrossRef] [PubMed]
- Hannivoort, L.N.; Eleveld, D.J.; Proost, J.H.; Reyntjens, K.M.; Absalom, A.R.; Vereecke, H.E.; Struys, M.M. Development of an Optimized Pharmacokinetic Model of Dexmedetomidine Using Target-controlled Infusion in Healthy Volunteers. Anesthesiology 2015, 123, 357–367. [Google Scholar] [CrossRef]
- Ihmsen, H.; Geisslinger, G.; Schuttler, J. Stereoselective pharmacokinetics of ketamine: R(-)-ketamine inhibits the elimination of S(+)-ketamine. Clin. Pharmacol. Ther. 2001, 70, 431–438. [Google Scholar] [CrossRef]
- Kataria, B.K.; Ved, S.A.; Nicodemus, H.F.; Hoy, G.R.; Lea, D.; Dubois, M.Y.; Mandema, J.W.; Shafer, S.L. The pharmacokinetics of propofol in children using three different data analysis approaches. Anesthesiology 1994, 80, 104–122. [Google Scholar] [CrossRef]
- Marsh, B.; White, M.; Morton, N.; Kenny, G.N. Pharmacokinetic model driven infusion of propofol in children. Brit. J. Anaesth. 1991, 67, 41–48. [Google Scholar] [CrossRef]
- Rigby-Jones, A.E.; Priston, M.J.; Sneyd, J.R.; McCabe, A.P.; Davis, G.I.; Tooley, M.A.; Thorne, G.C.; Wolf, A.R. Remifentanil-midazolam sedation for paediatric patients receiving mechanical ventilation after cardiac surgery. Brit. J. Anaesth. 2007, 99, 252–261. [Google Scholar] [CrossRef] [PubMed]
- Potts, A.L.; Warman, G.R.; Anderson, B.J. Dexmedetomidine disposition in children: A population analysis. Pediatr. Anesth. 2008, 18, 722–730. [Google Scholar] [CrossRef]
- Ross, A.K.; Davis, P.J.; Dear, G.D.; Ginsberg, B.; McGowan, F.X.; Stiller, R.D.; Henson, L.G.; Huffman, C.; Muir, K.T. Pharmacokinetics of remifentanil in anesthetized pediatric patients undergoing elective surgery or diagnostic procedures. Anesth. Analg. 2001, 93, 1393–1401. [Google Scholar] [CrossRef] [PubMed]
- Eleveld, D.J.; Proost, J.H.; Cortinez, L.I.; Absalom, A.R.; Struys, M.M. A general purpose pharmacokinetic model for propofol. Anesth. Analg. 2014, 118, 1221–1237. [Google Scholar] [CrossRef]
- Eleveld, D.J.; Proost, J.H.; Vereecke, H.; Absalom, A.R.; Olofsen, E.; Vuyk, J.; Struys, M. An allometric model of remifentanil pharmacokinetics and pharmacodynamics. Anesthesiology 2017, 126, 1005–1018. [Google Scholar] [CrossRef] [PubMed]
- Cortinez, L.I.; Anderson, B.J.; Holford, N.H.; Puga, V.; de la Fuente, N.; Auad, H.; Solari, S.; Allende, F.A.; Ibacache, M. Dexmedetomidine pharmacokinetics in the obese. Eur. J. Clin. Pharmacol. 2015, 71, 1501–1508. [Google Scholar] [CrossRef] [PubMed]
- Rolle, A.; Paredes, S.; Cortinez, L.I.; Anderson, B.J.; Quezada, N.; Solari, S.; Allende, F.; Torres, J.; Cabrera, D.; Contreras, V.; et al. Dexmedetomidine metabolic clearance is not affected by fat mass in obese patients. Br. J. Anaesth. 2018, 120, 969–977. [Google Scholar] [CrossRef]
- Potts, A.L.; Anderson, B.J.; Holford, N.H.; Vu, T.C.; Warman, G.R. Dexmedetomidine hemodynamics in children after cardiac surgery. Pediatr. Anesth. 2010, 20, 425–433. [Google Scholar] [CrossRef]
- Potts, A.L.; Anderson, B.J.; Warman, G.R.; Lerman, J.; Diaz, S.M.; Vilo, S. Dexmedetomidine pharmacokinetics in pediatric intensive care—A pooled analysis. Pediatr. Anesth. 2009, 19, 1119–1129. [Google Scholar] [CrossRef]
- Holford, N.; Heo, Y.A.; Anderson, B. A pharmacokinetic standard for babies and adults. J. Pharm. Sci. 2013, 102, 2941–2952. [Google Scholar] [CrossRef]
- Holford, N.H.G.; Anderson, B.J. Allometric size: The scientific theory and extension to normal fat mass. Eur. J. Pharm. Sci. 2017, 109S, S59–S64. [Google Scholar] [CrossRef]
- Dyck, J.B.M.D.; Maze, M.; Haack, C.; Vuorilehto, L.; Shafer, S.L. The Pharmacokinetics and Hemodynamic Effects of Intravenous and Intramuscular Dexmedetomidine Hydrochloride in Adult Human Volunteers. Anesthesiology 1993, 78, 813–820. [Google Scholar] [CrossRef] [PubMed]
- Sumpter, A.L.; Holford, N.H.G. Predicting weight using postmenstrual age–neonates to adults. Pediatr. Anesth. 2011, 21, 309–315. [Google Scholar] [CrossRef]
- Petroz, G.C.; Sikich, N.; James, M.; van Dyk, H.; Shafer, S.L.; Schily, M.; Lerman, J. A phase I, two-center study of the pharmacokinetics and pharmacodynamics of dexmedetomidine in children. Anesthesiology 2006, 105, 1098–1110. [Google Scholar] [CrossRef] [PubMed]
- Vilo, S.; Rautiainen, P.; Kaisti, K.; Aantaa, R.; Scheinin, M.; Manner, T.; Olkkola, K.T. Pharmacokinetics of intravenous dexmedetomidine in children under 11 yr of age. Br. J. Anaesth. 2008, 100, 697–700. [Google Scholar] [CrossRef] [PubMed]
- Talke, P.; Anderson, B.J. Pharmacokinetics and pharmacodynamics of dexmedetomidine-induced vasoconstriction in healthy volunteers. Br. J. Clin. Pharmacol. 2018, 84, 1364–1372. [Google Scholar] [CrossRef] [PubMed]
- Sheiner, L.B.; Beal, S.L.J. Some suggestions for measuring predictive performance. J. Pharmacokinet. Biopharm. 1981, 9, 503–512. [Google Scholar] [CrossRef]
- Cortinez, L.I.; Anderson, B.J.; Penna, A.; Olivares, L.; Munoz, H.R.; Holford, N.H.; Struys, M.M.; Sepulveda, P. Influence of obesity on propofol pharmacokinetics: Derivation of a pharmacokinetic model. Brit. J. Anaesth. 2010, 105, 448–456. [Google Scholar] [CrossRef]
- Anderson, B.J.; Holford, N.H. What is the best size predictor for dose in the obese child? Pediatr. Anesth. 2017, 27, 1176–1184. [Google Scholar] [CrossRef]
- Anderson, B.J.; Meakin, G.H. Scaling for size: Some implications for paediatric anaesthesia dosing. Pediatr. Anesth. 2002, 12, 205–219. [Google Scholar] [CrossRef]
- Anderson, B.J.; Holford, N.H. Mechanism-based concepts of size and maturity in pharmacokinetics. Annu. Rev. Pharmacol. Toxicol. 2008, 48, 303–332. [Google Scholar] [CrossRef] [PubMed]
- Holford, N.H. A size standard for pharmacokinetics. Clin. Pharmacokinet. 1996, 30, 329–332. [Google Scholar] [CrossRef] [PubMed]
- Al-Sallami, H.S.; Goulding, A.; Grant, A.; Taylor, R.; Holford, N.; Duffull, S.B. Prediction of Fat-Free Mass in Children. Clin. Pharmacokinet. 2015, 54, 1169–1178. [Google Scholar] [CrossRef]
- Janmahasatian, S.; Duffull, S.B.; Ash, S.; Ward, L.C.; Byrne, N.M.; Green, B. Quantification of lean bodyweight. Clin. Pharmacokinet. 2005, 44, 1051–1065. [Google Scholar] [CrossRef]
- Anderson, B.J.; Holford, N.H.G. Mechanistic basis of using body size and maturation to predict clearance in humans. Drug Metab. Pharmacokinet. 2009, 24, 25–36. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, T.H.; Mouksassi, M.S.; Holford, N.; Al-Huniti, N.; Freedman, I.; Hooker, A.C.; John, J.; Karlsson, M.O.; Mould, D.R.; Perez Ruixo, J.J.; et al. Model Evaluation of Continuous Data Pharmacometric Models: Metrics and Graphics. CPT Pharmacometrics Syst. Pharmacol. 2017, 6, 87–109. [Google Scholar] [CrossRef]
- Efron, B.; Tibshirani, R. Bootstrap methods for standard errors, confidence intervals, and other measures of statistical accuracy. Stat. Sci. 1986, 1, 54–77. [Google Scholar] [CrossRef]
- Hsu, Y.W.; Cortinez, L.I.; Robertson, K.M.; Keifer, J.C.; Sum-Ping, S.T.; Moretti, E.W.; Young, C.C.; Wright, D.R.; Macleod, D.B.; Somma, J. Dexmedetomidine pharmacodynamics: Part I: Crossover comparison of the respiratory effects of dexmedetomidine and remifentanil in healthy volunteers. Anesthesiology 2004, 101, 1066–1076. [Google Scholar] [CrossRef]
- Li, A.; Yuen, V.M.; Goulay-Dufay, S.; Sheng, Y.; Standing, J.F.; Kwok, P.C.L.; Leung, M.K.M.; Leung, A.S.; Wong, I.C.K.; Irwin, M.G. Pharmacokinetic and pharmacodynamic study of intranasal and intravenous dexmedetomidine. Br. J. Anaesth. 2018, 120, 960–968. [Google Scholar] [CrossRef]
- Colin, P.J.; Hannivoort, L.N.; Eleveld, D.J.; Reyntjens, K.; Absalom, A.R.; Vereecke, H.E.M.; Struys, M. Dexmedetomidine pharmacokinetic-pharmacodynamic modelling in healthy volunteers: 1. Influence of arousal on bispectral index and sedation. Br. J. Anaesth. 2017, 119, 200–210. [Google Scholar] [CrossRef]
- Bhana, N.; Goa, K.L.; McClellan, K.J. Dexmedetomidine. Drugs 2000, 59, 263–268. [Google Scholar] [CrossRef] [PubMed]
- Mantz, J. Dexmedetomidine. Drugs. Today 1999, 35, 151–157. [Google Scholar] [CrossRef] [PubMed]
- Dyck, J.B.; Maze, M.; Haack, C.; Azarnoff, D.L.; Vuorilehto, L.; Shafer, S.L. Computer-controlled infusion of intravenous dexmedetomidine hydrochloride in adult human volunteers. Anesthesiology 1993, 78, 821–828. [Google Scholar] [CrossRef] [PubMed]
- Dutta, S.; Karol, M.D.; Cohen, T.; Jones, R.M.; Mant, T. Effect of dexmedetomidine on propofol requirements in healthy subjects. J. Pharm. Sci. 2001, 90, 172–181. [Google Scholar] [CrossRef]
- Talke, P.; Richardson, C.A.; Scheinin, M.; Fisher, D.M. Postoperative pharmacokinetics and sympatholytic effects of dexmedetomidine. Anesth. Analg. 1997, 85, 1136–1142. [Google Scholar] [CrossRef]
- Lin, L.; Guo, X.; Zhang, M.Z.; Qu, C.J.; Sun, Y.; Bai, J. Pharmacokinetics of dexmedetomidine in Chinese post-surgical intensive care unit patients. Acta Anaesthesiol. Scand. 2011, 55, 359–367. [Google Scholar] [CrossRef] [PubMed]
- Valitalo, P.A.; Ahtola-Satila, T.; Wighton, A.; Sarapohja, T.; Pohjanjousi, P.; Garratt, C. Population pharmacokinetics of dexmedetomidine in critically ill patients. Clin. Drug Investig. 2013, 33, 579–587. [Google Scholar] [CrossRef]
- Perez-Guille, M.G.; Toledo-Lopez, A.; Rivera-Espinosa, L.; Alemon-Medina, R.; Murata, C.; Lares-Asseff, I.; Chavez-Pacheco, J.L.; Gomez-Garduno, J.; Zamora Gutierrez, A.L.; Orozco-Galicia, C.; et al. Population pharmacokinetics and pharmacodynamics of dexmedetomidine in children undergoing ambulatory surgery. Anesth. Analg. 2018, 127, 716–723. [Google Scholar] [CrossRef]
- Su, F.; Nicolson, S.C.; Gastonguay, M.R.; Barrett, J.S.; Adamson, P.C.; Kang, D.S.; Godinez, R.I.; Zuppa, A.F. Population pharmacokinetics of dexmedetomidine in infants after open heart surgery. Anesth. Analg. 2010, 110, 1383–1392. [Google Scholar] [CrossRef] [PubMed]
- Chrysostomou, C.; Schulman, S.R.; Herrera Castellanos, M.; Cofer, B.E.; Mitra, S.; da Rocha, M.G.; Wisemandle, W.A.; Gramlich, L. A phase II/III, multicenter, safety, efficacy, and pharmacokinetic study of dexmedetomidine in preterm and term neonates. J. Pediatr. 2014, 164, 276–282. [Google Scholar] [CrossRef]
- Greenberg, R.G.; Wu, H.; Laughon, M.; Capparelli, E.; Rowe, S.; Zimmerman, K.O.; Smith, P.B.; Cohen-Wolkowiez, M. Population Pharmacokinetics of Dexmedetomidine in Infants. J. Clin. Pharmacol. 2017, 57, 1174–1182. [Google Scholar] [CrossRef] [PubMed]
- Weerink, M.A.S.; Struys, M.; Hannivoort, L.N.; Barends, C.R.M.; Absalom, A.R.; Colin, P. Clinical pharmacokinetics and pharmacodynamics of dexmedetomidine. Clin. Pharmacokinet. 2017, 56, 893–913. [Google Scholar] [CrossRef] [PubMed]


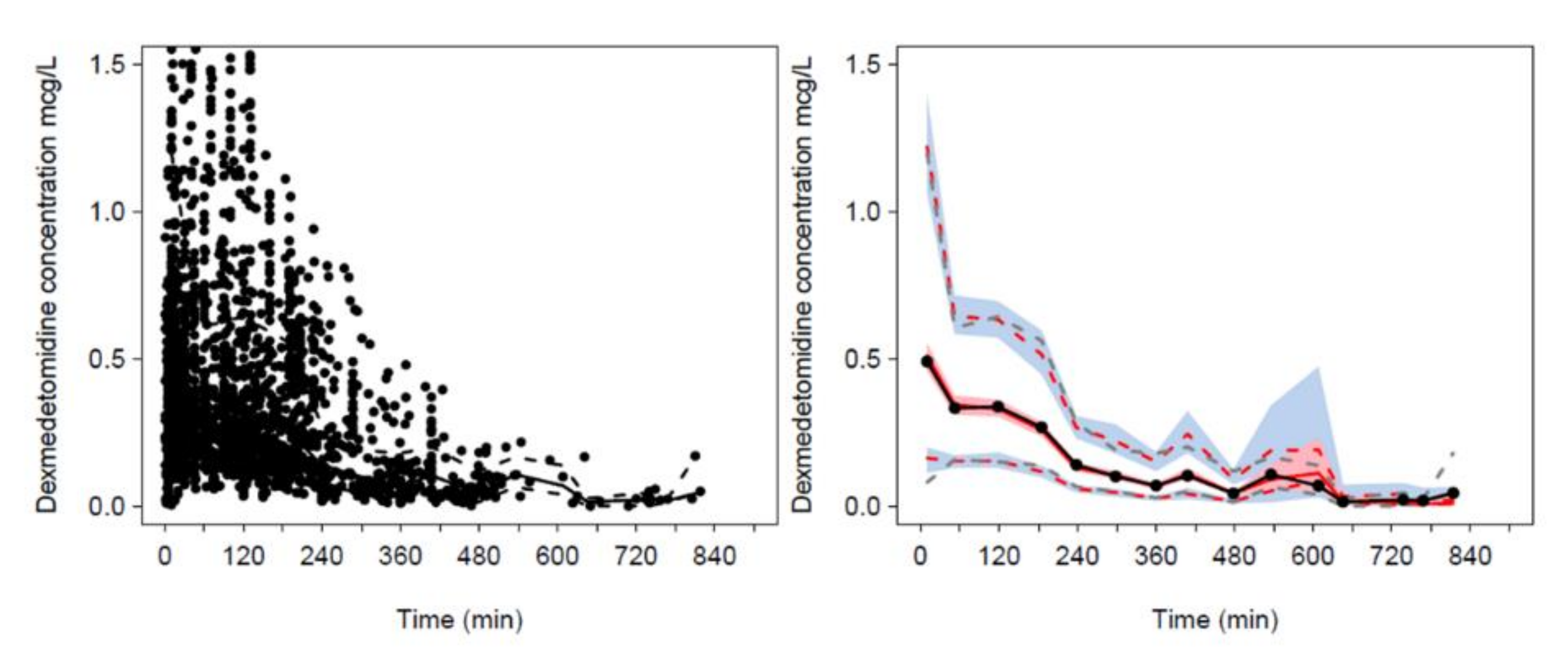
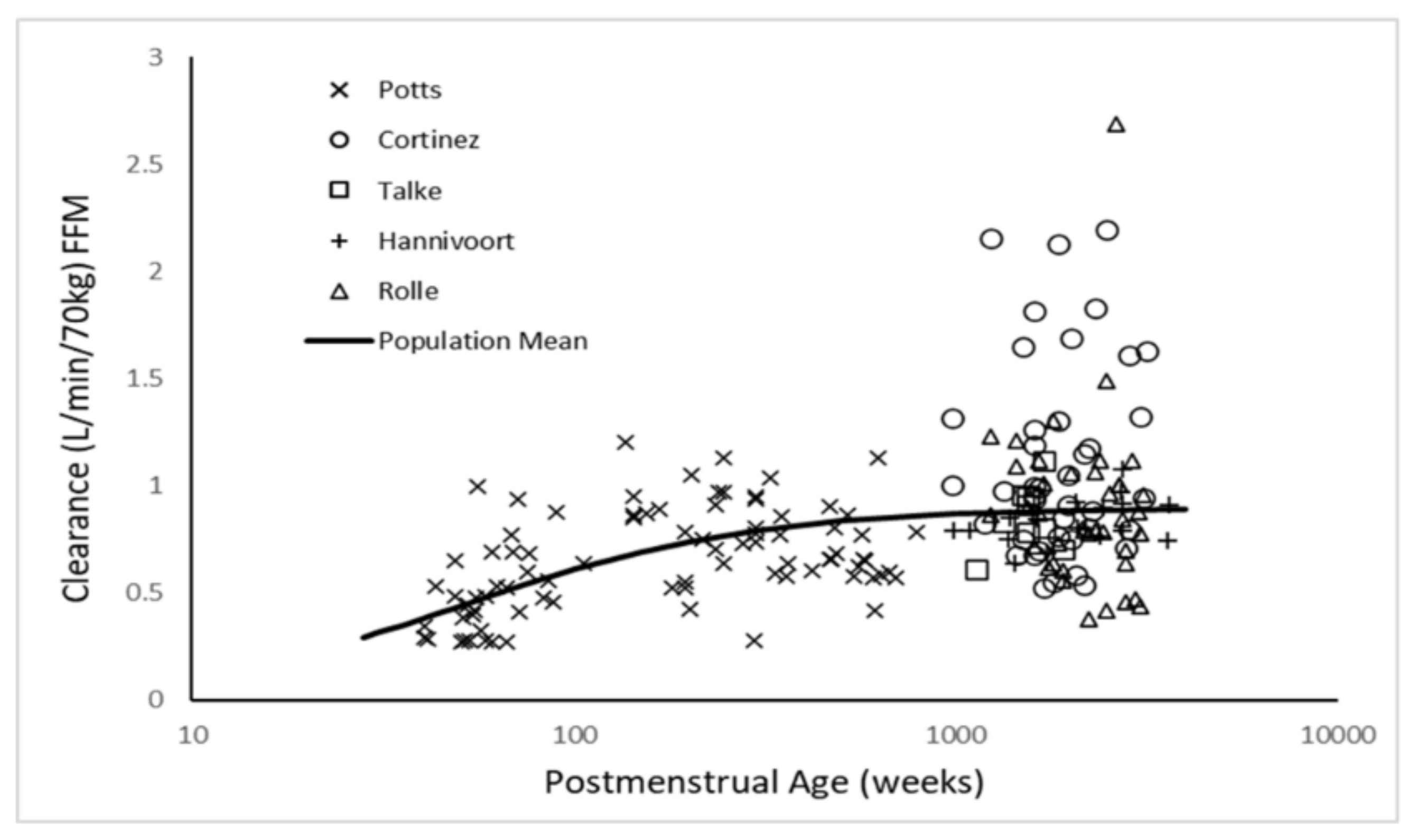
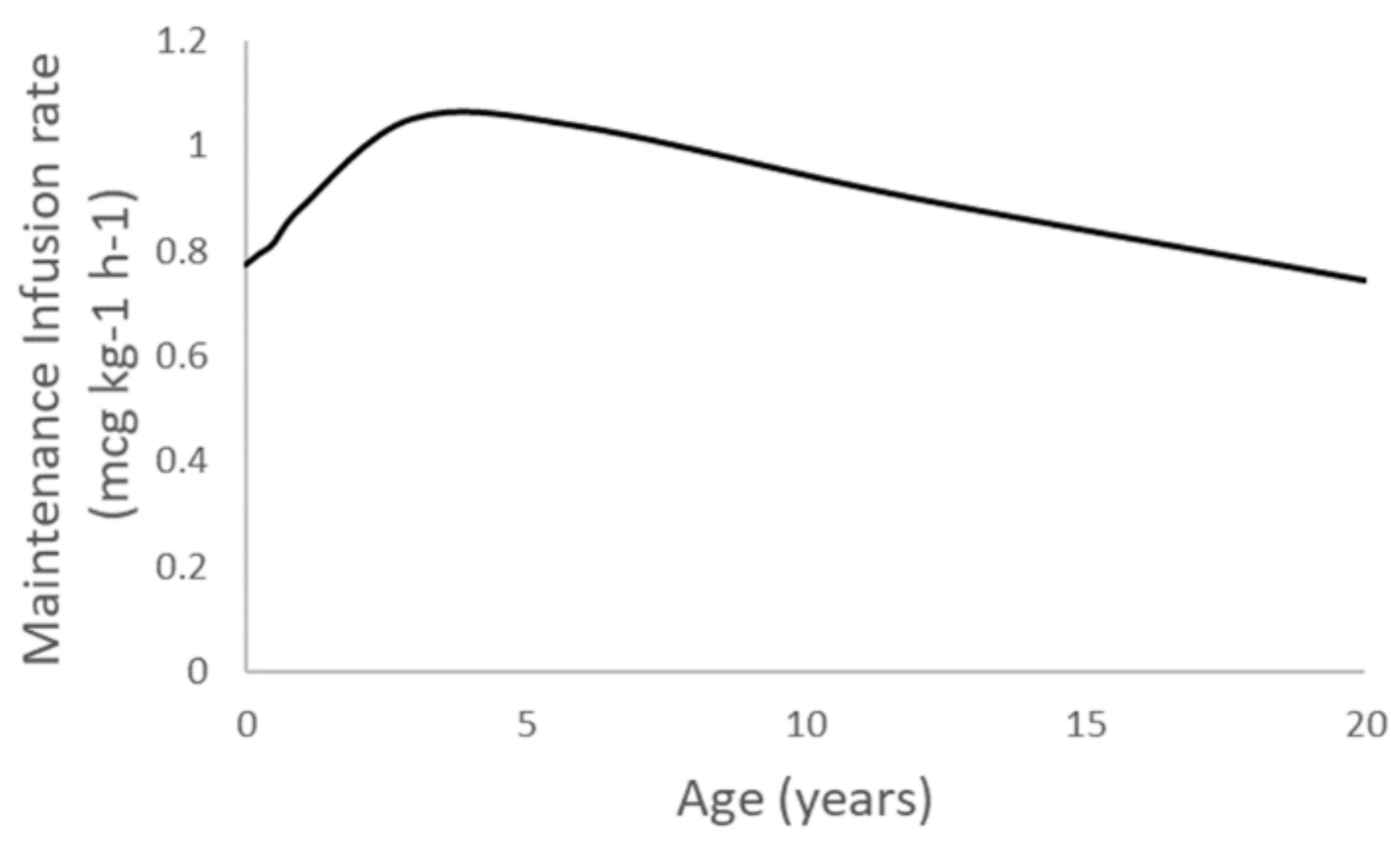
| Parameter | Estimate | PPV (%) | 95% CI | Sh% |
|---|---|---|---|---|
| V1 (L/70 kg) | 25.2 | 103.9 | 20.9, 31.3 | 16.4 |
| V2 (L/70kg) | 34.4 | 41.8 | 24.3, 44.2 | 15.5 |
| V3 (L/70 kg) | 65.4 | 61.6 | 53.4, 74.5 | 8.4 |
| CL (L/min/70 kg) | 0.897 | 35.8 | 0.81, 1.02 | 4.1 |
| Q2 (L/min/70kg) | 1.68 | 63.2 | 1.22, 1.97 | 12.5 |
| Q3 (L/min/70 kg) | 0.62 | 89.7 | 0.45, 0.83 | 21.4 |
| FFATV | 0.293 | - | 0.13, 0.55 | - |
| FFATCL | 0 FIX | - | - | - |
| TM50 | 52.4 | - | 43.5, 68.8 | - |
| Hill | 1 FIX | - | - | - |
| Additive Residual Error (µg/mL) | 0.004 | ηRUV 0.32 | - | |
| Proportional Residual Error (%) | 0.19 | - | 0.18, 0.20 |
| Sequential Nested Model | PPVt2 | BSVR2 | BSVP2 | BSVP2/PPVt2 |
|---|---|---|---|---|
| Clearance | ||||
| no covariates | 0.861 * | 0.861 * | 0 | 0 |
| TBW with allometric scaling (EXP = 3/4) | 0.861 * | 0.140 | 0.721 | 0.838 |
| TBW with PMA on CL | 0.861 * | 0.136 | 0.725 | 0.842 |
| FFM with PMA on CL | 0.861 * | 0.114 | 0.747 | 0.867 |
| Central compartment (V1) | ||||
| no covariates | 1.5 * | 1.5* | 0 | 0 |
| TBW allometric scaling (EXP = 1) | 1.5 * | 1.02 | 0.48 | 0.320 |
| Peripheral compartment (V2) | ||||
| no covariates | 1.46 * | 1.46* | 0 | 0 |
| TBW allometric scaling (EXP = 1) | 1.46 * | 0.25 | 1.209 | 0.823 |
| Age | Weight (kg) | Height (cm) | Clearance (L/min) | Loading Dose (mcg/kg) | Maintenance (mcg/kg/h) |
|---|---|---|---|---|---|
| Term neonate | 3.6 | 50 | 0.05 | 0.40 | 0.77 |
| 3 months | 6 | 62 | 0.08 | 0.38 | 0.80 |
| 6 months | 7.8 | 67 | 0.11 | 0.37 | 0.81 |
| 1 year | 10 | 75 | 0.15 | 0.37 | 0.88 |
| 3 years | 14 | 95 | 0.25 | 0.40 | 1.04 |
| 6 years | 21 | 115 | 0.36 | 0.39 | 1.02 |
| 12 years | 40 | 149 | 0.60 | 0.35 | 0.90 |
| 20 years | 70 | 175 | 0.87 | 0.31 | 0.75 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morse, J.D.; Cortinez, L.I.; Anderson, B.J. A Universal Pharmacokinetic Model for Dexmedetomidine in Children and Adults. J. Clin. Med. 2020, 9, 3480. https://doi.org/10.3390/jcm9113480
Morse JD, Cortinez LI, Anderson BJ. A Universal Pharmacokinetic Model for Dexmedetomidine in Children and Adults. Journal of Clinical Medicine. 2020; 9(11):3480. https://doi.org/10.3390/jcm9113480
Chicago/Turabian StyleMorse, James D., L. Ignacio Cortinez, and Brian J. Anderson. 2020. "A Universal Pharmacokinetic Model for Dexmedetomidine in Children and Adults" Journal of Clinical Medicine 9, no. 11: 3480. https://doi.org/10.3390/jcm9113480
APA StyleMorse, J. D., Cortinez, L. I., & Anderson, B. J. (2020). A Universal Pharmacokinetic Model for Dexmedetomidine in Children and Adults. Journal of Clinical Medicine, 9(11), 3480. https://doi.org/10.3390/jcm9113480





