Modeling and Design Optimization of Multifunctional Membrane Reactors for Direct Methane Aromatization
Abstract
:1. Introduction
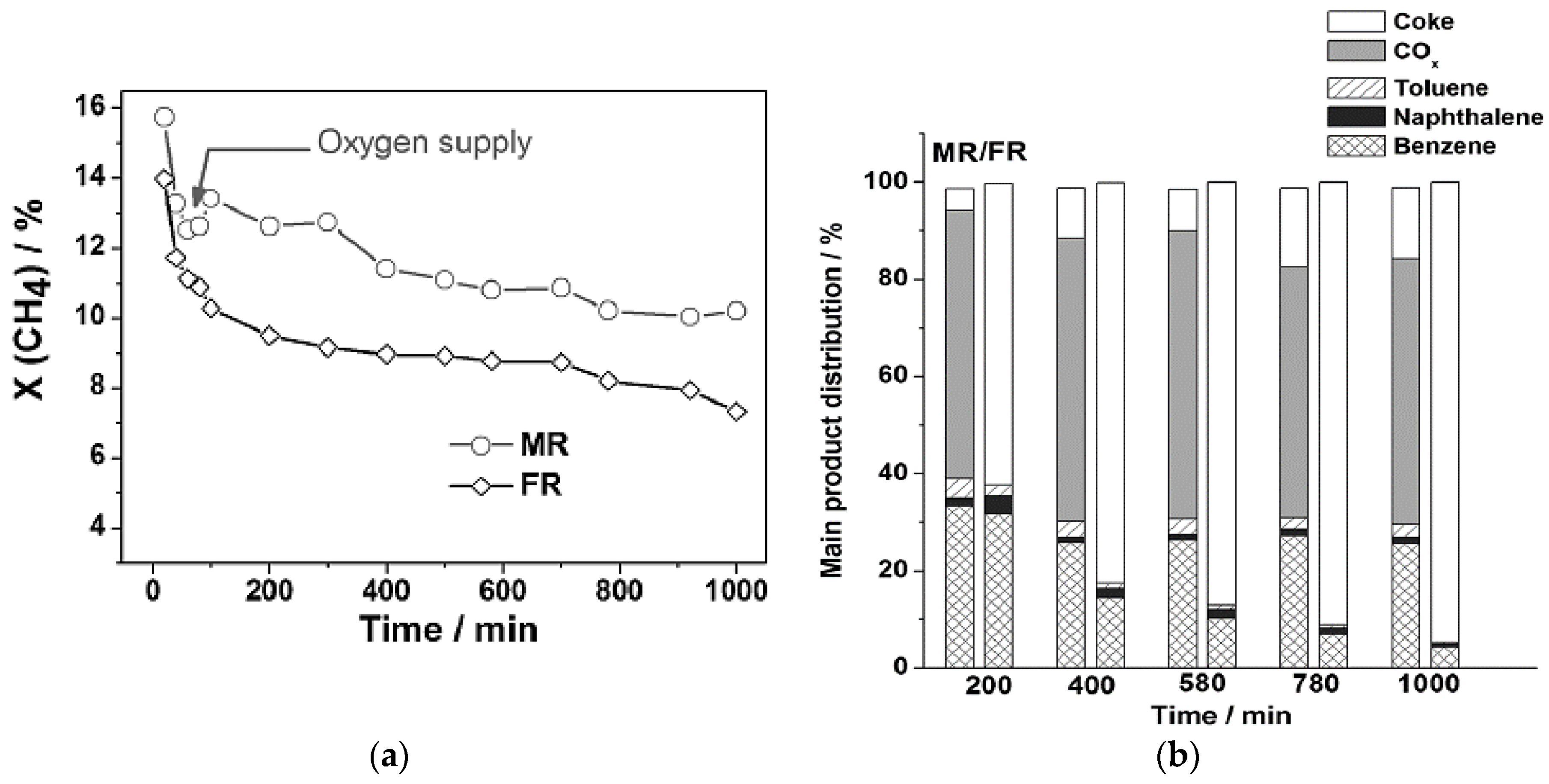
2. Literature Review
3. Background
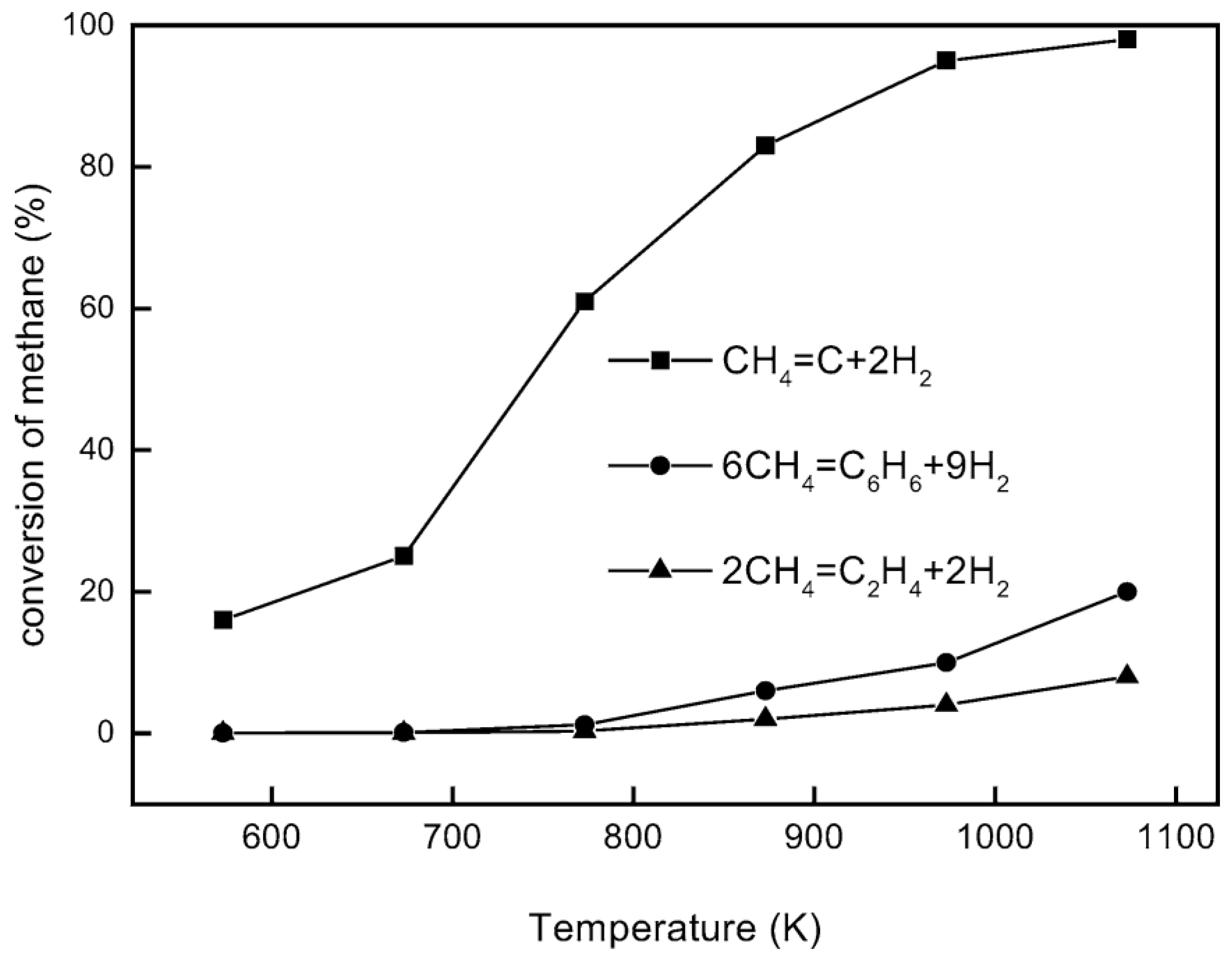
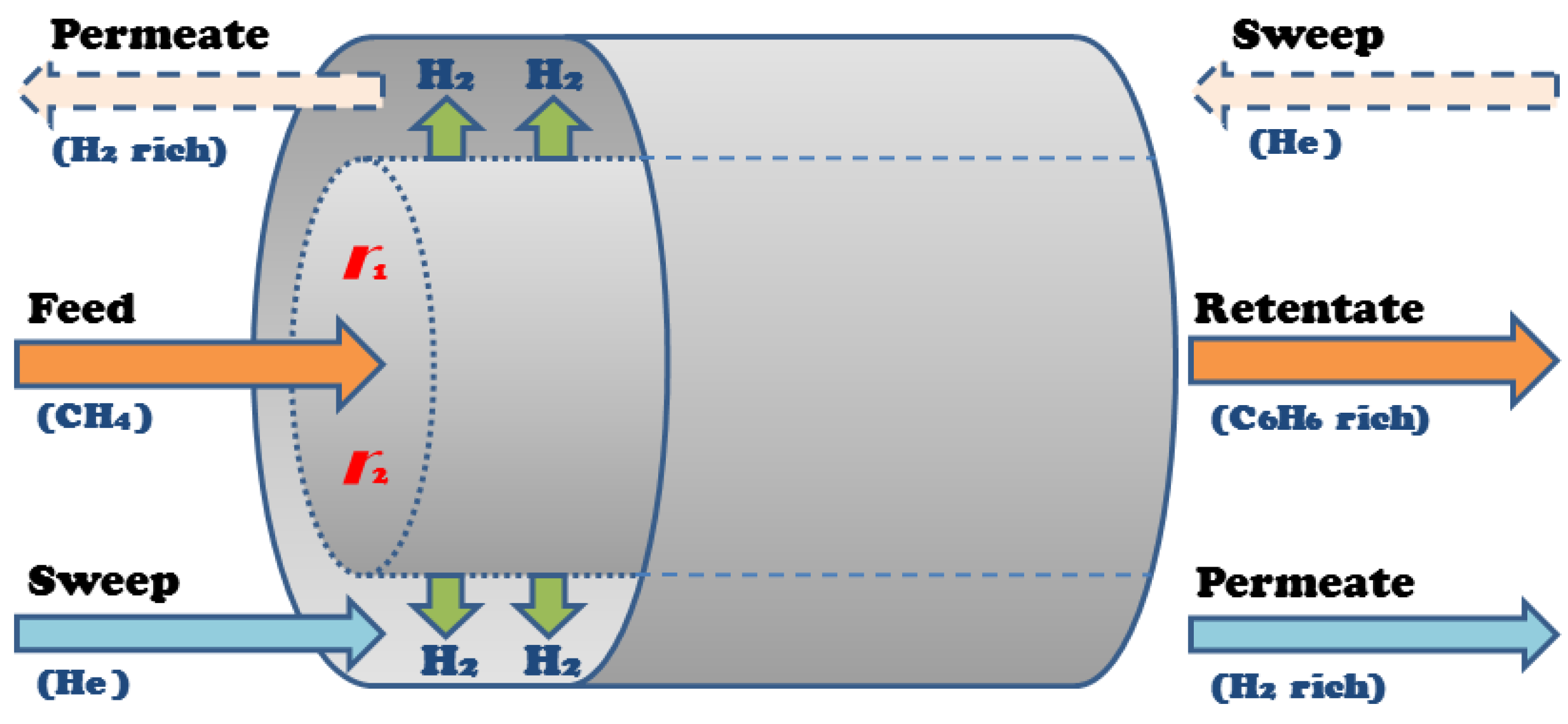
3.1. DMA Membrane Reactor Model
3.2. Role of Selective Oxidation
4. Proposed Approach
4.1. Reaction Modeling
4.2. Membrane Modeling
4.3. Simulation and Optimization Setup
5. Results and Discussion
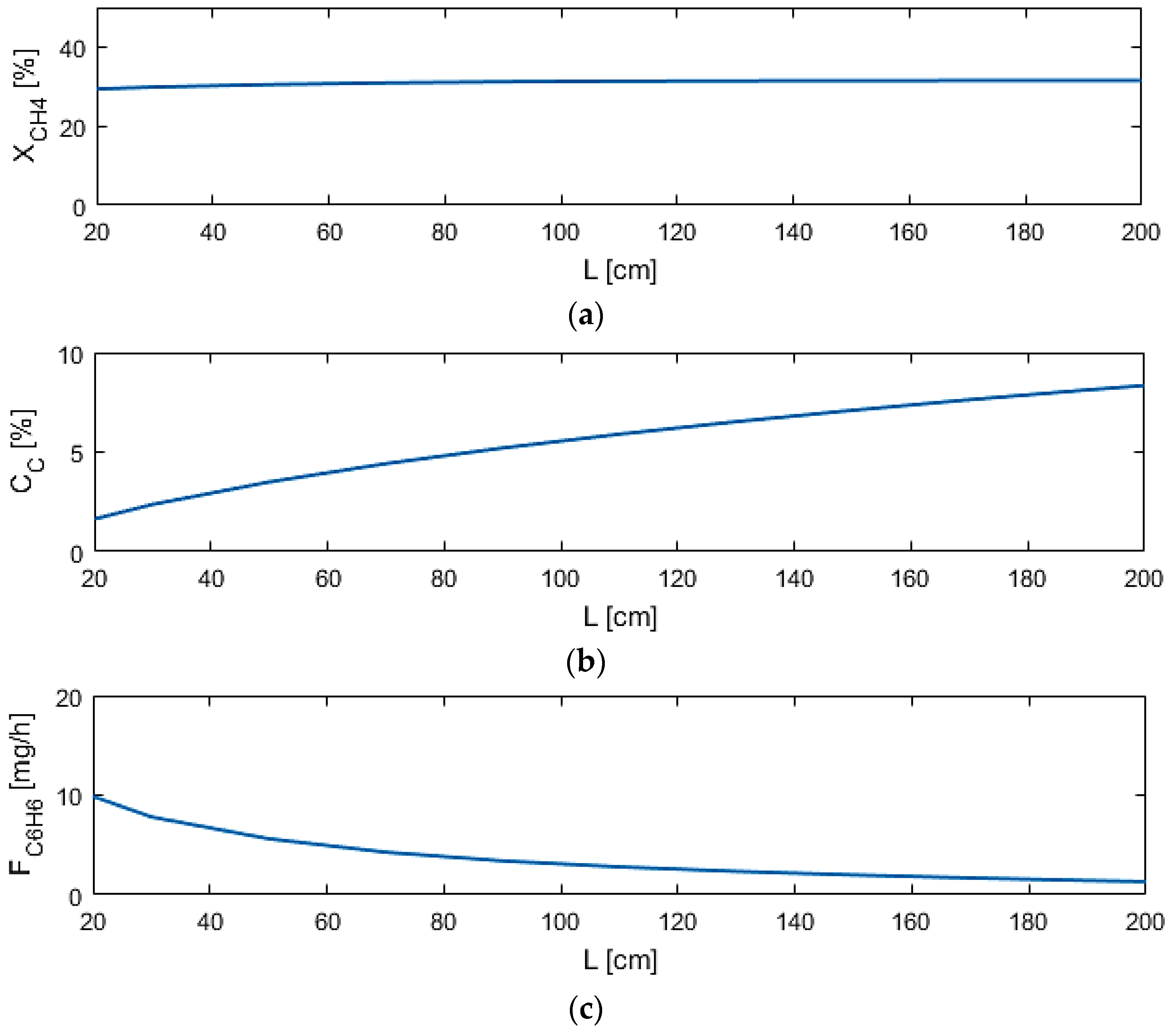
5.1. Base Case Performance Studies
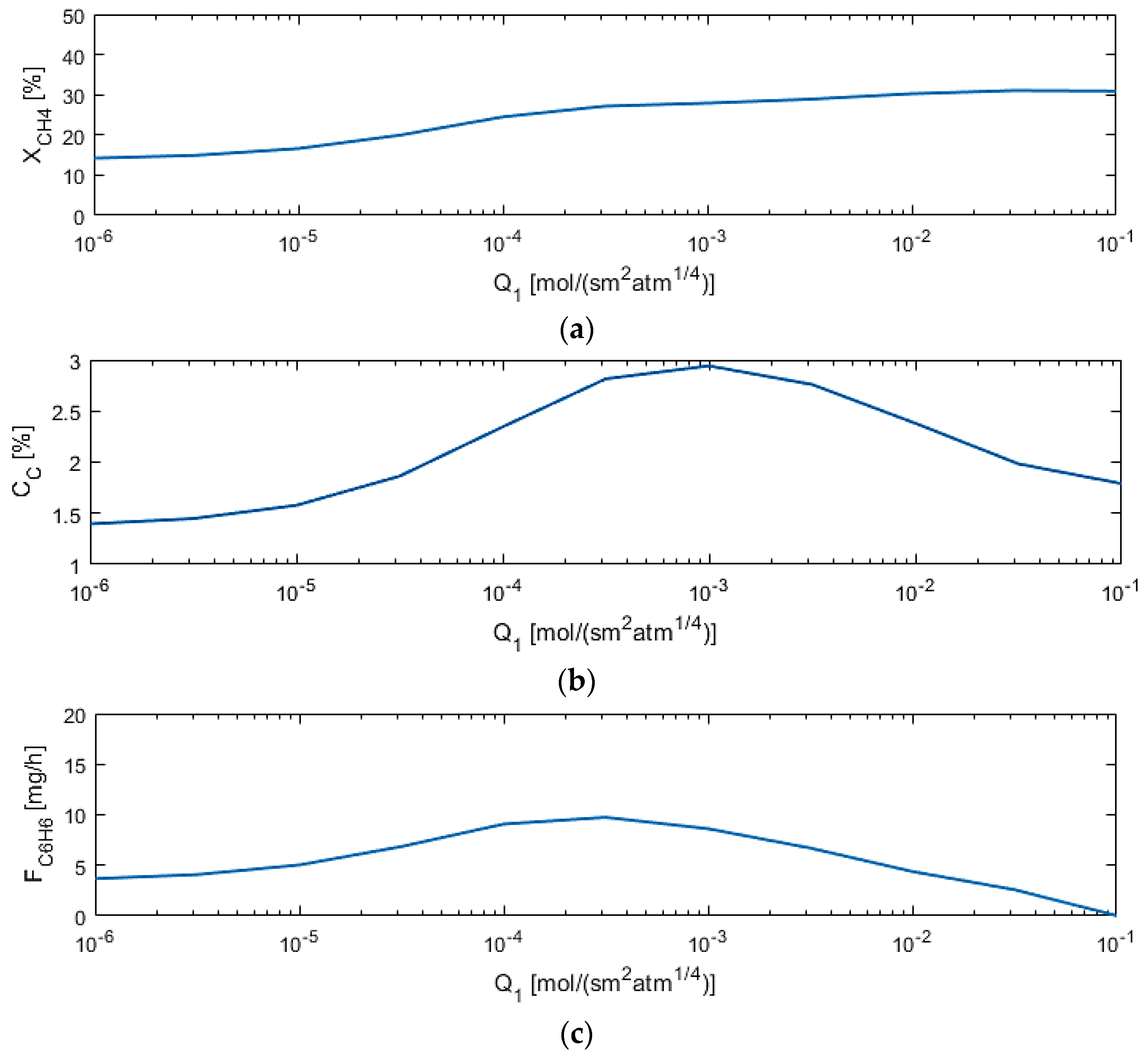
5.2. Sensitivity Studies
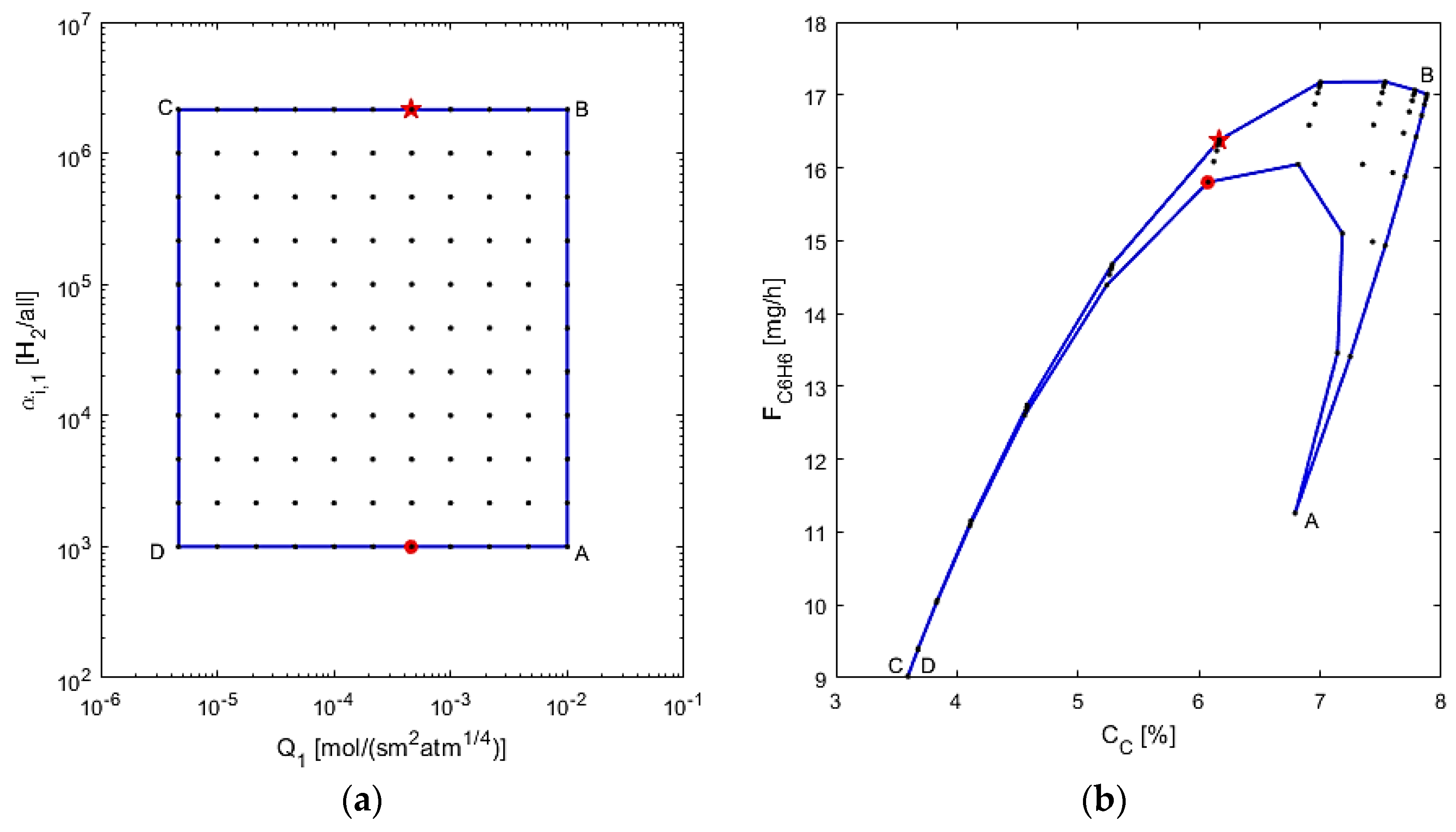
5.3. Optimization and Operability Mapping
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- U.S. EIA. Where Our Natural Gas Comes From. Available online: https://www.eia.gov/energyexplained/index.cfm?page=natural_gas_where (accessed on 16 February 2017).
- U.S. EIA. Independent Statistics and Analysis. Available online: https://www.eia.gov/outlooks/steo/report/natgas.cfm (accessed on 16 February 2017).
- Lunsford, J.H. Catalytic conversion of methane to more useful chemicals and fuels: a challenge for the 21st century. Catal. Today 2000, 63, 165–174. [Google Scholar] [CrossRef]
- Skutil, K.; Taniewski, M. Some technological aspects of methane aromatization (direct and via oxidative coupling). Fuel Proc. Technol. 2006, 87, 511–521. [Google Scholar] [CrossRef]
- Wang, L.; Tao, L.; Xie, M.; Xu, G. Dehydrogenation and aromatization of methane under non-oxidizing conditions. Catal. Lett. 1993, 21, 35–41. [Google Scholar] [CrossRef]
- Xu, Y.; Bao, X.; Lin, L. Direct conversion of methane under nonoxidative conditions. J. Catal. 2003, 216, 386–395. [Google Scholar] [CrossRef]
- Gao, K.; Yang, J.; Seidel-Morgenstern, A.; Hamel, C. Methane dehydro-aromatization: Potential of a Mo/MCM-22 catalyst and hydrogene-Selective Membranes. Chem. Ing. Tech. 2016, 88, 168–176. [Google Scholar] [CrossRef]
- Iliuta, M.; Grandjean, B.; Larachi, F. Methane nonoxidative aromatization over Ru-Mo/HZSM-5 at temperatures up to 973 K in a palladium-silver/stainless steel membrane reactor. Ind. Eng. Chem. Res. 2003, 42, 323–330. [Google Scholar] [CrossRef]
- Ismagliov, Z.; Matus, E.; Tsikoza, L. Direct conversion of methane on Mo/ZSM-5 catalysts to produce benzene and hydrogen: Achievements and perspectives. Energy Environ. Sci. 2008, 1, 526–541. [Google Scholar] [CrossRef]
- Anunziata, O.; Cussa, J.; Beltramone, A. Simultaneous optimization of methane conversion and aromatic yields by catalytic activation with ethane over Zn-ZSM-11 zeolite: The influence of the Zn-loading factor. Catal. Today 2011, 171, 36–42. [Google Scholar] [CrossRef]
- Choudhary, V.; Kinage, A.; Choudhary, T. Low-temperature nonoxidative activation of methane over H-galloaluminosilicate (MFI) Zeolite. Science 1997, 275, 1286–1288. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Ohnishi, R.; Ichikawa, M. Promotional role of water added to methane feed on catalytic performance in the methane dehydroaromatization reaction on Mo/HZSM-5 catalyst. J. Catal. 2003, 220, 57–65. [Google Scholar] [CrossRef]
- Wang, L.; Ohnishi, R.; Ichikawa, M. Novel rhenium-based catalysts for dehydrocondensation of methane with CO/CO2 towards ethylene and benzene. Catal. Lett. 1999, 62, 29–33. [Google Scholar] [CrossRef]
- Qiu, P.; Lunsford, J.; Rosynek, M. Steady-state conversion of methane to aromatics in high yields using an integrated recycle reaction system. Catal. Lett. 1997, 48, 11–15. [Google Scholar] [CrossRef]
- Karakaya, C.; Zhu, H.; Kee, R. Kinetic modeling of methane dehydroaromatization chemistry on Mo/Zeolite catalysts in packed-bed reactors. Chem. Eng. Sci. 2015, 123, 474–486. [Google Scholar] [CrossRef]
- Wong, K.; Thybaut, J.; Tangstad, E.; Stocker, M.; Marin, G. Methane aromatisation based upon elementary steps: Kinetic and catalyst descriptors. Microporous Mesoporous Mater. 2012, 164, 302–312. [Google Scholar] [CrossRef]
- Wang, L.; Ohnishi, R.; Ichikawa, M. Selective dehydroaromatization of methane toward benzene on Re/HZSM-5 catalysts and effects of CO/CO2 Addition. J. Catal. 2000, 190, 276–283. [Google Scholar] [CrossRef]
- Zeng, J.; Xiong, Z.; Zhang, H.; Lin, G.; Tsai, K. Nonoxidative dehydrogenation and aromatization of methane over W/HZSM-5-based catalysts. Catal. Lett. 1998, 53, 119–124. [Google Scholar] [CrossRef]
- Liu, S.; Wang, L.; Ohnishi, R.; Ichikawa, M. Bifunctional catalysis of Mo/HZSM-5 in the dehydroaromatization of methane to benzene and naphthalene XAFS/TG/DTA/MASS/FTIR Characterization and Supporting Effects. J. Catal. 1999, 181, 175–188. [Google Scholar] [CrossRef]
- Wu, Y.; Emdadi, L.; Wang, Z.; Fan, W.; Liu, D. Textural and catalytic properties of Mo loaded hierarchical meso-/microporous lamellar MFI and MWW zeolites for direct methane conversion. Appl. Catal. A 2014, 470, 344–354. [Google Scholar] [CrossRef]
- Wu, Y.; Emdadi, L.; Oh, S.; Sakbodin, M.; Liu, D. Spatial distribution and catalytic performance of metal–acid sites in Mo/MFI catalysts with tunable meso-/microporous lamellar zeolite structures. J. Catal. 2015, 323, 100–111. [Google Scholar] [CrossRef]
- Ismagliov, Z.; Matus, E.; Kerzhentsev, M.; Ismagliov, I.; Dosumov, K.; Mustafin, A. Methane conversion to valuable chemicals over nanostructured Mo/ZSM-5 catalysts. Pet. Chem. 2011, 51, 174–186. [Google Scholar] [CrossRef]
- Tempelman, C.H.; Hensen, E.J. On the deactivation of Mo/HZSM-5 in the methane dehydroaromatization reaction. Appl. Catal. B 2015, 176–177, 731–739. [Google Scholar] [CrossRef]
- Tempelman, C.H.; Hensen, E.J.; Zhu, X. Activation of Mo/HZSM-5 for methane aromatization. Chin. J. Catal. 2015, 36, 829–837. [Google Scholar] [CrossRef]
- Tessonnier, J.; Louis, B.; Rigolet, S.; Ledoux, M.; Pham-Huu, C. Methane dehydro-aromatization on Mo/ZSM-5: About the hidden role of Brønsted acid sites. Appl. Catal. A 2008, 336, 79–88. [Google Scholar] [CrossRef]
- Li, L.; Borry, R.; Iglesia, E. Reaction-transport simulations of non-oxidative methane conversion with continuous hydrogen removal—Homogeneous-heterogeneous reaction pathways. Chem. Eng. Sci. 2001, 56, 1869–1881. [Google Scholar] [CrossRef]
- Natesakhawat, S.; Means, N.C.; Howard, B.H.; Smith, M.; Abdelsayed, V.; Baltrus, J.; Cheng, Y.; Lekse, J.; Link, D.; Morreale, B. Improved benzene production from methane dehydroaromatization over Mo/HZSM-5 catalysts via hydrogen-permselective palladium membrane reactors. Catal. Sci. Technol. 2015, 5, 5023–5036. [Google Scholar] [CrossRef]
- Rival, O.; Grandjean, B.; Guy, C.; Sayari, A.; Larachi, F. Oxygen-free methane aromatization in a catalytic membrane reactor. Ind. Eng. Chem. Res. 2001, 40, 2212–2219. [Google Scholar] [CrossRef]
- Iliuta, M.; Iliuta, I.; Grandjean, B.; Larachi, F. Kinetics of methane nonoxidative aromatization over Ru-Mo/HZSM-5 catalyst. Ind. Eng. Chem. Res. 2003, 42, 3203–3209. [Google Scholar] [CrossRef]
- Yuan, S.; Li, J.; Hao, Z.; Feng, Z.; Xin, Q.; Ying, P.; Li, C. The effect of oxygen on the aromatization of methane over the Mo/HZSM-5 catalyst. Catal. Lett. 1999, 63, 73–77. [Google Scholar] [CrossRef]
- Cao, Z.; Jiang, H.; Luo, H.; Baumann, S.; Meulenberg, W.; Assmann, J.; Mleczko, L.; Liu, Y.; Caro, J. Natural gas to fuels and chemicals: Improved methane aromatization in an oxygen-permeable membrane reactor. Angew. Chem. 2013, 125, 14039–14042. [Google Scholar] [CrossRef]
- Kosinov, N.; Coumans, F.; Uslamin, E.; Kapteijn, F.; Hensen, E. Selective coke combustion by oxygen pulsing during Mo/ZSM-5-catalyzed methane dehydroaromatization. Angew. Chem. Int. Ed. 2016, 55, 15086–15090. [Google Scholar] [CrossRef] [PubMed]
- Cook, B.; Mousko, D.; Hoelderich, W.; Zennaro, R. Conversion of methane to aromatics over Mo2C/ZSM-5 catalyst in different reactor types. Appl. Catal. A 2009, 365, 34–41. [Google Scholar] [CrossRef]
- Carrasco, J.C.; Lima, F.V. Nonlinear operability of a membrane reactor for direct methane aromatization. In Proceedings of the 2015 IFAC ADCHEM Symposium, Whistler, BC, Canada, 7–10 June 2015. [Google Scholar]
- Carrasco, J.C.; Lima, F.V. An optimization-based operability framework for process design and intensification of modular natural gas utilization systems. Comput. Chem. Eng. 2017, 105, 246–258. [Google Scholar] [CrossRef]
- Carrasco, J.C.; Lima, F.V. Novel operability-based approach for process design and intensification: Application to a membrane reactor for direct methane aromatization. AIChE J. 2017, 63, 975–983. [Google Scholar] [CrossRef]
- Li, L.; Borry, R.; Iglesia, E. Design and optimization of catalysts and membrane reactors for the non-oxidative conversion of methane. Chem. Eng. Sci. 2002, 57, 4595–4604. [Google Scholar] [CrossRef]
- Yaws, C.L. Chemical Properties Handbook; McGraw-Hill Education: New York, NY, USA, 2003. [Google Scholar]
- Stansch, Z.; Mleczko, L.; Baerns, M. Comprehensive kinetics of oxidative coupling of methane over the La2O3/CaO Catalyst. Ind. Eng. Chem. Res. 1997, 36, 2568–2579. [Google Scholar] [CrossRef]
- Fuentes-Cano, D.; Gómez-Barea, A.; Nilsson, S.; Ollero, P. Kinetic modeling of tar and light hydrocarbons during the thermal conversion of biomass. Energy Fuels 2016, 30, 377–385. [Google Scholar] [CrossRef]
- Li, J.; Yoon, H.; Wachsman, E.D. Hydrogen permeation through thin supported SrCe0.7Zr0.2Eu0.1O3−δ membranes; dependence of flux on defect equilibria and operating conditions. J. Membr. Sci. 2011, 381, 126–131. [Google Scholar]
- Mancini, N.; Mitsos, A. Ion transport membrane reactors for oxy-combustion—Part I: Intermediate-fidelity modeling. Energy 2011, 36, 4701–4720. [Google Scholar] [CrossRef]
- Paidoussis, M.P. Fluid-Structure Interactions: Slender Structures and Axial Flow; Academic Press: San Diego, CA, USA, 1998; Volume 1, p. 240. [Google Scholar]
- Elyassi, B.; Zhang, X.; Tsapatsis, M. Long-term steam stability of MWW structure zeolites (MCM-22 and ITQ-1). Microporous Mesoporous Mater. 2014, 193, 134–144. [Google Scholar] [CrossRef]





| Parameter (Unit) | Value | Parameter (Unit) | Value |
|---|---|---|---|
| Temperature (K) | 1050 | d2 (cm) | 0.5 |
| Pressure (atm) | 1 | Q1 (mol/s·m2·atm1/4) | 0.01 |
| FCH4,feed (mmol/h) | 4.98 | Q2 (mol/s·m2·atm1/4) | 1.3 × 10−3 |
| Fair,feed (mmol/h) | 23.8 | αi,1 (H2/all) | 106 |
| FHe,sweep (mmol/h) | 6.24 | αi,2 (O2/all) | 106 |
| L (cm) | 25 | B (K) | 10,240 |
| d1 (cm) | 1.25 | – | – |
| Output (Unit) | Base | M1 | M2 | Multifunctional |
|---|---|---|---|---|
| XCH4 (%) | 19.52 | 38.36 | 19.57 | 38.15 |
| FC6H6 (mg/h) | 10.49 | 19.85 | 8.65 | 18.09 |
| CC (%) | 2.28 | 4.95 | 2.06 | 4.64 |
| Input (Unit) | Range |
|---|---|
| L (cm) | 20–200 |
| d1 (cm) | 0.5–3 |
| d2 (cm) | 0.2–2 |
| Q1 (mol/s·m2·atm1/4) | 10−6–0.1 |
| Q2 (mol/s·m2·atm1/4) | 10−7–10−2 |
| αi,1 (H2/all) | 102–107 |
| Input/Output (Unit) | Case 1 | Case 2 | Case 3 | Case 4 |
|---|---|---|---|---|
| L (cm) | 25 | 13 | 37 | 9 |
| d1 (cm) | 0.7 | 1.1 | 2.1 | 0.5 |
| Q1 (mol/s·m2·atm1/4) | 0.01 * | 0.01 | 0.01 | 2.15 × 10−5 # |
| αi,1 (H2/all) | 4.64 × 105 * | 4.64 × 105 | 1000 # | 4.64 × 105 |
| FC6H6 (mg/h) | 20.66 | 20.88 | 5.22 | 5.97 |
| XCH4 (%) | 37.82 | 38.18 | 42.17 | 13.06 |
| CC (%) | 1.30 | 1.99 | 15.32 | 0.064 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fouty, N.J.; Carrasco, J.C.; Lima, F.V. Modeling and Design Optimization of Multifunctional Membrane Reactors for Direct Methane Aromatization. Membranes 2017, 7, 48. https://doi.org/10.3390/membranes7030048
Fouty NJ, Carrasco JC, Lima FV. Modeling and Design Optimization of Multifunctional Membrane Reactors for Direct Methane Aromatization. Membranes. 2017; 7(3):48. https://doi.org/10.3390/membranes7030048
Chicago/Turabian StyleFouty, Nicholas J., Juan C. Carrasco, and Fernando V. Lima. 2017. "Modeling and Design Optimization of Multifunctional Membrane Reactors for Direct Methane Aromatization" Membranes 7, no. 3: 48. https://doi.org/10.3390/membranes7030048
APA StyleFouty, N. J., Carrasco, J. C., & Lima, F. V. (2017). Modeling and Design Optimization of Multifunctional Membrane Reactors for Direct Methane Aromatization. Membranes, 7(3), 48. https://doi.org/10.3390/membranes7030048





