On the Ionic Conductivity of Cation Exchange Membranes in Mixed Sulfates Using the Two-Phase Model
Abstract
:1. Introduction
2. Theoretical Background
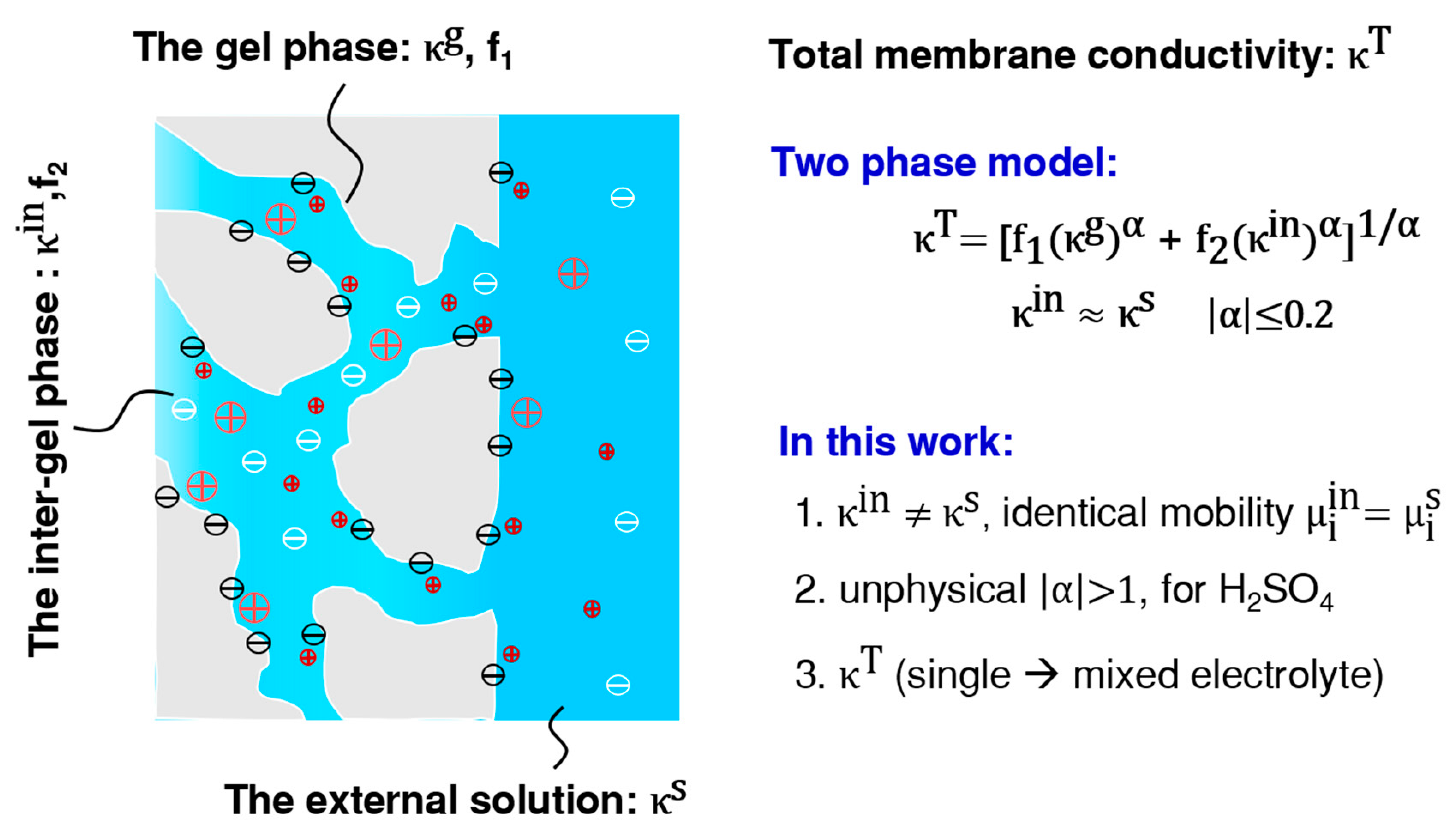
2.1. Contemporary Two-Phase Model
2.2. The Inter-Gel Phase Conductivity
3. Materials and Methods
3.1. Cation Exchange Membranes
3.2. Membrane Ionic Composition
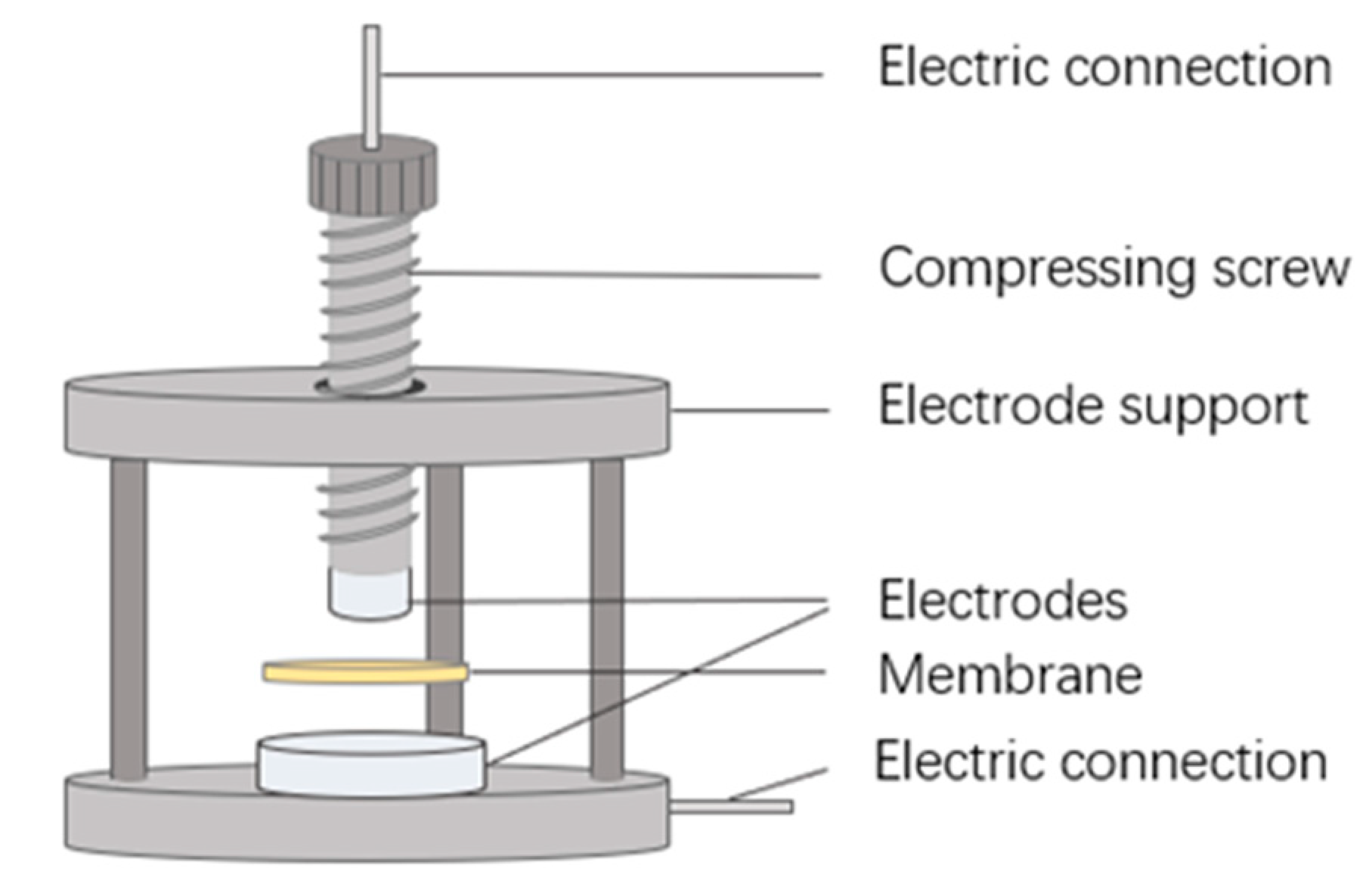
3.3. Conductivity Measurement
4. Results and Discussions
4.1. Determination of the Membrane Structural Parameters
4.1.1. Fitted from the External Solution Conductivity
4.1.2. Fitted from the Inter-Gel Phase Conductivity
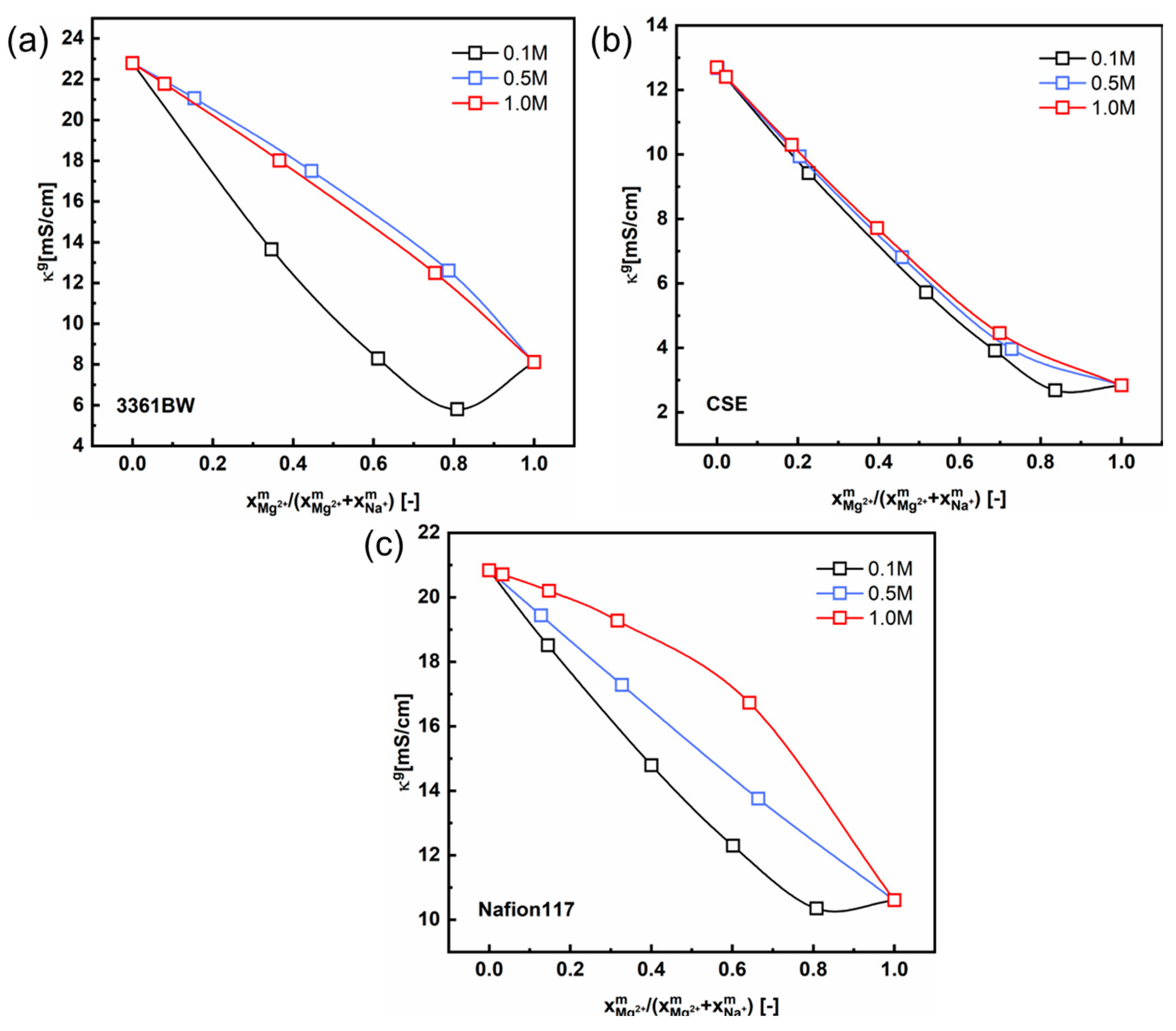
4.2. Prediction of Membrane Conductivity in Mixed Electrolytes
4.2.1. Prediction with the External Solution Conductivity
4.2.2. Prediction with the Inter-Gel Phase Conductivity
4.3. Correlation between Conductivity and Counter-Ions Composition in the Gel Phase
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jiang, S.; Sun, H.; Wang, H.; Ladewig, B.P.; Yao, Z. A comprehensive review on the synthesis and applications of ion exchange membranes. Chemosphere 2021, 282, 130817. [Google Scholar] [CrossRef] [PubMed]
- Shehzad, M.A.; Yasmin, A.; Ge, X.; Wu, L.; Xu, T. A Review of Nanostructured Ion-Exchange Membranes. Adv. Mater. Technol. 2021, 6, 2001171. [Google Scholar] [CrossRef]
- Luo, T.; Abdu, S.; Wessling, M. Selectivity of ion exchange membranes: A review. J. Membr. Sci. 2018, 555, 429–454. [Google Scholar] [CrossRef]
- Bryk, M.; Zabolotsky, V.; Atamanenko, D.; Dvorkina, G. Structural inhomogeneity of ion-exchange membranes in swelling state and methods of its investigations. Khim. Tekhnol. Vody 1989, 11, 491–498. [Google Scholar]
- Auclair, B.; Nikonenko, V.; Larchet, C.; Metayer, M.; Dammak, L. Correlation between transport parameters of ion-exchange membranes. J. Membr. Sci. 2002, 195, 89–102. [Google Scholar] [CrossRef]
- Xu, T.W.; Li, Y.; Wu, L.; Yang, W.H. A simple evaluation of microstructure and transport parameters of ion-exchange membranes from conductivity measurements. Sep. Purif. Technol. 2008, 60, 73–80. [Google Scholar] [CrossRef]
- Zabolotsky, V.I.; Nikonenko, V.V. Effect of Structural Membrane Inhomogeneity on Transport-Properties. J. Membr. Sci. 1993, 79, 181–198. [Google Scholar] [CrossRef]
- Pourcelly, G.R. Conductivity and selectivity of ion exchange membranes: Structure-correlations. Desalination 2003, 147, 359–361. [Google Scholar] [CrossRef]
- Strathmann, H. Ion Exchange Membrane Separation Processes; Elsevier: Amsterdam, The Netherlands, 2004. [Google Scholar]
- Lauger, P. Transport phenomena in membranes. Angew. Chem. Int. Ed. Engl. 1969, 8, 42–54. [Google Scholar] [CrossRef]
- Narębska, A.; Wódzki, R. Diffusion of electrolytes across inhomogeneous permselective membranes. Angew. Makromol. Chem. 1979, 80, 105–118. [Google Scholar] [CrossRef]
- Sata, T. Ion Exchange Membranes: Preparation, Characterization, Modification and Application; The Royal Society of Chemistry: Cambridge, UK, 2004. [Google Scholar]
- Petropoulos, J.H. Membrane-Transport Properties in Relation to Microscopic and Macroscopic Structural Inhomogeneity. J. Membr. Sci. 1990, 52, 305–323. [Google Scholar] [CrossRef]
- Steymans, C.L. Kurzvorträge zum Hauptthema. Untersuchungen über Leitfähigkeitsverhalten von Austauschermembranen. I. Mitteilung: Messung der Membranleitfähigkeit. Berichte der Bunsen-Gesellschaft fur Physikalische Chemie 1967, 71, 818–822. [Google Scholar]
- Koter, S.; Narebska, A. Conductivity of ion-exchange membranes-ii.Mobilities of ions and water. Electrochim. Acta 1987, 32, 455–458. [Google Scholar] [CrossRef]
- Narcbska, R.W.A.; Koter, S. Composition and structure of the cation permselective membranes I. Evaluation of the electrochemical models. Angew. Makromol. Chem. 1980, 86, 157–170. [Google Scholar] [CrossRef]
- Yamauchi, Y.H.A.A. Additivity between donnan salt and ion-exchanged salt in the specific conductance of membranes. J. Membr. Sci. 1990, 48, 25–31. [Google Scholar]
- Buck, R.P. Kinetics of bulk and interfacial ionic motion: Microscopic bases and limits for the Nernst—Planck equation applied to membrane systems. J. Membr. Sci. 1984, 17, 1–62. [Google Scholar] [CrossRef]
- Gnusin, N.P.; Berezina, N.P.; Kononenko, N.A.; Dyomina, O.A. Transport structural parameters to characterize ion exchange membranes. J. Membr. Sci. 2004, 243, 301–310. [Google Scholar] [CrossRef]
- Sedkaoui, Y.; Szymczyk, A.; Lounici, H.; Arous, O. A new lateral method for characterizing the electrical conductivity of ion-exchange membranes. J. Membr. Sci. 2016, 507, 34–42. [Google Scholar] [CrossRef]
- Sarapulova, V.; Pismenskaya, N.; Butylskii, D.; Titorova, V.; Wang, Y.; Xu, T.; Zhang, Y.; Nikonenko, V. Transport and Electrochemical Characteristics of CJMCED Homogeneous Cation Exchange Membranes in Sodium Chloride, Calcium Chloride, and Sodium Sulfate Solutions. Membranes 2020, 10, 165. [Google Scholar] [CrossRef]
- Sarapulova, V.; Pismenskaya, N.; Titorova, V.; Sharafan, M.; Wang, Y.; Xu, T.; Zhang, Y.; Nikonenko, V. Transport Characteristics of CJMAED™ Homogeneous Anion Exchange Membranes in Sodium Chloride and Sodium Sulfate Solutions. Int. J. Mol. Sci. 2021, 22, 1415. [Google Scholar] [CrossRef]
- Sarapulova, V.V.; Titorova, V.D.; Nikonenko, V.V.; Pismenskaya, N.D. Transport Characteristics of Homogeneous and Heterogeneous Ion-Exchange Membranes in Sodium Chloride, Calcium Chloride, and Sodium Sulfate Solutions. Membr. Membr. Technol. 2019, 1, 168–182. [Google Scholar] [CrossRef]
- Veerman, J. The Effect of the NaCl Bulk Concentration on the Resistance of Ion Exchange Membranes—Measuring and Modeling. Energies 2020, 13, 1946. [Google Scholar] [CrossRef]
- Kamcev, J.; Sujanani, R.; Jang, E.-S.; Yan, N.; Moe, N.; Paul, D.R.; Freeman, B.D. Salt concentration dependence of ionic conductivity in ion exchange membranes. J. Membr. Sci. 2018, 547, 123–133. [Google Scholar] [CrossRef]
- Zhu, S.; Kingsbury, R.S.; Call, D.F.; Coronell, O. Impact of solution composition on the resistance of ion exchange membranes. J. Membr. Sci. 2018, 554, 39–47. [Google Scholar] [CrossRef]
- Ziv, N.; Dekel, D.R. A practical method for measuring the true hydroxide conductivity of anion exchange membranes. Electrochem. Commun. 2018, 88, 109–113. [Google Scholar] [CrossRef]
- Barragán, V.M.; Izquierdo-Gil, M.A.; Maroto, J.C.; Antoranz, P.; Muñoz, S. Estimation of the through-plane thermal conductivity of polymeric ion-exchange membranes using finite element technique. Int. J. Heat Mass Transf. 2021, 176, 121469. [Google Scholar] [CrossRef]
- Díaz, J.C.; Kamcev, J. Ionic conductivity of ion-exchange membranes: Measurement techniques and salt concentration dependence. J. Membr. Sci. 2021, 618, 118718. [Google Scholar] [CrossRef]
- Pismenskaya, N.D.; Nevakshenova, E.E.; Nikonenko, V.V. Using a Single Set of Structural and Kinetic Parameters of the Microheterogeneous Model to Describe the Sorption and Kinetic Properties of Ion-Exchange Membranes. Pet. Chem. 2018, 58, 465–473. [Google Scholar] [CrossRef]
- Agmon, N. The Grotthuss mechanism. Chem. Phys. Lett. 1995, 244, 456–462. [Google Scholar] [CrossRef]
- Ponomarev, O.A.; Ionova, I.A. The thermodynamics of ionomers. Polym. Sci. U.S.S.R. 1974, 16, 1181–1190. [Google Scholar] [CrossRef]
- Wu, L.; Luo, T.; Yang, X.; Zhao, H.; Wang, X.; Zhang, Z. Impact of the Donnan electrolytes on selectivity of cation exchange membranes evaluated via the ionic membrane conductivity. Sep. Purif. Technol. 2023, 316, 123816. [Google Scholar] [CrossRef]
- Logette, S.; Eysseric, C.; Pourcelly, G.; Lindheimer, A.; Gavach, C. Selective permeability of a perfluorosulphonic membrane to different valency cations. Ion-exchange isotherms and kinetic aspects. J. Membr. Sci. 1998, 144, 259–274. [Google Scholar] [CrossRef]
- Nikonenko, V.; Nebavsky, A.; Mareev, S.; Kovalenko, A.; Urtenov, M.; Pourcelly, G. Modelling of Ion Transport in Electromembrane Systems: Impacts of Membrane Bulk and Surface Heterogeneity. Appl. Sci. 2019, 9, 25. [Google Scholar] [CrossRef]
- Dimov, A.D.; Alexandrova, I. Electrochemical properties of cation-exchange membranes. J. Chem. Soc. Faraday Trans. 1 Phys. Chem. Condens. Phases 1987, 83, 2841–2845. [Google Scholar] [CrossRef]
- Zou, Z.; Wu, L.; Luo, T.; Yan, Z.; Wang, X. Assessment of anion exchange membrane selectivity with ionic membrane conductivity, revised with Manning’s theory or the Kohlrausch’s law. J. Membr. Sci. 2021, 635, 119496. [Google Scholar] [CrossRef]
- Chaudhury, S.; Agarwal, C.; Goswami, A. Transport Properties of Multivalent Cations in Nafion-117 Membrane with Mixed Ionic Composition. J. Phys. Chem. B 2015, 119, 10566–10572. [Google Scholar] [CrossRef]


| Ions | Na+ | Mg2+ | H+ | SO42− |
|---|---|---|---|---|
| (m2s−1V−1) | 5.20 | 5.50 | 36.2 | 8.27 |
| Mem. | Area Resistance (Ω cm2) | Density of Dry Polymer (g cm−3) | Dry Membrane Thickness (μm) | Water Uptake (g g−1 Dry Polymer) | IEC (meq g−1 Dry Polymer) |
|---|---|---|---|---|---|
| CSE | 1.8 | 1.16 ± 0.03 | 160 | 0.29 ± 0.05 | 2.34 ± 0.01 |
| 3361BW | 11.0 | 0.93 ± 0.01 | 420 | 0.43 ± 0.08 | 2.45 ± 0.01 |
| Nafion117 | 1.0 | 1.94 ± 0.11 | 180 | 0.19 ± 0.01 | 0.99 ± 0.01 |
| Membranes | Electrolytes | α [-] | [-] | R2 [-] |
|---|---|---|---|---|
| 3361BW | Na2SO4 | 0.025 | 0.305 | 0.910 |
| MgSO4 | 0.281 | 0.317 | 0.868 | |
| H2SO4 | −1.072 | 0.818 | 0.987 | |
| CSE | Na2SO4 | −0.006 | 0.168 | 0.852 |
| MgSO4 | −0.732 | 0.998 | 0.924 | |
| H2SO4 | −1.561 | 0.999 | 0.959 | |
| Nafion117 | Na2SO4 | 0.427 | 0.128 | 0.971 |
| MgSO4 | 1.180 | 0.058 | 0.833 | |
| H2SO4 | −0.734 | 0.188 | 0.750 |
| Membranes | Electrolytes | Nonlinear | Linear | |||
|---|---|---|---|---|---|---|
| α [-] | [-] | R2 [-] | [-] | R2 [-] | ||
| 3361BW | Na2SO4 | −0.055 | 0.207 | 0.916 | 0.210 | 0.931 |
| MgSO4 | 0.213 | 0.167 | 0.740 | 0.157 | 0.801 | |
| H2SO4 | −1.393 | 0.155 | 0.999 | 0.397 | 0.932 | |
| CSE | Na2SO4 | −0.282 | 0.141 | 0.868 | 0.153 | 0.863 |
| MgSO4 | −0.382 | 0.099 | 0.868 | 0.098 | 0.879 | |
| H2SO4 | −2.439 | 0.005 | 0.884 | 0.150 | 0.610 | |
| Nafion117 | Na2SO4 | 0.256 | 0.120 | 0.935 | 0.098 | 0.931 |
| MgSO4 | 0.940 | 0.086 | 0.785 | 0.125 | 0.695 | |
| H2SO4 | −1.257 | 0.014 | 0.772 | 0.068 | 0.581 | |
| Membranes | Electrolytes | Nonlinear | Linear | |||
|---|---|---|---|---|---|---|
| α [-] | [-] | R2 [-] | [-] | R2 [-] | ||
| 3361BW | Na2SO4 | 0.162 | 0.310 | 0.919 | 0.269 | 0.932 |
| MgSO4 | 0.400 | 0.319 | 0.711 | 0.178 | 0.769 | |
| H2SO4 | - | - | - | 0.440 | 0.924 | |
| CSE | Na2SO4 | −0.356 | 0.152 | 0.866 | 0.218 | 0.858 |
| MgSO4 | −0.379 | 0.074 | 0.853 | 0.118 | 0.876 | |
| H2SO4 | −1.703 | 0.006 | 0.795 | 0.159 | 0.588 | |
| Nafion117 | Na2SO4 | 0.477 | 0.239 | 0.924 | 0.117 | 0.908 |
| MgSO4 | - | - | - | 0.163 | 0.640 | |
| H2SO4 | −1.866 | 0.002 | 0.754 | 0.072 | 0.569 | |
| Mem. | × 105 (cm2 s−1V−1) | × 105 (cm2 s−1V−1) | × 105 (cm2 s−1V−1) | |||
|---|---|---|---|---|---|---|
| Infinite Dilution | OLI Simulation | Infinite Dilution | OLI Simulation | Infinite Dilution | OLI Simulation | |
| CSE | 4.93 | 5.14 | 9.32 | 1.40 | 33.37 | 31.23 |
| 3361BW | 10.85 | 5.65 | 0.96 | 0.41 | 96.04 | 34.60 |
| Nafion117 | 13.24 | 13.52 | 1.55 | 1.95 | 59.00 | 57.30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, L.; Jiang, H.; Luo, T.; Wang, X. On the Ionic Conductivity of Cation Exchange Membranes in Mixed Sulfates Using the Two-Phase Model. Membranes 2023, 13, 811. https://doi.org/10.3390/membranes13100811
Wu L, Jiang H, Luo T, Wang X. On the Ionic Conductivity of Cation Exchange Membranes in Mixed Sulfates Using the Two-Phase Model. Membranes. 2023; 13(10):811. https://doi.org/10.3390/membranes13100811
Chicago/Turabian StyleWu, Liansheng, Haodong Jiang, Tao Luo, and Xinlong Wang. 2023. "On the Ionic Conductivity of Cation Exchange Membranes in Mixed Sulfates Using the Two-Phase Model" Membranes 13, no. 10: 811. https://doi.org/10.3390/membranes13100811
APA StyleWu, L., Jiang, H., Luo, T., & Wang, X. (2023). On the Ionic Conductivity of Cation Exchange Membranes in Mixed Sulfates Using the Two-Phase Model. Membranes, 13(10), 811. https://doi.org/10.3390/membranes13100811






