Analyses of the Effects of Electrolyte and Electrode Thickness on High Temperature Proton Exchange Membrane Fuel Cell (H-TPEMFC) Quality
Abstract
1. Introduction
2. Materials and Methods
2.1. H-TPEMF Advantages
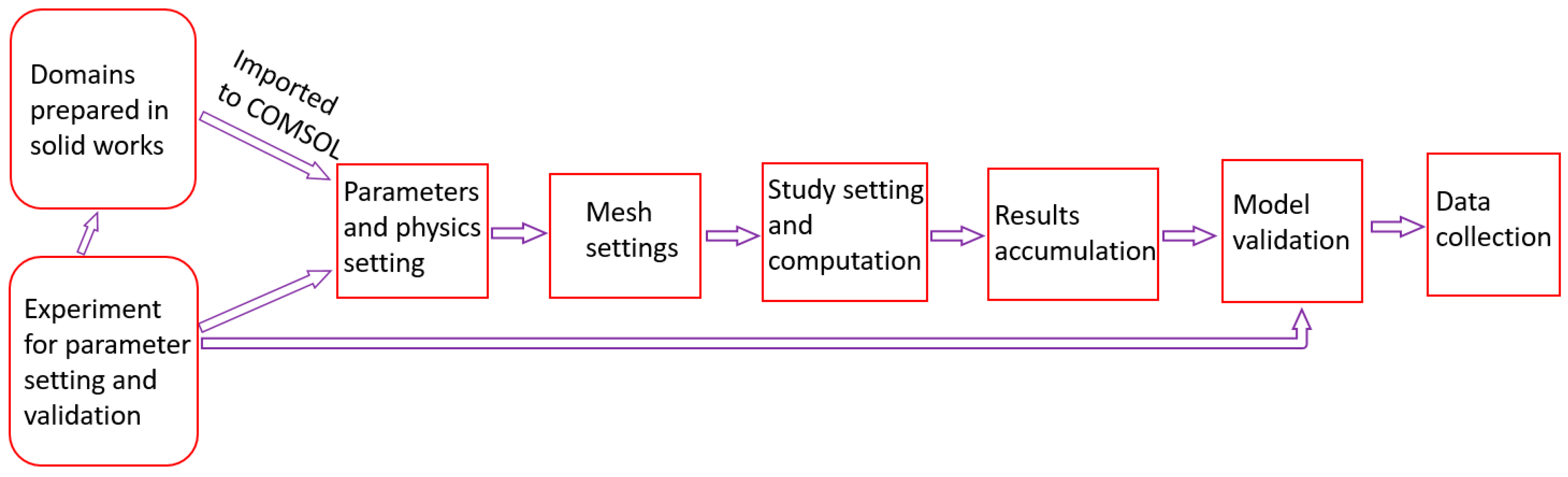
2.2. Modelling Set Up
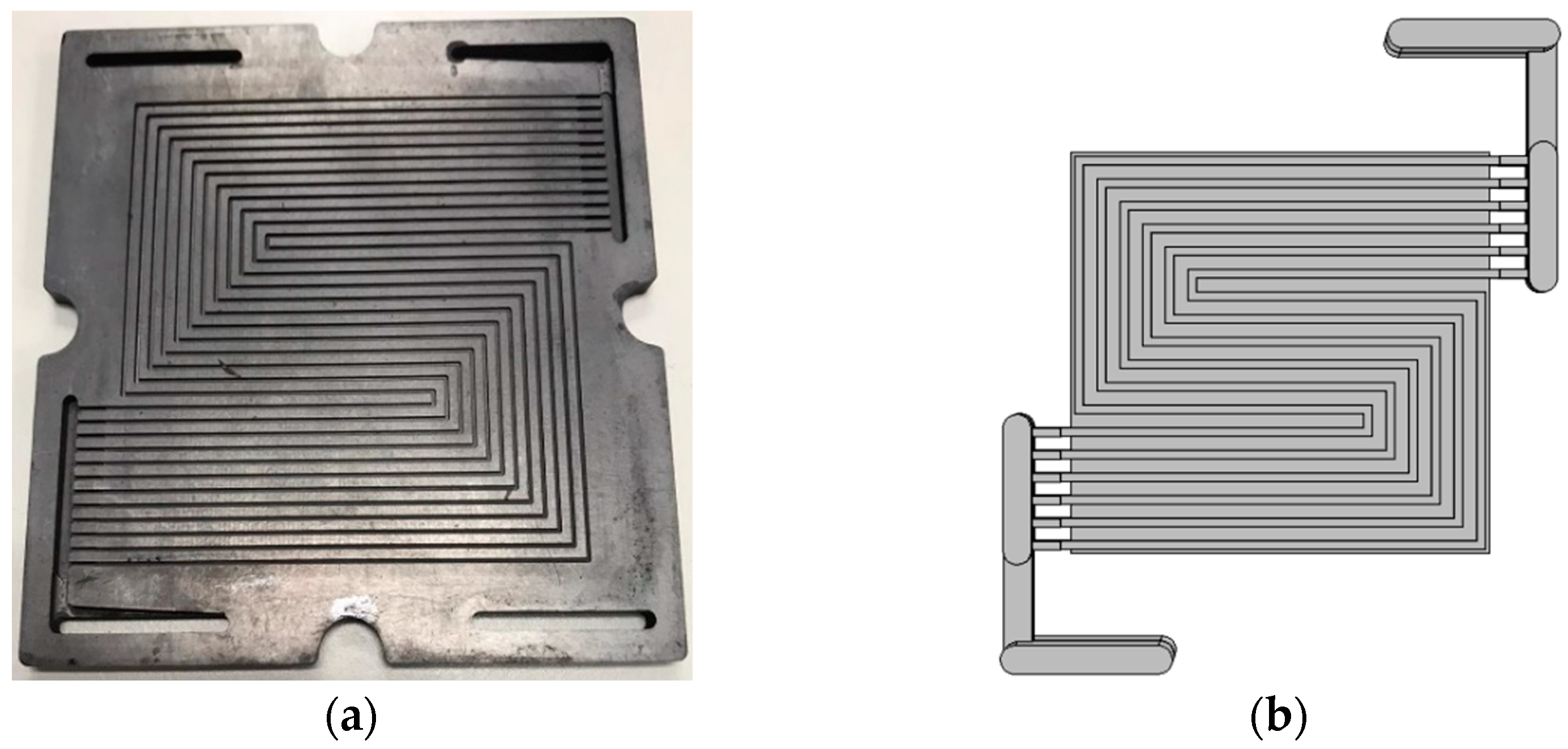
2.3. Components Geometry
2.4. Temperature Effect
2.5. Protonic Conductivity
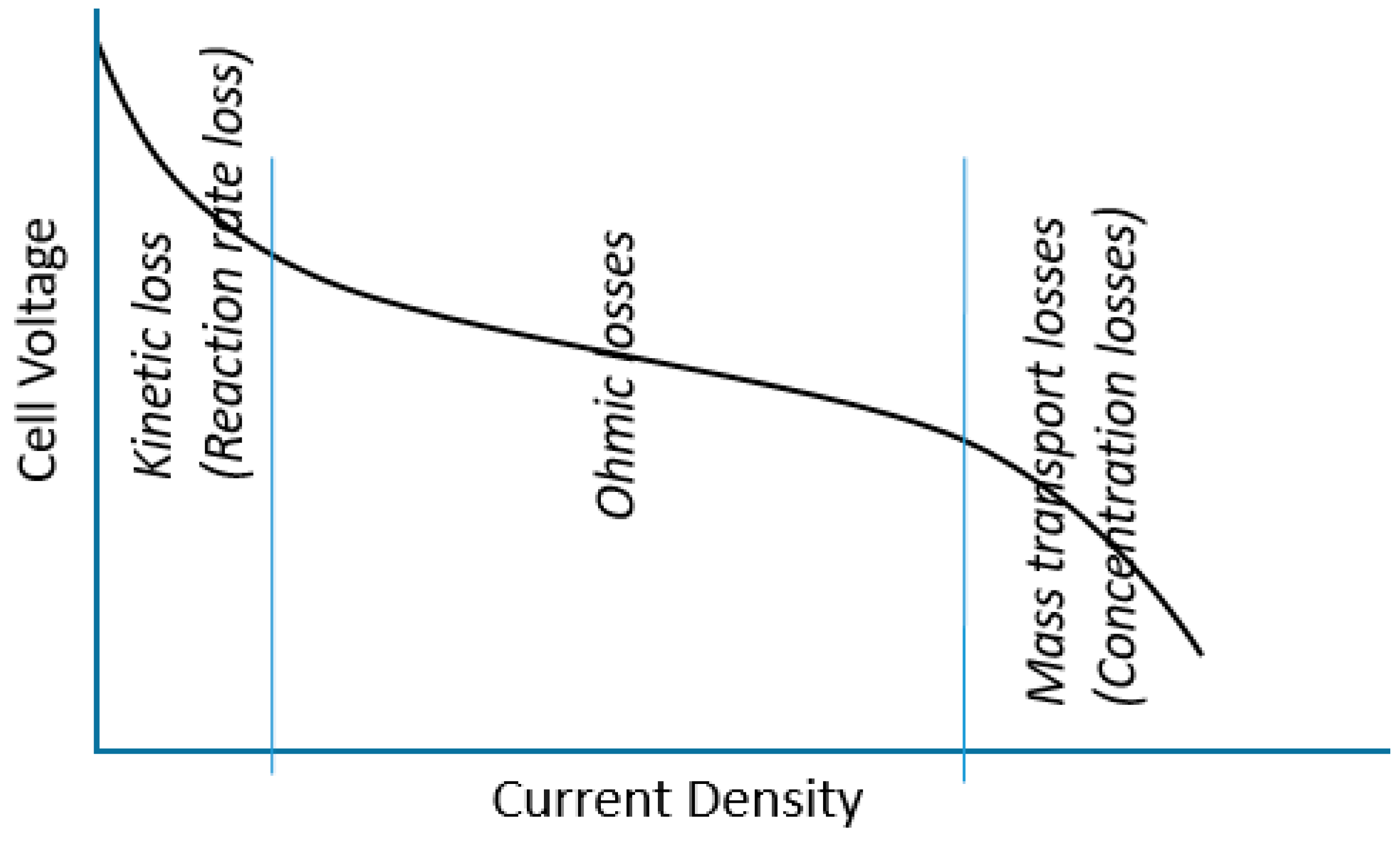
2.6. Resistance and Losses
2.7. Model Description
2.8. Grid Size
2.9. Numerical Methods and Governing Equations
3. Results
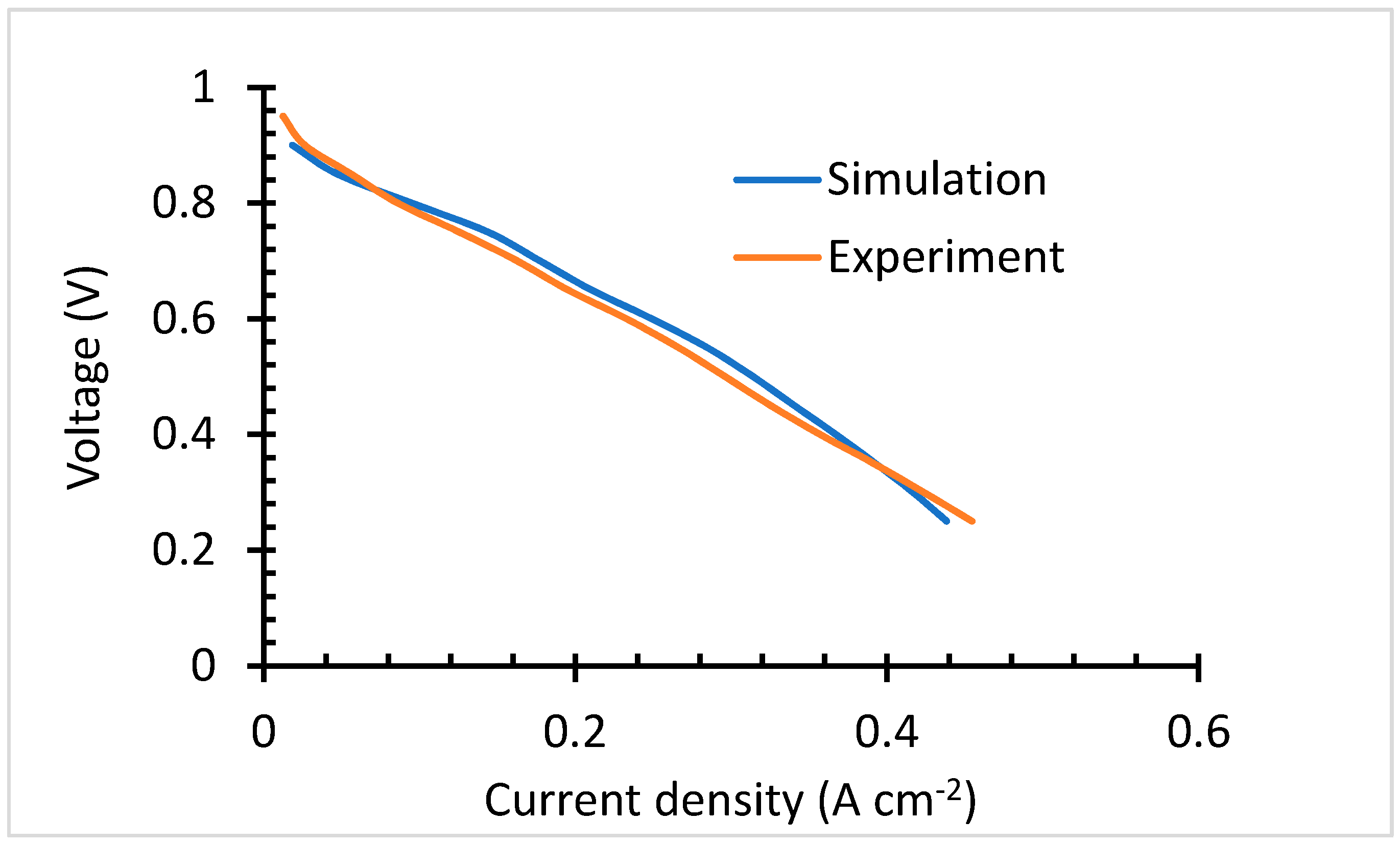
3.1. Parameter Validation
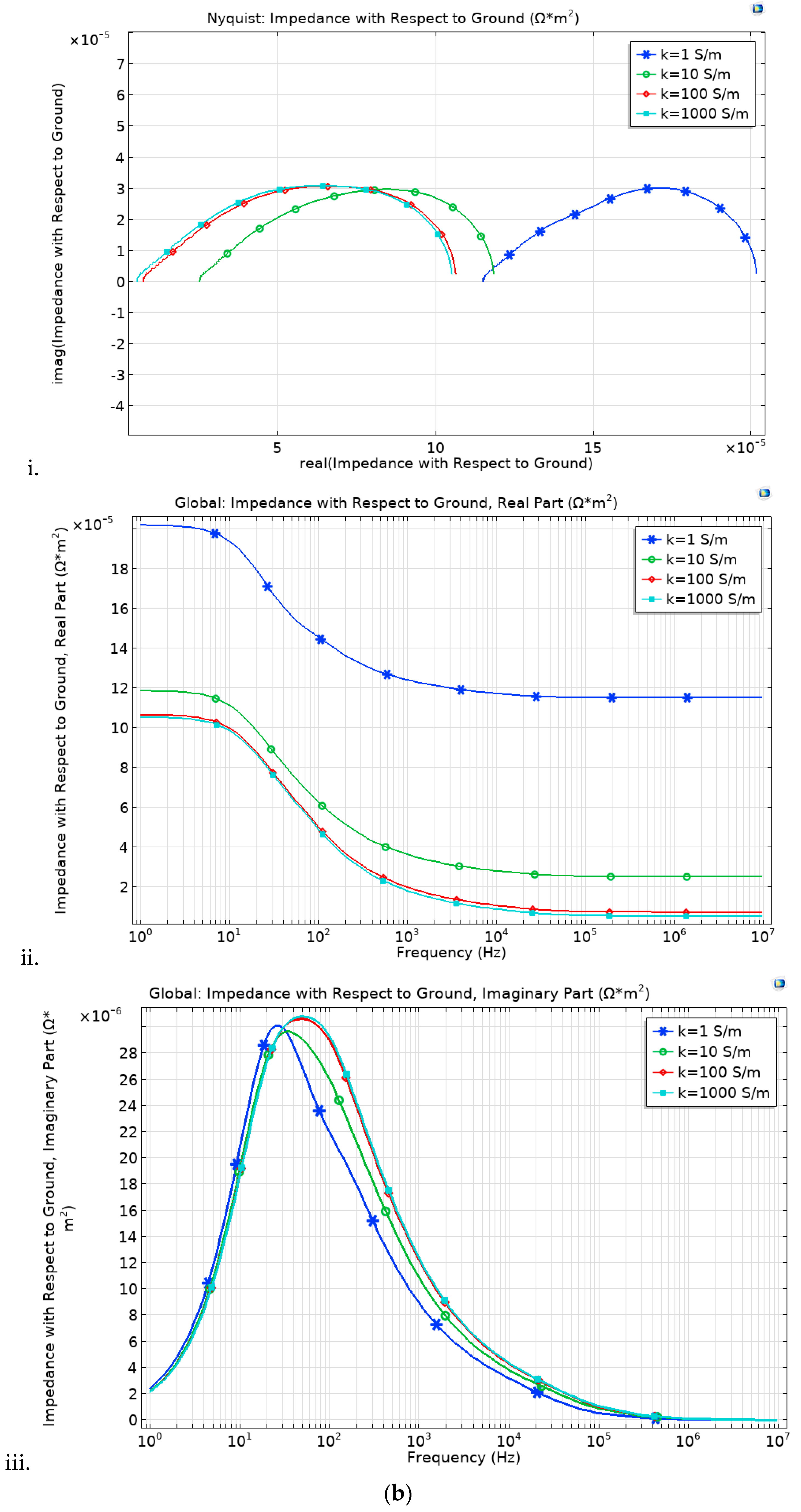
3.2. Impedance and ICR
3.3. Polarization I-V Curve
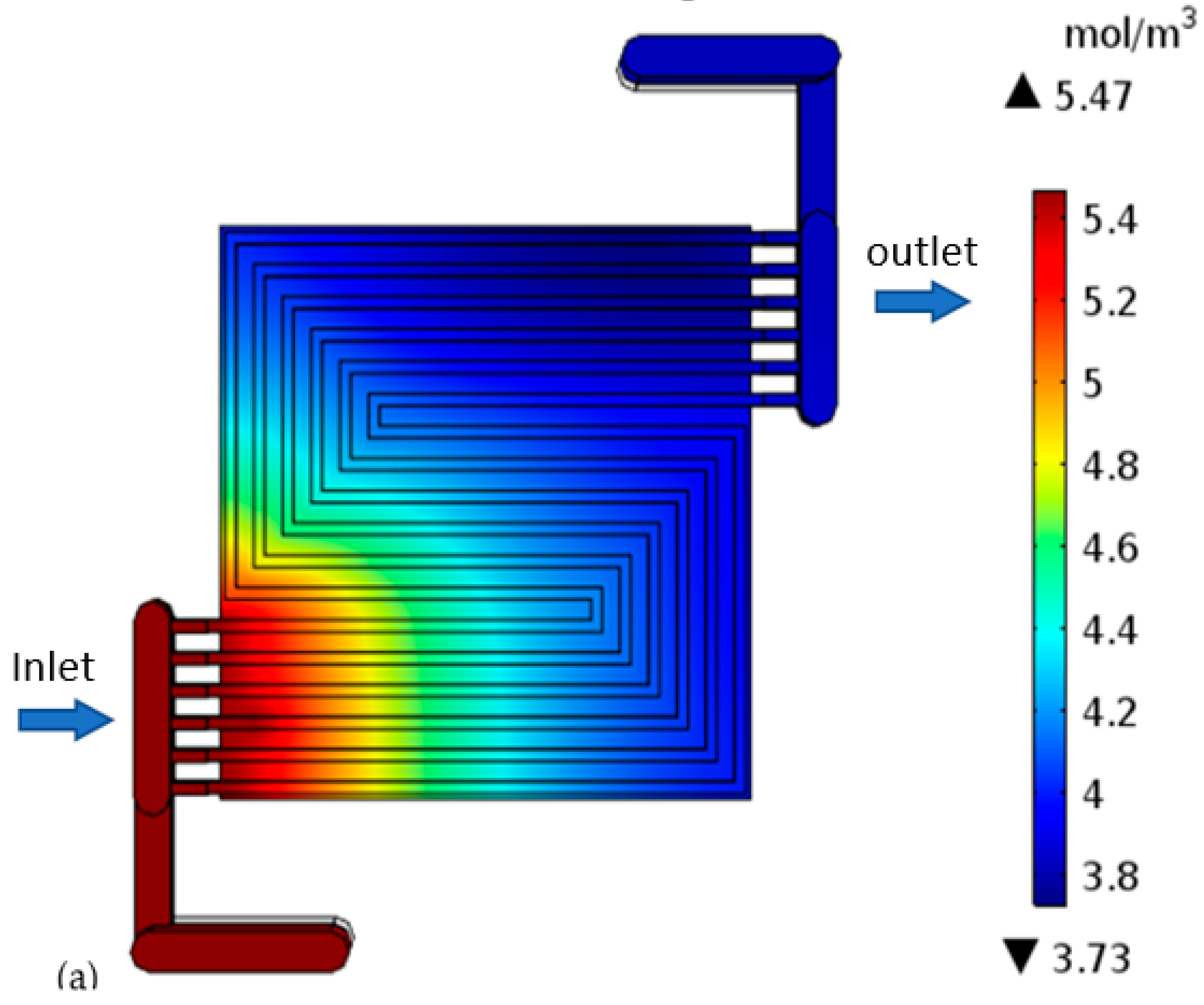
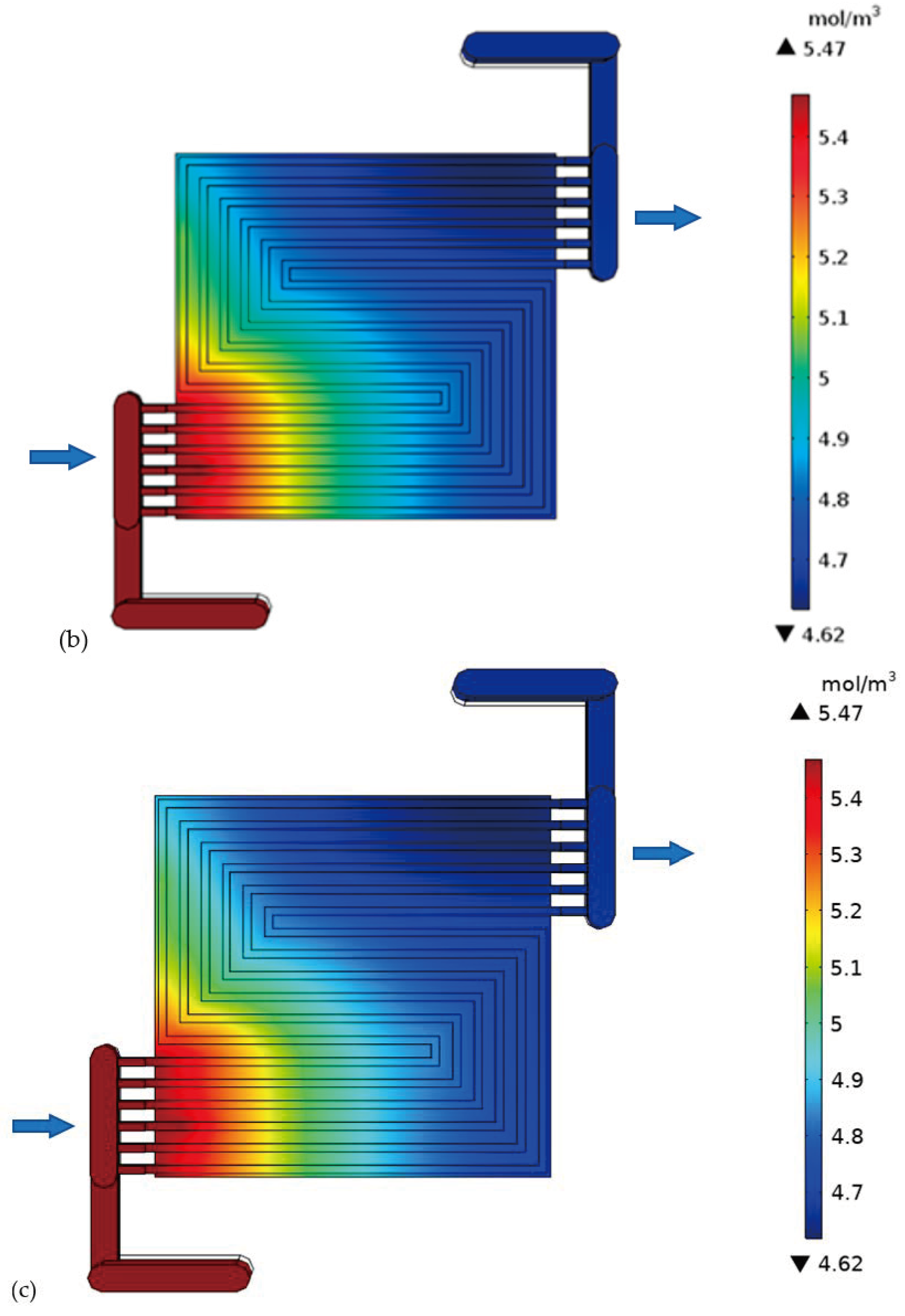
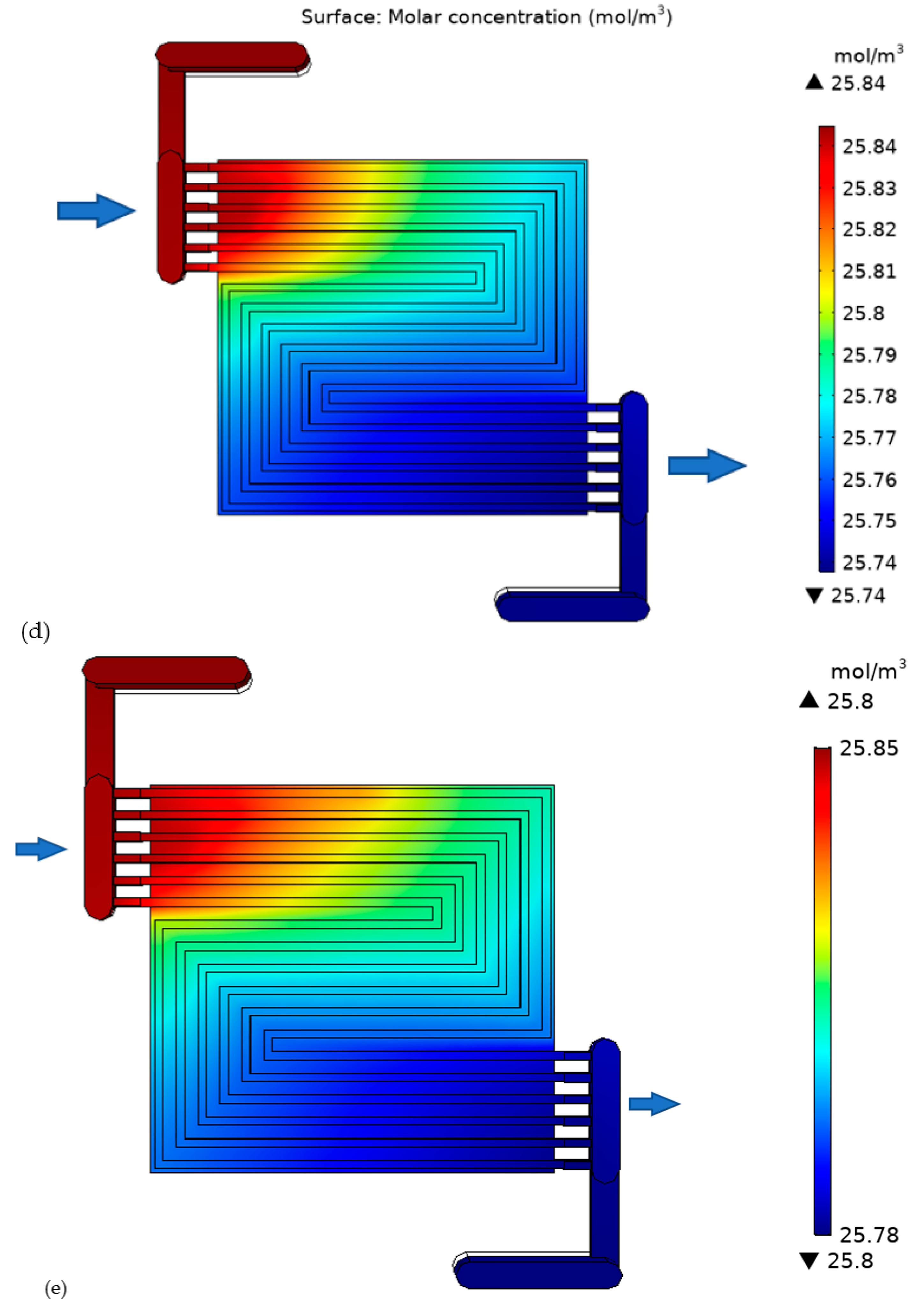
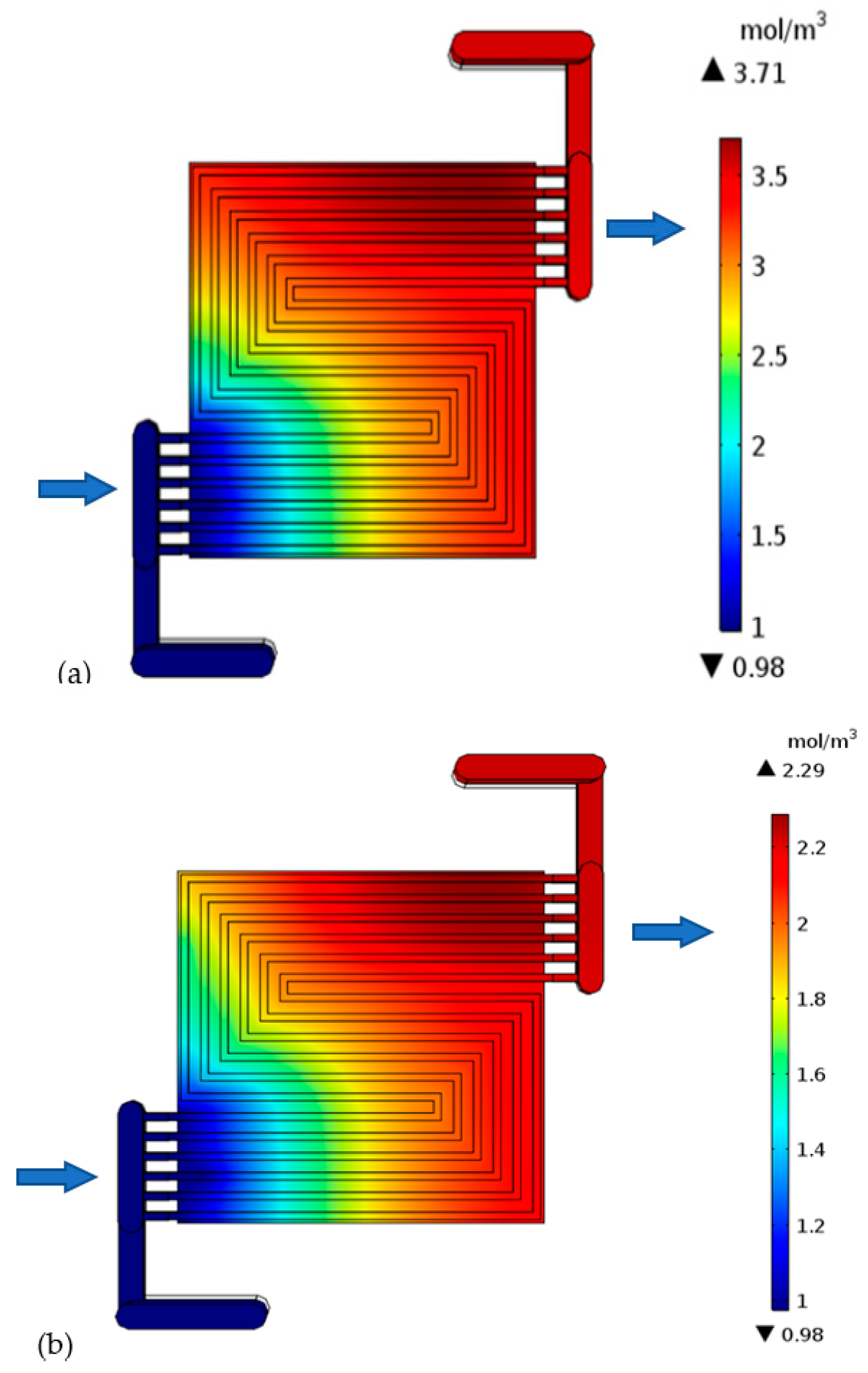
3.4. Species Concentration
3.4.1. Oxygen
3.4.2. Hydrogen
3.4.3. Electrochemically Generated Water
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee, H.; Lee, C.; Oh, T.; Choi, S.; Park, I.; Baek, K. Development of 1 kW class polymer electrolyte membrane fuel cell power generation system. J. Power Sources 2002, 107, 110–119. [Google Scholar] [CrossRef]
- Pei, P.; Yuan, X.; Chao, P.; Wang, X. Analysis on the PEM fuel cells after accelerated life experiment. Int. J. Hydrogen Energy 2010, 35, 3147–3151. [Google Scholar] [CrossRef]
- Jang, J.-H.; Chiu, H.-C.; Yan, W.-M.; Sun, W.-L. Effects of operating conditions on the performances of individual cell and stack of PEM fuel cell. J. Power Sources 2008, 180, 476–483. [Google Scholar] [CrossRef]
- Park, Y.H.; Caton, J.A. Development of a PEM stack and performance analysis including the effects of water content in the membrane and cooling method. J. Power Sources 2008, 179, 584–591. [Google Scholar] [CrossRef]
- Baik, K.D.; Yang, S.H. Development of cathode cooling fins with a multi-hole structure for open-cathode polymer electrolyte membrane fuel cells. Appl. Energy 2020, 279, 115815. [Google Scholar] [CrossRef]
- Baik, K.D.; Yang, S.H. Improving open-cathode polymer electrolyte membrane fuel cell performance using multi-hole separators. Int. J. Hydrogen Energy 2020, 45, 9004–9009. [Google Scholar] [CrossRef]
- Shen, K.-Y.; Park, S.; Kim, Y.-B. Hydrogen utilization enhancement of proton exchange membrane fuel cell with anode recirculation system through a purge strategy. Int. J. Hydrogen Energy 2020, 45, 16773–16786. [Google Scholar] [CrossRef]
- Zhao, C.; Xing, S.; Liu, W.; Wang, H. Air and H2 feed systems optimization for open-cathode proton exchange membrane fuel cells. Int. J. Hydrogen Energy 2021, 46, 11940–11951. [Google Scholar] [CrossRef]
- Gomez, A.; Sasmito, A.P.; Shamim, T. Investigation of the purging effect on a dead-end anode PEM fuel cell-powered vehicle during segments of a European driving cycle. Energy Convers. Manag. 2015, 106, 951–957. [Google Scholar] [CrossRef]
- Yan, W.-M.; Zeng, M.-S.; Yang, T.-F.; Chen, C.-Y.; Amani, M.; Amani, P. Performance improvement of air-breathing proton exchange membrane fuel cell stacks by thermal management. Int. J. Hydrogen Energy 2020, 45, 22324–22339. [Google Scholar] [CrossRef]
- Huang, Z.; Jian, Q.; Zhao, J. Thermal management of open-cathode proton exchange membrane fuel cell stack with thin vapor chambers. J. Power Sources 2020, 485, 229314. [Google Scholar] [CrossRef]
- Sasmito, A.P.; Ali, M.I.; Shamim, T. A Factorial Study to Investigate the Purging Effect on the Performance of a Dead-End Anode PEM Fuel Cell Stack. Fuel Cells 2015, 15, 160–169. [Google Scholar] [CrossRef]
- Siegel, J.B. Experiments and Modeling of PEM Fuel Cells for Dead-Ended Anode Operation. Ph.D. Thesis, University of Michigan, Ann Arbor, MI, USA, 2010. [Google Scholar]
- Steinberger, M.; Geiling, J.; Oechsner, R.; Frey, L. Anode recirculation and purge strategies for PEM fuel cell operation with diluted hydrogen feed gas. Appl. Energy 2018, 232, 572–582. [Google Scholar] [CrossRef]
- Kienitz, B. Optimizing polymer electrolyte membrane thickness to maximize fuel cell vehicle range. Int. J. Hydrogen Energy 2020, 46, 11176–11182. [Google Scholar] [CrossRef]
- Nandjou, F.; Poirot-Crouvezier, J.-P.; Chandesris, M.; Rosini, S.; Hussey, D.; Jacobson, D.; LaManna, J.; Morin, A.; Bultel, Y. A pseudo-3D model to investigate heat and water transport in large area PEM fuel cells—Part 2: Application on an automotive driving cycle. Int. J. Hydrogen Energy 2016, 41, 15573–15584. [Google Scholar] [CrossRef]
- Corbo, P.; Migliardini, F.; Veneri, O. Experimental analysis of a 20 kWe PEM fuel cell system in dynamic conditions representative of automotive applications. Energy Convers. Manag. 2008, 49, 2688–2697. [Google Scholar] [CrossRef]
- Haddad, A.; Mannah, M.; Bazzi, H. Nonlinear time-variant model of the PEM type fuel cell for automotive applications. Simul. Model. Pract. Theory 2015, 51, 31–44. [Google Scholar] [CrossRef]
- Kendall, M. Fuel cell development for New Energy Vehicles (NEVs) and clean air in China. Prog. Nat. Sci. 2018, 28, 113–120. [Google Scholar] [CrossRef]
- Gross, T.J.; Poche, A.J., Jr.; Ennis, K.C. Beyond Demonstration: The Role of Fuel Cells in DoD’s Energy Strategy; Logistics Management Institute (Lmi): Mclean, VA, USA, 2011. [Google Scholar]
- Barroso, J.; Renau, J.; Lozano, A.; Miralles, J.; Martín, J.; Sánchez, F.; Barreras, F. Experimental determination of the heat transfer coefficient for the optimal design of the cooling system of a PEM fuel cell placed inside the fuselage of an UAV. Appl. Therm. Eng. 2015, 89, 1–10. [Google Scholar] [CrossRef]
- Jiang, L.; Syed, J.A.; Zhang, G.; Ma, Y.; Ma, J.; Lu, H.; Meng, X. Enhanced anticorrosion performance of PPY-graphene oxide/PPY-camphorsulfonic acid composite coating for 304SS bipolar plates in proton exchange membrane fuel cell. J. Ind. Eng. Chem. 2019, 80, 497–507. [Google Scholar] [CrossRef]
- Park, J.E.; Hwang, W.; Lim, M.S.; Kim, S.; Ahn, C.-Y.; Kim, O.-H.; Shim, J.-G.; Lee, D.W.; Lee, J.H.; Cho, Y.-H.; et al. Achieving breakthrough performance caused by optimized metal foam flow field in fuel cells. Int. J. Hydrogen Energy 2019, 44, 22074–22084. [Google Scholar] [CrossRef]
- Perng, S.-W.; Wu, H.-W.; Chen, Y.-B.; Zeng, Y.-K. Performance enhancement of a high temperature proton exchange membrane fuel cell by bottomed-baffles in bipolar-plate channels. Appl. Energy 2019, 255, 113815. [Google Scholar] [CrossRef]
- Song, Y.; Zhang, C.; Ling, C.Y.; Han, M.; Yong, R.Y.; Sun, D.; Chen, J. Review on current research of materials, fabrication and application for bipolar plate in proton exchange membrane fuel cell. Int. J. Hydrogen Energy 2020, 45, 29832. [Google Scholar] [CrossRef]
- Available online: https://www.energy.gov/eere/fuelcells/doe-technical-targets-fuel-cell-systems-and-stacks-transportation-applications (accessed on 14 March 2022).
- Xia, L.; Zhang, C.; Hu, M.; Jiang, S.; Chin, C.S.; Gao, Z.; Liao, Q. Investigation of parameter effects on the performance of high-temperature PEM fuel cell. Int. J. Hydrogen Energy 2018, 43, 23441–23449. [Google Scholar] [CrossRef]
- Das, S.K.; Gibson, H.A. Three dimensional multi-physics modeling and simulation for assessment of mass transport impact on the performance of a high temperature polymer electrolyte membrane fuel cell. J. Power Sources 2021, 499, 229844. [Google Scholar] [CrossRef]
- Zhang, C.; Zhang, J.; Liu, Q.; Cai, L.; Ni, M.; Zeng, T.; Liang, C. Modeling and analysis of water vapor dynamics in high-temperature proton exchange membrane fuel cell coupling gas-crossover phenomena. Int. J. Hydrogen Energy 2022, 47, 18504–18517. [Google Scholar] [CrossRef]
- Pan, M.; Pan, C.; Li, C.; Zhao, J. A review of membranes in proton exchange membrane fuel cells: Transport phe-nomena, performance and durability. Renew. Sustain. Energy Rev. 2021, 141, 110771. [Google Scholar] [CrossRef]
- Xia, L.; Ni, M.; He, Q.; Xu, Q.; Cheng, C. Optimization of gas diffusion layer in high temperature PEMFC with the focuses on thickness and porosity. Appl. Energy 2021, 300, 117357. [Google Scholar] [CrossRef]
- Xia, L.; Ni, M.; Xu, Q.; Xu, H.; Zheng, K. Optimization of catalyst layer thickness for achieving high performance and low cost of high temperature proton exchange membrane fuel cell. Appl. Energy 2021, 294, 117012. [Google Scholar] [CrossRef]
- Lee, C.-Y.; Weng, F.-B.; Chiu, C.-W.; Nawale, S.-M.; Lai, B.-J. Real-Time Monitoring of the Temperature, Flow, and Pressure Inside High-Temperature Proton Exchange Membrane Fuel Cells. Micromachines 2022, 13, 1040. [Google Scholar] [CrossRef]
- Lee, C.-Y.; Weng, F.-B.; Yang, C.-Y.; Chiu, C.-W.; Nawale, S.-M. Real-Time Monitoring of HT-PEMFC. Membranes 2022, 12, 94. [Google Scholar] [CrossRef] [PubMed]
- Ribeirinha, P.; Abdollahzadeh, M.; Pereira, A.; Relvas, F.; Boaventura, M.; Mendes, A. High temperature PEM fuel cell integrated with a cellular membrane methanol steam reformer: Experimental and modelling. Appl. Energy 2018, 215, 659–669. [Google Scholar] [CrossRef]
- Tseng, C.-J.; Tsai, B.T.; Liu, Z.-S.; Cheng, T.-C.; Chang, W.-C.; Lo, S.-K. A PEM fuel cell with metal foam as flow distributor. Energy Convers. Manag. 2012, 62, 14–21. [Google Scholar] [CrossRef]
- Tsai, B.T.; Tseng, C.J.; Liu, Z.S.; Wang, C.H.; Lee, C.I.; Yang, C.C.; Lo, S.K. Effects of flow field design on the performance of a PEM fuel cell with metal foam as the flow distributor. Int. J. Hydrogen Energy 2012, 37, 13060–13066. [Google Scholar] [CrossRef]
- Heidary, H.; Kermani, M.J.; Dabir, B. Influences of bipolar plate channel blockages on PEM fuel cell performances. Energy Convers. Manag. 2016, 124, 51–60. [Google Scholar] [CrossRef]
- Hasanpour, S.; Ahadi, M.; Bahrami, M.; Djilali, N.; Akbari, M. Woven gas diffusion layers for polymer electrolyte membrane fuel cells: Liquid water transport and conductivity trade-offs. J. Power Sources 2018, 403, 192–198. [Google Scholar] [CrossRef]
- Öztürk, A.; Fıçıcılar, B.; Eroğlu, I.; Yurtcan, A.B. Facilitation of water management in low Pt loaded PEM fuel cell by creating hydrophobic microporous layer with PTFE, FEP and PDMS polymers: Effect of polymer and carbon amounts. Int. J. Hydrogen Energy 2017, 42, 21226–21249. [Google Scholar] [CrossRef]
- Chi, B.; Hou, S.; Liu, G.; Deng, Y.; Zeng, J.; Song, H.; Liao, S.; Ren, J. Tuning hydrophobic-hydrophilic balance of cathode catalyst layer to improve cell performance of proton exchange membrane fuel cell (PEMFC) by mixing polytetra-fluoroethylene (PTFE). Electrochim. Acta 2018, 277, 110–115. [Google Scholar] [CrossRef]
- Lee, C.-M.; Pai, Y.-H.; Zen, J.-M.; Shieu, F.-S. Characterization of Teflon-like carbon cloth prepared by plasma surface modification for use as gas diffusion backing in membrane electrode assembly. Mater. Chem. Phys. 2009, 114, 151–155. [Google Scholar] [CrossRef]
- Chandan, A.; Hattenberger, M.; El-Kharouf, A.; Du, S.; Dhir, A.; Self, V.; Pollet, B.G.; Ingram, A.; Bujalski, W. High temperature (HT) polymer electrolyte membrane fuel cells (PEMFC)—A review. J. Power Sources 2013, 231, 264–278. [Google Scholar] [CrossRef]
- Rosli, R.E.; Sulong, A.B.; Daud, W.R.W.; Zulkifley, M.A.; Husaini, T.; Rosli, M.I.; Majlan, E.H.; Haque, M.A. A review of high-temperature proton exchange membrane fuel cell (HT-PEMFC) system. Int. J. Hydrogen Energy 2017, 42, 9293–9314. [Google Scholar] [CrossRef]
- Xia, L.; Xu, Q.; He, Q.; Ni, M.; Seng, M. Numerical study of high temperature proton exchange membrane fuel cell (HT-PEMFC) with a focus on rib design. Int. J. Hydrogen Energy 2021, 46, 21098–21111. [Google Scholar] [CrossRef]
- Weng, F.B.; Dlamini, M.M.; Jung, G.B.; Lian, C.X. Analyses of reversible solid oxide cells porosity effects on tem-perature reduction. Int. J. Hydrogen Energy 2020, 45, 12170–12184. [Google Scholar] [CrossRef]
- Escorihuela, J.; García-Bernabé, A.; Montero, Á.; Sahuquillo, Ó.; Giménez, E.; Compañ, V. Ionic Liquid Composite Polybenzimidazol Membranes for High Temperature PEMFC Applications. Polymers 2019, 11, 732. [Google Scholar] [CrossRef] [PubMed]
- Teixeira, F.; de Sá, A.I.; Teixeira, A.P.S.; Ortiz-Martínez, V.; Ortiz, A.; Ortiz, I.; Rangel, C. New modified Nafion-bisphosphonic acid composite membranes for enhanced proton conductivity and PEMFC performance. Int. J. Hydrogen Energy 2021, 46, 17562–17571. [Google Scholar] [CrossRef]
- Larminie, J.; Dicks, A.; McDonald, M.S. Fuel Cell Systems Explained; J. Wiley: Chichester, UK, 2003; Volume 2, pp. 207–225. [Google Scholar]
- Lazarou, S.; Pyrgioti, E.; Alexandridis, A.T. A simple electric circuit model for proton exchange membrane fuel cells. J. Power Sources 2009, 190, 380–386. [Google Scholar] [CrossRef]
- Baroutaji, A.; Carton, J.G.; Sajjia, M.; Olabi, A.G. Materials in PEM fuel cells. In Reference Module in Materials Science and Materials Engineering; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Hwang, J.-J.; Dlamini, M.M.; Weng, F.-B.; Chang, T.; Lin, C.-H.; Weng, S.-C. Simulation of fine mesh implementation on the cathode for proton exchange membrane fuel cell (PEMFC). Energy 2021, 244, 122714. [Google Scholar] [CrossRef]
- Desai, A.N.; Mohanty, S.; Ramadesigan, V.; Singh, S.; Shaneeth, M. Simulating the effects of flow configurations on auxiliary power requirement and net power output of High-Temperature Proton Exchange Membrane Fuel Cell. Energy Convers. Manag. 2022, 259, 115557. [Google Scholar] [CrossRef]
- Davies, D.; Adcock, P.; Turpin, M.; Rowen, S. Bipolar plate materials for solid polymer fuel cells. J. Appl. Electrochem. 2000, 30, 101–105. [Google Scholar] [CrossRef]
- Electrical 4 U. Available online: https://www.electrical4u.com/electrical-resistance-and-laws-of-resistance/ (accessed on 14 March 2022).
- Ammosov, V.; Korablev, V.; Zaets, V. Electric field and currents in resistive plate chambers. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 1997, 401, 217–228. [Google Scholar] [CrossRef]
- Adelmann, C. On the extraction of resistivity and area of nanoscale interconnect lines by temperature-dependent resistance measurements. Solid-State Electron. 2019, 152, 72–80. [Google Scholar] [CrossRef]
- Grandi, M.; Mayer, K.; Gatalo, M.; Kapun, G.; Ruiz-Zepeda, F.; Marius, B.; Gaberšček, M.; Hacker, V. The Influence Catalyst Layer Thickness on Resistance Contributions of PEMFC Determined by Electrochemical Impedance Spectroscopy. Energies 2021, 14, 7299. [Google Scholar] [CrossRef]
- Lee, J.; Chevalier, S.; Banerjee, R.; Antonacci, P.; Ge, N.; Yip, R.; Kotaka, T.; Tabuchi, Y.; Bazylak, A. Investigating the effects of gas diffusion layer substrate thickness on polymer electrolyte membrane fuel cell performance via synchrotron X-ray radiography. Electrochim. Acta 2017, 236, 161–170. [Google Scholar] [CrossRef]
- Kulikovsky, A. Hydrogen crossover impedance of a PEM fuel cell at open circuit. Electrochim. Acta 2017, 247, 730–735. [Google Scholar] [CrossRef]
- Nguyen, T.-T.; Fushinobu, K. Effect of operating conditions and geometric structure on the gas crossover in PEM fuel cell. Sustain. Energy Technol. Assess. 2020, 37, 100584. [Google Scholar] [CrossRef]

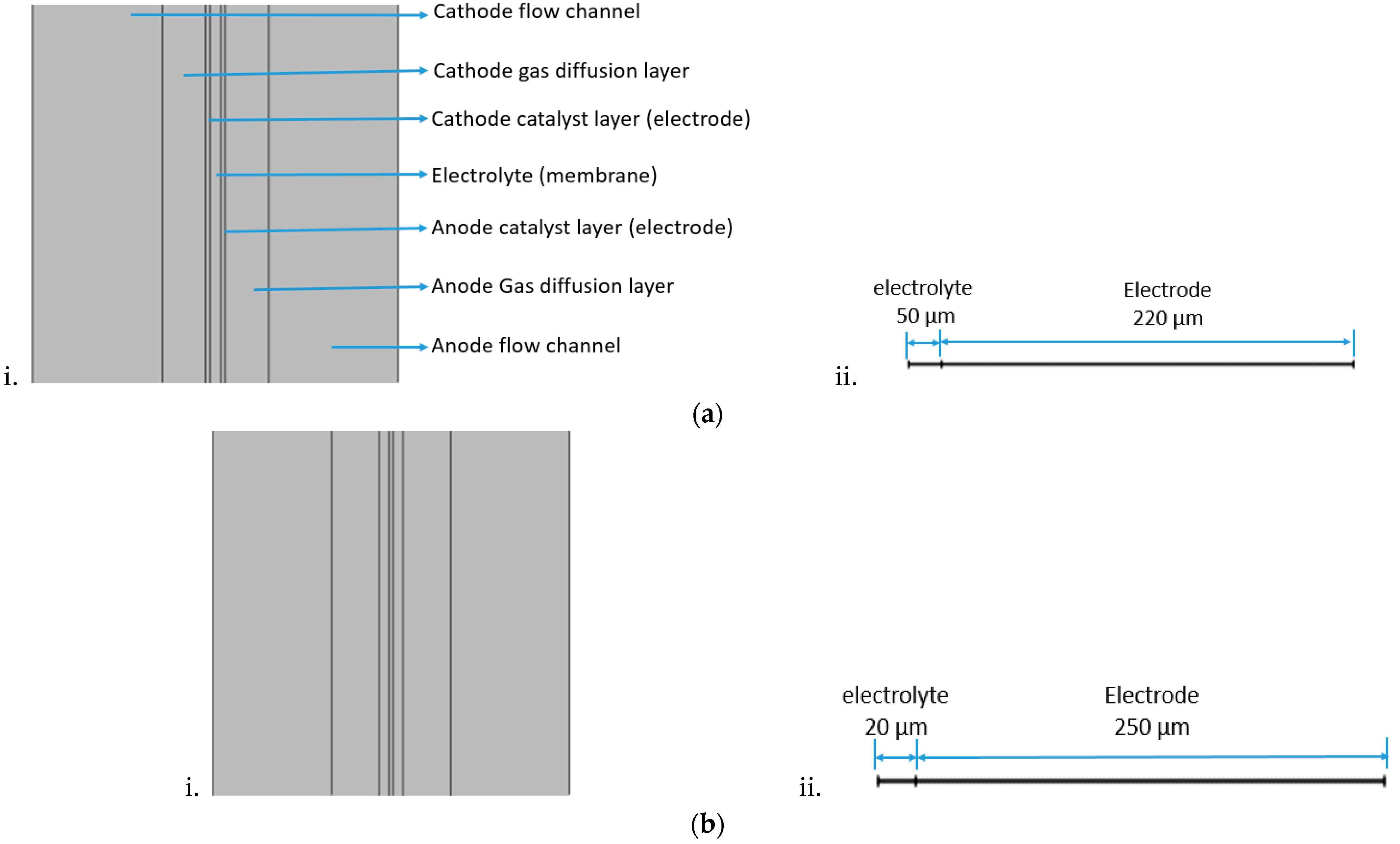




| Items | Cell 1 (Thin Electrode) | Cell 2 (Thick Electrode) | Cell 3 (Cell 1 with Improved Conductivity) |
|---|---|---|---|
| Electrolyte thickness (μm) | 50 | 20 | 50 |
| Electrode (μm) | 20 | 50 | 20 |
| GDL (μm) | 200 | 200 | 200 |
| Electrolyte Conductivity (S/m) | 9.825 | 9.825 | 49.825 |
| Electrode Conductivity (S/m) | 222 | 222 | 222 |
| Catalyst Porosity (%) | 35 | 35 | 35 |
| GDL Porosity (%) | 60 | 60 | 60 |
| Channel width (mm) | 1 | 1 | 1 |
| Channel depth (mm) | 1 | 1 | 1 |
| Rib width (mm) | 0.6 | 0.6 | 0.6 |
| Item | Values |
|---|---|
| Operating temperatures (°C) | 180 |
| Anode stoichiometry | 1.2 |
| Cathode stoichiometry | 3 |
| Reference pressure (Pa) | 101,325 |
| Anode velocity (m/s) | 0.2 |
| Cathode velocity (m/s) | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nawale, S.M.; Dlamini, M.M.; Weng, F.-B. Analyses of the Effects of Electrolyte and Electrode Thickness on High Temperature Proton Exchange Membrane Fuel Cell (H-TPEMFC) Quality. Membranes 2023, 13, 12. https://doi.org/10.3390/membranes13010012
Nawale SM, Dlamini MM, Weng F-B. Analyses of the Effects of Electrolyte and Electrode Thickness on High Temperature Proton Exchange Membrane Fuel Cell (H-TPEMFC) Quality. Membranes. 2023; 13(1):12. https://doi.org/10.3390/membranes13010012
Chicago/Turabian StyleNawale, Shubham Manoj, Mangaliso Menzi Dlamini, and Fang-Bor Weng. 2023. "Analyses of the Effects of Electrolyte and Electrode Thickness on High Temperature Proton Exchange Membrane Fuel Cell (H-TPEMFC) Quality" Membranes 13, no. 1: 12. https://doi.org/10.3390/membranes13010012
APA StyleNawale, S. M., Dlamini, M. M., & Weng, F.-B. (2023). Analyses of the Effects of Electrolyte and Electrode Thickness on High Temperature Proton Exchange Membrane Fuel Cell (H-TPEMFC) Quality. Membranes, 13(1), 12. https://doi.org/10.3390/membranes13010012





