A Simple 1D Convection-Diffusion Model of Oxalic Acid Oxidation Using Reactive Electrochemical Membrane
Abstract
1. Introduction
2. Mathematical Model
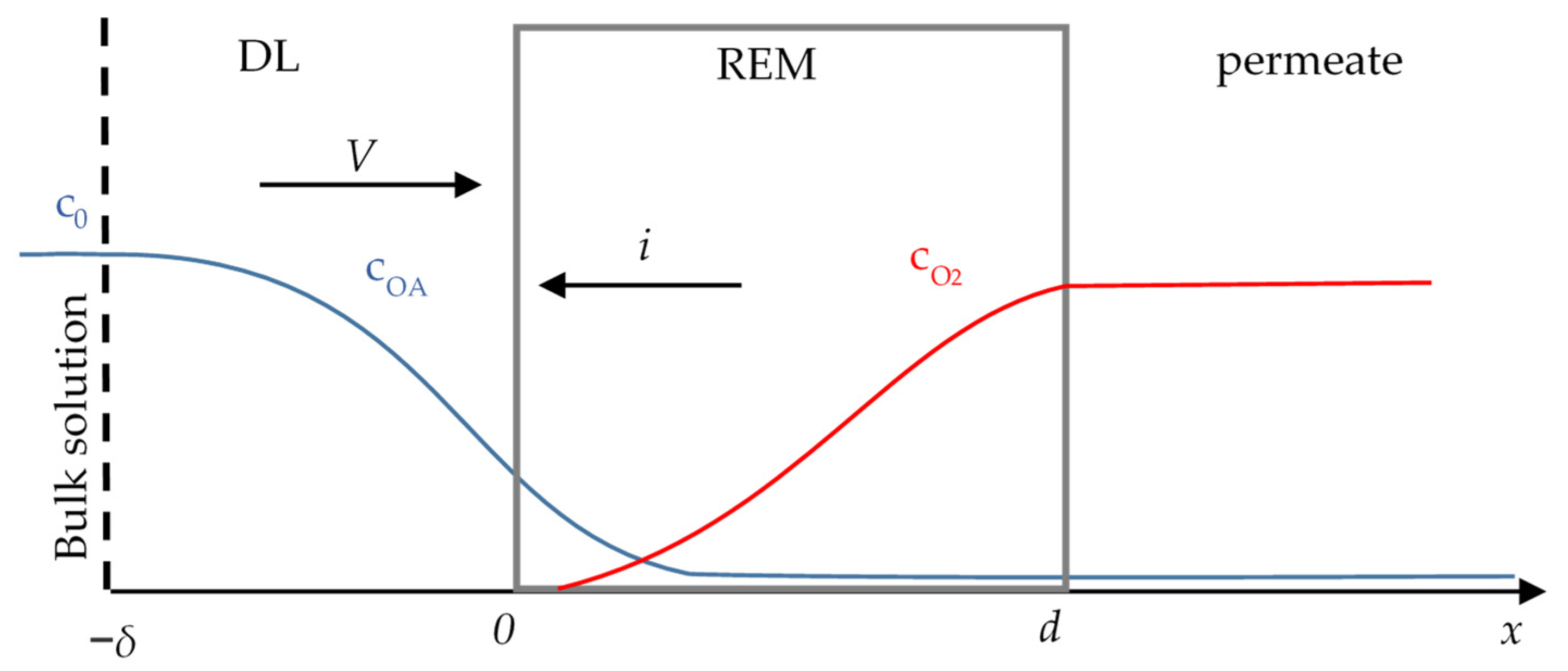
2.1. The Geometry of the System under Study
2.2. The Problem Formulation
- The transport number of organic compound is negligible compared to the transport number of the supporting electrolyte. Thus, only diffusion and convection fluxes are considered;
- The system under study is in a steady state, thus only the faradaic current is taken into account;
- Since the experiment proceeds under room conditions and the supporting electrolyte does not participate in the reactions, the gradients of temperature, activity coefficients, and density are ignored;
- The oxygen concentration in the solution exceeds the solubility limit only at the lowest TOC fluxes and is insufficient at given current density [31]; thus, the bubble-formation caused by oxygen evolution is not taken into account;
- The rate constant of oxalic acid oxidation by hydroxyl radicals is very small, thus, we assume that all the hydroxyl radicals are spent on the oxygen molecules formation;
- According to the conditions of the experiment [5], the bulk solution is considered perfectly mixed and renewable, so the oxalic acid concentration in it is assumed constant.
3. Results and Discussion
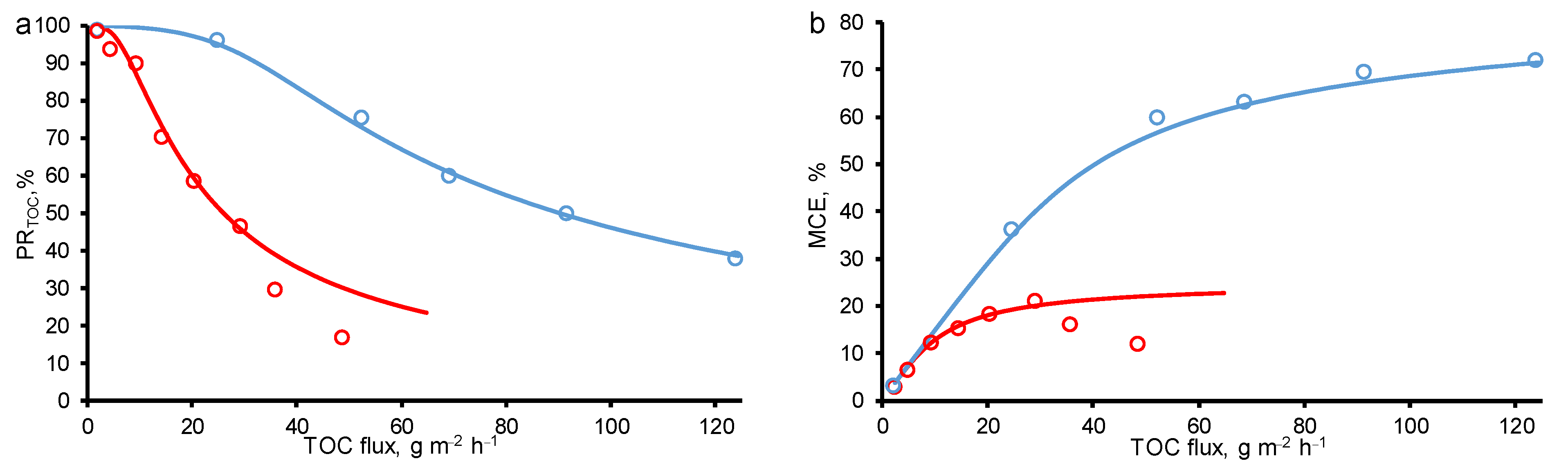
3.1. The Treatment of Experimental Data
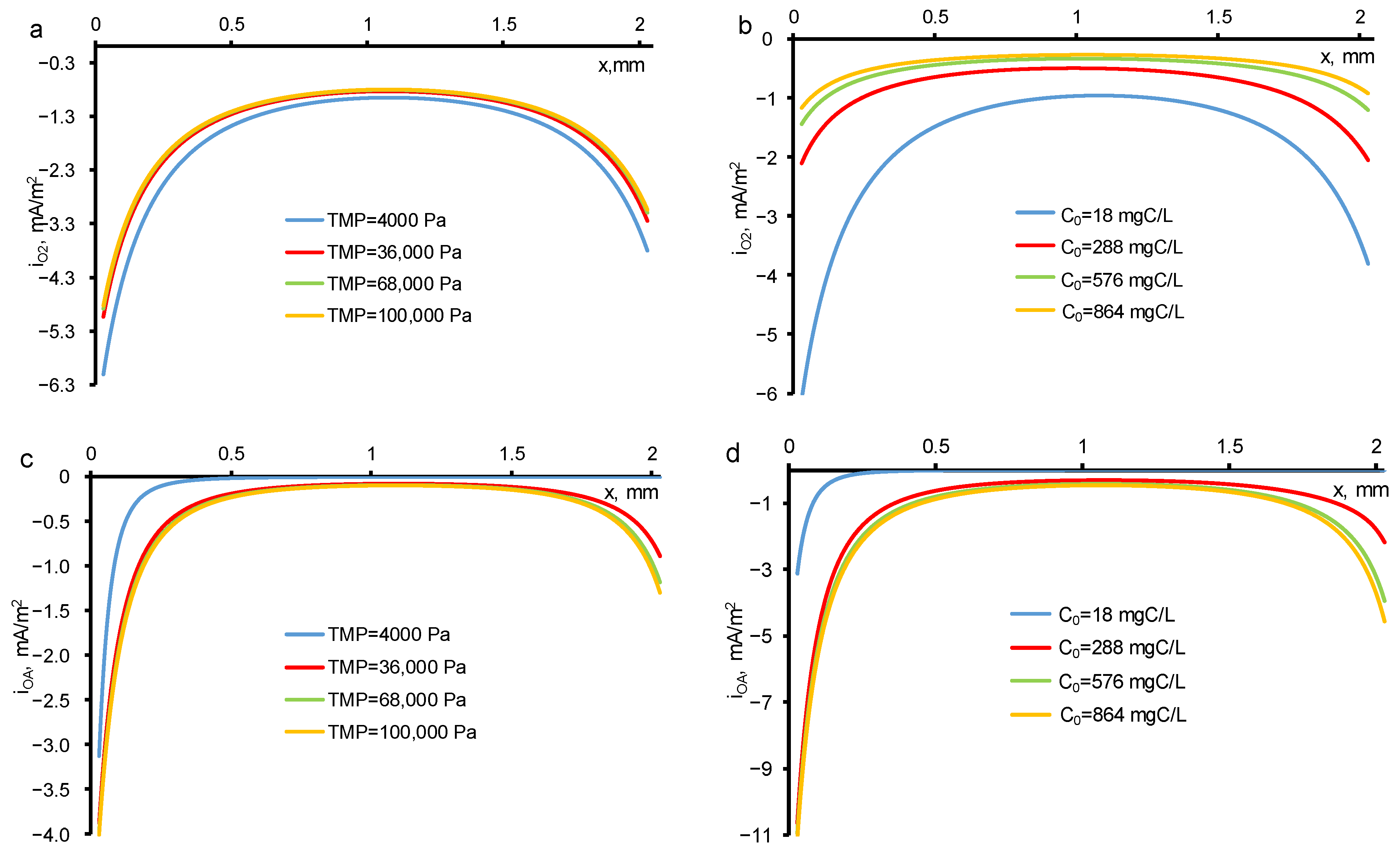
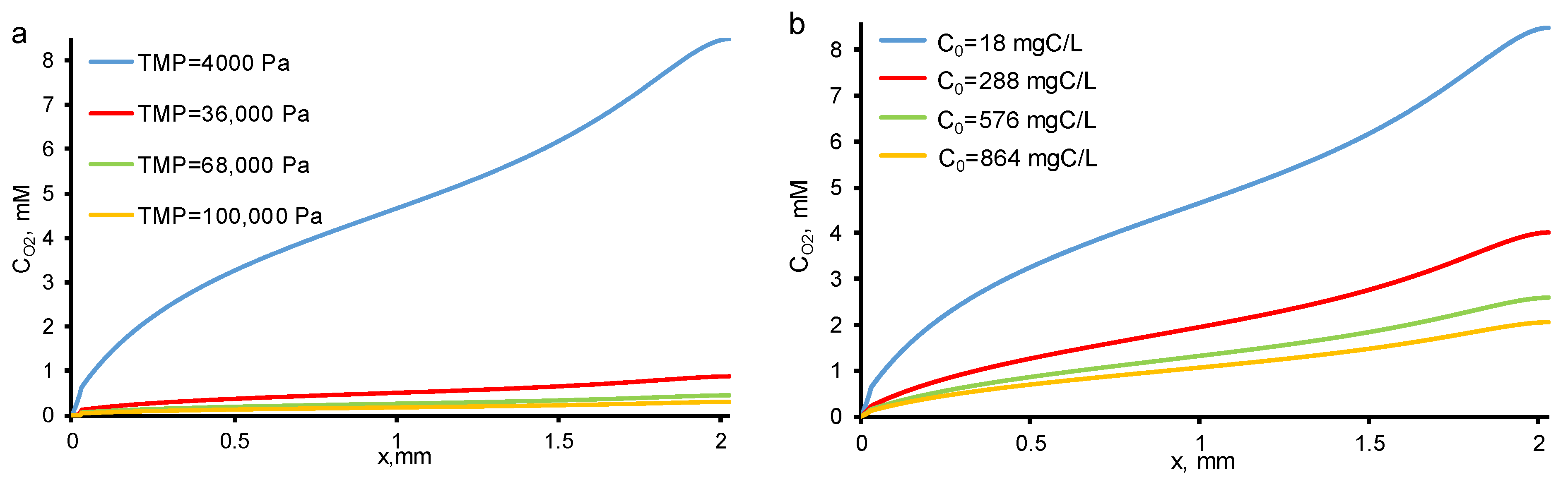
3.2. Effect of Increasing Concentration at a Constant Transmembrane Pressure
3.3. Effect of Increasing Transmembrane Pressure at Constant Concentration
3.4. The Oxygen Evolution in Pores of the Reactive Electrochemical Membrane
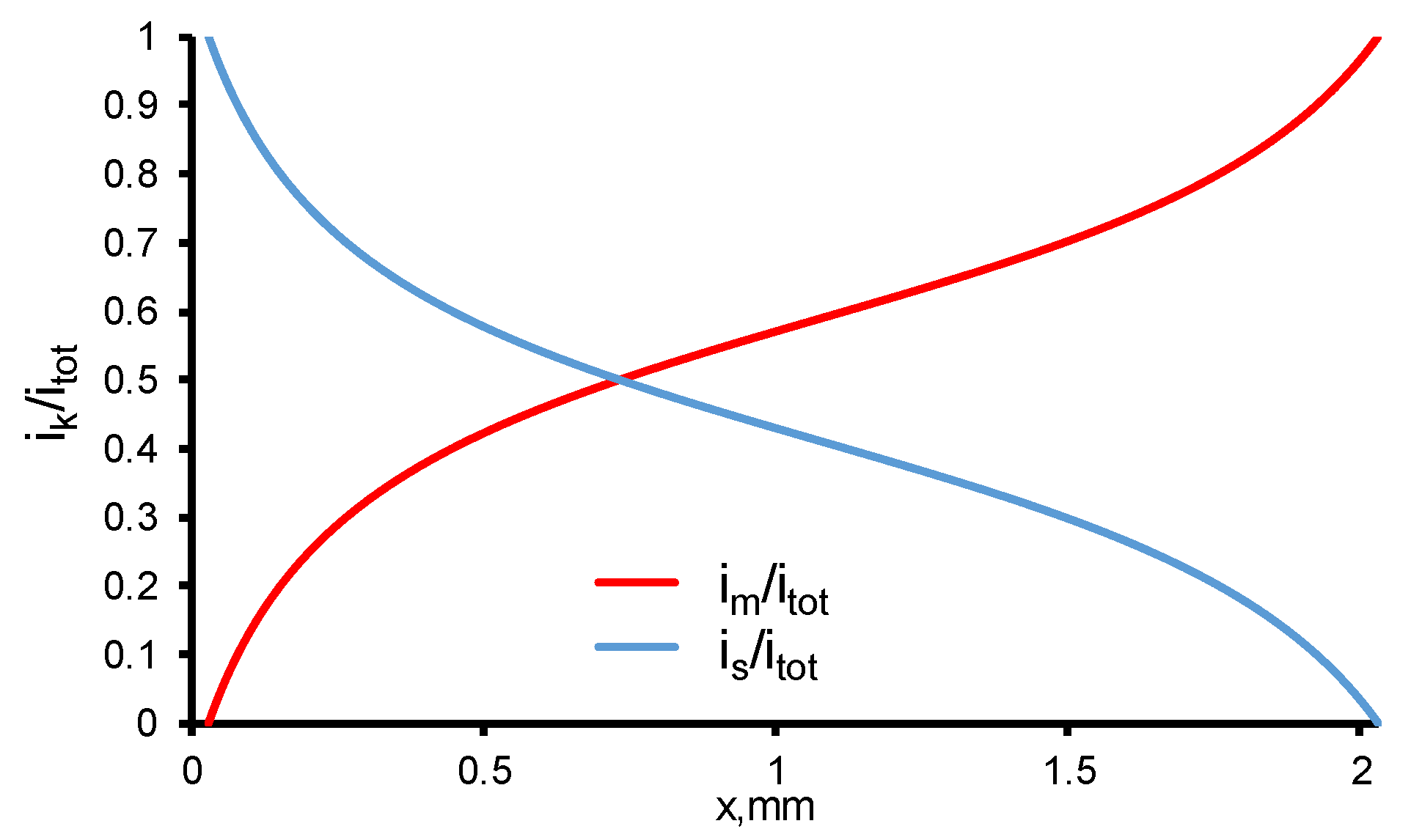
3.5. The Current Density, Potential, and Overpotential Distributions
3.6. Effect of Current Density on Mineralization Current Efficiency
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- The United Nations World Water Development Report 2020: Water and Climate Change; United Nations Educational, Scientific and Cultural Organization: Paris, France, 2020.
- Sirés, I.; Brillas, E.; Oturan, M.A.; Rodrigo, M.A.; Panizza, M. Electrochemical advanced oxidation processes: Today and tomorrow. A review. Environ. Sci. Pollut. Res. 2014, 21, 8336–8367. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Huitle, C.A.; Ferro, S. Electrochemical oxidation of organic pollutants for the wastewater treatment: Direct and indirect processes. Chem. Soc. Rev. 2006, 35, 1324–1340. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Huitle, C.A.; Rodrigo, M.A.; Sirés, I.; Scialdone, O. Single and Coupled Electrochemical Processes and Reactors for the Abatement of Organic Water Pollutants: A Critical Review. Chem. Rev. 2015, 115, 13362–13407. [Google Scholar] [CrossRef]
- Trellu, C.; Coetsier, C.; Rouch, J.-C.C.; Esmilaire, R.; Rivallin, M.; Cretin, M.; Causserand, C. Mineralization of organic pollutants by anodic oxidation using reactive electrochemical membrane synthesized from carbothermal reduction of TiO2. Water Res. 2018, 131, 310–319. [Google Scholar] [CrossRef] [PubMed]
- Comninellis, C. Electrocatalysis in the electrochemical conversion/combustion of organic pollutants for waste water treatment. Electrochim. Acta 1994, 39, 1857–1862. [Google Scholar] [CrossRef]
- Panizza, M.; Cerisola, G. Direct and Mediated Anodic Oxidation of Organic Pollutants. Chem. Rev. 2009, 109, 6541–6569. [Google Scholar] [CrossRef] [PubMed]
- Brillas, E.; Martínez-Huitle, C.A. Decontamination of wastewaters containing synthetic organic dyes by electrochemical methods. An updated review. Appl. Catal. B Environ. 2015, 166–167, 603–643. [Google Scholar] [CrossRef]
- Oturan, N.; Brillas, E.; Oturan, M.A. Unprecedented total mineralization of atrazine and cyanuric acid by anodic oxidation and electro-Fenton with a boron-doped diamond anode. Environ. Chem. Lett. 2012, 10, 165–170. [Google Scholar] [CrossRef]
- Oturan, N.; Van Hullebusch, E.D.; Zhang, H.; Mazeas, L.; Budzinski, H.; Le Menach, K.; Oturan, M.A. Occurrence and Removal of Organic Micropollutants in Landfill Leachates Treated by Electrochemical Advanced Oxidation Processes. Environ. Sci. Technol. 2015, 49, 12187–12196. [Google Scholar] [CrossRef]
- Trellu, C.; Oturan, N.; Pechaud, Y.; van Hullebusch, E.D.; Esposito, G.; Oturan, M.A. Anodic oxidation of surfactants and organic compounds entrapped in micelles—Selective degradation mechanisms and soil washing solution reuse. Water Res. 2017, 118, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Fernandes, A.; Pacheco, M.J.; Ciríaco, L.; Lopes, A. Anodic oxidation of a biologically treated leachate on a boron-doped diamond anode. J. Hazard. Mater. 2012, 199–200, 82–87. [Google Scholar] [CrossRef] [PubMed]
- Brillas, E.; Sirés, I.; Arias, C.; Cabot, P.L.; Centellas, F.; Rodríguez, R.M.; Garrido, J.A. Mineralization of paracetamol in aqueous medium by anodic oxidation with a boron-doped diamond electrode. Chemosphere 2005, 58, 399–406. [Google Scholar] [CrossRef] [PubMed]
- Andreozzi, R.; Caprio, V.; Marotta, R.; Vogna, D. Paracetamol oxidation from aqueous solutions by means of ozonation and H2O2/UV system. Water Res. 2003, 37, 993–1004. [Google Scholar] [CrossRef]
- Trellu, C.; Chaplin, B.P.; Coetsier, C.; Esmilaire, R.; Cerneaux, S.; Causserand, C.; Cretin, M. Electro-oxidation of organic pollutants by reactive electrochemical membranes. Chemosphere 2018, 208, 159–175. [Google Scholar] [CrossRef] [PubMed]
- Kapałka, A.; Fóti, G.; Comninellis, C. The importance of electrode material in environmental electrochemistry: Formation and reactivity of free hydroxyl radicals on boron-doped diamond electrodes. Electrochim. Acta 2009, 54, 2018–2023. [Google Scholar] [CrossRef]
- Kapałka, A.; Fóti, G.; Comninellis, C. Kinetic modelling of the electrochemical mineralization of organic pollutants for wastewater treatment. J. Appl. Electrochem. 2008, 38, 7–16. [Google Scholar] [CrossRef]
- Vecitis, C.D.; Gao, G.; Liu, H. Electrochemical carbon nanotube filter for adsorption, desorption, and oxidation of aqueous dyes and anions. J. Phys. Chem. C 2011, 115, 3621–3629. [Google Scholar] [CrossRef]
- Zaky, A.M.; Chaplin, B.P. Porous substoichiometric TiO2 anodes as reactive electrochemical membranes for water treatment. Environ. Sci. Technol. 2013, 47, 6554–6563. [Google Scholar] [CrossRef]
- Bakr, A.R.; Rahaman, M.S. Crossflow electrochemical filtration for elimination of ibuprofen and bisphenol a from pure and competing electrolytic solution conditions. J. Hazard. Mater. 2019, 365, 615–621. [Google Scholar] [CrossRef]
- Simond, O.; Schaller, V.; Comninellis, C. Theoretical model for the anodic oxidation of organics on metal oxide electrodes. Electrochim. Acta 1997, 42, 2009–2012. [Google Scholar] [CrossRef]
- Panizza, M.; Michaud, P.A.; Cerisola, G.; Comninellis, C.H. Anodic oxidation of 2-naphthol at boron-doped diamond electrodes. J. Electroanal. Chem. 2001, 507, 206–214. [Google Scholar] [CrossRef]
- Scialdone, O. Electrochemical oxidation of organic pollutants in water at metal oxide electrodes: A simple theoretical model including direct and indirect oxidation processes at the anodic surface. Electrochim. Acta 2009, 54, 6140–6147. [Google Scholar] [CrossRef]
- Lan, Y.; Coetsier, C.; Causserand, C.; Groenen Serrano, K. An experimental and modelling study of the electrochemical oxidation of pharmaceuticals using a boron-doped diamond anode. Chem. Eng. J. 2018, 333, 486–494. [Google Scholar] [CrossRef]
- Cañizares, P.; García-Gómez, J.; Lobato, J.; Rodrigo, M.A. Modeling of Wastewater Electro-oxidation Processes Part I. Application to Active Electrodes. Ind. Eng. Chem. Res. 2004, 43, 1923–1931. [Google Scholar] [CrossRef]
- Mascia, M.; Vacca, A.; Polcaro, A.M.; Palmas, S.; Ruiz, J.R.; Da Pozzo, A. Electrochemical treatment of phenolic waters in presence of chloride with boron-doped diamond (BDD) anodes: Experimental study and mathematical model. J. Hazard. Mater. 2010, 174, 314–322. [Google Scholar] [CrossRef]
- Savall, A.; Groenen-Serrano, K.; Weiss-Hortala, E.; Savall, A.; Spiteri, P. Role of Hydroxyl Radicals During the Competitive Electrooxidation of Organic Compounds on a Boron-Doped Diamond Anode. Electrocatalysis 2013, 4, 346–352. [Google Scholar] [CrossRef]
- Jing, Y.; Chaplin, B.P. Electrochemical impedance spectroscopy study of membrane fouling characterization at a conductive sub-stoichiometric TiO2 reactive electrochemical membrane: Transmission line model development. J. Memb. Sci. 2016, 511, 238–249. [Google Scholar] [CrossRef]
- Misal, S.N.; Lin, M.H.; Mehraeen, S.; Chaplin, B.P. Modeling electrochemical oxidation and reduction of sulfamethoxazole using electrocatalytic reactive electrochemical membranes. J. Hazard. Mater. 2020, 384, 121420. [Google Scholar] [CrossRef]
- Skolotneva, E.; Trellu, C.; Cretin, M.; Mareev, S. A 2D convection-diffusion model of anodic oxidation of organic compounds mediated by hydroxyl radicals using porous reactive electrochemical membrane. Membranes 2020, 10, 102. [Google Scholar] [CrossRef] [PubMed]
- Mareev, S.; Skolotneva, E.; Cretin, M.; Nikonenko, V. Modeling the Formation of Gas Bubbles inside the Pores of Reactive Electrochemical Membranes in the Process of the Anodic Oxidation of Organic Compounds. Int. J. Mol. Sci. 2021, 22, 5477. [Google Scholar] [CrossRef]
- Mascia, M.; Vacca, A.; Palmas, S.; Polcaro, A.M. Kinetics of the electrochemical oxidation of organic compounds at BDD anodes: Modelling of surface reactions. J. Appl. Electrochem. 2007, 37, 71–76. [Google Scholar] [CrossRef]
- Donaghue, A.; Chaplin, B.P. Effect of Select Organic Compounds on Perchlorate Formation at Boron-doped Diamond Film Anodes. Environ. Sci. Technol. 2013, 47, 12391–12399. [Google Scholar] [CrossRef] [PubMed]
- Doherty, T.; Sunderland, J.G.; Roberts, E.P.L.; Pickett, D.J. An improved model of potential and current distribution within a flow-through porous electrode. Electrochim. Acta 1996, 41, 519–526. [Google Scholar] [CrossRef]
- Trainham, J.A. A Flow-Through Porous Electrode Model: Application to Metal-Ion Removal from Dilute Streams. J. Electrochem. Soc. 1977, 124, 1528. [Google Scholar] [CrossRef]
- Siahrostami, S.; Li, G.-L.; Viswanathan, V.; Nørskov, J.K. One- or Two-Electron Water Oxidation, Hydroxyl Radical, or H2O2 Evolution. J. Phys. Chem. Lett. 2017, 8, 1157–1160. [Google Scholar] [CrossRef]
- Weiss, E.; Groenen-Serrano, K.; Savall, A.; Comninellis, C. A kinetic study of the electrochemical oxidation of maleic acid on boron doped diamond. J. Appl. Electrochem. 2007, 37, 41–47. [Google Scholar] [CrossRef]
- Martínez-Huitle, C.A.; Ferro, S.; De Battisti, A. Electrochemical incineration of oxalic acid: Role of electrode material. Electrochim. Acta 2004, 49, 4027–4034. [Google Scholar] [CrossRef]
- Garcia-Segura, S.; Brillas, E. Mineralization of the recalcitrant oxalic and oxamic acids by electrochemical advanced oxidation processes using a boron-doped diamond anode. Water Res. 2011, 45, 2975–2984. [Google Scholar] [CrossRef]
- Guo, L.; Jing, Y.; Chaplin, B.P. Development and Characterization of Ultra fi ltration TiO2 Magnéli Phase Reactive Electrochemical Membranes. Environ. Sci. Technol. 2016, 50, 1428–1436. [Google Scholar] [CrossRef]
- Šljukić, B.; Baron, R.; Compton, R.G. Electrochemical determination of oxalate at pyrolytic graphite electrodes. Electroanalysis 2007, 19, 918–922. [Google Scholar] [CrossRef]
- Jamnongwong, M.; Loubiere, K.; Dietrich, N.; Hébrard, G. Experimental study of oxygen diffusion coefficients in clean water containing salt, glucose or surfactant: Consequences on the liquid-side mass transfer coefficients. Chem. Eng. J. 2010, 165, 758–768. [Google Scholar] [CrossRef]




| Parameter | Definition | Value | Reference |
|---|---|---|---|
| εs | fraction volume of solution in REM (porosity) | 0.41 | [5] |
| εm | fraction volume of electrode material in REM | 1 − εs | [5] |
| DOA | diffusion coefficient of OA | 1.0 × 10−9 m2/s | [41] |
| DO2 | diffusion coefficient of O2 | 2.0 × 10−9 m2/s | [42] |
| dispersion coefficient | v × 3 × 10−4 m | * | |
| av | specific surface area of the electrode | 108 1/m | [5] |
| electrolyte conductivity | 1.3 S/m | [5] | |
| electrode conductivity | 1.3 S/m | ||
| σ | permeability coefficient | 1.7 × 10−14 m2 | [5] |
| μ | dynamic viscosity | 8.9 × 10−4 Pa×s | |
| d | REM thickness | 2 mm | [5] |
| exchange current density of OA | −10−6 A/m2 | * | |
| exchange current density of O2 | −10−6 A/m2 | * | |
| concentration of OA to which the exchange current density is referred | 0.75 mol/m3 | * | |
| electron transferred coefficient in reaction (11) | 0.5 | * | |
| electron transferred coefficient in reaction (10) | 0.125 | * | |
| number of electrons transferred in reaction (11) | 2 | ||
| number of electrons transferred in reaction (10) | 4 | ||
| formal potential for oxidation of OA | 2.02 V | * | |
| formal potential in reaction (11) | 1.8 V | * | |
| stoichiometric coefficient of OA in reaction (11) | −1 | ||
| stoichiometric coefficient of O2 in reaction (10) | 1 | ||
| itot | total current density | −150 A/m2 | [5] |
| temperature | 298.15 K | [5] | |
| δ | diffusion layer thickness | 30 μm | [30] |
| km | mass transfer coefficient | [35] | |
| ψ | shape factor | 0.86 | |
| ν | kinematic viscosity | 8.9 × 10−7 m2/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Skolotneva, E.; Cretin, M.; Mareev, S. A Simple 1D Convection-Diffusion Model of Oxalic Acid Oxidation Using Reactive Electrochemical Membrane. Membranes 2021, 11, 431. https://doi.org/10.3390/membranes11060431
Skolotneva E, Cretin M, Mareev S. A Simple 1D Convection-Diffusion Model of Oxalic Acid Oxidation Using Reactive Electrochemical Membrane. Membranes. 2021; 11(6):431. https://doi.org/10.3390/membranes11060431
Chicago/Turabian StyleSkolotneva, Ekaterina, Marc Cretin, and Semyon Mareev. 2021. "A Simple 1D Convection-Diffusion Model of Oxalic Acid Oxidation Using Reactive Electrochemical Membrane" Membranes 11, no. 6: 431. https://doi.org/10.3390/membranes11060431
APA StyleSkolotneva, E., Cretin, M., & Mareev, S. (2021). A Simple 1D Convection-Diffusion Model of Oxalic Acid Oxidation Using Reactive Electrochemical Membrane. Membranes, 11(6), 431. https://doi.org/10.3390/membranes11060431







