Exergy Analysis of a Direct Contact Membrane Distillation (DCMD) System Based on Computational Fluid Dynamics (CFD)
Abstract
:1. Introduction
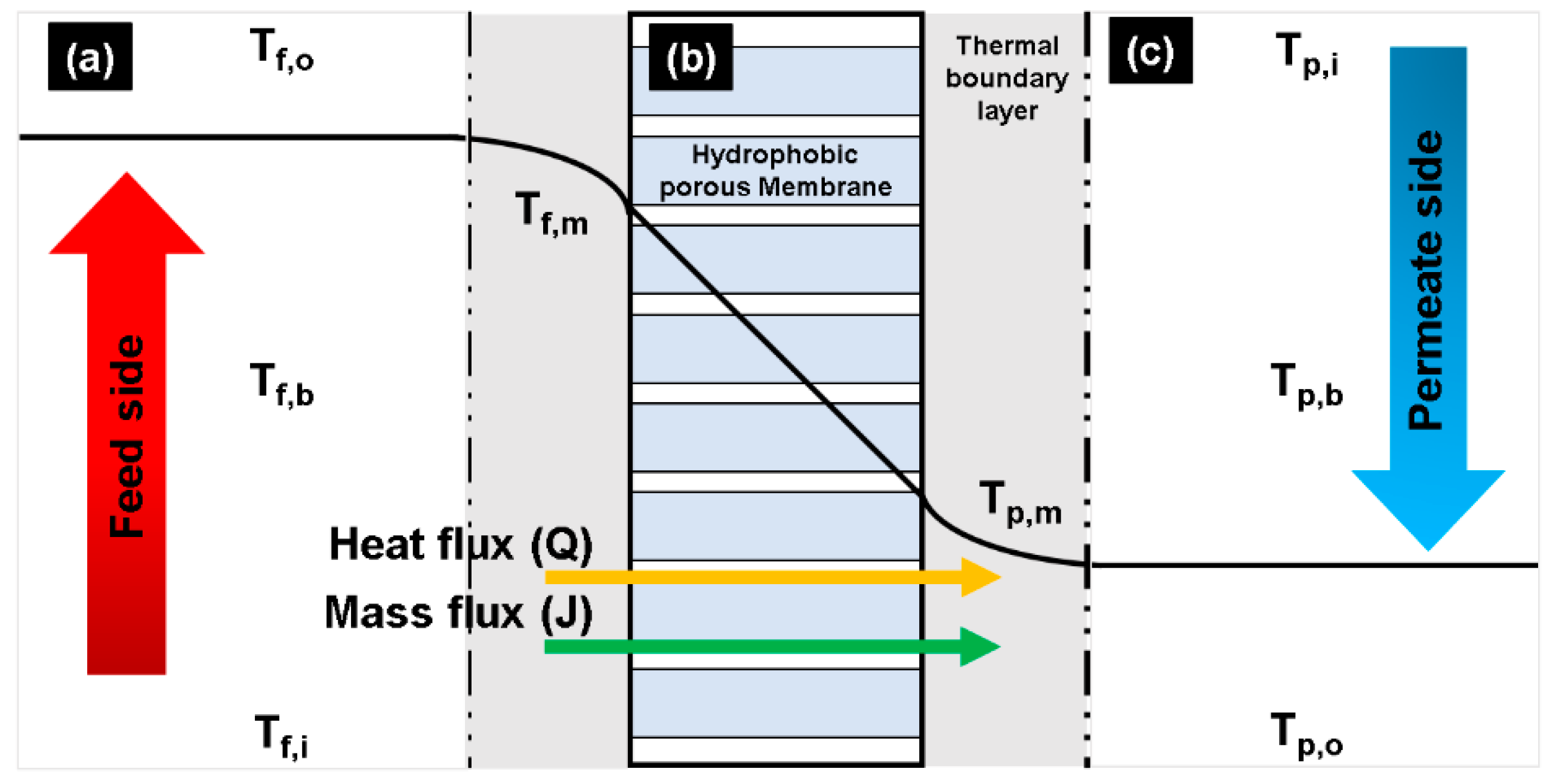
2. Theory
- Both the feed and permeate have a laminar flow regime and are in a steady state.
- Heat loss to the ambient environment is negligible.
- No chemical reaction occurs.
- Convective transport of water vapor via the membrane pore is negligible.
2.1. Computational Fluid Dynamics Model Equation
2.2. Temperature Polarization Coefficient
2.3. Analysis of Exergy Destruction
3. Materials and Methods
4. Results
4.1. Verification of the Computational Fluid Dynamics Model
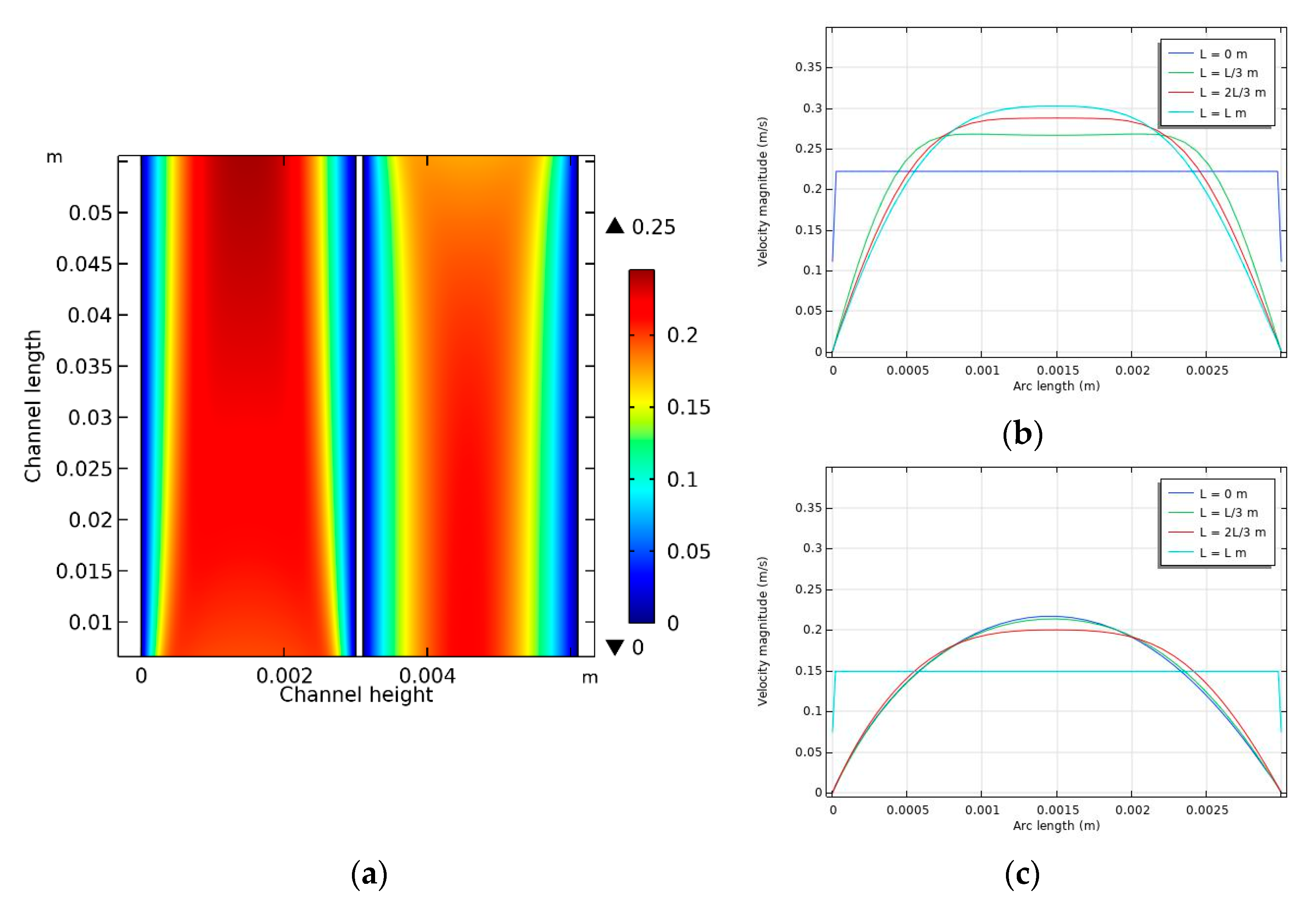
4.2. Velocity Distribution
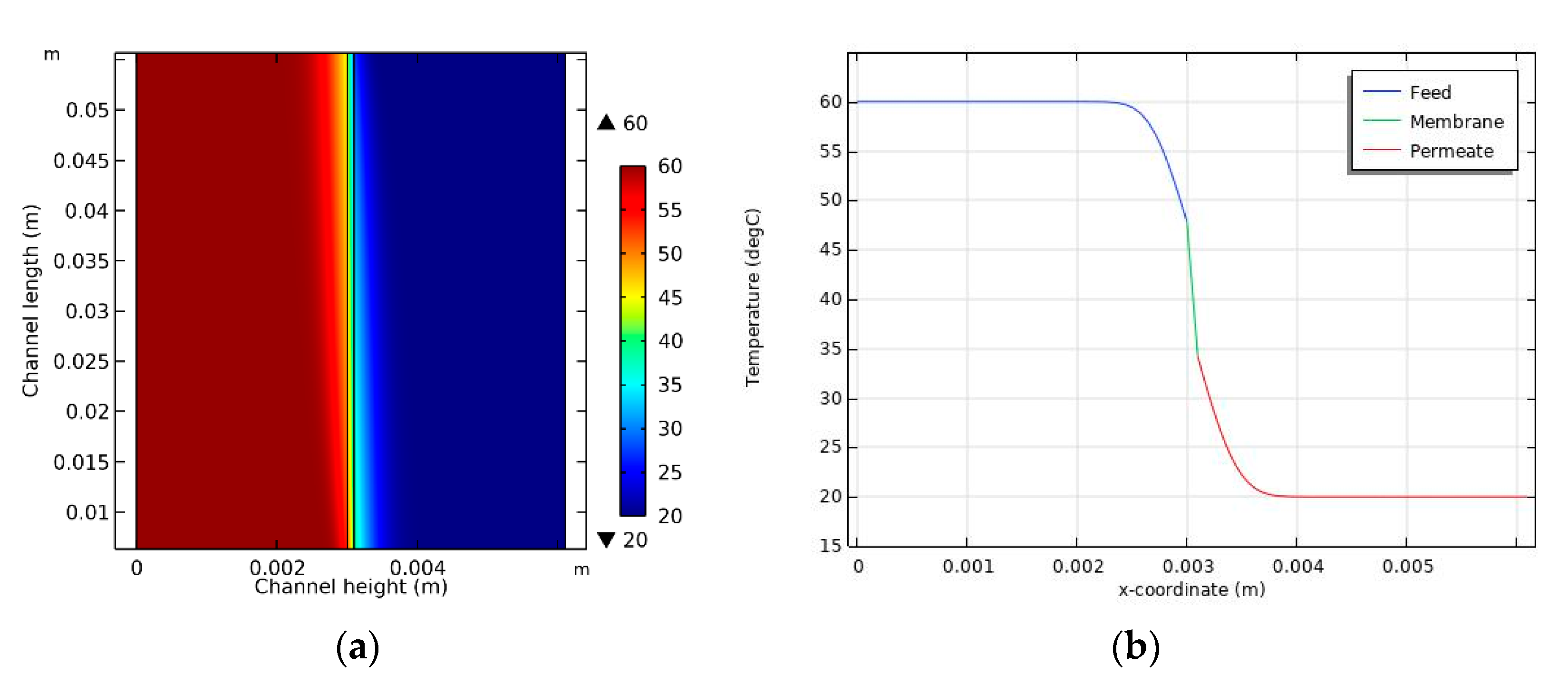
4.3. Temperature Distribution
4.4. Vapor Pressure and Flux
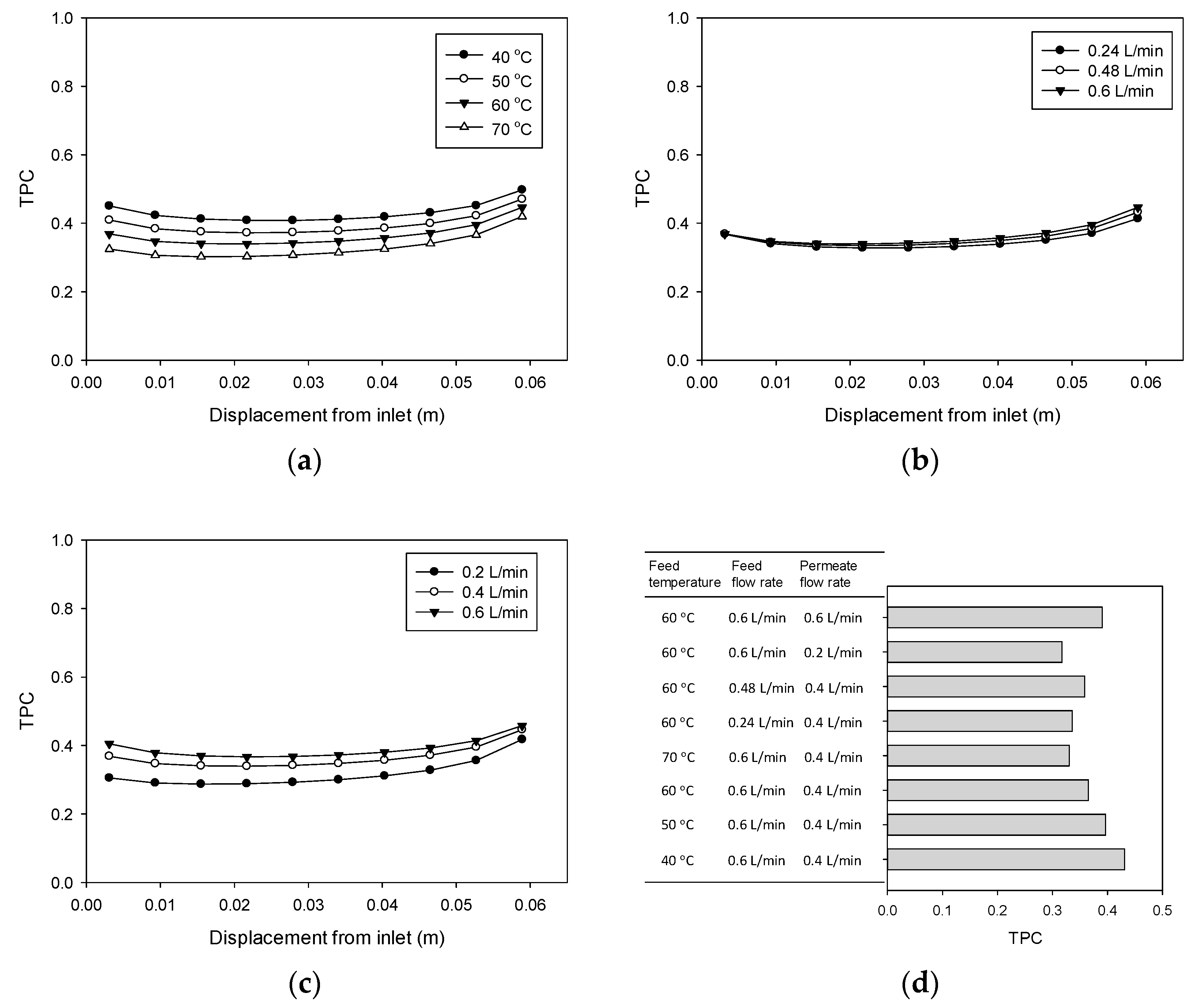
4.5. Temperature Polairzation (TP) Phenomenon
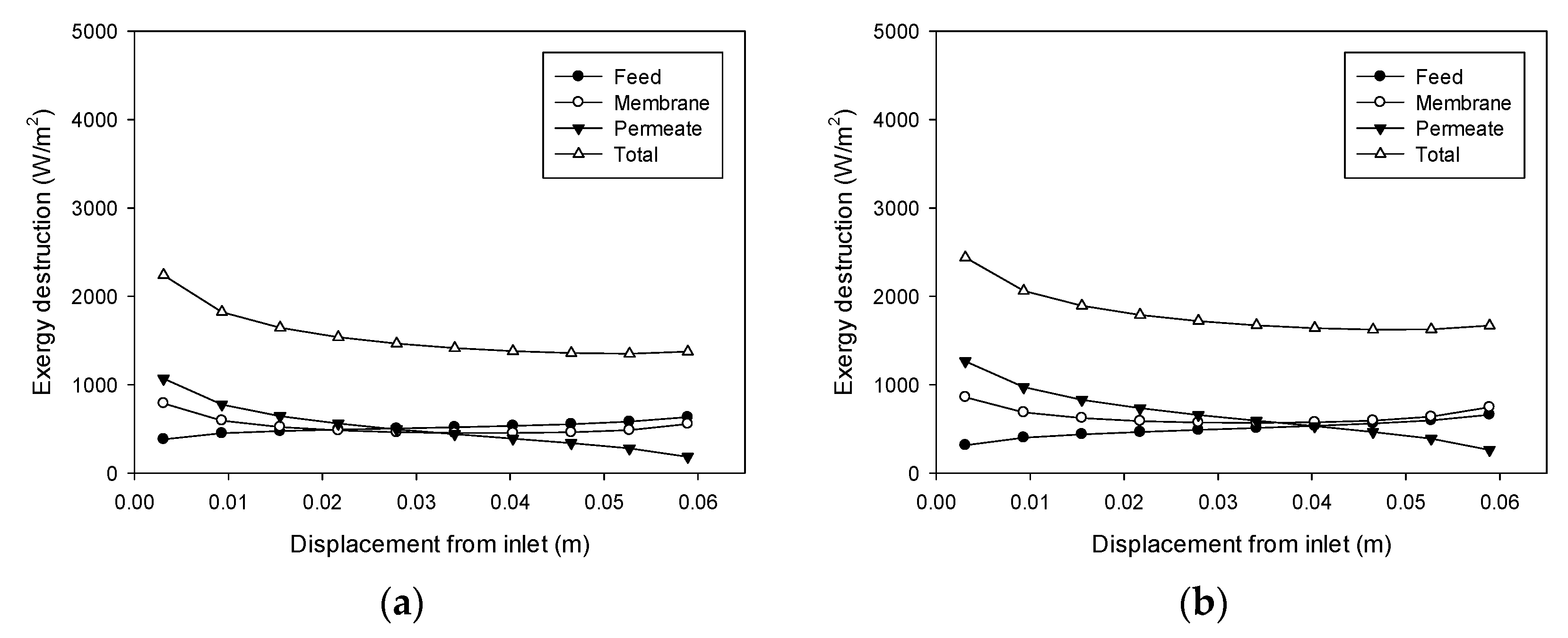
4.6. Exergy Destruction Profiles
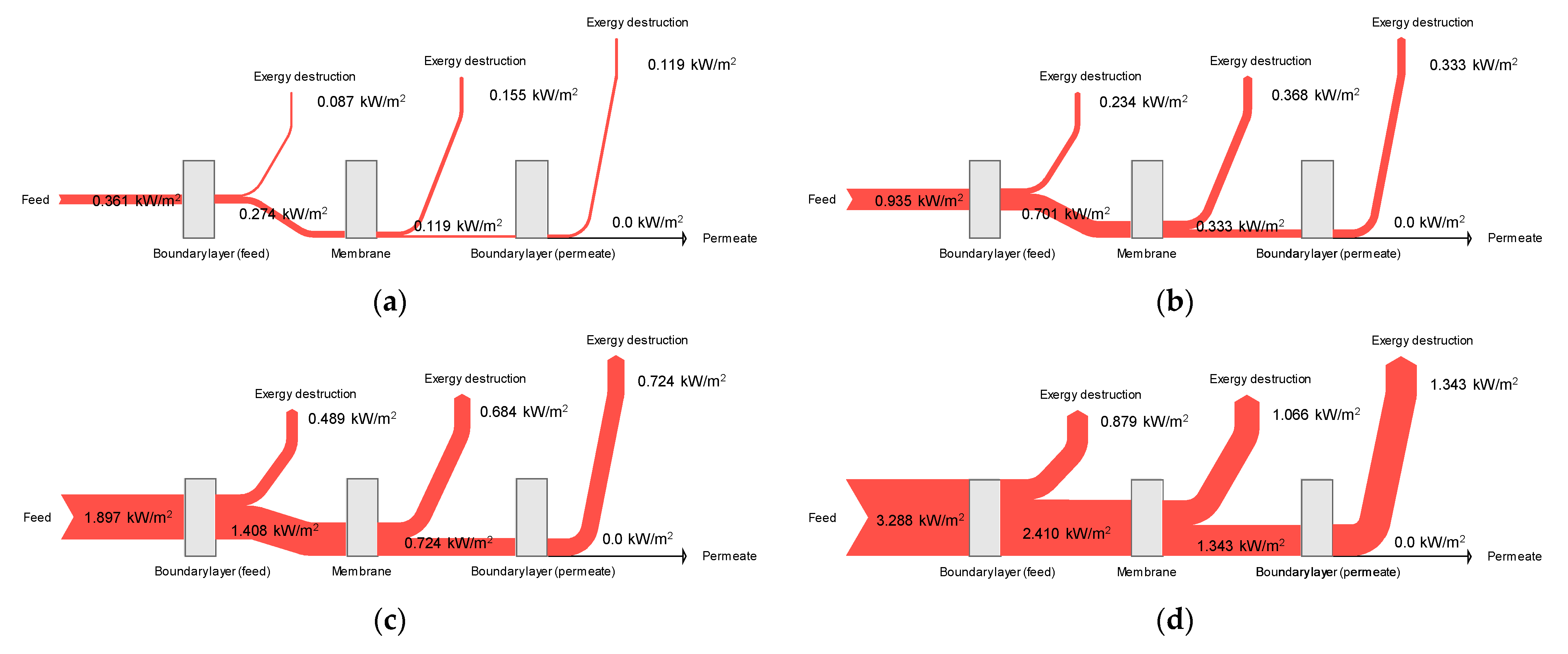
4.7. Exergy Flow Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Water activity in NaCl solution | |
| c | Concentration of water, mol/m3 |
| Feed-membrane concentration, mol/m3 | |
| Membrane-permeate concentration, mol/m3 | |
| Heat capacity, J/[kg·K] | |
| Membrane pore size, m | |
| Knudsen diffusion coefficient, m2/s | |
| Poiseuille flow coefficient, m2/s | |
| ED | Energy destruction, kW/m2 |
| Efeed | Exergy destruction on the feed side, kW/m2 |
| Emembrane | Exergy destruction in the membrane, kW/m2 |
| Epermeate | Exergy destruction on the permeate side, kW/m2 |
| F | Volume force vector |
| I | Unit tensor |
| Thermal conductivity coefficients of membrane, W/[m·K] | |
| Thermal conductivity coefficients of vapor, W/[m·K] | |
| Thermal conductivity coefficients of solid, W/[m·K] | |
| M | Water(vapor) molecular weight, g/mol |
| Dynamic pressure, Pa | |
| P | Mean pressure, Pa |
| Heat flux, W/m2 | |
| Q | Heat source, W/m3 |
| QT | Total heat transferred by the membrane, kW |
| R | Gas constant, J/[mol·K] |
| T | Temperature, K |
| T0 | Temperature of the environment, °C |
| Mean temperature, K | |
| Feed temperature on the membrane surface, °C | |
| Permeate temperature on the membrane surface, °C | |
| Feed temperature in bulk fluid, °C | |
| Permeated temperature in bulk fluid, °C | |
| Velocity vector, m/s | |
| Liquid mole fraction of water | |
| Liquid mole fraction of NaCl solution | |
| Density, kg/m3 | |
| Viscosity, N·s/m2 | |
| Porosity of the membrane | |
| Tortuosity of the membrane | |
| Dell operator |
References
- Khayet, M.; Matsuura, T. Membrane Distillation: Principles and Applications; Elsevier: Oxford, UK, 2011. [Google Scholar]
- Alkhudhiri, A.; Darwish, N.; Hilal, N. Membrane distillation: A comprehensive review. Desalination 2012, 287, 2–18. [Google Scholar] [CrossRef]
- Deshmukh, A.; Boo, C.; Karanikola, V.; Lin, S.; Straub, A.P.; Tong, T.; Warsinger, D.M.; Elimelech, M. Membrane distillation at the water-energy nexus: Limits, opportunities, and challenges. Energy Environ. Sci. 2018, 11, 1177–1196. [Google Scholar] [CrossRef]
- Ali, A.; Criscuoli, A.; Macedonio, F.; Drioli, E. A comparative analysis of flat sheet and capillary membranes for membrane distillation applications. Desalination 2019, 456, 1–12. [Google Scholar] [CrossRef]
- González, D.; Amigo, J.; Suárez, F. Membrane distillation: Perspectives for sustainable and improved desalination. Renew. Sustain. Energy Rev. 2017, 80, 238–259. [Google Scholar] [CrossRef]
- Quist-Jensen, C.A.; Macedonio, F.; Drioli, E. Membrane technology for water production in agriculture: Desalination and wastewater reuse. Desalination 2015, 364, 17–32. [Google Scholar] [CrossRef]
- Tow, E.W.; Warsinger, D.M.; Trueworthy, A.M.; Swaminathan, J.; Thiel, G.P.; Zubair, S.M.; Myerson, A.S. Comparison of fouling propensity between reverse osmosis, forward osmosis, and membrane distillation. J. Membr. Sci. 2018, 556, 352–364. [Google Scholar] [CrossRef] [Green Version]
- Amaya-Vías, D.; Nebot, E.; López-Ramírez, J.A. Comparative studies of different membrane distillation configurations and membranes for potential use on board cruise vessels. Desalination 2018, 429, 44–51. [Google Scholar] [CrossRef]
- Eykens, L.; De Sitter, K.; Dotremont, C.; Pinoy, L.; Van der Bruggen, B. How to optimize the membrane properties for membrane distillation: A review. Ind. Eng. Chem. Res. 2016, 55, 9333–9343. [Google Scholar] [CrossRef]
- Wang, P.; Chung, T.-S. Recent advances in membrane distillation processes: Membrane development, configuration design and application exploring. J. Membr. Sci. 2015, 474, 39–56. [Google Scholar] [CrossRef]
- Eykens, L.; Hitsov, I.; De Sitter, K.; Dotremont, C.; Pinoy, L.; Van der Bruggen, B. Direct contact and air gap membrane distillation: Differences and similarities between lab and pilot scale. Desalination 2017, 422, 91–100. [Google Scholar] [CrossRef]
- Swaminathan, J.; Chung, H.W.; Warsinger, D.M. Membrane distillation model based on heat exchanger theory and configuration comparison. Appl. Energy 2016, 184, 491–505. [Google Scholar] [CrossRef] [Green Version]
- Khalifa, A.; Ahmad, H.; Antar, M.; Laoui, T.; Khayet, M. Experimental and theoretical investigations on water desalination using direct contact membrane distillation. Desalination 2017, 404, 22–34. [Google Scholar] [CrossRef]
- Politano, A.; Di Profio, G.; Fontananova, E.; Sanna, V.; Cupolillo, A.; Curcio, E. Overcoming temperature polarization in membrane distillation by thermoplasmonic effects activated by Ag nanofillers in polymeric membranes. Desalination 2019, 451, 192–199. [Google Scholar] [CrossRef]
- Gil, J.D.; Mendes, P.R.; Camponogara, E.; Roca, L.; Alvarez, J.D.; Normey-Rico, J.E. A general optimal operating strategy for commercial membrane distillation facilities. Renew. Energy 2020, 156, 220–234. [Google Scholar] [CrossRef]
- Choi, J.; Choi, Y.; Ju, J.; Park, Y.; Lee, S. Inorganic fouling mitigation using the baffle system in direct contact membrane distillation (DCMD). Desalination 2020, 496, 114714. [Google Scholar] [CrossRef]
- Anvari, A.; Azimi Yancheshme, A.; Kekre, K.M.; Ronen, A. State-of-the-art methods for overcoming temperature polarization in membrane distillation process: A review. J. Membr. Sci. 2020, 616, 118413. [Google Scholar] [CrossRef]
- Bhoumick, M.C.; Roy, S.; Mitra, S. Synergistic effect of air sparging in direct contact membrane distillation to control membrane fouling and enhancing flux. Sep. Purif. Technol. 2021, 272, 118681. [Google Scholar] [CrossRef]
- Ve, Q.L.; Koirala, R.; Bawahab, M.; Faqeha, H.; Do, M.C.; Nguyen, Q.L.; Date, A.; Akbarzadeh, A. Experimental investigation of the effect of the spacer and operating conditions on mass transfer in direct contact membrane distillation. Desalination 2021, 500, 114839. [Google Scholar] [CrossRef]
- Janajreh, I.; Suwwan, D.; Hashaikeh, R. Assessment of direct contact membrane distillation under different configurations, velocities and membrane properties. Appl. Energy 2017, 185, 2058–2073. [Google Scholar] [CrossRef]
- Ullah, R.; Khraisheh, M.; Esteves, R.J.; McLeskey Jr, J.T.; AlGhouti, M.; Gad-el-Hak, M.; Tafreshi, H.V. Energy efficiency of direct contact membrane distillation. Desalination 2018, 433, 56–67. [Google Scholar] [CrossRef]
- Santoro, S.; Vidorreta, I.; Coelhoso, I.; Lima, J.C.; Desiderio, G.; Lombardo, G.; Drioli, E.; Mallada, R.; Crespo, J.; Criscuoli, A. Experimental evaluation of the thermal polarization in direct contact membrane distillation using electrospun nanofiber membranes doped with molecular probes. Molecules 2019, 24, 638. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Dow, N.; Duke, M.; Ostarcevic, E.; Gray, S. Identification of material and physical features of membrane distillation membranes for high performance desalination. J. Membr. Sci. 2010, 349, 295–303. [Google Scholar] [CrossRef] [Green Version]
- Janajreh, I.; Suwwan, D.; Hashaikeh, R. Theoretical and experimental study of direct contact membrane distillation. Desalination Water Treat. 2016, 57, 15660–15675. [Google Scholar] [CrossRef]
- Ahadi, H.; Karimi-Sabet, J.; Shariaty-Niassar, M. The effect of module geometry on heat and mass transfer in membrane distillation. Chem. Prod. Process. Modeling 2016, 11, 35–39. [Google Scholar] [CrossRef]
- Sreedhar, N.; Thomas, N.; Al-Ketan, O.; Rowshan, R.; Hernandez, H.H.; Al-Rub, R.K.A.; Arafat, H.A. Mass transfer analysis of ultrafiltration using spacers based on triply periodic minimal surfaces: Effects of spacer design, directionality and voidage. J. Membr. Sci. 2018, 561, 89–98. [Google Scholar] [CrossRef]
- Hagedorn, A.; Fieg, G.; Winter, D.; Koschikowski, J.; Grabowski, A.; Mann, T. Membrane and spacer evaluation with respect to future module design in membrane distillation. Desalination 2017, 413, 154–167. [Google Scholar] [CrossRef]
- Li, Q.; Lian, B.; Zhong, W.; Omar, A.; Razmjou, A.; Dai, P.; Guan, J.; Leslie, G.; Taylor, R.A. Improving the performance of vacuum membrane distillation using a 3D-printed helical baffle and a superhydrophobic nanocomposite membrane. Sep. Purif. Technol. 2020, 248, 117072. [Google Scholar] [CrossRef]
- Anvari, A.; Yancheshme, A.A.; Ronen, A. Enhanced performance of membrane distillation using radio-frequency induction heated thermally conducting feed spacers. Sep. Purif. Technol. 2020, 250, 117276. [Google Scholar] [CrossRef]
- Lou, J.; Johnston, J.; Cath, T.Y.; Martinand, D.; Tilton, N. Computational fluid dynamics simulations of unsteady mixing in spacer-filled direct contact membrane distillation channels. J. Membr. Sci. 2021, 622, 118931. [Google Scholar] [CrossRef]
- Alanezi, A.A.; Safaei, M.R.; Goodarzi, M.; Elhenawy, Y. The Effect of Inclination Angle and Reynolds Number on the Performance of a Direct Contact Membrane Distillation (DCMD) Process. Energies 2020, 13, 2824. [Google Scholar] [CrossRef]
- Yazgan-Birgi, P.; Ali, M.I.H.; Arafat, H.A. Comparative performance assessment of flat sheet and hollow fiber DCMD processes using CFD modeling. Sep. Purif. Technol. 2019, 212, 709–722. [Google Scholar] [CrossRef]
- Ni, W.; Li, Y.; Zhao, J.; Zhang, G.; Du, X.; Dong, Y. Simulation Study on Direct Contact Membrane Distillation Modules for High-Concentration NaCl Solution. Membranes 2020, 10, 179. [Google Scholar] [CrossRef]
- Sarpkaya, T. Computational Methods with Vortices—the 1988 Freeman Scholar Lecture. Trans. ASME J. Fluids Engng. 1989, 111, 5–52. [Google Scholar] [CrossRef]
- Khatir, Z.; Lucey, A.D. A combined boundary integral and vortex method for the numerical study of three-dimensional fluid flow systems. Int. J. Comput. Math. 2012, 89, 1504–1524. [Google Scholar] [CrossRef]
- Bimbato, A.; Pereira, L.A.; Hirata, M. Development of a new Lagrangian vortex method for evaluating effects of surfaces roughness. Eur. J. Mech. B/Fluids 2019, 74, 291–301. [Google Scholar] [CrossRef]
- Kornev, N.; Samarbakhsh, S. Large eddy simulation with direct resolution of subgrid motion using a grid free vortex particle method. Int. J. Heat Fluid Flow 2019, 75, 86–102. [Google Scholar] [CrossRef]
- Marques Lisboa, K.; Busson de Moraes, D.; Palma Naveira-Cotta, C.; Machado Cotta, R. Analysis of the membrane effects on the energy efficiency of water desalination in a direct contact membrane distillation (DCMD) system with heat recovery. Appl. Therm. Eng. 2021, 182, 116063. [Google Scholar] [CrossRef]
- Najib, A.; Orfi, J.; Ali, E.; Saleh, J. Thermodynamics analysis of a direct contact membrane distillation with/without heat recovery based on experimental data. Desalination 2019, 466, 52–67. [Google Scholar] [CrossRef]
- Mahian, O.; Mirzaie, M.R.; Kasaeian, A.; Mousavi, S.H. Exergy analysis in combined heat and power systems: A review. Energy Convers. Manag. 2020, 226, 113467. [Google Scholar] [CrossRef]
- Mehdizadeh-Fard, M.; Pourfayaz, F.; Maleki, A. Exergy analysis of multiple heat exchanger networks: An approach based on the irreversibility distribution ratio. Energy Rep. 2021, 7, 174–193. [Google Scholar] [CrossRef]
- Signorato, F.; Morciano, M.; Bergamasco, L.; Fasano, M.; Asinari, P. Exergy analysis of solar desalination systems based on passive multi-effect membrane distillation. Energy Rep. 2020, 6, 445–454. [Google Scholar] [CrossRef]
- Si, Z.; Han, D.; Gu, J.; Song, Y.; Liu, Y. Exergy analysis of a vacuum membrane distillation system integrated with mechanical vapor recompression for sulfuric acid waste treatment. Appl. Therm. Eng. 2020, 178, 115516. [Google Scholar] [CrossRef]
- Chen, T.-C.; Ho, C.-D.; Yeh, H.-M. Theoretical modeling and experimental analysis of direct contact membrane distillation. J. Membr. Sci. 2009, 330, 279–287. [Google Scholar] [CrossRef]
- Hwang, H.J.; He, K.; Gray, S.; Zhang, J.; Moon, I.S. Direct contact membrane distillation (DCMD): Experimental study on the commercial PTFE membrane and modeling. J. Membr. Sci. 2011, 371, 90–98. [Google Scholar] [CrossRef] [Green Version]
- Ghadiri, M.; Fakhri, S.; Shirazian, S. Modeling and CFD simulation of water desalination using nanoporous membrane contactors. Ind. Eng. Chem. Res. 2013, 52, 3490–3498. [Google Scholar] [CrossRef]
- Chang, H.; Ho, C.-D.; Hsu, J.-A. Analysis of Heat Transfer Coefficients in Direct Contact Membrane Distillation Modules Using CFD Simulation. J. Appl. Eng. Sci. 2016, 19, 197–206. [Google Scholar]
- Cath, T.Y.; Adams, V.D.; Childress, A.E. Experimental study of desalination using direct contact membrane distillation: A new approach to flux enhancement. J. Membr. Sci. 2004, 228, 5–16. [Google Scholar] [CrossRef]
- Martinez-Diez, L.; Vazquez-Gonzalez, M.; Florido-Diaz, F. Temperature Polarization Coefficients in Membrane Distillation. Sep. Sci. Tech. 1998, 33, 787–799. [Google Scholar] [CrossRef]





| Parameter | Value |
|---|---|
| Feed channel height | 0.003 m |
| Permeate channel height | 0.003 m |
| Channel length | 0.06 m |
| Heat of evaporation | 2.333 × 106 [W·s]/kg |
| Feed thermal conductivity | 0.64 W/[m·K] |
| Permeate thermal conductivity | 0.6 W/[m·K] |
| Membrane thermal conductivity | 0.04 W/[m·K] |
| Membrane pore size | 0.22 μm |
| Membrane thickness | 100 μm |
| Membrane tortuosity | 2 |
| Case | Feed Side | Permeate Side | ||
|---|---|---|---|---|
| Temperature (°C) | Flow Rate (L/min) | Temperature (°C) | Flow Rate (L/min) | |
| 1 | 40 | 0.6 | 20 | 0.4 |
| 2 | 50 | |||
| 3 | 60 | |||
| 4 | 70 | |||
| 5 | 60 | 0.24 | ||
| 6 | 0.48 | |||
| 7 | 0.6 | 0.2 | ||
| 8 | 0.6 | 0.6 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, J.; Choi, Y.; Lee, J.; Kim, Y.; Lee, S. Exergy Analysis of a Direct Contact Membrane Distillation (DCMD) System Based on Computational Fluid Dynamics (CFD). Membranes 2021, 11, 525. https://doi.org/10.3390/membranes11070525
Choi J, Choi Y, Lee J, Kim Y, Lee S. Exergy Analysis of a Direct Contact Membrane Distillation (DCMD) System Based on Computational Fluid Dynamics (CFD). Membranes. 2021; 11(7):525. https://doi.org/10.3390/membranes11070525
Chicago/Turabian StyleChoi, Jihyeok, Yongjun Choi, Juyoung Lee, Yusik Kim, and Sangho Lee. 2021. "Exergy Analysis of a Direct Contact Membrane Distillation (DCMD) System Based on Computational Fluid Dynamics (CFD)" Membranes 11, no. 7: 525. https://doi.org/10.3390/membranes11070525
APA StyleChoi, J., Choi, Y., Lee, J., Kim, Y., & Lee, S. (2021). Exergy Analysis of a Direct Contact Membrane Distillation (DCMD) System Based on Computational Fluid Dynamics (CFD). Membranes, 11(7), 525. https://doi.org/10.3390/membranes11070525







